Near-Wall Flow in Turbomachinery Cascades—Results of a German Collaborative Project
Abstract
1. Introduction
2. Sub-Project A—Periodically Transient Near-Wall Flow within Rotating Compressor Cascades
2.1. Scope of Sub-Project A
2.2. Experimental Setup
2.2.1. Test Facility
2.2.2. Measurement Techniques
2.3. Numerical Setup
2.4. Current Investigations and Results
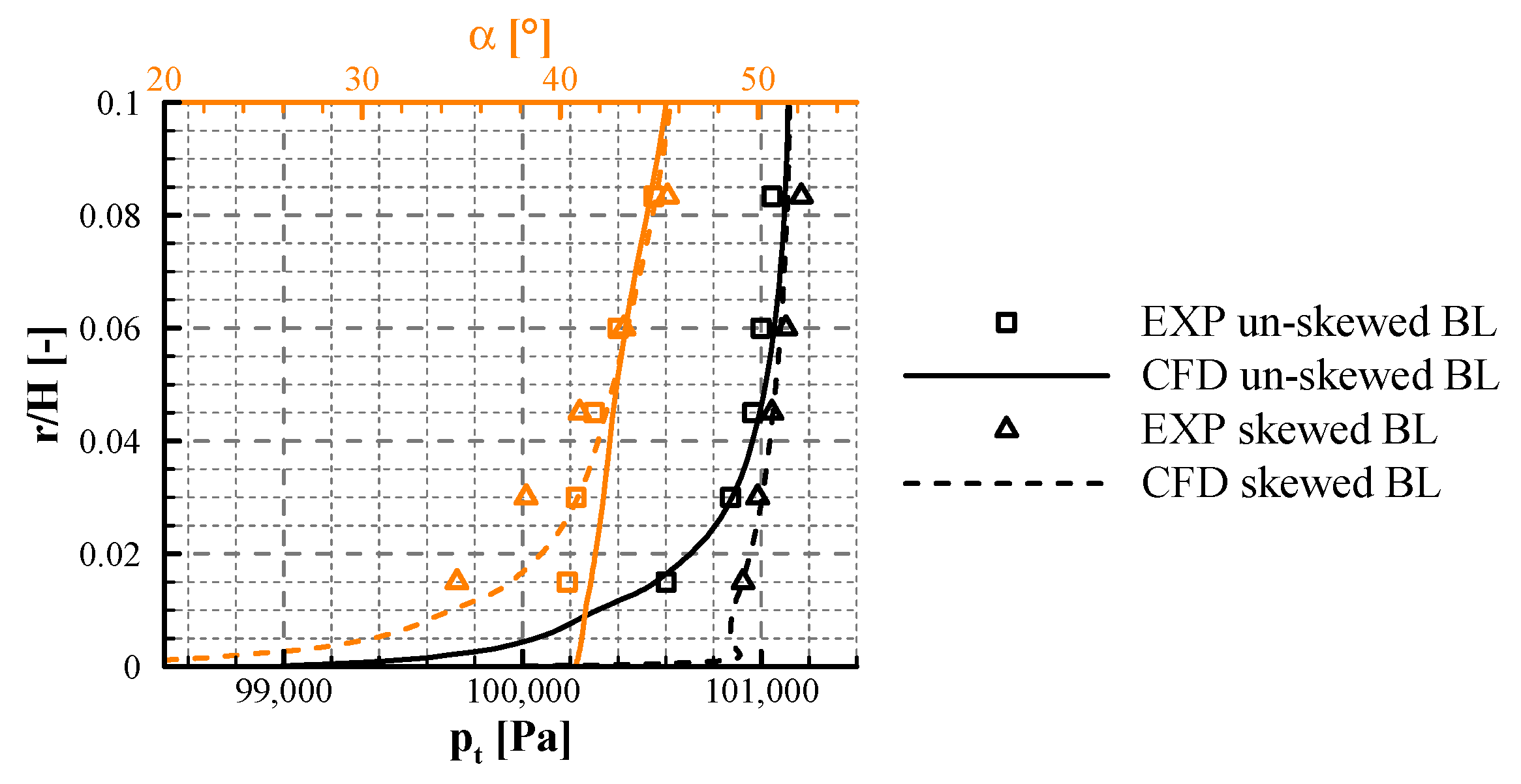
2.4.1. Influence of the Inlet Boundary Layer Skew
2.4.2. Influence of Relative Motion between Vane Tip and Corresponding Endwall
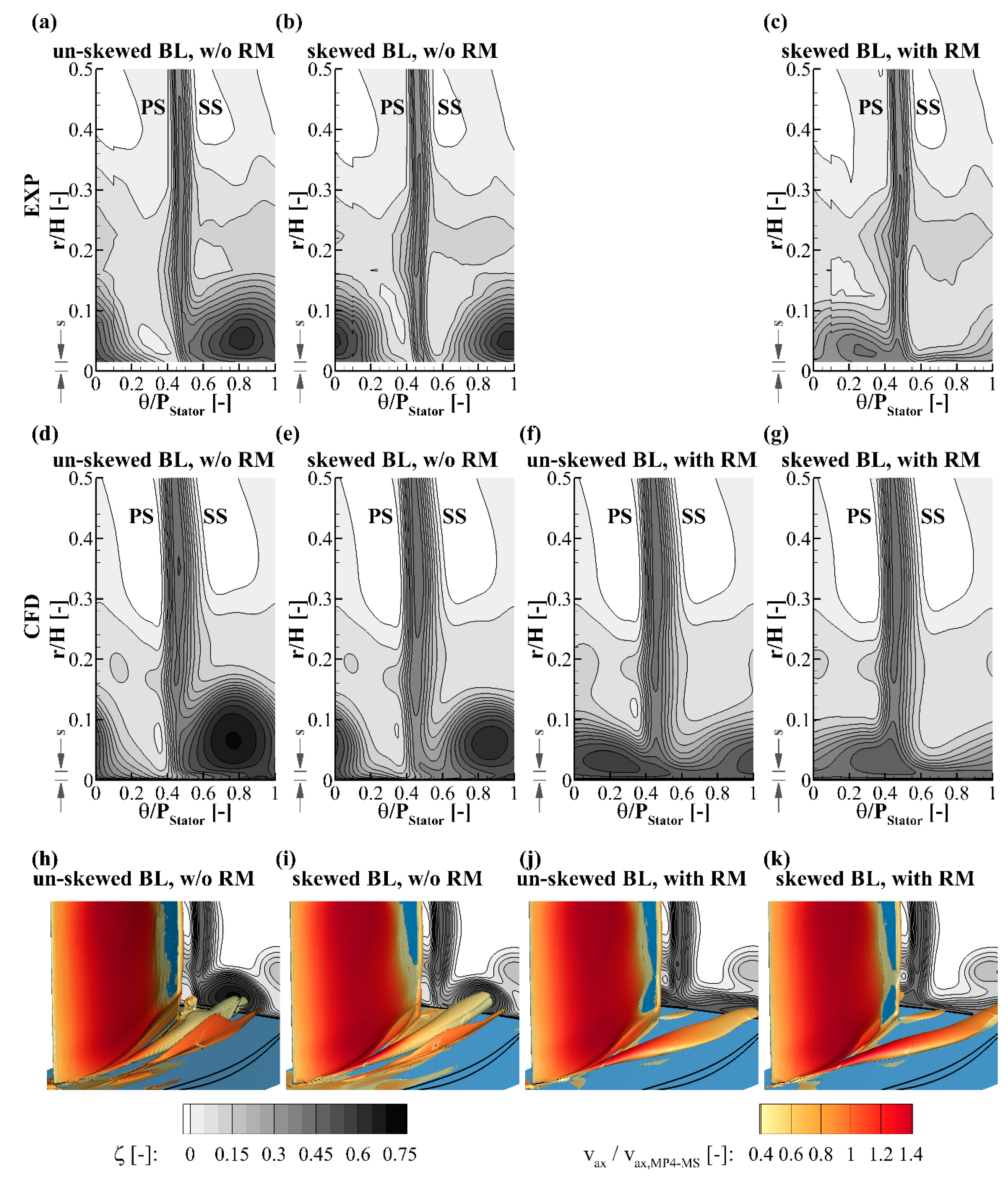
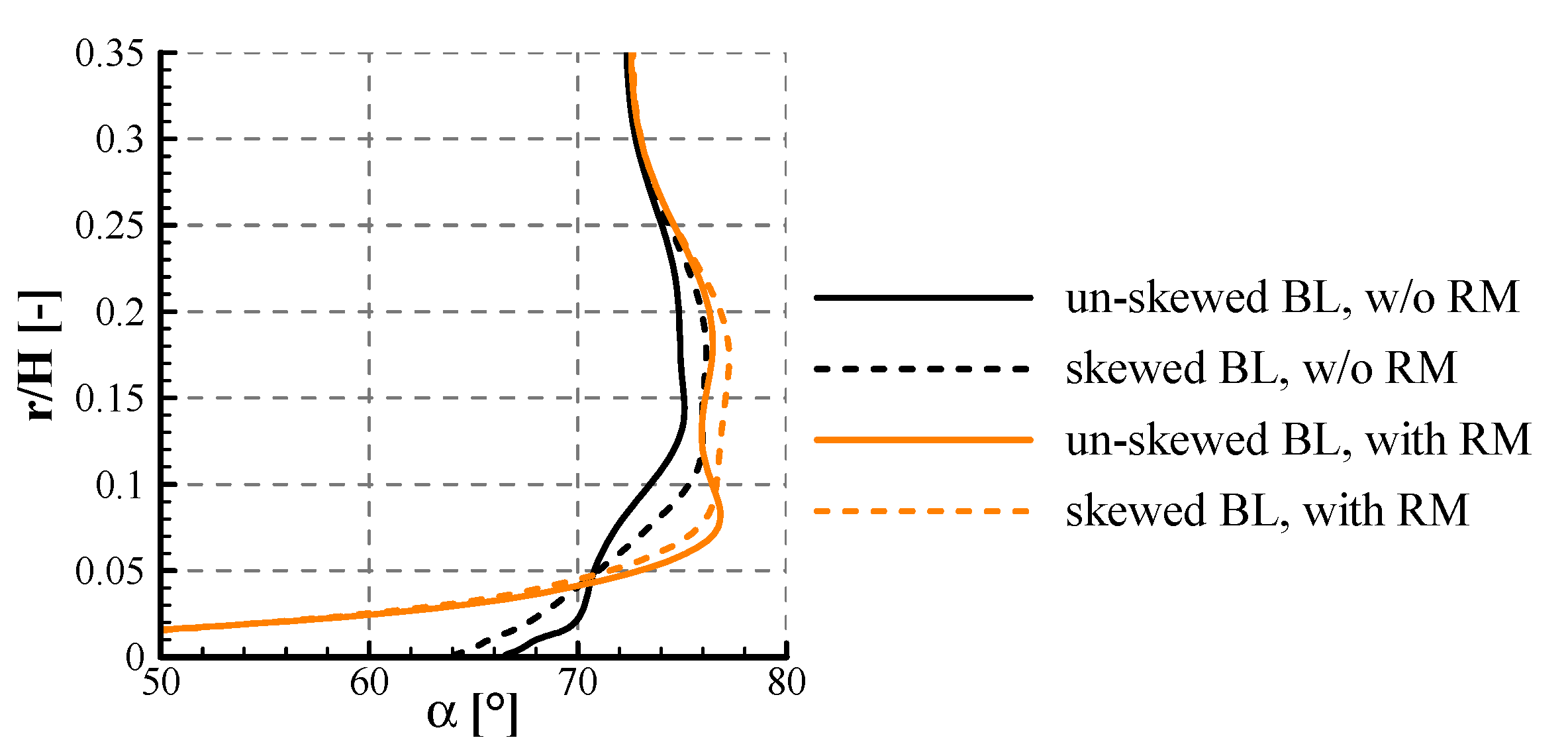
2.4.3. Combined Influence of Boundary Layer Skew and Relative Motion between Vane Tip and Corresponding Endwall
2.4.4. Influence of Incoming Periodic Wakes
2.5. Work in Progress
3. Sub-Project B—High Fidelity Numerical Investigations of the Secondary Flow in a Linear Compressor Cascade
3.1. Scope of Sub-Project B
3.2. Geometry
3.3. Numerical Setup and Grid
3.4. Current Investigations and Results
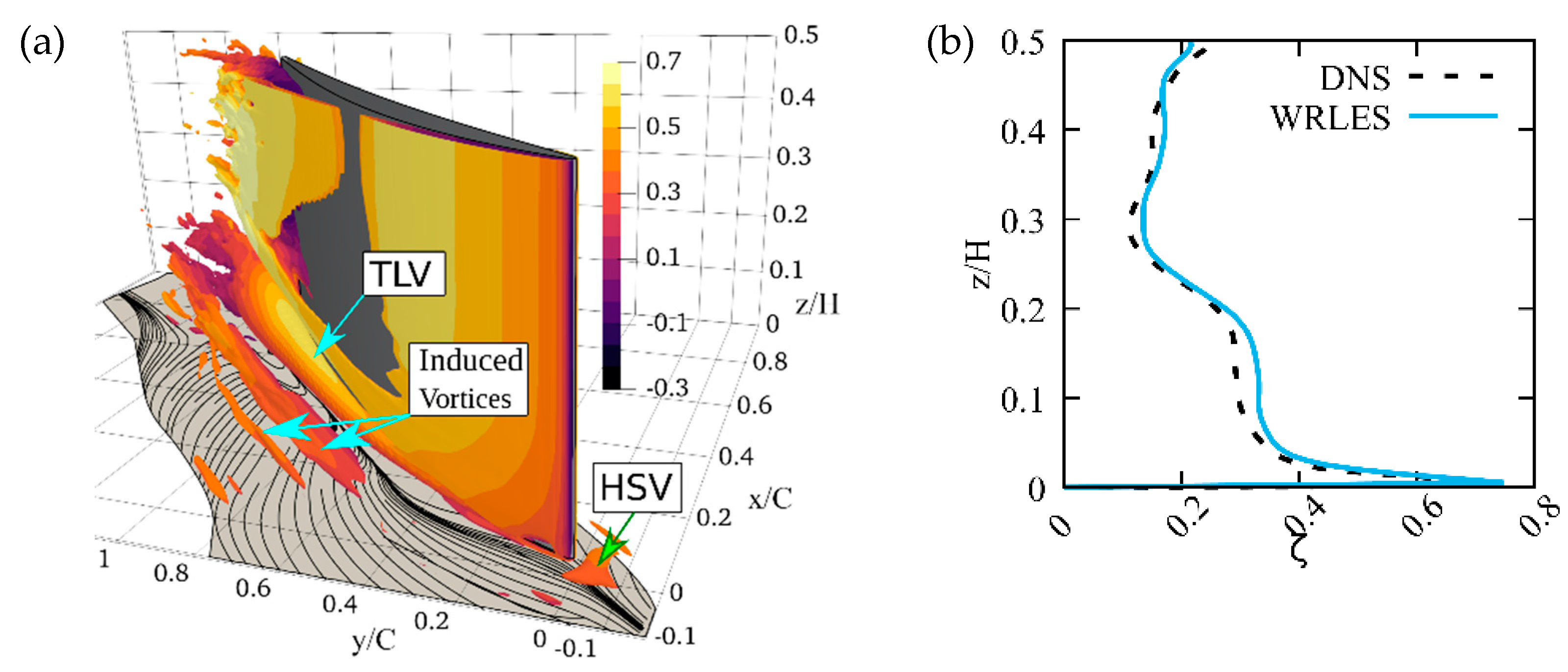
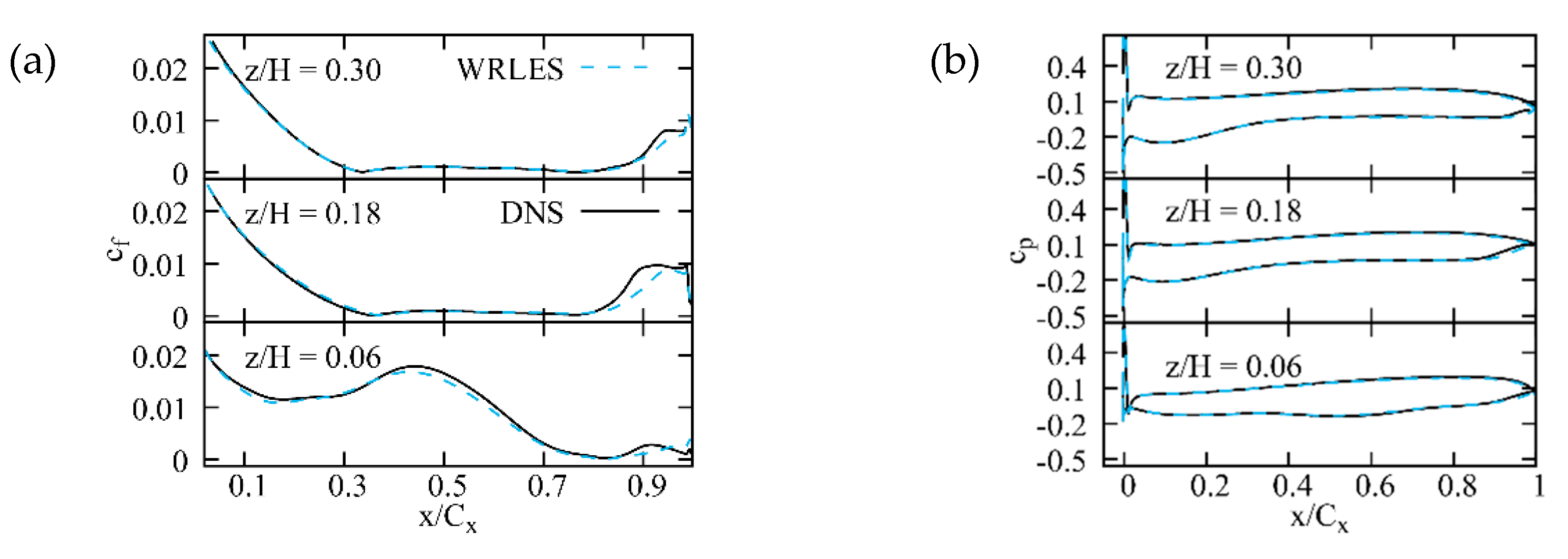
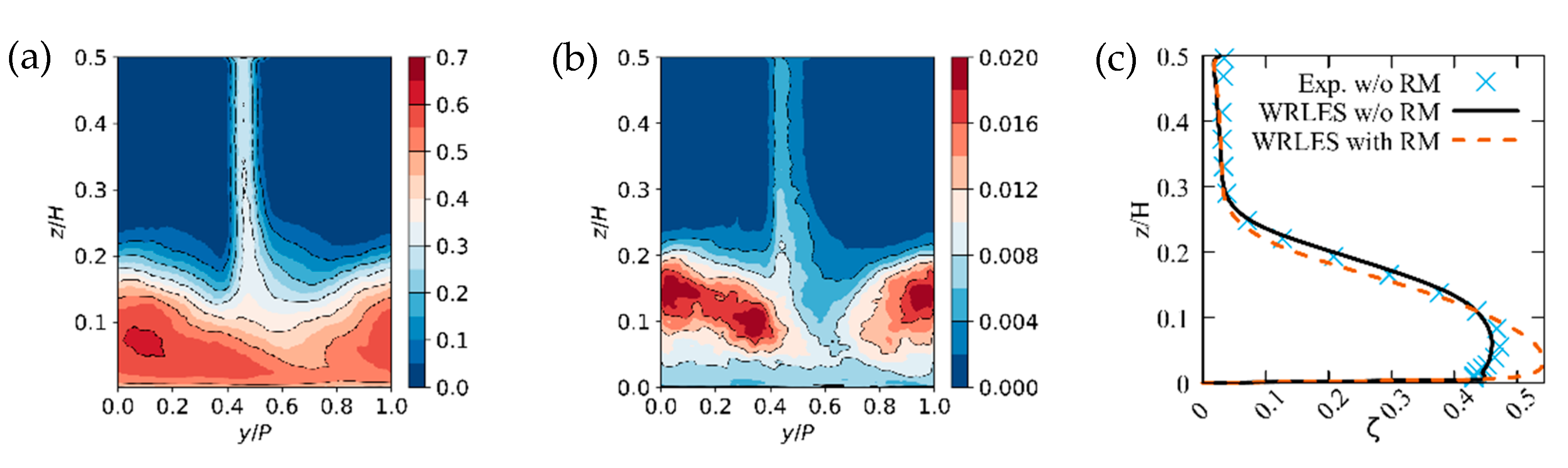
3.4.1. Validation of WRLES with Respect to DNS Data
3.4.2. Validation of WRLES with Respect to Experimental Data
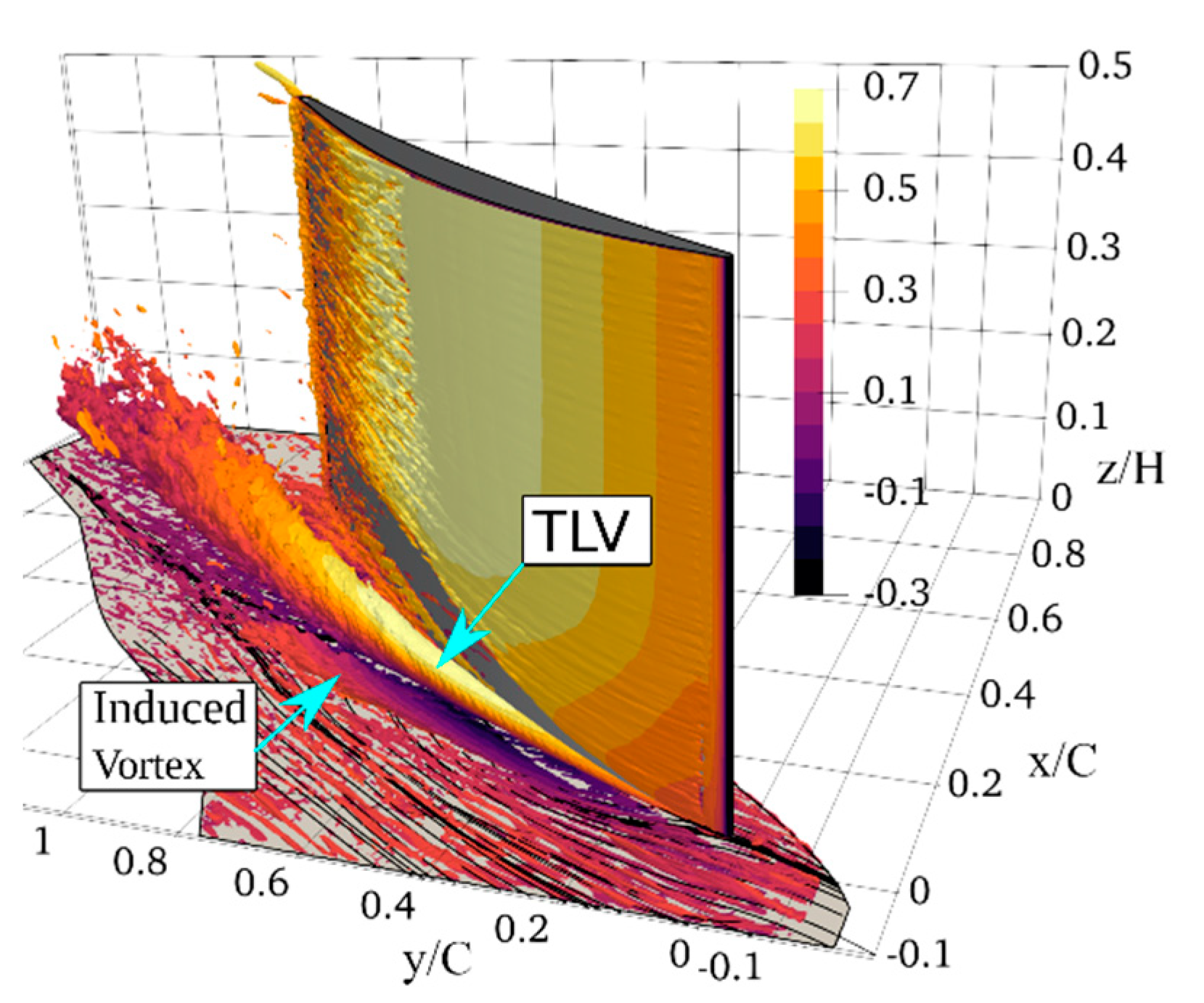
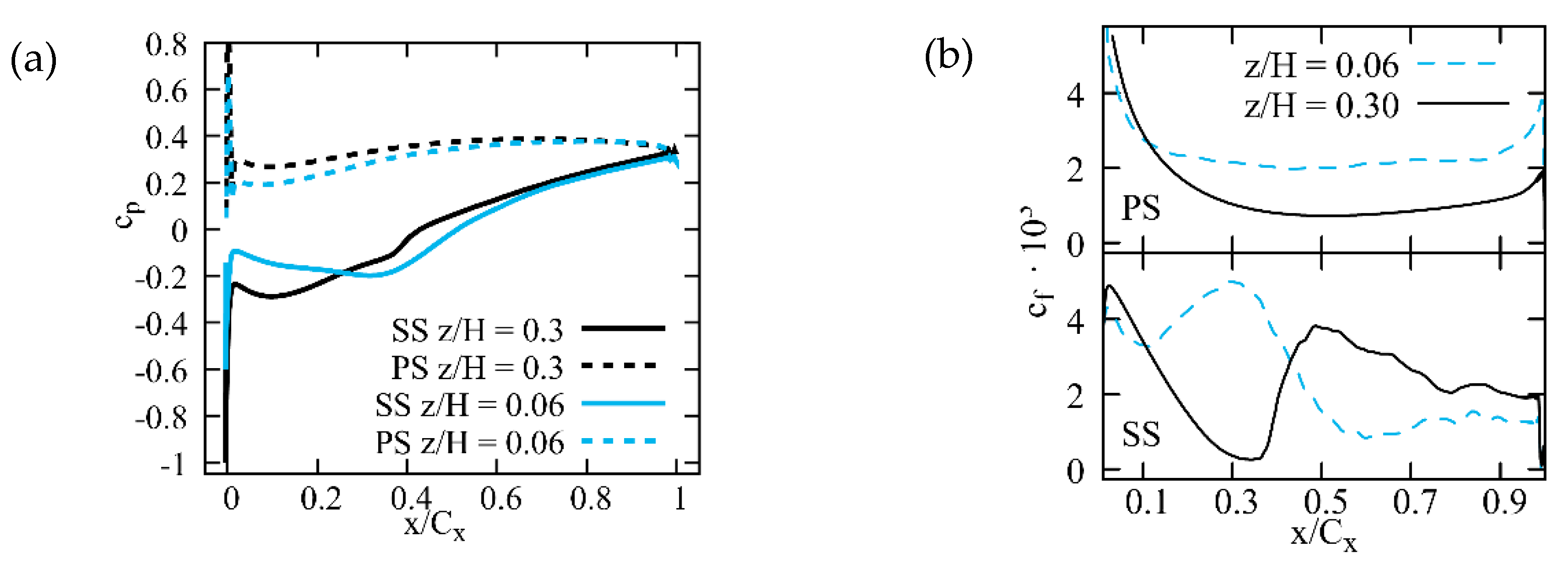
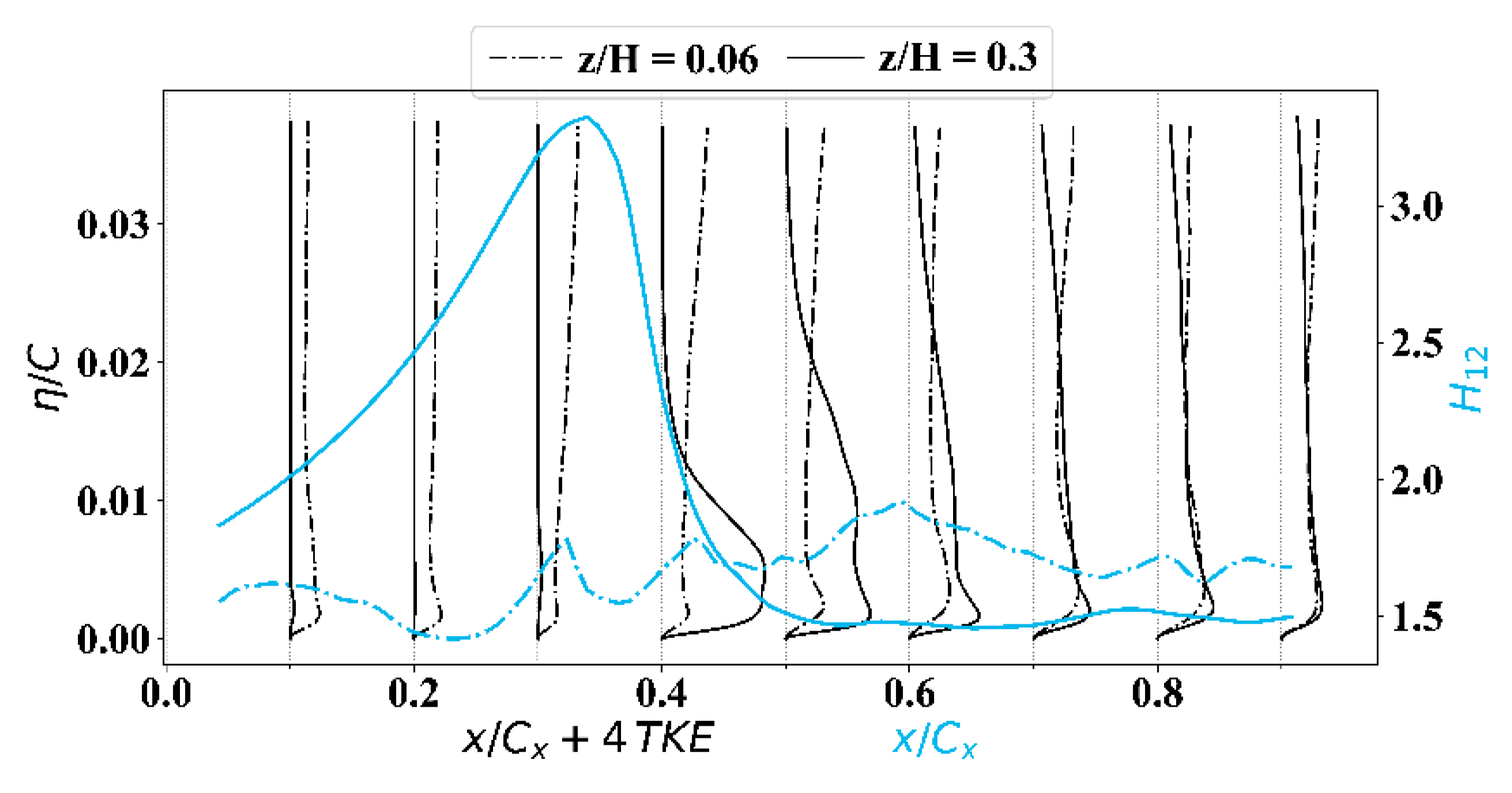
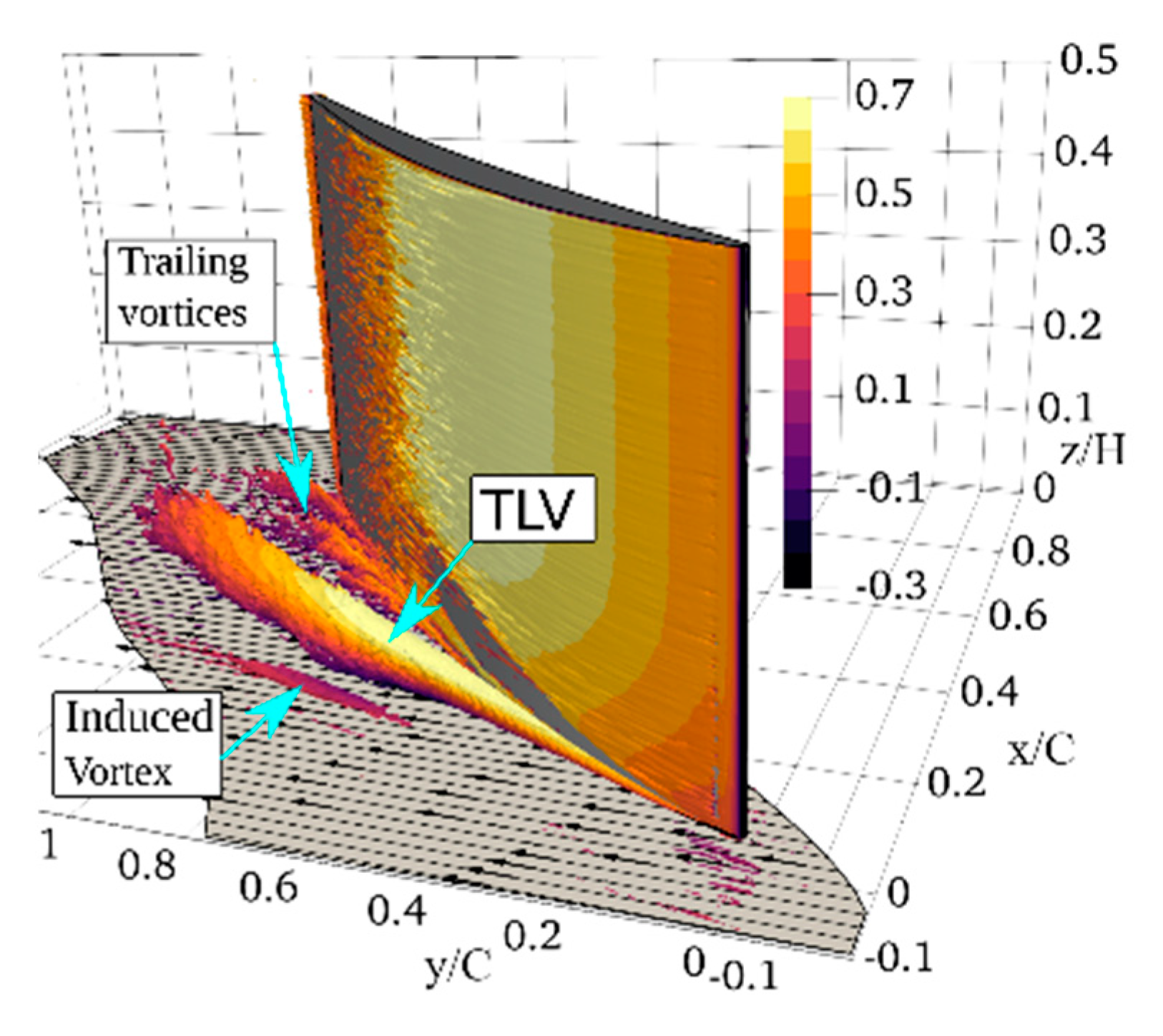
3.4.3. Secondary Flow Effects in Compressor Cascade
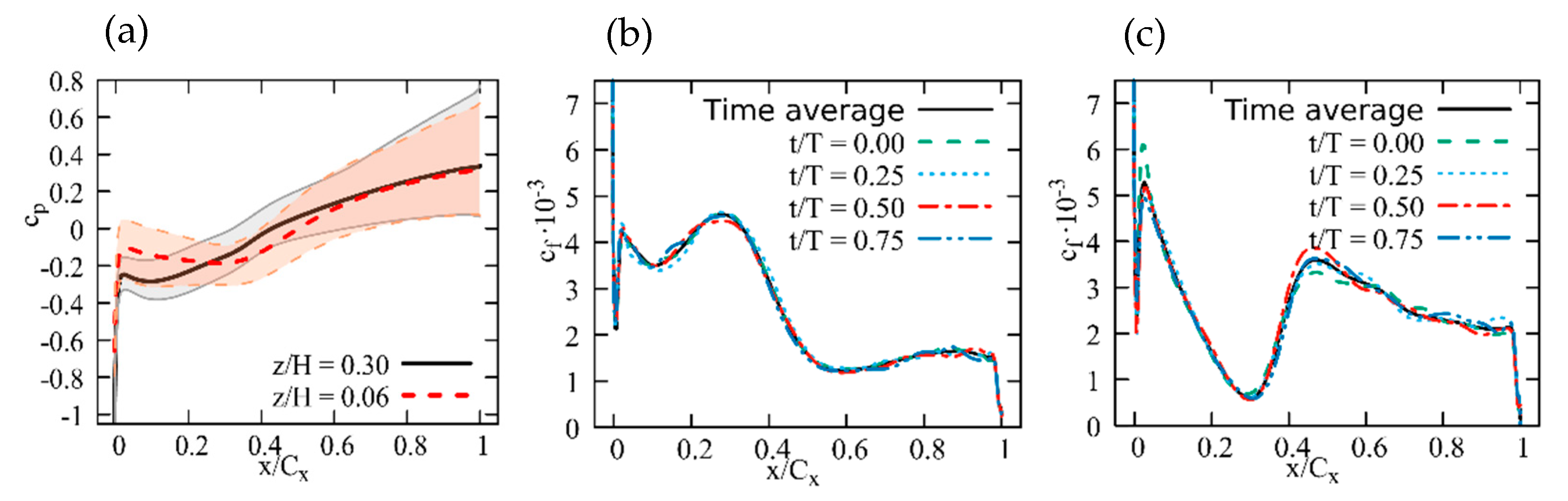
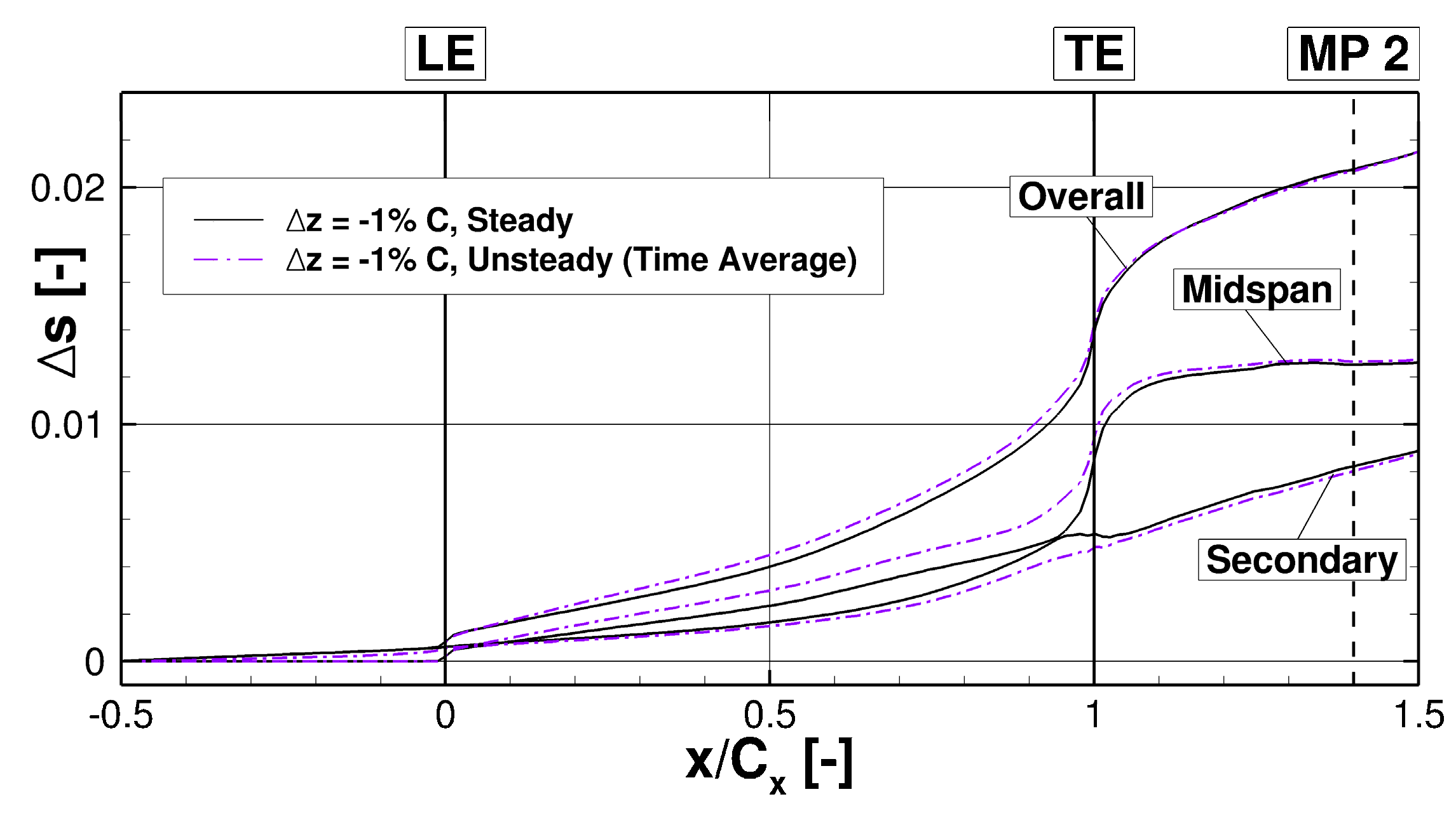
3.4.4. Effect of Relative Endwall Motion
3.4.5. Incoming, Periodically Perturbed Flow Field
3.5. Work in Progress
4. Sub-Project C – Periodically Transient Near-Wall Flow in the T106 Turbine Row
4.1. Scope of Sub-Project C
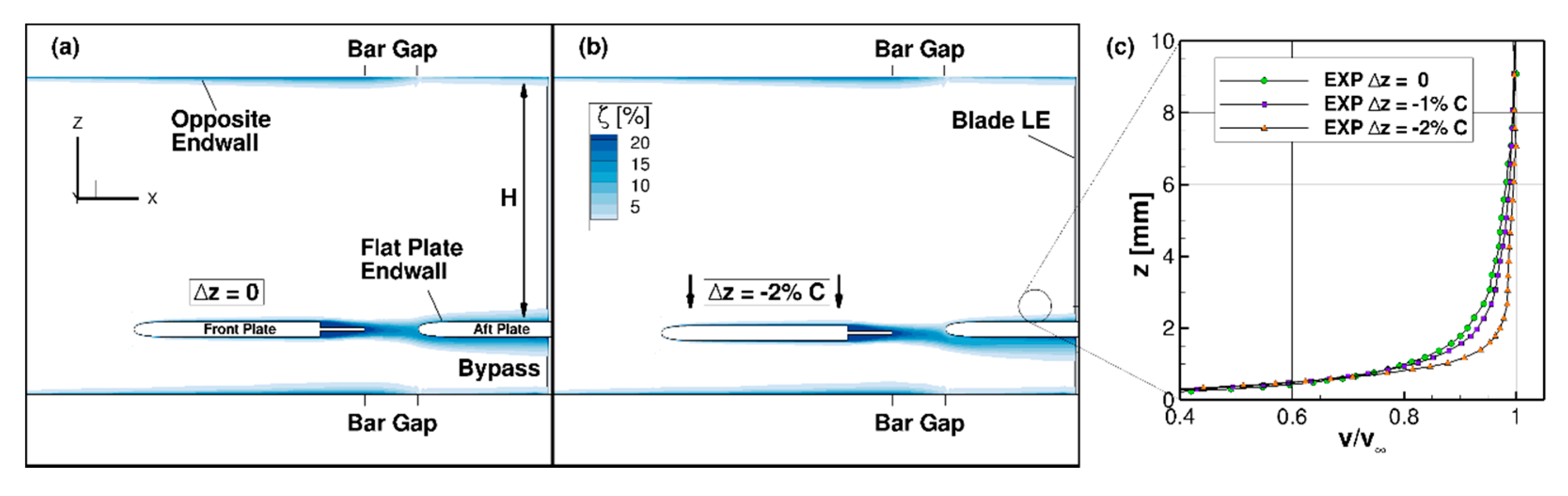
4.2. Experimental Setup
Measurement Techniques
4.3. Numerical Setup
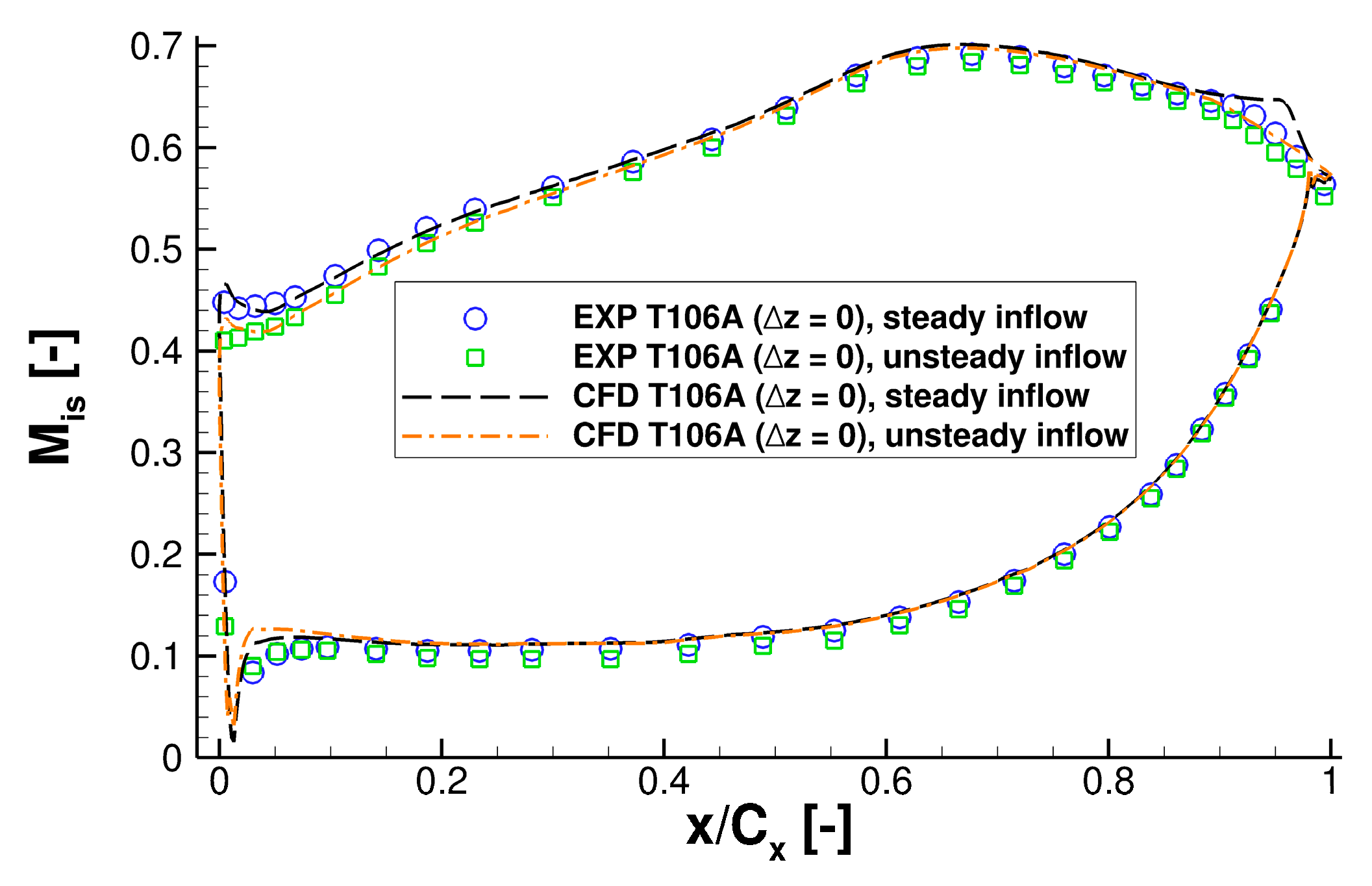
4.4. Current Investigations and Results
4.5. Work in Progress
5. Sub-Project D—Influence of Periodic Wakes on the Transient Near-Wall Flow in an Annular Axial Turbine Cascade
5.1. Scope of Sub-Project D
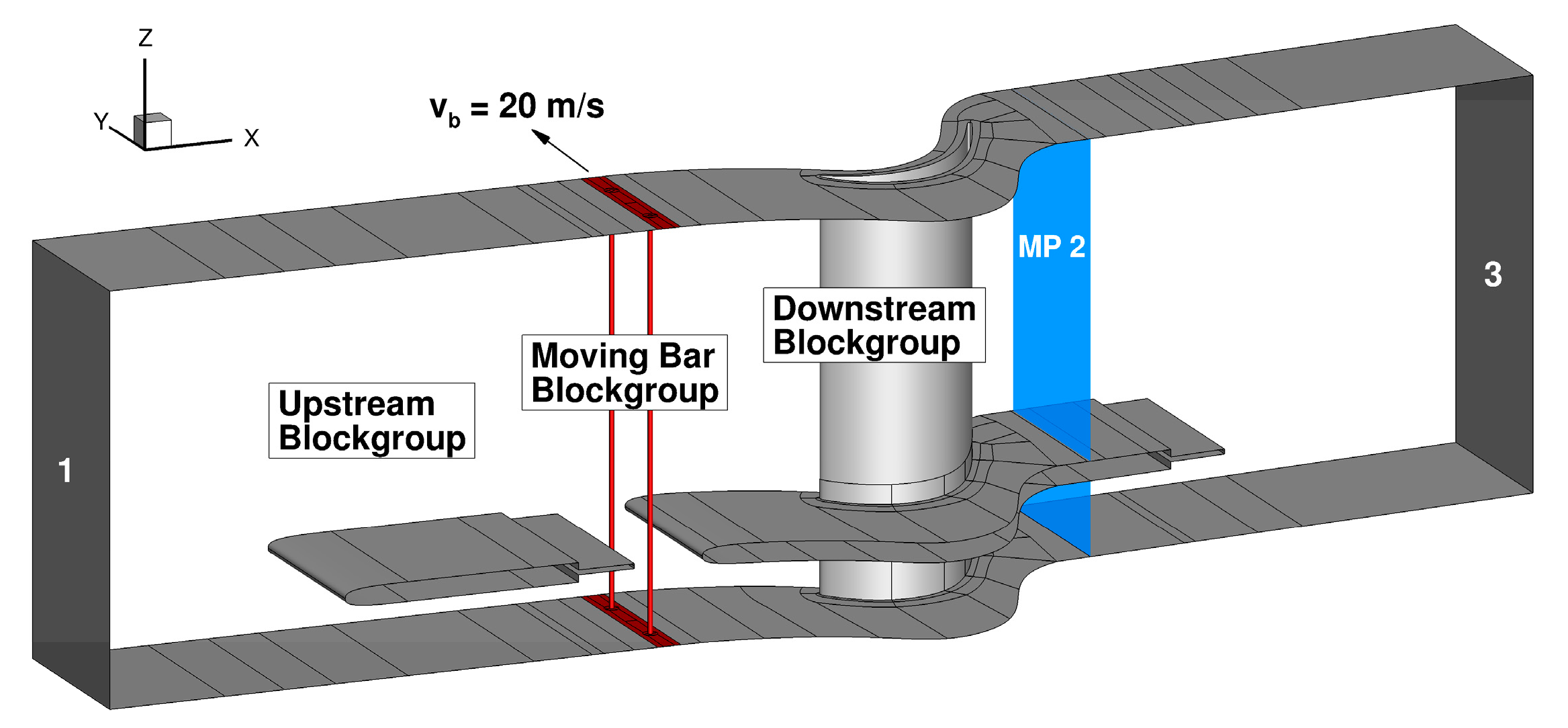
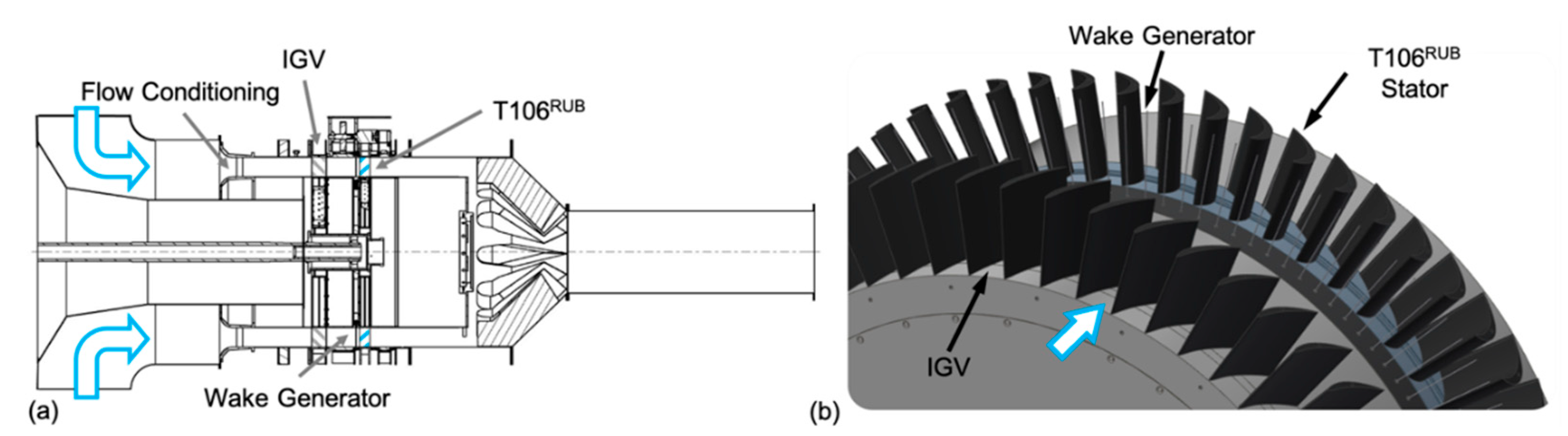
5.2. Experimental Setup
5.3. Measurement Techniques
5.4. Current Investigations and Results
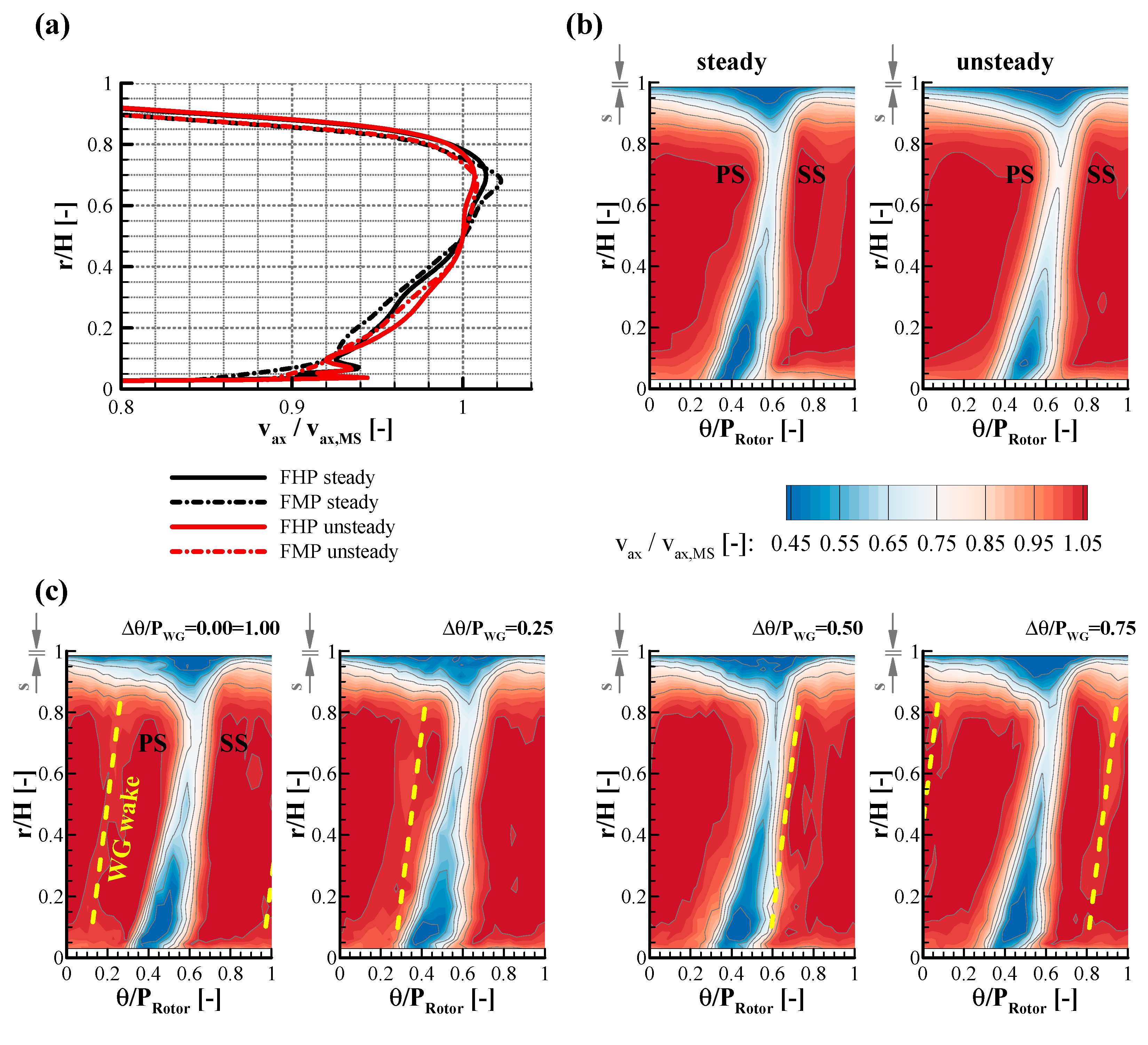
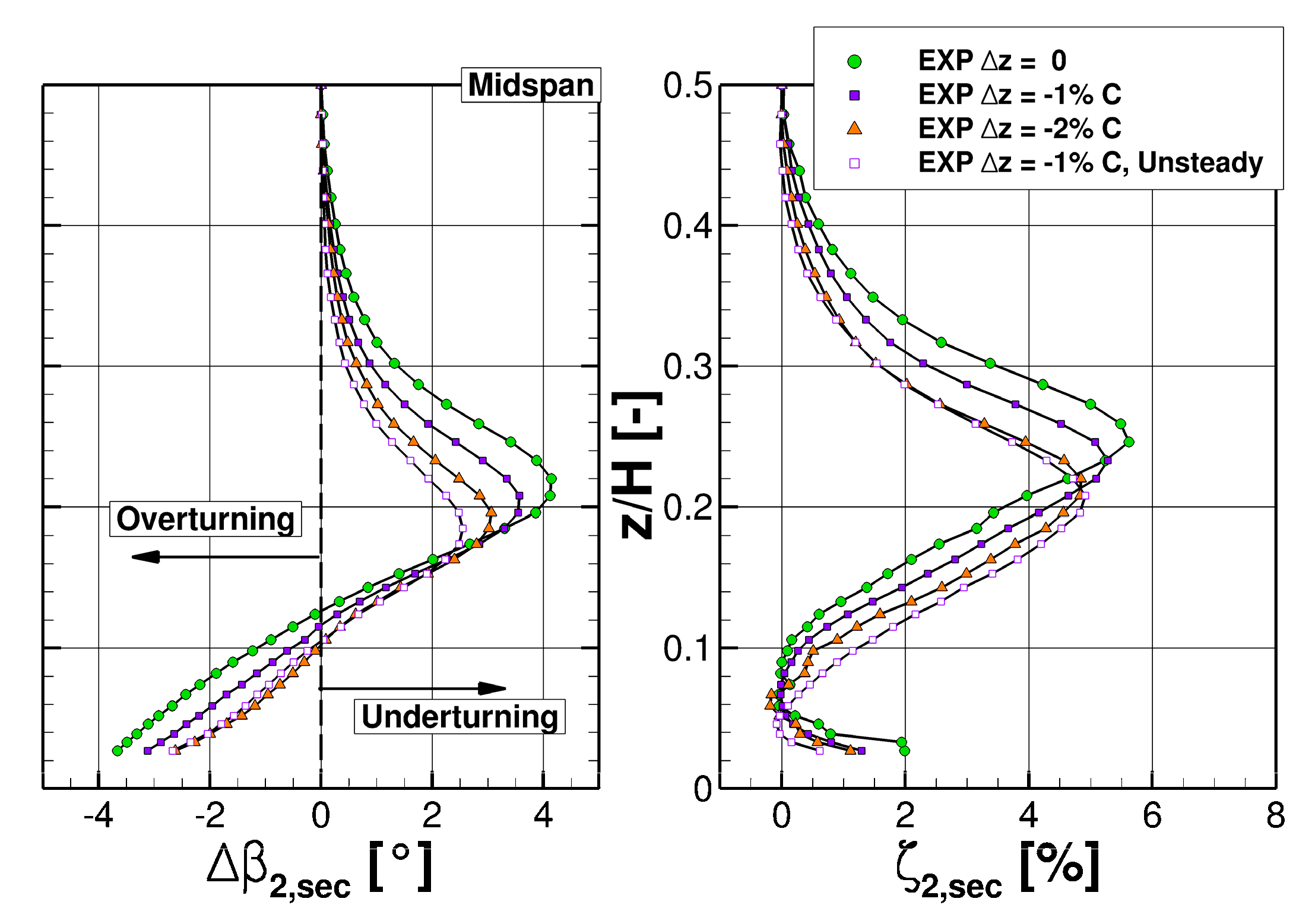
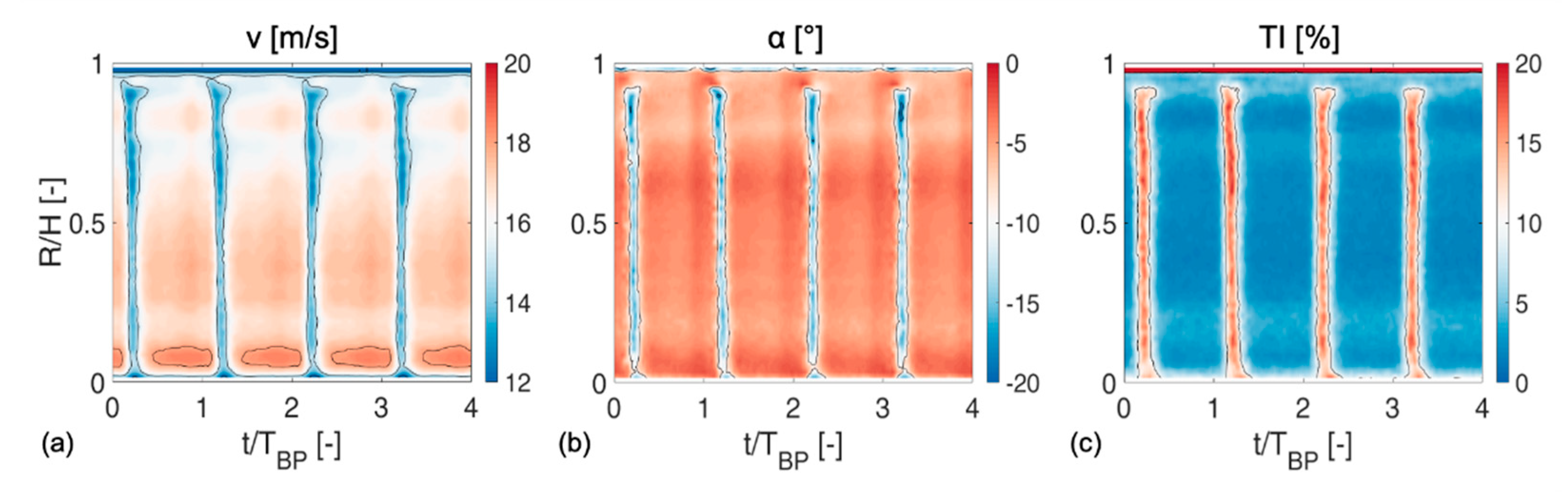
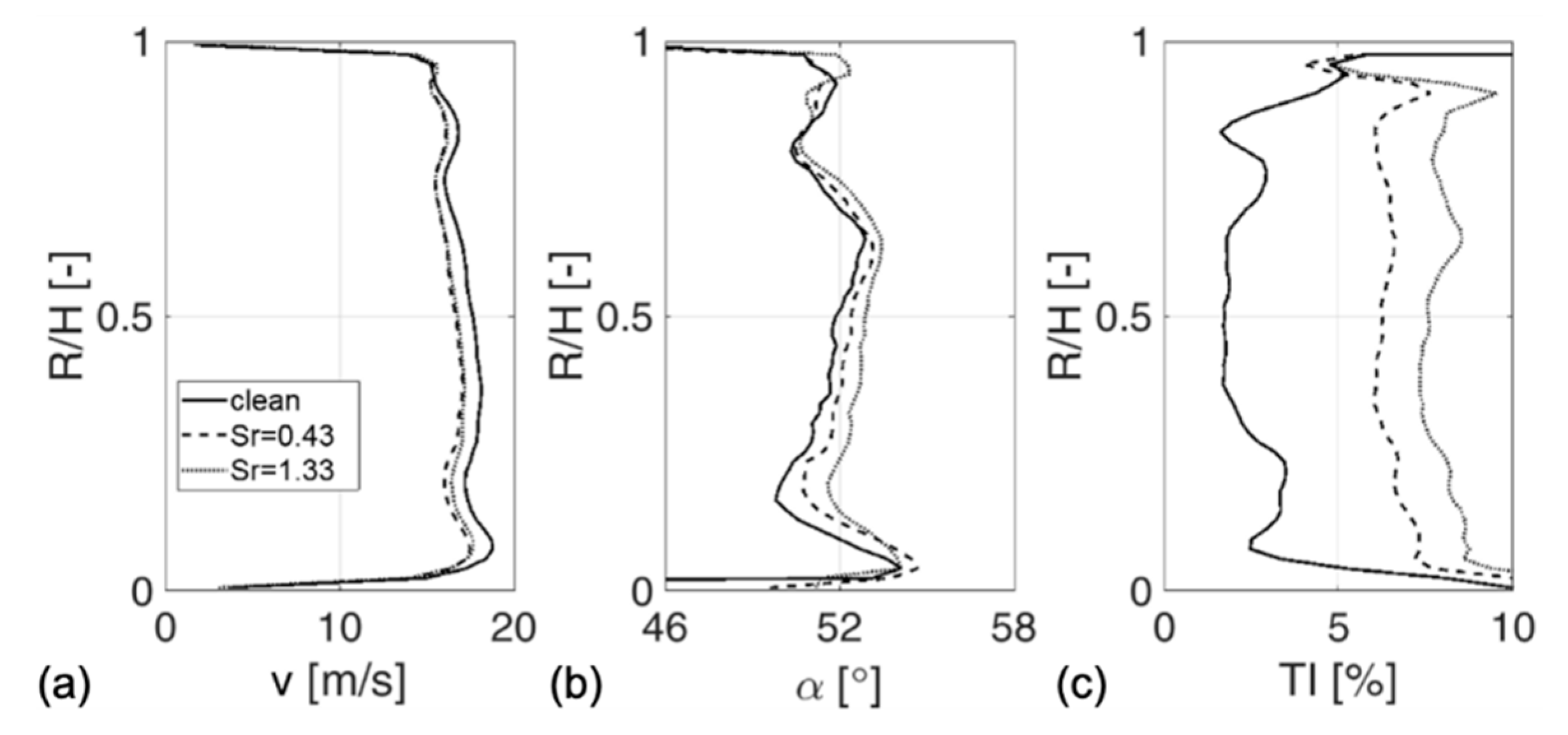
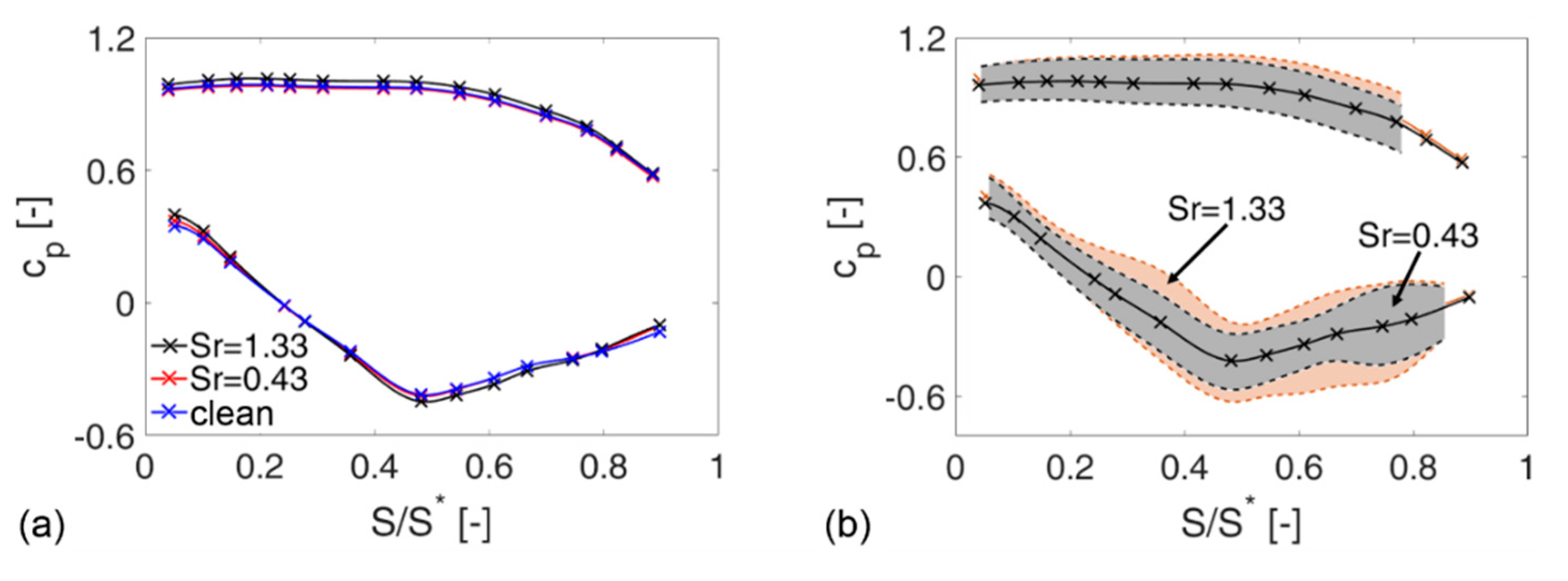
5.4.1. Incoming, Periodically Perturbed Flow Field
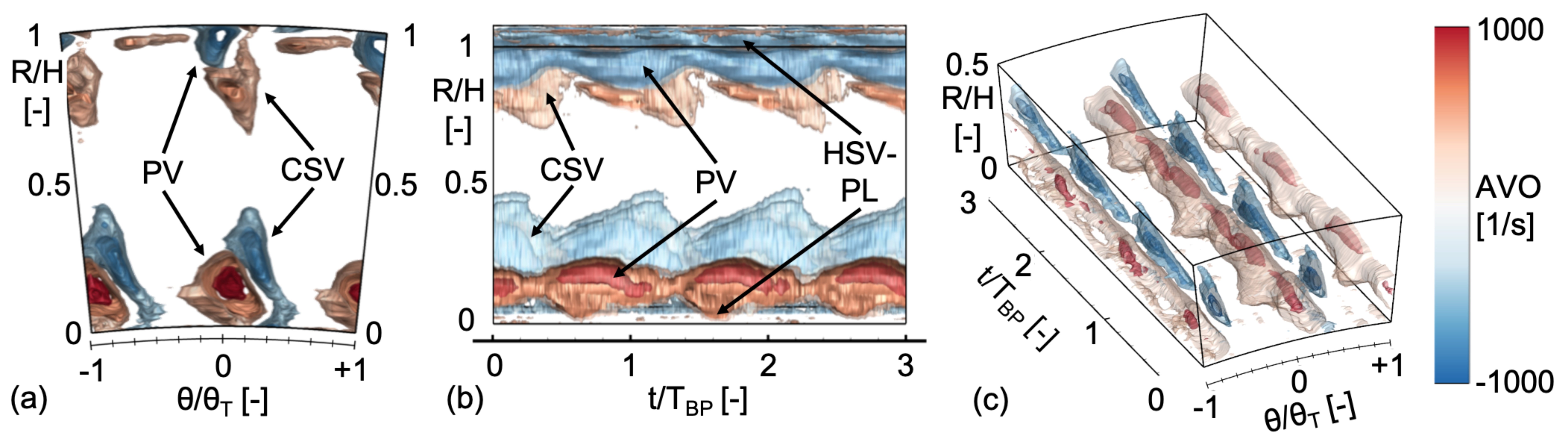
5.4.2. Situation within the T106RUB Blade Row
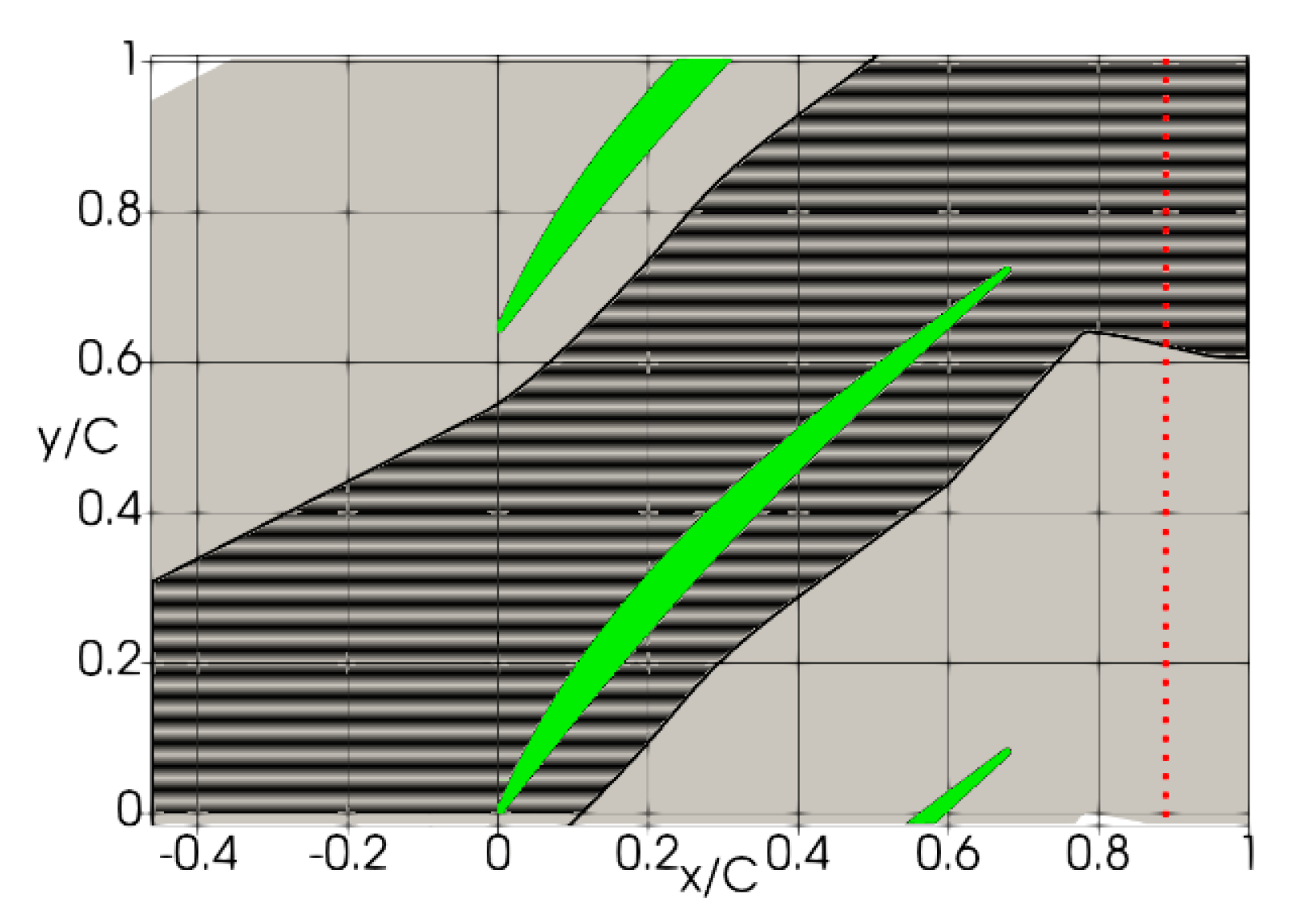
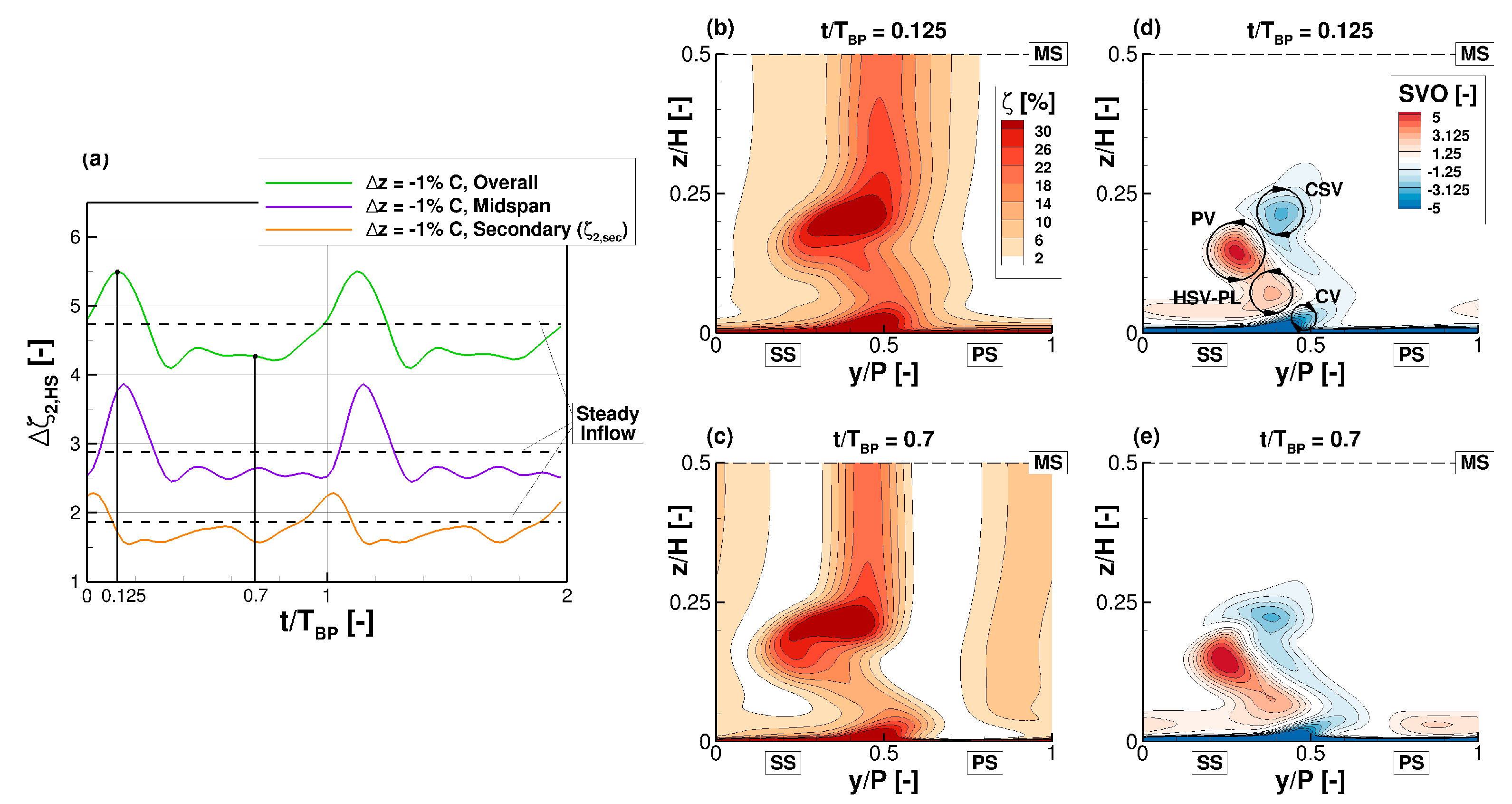
- Wake-induced boundary layer instabilities, like locally confined turbulent patches or Klebanoff-Streaks, which are induced in the front part of the profile boundary layer far upstream, propagate slower (0.5 < v/vFS < 0.88) than the free stream (FS) and the wakes [62].
- Calmed regions exert a damping effect on the boundary layer instabilities, thus counteract transition and separation and spread while propagating downstream [63], while their velocity of convection is also considerably reduced (0.3 < v/vFS < 0.5).
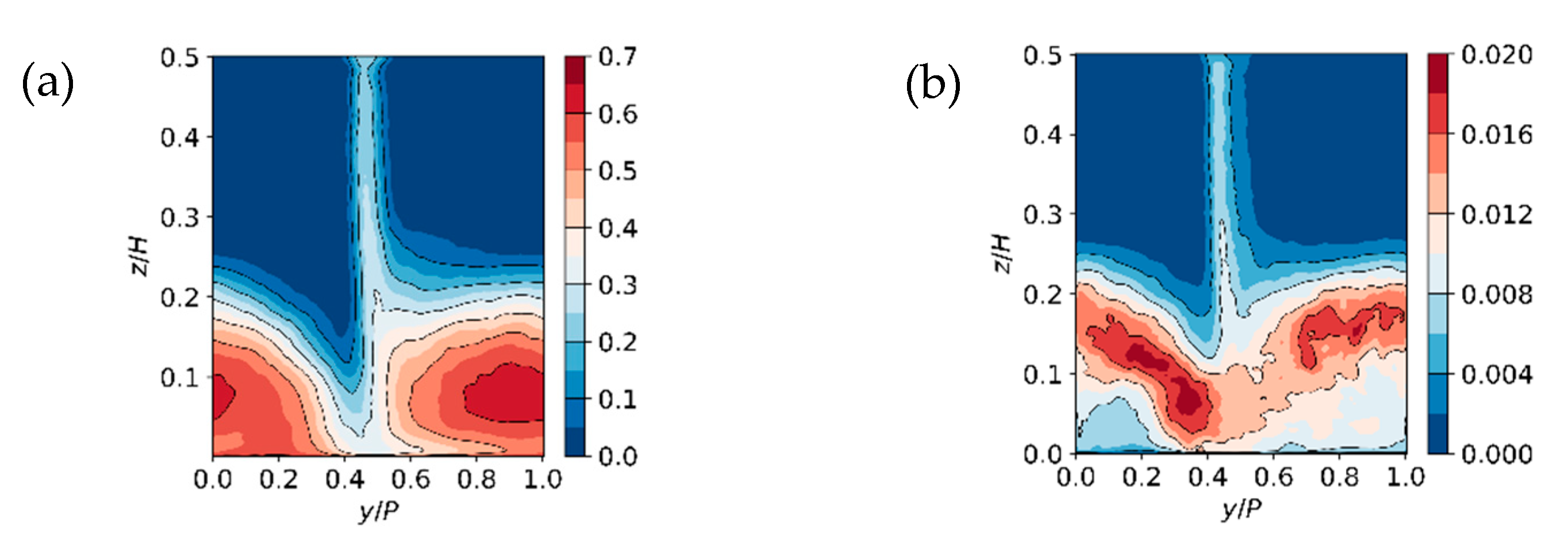
5.4.3. Impact on the Secondary Flow Structures
5.5. Work in Progress
6. Summary and Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| Roman Symbols | |
| ax | Axial Direction (for the annular cascade) |
| C | Chord |
| cf | Friction Coefficient |
| cp | Pressure Coefficient |
| D | Diameter |
| H | Passage Height, Channel Height |
| H12 | Shape Factor |
| Δht | Change in Total Enthalpy |
| M | Mach Number |
| Mass Flow | |
| P | Pitch Distance |
| p, pdyn, pt | Static, Dynamic and Total Pressure |
| r | Spanwise Direction (for the annular cascade) |
| Re | Reynolds Number |
| s | Gap Size |
| S | Distance along Blade Profile |
| Δs | Change in Entropy |
| Sr | Strouhal Number |
| t | Time |
| TBP | Bar Passing Period |
| TI | Turbulence Intensity [%] |
| u | Circumferential speed |
| v | Velocity |
| x | Axial Direction |
| y | Pitchwise Direction (for the linear cascade) |
| y+ | Non-Dimensional Wall Distance |
| z | Spanwise Direction (for the linear cascade) |
| Greek Symbols | |
| α | Yaw Angle, Flow Angle (1 for inflow, 2 for outflow) with respect to pitchwise direction |
| β | Flow Angle in Pitchwise Direction with respect to axial direction |
| δ | Boundary Layer Thickness |
| η | Normal Distance to a Wall |
| ζ | Total Pressure Loss Coefficient (Equation (1) for compressor, Equation (6) for turbine) |
| θ | Pitch Distance (For the annular cascade) |
| λ2 | 2nd Eigenvalue of Velocity Tensor |
| ρ | Density |
| τw | Wall Shear Stress |
| φ | Flow Coefficient |
| Abbreviations | |
| AVO | Axial Vorticity |
| b | Bar (used as a subscript) |
| BL | Boundary Layer |
| CFD | Computational Fluid Dynamics |
| CSV | Concentrated Shed Vortex |
| CTA | Constant Temperature Anemometry |
| CV | Corner Vortex |
| DNS | Direct Numerical Simulation |
| DP | Design Point |
| EARSM | Explicit Algebraic Reynolds Stress Model |
| EXP | Experiment |
| FHP | Five Hole Probe |
| FMP | Fast Measuring Pressure Probe |
| FS | Free Stream |
| FTT | Flow Through Time |
| HGK | High-Speed Cascade Wind Tunnel (Hochgeschwindigkeits-Gitterwindkanal) |
| HS | Half-Span |
| HSV | Horse Shoe Vortex |
| IGV | Inlet Guide Vane |
| LSRC | Low-Speed Research Compressor |
| LE | Leading Edge |
| LES | Large Eddy Simulation |
| LPT | Low Pressure Turbine |
| MCV | Million Control Volumes |
| MP | Measuring Plane |
| MS | Midspan |
| NI | National Instruments |
| PIV | Particle Image Velocimetry |
| PL | Pressure Side Leg |
| PS | Pressure Side |
| PV | Passage Vortex |
| QWSS | Quasi Wall Shear Stress |
| R1 | Rotor of Stage 1 |
| RANS | Reynolds Averaged Navier Stokes |
| Re | Reynolds Number |
| ref | Reference Value |
| RM | Relative Motion between Blade Tip and Adjacent Wall |
| rms | Root Mean Squared |
| S1 | Stator of Stage 1 |
| SAS | Scale Adaptive Simulation |
| sec | Secondary |
| SS | Suction Side |
| SVO | Streamwise Vorticity |
| TE | Trailing Edge |
| TKE | Turbulent Kinetic Energy |
| th | Theoretical, Thickened |
| TLV | Tip Leakage Vortex |
| URANS | Unsteady Reynolds Averaged Navier Stokes |
| WG | Wake Generator |
| WRLES | Wall-resolving LES |
References
- Von der Bank, R.; Donnerhack, S.; Rae, A.; Cazalens, M.; Lundbladh, A.; Dietz, M. LEMCOTEC: Improving the Core-Engine Thermal Efficiency. In Proceedings of the ASME Turbo Expo, Düsseldorf, Germany, 16–20 June 2014. GT2014-25040. [Google Scholar] [CrossRef]
- ETN Global. R&D Recommendation Report 2016—For the Next Generation of Gas Turbines—Revised Edition; ETN a.i.s.b.l.: Brussels, Belgium, 2017. [Google Scholar]
- To, H.; Miller, R. The Effect of Aspect Ratio on Compressor Performance. ASME J. Turbomach. 2019, 141, 081011. [Google Scholar] [CrossRef]
- Horlock, J.H.; Lakshminarayana, B. Secondary Flows: Theory, Experiment and Application in Turbomachinery Aerodynamics. Annu. Rev. Fluid Mech. 1973, 5, 247–280. [Google Scholar] [CrossRef]
- Peacock, R.E. A Review of Turbomachinery Tip Gap Effects: Part 1: Cascades. Int. J. Heat Fluid Flow 1982, 3, 185–193. [Google Scholar] [CrossRef]
- Peacock, R.E. A Review of Turbomachinery Tip Gap Effects: Part 2: Rotating Machinery. Int. J. Heat Fluid Flow 1983, 4, 3–16. [Google Scholar] [CrossRef]
- Sieverding, C.H. Recent Progress in the Understanding of Basic Aspects of Secondary Flows in Turbine Blade Passages. J. Eng. Gas Turbines Power 1985, 107, 248–257. [Google Scholar] [CrossRef]
- Liu, Y.; Tan, L.; Wang, B. A Review of Tip Clearance in Propeller, Pump and Turbine. Energies 2018, 11, 2202. [Google Scholar] [CrossRef]
- Bunker, R.S. A Review of Turbine Blade Tip Heat Transfer. Ann. N. Y. Acad. Sci. 2001, 934, 64–79. [Google Scholar] [CrossRef]
- Langston, L.S. Secondary Flows in Axial Turbines-A Review. Ann. N. Y. Acad. Sci. 2006, 934, 11–26. [Google Scholar] [CrossRef]
- Tyacke, J.; Vadlamani, N.R.; Trojak, W.; Watson, R.; Ma, Y.; Tucker, P.G. Turbomachinery Simulation Challenges and the Future. Prog. Aerosp. Sci. 2019, 110, 100554. [Google Scholar] [CrossRef]
- Mailach, R.; Vogeler, K. Recent German Research on Periodical Unsteady Flow in Turbomachinery. Flow Turbul. Combust. 2009, 83, 449–484. [Google Scholar] [CrossRef]
- Dring, R.P.; Joslyn, H.D.; Hardin, L.W.; Wagner, J.H. Turbine Rotor-Stator Interaction. ASME J. Eng. Power 1982, 104, 729–742. [Google Scholar] [CrossRef]
- Hodson, H.P.; Howell, R.J. Bladerow Interactions, Transition, and High-Lift Aerofoils in Low-Pressure Turbines. Annu. Rev. Fluid Mech. 2005, 37, 71–98. [Google Scholar] [CrossRef]
- Tucker, P.G. Computation of Unsteady Turbomachinery Flows: Part 1—Progress and Challenges. Prog. Aerosp. Sci. 2011, 47, 522–545. [Google Scholar] [CrossRef]
- Mailach, R. Unsteady Flow in Turbomachinery, Habilitation, Technische Universität Dresden, Dresden, 2020; TUD Press: Dresden, Germany, 2010; ISBN 978-3-941298-92-7. [Google Scholar]
- Pfeil, H.; Herbst, R.; Schröder, T. Investigation of the Laminar-Turbulent Transition of Boundary Layers Disturbed by Wakes. ASME J. Turbomach. 1983, 105, 130–137. [Google Scholar] [CrossRef]
- Hilgenfeld, L.; Pfitzner, M. Unsteady Boundary Layer Development Due to Wake Passing Effects on a Highly Loaded Linear Compressor Cascade. ASME J. Turbomach. 2004, 126, 493–500. [Google Scholar] [CrossRef]
- Ernst, M.; Michel, A.; Jeschke, P. Analysis of Rotor-Stator-Interaction and Blade-to-Blade Measurements in a Two Stage Axial Flow Compressor. ASME J. Turbomach. 2011, 133, 011027. [Google Scholar] [CrossRef]
- Smith, N.R.; Key, N.L. A Comprehensive Investigation of Blade Row Interaction Effects on Stator Loss Utilizing Vane Clocking. ASME J. Turbomach. 2018, 140, 071004. [Google Scholar] [CrossRef]
- Boos, P.; Möckel, H.; Henne, J.M.; Selmeier, R. Flow Measurement in a Multistage Large Scale Low Speed Axial Flow Research Compressor. In Proceedings of the ASME Int. Gas Turbine Aeroengine Congr. Exhib, Stockholm, Sweden, 2–5 June 1998. 98-GT-432. [Google Scholar]
- Künzelmann, M.; Mailach, R.; Müller, R.; Vogeler, K. Steady and Unsteady Flow Field in a Multistage Low-Speed Axial Compressor: A Test Case. In Proceedings of the ASME Turbo Expo, Berlin, Germany, 9–13 June 2008. GT2008-50793. [Google Scholar]
- Krug, A.; Busse, P.; Vogeler, K. Experimental investigation into the effects of the steady wake-tip clearance vortex interaction in a compressor cascade. ASME J. Turbomach. 2015, 137, 061006. [Google Scholar] [CrossRef]
- Lange, M.; Rolfes, M.; Mailach, R.; Schrapp, H. Periodic Unsteady Tip Clearance Vortex Development in a Low-Speed Axial Research Compressor at Different Tip Clearances. ASME J. Turbomach. 2018, 140, 031005. [Google Scholar] [CrossRef]
- Busse, P.; Krug, A.; Vogeler, K. Effects of the Steady Wake-Tip Clearance Vortex Interaction in a Compressor Cascade: Part II—Numerical Investigations. In Proceedings of the ASME Turbo Expo, Düsseldorf, Germany, 16–20 June 2014. GT2014-26121. [Google Scholar]
- Moore, R.W., Jr.; Richardson, D.L. Skewed Boundary-Layer Flow Near the End Walls of a Compressor Cascade. Trans. ASME 1957, 79, 1789–1800. [Google Scholar]
- Koppe, B.; Lange, M.; Mailach, R. Influence of Boundary Layer Skew on the Tip Leakage Vortex of an Axial Compressor Stator. In Proceedings of the ASME Turbo Expo, Virtual, Online, 21–25 September 2020. GT2020-15940. [Google Scholar]
- Jeong, J.; Hussain, F. On the identification of a vortex. J. Fluid Mech. 1995, 285, 69–94. [Google Scholar] [CrossRef]
- Hinterberger, C.; Fröhlich, J.; Rodi, W. 2D and 3D Turbulent Fluctuations in Open Channel Flow with Re τ = 590 Studied by Large Eddy Simulation. Flow Turbul. Combust. 2008, 80, 225–253. [Google Scholar] [CrossRef]
- Koschichow, D.; Fröhlich, J.; Kirik, I.; Niehuis, R. DNS of the flow near the endwall in a linear low pressure turbine cascade with periodically passing wakes. In Proceedings of the ASME Turbo Expo, Düsseldorf, Germany, 16–20 June 2014. GT2014-25071. [Google Scholar]
- Koschichow, D.; Fröhlich, J.; Ciorciari, R.; Niehuis, R. Analysis of the influence of periodic passing wakes on the secondary flow near the endwall of a linear LPT cascade using DNS and U-RANS. In Proceedings of the 11th European Conference on Turbomachinery Fluid Dynamics & Thermodynamics, Madrid, Spain, 23–25 March 2015. [Google Scholar]
- Baum, O.; Koschichow, D.; Fröhlich, J. Influence of the Coriolis Force on the Flow in a Low Pressure Turbine Cascade T106. In Proceedings of the ASME Turbo Expo, Seoul, Korea, 13–17 June 2016. GT2016-57399. [Google Scholar]
- Nicoud, F.; Ducros, F. Subgrid-Scale Stress Modelling Based on the Square of the Velocity Gradient Tensor. FlowTurbul. Combust. 1999, 62, 183–200. [Google Scholar] [CrossRef]
- Piomelli, U.; Chasnov, J.R. Large-Eddy Simulations: Theory and Applications. In Turbulence and Transition Modelling; Hallbäck, M., Henningson, D.S., Johansson, A.V., Alfredsson, P.H., Eds.; Springer: Dordrecht, The Netherlands, 1996; pp. 269–336. [Google Scholar] [CrossRef]
- Ventosa-Molina, J.; Lange, M.; Mailach, R.; Fröhlich, J. Study of Relative Endwall Motion Effects in a Compressor Cascade Through Direct Numerical Simulations. ASME J. Turbomach. 2021, 143, 011005. [Google Scholar] [CrossRef]
- Michelassi, V.; Wissink, J.G.; Fröhlich, J.; Rodi, W. Large-Eddy Simulation of Flow Around Low-Pressure Turbine Blade with Incoming Wakes. Aiaa J. 2003, 41, 2143–2156. [Google Scholar] [CrossRef]
- Inoue, M.; Kuroumaru, M. Structure of Tip Clearance Flow in an Isolated Axial Compressor Rotor. ASME J. Turbomach. 1989, 111, 250–256. [Google Scholar] [CrossRef]
- Storer, J.; Cumpsty, N. Tip Leakage Flow in Axial Compressors. ASME J. Turbomach. 1991, 113, 252–259. [Google Scholar] [CrossRef]
- Wissink, J.G.; Rodi, W. Numerical study of the near wake of a circular cylinder. Int. J. Heat Fluid Flow 2008, 29, 1060–1070. [Google Scholar] [CrossRef]
- Niehuis, R.; Bitter, M. The High-Speed Cascade Wind Tunnel at the Bundeswehr University Munich after a Major Revision and Upgrade. In Proceedings of the 14th European Conference on Turbomachinery Fluid dynamics & Thermodynamics, Gdansk, Poland, 12–16 April 2021. [Google Scholar]
- Ciorciari, R.; Kirik, I.; Niehuis, R. Effects of Unsteady Wakes on Secondary Flows in the Linear T106 Turbine Cascade. ASME J. Turbomach. 2014, 136, 091010. [Google Scholar] [CrossRef]
- Kirik, I.; Niehuis, R. Comparing the Effect of Unsteady Wakes on Parallel and Divergent Endwalls in a LP Turbine Cascade. In Proceedings of the International Gas Turbine Congress, Tokyo, Japan, 15–20 November 2015. IGTC2015-137. [Google Scholar]
- Kirik, I.; Niehuis, R. Experimental Investigation on Effects of Unsteady Wakes on the Secondary Flows in the Linear T106 Turbine Cascade. In Proceedings of the ASME Turbo Expo, Montreal, Canada, 15–19 June 2015. GT2015-43170. [Google Scholar]
- Kirik, I.; Niehuis, R. Influence of Unsteady Wakes on the Secondary Flows in the Linear T106 Turbine Cascade. In Proceedings of the ASME Turbo Expo, Seoul, Korea, 13–17 June 2016. GT2016-56350. [Google Scholar]
- Schubert, T.; Chemnitz, S.; Niehuis, R. The Effects of Inlet Boundary Layer Condition and Periodically Incoming Wakes on Secondary Flow in a Low Pressure Turbine Cascade. ASME J. Turbomach. 2021, 143, 041001. [Google Scholar] [CrossRef]
- Wilcox, D.C. Turbulence Modeling for CFD, 4th ed.; DCW Industries: La Canada, FL, USA, 2004. [Google Scholar]
- Langtry, R.B.; Menter, F.R. Transition Modeling for General CFD Applications in Aeronautics. In Proceedings of the 43rd AIAA Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 10–13 January 2005. AIAA 2005-522. [Google Scholar]
- Schubert, T.; Niehuis, R. Numerical Investigation of Loss Development in a Low-Pressure Turbine Cascade with Unsteady Inflow and Varying Inlet Endwall Boundary Layer. In Proceedings of the ASME Turbo Expo, Virtual, Online, 7–11 June 2021. GT2021-59696, to be published. [Google Scholar]
- Ciorciari, R.; Schubert, T.; Niehuis, R. Numerical Investigation of Secondary Flow and Loss Development in a Low Pressure Turbine Cascade with Divergent Endwalls. Int. J. Turbomach. Propuls. Power 2018, 3, 5. [Google Scholar] [CrossRef]
- Mayle, R. The 1991 IGTI Scholar Lecture: The Role of Laminar-Turbulent Transition in Gas Turbine Engines. ASME J. Turbomach. 1991, 113, 509–536. [Google Scholar] [CrossRef]
- Sinkwitz, M.; Engelmann, D.; Mailach, R. Experimental investigation of periodically unsteady wake impact on the secondary flow in a 1.5 stage full annular LPT cascade with modified T106 blading. In Proceedings of the ASME Turbo Expo, Charlotte, NC, USA, 26–30 June 2017. GT2017-64390. [Google Scholar]
- Sinkwitz, M.; Winhart, B.; Engelmann, D.; di Mare, F.; Mailach, R. Experimental and Numerical Investigation of Secondary Flow Structures in an Annular LPT Cascade under Periodic Wake Impact–Part 1: Experimental Results. ASME J. Turbomach. 2019, 141, 021008. [Google Scholar] [CrossRef]
- Sinkwitz, M.; Winhart, B.; Engelmann, D.; di Mare, F.; Mailach, R. On the Periodically Unsteady Interaction of Wakes, Secondary Flow Development, and Boundary Layer Flow in An Annular Low-Pressure Turbine Cascade: An Experimental Investigation. ASME J. Turbomach. 2019, 141, 091001. [Google Scholar] [CrossRef]
- Sinkwitz, M.; Winhart, B.; Engelmann, D.; di Mare, F. Time-Resolved Measurements of the Unsteady Boundary Layer in an Annular Low-Pressure Turbine Configuration with Perturbed Inlet. In Proceedings of the ASME Turbo Expo, Virtual, Online, 21–25 September 2020. GT2020-15319. [Google Scholar]
- Winhart, B.; Sinkwitz, M.; Engelmann, D.; di Mare, F.; Mailach, R. On the Periodically Unsteady Interaction of Wakes, Secondary Flow Development and Boundary Layer Flow in an Annular LPT Cascade. Part 2–Numerical Investigation. In Proceedings of the ASME Turbo Expo, Oslo, Norway, 11–15 June 2018. GT2020-76873. [Google Scholar]
- Winhart, B.; Sinkwitz, M.; Schramm, A.; Engelmann, D.; di Mare, F.; Mailach, R. Experimental and Numerical Investigation of Secondary Flow Structures in an Annular LPT Cascade under Periodic Wake Impact–Part 2: Numerical Results. ASME J. Turbomach. 2019, 141, 021009. [Google Scholar] [CrossRef]
- Winhart, B.; Sinkwitz, M.; Schramm, A.; Post, P.; di Mare, F. Large-Eddy Simulation of Periodic Wake Impact on Boundary Layer Transition Mechanisms on a Highly Loaded Low-Pressure Turbine Blade. In Proceedings of the ASME Turbo Expo, Virtual, Online, 21–25 September 2020. GT2020-14555. [Google Scholar]
- Sinkwitz, M. Experimentelle Untersuchung der Entstehung von Sekundärstömung in Turbinen-Ringgittern unter Periodisch-Instationärer Zuströmung. Ph.D. Thesis, Ruhr-Universität Bochum, Universitätsbibliothek, Bochum, Germany, 2021. [Google Scholar] [CrossRef]
- Hodson, H.P.; Howell, R.J. The Role of Transition in High-Lift Low-Pressure Turbines for Aeroengines. Prog. Aerosp. Sci. 2005, 41, 419–454. [Google Scholar] [CrossRef]
- Stieger, R.D.; Hodson, H.P. The Transition Mechanism of Highly Loaded Low-Pressure Turbine Blades. ASME J. Turbomach. 2004, 126, 536–543. [Google Scholar] [CrossRef]
- Hodson, H.P.; Huntsman, I.; Steele, A.B. An Investigation of Boundary Layer Development in a Multistage LP Turbine. ASME J. Turbomach. 1994, 116, 375–383. [Google Scholar] [CrossRef]
- Halstead, D.E.; Wisler, D.C.; Okiishi, T.H.; Walker, G.J.; Hodson, H.P.; Shin, H.-W. Boundary Layer Development in Axial Compressors and Turbines: Part 1 of 4—Composite Picture. ASME J. Turbomach. 1997, 119, 114–127. [Google Scholar] [CrossRef]
- Mahallati, A.; Sjolander, S.A. Aerodynamics of a Low-Pressure Turbine Airfoil at Low-Reynolds Numbers -Part II: Blade-Wake Interaction. ASME J. Turbomach. 2013, 135, 011011. [Google Scholar] [CrossRef]
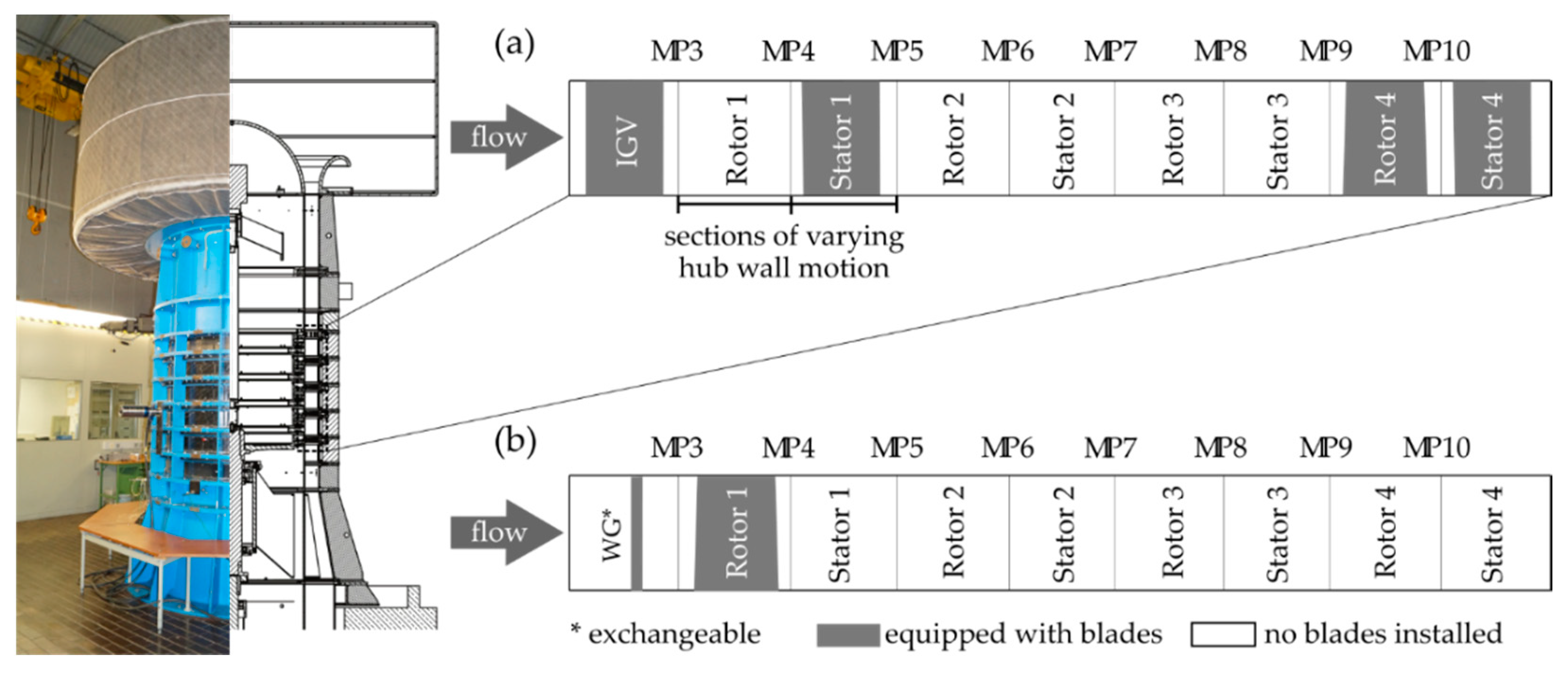







| Test Rig | Operating Point | ||
|---|---|---|---|
| Shroud diameter | 1500 mm | Rotational speed at DP | 1000 rpm |
| Hub to tip ratio | 0.84 | Mass flow at DP | 25.35 kg/s |
| Rotor R1 | Stator S1 | ||
| No. of blades | 63 | No. of vanes | 83 |
| Chord length | 110 mm | Chord length | 89 mm |
| Solidity, MS | 1.597 | Solidity, MS | 1.709 |
| Reynolds number at entry, MS | Reynolds number at entry, MS | ||
| Mach number at entry, MS | 0.25 | Mach number at entry, MS | 0.18 |
| Flow coefficient φ, MS 1 | 0.651 | Diffusion factor, MS | 0.37 |
| Loading coefficient , MS 1 | 0.489 | ||
| Configuration | Rotor R1 Hub Wall | Stator S1 Hub Wall |
|---|---|---|
| Un-skewed, w/o RM | Stationary | Stationary |
| Skewed, w/o RM | Rotating | Stationary |
| Skewed, with RM | Rotating | Rotating |
| Geometric Parameters: | |
|---|---|
| Chord length C | 100 mm |
| Pitch-to-chord ratio P/C | 0.799 |
| Aspect ratio H/C | 1.31 |
| Flow Conditions: | |
| Mach number at exit | 0.59 |
| Reynolds number at exit | |
| Design inflow pitch angle | 127.7° |
| Design outflow pitch angle | 26.8° |
| Turbulence intensity TI | 6.8% |
| Unsteady Inflow Conditions: | |
| Strouhal number Sr | 0.66 |
| Flow coefficient φ | 3.8 |
| Test Rig | Turbine Stage | ||
|---|---|---|---|
| Outer diameter (Casing) | 1660 mm | Chord length IGV | 137 mm |
| Inner diameter (Hub) | 1320 mm | Stagger angle IGV | −25.5° |
| Chord length T106RUB | 100 mm | ||
| Stagger angle T106RUB | 30.7° | ||
| Blade count IGV, T106RUB | 60 | ||
| Operating Point, Design Point | Design Flow Angles, Midspan | ||
| Mass flow | 12.8 kg/s | IGV inlet | 90.0° |
| Reynolds number at exit | IGV outlet = T106RUB inlet | 52.3° | |
| Mach number at exit | 0.091 | T106RUB outlet | 153.2° |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY-NC-ND) license (https://creativecommons.org/licenses/by-nc-nd/4.0/).
Share and Cite
Engelmann, D.; Sinkwitz, M.; di Mare, F.; Koppe, B.; Mailach, R.; Ventosa-Molina, J.; Fröhlich, J.; Schubert, T.; Niehuis, R. Near-Wall Flow in Turbomachinery Cascades—Results of a German Collaborative Project. Int. J. Turbomach. Propuls. Power 2021, 6, 9. https://doi.org/10.3390/ijtpp6020009
Engelmann D, Sinkwitz M, di Mare F, Koppe B, Mailach R, Ventosa-Molina J, Fröhlich J, Schubert T, Niehuis R. Near-Wall Flow in Turbomachinery Cascades—Results of a German Collaborative Project. International Journal of Turbomachinery, Propulsion and Power. 2021; 6(2):9. https://doi.org/10.3390/ijtpp6020009
Chicago/Turabian StyleEngelmann, David, Martin Sinkwitz, Francesca di Mare, Björn Koppe, Ronald Mailach, Jordi Ventosa-Molina, Jochen Fröhlich, Tobias Schubert, and Reinhard Niehuis. 2021. "Near-Wall Flow in Turbomachinery Cascades—Results of a German Collaborative Project" International Journal of Turbomachinery, Propulsion and Power 6, no. 2: 9. https://doi.org/10.3390/ijtpp6020009
APA StyleEngelmann, D., Sinkwitz, M., di Mare, F., Koppe, B., Mailach, R., Ventosa-Molina, J., Fröhlich, J., Schubert, T., & Niehuis, R. (2021). Near-Wall Flow in Turbomachinery Cascades—Results of a German Collaborative Project. International Journal of Turbomachinery, Propulsion and Power, 6(2), 9. https://doi.org/10.3390/ijtpp6020009







