Aerodynamic and Aeroelastic Effects of Design-Based Geometry Variations on a Low-Pressure Compressor
Abstract
1. Introduction
1.1. Engine Performance and Aerodynamics
1.2. Forced Response Analysis
1.3. Main Objectives
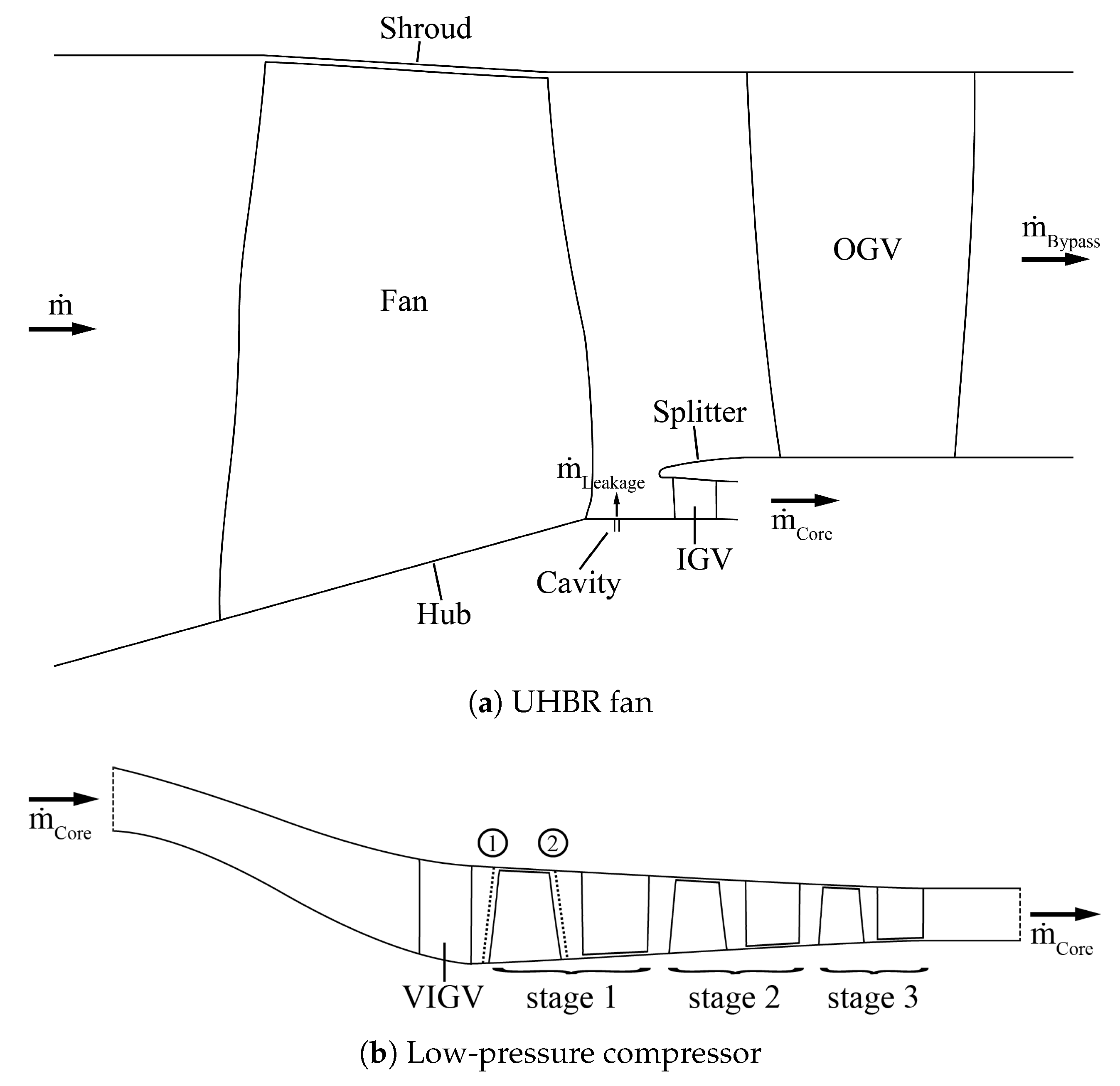
2. Fan Configuration and Low-Pressure Compressor
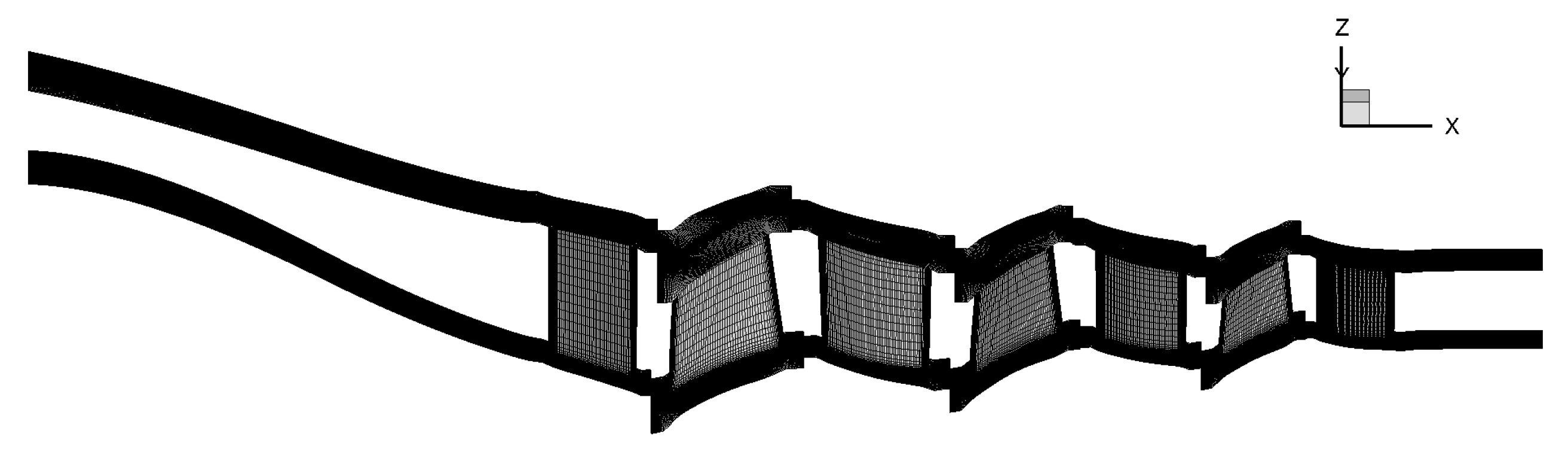
3. Numerical Setup
3.1. Aerodynamic Simulations
3.2. Aeroelastic Simulations
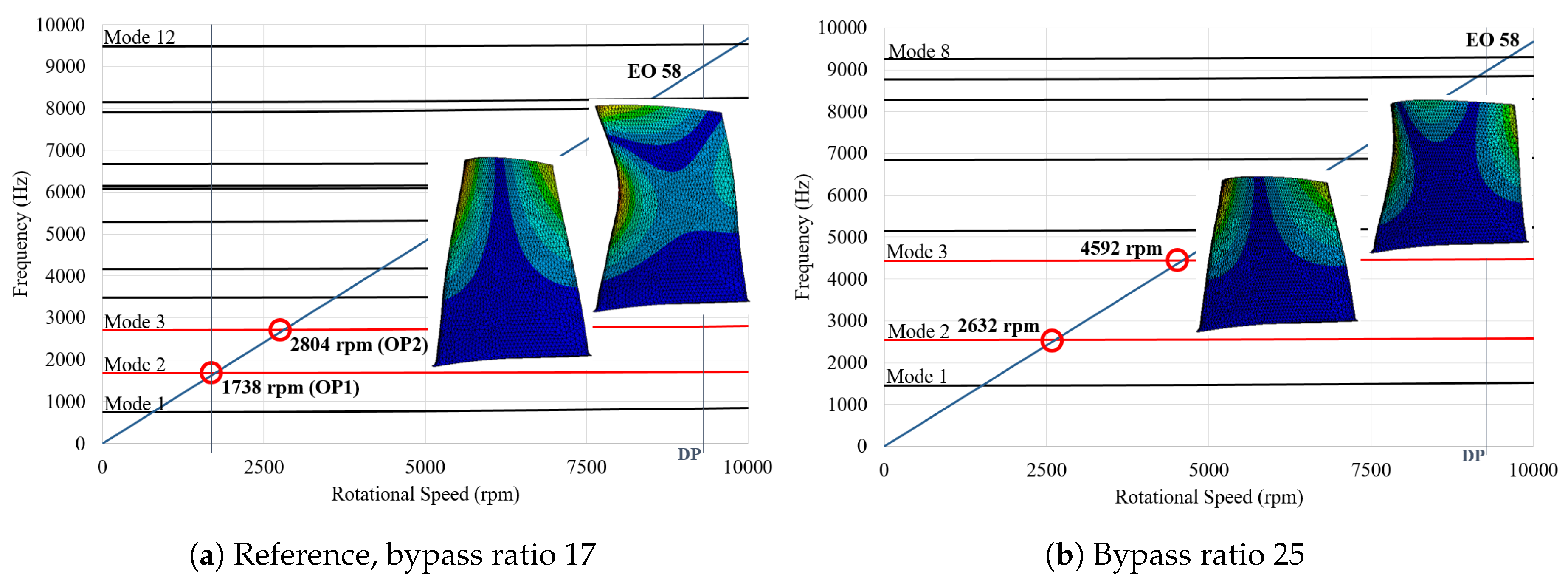
3.2.1. Modal Analysis and Campbell Diagram
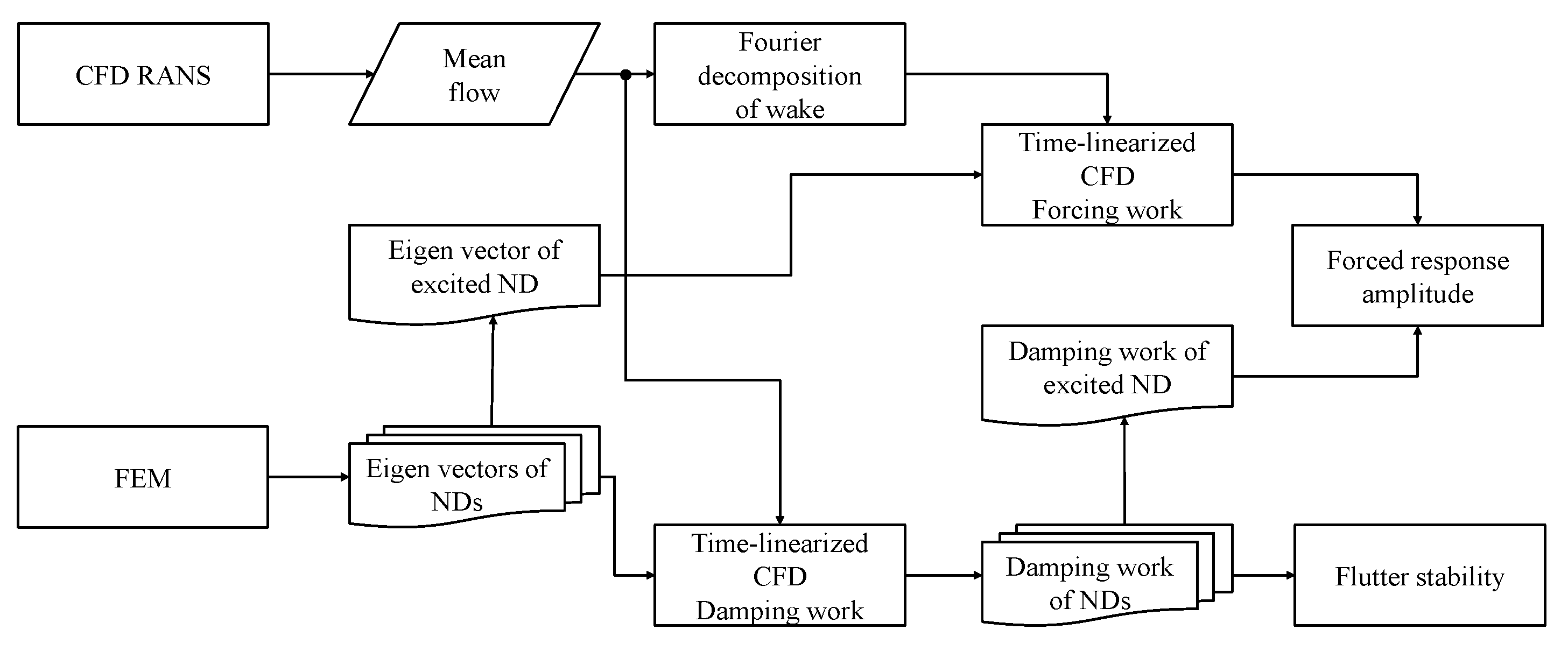
3.2.2. Time-Linearized Aeroelastic Simulation
4. Results and Discussion
4.1. General
4.1.1. Aerodynamics
4.1.2. Aeroelasticity
4.2. Results of Parametric Variations
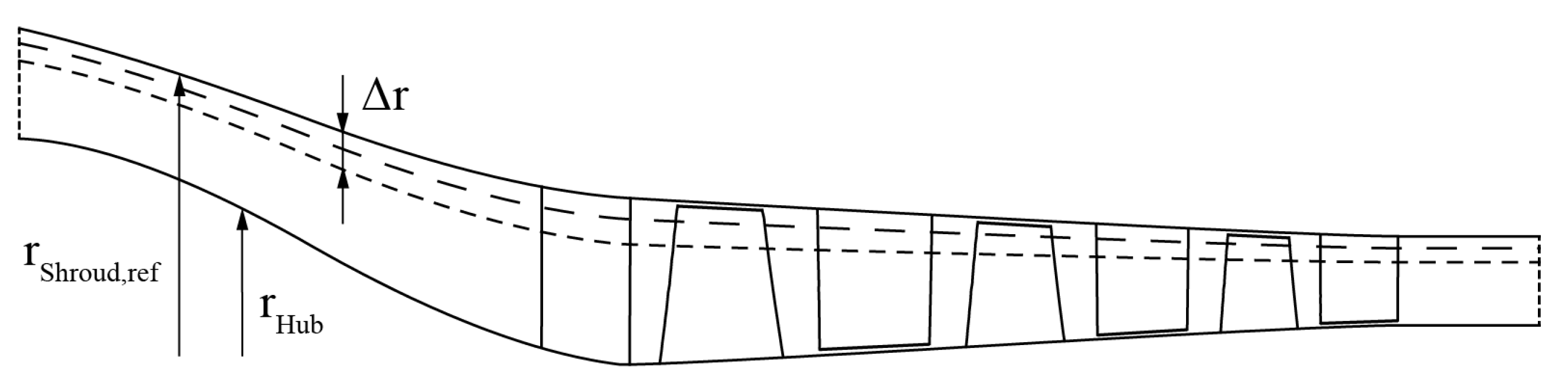
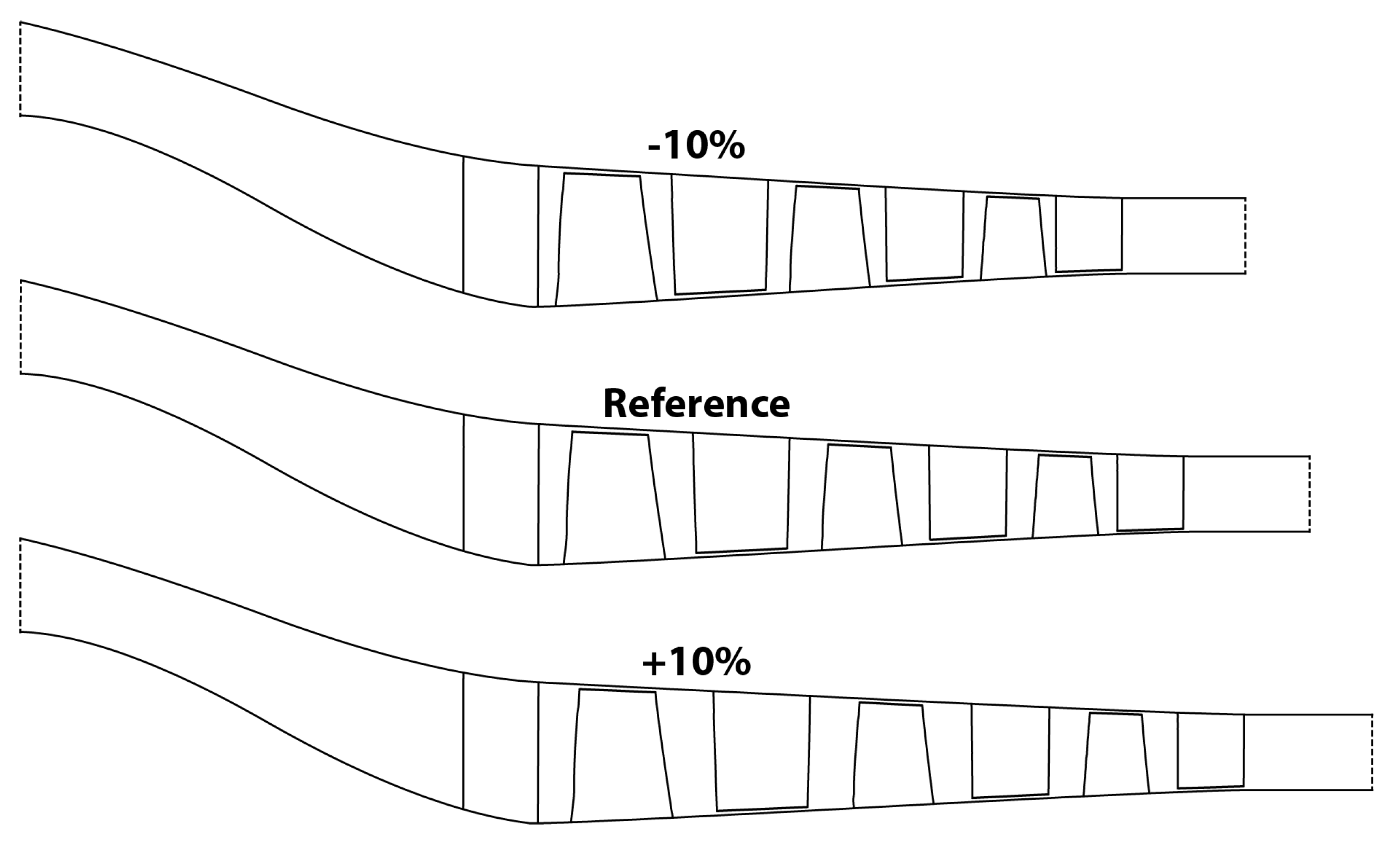
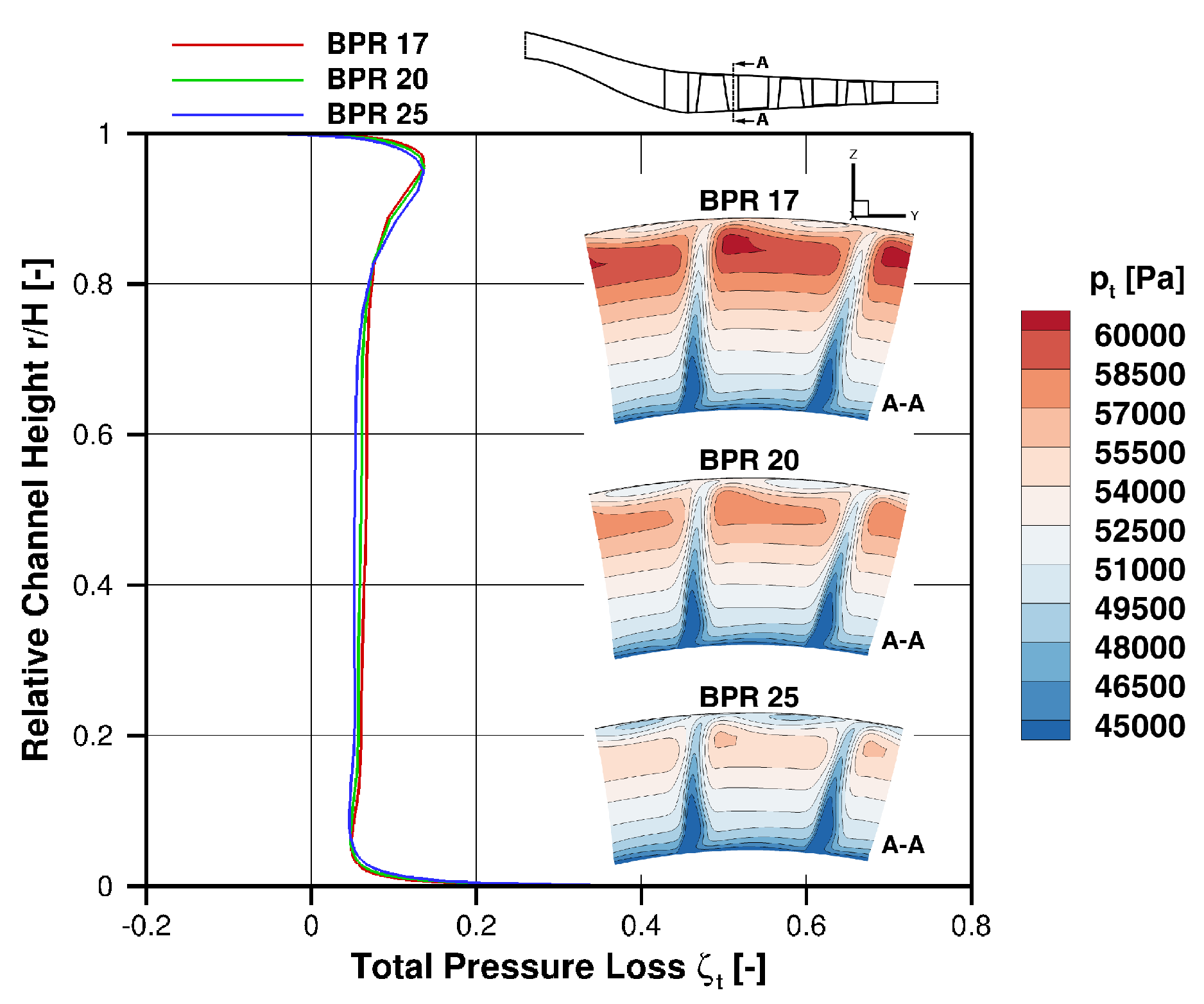
4.2.1. Increased Bypass Ratio
Aerodynamic Results
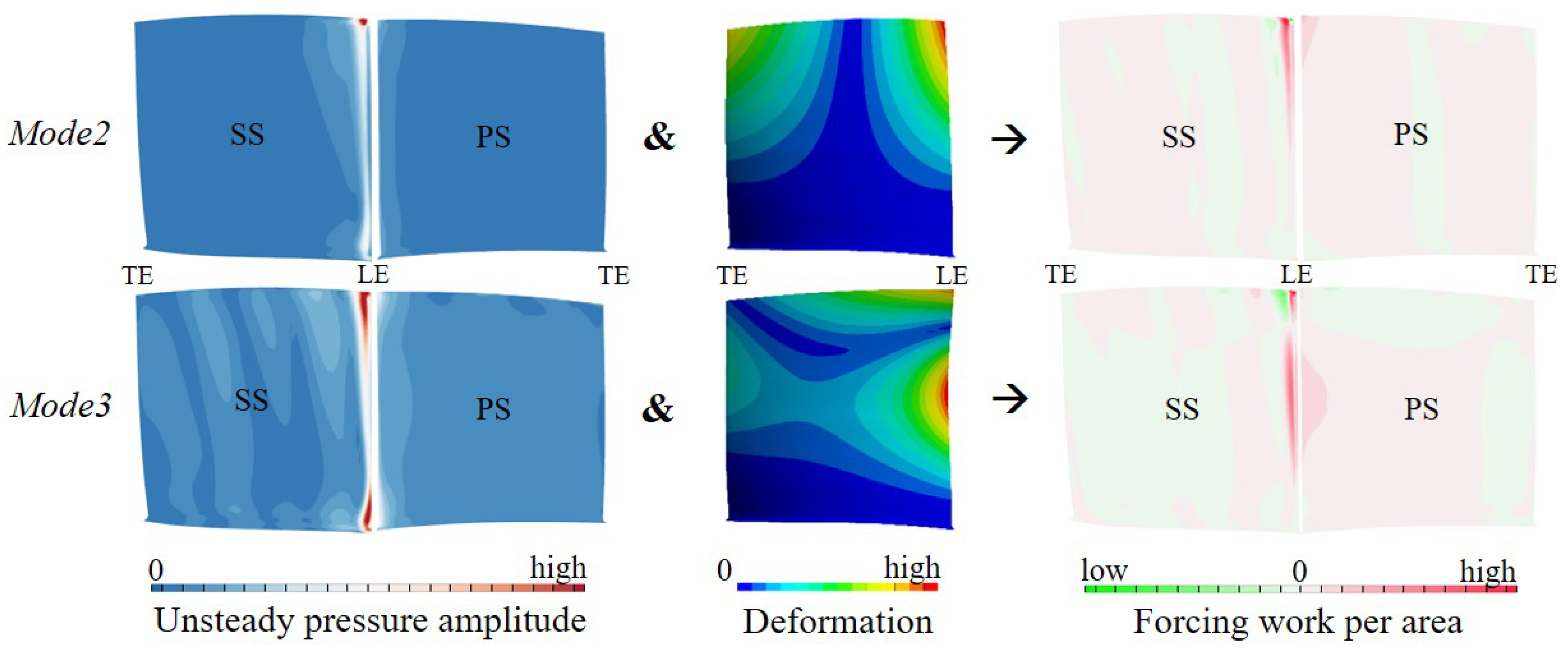
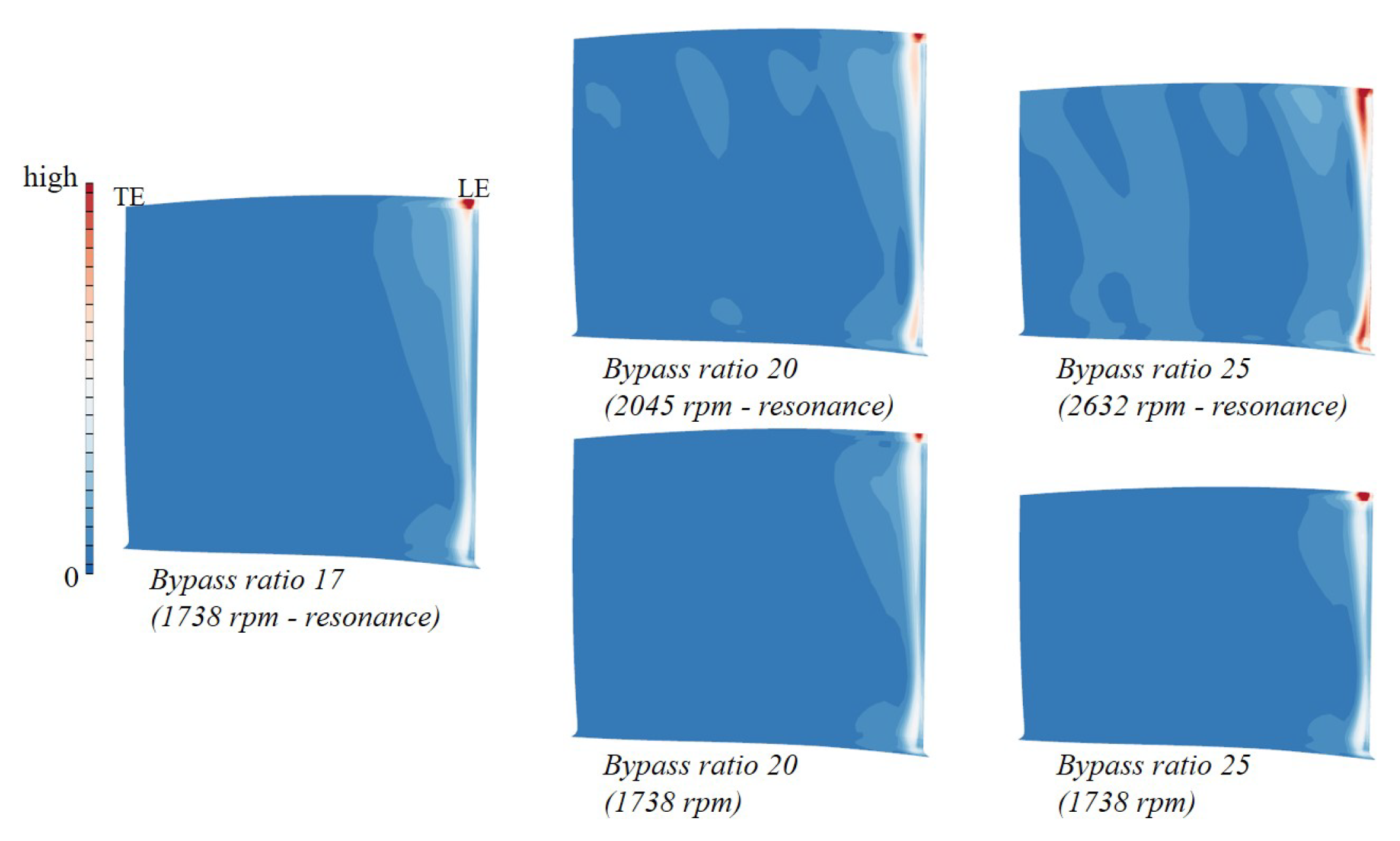
Aeroelastic Results
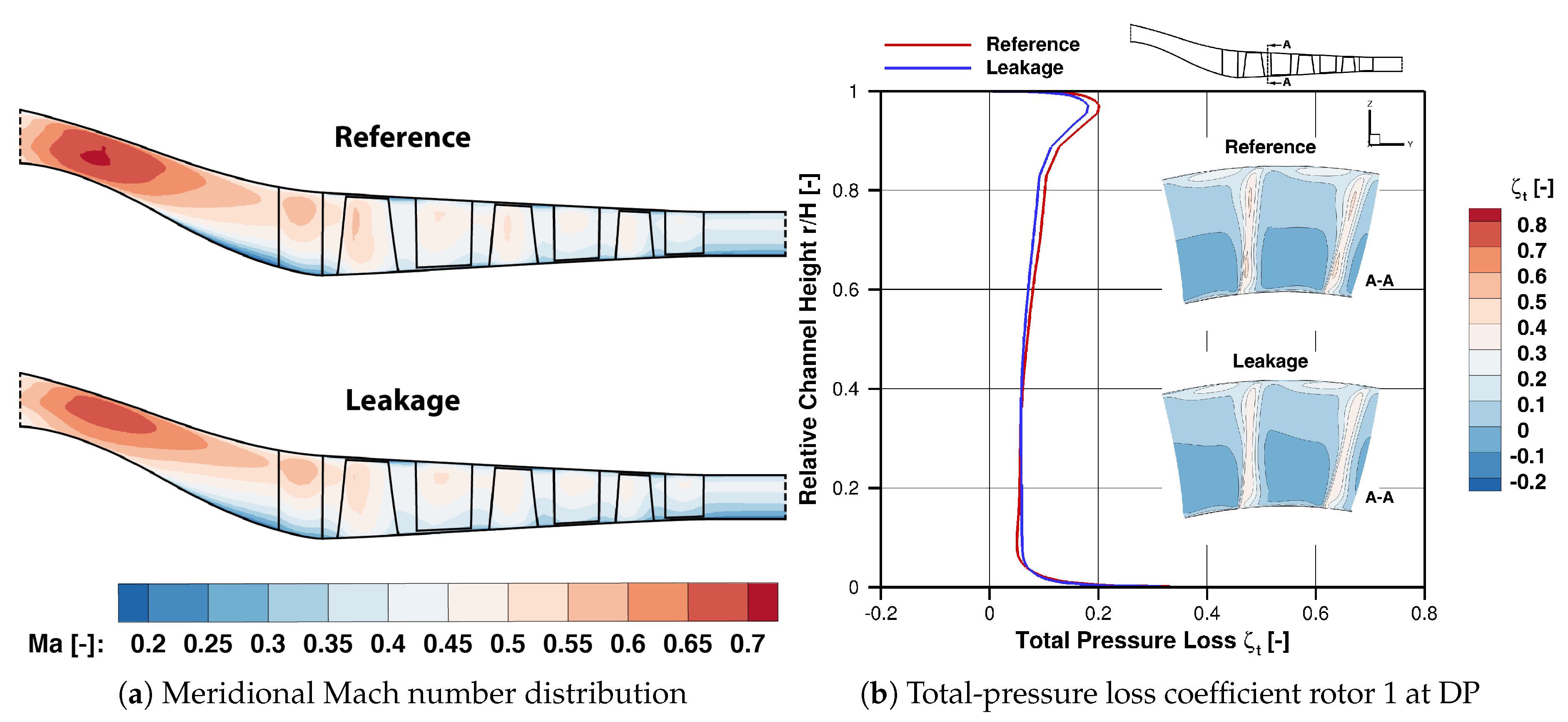
4.2.2. Effect of the Fan Rotor Cavity Flow
Aerodynamic Results
Aeroelastic Results
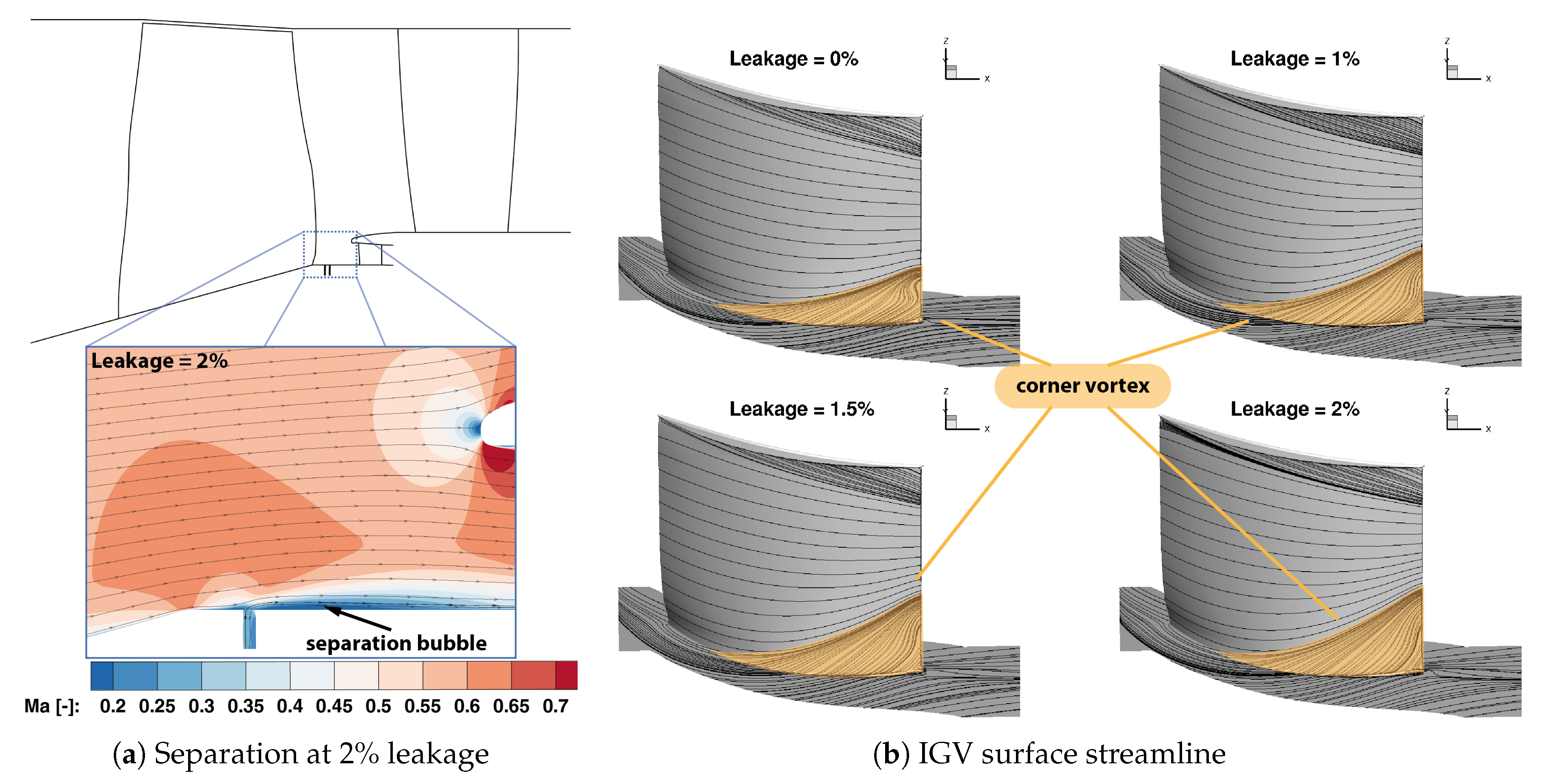
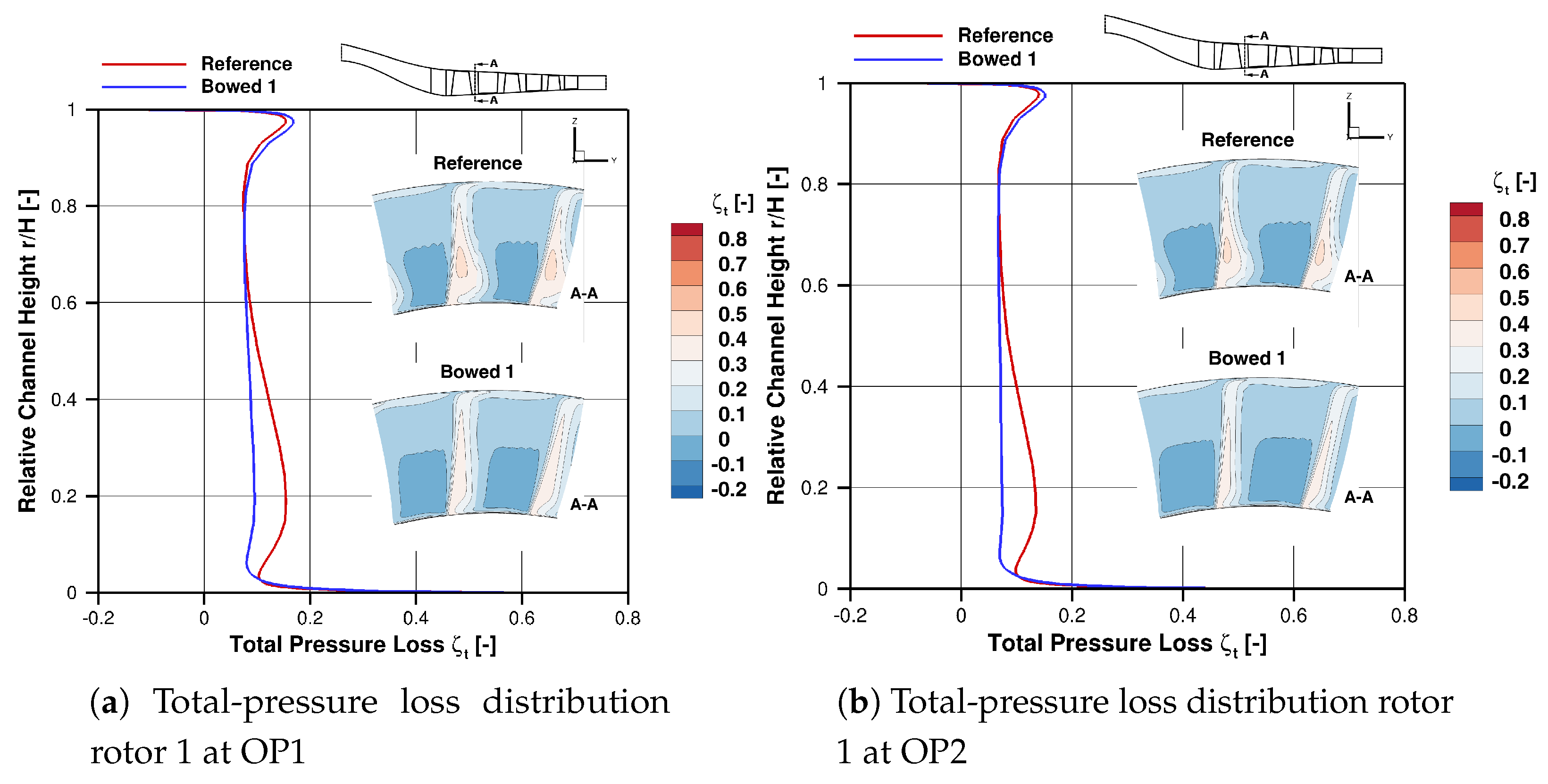
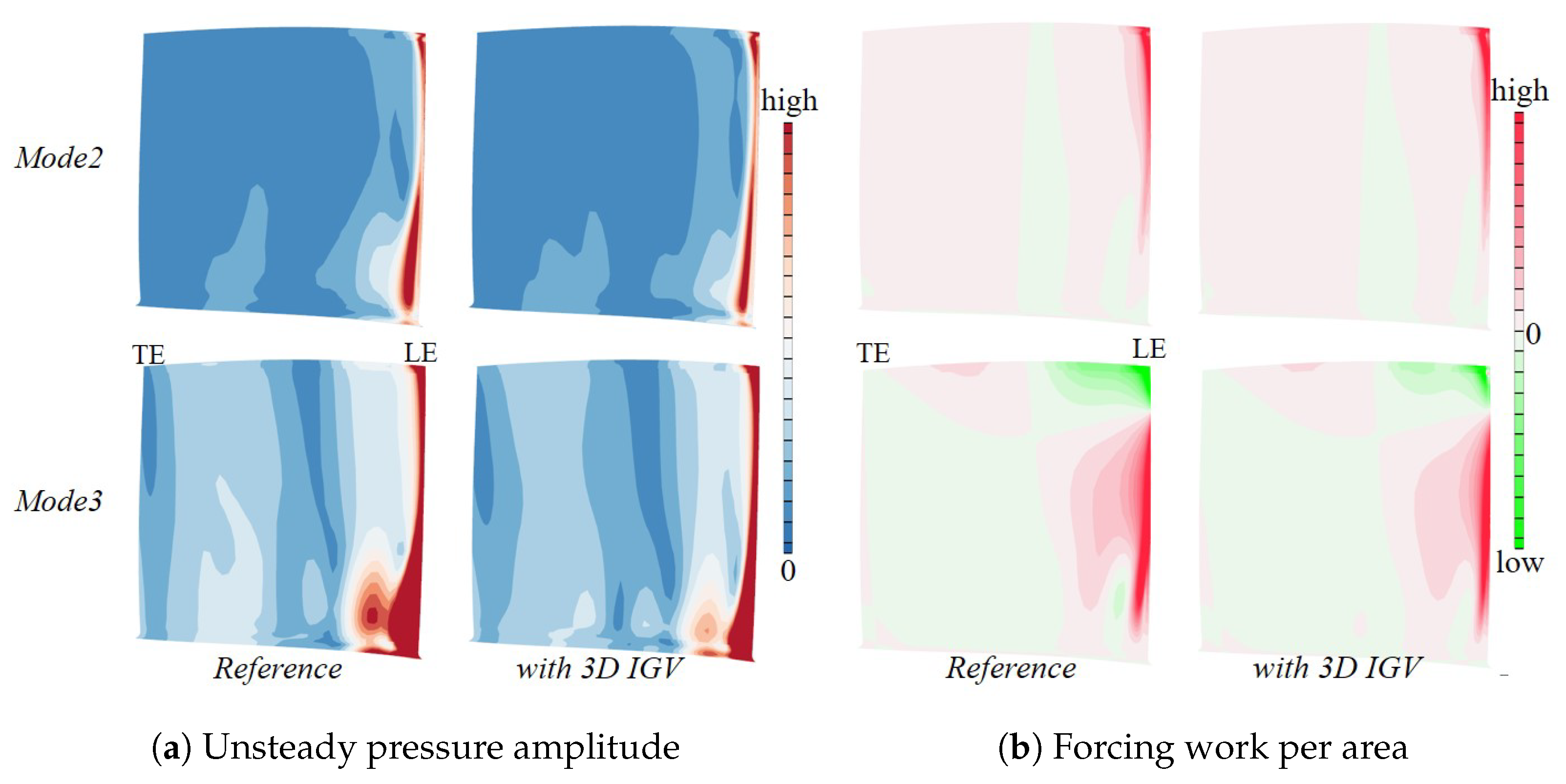
4.2.3. Sweep and Lean of the IGV
Aerodynamic Results
Aeroelastic Results
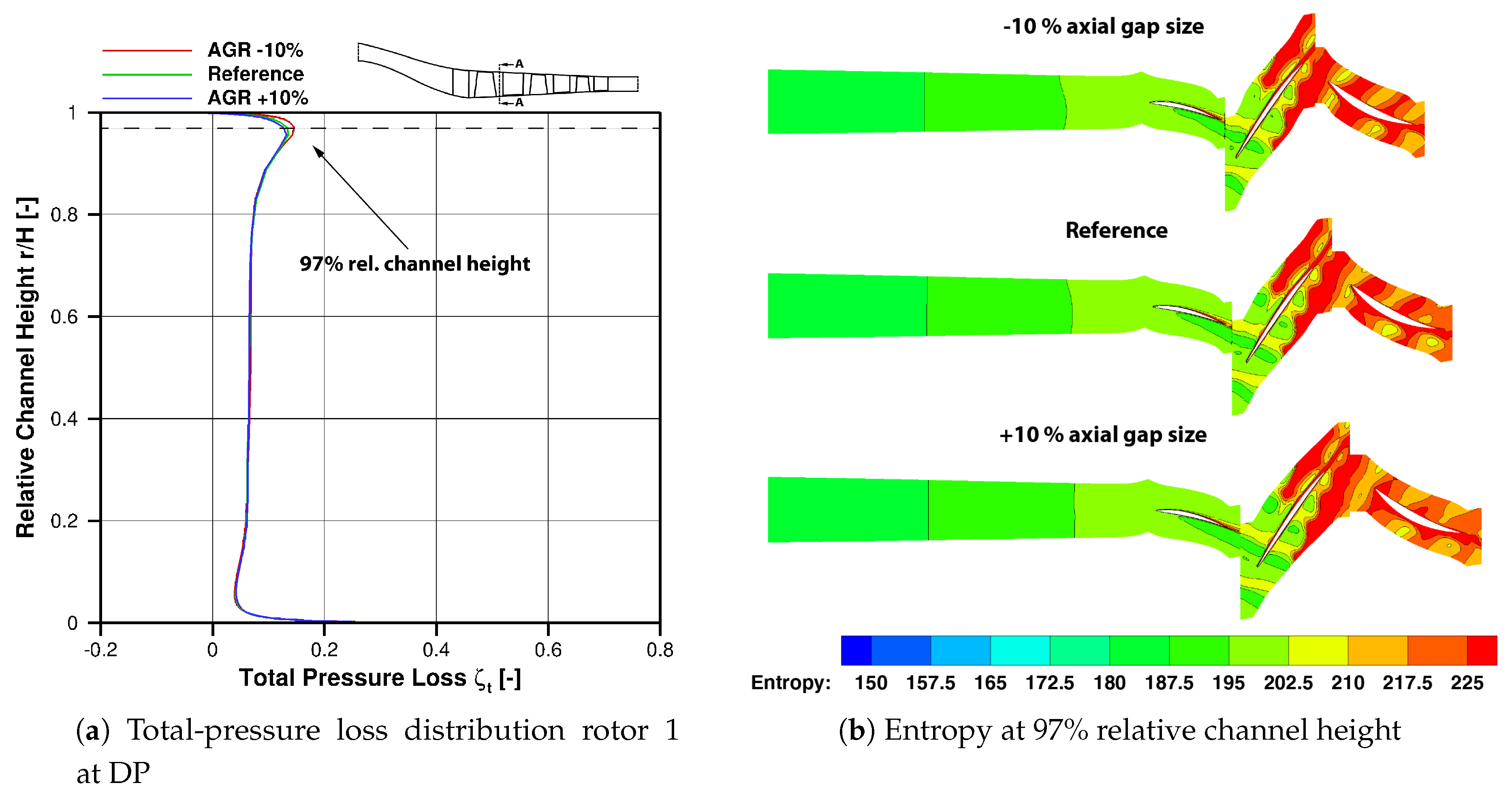
4.2.4. Impact of the Axial Gap Size
Aerodynamic Results
Aeroelastic Results
5. Conclusions
- The increase in the bypass ratio by lowering the compressor channel height increases the blockage of the flow and leads to a decrease in the overall efficiency of up to for the LPC. However, the forced response amplitude is mainly sensitive to the change of the incidence angle while the increased blockage effect is negligible.
- The flow from the fan rotor cavity increases the mixing loss in the s-duct () and redistributes the flow, eventually decreases total-pressure loss of rotor 1 by . This should be taken into account during the design process, because it causes a shift in the operating point. However, the fan leakage flow degrades the aerodynamic performance at off-design, and leads to an increase in vibration amplitude of for Mode 2 and for Mode 3. The implementation of an optimized cavity geometry is necessary to minimize the influence of the leakage flow on the core engine flow.
- The sweep and lean of the IGV downstream of the fan has a negligible effect on the aerodynamic performance of the LPC at the design point but leads to significant improvement of the off-design performance by reducing near hub corner vortices. The total-pressure loss of rotor 1 is reduced by up to at OP1 compared to the reference case. This achieves a and reduction of vibration amplitude for Modes 2 and 3, respectively.
- Varying the axial gap between blade rows in the LPC within +/− shows no visible aerodynamic and aeroelastic sensitivity which is promising to reduce the length of the LPC. The vibration amplitude of Mode 3 increases slightly with increasing axial gap because of the bending mode shape. The general correlation should be addressed by further investigations on higher modes.
- The bypass ratio variation and the fan cavity flow have the greatest importance on forced response within this study since these considerably alter the VIGV wake over the entire span. This also applies to the aerodynamic behavior, as the bypass ratio and the fan cavity leakage directly influence the fan design point. For off-design, the three-dimensional blade design has the greatest impact on the aerodynamic performance.
6. Suggestions of Future Works
- Whereas RANS simulations have been used for the aerodynamic analysis in the present paper, URANS methods should be applied in the future. The sensitivities might be more accurately evaluated using URANS.
- For the forced response evaluation, the harmonic balance method could be adapted instead of time-linearized RANS to determine the influence of other harmonics.
- The range of parameter variations could be extended for a more comprehensive sensitivity study.
- To adjust for the changing blade loading at higher bypass ratios, the LPC blades should be redesigned.
- The forced response of higher eigenmodes, especially those expected near the design speed of the LPC, should be investigated in order to further improve the design validation.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
Abbreviations
| 1 | inlet | |
| 2 | outlet | |
| aspect ratio | ||
| axial velocity | ||
| H | channel height | |
| specific stagnation enthalpy | ||
| axial chord length from meridional view | ||
| mass flow | ||
| Mach number | ||
| p | static pressure | |
| total pressure | ||
| r | radius | |
| r | reference radius | |
| total temperature | ||
| U | circumferential velocity | |
| v | absolute velocity | |
| vibration amplitude of forced response | ||
| coordinate system | ||
| radius offset | ||
| flow angle | ||
| relative flow angle | ||
| polytropic efficiency | ||
| isentropic exponent | ||
| density | ||
| flow coefficient | ||
| work coefficient | ||
| circumferential direction | ||
| total-pressure loss coefficient | ||
| BPR | bypass ratio | |
| CFD | computational fluid dynamics | |
| DP | design point | |
| EO | engine order | |
| GCI | grid convergence index | |
| IGV | inlet guide vane | |
| LE | leading edge | |
| LPC | low-pressure compressor | |
| OGV | outlet guide vane | |
| PS | puction side | |
| SS | suction side | |
| TE | trailing edge | |
| TOC | top of climb | |
| UHBR | ultra-high bypass ratio | |
| VIGV | variable inlet guide vane |
References
- Horlock, J.H.; Lakshminarayana, B. Secondary Flows: Theory, Experiment, and Application in Turbomachinery Aerodynamics. Annu. Rev. Fluid Mech. 1973, 5, 247–280. [Google Scholar] [CrossRef]
- Zamboni, G.; Xu, L. Fan Root Aerodynamics for Large Bypass Gas Turbine Engines: Influence on the Engine Performance and 3D Design. J. Turbomach. 2012, 134, 061017. [Google Scholar] [CrossRef]
- Dawes, W.N. Multi-Blade Row Navier-Stokes Simulations of Fan-Bypass Configurations. In Proceedings of the ASME 1991 International Gas Turbine and Aeroengine Congress and Exposition, Orlando, FL, USA, 3–6 June 1991; American Society of Mechanical Engineers: New York, NY, USA, 1991. 7p. [Google Scholar] [CrossRef]
- Eggers, T.; Friedrichs, J. Effects of Increasing Bypass Ratio of UHBR Fans on Near Hub Flow. J. Glob. Power Propuls. Soc. 2019. [Google Scholar] [CrossRef]
- Goyal, R.K.; Dawes, W.N. A Comparison of the Measured and Predicted Flow Field in a Modern Fan-Bypass Configuration. J. Turbomach. 1993, 115, 273. [Google Scholar] [CrossRef]
- Wellborn, S.R.; Okiishi, T.H. The Influence of Shrouded Stator Cavity Flows on Multistage Compressor Performance. J. Turbomach. 1999, 121, 486. [Google Scholar] [CrossRef]
- Gallimore, S.J.; Bolger, J.J.; Cumpsty, N.A.; Taylor, M.J.; Wright, P.I.; Place, J.M.M. The Use of Sweep and Dihedral in Multistage Axial Flow Compressor Blading—Part I: University Research and Methods Development. J. Turbomach. 2002, 124, 521. [Google Scholar] [CrossRef]
- Kaplan, B.; Nicke, E.; Voss, C. Design of a Highly Efficient Low-Noise Fan for Ultra-High Bypass Engines. In Proceedings of the ASME Turbo Expo 2006, Barcelona, Spain, 8–11 May 2006; ASME: New York, NY, USA, 2006; pp. 185–194. [Google Scholar] [CrossRef]
- Lewis, R.I.; Hill, J.M. The Influence of Sweep and Dihedral in Turbomachinery Blade Rows. J. Mech. Eng. Sci. 2006, 13, 266–285. [Google Scholar] [CrossRef]
- Smith, L.H.; Yeh, H. Sweep and Dihedral Effects in Axial-Flow Turbomachinery. J. Basic Eng. 1963, 85, 401. [Google Scholar] [CrossRef]
- Gümmer, V.; Goller, M.; Swoboda, M. Numerical Investigation of End Wall Boundary Layer Removal on Highly Loaded Axial Compressor Blade Rows. J. Turbomach. 2008, 130, 011015. [Google Scholar] [CrossRef]
- Place, J.M.M. Three-Dimensional flow in Core Compressors. Ph.D. Thesis, University of Cambridge, Cambridge, UK, 1997. [Google Scholar]
- Friedrichs, J.; Baumgarten, S.; Kosyna, G.; Stark, U. Effect of Stator Design on Stator Boundary Layer Flow in a Highly Loaded Single-Stage Axial-Flow Low-Speed Compressor. J. Turbomach. 2001, 123, 483. [Google Scholar] [CrossRef]
- van Zante, D.E.; Adamczyk, J.J.; Strazisar, A.J.; Okiishi, T.H. Wake Recovery Performance Benefit in a High-Speed Axial Compressor. J. Turbomach. 2002, 124, 275. [Google Scholar] [CrossRef]
- Wo, A.M.; Chung, M.H.; Chang, S.J.; Lee, S.F. Wake Vorticity Decay and Blade Response in an Axial Compressor With Varying Axial Gap. In Proceedings of the ASME 1999 International Gas Turbine and Aeroengine Congress and Exhibition, Indianapolis, IN, USA, 7–10 June 1999; American Society of Mechanical Engineers: New York, NY, USA, 1999. 10p. [Google Scholar] [CrossRef]
- Smith, L.H. Wake Dispersion in Turbomachines. J. Basic Eng. 1966, 88, 688. [Google Scholar] [CrossRef]
- Hah, C. Impact of Wake Dispersion on Axial Compressor Performance. In Proceedings of the ASME Turbo Expo: Turbine Technical Conference and Exposition—2017, Charlotte, NC, USA, 26–30 June 2017; The American Society of Mechanical Engineers: New York, NY, USA, 2017. 14p. [Google Scholar] [CrossRef]
- Przytarski, P.J.; Wheeler, A.P.S. The Effect of Rotor-Stator Gap on Repeating-Stage Compressor Loss. In Proceedings of the ASME Turbo Expo 2019: Turbomachinery Technical Conference and Exposition, Phoenix, AZ, USA, 17–21 June 2019. [Google Scholar]
- Gorrell, S.E.; Okiishi, T.H.; Copenhaver, W.W. Stator-Rotor Interactions in a Transonic Compressor— Part 1: Effect of Blade-Row Spacing on Performance. J. Turbomach. 2003, 125, 328. [Google Scholar] [CrossRef]
- Gorrell, S.E.; Okiishi, T.H.; Copenhaver, W.W. Stator-Rotor Interactions in a Transonic Compressor—Part 2: Description of a Loss-Producing Mechanism. J. Turbomach. 2003, 125, 336. [Google Scholar] [CrossRef]
- Zachcial, A.; Nürnberger, D. A Numerical Study on the Influence of Vane-Blade Spacing on a Compressor Stage at Sub- and Transonic Operating Conditions. In Proceedings of the ASME Turbo Expo 2003, Atlanta, GA, USA, 16–19 June 2003; American Society of Mechanical Engineers: New York, NY, USA, 2003; pp. 807–818. [Google Scholar] [CrossRef]
- Kielb, R.; Chiang, H.W. Recent advancements in turbomachinery forced response analyses. In Proceedings of the 30th Aerospace Sciences Meeting and Exhibit, Reno, NV, USA, 6–9 January 1992; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 1992. [Google Scholar] [CrossRef]
- Aschenbruck, J.; Seume, J.R. Experimentally Verified Study of Regeneration-Induced Forced Response in Axial Turbines. J. Turbomach. 2015, 137, 031006. [Google Scholar] [CrossRef]
- Bittner, S.L.; Keller, C.; Meinzer, C.E.; Seume, J.R. Experimental Validation of a Forced Response Analysis Using a Time-Linearized Method. In Proceedings of the 2018 AIAA/ASCE/AHS/ASC Structures Structural Dynamics and Materials Conference, Kissimmee, FL, USA, 8–12 January 2018; American Institute of Aeronautics and Astronautics: Reston, FL, USA, 2018. [Google Scholar] [CrossRef]
- Jöcker, M.; Fransson, T.H. Mode Shape Sensitivity of the High Pressure Turbine Rotor Excitation Due to Upstream Stators. In Proceedings of the Turbo Expo 2002, Volume 4: Parts A and B, Amsterdam, The Netherlands, 3–6 June 2002; pp. 1047–1056. [Google Scholar] [CrossRef]
- May, M. Sensitivity Analysis With Respect to Flutter-Free Design of Compressor Blades. In Proceedings of the ASME Turbo Expo 2010, Volume 6: Structures and Dynamics, Parts A and B, Glasgow, UK, 14–18 June 2010; pp. 1263–1275. [Google Scholar] [CrossRef]
- Chen, T.; Rogerson, J.; Patel, K. Effects of Both Axial Gap and Blade Count Ratio on the Forced Response of a Steam Turbine Stage. In Proceedings of the Volume 5B: Oil and Gas Applications; Steam Turbines, San Antonio, TX, USA, 3–7 June 2013; American Society of Mechanical Engineers: New York, NY, USA, 2013. [Google Scholar] [CrossRef]
- Korakianitis, T. On the Prediction of Unsteady Forces on Gas Turbine Blades: Part 2—Analysis of the Results. J. Turbomach. 1992, 114, 123–131. [Google Scholar] [CrossRef]
- Marn, A.; Schönleitner, F.; Mayr, M.; Selic, T.; Heitmeir, F. On the Effect of Axial Spacing Between Rotor and Stator Onto the Blade Vibrations of a Low Pressure Turbine Stage at Engine Relevant Operating Conditions. In Proceedings of the Volume 7B: Structures and Dynamics, Seoul, Korea, 13–17 June 2016; American Society of Mechanical Engineers: New York, NY, USA, 2016. [Google Scholar] [CrossRef]
- Giesecke, D.; Friedrichs, J.; Stark, U. Preliminary Aerodynamic Design of a Fan Stage for an Ultra High Bypass Ratio Engine. In Proceedings of the 23rd ISABE Conference, Manchester, UK, 3–8 September 2017. [Google Scholar]
- Schlichting, H.; Truckenbrodt, E. Aerodynamik des Flugzeuges: Erster Band: Grundlagen aus der Strömungstechnik Aerodynamik des Tragflügels (Teil I), 3rd ed.; Springer eBook Collection; Springer: Berlin/Heidelberg, Germany, 2001. [Google Scholar] [CrossRef]
- Wennerstrom, A.J. On the Treatment of Body Forces in the Radial Equilibrium Equation of Turbomachinery; USAF Aerospace Research Laboratories: Dayton, OH, USA, 1975; pp. 352–368. [Google Scholar]
- Denton, J.D.; Xu, L. The Effects of Lean and Sweep on Transonic Fan Performance. In Proceedings of the ASME Turbo Expo 2002, Amsterdam, The Netherlands, 3–6 June 2002; American Society of Mechanical Engineers: New York, NY, USA, 2002; pp. 23–32. [Google Scholar] [CrossRef]
- Giesecke, D.; Lehmler, M.; Friedrichs, J.; Blinstrub, J.; Bertsch, L.; Heinze, W. Evaluation of ultra-high bypass ratio engines for an over-wing aircraft configuration. J. Glob. Power Propuls. Soc. 2018, 2, 8SHP7K. [Google Scholar] [CrossRef]
- Celik, I.B.; Ghia, U.; Roache, P.J.; Freitas, C.J.; Coleman, H.; Raad, P.E. Procedure for Estimation and Reporting of Uncertainty Due to Discretization in CFD Applications. J. Fluids Eng. 2008, 130, 078001. [Google Scholar] [CrossRef]
- Nürnberger, D.; Eulitz, F.; Schmitt, S.; Zachcial, A. Recent Progress in the Numerical Simulation of Unsteady Viscous Multistage Turbomachinery Flow ISABE 2001–1081. In Proceedings of the 15th International Symposium on Air Breathing Engines, Bangalore, India, 3–7 September 2001. [Google Scholar]
- Schönweitz, D.; Becker, R.; Ebel, P.B.; Schnell, R.; Schroll, M. Aerodynamic Performance Characteristics of the Installed V2527 Fan at Ground Operation. In Proceedings of the 54th AIAA Aerospace Sciences Meeting 2016, San Diego, CA, USA, 4–8 January 2016; Curran Associates Inc.: Red Hook, NY, USA, 2016. [Google Scholar] [CrossRef]
- Wilcox, D.C. Reassessment of the scale-determining equation for advanced turbulence models. AIAA J. 1988, 26, 1299–1310. [Google Scholar] [CrossRef]
- Hall, K.; Thomas, J.; Ekici, K.; Voytovich, D. Frequency Domain Techniques for Complex and Nonlinear Flows in Turbomachinery. In Proceedings of the 3rd AIAA Fluid Dynamics Conference and Exhibit, Orlando, FL, USA, 23–26 June 2003. [Google Scholar] [CrossRef]
- Kersken, H.P.; Frey, C.; Voigt, C.; Ashcroft, G. Time-Linearized and Time-Accurate 3D RANS Methods for Aeroelastic Analysis in Turbomachinery. J. Turbomach. 2012, 134. [Google Scholar] [CrossRef]
- Blocher, M.; Gómez Fernández, I.E. Time-Linearized Forced Response Analysis of a Counter Rotating Fan: Part I—Theoretical Concept of a Fully Time-Linear Forced Response Analysis. In Proceedings of the Volume 7B: Structures and Dynamics, Düsseldorf, Germany, 16–20 June 2014; American Society of Mechanical Engineers: New York, NY, USA, 2014. [Google Scholar] [CrossRef]
- Schoenenborn, H.; Ashcroft, G. Comparison of Non-Linear and Linearized CFD Analysis of the Stator-Rotor Interaction of a Compressor Stage. In Proceedings of the Volume 7B: Structures and Dynamics, Düsseldorf, Germany, 16–20 June 2014; American Society of Mechanical Engineers: New York, NY, USA, 2014. [Google Scholar] [CrossRef]
- Walker, A.D.; Mariah, I.; Tsakmakidou, D.; Vadhvana, H.; Hal, C. The influence of fan root flow on the aerodynamics of a low-pressure compressor transition duct. In Proceedings of the ASME Turbo Expo 2019: Turbomachinery Technical Conference and Exposition, Volume 2B: Turbomachinery, Phoenix, AZ, USA, 17–21 June 2019. [Google Scholar]
- Gümmer, V.; Wenger, U.; Kau, H.P. Using Sweep and Dihedral to Control Three-Dimensional Flow in Transonic Stators of Axial Compressors. J. Turbomach. 2001, 123, 40–48. [Google Scholar] [CrossRef]
- Wadia, A.R.; Szucs, P.N.; Gundy-Burlet, K.L. Design and Testing of Swept and Leaned Outlet Guide Vanes to Reduce Stator-Strut-Splitter Aerodynamic Flow Interactions (98-GT-70). J. Turbomach. 1999, 121, 416–427. [Google Scholar] [CrossRef]
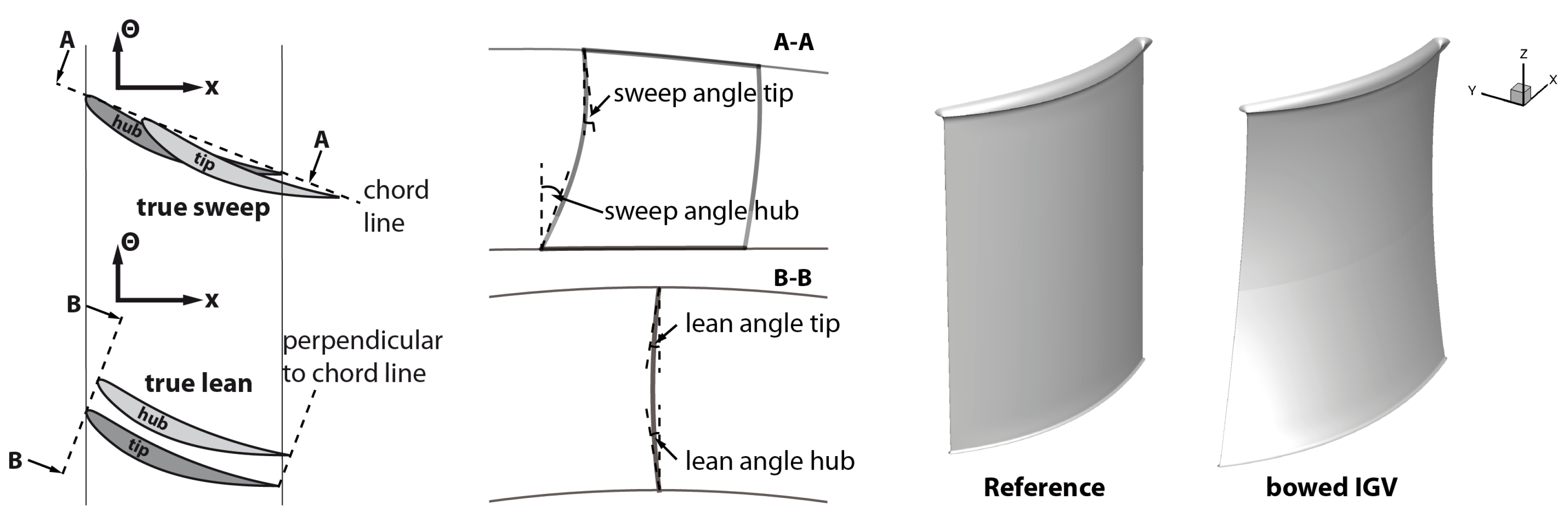




| Design | Sweep Angle [] | Lean Angle [] | ||
|---|---|---|---|---|
| Hub | Tip | Hub | Tip | |
| Reference | 0 | 0 | 0 | 0 |
| Bowed 1 | 30 | 10 | 10 | 10 |
| Bowed 2 | 30 | 10 | 15 | 15 |
| Bowed 3 | 30 | 10 | 20 | 20 |
| Bowed 4 | 40 | 15 | 15 | 15 |
| Bowed 5 | 50 | 20 | 15 | 15 |
| Mode 2 | Modal Force [N] | Aero Damping [N/m] | [m] | Relative |
|---|---|---|---|---|
| Bypass ratio 17 | 803 | 1 | ||
| Bypass ratio 20 | 1061 | |||
| Bypass ratio 25 | 1907 | |||
| Mode 3 | Modal Force [N] | Aero Damping [N/m] | [m] | Relative |
| Bypass ratio 17 | 2041 | 1 | ||
| Bypass ratio 20 | 2816 | |||
| Bypass ratio 25 | 2583 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY-NC-ND) license (https://creativecommons.org/licenses/by-nc-nd/4.0/).
Share and Cite
Eggers, T.; Kim, H.R.; Bittner, S.; Friedrichs, J.; Seume, J.R. Aerodynamic and Aeroelastic Effects of Design-Based Geometry Variations on a Low-Pressure Compressor. Int. J. Turbomach. Propuls. Power 2020, 5, 26. https://doi.org/10.3390/ijtpp5040026
Eggers T, Kim HR, Bittner S, Friedrichs J, Seume JR. Aerodynamic and Aeroelastic Effects of Design-Based Geometry Variations on a Low-Pressure Compressor. International Journal of Turbomachinery, Propulsion and Power. 2020; 5(4):26. https://doi.org/10.3390/ijtpp5040026
Chicago/Turabian StyleEggers, Torben, Hye Rim Kim, Simon Bittner, Jens Friedrichs, and Joerg R. Seume. 2020. "Aerodynamic and Aeroelastic Effects of Design-Based Geometry Variations on a Low-Pressure Compressor" International Journal of Turbomachinery, Propulsion and Power 5, no. 4: 26. https://doi.org/10.3390/ijtpp5040026
APA StyleEggers, T., Kim, H. R., Bittner, S., Friedrichs, J., & Seume, J. R. (2020). Aerodynamic and Aeroelastic Effects of Design-Based Geometry Variations on a Low-Pressure Compressor. International Journal of Turbomachinery, Propulsion and Power, 5(4), 26. https://doi.org/10.3390/ijtpp5040026





