Pulsation and Vibration Measurement on Stator Side for Turbocharger Turbine Blade Vibration Monitoring
Abstract
:1. Introduction
2. Materials and Methods
2.1. Review of Mengle’s Theory
- ω’: Observed frequency (in stationary frame)
- ω: Blade vibration frequency (in rotating frame)
- k: Traveling-wave mode number (in rotor structure)
- m: Integer (Fourier coefficient order for airborne wave between blades)
- B: Number of rotor blades
- Ω: Rotor rotational speed
- EO: Engine order,
- ND = k: Nodal diameter
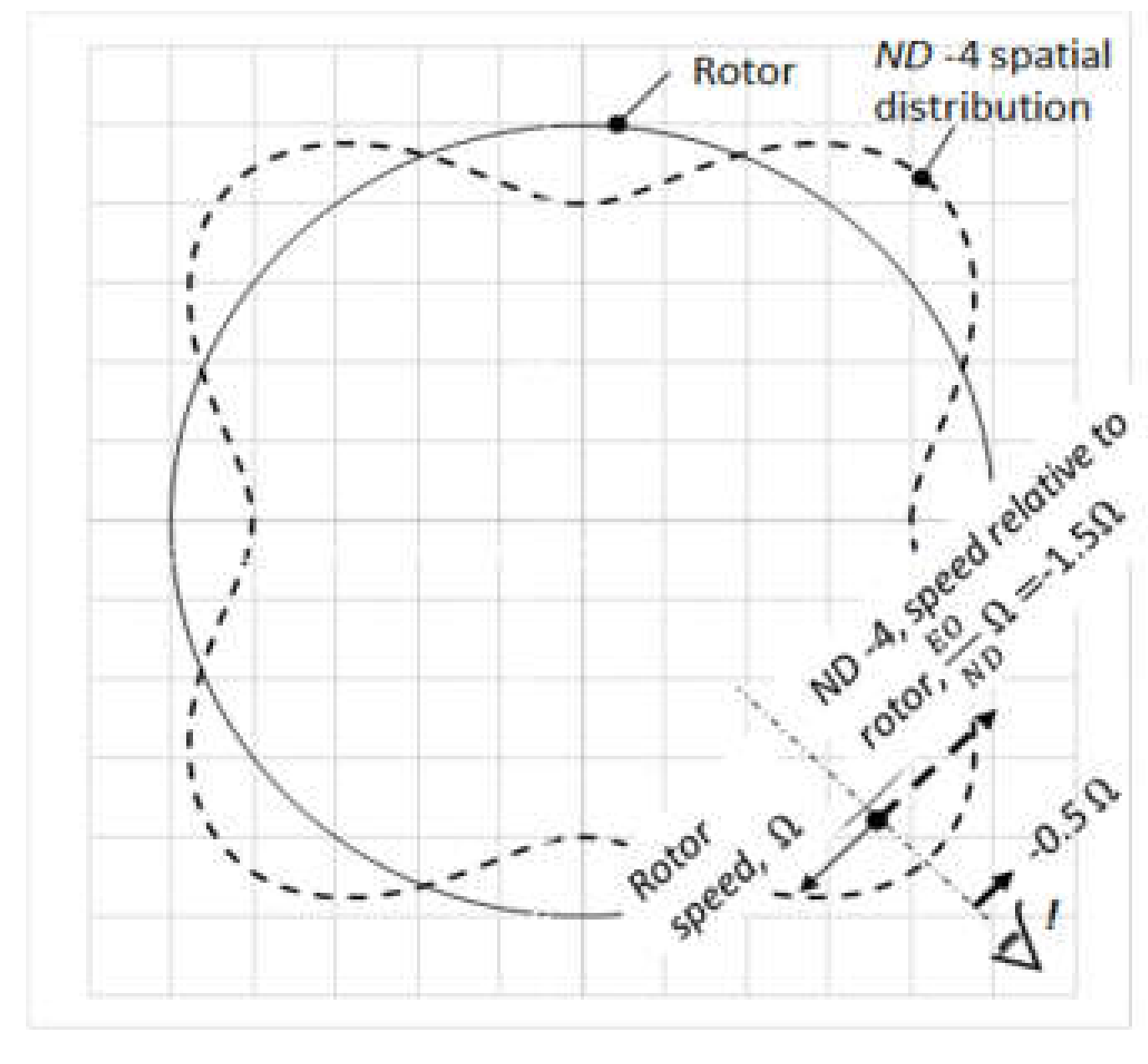
2.1.1. Rotor Structure-Borne Traveling Wave
2.1.2. Airborne Wave Shape between Blades
2.1.3. Pulsation Amplitude Relation with Blade Deflection
- ρ0: Density of medium
- c0: Speed of sound
- p0: Bulk gas static pressure
- T0: Bulk gas temperature
- τ ≤ 1: Fraction of gas flow travel time on the vibrating surface and one blade vibration cycle duration
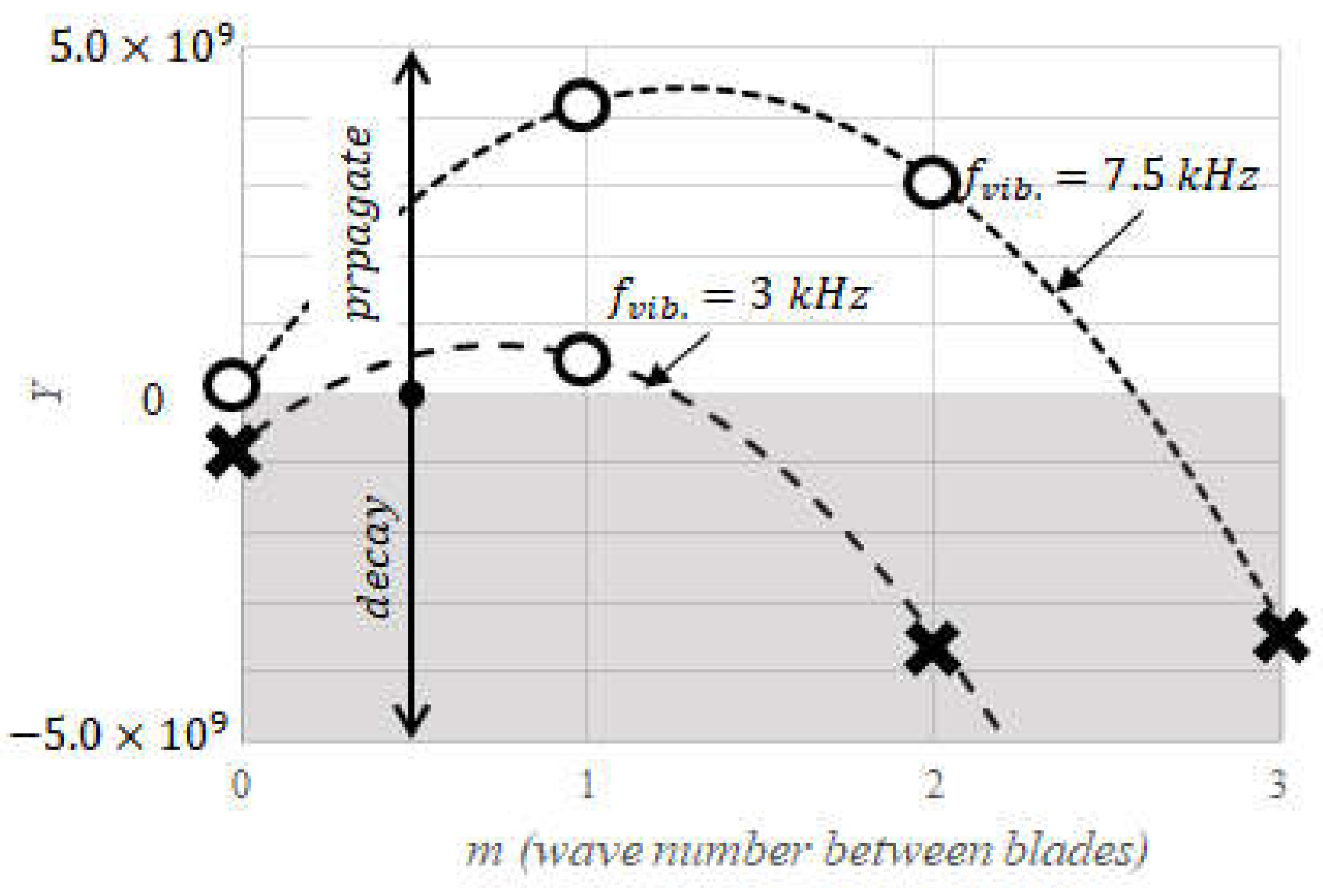
2.1.4. Pulsation Amplitude Axial Decay Behaviour
- U: Axial bulk gas flow rate
- V: Circumferential bulk gas flow rate (= blade tip velocity)
- ky: Wave number between blades in circumferential direction
- s: Spacing between two blades
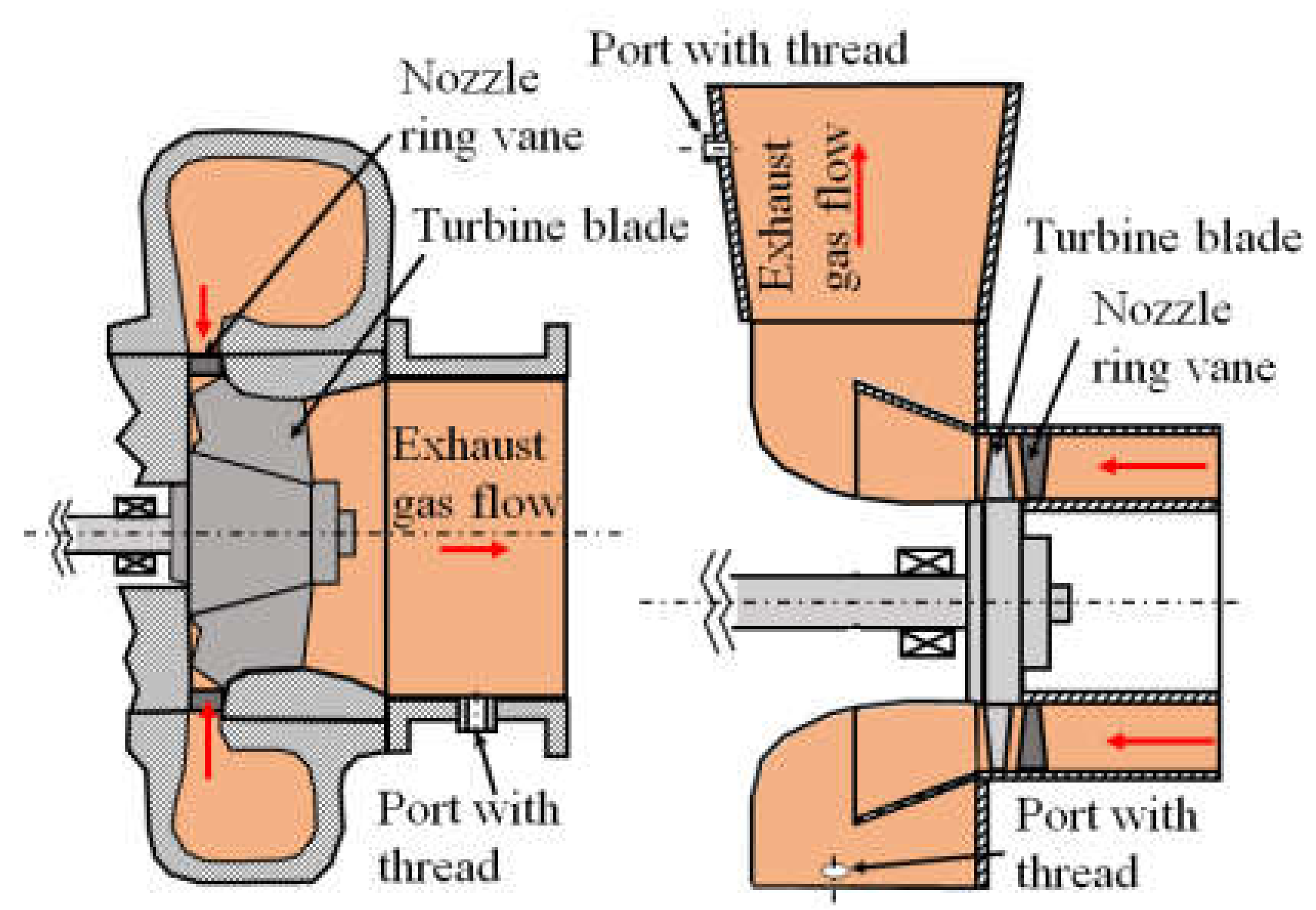
2.2. Experimental Setup
3. Results and Discussion
3.1. Fundamental Vibration Mode Detection in a Radial Turbine
- (1)
- By BTT measurement and analysis, ND = −4 was identified as the dominant vibration mode;
- (2)
- By inserting ND = −4, EO = 6, B = 11 in (2), expected engine orders along which pulsation measurement reactions to the blade vibration mode will be processed as EO = 2 (m = 0), EO = 13 (m = 1), EO = 24 (m = 2) and so on;
- (3)
- By taking the aspect of airborne acoustic wave shape between two blades into account, the modes with m = 0 and m = 1 are expected to have high pulsation amplitudes;
- (4)
- Finally, by taking the axial amplitude decay behaviour into account (refer to Figure 3, the line with 3 kHz), only the mode with m = 1 (EO = 13) can propagate undiminished downstream (the pulsation sensors were located approx. 1.5 times the chord length downstream from turbine blade trailing edge).
3.2. Fundamental Vibration Mode Detection in an Axial Turbine
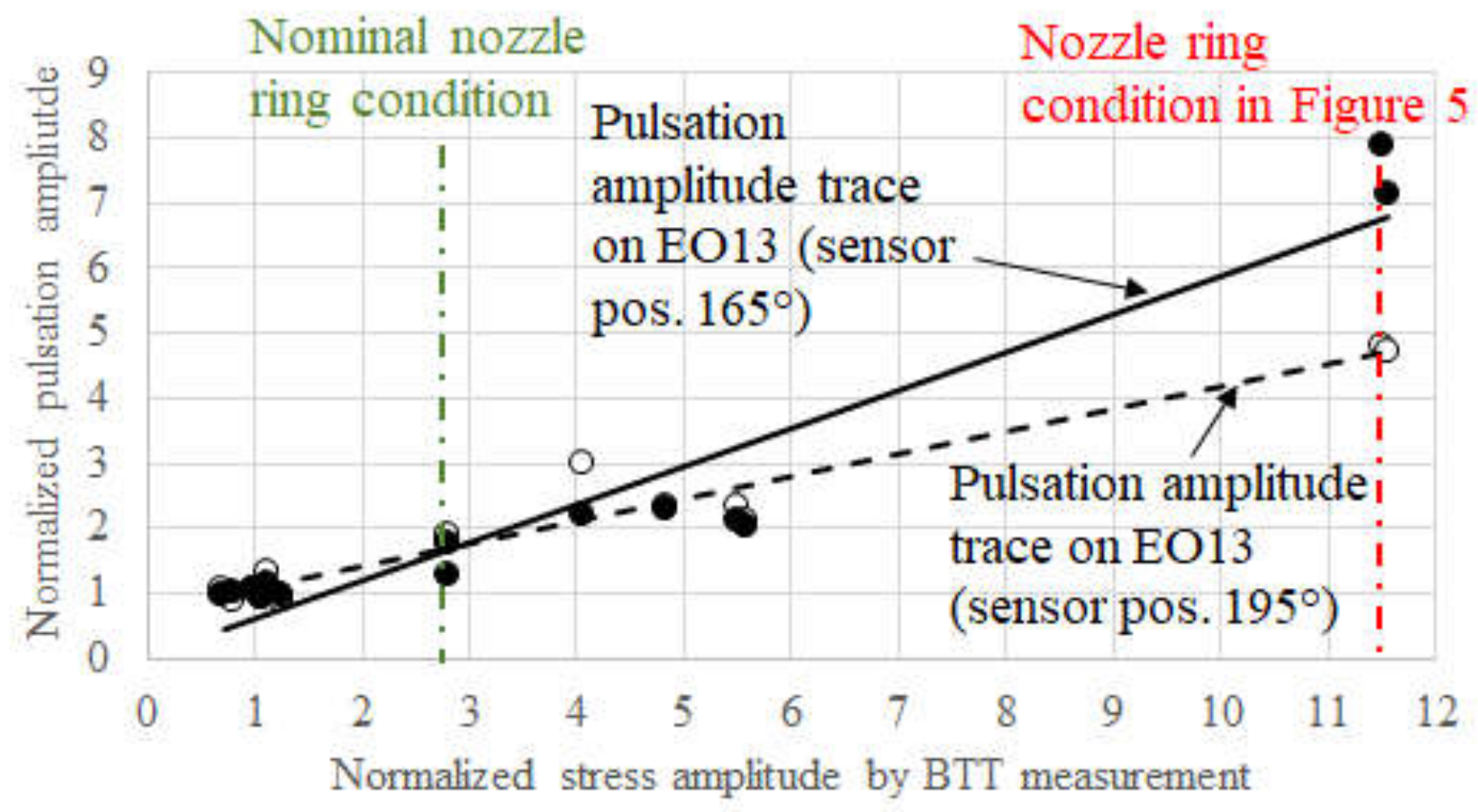
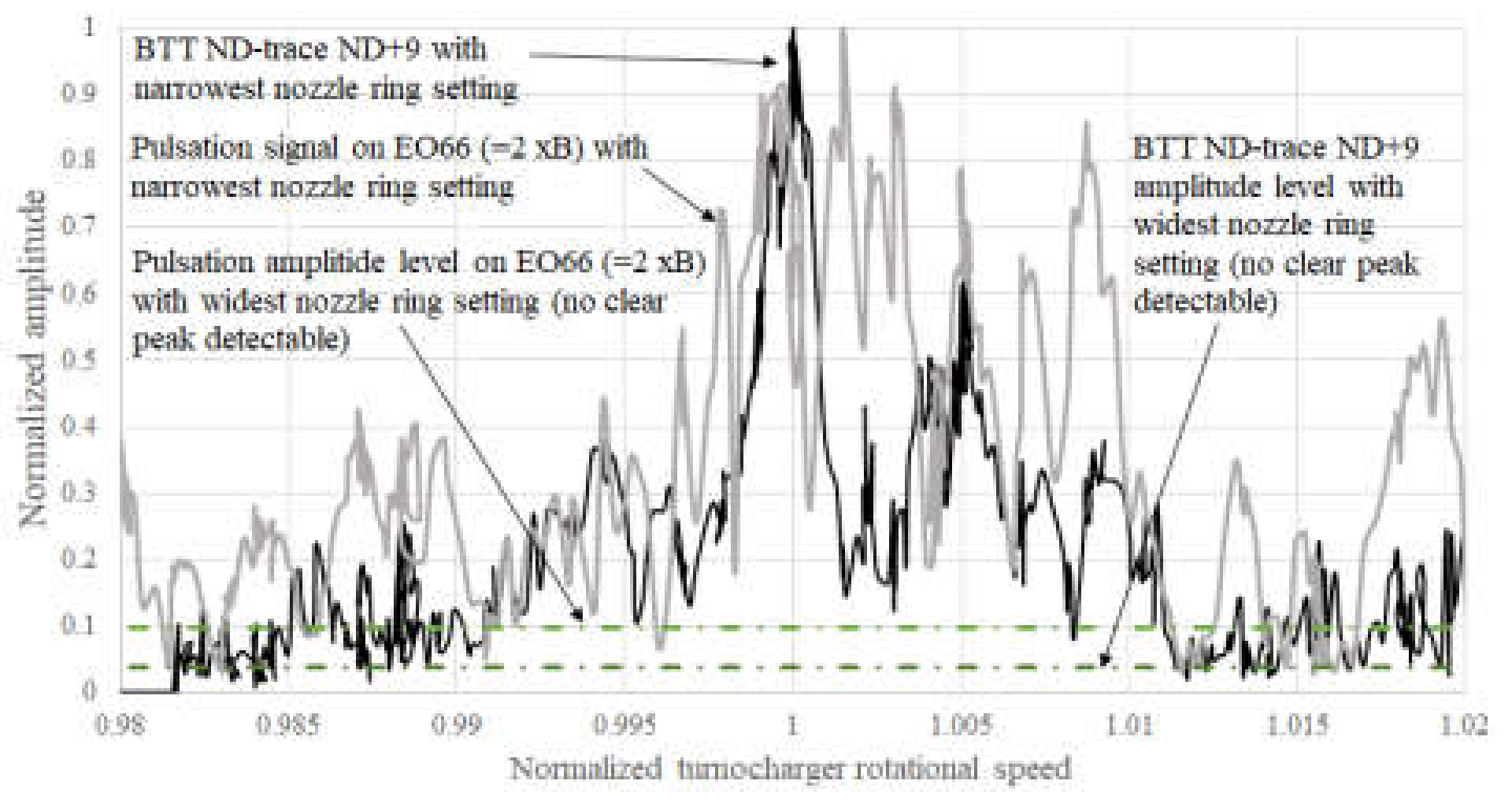
3.3. Nozzle-Ring-Induced Higher Vibration Mode Detection
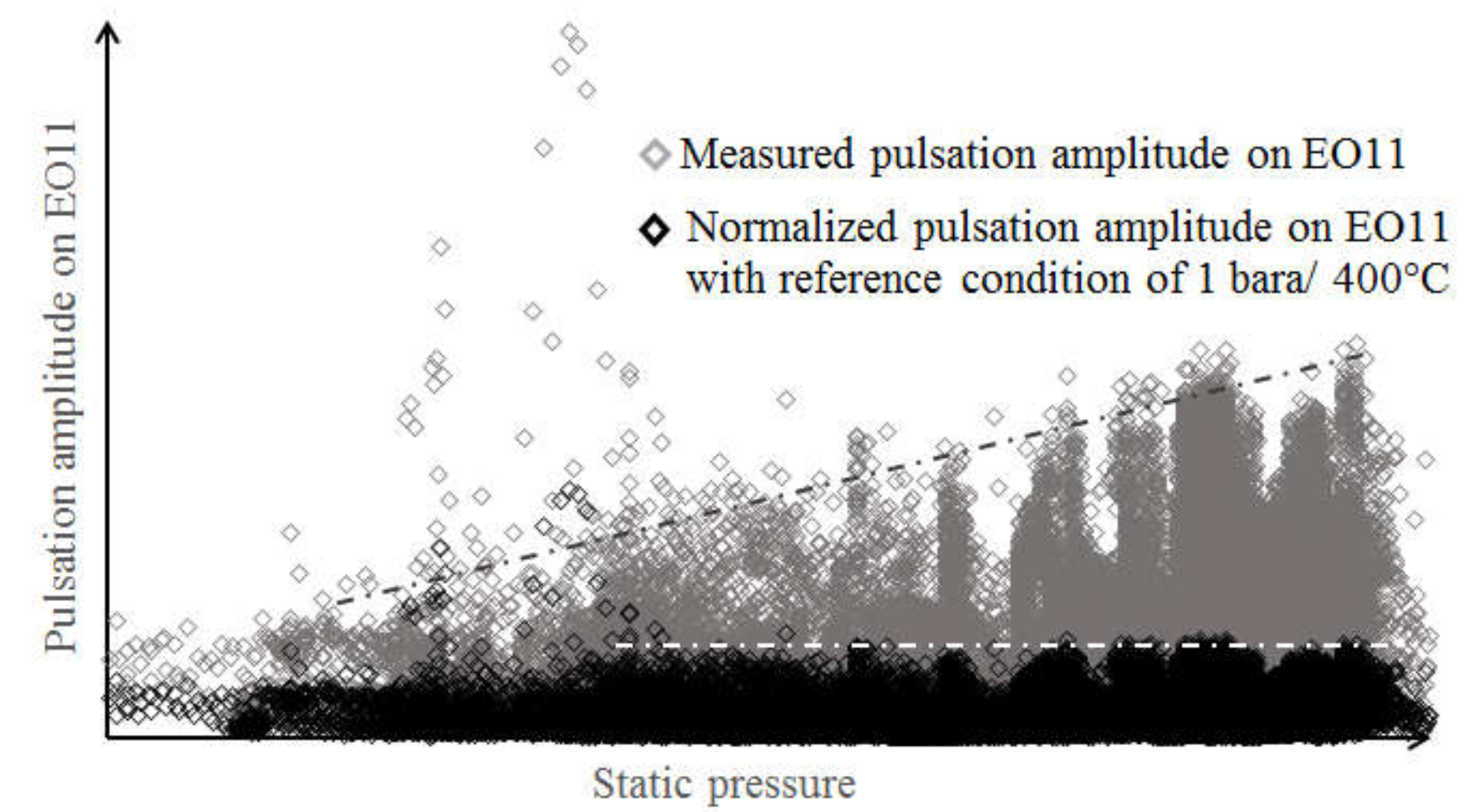
3.4. Long-Term Field Experience
4. Conclusions
Funding
Conflicts of Interest
Nomenclature
| Adefl | Blade deflection amplitude |
| B | Blade number |
| BTT | Blade Tip Timing |
| CFD | Computational Fluid Dynamics |
| EO | Engine or Excitation Order |
| FEM | Finite Element Method |
| FFT | First Fourier Transform |
| HCF | High Cycle Fatigue |
| HFO | Heavy Fuel Oil |
| LSMF | Least Squares Model Fit |
| ND | Nodal Diameter |
| T0 | Bulk gas temperature |
| U | Axial bulk gas flow rate |
| V | Circumferential bulk gas flow rate (= blade tip velocity) |
| Ω | Rotor rotational speed |
| Speed of sound | |
| k | Traveling-wave mode number (in rotor structure) |
| kx | Airborne acoustic wave number in the axial direction |
| ky | Wave number between blades in circumferential direction |
| m | Integer (Fourier coefficient order for airborne wave between blades) |
| p | Pulsation amplitude |
| s | Spacing between two blades |
| ρ0 | Density of medium |
| τ | Fraction of gas flow travel time on the vibrating surface and one blade vibration cycle duration |
| ω | Blade vibration frequency (in rotating frame) |
| ω’ | Observed frequency (in stationary frame) |
References
- Kurkov, A. Flutter Spectral Measurements Using Stationary Pressure Transducers. J. Eng. Power. 1981, 103, 461–467. [Google Scholar] [CrossRef] [Green Version]
- Kurkov, A.P. Formulation of Blade Flutter Spectral Analyses in Stationary Reference Frame; NASA TP-2296; NASA: Washington, DC, USA, March 1984. [Google Scholar]
- Mengle, V.G. Acoustic spectra and detection of vibrating rotor blades including row-to-row interference. Presented at the AIAA 13th Aeroacoustics Conference, Tallahassee, FL, USA, 22–24 October 1990. [Google Scholar]
- Gill, J.D.; Capece, V.R.; Fost, R.B. Experimental methods applied in a study of stall flutter in an axial flow fan. Shock. Vib. 2004, 11, 597–613. [Google Scholar] [CrossRef] [Green Version]
- Murray, W.L., III; Key, N.L. Detection of Rotor Forced Response Vibrations Using Stationary Pressure Transducers in a Multistage Axial Compressor. Int. J. Rotating Mach. 2015, 2015, 198534. [Google Scholar] [CrossRef]
- Agilis Measurement Systems. Available online: https://agilismeasurementsystems.com/ (accessed on 20 January 2020).


© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY-NC-ND) license (http://creativecommons.org/licenses/by-nc-nd/4.0/).
Share and Cite
Ando, T. Pulsation and Vibration Measurement on Stator Side for Turbocharger Turbine Blade Vibration Monitoring. Int. J. Turbomach. Propuls. Power 2020, 5, 11. https://doi.org/10.3390/ijtpp5020011
Ando T. Pulsation and Vibration Measurement on Stator Side for Turbocharger Turbine Blade Vibration Monitoring. International Journal of Turbomachinery, Propulsion and Power. 2020; 5(2):11. https://doi.org/10.3390/ijtpp5020011
Chicago/Turabian StyleAndo, Takashi. 2020. "Pulsation and Vibration Measurement on Stator Side for Turbocharger Turbine Blade Vibration Monitoring" International Journal of Turbomachinery, Propulsion and Power 5, no. 2: 11. https://doi.org/10.3390/ijtpp5020011
APA StyleAndo, T. (2020). Pulsation and Vibration Measurement on Stator Side for Turbocharger Turbine Blade Vibration Monitoring. International Journal of Turbomachinery, Propulsion and Power, 5(2), 11. https://doi.org/10.3390/ijtpp5020011



