Inferior Education or Killing Grandma: The Dilemma Facing the Public School Systems in the United States

Abstract

Due to COVID-19, school districts in the fall of 2020 had to decide whether to conduct all classes in person or offer some or all of these classes virtually. Many school districts would decide on a program and then change their decision based on the COVID-19 situation in their area. These changes caused education and personal issues for the students, teachers, parents, and others who worked in their school system. This paper explores how the Analytic Hierarchy Process (AHP), a well-regarded, multi-criteria decision analysis approach, can be used to analyze this situation by developing two prototype versions of the AHP and illustrating these versions of the AHP with a detailed example. The team approach for analyzing this issue is also described. Additionally, the current situation of this problem in the United States and elsewhere is discussed. Finally, Professor Bodin is making software that he has developed available (at no cost) for carrying out many of the computations for the AHP versions described in this paper.

1. Problem Setting

Due to COVID-19, a major issue in the United States is deciding on the “Back to School” program to implement in a school district. This important decision affects the education programs in the school district as well as the performance of the students who reside in these school districts. Furthermore, this decision can affect the safety of the students, faculty, and other people as well as the economy in the area in the surrounding school district. Other factors that affect this decision include the ability of the students to access the Internet and the possibility of students dropping out of school prematurely.

In the United States, the school district administration (with input and guidelines from the Federal government and the appropriate state and local governments) makes the decision as to the program to implement in a school district. As a result, (i) some school districts plan to offer all of their classes solely on the Internet, (ii) other school districts plan to offer all of their classes at schools in the district, (most likely, the school that each student in the district is scheduled to attend), and (iii) some school districts decide to implement a hybrid program where students spend part of each week at home in a virtual learning environment and the remainder of their week in classes at their school. The alternative where all students have all of their classes over the Internet is called the Virtual Alternative. The alternative where the students attend the school they were supposed to attend before COVID-19 is called the School Alternative. The alternative where the student attends some of their classes at their school and the remainder of their classes over the Internet and, most likely at home, is called the Hybrid Alternative.

It is important to note that deciding on whether a Virtual, In-School, or Hybrid environment is used in a public school is an important global issue. The analysis in this paper is restricted to the United States. To describe the current situation with regard to the education environment outside of the United States, we cite the following four articles that have been published or updated in 2021.

In [1], Stefania in a UNESCO report, wrote the following. “For months, the schools and universities were shut down, leading to a massive pivot to remote learning on which close to 500 million students missed out altogether. Now, governments are juggling with restrictive measures to contain the virus. As a result, around 800 million students are still facing major interruptions to their schooling, ranging from across-the-board school closures in 31 countries to reduced or part-time academic schedule.”

In [2], Bender, in the Wall Street Journal, wrote the following: “Children are a considerable factor in the spread of COVID-19—and more countries are shutting schools for the first time since the spring. Closures have been announced recently in the U.K., Germany, Ireland, Austria, Denmark and the Netherlands on concerns about the emergence of a more infectious variant of COVID-19 first detected in the U.K. and rising case counts despite lockdowns.” In [3], Magome discussed the education situation in South Africa. In [4], Parth describes the closing of schools in India.

The goal of this paper is to explore the use of the Analytic Hierarchy Process (AHP) in determining whether a school system should operate in a Virtual environment, an In-School environment, or a Hybrid environment. There are multiple constraints that can affect this decision. A major feature of the AHP is that it can be used to analyze problems that contain both quantitative and qualitative criteria. A solution to the AHP recommends the alternative that appears best for the school district to use or a solution that ranks a collection of alternatives from best to worst. Then, the AHP team approach is discussed and illustrated with an example. The team approach is a process for combining the analysis of more than one decision maker into a more general decision model that the organization makes. Thomas Saaty developed the AHP. Three basic and important references on Saaty’s AHP research are [5,6,7].

When using the AHP, it is important to identify the decision-makers who will carry out this analysis, since different individuals or teams can generate different results. Unless specified, the team carrying out this analysis has the following priorities: (1) to reduce the risk that individuals in the school district become infected by COVID-19 and (2) to ensure that students receive the best education possible. An interesting exercise for the reader is to rerun the analysis in this paper from the viewpoint of a person or team whose priority is to enhance the local economy. The results will, most likely, be different.

2. Description of the Alternatives and Criteria for This Study

In Section 2.1, we describe the three alternatives that we consider in this study. Then, in Section 2.2, we describe the three criteria that are used in this study and include a description of other criteria that could be used.

2.1. The Three Alternatives in This Study

The three alternatives considered in this paper are called the School Alternative (described in Section 2.1.1), the Virtual Alternative (described in Section 2.1.2), and the Hybrid Alternative (described in Section 2.1.3). We limit the discussion in this paper to these three alternatives and three criteria in order to make the discussion in this paper easier to follow and to not clutter the figures in this paper. The AHP models described in this paper can easily be expanded to more than three alternatives and/or more than three criteria.

2.1.1. The School Alternative

Under the School alternative, children will return to school just as they have done prior to the pandemic. All classes will be taught at the schools in the school district and staffed by regular teachers and support staff in the same way as they were taught before the pandemic. The advantage of this model, as articulated by its proponents, is that once parents are free from taking care of their children during the day, they will return to work, and boost the local and national economy.

Some believe that students will be well-served by having conventional instructors who use customary delivery systems. Furthermore, mental health experts have noticed that children suffer from isolation caused by home confinement and need to socialize with their peers. This benefit would occur under the School alternative.

A major downside to the School alternative is that it puts children, their families, teachers, staff, and support people at great risk of being infected by the COVID-19 virus. Government health officials and medical experts have advised citizens to avoid being in crowds of people and to wear a mask in crowded situations. By the nature of the school experience, children are in crowds.

Medical experts note that many children might be infected with the COVID-19 virus under the School alternative. Although these children initially will be asymptomatic, they will still be able to infect others and transmit the virus to their parents, families, and friends. Teachers and staff members at the schools as well as those who have underlying medical conditions can become infected and die. Persons over the age of 55 are in an age group that is vulnerable to being infected by COVID-19 and death. A school district, in deciding to implement the School alternative, is choosing the health of the economy over the health of children, their families, teachers, school staff, bus drivers, etc.

2.1.2. The Virtual Alternative

With the Virtual alternative, schools will be closed to children and teachers. Students will receive their education virtually using distance education techniques. This technique has been used by most public schools in the United States, beginning in March 2020 when the pandemic first struck the country.

Under the Virtual alternative, in-person contact between children and teachers is eliminated, and students receive their education online using a computer or tablet. Many schools use the Zoom software to hold their sessions. The advantage of the Virtual alternative is that it protects the health of all concerned—children, families, teachers, staff, and others with whom the children come in to contact.

There are challenges that arise with the Virtual alternative. When it was first used in March 2020, educators had to develop teaching techniques using distance learning with little or no time to plan or develop well thought out distance learning techniques. Ideally, with time to develop the curriculum over the 2020 summer, classroom teachers have been able to develop methods that better meet the needs of the children. Budget constraints have affected this development.

A major disadvantage to the Virtual alternative is that students will not meet their classmates in person, but they will see them electronically. As such, implementing this model increases the isolation of children who need interaction with their peers. Money is needed by school systems to develop curriculum materials, train teachers, and purchase computers for staff and tablets for students to ensure that they all have the tools to use this system that depends on technology. This situation is discussed further in Section 3 of this paper when we discuss urban school district issues.

Another limit to this distance learning approach is that some homes in the United States, especially in rural areas, are not connected to the Internet. Additionally, home Internet service is not free, and this cost is a burden to many parents who have lost their jobs due to the pandemic as well as other citizens in the school district.

The bottom line is that the Virtual alternative is the best alternative to protect the health of all concerned. However, it is imperative with young children that a parent or guardian is in the home to supervise the children. This can be a severe burden to parents who need to work away from home as well as persons working successfully at home under the environment that has been created.

2.1.3. The Hybrid Alternative

The Hybrid alternative can be thought of as a compromise between the other two alternatives. There are many possible versions of the Hybrid alternative. One example of the Hybrid alternative is the following. The students at the school are split into two groups. One group attends classes at the school on Monday and Thursday and operates like the Virtual alternative the other 3 days in the week. The other group attends classes at the school on Tuesday and Friday and operates like the Virtual alternative the other 3 days in the week. Under this structure, all students operate like the Virtual alternative on Wednesday.

Experts in Childhood Education question whether young children will be able to maintain social distancing and wear masks in programs such as the programs for 4-year-olds and kindergarten through grade three programs. Educators are concerned that these young students need group learning experiences and other best practices of active learning since these students are still operating like the Virtual alternative several days a week. Parents will still have to provide supervision on the days that children are not in school. From a family perspective, tradeoffs between taking care of family and working one or more jobs are a major issue since young students are in a Virtual experience several days a week.

Teachers of middle school and high school students are concerned about the developmental thinking of adolescents to feel “bullet proof” and compel them not to wear masks or be concerned about keeping a social distance. In terms of their stage of development, adolescents are often not able to realize that they can potentially infect others.

In summary, under the Hybrid alternative, the children will benefit from having normal face-to-face interaction with teachers and classmates. Going to school will reduce isolation and resulting fears that many children will have developed while being in isolation. This model still puts the health of children, parents, family members, teachers, staff, and other service providers at a high risk due to COVID-19, although this risk is not as high as the risk with the School alternative.

2.2. The Three Criteria Used in This Study

The three criteria used in the analysis in this paper are the following.

• Safety: How safe is the school district’s system with respect to being infected by COVID-19? School districts wish to avoid students, faculty members, or other persons becoming infected and spreading the COVID-19 virus to others.
• Education: Are the students able to learn at an acceptable level to at least maintain their grade level?
• Economy: Can the economy grow when the school district removes impediments to business activities in (and around) the school district?

These criteria are simple to define, easy for the decision-makers in a school district to analyze and comprehensive. The examples in the remainder of this paper use these three criteria in order to demonstrate the AHP models that can be developed. These three criteria are not the only criteria that can be used in this analysis.

Three additional criteria that can be used in this analysis are the following:

• Budget (or Cost) is a criterion that will play a significant role in analyzing a school district’s situation. Using pairwise comparisons, an analyst can compare the budgets of two alternatives as well as comparing the magnitude of a budget versus the other criteria that are part of the analysis.
• Number of dropouts (or percentage of students who drop out) is a criterion that concerns a school district. Dropouts can be a more severe situation in some school districts (for example, urban school districts) and less severe in other school districts.
• Hardware and software availability is a concern a school district faces, especially if the district is going to use the Virtual alternative. The issue with this criterion is how many students cannot attend the classes they want (or need) because they cannot obtain the appropriate hardware, software, and/or Internet access. As a result of these hardware and software shortages, these students are not able to attend their classes or do the required work for their classes at no fault of their own.

3. Urban School Districts

Many urban school districts struggle to make the decision as to the alternative to use for the school year or to change the alternative that is being used during the school year. These districts need additional funding to meet their needs. Particularly vulnerable in these urban school districts are the lower income families and the families who have lost their jobs. In 2020, President Trump wanted to open the schools in the United States as a boost to the economy, even though the residents in these school districts had a greater chance of being infected by COVID-19. Many school districts rejected Trump’s wishes and adopted the Virtual alternative.

However, there are issues with the Virtual alternative. If the urban school district wanted to go for the Virtual alternative, then some of the students in the school district and the school district itself may not have the resources to purchase this equipment. Additionally, there may not be enough hotspots around these urban school districts to allow students to participate in classes even if they had the appropriate Wi-Fi hardware and software. Obviously, students cannot attend Virtual classes if they are not able to access the Internet.

Reed and Bowie (2020) [8], in an article in the Baltimore Sun, reported that there are an estimated 11 million to 14 million homes across the United States that do not have internet access and that many of these homes are in urban areas. As a result of this situation, students drop out of school—a price that society will pay for in the future.

In many urban school districts, administrators are in an impossible position. These districts face a situation where there is basically no alternative that is best for the physical health of families and their children and of the school personnel of the school district, and that would allow all students to attend and participate in the Virtual classes held by the school district.

The students and their families in urban districts are vulnerable and need help. If the economy improves, the residents of these urban school districts get vaccinated for COVID-19, and additional funds for these school districts become available, then, hopefully, some of these negative issues will disappear or become less of a factor in the operations of these urban school districts.

As noted earlier, the AHP, originally developed by Professor Saaty, has been successfully used in analyzing and solving multiple-criteria decision analysis problems with both quantitative and qualitative criteria. References [1,2,3,4,5,6,7,8] are discussed earlier in this paper. References [9,10,11,12,13,14,15,16,17] are references on the AHP. References [18,19] are recent newspaper articles that discuss issues related to the topics in this paper.

Section 4, Section 5 and Section 6 contain an analysis of different aspects of the AHP. In Section 4, we describe the steps involved in formulating and solving the Direct Comparison Model (DCM) of the AHP and illustrate this approach with an example. In Section 5, we describe the steps involved in formulating and solving the Ratings Model (RM) of the AHP and illustrate this approach with an example. In Section 4 and Section 5, we assume there is a single decision-maker or the members of the team have agreed upon the value of the pairwise comparison values before carrying out the analysis. In Section 6, we give an overview of the AHP team approach. This approach is useful if the team members have different pairwise comparison values for entries in the DCM and RM.

4. The Direct Comparison Model (DCM) of the Analytic Hierarchy Process (AHP)

Suppose a DCM has three alternatives, labeled Alternative A, Alternative B, and Alternative C. The weights assigned to the three alternatives found by solving the DCM are the following: (i) the weight of Alternative A = 0.41, (ii) the weight of Alternative B = 0.25, and (iii) the weight of Alternative C = 0.34. Alternative A is the winner, since Alternative A earned the largest weight in this solution. In a solution to the DCM, the sum of the weights of the alternatives always equals 1. We call the solution to a DCM the “Splitting of the Pie” solution.

Assume that the RM has the same three alternatives (Alternative A, Alternative B, and Alternative C). A solution to RM is the following: the weight of Alternative A = 0.62, the weight of Alternative B = 0.45, and the weight of Alternative C = 0.65. In the case of the RM, Alternative C is the winning alternative because it had the largest weight. In a solution to the RM, the sum of the weights of the alternatives almost never equals 1. In this case, we try to find the alternative with the largest weight, where this weight is always less than or equal to 1. If an alternative earns a weight of 1, then this alternative is called perfect alternative. The RM is called the “Search for Perfection”.

We recommend school districts use both the DCM and the RM to analyze its situation and compare the results. Interesting insights into a school district’s decision process can be gained by comparing the results from the DCM and the RM. In the remainder of Section 4, we present an example of the DCM. Then, in Section 5, we work out an example of the RM.

The three criteria in this example are labeled Safety, Education, and Economic and the three alternatives for this problem are labeled Virtual, Hybrid, and School. These criteria and alternatives were discussed in Section 2 of this paper.

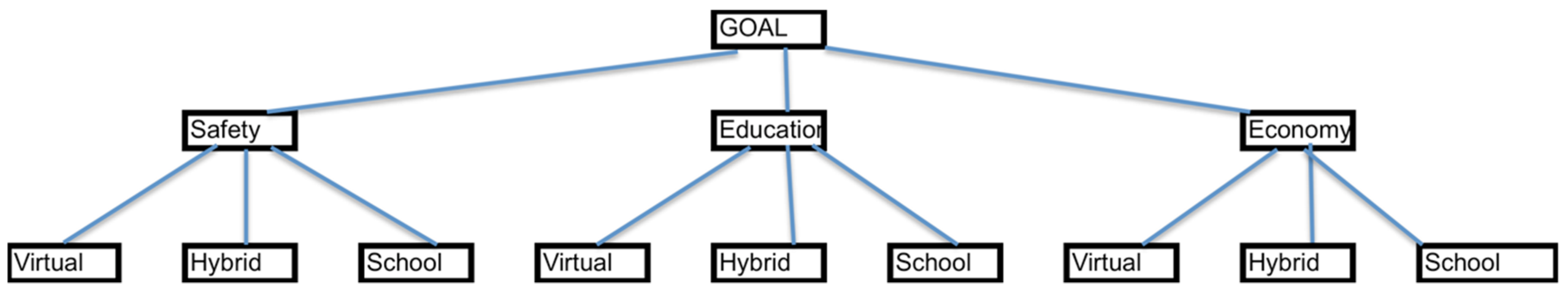
The first step in the DCM is to create the AHP tree. This AHP tree is a schematic of the associations between the goal and the different criteria and the association between each criterion and the different alternatives. The AHP tree for this problem is presented in Figure 1. As can be seen in Figure 1, the AHP tree is an acyclic network with nodes labeled as follows:

• The Level 1 node is called the Goal Node (or Root Node) of the AHP tree.
• The Level 2 nodes for this AHP tree are the Safety, Education, and Economic criteria.
• The Level 3 nodes for this AHP tree are the Virtual, Hybrid, and School alternatives connected to each criterion listed in Level 2 of the AHP tree. In this example, the nodes in Level 3 are repeated for each criterion in the construction of the AHP tree.

Professor Saaty recommends that if any level of the AHP tree contains more than seven criteria or alternatives, then this level of the tree should be broken up into two or more levels; see [5,6,7] for a further explanation and see [10] for an example of an AHP tree with criteria on two levels.

4.1. Verbal to Numeric Scale

The Verbal to Numeric Scale, developed by Professor Saaty, is key in using the AHP. We have used Saaty’s Verbal to Numeric Scale in every AHP problem that we have studied and recommend using Saaty’s Verbal to Numeric Scale for determining the value of a pairwise comparison in any AHP application.The numeric scale for any pairwise comparison can be any real number between 1 and 9 or between 0.1 and 0.9.

Saaty’s Verbal and Numeric Scale is presented in

Table 1. Saaty’s Verbal Scale and Numeric Scales.

Numeric Scale	Verbal Scale
1	Equal Importance
3	Moderate Importance
5	Strong Importance
7	Very Strong Importance
9	Extremely Strong Importance

. The analyst can determine the value of the Pairwise Comparison to use by answering the following two questions. The Verbal and Numeric scale can be used if the analyst is determining a pairwise comparison value between two criteria or two alternatives.

Question 1: Are criterion A and criterion B of equal importance to the analyst? If the answer to this question is YES, then the values of the pairwise comparison of A to B and B to A are equal to 1 and Question 2 is skipped. Otherwise, the analyst must answer Question 2 since the analyst believes either criterion A is more important than criterion B or criterion B is more important than criterion A.

Question 2: If the analyst believes criterion A is more important than criterion B, then the analyst must set the pairwise comparison value of A to B equal to a number between 1 and 9 and the pairwise comparison value of B to A is set equal to 1 divided by the pairwise comparison value of A to B. For example, if the analyst believes that criterion A is moderately preferred to strongly preferred to criterion B, the pairwise comparison value of A to B is equal to 4 and the pairwise comparison value of B to A is ¼. On the other hand, if the analyst believes that criterion B is moderately more important than criterion A, then the pairwise comparison value of B to A is equal to 3 and the pairwise comparison value of A to B is equal to 1/3.

4.2. Properties of the Pairwise Comparative Matrix (PCM)

The Pairwise Comparison Matrix is defined as follows:

• The PCM has the same number of rows and columns.
• The PCM cannot be made up of a mixture of criteria and alternatives.
• The identification of the rows in the PCM must be in the same order as the identification of the columns in the PCM. Otherwise, entering the values of the different pairwise comparisons in the PCM can become messy.
• If j = k, then A(j, j) = 1 as long as the rows and columns are numbered in the same order. In other words, the value of the pairwise comparisons for each diagonal cell of the PCM is 1.
• The Reciprocal Rule, A(k,j) = 1/A(j,k), holds for every entry in the PCM.
• If the Reciprocal Rule holds for each entry in the PCM, then the PCM is called a Positive Reciprocal Matrix since all entries in the PCM are positive.
• Further details of a Reciprocal Matrix with Positive Entries can be found in [9].

4.3. Finding the Value of the Weights for a PCM Using the Black Box

The Black Box is a procedure that computes the weights for any PCM that has been created under the rules described in Section 4.2. There are several sites on the Internet that have software (some at no charge) for determining the AHP weights for a PCM. Bodin wrote a procedure in Excel for computing the weights of a PCM where the PCM has between 3 to 7 rows and columns. A procedure that is virtually the same as the procedure Bodin implemented in the Black Box is presented in [11].

If the reader sends an email to Bodin at [email protected], then Bodin will email the reader the Excel file and documentation (one page) for the Black Box code (a simple Excel file). The Black Box is not a commercial code but can handle any 3 × 3 to 7 × 7 PCM. Bodin makes no guarantees and no liability protection with respect to this software. Furthermore, Bodin wishes every user of this software, good luck, good health, and great solutions.

An example of this software is shown in

Table 2. Example of a 3 × 3 PCM.

	A	B	C
A	1	3	4
B	0.333	1	2
C	0.25	0.5	1

. The yellow cells in Table 2 (and in the remainder of this paper) represent the cells whose values were manually entered into the Black Box. The remaining values in the PCM were computed by the Black Box since the PCM is a Reciprocal Matrix. The Black Box determined that the weights to the PCM in Table 2 are the following: A = 0.623, B = 0.24, and C = 0.137. The sum of the weights equals 1.

4.4. Finding the AHP Weights for the DCM

We now show how to find the AHP weights for the example that is introduced in Figure 1. In this example, there are three criteria and three alternatives. Therefore, four different PCMs are created. One of these PCMs computes the weights for the three criteria with respect to the Goal Node. The other three PCMs determine the weights for the three alternatives with respect to one of the criteria. Bodin and Frieman decided on the pairwise comparison values for each of these four PCMs.

4.4.1. Determining the Weights for the Three Criteria Adjacent to the Goal Node

The PCM for the three criteria, Safety, Education, and Economy, adjacent to the Goal Node, is presented in

Table 3. Pairwise Comparison Matrix for the Three Criteria.

	Safety	Education	Economy
Safety	1	1	6
Education	1	1	6
Economy	0.1667	0.1667	1

. We assumed that both the Safety and Education criteria are equally important and that the school district strongly preferred these criteria to the criterion of improving the economy in the area of the school district. The Black Box determined that the weights for the three criteria are the following: Safety = 0.4615, Education = 0.4615, and Economy = 0.077. The sum of these weights = 1.

4.4.2. Weights for the Three Alternatives under the Safety Criterion

Table 4. Pairwise Comparison and Weights for the 3 Alternatives under the Safety Criterion.

	Virtual	Hybrid	School
Virtual	1	5	7
Hybrid	0.2	1	5
School	0.1433	0.2	1

displays three alternatives adjacent to the Safety criterion. The Virtual alternative was strongly preferred to the Hybrid alternative (pairwise comparison = 5) and very strongly preferred to the School alternative (pairwise comparison = 7). Additionally, the Hybrid alternative was strongly preferred to the School alternative because about half of the students are on remote learning under the Hybrid alternative at any time, while no student is on remote learning under the School alternative at any time. The Black Box determined that the weights for the three alternatives under the Safety criterion are the following: Virtual = 0.696, Hybrid = 0.232, and School = 0.072.

4.4.3. Weights for the Alternatives under the Education Criterion

Table 5. Pairwise Comparison and Weights for the 3 Alternatives under the Education Criterion.

	Virtual	Hybrid	School
Virtual	1	0.3333	0.2
Hybrid	3	1	0.4
School	5	2.5	1

displays the pairwise comparisons and weights for the three alternatives adjacent to the Education criterion. In this case, the School alternative was strongly preferred compared to the Virtual alternative with a pairwise comparison value = 5 and somewhat moderately preferred to the Hybrid alternative with a pairwise comparison value equal to 2.5. The Hybrid alternative was moderately preferred to the Virtual alternative with a pairwise comparison value = 3. The Black Box found that the weights for the alternatives under the Education criterion are the following: Virtual = 0.108, Hybrid = 0.281, and School = 0.611.

As a note, in the solution shown in Table 5 for the Education criterion, the weight for the School alternative was greater than the weight for the Hybrid alternative. This result indicates that the Hybrid alternative may not be considered positively when compared to the other alternatives in the final analysis.

4.4.4. Weights for the Alternatives under the Economy Criterion

The pairwise comparisons and weights for the three alternatives adjacent to the Economy criterion, are displayed in

Table 6. Pairwise Comparison and Weights for the 3 Alternatives under the Economy Criterion.

	Virtual	Hybrid	School
Virtual	1	0.4	0.25
Hybrid	2.5	1	0.5
School	4	2	1

. The School alternative is slightly preferred when compared with the Hybrid alternative and moderately preferred when compared with the Virtual alternative. Additionally, the Hybrid alternative is slightly more preferred than the Virtual alternative. The Black Box found that the weights for the alternatives under the Economy criterion as determined by the Black Box are the following: Virtual = 0.134, Hybrid = 0.290 and School = 0.576.

4.5. Computing the Overall Score for the Alternatives Using the DCM

The overall scores for the three alternatives are computed as follows.

• The Virtual alternative score = 0.461 × 0.696 + 0.461 × 0.108 + 0.0769 × 0.134 = 0.3809.
• The Hybrid alternative score = 0.461 × 0.232 + 0.461 × 0.281 + 0.0769 × 0.290 = 0.2588.
• The School alternative score = 0.461 × 0.072 + 0.461 × 0.611 + 0.0769 × 0.576 = 0.3602.

4.6. Matrix Representation of Determining the Weights of the Alternatives

A matrix representation of the determination of the weights of the three alternatives is the following. Let R be a 1 × 3 row matrix (row vector) representing the three criteria whose order is Safety, Education, and Economy (

Table 7. 3 Component Vector Weights for the 3 Criteria.

	Safety	Education	Economy
R =	0.461	0.461	0.0769

). Let S be a 3 × 3 matrix where the rows of the matrix S are the three criteria whose order is Safety, Education, and Economy and the columns of the matrix S are the three alternatives whose order is Virtual, Hybrid, and School (

Table 8. 3 × 3 matrix – Black Box Solution for the 3 criteria and 3 alternative.

	Virtual	Hybrid	School
S =	0.696	0.108	0.134
	0.232	0.281	0.29
	0.072	0.611	0.576

). Then, R × S is a 1 × 3 row matrix (or row vector) representing the three alternatives whose order is Virtual, Hybrid, and School (

Table 9. Component Vector Weights for the 3 Alternatives.

	Virtual	Hybrid	School
R × S =	0.3809	0.2588	0.3602

). The vector R, matrix S, and vector R × S are displayed next.

4.7. Conclusions and Implications

In this example, the Virtual alternative receives the biggest “Piece of the Pie” with a value of 0.3809. The School alternative, is a close second with a “Piece of the Pie” value of 0.3602. The Hybrid alternative did not fare well with a “Piece of the Pie” value of 0.2588.

This solution implies that if the school district wants to protect the health of the students, faculty, and others, then the district will close all of its schools and go for a Virtual implementation. However, three criticisms of the Virtual alternative are the following: (i) the the school district is not able to maintain the educational levels of the students, (ii) more students drop out of school due to COVID-19, and (iii) students are not able to obtain the necessary hardware, software, and Internet access. We are aware of teachers who will take a leave of absence from a school district or resign from the school district if a school district decides to prematurely implement the School alternative, as they fear they will suffer a COVID-19 infection.

4.8. Notes on Pairwise Comparisons and Using the AHP

We expect that some of the readers of this paper may not agree with the above analysis. We remind the reader that this example illustrates the DCM methodology. However, disagreements like the above will occur in actual situations since different individuals and teams will create different AHP trees and pairwise comparisons for the same problem. However, this type of analysis, if properly managed, can create a healthy situation that leads to an intelligent discussion and a wise, consensus decision. We encourage these discussions and believe these discussions are a positive benefit if the AHP is properly used. This issue is further discussed in Section 6.

5. The Ratings Model (The Search for Perfection)

In Section 4, we described the Direct Comparison Model (DCM) for determining the weights of the three alternatives. In Section 5, we describe the Ratings Model (RM), a procedure that computes a Rating Score each alternative. The Ratings Score for an alternative ranges from 0 to 1 and the best Ratings Score that an alternative can achieve is 1.

A major benefit of the Ratings Model is that there is no restriction on the number of alternatives that can be evaluated as each alternative is evaluated individually. As with the DCM, the Ratings Model can analyze situations with both qualitative and quantitative constraints. For example, in [11], a version of the Ratings Model is used to analyze an accounting issue with thousands of alternatives. In [12], the Ratings Model is used to assist a major league baseball team in deciding the 15 out of the 42 players who are to be saved by the team preceding the 1997 Major League Baseball expansion draft.

We believe that the Ratings Model is a great approach for solving multi-criteria decision analysis problems, especially when these problems have both quantitative and qualitative criteria and alternatives. We call the Ratings Model the “Search for Perfection” because we are computing the Ratings Score for each alternative and observing how close this Ratings Score is to the perfect Ratings Score of 1.

5.1. Key Aspects of the Ratings Model

• We assume in this paper that the same criteria can be used in analyzing a DCM and a Ratings Model. More general AHPs can have subcriteria and subalternatives.
• In the RM in this paper, there is a set of intensities ssociated with each criterion
• A criterion will have between 3 and 7 intensities. The set of intensities must cover the full range of possible outcomes of the criterion. Furthermore, there is a different collection of intensities for each criterion.
• The alternatives are not analyzed in the Ratings Model until late in the procedure.
• The intensities are processed one criterion at a time. A PCM is set up for the intensities associated with a criterion. The Black Box is then used to compute the AHP Scores for these intensities. This process is repeated for the intensities associated with each criterion.
• The Normalized Score for a criterion is equal to the value of the AHP score for this intensity divided by the value of the maximum AHP score for all intensities associated with the criterion. In this way, at least one Normalized Score is equal to 1 for each criterion and the Normalized Scores of all intensities are between 0 and 1.

We now show how to compute the AHP score and the Normalized Score for the Safety criterion, the Education criterion, and the Economy criterion.

5.2. Pairwise Comparisons and Normalized Scores for the Safety Intensities

In this example, the four intensities for the Safety criterion are labeled Sa-Excellent, Sa-Good, Sa-Fair, and Sa-Poor where Sa- stands for the Safety criterion. The pairwise comparison matrix PCM for these four intensities is given in

Table 10. PCM for the Safety Criterion.

	Sa-Excellent	Sa-Good	Sa-Fair	Sa-Poor
Sa-Excellent	1	4	7	9
Sa-Good	0.25	1	4	7
Sa-Fair	0.143	0.25	1	3
Sa-Poor	0.111	0.143	0.333	1

. Given these pairwise comparisons, the Black Box computes the AHP scores for these intensities. Then, knowing the AHP scores for the Safety criterion, the Normalized Scores for the Safety criterion are computed. The normalized scores for the Safety Intensities can be found in

Table 11. AHP Score and Normalized Score for the Safety Criterion.

	AHP Score	Normalized Score
Sa-Excellent	0.611	0.611/0.611 = 1
Sa-Good	0.2	0.3273 = 0.2/0.611
Sa-Fair	0.143	0.234 = 0.143/0.611
Sa-Poor	0.046	0.0753 = 0.046/0.611

.

5.3. Pairwise Comparisons and Normalized Score for the Education Criterion’s Intensities

In

Table 12. Pairwise Comparisons for the Education Criterion.

	Ed-Excellent	Ed-Good	Ed-Fair	Ed-Poor
Ed-Excellent	1	2.5	5	7
Ed-Good	0.4	1	2.5	5
Ed-Fair	0.2	0.4	1	2.5
Ed-Poor	0.143	0.2	0.4	1

, the pairwise comparison matrix for the intensities for the Education criterion is displayed. The four intensities for the Education criterion are labeled Ed-Excellent, Ed-Good, Ed-Fair, and Ed-Poor where Ed- stands for Education. Given these pairwise comparisons, the Black Box computes the AHP scores for the intensities. Using the AHP scores, the Normalized Scores for these intensities are computed. These Normalized Scores are displayed in

Table 13. AHP Score and Normalized Score for the Education Criterion.

	AHP Score	Normalized Score
Ed-Excellent	0.549	1
Ed-Good	0.269	0.49
Ed-Fair	0.122	0.22
Ed-Poor	0.06	0.109

.

5.4. Pairwise Comparisons and Normalized for the Economy Criterion’s Intensities

In

Table 14. Pairwise Comparisons for the Economy Criterion.

	Ec-Excellent	Ec-Good	Ec-Fair	Ec-Poor
Ec-Excellent	1	2	3	4
Ec-Good	0.5	1	2	3
Ec-Fair	0.333	0.5	1	2
Ec-Poor	0.25	0.333	0.5	1

we set up the pairwise comparison matrix for the intensities for the Economy criterion. In this example, the four intensities for the Economy criterion are labeled Ec-Excellent, Ec-Good, Ec-Fair, and Ec-Poor. Given these pairwise comparisons, the Black Box then computes the AHP scores for these intensities. Then, using the AHP scores, the Normalized Scores for these alternatives are computed. The Normalized Scores are displayed in

Table 15. AHP Score and Normalized Score for the Economy criterion.

	AHP Score	Normalized Score
Ec-Excellent	0.466	1
Ec-Good	0.277	0.594
Ec-Fair	0.161	0.345
Ec-Poor	0.096	0.206

.

Table 16. Summary Normalized Scores for the 12 intensities.

Safety	Norm. Score	Education	Norm. Score	Economy	Norm. Score
Sa-Excellent	1	Ed-Excellent	1	Ec-Excellent	1
Sa-Good	0.3273	Ed-Good	0.49	Ec-Good	0.594
Sa-Fair	0.234	Ed-Fair	0.22	Ec-Fair	0.345
Sa-Poor	0.0753	Ed-Poor	0.109	Ec-Poor	0.206

summarizes the Normalized Scores for the 12 intensities created in Table 10, Table 11, Table 12, Table 13, Table 14 and Table 15. For example, the Normalized Score for the Good intensity for the Safety criterion, Sa-Good, is equal to 0.3273.

We next merge the information in Table 16 with the analysis of the criteria data given in Section 4.4.2 to obtain the Ratings Score for the three alternatives. For example, the Virtual alternative for the Safety criterion (Sa-excellent) has a Ratings Score of 1 since it is rated Excellent. The Normalized Scores for the intensities are given in

Table 17. Winning Intensity for the 3 alternatives.

	Safety	Education	Economy
Virtual	1 (Sa-excellent)	0.22 (Ed-fair)	0.206 (Ec-poor)
Hybrid	0.234 (Sa-fair)	0.49 (Ed-good)	0.345 (Ec-fair)
School	0.075 (Sa-poor)	0.49 (Ed-good)	1 (Ec-Excellent)

.

5.5. Computing the Ratings Score for Each Alternative

Given the above information, the Ratings Scores for the three alternatives are computed by using the results in Table 17 along with the weights for the three criteria determined in Section 4.4.2.

• Ratings Score for the Virtual Alternative = 0.461 × 1 + 0.461 × 0.22 + 0.0769 × 0.206 = 0.578.
• Ratings Score for the Hybrid Alternative = 0.461 × 0.234 + 0.461 × 0.49 + 0.0769 × 0.345 = 0.360.
• Ratings Score for the School Alternative = 0.461 × 0.75 + 0.461 × 0.49 + 0.0769 × 1 = 0.337.

5.6. Analysis of the Results

The final results of this analysis are as follows. The Virtual alternative’s Ratings Score is 0.578, the Hybrid alternative’s Ratings Score is 0.36, and the School alternative’s Ratings Score is 0.331. Based on these results, the Rating Score of the Virtual alternative is superior to the Rating Scores of the Hybrid and School alternatives even though the Virtual alternative’s Ratings Score is not high. Combining the results in Section 4 and Section 5, the Virtual Alternative appears to be the superior alternative.

It appears difficult to come up with an alternative that is superior with respect to both the Safety and the Economy criteria. This conclusion makes sense since the Safety and the Economy criterion are the two extreme criteria in this analysis.

6. The Team Approach

6.1. Introduction to the AHP Team Approach

In Section 4 and Section 5, we assumed that one person determined the values of the pairwise comparisons and carried out the procedures outlined in Section 4 and Section 5. In Section 6, we assume that a team called Team2 is assigned to carry out this analysis. The members of Team2 have decided upon the AHP tree and have submitted their values of the pairwise comparisons in advance of the meeting but these pairwise comparisons were not revealed to the Team2 members in advance of the meeting.

The submitted pairwise comparison values are revealed to the members at the beginning of the meeting. It is possible (and, likely) that some pairwise comparisons have more than one value assigned to them. As an example, if Team2 has five members, then it is possible that a pairwise comparison has five values assigned to it—one for each member of Team2.

The Geometric Average converts any pairwise comparison that has more than one value assigned to it into a pairwise comparison with one value assigned to it. More formally, the Geometric Average is defined as follows. If Team2 has T members who enter values for a particular pairwise comparison, then the Geometric Average for this pairwise comparison is equal to the T’th root of the product of the values submitted for this pairwise comparison. Each member of Team2 may contribute a value for this pairwise comparison. This process of using the Geometric Average to convert a collection of pairwise comparisons into a single pairwise comparison is carried out for each non-diagonal entry in the PCM.

An important property of the Geometric Average is that if the Geometric Average is computed for all pairwise comparisons in a Pairwise Comparison Matrix (PCM), then this PCM is a Reciprocal Matrix. Metrics such as the Arithmetic Average do not generate a Reciprocal Matrix.

6.2. Examples of the Geometric Average

Two examples of computing the Geometric Average are as follows:

• The Geometric Average (GA) of 4 and 9 is equal to square root of (4 × 9) = square root of 36 = 6. On the other hand, the Arithmetic Average of 4 and 9 = 13/2 = 6.5.
• The Geometric Average (GA) of 8, 27, and 64 = cube root of (8 × 27 × 64) = cube root of 13,824 = 24. On the other hand, the Arithmetic Average of 8, 27, and 64 is equal to (8 + 27 + 64)/3 = 33.

6.3. Computing the Geometric Average in Excel

The Geometric Average (GA) can be computed in Excel as follows. Assume we want to compute the GA of the following P numbers: whose values are Q(1), Q(2),…, Q(P). Then, the format for computing the Geometric Average in Excel for these P numbers is as follows

GA = [Q1 × Q2 ×…× Q(P)] ^ (1/P),

where (1/P) is the P’th root of [Q1 × Q2 ×…× Q(P)].

6.4. Why Is the Geometric Average Used in the AHP?

Let A and B be two criteria or two alternatives. If GA(A,B) is the geometric average of pairwise comparison (A,B) and GA(B,A) is the geometric average of pairwise comparison (B,A), then (A,B) and (B,A) are reciprocal pairwise comparisons and the following relationship must hold:

Value of GA(B,A) = 1/[Value of GA(A,B)].

In other words, if you generate GA(A,B) using the Geometric Average, then you know that 1/Value of GA(A,B) = GA(B,A). Thus, once all pairwise comparisons are converted to a single value, a procedure that uses the methodology in Section 4 and Section 5 can be applied to come up with a solution to the original problem.

6.5. Interactive Features of the Geometric Average and the AHP

When we introduced the GA at the beginning of Section 6, we stated that Team2 generates the pairwise comparisons in advance and then, at the meeting, these individual pairwise comparison values are scrutinized by the member of Team2. Any pairwise comparison value that appears to be out of line should be discussed and changed if necessary. This discussion can lead to changes in the pairwise comparison values as well as the reworking of parts of the analysis.

Several years ago, Bodin attended a couple of educational sessions at a professional meeting where the pairwise comparisons were entered real time using a handheld device. A pairwise comparison was announced and each participant would enter his pairwise comparison value for this pairwise comparison. Participants challenged the pairwise comparison value of a participant and a discussion on the values of this pairwise comparison would begin. A vote was held. If the team agreed to change the value of the pairwise comparison in question or change the value of any other pairwise comparison, then the Weights would be recomputed. This process continued until all issues of this type were handled. This interactive feature of the AHP is valuable since it can lead to an excellent discussion, analysis, and decision making by an organization.

When we teach the AHP at the University of Maryland, we use a simplified approach to the approach described above to determine the values of the AHP pairwise comparisons. We state a problem and present the AHP tree. The students in the class are the Team2 members. The teacher plays the role of the facilitator at the meeting.

A pairwise comparison is introduced and the students vote on the value to use for this pairwise comparison. We have had some interesting discussions in carrying out this exercise. Since we do not have much time to complete this exercise, we use the Mode of the pairwise comparisons values rather than the geographic average. We then take a short break. On this break, we use the Black Box to determine the weights. Over the years, the results have been remarkably close to the actual value of the candidate weights. We believe this exercise enforces the fact that this process actually gives reasonable results.

6.6. An Example of the TEAM Approach

We now present a not very complicated example of the team approach. Assume that Team2 is made up of three analysts named Mo, Shemp, and Curly. The pairwise comparison matrices for Mo, Shemp, and Curly are given in Table 18, Table 19 and Table 20. We, further, assume that the pairwise comparison matrices for Mo, Shemp, and Curly for the alternatives are the same as the pairwise comparison matrices for the alternatives in Section 4 of this paper—a real surprise to the organization as Mo, Shemp, and Curly rarely agree on anything.

6.6.1. Mo’s Pairwise Comparison Matrix for the Criteria

Pairwise Comparison Matrix for Mo is given in Table 18.

Table 18. Pairwise Comparison Matrix for Mo.

	Safety	Education	Economy
Safety	1	1	6
Education	1	1	6
Economy	0.1667	0.1667	1
Weights	0.461	0.461	0.078

Table 18. Pairwise Comparison Matrix for Mo.

	Safety	Education	Economy
Safety	1	1	6
Education	1	1	6
Economy	0.1667	0.1667	1
Weights	0.461	0.461	0.078

6.6.2. Shemp’s Pairwise Comparison Matrix

Pairwise Comparison Matrix for Shemp is given in Table 19.

Table 19. Pairwise Comparison Matrix for Shemp.

	Safety	Education	Economy
Safety	1	3	8
Education	0.3333	1	6
Economy	0.125	0.1667	1
Weights	0.6464	0.2895	0.0641

Table 19. Pairwise Comparison Matrix for Shemp.

	Safety	Education	Economy
Safety	1	3	8
Education	0.3333	1	6
Economy	0.125	0.1667	1
Weights	0.6464	0.2895	0.0641

6.6.3. Curly’s Pairwise Comparison Matrix

Pairwise Comparison Matrix for Curly is given in Table 20.

Table 20. Pairwise Comparison Matrix for Curly.

	Safety	Education	Economy
Safety	1	0.3571	0.1333
Education	2.8	1	0.2222
Economy	7.5	4.5	1
Weights	0.0826	0.1942	0.7232

Table 20. Pairwise Comparison Matrix for Curly.

	Safety	Education	Economy
Safety	1	0.3571	0.1333
Education	2.8	1	0.2222
Economy	7.5	4.5	1
Weights	0.0826	0.1942	0.7232

6.6.4. Geometric Average of the Pairwise Comparisons in Table 18, Table 19 and Table 20

Table 21. Geometric Average for Non-diagonal Entries in Table 18, Table 19 and Table 20.

Cell ID	Mo (A1)	Shemp (A2)	Curly (A3)	Geometric Average
(A1,A2)	1	3	0.3571	1.0233
(A2,A1)	1	0.3333	2.8	0.9769
(A1,A3)	6	8	0.1333	1.8566
(A3,A1)	0.1667	0.125	7.5	0.5386
(A2,A3)	6	6	0.2222	0.5
(A3,A2)	0.1667	0.1667	4.5	2

show the computation of the Geometric Averages for the six non-diagonal entries (that are called Cell ID) in Table 18, Table 19 and Table 20 in order to verify that the Geometric Averages in Table 21 satisfy the reciprocal requirement.

We, next verify that the reciprocal cells in Table 21 satisfy the reciprocal relationship desired by the Geometric Average. Further, we note that the values of the 6 non-diagonal elements in Table 21 were computed through the definition of the Geometric Average and did not assume that the reciprocal relation held a-priori to running this analysis:

(A1,A2) times (A2,A1) = 1.0233 × 0.9769 = 1 (up to four decimal places)

(A1,A3) times (A3,A1) = 1.8566 × 0.5386 = 1 (up to four decimal places)

(A2,A3) times (A3,A2) = 0.5 × 2 = 1.

6.6.5. The Team2 Pairwise Comparison Matrix and Weights

Based on the above computation, the Team2 Pairwise Comparison Matrix is presented in

Table 22. Weights for the Criteria in the Team2 Example.

	Safety	Education	Economy
Safety	1	1.02326	1.85662
Education	0.97693	1	0.5
Economy	0.53861	2	1
Weights	0.4016	0.2620	0.3364

. Then, the Black Box found the Weights to assign to each criterion for Team2. These weights are given in the last line in Table 22.

6.7. Summary

In summary, we encourage the use of the AHP team approach. It is an excellent approach for making a consensus decision and an organization’s key decision-makers are active participants in this analysis. Furthermore, pairwise comparisons that are outliers can be identified and modified. Finally, issues that come up in the overall process can be discussed and dealt with.

7. Conclusions

A school district must be concerned with the possibility that students can ‘fall through the cracks’ under an implementation program and, in some cases, drop out of school. Some students need a more structured environment than what can be offered in a Virtual implementation. How to handle this situation should be considered in any decision that the school district makes.

One goal of a school district should be to ensure that every student has access to a computer (or its equivalent) and Wi-Fi if it decides to implement either a Virtual or Hybrid plan. A Virtual plan may be difficult to operate in a rural district since the families in this district may not have access to Wi-Fi. A plan that opens up the schools from the beginning of the school year may be successful in a rural school district if the school district has the classroom space (and other spaces) available to handle its student body.

COVID-19 is seriously affecting school districts in the United States. A new peak of COVID-19 with increased intensity began near the end of the 2020 and continues as of the writing of this paper. There has been a major increase in the number of deaths each day although, at the end of ‘February 2021, the number appears to be deceasing. Three vaccines have been approved for vaccinating the public in the United States. Due to logistical issues and weak political leadership until the beginning of 2021, the implementation of this vaccination program in the United States is slow. At the end of January 2021, over 3000 Americans are dying daily because of COVID-19.

Yet, there is some positive news. It appears that the number of vaccines being manufactured is increasing and the distribution of vaccines is increasing as well (although at a slower pace than is desired).

School districts are moving from Virtual to Hybrid and some school districts are considering a move to In-School from Virtual or Hybrid. An issue with these decisions is that the teachers and personnel may not have been vaccinated before these moves are made. Alternatives such as forcing certain grades and special education students to become full-time in-school students and the remaining students to continue as Virtual or Hybrid students have been recently implemented.

President Joe Biden regards the vaccination of as many persons as possible by July 4, 2021 to be a primary issue in his administration. In the middle of March 2021, over 2 million persons a day are vaccinated and this inoculation program is increasing in size and intensity. Further, new vaccines are emerging and the supply available for vaccinating the public is increasing. There are still logistical and supply chain issues to be solved but the process is functioning smoothly at this time. The effectiveness of these vaccinations to new strains of COVID-19 and the lifetime of these vaccinations still have to be determined.

On a personal level, we believe that any plan that allows thousands of United States residents to die because of the pandemic is unacceptable. We further believe that any plan that reopens schools before teachers and staff have been inoculated for COVID-19 may be dangerous. We recommend the decision to open schools be made with caution and with analysis.