Elevated Temperature Effects on FRP–Concrete Bond Behavior: A Comprehensive Review and Machine Learning-Based Bond Strength Prediction
Abstract
1. Introduction
2. Previous Work
2.1. The Behavior of FRP Bonded with Epoxy Adhesives
2.2. The Behavior of FRP Bonded with Cementitious Adhesives
3. Proposed Bond–Slip Models
4. Machine Learning Models
5. Conclusions and Gaps in the Literature
- There are limited models for the FRP–concrete bond behavior under high temperatures. The development and validation of an extensive bond–slip model under high temperatures incorporating temperature-dependent properties of FRPs and adhesives are needed.
- The work performed in the literature is insufficient regarding the long-term performance and life of adhesives at elevated temperatures.
- In most of the previous works, researchers focus on specific scenarios or materials; the vast majority of the experimental campaigns are conducted for CFRP-strengthened beams. This has created an evident lack of general experimental research works covering a wide range of other FRP materials. It would be useful to have detailed studies concerning different types of FRP and adhesives so that their particular behavior under high-temperature conditions could be understood and appropriate materials could be selected for a whole range of applications.
- Some studies consider thermal effects on mechanical properties but, generally, no deep discussions concerning micro-mechanisms of bond failure are performed. Researching the micro-mechanisms of bond failure under thermal stress with state-of-the-art imaging and analytical techniques is desirable.
- Regarding real-world application scenarios, most studies are conducted under a highly controlled laboratory setup, accounting just for mere simulation in real applications. Real case application scenarios should be established and validated by case studies, followed by model adjustment as per the results.
6. Recommendations
- Identify advanced tools for analysis that will be used to investigate microstructural change and failure mechanisms of FRP–concrete bonds under elevated temperatures, such as digital image correlation, scanning electron microscopy, and X-ray diffraction.
- Field studies, inclusive of real-life case studies of the application of FRPs in concrete structures in elevated temperature conditions, could become very instrumental with valuable insights and important empirical data for consideration in the refinement of theoretical models and results of experiments.
- Newer developments in materials, such as nanocomposites or advanced epoxy formulations, have huge potential for improving the durability of FRP composites at higher temperatures. In contrast, additional research work is still needed to develop models for the prediction of service life with accuracy by considering multiple factors of bond degradation in real-world conditions.
- Standard test methods and guidelines should be developed for adoption in the evaluation of the bond performance of FRP–concrete systems at elevated temperatures. This will help provide consistency and reliability in research findings on the derived values for practical applications.
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| Specimen Code * | Ef (GPa) | bf (mm) | tf (mm) | Tg (°C) | Test Temperature (°C) | L (mm) | τmax (MPa) |
|---|---|---|---|---|---|---|---|
| B1-20 | 165 | 50 | 1.2 | 62 | 20 | 300 | 1.757 |
| B1-40 | 165 | 50 | 1.2 | 62 | 40 | 300 | 1.7416 |
| B1-50 | 165 | 50 | 1.2 | 62 | 50 | 300 | 2.3115 |
| B1-70 | 165 | 50 | 1.2 | 62 | 70 | 300 | 2.1695 |
| B1-80 | 165 | 50 | 1.2 | 62 | 80 | 300 | 1.7753 |
| B2-20 | 165 | 50 | 1.2 | 62 | 20 | 300 | 2.0382 |
| B2-40 | 165 | 50 | 1.2 | 62 | 40 | 300 | 2.6586 |
| B2-50 | 165 | 50 | 1.2 | 62 | 50 | 300 | 2.0212 |
| B2-70 | 165 | 50 | 1.2 | 62 | 70 | 300 | 1.4361 |
| B2-90 | 165 | 50 | 1.2 | 62 | 90 | 300 | 1.1315 |
| O-26 | 230 | 50 | 0.111 | 38 | 26 | 200 | 1.2606 |
| O-30 | 230 | 50 | 0.111 | 38 | 30 | 200 | 1.1301 |
| O-40 | 230 | 50 | 0.111 | 38 | 40 | 200 | 0.7165 |
| O-50 | 230 | 50 | 0.111 | 38 | 50 | 200 | 0.4874 |
| T-26 | 230 | 50 | 0.111 | 38 | 26 | 200 | 1.283 |
| T-40 | 230 | 50 | 0.111 | 38 | 40 | 200 | 1.194 |
| T-50 | 230 | 50 | 0.111 | 38 | 50 | 200 | 0.8323 |
| T-60 | 230 | 50 | 0.111 | 38 | 60 | 200 | 0.6427 |
| T-SL20 | 210 | 60 | 1.4 | 85 | 20 | 150 | 1.5213 |
| T-SL70 | 210 | 60 | 1.4 | 85 | 70 | 150 | 1.9423 |
| T-SL80 | 210 | 60 | 1.4 | 85 | 80 | 150 | 2.0705 |
| T-SL85 | 210 | 60 | 1.4 | 85 | 85 | 150 | 2.2996 |
| CS-4 | 390 | 100 | 0.167 | 44 | 4 | 120 | 1.0261 |
| CS-40 | 390 | 100 | 0.167 | 44 | 40 | 120 | 1.4335 |
| CS-60 | 390 | 100 | 0.167 | 44 | 60 | 120 | 0.6594 |
| CS-80 | 390 | 100 | 0.167 | 44 | 80 | 120 | 0.3251 |
| CS-100 | 390 | 100 | 0.167 | 44 | 100 | 120 | 0.345 |
| CS-120 | 390 | 100 | 0.167 | 44 | 120 | 120 | 0.3593 |
| CS-140 | 390 | 100 | 0.167 | 44 | 140 | 120 | 0.3478 |
| CS-160 | 390 | 100 | 0.167 | 44 | 160 | 120 | 0.342 |
| CS-180 | 390 | 100 | 0.167 | 44 | 180 | 120 | 0.3506 |
| ZS-27 | 245 | 100 | 0.167 | 44 | 27 | 110 | 0.8627 |
| ZS-40 | 245 | 100 | 0.167 | 44 | 40 | 110 | 0.8121 |
| ZS-50 | 245 | 100 | 0.167 | 44 | 50 | 110 | 0.6178 |
| ZS-65 | 245 | 100 | 0.167 | 44 | 65 | 110 | 0.2769 |
| ZS-85 | 245 | 100 | 0.167 | 44 | 85 | 110 | 0.2061 |
| ZS-110 | 245 | 100 | 0.167 | 44 | 110 | 110 | 0.2076 |
References
- Ferracuti, B.; Savoia, M.; Mazzotti, C. Interface law for FRP-concrete delamination. Compos. Struct. 2007, 80, 523–531. [Google Scholar] [CrossRef]
- Shier, G. Flexural Behaviour of Fibre Reinforced Polymer Strengthened Reinforced Concrete Beams at Elevated Temperatures; Library and Archives Canada = Bibliothèque et Archives Canada: Ottawa, ON, Canada, 2014. [Google Scholar]
- Correia, J.R.; Gomes, M.M.; Pires, J.M.; Branco, F.A. Mechanical behaviour of pultruded glass fibre reinforced polymer composites at elevated temperature: Experiments and model assessment. Compos. Struct. 2013, 98, 303–313. [Google Scholar] [CrossRef]
- Bazli, M.; Abolfazli, M. Mechanical properties of fibre reinforced polymers under elevated temperatures: An overview. Polymers 2020, 12, 2066. [Google Scholar] [CrossRef]
- Sharifianjazi, F.; Zeydi, P.; Bazli, M.; Esmaeilkhanian, A.; Rahmani, R.; Bazli, L.; Khaksar, S. Fibre-Reinforced Polymer Reinforced Concrete Members under Elevated Temperatures: A Review on Structural Performance. Polymers 2022, 14, 472. [Google Scholar] [CrossRef]
- Haddad, R.H.; Al-Rousan, R.; Almasry, A. Bond-slip behavior between carbon fiber reinforced polymer sheets and heat-damaged concrete. Compos. B Eng. 2013, 45, 1049–1060. [Google Scholar] [CrossRef]
- Hawileh, R.A.; Assad, M.A.; Abdalla, J.A.; Naser, M.Z. Finite element modeling of reinforced concrete beams externally bonded with PET-FRP laminates. Comput. Concr. 2024, 33, 163–173. [Google Scholar] [CrossRef]
- Hawileh, R.; Abdalla, J.; Thomas, B.S.; Bahurudeen, P.H.D.B.; Jittin, V.; Syed, K.I.; Kabeer, A.; Hadi, M.; Abu-Obeidah, A. Influence of Synthesized Nanomaterials on the Strength and Durability of Cementitious Composites; Manuscript Draft-Powered by Editorial Manager® and ProduXion Manager® from Aries Systems Corporation; Aries Systems Corporation: North Andover, MA, USA, 2023. [Google Scholar]
- Hawileh, R.A.; Abu-Obeidah, A.; Abdalla, J.A.; Al-Tamimi, A. Temperature effect on the mechanical properties of carbon, glass and carbon-glass FRP laminates. Constr. Build. Mater. 2015, 75, 342–348. [Google Scholar] [CrossRef]
- Hawileh, R.A.; Rasheed, H.A.; Abdalla, J.A.; Al-Tamimi, A.K. Behavior of reinforced concrete beams strengthened with externally bonded hybrid fiber reinforced polymer systems. Mater. Des. 2014, 53, 972–982. [Google Scholar] [CrossRef]
- Hawileh, R.A.; Abdalla, J.A.; Hasan, S.S.; Ziyada, M.B.; Abu-Obeidah, A. Models for predicting elastic modulus and tensile strength of carbon, basalt and hybrid carbon-basalt FRP laminates at elevated temperatures. Constr. Build. Mater. 2016, 114, 364–373. [Google Scholar] [CrossRef]
- Firmo, J.P.; Correia, J.R.; Pitta, D.; Tiago, C.; Arruda, M.R.T. Experimental characterization of the bond between externally bonded reinforcement (EBR) CFRP strips and concrete at elevated temperatures. Cem. Concr. Compos. 2015, 60, 44–54. [Google Scholar] [CrossRef]
- Firmo, J.P.; Roquette, M.G.; Correia, J.R.; Azevedo, A.S. Influence of elevated temperatures on epoxy adhesive used in CFRP strengthening systems for civil engineering applications. Int. J. Adhes. Adhes. 2019, 93, 102333. [Google Scholar] [CrossRef]
- Zhang, Y.; Vassilopoulos, A.P.; Keller, T. Effects of low and high temperatures on tensile behavior of adhesively-bonded GFRP joints. Compos. Struct. 2010, 92, 1631–1639. [Google Scholar] [CrossRef]
- Di Tommaso, A.; Neubauer, U.; Pantuso, A.; Rostasy, F.S. Behavior of Adhesively Bonded Concrete-CFRP Joints at Low and High Temperatures. Mech. Compos. Mater. 2001, 37, 327–338. [Google Scholar] [CrossRef]
- Biscaia, H.C. The influence of temperature variations on adhesively bonded structures: A non-linear theoretical perspective. Int. J. Non-Linear Mech. 2019, 113, 67–85. [Google Scholar] [CrossRef]
- Jadooe, A.; Al-Mahaidi, R.; Abdouka, K. Bond Behavior between NSM CFRP Strips and Concrete Exposed to Elevated Temperature Using Cement-Based and Epoxy Adhesives. J. Compos. Constr. 2017, 21, 04017033. [Google Scholar] [CrossRef]
- Arruda, M.R.T.; Firmo, J.P.; Correia, J.R.; Tiago, C. Numerical modelling of the bond between concrete and CFRP laminates at elevated temperatures. Eng. Struct. 2016, 110, 233–243. [Google Scholar] [CrossRef]
- Liu, Y.; Chen, W.; Liu, C.; Li, N. Bond Performance of CFRP/Steel Double Strap Joint at Elevated Temperatures. Sustainability 2022, 14, 15537. [Google Scholar] [CrossRef]
- Gamage, J.C.P.H.; Al-Mahaidi, R.; Wong, M.B. Bond characteristics of CFRP plated concrete members under elevated temperatures. Compos. Struct. 2006, 75, 199–205. [Google Scholar] [CrossRef]
- Ferrier, E.; Rabinovitch, O.; Michel, L. Mechanical behavior of concrete–resin/adhesive–FRP structural assemblies under low and high temperatures. Constr. Build. Mater. 2016, 127, 1017–1028. [Google Scholar] [CrossRef]
- He, J.; Xian, G.; Zhang, Y.X. Effect of moderately elevated temperatures on bond behaviour of CFRP-to-steel bonded joints using different adhesives. Constr. Build. Mater. 2020, 241, 118057. [Google Scholar] [CrossRef]
- Ahmed, A.; Rahman, M.Z.; Ou, Y.; Liu, S.; Mobasher, B.; Guo, S.; Zhu, D. A review on the tensile behavior of fiber-reinforced polymer composites under varying strain rates and temperatures. Constr. Build. Mater. 2021, 294, 123565. [Google Scholar] [CrossRef]
- Foster, S.K.; Bisby, L.A. High Temperature Residual Properties of Externally-Bonded FRP Systems. In Proceedings of the 7th International Symposium on Fiber Reinforced Polymer Reinforcement for Reinforced Concrete Structures (FRPRCS-7), Kansas City, MO, USA, 6–9 November 2005. [Google Scholar]
- Li, Y.; Liu, X.; Wu, M. Mechanical properties of FRP-strengthened concrete at elevated temperature. Constr. Build. Mater. 2017, 134, 424–432. [Google Scholar] [CrossRef]
- Mikami, C.; Wu, H.C.; Elarbi, A. Effect of hot temperature on pull-off strength of FRP bonded concrete. Constr. Build. Mater. 2015, 91, 180–186. [Google Scholar] [CrossRef]
- Dai, J.-G.; Gao, W.Y.; Teng, J.G. Bond-slip model for FRP laminates externally bonded to concrete at elevated temperature. J. Compos. Constr. 2013, 17, 217–228. [Google Scholar] [CrossRef]
- Al-Shawaf, A.; Al-Mahaidi, R.; Zhao, X.L. Effect of elevated temperature on bond behaviour of high modulus CFRP/steel double-strap joints. Aust. J. Struct. Eng. 2009, 10, 63–74. [Google Scholar] [CrossRef]
- Zhou, A.; Qiu, Q.; Chow, C.L.; Lau, D. Interfacial performance of aramid, basalt and carbon fiber reinforced polymer bonded concrete exposed to high temperature. Compos. Part A Appl. Sci. Manuf. 2020, 131, 105802. [Google Scholar] [CrossRef]
- Selvaratnam, A.; Gamage, J.C.P.H. A Review on Thermo-mechanical Behaviour of CFRP-Concrete Composites at Elevated Temperature and Available Insulation Systems. Lect. Notes Civ. Eng. 2021, 94, 533–541. [Google Scholar] [CrossRef]
- Assad, M.; Hawileh, R.A.; Abdalla, J.A. Modeling the behavior of CFRP-strengthened RC slabs under fire exposure. Procedia Struct. Integr. 2022, 42, 1668–1675. [Google Scholar] [CrossRef]
- Gao, W.Y.; Teng, J.G.; Asce, M.; Dai, J.-G. Effect of Temperature Variation on the Full-Range Behavior of FRP-to-Concrete Bonded Joints. J. Compos. Constr. 2012, 16, 671–683. [Google Scholar] [CrossRef]
- Moussa, O.; Vassilopoulos, A.P.; De Castro, J.; Keller, T. Time-temperature dependence of thermomechanical recovery of cold-curing structural adhesives. Int. J. Adhes. Adhes. 2012, 35, 94–101. [Google Scholar] [CrossRef]
- Tatar, J.; Milev, S. Durability of externally bonded fiber-reinforced polymer composites in concrete structures: A critical review. Polymers 2021, 13, 765. [Google Scholar] [CrossRef] [PubMed]
- Firmo, J.P.; Correia, J.R.; França, P. Fire behaviour of reinforced concrete beams strengthened with CFRP laminates: Protection systems with insulation of the anchorage zones. Compos. B Eng. 2012, 43, 1545–1556. [Google Scholar] [CrossRef]
- Firmo, J.P.; Correia, J.R.; Bisby, L.A. Fire behaviour of FRP-strengthened reinforced concrete structural elements: A state-of-the-art review. Compos. B Eng. 2015, 80, 198–216. [Google Scholar] [CrossRef]
- Chowdhury, E.U.; Eedson, R.; Bisby, L.A.; Green, M.F.; Benichou, N. Mechanical Characterization of Fibre Reinforced Polymers Materials at High Temperature. Fire Technol. 2011, 47, 1063–1080. [Google Scholar] [CrossRef]
- Selvaratnam, A.; Arachchi, K.A.D.Y.T.K.; Gamage, J.C.P.H.; Attanayake, V. Investigation on an effective bond arrangement of insulated CFRP-strengthened flexural members for improved thermo-mechanical performance. Case Stud. Constr. Mater. 2021, 14, e00544. [Google Scholar] [CrossRef]
- Liu, K. Analytical study on bond characterization of hybrid-bonded FRP to concrete interfaces. Appl. Mech. Mater. 2013, 405–408, 2528–2533. [Google Scholar] [CrossRef]
- Yu, B.; Kodur, V.K.R. Effect of high temperature on bond strength of near-surface mounted FRP reinforcement. Compos. Struct. 2014, 110, 88–97. [Google Scholar] [CrossRef]
- Dai, J.; Ueda, T.; Sato, Y. Development of the Nonlinear Bond Stress–Slip Model of Fiber Reinforced Plastics Sheet–Concrete Interfaces with a Simple Method. J. Compos. Constr. 2005, 9, 52–62. [Google Scholar] [CrossRef]
- Blontrock, H.; Taerwe, L.; Vanwalleghem, H. Bond testing of externally glued FRP laminates at elevated temperatures. In Proceedings Bond in Concrete-From Research to Standards; Ghent University: Ghent, Belgium, 2002; pp. 648–654. [Google Scholar]
- Guo, D.; Liu, Y.L.; Gao, W.Y.; Dai, J.G. Bond behavior of CFRP-to-steel bonded joints at different service temperatures: Experimental study and FE modeling. Constr. Build. Mater. 2023, 362, 129836. [Google Scholar] [CrossRef]
- Hawileh, R.A.; Naser, M.; Zaidan, W.; Rasheed, H.A. Modeling of insulated CFRP-strengthened reinforced concrete T-beam exposed to fire. Eng. Struct. 2009, 31, 3072–3079. [Google Scholar] [CrossRef]
- Kodur, V.K.R.; Bisby, L.A. Evaluation of Fire Endurance of Concrete Slabs Reinforced with Fiber-Reinforced Polymer Bars. J. Struct. Eng. 2005, 131, 34–43. [Google Scholar] [CrossRef]
- Bhatt, P.P.; Kodur, V.K.R.; Shakya, A.M.; Alkhrdaji, T. Performance of insulated FRP-strengthened concrete flexural members under fire conditions. Front. Struct. Civ. Eng. 2021, 15, 177–193. [Google Scholar] [CrossRef]
- Zhu, H.; Wu, G.; Zhang, L.; Zhang, J.; Hui, D. Experimental study on the fire resistance of RC beams strengthened with near-surface-mounted high-Tg BFRP bars. Compos. B Eng. 2014, 60, 680–687. [Google Scholar] [CrossRef]
- Saafi, M. Effect of Fire on FRP Reinforced Concrete Members. Compos. Struct. 2002, 58, 11–20. [Google Scholar] [CrossRef]
- Maraveas, C.; Miamis, K.; Vrakas, A.A. Fiber-reinforced polymer-strengthened/reinforced concrete structures exposed to fire: A review. Struct. Eng. Int. J. Int. Assoc. Bridge Struct. Eng. (IABSE) 2012, 22, 500–513. [Google Scholar] [CrossRef]
- Chowdhury, E.U.; Bisby, L.A.; Green, M.F.; Kodur, V.K.R.; Asce, F. Residual Behavior of Fire-Exposed Reinforced Concrete Beams Prestrengthened in Flexure with Fiber-Reinforced Polymer Sheets. J. Compos. Constr. 2008, 12, 61–68. [Google Scholar] [CrossRef]
- Ahmed, A.; Kodur, V.K.R. Effect of bond degradation on fire resistance of FRP-strengthened reinforced concrete beams. Compos. B Eng. 2011, 42, 226–237. [Google Scholar] [CrossRef]
- Li, J.; Gravina, R.J.; Smith, S.T.; Visintin, P. Bond strength and bond stress-slip analysis of FRP bar to concrete incorporating environmental durability. Constr. Build. Mater. 2020, 261, 119860. [Google Scholar] [CrossRef]
- Dong, K.; Hu, K.; Gao, W. Fire behavior of full-scale CFRP-strengthened RC beams protected with different insulation systems. J. Asian Archit. Build. Eng. 2016, 15, 581–588. [Google Scholar] [CrossRef]
- Lu, X.Z.; Teng, J.G.; Ye, L.P.; Jiang, J.J. Bond-slip models for FRP sheets/plates bonded to concrete. Eng. Struct. 2005, 27, 920–937. [Google Scholar] [CrossRef]
- Chang, Y.F.; Chen, Y.H.; Sheu, M.S.; Yao, G.C. Residual stress-strain relationship for concrete after exposure to high temperatures. Cem. Concr. Res. 2006, 36, 1999–2005. [Google Scholar] [CrossRef]
- Wang, K.; Young, B.; Smith, S.T. Mechanical properties of pultruded carbon fibre-reinforced polymer (CFRP) plates at elevated temperatures. Eng. Struct. 2011, 33, 2154–2161. [Google Scholar] [CrossRef]
- Wang, Z.; Dai, J.G.; Wang, M.; Chen, L.; Zhang, F.; Xu, Q. Residual bond strengths of epoxy and cement-bonded CFRP reinforcements to concrete interfaces after elevated temperature exposure. Fire Saf. J. 2021, 124, 103393. [Google Scholar] [CrossRef]
- Chandrathilaka, E.R.K.; Gamage, J.C.P.H.; Fawzia, S. Numerical modelling of bond shear stress slip behavior of CFRP/steel composites cured and tested at elevated temperature. Compos. Struct. 2019, 212, 1–10. [Google Scholar] [CrossRef]
- Biscaia, H.C.; Chastre, C.; Viegas, A.; Franco, N. Numerical modelling of the effects of elevated service temperatures on the debonding process of FRP-to-concrete bonded joints. Compos. B Eng. 2015, 70, 64–79. [Google Scholar] [CrossRef]
- Krzywoń, R. Behavior of EBR FRP Strengthened Beams Exposed to Elevated Temperature. Procedia Eng. 2017, 193, 297–304. [Google Scholar] [CrossRef]
- Pellegrino, C.; Tinazzi, D.; Modena, C. Experimental Study on Bond Behavior between Concrete and FRP Reinforcement. J. Compos. Constr. 2008, 12, 180–189. [Google Scholar] [CrossRef]
- Aguiar, J.B.; Camões, A.; Vaz, N.F. Effect of temperature on RC elements strengthened with CFRP. Mater. Struct./Mater. Constr. 2008, 41, 1133–1142. [Google Scholar] [CrossRef]
- Gamage, J.C.P.H.; Al-Mahaidi, R.; Wong, B.; Ariyachandra, M.R.E.F. Bond characteristics of CFRP-strengthened concrete members subjected to cyclic temperature and mechanical stress at low humidity. Compos. Struct. 2017, 160, 1051–1059. [Google Scholar] [CrossRef]
- Zhou, H.; Torres, J.P.; Fernando, D.; Law, A.; Emberley, R. The bond behaviour of CFRP-to-steel bonded joints with varying bond properties at elevated temperatures. Eng. Struct. 2019, 183, 1121–1133. [Google Scholar] [CrossRef]
- Kalyani, V.J.; Joshi, D.D.; Patel, P.V. Study of the behaviour of FRP materials and epoxy adhesives under elevated temperature. Mater. Today Proc. 2023, in press. [Google Scholar] [CrossRef]
- Hashemi, S.; Al-Mahaidi, R. Flexural performance of CFRP textile-retrofitted RC beams using cement-based adhesives at high temperature. Constr. Build. Mater. 2012, 28, 791–797. [Google Scholar] [CrossRef]
- Widanage, C.; Gamage, J.C.P.H.; De Silva, G.I.P. Bond characteristics of CFRP strengthened concrete members bonded using Modified Engineered Cementitious Composite. Case Stud. Constr. Mater. 2021, 15, e00766. [Google Scholar] [CrossRef]
- Leone, M.; Matthys, S.; Aiello, M.A. Effect of elevated service temperature on bond between FRP EBR systems and concrete. Compos. B Eng. 2009, 40, 85–93. [Google Scholar] [CrossRef]
- Dong, K.; Hu, K. Development of bond strength model for CFRP-to-concrete joints at high temperatures. Compos. B Eng. 2016, 95, 264–271. [Google Scholar] [CrossRef]
- Mofrad, M.H.; Mostofinejad, D.; Hosseini, A. A generic non-linear bond-slip model for CFRP composites bonded to concrete substrate using EBR and EBROG techniques. Compos. Struct. 2019, 220, 31–44. [Google Scholar] [CrossRef]
- Wang, Y.J.; Wu, Z.M.; Liu, H.B.; Zhang, Q.M.; Yang, S.T.; Li, Y.C. Influence of thermal effects on debonding response of CFRP-to-concrete/steel interfaces under thermal and mechanical loads: An analytical solution. Compos. Struct. 2023, 303, 116333. [Google Scholar] [CrossRef]
- Klamer, E.L.; Hordijk, D.A.; Janssen, H.J.M. The Influence of Temperature on the Debonding of Externally Bonded CFRP. Spec. Issue ACI 2005, 23, 1551–1570. [Google Scholar]
- Wu, Z.S.; Iwashita, K.; Yagashiro, S.; Ishikawa, T.; Hamaguchi, Y. Temperature effect on bonding and debonding behavior b tween FRP sheets and concrete. J. Soc. Mater. Sci. 2005, 54, 474–480. [Google Scholar] [CrossRef]
- Camata, G.; Pasquini, F.; Spacone, E. High temperature flexural strengthening with externally bonded FRP reinforcement. In Proceedings of the 8th International Symposium on Fiber Reinforced Polymer (FRP) Reinforcement for Concrete Structures (FRP8RCS), Patras, Greece, 16–18 July 2007; pp. 1–10. [Google Scholar]
- Hu, K.X.; Lu, F.; Cai, Z.H. Researches on mechanical property of CFRP-concrete interface at elevated temperatures. J. Tongji Univ. 2009, 37, 1592–1597. [Google Scholar]
- Hu, K.X.; Peng, D.P.; Zhao, C.X. Influence of temperature on the bond-slip behavior of CFRP-concrete interface. J. Disaster Prev. Mitig. Eng. 2012, 32, 45–49. [Google Scholar]

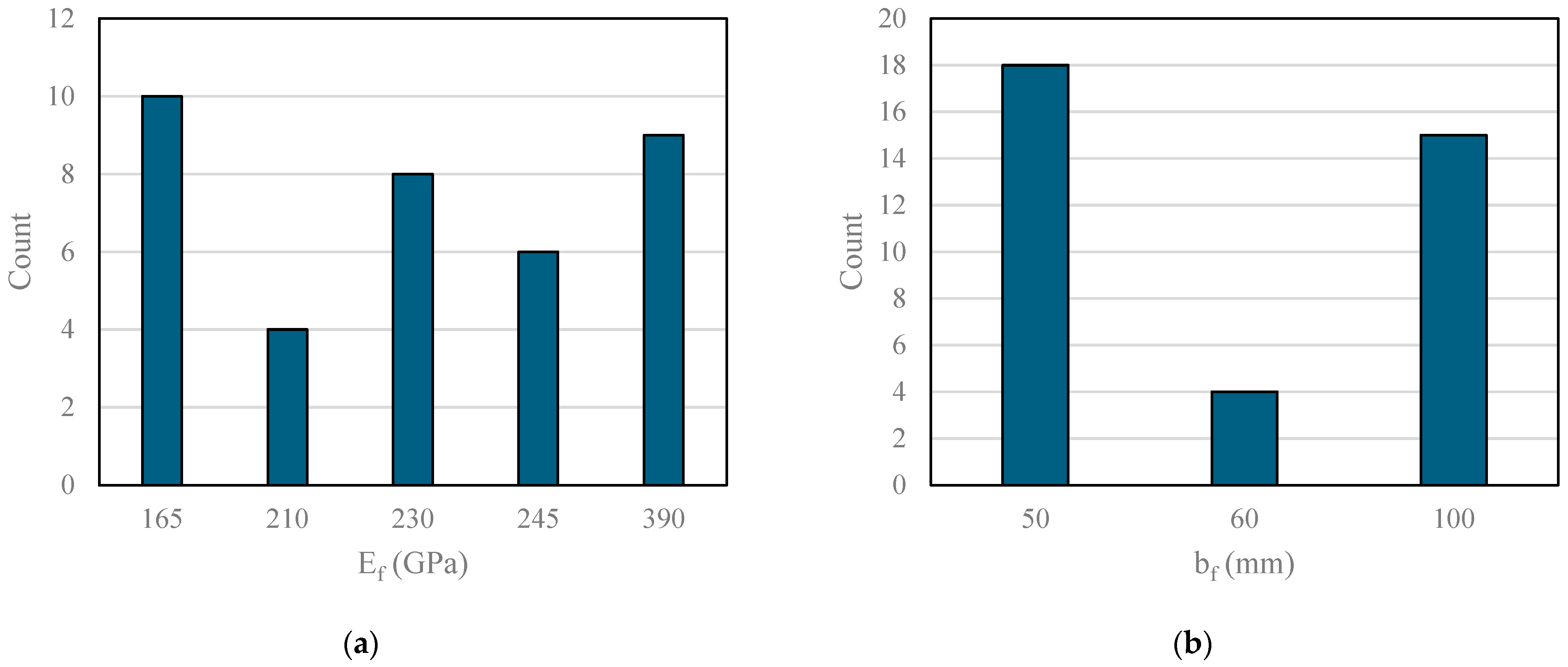
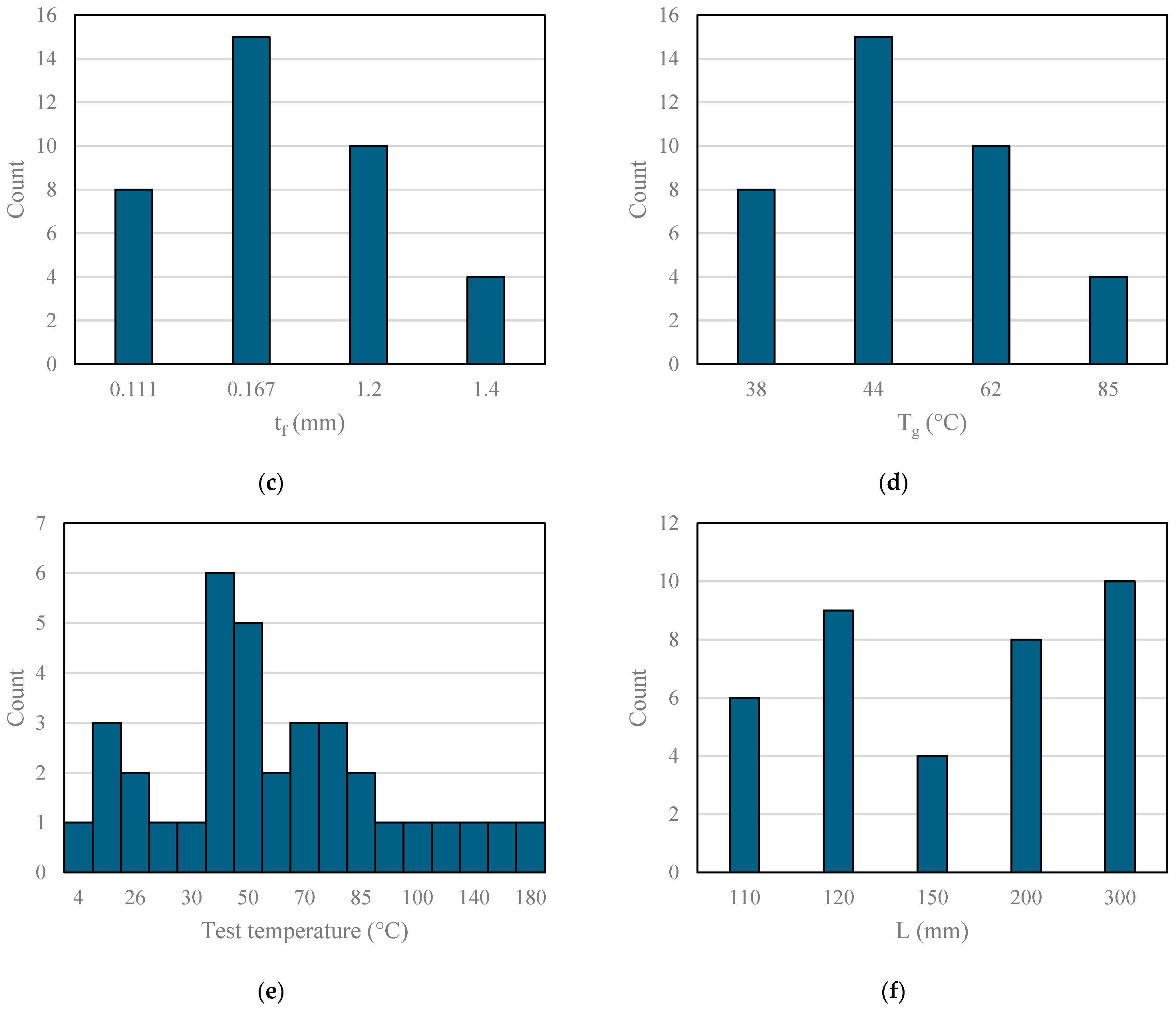
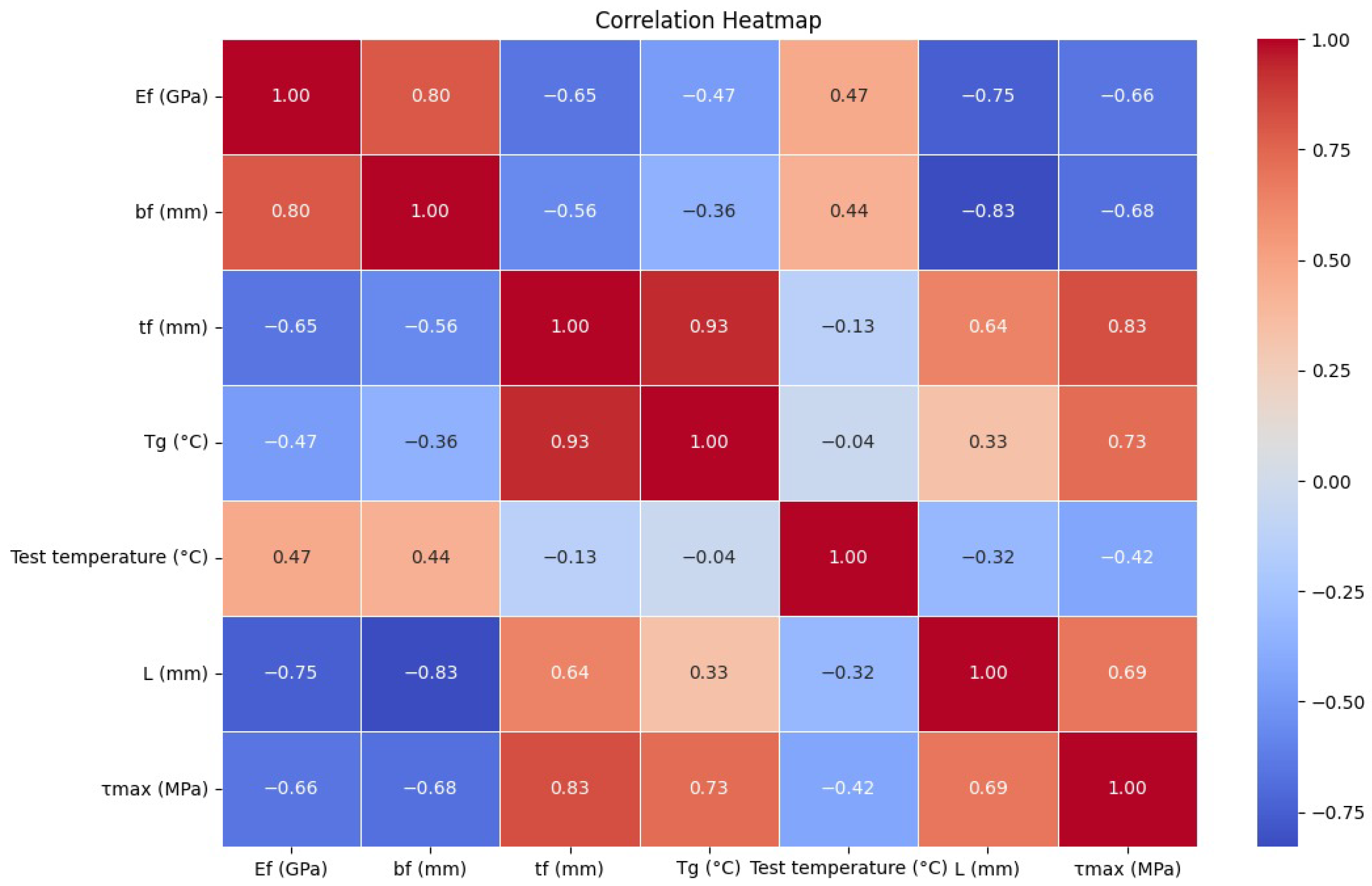


| Temp. Range | Bond Strength Reduction | Failure Modes | Bond–Slip Relationship |
|---|---|---|---|
| Up to 100 | A 30% reduction compared with ambient temperatures. | A combination of cohesive failure in concrete and adhesive failure at the interface. | Decrease in stiffness and an increase in slip values. |
| 100–300 | Reduction of up to 50% | Adhesive failure at the FRP–concrete interface (debonding of the FRP). | A substantial decrease in maximum bond stress, decreased stiffness, and increased slip values. |
| 300–600 | Reduction up to 70% compared with ambient conditions | Adhesive failure at the interface or debonding of the FRP system becomes more prevalent. | A significant decrease in maximum bond stress and increased slip values. |
| More than 600 | Potential complete loss of bond strength. | Delamination of the FRP system is commonly observed because of thermal degradation and weakening of the bond interface. | Very low bond stress values and large slip values, indicating a near-complete loss of bond integrity [19,20]. |
| Author(s) | Model Type | Key Features | Advantages | Disadvantages |
|---|---|---|---|---|
| Dai et al. [41] | Nonlinear | - Based on extensive experimental data; considers residual stresses. | - Accounts for thermal degradation up to 120 °C. | - Complexity in parameter determination. |
| Arruda et al. [18] | Bilinear | - Uses the cohesive zone model (CZM) and is implemented with the finite element method. | - Good agreement with experimental results. - Captures reduction in bond strength and stiffness. - Applicable to both NSM and EBR techniques. | - Requires empirical parameters from experiments. - It may be computationally intensive. |
| Leone et al. [68] | Exponential | - Focused on temperatures up to 60 °C. -Based on double-shear pull-out tests. | - Relatively simple formulation. | - Limited temperature range and may not be applicable to extreme temperature conditions. |
| Dong et al. [69] | Two-parameters exponential | - Considers bond length and free-end slip. | - Simplified formulas for peak load prediction. - Good agreement with experimental data for bond strength reduction. | - May not capture complex behaviors at very high temperatures. - Accuracy depends on the quality of regression analysis. |
| Jia D et al. [27] | Nonlinear equation | - Isolates interfacial thermal stress effects. | - Provides comprehensive thermal stress analysis. - Versatile across different structural contexts. | - Mathematically complex for practical application. - Requires detailed material property data. |
| Wang Y et al. [71] | Trilinear | - Considers arbitrary bond lengths. - Includes interfacial friction. - For thermomechanical loading. | - Captures the whole debonding process. - Introduces the concept of effective bond length change with temperature. - Efficient as a coupled thermal–mechanical tool. | - Assumes uniform thermal expansion and shear deformation. - May require complex numerical implementation. |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Salameh, A.; Hawileh, R.; Safieh, H.; Assad, M.; Abdalla, J. Elevated Temperature Effects on FRP–Concrete Bond Behavior: A Comprehensive Review and Machine Learning-Based Bond Strength Prediction. Infrastructures 2024, 9, 183. https://doi.org/10.3390/infrastructures9100183
Salameh A, Hawileh R, Safieh H, Assad M, Abdalla J. Elevated Temperature Effects on FRP–Concrete Bond Behavior: A Comprehensive Review and Machine Learning-Based Bond Strength Prediction. Infrastructures. 2024; 9(10):183. https://doi.org/10.3390/infrastructures9100183
Chicago/Turabian StyleSalameh, Aseel, Rami Hawileh, Hussam Safieh, Maha Assad, and Jamal Abdalla. 2024. "Elevated Temperature Effects on FRP–Concrete Bond Behavior: A Comprehensive Review and Machine Learning-Based Bond Strength Prediction" Infrastructures 9, no. 10: 183. https://doi.org/10.3390/infrastructures9100183
APA StyleSalameh, A., Hawileh, R., Safieh, H., Assad, M., & Abdalla, J. (2024). Elevated Temperature Effects on FRP–Concrete Bond Behavior: A Comprehensive Review and Machine Learning-Based Bond Strength Prediction. Infrastructures, 9(10), 183. https://doi.org/10.3390/infrastructures9100183







