Topographic Measurements and Statistical Analysis in Static Load Testing of Railway Bridge Piers

Abstract

The aim of the paper is to identify a suitable method for assessing the deformation of structures (buildings, bridges, walls, etc.) by means of topographic measurements of significant targets positioned on the infrastructure under consideration. In particular, the paper describes an approach to testing a bridge in a mixed structure (concrete and steel). The methodological approach developed can be schematised into the following main phases: (i) surveying using total stations (TSs) in order to obtain the spatial coordinates of the targets by means of the three-dimensional intersection technique (planimetric and altimetric measurements); (ii) least-squares compensation for the measurements performed; (iii) displacement analysis; and (iv) statistical evaluation of the reliability of the results. This method was evaluated on a case study of a newly built double-track railway bridge, located near the metropolitan area of the city of Bari, Italy, during various loading and unloading activities. The results obtained, evaluated by means of certain statistical tests, made it possible to verify the structural suitability of the bridge.

1. Introduction

The monitoring of structures plays an important role in the field of civil engineering, especially in the testing phase, as it allows for the verification of its structural behaviour in the different load phases [1,2,3]. The monitoring of civil engineering or buildings can be performed using several geomatics techniques [4,5,6]. For example, Global Navigation Satellite System (GNSS) technology has been widely applied as an essential part of a Structural Health Monitoring (SHM) system [7]. Its first applications in monitoring bridges were carried out by Roberts et al. [8], attaching Ashtech ZXII GPS receivers onto the “Humber Bridge” parapet and gathering and further analysing the resulting 1 Hz Real-Time Kinematic (RTK) GNSS data; in this way, certain authors have performed several experiments on the Humber Bridge (Kingston upon Hull, England), the Millennium Bridge (London, England), the Forth Road Bridge (from Edinburgh to Fife, England), the Severn Suspension Bridge (South West England/South East Wales), and the Avonmouth Viaduct (Avonmouth—Bristol, England). In the literature, several applications based on the GNSS approach have been carried out [9,10]. This is due to the ease of application and the ability to quickly, reliably, and continuously obtain and store data on the structure’s movements. However, GNSS-based approaches require an unobstructed environment to receive satellites; as a result, monitoring certain parts of the structure may not be possible since good satellite coverage cannot be obtained. For this reason, the use of total stations (TSs) is widely used for monitoring structures [11]. In addition, TSs allow for higher precision than satellite techniques, especially in specific parts of infrastructure. With the technological development of increasingly accurate total stations and least-squares adjustment using computer programs, deformation control and monitoring activities become increasingly available and accurate [12].

Costantino et al. [13] discussed about 12 survey campaigns performed using total stations in order to verify the movements of a building located in the city of Lecce (Italy). Lienhart et al. [14] wrote about the monitoring of bridge vibrations using Robotic Total Stations (RTSs); in particular, the authors discussed several methods to increase the measurement frequency of commercially available total stations. Marendić et al. [15] discussed the use of RTSs for measuring displacements in the Sava railway bridge (Croatia). Jankauskiene et al. [16] discussed an analysis of the results achieved by using TSs in the process of diagnostics of the geometrical position of the pier structures built at Klaipeda Seaport (Lithuania). Considering this latest research [11,12,13,14,15,16], it is evident that the use of TSs for the structural monitoring of infrastructures continues to be a useful technology due to the flexibility and precision achievable in the determination of possible displacements. Therefore, the research to be discussed in this paper concerns a topographic approach based on the use of least-squares approach and specific statistical criteria for analysing the measurements performed with the use of TS.

2. Methods

In order to determine and evaluate the displacements and/or deformations of a structure, it is first necessary to carry out a survey with the appropriate topographical instrumentation in order to obtain the spatial coordinates of the targets using the three-dimensional intersection technique (planimetric and altimetric measurements). The values of the topographic measurements obtained during the survey phase are LS-compensated; the reliability of these values, analyses, and results obtained are validated through appropriate statistical tests. The approach used in monitoring with the LS method for the topographic measurements and the statistical analyses for the reliability of the results and observed values are discussed in the following sections.

The following pipeline (Figure 1) describes the operational approach to the verification of the deformation and possible displacement/rotation of the bridge piers by means of topographical survey activities connected to the static testing of this construction.

2.1. Least-Squares Approach to the Topographic Measurements

Topographical monitoring is performed using a TS, which is an electronic/optical instrument that combines an electronic distance measuring (EDM) device with an electronic theodolite and a computer. The TS measures the angle on two planes, the X–Y (horizontal plane) and the X–Z plane (vertical). The EDM measures the slope distance from the prisms it is pointed at, while the on-board computer stores and calculates a large number of values from these three measurements. Two different methods of measurement can be distinguished: “phase shift” and “time of flight” (TOF), also known as “impulse” [17]. In phase shift measurements, by measuring the phase shift between the emitted and received wave, more accurate measurements are obtained but with the disadvantage of reduced ranges. TOF measurements, on the other hand, are characterised by higher ranges but lower accuracy than the previous ones [18]. Consequently, in the monitoring of structures, it is preferred to adopt a phase-based TS. In addition, the TS to be used for monitoring must have a high accuracy in angular and distance measurements. In fact, it is desirable to have a TS with an angular accuracy less than or equal to 1” in order to obtain accurate measurements [19,20]; however, the choice of TS must take into account the order of magnitude of the expected displacements and/or deformations under specific loading conditions and the required specifications in terms of the quality of the results.

The measurements (angles and distances, i.e., the polar coordinates) of the targets positioned on the structure are transformed into Cartesian coordinates. The position of the targets is chosen in relation to the specific parts of the structure to be investigated; furthermore, in order to have redundant and reliable measurements, a scheme similar to the forward intersection scheme is adopted, i.e., each point to be determined must be collimated by at least two vertices, according to the scheme illustrated in Figure 2.

According to the scheme shown in Figure 2, the coordinates of the two vertices $(x_{s1}{,y}_{s1}{,z}_{s1};x_{s2}{,y}_{s2}{,z}_{s2})$ are known and, without loss of generality, let us assume that S₁ is the origin of the considered reference system. In addition, it is necessary to measure the angles ${\theta }_{1P}$,${ \theta }_{2P}$ and the distances $d_{1P}$, $d_{2P}$. The target coordinates are determined by means of a plano-altimetric compensation with the implementation of the least-squares approach (see Appendix A).

2.2. Statistical Analysis

The results for the object to be investigated are typically represented by a cluster of points, whose positions are fixed using topographic processing at different epochs. The displacement of the clusters is consequent to any displacement of the points; however, the observed differences should be the same order of magnitude as the observational errors. To this end, it is necessary to perform a series of statistical analyses to reasonably identify significant shifts in each individual control point, generally materialised through reflecting prisms (also called targets). In the present case study, two statistical approaches were applied: the classical approach and the Bayesian approach (Appendix B). Specifically, in the first approach, the data are considered realisations of random variables, and the unknown parameters deterministic. In the second approach, the data are considered constant, and the unknown parameters are considered random variables characterised by $a priori pdfP\left(\theta \right)$. Therefore, the Bayesian approach allows the a priori information of the parameters to be updated and, consequently, the update is reflected in the definition of a $pdfP(\theta /d)a posteriori$.

3. Case Study

The bridge to be tested is located in the Apulia region, Italy (Figure 3a), and was built for the Bari–Taranto railway line. In particular, the structure under investigation is located in the municipality of Bitetto, near the city of Bari (Figure 3b,c). The deck consists of a mixed steel–concrete structure, as shown in Figure 3d.

The bridge consists of a sequence of eight spans with a static scheme of simply supported beams. The central spans have a span of 40 m in the pile axis, while the bank spans have a span of 39 m between the pile axis and the abutment axis.

The verifications were carried out for the testing activities relating to the commissioning of a newly constructed railway bridge. For such verifications, the load is usually applied by means of the transit and stationing at known positions of suitably loaded heavy vehicles whose real weight is certified using electronic weighing or, as in the present case, by means of loads consisting of layers of concrete blocks, each weighing 21 kN (Figure 3e). 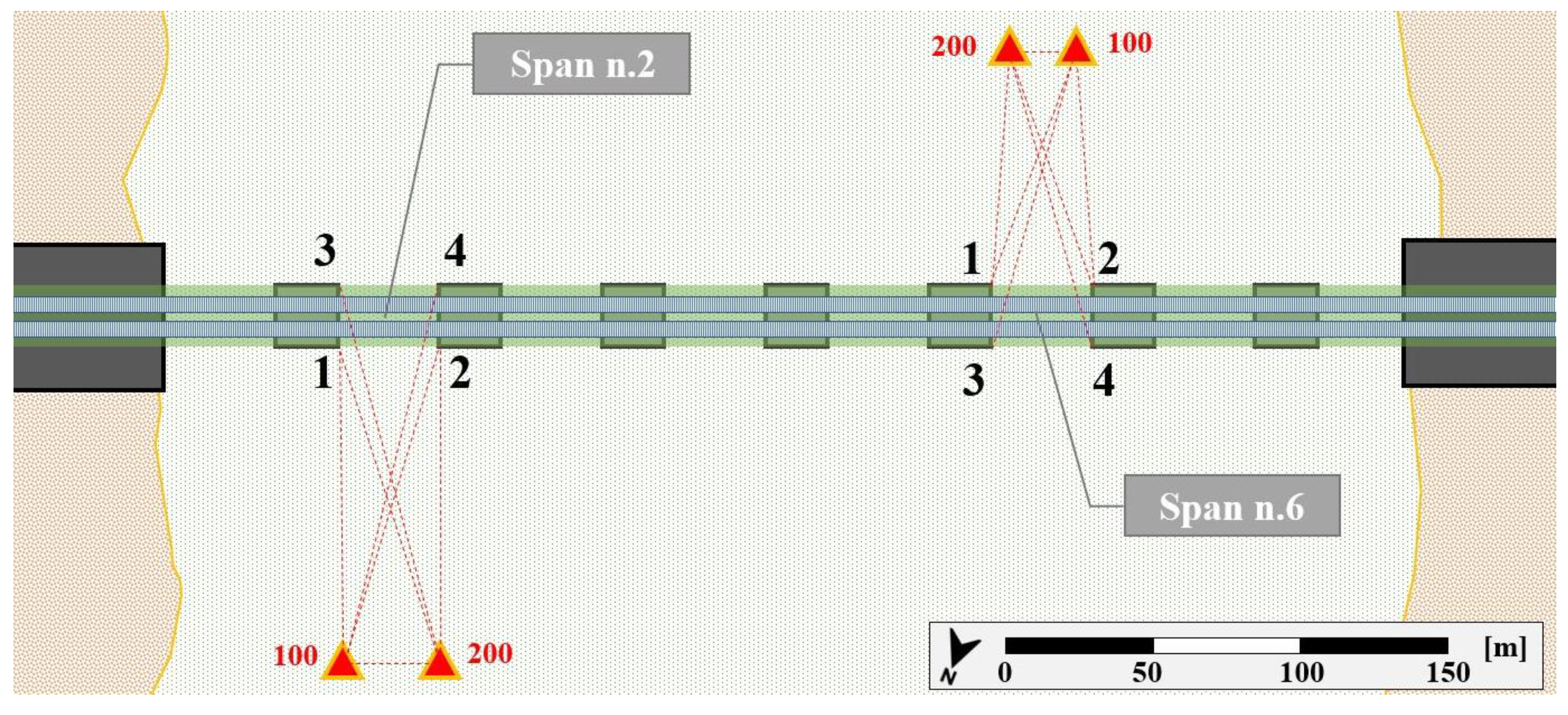In accordance with the Italian network guidelines: “Guidelines for the static testing of works of art on a railway line”, which envisage at least one test every five spans, separate load tests were carried out on spans n.2 and n.6.

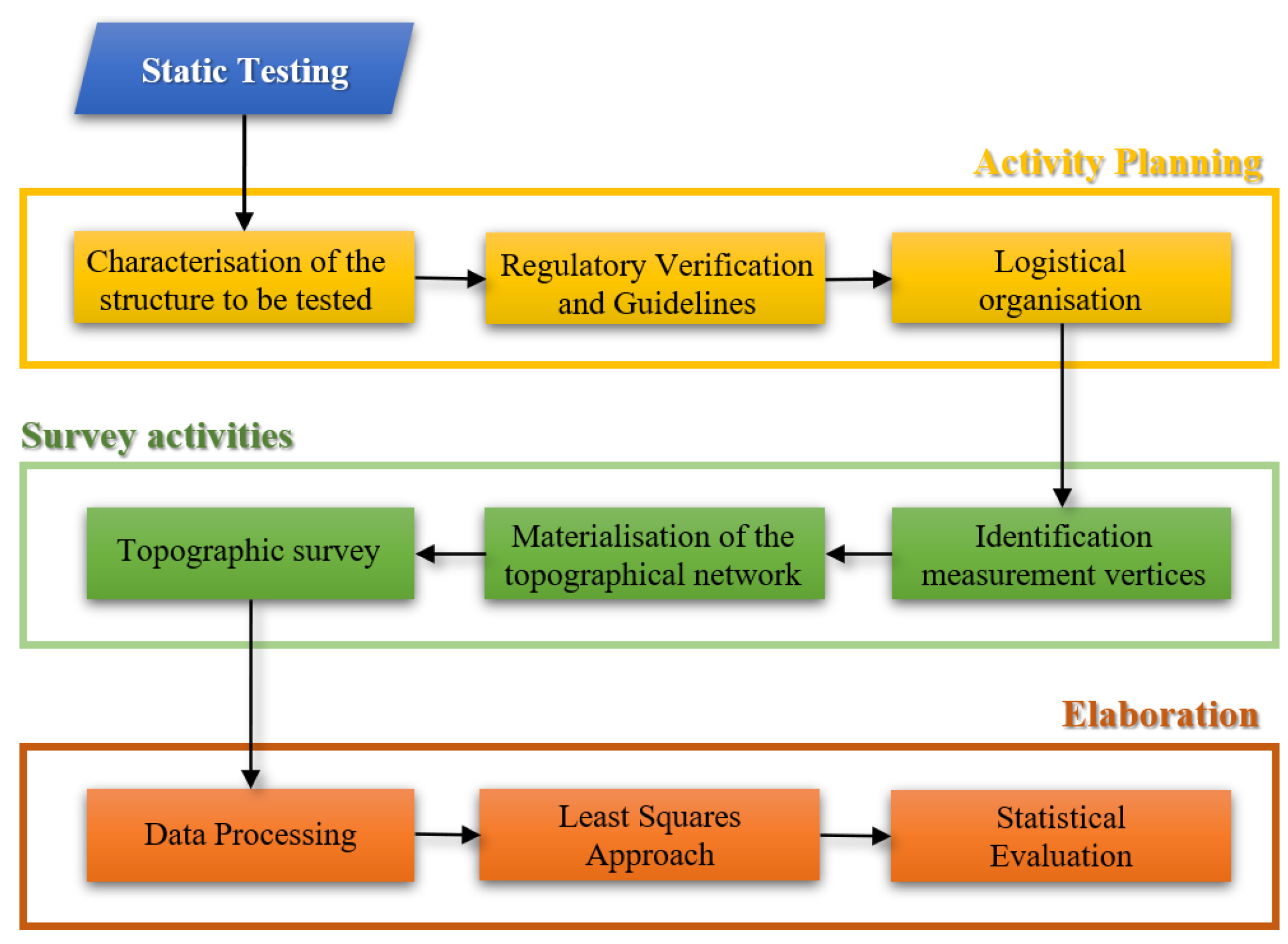
In order to plan the survey activities, it was necessary to carry out an accurate inspection of the infrastructure to be surveyed and the relative landscape and environmental context into which it is inserted. In fact, in this phase, it is important to precisely define the positions of the station vertices and the points to be surveyed, in order to guarantee optimal visibility between them, excluding all the elements that may possibly create disturbance or interference to the subsequent survey activities.

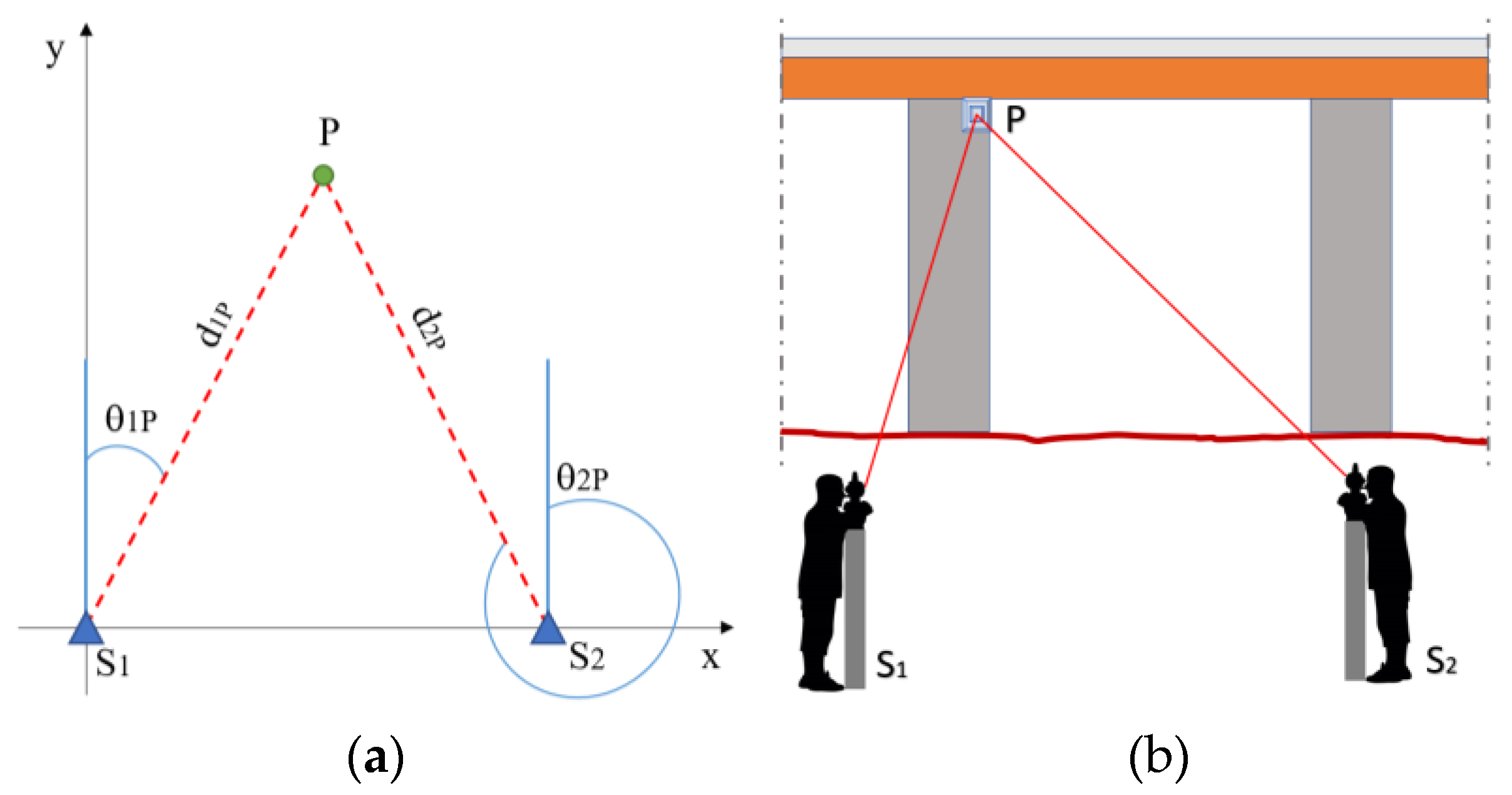
Regarding the design of the topographical network, the installation of four prisms was planned, two for each span, as shown in Figure 4, with respect to which the reference axes were defined; in particular, the x-axis is identified by the conjunction of the two station vertices, the y-axis normal to that direction, and finally the z-axis, coinciding with the local vertical of the station point.

In general, as a reference system, to measure three-dimensional displacements, a local reference system referring to the topographical field was used, given the minimal planimetric extensions of the structure.

Furthermore, the materialisation of the points to be measured and the station vertices is as such to allow the correct interpretation of the structure’s behaviour in terms of three-dimensional displacements. The materialisation of points includes all those construction operations that allow the various topographical operations to be carried out accurately: pillars for the materialisation of station vertices and the installation of mini prisms on the points to be monitored. For each vertex, it was decided to build a foundation with a square base (1 m × 1 m base and 0.20 m height); a pillar with a circular cross-section (0.20 m diameter, 1.60 m height) was realised on this type of foundation (Figure 5a,b).

In the centre of the top plane of the pillar, an aluminium plate was installed for the fixed anchorage. The plate contains, in its geometric centre, the exact vertical of the station vertex and the invitation for the instrumentation base (Figure 5c).

In the design phase, the targets were located on the structure to ensure visibility from the two station vertices. The targets were materialised on the structural elements of the bridge through the installation of miniature monitoring prisms anchored to the piers (Figure 5d). The survey scheme was carried out in order to perform a rigorous least-squares compensation starting from the two station vertices visible from each other.

The survey was carried out using a total station characterised by an accuracy of 1 mm + 1.5 ppm for the EDM measurements and, thanks to a four-axis compensator, allowed for reliable angular measurements with an angular accuracy of 1” (

Table 1. Technical characteristics of the total station TS11.

Image	Angle Measurement
Leica Viva TS11	Accuracy	1″ (0.3 mgon)
	Method	Absolute, continuous, diametrical
	Compensator setting accuracy	Quadruple-axis compensation
	Distance Measurement with Reflector
	Range round prism	3500 m
	Accuracy	Standard: 1.0 mm + 1.5 ppm Fast: 2.0 mm + 1.5 ppm Tracking: 3.0 mm + 1.5 ppm
	Measurement time	1.0 s
	Enhanced measurement accuracy to prism	1.0 mm + 1.5 ppm
	Distance Measurement without Reflector
	Range PinPoint R1000	>1000 m
	Accuracy	2 mm + 2 ppm

).

In order to obtain a functional dataset, each collimation direction was uniquely identified by a pair of numbers consisting of, in order, the station vertex number and the collimated vertex number. In addition, in order for the accuracy of the measurement to depend only on accidental errors, it was necessary to define surveying operating procedures, which consist of carrying out the measurements in a condition where there are no systematic errors. For this reason, the survey was conducted using the methodology of direct and conjugate measurements, allowing the measurements to be averaged and a result unaffected by any systematic errors to be obtained.

Once the topographical operations were completed, the surveyed data were exported in ASCII format in order to carry out subsequent processing in suitably prepared spreadsheets. In particular, the algorithms and processes were implemented in Microsoft Excel, in which the measurements of the azimuth angles (with respect to the instrument zero whose direction, in turn, is treated as an unknown in the problem), distances, zenith angles, and elevation differences, each with the a priori root mean square error (RMS), were reported in precise order. In addition, the approximate coordinates of the points and constraints were assigned, i.e., the coordinates of the known points (coordinates calculated a priori) and the azimuths of the known directions, which define the reference system. Once the input data were defined, it was possible to compensate for the topographical network using the method of least squares. In addition, iteration processes were set up in order to assess the convergence of the results as a function of the error factor. Generally, after three iterations, the result showed convergence.

According to the project for the testing of the structure, the load test of each of the two decks was to be preceded by a 24 h unloaded deck thermal monitoring during which eight reading cycles were to be performed. At the end of the thermal monitoring, the decks were to be loaded and unloaded in 14 steps. Two reading cycles were required for each step. The structure was monitored under different environmental and load conditions. Regarding monitoring under different environmental conditions, referred to as “thermal monitoring”, eight measurement cycles were carried out over a 24 h period under visibility conditions and from the minimum to maximum temperature (temperature range). Specifically, the eight cycles were performed every 3 h starting at 06:00 a.m.

The monitoring performed with the different load conditions included two cycles for each loading and unloading every 30 min, as shown in

Table 2. Load test span n.6.

Step n°	Date	Time I° Session	Time II° Session
0 (Zero Load)	Day 2	09:30	10:00
2 (1/3 Load)	Day 3	06:45	07:15
6 (2/3 Load)	Day 3	13:45	14:30
10 (Max Load)	Day 4	10:00	10:30
10 (Max Load)	Day 5	07:00	07:30
6 (2/3 Load)	Day 5	15:15	15:50
2 (1/3 Load)	Day 6	09:30	11:20
0 (Zero Load)	Day 7	09:40	10:10

and

Table 3. Load test span n.2.

Step n°	Date	Time I° Session	Time II° Session
0 (Zero Load)	Day 7	12:10	12:50
0 (Zero Load)	Day 7	16:00	16:30
2 (1/3 Load)	Day 8	09:30	10:15
6 (2/3 Load)	Day 8	17:15	17:45
10 (Max Load)	Day 9	11:30	12:30
6 (2/3 Load)	Day 10	08:30	09:00
2 (1/3 Load)	Day 11	09:45	10:30
0 (Zero Load)	Day 11	15:15	15:45

.

4. Results

The monitoring sessions were carried out taking into account specific planning (see Table 2 and Table 3). Regarding the thermal monitoring that preceded (eight measurement sessions) and concluded (six measurement sessions) the topographical tasks, the relative spatial coordinates of the points investigated, were obtained, as shown in

Table 4. Thermal monitoring—span n.6.

ID	Day 1–Cycle 1			Day 1–Cycle 2			Day 1–Cycle 3			Day 1–Cycle 4
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	58.235	−10.373	15.681	58.235	−10.373	15.681	58.235	−10.373	15.681	58.233	−10.373	15.682
2	65.040	−10,214	15.603	65.040	−10.214	15.603	65.040	−10.214	15.603	65.039	−10.213	15.603
3	63.906	23.665	16.173	63.906	23.665	16.173	63.906	23.665	16.173	63.905	23.665	16.173
4	57.112	23.359	16.216	57.112	23.359	16.216	57.112	23.359	16.216	57.111	23.358	16.216
ID	Day 1–Cycle 5			Day 1–Cycle 6			Day 2–Cycle 7			Day 2–Cycle 8
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	58.233	−10.373	15.681	58.235	−10.373	15.681	58.233	−10.373	15.681	58.235	−10.373	15.681
2	65.039	−10.213	15.603	65.040	−10.214	15.602	65.040	−10.214	15.603	65.040	−10.214	15.603
3	63.905	23.665	16.173	63.906	23.665	16.173	63.906	23.665	16.173	63.906	23.665	16.173
4	57.111	23.358	16.216	57.112	23.359	16.215	57.112	23.359	16.216	57.112	23.359	16.216

for span 6 and

Table 5. Thermal monitoring—span n.2.

ID	Day 13–Cycle 17			Day 13–Cycle 18			Day 13–Cycle 19
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	85.458	−9.060	24.605	85.457	−9.060	24,605	85.458	−9.059	24.605
2	92.227	−8.705	24.521	92.227	−8.705	24.521	92.227	−8.705	24.521
3	90.856	25.035	24.006	90.856	25.035	24.006	90.856	25.035	24.006
4	83.891	24.785	24.171	83.891	24.785	24.171	83.891	24.785	24.171
ID	Day 14–Cycle 20			Day 14–Cycle 21			Day 14–Cycle 22
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	85.459	−9.060	24.605	85.458	−9.060	24.605	85.458	−9.060	24.605
2	92.227	−8.705	24.521	92.227	−8.705	24.521	92.226	−8.705	24.521
3	90.856	25.035	24.005	90.856	25.035	24.006	90.856	25.035	24.006
4	83.890	24.785	24.171	83.891	24.785	24.171	83.891	24.785	24.171

for span 2.

The results of the several measurements can be summarised in the following tables and, in particular, the data for the span n.6 are summarised in

Table 6. Load test step 0—span n.6.

ID	Day 2–Cycle 9			Day 2–Cycle 10
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	58.234	−10.373	15.681	58.233	−10.372	15.681
2	65.041	−10.214	15.603	65.041	−10.214	15.603
3	63.906	23.665	16.173	63.906	23.665	16.173
4	57.112	23.359	16.216	57.112	23.359	16.216

,

Table 7. Load test step 2—span n.6.

ID	Day 3–Cycle 11			Day 3–Cycle 12
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	58.233	−10.373	15.683	58.233	−10.373	15.678
2	65.039	−10.213	15.602	65.039	−10.213	15.602
3	63.905	23.665	16.173	63.905	23.665	16.170
4	57.111	23.358	16.216	57.111	23.358	16.215

,

Table 8. Load test step 6—span n.6.

ID	Day 3–Cycle 13			Day 3–Cycle 14
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	58.233	−10.373	15.680	58.233	−10.373	15.681
2	65.039	−10.213	15.603	65.039	−10.213	15.602
3	63.905	23.665	16.173	63.905	23.665	16.173
4	57.111	23.358	16.216	57.111	23.358	16.215

,

Table 9. Load test step 10—span n.6.

ID	Day 4–Cycle 15			Day 4–Cycle 16
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	58.233	−10.373	15.681	58.233	−10.373	15.681
2	65.039	−10.213	15.604	65.039	−10.213	15.603
3	63.905	23.665	16.172	63.905	23.665	16.173
4	57.111	23.358	16.216	57.111	23.358	16.216

,

Table 10. Load test step 10—span n.6.

ID	Day 4–Cycle 17			Day 4–Cycle 18
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	58.233	−10.373	15.681	58.233	−10.373	15.681
2	65.039	−10.213	15.602	65.039	−10.213	15.602
3	63.905	23.665	16.173	63.905	23.665	16.173
4	57.111	23.358	16.216	57.111	23.358	16.216

,

Table 11. Load test step 6—span n.6.

ID	Day 5–Cycle 19			Day 5–Cycle 20
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	58.233	−10.373	15.681	58.232	−10.373	15.681
2	65.039	−10.213	15.602	65.038	−10.213	15.602
3	63.905	23.665	16.173	63.903	23.664	16.173
4	57,111	23.358	16.216	57.111	23.358	16.216

,

Table 12. Load test step 2—span n.6.

ID	Day 6–Cycle 21			Day 6–Cycle 22
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	58.233	−10.373	15.680	58.233	−10.373	15.680
2	65.039	−10.213	15.603	65.039	−10.213	15.603
3	63.905	23.665	16.173	63.905	23.665	16.173
4	57.111	23.358	16.216	57.111	23.358	16.216

and

Table 13. Load test step 0—span n.6.

ID	Day 7–Cycle 23			Day 7–Cycle 24
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	58.232	−10.373	15.680	58.232	−10.373	15.681
2	65.038	−10.213	15.603	65.038	−10.213	15.603
3	63.903	23.664	16.172	63.903	23.664	16.171
4	57.110	23.358	16.216	57.110	23.358	16.217

, while, for span n.2, the data are shown in

Table 14. Load test step 0—span n.2.

ID	Day 7–Cycle 1			Day 7–Cycle 2
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	85.458	−9.060	24.605	85.458	−9.060	24.605
2	92.227	−8.705	24.521	92.227	−8.705	24.521
3	90.856	25.035	24.006	90.856	25.035	24.006
4	83.890	24.785	24.171	83.890	24.785	24.171

,

Table 15. Load test step 0—span n.2.

ID	Day 7–Cycle 3			Day 7–Cycle 4
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	85.459	−9.060	24.605	85.458	−9.060	24.605
2	92.227	−8.705	24.521	92.227	−8.705	24.521
3	90.856	25.035	24.006	90.856	25.035	24.006
4	83.891	24.785	24.171	83.891	24.785	24.171

,

Table 16. Load test step 2—span n.2.

ID	Day 8–Cycle 5			Day 8–Cycle 6
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	85.458	−9.060	24.605	85.459	−9.060	24.605
2	92.227	−8.705	24.521	92.227	−8.705	24.521
3	90.856	25.035	24.006	90.856	25.035	24.006
4	83.891	24.785	24.171	8.891	24.785	24.171

,

Table 17. Load test step 6—span n.2.

ID	Day 8–Cycle 7			Day 8–Cycle 8
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	85.458	−9.060	24.605	85.459	−9.060	24.605
2	92.228	−8.705	24.521	92.227	−8.705	24.521
3	90.856	25.035	24.006	90.856	25.035	24.006
4	83.891	24.785	24.171	83.891	24.785	24.171

,

Table 18. Load test step 10—span n.2.

ID	Day 9–Cycle 9			Day 9–Cycle 10
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	85.458	−9.060	24.605	85.458	−9.060	24.605
2	92.227	−8.705	24,521	92.226	−8.705	24.521
3	90.857	25.035	24.006	90.856	25.035	24.006
4	83.891	24.785	24.171	83.890	24.785	24.171

,

Table 19. Load test step 6—span n.2.

ID	Day 10–Cycle 11			Day 10–Cycle 12
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	85.459	−9.060	24.605	85.458	−9.060	24,605
2	92.227	−8.705	24.521	92.227	−8.705	24.521
3	90.856	25.035	24.006	90.856	25.035	24.006
4	83.890	24.785	24.171	83.890	24.785	24.171

,

Table 20. Load test step 2—span n.2.

ID	Day 11–Cycle 13			Day 11–Cycle 14
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	85.460	−9.060	24.605	85.459	−9060	24,605
2	92.227	−8.705	24.521	92.228	−8.705	24.521
3	90.856	25.035	24.006	90.856	25.035	24.006
4	83.891	24.785	24.171	83.891	24.785	24.171

and

Table 21. Load test step 0—span n.2.

ID	Day 11–Cycle 15			Day 11–Cycle 16
	x [m]	y [m]	Q [m]	x [m]	y [m]	Q [m]
1	85.459	−9.060	24.605	85.459	−9060	24.605
2	92.226	−8.705	24.521	92.227	−8.705	24.521
3	90.856	25.035	24.006	90.856	25.035	24.006
4	83.891	24.785	24.171	83.890	24.785	24.171

.

For each table, the IDs attributed to each reflector relative to each span, the planimetric x and y coordinates, and the relative elevation value, relative to the monitoring base, the origin of the reference system, are shown.

As can be seen from Table 4 and Table 5, no significant changes in displacements were detected during the thermal monitoring.

In terms of the statistical analysis, two different approaches were conducted on the spans under investigation. In particular, the analyses were carried out considering the elaborations performed in the case of zero load and the respective elaborations in the case of the maximum load. The values obtained from the classical statistical approach are shown below (

Table 22. Z_test of span n.6.

ID	Day 2–Day 5
	$∆\mathit{X}$ [m]	$∆\mathit{Y}$ [m]	$∆\mathit{Q}$ [m]	${\mathit{Z}}_{\raisebox{1ex}{$\mathit{\alpha }$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}\mathit{X}$	${\mathit{Z}}_{\raisebox{1ex}{$\mathit{\alpha }$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}\mathit{Y}$	${\mathit{Z}}_{\raisebox{1ex}{$\mathit{\alpha }$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}\mathit{Q}$
1	0.0001	0.0002	0.0003	0.997	0.998	0.968
2	0.0014	0.0002	0.0016	0.587	0.977	0.635
3	0.0013	0.0005	0.0016	1.298	2.678	0.618
4	0.0013	0.0005	0.0003	0.847	0.042	0.977

and

Table 23. Z_test of span n.2.

ID	Day 7–Day 9
	$∆\mathit{X}$ [m]	$∆\mathit{Y}$ [m]	$∆\mathit{Q}$ [m]	${\mathit{Z}}_{\raisebox{1ex}{$\mathit{\alpha }$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}\mathit{X}$	${\mathit{Z}}_{\raisebox{1ex}{$\mathit{\alpha }$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}\mathit{Y}$	${\mathit{Z}}_{\raisebox{1ex}{$\mathit{\alpha }$}\!\left/ \!\raisebox{-1ex}{$2$}\right.}\mathit{Q}$
1	0.0001	0.0000	0.0000	0.999	1.000	1.000
2	0.0006	0.0002	0.0000	0.859	0.988	1.000
3	0.0002	0.0001	0.0000	4.851	2.992	1.100
4	0.0003	0.0001	0.0000	0.988	0.251	1.000

).

For the Bayesian analysis, different statistical elaborations were considered depending on the a priori values of $\mu$ and ${\sigma }_0$ assigned (

Table 24. Bayesian analysis with prior data ($\mu$ = 0.005; ${\sigma }_0$ = 0.006) of span n.6.

ID	Day 2–Day 5
	$$\mathit{P}(\mathit{\delta }\mathit{h}\ne 0)|∆\mathit{X}$$	$$\mathit{P}(\mathit{\delta }\mathit{h}\ne 0)|∆\mathit{Y}$$	$$\mathit{P}(\mathit{\delta }\mathit{h}\ne 0)|∆\mathit{Q}$$
1	1.000	1.000	1.000
2	0.998	1.000	1.000
3	0.996	1.000	1.000
4	0.999	1.000	1.000

,

Table 25. Bayesian analysis with prior data ($\mu$ = 0.010; ${\sigma }_0$ = 0.006) of span n.6.

ID	Day 2–Day 5
	$$\mathit{P}(\mathit{\delta }\mathit{h}\ne 0)|∆\mathit{X}$$	$$\mathit{P}(\mathit{\delta }\mathit{h}\ne 0)|∆\mathit{Y}$$	$$\mathit{P}(\mathit{\delta }\mathit{h}\ne 0)|∆\mathit{Q}$$
1	1.000	1.000	1.000
2	0.995	1.000	1.000
3	0.993	0.999	1.000
4	0.998	1.000	1.000

,

Table 26. Bayesian analysis with prior data ($\mu$ = 0.008; ${\sigma }_0$ = 0.006) of span n.6.

ID	Day 2–Day 5
	$$\mathit{P}(\mathit{\delta }\mathit{h}\ne 0)|∆\mathit{X}$$	$$\mathit{P}(\mathit{\delta }\mathit{h}\ne 0)|∆\mathit{Y}$$	$$\mathit{P}(\mathit{\delta }\mathit{h}\ne 0)|∆\mathit{Q}$$
1	1.000	1.000	1.000
2	0.995	1.000	1.000
3	0.993	0.999	1.000
4	0.998	1.000	1.000

,

Table 27. Bayesian analysis with prior data ($\mu$ = 0.005; ${\sigma }_0=$0.006) of span n.2.

ID	Day 2–Day 5
	$$\mathit{P}(\mathit{\delta }\mathit{h}\ne 0)|∆\mathit{X}$$	$$\mathit{P}(\mathit{\delta }\mathit{h}\ne 0)|∆\mathit{Y}$$	$$\mathit{P}(\mathit{\delta }\mathit{h}\ne 0)|∆\mathit{Q}$$
1	1.000	1.000	1.000
2	0.998	1.000	1.000
3	0.996	1.000	1.000
4	0.999	1.000	1.000

,

Table 28. Bayesian analysis with prior data ($\mu$ = 0.010; ${\sigma }_0$ = 0.006) of span n.2.

ID	Day 2–Day 5
	$$\mathit{P}(\mathit{\delta }\mathit{h}\ne 0)|∆\mathit{X}$$	$$\mathit{P}(\mathit{\delta }\mathit{h}\ne 0)|∆\mathit{Y}$$	$$\mathit{P}(\mathit{\delta }\mathit{h}\ne 0)|∆\mathit{Q}$$
1	1.000	1.000	1.000
2	0.995	1.000	1.000
3	0.993	0.999	1.000
4	0.998	1.000	1.000

and

Table 29. Bayesian analysis with prior data ($\mu$ = 0.008; ${\sigma }_0$ = 0.006) of span n.2.

ID	Day 2–Day 5
	$$\mathit{P}(\mathit{\delta }\mathit{h}\ne 0)|∆\mathit{X}$$	$$\mathit{P}(\mathit{\delta }\mathit{h}\ne 0)|∆\mathit{Y}$$	$$\mathit{P}(\mathit{\delta }\mathit{h}\ne 0)|∆\mathit{Q}$$
1	1.000	1.000	1.000
2	0.995	1.000	1.000
3	0.993	0.999	1.000
4	0.998	1.000	1.000

).

5. Discussion

The analysis of the differences between the coordinates in the different measurement cycles confirmed the displacements intrinsic to the structure and the different phases of the loading/unloading conditions. In fact, the points taken into consideration, relative to each span and to the different piers, show dispersion values contained in the order of a few millimetres, as shown in Figure 6.

From the analysis in Figure 6, it was found that the differences between the different measurement cycles are in the order of a few millimetres. In fact, for span no. 2, 60% of the measurements are below one millimetre, and 40% show a difference of between 1 and 2 mm. On the other hand, for span no. 6, 23% of the measurements are below one millimetre, 36% show a difference of between 1 and 2 mm, 31% show a difference of between 2 and 3 mm, and the remaining 10% show values of more than 3 mm but less than 4 mm. As far as the reliability of the measurements is concerned, for an a priori estimate of the mean and the variance, respectively, equal to $\mu$ = 0.005 and ${\sigma }_0$ = 0.006, values close to 1 are obtained. Also, by varying the estimate of the mean and variance under worst-case assumptions, it can be seen that the statistics confirm the reliability of the results as the values obtained are, again, close to 1.

The estimates made indicate the reliability of the topographical differences obtained in the different measurement cycles relative to the analysis of the possible displacements of the bridge piers. This approach is necessary in order to validate the data, especially if the displacements can be compared with or exceed the accuracy of the topographic method adopted, which takes instrumental accuracy into account.

6. Conclusions

The proposed method, based on the use of TSs and the calculation of coordinates using the LS approach, made it possible to monitor the displacements of the structure taken into consideration under different loading conditions.

The measurement errors of each planimetric and altimetric compensation are less than one millimetre. In relation to the results obtained, no important displacements of the piles were verified under any loading and unloading conditions. To obtain further confirmation of the metric data acquired and processed, two different types of statistical approaches were conducted. For both approaches, the values obtained confirm the reliability of the results obtained and therefore support the hypothesis of the insignificant displacements and deformations of the analysed infrastructure.

To validate the results of monitoring for deformations and displacements, the statistical approach cannot be ignored, which must take into account the type of structure and any expected movements. Lastly, the paper described the topographical and statistical contribution to the verification of a bridge; this method can also be applied to other infrastructures (dams, buildings, slabs, etc.), guaranteeing the reliability of the results obtained.