Free Shrinkage Strains of Box Girders with Concrete Overlays
Abstract
1. Introduction
2. Materials and Methods
2.1. Geometry and Mesh Size
2.2. Humidity Profiles
2.2.1. Initial Drying Humidity Profile
2.2.2. Drying Humidity: Solution Method
2.3. Free Shrinkage Strains
3. Results
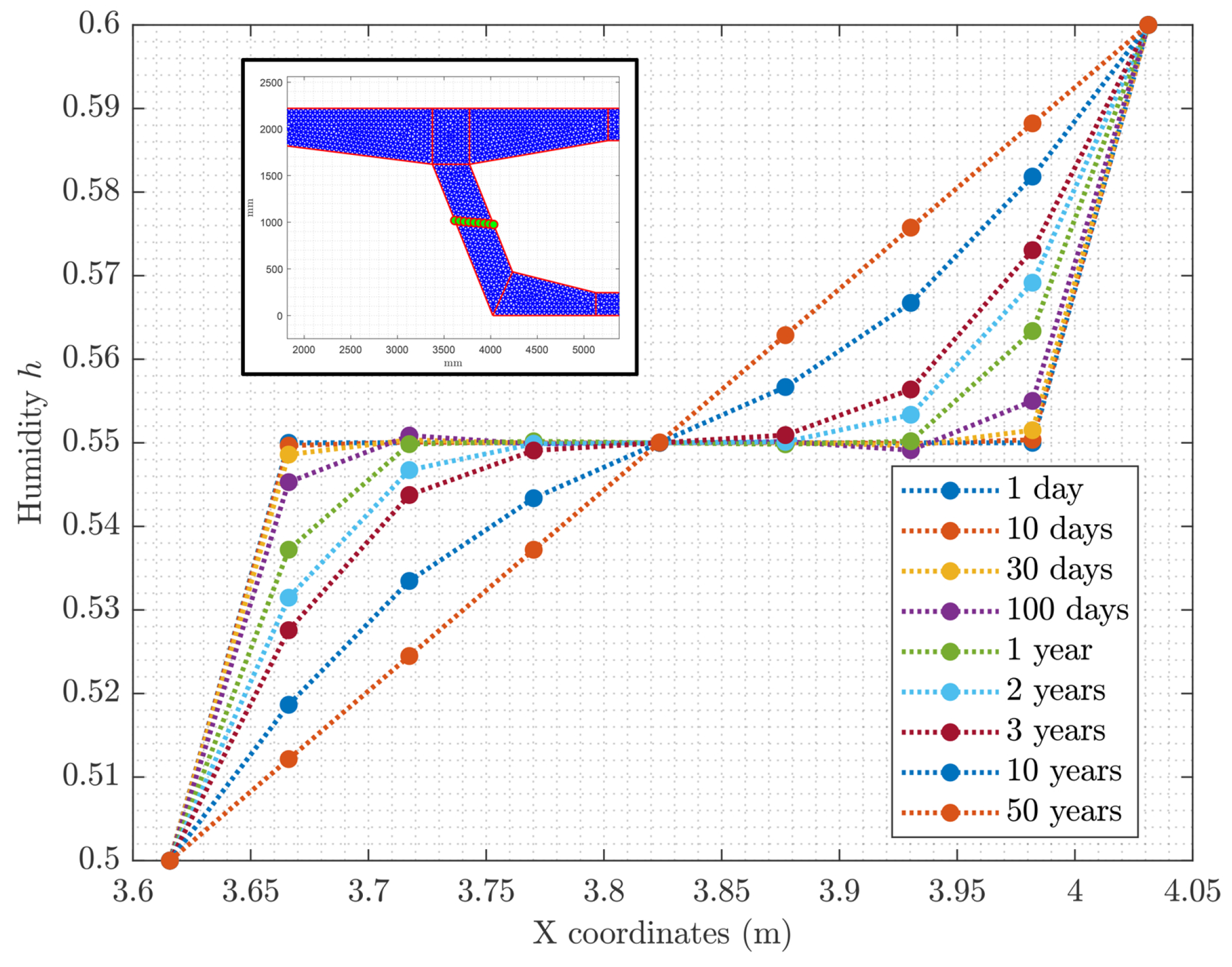
3.1. Humidity Profiles
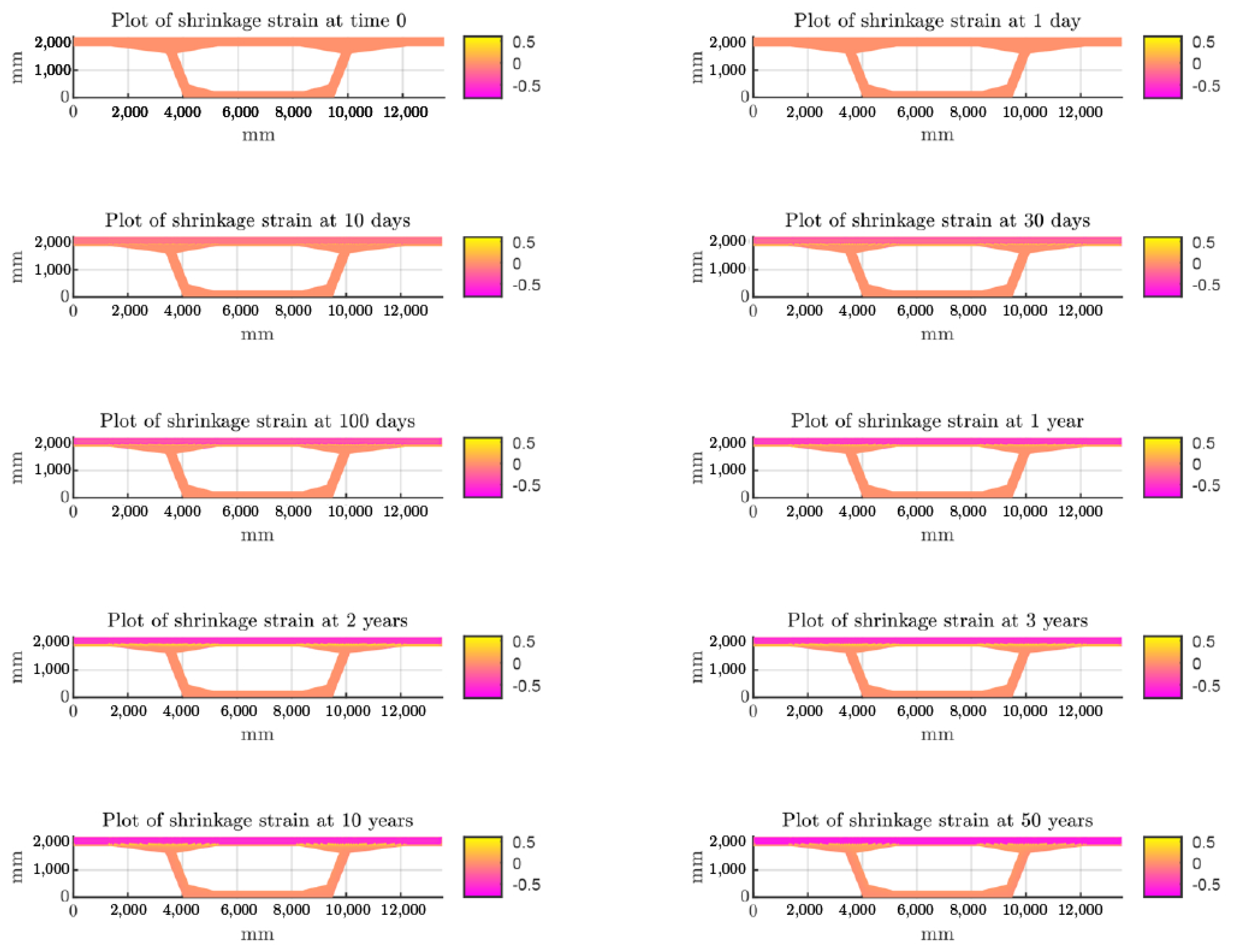
3.2. Free Shrinkage Strain Profiles
4. Discussion
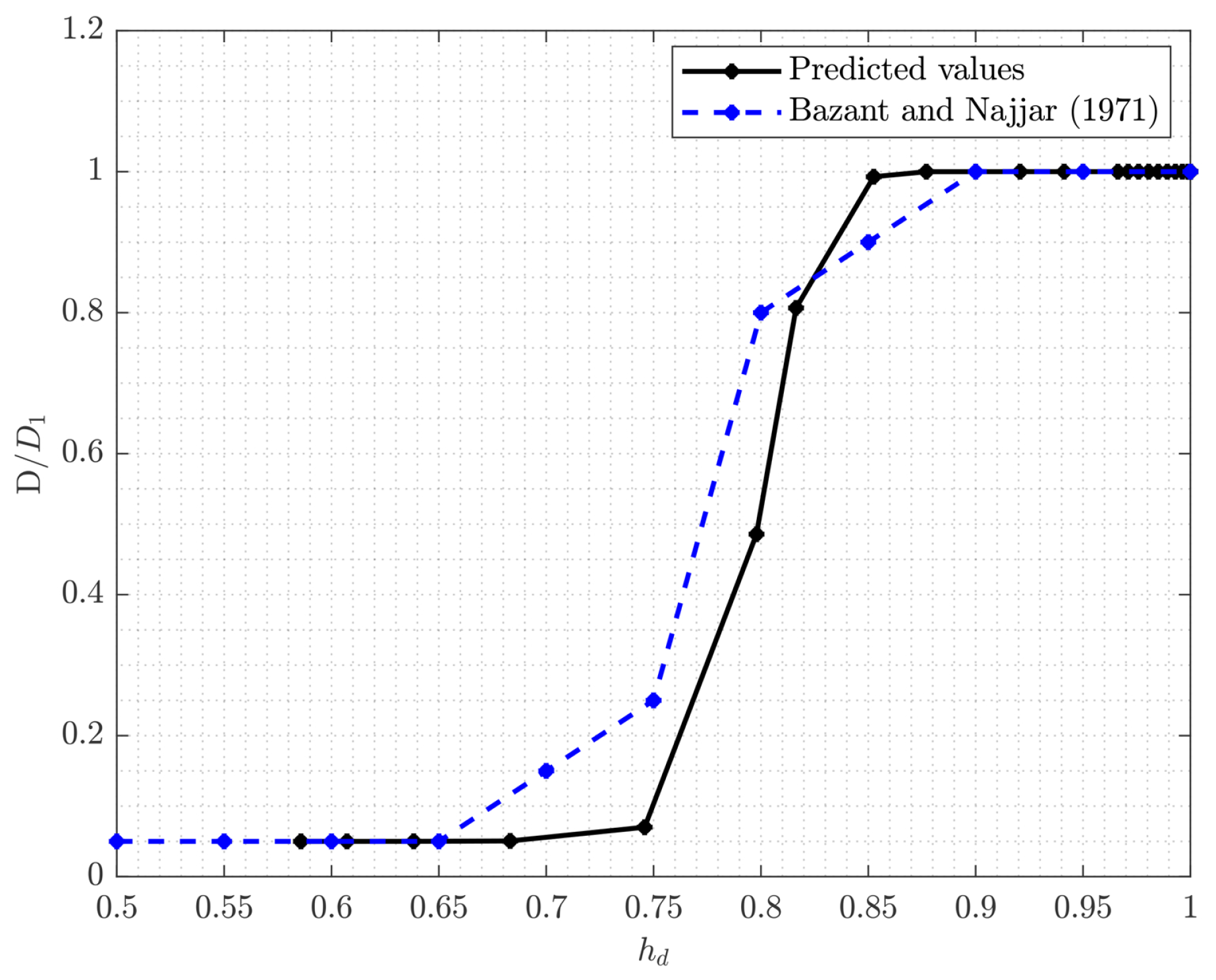
4.1. Humidity Profiles: Discussion of the Results
4.2. Free Shrinkage Strains: Discussion of the Results
5. Conclusions
- The humidity gradient between the overlay and the substrate generates differential volume changes between them. The substrate deformations are negligible while the overlay is subjected to high shrinkage strains.
- The internal humidity gradient must be considered because it influences the drying shrinkage and it may result in increased tensile stress, which may lead to early age cracking.
- The predicted free shrinkage strain values suggest that the overlay might be quite susceptible to cracking.
- The computation of shrinkage strains increases with time, and after 50 years, full equilibrium is reached; however, an analysis of about 10 years seems to be appropriate for practical applications and standard designs conducted in engineering firms, leading to a reduction in computational work for the prediction of stresses, probability of cracking, or displacements due to restrained shrinkage of new concrete overlays.
6. Future Work
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Lukovic, M.; Ye, G.; Van Breugel, K. Reliable concrete repair: A critical review. In Proceedings of the 14th International Conference Structural Faults and Repair, Edinburgh, UK, 3–5 July 2012. [Google Scholar]
- Federal Highway Administration. Bridge Condition by Highway System 2022. U.S. Department of Transportation. Available online: https://www.fhwa.dot.gov/bridge/nbi/no10/condition22.cfm (accessed on 15 May 2023).
- Parmiani, M.G.; Orta, L. Reliability Assessment of a Continuous Bridge Beam with Exposed Reinforcement. Eng. Struct. 2022, 262, 1–9. [Google Scholar] [CrossRef]
- Zhang, Y.; Chai, Y.H. Numerical analysis of bridge deck rehabilitation by ultra-high-performance concrete (UHPC) overlay. Structures 2021, 33, 4791–4802. [Google Scholar] [CrossRef]
- Gucunski, N.; Imani, A.; Romero, F.; Nazarian, S.; Yuan, D.; Wiggenhauser, H.; Shokouhi, P.; Taffe, A.; Kutrubes, D. Nondestructive Testing to Identify Concrete Bridge Deck Deterioration; Transportation Research Board: Washington, DC, USA, 2012. [Google Scholar]
- Silfwerbrand, J.L. 8—Bonded concrete overlays for repairing concrete structures. In Failure, Distress and Repair of Concrete Structures; Delatte, N., Ed.; Woodhead Publishing Series in Civil and Structural Engineering; Woodhead Publishing: Sawston, UK, 2009; pp. 208–243. [Google Scholar] [CrossRef]
- Beushausen, H.; Alexander, M.G. Failure mechanisms and tensile relaxation of bonded concrete overlays subjected to differential shrinkage. Cem. Concr. Res. 2006, 36, 1908–1914. [Google Scholar] [CrossRef]
- Orta, L.; Bartlett, F.M. Free shrinkage strains in concrete overlays. ACI Mater. J. 2014, 111, 263–271. [Google Scholar] [CrossRef]
- Delatte, N.J.; Scott Williamson, M.; Fowler, D.W. Bond strength development with maturity of high-earlystrength bonded concrete overlays. ACI Struct. J. 2000, 97, 201–207. [Google Scholar] [CrossRef]
- Lange, D.A.; Shin, H.C. Early age stresses and debonding in bonded concrete overlays. Transp. Res. Rec. 2001, 1778, 174–181. [Google Scholar] [CrossRef]
- Beushausen, H.; Alexander, M.G. Localised strain and stress in bonded concrete overlays subjected to differential shrinkage. Mater. Struct. Mater. Constr. 2007, 40, 189–199. [Google Scholar] [CrossRef]
- Orta, L.; Bartlett, F.M. Stresses due to restrained shrinkage in concrete deck overlays. ACI Mater. J. 2014, 111, 701–710. [Google Scholar] [CrossRef]
- Tang, F. Overlay for Concrete Segmental Box-Girder Bridges. J. Bridge Eng. 2000, 5, 311–321. [Google Scholar] [CrossRef]
- Zhang, Y.; Chai, Y.H. An Analytical Model to Evaluate Short- and Long-Term Performances of Post-Tensioned Concrete Box-Girder Bridges Rehabilitated by an Ultrahigh-Performance Concrete Overlay. J. Bridge Eng. 2023, 28. [Google Scholar] [CrossRef]
- AASHTO. Segmental Box Girder Standards for Span-by-Span and Balanced Cantilever Construction; Technical Report, AASHTO-PCI-ASBI; American Association of State Highway and Transportation Officials: Washington, DC, USA, 1997. [Google Scholar]
- Parmiani, M.G. Evaluation of Aging and Repaired Reinforced Concrete Structures. Ph.D. Thesis, Tecnologico de Monterrey, Monterrey, Mexico, 2023. [Google Scholar]
- Orta, L.; Bartlett, F.M. Sensitivity analysis of restrained shrinkage stresses of concrete deck overlays. Eng. Struct. 2020, 210, 110396. [Google Scholar] [CrossRef]
- Neville, A. Properties of Concrete, 5th ed.; Pearson: London, UK, 2012. [Google Scholar]
- Tazawa, E.I. (Ed.) Autogenous Shrinkage of Concrete: Proceedings of the International Workshop Organized by JCI, Hiroshima June 13–14, 1998; Taylor & Francis: Oxfordshire, UK, 1999; pp. 1–411. [Google Scholar]
- Bažant, Z.P.; Najjar, L.J. Drying of concrete as a nonlinear diffusion problem. Cem. Concr. Res. 1971, 1, 461–473. [Google Scholar] [CrossRef]
- CEB-FIP. CEB-FIP Model Code 1990: Design Code; Thomas Telford: Lausanne, Switzerland, 1993. [Google Scholar]
- Zhang, J.; Dongwei, H.; Wei, S. Experimental study on the relationship between shrinkage and interior humidity of concrete at early age. Mag. Concr. Res. 2010, 62, 191–199. [Google Scholar] [CrossRef]
- Bažant, Z.P.; Křístek, V.; Vitek, J.L. Drying and Cracking Effects in Box-Girder Bridge Segment. J. Struct. Eng. 1992, 118, 305–321. [Google Scholar] [CrossRef]
- Chandrupatla, T.; Belegundu, A. Introduction to Finite Elements in Engineering, 4th ed.; Pearson: Upper Saddle River, NJ, USA, 2012. [Google Scholar]
- Hughes, T. Unconditionally stable algorithms for nonlinear heat conduction. Comput. Methods Appl. Mech. Eng. 1977, 10, 135–139. [Google Scholar] [CrossRef]
- Bazant, Z.P.; Chern, J.C. Concrete creep at variable humidity: Constitutive law and mechanism. Mater. Constr. 1985, 18, 1–20. [Google Scholar] [CrossRef]
- Bazant, Z.; Baweja, S. Creep and shrinkage prediction model for analysis and design of concrete structures: Model 83. In Adam Neville Simposium: Creep and Shrinkage—Structural Design Effects; Al-Manaseer, A., Ed.; American Concrete Institute: Farmington Hills, MI, USA, 2000; pp. 1–83. [Google Scholar]
- ACI Committee 318. Building Code Requirements for Structural Concrete; American Concrete Institute: Farmington Hills, MI, USA, 2019. [Google Scholar]
- Wu, L.; Farzadnia, N.; Shi, C.; Zhang, Z.; Wang, H. Autogenous shrinkage of high performance concrete: A review. Constr. Build. Mater. 2017, 149, 62–75. [Google Scholar] [CrossRef]
- Grasley, Z.C.; Lange, D.A. Modeling drying shrinkage stress gradients in concrete. Cem. Concr. Aggreg. 2004, 26, 1–13. [Google Scholar] [CrossRef]





Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Parmiani, M.G.; Orta, L. Free Shrinkage Strains of Box Girders with Concrete Overlays. Infrastructures 2023, 8, 96. https://doi.org/10.3390/infrastructures8050096
Parmiani MG, Orta L. Free Shrinkage Strains of Box Girders with Concrete Overlays. Infrastructures. 2023; 8(5):96. https://doi.org/10.3390/infrastructures8050096
Chicago/Turabian StyleParmiani, Maria Giulia, and Luis Orta. 2023. "Free Shrinkage Strains of Box Girders with Concrete Overlays" Infrastructures 8, no. 5: 96. https://doi.org/10.3390/infrastructures8050096
APA StyleParmiani, M. G., & Orta, L. (2023). Free Shrinkage Strains of Box Girders with Concrete Overlays. Infrastructures, 8(5), 96. https://doi.org/10.3390/infrastructures8050096





