Dynamic Sustainable Processes Simulation to Study Transport Object Efficiency
Abstract
:1. Introduction
- -
- Using the elastic wave theory makes it possible to overcome five main drawbacks of the elasticity theory;
- -
- Opportunity to describe any process of interaction as a chain of processes, incidence-reflection-refraction of force impulses;
- -
- The wording of the basic law of mechanics and the description of the characteristics of the impulse of action have been expanded.
2. Materials and Methods
- -
- -
- Determination of wave propagation velocity, Formula (1);
- -
- Dependences for determining the magnitude of wave amplitudes, Formulas (2)–(7);
- -
- Record of Newton’s second law for the relationship between external and internal forces, Formula (8).where —the Young modulus or the modulus of elasticity;—Poisson’s ratio;—are the transverse and longitudinal wave propagation speeds in a particular medium.
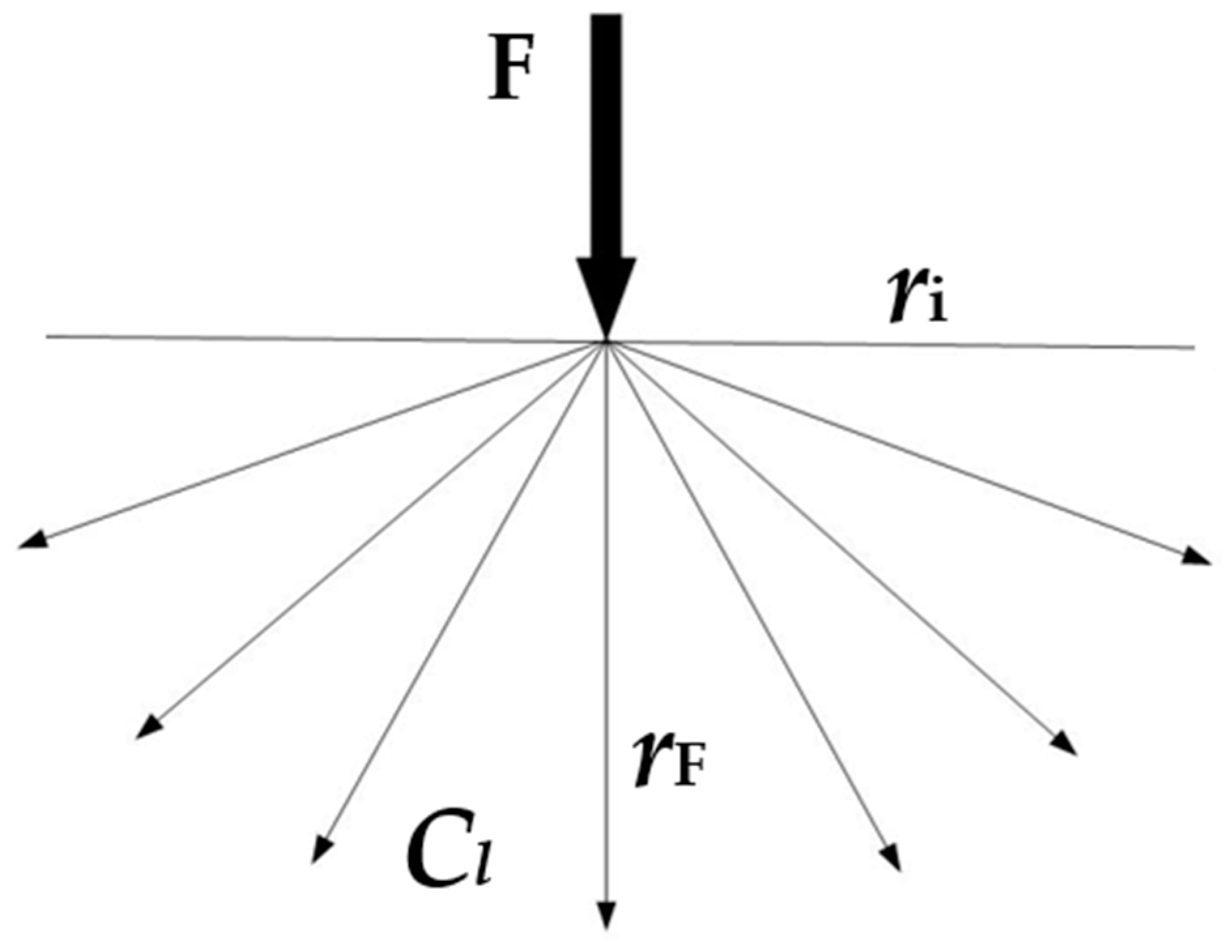
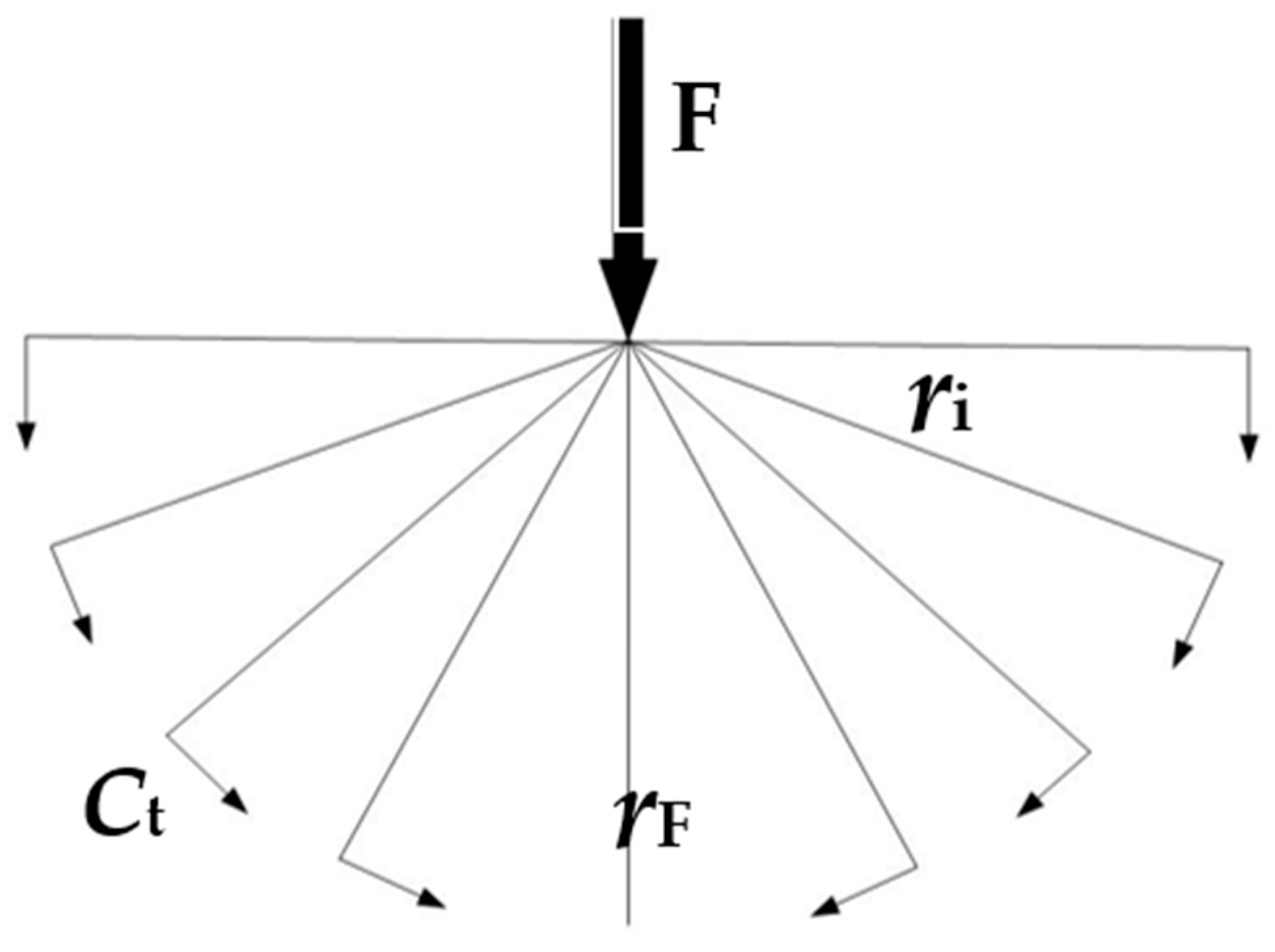
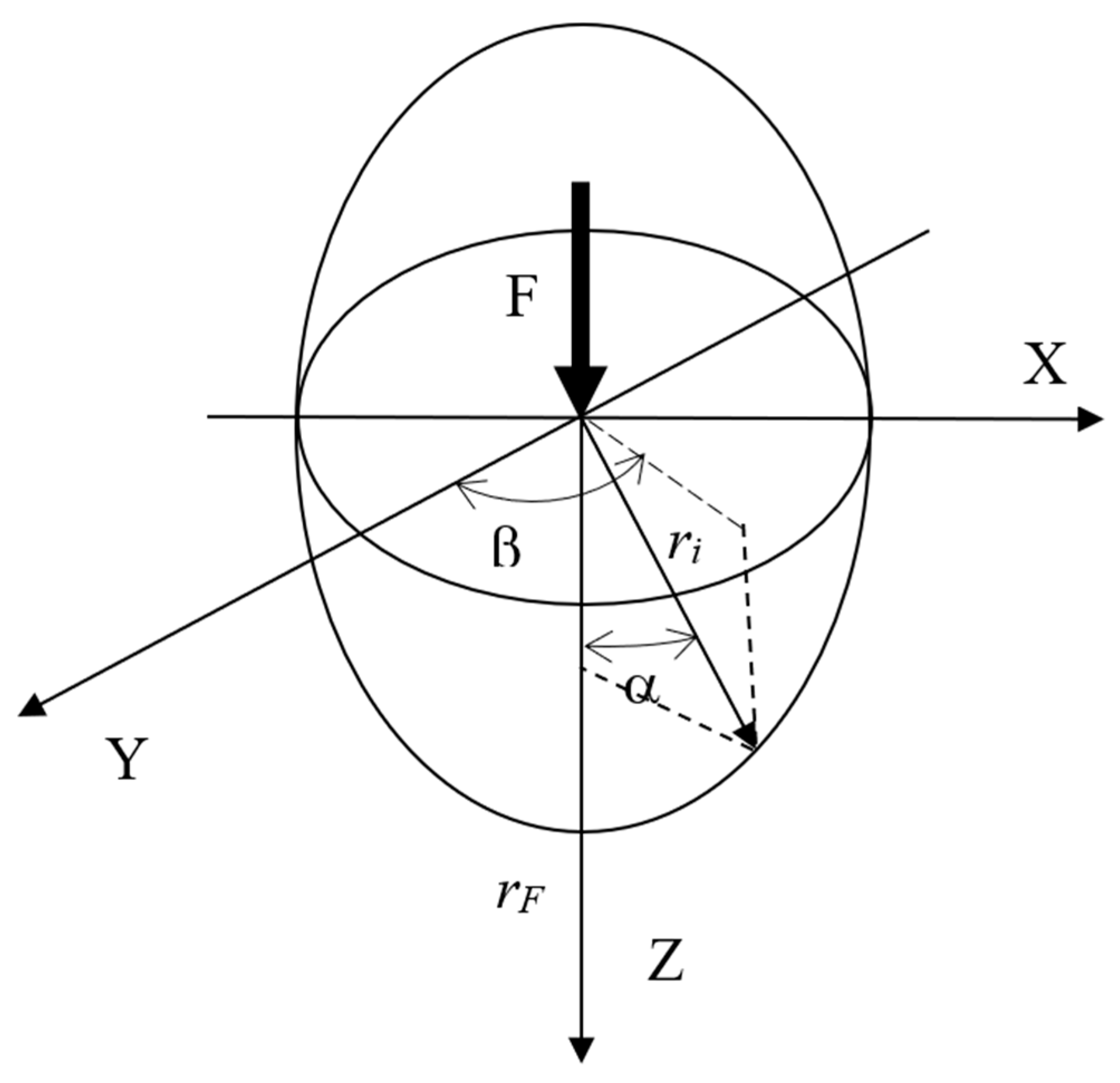
3. Results
3.1. Expansion of the Capabilities of the Mathematical Apparatus to Describe the Dynamics of the Process in Determining the Issues of Facility Efficiency
- The main limitation of the elasticity theory is the absence of a time component. There are no dependencies in the timescale that connect the duration of the action of force, deformation, or stress. The laws of elastic wave propagation over time in different mediums and during the transition from one medium to another completely negate this limitation.
- In the theory of stresses, internal forces are studied that arise in solid deformable materials or objects as a result of physical influences on them. With an external physical impact, the distances between the internal points of the material (material particles) change, resulting in internal forces that represent the macroscopic interaction between atoms or molecules. The method of sections and the connection axiom are used to describe internal forces in stress theory. Internal forces can change during the transition from one particle to another; therefore, the stress state in the object is generally inhomogeneous (as well as the deformed state). However, deformations can be measured, but stresses cannot because deformations are physically measured to measure stresses. Consequently, the stress theory is axiomatic, and stress is an artificial measure of the internal forces arising in the object. By their physical nature, stresses are pressures caused by a force field inside an object. The calculated amplitudes of the oscillations of the particles under the influence of force waves allow for determining the deformations at the points of the sensor established. Thus, it is possible to directly correlate the quantities measured in the experiment and actually occurring in the objects of measurement. Understanding the dynamic processes within an object allows the selection of equipment for recording specific types of pulses and interpreting and analyzing the recorded signals in terms of noise.
- In the theory of deformations, the kinematics of a continuous medium is considered regardless of physical influences. Stress theory studies the internal forces generated in the object or in the material as a result of physical pressure. Neither the deformation theory nor the stress theory took into account the specific properties of the material to describe the process of propagation of force influences within objects. Even with numerical modeling methods such as finite element methods, the partitioning of the inner mesh is independent of the material properties. The laws of elastic wave propagation over time in various mediums allow considering changes occurring inside objects under the influence of force fields over time. This makes it possible to modify the object’s geometric and elastic characteristics to specific operating conditions, taking into account the deformability processes within the objects.
- The behavior of specific materials and structures of objects, as well as the relationships between them, under different influences, are given to the studied models by defining ratios. In order to construct defining ratios, experiments are carried out on the physical influence on samples of objects. From the processing of these experiments, a specific type of dependency and the values of the constants of the constituent dependencies are set. Such experiments are called set-up experiments, and dependency constants are called material constants. Both experiments and constants are applicable only for the conditions under consideration and present actual physical processes in separate correlated aspects, the physical nature of which is not taken into account. The laws of elastic wave propagation are universal because they eliminate the following limitations:
- Applications of different mathematical apparatus for problems of mechanics in Newtonian, relativistic, and ultra-relativistic areas. It is believed that momentum increases only by increasing velocity in Newtonian mechanics, as the change in mass can be ignored. In the ultra-relativistic area, the object’s velocity is practically unchanged, and the momentum increases only due to the increase in mass. It is considered in the relativistic area that the increase in momentum is due to an increase in both the velocity and mass. In this case, the speed increases more slowly than in Newtonian mechanics, precisely because of the increase in the mass of the object being accelerated. Elastic wave theory allows the consideration of momentum propagation problems in any mechanics area (see Formula (8)), as the time accounting for the propagation of force waves in an object, automatically considers the change in mass over time and changes in the velocity of the deformation process within the object in time.
- The need to divide the energy spent on the dynamics of the process into types, as well as the concepts that characterize the dynamics of the process into types. For example, the process of moving an object in mechanics is characterized by the following two types of energy: kinetic and potential. The first describes the movement process in terms of velocity change and the second in terms of coordinate changes. This artificial separation of the energy used in the movement process is related to the history of technical calculations, which led to the existence of several systems of measures at a certain stage, as follows: physical and technical. In the physical system of measures, the basic unit of measurement is the mass, and force is a derivative unit, the value of which is obtained by multiplying the basic unit of mass by the dimension of the acceleration. In a technical system of measures, mass is a derivative of the unit obtained by dividing the basic unit of force by the acceleration dimension. However, since any amount of force involved in the experiment is easier to attach or measure than to determine the mass involved in the experiment in dynamic processes, the technical calculations and logic of their development are invisibly based on the technical system of measurement, where equivalent/effective mass values are used.
- 5.
- The theory of elasticity perfectly describes the material of objects using the concept of a material continuum but completely excludes the possibility of studying the behavior of internal forces. Knowledge of internal force change mechanisms is used, for example, to create high- or low-damping materials for mechanical applications in various fields of technology. In addition, this knowledge will help select the charge for a specific type of wall in explosive wall breaking, which is an important operation used by firefighters or special forces as a method of gaining immediate access to a structure in an emergency as a direct replacement for conventional breaking methods. Even when studying the internal state in the elasticity of anisotropic materials, the macroscopic behavior of materials is considered, that is, the atomic or molecular structure is not considered. In the proposed method, local volumes and material density, which vary over time due to force waves, must be taken into account when calculating the amplitude. Therefore, it is necessary to take into account the influence of a change in the distance between particles on the interaction forces of particles in order to determine the probability of the occurrence of irreversible deformation or the impossibility of deformation, as well as the probability of a defect and a change in the structure of the material. Thus, the application of the first law of thermodynamics and the extended use of material properties in the calculation makes it possible to develop modeling in this direction, linking the macro- and microscopic behavior of materials.
3.2. Influence of Characteristics of an Impulse on the Dynamics of the Process in the Study of Facility Efficiency
- In the proposed method, impulses are considered as a measure of any action of different physical quantities on an object over time at which there is an exchange of energy over time. The action of the impulse on the object has clear time characteristics of beginning and end of action, that is, duration of action. This makes it possible to determine the additional characteristics of the pulse as follows: at the time of transmission of the pulse. Therefore, the influence of any physical quantity, when cyclically acting in a dynamic process, is characterized by the following two types of frequencies:
- 3.
- The frequency (or frequencies) of pulse transmission acting on a specific contact site. It is characterized by its action duration;
- 4.
- The frequency of pulse repetition (repetition rate) in a certain cross-section. It is characterized by the time through which the repetition of the action occurs.
This provides a clear separation between single and repetitive impulses, leading to increased simulation capabilities. For example, when considering the impact on the rail of a rolling stock wheel (force pulse) moving at a constant speed, the same rail cross-section is subjected to different force pulses from different wheels because each wheel has different impact characteristics at the time of passage, namely, the following:- 5.
- The amplitude of the force impulse is determined as it is an oscillation superposition of the wagon, bogie, damping system, and railway track structure state;
- 6.
- The direction of the pulse depends on the position of the bogie relative to the railway track axis;
- 7.
- The number, area, and location of rail/wheel contacts depend on the position of the wheelset relative to the track axis.
- 2.
- Since there is an energy exchange that occurs during the action of an impulse, one of its characteristics is the law of change of the acting physical quantity over time. This value characterizes the intensity of the effect of the force impulse per unit time during the force action. For example, according to Hooke’s law, the force acting on an object causes directly proportional deformations or displacements. When a force acts on an object, the latter will do work equal to the product of the force and the displacement. The action of a constant force per unit time on an object, regardless of the time of its impact, is characterized by the same value of the amount of motion per unit of time, which serves as a potential for performing the same amount of work on the object per unit of time. This means that it transfers the same amount of energy per unit time during the action of the force. So, impulses of constant forces transfer a constant amount of energy per unit of time and force the object to have a permanent deformation under their influence, that is, behave according to the laws of statics from the moment equilibrium is established. Or, if the object does not interact with other objects, it will move in a straight line and uniformly with the speed established in this medium. If the force has a variable value over time, then the impulses of variable forces cause the exchange of a variable amount of energy per unit of time during the duration of the force impulse. When describing impacts, the amplitude of the force impulse characterizes the impact force in mechanical systems or loudness in music. Additionally, the intensity of the impact allows you to describe such characteristics of the impact as “legato”, “staccato” and sound density in music, or “soft”, “hard/sharp” impact, and flow density per unit time in mechanical systems.
- 3.
- Unlike modern equipment, previously used instruments used in the study of oscillations were very inertial. Such instruments respond only to a change in the average energy value over a period of time significantly exceeding the oscillation period. In this case, knowledge of the spectrum was sufficient, as it could be used to determine the energy of each harmonic and thus determine the average energy of the total oscillation. This is why spectral decomposition plays an extremely important role in the study of oscillations. However, knowledge of the spectrum of some non-sinusoidal oscillations makes it impossible to determine the shape of this oscillation and build its graph. In addition, the energies of harmonics depend only on the amplitude and frequency, and do not depend on the phase of the oscillations. When recording vibrations, it is impossible to divide them into the incident and reflected processes, so it is necessary to understand the energy of which the oscillating process is recorded. An introduction to the calculation of the time characteristic allows evaluating what oscillatory processes were recorded at the time of recording.
- 4.
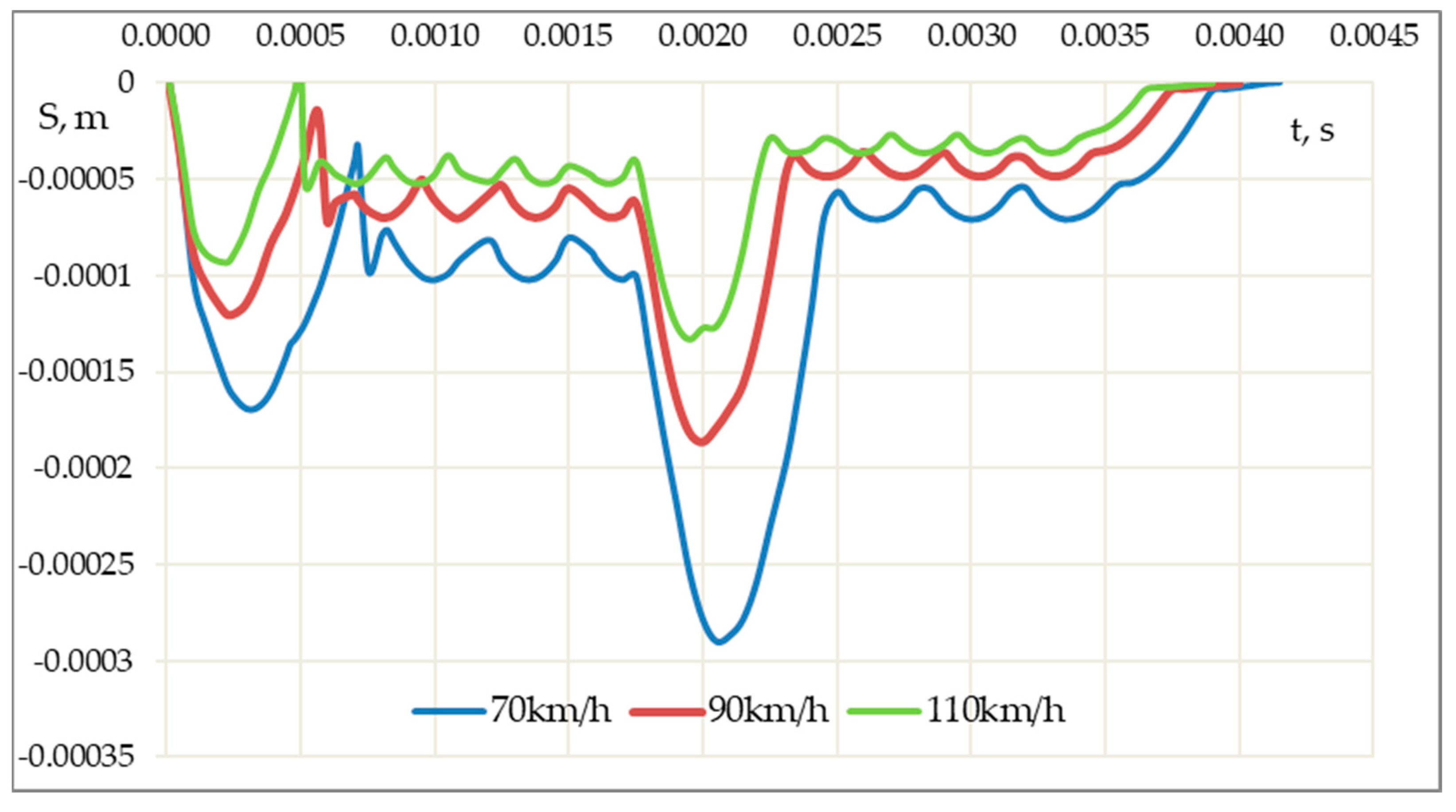
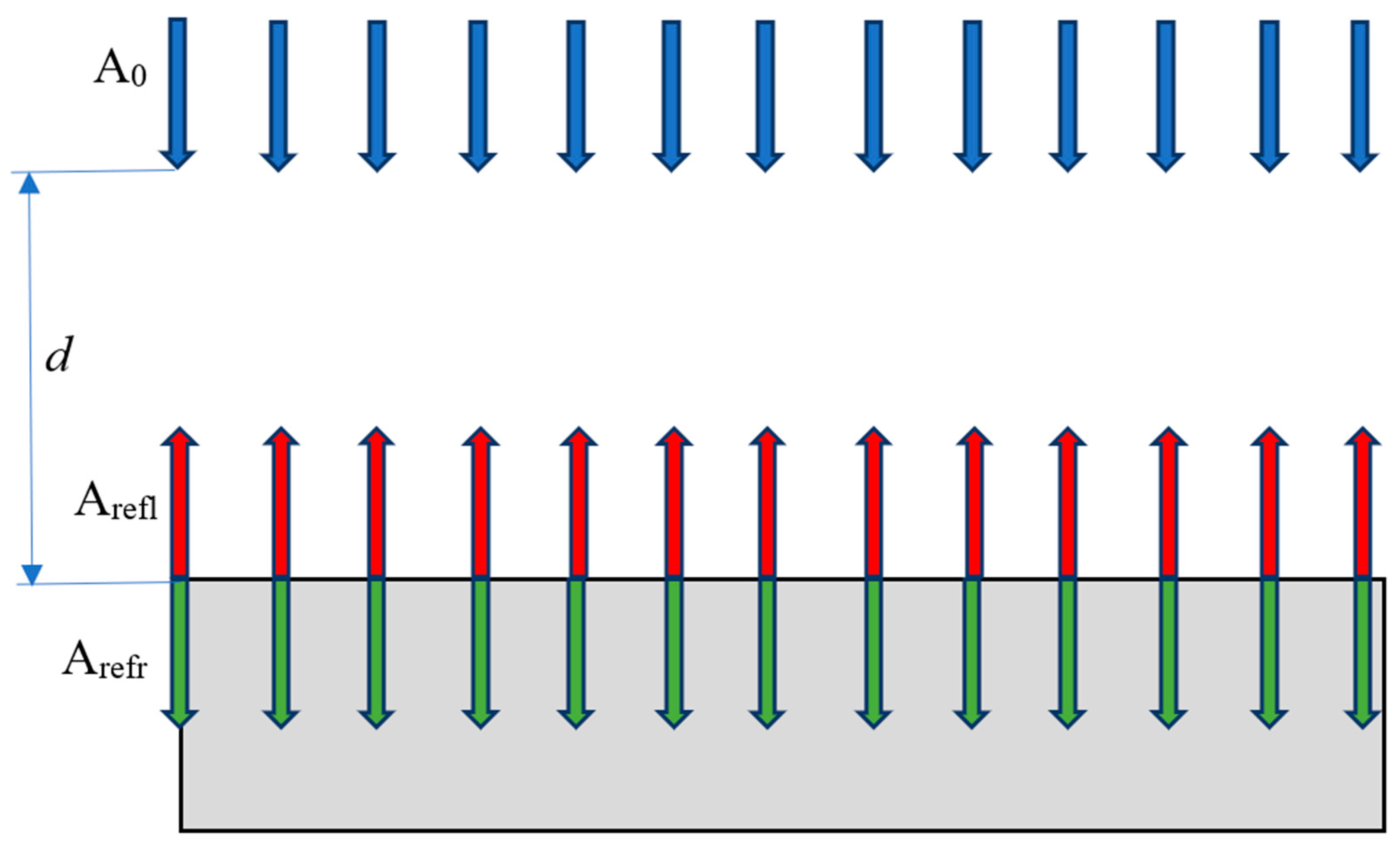
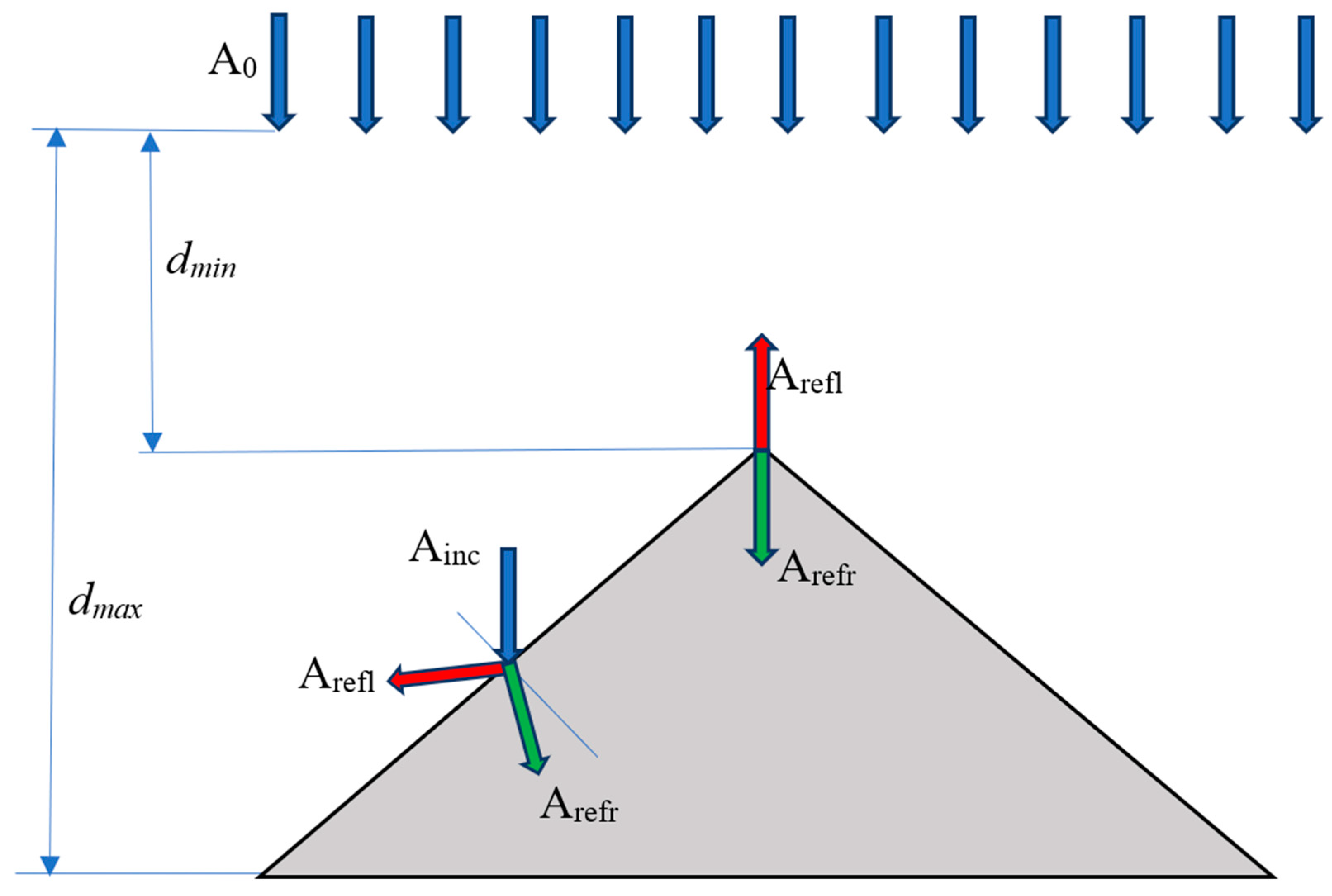
- The introduction of the time component allows describing and comparing changes in the values of different vectors in time and space. Additionally, the use of the properties of elastic longitudinal and transverse waves makes it possible to consider changes in the values of vectors without observing mechanical equilibrium. This enables considering the change in impulses depending on specific conditions, which expands the possibilities of applying an impulse not only to a specific object but also outside the object. So, for example, suppose that the initial impulse of force was a uniformly distributed pressure moving through the air by the propagation of longitudinal waves from a source located at a distance . Figure 6 shows an example of changing the parameters of the impact of the primary force impulse on a rectangular object located perpendicular to the incident flow of the force impulse. Upon reaching the object, the amplitude of the incident waves will be equal to . The values of the amplitudes of reflection and refraction will be calculated using Formulas (2) and (3). It is supposed that the object is a solid shell with air inside. Since the force impulse falls perpendicular to the incident surface, the reflection and refraction waves will have equal amplitudes over the entire loaded surface of the object. Thus, the law of impact of a force impulse applied to an object remained completely identical to the original one in form but with a smaller impact.
- 5.
- There are the following three types of deformability:
- Deformability of manerial—the ability of a material to respond to impulses from physical factors;
- Deformability of elements—the ability of elements, as products from a certain material with a certain geometry, to react to mechanical impulses;
- Deformability of the structure—total deformability of its elements, in which the elements of the structure can not only change shape, dimensions, and volume but also move due to deformability of other elements that make up the structure.
4. Discussion, Conclusions and Future Recommendation
- The description of physical processes in the most approximate way to the course of natural phenomena. For this purpose, the time component is taken into account when describing the transmission of impulses by elastic waves. This description of the dynamics of the process regulates the pattern of natural phenomena, the sequence of which determines the cause-and-effect relationship, giving an answer to the question of what consequences will arise from the influence of physical impulses in the future. Without knowledge of the mechanism of cause-and-effect relationships, it is impossible to determine risk in a universal way. A person, in many cases, assesses the risks of various actions subconsciously. If we look at a person’s ordinary life, we will see that he automatically uses the importance of taking into account the duration of exposure in his life. For example, someone decided to make a new dish. In preparation for the realization of his wish, he is sure to learn the duration of necessary operations when cooking, for instance, meat, as follows: the duration of marinating in a particular sauce, the duration of baking or extinguishing a dish of a particular type of meat, set weight and under certain temperature and/or power conditions. Or if someone decides to dye fabric or remove a stain on clothes at home, he will certainly be aware of exactly what (reagent) and what (type of fabric) will be affected and how long its effect is needed. When someone chooses shoes for a hike, he necessarily decides for himself how much he is willing to pay for the comfortable and guaranteed performance of these shoes for the duration of the hike (a certain period of time) under the specific operating conditions of the shoes. A person, in many cases, assesses the risks of various actions regardless of paying attention.
- 2.
- Ensuring the functional security of the technosphere objects of the human habitat. Throughout its existence, mankind has been constantly creating and modifying artificial technological and technical objects to meet its socio-economic needs both in the engineering field (factories, transport infrastructure, mechanisms, etc.) and in everyday life (vehicles, household appliances, medical equipment, etc.). The operation of the technosphere objects in the human habitat affects both the ecological envelope of the Earth and the health of the person himself.The wave process can have a variety of physical natures as follows: mechanical, chemical, electromagnetic, gravitational, spin, probability density, etc. Therefore, a universal tool is needed to study the influence of technosphere objects on both people’s lives and the environment. The proposed method uses universal properties and laws of elastic wave propagation for different materials and object geometries. This allows analytical simulation of the behavior of the object under the influence of various impulses. Development of this direction in the future will allow answering such questions as the following:
- What actual changes in the characteristics of the object itself can occur during the period of operation;
- What maintenance is required by the facility under any operating conditions;
- Whether the object is able to safely perform the intended functions for the total period of operation;
- Whether the object is able to give the required level of economic efficiency of use, that is, whether the object is competitive in the market.
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Erhova, N.; Bondarenko, I.; Shibko, O.; Velmagina, N. Development of the procedure for verifying the feasibility of designing an active suspension system for transport carriages. East.-Eur. J. Enterp. Technol. 2018, 3, 53–63. [Google Scholar] [CrossRef]
- Kovalchuk, V.; Luchko, J.; Bondarenko, I.; Markul, R.; Parneta, B. Research and analysis of the stressed-strained state of metal corrugated structures of railroad tracks. East.-Eur. J. Enterp. Technol. 2016, 6, 4–9. [Google Scholar] [CrossRef]
- Magalhães, K.M.M.; Brasil, R.M.L.R.F.; Wahrhaftig, A.M.; Siqueira, G.H.; Bondarenko, I.; Neduzha, L. Influence of Atmospheric Humidity on the Critical Buckling Load of Reinforced Concrete Columns. Int. J. Struct. Stab. Dyn. 2022, 22, 2250011. [Google Scholar] [CrossRef]
- Radulović, S.; Milković, D.; Raković, M.; Simić, G.; Kostić, A. Influence of the head wind on determining brake performance of Zacns tank wagon. Acta Polytech. Hung. 2022, 19, 81–98. [Google Scholar]
- Goolak, S.; Gubarevych, O.; Yermolenko, E.; Slobodyanyuk, M.; Gorobchenko, O. Mathematical modeling of an induction motor for vehicles. East. -Eur. J. Enterp. Technol. 2020, 2, 25–34. [Google Scholar] [CrossRef]
- Xu, L.; Zhao, Y.; Li, Z.; Shi, C.; Yu, Z. Three-dimensional vehicle-ballasted track-subgrade interaction: Model construction and numerical analysis. Appl. Math. Model. 2020, 86, 424–445. [Google Scholar] [CrossRef]
- Fan, D.; Sebès, M.; Qazi, A.; Bourgeois, S.; Chollet, H.; Pozzolini, C. A new dynamic vehicle/track interaction method with a finite element track model. In Proceedings of the Second International Conference on Rail Transportation (ICRT), Chengdu, China, 5–6 July 2021. [Google Scholar]
- Xu, L.; Zhai, W. Vehicle–track–tunnel dynamic interaction: A finite/infinite element modelling method. Railw. Eng. Sci. 2021, 29, 109–126. [Google Scholar] [CrossRef]
- Meacci, M.; Meli, E.; Pugi, L.; Rindi, A. Development and Validation of a Vertical Vehicle-Track Interaction Model. In Proceedings of the First International Conference on Rail Transportation, Chengdu, China, 10–12 July 2017. [Google Scholar] [CrossRef]
- Kepka, M.; Václavík, J.; Chvojan, J. Fatigue life of a bus structure in normal operation and in accelerated testing on special tracks. Procedia Struct. Integr. 2019, 17, 44–50. [Google Scholar] [CrossRef]
- Zeng, Z.; Xiao, Y.; Wang, W.; Huang, X.; Du, X.; Liu, L.; Victor, J.; Xie, Z.; Yuan, Y.; Wang, J. The Influence of Track Structure Parameters on the Dynamic Response Sensitivity of Heavy Haul Train-LVT System. Appl. Sci. 2021, 11, 11830. [Google Scholar] [CrossRef]
- Escalona, J.L.; Aceituno, J.F.; Urda, P.; Balling, O. Railway multibody simulation with the knife-edge-equivalent wheel–rail constraint equations. Multibody Syst. Dyn. 2020, 48, 373–402. [Google Scholar] [CrossRef]
- Spiroiu, M.A.; Camil, C. Wheel flat effect on wheel-rail dynamic interaction. In IOP Conference Series Materials Science and Engineering; IOP Publishing: Bristol, UK, 2018; Volume 444, p. 042002. [Google Scholar] [CrossRef]
- Guo, Y.; Shi, C.; Zhao, C.; Markine, V.; Jing, G. Numerical Analysis of Train-Track-Subgrade Dynamic Performance with Tire-Derived Aggregate in Ballast Layer. Constr. Build. Mater. 2022, 336, 127599. [Google Scholar] [CrossRef]
- Qi, Y.; Indraratna, B.; Ngo, T.; Ferreira, F.B. Advancements in Geo-Inclusions for Ballasted Track: Constitutive Modelling and Numerical Analysis. Sustainability 2021, 13, 9048. [Google Scholar] [CrossRef]
- Bruner, M.; Cortis, D.; Malavasi, G. Rail Strain under Different Loads and Conditions as a Source of Information for Operation. In Proceedings of the Third International Conference on Railway Technology: Research, Development and Maintenance, Cagliari, Italy, 5–8 April 2016. [Google Scholar] [CrossRef]
- Rabbi, M.F.; Johnson, D.K.; Mishra, D.; Bruzek, R. Effect of Track Configuration and Loading Conditions on Vertical Wheel Load Measurements using the Differential Shear Approach. Transp. Res. Rec. 2019, 2673, 279–288. [Google Scholar] [CrossRef]
- Idczak, W.; Lewandrowski, T.; Pokropski, D.; Rudnicki, T.; Trzmiel, J. Dynamic Impact of a Rail Vehicle on a Rail Infrastructure with Particular Focus on the Phenomenon of Threshold Effect. Energies 2022, 15, 2119. [Google Scholar] [CrossRef]
- Mirković, N.; Brajović, L.; Popović, Z.; Todorović, G.; Lazarević, L.; Petrović, M. Determination of temperature stresses in CWR based on measured rail surface temperatures. Constr. Build. Mater. 2021, 284, 122713. [Google Scholar] [CrossRef]
- Weston, P.; Roberts, C.; Yeo, G.; Stewart, E. Perspectives on railway track geometry condition monitoring from in-service railway vehicles. Veh. Syst. Dyn. 2015, 53, 1063–1091. [Google Scholar] [CrossRef]
- Jiang, J.Z.; Matamoros, A.; Zolotas, A.; Goodall, R.M.; Smith, M.C. Passive suspensions for ride quality improvement of two-axle railway vehicles. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2013, 229, 315–329. [Google Scholar] [CrossRef]
- Jukowski, M.; Śledziewski, K.; Hypki, M. Assessment of the impact of vibrations on the track surfacefrom impact loads. In Proceedings of the MATEC Web of Conferences, Moscow, Russia, 4–7 August 2019; Volume 252, p. 01007. [Google Scholar] [CrossRef] [Green Version]
- Naeimi, M.; Zakeri, J.A.; Shadfar, M.; Esmaeili, M. 3D dynamic model of the railway wagon to obtain the wheel-rail forces under track irregularities. Proc. Inst. Mech. Eng. Part K J. Multi-Body Dyn. 2015, 229, 357–369. [Google Scholar] [CrossRef]
- Drozdziel, P.G.; Stastniak, P.; Smetanka, L. Computer aided simulation analysis for wear investigation of railway wheel running surface. Diagnostyka 2019, 20, 63–68. [Google Scholar] [CrossRef]
- Konowrocki, R.; Walczak, S. Influence of Flexibility Parameters of Wheels and Wheelset on the Railway Bogie Dynamics: Experimental and Theoretical Investigations. Mach. Dyn. Res. 2017, 41, 128–142. [Google Scholar]
- Sunbuloglu, E.; Bozdag, E. A design strategy on the talbot type articulation of a freight wagon. WIT Trans. Built Environ. 2018, 181, 145–156. [Google Scholar] [CrossRef]
- Xiao, Y.; Tutumluer, E. Performance Checks for Unbound Aggregate Base Permanent Deformation Prediction Models under Dynamic Stress States Induced by Moving Wheel Loading. Procedia Eng. 2016, 143, 979–990. [Google Scholar] [CrossRef]
- Sharma, R.C.; Sharmab, S.K.; Pallic, S. Rail Vehicle Modelling and Simulation using Lagrangian Method. Veh. Struct. Syst. 2018, 10, 188–194. [Google Scholar] [CrossRef]
- Kaiser, I.; Poll, G.; Vinolas, J. Modelling the impact of structural flexibility of wheelsets and rails on the wheel-rail contact and the wear. Wear 2020, 504–505, 203445. [Google Scholar] [CrossRef]
- Sharma, R.C.; Palli, S. Analysis of creep force and its sensitivity on stability and vertical-lateral ride for railway vehicle. Int. J. Veh. Noise Vib. 2016, 12, 60. [Google Scholar] [CrossRef]
- Dusza, M. Rail vehicle model motion analysis on curved track with vertical irregularity. WUT J. Transp. Eng. 2020, 130, 75–84. [Google Scholar] [CrossRef]
- Pehlivan, F.; Mizrak, C.; Esen, I. Modeling and Validation of 2-DOF Rail Vehicle Model Based on Electro–Mechanical Analogy Theory Using Theoretical and Experimental Methods. Eng. Technol. Appl. Sci. Res. 2018, 8, 3603–3608. [Google Scholar] [CrossRef]
- Wu, Q.; Sun, Y.Q.; Spiryagin, M.; Cole, C. Railway track longitudinal force model. Veh. Syst. Dyn. 2019, 59, 155–170. [Google Scholar] [CrossRef]
- Sobolevska, M.; Horobets, D.; Syrota, S. 2020. Development of passive protection devices for a power head of a high-speed multiple unit train at its collisions. IOP Conf. Ser. Mater. Sci. Eng. 2020, 985, 012016. [Google Scholar] [CrossRef]
- Liu, X.; Thompson, D.; Squicciarini, G.; Rissmann, M.; Bouvet, P.; Xie, G.; Martínez-Casas, J.; Carballeira, J.; Arteaga, I.L.; Garralaga, M.A.; et al. Measurements and modelling of dynamic stiffness of a railway vehicle primary suspension element and its use in a structure-borne noise transmission model. Appl. Acoust. 2021, 182, 108232. [Google Scholar] [CrossRef]
- Xu, L.; Xin, L.; Yu, Z.; Zhu, Z. Construction of a dynamic model for the interaction between the versatile tracks and a vehicle. Eng. Struct. 2020, 206, 110067. [Google Scholar] [CrossRef]
- Sichani, M.S.; Enblom, R.; Berg, M. A fast wheel–rail contact model for application to damage analysis in vehicle dynamics simulation. Wear 2016, 366–367, 123–130. [Google Scholar] [CrossRef]
- Cruceanu, C.; Crǎciun, C. Aspects regarding braking process of passenger trains with different braking systems in composition. IOP Conf. Ser. Mater. Sci. Eng. 2018, 400, 042012. [Google Scholar] [CrossRef]
- Stoilov, V.; Simić, G.; Purgić, S.; Milković, D.; Slavchev, S.; Radulović, S.; Maznichki, V. Comparative analysis of the results of theoretical and experimental studies of freight wagon Sdggmrss-twin. IOP Conf. Ser. Mater. Sci. Eng. 2019, 664, 012026. [Google Scholar] [CrossRef]
- Silva, R.; Ribeiro, D.; Bragança, C.; Costa, C.; Arêde, A.; Calçada, R. Model Updating of a Freight Wagon Based on Dynamic Tests under Different Loading Scenarios. Appl. Sci. 2021, 11, 10691. [Google Scholar] [CrossRef]
- Wei, X.; Liu, F.; Jia, L. Urban rail track condition monitoring based on in-service vehicle acceleration measurements. Measurement 2016, 80, 217–228. [Google Scholar] [CrossRef]
- Kang, C.; Wenner, M.; Marx, S. Experimental investigation on the rail residual stress distribution and its influence on the bending fatigue resistance of rails. Constr. Build. Mater. 2021, 284, 122856. [Google Scholar] [CrossRef]
- Bondarenko, I.; Keršys, R.; Neduzha, L. Analysis of problem related to experimental data processing in the study of the crew’s influence on the track. In Proceedings of the 26th International Conference Transport Means, Kaunas, Lithuania, 5–7 October 2022; pp. 1–6. [Google Scholar]
- Borucka, A.; Mazurkiewicz, D.; Łagowska, E. Mathematical modelling as an element of planning rail transport strategies. Transport 2021, 36, 354–363. [Google Scholar] [CrossRef]
- Aimé, L.S.; Lebon, F.; Saulot, A. Thermomechanical modelling of the tribological surface transformations in the railroad network (white etching layer). Mech. Mater. 2020, 151, 103636. [Google Scholar] [CrossRef]
- Jarašūnienė, A.; Sinkevičius, G.; Čižiūnienė, K.; Čereška, A. Adaptation of the Management Model of Internationalization Processes in the Development of Railway Transport Activities. Sustainability 2020, 12, 6248. [Google Scholar] [CrossRef]
- Milenkovic, M.; Svadlenka, L.; Melichar, V.; Bojovic, N.; Avramović, Z. SARIMA modelling approach for railway passenger flow forecasting. Transport 2018, 33, 1113–1120. [Google Scholar] [CrossRef]
- Stopka, O.; Zitrický, V.; Abramović, B.; Marinov, M.; Ricci, S. Innovative Technologies for Sustainable Passenger Transport. J. Adv. Transp. 2019, 2019, 4197246. [Google Scholar] [CrossRef]
- Severino, A.; Martseniuk, L.; Curto, S.; Neduzha, L. Routes Planning Models for Railway Transport Systems in Relation to Passengers’ Demand. Sustainability 2021, 13, 8686. [Google Scholar] [CrossRef]
- Rehak, D.; Patrman, D.; Foltin, P.; Dvořák, Z.; Skrickij, V. Negative impacts from disruption of road infrastructure element performance on dependent subsystems: Methodological framework. Transport 2021, 36, 510–524. [Google Scholar] [CrossRef]
- Pipitone, E.; Caltabellotta, S.; Occhipinti, L. A Life Cycle Environmental Impact Comparison between Traditional, Hybrid, and Electric Vehicles in the European Context. Sustainability 2021, 13, 10992. [Google Scholar] [CrossRef]
- Mikušová, N.; Fedorko, G.; Molnár, V.; Hlatká, M.; Kampf, R.; Sirková, V. Possibility of a Solution of the Sustainability of Transport and Mobility with the Application of Discrete Computer Simulation—A Case Study. Sustainability 2021, 13, 9816. [Google Scholar] [CrossRef]
- Vojtek, M.; Kendra, M.; Zitrický, V.; Široký, J. Mathematical approaches for improving the eflciency of railway transport. Open Eng. 2020, 10, 57–63. [Google Scholar] [CrossRef]
- Wróblewski, P.; Lewicki, W. A Method of Analyzing the Residual Values of Low-Emission Vehicles Based on a Selected Expert Method Taking into Account Stochastic Operational Parameters. Energies 2021, 14, 6859. [Google Scholar] [CrossRef]
- Sañudo, O.R.; Pombo, J.; Ricci, S.; Miranda, M. The importance of sleepers spacing in railways. Constr. Build. Mater. 2021, 300, 124326. [Google Scholar] [CrossRef]
- Twumasi-Boakye, R.; Sobanjo, J. Civil infrastructure resilience: State-of-the-art on transportation network systems. Transp. A Transp. Sci. 2019, 15, 455–484. [Google Scholar] [CrossRef]
- Frilli, A.; Meli, R.; Nocciolini, D.; Pugi, L.; Rindi, A.; Romani, B.; Ceraolo, M.; Lutzemberger, G. The Tesys Rail Project: Innovative Models to Enhance the Energy Sustainability of Railway Systems. Int. J. Railw. Technol. 2017, 6, 1–28. [Google Scholar] [CrossRef]
- Rausand, M.; Høyland, A. System Reliability Theory: Models, Statistical Methods, and Applications, 2nd ed.; John Wiley & Sons: Hoboken, NJ, USA, 2003; 672p, ISBN 978-0-471-47133-2. [Google Scholar] [CrossRef]
- Kumar, A.; Sharma, P.; Agarwal, A. RAMS of HVAC for Rolling Stock Application. Int. J. Eng. Tech. Res. 2021, 10, 157. [Google Scholar]
- Loidolt, M.; Marschnig, S. Evaluating Short-Wave Effects in Railway Track Using the Rail Surface Signal. Appl. Sci. 2022, 12, 2529. [Google Scholar] [CrossRef]
- Malekjafarian, A.; OBrien, E.J.; Quirke, P.; Cantero, D.; Golpayegani, F. Railway Track Loss-of-Stiffness Detection Using Bogie Filtered Displacement Data Measured on a Passing Train. Infrastructures 2021, 6, 93. [Google Scholar] [CrossRef]
- Zhang, K.; Yang, J.; Liu, C.; Wang, J.; Yao, D. Dynamic Characteristics of a Traction Drive System in High-Speed Train Based on Electromechanical Coupling Modeling under Variable Conditions. Energies 2022, 15, 1202. [Google Scholar] [CrossRef]
- Heleno, R.; Montenegro, P.A.; Carvalho, H.; Ribeiro, D.; Calcada, R.; Baker, C.J. Influence of the railway vehicle properties in the running safety against crosswinds. J. Wind Eng. Ind. Aerodyn. 2021, 217, 104732. [Google Scholar] [CrossRef]
- Tułecki, A.; Szkoda, M. Ecology, energy efficiency and resource efficiency as the objectives of rail vehicles renewal. Transp. Res. Procedia 2017, 25, 386–406. [Google Scholar] [CrossRef]
- Alfonso, J.; Rodriguez, J.M.; Salazar, J.C.; Orús, J.; Schreiber, V.; Ivanov, V.; Augsburg, K.; Molina, J.V.; Sakka, M.A.; Castellano, J.A. Distributed simulation and testing for the design of a smart suspension. SAE Int. J. Connect. Autom. Veh. 2020, 3, 129–138. [Google Scholar] [CrossRef]
- Kendra, M.; Skrúcaný, T.; Synák, F.; Škorupa, M.; Grenčík, J. Energy intensity of railway and road passenger transport and its breaking point according to vehicle capacity usage. In Proceedings of the 7th Transport Research Arena TRA, Vienna, Austria, 16–19 April 2018; pp. 1–10. [Google Scholar] [CrossRef]
- Babyak, M.; Keršys, R.; Neduzha, L. Improving the dependability evaluation technique of a transport vehicle. In Proceedings of the International Conference Transport Means, Kaunas, Lithuania, 30 September–2 October 2020; pp. 646–651. [Google Scholar]
- Bosso, N.; Gugliotta, A.; Magelli, M.; Zampieri, N. Monitoring of railway freight vehicles using onboard systems. Procedia Struct. Integr. 2019, 24, 692–705. [Google Scholar] [CrossRef]
- Komorski, P.; Nowakowski, T.; Firlik, B.; Szymański, G.M. Analysis of wheel and track irregularities impact on the vibroacoustic signals emission in rail vehicles. In Proceedings of the 25th International Congress on Sound and Vibration ICSV25, Hiroshima, Japan, 8–12 July 2018. [Google Scholar]
- Bondarenko, I. Modeling for establishment of evaluation conditions of functional safety of the railway track. East. -Eur. J. Enterp. Technol. 2016, 1, 4–10. [Google Scholar] [CrossRef]
- Zelenko, Y.; Zelenko, D.; Neduzha, L. Contemporary principles for solving the problem in noise reduction from railway rolling stock. IOP Conf. Ser. Mater. Sci. Eng. 2020, 985, 012015. [Google Scholar] [CrossRef]
- Komorski, P.; Szymański, G.M.; Nowakowski, T. Development of the urban rail vehicle acoustic model. Appl. Acoust. 2022, 195, 108807. [Google Scholar] [CrossRef]
- Harrison, T.; Midgley, W.; Goodall, R.M.; Ward, C.P. Development and control of a rail vehicle model to reduce energy consumption and carbon dioxide emissions. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2021, 235, 1237–1248. [Google Scholar] [CrossRef]
- Boccaccio, M.; Fierro, G.P.M.; Bucciarelli, F.; Meo, M. Multi-tonal subwavelength metamaterial for absorption and amplification of acoustic and ultrasonic waves. Eng. Res. Express 2021, 3, 025024. [Google Scholar] [CrossRef]
- Liu, Q.; Lei, X.; Rose, J.G.; Chen, H.P.; Feng, Q.; Luo, X. Vertical wheel-rail force waveform identification using wavenumber domain method. Mech. Syst. Signal Process. 2021, 159, 107784. [Google Scholar] [CrossRef]
- Velletrani, F.; Licciardello, R.; Bruner, M. Intelligent Wheelsets for the trains of the future: The role of in-service wheel-rail force measurement. Ing. Ferrov. 2020, 10, 701. [Google Scholar]
- Iwnicki, S.D.; Grassie, S.L.; Kik, W. Track Settlement Prediction Using Computer Simulation Tools. Vehicle System Dynamics. In The Dynamics of Vehicles on Roads and on Tracks; CRC Press: Boca Raton, FL, USA, 2021. [Google Scholar] [CrossRef]
- True, H.; Christiansen, L.E.; Plesner, A.L.; Ammitzbøll, A.L.; Dahl, B.J. Why is it so difficult to determine the lateral Position of the Rails by a Measurement of the Motion of an Axle on a moving Vehicle? In Proceedings of the First International Conference on Rail Transportation, Chengdu, China, 10–12 July 2017.
- Mosleh, A.; Montenegro, P.A.; Costa, P.A.; Calçada, R. Railway Vehicle Wheel Flat Detection with Multiple Records Using Spectral Kurtosis Analysis. Appl. Sci. 2021, 11, 4002. [Google Scholar] [CrossRef]
- Enjalbert, S.; Gandini, L.M.; Pereda-Baños, A.; Ricci, S.; Frédéric, V. Human–Machine Interface in Transport Systems: An Industrial Overview for More Extended Rail Applications. Machines 2021, 9. [Google Scholar] [CrossRef]
- Bondarenko, I.; Keršys, R.; Neduzha, L. Studying of Dynamic Parameters Impulse Impact of the Vehicle Taking into Account the Track Stiffness Variations. In Proceedings of the 25th International Conference Transport Means, Kaunas, Lithuania, 6–8 October 2021; pp. 684–689. [Google Scholar]
- Borghetti, F.; Petrenj, B.; Trucco, P.; Marchionni, G.; Ponti, M.; Calabrese, V. Multi-level approach to assessing the resilience of road network infrastructure. Int. J. Crit. Infrastruct. 2021, 17, 97. [Google Scholar] [CrossRef]
- Ngo, D.; Parkinson, H.; Bamford, G.; Bamford, J.; Rayson, P. Opportunities for resilient rail system development using natural language processing. In Proceedings of the Conference: 7th Transport Research Arena TRA 2018, Vienna, Austria, 16–19 April 2018. [Google Scholar] [CrossRef]
- Knapcikova, L.; Konings, R. European railway infrastructure: A review. Acta Logist.-Int. Sci. J. About Logist. 2018, 5, 71–77. [Google Scholar] [CrossRef]
- Falamarzi, A.; Moridpour, S.; Nazem, M. A reviewof rail track degradation prediction models. Aust. J. Civ. Eng. 2019, 17, 152–166. [Google Scholar] [CrossRef]
- Bernacki, D.; Lis, C. Exploring the sustainable effects of urban-port road system reconstruction. Energies 2021, 14, 6512. [Google Scholar] [CrossRef]
- Scholte, W.J.; Marco, V.R.; Nijmeijer, H. Experimental Validation of Vehicle Velocity, Attitude and IMU Bias Estimation. IFAC-Pap. 2019, 52, 118–123. [Google Scholar] [CrossRef]
- Olaby, O.; Hamadache, M.; Soper, D.; Winship, P.; Dixon, R. Development of a Novel Railway Positioning System Using RFID Technology. Sensors 2022, 22, 2401. [Google Scholar] [CrossRef]
- Galar, D.; Seneviratne, D.; Kumar, U. Big Data in Railway O&M: A Dependability Approach. In Research Anthology on Big Data Analytics, Architectures, and Applications; IGI Global: Hershey, PA, USA, 2022. [Google Scholar] [CrossRef]
- Sasidharan, M.; Burrow, M.P.N.; Ghataora, G.S.; Marathu, R. A risk-informed decision support tool for the strategic asset management of railway track infrastructure. Proc. Inst. Mech. Eng. Part F J. Rail Rapid Transit 2021, 236, 183–197. [Google Scholar] [CrossRef]
- Yan, H.; Gao, C.; Elzarka, H.; Mostafa, K.; Tang, W. Risk assessment for construction of urban rail transit projects. Saf. Sci. 2019, 118, 583–594. [Google Scholar] [CrossRef]
- Bondarenko, I.; Lunys, O.; Neduzha, L.; Keršys, R. Dynamic Track Irregularities Modeling when Studying Rolling Stock Dynamics. In Proceedings of the 23rd International Conference Transport Means, Palanga, Lithuania, 2–4 October 2019; pp. 1014–1019. [Google Scholar]
- Domin, R.; Domin, Y.; Chernyak, G.; Nozhenko, V. Method of operational investigation of rolling stock derailment. Transp. Syst. Technol. 2021, 37, 173–186. [Google Scholar] [CrossRef]
- Nandan, S.; Trivedi, R.; Kant, S.; Ahmad, J.; Maniraj, M. Design, Analysis and Prototype Development of Railway Wagons on Different Loading Conditions. Int. J. Eng. Appl. Sci. Technol. 2020, 4, 122–129. [Google Scholar] [CrossRef]
- Liu, Z.; Kang, J.; Zhao, X.; Zuo, M.J.; Qin, Y.; Jia, L. Modelling of the Safe Region Based on Support Vector Data Description for Health Assessment of Wheelset Bearings. Appl. Math. Model. 2019, 73, 19–29. [Google Scholar] [CrossRef]
- Kulbovskyi, I.; Holub, H.; Andonova, S.; Saiapina, I.; Tkachuk, M. Model and methods of management of efficiency of use of production and technological potential of rolling stock of rail transport. Transp. Syst. Technol. 2021, 38, 232–238. [Google Scholar] [CrossRef]
- Sichani, M.S.; Enblom, R.; Berg, M. A novel method to model wheel–rail normal contact in vehicle dynamics simulation. Veh. Syst. Dyn. 2014, 52, 1752–1764. [Google Scholar] [CrossRef]
- Liu, W.; Xiong, W. Rethinking the Transport Infrastructure-Led Development Model. Sustainability 2022, 14, 407. [Google Scholar] [CrossRef]
- Poveda-Reyes, S.; Rizzetto, L.; Triti, C.; Shi, D.; García-Jiménez, E.; Molero, G.M.; Santarremigia, F.E. Risk evaluation of failures of the running gear with effects on rail infrastructure. Eng. Fail. Anal. 2021, 128, 105613. [Google Scholar] [CrossRef]
- Pons, J.J.; Sanchis, I.V.; Franco, R.I.; Yepes, V. Life cycle assessment of a railway tracks substructures: Comparison of ballast and ballastless rail tracks. Environ. Impact Assess. Rev. 2020, 85, 106444. [Google Scholar] [CrossRef]
- Procházková, D.; Prochazka, J. Risk Management Plan for Technical Facility Operation. In Proceedings of the 31st European Safety and Reliability Conference, Angers, France, 19–23 September 2021. [Google Scholar] [CrossRef]
- Gerum, P.C.L.; Altay, A.; Baykal-Gürsoy, M. Data-driven Predictive Maintenance Scheduling Policies for Railways. Transp. Res. Part C Emerg. Technol. 2019, 107, 137–154. [Google Scholar] [CrossRef]
- Bondarenko, I.; Keršys, A.; Neduzha, L. Assessment of the Railway Track Deformability Behaviour as the Parameter of Operational Availability Function. In Proceedings of the 25th International Conference Transport Means, Kaunas, Lithuania, 6–8 October 2021; pp. 644–648. [Google Scholar]
- Xie, J.; Huang, J.; Zeng, C.; Jiang, S.-H.; Podlich, N. Systematic Literature Review on Data-Driven Modelsfor Predictive Maintenance of Railway Track: Implications in Geotechnical Engineering. Geosciences 2020, 10, 425. [Google Scholar] [CrossRef]
- Kamburjan, E.; Hähnle, R. Formal modeling and analysis of railway operations with active objects. Sci. Comput. Program. 2018, 166, 167–193. [Google Scholar] [CrossRef]
- Leite, M.; Costa, M.A.; Alves, T.; Infante, V.; Andrade, A.R. Reliability and availability assessment of railway locomotive bogies under correlated failures. Eng. Fail. Anal. 2022, 135, 106104. [Google Scholar] [CrossRef]
- Grossoni, I.; Powrie, W.; Zervos, A.; Bezin, Y.; Pen, L.P. Modelling railway ballasted track settlement in vehicle-track interaction analysis. Transp. Geotech. 2021, 26, 100433. [Google Scholar] [CrossRef]
- Liu, Z.; Song, H.; Tan, H.; Hao, H.; Zhao, F. Evaluation of the Cost of Intelligent Upgrades of Transportation Infrastructure for Intelligent Connected Vehicles. J. Adv. Transp. 2022, 2022, 5841373. [Google Scholar] [CrossRef]
- Rahimi, M.; Liu, H.; Cardenas, I.D.; Starr, A.; Hall, A.; Anderson, R. A Review on Technologies for Localisation and Navigation in Autonomous Railway Maintenance Systems. Sensors 2022, 22, 4185. [Google Scholar] [CrossRef] [PubMed]
- Sedghi, M.; Kauppila, O.; Bergquist, B.; Vanhatalo, E.; Kulahci, M. A taxonomy of railway track maintenance planning and scheduling: A review and research trends. Reliab. Eng. Syst. Saf. 2021, 215, 107827. [Google Scholar] [CrossRef]
- Maciej, A.; Ryszard, S.; Kinga, S.; Mateusz, N. Analysis of driver assistance systems in rail vehicles. Pojazdy Szyn. 2020, 4, 25–32. [Google Scholar] [CrossRef]
- Zolotas, A.; Hassan, F. Impact of Fractional Order Methods on Optimized Tilt Control for Rail Vehicles. Fract. Calc. Appl. Anal. 2017, 20, 765–789. [Google Scholar] [CrossRef]
- Kuźma, K.; Pyrek, K.; Kocik, W.; Kalinowski, P.; Kamiński, P. Individual rail transport and the conception of a means of transport using a suspended railway track. Min.-Inform. Autom. Electr. Eng. 2019, 3, 47. [Google Scholar] [CrossRef]
- Liu, B.; Yue, H.; Lin, J.; Wu, X.; Shi, Z. Analysis and Optimization of Driving Attitude and Oscillation Characteristics of Suspension-Type Small Rail Vehicles. Shock Vib. 2020, 2020, 8894597. [Google Scholar] [CrossRef]
- Pillai, N.; Shih, J.Y.; Roberts, C. Evaluation of Numerical Simulation Approaches for Simulating Train-Track Interactions and Predicting Rail Damage in Railway Switches and Crossings (S&Cs). Infrastructures 2021, 6, 63. [Google Scholar] [CrossRef]
- Chang, C.; Ling, L.; Chen, S.; Zhai, W.; Wang, K.; Wang, G. Dynamic performance evaluation of an inspection wagon for urban railway tracks. Measurement 2020, 170, 108704. [Google Scholar] [CrossRef]
- Yang, Y.B.; Wang, Z.L.; Shi, K.; Xu, H.; Mo, X.Q.; Wu, Y.T. Two-axle test vehicle for damage detection for railway tracks modeled as simply supported beams with elastic foundation. Eng. Struct. 2020, 319, 110908. [Google Scholar] [CrossRef]
- Lu, Y.; Zhang, J.; Yang, S.; Li, Z. Study on improvement of LuGre dynamical model and its application in vehicle handling dynamics. J. Mech. Sci. Technol. 2019, 33, 545–558. [Google Scholar] [CrossRef]
- Polak, K.; Korzeb, J. Acoustic Signature and Impact of High-Speed Railway Vehicles in the Vicinity of Transport Routes. Energies 2022, 15, 3244. [Google Scholar] [CrossRef]
- Lesgidis, N.; Sextos, A.; Moschen, L.; Gomez, J.S.G.; Pistone, E. Rigorous vehicle-soil-track simulation of high-speed rail through optimization-based model order reduction. Transp. Geotech. 2020, 23, 100350. [Google Scholar] [CrossRef]
- Liang, Y.; Feng, Q.; Lu, J.; Guo, W.; Yang, Z. A Double-Rail Phononic Crystal Model for the Ballasted Track. International J. Struct. Stab. Dyn. 2022, 22, 2250066. [Google Scholar] [CrossRef]
- Minda, A.; Lucian, P.; Burtea, D.G.; Gillich, G.R. Smoothing the Time-Frequency Representations to Improve the Diagnosis for Engineering Structures. Annals of the “Constantin Brancusi” University of Targu Jiu, Engineering Series. No. 1/2021. Available online: https://www.utgjiu.ro/rev_ing/pdf/2021-1/01_Andrea%20Amalia%20Minda_SMOOTHING%20THE%20TIME-FREQUENCY%20REPRESENTATIONS%20TO%20IMPROVE%20THE%20DIAGNOSIS%20FOR%20ENGINEERING%20STRUCTURES.pdf (accessed on 1 August 2022).
- Han, J.; He, Y.; Wang, J.; Xiao, X. Simulation and experimental study on vibration and acoustic characteristics of a continuous supported embedded track. Appl. Acoust. 2021, 180, 108103. [Google Scholar] [CrossRef]
- Xiao, J.; Yan, Z.; Shi, J.; Ma, D. Effects of Wheel-Rail Impact on the Fatigue Performance of Fastening Clips in Rail Joint Area of High-Speed Railway. KSCE J. Civ. Eng. 2022, 26, 120–130. [Google Scholar] [CrossRef]
- Xu, L.; Zhai, W.M. A novel model for determining the amplitude-wavelength limits of track irregularities accompanied by a reliability assessment in railway vehicle-track dynamics. Mech. Syst. Signal Process. 2017, 86, 260–277. [Google Scholar] [CrossRef]
- Sadeghi, J.; Khajehdezfuly, A.; Esmaeili, M.; Poorveis, D. Investigation of rail irregularity effects on wheel/rail dynamic force in slab track: Comparison of two and three dimensional models. J. Sound Vib. 2016, 374, 228–244. [Google Scholar] [CrossRef]
- Auersch, L. Different Types of Continuous Track Irregularities as Sources of Train-Induced Ground Vibration and the Importance of the Random Variation of the Track Support. Appl. Sci. 2022, 12, 1463. [Google Scholar] [CrossRef]
- Paudel, M.; Lim, L.; Yap, F.; Kho, K. Vibration Analysis of the Third Rail Structure of a Mass Rapid Transit System with Structural Defects. Appl. Sci. 2021, 11, 8410. [Google Scholar] [CrossRef]
- Costa, M.A.; Costa, J.N.; Andrade, A.R.; Ambrósio, J. Combining wavelet analysis of track irregularities and vehicle dynamics simulations to assess derailment risks. Int. J. Veh. Mech. Mobil. 2022, 1–27. [Google Scholar] [CrossRef]
- Sekulic, D.; Dedović, V.; Mladenović, D.M. General oscillatory model of vehicles for passenger transport. In Proceedings of the Second International Conference on Traffic and Transport Engineering (ICTTE), Belgrade, Serbia, 27–28 November 2014. [Google Scholar]
- Papaelias, M.; Amini, A.; Culwick, R.; Heesom, J.; Huang, Z.; Junior, V.L.J.; Kaenwunruen, S.; Kerkyras, S.; Kongpuang, M.; Marquez, F.P.G.; et al. Advanced remote condition monitoring of railway infrastructure and rolling stock. In Proceedings of the 1st International Conference on Welding & Non Destructive Testing, Athens, Greece, 22–23 October 2018. [Google Scholar]
- Vlachospyros, G.; Iliopoulos, I.A.; Kritikakos, K.; Kaliorakis, N.; Fassois, S.D.; Sakellariou, J.S.; Deloukas, A.; Leoutsakos, G.; Giannakis, C.; Chronopoulos, E.; et al. The MAIANDROS System for Random-Vibration-Based On-Board Railway Vehicle and Track Monitoring. In Proceedings of the ASME 2021 International Design Engineering Technical Conferences and Computers and Information in Engineering Conference, Virtual, Online, 17–19 August 2021. [Google Scholar] [CrossRef]
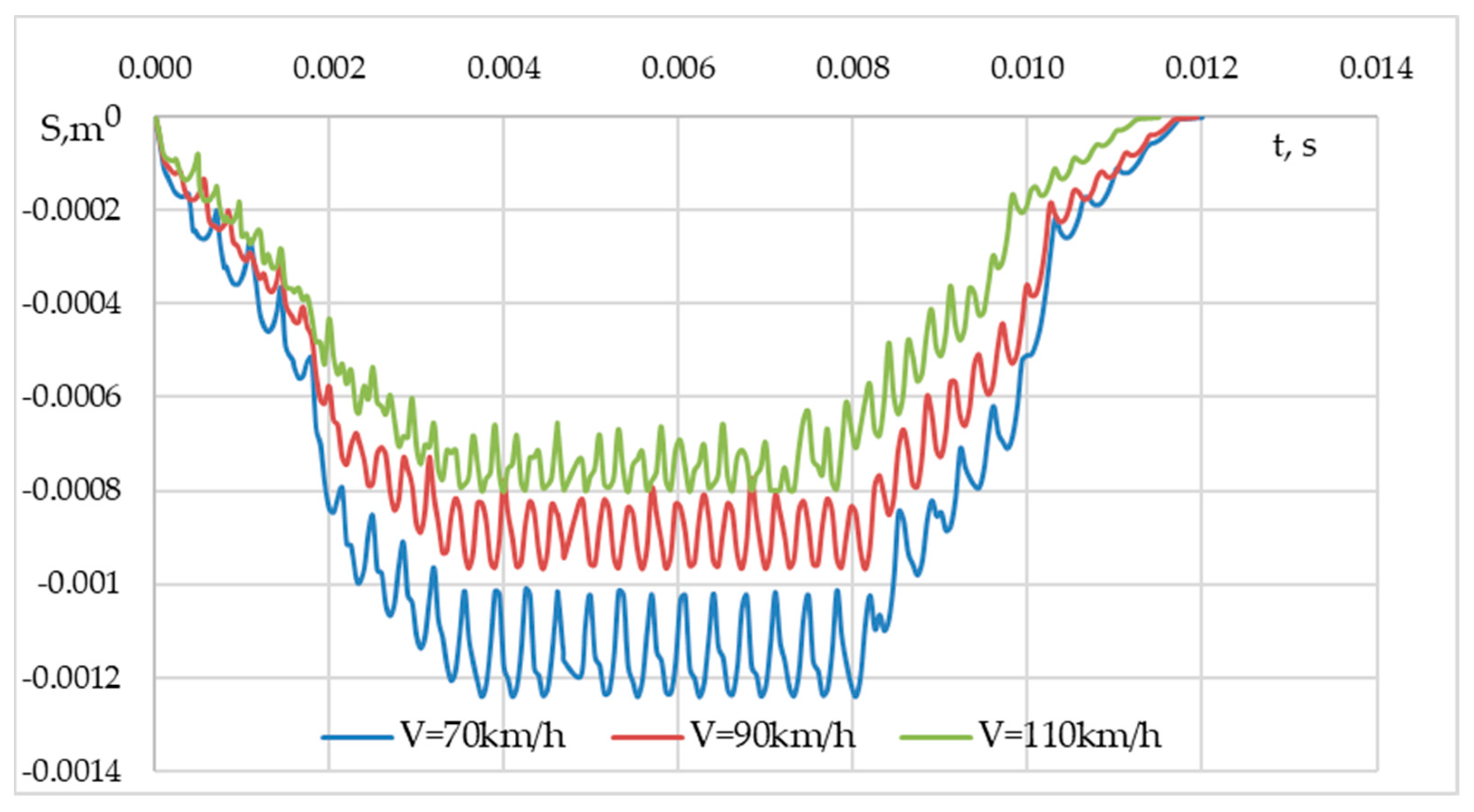
- Bondarenko, I. Development of algorithm for calculating dynamic processes of railroad track deformability work. East. -Eur. J. Enterp. Technol. 2016, 6, 28–36. [Google Scholar] [CrossRef]
- Bondarenko, I. Advantages of applying elastic waves theory in solving dynamic problems. Publ. House “Balt. Publ.” 2022, 328–352. [Google Scholar] [CrossRef]
| Element | Density, kg/m3 | Poisson’s Coefficient | Young’s Module , MPa | ||
|---|---|---|---|---|---|
| first option | |||||
| rail | 7830 | 0.24 | 2.1 × 105 | 5622 | 3288 |
| pad | 918 | 0.3 | 100 | 382 | 204 |
| second option | |||||
| rail | 7830 | 0.3 | 2.1 × 105 | 6008 | 3211 |
| pad | 935 | 0.485 | 200 | 572 | 268 |
| Angles, Degree | ||||
|---|---|---|---|---|
| Incidence | Reflection | Refraction | ||
| longitudinal wave | ||||
| 10 | 10 | 5.33 | 0.68 | 0.34 |
| 20 | 20 | 10.53 | 1.33 | 0.67 |
| 30 | 30 | 15.50 | 1.95 | 0.98 |
| 40 | 40 | 20.10 | 2.51 | 1.25 |
| 50 | 50 | 24.17 | 2.9 | 1.50 |
| 60 | 60 | 27.58 | 3.38 | 1.69 |
| 70 | 70 | 30.15 | 3.67 | 1.83 |
| 80 | 80 | 31.76 | 3.85 | 1.92 |
| transverse wave | ||||
| 10 | 10 | 17.27 | 1.16 | 0.62 |
| 20 | 20 | 35.79 | 2.28 | 1.22 |
| 30 | 30 | 58.74 | 3.3 | 1.78 |
| 40 | 40 | - | 3.88 | 2.07 |
| 50 | 50 | - | 5.12 | 2.73 |
| 60 | 60 | - | 5.79 | 3.09 |
| 70 | 70 | - | 6.28 | 3.35 |
| 80 | 80 | - | 6.58 | 3.51 |
| Longitudinal Wave | ||||
|---|---|---|---|---|
| , degree | ||||
| 10 | 0.941 | 0.392 | 1.950 | 0.380 |
| 20 | 0.820 | 0.727 | 1.852 | 0.732 |
| 30 | 0.646 | 0.963 | 1.701 | 1.047 |
| 40 | 0.450 | 1.081 | 1.512 | 1.302 |
| 50 | 0.272 | 1.085 | 1.302 | 1.482 |
| 60 | 0.148 | 0.997 | 1.083 | 1.558 |
| 70 | 0.131 | 0.835 | 0.855 | 1.485 |
| 80 | 0.318 | 0.573 | 0.569 | 1.120 |
| Transverse wave | ||||
| Angles , degree | ||||
| 10 | 0.847 | 0.389 | 0.378 | 1.943 |
| 20 | 0.467 | 0.712 | 0.722 | 1.824 |
| 30 | 0.029 | 0.968 | 0.965 | 1.725 |
| 40 | 0.708 | 5.656 | 0.064 | 6.964 |
| 50 | 0.799 | 5.960 | 0.383 | 4.774 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Bondarenko, I.; Severino, A.; Olayode, I.O.; Campisi, T.; Neduzha, L. Dynamic Sustainable Processes Simulation to Study Transport Object Efficiency. Infrastructures 2022, 7, 124. https://doi.org/10.3390/infrastructures7090124
Bondarenko I, Severino A, Olayode IO, Campisi T, Neduzha L. Dynamic Sustainable Processes Simulation to Study Transport Object Efficiency. Infrastructures. 2022; 7(9):124. https://doi.org/10.3390/infrastructures7090124
Chicago/Turabian StyleBondarenko, Iryna, Alessandro Severino, Isaac Oyeyemi Olayode, Tiziana Campisi, and Larysa Neduzha. 2022. "Dynamic Sustainable Processes Simulation to Study Transport Object Efficiency" Infrastructures 7, no. 9: 124. https://doi.org/10.3390/infrastructures7090124
APA StyleBondarenko, I., Severino, A., Olayode, I. O., Campisi, T., & Neduzha, L. (2022). Dynamic Sustainable Processes Simulation to Study Transport Object Efficiency. Infrastructures, 7(9), 124. https://doi.org/10.3390/infrastructures7090124










