Sensitivity Investigation on the Pressure Coefficients Non-Dimensionalization
Abstract
:1. Introduction
2. Wind Tunnel Experimental Setup: Pressure Random Processes
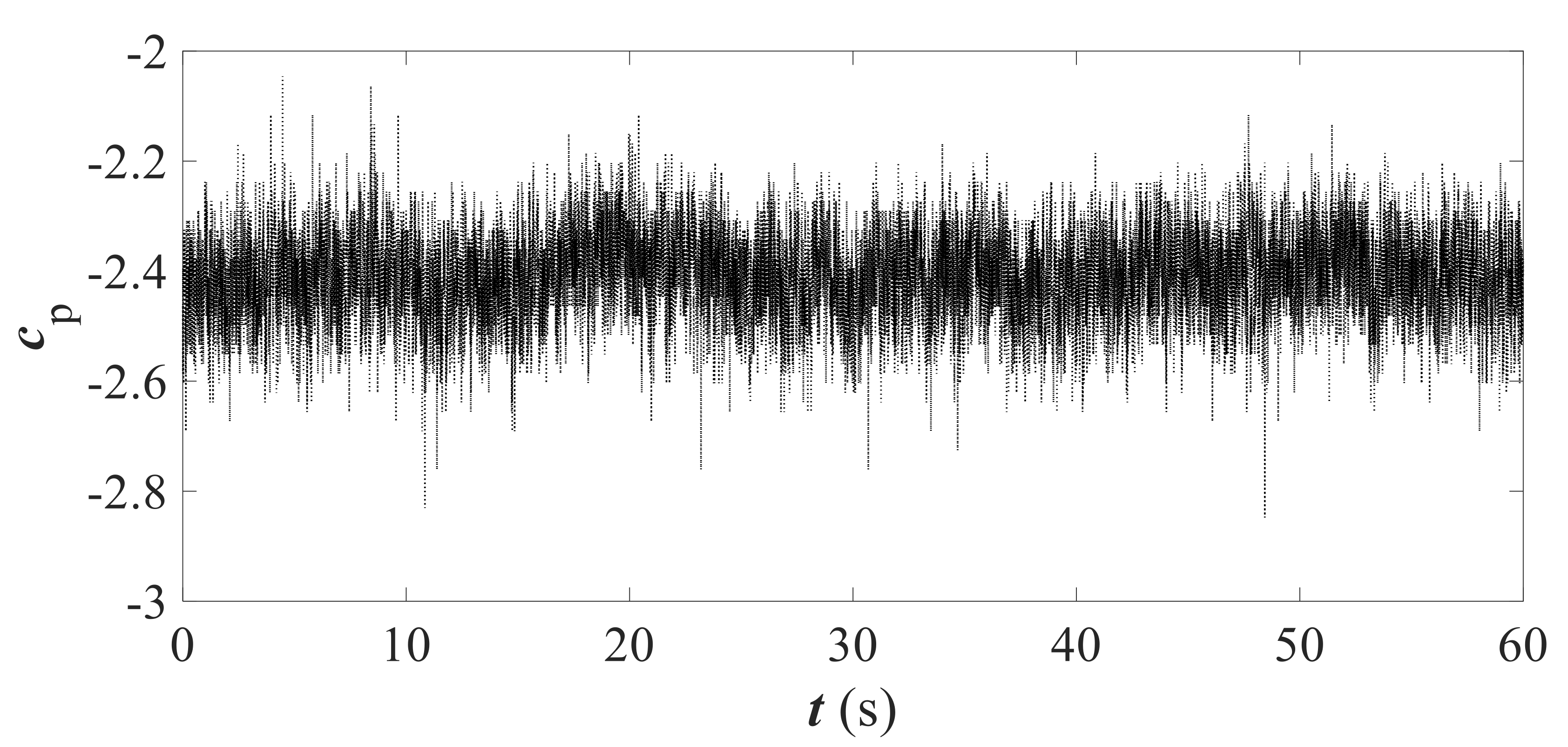
2.1. Pressure Coefficient Estimation: Methodology
2.2. The Blockage Effect on the Pressure Coefficient
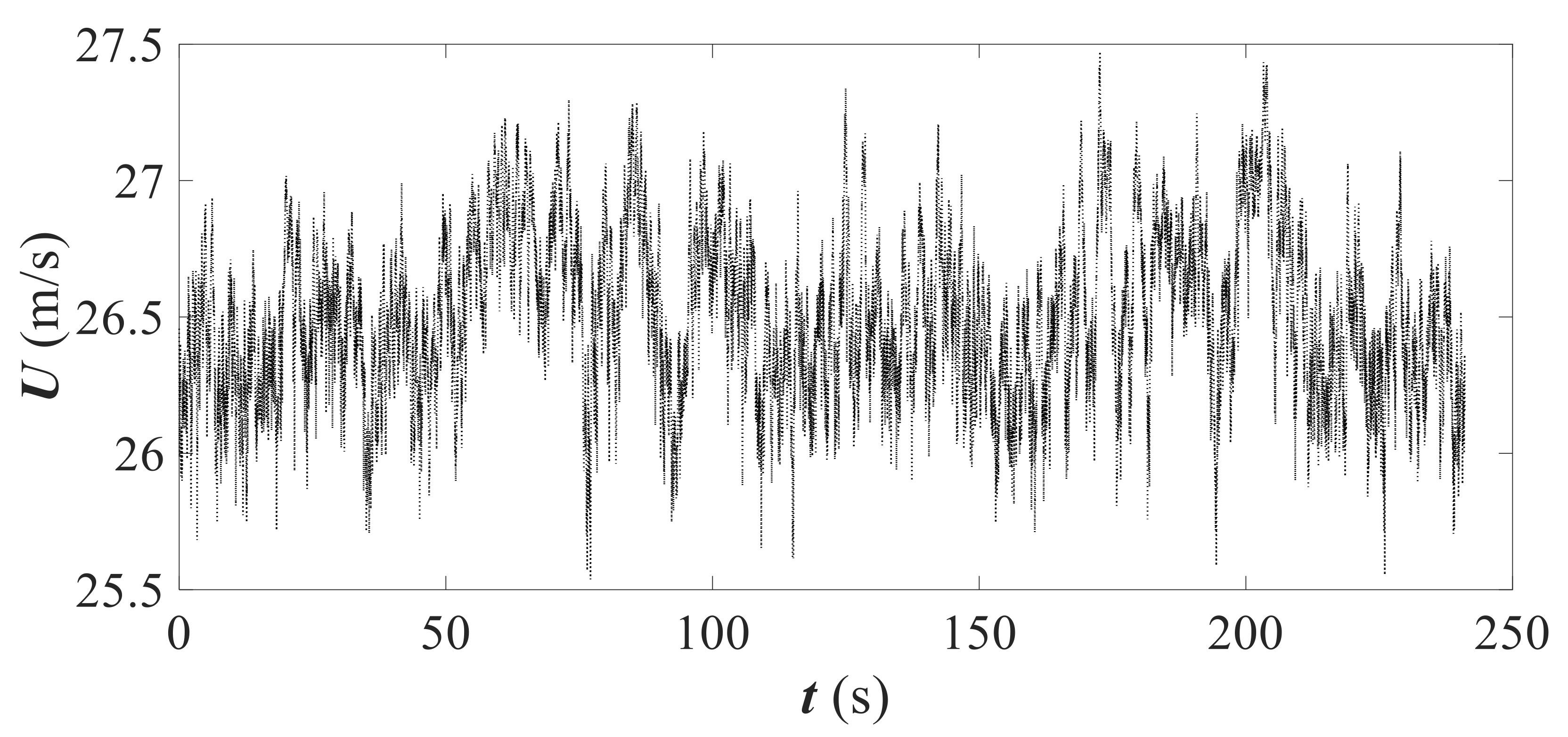
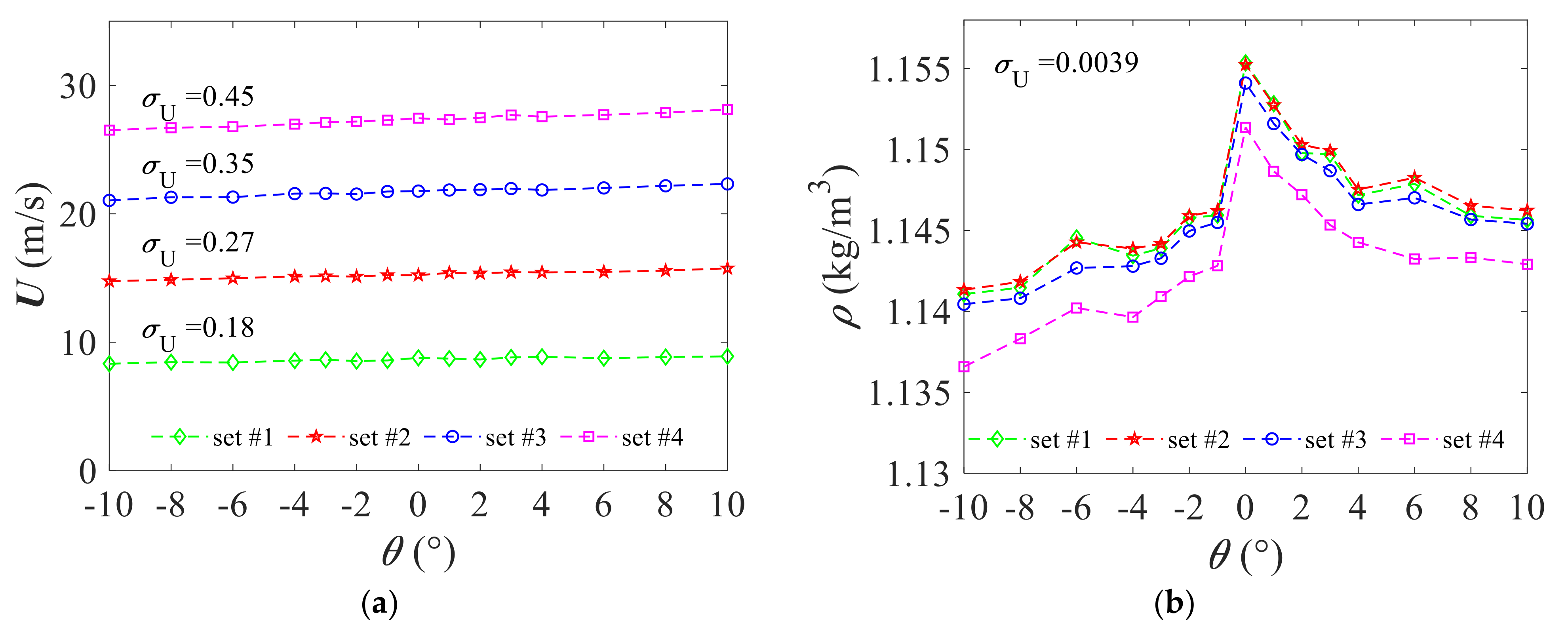
3. Wind Tunnel Experimental Setup: Wind Velocity Random Processes
3.1. The Kinetic Pressure Variability
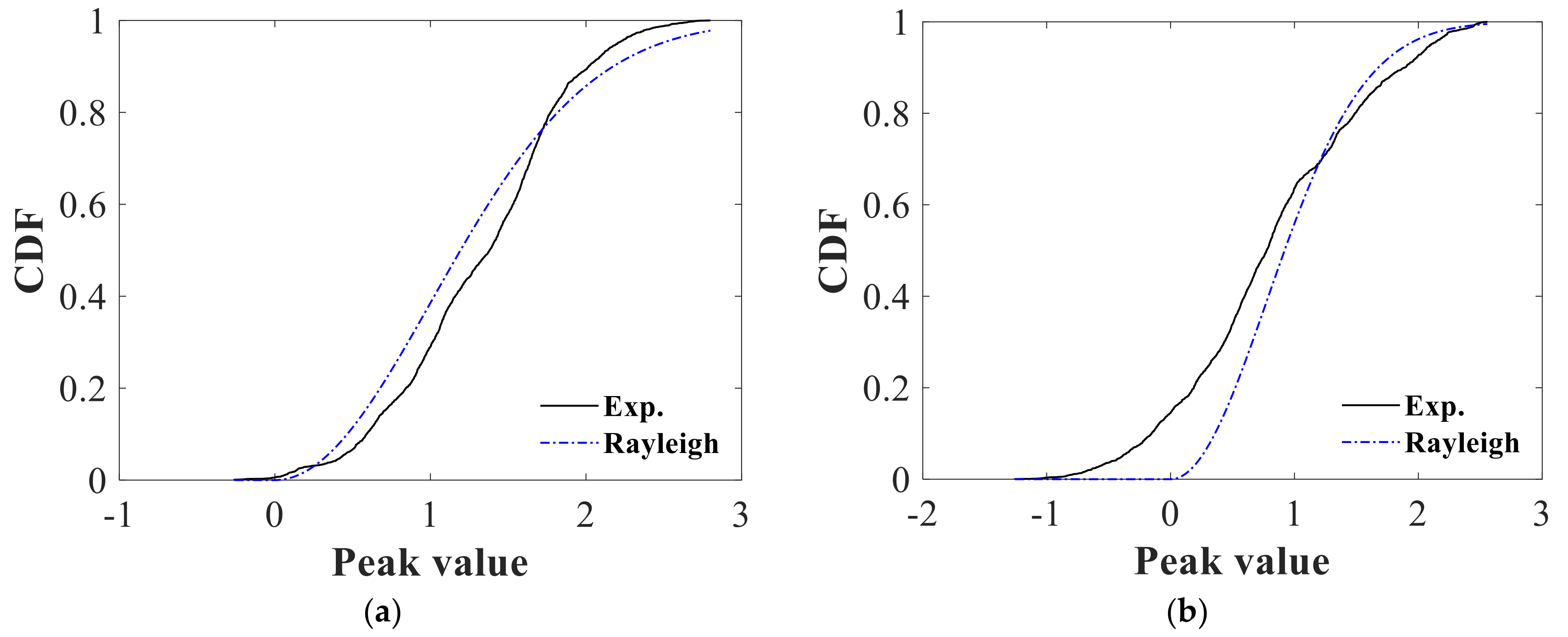
3.2. Non-Stationarity and Non-Gaussianity of the Wind Velocity Random Processes
4. Remarks
5. Conclusions
- The uncertainty related to different sets of wind velocity used to non-dimensionalize the pressure coefficient.
- The uncertainty related to the stationary assumption of the wind tunnel velocity time history.
- The uncertainty related to the Gaussianity of the wind tunnel velocity time history.
- The uncertainty related to the blockage estimation.
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Rizzo, F.; Ricciardelli, F.; Maddaloni, G.; Bonati, A.; Occhiuzzi, A. Experimental error analysis of dynamic properties for a reduced-scale high-rise building model and implications on full-scale behavior. J. Build. Eng. 2020, 28, 101067. [Google Scholar] [CrossRef]
- Rizzo, F.; Caracoglia, L. Examining wind tunnel errors in Scanlan derivatives and flutter speed of a closed-box. J. Wind Struct. 2018, 26, 231–251. [Google Scholar]
- Rizzo, F.; Caracoglia, L.; Montelpare, S. Predicting the flutter speed of a pedestrian suspension bridge through examination of laboratory experimental errors. Eng. Struct. 2018, 172, 589–613. [Google Scholar] [CrossRef]
- Desceliers, C.; Soize, C.; Ghanem, R. Identification of chaos representations of elastic properties of random media using experimental vibration tests. Comput. Mech. 2007, 39, 831–838. [Google Scholar] [CrossRef]
- Schoefs, F.; Yáñez-Godoy, H.; Lanata, F. Polynomial chaos representation for identification of mechanical characteristics of instrumented structures. Comput. Aided Civ. Infrastruct. 2011, 26, 173–189. [Google Scholar] [CrossRef]
- Seo, D.W.; Caracoglia, L. Derivation of equivalent gust effect factors for wind loading on low-rise buildings through Database-Assisted-Design approach. Eng. Struct. 2010, 32, 328–336. [Google Scholar] [CrossRef]
- Rizzo, F.; Barbato, M.; Sepe, V. Peak factor statistics of wind effects for hyperbolic paraboloid roofs. Eng. Struct. 2018, 173, 313–330. [Google Scholar] [CrossRef]
- CNR. Guide for the Assessment of Wind Actions and Effects on Structures; CNR-DT; National Research Council of Italy: Rome, Italy, 2010. [Google Scholar]
- CEN. Eurocode 1: Actions on Structures–Part 1-4: General Actions–Wind Actions, EN-1991-1-4; Comité Européen de Normalization: Brussels, Belgium, 2008. [Google Scholar]
- Isyumov, N. The Aeroelastic Modelling of Tall Buildings. In International Workshop on Wind Tunnel Modelling Criteria and Technique in Civil Engineering Applications; Reinhold, T., Ed.; Cambridge University Press: Cambridge, UK, 1982. [Google Scholar]
- Rizzo, F.; Kopp, A.G.; Giaccu, G. Investigation of wind-induced dynamics of a cable net roof with aeroelastic wind tunnel tests. Eng. Struct. 2021, 229, 111569. [Google Scholar] [CrossRef]
- Barlow, J.B.; Rae, W.H.; Pope, A. Low-Speed Wind Tunnel Testing, 3rd ed.; John Wiley and Sons: New York, NY, USA, 2018. [Google Scholar]
- Bartoli, G.; Mannini, C. Reliability of bridge deck flutter derivative measurement in wind tunnel tests. In Proceedings of the ICOSSAR, Shangai, China, 21–25 June 2005. [Google Scholar]
- Ghanem, R.; Spanos, P.D. Stochastic Finite Elements: A Spectral Approach; Springer-Verlag: New York, NY, USA, 1991. [Google Scholar]
- Gimsing, N.J.; Georgakis, C.T. Cable Supported Bridges: Concept and Design, 3rd ed.; Wiley: Chichester, UK, 2011. [Google Scholar]
- Kwon, S.-D. Uncertainty of bridge flutter velocity measured at wind tunnel tests. In Proceedings of the 5th International Symposium on Computational Wind Engineering (CWE2010), Chapel Hill, NC, USA, 23–25 May 2010. [Google Scholar]
- Jakobsen, J.B.; Tanaka, H. Modelling uncertainties in prediction of aeroelastic bridge behaviour. J. Wind Eng. Ind. Aerodyn. 2003, 91, 1485–1498. [Google Scholar] [CrossRef]
- Le Maître, O.P.; Knio, O.M. Spectral Methods for Uncertainty Quantification; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Mannini, C.; Bartoli, G. The problem of uncertainty in the measurement of aerodynamic derivatives. Safety, Reliability and Risk of Structures. Infrastruct. Eng. Syst. 2010, 824–831. [Google Scholar]
- Ricciardelli, F.; Hangan, H. Pressure distribution and aerodynamic forces on stationary box bridge sections. Wind Struct. 2001, 4, 399–412. [Google Scholar] [CrossRef]
- Ricciardelli, F.; de Grenet, E.T.; Hangan, H. Pressure distribution, aerodynamic forces and dynamic response of box bridge sections. J. Wind Eng. Ind. Aerodyn. 2002, 90, 1135–1150. [Google Scholar] [CrossRef]
- Reinhold, T.A.; Brinch, M.; Damsgaard, A. Wind tunnel tests for the Great Belt Link. In Aerodynamics of Large Bridges, Proceedings of the 1st International Symposium on Aerodynamics of Large Bridges, Copenhagen, Denmark, 19–21 February 1992; Larsen, A., Ed.; CRC Press: Boca Raton, FL, USA, 1992; ISBN 905410 042 7. [Google Scholar]
- Scotta, R.; Lazzari, M.; Stecca, E.; Cotela, J.; Rossi, R. Numerical wind tunnel for aerodynamic and aeroelastic characterization of bridge deck sections. Comput. Struct. 2016, 167, 96–114. [Google Scholar] [CrossRef]
- Rizzo, F.; Caracoglia, L. Artificial Neural Network model to predict the flutter velocity of suspension bridges. Comput. Struct. 2020, 233, 1062362020. [Google Scholar] [CrossRef]
- Rizzo, F.; D’Alessandro, V.; Montelpare, S.; Giammichele, L. Computational study of a bluff body aerodynamics: Impact of the laminar-to-turbulent transition modelling. Int. J. Mech. Sci. 2020, 178, 105620. [Google Scholar] [CrossRef]
- Jones, N.P.; Scanlan, R.H. Theory and full-bridge modeling of wind response of cable-supported bridges. J. Bridge Eng. 2001, 6, 365–375. [Google Scholar] [CrossRef]
- Lau, C.K.; Wong, K.Y. Aerodynamic stability of Tsing Ma Bridge. In Proceedings of the Fourth International Kerensky Conference on Structures in the New Millennium, Hong Kong, China, 3–5 September 1997. [Google Scholar]
- Matsumoto, M.; Kobayashi, Y.; Shirato, H. The influence of aerodynamic derivatives on flutter. J. Wind Eng. Ind. Aerodyn. 1996, 60, 227–239. [Google Scholar] [CrossRef]
- Ostenfeld-Rosenthal, P.; Madsen, H.O.; Larsen, A. Probabilistic flutter criteria for long span bridges. J. Wind Eng. Ind. Aerodyn. 1992, 42, 1265–1276. [Google Scholar] [CrossRef]
- Sarkar, P.P.; Caracoglia, L.; Haan, F.L.; Sato, H.; Murakoshi, J. Comparative and sensitivity study of flutter derivatives of selected bridge deck sections. Part 1: Analysis of inter-laboratory experimental data. Eng. Struct. 2009, 31, 158–169. [Google Scholar] [CrossRef]
- Scanlan, R.H.; Tomko, J.J. Airfoil and bridge deck flutter derivatives. J. Eng. Mech. 1971, 97, 1717–1737. [Google Scholar]
- Scanlan, R.H.; Jones, N.P. Aeroelastic analysis of cable-stayed bridges. J. Struct. Eng. 1990, 116, 279–297. [Google Scholar] [CrossRef]
- Scanlan, R.H.; Jones, N.P.; Singh, L. Inter-relations among flutter derivatives. J. Wind Eng. Ind. Aerodyn. 1997, 69–71, 829–837. [Google Scholar] [CrossRef]
- Simiu, E.; Scanlan, R.H. Wind Effects on Structures: Fundamentals and Applications to Design, 3rd ed.; John Wiley: New York, NY, USA, 1996. [Google Scholar]
- Davenport, A.G. Note on the distribution of the largest value of a random function with application to gust loading. Inst. Civ. Eng. 1964, 28, 187–196. [Google Scholar] [CrossRef]
- Massey, F.J. The Kolmogorov-Smirnov test for goodness of fit. J. Am. Stat. Assoc. 1951, 46, 68–78. [Google Scholar] [CrossRef]
- Suresh Kumar, K.; Stathopoulos, T. Wind loads on low building roofs: A stochastic perspective. J. Struct. Eng. 2000, 126, 944–956. [Google Scholar] [CrossRef]
- Ross, I.; Altman, A. Wind tunnel blockage corrections: Review and application to Savonius vertical-axis wind turbines. J. Wind Eng. Ind. Aerodyn. 2011, 99, 523–538. [Google Scholar] [CrossRef]
- Pope, A.; Harper, J.J. Low Speed Wind Tunnel Testing; John Wiley and Sons: New York, NY, USA, 1996. [Google Scholar]
- Pankhurst, R.C.; Holder, D.W. Wind-Tunnel Technique: An Account of Experimental Methods in Low-and High-Speed Wind Tunnels; Pitman: London, UK, 1952. [Google Scholar]
- Hackett, J.E.; Wilsden, D.J. Determination of low speed wake blockage corrections via tunnel wall static pressure measurements. In Proceedings of the AGARD Fluid Dynamic Panel Symposium on Wind tunnel Design and Testing Techniques, London, UK, 6–8 October 1975. [Google Scholar]
- Hackett, J.E.; Lilley, D.E.; Wilsden, D.J. Estimation of Tunnel Blockage from Wall Pressure Signatures: A Review and Data Correlation; NASA CR-15 224; Lockheed-Georgia Co: Calabasas, CA, USA, 1979. [Google Scholar]
- Hackett, J.E. Recent developments in the calculation of low-speed solidwalled wind tunnel wall interference in tests on large models part I: Evaluation of three interference assessment methods. Prog. Aerosp. Sci. 2003, 39, 537–583. [Google Scholar] [CrossRef]
- Ashill, P.R.; Weeks, D.J. A Method of Determining Wall Interference Corrections in Solid-Wall Tunnels from Measurements of Static Pressure at the Walls; AGARD-CP-335; Nato: Washington, DC, USA, 1982. [Google Scholar]
- Maskell, E.C. A Theory of the Blockage Effects on Bluff Bodies and Stalled Wings in a Closed Wind Tunnel; ARC R and M 3400; The Stationery Office: London, UK, 1965. [Google Scholar]
- Alexander, A.J. Wind tunnel corrections for Savonius rotors. In Proceedings of the Second International Symposium on Wind Energy Systems, Paper E6, Amsterdam, The Netherlands, 3–6 October 1978; pp. 69–80. [Google Scholar]
- Hensel, R.W. Rectangular-wind-tunnel blocking corrections using the velocity ratio method. In NACA TN 2372; NACA: Washington, DC, USA, 1951. [Google Scholar]



| h1 | h2 | d1 | b1 | d2 | b2 | |
|---|---|---|---|---|---|---|
| (mm) | (mm) | (mm) | (mm) | (mm) | (mm) | |
| Mod01 | 13 | 27 | 21 | 250 | 100 | 96 |
| Mod02 | 13 | 27 | 21 | 250 | 46 | 204 |
(°) | (m/s) | (m/s) | ||||||
|---|---|---|---|---|---|---|---|---|
| Set | Set | |||||||
| #1 | #2 | #3 | #4 | #1 | #2 | #3 | #4 | |
| −10 | 8.33 | 14.76 | 21.05 | 26.52 | 0.13 | 0.22 | 0.31 | 0.30 |
| −8 | 8.45 | 14.86 | 21.29 | 26.70 | 0.15 | 0.22 | 0.31 | 0.32 |
| −6 | 8.42 | 14.98 | 21.30 | 26.78 | 0.12 | 0.24 | 0.26 | 0.34 |
| −4 | 8.57 | 15.12 | 21.57 | 26.98 | 0.17 | 0.25 | 0.27 | 0.33 |
| −3 | 8.64 | 15.14 | 21.59 | 27.13 | 0.14 | 0.22 | 0.29 | 0.37 |
| −2 | 8.53 | 15.11 | 21.54 | 27.19 | 0.15 | 0.22 | 0.28 | 0.32 |
| −1 | 8.58 | 15.23 | 21.74 | 27.29 | 0.17 | 0.22 | 0.28 | 0.31 |
| 0 | 8.79 | 15.23 | 21.77 | 27.44 | 0.16 | 0.21 | 0.28 | 0.34 |
| 1 | 8.73 | 15.40 | 21.85 | 27.34 | 0.18 | 0.23 | 0.28 | 0.31 |
| 2 | 8.65 | 15.37 | 21.88 | 27.48 | 0.14 | 0.21 | 0.29 | 0.33 |
| 3 | 8.82 | 15.46 | 21.96 | 27.69 | 0.19 | 0.20 | 0.29 | 0.29 |
| 4 | 8.87 | 15.43 | 21.86 | 27.56 | 0.15 | 0.21 | 0.22 | 0.26 |
| 6 | 8.76 | 15.48 | 22.01 | 27.71 | 0.13 | 0.19 | 0.24 | 0.25 |
| 8 | 8.85 | 15.58 | 22.18 | 27.88 | 0.14 | 0.19 | 0.22 | 0.22 |
| 10 | 8.90 | 15.76 | 22.33 | 28.12 | 0.13 | 0.21 | 0.22 | 0.24 |
(°) | ||||
|---|---|---|---|---|
| Set | ||||
| #1 | #2 | #3 | #4 | |
| −10 | 39.59 | 124.32 | 252.67 | 399.68 |
| −8 | 40.75 | 126.07 | 258.55 | 405.75 |
| −6 | 40.57 | 128.39 | 259.21 | 408.86 |
| −4 | 41.99 | 130.75 | 265.85 | 414.79 |
| −3 | 42.70 | 131.13 | 266.46 | 419.88 |
| −2 | 41.68 | 130.81 | 265.62 | 422.19 |
| −1 | 42.18 | 132.93 | 270.70 | 425.55 |
| 0 | 44.63 | 133.98 | 273.48 | 433.46 |
| 1 | 43.93 | 136.69 | 274.90 | 429.29 |
| 2 | 43.01 | 135.87 | 275.20 | 433.16 |
| 3 | 44.72 | 137.42 | 276.97 | 439.09 |
| 4 | 45.13 | 136.60 | 273.96 | 434.57 |
| 6 | 44.04 | 137.58 | 277.83 | 438.91 |
| 8 | 44.88 | 139.15 | 281.81 | 444.35 |
| 10 | 45.37 | 142.35 | 285.57 | 451.87 |
(°) | ||||
|---|---|---|---|---|
| Set | ||||
| #1 | #2 | #3 | #4 | |
| −10 | 0.10 | 0.17 | 0.26 | 0.22 |
| −8 | 0.12 | 0.18 | 0.27 | 0.26 |
| −6 | 0.08 | 0.20 | 0.20 | 0.29 |
| −4 | 0.15 | 0.22 | 0.22 | 0.27 |
| −3 | 0.11 | 0.18 | 0.24 | 0.32 |
| −2 | 0.11 | 0.17 | 0.22 | 0.26 |
| −1 | 0.14 | 0.17 | 0.23 | 0.25 |
| 0 | 0.13 | 0.17 | 0.23 | 0.28 |
| 1 | 0.15 | 0.19 | 0.22 | 0.24 |
| 2 | 0.10 | 0.17 | 0.24 | 0.27 |
| 3 | 0.17 | 0.15 | 0.24 | 0.22 |
| 4 | 0.13 | 0.16 | 0.15 | 0.19 |
| 6 | 0.09 | 0.14 | 0.18 | 0.17 |
| 8 | 0.10 | 0.13 | 0.15 | 0.14 |
| 10 | 0.10 | 0.16 | 0.16 | 0.16 |
(°) | Set | |||
|---|---|---|---|---|
| #1 | #2 | #1 | #2 | |
| −10 | 0 | 0 | 1 | 0 |
| −8 | 0 | 0 | 1 | 0 |
| −6 | 0 | 1 | 0 | 1 |
| −4 | 1 | 1 | 0 | 1 |
| −3 | 0 | 0 | 0 | 1 |
| −2 | 0 | 0 | 0 | 0 |
| −1 | 0 | 1 | 0 | 0 |
| 0 | 0 | 0 | 1 | 1 |
| 1 | 1 | 0 | 0 | 0 |
| 2 | 0 | 0 | 0 | 0 |
| 3 | 1 | 1 | 1 | 0 |
| 4 | 1 | 0 | 0 | 0 |
| 6 | 0 | 0 | 0 | 0 |
| 8 | 0 | 0 | 0 | 1 |
| 10 | 0 | 0 | 1 | 0 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rizzo, F. Sensitivity Investigation on the Pressure Coefficients Non-Dimensionalization. Infrastructures 2021, 6, 53. https://doi.org/10.3390/infrastructures6040053
Rizzo F. Sensitivity Investigation on the Pressure Coefficients Non-Dimensionalization. Infrastructures. 2021; 6(4):53. https://doi.org/10.3390/infrastructures6040053
Chicago/Turabian StyleRizzo, Fabio. 2021. "Sensitivity Investigation on the Pressure Coefficients Non-Dimensionalization" Infrastructures 6, no. 4: 53. https://doi.org/10.3390/infrastructures6040053
APA StyleRizzo, F. (2021). Sensitivity Investigation on the Pressure Coefficients Non-Dimensionalization. Infrastructures, 6(4), 53. https://doi.org/10.3390/infrastructures6040053






