Sensitivity Assessment of the Seismic Response of a Masonry Palace via Non-Linear Static Analysis: A Case Study in L’Aquila (Italy)
Abstract
:1. Introduction
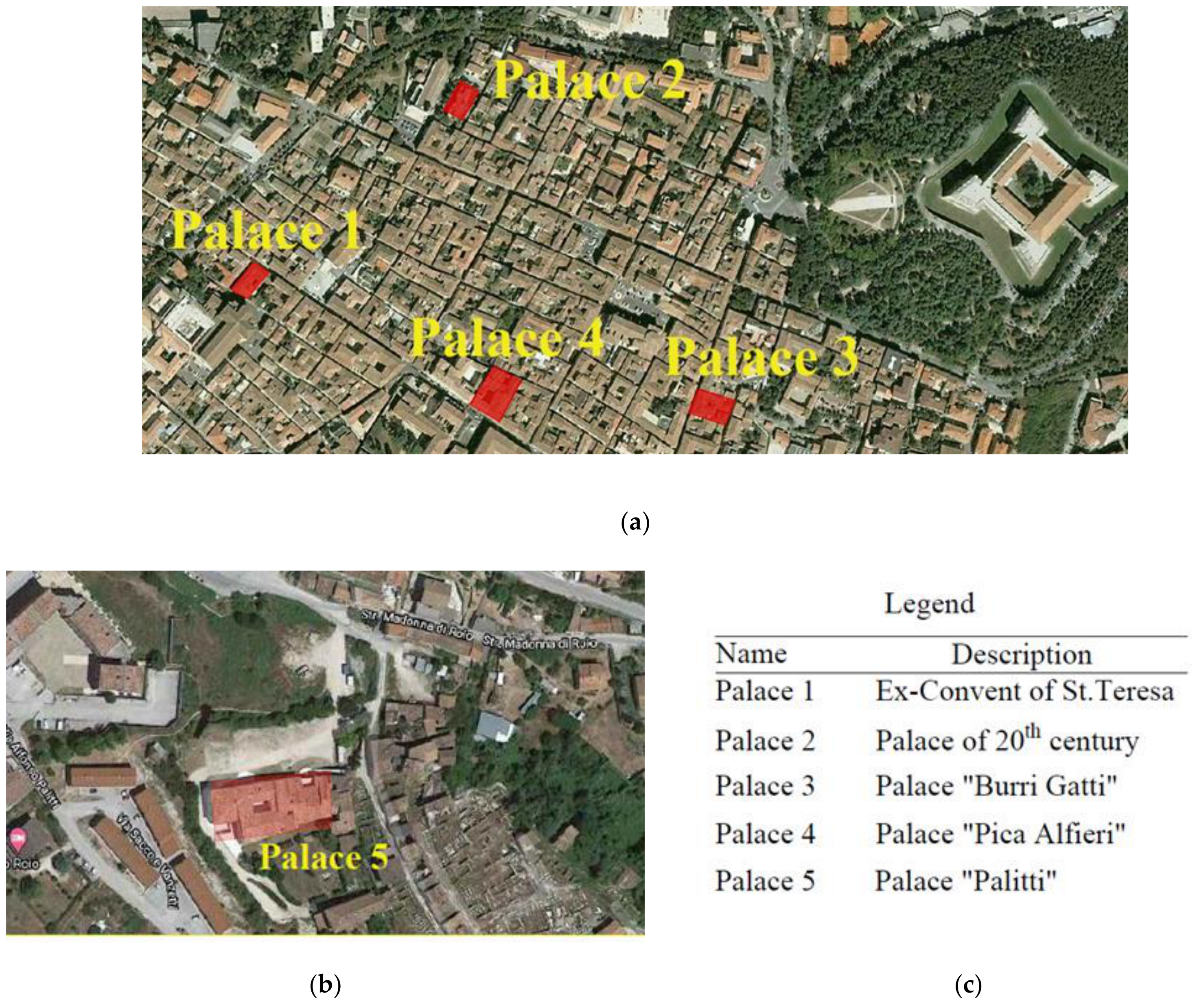
2. Research Significance
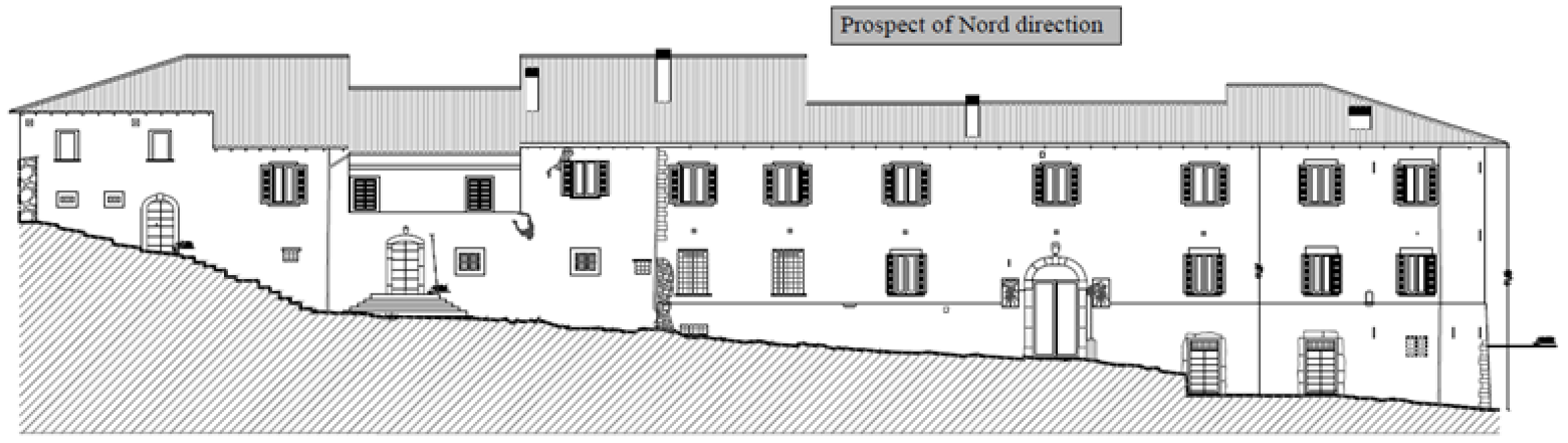
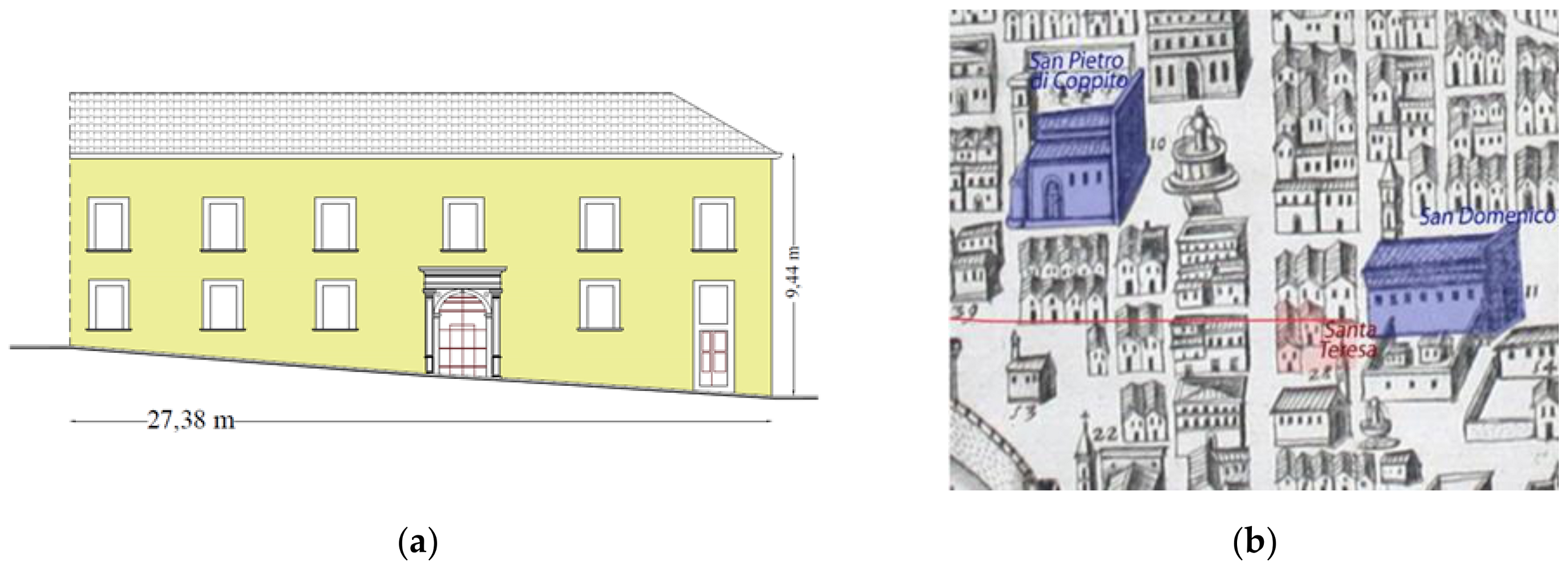
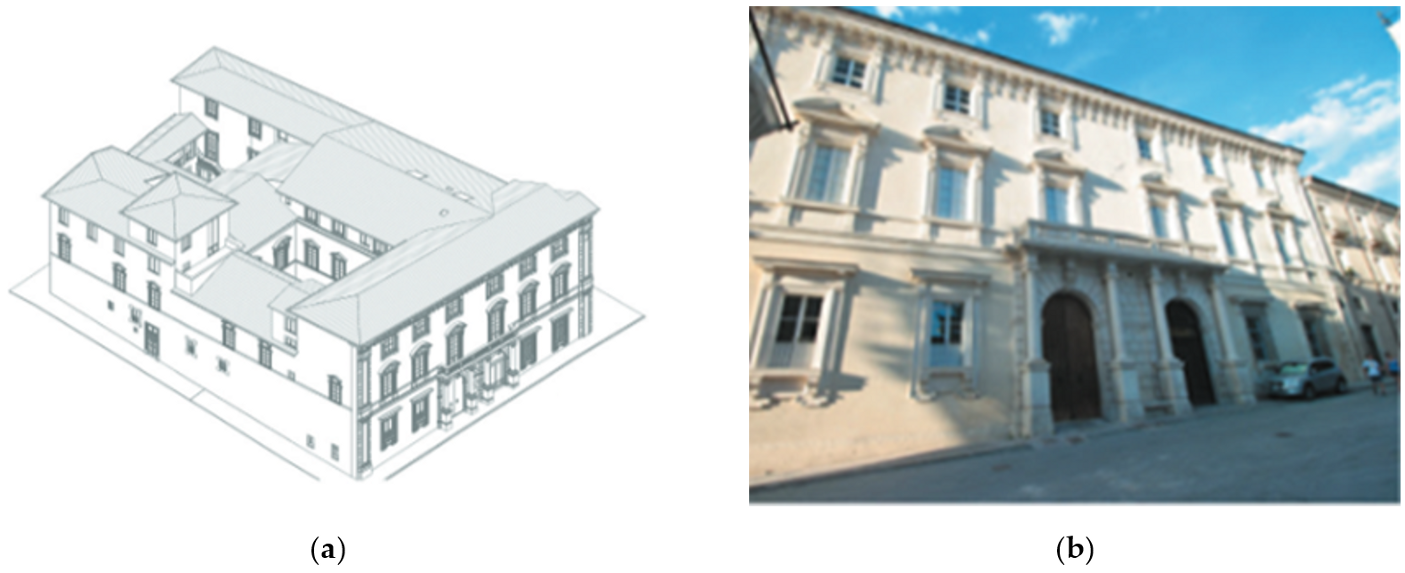
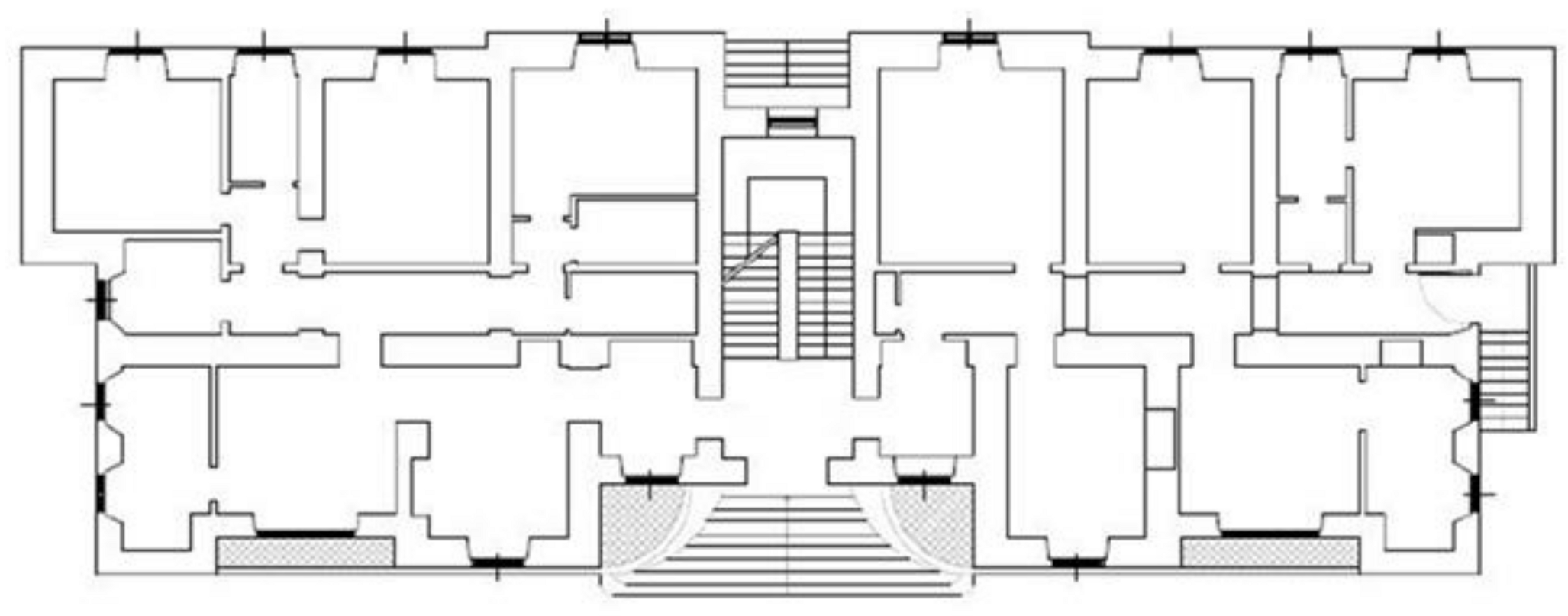
3. The Case Study: Palace 2
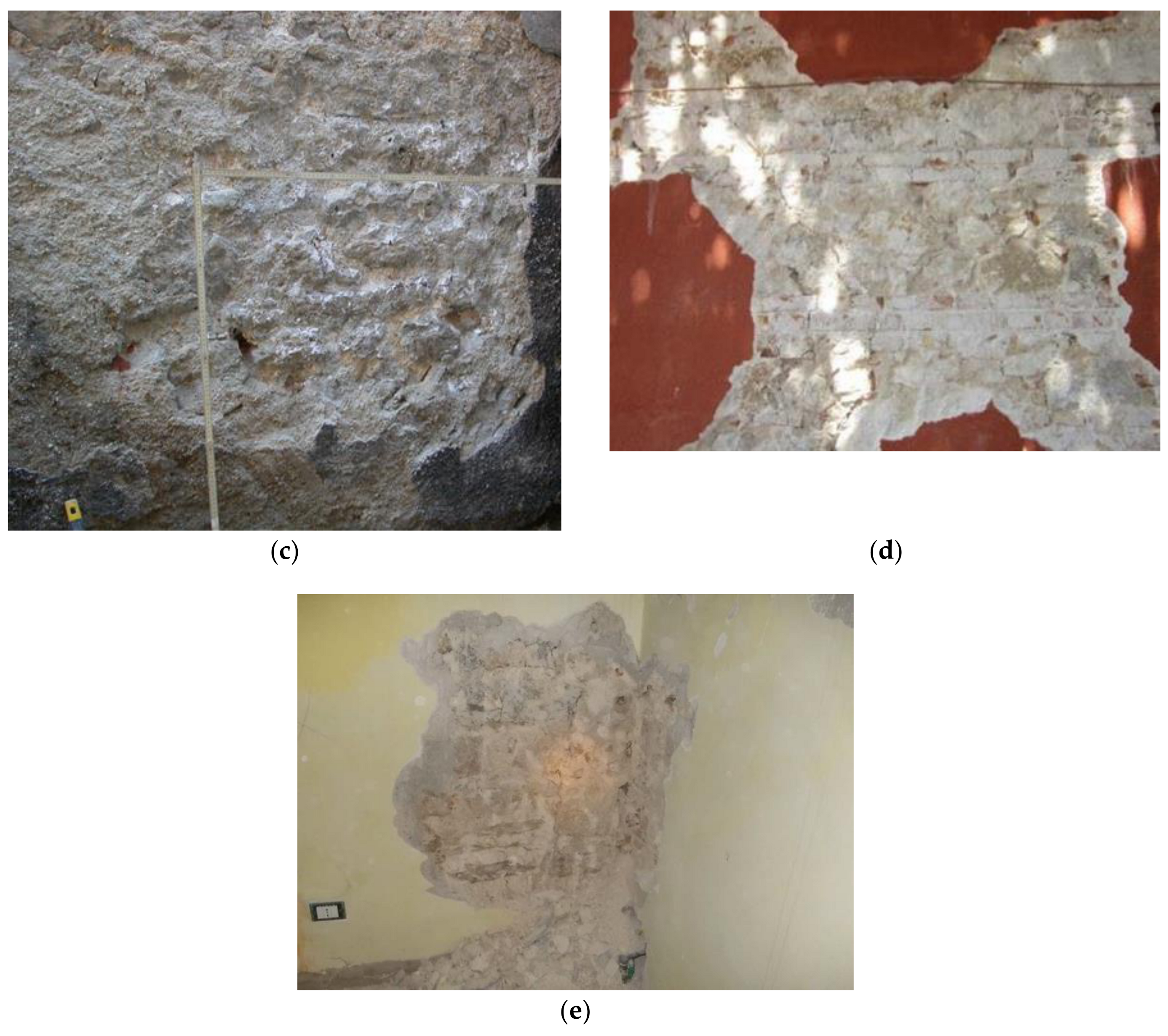
Seismic Response after the 2009 Earthquake in L’Aquila
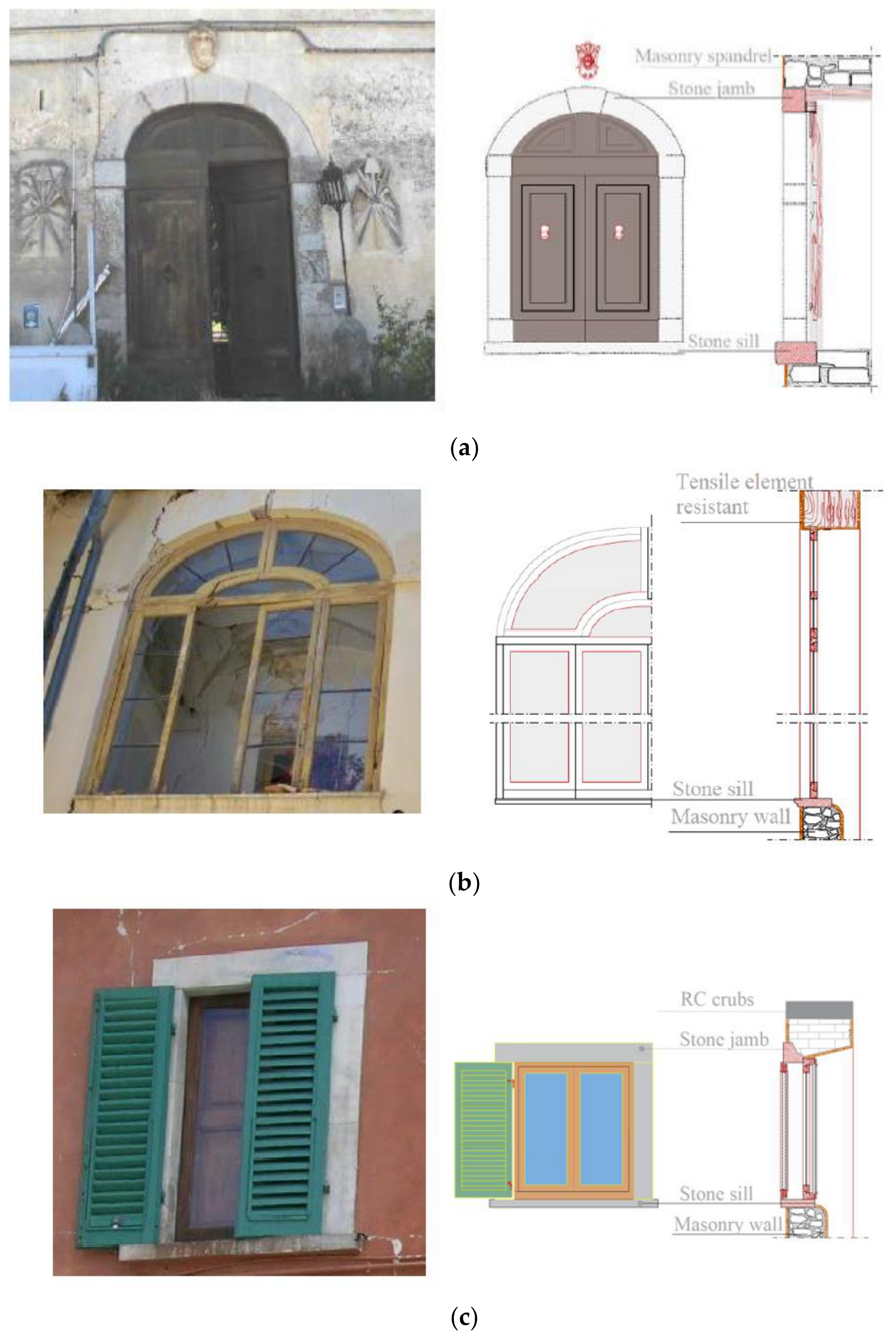
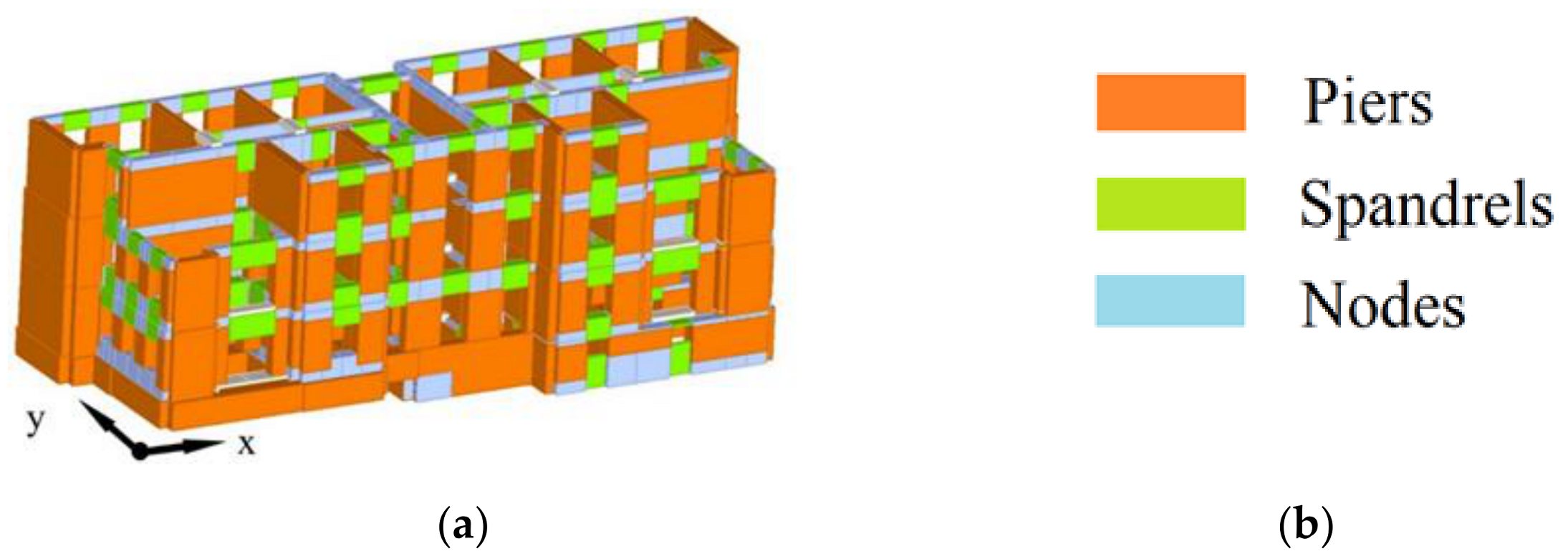
4. Numerical Analyses
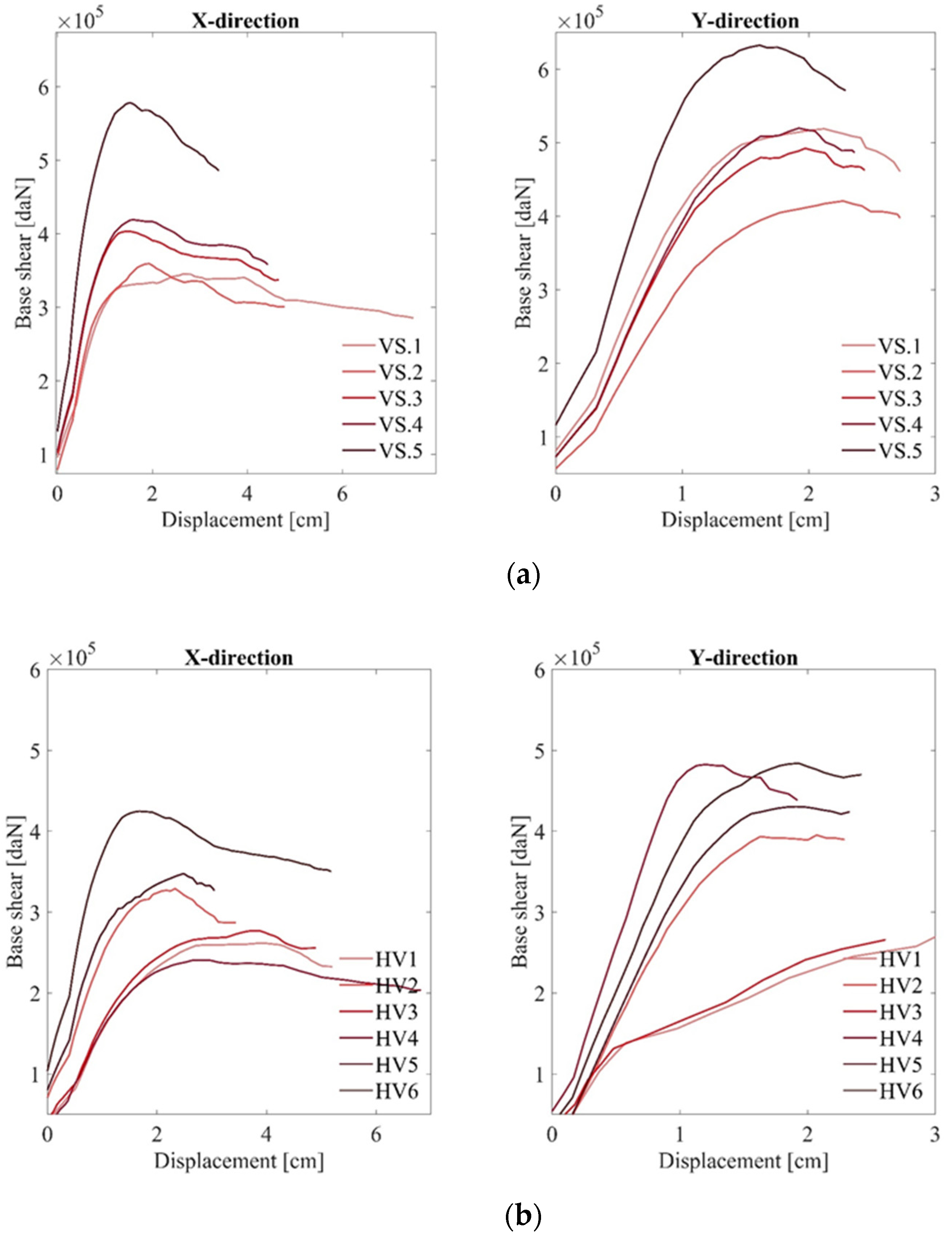
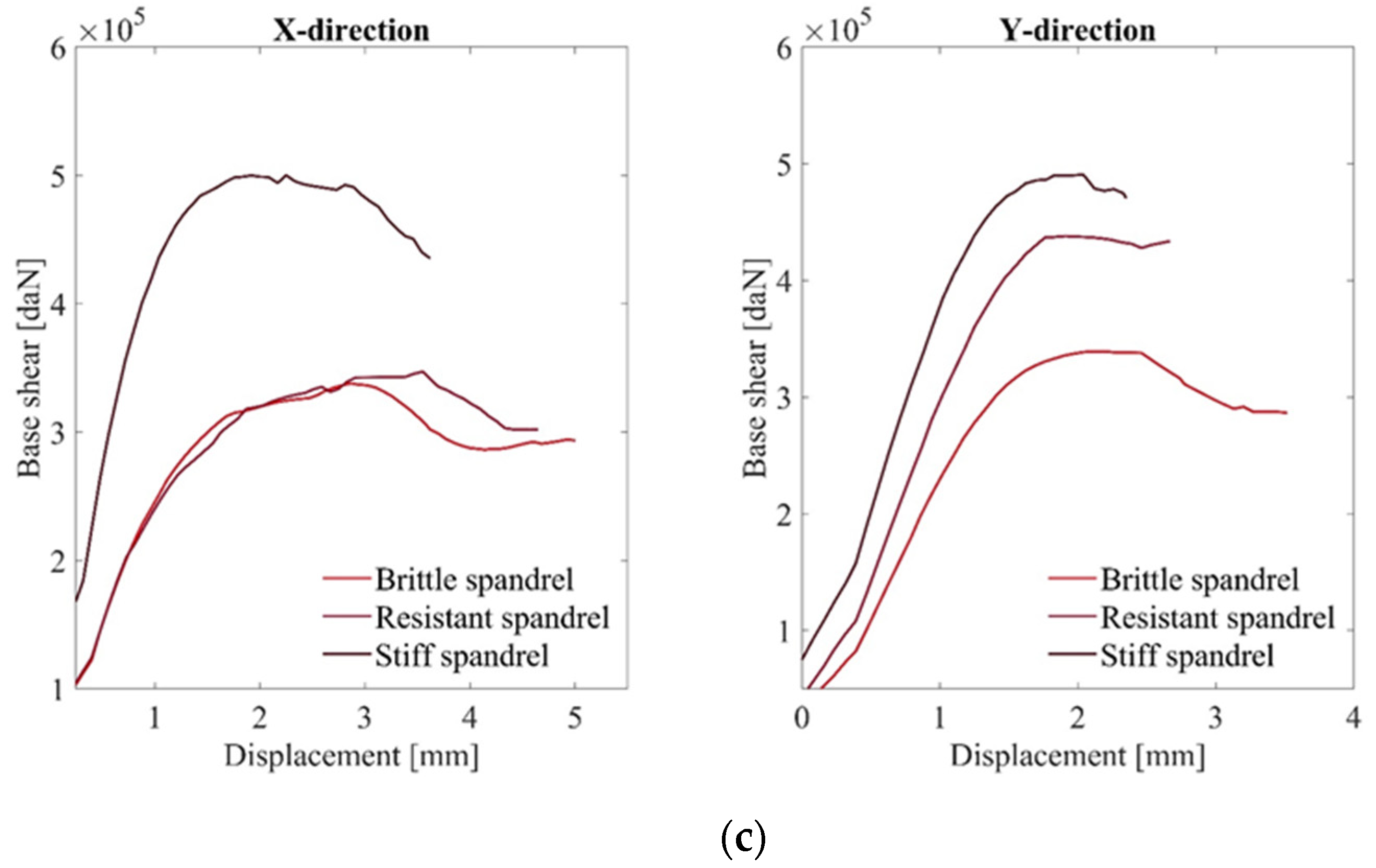
5. Parametric Study
- The variation in the floor structure’s mechanical properties does not cause a manifest variation of the behavior factors. Conversely, the behavior factors are more sensitive to the masonry’s mechanical properties: increasing values of strength reduce the behavior factors, which attain the value of 3 in the case with the most considerable compression strength. Likewise, the behavior factors are not markedly affected by the spandrel properties. However, ring beams cause a notable reduction in the behavior factor, whose value nearly attains 3.
- The ultimate displacement capacity is hardly sensitive to the floors’ in-plane stiffness, ranging between 5 and 4 cm in the x-direction, and approaching 3 cm in the y-direction. Accordingly, the masonry compression strength does not cause significant effects on the ultimate displacement, except for the case with lower resistance, which attains values higher than 9 and 4 cm in the x- and y-direction, respectively. The spandrels’ properties significantly affect the structural response in the last case, as it maximizes the coupling between the supporting walls. The ultimate displacement almost attains the value of 3—the more robust the spandrels, the lower the ultimate displacement capacity.
- The blue sets of histograms illustrate the structural parameters’ impact on the ultimate strength capacity. The stiffer and more resistant are the floors, the larger the shear capacity. Similarly, effective spandrels and more resistant supporting walls cause an increment in the base shear forces.
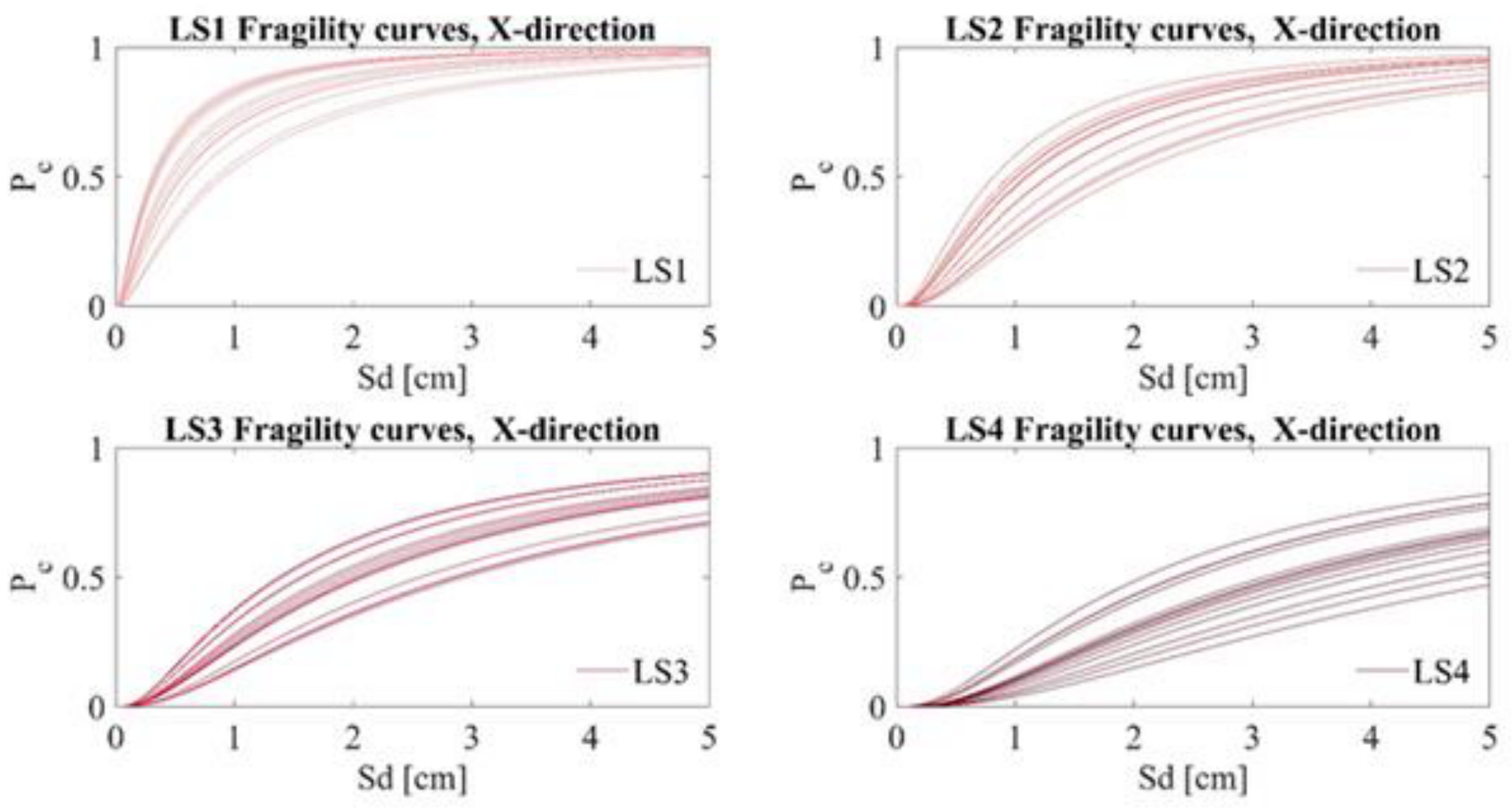
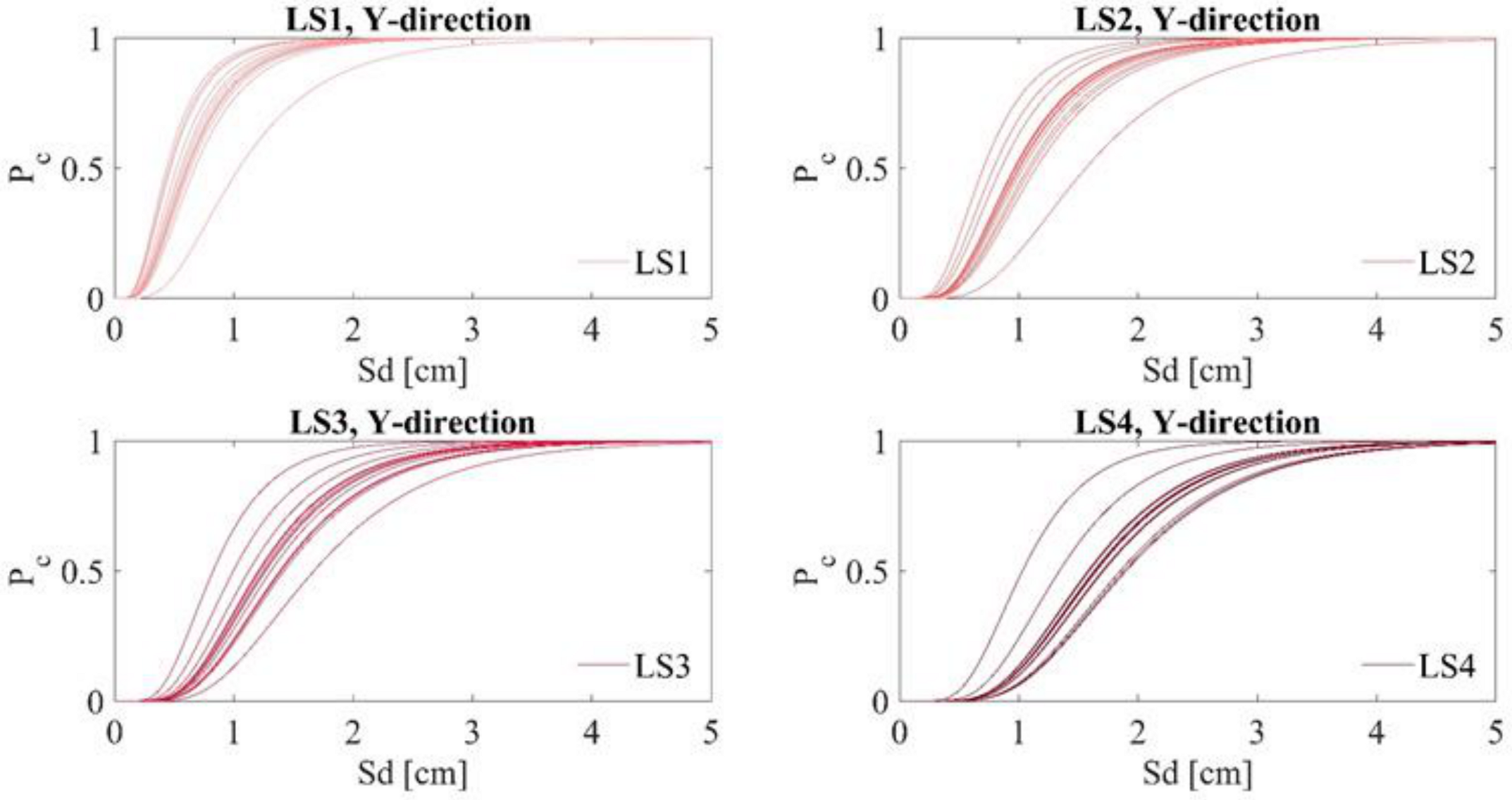
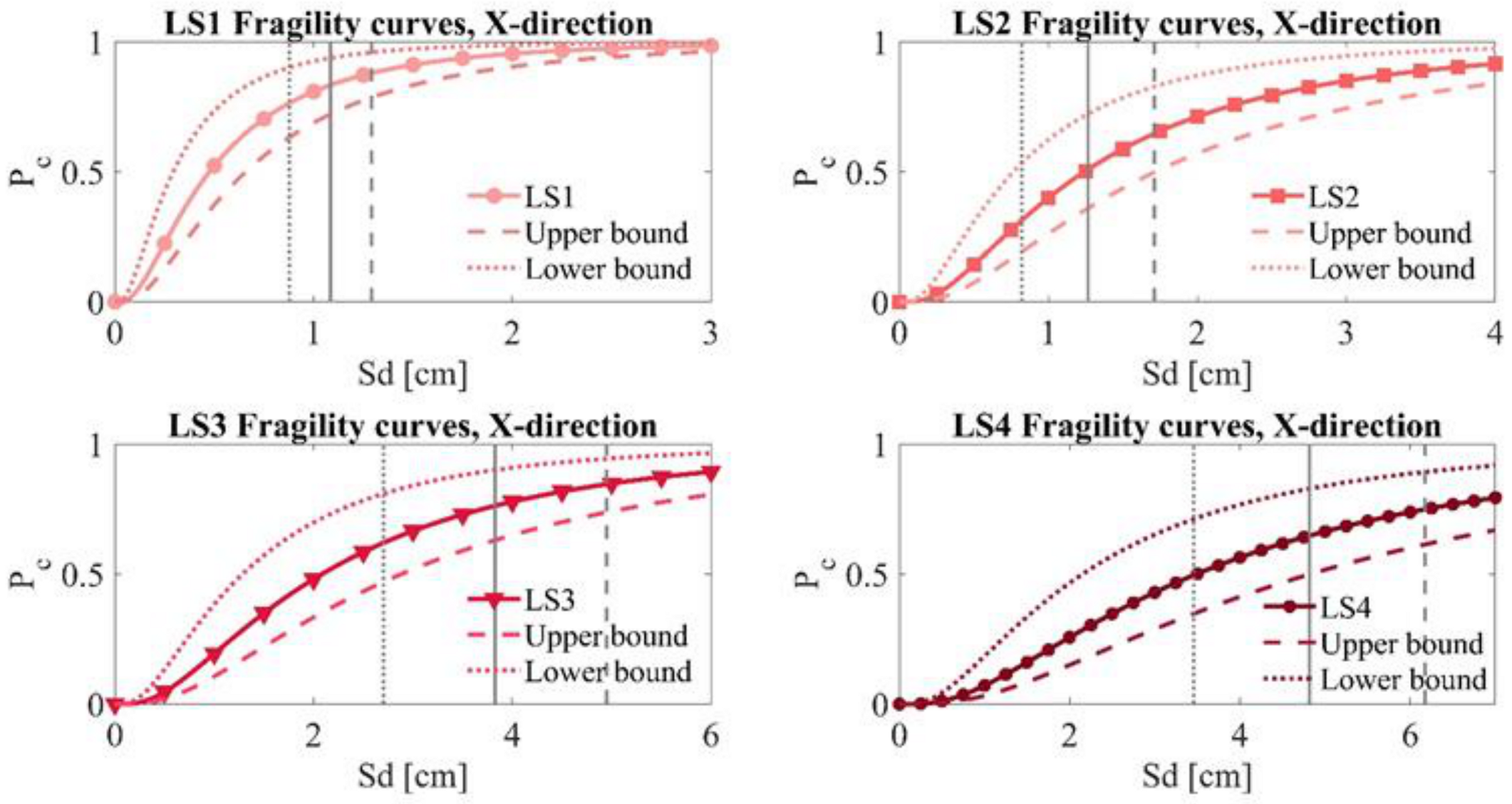
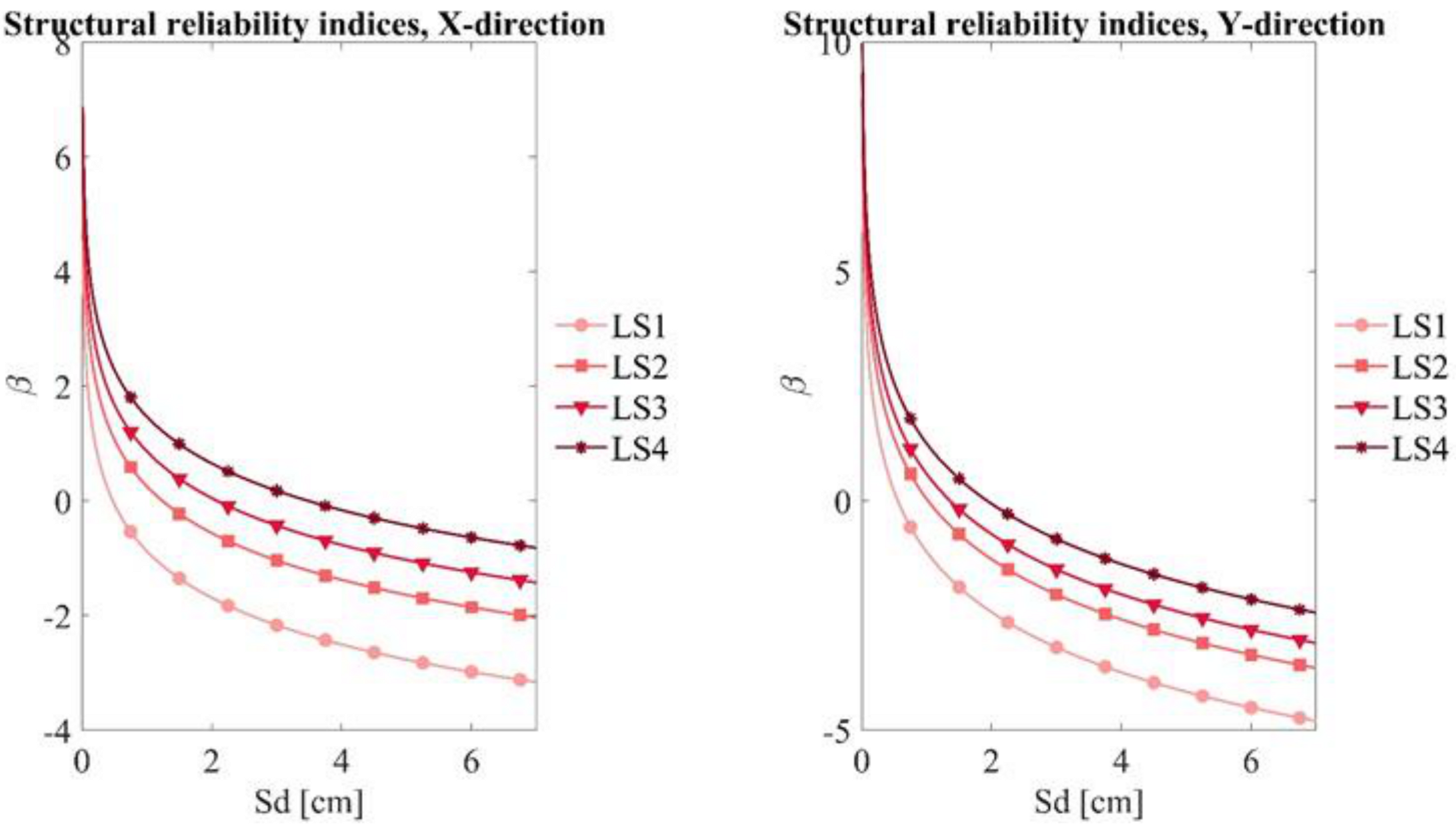
6. Derivation of the Fragility Curves
7. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Acknowledgments
Conflicts of Interest
References
- Maio, R.; Vicente, R.; Formisano, A.; Varum, H. Seismic vulnerability of building aggregates through hybrid and indirect assessment techniques. Bull. Earthq. Eng. 2015, 13, 2995–3014. [Google Scholar] [CrossRef] [Green Version]
- Brando, G.; De Matteis, G.; Spacone, E. Predictive model for the seismic vulnerability assessment of small historic centres: Application to the inner Abruzzi Region in Italy. Eng. Struct. 2017, 153, 81–96. [Google Scholar] [CrossRef]
- Rapone, D.; Brando, G.; Spacone, E.; De Matteis, G. Seismic vulnerability assessment of historic centres: Description of a predictive method and application to the case study of Scanno (Abruzzi, Italy). Int. J. Archit. Herit. 2018, 12, 1171–1195. [Google Scholar] [CrossRef]
- D’Ayala, D.F.; Paganoni, S. Assessment and analysis of damage in L’Aquila historic city center after 6th April 2009. Bull. Earthq. Eng. 2011, 9, 81–104. [Google Scholar] [CrossRef]
- Sorrentino, L.; Liberatore, L.; Decanini, L.; Liberatore, D. The performance of churches in the 2012 Emilia Earthquakes. Bull. Earthq. Eng. 2012, 12, 2299–2331. [Google Scholar] [CrossRef]
- Ferreira, T.M.; Vicente, R.; Mendes Da Silva, J.A.R.; Varum, H.; Costa, A. Seismic vulnerability assessment of historical urban centres: Case study of the old city centre in Seixal, Portugal. Bull. Earthq. Eng. 2013, 11, 1735–1773. [Google Scholar] [CrossRef]
- Dolce, M.; Goretti, A. Building damage assessment after the 2009 Abruzzi earthquake. Bull. Earthq. Eng. 2015, 13, 2241–2264. [Google Scholar] [CrossRef]
- Zuccaro, G.; Cacace, F. Seismic vulnerability assessment based on typological characteristics. The first level procedure “SAVE”. Soil Dyn. Earthq. Eng. 2015, 69, 262–269. [Google Scholar] [CrossRef]
- Sorrentino, L.; Cattari, S.; da Porto, F.; Magenes, G.; Penna, A. Seismic behaviour of ordinary masonry buildings during the 2016 central Italy earthquakes. Bull. Earthq. Eng. 2019, 17, 5583–5607. [Google Scholar] [CrossRef] [Green Version]
- Calderoni, B.; Cordasco, E.; Leanza, P. Il ruolo della fascia di piano nel comportamento sismico degli edifici in muratura. Ingegneria Sismica 2007, 24, 26. [Google Scholar]
- Rizzano, G.; Sabatino, R.; Zambrano, M. L’influenza delle fasce di piano sulla resistenza di pareti in muratura. In Proceedings of the 13th Italian Conference on Earthquake Engineering, Bologna, Italy, 28 June–2 July 2009. [Google Scholar]
- Rinaldin, G.; Amadio, C.; Gattesco, N. Experimental and numerical characterization of the cyclic behaviour of unreinforced and reinforced masonry spandrels. In Proceedings of the 9th International Masonry Conference, Guimarăes, Portugal, 7–9 July 2014. [Google Scholar]
- Senaldi, I.; Magenes, G.; Penna, A.; Galasco, A.; Rota, M. The effect of stiffened floor and roof diaphragms on the experimental seismic response of a full scale unreinforced stone masonry building. J. Earthq. Eng. 2014, 18, 3. [Google Scholar] [CrossRef]
- De Felice, G.; De Santis, S.; Lourenco, P.B.; Mendes, N. Methods and challenges for the seismic assessment of historic masonry structures. Int. J. Archit. Herit. 2017, 11, 143–160. [Google Scholar] [CrossRef] [Green Version]
- Ceci, A.; Contento, A.; Fanale, L.; Galeota, D.; Gattulli, V.; Lepidi, M.; Potenza, F. Structural performance of the historic and modern buildings of the University of L’Aquila during the seismic events of April 2009. Eng. Struct. 2010, 32, 1899–1924. [Google Scholar] [CrossRef]
- Indirli, M.; Kouris Leonidas, A.S.; Formisano, A.; Borg Ruben, P.; Mazzolani, F.M. Seismic damage assessment of unreinforced masonry structures after the Abruzzo 2009 earthquake: The case study of the historical centers of L’Aquila and Castelvecchio Calvisio. Int. J. Archit. Herit. 2012, 7, 536–578. [Google Scholar] [CrossRef]
- Lucibello, G.; Brandonisio, G.; Mele, E.; Antonello, L. Seismic damage and performance of Palazzo Centi after L’Aquila earthquake: A paradigmatic case study of effectiveness of mechanical steel ties. Eng. Fail. Anal. 2013, 34, 407–430. [Google Scholar] [CrossRef]
- Cannizzaro, F.; Pantò, B.; Lepidi, M.; Caddemi, S.; Caliò, I. Multi-directional seismic assessment of historical masonry buildings by means of macro-element modelling: Application to a building damaged during the L’Aquila earthquake (Italy). Buildings 2017, 7, 106. [Google Scholar] [CrossRef] [Green Version]
- Formisano, A.; Krstevska, L.; Di Lorenzo, G.; Landolfo, R.; Tashkov, L. Experimental ambient vibration tests and numerical investigation on the Sidoni Palace in Castelnuovo of San Pio (L’Aquila, Italy). IJMRI 2018, 3, 269. [Google Scholar] [CrossRef]
- Del Gaudio, C.; De Martino, G.; Di Ludovico, M. Empirical fragility curves for masonry buildings after the 2009 L’Aquila, Italy, earthquake. Bull. Earthq. Eng. 2019, 17, 6301–6330. [Google Scholar] [CrossRef]
- Aloisio, A.; Fragiacomo, M.; D’Alò, G. The 18th-century baraccato of l’aquila. Int. J. Archit. Heritage 2019, 14, 1–15. [Google Scholar] [CrossRef]
- Aloisio, A.; Alaggio, R.; Fragiacomo, M. The architrave a tasselli. Case Stud. Constr. Mater. 2019, 11, e00252. [Google Scholar] [CrossRef]
- Aloisio, A.; Fragiacomo, M.; D’Alò, G. Traditional TF masonries in the city centre of L’Aquila–The baraccato aquilano. Int. J. Archit. Heritage 2019, 1–18. [Google Scholar] [CrossRef]
- Formisano, A. Theoretical and numerical seismic analysis of masonry building aggregates: Case studies in San Pio Delle Camere (L’Aquila, Italy). J. Earthq. Eng. 2017, 21, 227–245. [Google Scholar] [CrossRef] [Green Version]
- Aloisio, A.; Capanna, I.; Cirella, R.; Alaggio, R.; Di Fabio, F.; Fragiacomo, M. Identification and model update of the dynamic properties of the San Silvestro belfry in L’Aquila and estimation of Bell’s Dynamic Actions. Appl. Sci. 2020, 10, 4289. [Google Scholar] [CrossRef]
- Galeota, D.; Iacovella, C.C.; Elicio, L. Miglioramento sismico e restauro architettonico: Proposte progettuali per l’ex convento di S. Teresa a L’Aquila. Ingenio 2019. (In Italian) [Google Scholar]
- Aloisio, A.; Di Pasquale, A.; Alaggio, R.; Fragiacomo, M. Assessment of seismic retrofitting interventions of a masonry palace using operational modal analysis. Int. J. Archit. Herit. 2020. [Google Scholar] [CrossRef]
- Asteris, P.G.; Moropoulou, A.; Skentou, A.; Apostolopoulou, M.; Mohebkhah, A.; Cavaleri, L.; Rodirgues, H.; Varum, H. Stochastic vulnerability assessment of masonry structures: Concepts, modeling and restoration aspects. Appl. Sci. 2019, 9, 243. [Google Scholar] [CrossRef] [Green Version]
- Asteris, P.G.; Douvika, M.G.; Apostolopoulou, M.; Moropoulou, A. Seismic and restoration assessment of monumental masonry structures. Materials 2017, 10, 895. [Google Scholar] [CrossRef] [Green Version]
- Asteris, P.G.; Chronopoulos, M.P.; Chrysostomou, C.; Varum, H.; Plevris, V.; Kyriakides, N.; Silva, V. Seismic vulnerability assessment of historical masonry structural system. Eng. Struct. 2014, 62, 118–134. [Google Scholar] [CrossRef]
- Apostolopoulou, M.; Aggelakopoulou, E.; Siouta, L.; Bakolas, A.; Douvika, M.; Asteris, P.G.; Moropoulou, A. A methodological approach for the selection of compatible and performable restoration mortars in seismic hazard areas. Constr Build. Mater. 2017, 155, 1–14. [Google Scholar] [CrossRef]
- Tzamtzis, A.; Vouthouni, P.; Sophianopoulos, D. Earthquake resistant design and rehabilitation of masonry historical structures. Pract. Period. Struct. Des. Constr. 2005, 10, 49–55. [Google Scholar] [CrossRef] [Green Version]
- Chrysostomou, C.; Demetriou, T.; Pittas, M.; Stassis, A. Retrofit of a church with linear viscous dampers. Struct. Control. Health Monit. 2005, 12, 197–212. [Google Scholar] [CrossRef]
- Ministerial Decree of Public Works. Updating of Technical codes for constructions. M.D. 17/01/2018. Official Gazette of the Italian Republic n.42, 20 February 2018. [Google Scholar]
- Ministerial Decree of Public Works. Instructions for the application of the updating. Technical codes for constructions. Official Gazette of the Italian Republic n.7, 17 January 2019. [Google Scholar]
- Brusaporci, S. Le murature nell’architettura del versante meridionale del Gran Sasso (secc. XI-XIV); Gangemi Editore: Rome, Italy, 2007. [Google Scholar]
- Milani, G.; Venturini, G. Automatic fragility curve evaluation of masonry churches accounting for partial collapses by means of 3D FE homogenized limit analysis. Comput. Struct. 2011, 89, 1628–1648. [Google Scholar] [CrossRef]
- Murat, A.E. Generation of fragility curves for Turkish masonry buildings considering in-plane failure modes. Earthq. Eng. Struct. Dyn. 2008, 37, 387–405. [Google Scholar] [CrossRef]
- Mendes, N.; Lourenco, P.B. Sensitivity analysis of the seismic performance of existing masonry buildings. Eng. Struct. 2014, 80. [Google Scholar] [CrossRef] [Green Version]
- 3MURI. Seismic Calculation of masonry structures according to M.D.14/01/2018; 3MURI: Torino, Italy, 2009. [Google Scholar]
- Asteris, P.G.; Plevris, V. Handbook of Research on Seismic Assessment and Rehabilitation of Historic Structures. In Numerical Modeling of Historic Masonry Structures; IGI Global: Hershey, PA, USA, 2015; pp. 213–256. [Google Scholar] [CrossRef]
- Gambarotta, L.; Lagomarsino, S. On dynamic response of masonry panels. In Proceedings of the Italian National Conference Masonry mechanics between theory and practice, Messina, Italy, 18–20 September 1996. [Google Scholar]
- Gambarotta, L.; Lagomarsino, S. Damage models for the seismic response of brick masonry shear walls, Part II: The continuum model and its applications. Earthq. Eng. Struct. Dyn. 1997, 26, 441–462. [Google Scholar] [CrossRef]
- Lagomarsino, S.; Penna, A.; Galasco, A.; Cattari, S. TREMURI program: An equivalent frame model for the non-linear seismic analysis of masonry buildings. Eng. Struct. 2013, 56, 1787–1799. [Google Scholar] [CrossRef]
- European Committee for Standardization (CEN). Eurocode 8: Design of Structures for Earthquake Resistance—Part 1: General Rules, Seismic Actions and Rules for Buildings (EC8-1); CEN: Brussels, Belgium, 2004. [Google Scholar]
- Magenes, G.; Calvi, G. In-plane seismic response of brick masonry walls. Earthq. Eng. Struct. Dyn. 1997, 26, 1091–1112. [Google Scholar] [CrossRef]
- Formisano, A.; Massimilla, A. A novel procedure for simplified non-linear numerical modelling of structural units in masonry aggregates. Int. J. Archit. Herit. 2018, 12, 1162–1170. [Google Scholar] [CrossRef]
- Monaco, P.; Totani, G.; Totani, F.; Grasso, S.; Maugeri, M. Site effects in the urban area of L’Aquila damaged by the April 6, 2009 earthquake. In Earthquake-Soil Interaction; WIT Press: Ashurst, UK, 2014; Volume 79, pp. 71–86. [Google Scholar] [CrossRef]
- Chopra, A.K.; Goel, R.K. Capacity-demand-diagram methods based on inelastic design spectrum. Earthq. Spectra 1999, 15, 637–656. [Google Scholar] [CrossRef]
- Lagomarsino, S.; Giovinazzi, S. Macroseismic and mechanical models for the vulnerability and damage assessment of current buildings. Bull. Earthq. Eng. 2006, 4, 415–443. [Google Scholar] [CrossRef]
- Aloisio, A.; Fragiacomo, M. Reliability-based overstrength factors of cross-laminated timber shear walls for seismic design. Eng. Struct. 2021, 228, 111547. [Google Scholar] [CrossRef]
- European Committee for Standardization (CEN). Eurocode 0: Basis of Structural Design (EC0); CEN: Brussels, Belgium, 2002. [Google Scholar]






| Masonry Typology (VS) | Compressive Strength fm (MPa) | Shear Strength τ0 (MPa) | Young Modulus E (MPa) | Shear Modulus G (MPa) | Specific Weight γ (kN/m3) |
|---|---|---|---|---|---|
| VS1 | 1.00 | 0.018 | 870 | 290 | 19 |
| VS2 | 1.30 | 0.023 | 870 | 290 | 19 |
| VS3 | 2.00 | 0.035 | 1230 | 410 | 20 |
| VS4 | 2.40 | 0.042 | 1230 | 410 | 20 |
| VS5 | 3.38 | 0.073 | 2262 | 754 | 21 |
| Limit State | ag (m/sec2) | F0 | Tc (sec) |
|---|---|---|---|
| LS1 | 0.77 | 2.40 | 0.27 |
| LS2 | 1.02 | 2.33 | 0.28 |
| LS3 | 2.56 | 2.36 | 0.35 |
| LS4 | 3.28 | 2.40 | 0.36 |
| Analysis | X-Direction | Y-Direction | ||||||
|---|---|---|---|---|---|---|---|---|
| Fy (kN) | dy (mm) | du (mm) | T (sec) | Fy (kN) | dy (mm) | du (mm) | T (sec) | |
| VS1 | 2287.29 | 0.51 | 5.37 | 0.36 | 3498.54 | 0.74 | 1.88 | 0.36 |
| VS2 | 2330.65 | 0.51 | 3.37 | 0.36 | 2845.3 | 0.87 | 1.90 | 0.43 |
| VS3 | 2656.49 | 0.45 | 3.27 | 0.32 | 3363.95 | 0.82 | 1.70 | 0.32 |
| VS4 | 2794.00 | 0.48 | 3.11 | 0.32 | 3582.58 | 0.88 | 1.65 | 0.39 |
| VS5 | 3814.00 | 0.43 | 2.37 | 0.26 | 4335.00 | 0.67 | 1.58 | 0.32 |
| HS1 | 2108.60 | 1.3 | 4.39 | 0.57 | 2224.60 | 1.67 | 1.97 | 0.61 |
| HS2 | 2207.50 | 0.79 | 2.36 | 0.42 | 2617.30 | 0.82 | 1.59 | 0.40 |
| HS3 | 2097.30 | 1.21 | 3.93 | 0.54 | 2165.30 | 1.49 | 1.85 | 0.59 |
| HS4 | 1563.80 | 0.93 | 4.79 | 0.56 | 3289.60 | 0.65 | 1.34 | 0.33 |
| HS5 | 2326.61 | 0.67 | 2.08 | 0.39 | 2887.16 | 0.85 | 1.63 | 0.40 |
| HS6 | 2757.77 | 0.47 | 3.65 | 0.33 | 3272.90 | 0.82 | 1.71 | 0.40 |
| SP1 | 2185.15 | 0.73 | 3.46 | 0.43 | 2931.84 | 0.91 | 1.88 | 0.43 |
| SP2 | 2302.11 | 0.80 | 3.23 | 0.44 | 3008.04 | 0.96 | 1.88 | 0.43 |
| SP3 | 3403.50 | 0.63 | 2.50 | 0.32 | 3366.30 | 0.79 | 1.64 | 0.37 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Capanna, I.; Aloisio, A.; Di Fabio, F.; Fragiacomo, M. Sensitivity Assessment of the Seismic Response of a Masonry Palace via Non-Linear Static Analysis: A Case Study in L’Aquila (Italy). Infrastructures 2021, 6, 8. https://doi.org/10.3390/infrastructures6010008
Capanna I, Aloisio A, Di Fabio F, Fragiacomo M. Sensitivity Assessment of the Seismic Response of a Masonry Palace via Non-Linear Static Analysis: A Case Study in L’Aquila (Italy). Infrastructures. 2021; 6(1):8. https://doi.org/10.3390/infrastructures6010008
Chicago/Turabian StyleCapanna, Ilaria, Angelo Aloisio, Franco Di Fabio, and Massimo Fragiacomo. 2021. "Sensitivity Assessment of the Seismic Response of a Masonry Palace via Non-Linear Static Analysis: A Case Study in L’Aquila (Italy)" Infrastructures 6, no. 1: 8. https://doi.org/10.3390/infrastructures6010008
APA StyleCapanna, I., Aloisio, A., Di Fabio, F., & Fragiacomo, M. (2021). Sensitivity Assessment of the Seismic Response of a Masonry Palace via Non-Linear Static Analysis: A Case Study in L’Aquila (Italy). Infrastructures, 6(1), 8. https://doi.org/10.3390/infrastructures6010008






