Analysis of Oil-Injected Twin-Screw Compressor with Multiphase Flow Models
Abstract
1. Introduction
- How is Fluent solver customised to solve for oil-injected twin screw compressor?
- Are pseudo-SFM models capable of predicting integral performance comparable to experimental data?
- How is the oil distributed within the compression chamber for various multiphase flow models and what is its effect on integral performance?
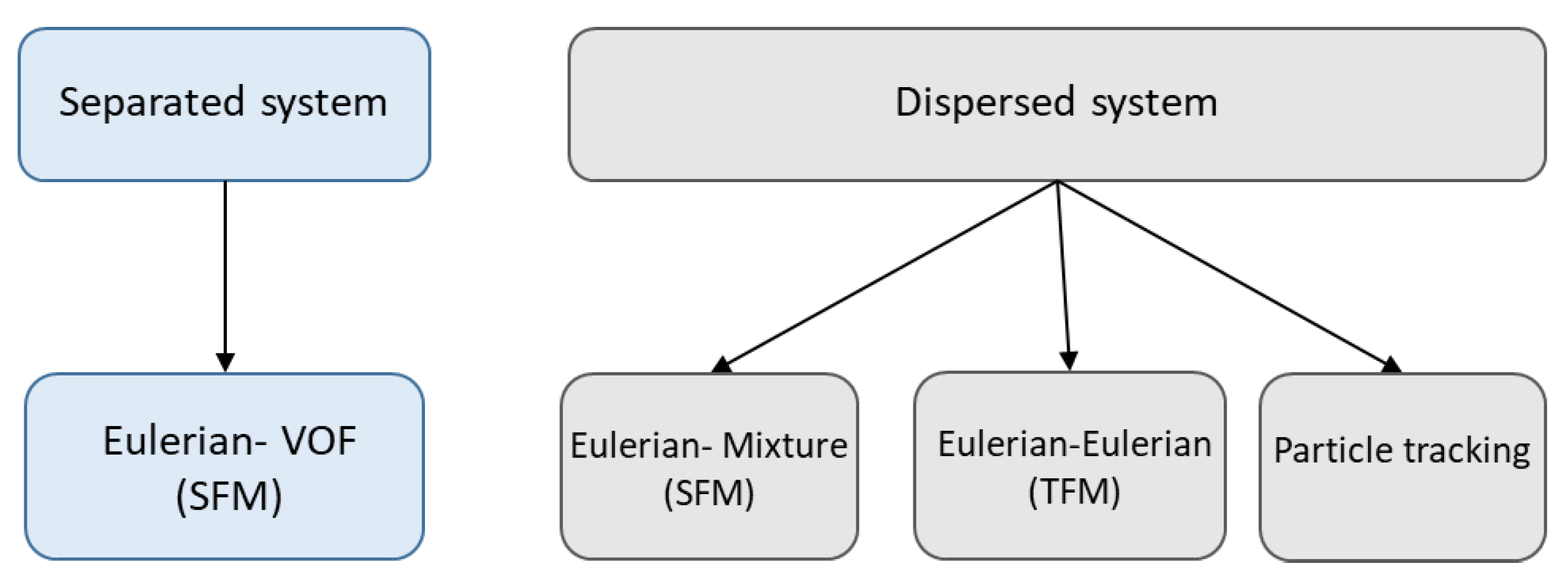
2. Multiphase Flow Modelling
- i.
- Binary fluids with high-density ratios
- ii.
- Compressible fluids
- iii.
- Mesh quality
2.1. Governing Equations
2.1.1. Eulerian-Eulerian Model
2.1.2. VOF Model
2.1.3. Mixture Model
- Including the additional term for the interphase momentum transfer in the momentum Equation (16).
- Treating the phases to be interpenetrating continua similar to the Eulerian-Eulerian model.
2.2. Comparison of Computational Multiphase Flow Models
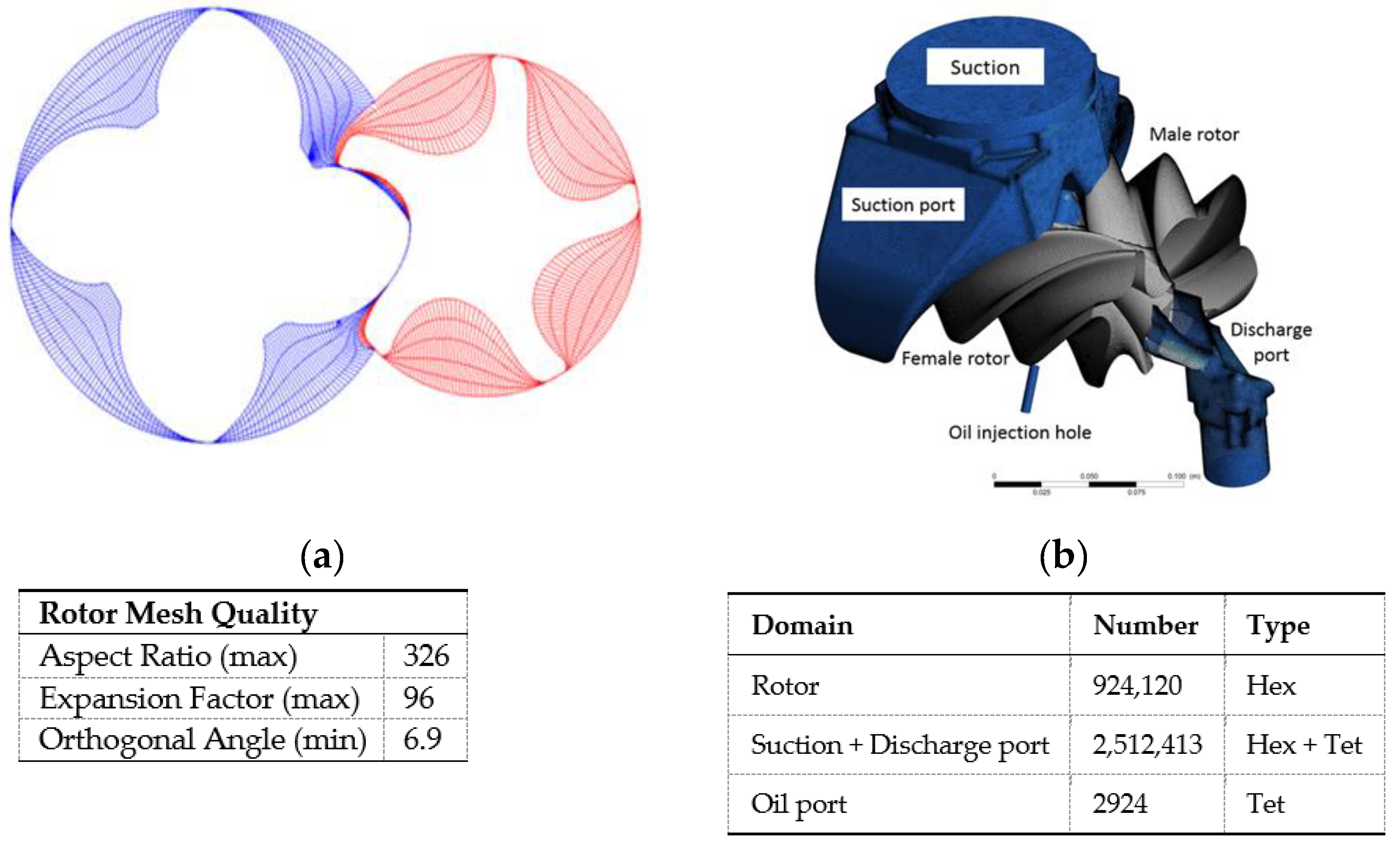
3. Case Study
3.1. Grids
3.2. Interface with Fluent
3.3. Numerical Set-Up
4. Results and Discussions
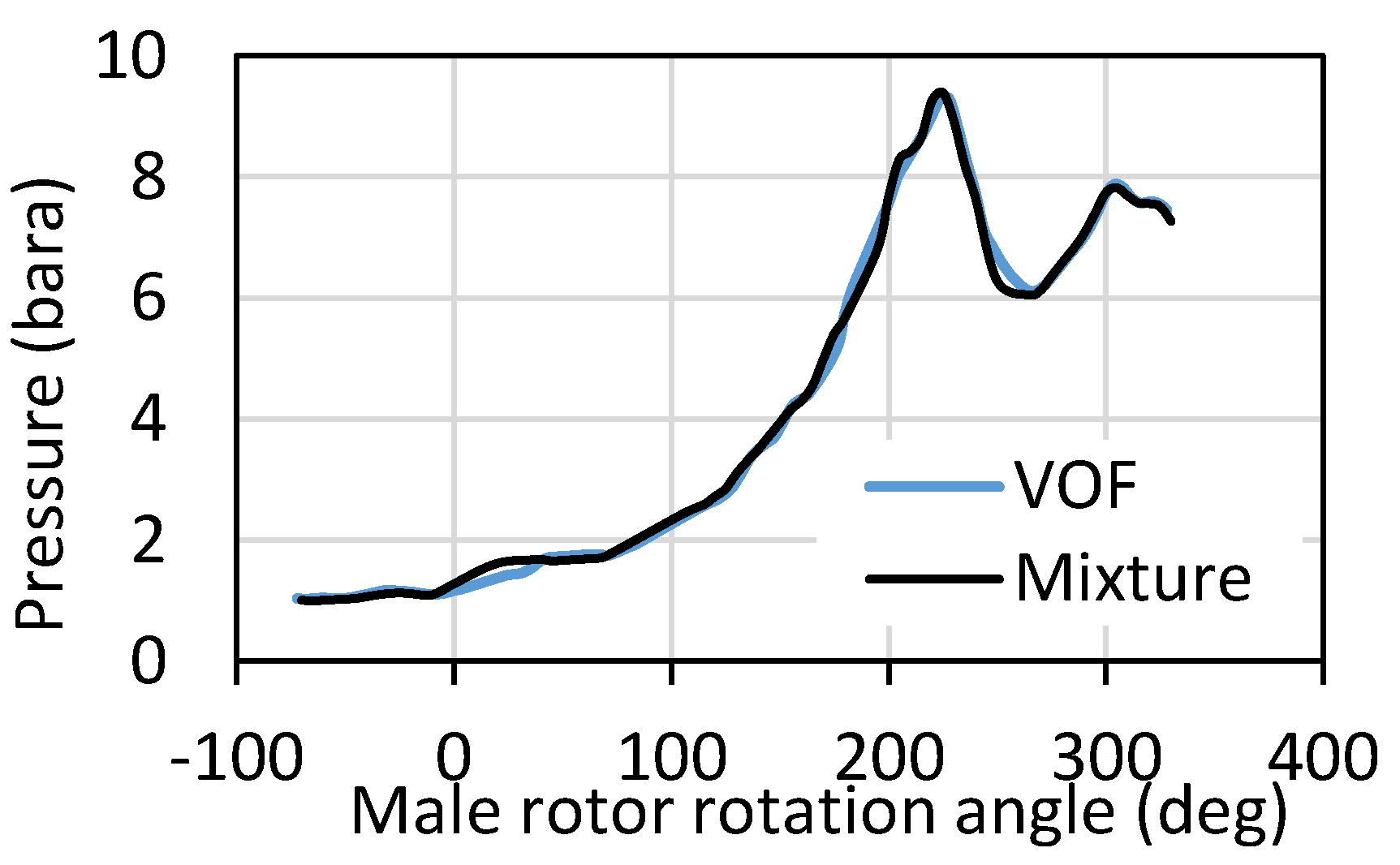
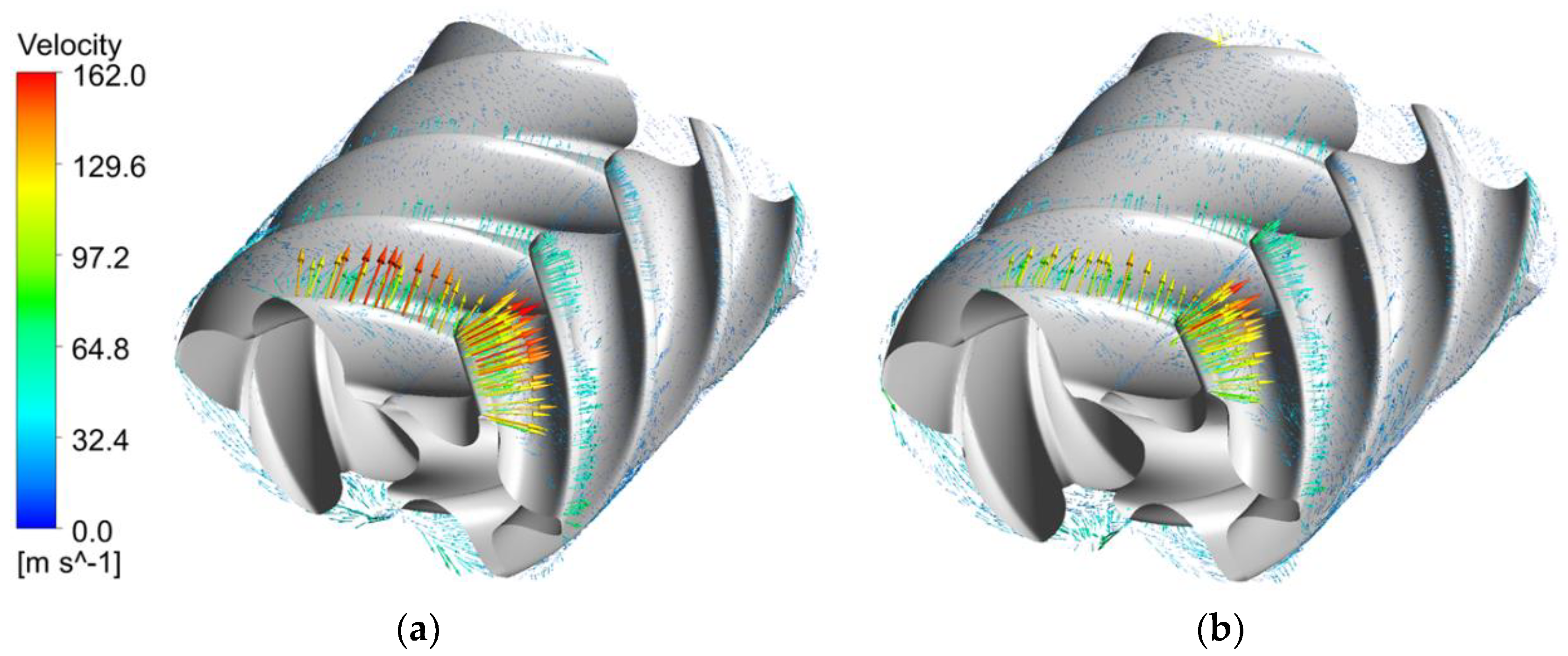
4.1. Pressure Distribution
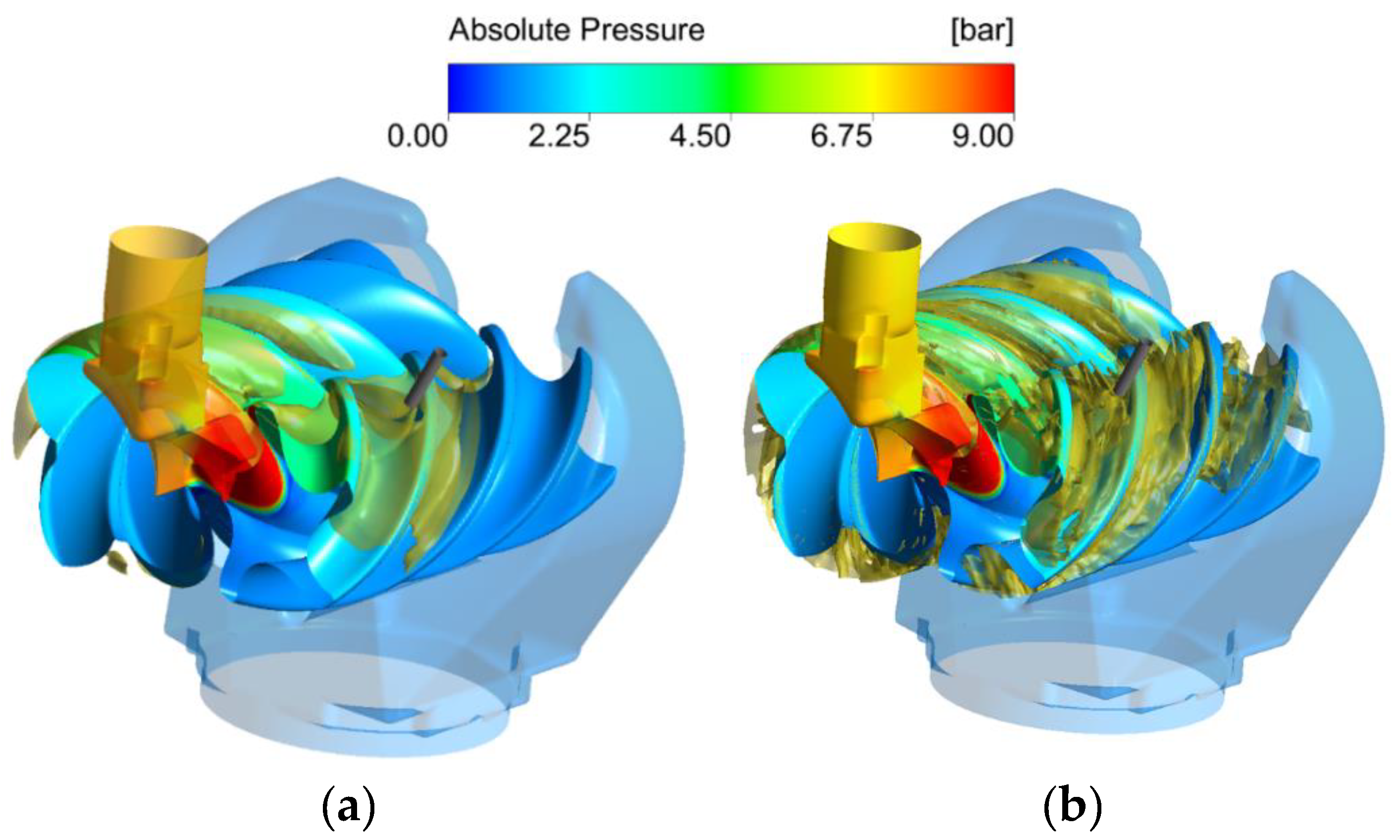
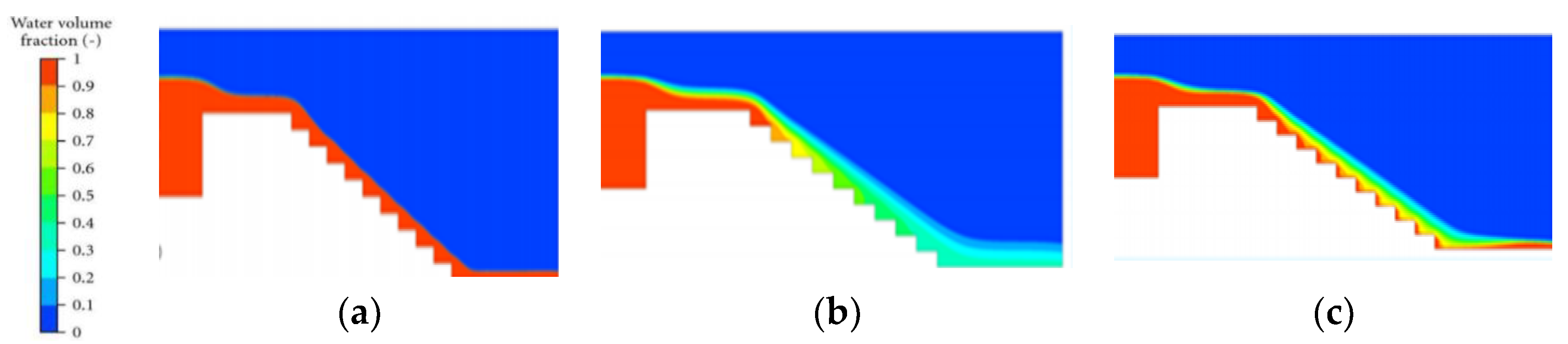
4.2. Oil Distribution
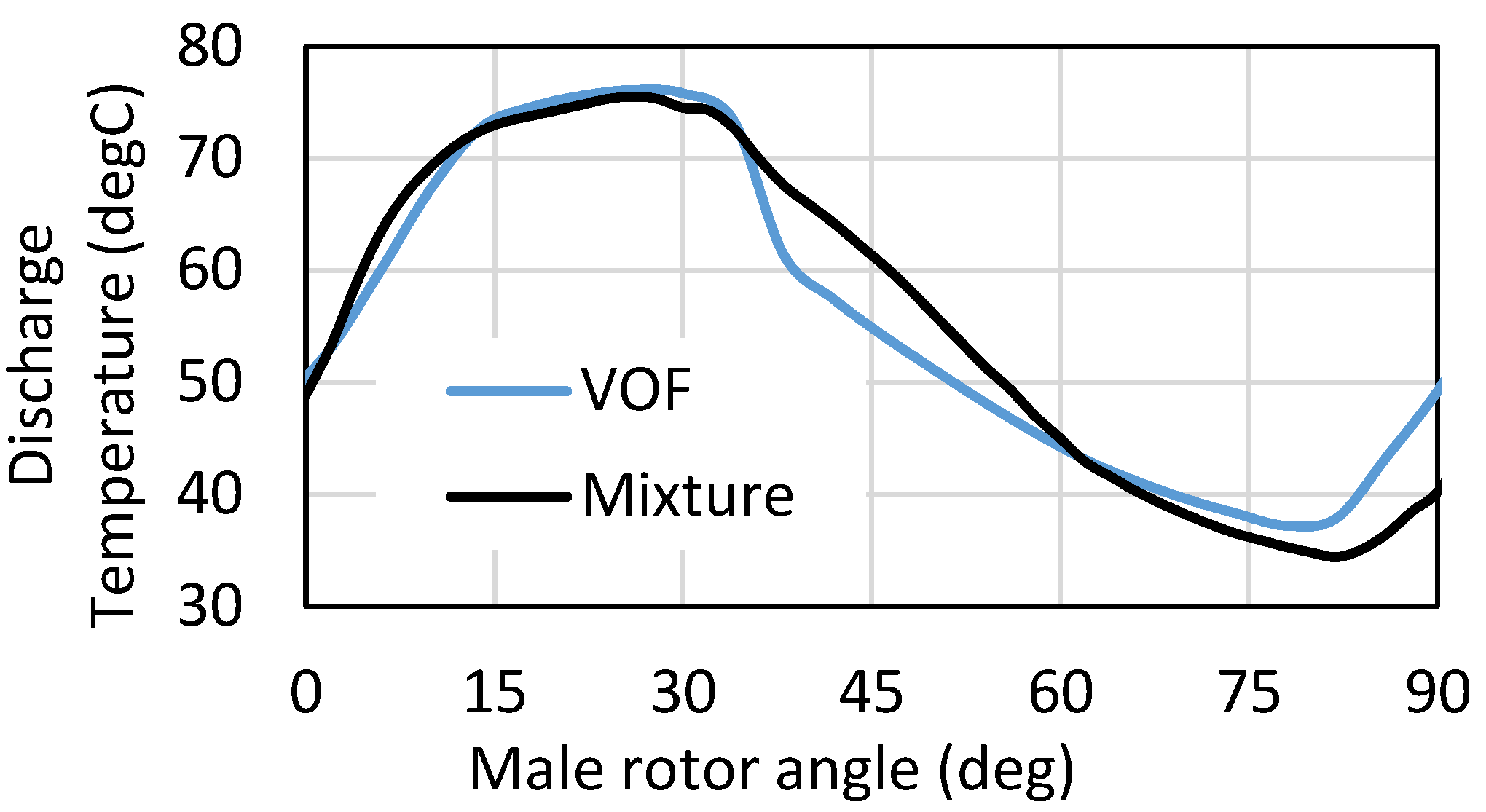
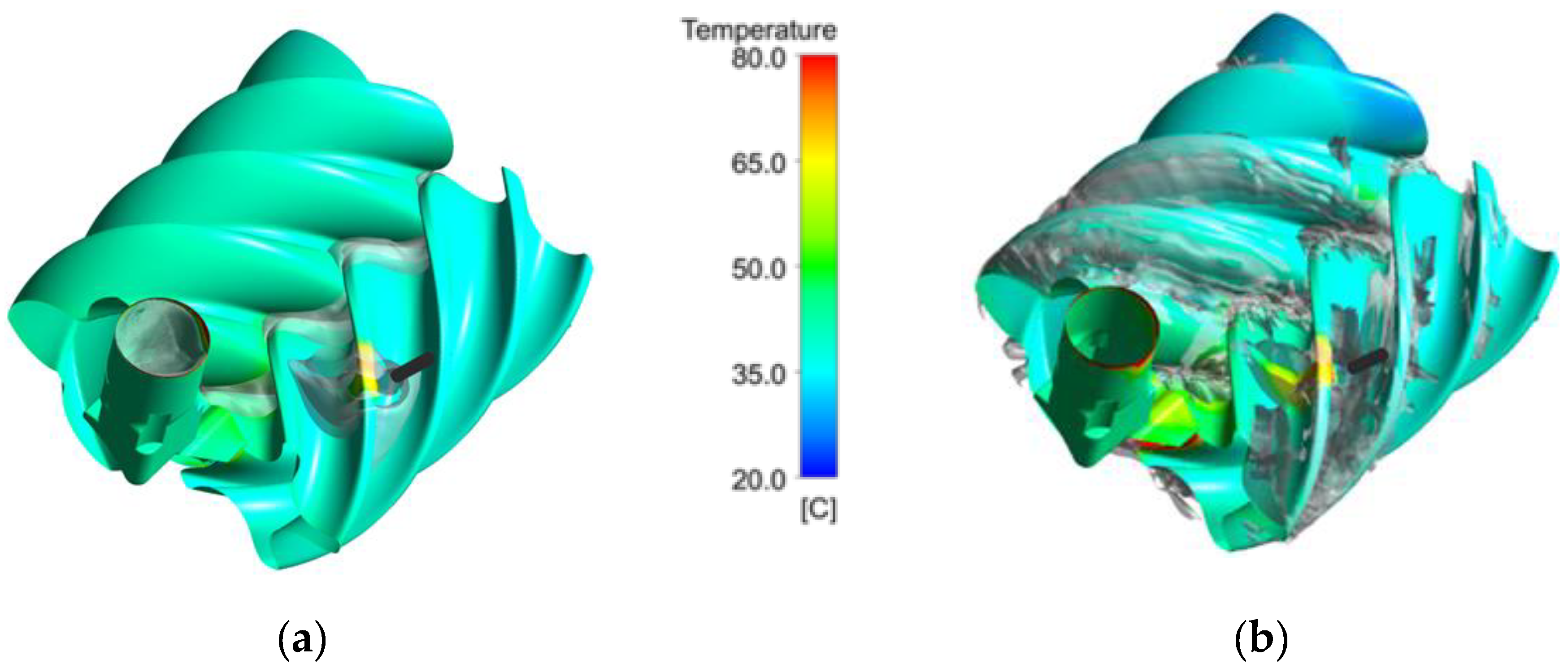
4.3. Temperature Distribution
4.4. Overall Performance with Measurements
5. Conclusions
- Both mixture and VOF models are capable of predicting overall performance of the flow and power close to measured values. The mixture model predicts flow with 0.9% and power with 4.5% error compared with measured values. The VOF model predicts flow with 3.5% and power with 5.7% error compared with measured values.
- Pseudo single-phase models are computationally economical, and on average a 22% improvement in time was observed with pseudo-single fluid models compared to the Eulerian–Eulerian TFM model.
- This study shows a good comparison of oil distribution within the compression chamber, which differs between mixture and VOF models. With the VOF model, smooth distribution of oil volume fraction was observed due to the air and oil phases being treated as non-interpenetrating. With the mixture model, the distribution of oil phase was different since the phases are penetrating and the slip between phases is included. However, the Eulerian–Eulerian model which was originally solved in CFX still shows distribution closer to expected reality.
- With the mixture model there is flexibility and opportunity for further improvements in terms of customised empirical model for interphase drag and slip forces which can lead to more realistic oil distribution. The mixture model can be a good replacement for the full Eulerian–Eulerian models as it is computationally more efficient, but further investigation into better interphase modelling forces will be required.
Author Contributions
Funding
Conflicts of Interest
References
- Stosic, N.; Smith, I.; Kovacevic, A.; Mujic, E. Three Decades of Modern Practice in Screw Compressors. In Proceedings of the International Compressor Engineering Conference, Purdue, IN, USA, 12–15 July 2010. [Google Scholar]
- Wood, L. Screw Compressor Market 2017—Global Forecast to 2021. Research and Markets. Available online: https://www.globenewswire.com/news-release/2017/01/26/911099/0/en/Global-Screw-Compressor-Market-2017-2021-Atlas-Copco-Ingersoll-Rand-GE-Oil-Gas-Gardner-Denver-and-Siemens-Lead-the-11-Billion-Market.html (accessed on 26 January 2017).
- Abdan, S.; Basha, N.; Kovacevic, A.; Stosic, N.; Birari, A.; Asati, N. Development and Design of Energy Efficient Oil-Flooded Screw Compressors. In IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2019; Volume 604. [Google Scholar]
- Deipenwisch, R.; Kauder, K. Oil as a design parameter in screw-type compressors: Oil distribution and power losses caused by oil in the working chamber of a screw-type compressor. In Proceedings of the International Conference on Compressor and their Systems, London, UK, 13–15 September 1999; pp. 49–58. [Google Scholar]
- Singh, P.; Bowman, J. Heat Transfer in Oil-Flooded Screw Compressors. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 4–7 August 1986; pp. 135–153. [Google Scholar]
- Stošic, N.; Milutinović, L.; Hanjalić, K.; Kovačević, A. Investigation of the influence of oil injection upon the screw compressor working process. Int. J. Refrig. 1992, 15, 206–220. [Google Scholar] [CrossRef]
- Peng, X.; Xing, Z.; Cui, T.; Shu, P. Experimental Study of Oil Injection and Its Effect On Performance of Twin Screw Compressors. In Proceedings of the International Compressor Engineering Conference, West Lafayette, IN, USA, 25–28 July 2000. [Google Scholar]
- De Paepe, M.; Bogaert, W.; Mertens, D. Cooling of oil injected screw compressors by oil atomisation. Appl. Therm. Eng. 2005, 25, 2764–2779. [Google Scholar] [CrossRef]
- Basha, N.; Kovacevic, A.; Stosic, N.; Smith, I. Effect of oil-injection on twin screw compressor performance. In IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2018. [Google Scholar]
- Kovacevic, A. Three-Dimensional Numerical Analysis for Flow Prediction in Positive Displacement Screw Machines. Ph.D. Thesis, City University London, Lodon, UK, 2002. [Google Scholar]
- Kovacevic, A. Boundary adaptation in grid generation for CFD analysis of screw compressors. Int. J. Numer. Methods Eng. 2005, 64, 401–426. [Google Scholar] [CrossRef]
- Kovacevic, A.; Stosic, N.; Smith, I. Screw Compressors: Three Dimensional Computational Fluid Dynamics and Solid Fluid Interaction; Springer: Berlin, Germany, 2005. [Google Scholar]
- Kovacevic, A.; Rane, S.; Stosic, N.; Jiang, Y.; Furmanczyk, M.; Lowry, S. Influence of approaches in CFD Solvers on Performance Prediction in Screw Compressors. In Proceedings of the International Compressor Engineering Conference, Purdue, IN, USA, 14–17 July 2014. [Google Scholar]
- Kovacevic, A.; Rane, S. Algebraic generation of single domain computational grid for twin screw machines Part II—Validation. Adv. Eng. Softw. 2017, 109, 31–43. [Google Scholar] [CrossRef]
- Rane, S.; Kovacevic, A.; Stosic, N. CFD Analysis of Oil Flooded Twin Screw Compressors. In Proceedings of the 23rd International Compressor Engineering Conference, West Lafayette, IN, USA, 11–14 July 2016. [Google Scholar]
- Basha, N.; Rane, S.; Kovacevic, A. Multiphase Flow Analysis in Oil-injected Twin Screw Compressor. In Proceedings of the 3rd International Conference on Multiphase Flow and Heat Transfer, Budapest, Hungary, 12–14 April 2018. [Google Scholar]
- Yeoh, G.H.; Barber, T. Assessment of interface capturing methods in Computational Fluid Dynamics (CFD) codes—A case study. J. Comput. Multiph. Flows 2009, 1, 201–215. [Google Scholar] [CrossRef]
- Ding, H.; Jiang, Y. CFD simulation of a screw compressor with oil injection. In Proceedings of the 10th International Conference on Compressors and their Systems, London, UK, 11–13 September 2017. [Google Scholar]
- Randi, S.; Suman, A.; Casari, N.; Pinelli, M.; Ziviani, D. Numerical analysis of oil injection effects in a single screw expander. In IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2018. [Google Scholar]
- Papes, I.; Degroote, J.; Vierendeels, J. 3D CFD Analysis of an Oil Injected Twin Screw Expander. In Proceedings of the ASME 2013 International Mechanical Engineering Congress and Exposition IMECE2013, Fairfield, CT, USA, 15–21 November 2014. [Google Scholar]
- Manik, J.; Dalal, A.; Natarajan, G. International Journal of Multiphase Flow A generic algorithm for three-dimensional multiphase flows on unstructured meshes. Int. J. Multiph. Flow 2018, 106, 228–242. [Google Scholar] [CrossRef]
- Fluent, A. ANSYS Fluent 12.0 User’s Guide; Ansys Inc.: Pittsburgh, PA, USA, 2009; Volume 15317, pp. 1–2498. [Google Scholar]
- Ubbink, O.; Issa, R.I. A Method for Capturing Sharp Fluid Interfaces on Arbitrary Meshes. J. Comput. Phys. 1999, 153, 26–50. [Google Scholar] [CrossRef]
- Kallio, S.; Akademi, A. On the Mixture Model for Multiphase Flow; VTT: Espoo, Finland, 1996; Volume 288. [Google Scholar]
- Bianchi, G.; Rane, S.; Kovacevic, A.; Cipollone, R. Deforming grid generation for numerical simulations of fluid dynamics in sliding vane rotary machines. Adv. Eng. Softw. 2017, 112, 180–191. [Google Scholar] [CrossRef]
- Basha, N.; Kovacevic, A.; Rane, S. User defined nodal displacement of numerical mesh for analysis of screw machines in FLUENT. In IOP Conference Series: Materials Science and Engineering; IOP Publishing: Bristol, UK, 2019; Volume 604. [Google Scholar]
- Lu, Y.; Kovacevic, A.; Basha, N.; Read, M. CFD Analysis of Twin Screw Vacuum Pump. In Proceedings of the 9th International Conference on Compressor and Refrigeration, Xi′an, China, 10–12 July 2019. [Google Scholar]
- Lu, Y.; Kovacevic, A.; Read, M.; Basha, N. Numerical Study of Customised Mesh for Twin Screw Vacuum Pumps. Designs 2019, 3, 52. [Google Scholar] [CrossRef]
- Bannari, R.; Kerdouss, F.; Selma, B.; Bannari, A.; Proulx, P. Three-dimensional mathematical modelling of dispersed two-phase flow using class method of population balance in bubble columns. Comput. Chem. Eng. 2008, 32, 3224–3237. [Google Scholar] [CrossRef]
- Van Alwon, J.; Borman, D.; Sleigh, A.; Kapur, N.I.K. Experimental and numerical modelling of aerated flows over stepped spillways. In Proceedings of the IAHR 2017 37th IAHR World Congress, Kuala Lumpur, Malaysia, 13–18 August 2017. [Google Scholar]






| Suitability | Continuity Equation (C) | Momentum Equation (M) | Energy Equation (E) | Droplet Dia. | Equations Solved | |
|---|---|---|---|---|---|---|
| Eulerian-Eulerian | Strong coupling between phases. Ex: bubble columns, particle suspension and fluidised beds | Solved for each phase |
|
| Yes | C-2 M-6 E-2 Total: 10 |
| VOF | Suitable when a clear interface is present between the phases and this interface is of interest. Ex: Stratified or free surface flows | Single equation |
|
| No | C-1 M-3 E-1 VF-1 Total: 6 |
| Mixture | Suitable for a wide range of dispersed phases and flows. Ex. Droplet laiden flows, sedimentation or cyclone separators | Single equation. Presence of drift & mixture velocities |
| Similar to VOF | Yes | C-1 M-3 E-1 VF-1 Total: 6 |
| Phase | Density (kg/m3) | Specific Heat Capacity (J/kg·K) | Dynamic Viscosity (kg/m·s) | Thermal Conductivity (W/m·K) |
|---|---|---|---|---|
| Air | 1.225 | 1.0044 | 1.831 × 10−5 | 2.61 × 10−2 |
| Oil | 800 | 1800.0 | 0.0088 | 0.18 |
| Criteria | Selection-FLUENT |
|---|---|
| Turbulence Model | SST (Menter’s Shear Stress Transport) k-omega (Mixture) |
| Inlet Boundary Condition | Inlet (specified pressure and temperature) |
| Outlet Boundary Condition | Pressure outlet (with specified pressure and temperature) |
| Pressure-Velocity Coupling | Coupled (second order upwind) |
| Gradient | Green-Gauss Node Based |
| Volume Fraction | Compressive (with phase localised zonal discretisation schemes) |
| Turbulence Scheme | Second order upwind |
| Transient Scheme | Second order implicit |
| Iterations per time step | 200 |
| Convergence Criteria | Continuity 0.07, Velocity 0.001, Energy 1 × 10−6, Turbulence 0.001, Volume Fraction 0.001 |
| Relaxation parameters | 0.01 |
| Serial No. | Air Flow | Power | Air Flow Error | Power Error | Specific Power Error | Mass Imbalance Error | |
|---|---|---|---|---|---|---|---|
| (kg/s) | (kW) | (%) | (%) | (%) | (%) | ||
| 1 | Expt. | 0.0706 | 18.434 | ||||
| 2 | VOF | 0.0682 | 17.387 | −3.46 | 5.68 | 2.30 | 9.54 |
| 3 | Mixture | 0.0713 | 17.595 | 0.88 | 4.55 | 4.39 | 0.10 |
| Parameter | VOF (Fluent) | Mixture (Fluent) | Eulerian–Eulerian (CFX) |
|---|---|---|---|
| Iterations per time step | 2.00 × 102 | 2.00 × 102 | 5.00 ×100 |
| Calculation time per time step per core (mins) | 40.5 | 42 | 50.5 |
| Time improvement (%) (compared to Eulerian-Eulerian) | 24.7 | 20.2 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Basha, N.; Kovacevic, A.; Rane, S. Analysis of Oil-Injected Twin-Screw Compressor with Multiphase Flow Models. Designs 2019, 3, 54. https://doi.org/10.3390/designs3040054
Basha N, Kovacevic A, Rane S. Analysis of Oil-Injected Twin-Screw Compressor with Multiphase Flow Models. Designs. 2019; 3(4):54. https://doi.org/10.3390/designs3040054
Chicago/Turabian StyleBasha, Nausheen, Ahmed Kovacevic, and Sham Rane. 2019. "Analysis of Oil-Injected Twin-Screw Compressor with Multiphase Flow Models" Designs 3, no. 4: 54. https://doi.org/10.3390/designs3040054
APA StyleBasha, N., Kovacevic, A., & Rane, S. (2019). Analysis of Oil-Injected Twin-Screw Compressor with Multiphase Flow Models. Designs, 3(4), 54. https://doi.org/10.3390/designs3040054







