Estimation of Resilient Modulus for Coarse-Grained Subgrade Soils from Quick Shear Tests for Mechanistic-Empirical Pavement Designs
Abstract
:1. Introduction
2. Background
3. Objectives of the Study
- Perform index property tests, resilient modulus tests, and QS tests on reconstituted samples of coarse-grained soils collected from different regions of South Carolina.
- Develop correlation models for estimating the resilient modulus of coarse-grained soils from results obtained from QS tests and soil index properties.
- Perform resilient modulus and QS tests on Shelby tube samples obtained from different regions of South Carolina to validate the developed models.
4. Methodology
4.1. Soil Collection and Laboratory Index Tests
4.2. Sample Preparation for Resilient Modulus Tests
4.3. Resilient Modulus Tests
4.4. Quick Shear Test
4.5. Multiple Linear Regression
5. Results and Discussions
5.1. Index Test Results
5.2. Resilient Modulus Test Results
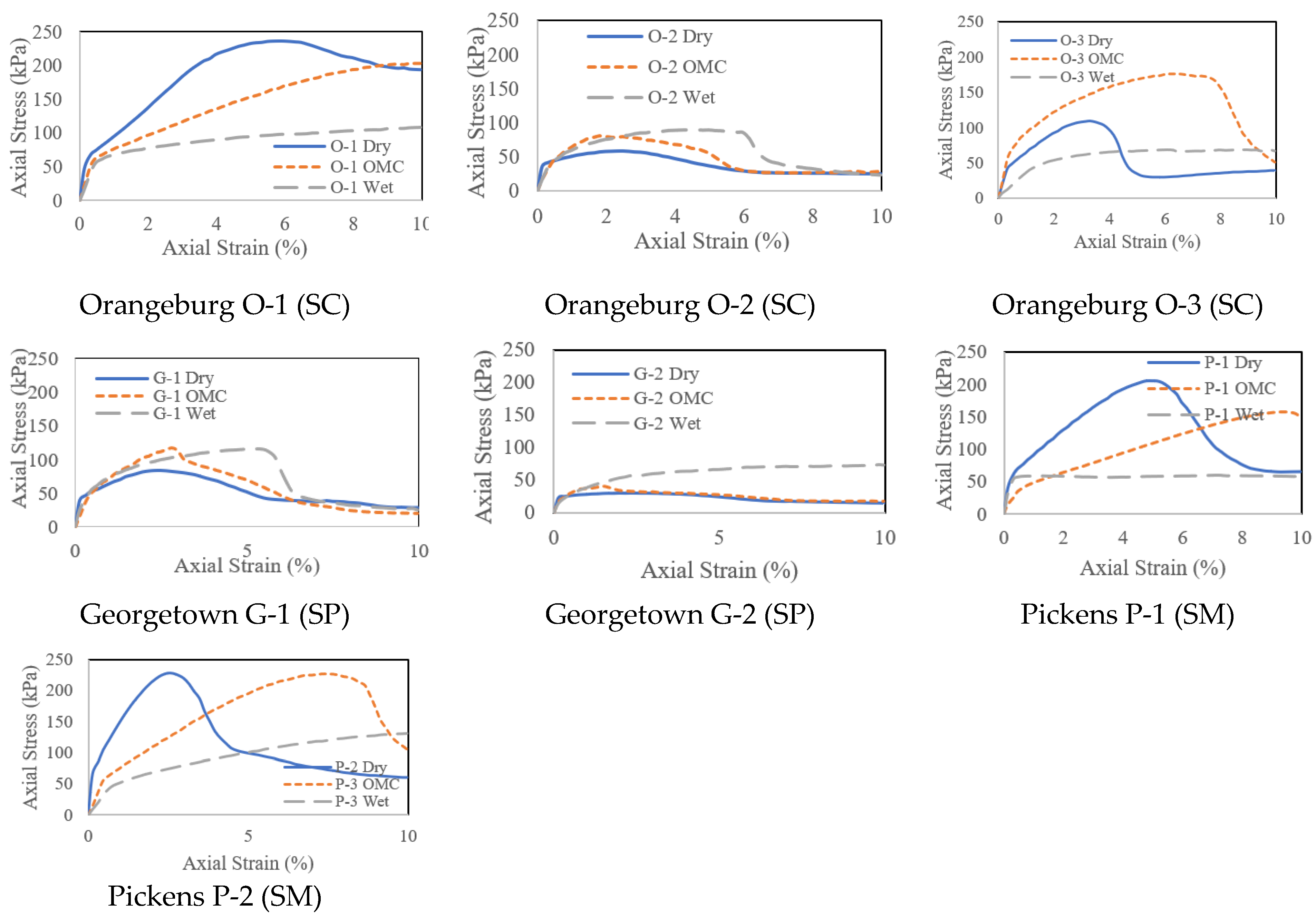
5.3. Quick Shear Test Results
6. Development of Resilient Modulus Model
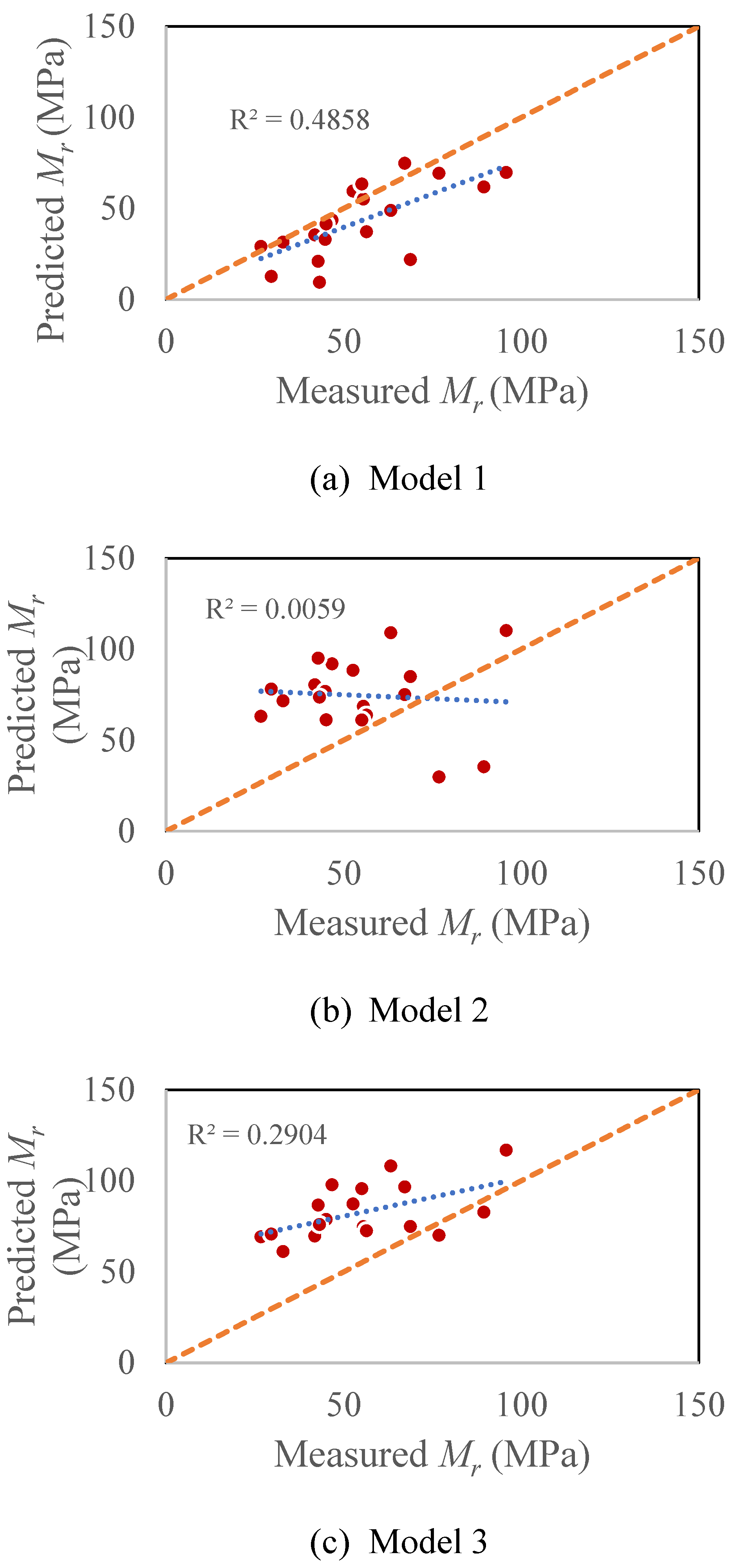
7. Validation of the Developed Model
8. Limitations of Models and Future Recommendations
9. Conclusions
- The resilient modulus value for coarse-grained subgrade soil can be estimated from Eavg and the index test results, with an R2 value of 0.76. Among 24 different variables only 5 variables showed a statistically significant effect on resilient modulus prediction model: Moisture content, optimum moisture content, dry unit weight, maximum dry unit weight, and average tangent modulus from a QS test.
- No distinct relation between moisture content and qu or S1% from the QS tests was observed for different coarse-grained soils. However, Eavg increased with decreasing moisture content, with few exceptions.
- The validation study, performed using field samples collected from Shelby tubes, showed that most of the data points, of measured and predicted resilient modulus, are close to the line of equity. A fair coefficient of determination was observed (R2 = 0.49) for the model developed from average tangent modulus and index properties (moisture content, optimum moisture content, dry unit weight, and maximum dry unit weight). Therefore, this model can be used for predicting resilient modulus for South Carolina coarse-grained soils.
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Gassman, S.L.; Rahman, M.M. Calibration of the AASHTO Pavement Design Guide to South Carolina Conditions-Phase I; Report FHWA-SC-16-02; Federal Highway Administration: Washington, DC, USA, 2016.
- Rahman, M.T.; Tarefder, R. Assessment of Molding Moisture and Suction on Resilient Modulus of Lime Stabilized Clayey Subgrade Soils. Geotech. Test. J. 2015, 38, 840–850. [Google Scholar] [CrossRef]
- Ng, K.; Henrichs, Z.R.; Ksaibati, K.; Wulff, S.S. Measurement and Estimation of the Resilient Modulus of Subgrade Materials for Mechanistic-Empirical Pavement Design Guide in Wyoming. In Proceedings of the Transportation Research Board Annual Meeting, Washington, DC, USA, 10 January 2016; pp. 1–17. [Google Scholar]
- Yau, Q.; Von Quintus, H.L. Predicting Elastic Response Characteristics of Unbound Materials and Soils. Transp. Res. Rec. J. Transp. Res. Board 2004, 1874, 47–56. [Google Scholar] [CrossRef]
- Titi, H.H.; Elias, M.B.; Helwany, S. Determination of Typical Resilient Modulus Values for Selected Soils in Wisconsin; Mitchell Hall, Office of Research Services and Administration, University of Wisconsin-Milwaukee: Milwaukee, WI, USA, 2006. [Google Scholar]
- Louay, M.N.; Huang, B.; Puppala, A.J. Regression Model for Resilient Modulus of Subgrade Soils. Transp. Res. Rec. J. Transp. Res. Board 1999, 1687, 47–54. [Google Scholar]
- Lee, W.; Bohra, N.C.; Altschaeffl, A.G.; White, T.D. Resilient Modulus of Cohesive Soils. J. Geotech. Geoenviron. Eng. 1997, 105, 131–135. [Google Scholar] [CrossRef]
- AASHTO T 307. Determining the Resilient Modulus of Soils and Aggregate Materials. In Standard Specifications for Transportation Materials and Methods of Sampling and Testing; American Association of State Highway and Transportation Officials (AASHTO): Washington, DC, USA, 2003. [Google Scholar]
- Hossain, M.S.; Kim, W.S. Estimation of Subgrade Resilient Modulus for Fine-Grained Soil from Unconfined Compression Test. Transp. Res. Rec. J. Transp. Res. Board 2015, 2473, 126–135. [Google Scholar] [CrossRef]
- Rahman, M.M.; Gassman, S.L. Effect of Resilient Modulus of Undisturbed Subgrade Soils on Pavement Rutting. Int. J. Geotech. Eng. 2017, 13, 152–161. [Google Scholar] [CrossRef]
- Thompson, M.R.; Robnett, Q.L. Resilient Properties of Subgrade Soils. J. Transp. Eng. 1979, 105, 71–89. [Google Scholar]
- Rahman, M.M.; Gassman, S.L. Data Collection Experience for Preliminary Calibration of the AASHTO Pavement Design Guide for Flexible Pavements in South Carolina. Int. J. Pavement Res. Technol. 2018, 11, 445–457. [Google Scholar] [CrossRef]
- NCHRP 1-28A. Laboratory Determination of Resilient Modulus for Flexible Pavement Design. In Research Results Digest; Transportation Research Board: Washington, DC, USA, 2004; pp. 1–52. [Google Scholar]
- Butalia, T.S.; Huang, J.; Kim, D.G.; Croft, F. Effect of Moisture Content and Pore Water Pressure Build on Resilient Modulus of Cohesive Soils. In Resilient Modulus Testing for Pavement Components; ASTM STP 1437; ASTM International: West Conshohocken, PA, USA, 2003. [Google Scholar]
- Drumm, E.C.; Reeves, J.S.; Madgett, M.R.; Trolinger, W.D. Subgrade Resilient Modulus Correction for Saturation Effects. J. Geotech. Geoenviron. Eng. 1997, 123, 663–670. [Google Scholar] [CrossRef]
- Fredlund, D.G.; Bergan, A.T.; Wong, P.K. Relation between Resilient Modulus and Stress Conditions for Cohesive Subgrade Soils. Transp. Res. Rec. J. Transp. Res. Board 1977, 642, 73–81. [Google Scholar]
- Heydinger, A.G. Evaluation of Seasonal Effects on Subgrade Soils. Transp. Res. Rec. J. Transp. Res. Board 2003, 1619, 94–101. [Google Scholar] [CrossRef]
- Huang, J. Degradation of Resilient Modulus of Saturated Clay Due to Pore Water Pressure Buildup under Cyclic Loading. Master’s Thesis, Ohio State University, Columbus, OH, USA, 2001. [Google Scholar]
- Mohammad, L.N.; Puppala, A.J.; Alavilli, P. Influence of Testing Procedure and LVDT Location on Resilient Modulus of Soils. Transp. Res. Rec. J. Transp. Res. Board 1994, 1462, 91–101. [Google Scholar]
- Chou, Y.T. A Computer Program for Evaluation and Overlay Design of Roads, Streets and Open Storage Areas Using Nondestructive Testing and Elastic Layered Method-WESROAD (No. WES/MP/GL-94-2); Army Engineer Waterways Experiment Station Vicksburg Ms Geotechnical Lab: Vicksburg, MS, USA, 1994. [Google Scholar]
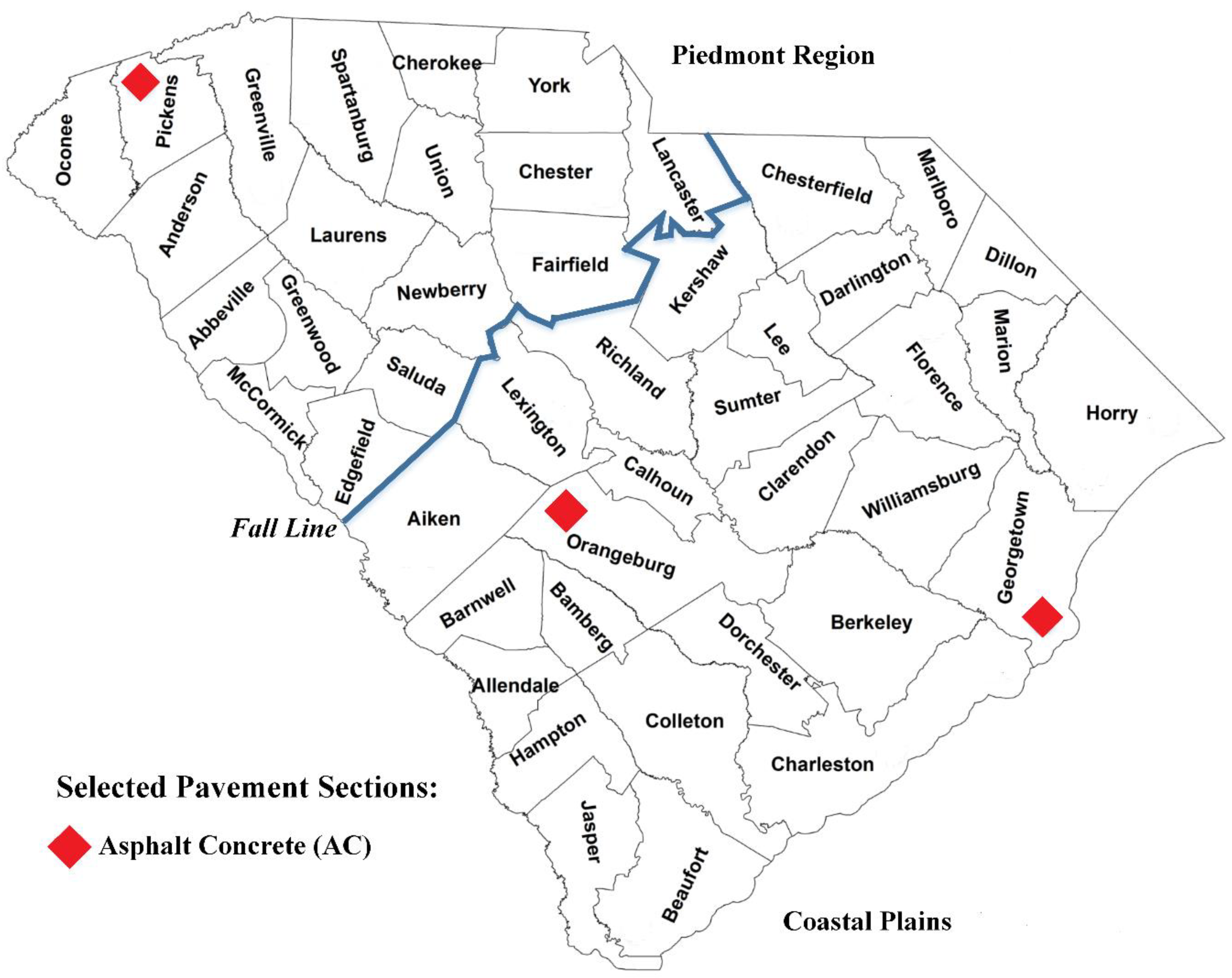



| County | Locations | P200 | LL (%) | PL (%) | PI (%) | Soil Classification | |||
|---|---|---|---|---|---|---|---|---|---|
| USCS | |||||||||
| Orangeburg | O-1 | 24.7 | 26 | 17 | 9 | 2.66 | 10.1 | 19.8 | SC |
| O-2 | 20.6 | 18 | 17 | 1 | 2.39 | 10.7 | 19.4 | SM | |
| O-3 | 22.8 | 20 | 16 | 4 | 2.6 | 10.6 | 19.5 | SC-SM | |
| Georgetown | G-1 | 1.5 | NA | NA | NA | 2.65 | 9.3 | 19.5 | SP |
| G-2 | 0.8 | NA | NA | NA | 2.71 | 12.2 | 17 | SP | |
| Pickens | P-1 | 43.8 | 45 | 29 | 16 | 2.55 | 15.1 | 17.6 | SM |
| P-2 | 44 | 42 | 28 | 14 | 2.51 | 13.8 | 18.5 | SC |
| Site | Soil | State | MC (%) | DUW (kN/m3) | Mr (MPa) | qu (kPa) | S1% (kPa) | Eavg (kPa) |
|---|---|---|---|---|---|---|---|---|
| Orangeburg | O-1 | Dry | 8.5 | 19.4 | 125 | 236 | 230 | 241 |
| OMC | 10.2 | 19.6 | 65 | 202 | 76 | 87 | ||
| Wet | 12.0 | 18.6 | 42 | 108 | 67 | 124 | ||
| O-2 | Dry | 7.0 | 18.5 | 114 | 58 | 50 | 270 | |
| Dry | 7.0 | 19.0 | 81 | 57 | 48 | 133 | ||
| OMC | 8.9 | 19.0 | 87 | 80 | 64 | 101 | ||
| OMC | 8.6 | 19.1 | 107 | 63 | 53 | 214 | ||
| Wet | 10.5 | 18.7 | 68 | 89 | 59 | 104 | ||
| O-3 | Dry | 8.0 | 19.4 | 97 | 110 | 64 | 107 | |
| OMC | 9.3 | 19.6 | 79 | 177 | 92 | 140 | ||
| Wet | 11.9 | 18.1 | 26 | 69 | 17 | 23 | ||
| Georgetown | G-1 | Dry | 7.8 | 19.0 | 121 | 84 | 64 | 205 |
| OMC | 9.5 | 19.3 | 89 | 118 | 72 | 108 | ||
| Wet | 11.2 | 18.7 | 62 | 116 | 73 | 115 | ||
| G-2 | Dry | 10.3 | 17.0 | 107 | 29 | 28 | 167 | |
| Dry | 10.0 | 17.0 | 74 | 29 | 27 | 154 | ||
| OMC | 11.9 | 17.1 | 103 | 41 | 37 | 93 | ||
| OMC | 11.6 | 17.1 | 111 | 38 | 35 | 161 | ||
| Wet | 13.7 | 16.7 | 90 | 73 | 38 | 56 | ||
| Pickens | P-1 | Dry | 13.2 | 17.4 | 89 | 188 | 118 | 202 |
| Dry | 13.1 | 17.0 | 71 | 115 | 85 | 176 | ||
| OMC | 14.7 | 17.7 | 81 | 205 | 90 | 141 | ||
| Wet | 16.7 | 17.4 | 20 | 158 | 48 | 38 | ||
| P-2 | Dry | 11.2 | 18.2 | 122 | 228 | 148 | 310 | |
| Dry | 10.6 | 17.8 | 34 | 228 | 95 | 97 | ||
| OMC | 13.2 | 18.5 | 76 | 227 | 75 | 70 | ||
| Wet | 14.3 | 18.1 | 25 | 131 | 52 | 51 |
| Model 1 | Model 2 | Model 3 | |
|---|---|---|---|
| Predictor Variables | β | β | β |
| Intercept | 343.77 *** | 55.62 *** | 41.68 |
| qu (kPa) | - | −0.22 * | - |
| S1% (kPa) | - | 0.27 | - |
| E (kPa) | 0.26 *** | 0.23 *** | 0.28 *** |
| MC (%) | −0.59 * | - | - |
| OMC (%) | −5.80 * | - | - |
| DUW (kN/m2) | 25.07 ** | - | - |
| MDUW (kN/m2) | −36.96 ** | - | - |
| R2 | 0.75 | 0.57 | 0.49 |
| Adjusted R2 | 0.71 | 0.51 | 0.48 |
| Overall Model Significance, F | 13.55 *** | 10.18 *** | 24.37 *** |
| Site | Soil | Sample No. | FMC (%) | FDUW (kN/m3) | Mr (MPa) | qu (kPa) | S1% (kPa) | Eavg (kPa) |
|---|---|---|---|---|---|---|---|---|
| Orangeburg | O-1 | 1 | 18.7 | 18.7 | 53 | 152 | 107 | 163 |
| 2 | 19.0 | 19.0 | 56 | 165 | 81 | 118 | ||
| O-2 | 1 | 17.9 | 17.9 | 42 | 42 | 41 | 100 | |
| 2 | 18.1 | 18.1 | 33 | 48 | 40 | 69 | ||
| O-3 | 1 | 17.3 | 17.3 | 69 | 47 | 46 | 118 | |
| Georgetown | G-1 | 1 | 17.1 | 17.1 | 47 | 129 | 69 | 200 |
| 2 | 16.7 | 16.7 | 43 | 61 | 59 | 160 | ||
| 3 | 17.9 | 17.9 | 57 | 170 | 75 | 110 | ||
| 4 | 18.5 | 18.5 | 67 | 229 | 92 | 196 | ||
| G-2 | 1 | 17.4 | 17.4 | 90 | 408 | 133 | 146 | |
| 2 | 16.5 | 16.5 | 96 | 221 | 154 | 268 | ||
| 3 | 16.0 | 16.0 | 64 | 159 | 125 | 237 | ||
| 4 | 17.0 | 17.0 | 55 | 325 | 121 | 193 | ||
| 5 | 18.2 | 18.2 | 77 | 345 | 99 | 101 | ||
| Pickens | P-1 | 1 | 17.0 | 17.0 | 45 | 167 | 102 | 132 |
| P-2 | 1 | 17.2 | 17.2 | 27 | 180 | 91 | 98 | |
| 2 | 16.6 | 16.6 | 30 | 112 | 86 | 103 | ||
| 3 | 18.2 | 16.2 | 43 | 167 | 99 | 122 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Rahman, M.M.; Islam, K.M.; Gassman, S. Estimation of Resilient Modulus for Coarse-Grained Subgrade Soils from Quick Shear Tests for Mechanistic-Empirical Pavement Designs. Designs 2019, 3, 48. https://doi.org/10.3390/designs3040048
Rahman MM, Islam KM, Gassman S. Estimation of Resilient Modulus for Coarse-Grained Subgrade Soils from Quick Shear Tests for Mechanistic-Empirical Pavement Designs. Designs. 2019; 3(4):48. https://doi.org/10.3390/designs3040048
Chicago/Turabian StyleRahman, Md Mostaqur, Kazi Moinul Islam, and Sarah Gassman. 2019. "Estimation of Resilient Modulus for Coarse-Grained Subgrade Soils from Quick Shear Tests for Mechanistic-Empirical Pavement Designs" Designs 3, no. 4: 48. https://doi.org/10.3390/designs3040048
APA StyleRahman, M. M., Islam, K. M., & Gassman, S. (2019). Estimation of Resilient Modulus for Coarse-Grained Subgrade Soils from Quick Shear Tests for Mechanistic-Empirical Pavement Designs. Designs, 3(4), 48. https://doi.org/10.3390/designs3040048




