Harmonics Mitigation Based on the Minimization of Non-Linearity Current in a Power System
Abstract
1. Introduction
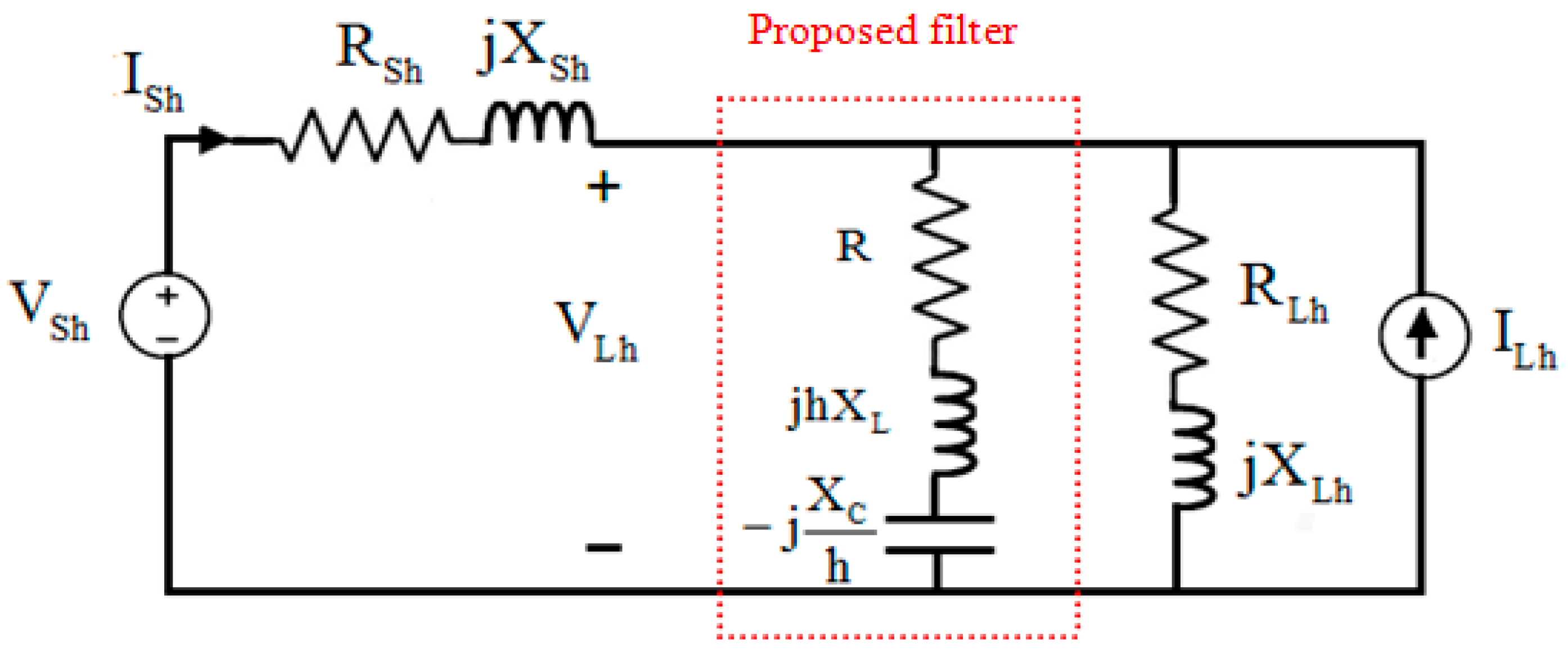
2. Formulation of the NLCI Minimized STPF
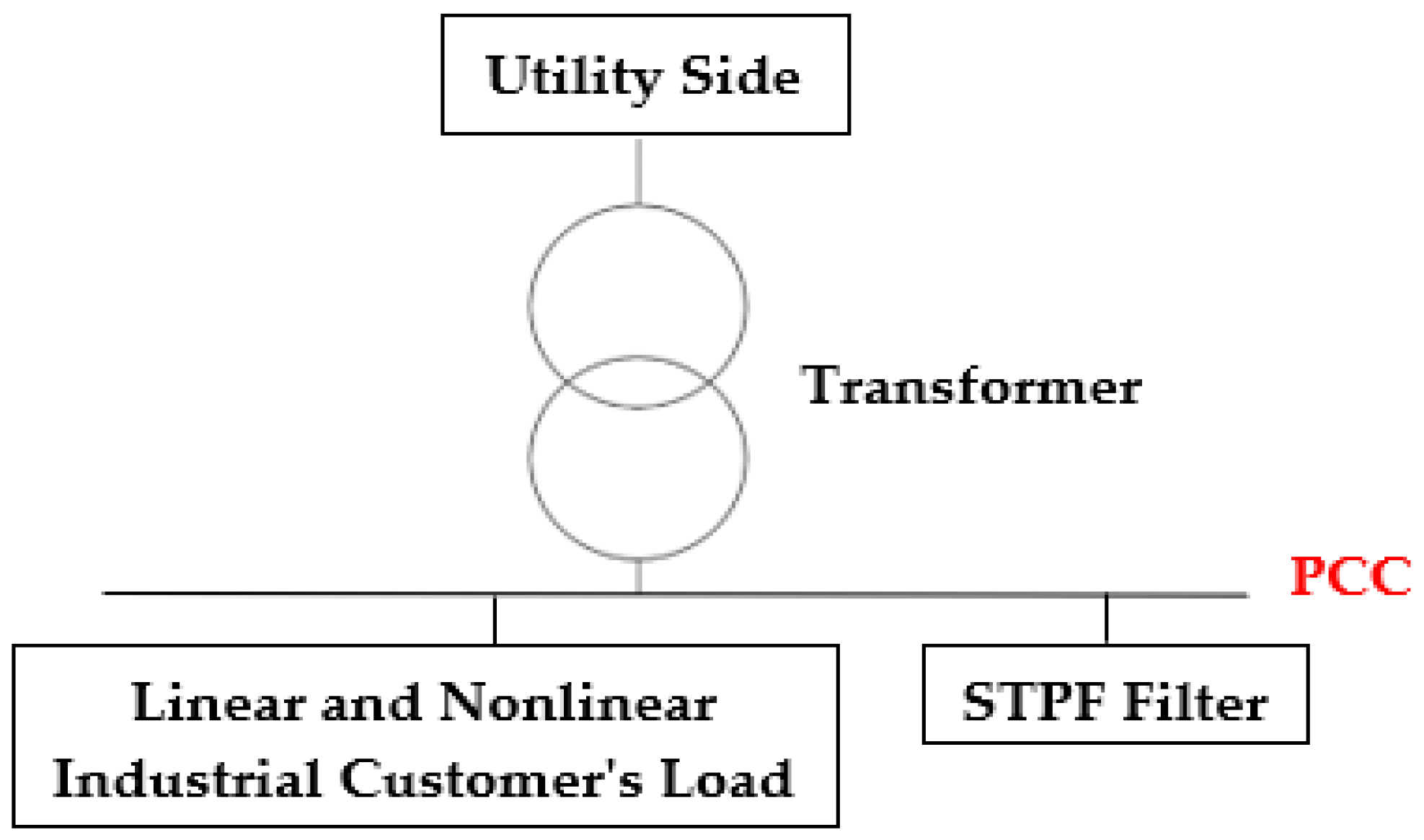
- The effect of transmission line impedance on the load voltage at the PCC.
- Source nonlinearity is included in the problem formulation by incorporating as separate parameters the source harmonic current ISh and the source harmonic voltage VSh.
- Load nonlinearity is included in the problem formulation as separate parameters, the harmonic load current ILh and the harmonic load voltage VLh.
- The frequency dependence of the solution is taken into consideration.
- Compensator values that would generate resonance phenomena are identified and excluded from the domain of possible solutions.
3. Constraints
3.1. Formulation of the Harmonic Resonance Constraint
3.2. Shunt Capacitor Constraints Based on the IEEE 18-2012 Standard
3.3. Other Constraints Based on the IEEE 519-2014 Standard
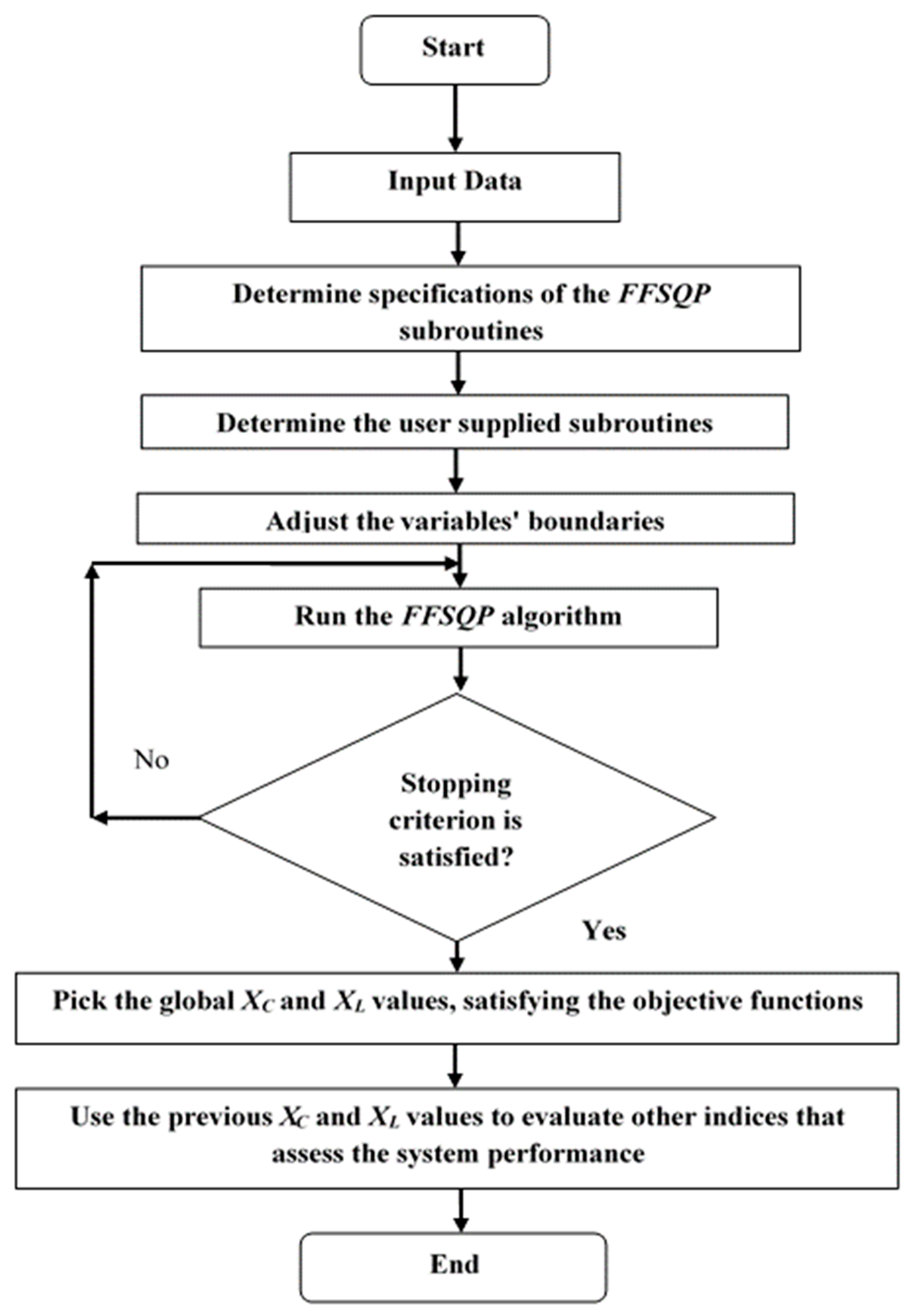
4. Optimization Technique and Search Algorithm
- Construct the needed subroutines (objective subroutine (f), constraints subroutine (g)) to develop the FFSQP search.
- Construct other subroutines that describe the mathematical modelling of the system.
- Run the search algorithm considering constraints.
- Choose the first value of the reactive power QCi rating of capacitor in kvar, so that QCi = {QC1, QC2… QCj}, where ΔQC or (QCj-QCj-1) is the kvar step. Also, j is the number of discrete values for the used voltage rating and i is a counter that has a starting value of 1.
- Calculate from
- Calculate XLi from equation (27).
- Run the search algorithm considering the filter component values to be the initial values at the beginning of each search in each region.
- Find the local solutions that achieve the best fitness of the considered objective.
- The algorithm will stop when a feasible point is reached or when the stopping criterion defined in terms of ε is met, where ε represents the relative difference in the objective function as a convergence-stopping criterion.
- After stopping, scan through local solutions to get the global one.
- Determine the filter parameters values corresponding to the global solution.
5. Case Studies and Simulated Results
6. Conclusions
- Tolerance to high levels of MVAr and almost maintenance-free service;
- They are more economical to implement than their rotating counterparts;
- A fast response time, of the order of one cycle or less, (which is particularly important in the presence of nonlinear loads).
Author Contributions
Funding
Conflicts of Interest
References
- Sher, H.A.; Addoweesh, K.E.; Khan, K.E. Harmonics generation, propagation and purging techniques in non-linear loads. In an Update on Power Quality; Lu, D., Ed.; InTech: Rijeka, Croatia, 2013. [Google Scholar] [CrossRef]
- Das, J.C. Power System Harmonics and Passive Filter Designs; Wiley: Piscataway, NJ, USA, 2015. [Google Scholar]
- Dugan, R.C.; McGranaghan, M.F.S.; Beaty, H.W. Electric Power Systems Quality; McGraw-Hill: New York, NY, USA, 2002. [Google Scholar]
- Ribeiro, R.L.D.; de Azevedo, C.C.; de Sousa, R.M. A Robust Adaptive Control Strategy of Active Power Filters for Power Factor Correction, Harmonic Compensation, and Balancing of Nonlinear Loads. IEEE Trans. Power Electron. 2012, 27, 718–730. [Google Scholar] [CrossRef]
- Wen, H.; Teng, Z.; Wang, Y.; Hu, X. Spectral correction approach based on desirable sidelobe window for harmonic analysis of industrial power system. IEEE Trans. Ind. Electron. 2013, 60, 1001–1010. [Google Scholar] [CrossRef]
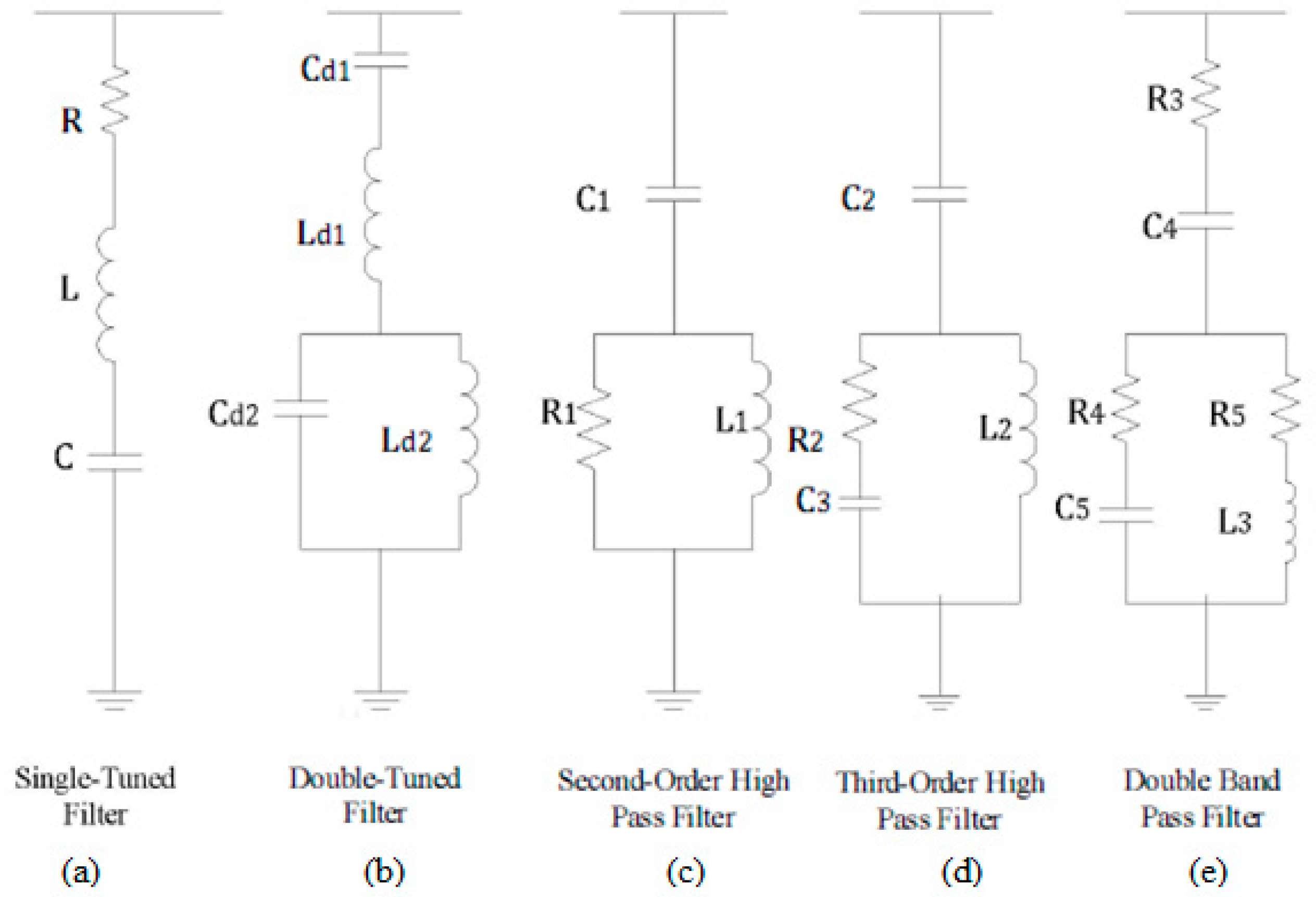
- Nassif, A.B.; Xu, W.; Freitas, W. An investigation on the selection of filter topologies for passive filter applications. IEEE Trans. Power Deliv. 2009, 24, 1710–1718. [Google Scholar] [CrossRef]
- Zobaa, A.F.; Vaccaro, A.; Zeineldin, H.H.; Lecci, A.; Monem, A.M.A. Sizing of passive filters in time-varying nonsinusoidal environments. In Proceedings of the 14th International Conference on Harmonics and Quality of Power (ICHQP’10), Bergamo, Italy, 26–29 September 2010; pp. 1–8. [Google Scholar]
- Izhar, M.; Hadzer, C.M.; Syafrudin, M.; Taib, S.; Idris, S. Performance for passive and active power filter in reducing harmonics in the distribution system. In Proceedings of the PECon 2004—IEEE National Power Energy Conference, Kuala Lumpur, Malaysia, 29–30 November 2004; pp. 104–108. [Google Scholar]
- Bagheri, P.; Xu, W. A technique to mitigate zero-sequence harmonics in power distribution systems. IEEE Trans. Power Deliv. 2014, 29, 215–223. [Google Scholar] [CrossRef]
- Ghorbani, A. An adaptive distance protection scheme in the presence of phase shifting transformer. Electr. Power Syst. Res. 2015, 129, 170–177. [Google Scholar] [CrossRef]
- Leite, J.C.; Nascimento, M.H.R.; Júnior, J.D.A.B.; Fonseca, M.; de Freitas, C.A.O.; Carvajal, T.L.R. Optimization using NSGA II for Passive Filters in Industrial Networks. In Proceedings of the 13th IEEE International Conference on Industry Applications (INDUSCON), São Paulo, Brazil, 12–14 November 2018; pp. 339–346. [Google Scholar]
- Parkatti, P.; Tuusa, H.; Sarén, H.; Kuusela, K.; Björkman, M. Analysis and Performance of a High-Efficiency Transformerless Hybrid Active Filter. Int. Rev. Electr. Eng. 2011, 6, 537–546. [Google Scholar]
- Rozlan, M.B.M.; Zobaa, A.F.; Aleem, S.H.E.A. The Optimisation of Stand-Alone Hybrid Renewable Energy Systems Using HOMER. Int. Rev. Electr. Eng. 2011, 6, 1802–1810. [Google Scholar]
- Farhoodnea, M.; Mohamed, A.; Shareef, H.; Zayandehroodi, H. Localization of Multiple Harmonic Sources in Non-Radial Power Distribution Systems. Int. Rev. Electr. Eng. 2012, 7, 4134–4145. [Google Scholar]
- Jou, H.L.; Wu, J.C.; Wu, K.D.; Huang, M.S.; Lin, C.A. A Hybrid Compensation System Comprising Hybrid Power Filter and AC Power Capacitor. Electr. Power Energy Syst. J. 2006, 28, 448–458. [Google Scholar] [CrossRef]
- Purwoharjono, M.A.; Penangsang, O.; Soeprijanto, A. Optimal Placement of SVC for Losses Reduction and Voltage Profile Improvement in Electrical Power System Using Improved Gravitational Search Algorithm. Int. Rev. Electr. Eng. 2013, 8, 329–339. [Google Scholar]
- Baitha, A.; Gupta, N. A comparative analysis of passive filters for power quality improvement. In Proceedings of the 2015 International Conference on Technological Advancements in Power and Energy (TAP Energy), Kollam, India, 24–26 June 2015; pp. 327–332. [Google Scholar]
- Kumar, D.R.; Anuradha, K.; Saraswathi, P.; Gokaraju, R.; Ramamoorty, M. New low cost passive filter configuration for mitigating bus voltage distortions in distribution systems. In Proceedings of the 2015 IEEE International Conference on Building Efficiency and Sustainable Technologies, Singapore, 31 August–1 September 2015; pp. 79–84. [Google Scholar]
- Zobaa, A.F. The Optimal Passive Filters to Minimize Voltage Harmonic Distortion at a Load Bus. IEEE Trans. Power Del. 2005, 20, 1592–1597. [Google Scholar] [CrossRef]
- Abdel-Aziz, M.M.; El-Zahab, E.E.A.; Ibrahim, A.M.; Zobaa, A.F. LC compensators for power factor correction of nonlinear loads. IEEE Trans. Power Deliv. 2004, 19, 331–336. [Google Scholar] [CrossRef]
- Aleem, S.H.E.; Zobaa, A.F.; Balci, M.E. Optimal resonance-free third-order high-pass filters based on minimization of the total cost of the filters using Crow Search Algorithm. Electr. Power Syst. Res. 2017, 151, 381–394. [Google Scholar] [CrossRef]
- Richards, G.G.; Klinkhachorn, P.; Tan, O.T.; Hartana, R.K. Optimal LC compensators for nonlinear loads with uncertain nonsinusoidal source and load characteristics. IEEE Trans. Power Syst. 1989, 4, 30–36. [Google Scholar] [CrossRef]
- Hartana, R.K.; Richards, G.G. Comparing capacitive and LC compensators for power factor correction and voltage harmonic reduction. Electr. Power Syst. Res. 1989, 17, 57–64. [Google Scholar] [CrossRef]
- Sakar, S.; Karaoglan, A.D.; Balci, M.E.; Aleem, S.H.A.; Zobaa, A.F. Optimal design of single-tuned passive filters using response surface methodology. In Proceedings of the 2015 International School on Nonsinusoidal Currents and Compensation (ISNCC), Lagow, Poland, 15–18 June 2015; pp. 1–6. [Google Scholar]
- Zacharia, P.; Menti, A.; Zacharias, T. Genetic algorithm based optimal design of shunt compensators in the presence of harmonics. Electr. Power Syst. Res. 2008, 78, 728–735. [Google Scholar] [CrossRef]
- Menti, A.; Zacharias, T.; Argitis, J.M. Optimal sizing and limitations of passive filters in the presence of background harmonic distortion. Electr. Eng. 2009, 91, 89–100. [Google Scholar] [CrossRef]
- Abdel Aleem, S.H.E.; Balci, M.E.; Zobaa, A.F.; Sakar, S. Optimal passive filter design for effective utilization of cables and transformers under non-sinusoidal conditions. In Proceedings of the 2014 16th International Conference on Harmonics and Quality of Power (ICHQP’14), Bucharest, Romania, 25–28 May 2014; pp. 626–630. [Google Scholar]
- Abdel-Aziz, M.M.; El-Zahab, E.E.A.; Ibrahim, A.M.; Zobaa, A.F. Comparing capacitive and LC compensators for power factor correction. In Proceedings of the 10th International Conference on Harmonics and Quality of Power, Rio de Janeiro, Brazil, 6–9 October 2002; pp. 42–45. [Google Scholar]
- Abdel-Aziz, M.M.; El-Zahab, E.E.A.; Ibrahim, A.M.; Zobaa, A.F. Practical considerations on power factor correction for nonlinear loads. In Proceedings of the 10th International Conference on Harmonics and Quality of Power (ICHQP’02), Rio de Janeiro, Brazil, 6–9 October 2002; pp. 46–49. [Google Scholar]
- Abdel Aleem, S.H.E.; Zobaa, A.F.; Aziz, M.M.A. Optimal C-type passive filter based on minimization of the voltage harmonic distortion for nonlinear loads. IEEE Trans. Ind. Electron. 2012, 59, 281–289. [Google Scholar] [CrossRef]
- Baici, M.E.; Karaoglan, A.D. Optimal design of C-type passive filters based on response surface methodology for typical industrial power systems. Electr. Power Compon. Syst. 2013, 41, 653–668. [Google Scholar]
- Zeineldin, H.H.; Zobaa, A.F. Particle swarm optimization of passive filters for industrial plants in distribution networks. Electr. Power Compon. Syst. 2011, 39, 1795–1808. [Google Scholar] [CrossRef]
- Zhu, X.-R.; Shi, X.-C.; Peng, Y.-L.; Li, H.-M. Simulated annealing based multi-object optimal planning of passive power filters. In Proceedings of the 2005 IEEE/PES Transmission & Distribution Conference & Exposition: Asia and Pacific, Dalian, China, 18 August 2005; pp. 1–5. [Google Scholar]
- Dell’Aquila, A.; Marinelli, M.; Monopoli, V.G.; Zanchetta, P. New power-quality assessment criteria for supply systems under unbalanced and nonsinusoidal conditions. IEEE Trans. Power Deliv. 2004, 19, 1284–1290. [Google Scholar] [CrossRef]
- Kahar, N.H.A.; Zobaa, A.F. Application of mixed integer distributed ant colony optimization to the design of undamped single-tuned passive filters based harmonics mitigation. Swarm Evol. Comput. 2019, 44, 187–199. [Google Scholar] [CrossRef]
- Piel, J.K.; Carnovale, D.J. Economic and Electrical Benefit of Harmonic Reduction Methods in Commercial Facilities; Eaton Electrical Inc.: Moon Township, PA, USA, 2004; pp. 1–9. [Google Scholar]
- Elmathana, M.T.; Zobaa, A.F.; Hegazy, Y. Optimal Harmonic Filters Design Based Mean Value Estimation of the Source and Load Characteristics. Recent Pat. Electr. Electron. Eng. 2012, 5, 155–163. [Google Scholar] [CrossRef]
- IEEE Standard 18-2012. IEEE Standard for Shunt Power Capacitors; Institute of Electrical and Electronics Engineers: New York, NY, USA, 2012. [Google Scholar]
- IEEE Standard 519-2014. IEEE Recommended Practice and Requirements for Harmonic Control. In Electrical Power Systems; Institute of Electrical and Electronics Engineers: New York, NY, USA, 2014. [Google Scholar]
- IEEE Standard 519-1992. IEEE Recommended Practices and Requirements for Harmonic Control. In Electrical Power Systems; Institute of Electrical and Electronics Engineers: New York, NY, USA, 1992. [Google Scholar]
- Gao, H.; Wu, B.; Xu, D.; Zargari, N.R. A model predictive power factor control scheme with active damping function for current source rectifiers. IEEE Trans. Power Electron. 2018, 33, 2655–2667. [Google Scholar] [CrossRef]
- Soomro, D.M.; Almelian, M.M. Optimal design of a single tuned passive filter to mitigate harmonics in power frequency. ARPN J. Eng. Appl. Sci. 2015, 10, 9009–9014. [Google Scholar]
- Abdollahi, R. Harmonic Mitigation using 36-Pulse AC-DC Converter for Direct Torque Controlled Induction Motor Drives. J. Appl. Res. Technol. 2015, 13, 135–144. [Google Scholar] [CrossRef][Green Version]
- Jacyntho, L.A.; Teixeira, M.C.M.; Cardim, E.A.R.; Galvão, R.K.H.; Hadjiloucas, S. Identification of fractional-order transfer functions using a step excitation. IEEE Trans. Circuits Syst. II, Express Briefs 2015, 62, 896–900. [Google Scholar] [CrossRef]
- Galvão, R.K.H.; Kienitz, K.H.; Hadjiloucas, S. Conversion of descriptor representations to state-space form: an extension of the shuffle algorithm. Int. J. Control 2018, 91, 2199–2213. [Google Scholar] [CrossRef]

| Parameters & Cases | Case 1 | Case 2 | Case 3 | Case 4 |
|---|---|---|---|---|
| MVASC (MVA) | 80 | 150 | ||
| RS1 (Ω) | 0.02163 | 0.02163 | 0.01154 | 0.01154 |
| XS1 (Ω) | 0.2163 | 0.2163 | 0.1154 | 0.1154 |
| RL1 (Ω) | 1.7421 | 1.7421 | 1.7421 | 1.7421 |
| XL1 (Ω) | 1.6960 | 1.6960 | 1.6960 | 1.6960 |
| VS1 (V) | 2400.00 | 2400.00 | 2400.00 | 2400.00 |
| VS5 (V) | 0.00 | 96.00 | 0.00 | 96.00 |
| VS7 (V) | 0.00 | 72.00 | 0.00 | 72.00 |
| VS11 (V) | 0.00 | 48.00 | 0.00 | 48.00 |
| VS13 (V) | 0.00 | 24.00 | 0.00 | 24.00 |
| IL5 (A) | 33 | 33 | 33 | 33 |
| IL7 (A) | 25 | 25 | 25 | 25 |
| IL11 (A) | 9 | 9 | 9 | 9 |
| IL13 (A) | 8 | 8 | 8 | 8 |
| Uncompensated Indices | ||||
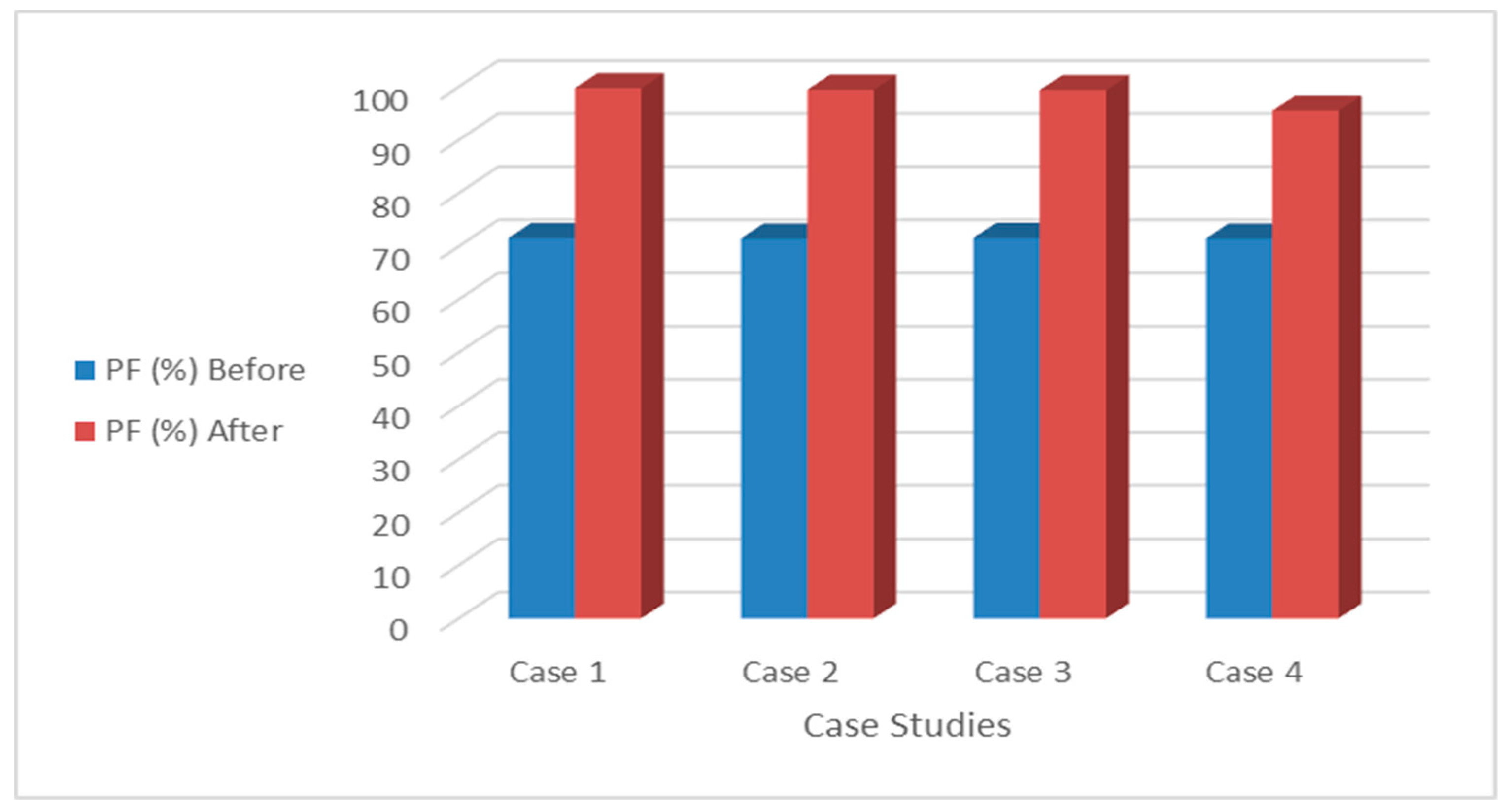
| PF (%) | 71.57 | 71.48 | 71.58 | 71.48 |
| DPF (%) | 71.65 | 71.65 | 71.65 | 71.65 |
| TL (kW) | 18.44 | 18.45 | 10.47 | 10.48 |
| η (%) | 98.77 | 98.77 | 99.34 | 99.34 |
| Load Type | Active Power (kW) | Reactive Power (kvar) | PF (%) |
|---|---|---|---|
| Linear loads | 1200 | 900 | 80.00 |
| 900 | 918 | 80.00 | |
| Nonlinear loads | 600 | 612 | 70.00 |
| 1100 | 1902 | 50.00 | |
| Other loads | 1300 | 630 | 90.00 |
| Total loads | 5100 | 4965 | 71.65 |
| XC (Ω) | XL (Ω) | PF (%) | η (%) | TL (kW) |
|---|---|---|---|---|
| Case 1 | ||||
| 4.11 | 0.40 | 99.75 | 99.36 | 10.61 |
| Case 2 | ||||
| 4.11 | 0.40 | 99.45 | 99.36 | 10.67 |
| Case 3 | ||||
| 4.19 | 0.27 | 99.38 | 99.66 | 5.76 |
| Case 4 | ||||
| 5.06 | 0.28 | 95.54 | 99.63 | 6.16 |
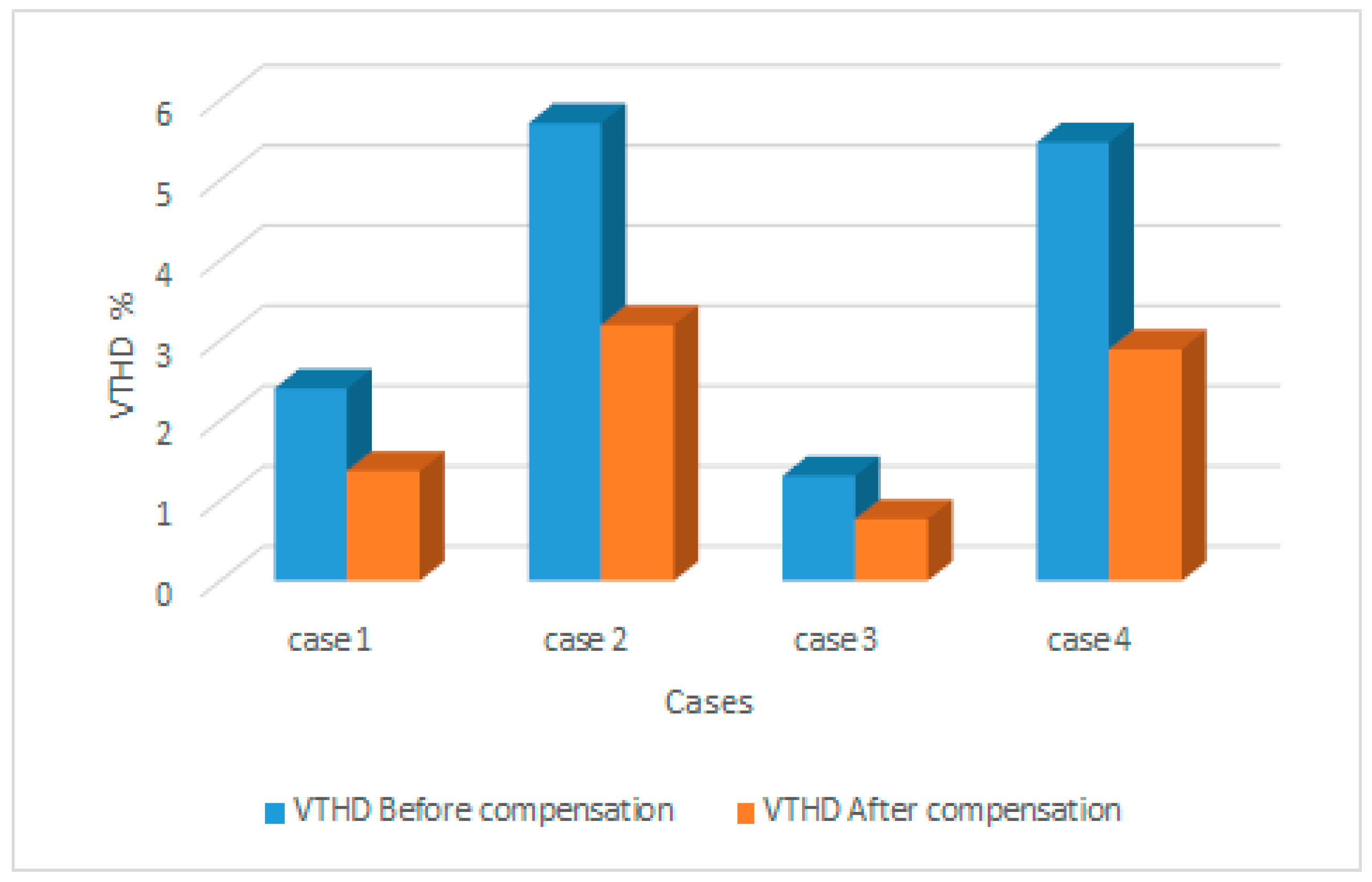
| Case | ITHD (%) | VTHD (%) | DPF (%) |
|---|---|---|---|
| 1 | 3.18 | 1.37 | 99.81 |
| 2 | 8.05 | 3.19 | 99.81 |
| 3 | 3.21 | 0.77 | 99.43 |
| 4 | 15.29 | 2.89 | 96.69 |
| Item | Calculated Values | Calculated (%) | IEEE 18-2012 Limit (%) | Exceeds Limit |
|---|---|---|---|---|
| Case 1 | ||||
| VC | 2620.835 | 109.201 | 110 | No |
| VCP | 2643.028 | 110.121 | 120 | No |
| IC | 637.804 | 109.338 | 135 | No |
| QC | 1685.735 | 120.410 | 135 | No |
| Case 2 | ||||
| VC | 2621.056 | 109.211 | 110 | No |
| VCP | 2674.345 | 111.431 | 120 | No |
| IC | 639.361 | 109.605 | 135 | No |
| QC | 1709.871 | 122.134 | 135 | No |
| Case 3 | ||||
| VC | 2547.008 | 106.125 | 110 | No |
| VCP | 2569.108 | 107.046 | 120 | No |
| IC | 608.496 | 106.210 | 135 | No |
| QC | 1563.293 | 113.694 | 135 | No |
| Case 4 | ||||
| VC | 2508.601 | 104.525 | 110 | No |
| VCP | 2640.681 | 110.028 | 120 | No |
| IC | 506.375 | 106.840 | 135 | No |
| QC | 1337.174 | 117.554 | 135 | No |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Almutairi, M.S.; Hadjiloucas, S. Harmonics Mitigation Based on the Minimization of Non-Linearity Current in a Power System. Designs 2019, 3, 29. https://doi.org/10.3390/designs3020029
Almutairi MS, Hadjiloucas S. Harmonics Mitigation Based on the Minimization of Non-Linearity Current in a Power System. Designs. 2019; 3(2):29. https://doi.org/10.3390/designs3020029
Chicago/Turabian StyleAlmutairi, Mohammed S., and Sillas Hadjiloucas. 2019. "Harmonics Mitigation Based on the Minimization of Non-Linearity Current in a Power System" Designs 3, no. 2: 29. https://doi.org/10.3390/designs3020029
APA StyleAlmutairi, M. S., & Hadjiloucas, S. (2019). Harmonics Mitigation Based on the Minimization of Non-Linearity Current in a Power System. Designs, 3(2), 29. https://doi.org/10.3390/designs3020029





