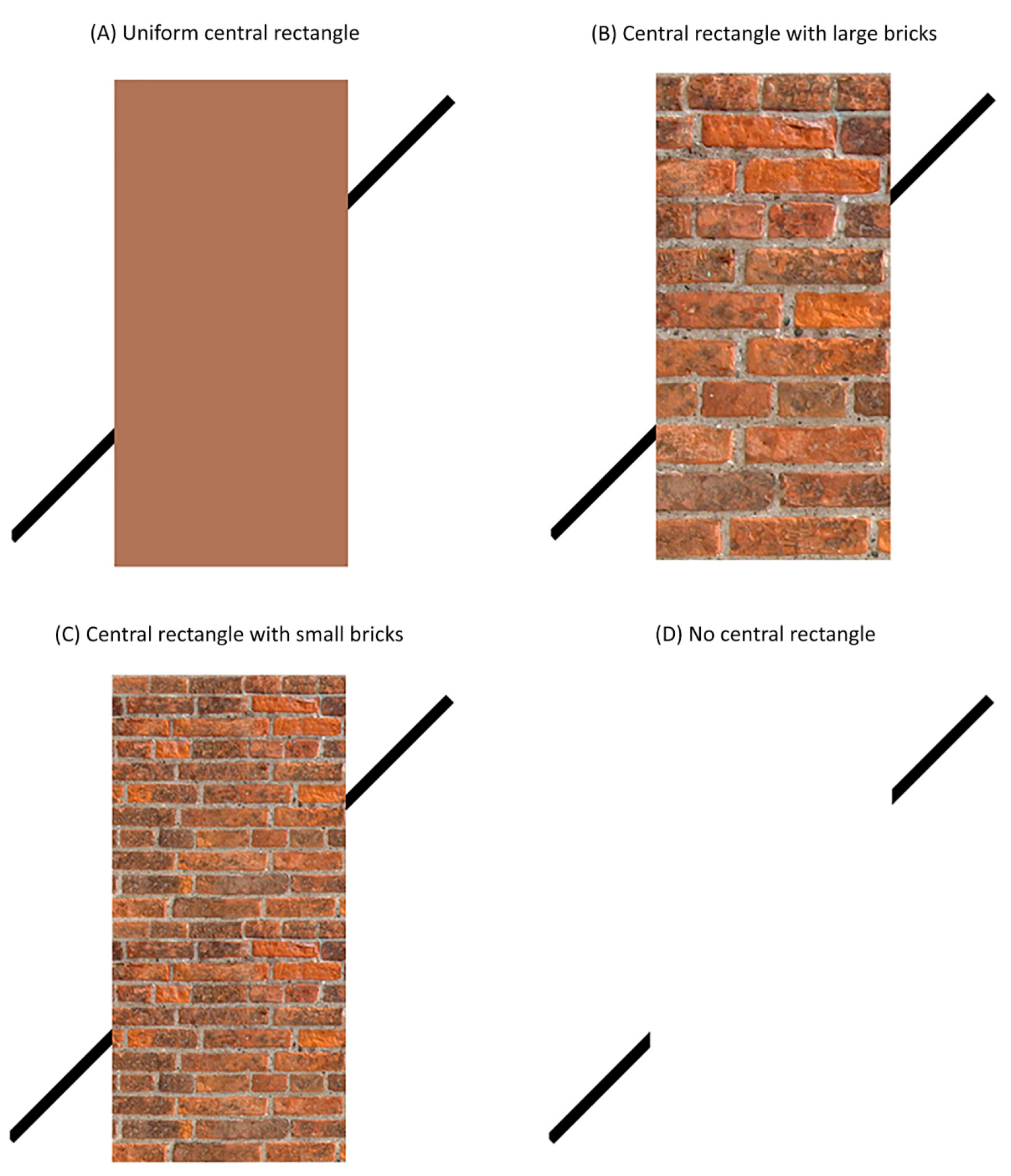
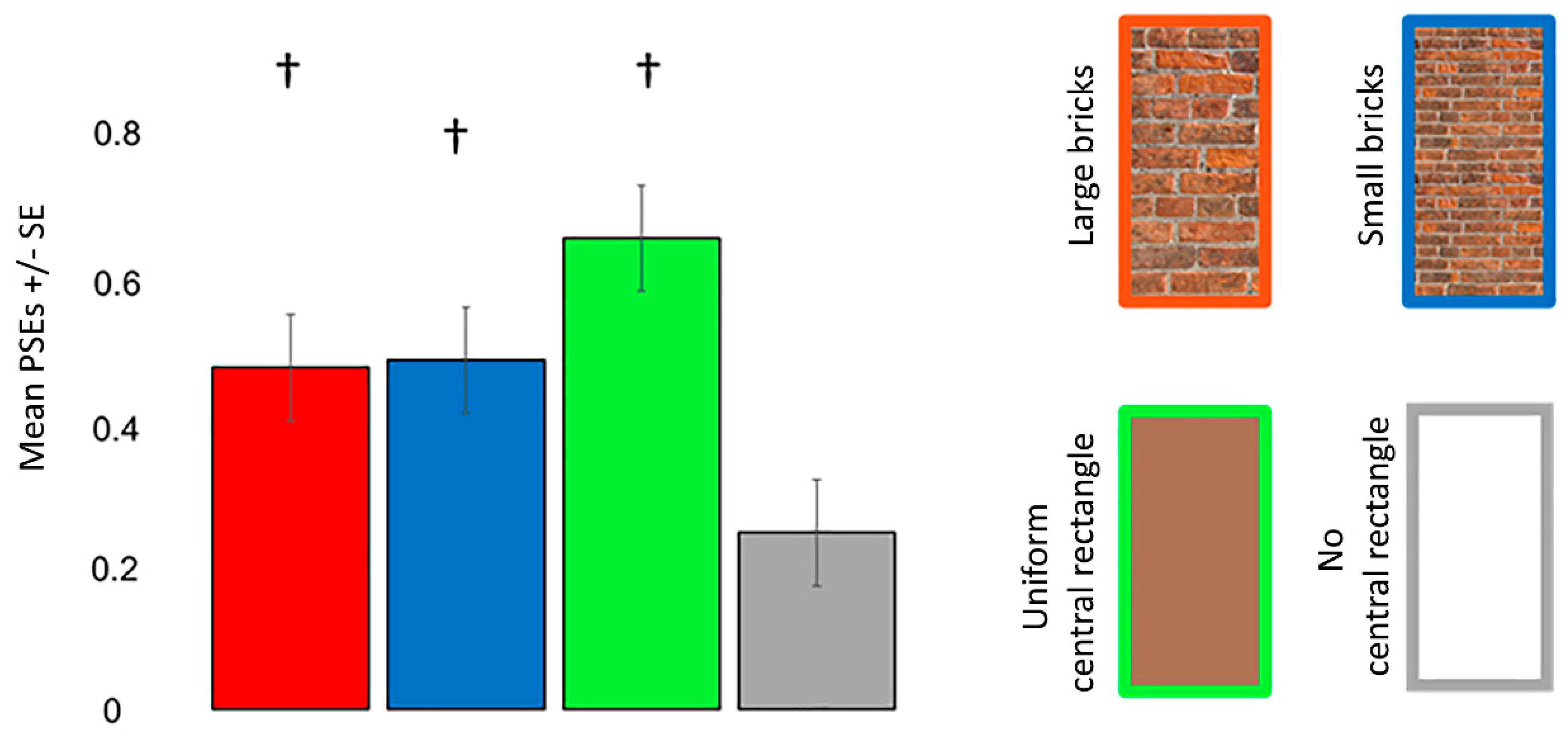
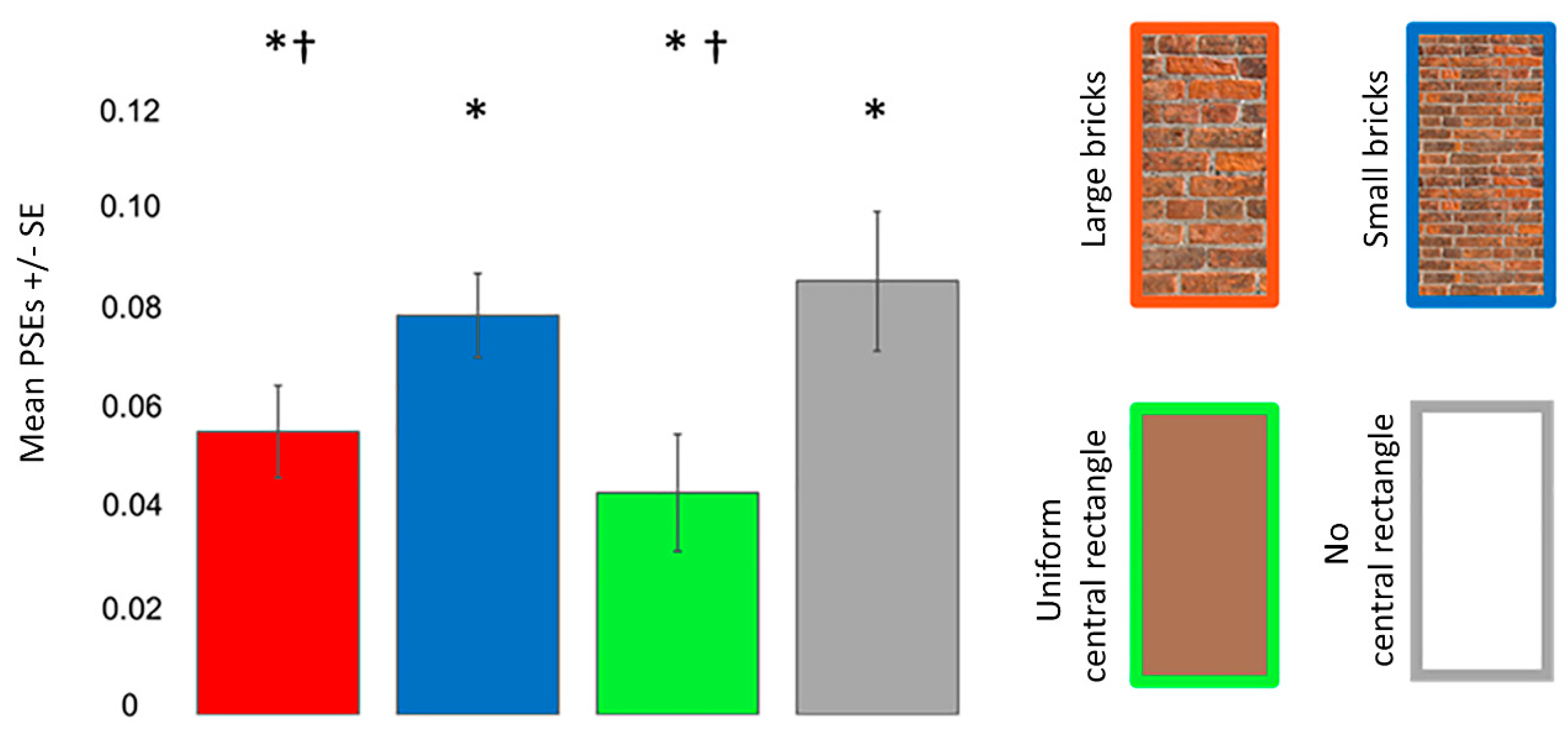
The present investigation examined the effects of adding pictorial depth cues to the Poggendorff illusion. For experiments 1 and 2, we reasoned that if the illusion is driven by perceptual size rescaling mechanisms, then the central rectangle with large bricks, relative to the one with small bricks, would be perceived to be more narrow and would also produce a greater perceptual misalignment of the oblique lines. More broadly, we expected that the illusion strength would increase under conditions where participants perceived a narrower central rectangle. Our results did not reveal any differences in illusion strength between the central rectangles with large and small bricks, nor did they indicate differences between their perceived widths. Although there was evidence of perceptual size rescaling whereby the central rectangle with large bricks was perceived as narrower than the no central rectangle condition and the central rectangle with small bricks was perceived as wider than the uniform central rectangle, these changes did not affect the strength of the illusion. Further, illusion strength in experiment 1 did not correlate with the perceived width of the central rectangle in experiment 2.
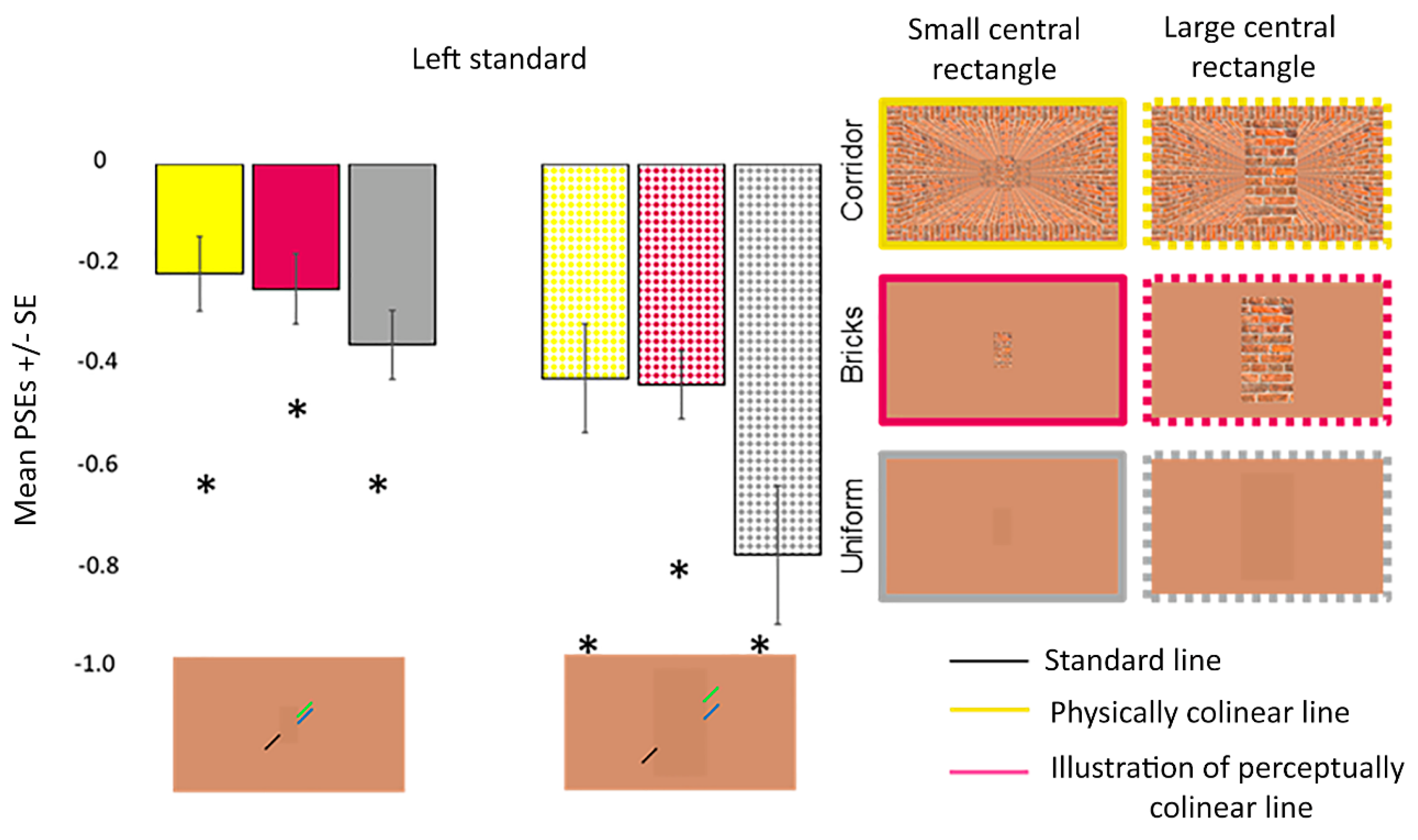
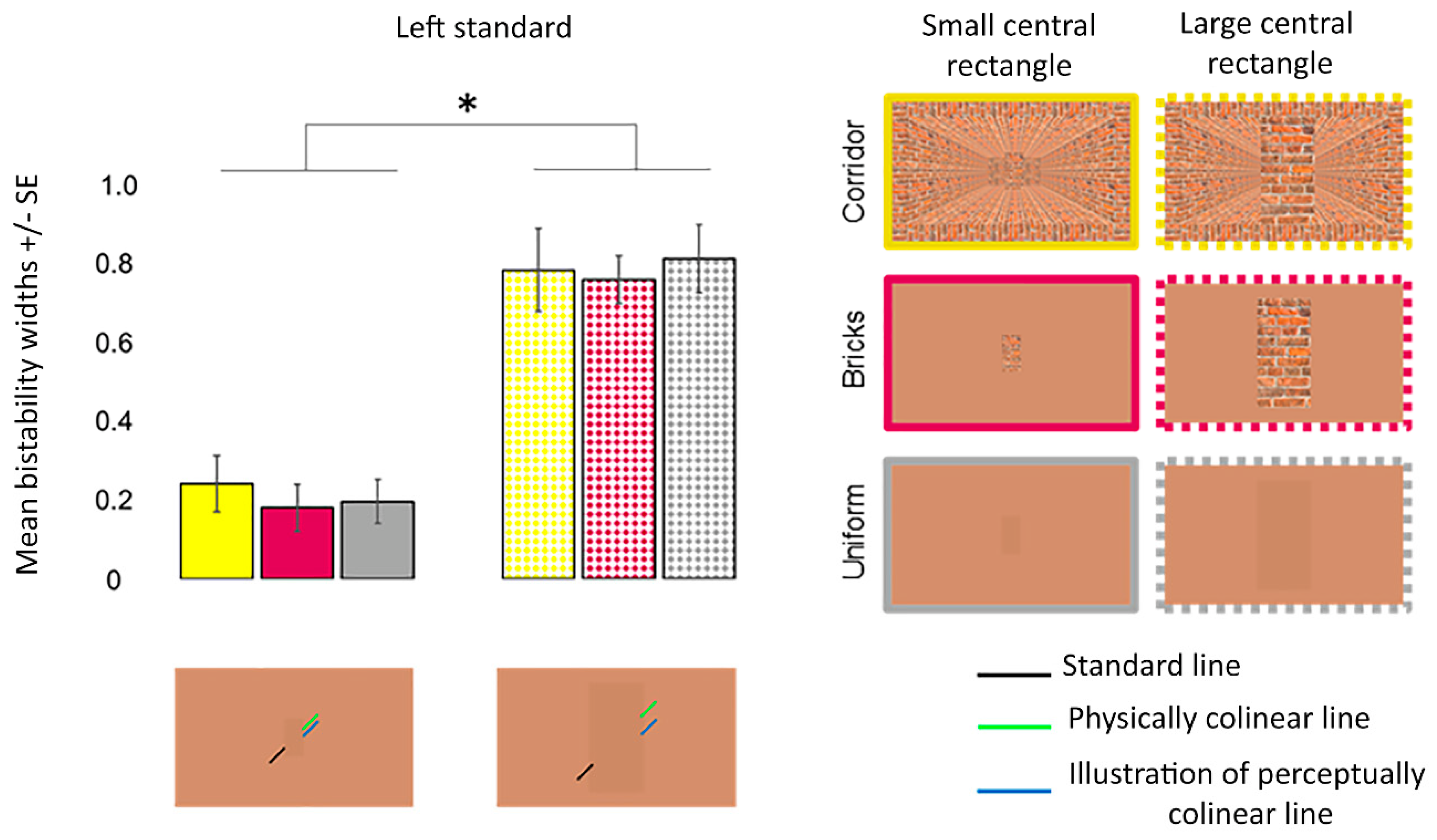
In experiment 3, we tested whether adding pictorial depth cues to both the central rectangles and the background image display would affect the Poggendorff illusion. Contrary to our hypothesis, our results revealed that adding pictorial depth cues to both the central rectangles and the background decreased rather than increased the strength of the illusion. Illusion strength also changed depending on the size of the central rectangle. The large central rectangle produced a stronger illusion than the small central rectangle. Overall, the results of the three experiments suggest that the illusion is not driven by perceptual rescaling mechanisms that shrink the apparent width of the central rectangle. On the other hand, adding pictorial depth cues seems to decrease the strength of the illusion, which is what Gillam [
11], as we will discuss in more detail later, predicted based on her reasoning that adding pictorial depth cues increases rather than decreases the certainty of the linear perspective created by the oblique lines. The following discussion focuses on how the results might relate to low-level and more ‘cognitive’ based theories to explain the illusion and argue that it could be the combination of both given our results. Throughout the discussion, we use the term ‘cognitive’ loosely to denote explanations that are not predominantly based on bottom-up processes fulfilled by retinal, thalamic and early visual areas and that place more importance on other processes mediated elsewhere in the brain or their top-down influences.
4.1. Empirical Arguments for and against Theories That Explain the Poggendorff Illusion with Mechanisms Related to Depth Perception
Talasli and Inan [
3] explained the perceived misalignment in the Poggendorff illusion by mechanisms that play a role in extracting depth information from 2D images. The authors proposed that the central rectangle is treated as an occluder and that its supposed nearness causes an illusory misalignment in the oblique lines by inappropriately triggering perceptual size rescaling mechanisms. Namely, the central rectangle acting as an occluder is treated as being more narrow than it really is, consequently causing a supposed shift in the positioning of the oblique lines towards the midline and making them appear more misaligned. Our findings in experiment 3 demonstrating a stronger illusion for uniform central rectangles compared to central rectangles with bricks contradicts the Talasli and Inan [
3] study. However, our findings agree more with a different study showing that the presence of dot patterns can cause a decline in the strength of the Poggendorff illusion [
23]. Masini et al. [
23] examined how the magnitude of the Poggendorff illusion changes with variations in the textural composition of the central rectangle. They demonstrated that the strength of the Poggendorff illusion was greater when the central rectangle was either empty or completely filled with dot patterns. Based on these results, the authors suggested that the Poggendorff illusion’s strength decreases when there are dot patterns with gaps because these patterns can cause a perceptual enlargement in the width of the central rectangle. In line with this idea, our results revealed that the central rectangle with small bricks was perceived to be wider than the uniform one in experiment 2.
Gillam [
11] also postulated that learned processes related to the extraction of depth play an important role in the Poggendorff illusion but in a manner that differed from the one proposed by Talasli and Inan [
3] and that originally formulated the hypotheses for our study. Gillam [
11] did not predict an increase in the strength of the illusion with the perceived shrinkage of the central rectangle nor did she predict that adding pictorial depth cues like we did would increase its strength. Rather, Gillam [
11] proposed that the illusion is driven primarily by the brain treating the two oblique lines as different lines receding into depth, which in turn causes a perceptual distortion in their alignment. According to her account, known as depth processing theory, the addition of other pictorial depth cues in the background adds greater certainty to whether the oblique lines can be considered to be one line receding into the distance, causing a decline in the magnitude of their perceived misalignment.
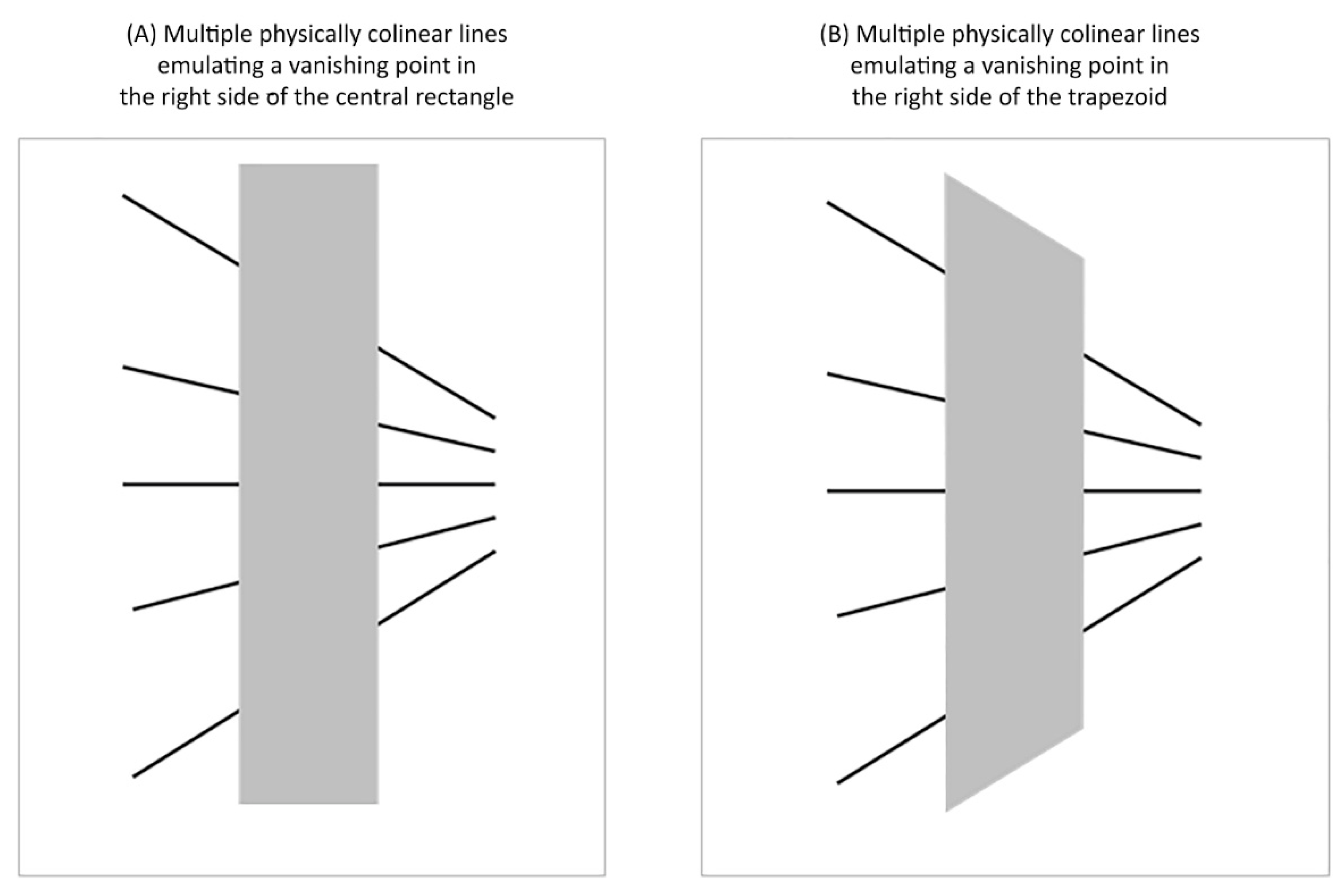
To aid clarity, imagine you are walking from point A to B inside a corridor. You see a yellow rectangle placed in front of a horizontal red line on one of the sidewalls (see
Figure 11A and
Video S1). If you take pictures of the yellow rectangle from the end of the corridor (e.g., point B in
Figure 11B,C, also see
Video S1), the rectangle and the line on the left sidewall would appear as in
Figure 11B, while the rectangle and the line on the right sidewall would appear as in
Figure 11C. Gillam [
11] proposed that when the parts of the central area are rescaled in size, as in
Figure 11B,C, the visual system processes the lines attached to the central area as if they are colinear horizontal lines receding into the distance. Thus, the perceptual rescaling of the central area serves as a pictorial depth cue, and this pictorial depth cue adds greater certainty as to whether the oblique lines can be considered to be one line receding into the distance, consequently causing a decline in the magnitude of their perceived misalignment. Contrarily, when the parts of the central area are not rescaled in size, as in the yellow central rectangle in
Figure 11D, the visual system does not process the oblique lines as a single horizontal line as in
Video S2. Rather, the visual system processes the points attached to the central rectangle as if they were placed at the same distance from the viewer in the 3D environment (see
Videos S3 and S4).
Gillam [
11] argued that when the attached points are at the same distance, this 2D representation could only result if the receding right line was placed at a lower position than the left one. Importantly, multiple lines and central rectangle positions might result in the same 2D representation (see
Figure 11E,F and
Videos S3 ans S4) [
6,
7,
24,
25]. Namely, the central rectangle can be interpreted as either an occluder placed in between the horizontal lines receding in depth (
Figure 11E,
Video S3) or a back wall placed at the termination point of two receding lines (
Figure 11F,
Video S4). As the physical properties of 3D objects are conflated in their 2D representations [
6,
7,
24], one may even argue that the central rectangle might be interpreted as a 2D representation of a 3D trapezoid with a shorter base placed at a nearer distance. Gillam’s [
11] theory asserts that it is this uncertainty about the position of oblique lines receding in the distance that creates an apparent misalignment in the oblique lines. In other words, when the central area is not perceptually rescaled, the absence of this pictorial depth cue creates an uncertainty about the positions of the receding lines in 3D space, which in turn causes an increase in the magnitude of their perceived misalignment.
Similar to these ideas, Gillam [
11] demonstrated that the strength of a typical Poggendorff illusion in
Figure 12A is stronger than the variant in
Figure 12B. She argued that this was due to a decrease in the certainty of the oblique lines representing continuous lines receding in the distance in the former case. Thus, according to Gillam, one way to diminish uncertainty about collinearity would be to increase the number of pictorial depth cues in the scene. In line with her predictions, we demonstrated that the strength of the Poggendorff illusion decreased as pictorial depth cues were added to the image display.
Many theories on the Poggendorff illusion do not consider depth processing and size constancy but instead ascribe a greater role to low-level mechanisms. For example, some have proposed that neural circuits in early visual areas related to the processing of acute and obtuse angles create the Poggendorff illusion [
26,
27,
28,
29]. Previously, Day and Dickinson [
30] demonstrated that the perceived misalignment of the oblique lines in the Poggendorff illusion could be driven by a combination of three different visual illusions driven by low-level mechanisms–namely, the horizontal–vertical, obtuse–acute angles and longitudinal–transversal illusions. The authors posited that mechanisms underlying the horizontal–vertical illusion operate so that the participants underestimate the horizontal distance between the two oblique lines while overestimating the vertical distance between them, similar to how Talasli and Inan [
3] explained how changes in the perceived width of the central rectangle could create a change in the apparent alignment of the oblique lines. In line with this explanation, Gregory [
2] proposed that the horizontal–vertical illusion is a simple visual illusion that is found in and drives many more complicated illusions.
With this in mind, there could be an alternative explanation as to why adding pictorial depth cues in the background might have decreased the illusion strength in experiment 3. Perhaps perceptual rescaling mechanisms drive the horizontal–vertical component of the Poggendorff illusion while low-level mechanisms drive the obtuse–acute angle component of the illusion. Our experiments provide evidence against the role of the horizontal–vertical component of the Poggendorff illusion by revealing that neither a physically nor perceptually narrower central rectangle produces greater misalignment.
Our findings cannot provide direct evidence for or against low-level explanations because our experiments were not designed to test them. Yet, some of our findings do corroborate these theories to some degree. For example, in experiment 3, we showed that the strength of the illusion varied as a function of the physical width of the central rectangle. Since effects of obtuse and acute angles on the strength of the Poggendorff illusion increase with an increase in the width of the central rectangle [
30], this finding can be seen as evidence for theories that explain the Poggendorff illusion with low-level mechanisms that play a role in processing obtuse and acute angles by early visual areas.
Yet, studies showing Poggendorff illusions during sequential [
31], dichoptic [
32] and illusory contour [
33] presentations have challenged these low-level explanations. For example, Sugita et al. [
31] investigated the temporal and spatial integration of the illusion by presenting the oblique lines and the central rectangle briefly (16.7 ms) sequentially in different orders (i.e., lines first versus occluder first) with various interstimulus intervals. The results showed that temporally separating the central rectangle 50 ms before to 200 ms after the presentation of the oblique lines induced a significant illusion with especially strong effects when the central rectangle was presented 100 to 150 ms after the oblique lines. Based on these findings, the authors concluded that the initial representation of oblique lines in iconic memory was altered by subsequent processing of the central rectangle, suggesting that the illusion might not be confined to low-level mechanisms.
Along the same vein, in an fMRI study, Shen et al. [
33] demonstrated that a Poggendorff illusion can occur with the creation of an apparent central area from Kanizsa-like illusory stimuli. The authors further reported common neural activation patterns for the Poggendorff illusion produced from physical and illusory contours, particularly in areas of the parietal lobe. Interestingly, we also report a significant Poggendorff illusion arising from the no central rectangle condition in experiment 1. It could be the case that these effects were driven by imagery. Taken together, these observations suggest once again that the illusion might not be confined to low-level mechanisms.
4.2. Methodological Considerations
One could argue that the effects induced by our central rectangles with small and large brick patterns on how the brain treats distance may have not been strong enough to cause a perceptual distortion in their widths. Talasli and Inan [
3] asked their participants to report the apparent distance of their central rectangles. In total, 27 of their 34 participants reported the central rectangle with the small bricks to appear more distant than the one with large bricks. We did not perform this test and remain sceptical about the validity of the perceived distances reported by Talasli and Inan [
3] because people are consciously aware that 2D images have no depth. It is more likely that these reports were influenced by response biases [
34].
Gregory [
9,
35,
36] acknowledged this problem years ago by noting the paradoxical nature of 2D images with pictorial depth cues. According to him, perceptual rescaling mechanisms operate so that the brain extracts depth information from 2D representations of 3D scenes automatically. This automaticity causes the visual system to still process depth information arising from pictorial cues despite people’s conscious awareness that pictures have no real depth and the conflicting extra-retinal cues, such as vergence and accommodation, telling the brain that pictures are flat. To overcome this problem and properly test for perceived distance differences induced by pictorial depth cues without these other influences, Gregory [
35] constructed a device called a Pandora’s box that made use of mirrors and polarising lights to create illusion stimuli viewed monocularly that appeared to subtend in space.
Several studies have used this device to examine how the brain might treat pictorial input as depth cues in geometrical illusions. For example, Chevrier and Delorme [
13] examined the relationship between the strength of a Ponzo-like illusion and perceived depth. Their results revealed increased perceptual distortions in stimulus size when participants perceived a greater distance between stimuli placed at locations where pictorial depth cues signal little depth and stimuli placed at locations where pictorial depth cues signal greater depth. Likewise, future work could examine the relationship between perceived distance and the strength of the Poggendorff illusion using a Pandora’s box. Alternatively, the same principles as a Pandora’s box can be applied in a virtual reality experiment to remove all depth cues other than those that are part of the illusion stimulus.
Another consideration is whether the effects of textures in experiment 3 were driven by differences in spatial frequency compositions rather than differences in how they might represent depth. Since textures are defined by spatial frequencies, it is impossible to disentangle their effects at a sensory level. Nonetheless, given that we found no difference between the strength of the Poggendorff illusion produced by the central rectangles with small and large bricks in experiment 1, it is more likely the case that the effects of adding bricks in experiment 3 were driven by their conceptual processing of depth rather than by low-level processes related to their spatial frequency compositions. If low-level processes related to processing the spatial frequency composition of visual input affected the illusion then one would expect to see illusory effects of textures in both experiments 1 and 3.
Finally, one could argue that the presence of brick patterns in the corridor background in experiment 3 might have influenced the strength of the Poggendorff illusion by decreasing the saliency of the oblique lines. Indeed, our results revealed that reaction times tended to be slower when the central rectangle was presented on the corridor versus the uniform backgrounds. Thus, there was some evidence that there was a decrease in the saliency of the oblique lines when brick patterns were added to the background. Whether or not this could explain the decrease in illusion strength in experiment 3 is difficult to ascertain. However, our previous studies demonstrating increases in Ponzo illusion strength with the addition of pictorial depth cues would suggest otherwise [
17,
19,
20]. Presumably, the stimuli in these studies were also less salient over busier backgrounds with more pictorial depth cues.