1. Introduction
Size constancy in visual perception is a cognitive mechanism that allows us to perceive an object as having the same size even when seen at different distances [
1,
2] or when two objects at the same distance are seen from different perspectives, resulting in differential foreshortening. Indeed, we can maintain a veridical perception of the object as having the same physical properties, even though the retinal input may be different in each case. For instance, we perceive a dog as remaining the same size when it is standing nearby us and when it walks to a more distant location. Another example is when two objects are projected to different regions of the retina (i.e., fovea vs. periphery) with different levels of photoreceptor density and cortical magnification. It has been shown that an object appears smaller in the periphery than in the centre of the visual field [
3]. It is suggested that this is due to the structural properties of the visual field [
4] and, to some extent, a lack of spatial attention [
5]. This issue of visual size constancy across different regions of the retina (fovea vs. periphery) has a very direct analogy with the issue of tactile size constancy across different regions of the skin with different mechanoreceptor densities, RF sizes, and cortical magnification [
6,
7,
8].
Therefore, size constancy provides us with a stable perception of the world, although the retinal image is constantly changing, given that we and the objects around us move in the environment [
9]. There is evidence that these cognitive mechanisms may be present from birth [
10]. Similar to the tactile domain, due to the different sizes and shapes of tactile receptive fields (RFs) across body parts, compensatory mechanisms of tactile size constancy are necessary to allow a stable perception of touch. However, such mechanisms are sometimes incomplete, producing distorted representations of touch. Here, we provide empirical evidence suggesting that tactile constancy is determined by the nature of the stimulus.
There are several lines of evidence in the literature showing that visual size constancy is achieved not only by using information that derives from the retinal inputs but also from other extra-retinal signals that come from the eyes as well as other sensory organs [
2]. For example, the eyes converge when a person directs their gaze on an object close to them and diverge when the gaze is directed to a more distant object. This process provides rich information to the brain about three-dimensional depth. Moreover, other sensory modalities such as audition [
11] and proprioception [
12] provide useful information about distance. For instance, when we hear a sound that is linked with a visual event (e.g., lightning), we can figure out the distance of the visual event by estimating the temporal gap between the two stimuli (vision and audition).
Critically, it has been recently shown that the primary visual cortex (V1) is an important area in which these different signals are integrated, well beyond the mere processing of retinal information [
2], playing a key role in visual perception and perceptual constancy. Neuroimaging evidence in humans suggests that V1 activity better reflects the final perceived size of an object rather than the size of the retinal projection [
13,
14]. Research in how size constancy occurs in other sensory modalities, such as touch, has not been as extensive as for vision. However, recent evidence suggests that the primary somatosensory cortex (S1) can be considered a critical brain area that mediates tactile distance perception [
15]. Like in visual studies of V1, activity in S1 closely mirrored the participant’s perception of stimulus size.
A classical illusion in tactile size perception is the one described by Weber in his pioneering studies [
7], in which he found that the distance between two points touching the skin felt larger when applied to highly sensitive regions than to less sensitive regions. This phenomenon is known as Weber’s illusion. In the classical version of this study, Weber showed the presence of this illusion by comparing touches between different parts of the body. He found that there was a direct relationship between tactile size perception and tactile spatial acuity, a result that has been subsequently replicated several times by other studies [
16,
17,
18,
19].
Later research extended this notion by showing that analogous perceptual illusions are also present when the same region of the skin is stimulated using stimuli with different orientations [
20,
21,
22,
23,
24,
25]. In this respect, Longo and Haggard [
22] found that the perceived distance between two touches on the width of the hand dorsum was about 40% bigger than the same distance along the length of the hand. This bias mirrors the anisotropy in tactile acuity on the limbs [
7,
26] and the shape of tactile receptive fields (RFs) [
27,
28]. Interestingly, it has recently been shown that such anisotropy is also present in the temporal domain, where temporal intervals between touches across the hand were perceived as longer than those along the hand [
29]. Consistent with spatial anisotropy, temporal anisotropy did not appear on the palmar side of the hand. Moreover, changes in the perceived size of body parts produce corresponding changes in tactile distance perception [
19,
30,
31], suggesting the need for a reference to a body model that includes the proportion of body parts [
32].
Importantly, however, the magnitude of this illusion is significantly smaller than would be expected on the bases of sensitivity, cortical magnification, or RF geometry alone [
22]. The origin of this anisotropy is suggested to derive from the RFs’ natural oval shape on certain body regions, such as the dorsum of the hand, and a process of tactile size constancy that compensates for the distortions incompletely [
33]. In most previous studies investigating tactile anisotropy, this phenomenon was tested by presenting two distinct touches on the skin surface and asking people to make judgments about how far apart they felt. However, in everyday life, it is much more common for our skin surface to get into contact with objects rather than single touches. Therefore, it may be that additional information or cues in the tactile signal (i.e., a more substantial touch rather than just single points touching the skin) are necessary in order to accomplish complete constancy compensation, possibly through a lateral inhibition mechanism.
An exception is a study by Anema and colleagues [
16] that used a modified version of Weber’s illusion in which a solid object was placed on the participants’ forearm and hand. They found a larger estimation of the size of an object on the palm of the hand than on the forearm, consistent with Weber’s illusion. Moreover, they also tested participants for grasping responses, which showed an opposite pattern, with a larger hand opening for objects when they were on the forearm [
16]. They suggested that the greater aperture when grasping objects on the forearm than on the hand was due to greater uncertainty about object dimension because of the reduced receptor density in the arm. This result could be derived from the presence of a significant motor component in the task, namely, the fact that responses were given using the fingers’ aperture. Therefore, it remains unexplored whether the perceived size of an object can elicit the same amount of anisotropy on the dorsum of the hand that has been shown to be present when the distance between two touches has to be estimated in a pure perceptual context. Moreover, Anema and colleagues [
16] asked participants to estimate the objects’ size in absolute value, whereas, in the present study, we will ask participants to provide a relative size estimation of the objects while they are presented on the same skin region at different orientations (i.e., across vs. along). This procedure allows us to directly quantify the amount of compensation necessary to correct the irregular shape of the tactile RFs.
To address this issue, the current study investigates whether the large and highly consistent distortions that emerge when participants have to estimate the distance between two touches on the skin also occur when the size of an object touching the skin surface has to be estimated. We measured the anisotropy of tactile size perception on the back of the hand [
22]. In the distance condition, two pairs of touches defining different tactile distances were applied sequentially to the hand, one pair oriented proximodistally (along the hand) and the other oriented mediolaterally (across the hand). Participants made two-alternative forced-choice (2AFC) judgments of which distance felt larger. The method of constant stimuli was used to estimate biases in the perception of size as a function of orientation. Similarly, in the object condition, two pairs of objects defining different tactile sizes were applied sequentially to the hand, as above; in this case, the participants made 2AFC judgments of which size felt bigger. If the same extent of tactile size constancy applies to both the distance and size estimation of tactile stimuli, we should have the same level of anisotropy for both distance and size estimation. Instead, if a different extent of tactile size constancy mediates tactile distance and size estimation, the anisotropy should vary depending on the type of stimulation—i.e., points or objects.
3. Experiment 2
As mentioned above, it is possible that the results of Experiment 1, when objects were used as stimulators, may in part derive from an artefact of the stimulation per se. To control for this possibility, in Experiment 2, we used different objects to stimulate the proximodistal (i.e., along) and mediolateral (i.e., across) hands’ axes. The former was the same we used in Experiment 1 (i.e., flat surface), whereas additional objects slightly bent were used to stimulate the dorsum in the mediolateral axis (
Figure 1C). This has the effect of making the across and along objects stimulations equally effective in terms of the amount of surface of the skin touched. If the result of Experiment 1 when using objects is due, at least to some extent, to an artefact of the stimulation, we expect a reduction in the PSE value in the objects condition.
3.1. Material and Methods
Participants. Twenty participants (mean ± SD = 29.4 ± 10.4 years; 13 females) took part in Experiment 2. Nine participants were the same participants who had taken part in Experiment 1. Participants reported normal or corrected-to-normal vision and normal touch. Participants were all right-handed, as assessed by the Edinburgh Handedness Inventory [
39] (M = 93, range 71–100).
Stimuli. These were the same as in Experiment 1 except for the fact that the objects stimuli used to stimulate in the mediolateral dimension were slightly bent (34 degrees) to better cover the whole skin surface.
Procedure. Procedures were identical to Experiment 1 except that the researcher used different stimuli, namely, flat or bent, when applying them to the across and along dimensions using objects, respectively.
3.2. Results and Discussion
One participant showed an extremely low R-squared value in the objects condition (0.178) and was excluded from subsequent analyses. For the remaining 19 participants, the average R-squared value for the posts condition was 0.954 (range = 0.837–1) and for the objects condition was 0.917 (range = 0.738–1), indicating a good fit to the data that was slightly better for the posts stimulation condition than the objects stimulation condition (t(18) = 2.49, p = 0.02, dz = 0.57).
As in Experiment 1, PSEs for stimuli presented using posts were again significantly less than 1 (M ± SE = 0.853 ± 0.03,
t(18) = −4.87,
p = 0.0001,
d = 1.12; see
Figure 3), indicating a large anisotropy. Further, the magnitude of bias was similar in the two experiments. Unlike Experiment 1, for the objects condition, we found that PSEs were lower than 1 (M ± SE = 0.937 ± 0.03,
t(18) = −2.25,
p = 0.037,
d = 0.52) in the same direction as for the posts. Moreover, we found that these distorted representations for posts and objects were still significantly different (
t(18) = −2.33,
p = 0.03,
dz = 0.54).
As for Experiment 1, the mean slope for the posts (M ± SE ß = 7.4 ± 0.58) stimulation condition was significantly greater compared to the objects (M ± SE ß = 4.2 ± 0.40) stimulation condition (t(18) = 6.15, p = 0.0001, dz = 1.41). There was no correlation between the two conditions, r(18) = 0.30, p = 0.22.
To compare the PSE values for the posts and objects conditions between Experiments 1 and 2, we performed a repeated measure analysis of variance (ANOVA) with type of stimulation (posts, objects) as a within-participant factor and Experiment as a between-participants factor. This analysis revealed the significant main effects of type of stimulation, F(1,36) = 51.76, p < 0.001, ηp2 = 0.59, and of Experiment, F(1,36) = 4.20, p = 0.05, ηp2 = 0.10, which were modulated by a significant interaction, F(1,36) = 14.16, p < 0.001, ηp2 = 0.28. This derives from the fact that there was no differences in anisotropy for the posts between Experiment 1 and Experiment 2 (t(18) = −0.67, p = 0.50). However, for objects, the anisotropy was significantly different for Experiment 1 compared to Experiment 2 (t(18) = −3.92, p < 0.001).
These results replicate the anisotropy using the posts condition found in Experiment 1 and in many other studies. Moreover, it suggests that the reversed anisotropy seen in the objects condition of Experiment 1 may relate to a possible artefact created by the type of object used. Indeed, in Experiment 2, using bent objects for the mediolateral axis stimulation abolished the bias in representing the hand as narrower than it really is for this condition. Intriguingly, however, we found that the bias in representing the hand as wider than it really is was significantly lower in the objects condition compared to the posts condition. This indicates that the type of stimuli used, namely, posts or objects, may generate a different type of processing/compensation.
There is another substantial difference between the posts and objects conditions. Indeed, when we used posts of different sizes (i.e., 2, 3 and 4 cm), the same amount of skin surface was always stimulated. Instead, when we used the objects, increasing the length of the stimulus at the same time increased the amount of skin surface stimulated. This difference between the two types of stimulation may have, to some extent, contributed to the different biases in the two conditions. This issue is addressed in Experiment 3.
4. Experiment 3
In Experiment 2, we found that object stimulation produces anisotropy in the same direction as for the posts simulation; however, it was significantly reduced in its magnitude. A possibility is that this difference derives from the fact that in the objects condition, the surface area of the skin touched increases together with the length of the stimuli (i.e., 2, 3, 4 cm) since all stimuli have the same width. Therefore, the reduced anisotropy in the objects condition could be caused by the greater information provided to the skin by the object—i.e., more skin surface touched—in the objects condition compared to the posts condition. To test for this possibility, in Experiment 3, stimuli were the posts, as in the previous experiments, and two different types of objects. The first type of objects condition, named the different-surface condition, was as for Experiment 2, where the object’s length also increased as the dimension of the surface increased. In contrast, in the same-surface condition, when the object’s length was increased, the width was proportionally reduced, keeping the surface area of skin stimulated constant at 120 mm2. If the smaller anisotropy for the object than the post in Experiment 2 was due to the amount of surface touched, we should expect different anisotropies for the same- and different-surface stimulation conditions—i.e., different PSE values. By contrast, if the amount of surface stimulated is not the critical factor that causes this effect, we should not find any difference between the same and different conditions.
4.1. Material and Methods
Participants. Twenty participants (mean ± SD = 31.8 ± 13.8 years; 11 females) took part in Experiment 3. Four participants were the same participants who had taken part in Experiment 1 and Experiment 2. Participants reported normal or corrected-to-normal vision and normal touch. Participants were all right-handed, as assessed by the Edinburgh Handedness Inventory [
39] (M = 94, range 48–100).
Stimuli. These were the same as in Experiment 2 except for the fact that there was an additional object condition (same surface) in which objects were weighted for their dimension (
Figure 4). In particular, 2, 3, and 4 cm plastic objects were built to have the same surface area of 120 mm
2, distributed for the three objects differently as a function of their dimension: 2 cm: 2 × 0.6, 3 cm: 3 × 0.4, and 4 cm: 4 × 0.3.
4.2. Results and Discussion
Two participants showed low R-squared values in the objects with the different-surface condition (0.451 and 0.461) and so were excluded from subsequent analyses. For the remaining 18 participants, the average R-squared value for the posts condition was 0.924 (range = 0.833–1), for the objects with the different-surface condition was 0.933 (range = 0.744–1), and for the objects with the same-surface condition was 0.937 (range = 0.783–1), indicating a good fit to the data.
As for previous experiments, PSEs for stimuli presented using posts were again significantly less than 1 (M ± SE = 0.816 ± 0.03,
t(17) = −5.76,
p = 0.0001,
d = 1.36; see
Figure 5), indicating a large anisotropy. Further, the magnitude of bias was similar to the previous experiments. For both the object conditions (i.e., same and different surfaces), we found that PSEs values were not significantly different from 1 (same surface: M ± SE = 0.950 ± 0.05,
t(17) = −1.47,
p = 0.16,
d = 0.35; different surface: M ± SE = 0.974 ± 0.05,
t(17) = −0.97,
p = 0.35,
d = 0.23). As for Experiment 1 and 2, we found that distance estimation for posts were still significantly different from both the objects with the same (
t(17) = −3.07,
p = 0.007,
dz = 0.72) and different surfaces (
t(17) = −3.54,
p = 0.003,
dz = 0.83). However, there was no significant difference between the same and different objects conditions (
t(17) = 1.44,
p = 0.17,
dz = 0.34).
Moreover, the mean slope for posts (M ± SE ß = 6.0 ± 0.54) was significantly greater compared to same-surface objects stimulation conditions (M ± SE ß = 4.8 ± 0.40; t(17) = 2.12, p = 0.05, dz = 0.50), but not for different-surface objects stimulation conditions (M ± SE ß = 5.3 ± 0.49; t(17) = 1.17, p = 0.25, dz = 0.28). In addition, the mean slope for the different-surface objects condition was different compared to the same-surface objects condition, t(17) = 2.12, p = 0.05, dz = 0.50. The mean slope between the two objects conditions were highly correlated, r(17) = 0.83, p = 0.0001. Moreover, the slope when posts were used were correlated with the same-surface objects condition, r(17) = 0.59, p = 0.01) but not with the different-surface objects condition, r(17) = 0.41, p = 0.09. The fact that the slopes of posts and different-surface objects were comparable may be an indication that the task was slightly easier in these conditions compared to the same-surface objects condition. We are not sure about the reason for such a difference, though it may have been caused by the fact that the amount of skin surface touched across different conditions was constant for the posts and different-surface objects conditions.
Overall, the results of Experiment 3 show once again the presence of anisotropy using the posts as stimuli. Moreover, we found that the bias in representing the hand as wider than it really is was significantly lower in the objects condition compared to the posts condition. Critically, this effect is not modulated by the amount of skin surface stimulated as we did not find any difference in terms of PSEs between the same- and different-surface objects conditions.
6. General Discussion
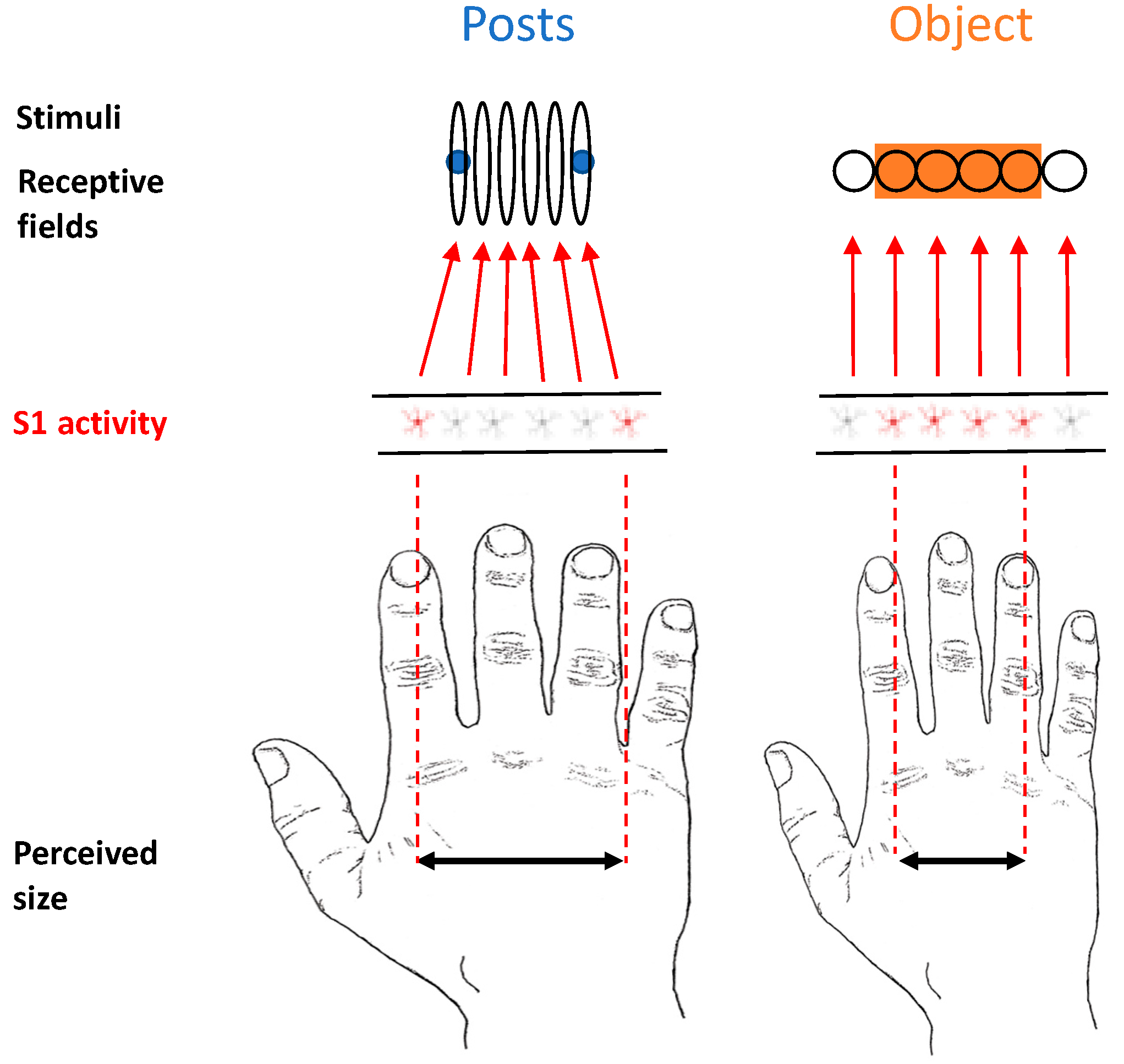
In the present study, we found that two touches on the dorsum of the hand are perceived as larger when they are oriented mediolaterally (across the hand) than proximodistally (along the hand). Critically, however, when controlling for artefacts deriving from the stimulation, we found that this bias was significantly reduced (Experiment 2) or vanished (Experiment 3) when the stimuli were continuous objects. Moreover, the absence of this bias with continuous objects was not due to the curvature of the objects (Experiment 2) and was independent of the amount of skin surface stimulated (Experiment 3). The anisotropy we found when stimulating with the posts is in accordance with early [
7] as well as more recent [
22,
26] reports that showed substantial anisotropy on the dorsum of the hand and in the shape of tactile RFs [
27,
28]. Therefore, our results for posts provide further evidence that such distortions may originate from primary somatosensory representations [
15].
As discussed elsewhere by Longo and Haggard [
22], such distortions are significantly reduced compared to what would be expected if they were simply reflections of the anisotropy of the RFs of individual neurons in SI. Therefore, it is likely that a process of tactile size constancy takes place to correct such distortions. In the case of objects, we propose that such a mechanism is simply more effective, given that the anisotropy was significantly reduced or absent. The somatosensory system thus appears capable of correcting substantially for anisotropic distortions but appears to do so less fully for distances than for continuous objects. In the next paragraphs, we will discuss a possible reason that may explain these effects and try to compare it with the size constancy effects and mechanisms that are currently proposed for visual perception.
The presence of anisotropy in tactile distance estimation when posts (
Figure 1A) are used to stimulate the dorsum of the hand has been previously explained as a consequence of several factors, such as the sensitivity of the skin, cortical magnification, or RF geometry [
33]. Moreover, given that such a factor would not be able to explain the magnitude of the anisotropy—i.e., such a phenomenon is reduced in magnitude compared to the difference in cortical magnification or tactile acuity—the authors proposed that a compensatory mechanism, though not complete, is taking place [
22]. We propose that a possible reason that leads to a more substantial form of
tactile size constancy when objects touch the skin rather than posts can be derived from a similar mechanism described for vision.
We propose that when objects rather than posts contact the skin, “background” information deriving from a lateral inhibition mechanism triggers a process in which tactile RFs, which are actually distorted on the hand dorsum, shift location, reducing their natural oval shape and becoming more circular (
Figure 7). In this respect, there is evidence in cats showing that injecting GABA antagonists in the primary somatosensory cortex produces asymmetric expansion of tactile RFs, making them even more elongated than they were at baseline [
44]. This suggests that one consequence of lateral inhibition is to make tactile RFs more circular than they would otherwise be. This can be considered a natural potential mechanism of size constancy. We now describe the rationale of our reasoning, discussing two lines of evidence that point us in this direction.
First, we know that lateral inhibition mechanisms affect the way in which we perceive an object that touches our skin. This is well described by the tactile version of the well-known optical Müller–Lyer illusion [
45]. In his book, von Békésy [
45] suggests that this illusion in touch is created by a lateral inhibitory effect. In that study, a cardboard stimulus with a rounded edge was applied to the skin with a rocking motion. The participants perceived the edge of the form as more obtuse than the actual pressure. Von Békésy interprets this result as an effect of lateral inhibition, which shifts the perceived peak of the cardboard towards the aperture of the shape. Therefore, we know that lateral inhibition plays a key role in the context of perception of an object on the skin surface.
Second, as we have described above, the shape of the tactile RFs on the hand dorsum in several regions of the nervous system is not circular but oval [
27,
28,
44], with the elongated part aligned with the proximodistal axis of the hand. It has been hypothesised that the tactile anisotropic effect for the posts can be due to this reason [
33]. Tactile RFs have different sizes and shapes across the body; in addition, they have also different distributions of their excitatory and inhibitory subfields. DiCarlo, Johnson, and Hsiao [
46], in a study on three alert monkeys, investigated the structure of tactile RFs in area 3b of the primary somatosensory cortex (S1) in terms of excitatory and inhibitory components of each RF. They studied neurons with RFs on the distal fingerpads with scanned random-dot stimuli. Overall, their results showed that most of the RFs (94% of the ones considered) contained a single central region of excitation. Interestingly, they also found that the RFs have one or more regions of inhibition located on one or more sides with respect to the excitatory centre. The shape area and strength of excitatory and inhibitory RF regions ranged widely. It is important to note that they reported that in 78% of the RFs, they found a continuous single region of inhibition positioned on one side of the excitation (52%), surrounding it partially (23%) or fully (3%). Interestingly, in the remaining 22% of the RFs, there were two regions of inhibition on opposite sides of the excitation [
46]. Finally, these authors reported an overall distal displacement of the inhibitory subfields relative to the excitatory centre that coincided with the scanning direction.
Posts’ stimuli have a sharp tip; therefore, it is reasonable to expect that they were producing a homogenous deformation of the skin surface in all directions. By contrast, objects have a wider shape; therefore, they were probably producing a lateralised deformation in one or more directions of the skin surface. Such a difference in stimulation may have, in turn, produced a distribution of the inhibitory areas in the tactile RFs that was different across the two conditions. We hypothesise that a non-uniform distribution of the inhibition could somehow provide information regarding the shape of the object. We know that different responses can be elicited as a function of the type of surface touching the skin, at least on the fingertip [
47,
48,
49]. Moreover, given the fact that tactile RFs on the hand dorsum have an oval shape, their inhibitory subfields may provide information about the actual shape of the RF itself. Namely, the elongated part in the proximodistal axis may have a greater inhibitory subfield in that direction compared to the not-elongated side. This information may allow the shape of the RF to be determined and, in turn, facilitate the computation of the actual skin surface stimulated. This may happen when an object is touching the skin and, therefore, continuously stimulating the RFs; however, this is not the case when the two posts touch the skin (i.e., uniform sharp touch). Such processing could have served as a tactile size compensatory mechanism that led to a greater correction for the distortions deriving from the tactile RFs’ shape in the case of the object compared to the posts. Therefore, lateral inhibition may provide information to overcome uniformity assumptions and produce a more effective compensatory process through a feedback signal sent to S1. This explanation would be compatible with a previously proposed hypothesis that suggests that distance and orientation on the skin are defined by the amount of skin intervening between two points [
50].
Our tactile RF-shifting theoretical model (
Figure 7) proposes that a lateral inhibition mechanism, when an object touches the skin, provides information through the distribution of the inhibitory subfields of the RF about the shape of the tactile RF itself. Such a process allows for an effective tactile size compensatory mechanism, where a good match between the physical and perceptual dimensions of the object is achieved. The fact that primary sensory areas play a critical role in size constancy has been shown in a recent study by Sperandio, Chouinard, and Goodale [
14] in the visual domain. In this study, the authors showed that the retinal size of an afterimage activity in the primary visual cortex (V1) reflects the perceived image. The central role of S1 in complex processing such as tactile size constancy is in accordance with previous literature showing that this “sensory” area may not be critical for solving simple tactile tasks—i.e., tactile simple detection—in both monkeys [
51] and humans [
52]. By contrast, S1 seems to be critically involved in processing that was thought to be performed by higher-level cortical areas such as the bilateral integration of touch [
53,
54,
55] as well as tactile working memory [
56,
57]. Moreover, in a recent fMRI study, we have shown that the tactile anisotropy on the hand dorsum seems to be mediated by the primary sensorimotor cortices [
15]. Such intimate relations with the sensory and motor cortices may play a critical role in the control of finely tuned movements and complex motor skills. In this respect, Tamè and colleagues [
53] found that activity in the somatosensory cortices (i.e., SI and SII) following repetitive (i.e., double) tactile stimulation causes finger-specific activation in the primary motor cortex, supporting the notion that spatial information is retained in SI and then transmitted to the motor cortex [
58]. Such a relation between the sensory and motor systems is particularly relevant in the context of haptic tasks, in which we are required to actively explore an object.
In vision, it has been shown that responses in the primary visual cortex reflect perceptual outcomes rather than retinal inputs in both microelectrode recordings of individual neurons in monkeys [
59] and fMRI responses in humans [
14,
60]. Recently, Ni and colleagues [
61], in a very interesting experiment in monkeys, in which they measured visual RF responses, have shown that the firing of neurons in V1 reflects the perceived size of an object rather than its actual size. Using extracellular recordings, they showed that neurons in V1 can shift the position of their RFs when subjected to complex monocular depth cues. During the task, monkeys looked at rings over a Ponzo Illusory background (i.e., a corridor). In this context the illusion makes monkeys perceive the two rings as different in size (one bigger than the other), even though their actual physical size is the same. In particular, the rings positioned on the back of the corridor are perceived as bigger compared to the rings at the front. While monkeys looked at the ring at the back of the corridor, their vRFs shifted towards the centre of the rings. In contrast, when the rings appeared at the front of the corridor, their vRFs shifted outward. Ni and colleagues proposed that the size illusion derives from this shift of vRFs. Moreover, they suggested that such movement cannot be explained by the low-level features of the stimuli or by attentional factors. They suggested that visual RF movement is guided by higher-level stages of processing, which extract complex depth information from the picture background and then feedback this information to V1 to shift the position of the vRFs (see the model they proposed in Ni and colleagues [
61]). A similar effect has also been found in a study conducted a year later in humans using fMRI-based population receptive fields (pRFs) in the visual cortex in combination with psychophysics [
62]. Compatible with the work of Ni and colleagues [
61], He and colleagues found that the far apparent rings in the scene caused the pRF positions of voxels in V1–V3 to shift toward the fovea, in line with participants’ percept of the Ponzo illusion. Moreover, they found that the pRF shift in V1 significantly correlated with the magnitude of the Ponzo Illusion [
62].
Finally, results showed quite consistently across experiments that the task was more difficult for the objects than the posts. Such a result can be explained by the fact that in the objects condition, differently from the posts condition, the same regions of the skin are stimulated in the comparison between the across and along stimuli. Indeed, the centre of the dorsum was both touched by the across and along stimulation. Therefore, it may be that the partially same region of the skin stimulated twice may have created some confusion or a masking effect that generally reduced participants’ performance.