New Equations for Hydrostatic Weighing without Head Submersion
Abstract
1. Introduction
- Derive new equations for HV prediction (HVPRED) from simple measurements without special equipment.
- Compare BF% by HWHBW to both:
- a.
- BF% by HWHAW with HVPRED;
- b.
- BF% by HWHAW from uncorrected “density”.
- Compare weight fluctuations in computer samples during HWHAW and HWHBW.
2. Materials and Methods
2.1. Participants
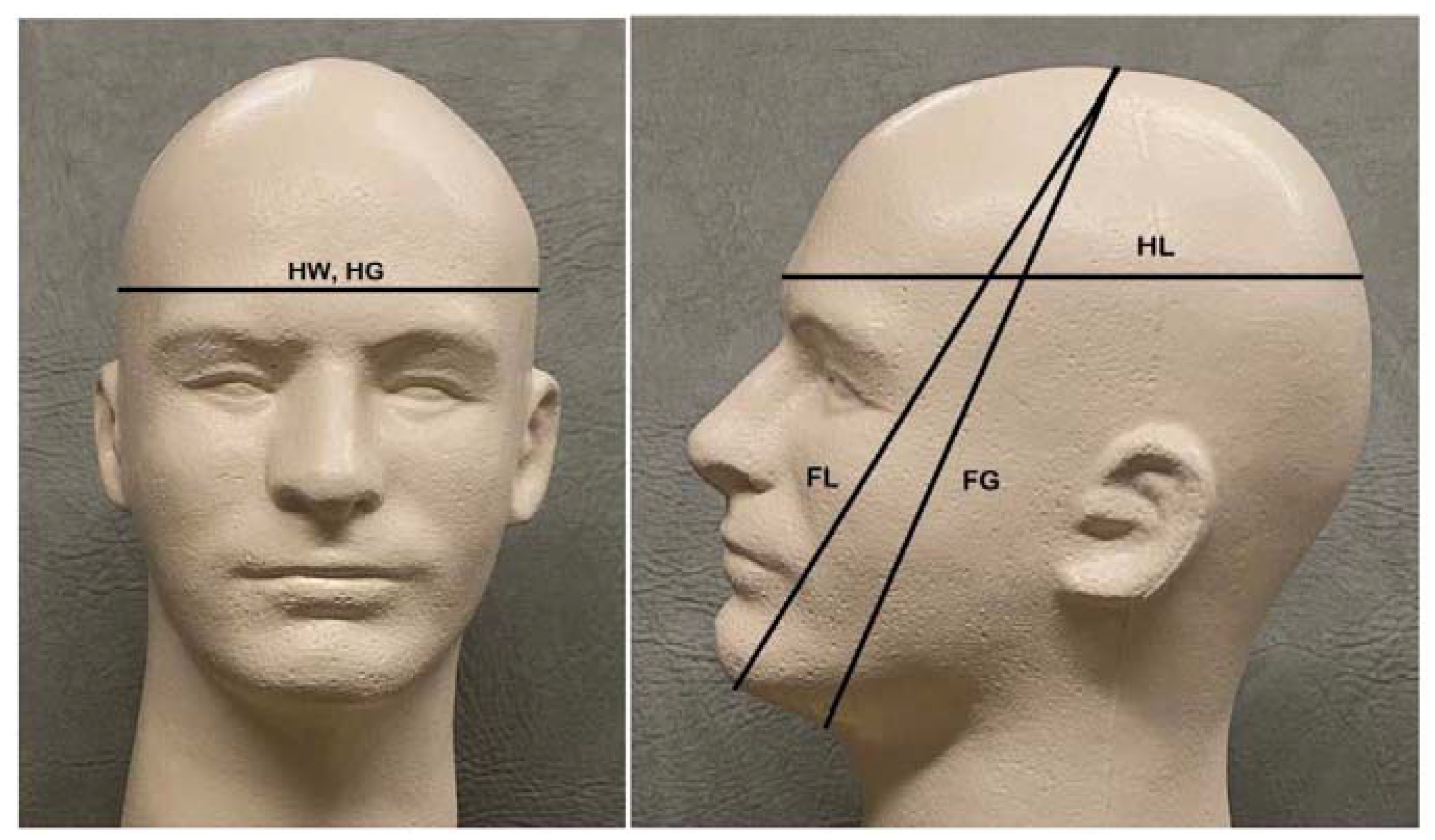
2.2. Anthropometrics
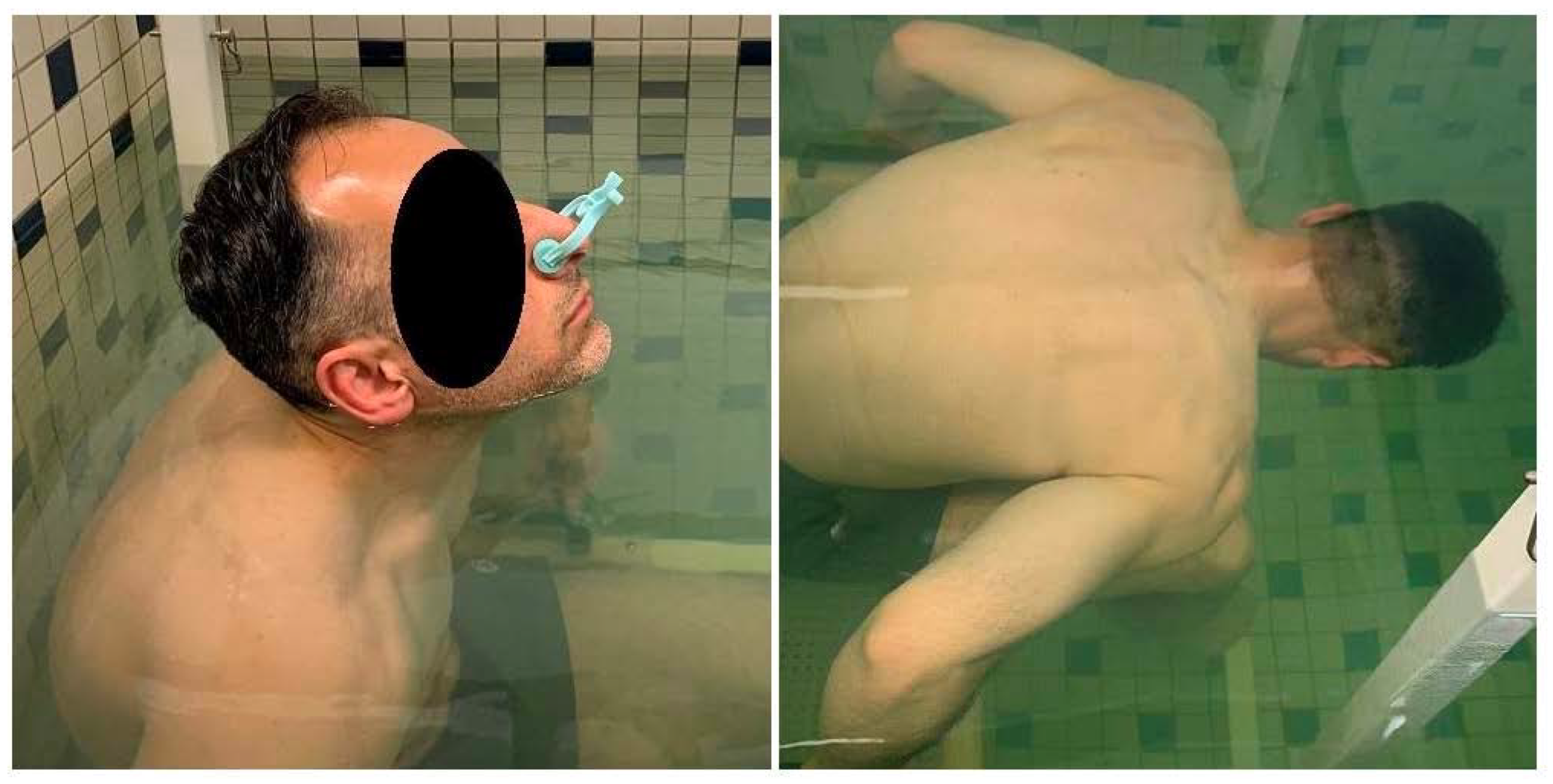
2.3. Hydrostatic Weighing
- Bend forward until the inferior surface of the chin and ear lobes just touched the water.
- Exhale maximally with the head positioned as described above and hold the breath at that level.
- Remain still for two or three seconds with the chin and ear lobes just touching the water.
- Hold breath at the same level and duck slowly under the water, until the head was completely immersed.
- Remain still for two or three seconds while completely immersed.
- Come up for air.
- Preliminary testing data collected before procedures became consistent (EXP group).
- Failure of potential participants to come for tests at appointed times.
- Fewer than 3 trials recorded for either MWHAW or MWHBW.
- A difference of more than 0.5 kg in MWHAW between any pair of the 3 trials.
- A difference of more than 0.5 kg in MWHBW between any pair of the 3 trials.
- Lack of a stable weight of 3 s duration for either MWHAW or MWHBW.
2.4. Statistical Analyses
3. Results
3.1. Head Volumes
3.2. Body Density and Percent of Fat
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Campa, F.; Toselli, S.; Mazzilli, M.; Gobbo, L.A.; Coratella, G. Assessment of Body Composition in Athletes: A Narrative Review of Available Methods with Special Reference to Quantitative and Qualitative Bioimpedance Analysis. Nutrients 2021, 13, 1620. [Google Scholar] [CrossRef]
- Kasper, A.M.; Langan-Evans, C.; Hudson, J.F.; Brownlee, T.E.; Harper, L.D.; Naughton, R.J.; Morton, J.P.; Close, G.L. Come Back Skinfolds, All Is Forgiven: A Narrative Review of the Efficacy of Common Body Composition Methods in Applied Sports Practice. Nutrients 2021, 13, 1075. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.M.; Pierson, R.N.; Heymsfield, S.B., Jr. The five-level model: A new approach to organizing body-composition research. Am. J. Clin. Nutr. 1992, 56, 19–28. [Google Scholar] [CrossRef] [PubMed]
- Garrow, J.S.; Stalley, S.; Diethelm, R.; Pittet, P.; Hesp, R.; Halliday, D. A new method for measuring the body density of obese adults. Br. J. Nutr. 1979, 42, 173–183. [Google Scholar] [CrossRef] [PubMed]
- Fields, D.A.; Hunter, G.R.; Goran, M.I. Validation of the BOD POD with hydrostatic weighing: Influence of body clothing. Int. J. Obes. Relat. Metab. Disord. 2000, 24, 200–205. [Google Scholar] [CrossRef]
- Wilmore, J.H. Body composition in sport and exercise: Directions for future research. Med. Sci. Sports Exerc. 1983, 15, 21–31. [Google Scholar] [CrossRef]
- Meyer, N.L.; Sundgot-Borgen, J.; Lohman, T.G.; Ackland, T.R.; Stewart, A.D.; Maughan, R.J.; Smith, S.; Müller, W. Body composition for health and performance: A survey of body composition assessment practice carried out by the Ad Hoc Research Working Group on Body Composition, Health and Performance under the auspices of the IOC Medical Commission. Br. J. Sports Med. 2013, 47, 1044–1053. [Google Scholar] [CrossRef]
- Dixon, C.B.; Deitrick, R.W.; Pierce, J.R.; Cutrufello, P.T.; Drapeau, L.L. Evaluation of the BOD POD and leg-to-leg bioelectrical impedance analysis for estimating percent body fat in National Collegiate Athletic Association Division III collegiate wrestlers. J. Strength Cond. Res. 2005, 19, 85–91. [Google Scholar]
- Hoeger, W.; Gonzalez, D.; Ransdell, L.; Gao, Y. A comparison of air displacement plethysmography and hydrostatic weighing techniques for the assessment of percent body fat in adults by gender and BMI category. Int. J. Body Comp. Res. 2011, 9, 89–94. [Google Scholar]
- Vescovi, J.D.; Zimmerman, S.L.; Miller, W.C.; Hildebrandt, L.; Hammer, R.L.; Fernhall, B. Evaluation of the BOD POD for estimating percentage body fat in a heterogeneous group of adult humans. Eur. J. Appl. Physiol. 2001, 85, 326–332. [Google Scholar] [CrossRef]
- Van Der Ploeg, G.E.; Withers, R.T.; Laforgia, J. Percent body fat via DEXA: Comparison with a four-compartment model. J. Appl. Physiol. 2003, 94, 499–506. [Google Scholar] [CrossRef] [PubMed]
- Goldman, R.; Buskirk, E. Body Volume Measurement by Underwater Weighing: Description of a Method; Brozek, J., Henschel, A., Eds.; National Academy of Sciences: Washington, DC, USA, 1961. [Google Scholar]
- Donnelly, J.E.; Smith-Sintek, S. Hydrostatic Weighing Without Head Submersion; Day, J., Ed.; Human Kinetics Publishers: Champaign, IL, USA, 1986. [Google Scholar]
- Evans, P.E.; Israel, R.G.; Flickinger, E.G.; O’Brien, K.F.; Donnelly, J.E. Hydrostatic weighing without head submersion in morbidly obese females. Am. J. Clin. Nutr. 1989, 50, 400–403. [Google Scholar] [CrossRef] [PubMed]
- Donnelly, J.E.; Brown, T.E.; Israel, R.G.; Smith-Sintek, S.; O’Brien, K.F.; Caslavka, B. Hydrostatic weighing without head submersion: Description of a method. Med. Sci. Sports Exerc. 1988, 20, 66–69. [Google Scholar] [CrossRef]
- Demura, S.; Sato, S.; Nakada, M.; Minami, M.; Kitabayashi, T. Comparison of estimation accuracy of body density between different hydrostatics weighing methods without head submersion. J. Physiol. Anthropol. Appl. Human Sci. 2003, 22, 175–179. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Nagao, N.; Matsueda, S.; Bunya, T.; Wakimoto, T.; Yamagata, T.; Nagao, M. A modified underwater weighing method without complete immersion. Kawasaki J. Med. Welfare 2008, 13, 75–82. [Google Scholar]
- Heath, E.M.; Adams, T.D.; Daines, M.M.; Hunt, S.C. Bioelectric impedance and hydrostatic weighing with and without head submersion in persons who are morbidly obese. J. Am. Diet. Assoc. 1998, 98, 869–875. [Google Scholar] [CrossRef]
- Buskirk, E. Underwater Weighing and Body Density: A Review of Procedures; Brozek, J., Henschel, A., Eds.; National Academy of Sciences: Washington, DC, USA, 1961. [Google Scholar]
- Weast, R.C.; Astle, M.J. (Eds.) Handbook of Chemistry and Physics, 60th ed.; CRC Press: Boca Raton, FL, USA, 1980. [Google Scholar]
- Katch, F.I. Practice curves and errors of measurement in estimating underwater weight by hydrostatic weighing. Med. Sci. Sports Exerc. 1969, 1, 212–216. [Google Scholar] [CrossRef]
- Quanjer, P.H. (Ed.) Standardized Lung Function Testing; Luxembourg European Community for Coal and Steel: Luxembourg, 1983. [Google Scholar]
- Brozek, J.; Grande, F.; Anderson, J.T.; Keys, A. Densitometric Analysis Of Body Composition: Revision Of Some Quantitative Assumptions. Ann. N. Y. Acad. Sci. 1963, 110, 113–140. [Google Scholar] [CrossRef]
- R Core Team. R: A Language and Environment for Statistical Computing; R Foundation for Statistical Computing: Vienna, Austria, 2019. [Google Scholar]
- Bland, J.M.; Altman, D.G. Statistical methods for assessing agreement between two methods of clinical measurement. Lancet 1986, 1, 307–310. [Google Scholar] [CrossRef]
- Lin, L.I. A concordance correlation coefficient to evaluate reproducibility. Biometrics 1989, 45, 255–268. [Google Scholar] [CrossRef]
- McBride, G. A Proposal for Strength-of-Agreement Criteria for Lin’s Concordance Correlation Coefficient; National Institute of Water & Atmospheric Research Ltd.: Hamilton, New Zealand, 2005. [Google Scholar]
- Coratella, G.; Campa, F.; Matias, C.N.; Toselli, S.; Koury, J.C.; Andreoli, A.; Sardinha, L.B.; Silva, A.M. Generalized bioelectric impedance-based equations underestimate body fluids in athletes. Scand. J. Med. Sci. Sports 2021, 31, 2123–2132. [Google Scholar] [CrossRef] [PubMed]
- Dixon, P.M.; Saint-Maurice, P.F.; Kim, Y.; Hibbing, P.; Bai, Y.; Welk, G.J. A Primer on the Use of Equivalence Testing for Evaluating Measurement Agreement. Med. Sci. Sports Exerc. 2018, 50, 837–845. [Google Scholar] [CrossRef] [PubMed]
- Burns, R.D.; Fu, Y.; Constantino, N. Measurement agreement in percent body fat estimates among laboratory and field assessments in college students: Use of equivalence testing. PLoS ONE 2019, 14, e0214029. [Google Scholar] [CrossRef]
- Harty, P.S.; Sieglinger, B.; Heymsfield, S.B.; Shepherd, J.A.; Bruner, D.; Stratton, M.T.; Tinsley, G.M. Novel body fat estimation using machine learning and 3-dimensional optical imaging. Eur. J. Clin. Nutr. 2020, 74, 842–845. [Google Scholar] [CrossRef] [PubMed]
- Tinsley, G.M.; Moore, M.L.; Benavides, M.L.; Dellinger, J.R.; Adamson, B.T. 3-Dimensional optical scanning for body composition assessment: A 4-component model comparison of four commercially available scanners. Clin. Nutr. 2020, 39, 3160–3167. [Google Scholar] [CrossRef]
- Weltman, A.; Katch, V. Comparison of hydrostatic weighing at residual volume and total lung capacity. Med. Sci. Sports Exerc. 1981, 13, 210–213. [Google Scholar] [CrossRef]
- Claros, G.; Hull, H.R.; Fields, D.A. Comparison of air displacement plethysmography to hydrostatic weighing for estimating total body density in children. BMC Pediatr. 2005, 5, 37. [Google Scholar] [CrossRef]
- Jackson, A.S.; Pollock, M.L.; Graves, J.E.; Mahar, M.T. Reliability and validity of bioelectrical impedance in determining body composition. J. Appl. Physiol. 1988, 64, 529–534. [Google Scholar] [CrossRef]
- Lukaski, H.C.; Bolonchuk, W.W.; Hall, C.B.; Siders, W.A. Validation of tetrapolar bioelectrical impedance method to assess human body composition. J. Appl. Physiol. 1986, 60, 1327–1332. [Google Scholar] [CrossRef]
- Wellens, R.; Chumlea, W.C.; Guo, S.; Roche, A.F.; Reo, N.V.; Siervogel, R.M. Body composition in white adults by dual-energy x-ray absorptiometry, densitometry, and total body water. Am. J. Clin. Nutr. 1994, 59, 547–555. [Google Scholar] [CrossRef]
- Biaggi, R.R.; Vollman, M.W.; Nies, M.A.; Brener, C.E.; Flakoll, P.J.; Levenhagen, D.K.; Sun, M.; Karabulut, Z.; Chen, K.Y. Comparison of air-displacement plethysmography with hydrostatic weighing and bioelectrical impedance analysis for the assessment of body composition in healthy adults. Am. J. Clin. Nutr. 1999, 69, 898–903. [Google Scholar] [CrossRef] [PubMed]
- Clark, R.R.; Kuta, J.M.; Sullivan, J.C. Prediction of percent body fat in adult males using dual energy x-ray absorptiometry, skinfolds, and hydrostatic weighing. Med. Sci. Sports Exerc. 1993, 25, 528–535. [Google Scholar] [CrossRef] [PubMed]
- Vescovi, J.D.; Hildebrandt, L.; Miller, W.; Hammer, R.; Spiller, A. Evaluation of the BOD POD for estimating percent fat in female college athletes. J. Strength Cond. Res. 2002, 16, 599–605. [Google Scholar]
- Utter, A.C.; Goss, F.L.; Swan, P.D.; Harris, G.S.; Robertson, R.J.; Trone, G.A. Evaluation of air displacement for assessing body composition of collegiate wrestlers. Med. Sci. Sports Exerc. 2003, 35, 500–505. [Google Scholar] [CrossRef] [PubMed]
- Kohrt, W.M. Body composition by DXA: Tried and true? Med. Sci. Sports Exerc. 1995, 27, 1349–1353. [Google Scholar] [CrossRef]
- Oliver, J.; Lambert, B.; Tanguay, J.; Bragg, A.; Green, J.; Crouse, S. Predicting body fat to the accuracy of DEXA using standard anthropometric measures. J. Strength Cond. Res. 2011, 25, S38–S39. [Google Scholar] [CrossRef]


| Males (Exp: n = 44, Val: n = 21) | Females (Exp: n = 46, Val: n = 24) | |||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| Mean ± SD | Range | r | Mean ± SD | Range | r | |||||||
| Exp | Val | Exp | Val | Exp | Val | Exp | Val | Exp | Val | Exp | Val | |
| Physical data | ||||||||||||
| Age (years) | 21.6 ± 3.3 | 22.1 ± 3.2 | 18–36 | 19–35 | 0.10 | −0.18 | 20.4 ± 1.2 | 20.5 ± 1.6 | 19–23 | 18–26 | 0.05 | 0.07 |
| Height (cm) | 182 ± 10 | 177 ± 8 | 166–210 | 161–189 | 0.22 | 0.49 | 168 ± 8 | 166 ± 7 | 152–185 | 144–175 | 0.49 | 0.05 |
| Weight (kg) | 83.3 ± 10.6 | 81.8 ± 13.5 | 64.7–116.8 | 62.5–118.2 | 0.37 | 0.48 | 65.2 ± 10.8 | 65.9 ± 8.2 | 41.0–96.6 | 51.6–79.8 | 0.65 | −0.05 |
| Girths (cm) | ||||||||||||
| Head girth | 57.7 ± 1.7 | 58.1 ± 1.5 | 55.0–62.0 | 55.5–61.0 | 0.72 | 0.77 | 55.5 ± 1.4 | 55.6 ± 1.7 | 51.5–58.5 | 53.0–60.0 | 0.79 | 0.58 |
| Face girth | 65.4 ± 2.0 | 66.3 ± 2.0 | 61.0–72.0 | 63.5–71.0 | 0.63 | 0.83 | 61.0 ± 1.9 | 61.8 ± 1.7 | 54.5–64.0 | 57.0–64.0 | 0.75 | 0.48 |
| Diameters (cm) | ||||||||||||
| Head length | 19.6 ± 0.9 | * | 17.0–21.5 | * | 0.50 | * | 18.9 ± 0.7 | * | 16.0–20.0 | * | 0.53 | * |
| Head width | 15.3 ± 0.6 | * | 14.0–17.0 | * | 0.39 | * | 14.6 ± 0.5 | * | 13.5–16.0 | * | 0.36 | * |
| Face length | 25.2 ± 0.8 | * | 23.0–26.5 | * | 0.45 | * | 23.7 ± 0.9 | * | 21.5–25.5 | * | 0.48 | * |
| Weights (kg) | ||||||||||||
| MWHAW | 8.51 ± 1.01 | 7.97 ± 1.10 | 6.22–10.92 | 5.47–9.95 | 0.43 | 0.36 | 5.76 ± 0.94 | 5.50 ± 0.80 | 3.35–8.29 | 4.04–7.66 | 0.70 | 0.52 |
| MWHBW | 4.39 ± 0.91 | 3.70 ± 1.03 | 2.33–6.57 | 1.52–5.39 | 0.06 | 0.02 | 2.13 ± 0.73 | 1.86 ± 0.68 | 0.60–3.86 | 0.21–3.12 | 0.37 | 0.04 |
| MWDH | 4.11 ± 0.38 | 4.27 ± 0.38 | 2.94–5.11 | 3.43–5.38 | 1.00 | 1.00 | 3.64 ± 0.39 | 3.65 ± 0.39 | 2.47–4.66 | 3.08–5.35 | 1.00 | 1.00 |
| Mean ± SD | CCC Analysis | SEE | ||||||||
|---|---|---|---|---|---|---|---|---|---|---|
| CCC | ρ | Cb | ||||||||
| Males (Exp: n = 44; Val: n = 21) | Exp | Val | Exp | Val | Exp | Val | Exp | Val | Exp | Val |
| Head volume (liters) | ||||||||||
| HVIMM (criterion) | 4.14 ± 0.38 | 4.30 ± 0.38 | ||||||||
| HVPRED | 4.14 ± 0.29 | 4.21 ± 0.28 | 0.72 | 0.76 | 0.75 | 0.83 | 0.96 | 0.93 | 0.2596 | 0.2333 |
| Body density (g·ml−1) | ||||||||||
| DBHBW (criterion) | 1.0727 ± 0.0102 | 1.0662 ± 0.0187 | ||||||||
| DBHAW[HV] | 1.0727 ± 0.0104 | 1.0674 ± 0.0187 | 0.94 | 0.99 | 0.94 | 0.99 | 1.00 | 1.00 | 0.0035 | 0.0029 |
| DBHAW[UD] | 1.0727 ± 0.0083 | 1.0709 ± 0.0157 | 0.79 | 0.91 | 0.81 | 0.96 | 0.98 | 0.95 | 0.0061 | 0.0052 |
| Fat percent (by weight) | ||||||||||
| BF%HBW (criterion) | 11.85 ± 4.08 | 14.55 ± 7.56 | ||||||||
| BF%HAW[HV] | 11.85 ± 4.14 | 14.07 ± 7.58 | 0.94 | 0.99 | 0.94 | 0.99 | 1.00 | 1.00 | 1.38 | 1.16 |
| BF%HAW[UD] | 11.85 ± 3.30 | 12.63 ± 6.30 | 0.79 | 0.91 | 0.81 | 0.96 | 0.98 | 0.95 | 2.41 | 2.10 |
| Females (Exp: n = 46; Val: n = 24) | ||||||||||
| Head volume (liters) | ||||||||||
| HVIMM (criterion) | 3.66 ± 0.39 | 3.67 ± 0.39 | ||||||||
| HVPRED | 3.66 ± 0.33 | 3.72 ± 0.29 | 0.84 | 0.54 | 0.85 | 0.57 | 0.99 | 0.95 | 0.2091 | 0.3425 |
| Body density (g·ml−1) | ||||||||||
| DBHBW (criterion) | 1.0528 ± 0.0134 | 1.0475 ± 0.0157 | ||||||||
| DBHAW[HV] | 1.0528 ± 0.0147 | 1.0469 ± 0.0170 | 0.97 | 0.94 | 0.97 | 0.95 | 1.00 | 1.00 | 0.0030 | 0.0052 |
| DBHAW[UD] | 1.0528 ± 0.0124 | 1.0491 ± 0.0154 | 0.92 | 0.92 | 0.93 | 0.93 | 0.99 | 0.99 | 0.0051 | 0.0061 |
| Fat percent (by weight) | ||||||||||
| BF%HBW (criterion) | 19.96 ± 5.56 | 22.16 ± 6.54 | ||||||||
| BF%HAW[HV] | 19.95 ± 6.08 | 22.43 ± 7.03 | 0.97 | 0.94 | 0.98 | 0.95 | 1.00 | 1.00 | 1.25 | 2.14 |
| BF%HAW[UD] | 19.95 ± 5.13 | 21.50 ± 6.35 | 0.92 | 0.92 | 0.93 | 0.93 | 1.00 | 0.99 | 2.11 | 2.50 |
| Comparison: | HVIMM, HVPRED | BF%HBW, BF%HAW[HV] | BF%HBW, BF%HAW[UD] |
|---|---|---|---|
| Males (n = 21) | |||
| Equivalence bounds | ±0.2 L | ±2.0% | ±2.0% |
| 90% CI (LL, UL) | (0.001, 0.165) | (0.052, 0.909) | (1.063, 2.767) |
| Equivalence? | Yes | Yes | No |
| Power | 0.99 | 1.00 | 0.98 |
| Minimum sample size 1 | 10 | 3 | 11 |
| t (p) | 1.75 (p = 0.095) | 1.93 (p = 0.067) | 3.87 (p = 0.001) * |
| Females (n = 24) | |||
| Equivalence bounds | ±0.2 L | ±2.0% | ±2.0% |
| 90% CI (LL, UL) | (−0.163, 0.065) | (−1.052, 0.525) | (−0.197, 1.527) |
| Equivalence? | Yes | Yes | Yes |
| Power | 0.83 | 0.99 | 0.98 |
| Minimum sample size 1 | 23 | 11 | 13 |
| t (p) | 0.74 (p = 0.466) | 0.57 (p = 0.572) | 1.32 (p = 0.199) |
| Investigation | N | Age ± SD | Criterion BF% | Estimated BF% | SEE | Method |
|---|---|---|---|---|---|---|
| Males | ||||||
| Biaggi et al., 1999 [38] | 23 | 33.3 ± 8.7 | 21.5 | 20.2 | 3.1 | ADP |
| Claros et al., 2005 [34] | 40 | 12.4 ± 1.3 | 18.7 | 18.4 | 3.0 | ADP |
| Dixon et al., 2005 [8] | 25 | 19.2 ± 1.2 | 14.5 | 13.8 | 1.7 | ADP |
| Utter et al., 2003 [41] | 66 | 20.2 ± 2.0 | 11.3 | 11.0 | 2.1 | ADP |
| Dixon et al., 2005 [8] | 25 | 19.2 ± 1.2 | 14.5 | 12.3 | 3.6 | BIA |
| Jackson et al., 1988 [35] | 50 | 36.7 ± 9.5 | 19.1 | 16.7 | 4.6 | BIA |
| Lukaski et al., 1986 [36] | 47 | 26.9 ± 8.0 | 16.2 | NR | 3.0 | BIA |
| Clark et al., 1993 [39] | 35 | 39.1 ± 14.0 | 17.4 | 21.3 | 3.0 | DXA |
| Wellens et al., 1994 [37] | 50 | 39.9 ± 13.7 | 22.5 | 21.7 | 2.3 * | DXA |
| Donnelly et al., 1988 [15] | 20 | NR | 15.9 | 15.9 | 1.6 | HWHAW[UD] |
| Present investigation | 21 | 22.1 ± 3.2 | 14.6 | 12.6 | 2.1 | HWHAW[UD] |
| Donnelly and Sintek, 1986 [13] | 11 | NR | NR | NR | 1.4 | HWHAW[HV] |
| Nagao et al., 2008 [17] | 27 | 21.8 ± 5.1 | 13.6 | NR | 1.3 | HWHAW[HV] |
| Present investigation | 21 | 22.1 ± 3.2 | 14.6 | 14.1 | 1.2 | HWHAW[HV] |
| Females | ||||||
| Biaggi et al., 1999 [38] | 24 | 30.7 ± 7.2 | 28.6 | 29.7 | 2.5 | ADP |
| Claros et al., 2005 [34] | 26 | 12.0 ± 1.9 | 20.4 | 18.9 | 3.8 | ADP |
| Vescovi et al., 2002 [40] | 80 | 20.2 ± 1.5 | 19.4 | 21.2 | 3.3 | ADP |
| Jackson et al., 1988 [35] | 82 | 28.5 ± 5.7 | 20.2 | 22.8 | 5.0 | BIA |
| Lukaski et al., 1986 [36] | 67 | 27.0 ± 6.4 | 25.1 | NR | 3.1 | BIA |
| Wellens et al., 1994 [37] | 78 | 42.5 ± 13.7 | 33.2 | 34.6 | 3.2 * | DXA |
| Donnelly et al., 1988 [15] | 20 | NR | 21.5 | 22.2 | 3.5 | HWHAW[UD] |
| Present investigation | 24 | 20.5 ± 1.6 | 22.2 | 21.5 | 2.5 | HWHAW[UD] |
| Nagao et al., 2008 [17] | 56 | 20.3 ± 1.8 | 20.1 | NR | 1.9 | HWHAW[HV] |
| Present investigation | 24 | 20.5 ± 1.6 | 22.2 | 22.4 | 2.1 | HWHAW[HV] |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tesch, J.C.; Papadopoulos, P.; Dolgener, F.; Tinsley, G.M. New Equations for Hydrostatic Weighing without Head Submersion. J. Funct. Morphol. Kinesiol. 2022, 7, 70. https://doi.org/10.3390/jfmk7030070
Tesch JC, Papadopoulos P, Dolgener F, Tinsley GM. New Equations for Hydrostatic Weighing without Head Submersion. Journal of Functional Morphology and Kinesiology. 2022; 7(3):70. https://doi.org/10.3390/jfmk7030070
Chicago/Turabian StyleTesch, Jeff C., Panayiotis Papadopoulos, Forrest Dolgener, and Grant M. Tinsley. 2022. "New Equations for Hydrostatic Weighing without Head Submersion" Journal of Functional Morphology and Kinesiology 7, no. 3: 70. https://doi.org/10.3390/jfmk7030070
APA StyleTesch, J. C., Papadopoulos, P., Dolgener, F., & Tinsley, G. M. (2022). New Equations for Hydrostatic Weighing without Head Submersion. Journal of Functional Morphology and Kinesiology, 7(3), 70. https://doi.org/10.3390/jfmk7030070






