Flow Instability Control in a Model Swirl-Stabilized Combustor with Central Jet Injection
Abstract
:1. Introduction
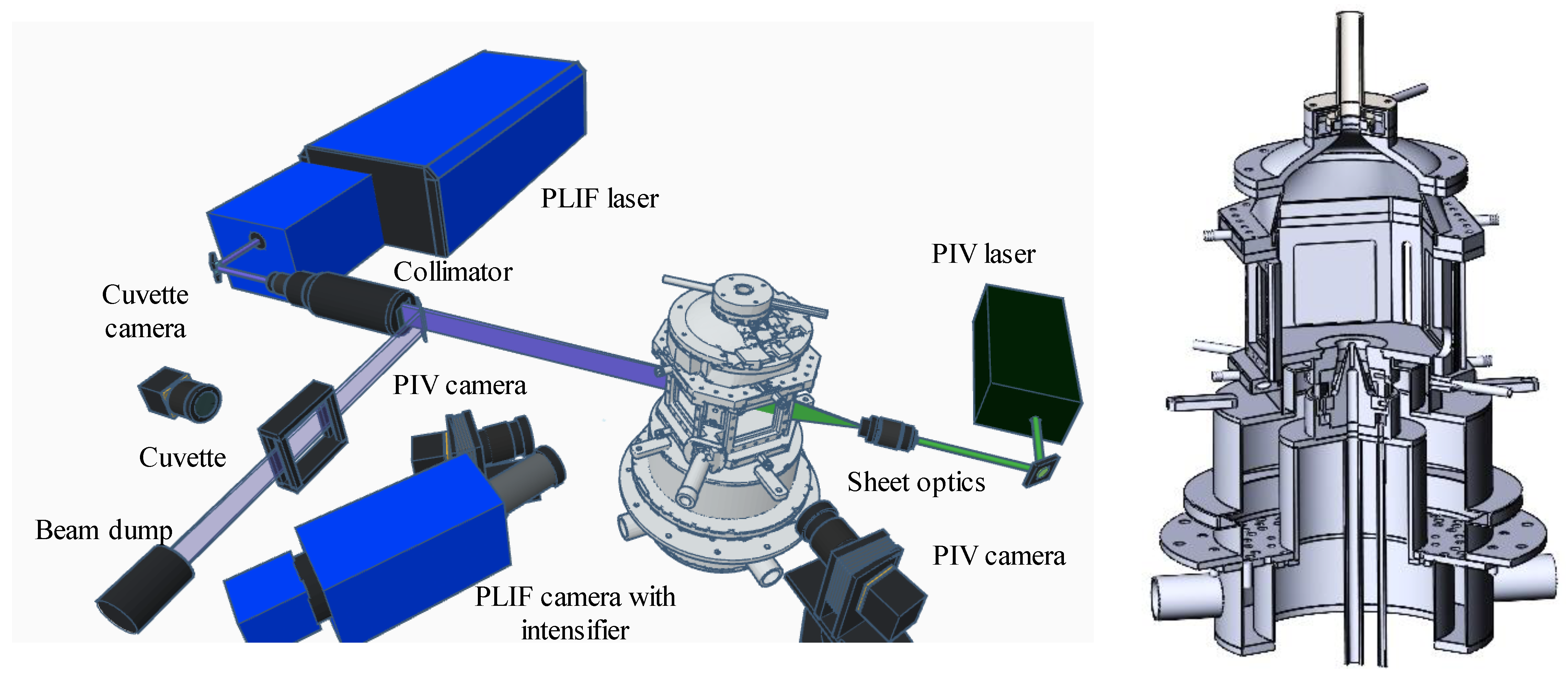
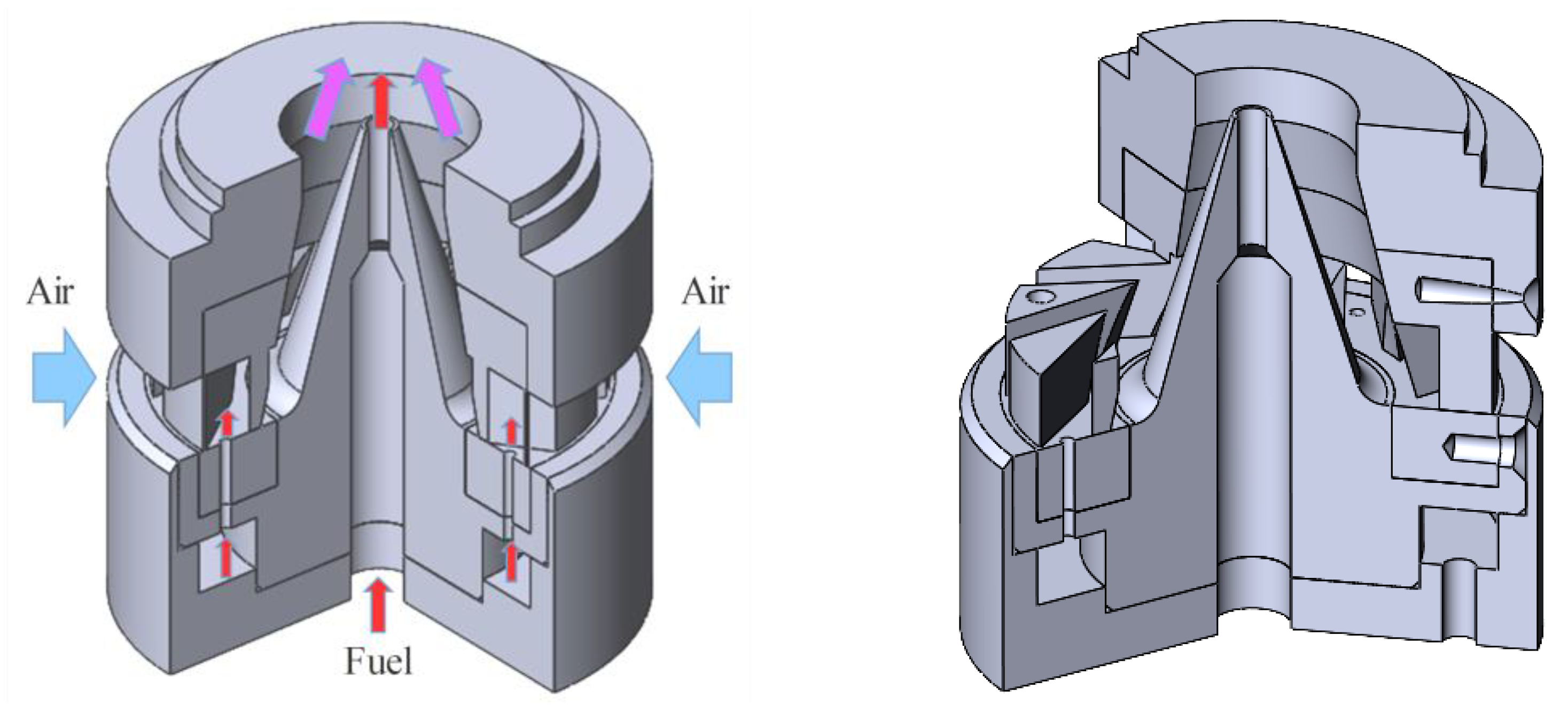
2. Materials and Methods
3. Results and Discussion
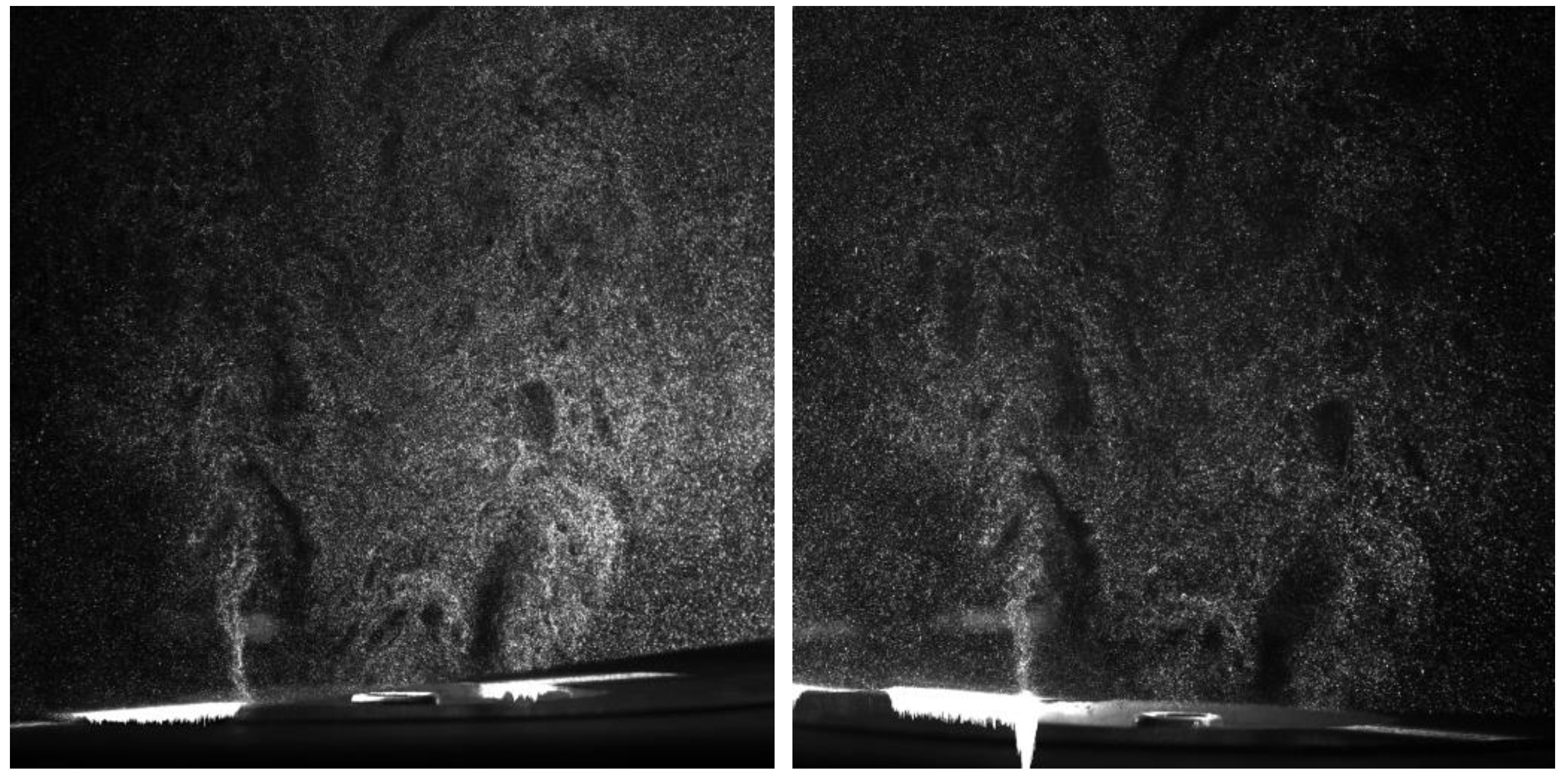
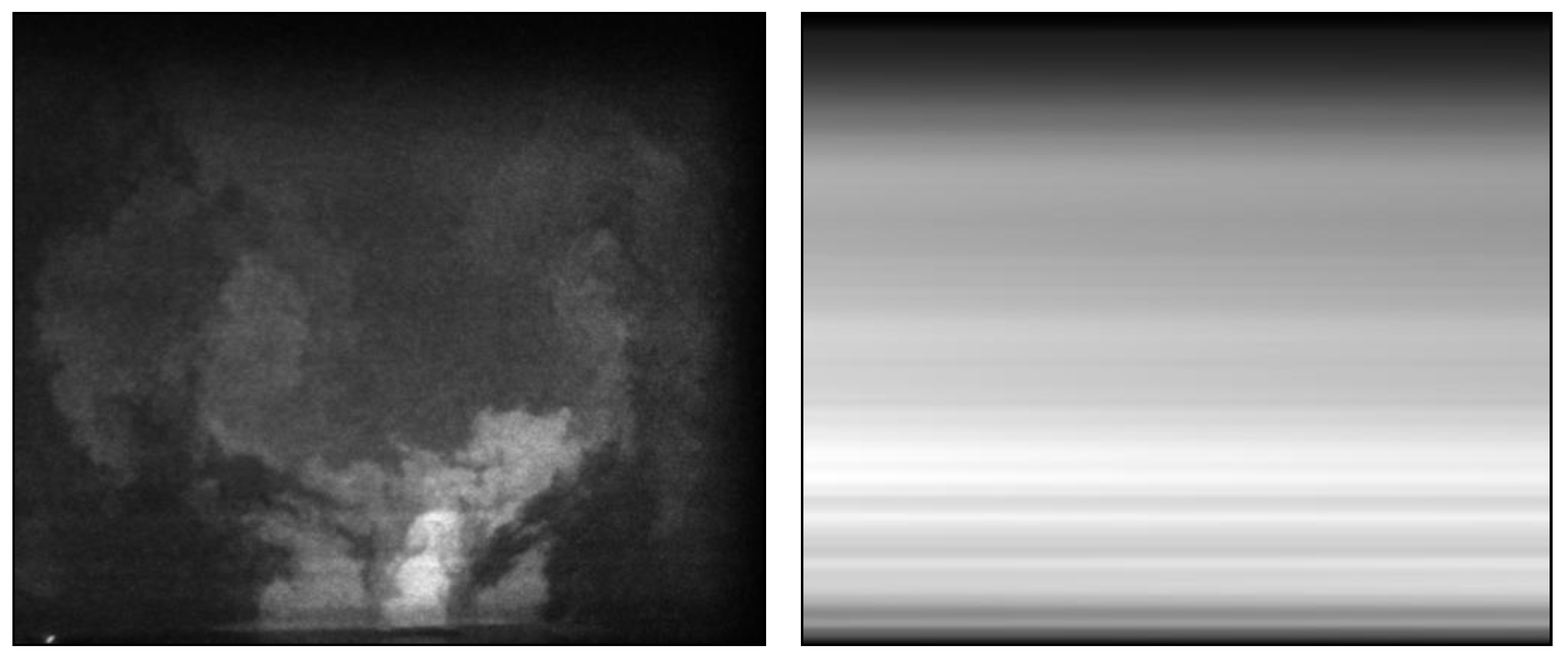
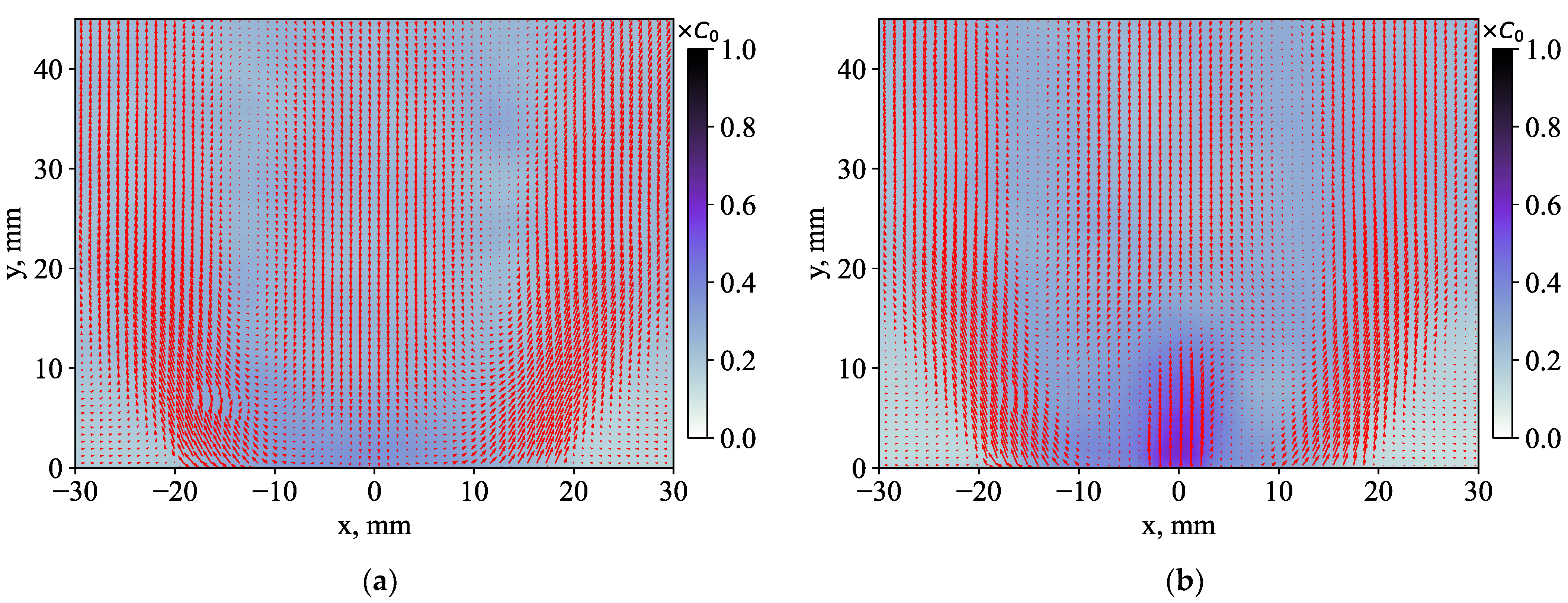
3.1. Instantenous Snapshots
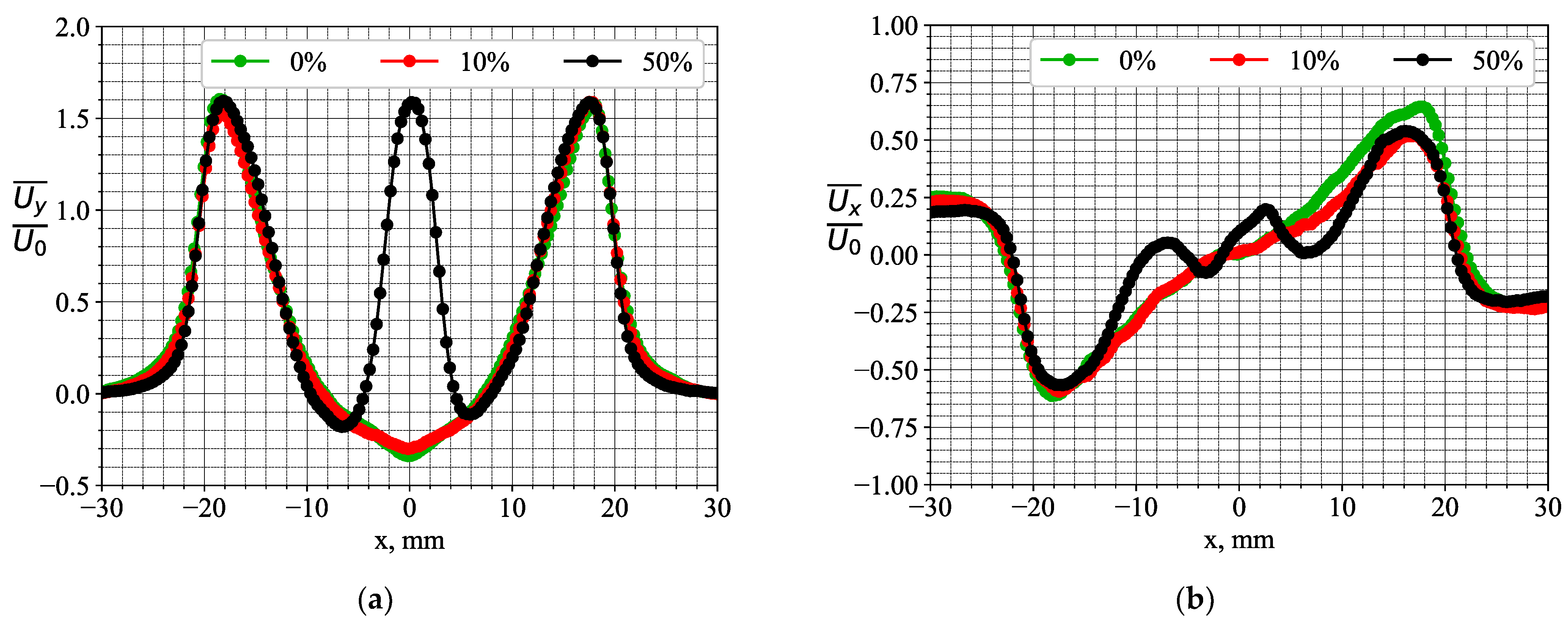
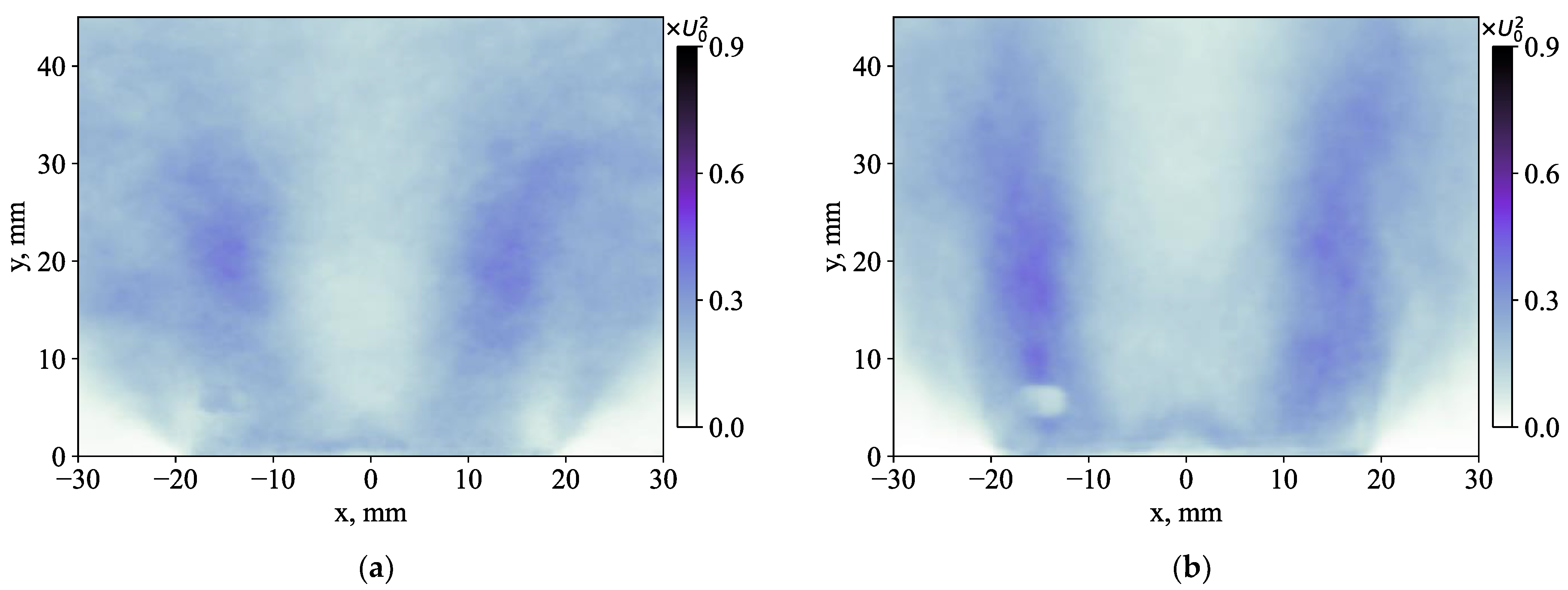
3.2. Time-Averaged Data
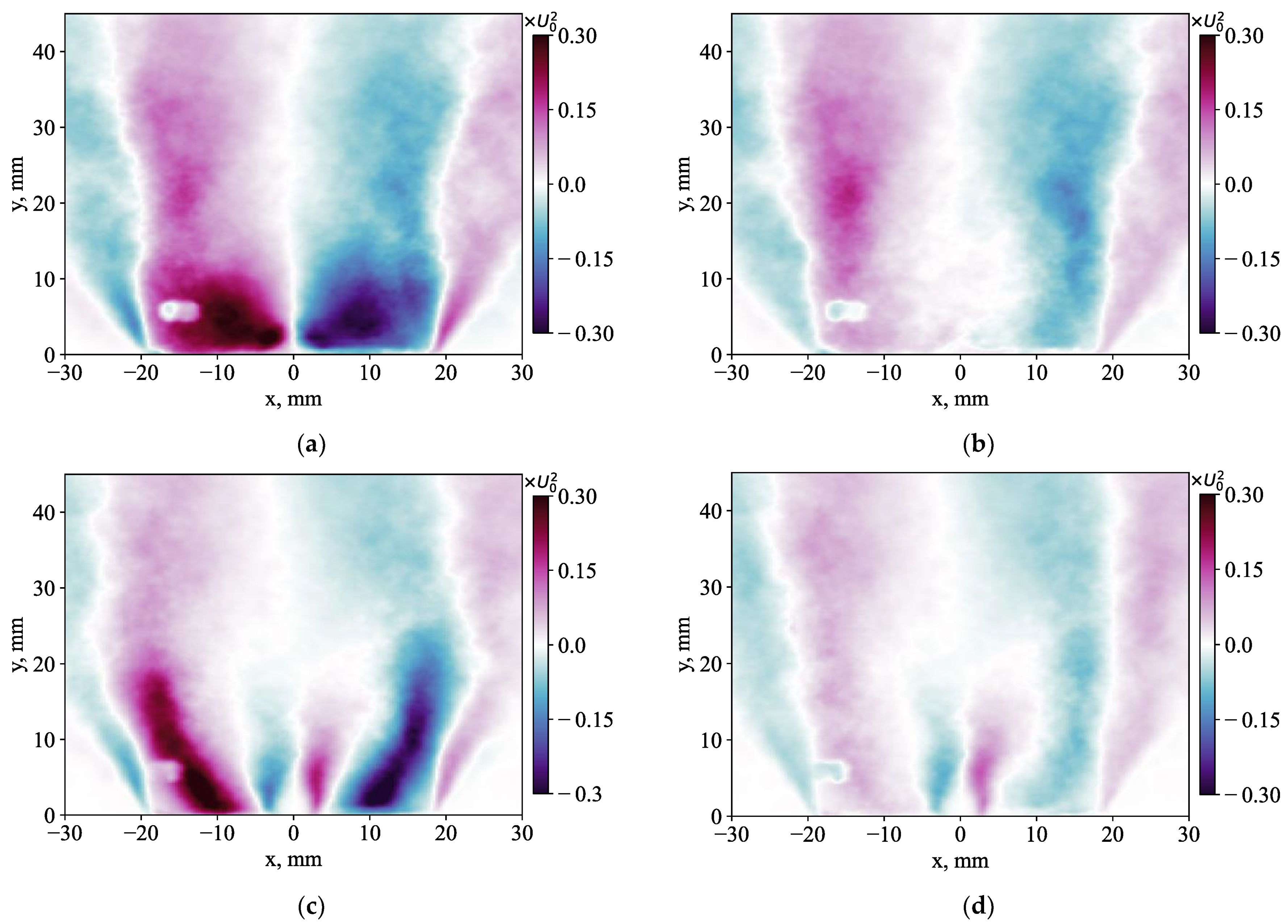
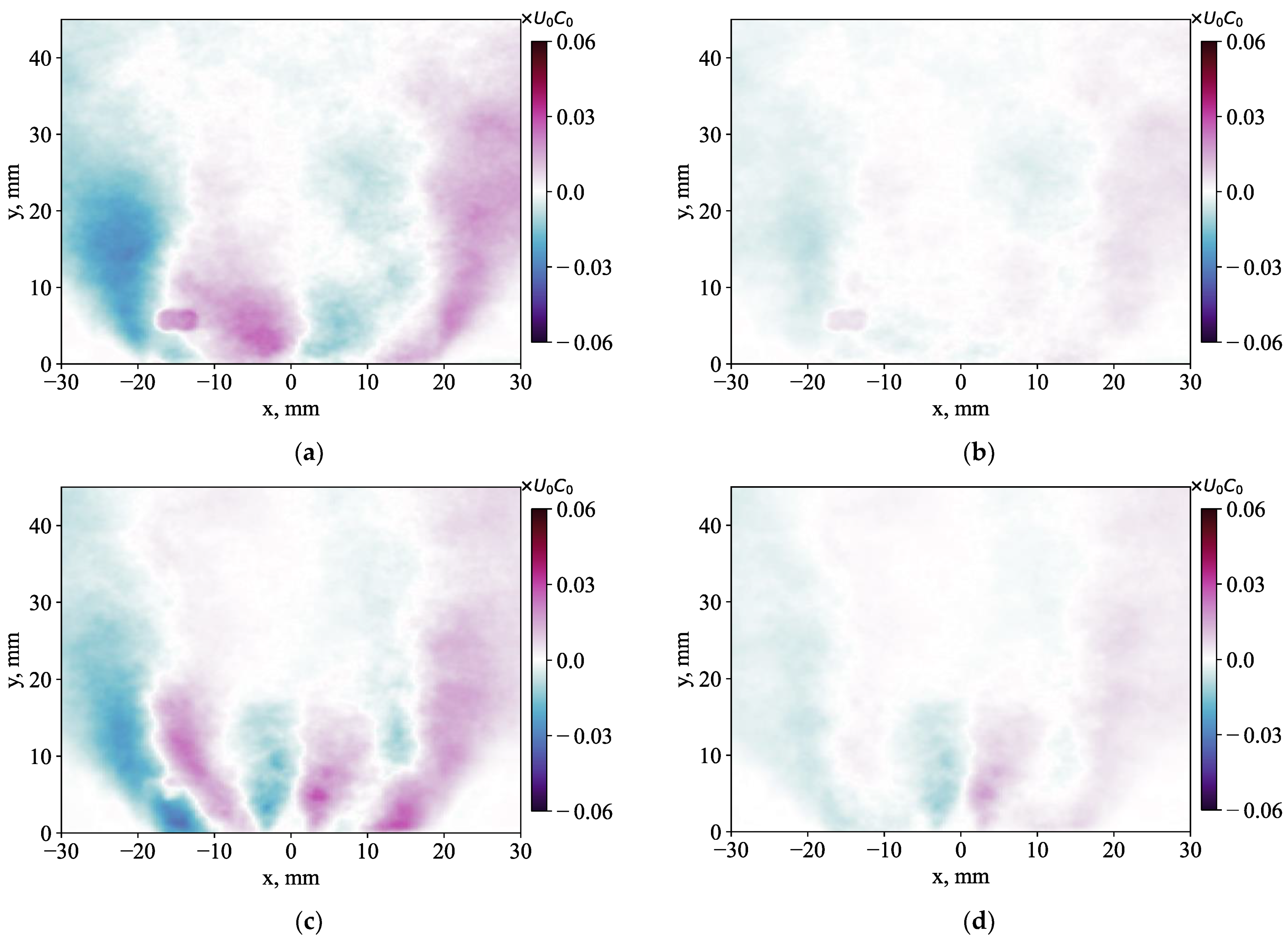
3.3. Coherent Fluctuations
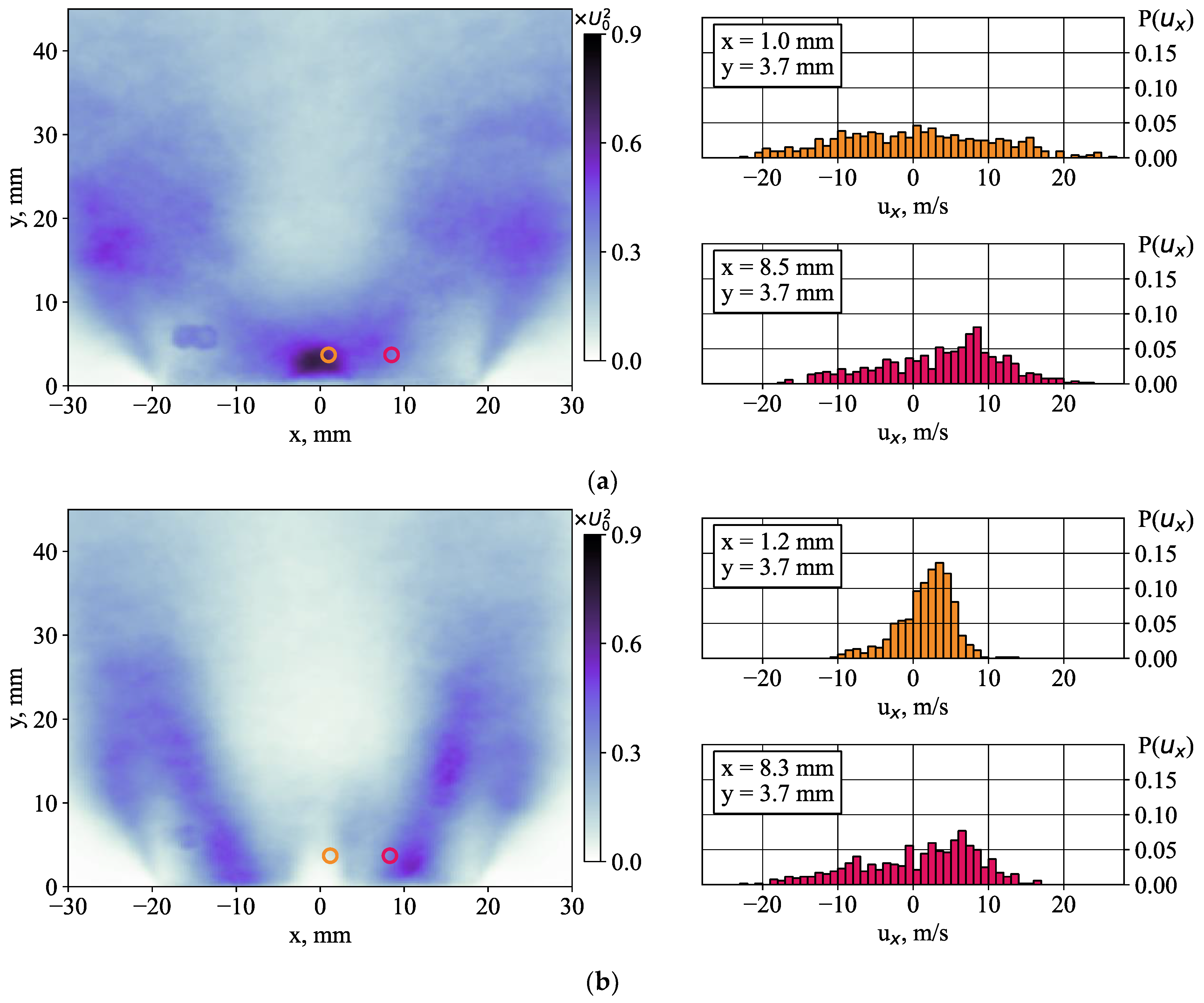
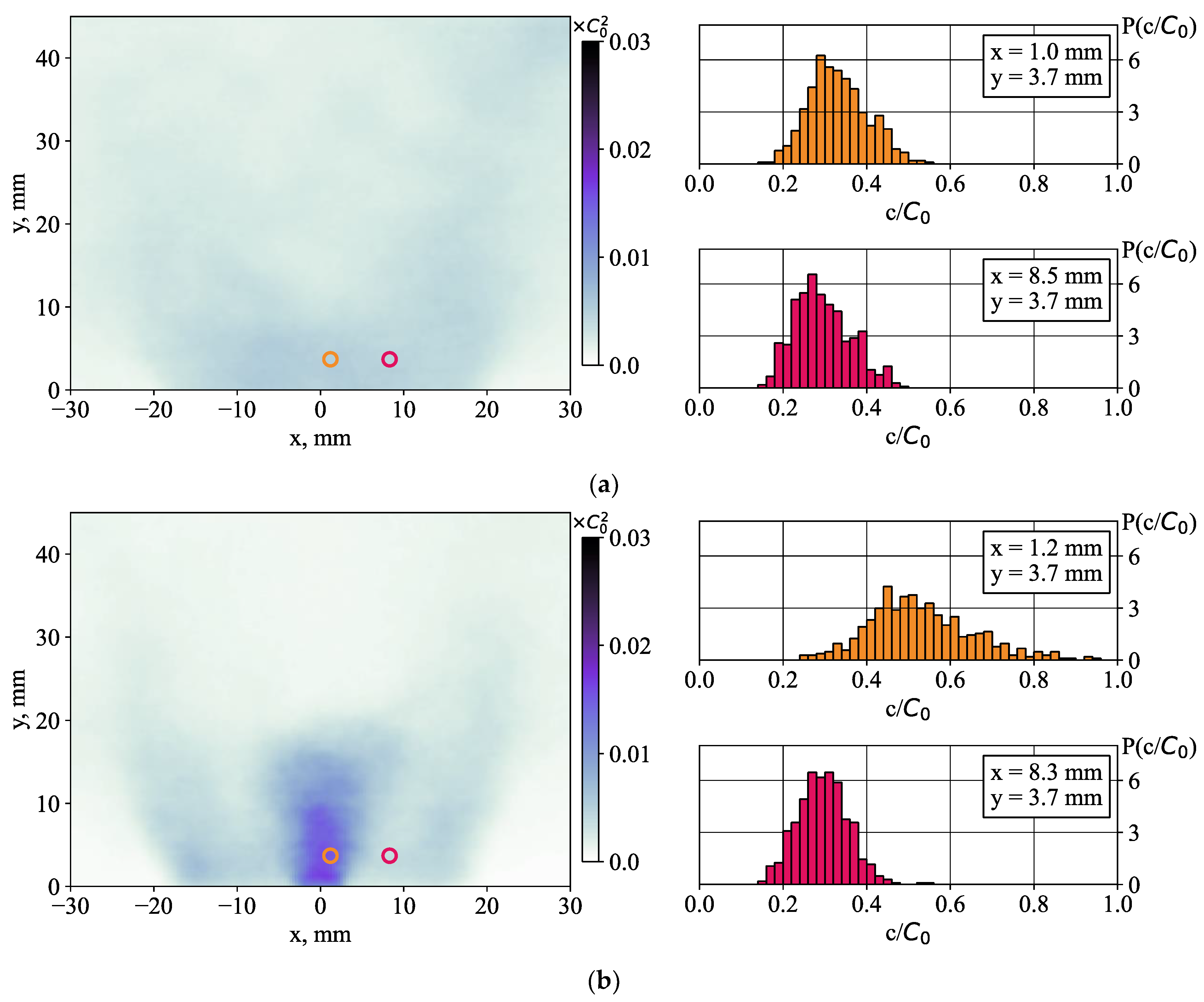
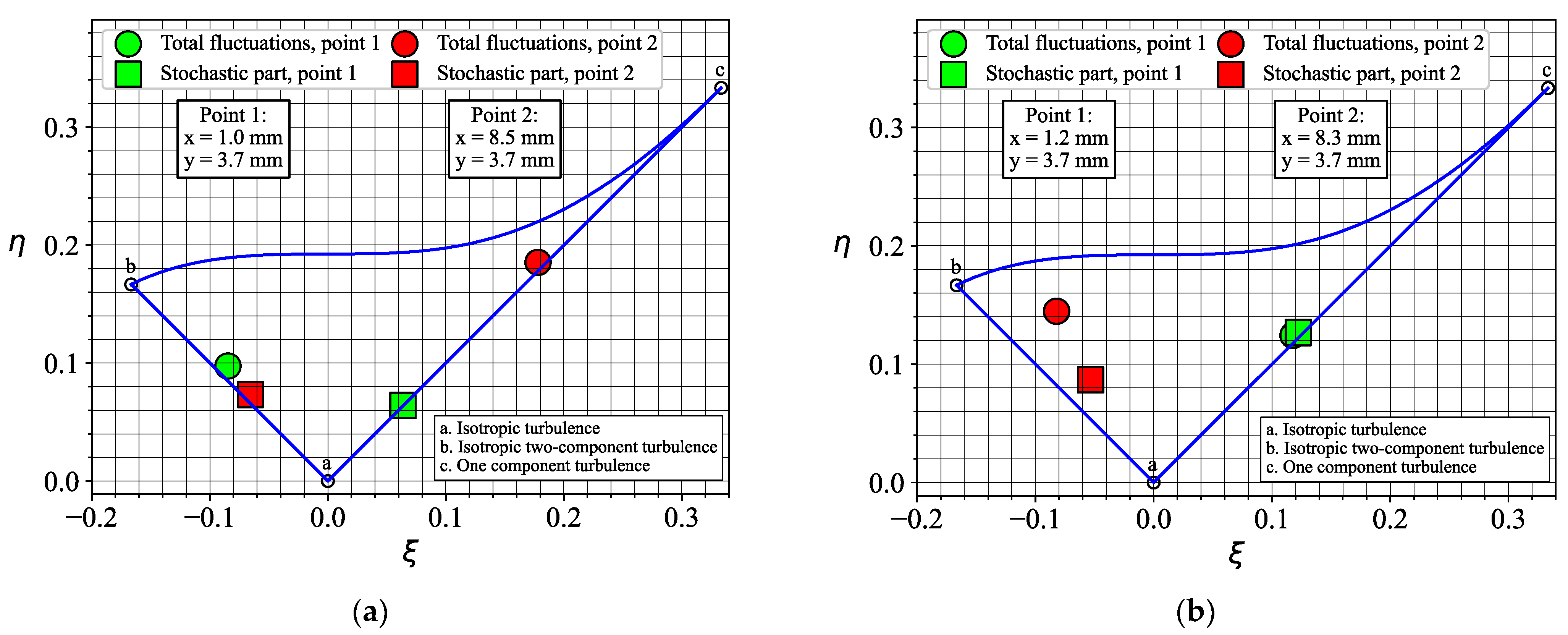
3.4. Stochastic Fluctuations
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Billant, P.; Chomaz, J.M.; Huerre, P. Experimental study of vortex breakdown in swirling jets. J. Fluid Mech. 1998, 376, 183–219. [Google Scholar] [CrossRef]
- Lucca-Negro, O.; O’doherty, T. Vortex breakdown: A review. Prog. Energy Combust. Sci. 2001, 27, 431–481. [Google Scholar] [CrossRef]
- Syred, N. A review of oscillation mechanisms and the role of the precessing vortex core (PVC) in swirl combustion systems. Prog. Energy Combust. Sci. 2006, 32, 93–161. [Google Scholar] [CrossRef]
- Stöhr, M.; Arndt, C.M.; Meier, W. Transient effects of fuel–air mixing in a partially-premixed turbulent swirl flame. Proc. Combust. Inst. 2015, 35, 3327–3335. [Google Scholar] [CrossRef]
- Stöhr, M.; Sadanandan, R.; Meier, W. Experimental study of unsteady flame structures of an oscillating swirl flame in a gas turbine model combustor. Proc. Combust. Inst. 2009, 32, 2925–2932. [Google Scholar] [CrossRef]
- Boxx, I.; Stöhr, M.; Carter, C.; Meier, W. Temporally resolved planar measurements of transient phenomena in a partially pre-mixed swirl flame in a gas turbine model combustor. Combust. Flame 2010, 157, 1510–1525. [Google Scholar] [CrossRef]
- Oberleithner, K.; Paschereit, C.O.; Seele, R.; Wygnanski, I. Formation of turbulent vortex breakdown: Intermittency, criticality, and global instability. AIAA J. 2012, 50, 1437–1452. [Google Scholar] [CrossRef]
- Shtork, S.; Suslov, D.; Skripkin, S.; Litvinov, I.; Gorelikov, E. An Overview of Active Control Techniques for Vortex Rope Mitigation in Hydraulic Turbines. Energies 2023, 16, 5131. [Google Scholar] [CrossRef]
- Midgley, K.; Spencer, A.; McGuirk, J.J. Unsteady Flow Structures in Radial Swirler Fed Fuel Injectors. ASME J. Eng. Gas Turbines Power 2005, 127, 755–764. [Google Scholar] [CrossRef]
- Janus, B.; Dreizler, A.; Janicka, J. Experimental study on stabilization of lifted swirl flames in a model GT combustor. Flow Turbul. Combust. 2005, 75, 293–315. [Google Scholar] [CrossRef]
- Meier, W.; Weigand, P.; Duan, X.R.; Giezendanner-Thoben, R. Detailed characterization of the dynamics of thermoacoustic pulsations in a lean premixed swirl flame. Combust. Flame 2007, 150, 2–26. [Google Scholar] [CrossRef]
- Meier, W.; Duan, X.R.; Weigand, P. Investigation of swirl flames in a gas turbine model combustor II. Turbulence-chemistry interactions. Combust. Flame 2006, 144, 225–236. [Google Scholar] [CrossRef]
- Renaud, A.; Ducruix, S.; Scouflaire, P.; Zimmer, L. Flame shape transition in a swirl stabilised liquid fueled burner. Proc. Combust. Inst. 2015, 35, 3365–3372. [Google Scholar] [CrossRef]
- Li, G.; Gutmark, E.J. Geometry effects on the flow field and spectral characteristics of a triple annular swirler. In Proceedings of the ASME Turbo Expo, Power for Land, See and Air, Atlanta, GA, USA, 16–19 June 2003; 2003. [Google Scholar] [CrossRef]
- Vashahi, F.; Lee, J. Effects of the Interaction Point of Adjacent Swirlers on Swirling Flow Using POD Analysis in a Triple Swirler Configuration. J. Eng. Gas Turbines Power 2019, 141, 0610113. [Google Scholar] [CrossRef]
- Wehr, L.; Meier, W.; Kutne, P.; Hassa, C. Single-pulse 1D laser Raman scattering applied in a gas turbine model combustor at elevated pressure. Proc. Combust. Inst. 2007, 31, 3099–3106. [Google Scholar] [CrossRef]
- Stöhr, M.; Sadanandan, R.; Meier, W. Phase-resolved characterization of vortex–flame interaction in a turbulent swirl flame. Exp. Fluids 2011, 51, 1153–1167. [Google Scholar] [CrossRef]
- Dunn-Rankin, D. Lean Combustion. Technology and Control; Elsevier: Oxford, UK, 2008. [Google Scholar]
- Lieuwen, T.; Torres, H.; Johnson, C.; Zinn, B.T. A mechanism of combustion instability in lean premixed gas turbine combustors. J. Eng. Gas Turbines Power 2001, 123, 182–189. [Google Scholar] [CrossRef]
- Candel, S.; Durox, D.; Schuller, T.; Bourgouin, J.-F.; Moeck, J.P. Dynamics of swirling flames. Ann. Rev. Fluid Mech. 2014, 46, 147–173. [Google Scholar] [CrossRef]
- Lieuwen, T.C. Unsteady Combustor Physics; Cambridge University Press: Cambridge, UK, 2021. [Google Scholar]
- Spencer, A.; McGuirk, J.; Midgley, K. Vortex breakdown in swirling fuel injector flows. J. Eng. Gas Turbines Power 2008, 130, 021503. [Google Scholar] [CrossRef]
- Palkin, E.V.; Hrebtov, M.Y.; Slastnaya, D.A.; Mullyadzhanov, R.I.; Vervisch, L.; Sharaborin, D.K.; Lobasov, A.S.; Dulin, V.M. Influence of a Central Jet on Isothermal and Reacting Swirling Flow in a Model Combustion Chamber. Energies 2022, 15, 1615. [Google Scholar] [CrossRef]
- Sharaborin, D.K.; Savitskii, A.G.; Bakharev, G.Y.; Lobasov, A.S.; Chikishev, L.M.; Dulin, V.M. PIV/PLIF investigation of unsteady turbulent flow and mixing behind a model gas turbine combustor. Exp. Fluids 2021, 62, 96. [Google Scholar] [CrossRef]
- Scarano, F. Iterative image deformation methods in PIV. Meas. Sci. Technol. 2001, 13, R1–R19. [Google Scholar] [CrossRef]
- Soloff, S.M.; Adrian, R.J.; Liu, Z.-C. Distortion compensation for generalized stereoscopic particle image velocimetry. Meas. Sci. Technol. 1997, 8, 1441–1454. [Google Scholar] [CrossRef]
- Lobasov, A.S.; Alekseenko, S.V.; Markovich, D.M.; Dulin, V.M. Mass and momentum transport in the near field of swirling turbulent jets. Effect of swirl rate. Int. J. Heat Fluid Flow 2020, 83, 108539. [Google Scholar] [CrossRef]
- Hussain, A.K.M.F.; Reynolds, W.C. The mechanics of an organized wave in turbulent shear flow. J. Fluid Mech. 1970, 41, 241–258. [Google Scholar] [CrossRef]
- Choi, K.-S.; Lumley, J.L. The return to isotropy of homogeneous turbulence. J. Fluid Mech. 2001, 436, 59–84. [Google Scholar] [CrossRef]






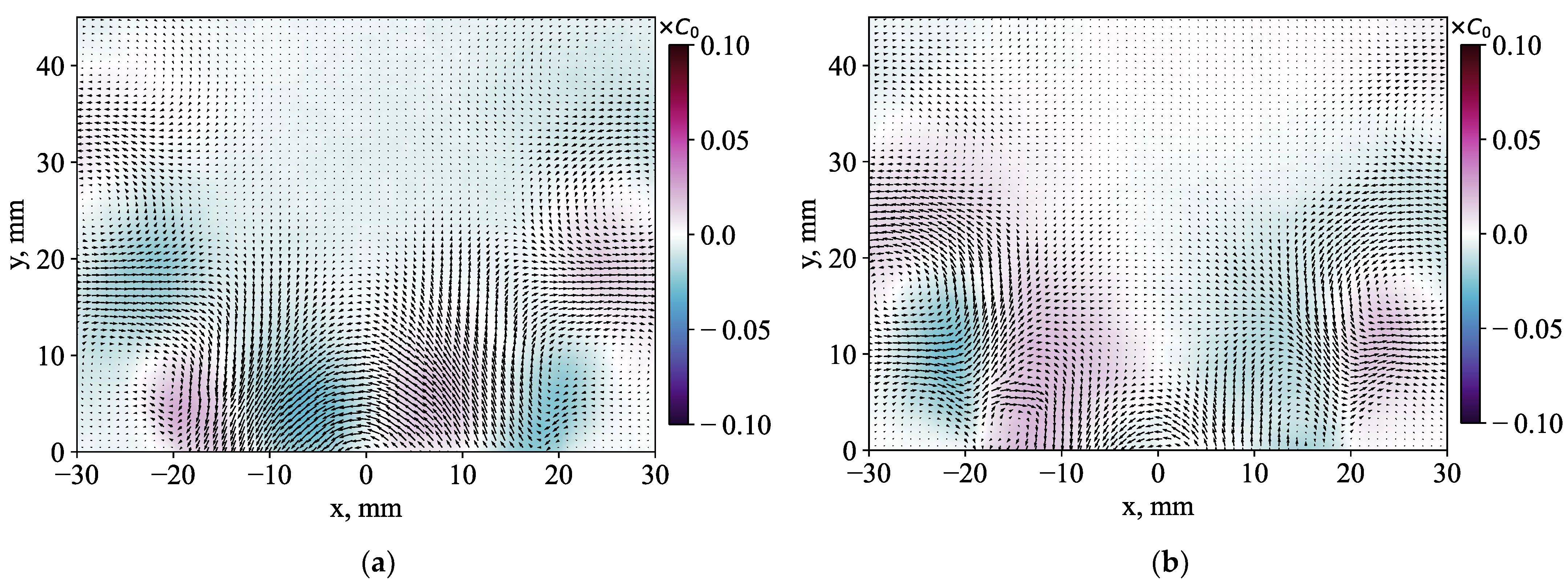
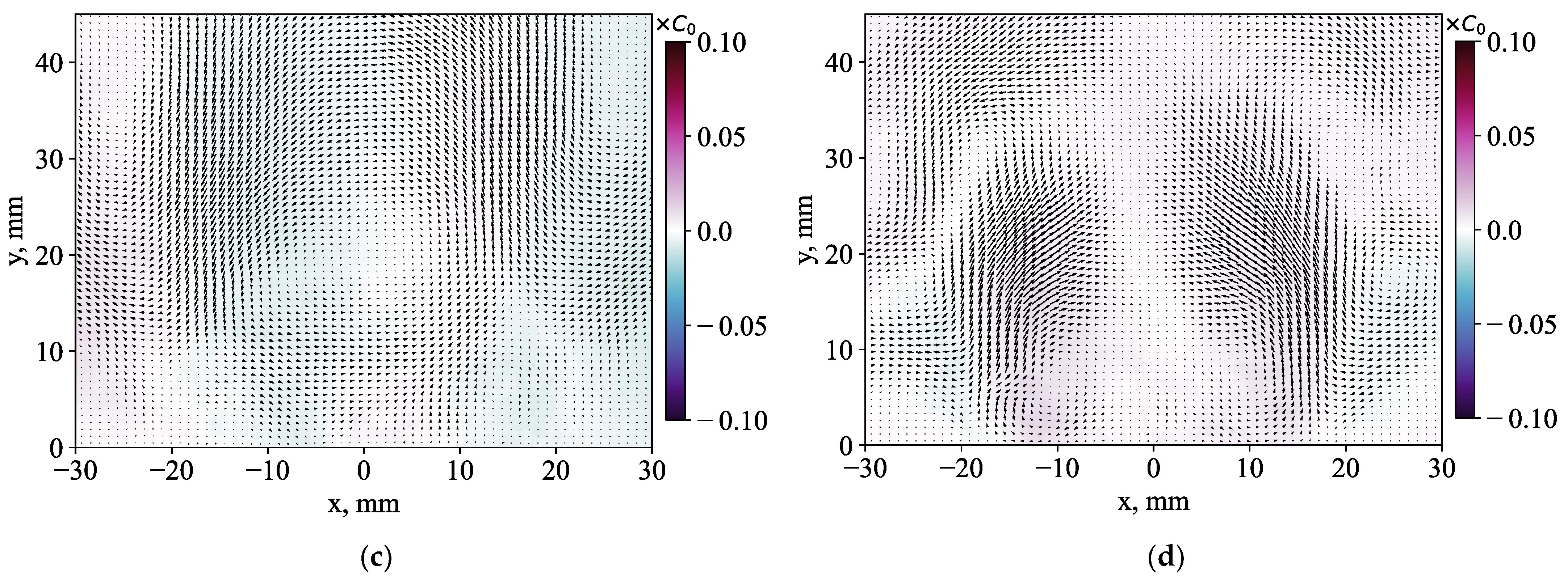
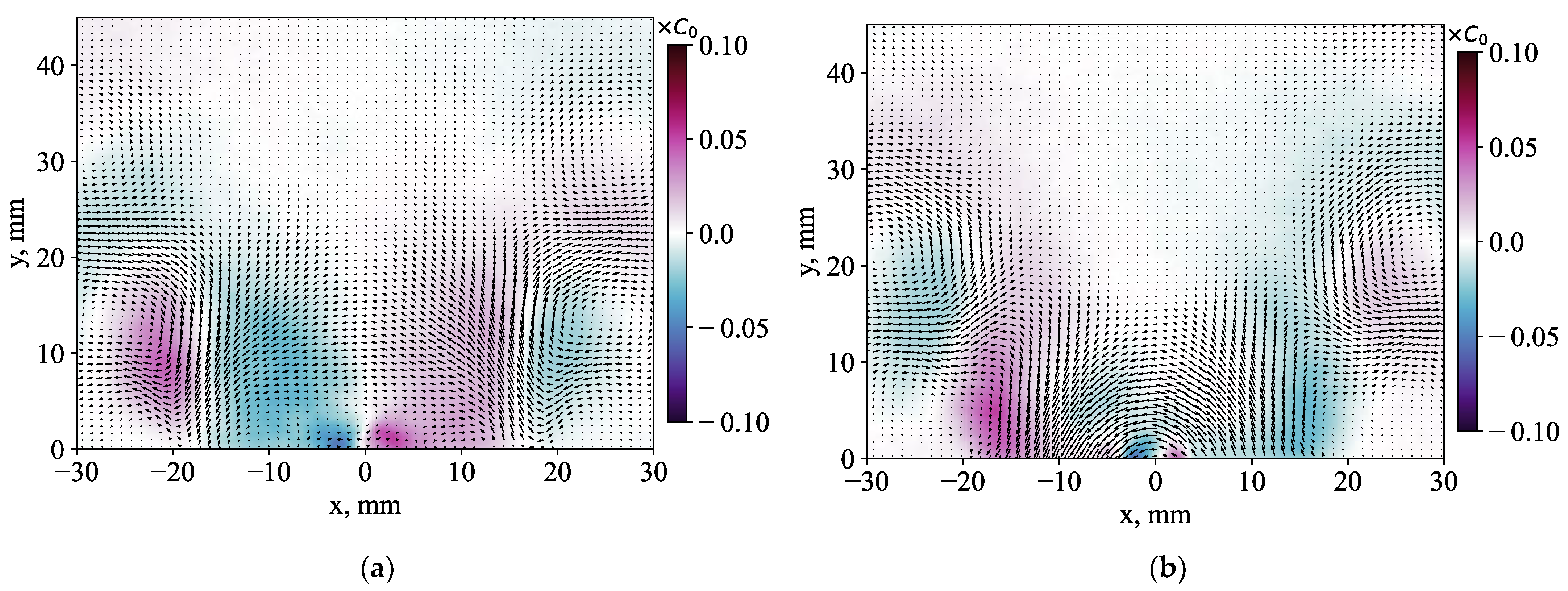

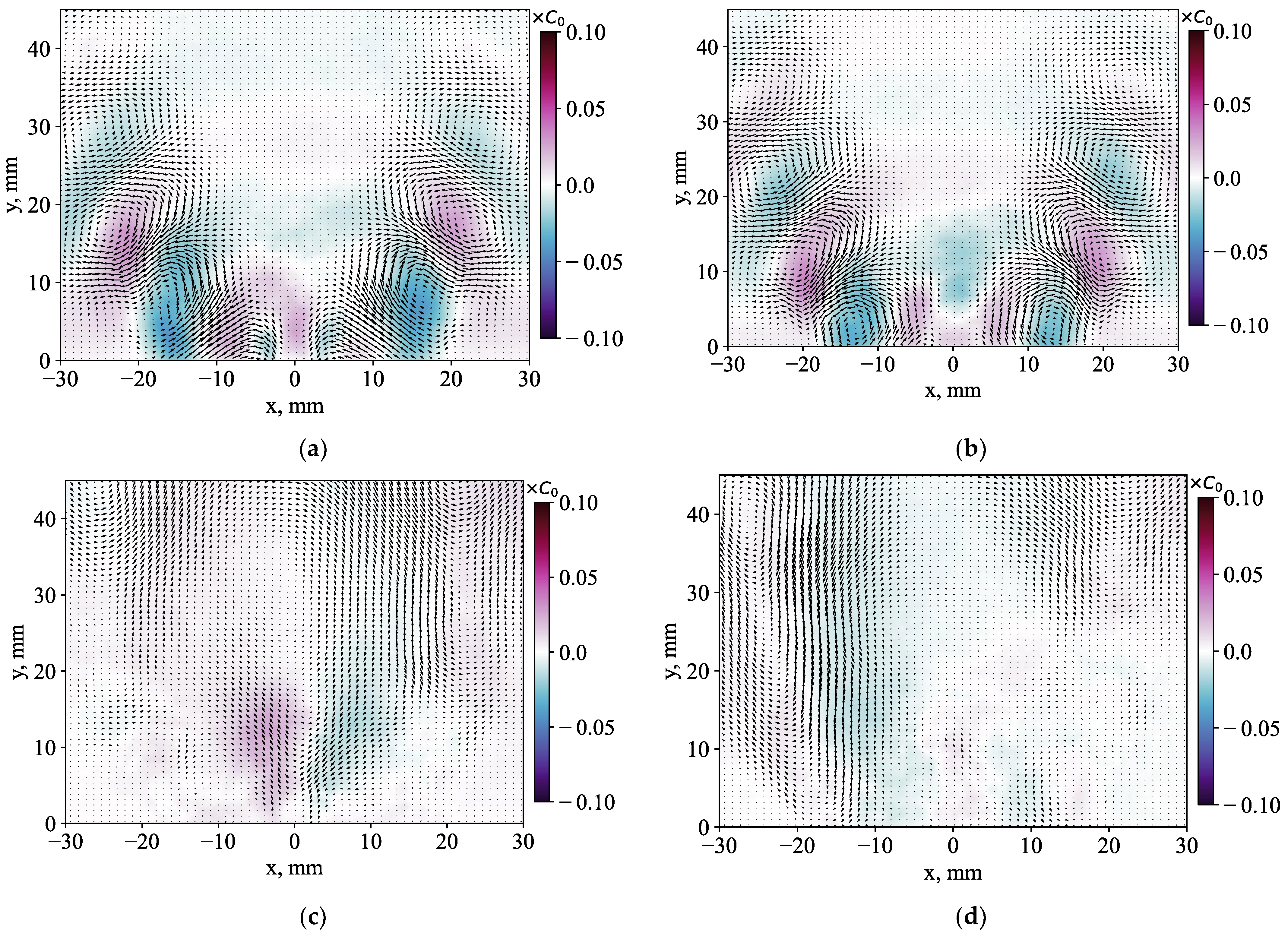

| Flowrate through Swirler (ln/min) | Flowrate between Vanes (ln/min) | Flowrate through Central Jet (ln/min) | Ratio through Central Jet |
|---|---|---|---|
| 799 | 58.3 | 0 | 0% |
| 799 | 52.5 | 5.8 | 10% |
| 799 | 29.15 | 29.15 | 50% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Savitskii, A.; Sharaborin, D.; Chikishev, L.; Dulin, V. Flow Instability Control in a Model Swirl-Stabilized Combustor with Central Jet Injection. Inventions 2023, 8, 148. https://doi.org/10.3390/inventions8060148
Savitskii A, Sharaborin D, Chikishev L, Dulin V. Flow Instability Control in a Model Swirl-Stabilized Combustor with Central Jet Injection. Inventions. 2023; 8(6):148. https://doi.org/10.3390/inventions8060148
Chicago/Turabian StyleSavitskii, Alexey, Dmitriy Sharaborin, Leonid Chikishev, and Vladimir Dulin. 2023. "Flow Instability Control in a Model Swirl-Stabilized Combustor with Central Jet Injection" Inventions 8, no. 6: 148. https://doi.org/10.3390/inventions8060148
APA StyleSavitskii, A., Sharaborin, D., Chikishev, L., & Dulin, V. (2023). Flow Instability Control in a Model Swirl-Stabilized Combustor with Central Jet Injection. Inventions, 8(6), 148. https://doi.org/10.3390/inventions8060148








