Development of the New Analytic Model for Sand Deposition Particles Downstream of a Fence
Abstract
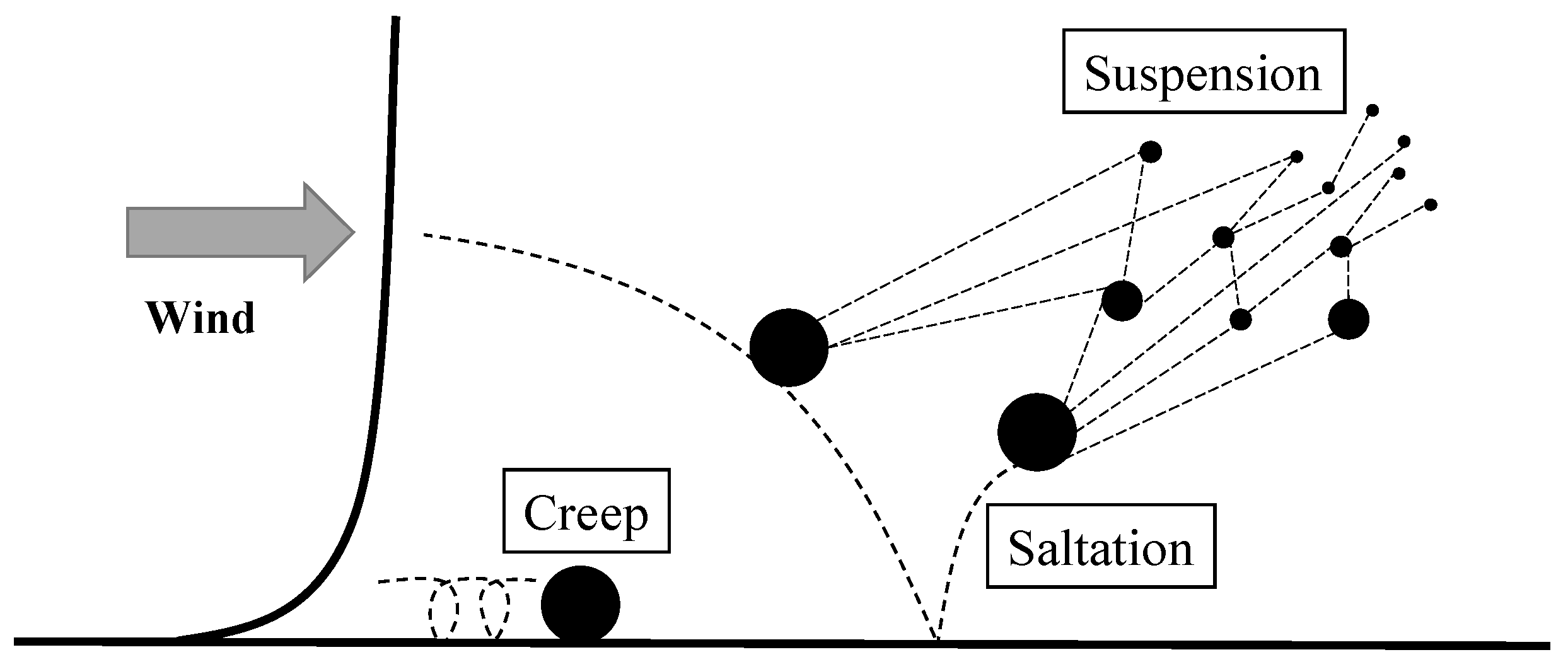
1. Introduction
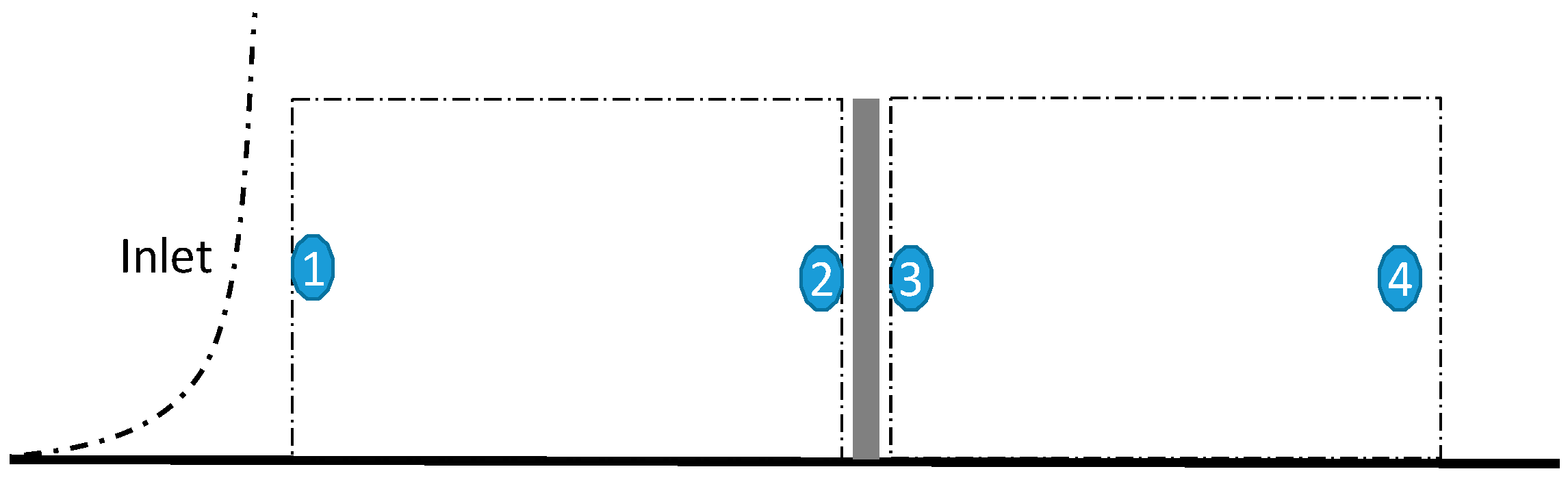
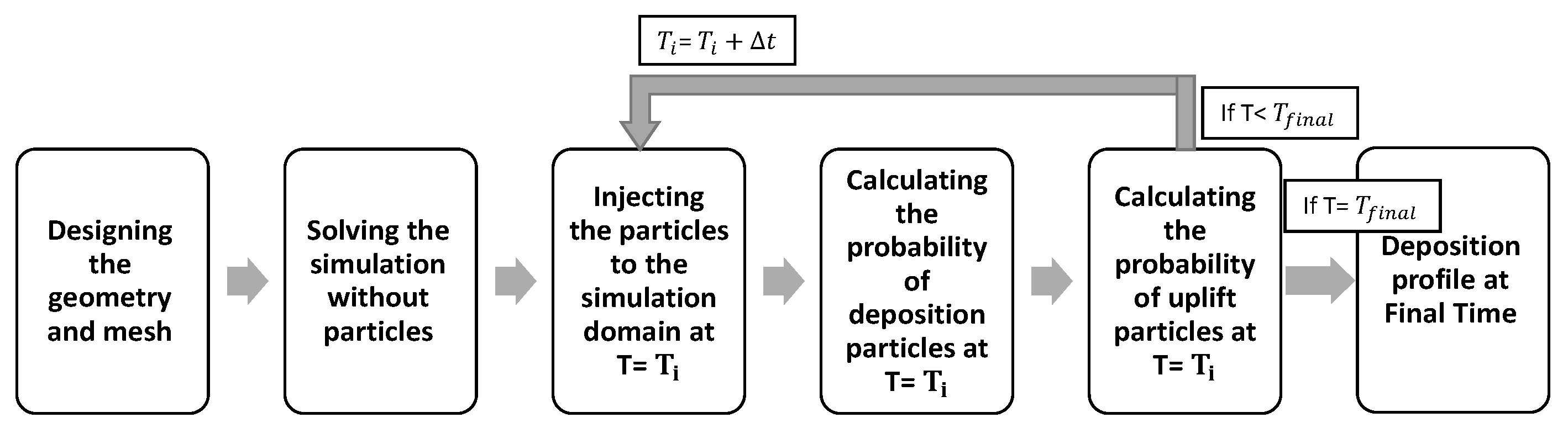
2. Method
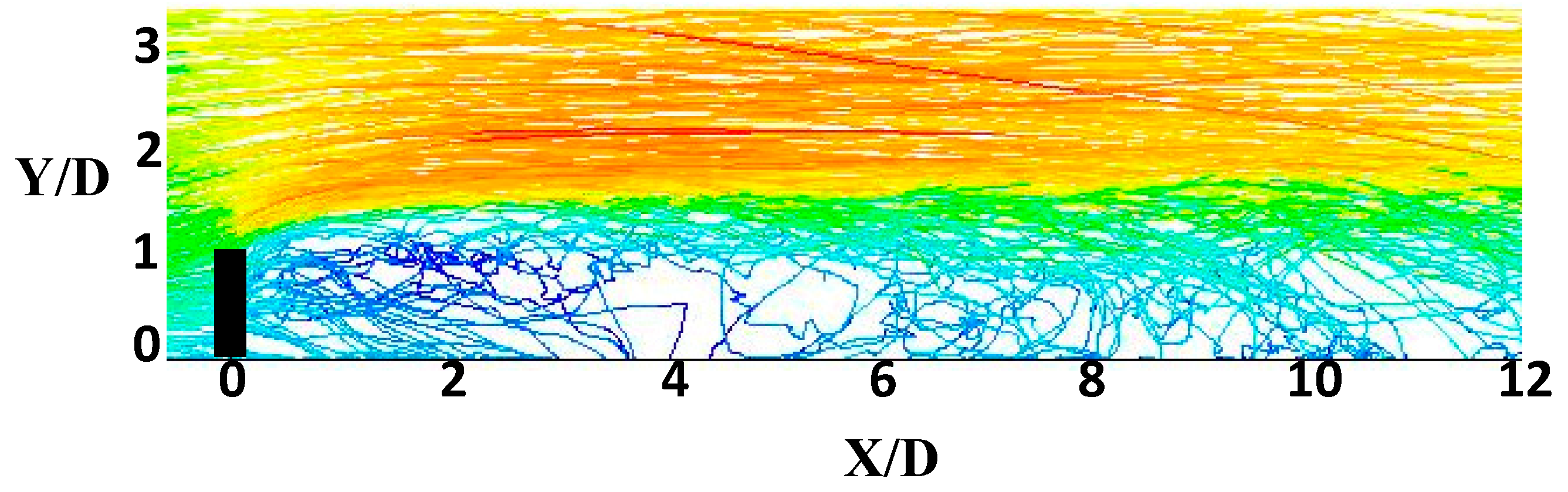
3. Simulation and Discussion
4. Conclusions
Funding
Conflicts of Interest
References
- Shen, Y.; Zhang, C.; Huang, X.; Wang, X.; Cen, S. The effect of wind speed averaging time on sand transport estimates. CATENA 2019, 175, 286–293. [Google Scholar] [CrossRef]
- Van Donk, S.; Wagner, L.E.; Skidmore, E.L.; Tatarko, J. Comparison of the Weibull model with measured wind speed distributions for stochastic wind generation. Trans. ASAE 2005, 48, 503–510. [Google Scholar] [CrossRef]
- Nickling, W.G. The initiation of particle movement by wind. Sedimentology 1988, 35, 499–511. [Google Scholar] [CrossRef]
- Shi, F.; Huang, N. Measurement and simulation of sand saltation movement under fluctuating wind in a natural field environment. Phys. A Stat. Mech. Its Appl. 2012, 391, 474–484. [Google Scholar] [CrossRef]
- Goudie, A.; Watson, A. The shape of desert sand dune grains. J. Arid Environ. 1981, 4, 185–190. [Google Scholar] [CrossRef]
- Kalinske, A.A. Criteria for determining sand-transport by surface-creep and saltation. Eos Trans. Am. Geophys. Union 1942, 23, 639–643. [Google Scholar] [CrossRef]
- Baas, A.C.W. Grains in Motion. In Aeolian Geomorphology; Livingstone, I., Warren, A., Eds.; Wiley-Blackwell: Hoboken, NJ, USA, 2019; pp. 27–60. [Google Scholar]
- Tominaga, Y.; Okaze, T.; Mochida, A. Wind tunnel experiment and CFD analysis of sand erosion/deposition due to wind around an obstacle. J. Wind Eng. Ind. Aerodyn. 2018, 182, 262–271. [Google Scholar] [CrossRef]
- Dupont, S.; Bergametti, G.; Simoëns, S. Modeling aeolian erosion in presence of vegetation. J. Geophys. Res. Earth Surf. 2014, 119, 168–187. [Google Scholar] [CrossRef]
- Wang, N.; Hu, B.-G. Aeolian sand movement and interacting with vegetation: A gpu based simulation and visualization method. In Proceedings of the 2009 Third International Symposium on Plant Growth Modeling, Simulation, Visualization and Applications, Beijing, China, 9–13 November 2009; pp. 401–408. [Google Scholar]
- Saif, A.; Mohamed, A.; Alam Eldein, A. Variable porosity wind fences to control aeolian sand transport. In Proceedings of the Tenth International Congress of Fluid Dynamics, Ain Soukhna Red Sea, Egypt, 16–19 December 2010. [Google Scholar]
- Bitog, J.P.; Lee, I.B.; Shin, M.H.; Hong, S.W.; Hwang, H.S.; Seo, I.H.; Yoo, J.I.; Kwon, K.S.; Kim, Y.H.; Han, J.W. Numerical simulation of an array of fences in Saemangeum reclaimed land. Atmos. Environ. 2009, 43, 4612–4621. [Google Scholar] [CrossRef]
- Xie, L.; Dong, Z.; Zheng, X. Experimental analysis of sand particles’ lift-off and incident velocities in wind-blown sand flux. Acta Mech. Sin. 2006, 21, 564–573. [Google Scholar] [CrossRef]
- Liu, B.; Qu, J.; Zhang, W.; Tan, L.; Gao, Y. Numerical evaluation of the scale problem on the wind flow of a windbreak. Sci. Rep. 2014, 4, 6619. [Google Scholar] [CrossRef] [PubMed]
- Lima, I.A.; Araújo, A.D.; Parteli, E.J.R.; Andrade, J.S.; Herrmann, H.J. Optimal array of sand fences. Sci. Rep. 2017, 7, 45148. [Google Scholar] [CrossRef] [PubMed]
- Lavasani, A.M.; Razia, P.; Mehdipourb, R. Numerical Solution of Fence Performance for Reduction of Sand Deposition on Railway Tracks. Int. J. Eng.-Trans. A Basics 2016, 29, 1014–1021. [Google Scholar]
- Li, B.; Sherman, D.J. Aerodynamics and morphodynamics of sand fences: A review. Aeolian Res. 2015, 17, 33–48. [Google Scholar] [CrossRef]
- Hotta, S.; Kraus, N.C.; Horikawa, K. Function of sand fences in controlling wind-blown sand. In Coastal Sediments; ASCE: Reston, VA, USA, 1987; pp. 772–787. [Google Scholar]
- Jensen, M.J.P. Shelter Effect, Investigations into the Aerodynamics of Shelter and its Effects on Climate and Crops; Danish Techn.: Krbenhavn, Denmark, 1954. [Google Scholar]
- Bruno, L.; Horvat, M.; Raffaele, L. Windblown sand along railway infrastructures: A review of challenges and mitigation measures. J. Wind Eng. Ind. Aerodyn. 2018, 177, 340–365. [Google Scholar] [CrossRef]
- Dong, Z.; Luo, W.; Qian, G.; Wang, H. A wind tunnel simulation of the mean velocity fields behind upright porous fences. Agric. For. Meteorol. 2007, 146, 82–93. [Google Scholar] [CrossRef]
- Nägeli, W. Weitere Untersuchungen über die Windverhältnisse im Bereich von Windschutzstreifen. Mitteilungen für die Schweizerische Anstalt für das Forstliche Versuchswesen 1946, 24, 660–737. [Google Scholar]
- Phillips, C.J.; Willetts, B.B. Predicting sand deposition at porous fences. J. Waterway Port Coast. Ocean Div. 1979, 105, 15–31. [Google Scholar]
- Chamorro, L.P.; A Arndt, R.E. Non-uniform velocity distribution effect on the Betz-Joukowsky limit. Wind Energy 2013, 16, 279–282. [Google Scholar] [CrossRef]
- Jensen, N.O. A Note on Wind Generator Interaction; Risø National Laboratory: Roskilde, Denmark, 1983; ISBN 87-550-0971-9. [Google Scholar]
- Darcy, H.P.G. Les Fontaines Publiques de la Ville de Dijon. Exposition et Application des Principes à Suivre et des Formules à Employer Dans les Questions de Distribution D’eau, Etc.; V. Dalamont: Paris, France, 1856. [Google Scholar]
- Idelchik, I.E. Handbook of Hydraulic Resistance; Hemisphere Publishing Corp.: Washington, DC, USA, 1986; 662p. [Google Scholar]
- Rosin, P. Laws governing the fineness of powdered coal. J. Inst. Fuel 1933, 7, 29–36. [Google Scholar]
- Mehdipour, R.; Baniamerian, Z. A new approach in reducing sand deposition on railway tracks to improve transportation. Aeolian Res. 2019, 41, 100537. [Google Scholar] [CrossRef]
- Fluent 6.2 User’s Guide; Fluent Inc.: Lebanon, IN, USA, 2006.
- Tabler, R.D. Geometry and Density of Drifts Formed by Snow Fences. J. Glaciol. 1980, 26, 405–419. [Google Scholar] [CrossRef][Green Version]
- Iversen, J.D. Comparison of wind-tunnel model and full-scale snow fence drifts. J. Wind Eng. Ind. Aerodyn. 1981, 8, 231–249. [Google Scholar] [CrossRef]

© 2020 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Razi, P. Development of the New Analytic Model for Sand Deposition Particles Downstream of a Fence. Inventions 2020, 5, 4. https://doi.org/10.3390/inventions5010004
Razi P. Development of the New Analytic Model for Sand Deposition Particles Downstream of a Fence. Inventions. 2020; 5(1):4. https://doi.org/10.3390/inventions5010004
Chicago/Turabian StyleRazi, Peyman. 2020. "Development of the New Analytic Model for Sand Deposition Particles Downstream of a Fence" Inventions 5, no. 1: 4. https://doi.org/10.3390/inventions5010004
APA StyleRazi, P. (2020). Development of the New Analytic Model for Sand Deposition Particles Downstream of a Fence. Inventions, 5(1), 4. https://doi.org/10.3390/inventions5010004




