Microwave Photon Emission in Superconducting Circuits
Abstract
1. Introduction
2. Devices and Experimental Setup
2.1. SQUID
2.2. JPA
2.3. JTWPA
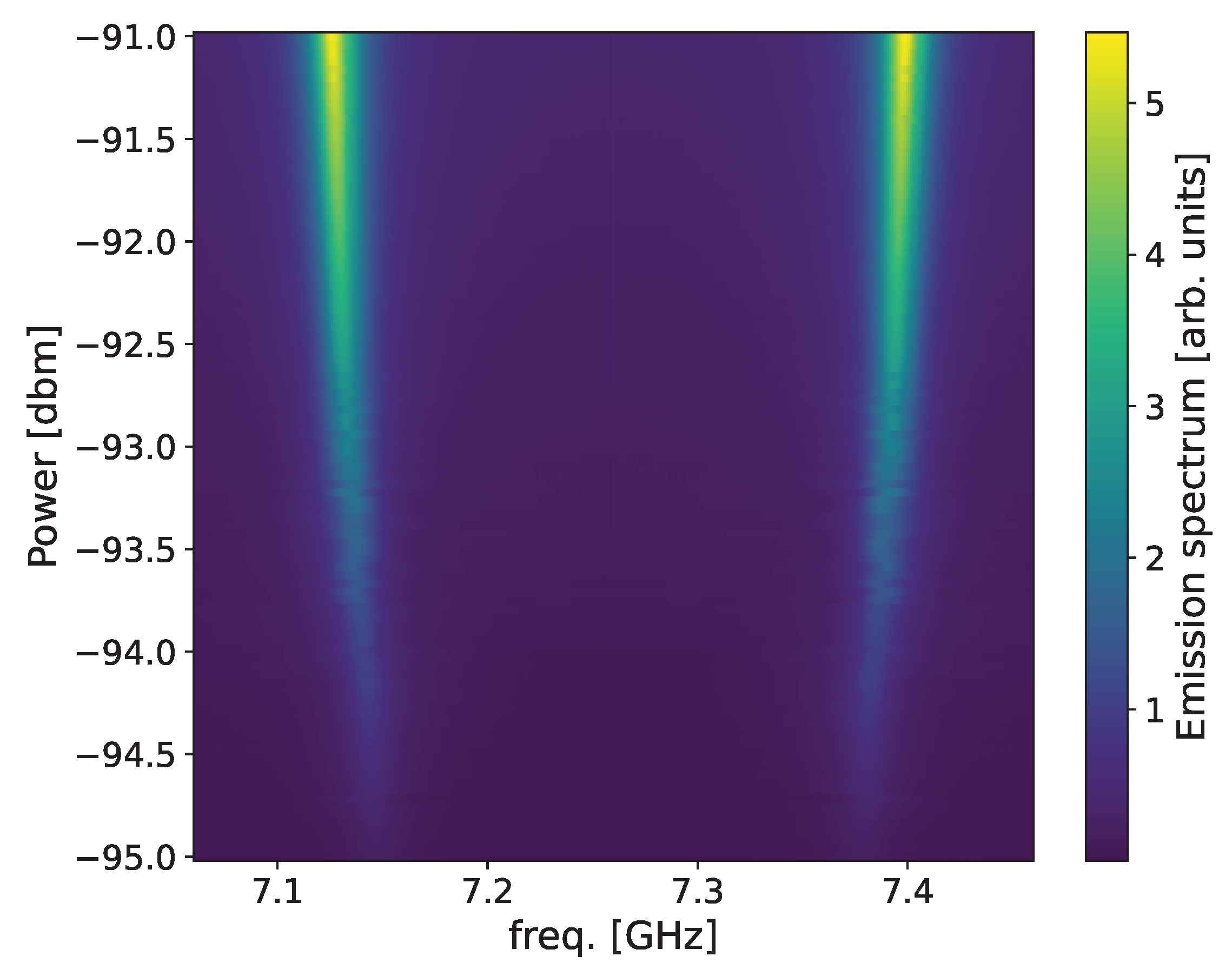
3. Results and Discussion
3.1. SQUID
3.2. JPA
3.3. JTWPA
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A. Rf Components Details
| Instrument | Model | Bandwidth |
|---|---|---|
| VNA | Agilent E5071C | 300 kHz–20 GHz |
| Signal generator (S1) | Rohde&Schwarz SMA100B | 8 kHz–20 GHz |
| Signal generator (S2) | Rohde&Schwarz SGS100A | 1 MHz–12.75 GHz |
| Spectrum Analyzer | Signal Hound SM200B | 100 kHz–20 GHz |
| FET +30 dB | Narda Miteq LNA-30-08001200-09-10P | 8 GHz–12 GHz |
| FET +35 dB | Narda Miteq LNA-30-04001200-15-10P | 4 GHz–12 GHz |
| HEMT +30 dB | Low Noise Factory LNF-LNC6_20C s/n 1403Z | 6 GHz–20 GHz |
| HEMT +36 dB | Low Noise Factory LNF-LNC4_16B s/n 2157Z | 4 GHz–16 GHz |
| Attenuators (A1) | Radiall R413806000/R411810121/R411820121 | DC–18 GHz |
| Attenuators (A2) | Narda Miteq 4768-6/4768-10/4768-20 | DC–40 GHz |
| Directional Coupler | Mini-Circuits ZUDC10-02183-S+ | 2 GHz–18 GHz |
| Circulators (C1) | Low Noise Factory LNF-CIC4_12A | 4 GHz–12 GHz |
| Double circulator (C2) | Quinstar Technology QCY-G0801202 | 8 GHz–12 GHz |
| Bias Tee (BT) | Marki Microwave BT-0018 | 40 kHz–18 GHz |
| Switch | Radiall R577443005 | DC–18 GHz |
| Splitter | Mini-Circuits ZX10-2-183-S+ | 1.5 GHz–18 GHz |
Appendix B. Derivation of SQUID Resonances with Direct Pump Drive
References
- Josephson, B.D. Possible new effects in superconductive tunnelling. Phys. Lett. 1962, 1, 251–253. [Google Scholar] [CrossRef]
- Josephson, B.D. Supercurrents through barriers. Adv. Phys. 1965, 14, 419–451. [Google Scholar] [CrossRef]
- Rettaroli, A.; Alesini, D.; Babusci, D.; Barone, C.; Buonomo, B.; Beretta, M.M.; Castellano, G.; Chiarello, F.; Di Gioacchino, D.; Felici, G.; et al. Josephson Junctions as Single Microwave Photon Counters: Simulation and Characterization. Instruments 2021, 5, 25. [Google Scholar] [CrossRef]
- D’Elia, A.; Rettaroli, A.; Tocci, S.; Babusci, D.; Barone, C.; Beretta, M.; Buonomo, B.; Chiarello, F.; Chikhi, N.; Di Gioacchino, D.; et al. Stepping closer to pulsed single microwave photon detectors for axions search. IEEE Trans. Appl. Supercond. 2022, 33, 1–9. [Google Scholar] [CrossRef]
- Navarathna, R.; Le, D.T.; Hamann, A.R.; Nguyen, H.D.; Stace, T.M.; Fedorov, A. Passive superconducting circulator on a chip. Phys. Rev. Lett. 2023, 130, 037001. [Google Scholar] [CrossRef]
- Xu, M.; Cheng, R.; Wu, Y.; Liu, G.; Tang, H.X. Magnetic field-resilient quantum-limited parametric amplifier. PRX Quantum 2023, 4, 010322. [Google Scholar] [CrossRef]
- Peugeot, A.; Ménard, G.; Dambach, S.; Westig, M.; Kubala, B.; Mukharsky, Y.; Altimiras, C.; Joyez, P.; Vion, D.; Roche, P.; et al. Generating two continuous entangled microwave beams using a dc-biased Josephson junction. Phys. Rev. X 2021, 11, 031008. [Google Scholar] [CrossRef]
- Esposito, M.; Ranadive, A.; Planat, L.; Leger, S.; Fraudet, D.; Jouanny, V.; Buisson, O.; Guichard, W.; Naud, C.; Aumentado, J.; et al. Observation of Two-Mode Squeezing in a Traveling Wave Parametric Amplifier. Phys. Rev. Lett. 2022, 128, 153603. [Google Scholar] [CrossRef]
- Luo, Y.H.; Chen, M.C.; Erhard, M.; Zhong, H.S.; Wu, D.; Tang, H.Y.; Zhao, Q.; Wang, X.L.; Fujii, K.; Li, L.; et al. Quantum teleportation of physical qubits into logical code spaces. Proc. Natl. Acad. Sci. USA 2021, 118, e2026250118. [Google Scholar] [CrossRef]
- Brehm, J.D.; Gebauer, R.; Stehli, A.; Poddubny, A.N.; Sander, O.; Rotzinger, H.; Ustinov, A.V. Slowing down light in a qubit metamaterial. Appl. Phys. Lett. 2022, 121, 204001. [Google Scholar] [CrossRef]
- Aumentado, J. Superconducting Parametric Amplifiers: The State of the Art in Josephson Parametric Amplifiers. IEEE Microw. Mag. 2020, 21, 45–59. [Google Scholar] [CrossRef]
- Clarke, J. SQUIDs. Sci. Am. 1994, 271, 46–53. [Google Scholar] [CrossRef]
- Krantz, P.; Bengtsson, A.; Simoen, M.; Gustavsson, S.; Shumeiko, V.; Oliver, W.D.; Wilson, C.M.; Delsing, P.; Bylander, J. Single-shot read-out of a superconducting qubit using a Josephson parametric oscillator. Nat. Commun. 2016, 7, 11417. [Google Scholar] [CrossRef] [PubMed]
- Shankar, S.; Hao, Z.; Hatefipour, M.; Strickland, W.; Shaw, T.; Shabani, J. Josephson parametric amplifiers for rapid, high-fidelity measurement of solid-state qubits. In Proceedings of the 2023 Device Research Conference (DRC), Santa Barbara, CA, USA, 25–28 June 2023; pp. 1–2. [Google Scholar] [CrossRef]
- Blais, A.; Huang, R.S.; Wallraff, A.; Girvin, S.M.; Schoelkopf, R.J. Cavity quantum electrodynamics for superconducting electrical circuits: An architecture for quantum computation. Phys. Rev. A 2004, 69, 062320. [Google Scholar] [CrossRef]
- Roy, A.; Devoret, M. Quantum-limited parametric amplification with Josephson circuits in the regime of pump depletion. Phys. Rev. B 2018, 98, 045405. [Google Scholar] [CrossRef]
- Du, N.; Force, N.; Khatiwada, R.; Lentz, E.; Ottens, R.; Rosenberg, L.J.; Rybka, G.; Carosi, G.; Woollett, N.; Bowring, D.; et al. Search for Invisible Axion Dark Matter with the Axion Dark Matter Experiment. Phys. Rev. Lett. 2018, 120, 151301. [Google Scholar] [CrossRef] [PubMed]
- Backes, K.M.; Palken, D.A.; Al Kenany, S.; Brubaker, B.M.; Cahn, S.B.; Droster, A.; Hilton, G.C.; Ghosh, S.; Jackson, H.; Lamoreaux, S.K.; et al. A quantum enhanced search for dark matter axions. Nature 2021, 590, 238. [Google Scholar] [CrossRef]
- Alesini, D.; Braggio, C.; Carugno, G.; Crescini, N.; D’Agostino, D.; Di Gioacchino, D.; Di Vora, R.; Falferi, P.; Gambardella, U.; Gatti, C.; et al. Search for invisible axion dark matter of mass ma = 43 μeV with the QUAX–aγ experiment. Phys. Rev. D 2021, 103, 102004. [Google Scholar] [CrossRef]
- Mück, M.; McDermott, R. Radio-frequency amplifiers based on dc SQUIDs. Supercond. Sci. Technol. 2010, 23, 093001. [Google Scholar] [CrossRef]
- Johansson, J.R.; Johansson, G.; Wilson, C.M.; Nori, F. Dynamical Casimir effect in superconducting microwave circuits. Phys. Rev. A 2010, 82, 052509. [Google Scholar] [CrossRef]
- Wilson, C.M.; Johansson, G.; Pourkabirian, A.; Simoen, M.; Johansson, J.R.; Duty, T.; Nori, F.; Delsing, P. Observation of the dynamical Casimir effect in a superconducting circuit. Nature 2011, 479, 376–379. [Google Scholar] [CrossRef] [PubMed]
- Lähteenmäki, P.; Paraoanu, G.S.; Hassel, J.; Hakonen, P.J. Dynamical Casimir effect in a Josephson metamaterial. Proc. Natl. Acad. Sci. USA 2013, 110, 4234–4238. [Google Scholar] [CrossRef]
- Suri, B.; Keane, Z.; Ruskov, R.; Bishop, L.S.; Tahan, C.; Novikov, S.; Robinson, J.; Wellstood, F.; Palmer, B. Observation of Autler–Townes effect in a dispersively dressed Jaynes–Cummings system. New J. Phys. 2013, 15, 125007. [Google Scholar] [CrossRef][Green Version]
- Li, J.; Paraoanu, G.; Cicak, K.; Altomare, F.; Park, J.I.; Simmonds, R.W.; Sillanpää, M.A.; Hakonen, P.J. Decoherence, Autler-Townes effect, and dark states in two-tone driving of a three-level superconducting system. Phys. Rev. B 2011, 84, 104527. [Google Scholar] [CrossRef]
- Baur, M.; Filipp, S.; Bianchetti, R.; Fink, J.; Göppl, M.; Steffen, L.; Leek, P.J.; Blais, A.; Wallraff, A. Measurement of Autler-Townes and Mollow transitions in a strongly driven superconducting qubit. Phys. Rev. Lett. 2009, 102, 243602. [Google Scholar] [CrossRef] [PubMed]
- Sillanpää, M.A.; Li, J.; Cicak, K.; Altomare, F.; Park, J.I.; Simmonds, R.W.; Paraoanu, G.S.; Hakonen, P.J. Autler-Townes effect in a superconducting three-level system. Phys. Rev. Lett. 2009, 103, 193601. [Google Scholar] [CrossRef] [PubMed]
- Braggio, C.; Cappelli, G.; Carugno, G.; Crescini, N.; Di Vora, R.; Esposito, M.; Ortolan, A.; Planat, L.; Ranadive, A.; Roch, N.; et al. A haloscope amplification chain based on a traveling wave parametric amplifier. Rev. Sci. Instrum. 2022, 93, 094701. [Google Scholar] [CrossRef]
- Rettaroli, A.; Barone, C.; Borghesi, M.; Capelli, S.; Carapella, G.; Caricato, A.; Carusotto, I.; Cian, A.; Di Gioacchino, D.; Enrico, E.; et al. Ultra low noise readout with traveling wave parametric amplifiers: The DARTWARS project. Nucl. Instrum. Methods Phys. Res. Sect. A 2023, 1046, 167679. [Google Scholar] [CrossRef]
- Chiarello, F.; Alesini, D.; Babusci, D.; Barone, C.; Beretta, M.M.; Buonomo, B.; D’Elia, A.; Gioacchino, D.D.; Felici, G.; Filatrella, G.; et al. Investigation of Resonant Activation in a Josephson Junction for Axion Search With Microwave Single Photon Detection. IEEE Trans. Appl. Supercond. 2022, 32, 1–5. [Google Scholar] [CrossRef]
- Orlando, T.P.; Mooij, J.E.; Tian, L.; van der Wal, C.H.; Levitov, L.S.; Lloyd, S.; Mazo, J.J. Superconducting persistent-current qubit. Phys. Rev. B 1999, 60, 15398–15413. [Google Scholar] [CrossRef]
- Yamamoto, T.; Inomata, K.; Watanabe, M.; Matsuba, K.; Miyazaki, T.; Oliver, W.D.; Nakamura, Y.; Tsai, J.S. Flux-driven Josephson parametric amplifier. Appl. Phys. Lett. 2008, 93, 042510. [Google Scholar] [CrossRef]
- Dolan, G.J. Offset masks for lift-off photoprocessing. Appl. Phys. Lett. 1977, 31, 337–339. [Google Scholar] [CrossRef]
- Greco, A.; Fasolo, L.; Meda, A.; Callegaro, L.; Enrico, E. Quantum model for rf-SQUID-based metamaterials enabling three-wave mixing and four-wave mixing traveling-wave parametric amplification. Phys. Rev. B 2021, 104, 184517. [Google Scholar] [CrossRef]
- Fasolo, L.; Barone, C.; Borghesi, M.; Carapella, G.; Caricato, A.P.; Carusotto, I.; Chung, W.; Cian, A.; Gioacchino, D.D.; Enrico, E.; et al. Bimodal Approach for Noise Figures of Merit Evaluation in Quantum-Limited Josephson Traveling Wave Parametric Amplifiers. IEEE Trans. Appl. Supercond. 2022, 32, 1–6. [Google Scholar] [CrossRef]
- Livreri, P.; Enrico, E.; Fasolo, L.; Greco, A.; Rettaroli, A.; Vitali, D.; Farina, A.; Marchetti, C.F.; Giacomin, A.S.D. Microwave Quantum Radar using a Josephson Traveling Wave Parametric Amplifier. In Proceedings of the 2022 IEEE Radar Conference (RadarConf22), New York, NY, USA, 21–25 March 2022; pp. 1–5. [Google Scholar] [CrossRef]








Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
D′Elia, A.; Rettaroli, A.; Chiarello, F.; Di Gioacchino, D.; Enrico, E.; Fasolo, L.; Ligi, C.; Maccarrone, G.; Mantegazzini, F.; Margesin, B.; et al. Microwave Photon Emission in Superconducting Circuits. Instruments 2023, 7, 36. https://doi.org/10.3390/instruments7040036
D′Elia A, Rettaroli A, Chiarello F, Di Gioacchino D, Enrico E, Fasolo L, Ligi C, Maccarrone G, Mantegazzini F, Margesin B, et al. Microwave Photon Emission in Superconducting Circuits. Instruments. 2023; 7(4):36. https://doi.org/10.3390/instruments7040036
Chicago/Turabian StyleD′Elia, Alessandro, Alessio Rettaroli, Fabio Chiarello, Daniele Di Gioacchino, Emanuele Enrico, Luca Fasolo, Carlo Ligi, Giovanni Maccarrone, Federica Mantegazzini, Benno Margesin, and et al. 2023. "Microwave Photon Emission in Superconducting Circuits" Instruments 7, no. 4: 36. https://doi.org/10.3390/instruments7040036
APA StyleD′Elia, A., Rettaroli, A., Chiarello, F., Di Gioacchino, D., Enrico, E., Fasolo, L., Ligi, C., Maccarrone, G., Mantegazzini, F., Margesin, B., Mattioli, F., Tocci, S., Vinante, A., & Gatti, C. (2023). Microwave Photon Emission in Superconducting Circuits. Instruments, 7(4), 36. https://doi.org/10.3390/instruments7040036









