First Demonstration of a Pixelated Charge Readout for Single-Phase Liquid Argon Time Projection Chambers
Abstract
1. Introduction
2. Experimental Set-Up
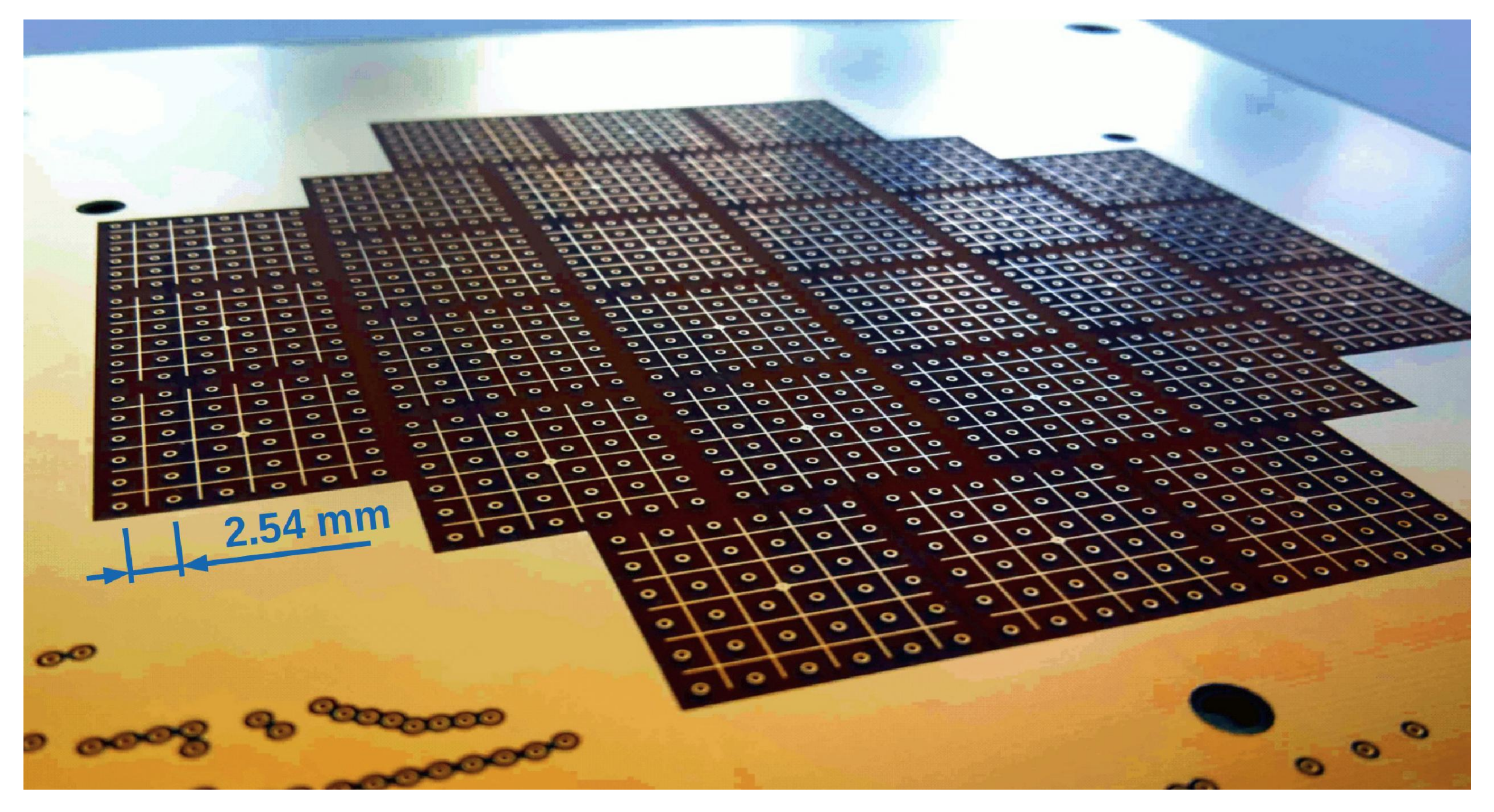
2.1. Pixel PCB Design
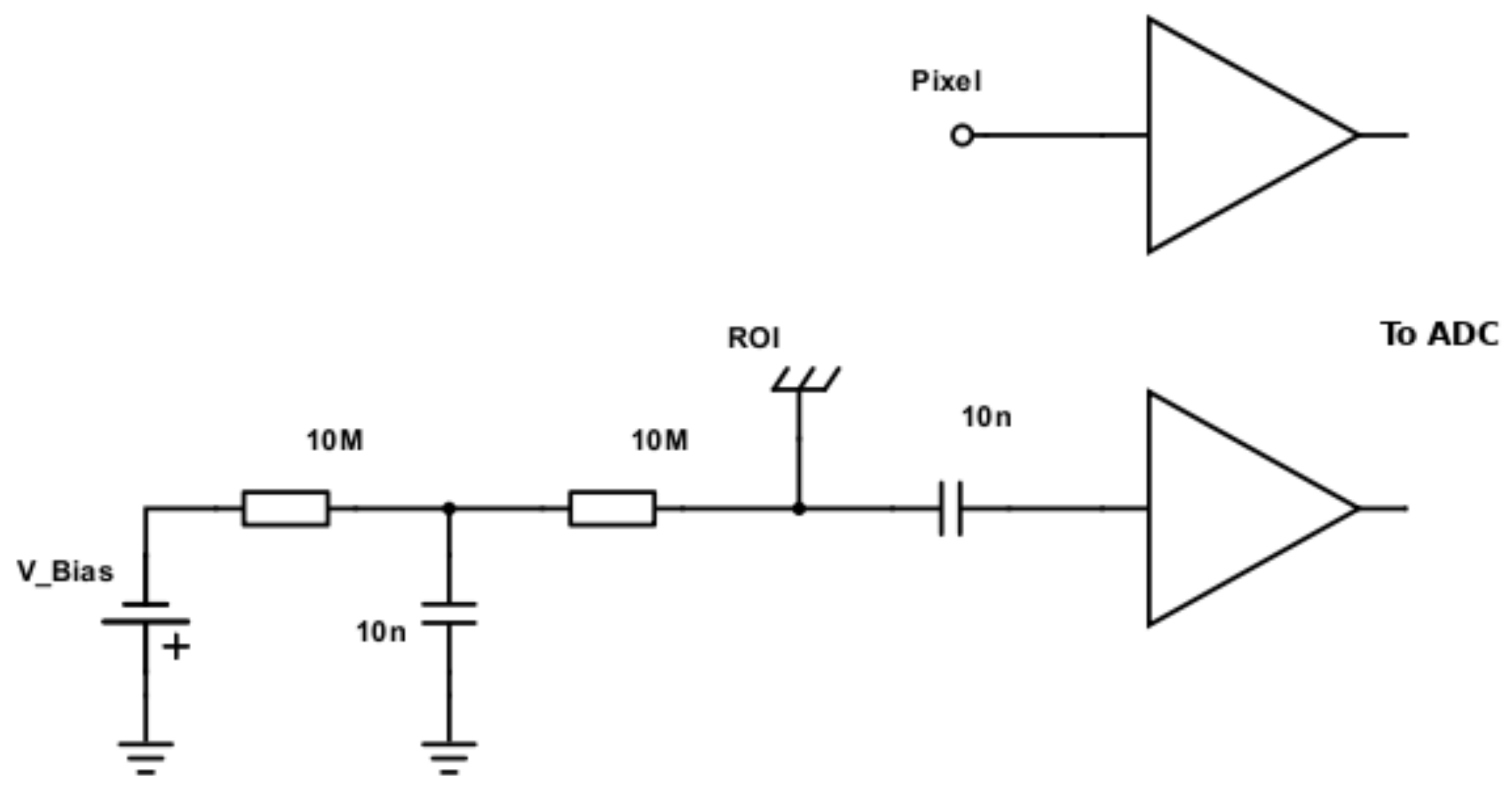
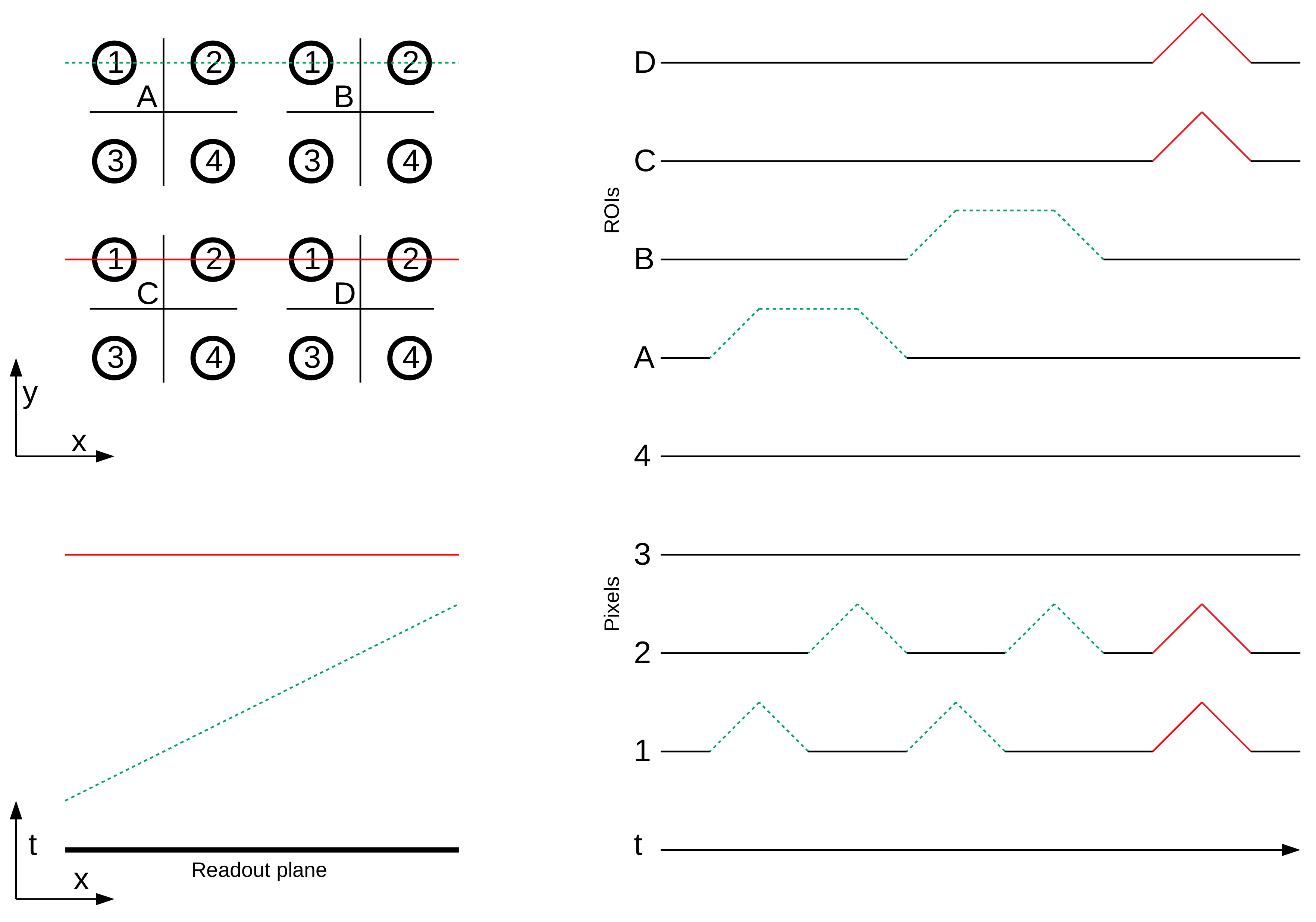
2.2. Readout Scheme
2.3. Pixel Demonstration TPC
2.4. Infrastructure
3. Data Analysis and Reconstruction
3.1. Signal-To-Noise Ratio
3.2. 3D Track Reconstruction
- Noise filtering
- Pulse finding
- 3D hit finding
- Ambiguity rejection
- Track fitting
4. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Nygren, D.R. The Time Projection Chamber: A New 4 pi Detector for Charged Particles. eConf 1974, C740805, 58. [Google Scholar]
- Willis, W.; Radeka, V. Liquid-argon ionization chambers as total-absorption detectors. Nucl. Instrum. Methods 1974, 120, 221–236. [Google Scholar] [CrossRef]
- Rubbia, C. The Liquid Argon Time Projection Chamber: A New Concept for Neutrino Detectors; Technical Report CERN-EP-INT-77-08; CERN: Geneva, Swizerland, 1977. [Google Scholar]
- Rubbia, C.; Antonello, M.; Aprili, P.; Baibussinov, B.; Ceolin, M.B.; Barze, L.; Benetti, P.; Calligarich, E.; Canci, N.; Carbonara, F.; et al. Underground operation of the ICARUS T600 LAr-TPC: First results. J. Instrum. 2011, 6, P07011. [Google Scholar] [CrossRef]
- Anderson, C.; Antonello, M.; Baller, B.; Bolton, T.; Bromberg, C.; Cavanna, F.; Church, E.; Edmunds, D.; Ereditato, A.; Farooq, S.; et al. The ArgoNeuT Detector in the NuMI Low-Energy beam line at Fermilab. JINST 2012, 7, P10019. [Google Scholar] [CrossRef]
- Acciarri, R.; Adams, C.; An, R.; Aparicio, A.; Aponte, S.; Asaadi, J.; Auger, M.; Ayoub, N.; Bagby, L.; Baller, B.; et al. Design and Construction of the MicroBooNE Detector. JINST 2017, 12, P02017. [Google Scholar] [CrossRef]
- Joshi, J.; Qian, X. Signal Processing in the MicroBooNE LArTPC. arXiv 2015, arXiv:1511.00317. [Google Scholar]
- Rossi, B.; Badhress, I.; Ereditato, A.; Haug, S.; Hänni, R.; Hess, M.; Janoŝ, S.; Juget, F.; Kreslo, I.; Lehmann, S.; et al. A prototype liquid Argon Time Projection Chamber for the study of UV laser multi-photonic ionization. J. Instrum. 2009, 4, P07011. [Google Scholar] [CrossRef][Green Version]
- Adamson, P.; Anderson, K.; Andrews, M.; Andrews, R.; Anghel, I.; Augustine, D.; Aurisano, A.; Avvakumov, S.; Ayres, D.S.; Baller, B.; et al. The NuMI Neutrino Beam. Nucl. Instrum. Meth. 2016, A806, 279–306. [Google Scholar] [CrossRef]
- Strait, J.; McCluskey, E.; Lundin, T.; Willhite, J.; Hamernik, T.; Papadimitriou, V.; Marchionni, A.; Kim, M.J.; Nessi, M.; Montanari, D.; et al. Long-Baseline Neutrino Facility (LBNF) and Deep Underground Neutrino Experiment (DUNE) Conceptual Design Report Volume 3: Long-Baseline Neutrino Facility for DUNE. arXiv 2016, arXiv:1601.05823. [Google Scholar]
- Auger, M.; Blatter, A.; Ereditato, A.; Göldi, D.; Janos, S.; Kreslo, I.; Lüthi, M.; Von Rohr, C.R.; Strauss, T.; Weber, M.S. On the Electric Breakdown in Liquid Argon at Centimeter Scale. JINST 2016, 11, P03017. [Google Scholar] [CrossRef][Green Version]
- Auger, M.; Ereditato, A.; Göldi, D.; Janos, S.; Kreslo, I.; Lüthi, M.; von Rohr, C.R.; Strauss, T.; Tolba, T.; Weber, M.S. A method to suppress dielectric breakdowns in liquid argon ionization detectors for cathode to ground distances of several millimeters. JINST 2014, 9, P07023. [Google Scholar] [CrossRef]
- Kubo, H.; Miuchi, K.; Nagayoshi, T.; Ochi, A.; Orito, R.; Takada, A.; Tanimori, T.; Ueno, M. Development of a time projection chamber with micro pixel electrodes. Nucl. Instrum. Meth. 2003, A513, 94–98. [Google Scholar] [CrossRef]
- Acciarri, R.; Acero, M.A.; Adamowski, M.; Adams, C.; Adamson, P.; Adhikari, S.; Ahmad, Z.; Albright, C.H.; Alion, T.; Amador, E.; et al. Long-Baseline Neutrino Facility (LBNF) and Deep Underground Neutrino Experiment (DUNE): Conceptual Design Report, Volume 4 The DUNE Detectors at LBNF. arXiv 2016, arXiv:1601.02984. [Google Scholar]
- De Geronimo, G.; D’Andragora, A.; Li, S.; Nambiar, N.; Rescia, S.; Vernon, E.; Chen, H.; Lanni, F.; Makowiecki, D.; Radeka, V.; et al. Front-End ASIC for a Liquid Argon TPC. IEEE Trans. Nucl. Sci. 2011, 58, 1376–1385. [Google Scholar] [CrossRef]
- Krieger, A.; Dwyer, D.; Garcia-Sciveres, M.; Gnani, D.; Grace, C. A micropower readout ASIC for pixelated liquid Ar TPCs. In Proceedings of the Topical Workshop on Electronics for Particle Physics, Santa Cruz, CA, USA, 11–15 September 2017. [Google Scholar]
- Acciarri, R.; Acero, M.A.; Adamowski, M.; Adams, C.; Adamson, P.; Adhikari, S.; Ahmad, Z.; Albright, C.H.; Alion, T.; Amador, E.; et al. Long-Baseline Neutrino Facility (LBNF) and Deep Underground Neutrino Experiment (DUNE) Conceptual Design Report Volume 1: The LBNF and DUNE Projects. arXiv 2016, arXiv:1601.05471. [Google Scholar]
- Acciarri, R.; Acero, M.A.; Adamowski, M.; Adams, C.; Adamson, P.; Adhikari, S.; Ahmad, Z.; Albright, C.H.; Alion, T.; Amador, E. Long-Baseline Neutrino Facility (LBNF) and Deep Underground Neutrino Experiment (DUNE) Conceptual Design Report Volume 2: The Physics Program for DUNE at LBNF. arXiv 2016, arXiv:1512.06148. [Google Scholar]
- Asaadi, J.; Auger, M.; Ereditato, A.; Goeldi, D.; Hänni, R.; Kose, U.; Kreslo, I.; Lorca, D.; Luethi, M.; von Rohr, C.R.; et al. A pixelated charge readout for Liquid Argon Time Projection Chambers. J. Instrum. 2018, 13, C02008. [Google Scholar] [CrossRef]
- Sarpeshkar, R.; Delbruck, T.; Mead, C. White noise in MOS transistors and resistors. IEEE Circuits Devices Mag. 1993, 9, 23–29. [Google Scholar] [CrossRef]
- Ereditato, A.; Göldi, D.; Janos, S.; Kreslo, I.; Luethi, M.; von Rohr, C.R.; Schenk, M.; Strauss, T.; Weber, M.S.; Zeller, M. Performance of cryogenic charge readout electronics with the ARGONTUBE LAr TPC. JINST 2014, 9, P11022. [Google Scholar] [CrossRef][Green Version]
- Cavanna, F.; Kordosky, M.; Raaf, J.; Rebel, B. LArIAT: Liquid Argon In A Testbeam. arXiv 2014, arXiv:1406.5560. [Google Scholar]
- Auger, M. New Micromegas Based Readout Techniques for Imaging in Time Projection Chambers. Ph.D. Thesis, University of Bern, Bern, Switzerland, 2012. [Google Scholar]
- Moss, Z.; Moon, J.; Bugel, L.; Conrad, J.M.; Sachdev, K.; Toups, M.; Wongjirad, T. A Factor of Four Increase in Attenuation Length of Dipped Lightguides for Liquid Argon TPCs Through Improved Coating. arXiv 2016, arXiv:1604.03103. [Google Scholar]
- Auger, M.; Ereditato, A.; Goeldi, D.; Kreslo, I.; Lorca, D.; Luethi, M.; Von Rohr, C.R.; Sinclair, J.; Weber, M.S. Multi-channel front-end board for SiPM readout. JINST 2016, 11, P10005. [Google Scholar] [CrossRef][Green Version]
- Auger, M.; Del Tutto, M.; Ereditato, A.; Fleming, B.T.; Goeldi, D.; Gramellini, E.; Guenette, R.; Ketchum, W.; Kreslo, I.; Laube, A.; et al. A Novel Cosmic Ray Tagger System for Liquid Argon TPC Neutrino Detectors. Instruments 2017, 1, 2. [Google Scholar] [CrossRef]
- Badhrees, I.; Ereditato, A.; Kreslo, I.; Messina, M.; Moser, U.; Rossi, B.; Weber, M.S.; Zeller, M.; Altucci, C.; Amoruso, S.; et al. Measurement of the two-photon absorption cross-section of liquid argon with a time projection chamber. New J. Phys. 2010, 12, 113024. [Google Scholar] [CrossRef]
- Blatter, A.; Ereditato, A.; Hsu, C.C.; Janos, S.; Kreslo, I.; Luethi, M.; von Rohr, C.R.; Schenk, M.; Strauss, T.; Weber, M.S.; et al. Experimental study of electric breakdowns in liquid argon at centimeter scale. JINST 2014, 9, P04006. [Google Scholar] [CrossRef]
- Patrignani, C.; Agashe, K.; Aielli, G.; Amsler, C.; Antonelli, M.; Asner, D.M.; Baer, H.; Banerjee, S.; Barnett, R.M.; Basaglia, T.; et al. Review of Particle Physics. Chin. Phys. C 2016, 40, 100001. [Google Scholar]
- Aprile, E.; Bolotnikov, A.E.; Bolozdynya, A.L.; Doke, T. Noble Gas Detectors; Wiley: Hoboken, NJ, USA, 2006. [Google Scholar]
- Amoruso, S.; Antonello, M.; Aprili, P.; Arneodo, F.; Badertscher, A.; Baiboussinov, B.; Ceolin, M.B.; Battistoni, G.; Bekman, B.; Benetti, P.; et al. Study of electron recombination in liquid argon with the ICARUS TPC. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2004, 523, 275–286. [Google Scholar] [CrossRef]
- Acciarri, R.; Adams, C.; Asaadi, J.; Baller, B.; Bolton, T.; Bromberg, C.; Cavanna, F.; Church, E.; Edmunds, D.; Ereditato, A. A study of electron recombination using highly ionizing particles in the ArgoNeuT Liquid Argon TPC. J. Instrum. 2013, 8, P08005. [Google Scholar] [CrossRef]
- Zeller, M.; Ereditato, A.; Haug, S.; Hsu, C.C.; Janos, S.; Kreslo, I.; Messina, M.; von Rohr, C.R.; Rossi, B.; Strauss, T.; et al. First measurements with ARGONTUBE, a 5m long drift Liquid Argon TPC. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2013, 718, 454–458. [Google Scholar] [CrossRef]
- Gushchin, E.M.; Kruglov, A.A.; Obodovskii, I.M. Electron dynamics in condensed argon and xenon. J. Exp. Theor. Phys. 1982, 55, 650. [Google Scholar]
- Chepel, V.; Araújo, H. Liquid noble gas detectors for low energy particle physics. J. Instrum. 2013, 8, R04001. [Google Scholar] [CrossRef]
- Jolliffe, I. Principal Component Analysis; Springer Series in Statistics; Springer: Berlin, Germany, 2002. [Google Scholar]
- Höppner, C.; Neubert, S.; Ketzer, B.; Paul, S. A novel generic framework for track fitting in complex detector systems. Nucl. Instrum. Methods Phys. Res. Sect. A Accel. Spectrometers Detect. Assoc. Equip. 2010, 620, 518–525. [Google Scholar] [CrossRef]
- Rauch, J.; Schlüter, T. GENFIT—A Generic Track-Fitting Toolkit. J. Phys. Conf. Ser. 2015, 608, 012042. [Google Scholar] [CrossRef]
- Bilka, T.; Braun, N.; Hauth, T.; Kuhr, T.; Lavezzi, L.; Metzner, F.; Paul, S.; Prencipe, E.; Prim, M.; Rauch, J.; et al. Implementation of GENFIT2 as an experiment independent track-fitting framework. arXiv 2019, arXiv:1902.04405. [Google Scholar]



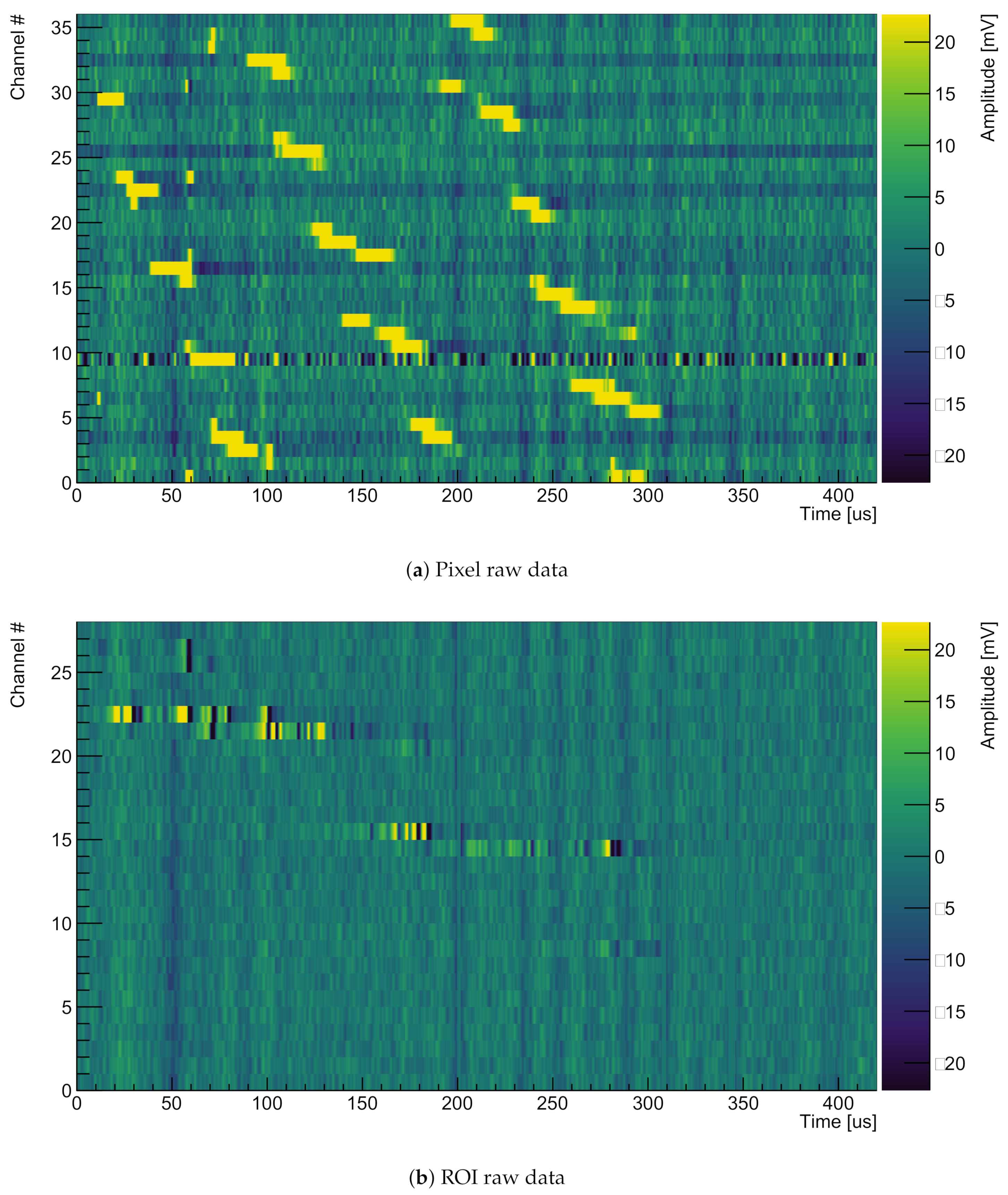



| Channel | MIP at | SNR |
|---|---|---|
| Pixel | Readout plane | 14 |
| Pixel | Cathode | 5.5 |
| ROI | Readout plane | 16 |
| ROI | Cathode | 6.1 |
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Asaadi, J.; Auger, M.; Ereditato, A.; Goeldi, D.; Kose, U.; Kreslo, I.; Lorca, D.; Luethi, M.; Rudolf Von Rohr, C.B.U.; Sinclair, J.; et al. First Demonstration of a Pixelated Charge Readout for Single-Phase Liquid Argon Time Projection Chambers. Instruments 2020, 4, 9. https://doi.org/10.3390/instruments4010009
Asaadi J, Auger M, Ereditato A, Goeldi D, Kose U, Kreslo I, Lorca D, Luethi M, Rudolf Von Rohr CBU, Sinclair J, et al. First Demonstration of a Pixelated Charge Readout for Single-Phase Liquid Argon Time Projection Chambers. Instruments. 2020; 4(1):9. https://doi.org/10.3390/instruments4010009
Chicago/Turabian StyleAsaadi, Jonathan, Martin Auger, Antonio Ereditato, Damian Goeldi, Umut Kose, Igor Kreslo, David Lorca, Matthias Luethi, Christoph Benjamin Urs Rudolf Von Rohr, James Sinclair, and et al. 2020. "First Demonstration of a Pixelated Charge Readout for Single-Phase Liquid Argon Time Projection Chambers" Instruments 4, no. 1: 9. https://doi.org/10.3390/instruments4010009
APA StyleAsaadi, J., Auger, M., Ereditato, A., Goeldi, D., Kose, U., Kreslo, I., Lorca, D., Luethi, M., Rudolf Von Rohr, C. B. U., Sinclair, J., Stocker, F., & Weber, M. (2020). First Demonstration of a Pixelated Charge Readout for Single-Phase Liquid Argon Time Projection Chambers. Instruments, 4(1), 9. https://doi.org/10.3390/instruments4010009






