Superlattices, Bonding-Antibonding, Fermi Surface Nesting, and Superconductivity
Abstract
1. Introduction
2. Results
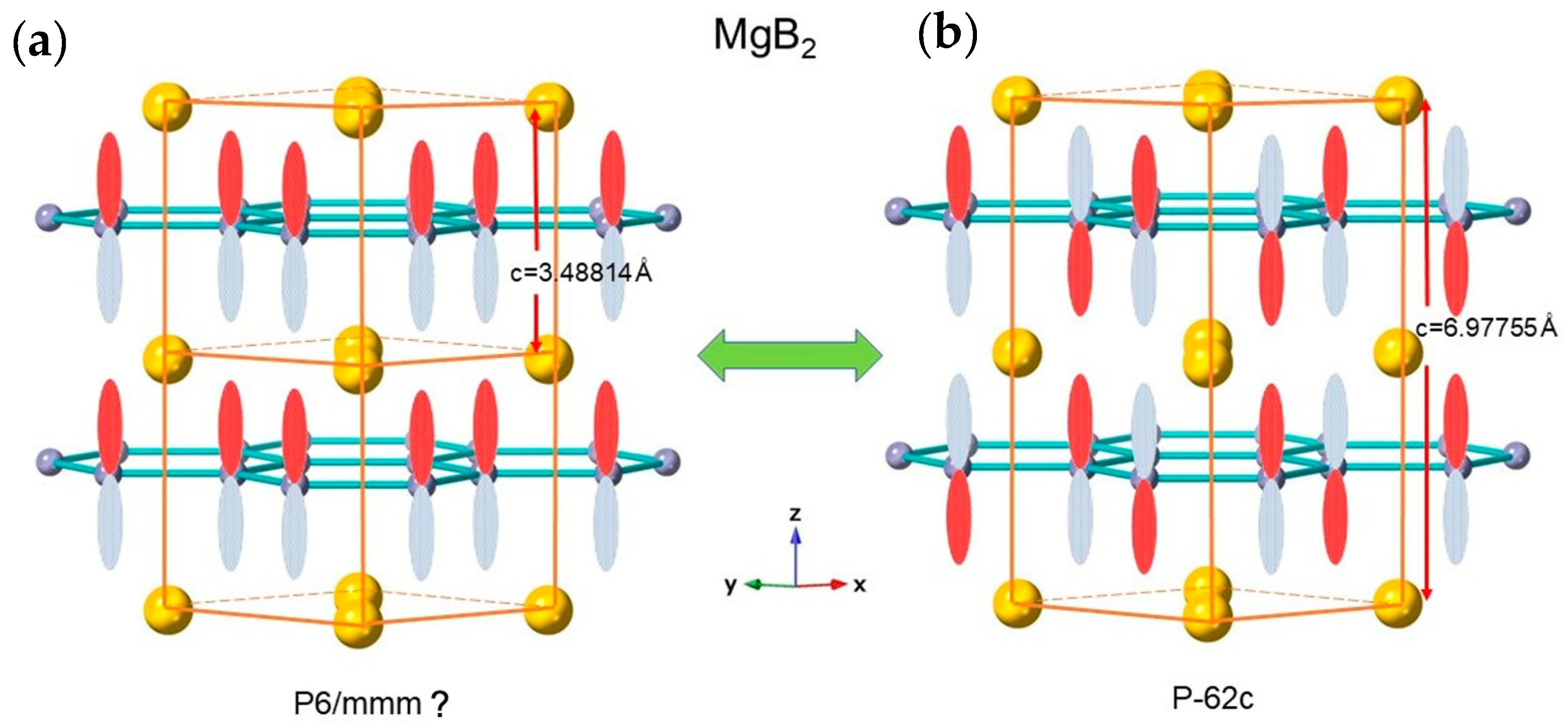
2.1. Electronic Band Structures: Unit Cells and Superlattices
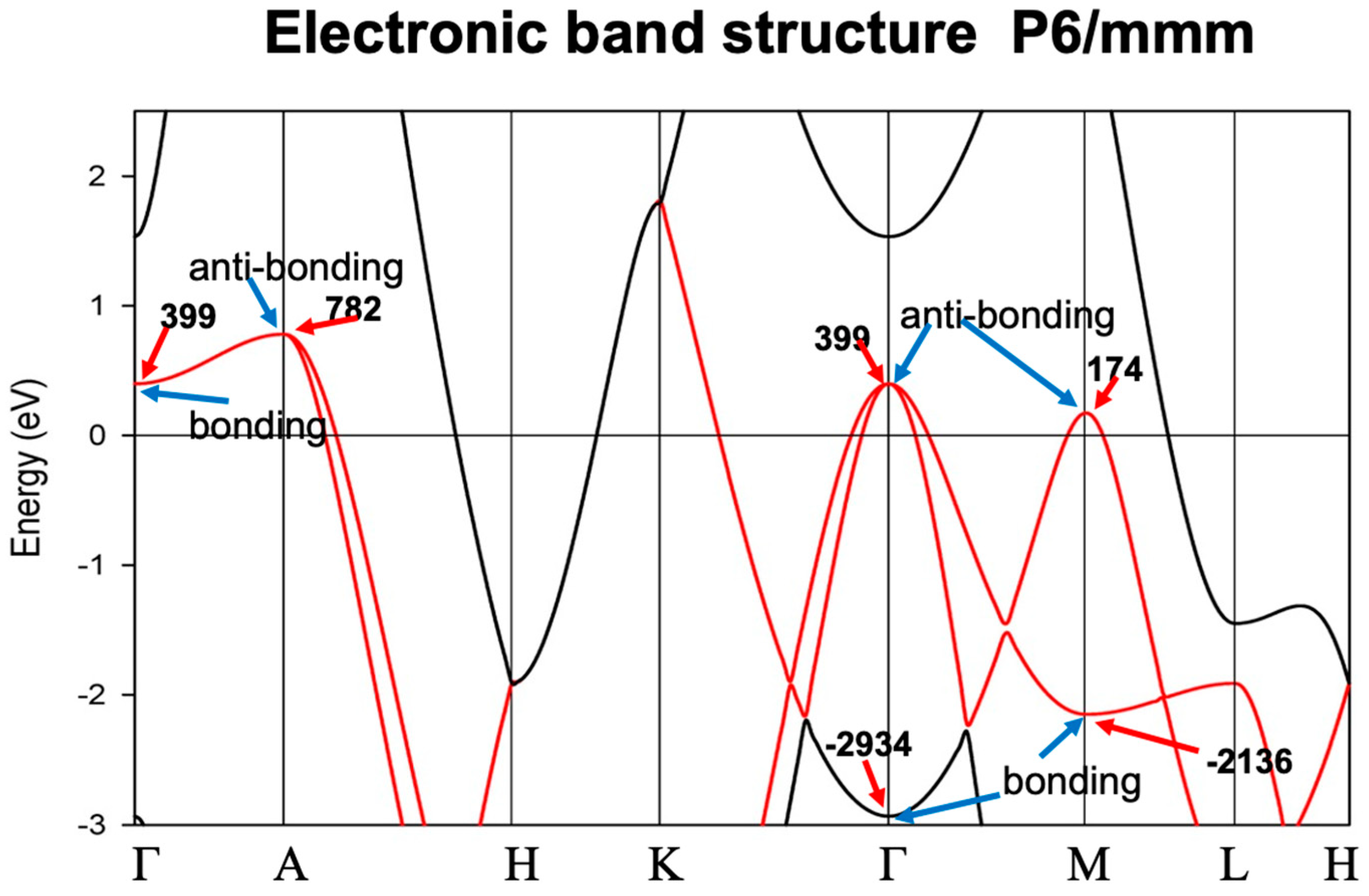
2.2. Energy Values and Fermi Surfaces
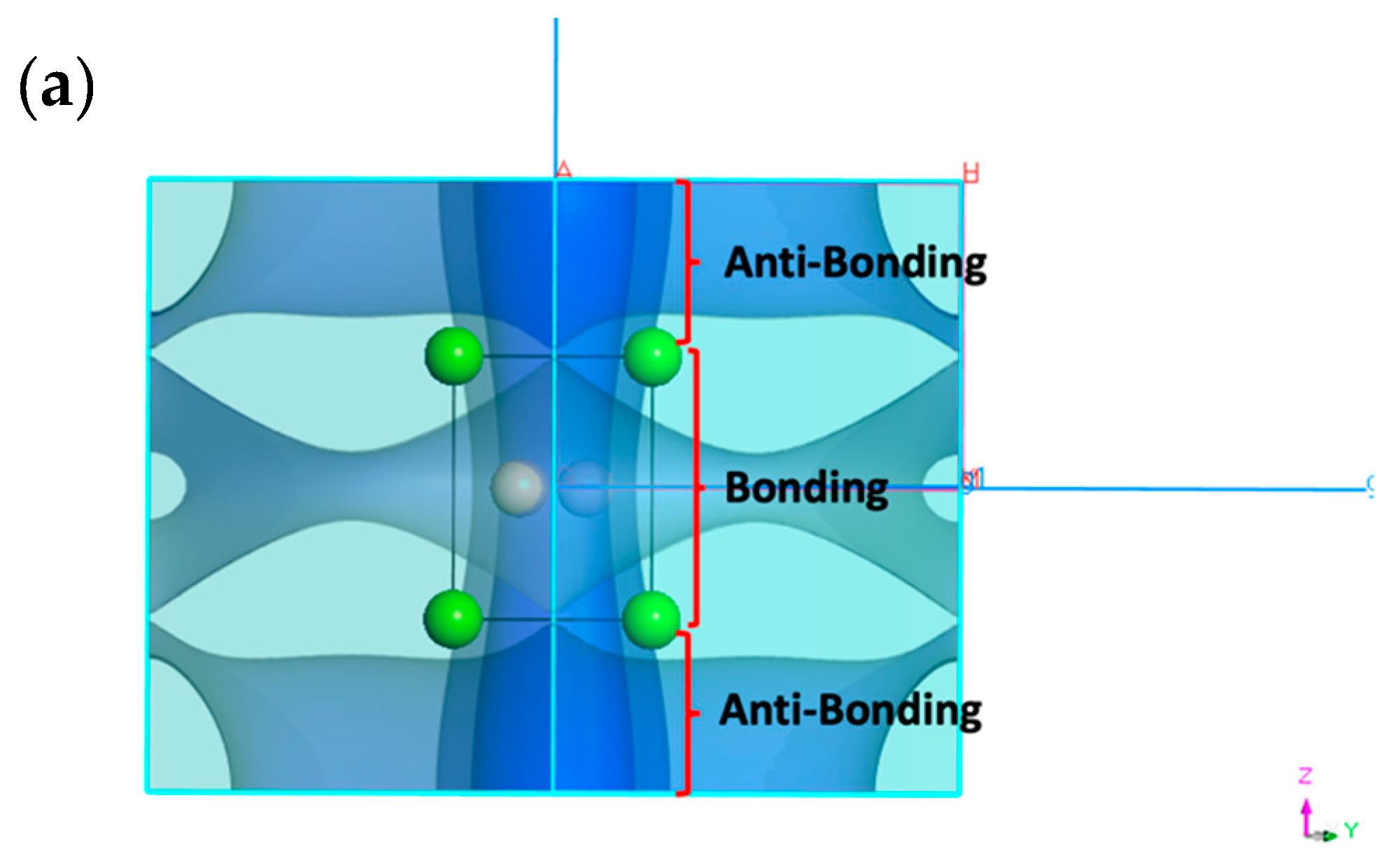
3. Discussion
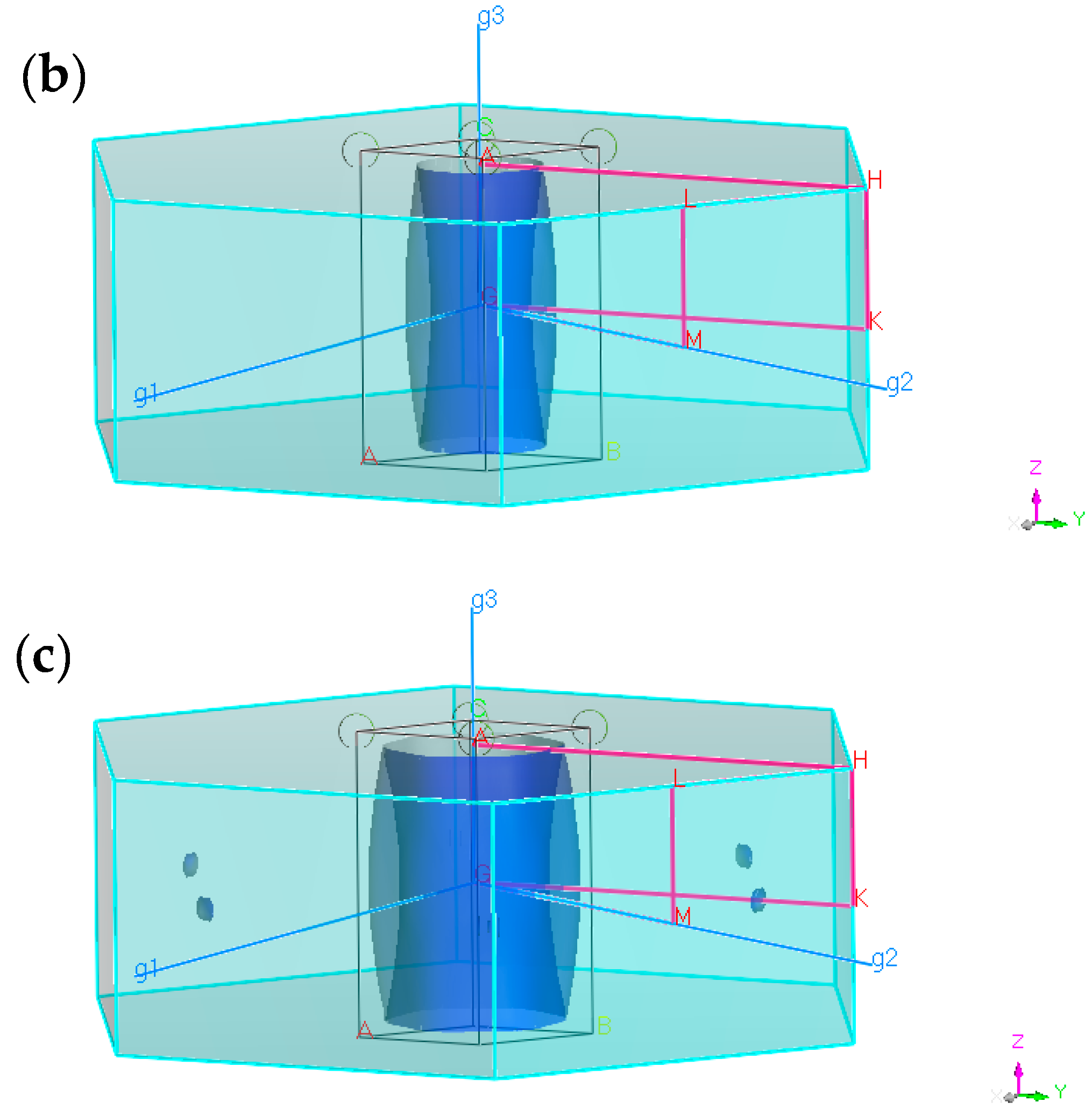
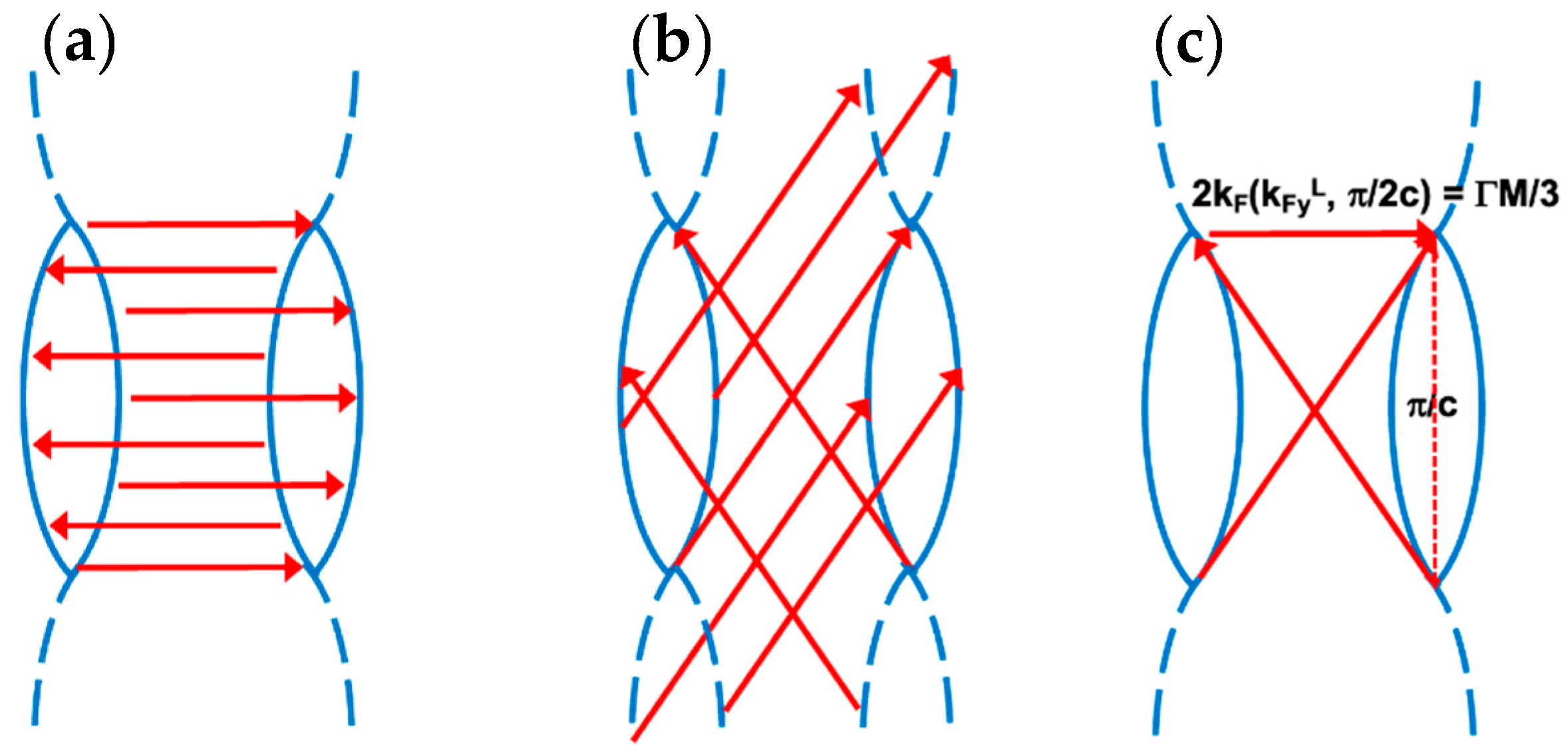
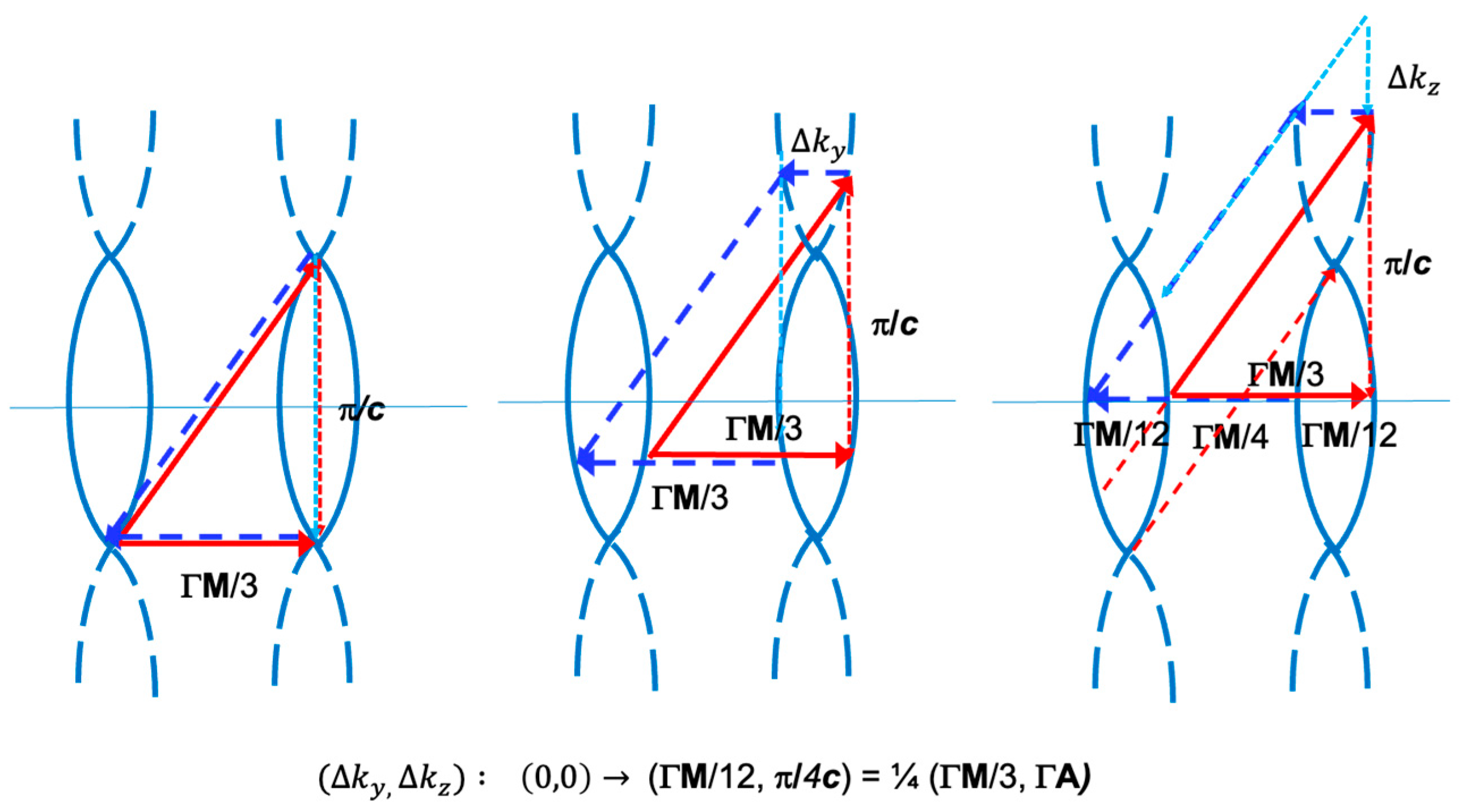
3.1. Fermi Surface Nesting: Density Waves
3.2. Revised Tight-Binding Equations
3.3. Identification of Cooper Pairing
4. Methods
5. Conclusions
Supplementary Materials
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Kohn, W. Fundamentals of density functional theory. In Density Functionals: Theory and Applications; Springer: Berlin/Heidelberg, Germany, 1998; Volume 500, pp. 1–7. [Google Scholar]
- Kohn, W. Nobel Lecture: Electronic structure of matter—Wave functions and density functionals. Rev. Mod. Phys. 1999, 71, 1253–1266. [Google Scholar] [CrossRef]
- Martin, R.M. Electronic Structure—Basic Theory and Practical Methods; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar]
- Sholl, D.S.; Steckel, J.A. Density Functional Theory—A Practical Introduction; Wiley: Hoboken, NJ, USA, 2009. [Google Scholar]
- Giustino, F. Materials Modelling Using Density Functional Theory—Properties and Predictions; Oxford University Press: Oxford, UK, 2014. [Google Scholar]
- Teale, A.M.; Helgaker, T.; Savin, A.; Adamo, C.; Aradi, B.; Arbuznikov, A.V.; Ayers, P.W.; Baerends, E.J.; Barone, V.; Calaminici, P.; et al. DFT exchange: Sharing perspectives on the workhorse of quantum chemistry and materials science. Phys. Chem. Chem. Phys. 2022, 24, 28700–28781. [Google Scholar] [CrossRef] [PubMed]
- Shankar, S.; Muller, R.; Dunning, T.; Chen, G.H. Computational Materials, Chemistry, and Biochemistry: From Bold Initiatives to the Last Mile: In Honor of William A. Goddard’s Contributions to Science and Engineering; Springer Nature: Berlin/Heidelberg, Germany, 2021. [Google Scholar]
- Cheng, Y.; Wang, T.; Zhang, G. Artificial Intelligence for Materials Science; Springer: Berlin/Heidelberg, Germany, 2021. [Google Scholar]
- Sanna, A. Introduction to superconducting density functional theory. In The Physics of Correlated Insulators, Metals, and Superconductors; Lecture Notes of the Autumns School on Correlated Electrons 2017, Schriften des Forschungszentrums Jülich Reihe Modeling and Simulation; Forschungszentrum Jülich GmbH, Institute for Advanced Simulation: Jülich, Germany, 2017. [Google Scholar]
- Tachibana, A. Density functional theory for hidden high-Tc superconductivity. In High-Temperature Superconducting Materials; CRC Press: Boca Raton, FL, USA, 2020; pp. 99–106. [Google Scholar]
- Nomura, Y. Ab Initio Studies on Superconductivity in Alkali-Doped Fullerides; Springer: Berlin/Heidelberg, Germany, 2016. [Google Scholar]
- Duan, D.; Liu, Y.; Tian, F.; Li, D.; Huang, X.; Zhao, Z.L.; Yu, H.; Liu, B.; Tian, W.; Cui, T. Pressure-induced metallization of dense (H2S)2H2 with high-Tc superconductivity. Sci. Rep. 2015, 4, 6968. [Google Scholar] [CrossRef]
- Quan, Y.; Pickett, W. Van Hove singularities and spectral smearing in high temperature superconducting H3S. Phys. Rev. B 2016, 93, 104526. [Google Scholar] [CrossRef]
- Errea, I.; Belli, F.; Monacelli, L.; Sanna, A.; Koretsune, T.; Tadano, T.; Bianco, R.; Calandra, M.; Arita, R.; Mauri, F.; et al. Quantum crystal structure in the 250-kelvin superconducting lanthanum hydride. Nature 2020, 578, 66–69. [Google Scholar] [CrossRef]
- Liu, L.; Wang, C.; Yi, S.; Kim, K.W.; Kim, J.; Cho, J.-H. Microscopic mechanism of room-temperature superconductivity in compressed LaH 10. Phys. Rev. B 2019, 99, 140501. [Google Scholar] [CrossRef]
- Geballe, Z.M.; Liu, H.; Mishra, A.K.; Ahart, M.; Somayazulu, M.; Meng, Y.; Baldini, M.; Hemley, R.J. Synthesis and stability of lanthanum superhydrides. Angew. Chem. 2018, 130, 696–700. [Google Scholar] [CrossRef]
- Chu, C.W.; Canfield, P.C.; Dynes, R.C.; Fisk, Z.; Batlogg, B.; Deutscher, G.; Geballe, T.H.; Zhao, Z.X.; Greene, R.L.; Hosono, H.; et al. Epilogue: Superconducting materials past, present and future. Phys. C-Supercond. Its Appl. 2015, 514, 437–443. [Google Scholar] [CrossRef][Green Version]
- Kruglov, I.A.; Semenok, D.V.; Song, H.; Szczęśniak, R.; Wrona, I.A.; Akashi, R.; Esfahani, M.M.D.; Duan, D.; Cui, T.; Kvashnin, A.G. Superconductivity of LaH 10 and LaH 16 polyhydrides. Phys. Rev. B 2020, 101, 024508. [Google Scholar] [CrossRef]
- Liu, H.; Naumov, I.I.; Geballe, Z.M.; Somayazulu, M.; John, S.T.; Hemley, R.J. Dynamics and superconductivity in compressed lanthanum superhydride. Phys. Rev. B 2018, 98, 100102. [Google Scholar] [CrossRef]
- Papaconstantopoulos, D.; Mehl, M.; Chang, P.-H. High-temperature superconductivity in LaH 10. Phys. Rev. B 2020, 101, 060506. [Google Scholar] [CrossRef]
- Quan, Y.; Ghosh, S.S.; Pickett, W.E. Compressed hydrides as metallic hydrogen superconductors. Phys. Rev. B 2019, 100, 184505. [Google Scholar] [CrossRef]
- Wang, C.; Yi, S.; Cho, J.-H. Pressure dependence of the superconducting transition temperature of compressed LaH 10. Phys. Rev. B 2019, 100, 060502. [Google Scholar] [CrossRef]
- Wang, C.; Yi, S.; Cho, J.-H. Multiband nature of room-temperature superconductivity in LaH 10 at high pressure. Phys. Rev. B 2020, 101, 104506. [Google Scholar] [CrossRef]
- Drozdov, A.P.; Eremets, M.I.; Troyan, I.A. Conventional superconductivity at 190 K at high pressures. arXiv 2014, arXiv:1412.0460. [Google Scholar]
- Drozdov, A.P.; Eremets, M.I.; Troyan, I.A.; Ksenofontov, V.; Shylin, S.I. Conventional superconductivity at 203 kelvin at high pressures in the sulfur hydride system. Nature 2015, 525, 73–76. [Google Scholar] [CrossRef]
- Einaga, M.; Sakata, M.; Masuda, A.; Nakao, H.; Shimizu, K.; Drozdov, A.; Eremets, M.; Kawaguchi, S.; Hirao, N.; Ohishi, Y. Two-year progress in experimental investigation on high-temperature superconductivity of sulfur hydride. Jpn. J. Appl. Phys. 2017, 56, 05FA13. [Google Scholar] [CrossRef]
- Drozdov, A.; Kong, P.; Minkov, V.; Besedin, S.; Kuzovnikov, M.; Mozaffari, S.; Balicas, L.; Balakirev, F.; Graf, D.; Prakapenka, V. Superconductivity at 250 K in lanthanum hydride under high pressures. Nature 2019, 569, 528–531. [Google Scholar] [CrossRef]
- Somayazulu, M.; Ahart, M.; Mishra, A.K.; Geballe, Z.M.; Baldini, M.; Meng, Y.; Struzhkin, V.V.; Hemley, R.J. Evidence for Superconductivity above 260 K in Lanthanum Superhydride at Megabar Pressures. Phys. Rev. Lett. 2019, 122, 027001. [Google Scholar] [CrossRef]
- Bose, S.K.; Kortus, J. Electron-Phonon Coupling in Metallic Solids from Density Functional Theory, Vibronic and Electron-Phonon Interactions and Their Role in Modern Chemistry and Physics; Transworld Research Network: Kerala, India, 2009; pp. 1–62. [Google Scholar]
- Heid, R. Electron-phonon coupling. In Lecture Notes of the Autumn School on Correlated Electrons; Pavarini, E., Koch, E., Scalettar, R., Martin, R., Eds.; Forschungszentrum Jülich GmbH Institute for Advanced Simulation: Julich, Germany, 2017; pp. 399–427. [Google Scholar]
- Marsiglio, F. Eliashberg theory: A short review. Ann. Phys. 2020, 417, 168102. [Google Scholar] [CrossRef]
- Sanna, A.; Pellegrini, C.; Gross, E. Combining Eliashberg theory with density functional theory for the accurate prediction of superconducting transition temperatures and gap functions. Phys. Rev. Lett. 2020, 125, 057001. [Google Scholar] [CrossRef]
- Xie, S.; Quan, Y.; Hire, A.; Deng, B.; DeStefano, J.; Salinas, I.; Shah, U.; Fanfarillo, L.; Lim, J.; Kim, J. Machine learning of superconducting critical temperature from Eliashberg theory. Npj Comput. Mater. 2022, 8, 14. [Google Scholar] [CrossRef]
- Jones, W.; March, N.H. Theoretical Solid State Physics, Volume 1: Perfect lattices in Equilibrium; Dover Books: Mineola, NY, USA, 1973. [Google Scholar]
- Alarco, J.A.; Almutairi, A.; Mackinnon, I.D.R. Progress towards a universal approach for prediction of the superconducting transition temperature. J. Supercond. Nov. Magn 2020, 33, 2287–2293. [Google Scholar] [CrossRef]
- Mackinnon, I.D.R.; Almutairi, A.; Alarco, J.A. Insights from systematic DFT calculations on superconductors. In Real Perspectives of Fourier Transforms and Current Developments in Superconductivity; Arcos, J.M.V., Ed.; IntechOpen Ltd.: London UK, 2021; pp. 1–29. [Google Scholar] [CrossRef]
- Johansson, E.; Tasnadi, F.; Ektarawong, A.; Rosen, J.; Alling, B. The effect of strain and pressure on the electron-phonon coupling and superconductivity in MgB2—Benchmark of theoretical methodologies and outlook for nanostructure design. J. Appl. Phys. 2022, 131, 063902. [Google Scholar] [CrossRef]
- Almutairi, A. Electronic Band Structure Equations and Fermi Surface Evolution from 2D Materials to 3D Layered Superconducting Compounds; Queensland University of Technology: Brisbane, Australia, 2019. [Google Scholar]
- Calandra, M.; Lazzeri, M.; Mauri, F. Review: Anharmonic and non-adiabatic effects in MgB2: Implications for the isotope effect and interpretation of Raman spectra. Phys. C 2007, 456, 38–44. [Google Scholar] [CrossRef]
- Liu, A.Y.; Mazin, I.I.; Kortus, J. Beyond Eliashberg Superconductivity in MgB2: Anharmonicity, Two-Phonon Scattering, and Multiple Gaps. Phys. Rev. Lett. 2001, 87, 087005. [Google Scholar] [CrossRef]
- Yildirim, T.; Gülseren, O.; Lynn, J.W.; Brown, C.M.; Udovic, T.J.; Huang, Q.; Rogado, N.; Regan, K.A.; Hayward, M.A.; Slusky, J.S.; et al. Giant Anharmonicity and Nonlinear Electron-Phonon Coupling in MgB2: A Combined First-Principles Calculation and Neutron Scattering Study. Phys. Rev. Lett. 2001, 87, 037001. [Google Scholar] [CrossRef] [PubMed]
- Alarco, J.A.; Talbot, P.C.; Mackinnon, I.D.R. Phonon dispersion models for MgB2 with application of pressure. Phys. C Supercond. Appl. 2017, 536, 11–17. [Google Scholar] [CrossRef]
- Alarco, J.A.; Talbot, P.C.; Mackinnon, I.D.R. Phonon anomalies predict superconducting Tc for AlB2-type structures. Phys. Chem. Chem. Phys. 2015, 17, 25090–25099. [Google Scholar] [CrossRef] [PubMed]
- Alarco, J.A.; Chou, A.; Talbot, P.C.; Mackinnon, I.D.R. Phonon Modes of MgB2: Super-lattice Structures and Spectral Response. Phys. Chem. Chem. Phys. 2014, 16, 24443–24456. [Google Scholar] [CrossRef]
- Harrison, W.A. Electronic Structure and the Properties of Solids: The Physics of the Chemical Bond; Dover Publications, Inc.: New York, NY, USA, 2012. [Google Scholar]
- Sutton, A.P. Electronic Structure of Materials; Clarendon Press, Oxford Science Publications: Oxford, UK, 2004. [Google Scholar]
- Canadell, E.; Doublet, M.-L.; Iung, C. Orbital Approach to the Electronic Structure of Solids; Oxford University Press: Oxford, UK, 2012. [Google Scholar]
- Evarestov, R.A.; Smirnov, V.P. Site symmetry in Crystals: Theory and Applications; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Urch, D.S. Orbitals and Symmetry; Penguin Books Ltd.: Harmondsworth, UK, 1970. [Google Scholar]
- Alarco, J.A.; Gupta, B.; Shahbazi, M.; Appadoo, D.; Mackinnon, I.D.R. THz/Far infrared synchrotron observations of superlattice frequencies in MgB2. Phys. Chem. Chem. Phys. 2021, 23, 23922–23932. [Google Scholar] [CrossRef] [PubMed]
- Stohr, J.; Siegmann, H.C. Magnetism—From Fundamentals to Nanoscale Dynamics; Springer: Berlin/Heidelberg, Germany, 2006. [Google Scholar]
- Askerzade, I. Unconventional Superconductors—Anisotropy and Multiband Effects; Springer: Berlin/Heidelberg, Germany, 2012. [Google Scholar]
- Askerzade, I.N. Effect of Coulomb Repulsion on the Critical Temperature in Layered Superconductors with Arbitrary Layer Thicknesses. J. Korean Phys. Soc. 2004, 45, 475–478. [Google Scholar]
- Askerzade, I.N. Josephson-effect samplers: A review. Tech. Phys. 2006, 51, 393–400. [Google Scholar] [CrossRef]
- Askerzade, I.N. Reviews of Topical Problems—Study of layered superconductors in the theory of an electron—Phonon coupling mechanism. Phys.-Uspekhi 2009, 52, 977–988. [Google Scholar] [CrossRef]
- Askerzade, I.N.; Tanatar, B. Effects of anisotropy on the critical temperature in layered nonadiabatic superconductors. Phys. C 2003, 384, 404–410. [Google Scholar] [CrossRef]
- Alarco, J.A.; Talbot, P.C.; Mackinnon, I.D.R. A complete and accurate description of superconductivity of AlB2-type structures from phonon dispersion calculations. J. Supercond. Nov. Magnet. 2018, 31, 727–731. [Google Scholar] [CrossRef]
- Campi, G.; Ricci, A.; Bianconi, A. Local Structure in Mg1−xAlxB2 System by High Resolution Neutron Diffraction. J. Supercond. Nov. Magn. 2012, 25, 1319–1322. [Google Scholar] [CrossRef]
- Wu, S.Y.; Shih, P.-H.; Ji, J.-Y.; Chan, T.-S.; Yang, C.C. Direct observation of charge re-distribution in a MgB2 superconductor. Supercond. Sci. Technol. 2016, 29, 045001. [Google Scholar] [CrossRef]
- Agrestini, S.; Metallo, C.; Filippi, M.; Simonelli, L.; Campi, G.; Sanipoli, C.; Liarokapis, E.; De Negri, S.; Giovannini, M.; Saccone, A.; et al. Substitution of Sc for Mg in MgB2: Effects on transition temperature and Kohn anomaly. Phys. Rev. B 2004, 70, 134514. [Google Scholar] [CrossRef]
- Bianconi, A. Multiband superconductivity in high Tc cuprates and diborides. J. Phys. Chem. Solids 2006, 67, 567–570. [Google Scholar] [CrossRef]
- Mazziotti, M.V.; Bianconi, A.; Raimondi, R.; Campi, G.; Valletta, A. Spin–orbit coupling controlling the superconducting dome of artificial superlattices of quantum wells. J. Appl. Phys. 2022, 132, 193908. [Google Scholar] [CrossRef]
- Grüner, G. Density Waves in Solids; CRC Press: Boca Raton, FL, USA, 1994. [Google Scholar]
- Ziman, J.M. Electrons and Phonons: The Theory of Transport Phenomena in Solids; Oxford University Press: Oxford, UK, 1960. [Google Scholar]
- Ziman, J.M. Principles of the Theory of Solids, 2nd ed.; Cambridge University Press: Cambridge, UK, 1972. [Google Scholar]
- Yu, P.Y.; Cardona, M. Fundamentals of Semiconductors—Physics and Materials Properties; Stanley, H.E., Rhodes, W.T., Eds.; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Alarco, J.A.; Talbot, P.C.; Mackinnon, I.D.R. Electron density response to phonon dynamics in MgB2: An indicator of superconducting properties. Mod. Numer. Sim. Mater. Sci. 2018, 8, 21–46. [Google Scholar]
- Alarco, J.A.; Mackinnon, I.D.R. Phonon dispersions as indicators of dynamic symmetry reduction in superconductors. In Phonons in Low Dimensional Structures; Stavrou, V.N., Ed.; InTech Open: London, UK, 2018; pp. 75–101. [Google Scholar]
- Kortus, J. Current progress in the theoretical understanding of MgB2. Phys. C 2007, 456, 54–62. [Google Scholar] [CrossRef]
- Coulson, C.A. Valence, 2nd ed.; Oxford University Press: London, UK, 1963. [Google Scholar]
- Annett, J.F. Superconductivity, Superfluids and Condensates; Oxford University Press: New York, NY, USA, 2013. [Google Scholar]
- Alarco, J.A.; Talbot, P.C.; Mackinnon, I.D. Coherent phonon decay and the boron isotope effect for MgB2. Phys. Chem. Chem. Phys. 2014, 16, 25386–25392. [Google Scholar] [CrossRef]
- Mackinnon, I.D.R.; Talbot, P.C.; Alarco, J.A. Phonon dispersion anomalies and superconductivity in metal substituted MgB2. Comp. Mater. Sci. 2017, 130, 191–203. [Google Scholar] [CrossRef]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Alarco, J.A.; Mackinnon, I.D.R. Superlattices, Bonding-Antibonding, Fermi Surface Nesting, and Superconductivity. Condens. Matter 2023, 8, 72. https://doi.org/10.3390/condmat8030072
Alarco JA, Mackinnon IDR. Superlattices, Bonding-Antibonding, Fermi Surface Nesting, and Superconductivity. Condensed Matter. 2023; 8(3):72. https://doi.org/10.3390/condmat8030072
Chicago/Turabian StyleAlarco, Jose A., and Ian D. R. Mackinnon. 2023. "Superlattices, Bonding-Antibonding, Fermi Surface Nesting, and Superconductivity" Condensed Matter 8, no. 3: 72. https://doi.org/10.3390/condmat8030072
APA StyleAlarco, J. A., & Mackinnon, I. D. R. (2023). Superlattices, Bonding-Antibonding, Fermi Surface Nesting, and Superconductivity. Condensed Matter, 8(3), 72. https://doi.org/10.3390/condmat8030072






