Electrical Manipulation of Spin-Dependent Anisotropy of a Dirac Cone in a Graphene Superlattice with Alternating Periodic Electrostatic and Exchange Fields
Abstract
1. Introduction
2. Theoretical Framework
3. Results and Discussion
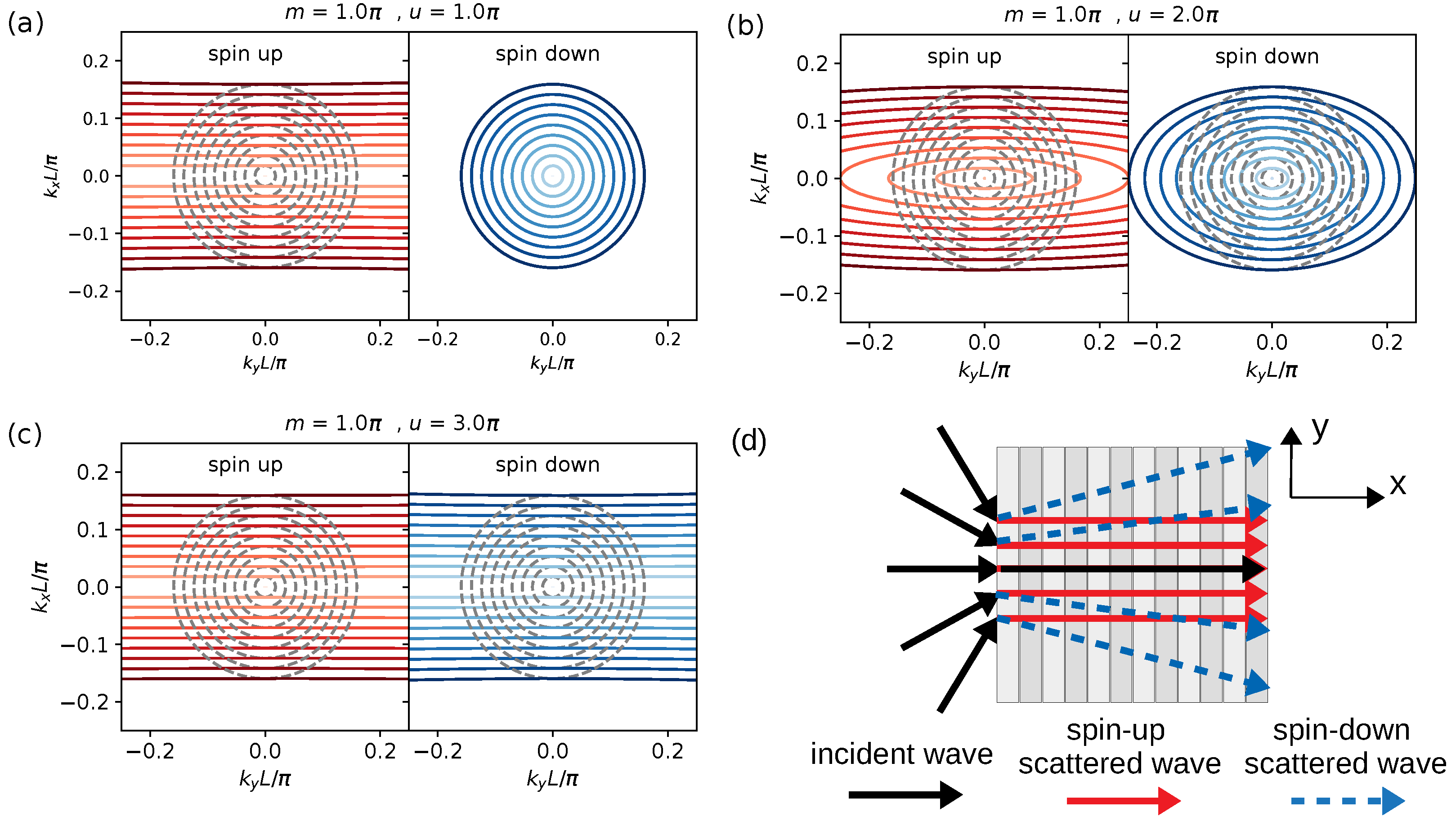
3.1. Spin-Dependent Anisotropy Miniband
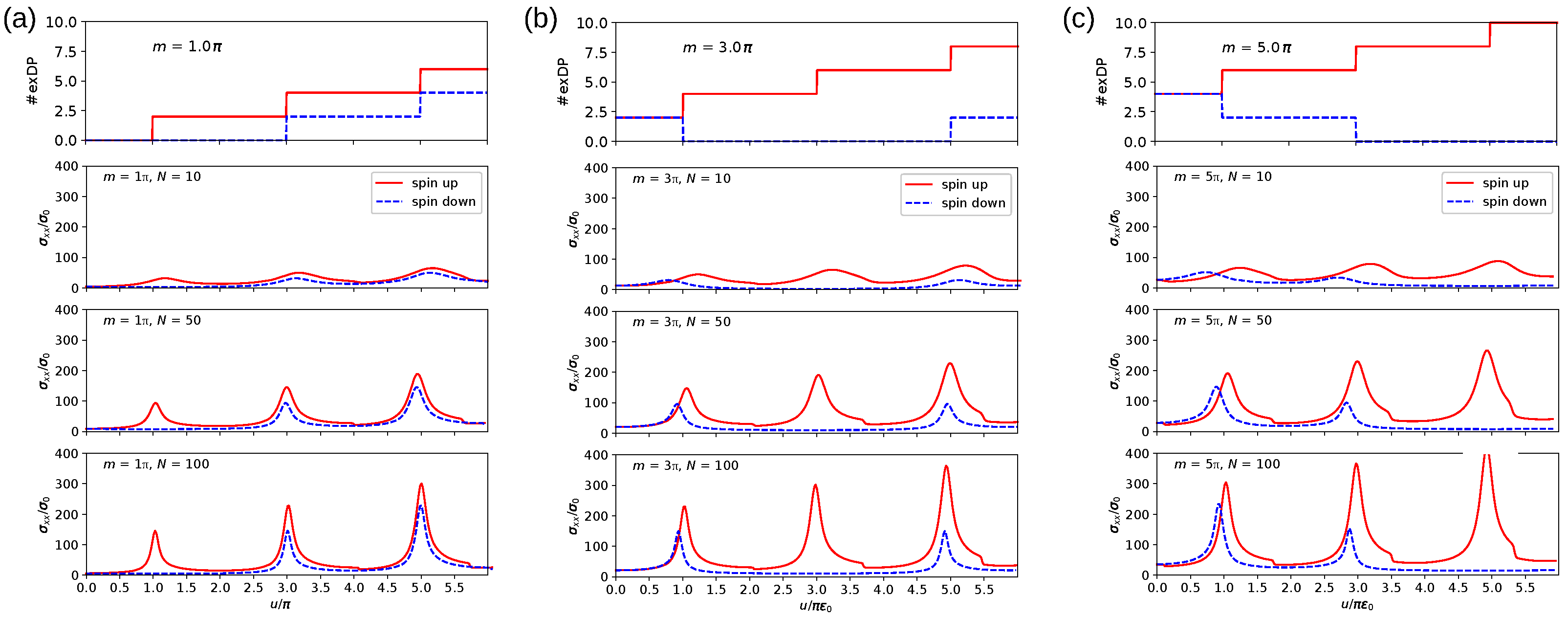
3.2. Spin-Polarized Transport and Extra Dirac Points
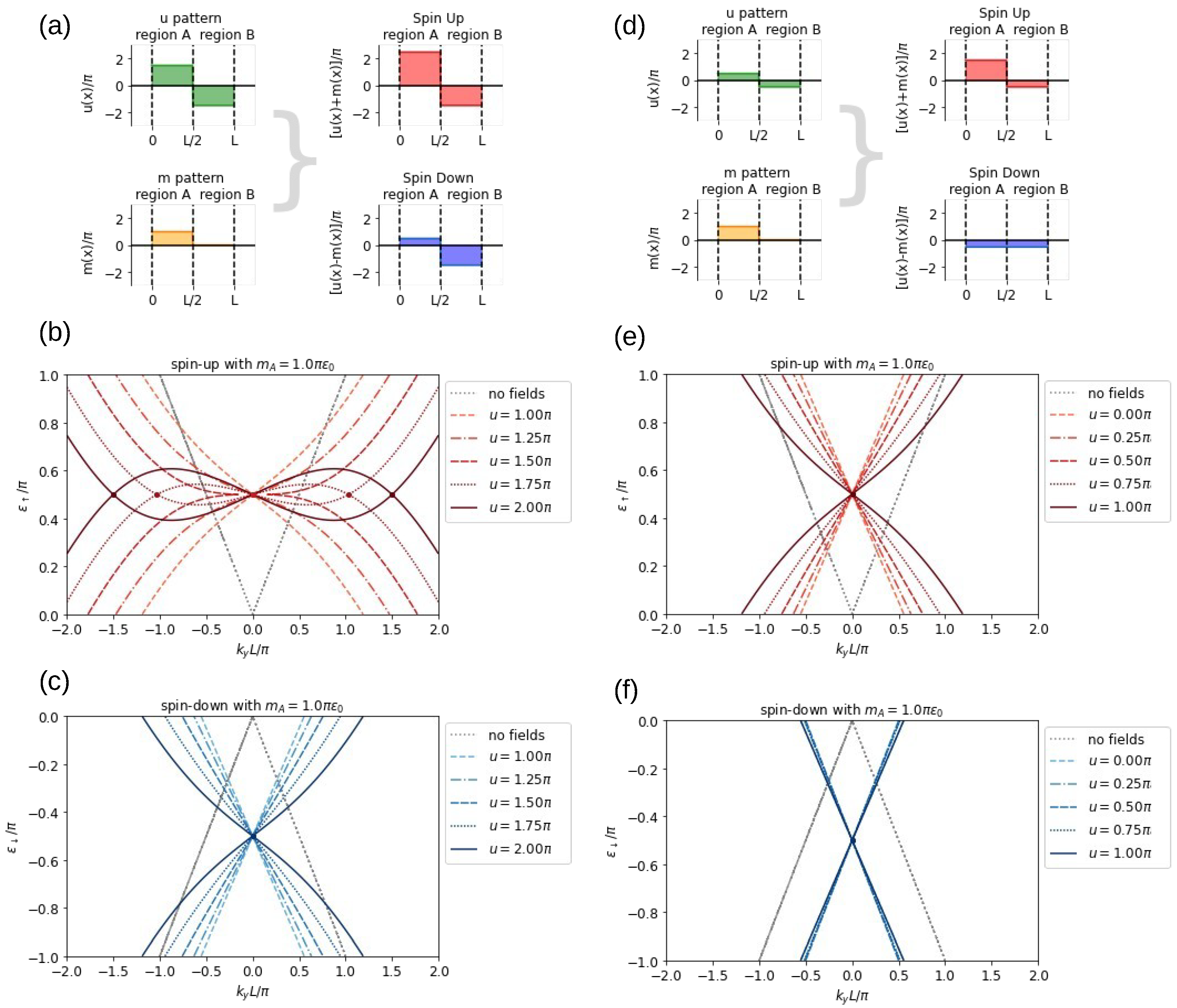
3.3. Electrical Controllable Spin-Dependent Band Structure
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Novoselov, K.S.; Geim, A.K.; Morozov, S.V.; Jiang, D.; Katsnelson, M.I.; Grigorieva, I.V.; Dubonos, S.; Firsov, A. Two-dimensional gas of massless Dirac fermions in graphene. Nature 2005, 438, 197–200. [Google Scholar] [CrossRef] [PubMed]
- Castro Neto, A.H.; Guinea, F.; Peres, N.M.R.; Novoselov, K.S.; Geim, A.K. The electronic properties of graphene. Rev. Mod. Phys. 2009, 81, 109–162. [Google Scholar] [CrossRef]
- Katsnelson, M.I.; Novoselov, K.S.; Geim, A.K. Chiral tunnelling and the Klein paradox in graphene. Nat. Phys. 2006, 2, 620–625. [Google Scholar] [CrossRef]
- Novoselov, K.S.; Jiang, Z.; Zhang, Y.; Morozov, S.V.; Stormer, H.L.; Zeitler, U.; Maan, J.C.; Boebinger, G.S.; Kim, P.; Geim, A.K. Room-Temperature Quantum Hall Effect in Graphene. Science 2007, 315, 1379. [Google Scholar] [CrossRef]
- Ingla-Aynés, J.; Guimarães, M.H.D.; Meijerink, R.J.; Zomer, P.J.; van Wees, B.J. 24-μm spin relaxation length in boron nitride encapsulated bilayer graphene. Phys. Rev. B 2015, 92, 201410. [Google Scholar] [CrossRef]
- Drögeler, M.; Franzen, C.; Volmer, F.; Pohlmann, T.; Banszerus, L.; Wolter, M.; Watanabe, K.; Taniguchi, T.; Stampfer, C.; Beschoten, B. Spin Lifetimes Exceeding 12 ns in Graphene Nonlocal Spin Valve Devices. Nano Lett. 2016, 16, 3533–3539. [Google Scholar] [CrossRef]
- Haugen, H.; Huertas-Hernando, D.; Brataas, A. Spin transport in proximity-induced ferromagnetic graphene. Phys. Rev. B 2008, 77, 115406. [Google Scholar] [CrossRef]
- Swartz, A.G.; Odenthal, P.M.; Hao, Y.; Ruoff, R.S.; Kawakami, R.K. Integration of the ferromagnetic insulator EuO onto graphene. ACS Nano 2012, 6, 10063–10069. [Google Scholar] [CrossRef]
- Yang, H.X.; Hallal, A.; Terrade, D.; Waintal, X.; Roche, S.; Chshiev, M. Proximity Effects Induced in Graphene by Magnetic Insulators: First-Principles Calculations on Spin Filtering and Exchange-Splitting Gaps. Phys. Rev. Lett. 2013, 110, 046603. [Google Scholar] [CrossRef] [PubMed]
- Su, S.; Barlas, Y.; Li, J.; Shi, J.; Lake, R.K. Effect of intervalley interaction on band topology of commensurate graphene/EuO heterostructures. Phys. Rev. B 2017, 95, 075418. [Google Scholar] [CrossRef]
- Hallal, A.; Ibrahim, F.; Yang, H.; Roche, S.; Chshiev, M. Tailoring magnetic insulator proximity effects in graphene: First-principles calculations. 2D Mater. 2017, 4, 025074. [Google Scholar] [CrossRef]
- Wei, P.; Lee, S.; Lemaitre, F.; Pinel, L.; Cutaia, D.; Cha, W.; Katmis, F.; Zhu, Y.; Heiman, D.; Hone, J.; et al. Strong interfacial exchange field in the graphene/EuS heterostructure. Nat. Mater. 2016, 15, 711–716. [Google Scholar] [CrossRef] [PubMed]
- Cao, Y.; Fatemi, V.; Fang, S.; Watanabe, K.; Taniguchi, T.; Kaxiras, E.; Jarillo-Herrero, P. Unconventional superconductivity in magic-angle graphene superlattices. Nature 2018, 556, 43–50. [Google Scholar] [CrossRef]
- Cao, Y.; Chowdhury, D.; Rodan-Legrain, D.; Rubies-Bigorda, O.; Watanabe, K.; Taniguchi, T.; Senthil, T.; Jarillo-Herrero, P. Strange Metal in Magic-Angle Graphene with near Planckian Dissipation. Phys. Rev. Lett. 2020, 124, 076801. [Google Scholar] [CrossRef]
- He, P.; Koon, G.K.W.; Isobe, H.; Tan, J.Y.; Hu, J.; Neto, A.H.C.; Fu, L.; Yang, H. Graphene moiré superlattices with giant quantum nonlinearity of chiral Bloch electrons. Nat. Nanotechnol. 2022, 17, 378–383. [Google Scholar] [CrossRef] [PubMed]
- Barbier, M.; Vasilopoulos, P.; Peeters, F.M. Dirac electrons in a Kronig-Penney potential: Dispersion relation and transmission periodic in the strength of the barriers. Phys. Rev. B 2009, 80, 205415. [Google Scholar] [CrossRef]
- Park, C.H.; Yang, L.; Son, Y.W.; Cohen, M.L.; Louie, S.G. Anisotropic behaviours of massless Dirac fermions in graphene under periodic potentials. Nat. Phys. 2008, 4, 213–217. [Google Scholar] [CrossRef]
- Park, C.h.; Son, Y.w.; Yang, L.; Cohen, M.L.; Louie, S.G. Electron Beam Supercollimation in Graphene Superlattices. Nano 2008, 8, 2920–2924. [Google Scholar] [CrossRef] [PubMed]
- Choi, S.; Park, C.H.; Louie, S.G. Electron Supercollimation in Graphene and Dirac Fermion Materials Using One-Dimensional Disorder Potentials. Phys. Rev. Lett. 2014, 113, 026802. [Google Scholar] [CrossRef]
- Masir, M.R.; Vasilopoulos, P.; Peeters, F. Kronig–Penney model of scalar and vector potentials in graphene. J. Phys. Condens. Matter 2010, 22, 465302. [Google Scholar] [CrossRef]
- Brey, L.; Fertig, H.A. Emerging Zero Modes for Graphene in a Periodic Potential. Phys. Rev. Lett. 2009, 103, 046809. [Google Scholar] [CrossRef]
- Barbier, M.; Vasilopoulos, P.; Peeters, F.M. Extra Dirac points in the energy spectrum for superlattices on single-layer graphene. Phys. Rev. B-Condens. Matter Mater. Phys. 2010, 81, 075438. [Google Scholar] [CrossRef]
- Dell’Anna, L.; De Martino, A. Magnetic superlattice and finite-energy Dirac points in graphene. Phys. Rev. B 2011, 83, 155449. [Google Scholar] [CrossRef]
- Burset, P.; Yeyati, A.L.; Brey, L.; Fertig, H.A. Transport in superlattices on single-layer graphene. Phys. Rev. B 2011, 83, 195434. [Google Scholar] [CrossRef]
- Yankowitz, M.; Xue, J.; Cormode, D.; Sanchez-Yamagishi, J.D.; Watanabe, K.; Taniguchi, T.; Jarillo-Herrero, P.; Jacquod, P.; LeRoy, B.J. Emergence of superlattice Dirac points in graphene on hexagonal boron nitride. Nat. Phys. 2012, 8, 382–386. [Google Scholar] [CrossRef]
- Ponomarenko, L.A.; Gorbachev, R.V.; Yu, G.L.; Elias, D.C.; Jalil, R.; Patel, A.A.; Mishchenko, A.; Mayorov, A.S.; Woods, C.R.; Wallbank, J.R.; et al. Cloning of Dirac fermions in graphene superlattices. Nature 2013, 497, 594–597. [Google Scholar] [CrossRef] [PubMed]
- Zhang, R.L.; Li, J.J.; Zhou, Y.; Peng, R.W.; Huang, R.S.; Wang, M. Tunable Dirac points and perfect transmission in asymmetric graphene superlattices. Appl. Phys. Express 2015, 8, 085102. [Google Scholar] [CrossRef]
- Somroob, P.; Sutthibutpong, T.; Tangwancharoen, S.; Liewrian, W. Tunable tilted anisotropy of massless Dirac fermion in magnetic Kronig-Penney-type graphene. Phys. E Low-Dimens. Syst. Nanostructures 2021, 127, 114501. [Google Scholar] [CrossRef]
- Wang, L.G.; Zhu, S.Y. Electronic band gaps and transport properties in graphene superlattices with one-dimensional periodic potentials of square barriers. Phys. Rev. B 2010, 81, 205444. [Google Scholar] [CrossRef]
- Kang, W.H.; Chen, S.C.; Liu, M.H. Cloning of zero modes in one-dimensional graphene superlattices. Phys. Rev. B 2020, 102, 195432. [Google Scholar] [CrossRef]
- Park, C.H.; Son, Y.W.; Yang, L.; Cohen, M.L.; Louie, S.G. Landau levels and quantum Hall effect in graphene superlattices. Phys. Rev. Lett. 2009, 103, 046808. [Google Scholar] [CrossRef]
- Forti, S.; Stöhr, A.; Zakharov, A.; Coletti, C.; Emtsev, K.; Starke, U. Mini-Dirac cones in the band structure of a copper intercalated epitaxial graphene superlattice. 2D Mater. 2016, 3, 035003. [Google Scholar] [CrossRef]
- Faizabadi, E.; Esmaeilzadeh, M.; Sattari, F. Spin filtering in a ferromagnetic graphene superlattice. Eur. Phys. J. B 2012, 85, 198. [Google Scholar] [CrossRef]
- Lu, W.T.; Li, W.; Wang, Y.L.; Jiang, H.; Xu, C.T. Tunable wavevector and spin filtering in graphene induced by resonant tunneling. Appl. Phys. Lett. 2013, 103, 062108. [Google Scholar] [CrossRef]
- Wu, Q.P.; Liu, Z.F.; Chen, A.X.; Xiao, X.B.; Miao, G.X. Tunable Dirac points and high spin polarization in ferromagnetic-strain graphene superlattices. Sci. Rep. 2017, 7, 14636. [Google Scholar] [CrossRef]
- Leutenantsmeyer, J.C.; Kaverzin, A.A.; Wojtaszek, M.; Van Wees, B.J. Proximity induced room temperature ferromagnetism in graphene probed with spin currents. 2D Mater. 2016, 4, 014001. [Google Scholar] [CrossRef]
- Arovas, D.; Brey, L.; Fertig, H.; Kim, E.A.; Ziegler, K. Dirac spectrum in piecewise constant one-dimensional (1D) potentials. New J. Phys. 2010, 12, 123020. [Google Scholar] [CrossRef]
- Büttiker, M. Four-Terminal Phase-Coherent Conductance. Phys. Rev. Lett. 1986, 57, 1761–1764. [Google Scholar] [CrossRef]
- Panda, J.; Ramu, M.; Karis, O.; Sarkar, T.; Kamalakar, M.V. Ultimate Spin Currents in Commercial Chemical Vapor Deposited Graphene. ACS Nano 2020, 14, 12771–12780. [Google Scholar] [CrossRef] [PubMed]
- Dubey, S.; Singh, V.; Bhat, A.K.; Parikh, P.; Grover, S.; Sensarma, R.; Tripathi, V.; Sengupta, K.; Deshmukh, M.M. Tunable superlattice in graphene to control the number of Dirac points. Nano Lett. 2013, 13, 3990–3995. [Google Scholar] [CrossRef]
- Forsythe, C.; Zhou, X.; Watanabe, K.; Taniguchi, T.; Pasupathy, A.; Moon, P.; Koshino, M.; Kim, P.; Dean, C.R. Band structure engineering of 2D materials using patterned dielectric superlattices. Nat. Nanotechnol. 2018, 13, 566–571. [Google Scholar] [CrossRef] [PubMed]
- Lin, X.; Wang, H.; Pan, H.; Xu, H. Gap opening in single-layer graphene in the presence of periodic scalar and vector potentials within the continuum model. Phys. Lett. A 2012, 376, 584–589. [Google Scholar] [CrossRef]
- Lu, W.T.; Li, Y.F.; Tian, H.Y. Spin- and Valley-Dependent Electronic Structure in Silicene Under Periodic Potentials. Nanoscale Res. Lett. 2018, 13, 84. [Google Scholar] [CrossRef] [PubMed]






Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Somroob, P.; Liewrian, W. Electrical Manipulation of Spin-Dependent Anisotropy of a Dirac Cone in a Graphene Superlattice with Alternating Periodic Electrostatic and Exchange Fields. Condens. Matter 2023, 8, 28. https://doi.org/10.3390/condmat8010028
Somroob P, Liewrian W. Electrical Manipulation of Spin-Dependent Anisotropy of a Dirac Cone in a Graphene Superlattice with Alternating Periodic Electrostatic and Exchange Fields. Condensed Matter. 2023; 8(1):28. https://doi.org/10.3390/condmat8010028
Chicago/Turabian StyleSomroob, Pattana, and Watchara Liewrian. 2023. "Electrical Manipulation of Spin-Dependent Anisotropy of a Dirac Cone in a Graphene Superlattice with Alternating Periodic Electrostatic and Exchange Fields" Condensed Matter 8, no. 1: 28. https://doi.org/10.3390/condmat8010028
APA StyleSomroob, P., & Liewrian, W. (2023). Electrical Manipulation of Spin-Dependent Anisotropy of a Dirac Cone in a Graphene Superlattice with Alternating Periodic Electrostatic and Exchange Fields. Condensed Matter, 8(1), 28. https://doi.org/10.3390/condmat8010028





