Fermi-Liquid Nonadiabatic Highly Compressed Cesium Iodide Superconductor
Abstract
1. Introduction
2. Results
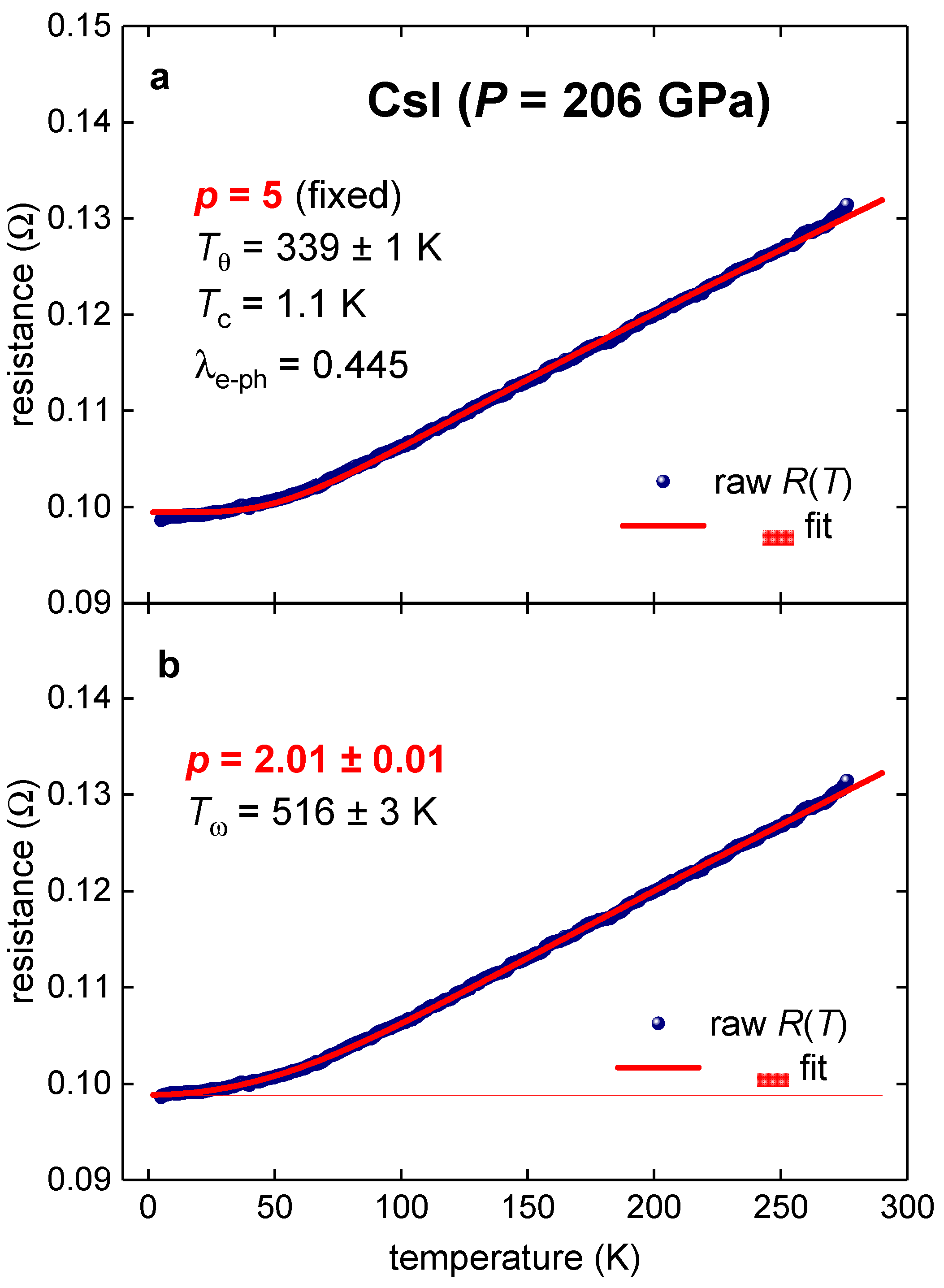
2.1. The Electron–Phonon Coupling Constant and the Debye Temperature in CsI at p = 206 GPa
2.2. Perfect Fermi Liquid Conductor CsI at p = 206 GPa
2.3. Compressed CsI (p = 206 GPa) in the Uemura Plot
2.4. Nonadiabalic Superconductivity in CsI (P = 206 GPa)
3. Discussion
4. Conclusions
- CsI is a perfect Fermi liquid metal.
- CsI exhibits the ratio of and, thus, it falls to the unconventional superconductors band in the Uemura plot. This level of the ratio is typical for many superconductors, including the electron–phonon-mediated A-15 compound V3Si. It should be mentioned that the magic-angle twisted bilayer graphene exhibits close and values [68,69].
Funding
Data Availability Statement
Conflicts of Interest
References
- Drozdov, A.P.; Eremets, M.I.; Troyan, I.A.; Ksenofontov, V.; Shylin, S.I. Conventional superconductivity at 203 kelvin at high pressures in the sulfur hydride system. Nature 2015, 525, 73–76. [Google Scholar] [CrossRef] [PubMed]
- Drozdov, A.P.; Kong, P.P.; Minkov, V.S.; Besedin, S.P.; Kuzovnikov, M.A.; Mozaffari, S.; Balicas, L.; Balakirev, F.F.; Graf, D.E.; Prakapenka, V.B.; et al. Superconductivity at 250 K in lanthanum hydride under high pressures. Nature 2019, 569, 528–531. [Google Scholar] [CrossRef] [PubMed]
- Somayazulu, M.; Ahart, M.; Mishra, A.K.; Geballe, Z.M.; Baldini, M.; Meng, Y.; Struzhkin, V.V.; Hemley, R.J. Evidence for superconductivity above 260 K in lanthanum superhydride at megabar pressures. Phys. Rev. Lett. 2019, 122, 027001. [Google Scholar] [CrossRef] [PubMed]
- Semenok, D.V.; Kvashnin, A.G.; Ivanova, A.G.; Svitlyk, V.; Fominski, V.Y.; Sadakov, A.V.; Sobolevskiy, O.A.; Pudalov, V.M.; Troyan, I.A.; Oganov, A.R. Superconductivity at 161 K in thorium hydride ThH10: Synthesis and properties. Mater. Today 2020, 33, 36–44. [Google Scholar] [CrossRef]
- Chen, W.; Semenok, D.V.; Kvashnin, A.G.; Huang, X.; Kruglov, I.A.; Galasso, M.; Song, H.; Duan, D.; Goncharov, A.F.; Prakapenka, V.B.; et al. Synthesis of molecular metallic barium superhydride: Pseudocubic BaH12. Nat. Commun. 2021, 12, 273. [Google Scholar] [CrossRef]
- Wang, N.; Shan, P.F.; Chen, K.Y.; Sun, J.P.; Yang, P.T.; Ma, X.L.; Wang, B.S.; Yu, X.H.; Zhang, S.; Chen, G.F.; et al. A low-Tc superconducting modification of Th4H15 synthesized under high pressure. Supercond. Sci. Technol. 2021, 34, 034006. [Google Scholar] [CrossRef]
- Troyan, I.A.; Semenok, D.V.; Kvashnin, A.G.; Sadakov, A.V.; Sobolevskiy, O.A.; Pudalov, V.M.; Ivanova, A.G.; Prakapenka, V.B.; Greenberg, E.; Gavriliuk, A.G.; et al. Anomalous high-temperature superconductivity in YH6. Adv. Mater. 2021, 33, 2006832. [Google Scholar] [CrossRef]
- Kong, P.; Minkov, V.S.; Kuzovnikov, M.A.; Drozdov, A.P.; Besedin, S.P.; Mozaffari, S.; Balicas, L.; Balakirev, F.F.; Prakapenka, V.B.; Chariton, S.; et al. Superconductivity up to 243 K in yttrium hydrides under high pressure. Nat. Commun. 2021, 12, 5075. [Google Scholar] [CrossRef]
- Ma, L.; Wang, K.; Xie, Y.; Yang, X.; Wang, Y.; Zhou, M.; Liu, H.; Yu, X.; Zhao, Y.; Wang, H.; et al. High-temperature superconducting phase in clathrate calcium hydride CaH6 up to 215 K at a pressure of 172 GPa. Phys. Rev. Lett. 2022, 128, 167001. [Google Scholar] [CrossRef]
- Semenok, D.V.; Troyan, I.A.; Ivanova, A.G.; Kvashnin, A.G.; Kruglov, I.A.; Hanfland, M.; Sadakov, A.V.; Sobolevskiy, O.A.; Pervakov, K.S.; Lyubutin, I.S.; et al. Superconductivity at 253 K in lanthanum–yttrium ternary hydrides. Mater. Today 2021, 48, 18–28. [Google Scholar] [CrossRef]
- Zhou, D.; Semenok, D.V.; Duan, D.; Xie, H.; Chen, W.; Huang, X.; Li, X.; Liu, B.; Oganov, A.R.; Cui, T. Superconducting praseodymium superhydrides. Sci. Adv. 2020, 6, eaax6849. [Google Scholar] [CrossRef] [PubMed]
- Hong, F.; Shan, P.F.; Yang, L.X.; Yue, B.B.; Yang, P.T.; Liua, Z.Y.; Sun, J.P.; Dai, J.H.; Yu, H.; Yin, Y.Y.; et al. Possible superconductivity at ∼70 K in tin hydride SnHx under high pressure. Mater. Today Phys. 2022, 22, 100596. [Google Scholar] [CrossRef]
- Chen, W.; Semenok, D.V.; Huang, X.; Shu, H.; Li, X.; Duan, D.; Cui, T.; Oganov, A.R. High-temperature superconducting phases in cerium superhydride with a Tc up to 115 K below a pressure of 1 Megabar. Phys. Rev. Lett. 2021, 127, 117001. [Google Scholar] [CrossRef] [PubMed]
- Osmond, I.; Moulding, O.; Cross, S.; Muramatsu, T.; Brooks, A.; Lord, O.; Fedotenko, T.; Buhot, J.; Friedemann, S. Clean-limit superconductivity in Im3m H3S synthesized from sulfur and hydrogen donor ammonia borane. Phys. Rev. B 2022, 105, L220502. [Google Scholar] [CrossRef]
- Semenok, D.V.; Troyan, I.A.; Sadakov, A.V.; Zhou, D.; Galasso, M.; Kvashnin, A.G.; Ivanova, A.G.; Kruglov, I.A.; Bykov, A.A.; Terent’ev, K.Y.; et al. Effect of magnetic impurities on superconductivity in LaH10. Adv. Mater. 2022, 34, 2204038. [Google Scholar] [CrossRef]
- Bi, J.; Nakamoto, Y.; Zhang, P.; Shimizu, K.; Zou, B.; Liu, H.; Zhou, M.; Liu, G.; Wang, H.; Ma, Y. Giant enhancement of superconducting critical temperature in substitutional alloy (La,Ce)H9. Nat. Commun. 2022, 13, 5952. [Google Scholar] [CrossRef]
- Li, Z.; He, X.; Zhang, C.; Wang, X.; Zhang, S.; Jia, Y.; Feng, S.; Lu, K.; Zhao, J.; Zhang, J.; et al. Superconductivity above 200 K discovered in superhydrides of calcium. Nat. Commun. 2022, 13, 2863. [Google Scholar] [CrossRef]
- Flores-Livas, J.A.; Boeri, L.; Sanna, A.; Profeta, G.; Arita, R.; Eremets, M. A perspective on conventional high-temperature superconductors at high pressure: Methods and materials. Phys. Rep. 2020, 856, 1–78. [Google Scholar] [CrossRef]
- Boeri, L.; Hennig, R.; Hirschfeld, P.; Profeta, G.; Sanna, A.; Zurek, E.; Pickett, W.E.; Amsler, M.; Dias, R.; Eremets, M.I.; et al. The 2021 room-temperature superconductivity roadmap. J. Phys. Condens. Matter 2022, 34, 183002. [Google Scholar] [CrossRef]
- Troyan, I.A.; Semenok, D.V.; Ivanova, A.G.; Kvashnin, A.G.; Zhou, D.; Sadakov, A.V.; Sobolevskiy, O.A.; Pudalov, V.M.; Lyubutin, I.S.; Oganov, A.R. High-temperature superconductivity in hydrides. Phys. Uspekhi 2022, 65, 748–761. [Google Scholar] [CrossRef]
- Satterthwaite, C.B.; Toepke, I.L. Superconductivity of hydrides and deuterides of thorium. Phys. Rev. Lett. 1970, 25, 741–743. [Google Scholar] [CrossRef]
- Wittig, J.; Matthias, B.T. Superconducting phosphorus. Science 1968, 160, 994–995. [Google Scholar] [CrossRef] [PubMed]
- Eliashberg, G.M. Interactions between electrons and lattice vibrations in a superconductor. Sov. Phys. JETP 1960, 11, 696–702. [Google Scholar]
- Hou, P.; Belli, F.; Bianco, R.; Errea, I. Strong anharmonic and quantum effects in -AlH3 under high pressure: A first-principles study. Phys. Rev. B 2021, 103, 134305. [Google Scholar] [CrossRef]
- Goncharenko, I.; Eremets, M.I.; Hanfland, M.; Tse, J.S.; Amboage, M.; Yao, Y.; Trojan, I.A. Pressure-induced hydrogen-dominant metallic state in aluminum hydride. Phys. Rev. Lett. 2008, 100, 045504. [Google Scholar] [CrossRef]
- Feng, J.; Grochala, W.; Jaroń, T.; Hoffmann, R.; Bergara, A.; Ashcroft, N.W. Structures and potential superconductivity in SiH4 at high pressure: En route to “metallic hydrogen”. Phys. Rev. Lett. 2006, 96, 017006. [Google Scholar] [CrossRef]
- Eremets, M.I.; Trojan, I.A.; Medvedev, S.A.; Tse, J.S.; Yao, Y. Superconductivity in hydrogen dominant materials: Silane. Science 2008, 319, 1506–1509. [Google Scholar] [CrossRef]
- Li, Y.; Hao, J.; Liu, H.; Li, Y.; Ma, Y. The metallization and superconductivity of dense hydrogen sulfide. J. Chem. Phys. 2014, 140, 174712. [Google Scholar] [CrossRef]
- Duan, D.; Liu, Y.; Tian, F.; Li, D.; Huang, X.; Zhao, Z.; Yu, H.; Liu, B.; Tian, W.; Cui, T. Pressure-induced metallization of dense (H2S)2H2 with high-Tc superconductivity. Sci. Rep. 2014, 4, 6968. [Google Scholar] [CrossRef]
- Xu, Y.; Tse, J.S.; Oganov, A.R.; Cui, T.; Wang, H.; Ma, Y.; Zou, G. Superconducting high-pressure phase of cesium iodide. Phys. Rev. B 2009, 79, 144110. [Google Scholar] [CrossRef]
- Eremets, M.I.; Shimizu, K.; Kobayashi, T.C.; Amaya, K. Metallic CsI at pressures of up to 220 gigapascals. Science 1998, 281, 1333–1335. [Google Scholar] [CrossRef] [PubMed]
- Knittle, E.; Jeanloz, R. Structural and bonding changes in cesium iodide at high pressures. Science 1984, 223, 53–56. [Google Scholar] [CrossRef] [PubMed]
- Makarenko, I.N.; Goncharov, A.F.; Stishov, S.M. Optical absorption of cesium iodide (CsI) at pressure up to 60 GPa. Phys. Rev. B 1984, 29, 6018–6019. [Google Scholar] [CrossRef]
- Bardeen, J.; Cooper, L.N.; Schrieffer, J.R. Theory of superconductivity. Phys. Rev. 1957, 108, 1175–1204. [Google Scholar] [CrossRef]
- Bloch, F. Zum elektrischen Widerstandsgesetz bei tiefen Temperaturen. Z. Phys. 1930, 59, 208–214. [Google Scholar] [CrossRef]
- Grüneisen, E. Die abhängigkeit des elektrischen widerstandes reiner metalle von der temperatur. Ann. Phys. 1933, 408, 530–540. [Google Scholar] [CrossRef]
- Matsumoto, R.; Song, P.; Adachi, S.; Saito, Y.; Hara, H.; Yamashita, A.; Nakamura, K.; Yamamoto, S.; Tanaka, H.; Irifune, T. Pressure-induced superconductivity in tin sulfide. Phys. Rev. B 2019, 99, 184502. [Google Scholar] [CrossRef]
- Kudo, K.; Hiiragi, H.; Honda, T.; Fujimura, K.; Idei, H.; Nohara, M. Superconductivity in Mg2Ir3Si: A fully ordered Laves phase. J. Phys. Soc. Jpn. 2020, 89, 013701. [Google Scholar] [CrossRef]
- Susner, M.A.; Bhatia, M.; Sumption, M.D.; Collings, E.W. Electrical resistivity, Debye temperature, and connectivity in heavily doped bulk MgB2 superconductors. J. Appl. Phys. 2009, 105, 195901. [Google Scholar] [CrossRef]
- Pyon, S.; Kudo, K.; Matsumura, J.-I.; Ishii, H.; Matsuo, G.; Nohara, M.; Hojo, H.; Oka, K.; Azuma, M.; Garlea, V.O.; et al. Superconductivity in noncentrosymmetric iridium silicide Li2IrSi3. J. Phys. Soc. Jpn. 2014, 83, 093706. [Google Scholar] [CrossRef]
- Talantsev, E.F. Advanced McMillan’s equation and its application for the analysis of highly-compressed superconductors. Supercond. Sci. Technol. 2020, 33, 094009. [Google Scholar] [CrossRef]
- Talantsev, E.F. An approach to identifying unconventional superconductivity in highly compressed superconductors. Supercond. Sci. Technol. 2020, 33, 124001. [Google Scholar] [CrossRef]
- Talantsev, E.F.; Stolze, K. Resistive transition of hydrogen-rich superconductors. Supercond. Sci. Technol. 2021, 34, 064001. [Google Scholar] [CrossRef]
- Talantsev, E.F. Comparison of highly-compressed C2/m-SnH12 superhydride with conventional superconductors. J. Phys. Condens. Matter 2021, 33, 285601. [Google Scholar] [CrossRef]
- Talantsev, E.F. The electron–phonon coupling constant and the Debye temperature in polyhydrides of thorium, hexadeuteride of yttrium, and metallic hydrogen phase III. J. Appl. Phys. 2021, 130, 195901. [Google Scholar] [CrossRef]
- Talantsev, E.F. Electron–phonon coupling constant and BCS ratios in LaH10−y doped with magnetic rare-earth element. Supercond. Sci. Technol. 2022, 35, 095008. [Google Scholar] [CrossRef]
- Carbotte, J.P. Properties of boson-exchange superconductors. Rev. Mod. Phys. 1990, 62, 1027–1157. [Google Scholar] [CrossRef]
- McMillan, W.L. Transition temperature of strong-coupled superconductors. Phys. Rev. 1968, 167, 331–344. [Google Scholar] [CrossRef]
- Poole, C.P., Jr. Properties of the normal metallic state. In Handbook of Superconductivity, 1st ed.; Poole, C.P., Jr., Ed.; Academic Press: New York, NY, USA, 1999; Volume 1, pp. 31–32. [Google Scholar]
- Allen, P.B.; Dynes, R.C. Transition temperature of strong-coupled superconductors reanalyzed. Phys. Rev. B 1975, 12, 905–922. [Google Scholar] [CrossRef]
- Jiang, H.; Bao, J.-K.; Zhai, H.-F.; Tang, Z.-T.; Sun, Y.-L.; Liu, Y.; Wang, Z.-C.; Bai, H.; Xu, Z.-A.; Cao, G.-H. Physical properties and electronic structure of Sr2Cr3As2O2 containing CrO2 and Cr2As2 square-planar lattices. Phys. Rev. B 2015, 92, 205107. [Google Scholar] [CrossRef]
- Zhang, C.; Wang, Y.; Wang, D.; Zhang, Y.; Liu, Z.-H.; Feng, Q.-R.; Gan, Z.-Z. Suppression of superconductivity in epitaxial MgB2 ultrathin films. J. Appl. Phys. 2013, 114, 023903. [Google Scholar] [CrossRef]
- Talantsev, E.F. Quantifying the charge carrier interaction in metallic twisted graphene superlattices. Nanomaterials 2021, 11, 1306. [Google Scholar] [CrossRef] [PubMed]
- Talantsev, E.F. The dominance of non-electron–phonon charge carrier interaction in highly-compressed superhydrides. Supercond. Sci. Technol. 2021, 34, 115001. [Google Scholar] [CrossRef]
- Talantsev, E.F. Classifying charge carrier interaction in highly compressed elements and silane. Materials 2021, 14, 4322. [Google Scholar] [CrossRef]
- White, G.K.; Woods, S.B. Electrical and thermal resistivity of the transition elements at low temperatures. Phil. Trans. R. Soc. Lond. A 1959, 251, 273–302. [Google Scholar] [CrossRef]
- Poker, D.B.; Klabunde, C.E. Temperature dependence of electrical resistivity of vanadium, platinum, and copper. Phys. Rev. B 1982, 26, 7012–7014. [Google Scholar] [CrossRef]
- Matula, R.A. Electrical resistivity of copper, gold, palladium, and silver. J. Phys. Chem. Ref. Data 1979, 8, 1147–1298. [Google Scholar] [CrossRef]
- Shang, T.; Amon, A.; Kasinathan, D.; Xie, W.; Bobnar, M.; Chen, Y.; Wang, A.; Shi, M.; Medarde, M.; Yuan, H.Q.; et al. Enhanced Tc and multiband superconductivity in the fully-gapped ReBe22 superconductor. New J. Phys. 2019, 21, 073034. [Google Scholar] [CrossRef]
- Stolze, K.; Tao, J.; von Rohr, F.O.; Kong, T.; Cava, R.J. Sc–Zr–Nb–Rh–Pd and Sc–Zr–Nb–Ta–Rh–Pd high-entropy alloy superconductors on a CsCl-type lattice. Chem. Mater. 2018, 30, 906–914. [Google Scholar] [CrossRef]
- Uemura, Y.J.; Luke, G.M.; Sternlieb, B.J.; Brewer, J.H.; Carolan, J.F.; Hardy, W.N.; Kadono, R.; Kempton, J.R.; Kiefl, R.F.; Kreitzman, S.R. Universal correlations between Tc and (carrier density over effective mass) in high-Tc cuprate. Phys. Rev. Lett. 1989, 62, 2317–2320. [Google Scholar] [CrossRef] [PubMed]
- Uemura, Y.J. Condensation, excitation, pairing, and superfluid density in high-Tc superconductors: The magnetic resonance mode as a roton analogue and a possible spin-mediated pairing. J. Phys. Condens. Matter 2004, 16, S4515–S4540. [Google Scholar] [CrossRef]
- Talantsev, E.F. Classifying superconductivity in compressed H3S. Mod. Phys. Lett. B 2019, 33, 1950195. [Google Scholar] [CrossRef]
- Talantsev, E.F.; Mataira, R.C. Classifying superconductivity in ThH-ThD superhydrides/superdeuterides. Mater. Res. Express 2020, 7, 016003. [Google Scholar] [CrossRef]
- Helfand, E.; Werthamer, N.R. Temperature and purity dependence of the superconducting critical field, Hc2. II. Phys. Rev. 1966, 147, 288–294. [Google Scholar] [CrossRef]
- Werthamer, N.R.; Helfand, E.; Hohenberg, P.C. Temperature and purity dependence of the superconducting critical field, Hc2. III. Electron spin and spin-orbit effects. Phys. Rev. 1966, 147, 295–302. [Google Scholar] [CrossRef]
- Orlando, T.P.; McNiff, E.J., Jr.; Foner, S.; Beasley, M.R. Critical fields, Pauli paramagnetic limiting, and material parameters of Nb3Sn and V3Si. Phys. Rev. B 1979, 19, 4545–4561. [Google Scholar] [CrossRef]
- Cao, Y.; Fatemi, V.; Fang, S.; Watanabe, K.; Taniguchi, T.; Kaxiras, E.; Jarillo-Herrero, P. Unconventional superconductivity in magic-angle graphene superlattices. Nat. Cell Biol. 2018, 556, 43–50. [Google Scholar] [CrossRef]
- Talantsev, E.F.; Mataira, R.C.; Crump, W.P. Classifying superconductivity in Moiré graphene superlattices. Sci. Rep. 2020, 10, 212. [Google Scholar] [CrossRef]
- Suzuki, Y.; Wakamatsu, K.; Ibuka, J.; Oike, H.; Fujii, T.; Miyagawa, K.; Taniguchi, H.; Kanoda, K. Mott-driven BEC-BCS crossover in a doped spin liquid candidate κ-(BEDT-TTF)4Hg2.89Br8. Phys. Rev. X 2022, 12, 011016. [Google Scholar] [CrossRef]
- Migdal, A.B. Interaction between electrons and lattice vibrations in a normal metal. Sov. Phys. JETP 1958, 7, 996–1001. [Google Scholar]
- Born, M.; Oppenheimer, J.R. Zur Quantentheorie der Molekeln [On the Quantum Theory of Molecules]. Ann. Der Phys. 1927, 389, 457–484. [Google Scholar] [CrossRef]
- Pietronero, L.; Strässler, S.; Grimaldi, C. Nonadiabatic superconductivity. I. Vertex corrections for the electron-phonon interactions. Phys. Rev. B 1995, 52, 10516–10529. [Google Scholar] [CrossRef] [PubMed]
- Grimaldi, C.; Pietronero, L.; Strässler, S. Nonadiabatic superconductivity. II. Generalized Eliashberg equations beyond Migdal’s theorem. Phys. Rev. B 1995, 52, 10530–10546. [Google Scholar] [CrossRef]
- Grimaldi, C.; Cappelluti, E.; Pietronero, L. Isotope effect on m* in high Tc materials due to the breakdown of Migdal’s theorem. Europhys. Lett. 1998, 42, 667–672. [Google Scholar] [CrossRef]
- Cappelluti, E.; Ciuchi, S.; Grimaldi, C.; Pietronero, L.; Strässler, S. High Tc superconductivity in MgB2 by nonadiabatic pairing. Phys. Rev. Lett. 2002, 88, 117003. [Google Scholar] [CrossRef] [PubMed]
- Pietronero, L.; Boeri, L.; Cappelluti, E.; Ortenzi, L. Conventional/unconventional superconductivity in high-pressure hydrides and beyond: Insights from theory and perspectives. Quantum Stud. Math. Found. 2018, 5, 5–21. [Google Scholar] [CrossRef]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Talantsev, E.F. Fermi-Liquid Nonadiabatic Highly Compressed Cesium Iodide Superconductor. Condens. Matter 2022, 7, 65. https://doi.org/10.3390/condmat7040065
Talantsev EF. Fermi-Liquid Nonadiabatic Highly Compressed Cesium Iodide Superconductor. Condensed Matter. 2022; 7(4):65. https://doi.org/10.3390/condmat7040065
Chicago/Turabian StyleTalantsev, Evgueni F. 2022. "Fermi-Liquid Nonadiabatic Highly Compressed Cesium Iodide Superconductor" Condensed Matter 7, no. 4: 65. https://doi.org/10.3390/condmat7040065
APA StyleTalantsev, E. F. (2022). Fermi-Liquid Nonadiabatic Highly Compressed Cesium Iodide Superconductor. Condensed Matter, 7(4), 65. https://doi.org/10.3390/condmat7040065






