Toward an Automated-Algebra Framework for High Orders in the Virial Expansion of Quantum Matter
Abstract
:1. Introduction
2. Basic Formalism
3. Calculation Methods for the Virial Expansion
3.1. Second Order
3.2. Third Order and Beyond
4. Homogeneous Fermi Gases with a Zero-Range Interaction
4.1. Factorizing the Transfer Matrix
4.2. From Transfer Matrices to Canonical Partition Functions
4.3. Computational Details of Automated Algebra
- Term generation: Expand the product symbolically, which will yield a large number of terms as is increased.
- Delta crunch: Contract indices to saturate all Kronecker deltas, thus simplifying each term into a product of Gaussian functions, namely the propagator , by integrating out a subset of variables. This is the most computationally expensive step.
- Gaussian integration: For each term, take the summation over the rest of the variables and take the continuum limit, ultimately turning each term into a multidimensional Gaussian integral whose results are analytically available as the well-known formulawhere n is the dimension of vector .
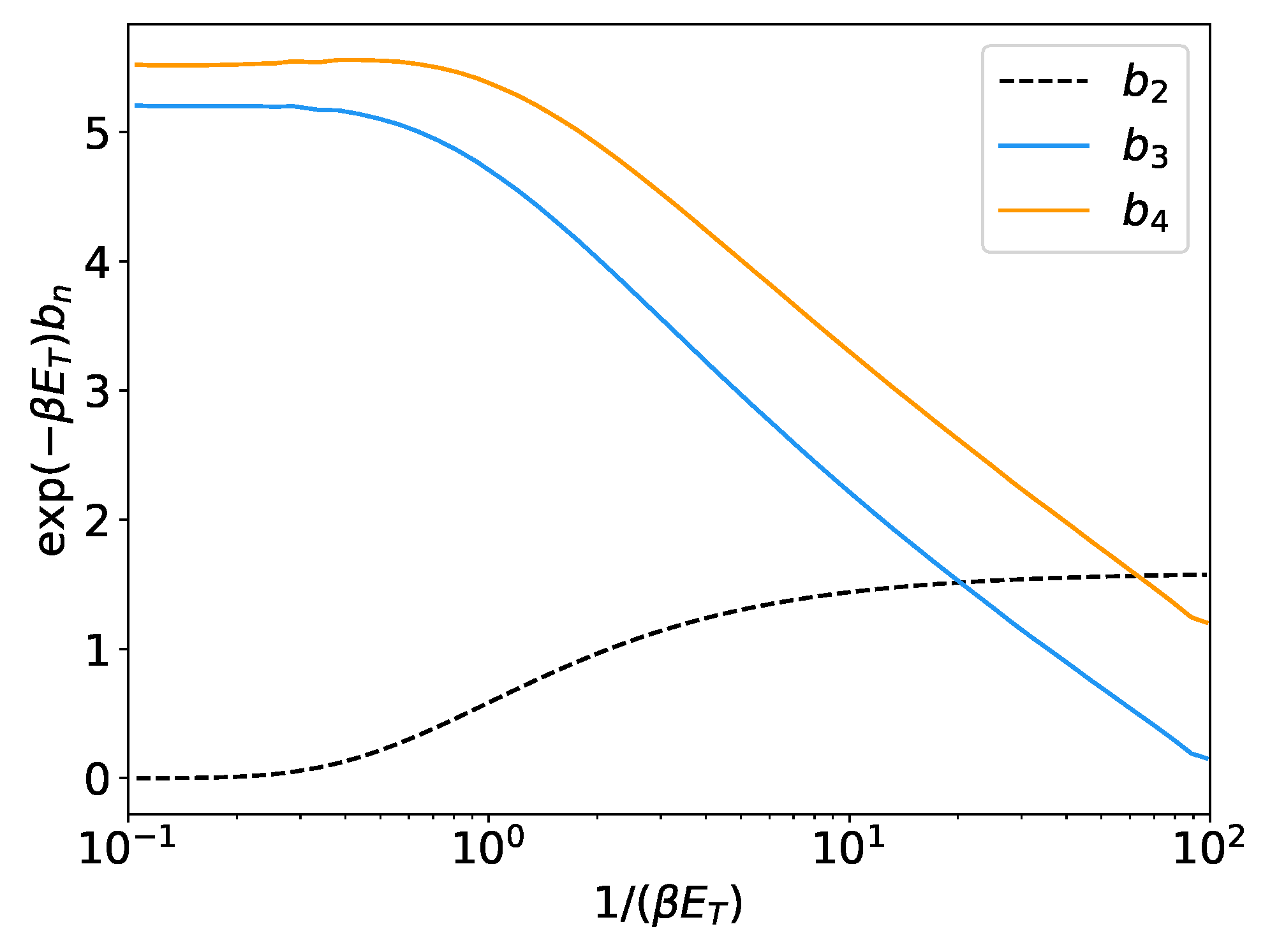
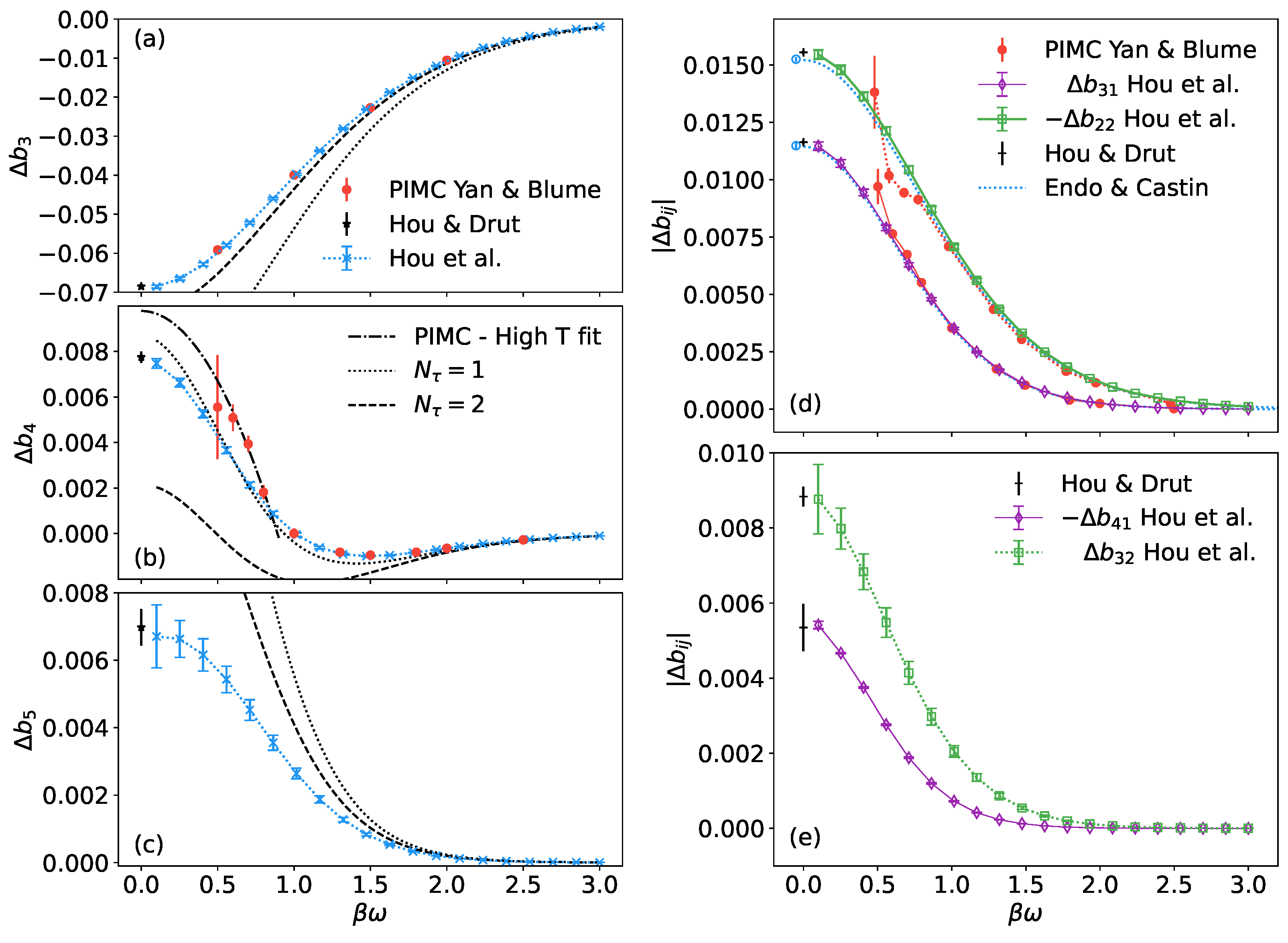
4.4. Selected Results
5. Generalization to Other Systems
5.1. Harmonic Traps
5.2. Neutron Matter
5.3. Unitary Bose Gas
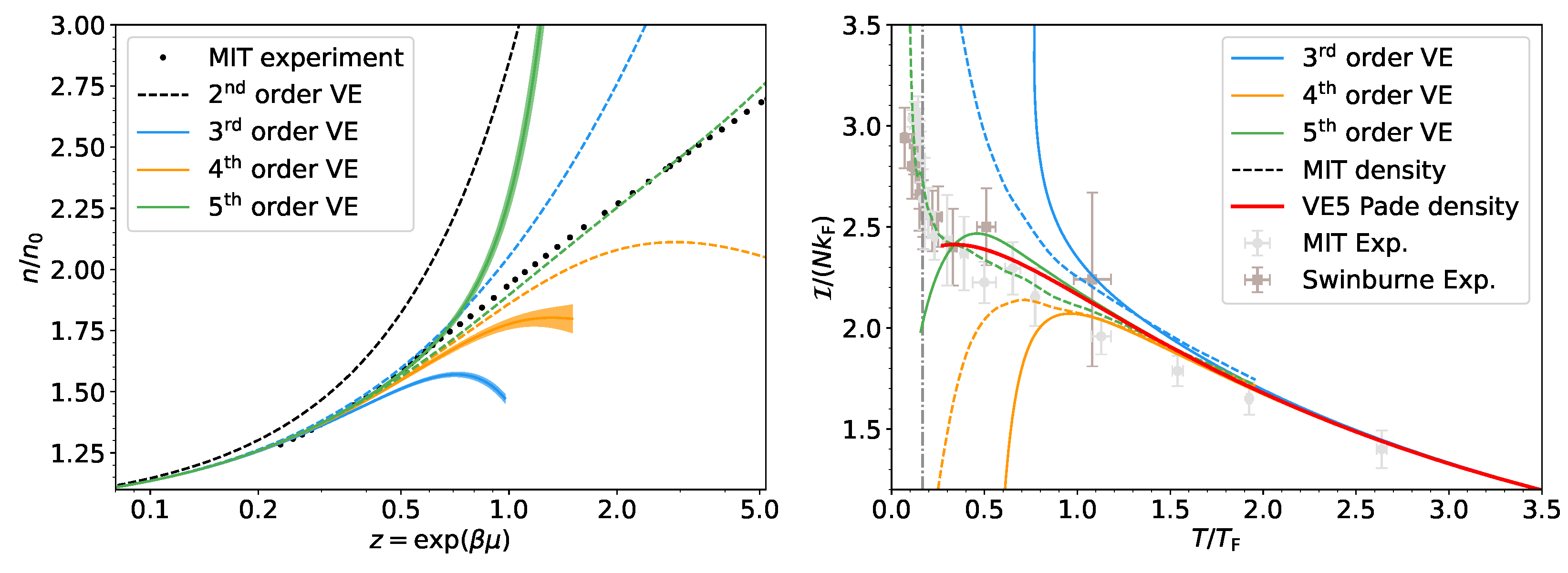
6. Pressure, Density, and Generalization to Other Observables
6.1. From Pressure to Density and Tan Contact
6.2. One-Body Operator Example: The Momentum Distribution
6.3. Two-Body Operators and Real-Time Evolution
7. Summary and Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Berger, C.; Rammelmueller, L.; Loheac, A.; Ehmann, F.; Braun, J.; Drut, J. Complex Langevin and other approaches to the sign problem in quantum many-body physics. Phys. Rep. 2021, 892, 1–54. [Google Scholar] [CrossRef]
- Pathria, R. Statistical Mechanics; International Series of Monographs in Natural Philosophy; Elsevier Science & Technology Books: Amsterdam, The Netherlands, 1972. [Google Scholar]
- Liu, X.J. Virial expansion for a strongly correlated Fermi system and its application to ultracold atomic Fermi gases. Phys. Rep. 2013, 524, 37–83. [Google Scholar] [CrossRef] [Green Version]
- Mayer, J.E.; Mayer, M.G. Statistical Mechanics; John Wiley and Sons, Inc.: New York, NY, USA, 1940. [Google Scholar]
- Kahn, B.; Uhlenbeck, G.E. On the theory of condensation. Physica 1938, 5, 399. [Google Scholar] [CrossRef]
- Lee, T.D.; Yang, C.N. Many-Body Problem in Quantum Statistical Mechanics. I. General Formulation. Phys. Rev. 1959, 113, 1165. [Google Scholar] [CrossRef]
- Lee, T.D.; Yang, C.N. Many-Body Problem in Quantum Statistical Mechanics. II. Virial Expansion for Hard-Sphere Gas. Phys. Rev. 1959, 116, 25. [Google Scholar] [CrossRef]
- Lee, T.D.; Yang, C.N. Many-Body Problem in Quantum Statistical Mechanics. III. Zero-Temperature Limit for Dilute Hard Spheres. Phys. Rev. 1960, 117, 12. [Google Scholar] [CrossRef]
- Lee, T.D.; Yang, C.N. Many-Body Problem in Quantum Statistical Mechanics. IV. Formulation in Terms of Average Occupation Number in Momentum Space. Phys. Rev. 1960, 117, 22. [Google Scholar] [CrossRef]
- Lee, T.D.; Yang, C.N. Many-Body Problem in Quantum Statistical Mechanics. V. Degenerate Phase in Bose-Einstein Condensation. Phys. Rev. 1960, 117, 897. [Google Scholar] [CrossRef]
- Tan, S. Energetics of a strongly correlated Fermi gas. Ann. Phys. 2008, 323, 2952–2970. [Google Scholar] [CrossRef] [Green Version]
- Tan, S. Large momentum part of a strongly correlated Fermi gas. Ann. Phys. 2008, 323, 2971–2986. [Google Scholar] [CrossRef] [Green Version]
- Tan, S. Generalized virial theorem and pressure relation for a strongly correlated Fermi gas. Ann. Phys. 2008, 323, 2987–2990. [Google Scholar] [CrossRef] [Green Version]
- Shill, C.R.; Drut, J.E. Virial coefficients of one-dimensional and two-dimensional Fermi gases by stochastic methods and a semiclassical lattice approximation. Phys. Rev. A 2018, 98, 053615. [Google Scholar] [CrossRef] [Green Version]
- Morrell, K.J.; Berger, C.E.; Drut, J.E. Third- and fourth-order virial coefficients of harmonically trapped fermions in a semiclassical approximation. Phys. Rev. A 2019, 100, 063626. [Google Scholar] [CrossRef] [Green Version]
- Hou, Y.; Czejdo, A.J.; DeChant, J.; Shill, C.R.; Drut, J.E. Leading- and next-to-leading-order semiclassical approximation to the first seven virial coefficients of spin-1/2 fermions across spatial dimensions. Phys. Rev. A 2019, 100, 063627. [Google Scholar] [CrossRef] [Green Version]
- Czejdo, A.J.; Drut, J.E.; Hou, Y.; McKenney, J.R.; Morrell, K.J. Virial coefficients of trapped and untrapped three-component fermions with three-body forces in arbitrary spatial dimensions. Phys. Rev. A 2020, 101, 063630. [Google Scholar] [CrossRef]
- Hou, Y.; Drut, J.E. Fourth- and Fifth-Order Virial Coefficients from Weak Coupling to Unitarity. Phys. Rev. Lett. 2020, 125, 050403. [Google Scholar] [CrossRef]
- Hou, Y.; Drut, J.E. Virial expansion of attractively interacting Fermi gases in one, two, and three dimensions, up to fifth order. Phys. Rev. A 2020, 102, 033319. [Google Scholar] [CrossRef]
- Hou, Y.; Morrell, K.J.; Czejdo, A.J.; Drut, J.E. Fourth- and fifth-order virial expansion of harmonically trapped fermions at unitarity. Phys. Rev. Res. 2021, 3, 033099. [Google Scholar] [CrossRef]
- Rammelmueller, L.; Hou, Y.; Drut, J.E.; Braun, J. Pairing and the spin susceptibility of the polarized unitary Fermi gas in the normal phase. Phys. Rev. A 2021, 103, 043330. [Google Scholar] [CrossRef]
- Uhlenbeck, G.E.; Beth, E. The quantum theory of the non-ideal gas I. Deviations from the classical theory. Physica 1936, 3, 729–745. [Google Scholar] [CrossRef]
- Beth, E.; Uhlenbeck, G.E. The quantum theory of the non-ideal gas. II. Behaviour at low temperatures. Physica 1937, 4, 915–924. [Google Scholar] [CrossRef]
- Hoffman, M.D.; Javernick, P.D.; Loheac, A.C.; Porter, W.J.; Anderson, E.R.; Drut, J.E. Universality in one-dimensional fermions at finite temperature: Density, pressure, compressibility, and contact. Phys. Rev. A 2015, 91, 033618. [Google Scholar] [CrossRef] [Green Version]
- Loheac, A.C.; Drut, J.E. Third-order perturbative lattice and complex Langevin analyses of the finite-temperature equation of state of nonrelativistic fermions in one dimension. Phys. Rev. D 2017, 95, 094502. [Google Scholar] [CrossRef] [Green Version]
- Chafin, C.; Schäfer, T. Scale breaking and fluid dynamics in a dilute two-dimensional Fermi gas. Phys. Rev. A 2013, 88, 043636. [Google Scholar] [CrossRef] [Green Version]
- Daza, W.; Drut, J.E.; Lin, C.; Ordóñez, C. Virial expansion for the Tan contact and Beth-Uhlenbeck formula from two-dimensional SO(2,1) anomalies. Phys. Rev. A 2018, 97, 033630. [Google Scholar] [CrossRef] [Green Version]
- Lee, D.; Schäfer, T. Cold dilute neutron matter on the lattice. I. Lattice virial coefficients and large scattering lengths. Phys. Rev. C 2006, 73, 015201. [Google Scholar] [CrossRef] [Green Version]
- Horowitz, C.; Schwenk, A. The virial equation of state of low-density neutron matter. Phys. Lett. B 2006, 638, 153–159. [Google Scholar] [CrossRef] [Green Version]
- Horowitz, C.; Schwenk, A. Cluster formation and the virial equation of state of low-density nuclear matter. Nucl. Phys. A 2006, 776, 55–79. [Google Scholar] [CrossRef] [Green Version]
- Stoks, V.G.J.; Klomp, R.A.M.; Rentmeester, M.C.M.; de Swart, J.J. Partial-wave analysis of all nucleon-nucleon scattering data below 350 MeV. Phys. Rev. C 1993, 48, 792–815. [Google Scholar] [CrossRef]
- Camblong, H.E.; Chakraborty, A.; Daza, W.S.; Drut, J.E.; Lin, C.L.; Ordóñez, C.R. Spectral density, Levinson’s theorem, and the extra term in the second virial coefficient for the one-dimensional δ-function potential. Phys. Rev. A 2019, 100, 062110. [Google Scholar] [CrossRef] [Green Version]
- Leyronas, X. Virial expansion with Feynman diagrams. Phys. Rev. A 2011, 84, 053633. [Google Scholar] [CrossRef] [Green Version]
- Kaplan, D.B.; Sun, S. New Field-Theoretic Method for the Virial Expansion. Phys. Rev. Lett. 2011, 107, 030601. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Rakshit, D.; Daily, K.M.; Blume, D. Natural and unnatural parity states of small trapped equal-mass two-component Fermi gases at unitarity and fourth-order virial coefficient. Phys. Rev. A 2012, 85, 033634. [Google Scholar] [CrossRef] [Green Version]
- Endo, S.; Castin, Y. The interaction-sensitive states of a trapped two-component ideal Fermi gas and application to the virial expansion of the unitary Fermi gas. J. Phys. A Math. Theor. 2016, 49, 265301. [Google Scholar] [CrossRef] [Green Version]
- Endo, S.; Castin, Y. Unitary boson-boson and boson-fermion mixtures: Third virial coefficient and three-body parameter on a narrow Feshbach resonance. Eur. Phys. J. D 2016, 70, 238. [Google Scholar] [CrossRef] [Green Version]
- Gao, C.; Endo, S.; Castin, Y. The third virial coefficient of a two-component unitary Fermi gas across an Efimov-effect threshold. EPL Europhys. Lett. 2015, 109, 16003. [Google Scholar] [CrossRef] [Green Version]
- Ngampruetikorn, V.; Parish, M.M.; Levinsen, J. High-temperature limit of the resonant Fermi gas. Phys. Rev. A 2015, 91, 013606. [Google Scholar] [CrossRef] [Green Version]
- Bedaque, P.F.; Rupak, G. Dilute resonating gases and the third virial coefficient. Phys. Rev. B 2003, 67, 174513. [Google Scholar] [CrossRef] [Green Version]
- Armstrong, J.R.; Zinner, N.T.; Fedorov, D.V.; Jensen, A.S. Virial expansion coefficients in the harmonic approximation. Phys. Rev. E 2012, 86, 021115. [Google Scholar] [CrossRef] [Green Version]
- Sakumichi, N.; Nishida, Y.; Ueda, M. Lee-Yang cluster expansion approach to the BCS-BEC crossover: BCS and BEC limits. Phys. Rev. A 2014, 89, 033622. [Google Scholar] [CrossRef] [Green Version]
- Liu, X.J.; Hu, H.; Drummond, P.D. Virial Expansion for a Strongly Correlated Fermi Gas. Phys. Rev. Lett. 2009, 102, 160401. [Google Scholar] [CrossRef] [PubMed]
- Ngampruetikorn, V.; Levinsen, J.; Parish, M.M. Pair Correlations in the Two-Dimensional Fermi Gas. Phys. Rev. Lett. 2013, 111, 265301. [Google Scholar] [CrossRef] [PubMed]
- Werner, F.; Castin, Y. Unitary Quantum Three-Body Problem in a Harmonic Trap. Phys. Rev. Lett. 2006, 97, 150401. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Yan, Y.; Blume, D. Incorporating exact two-body propagators for zero-range interactions into N-body Monte Carlo simulations. Phys. Rev. A 2015, 91, 043607. [Google Scholar] [CrossRef] [Green Version]
- Yan, Y.; Blume, D. Path-Integral Monte Carlo Determination of the Fourth-Order Virial Coefficient for a Unitary Two-Component Fermi Gas with Zero-Range Interactions. Phys. Rev. Lett. 2016, 116, 230401. [Google Scholar] [CrossRef] [PubMed]
- Pordes, R.; Petravick, D.; Kramer, B.; Olson, D.; Livny, M.; Roy, A.; Avery, P.; Blackburn, K.; Wenaus, T.; Würthwein, F.; et al. The open science grid. J. Phys. Conf. Ser. 2007, 78, 012057. [Google Scholar] [CrossRef]
- Sfiligoi, I.; Bradley, D.C.; Holzman, B.; Mhashilkar, P.; Padhi, S.; Wurthwein, F. The pilot way to grid resources using glideinWMS. In Proceedings of the 2009 WRI World Congress on Computer Science and Information Engineering, Los Angeles, CA, USA, 31 March–2 April 2009; Volume 2, pp. 428–432. [Google Scholar] [CrossRef] [Green Version]
- Ruskey, F.; Savage, C.; Wang, T.M.Y. Generating necklaces. J. Algorithms 1992, 13, 414–430. [Google Scholar] [CrossRef]
- Nascimbène, S.; Navon, N.; Jiang, K.J.; Chevy, F.; Salomon, C. Exploring the thermodynamics of a universal Fermi gas. Nature 2010, 463, 1057–1060. [Google Scholar] [CrossRef] [Green Version]
- Ku, M.J.H.; Sommer, A.T.; Cheuk, L.W.; Zwierlein, M.W. Revealing the Superfluid Lambda Transition in the Universal Thermodynamics of a Unitary Fermi Gas. Science 2012, 335, 563–567. [Google Scholar] [CrossRef] [Green Version]
- Liu, X.J.; Hu, H.; Drummond, P.D. Three attractively interacting fermions in a harmonic trap: Exact solution, ferromagnetism, and high-temperature thermodynamics. Phys. Rev. A 2010, 82, 023619. [Google Scholar] [CrossRef] [Green Version]
- Hu, H.; Liu, X.J.; Drummond, P.D. Universal contact of strongly interacting fermions at finite temperatures. New J. Phys. 2011, 13, 035007. [Google Scholar] [CrossRef]
- Thomas, L.H. The Interaction Between a Neutron and a Proton and the Structure of H3. Phys. Rev. 1935, 47, 903–909. [Google Scholar] [CrossRef]
- Efimov, V.N. Weakly-bound states of 3 resonantly interacting particles. Sov. J. Nucl. Phys. 1971, 12, 589. [Google Scholar]
- Efimov, V.N. Effective interaction of three resonantly interacting particles and the force range. Phys. Rev. C 1993, 47, 1876. [Google Scholar] [CrossRef]
- Bedaque, P.F.; Hammer, H.W.; van Kolck, U. Renormalization of the Three-Body System with Short-Range Interactions. Phys. Rev. Lett. 1999, 82, 463–467. [Google Scholar] [CrossRef] [Green Version]
- Castin, Y.; Werner, F. Le troisième coefficient du viriel du gaz de Bose unitaire. Can. J. Phys. 2013, 91, 382–389. [Google Scholar] [CrossRef]
- Barth, M.; Zwerger, W. Tan relations in one dimension. Ann. Phys. 2011, 326, 2544–2565. [Google Scholar] [CrossRef] [Green Version]
- Valiente, M.; Zinner, N.T.; Mølmer, K. Universal relations for the two-dimensional spin-1/2 Fermi gas with contact interactions. Phys. Rev. A 2011, 84, 063626. [Google Scholar] [CrossRef] [Green Version]
- Wild, R.J.; Makotyn, P.; Pino, J.M.; Cornell, E.A.; Jin, D.S. Measurements of Tan’s Contact in an Atomic Bose-Einstein Condensate. Phys. Rev. Lett. 2012, 108, 145305. [Google Scholar] [CrossRef] [Green Version]
- Lang, G.; Vignolo, P.; Minguzzi, A. Tan’s contact of a harmonically trapped one-dimensional Bose gas: Strong-coupling expansion and conjectural approach at arbitrary interactions. Eur. Phys. J. Spec. Top. 2017, 226, 1583–1591. [Google Scholar] [CrossRef]
- Zou, Y.Q.; Bakkali-Hassani, B.; Maury, C.; Le Cerf, É.; Nascimbene, S.; Dalibard, J.; Beugnon, J. Tan’s two-body contact across the superfluid transition of a planar Bose gas. Nat. Commun. 2021, 12, 1–6. [Google Scholar] [CrossRef] [PubMed]
- Mukherjee, B.; Patel, P.B.; Yan, Z.; Fletcher, R.J.; Struck, J.; Zwierlein, M.W. Spectral Response and Contact of the Unitary Fermi Gas. Phys. Rev. Lett. 2019, 122, 203402. [Google Scholar] [CrossRef] [Green Version]
- Kuhnle, E.; Dyke, P.; Hoinka, S.; Mark, M.; Hu, H.; Liu, X.J.; Drummond, P.; Hannaford, P.; Vale, C. Universal structure of a strongly interacting Fermi gas. J. Phys. Conf. Ser. 2011, 264, 012013. [Google Scholar] [CrossRef]
- Kuhnle, E.; Hoinka, S.; Hu, H.; Dyke, P.; Hannaford, P.; Vale, C. Studies of the universal contact in a strongly interacting Fermi gas using Bragg spectroscopy. New J. Phys. 2011, 13, 055010. [Google Scholar] [CrossRef]
- Yan, Z.; Patel, P.B.; Mukherjee, B.; Fletcher, R.J.; Struck, J.; Zwierlein, M.W. Boiling a Unitary Fermi Liquid. Phys. Rev. Lett. 2019, 122, 093401. [Google Scholar] [CrossRef] [Green Version]
- Carcy, C.; Hoinka, S.; Lingham, M.G.; Dyke, P.; Kuhn, C.C.N.; Hu, H.; Vale, C.J. Contact and Sum Rules in a Near-Uniform Fermi Gas at Unitarity. Phys. Rev. Lett. 2019, 122, 203401. [Google Scholar] [CrossRef] [Green Version]
- Horowitz, C.; Schwenk, A. The neutrino response of low-density neutron matter from the virial expansion. Phys. Lett. B 2006, 642, 326–332. [Google Scholar] [CrossRef] [Green Version]
- Alexandru, A.; Bedaque, P.; Berkowitz, E.; Warrington, N.C. Structure Factors of Neutron Matter at Finite Temperature. Phys. Rev. Lett. 2020, 126, 132701. [Google Scholar] [CrossRef]
- Alexandru, A.; Bedaque, P.F.; Warrington, N.C. Structure factors of the unitary gas under supernova conditions. Phys. Rev. C 2020, 101. [Google Scholar] [CrossRef] [Green Version]
- Enss, T. Bulk Viscosity and Contact Correlations in Attractive Fermi Gases. Phys. Rev. Lett. 2019, 123, 205301. [Google Scholar] [CrossRef] [Green Version]
- Nishida, Y. Viscosity spectral functions of resonating fermions in the quantum virial expansion. Ann. Phys. 2019, 410, 167949. [Google Scholar] [CrossRef] [Green Version]
- Hofmann, J. High-temperature expansion of the viscosity in interacting quantum gases. Phys. Rev. A 2020, 101, 013620. [Google Scholar] [CrossRef] [Green Version]
- Sun, M.; Zhang, P.; Zhai, H. High Temperature Virial Expansion to Universal Quench Dynamics. Phys. Rev. Lett. 2020, 125, 110404. [Google Scholar] [CrossRef] [PubMed]
- Eigen, C.; Glidden, J.A.P.; Lopes, R.; Cornell, E.A.; Smith, R.P.; Hadzibabic, Z. Universal prethermal dynamics of Bose gases quenched to unitarity. Nature 2018, 563, 221–224. [Google Scholar] [CrossRef] [Green Version]

Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Czejdo, A.J.; Drut, J.E.; Hou, Y.; Morrell, K.J. Toward an Automated-Algebra Framework for High Orders in the Virial Expansion of Quantum Matter. Condens. Matter 2022, 7, 13. https://doi.org/10.3390/condmat7010013
Czejdo AJ, Drut JE, Hou Y, Morrell KJ. Toward an Automated-Algebra Framework for High Orders in the Virial Expansion of Quantum Matter. Condensed Matter. 2022; 7(1):13. https://doi.org/10.3390/condmat7010013
Chicago/Turabian StyleCzejdo, Aleks J., Joaquin E. Drut, Yaqi Hou, and Kaitlyn J. Morrell. 2022. "Toward an Automated-Algebra Framework for High Orders in the Virial Expansion of Quantum Matter" Condensed Matter 7, no. 1: 13. https://doi.org/10.3390/condmat7010013
APA StyleCzejdo, A. J., Drut, J. E., Hou, Y., & Morrell, K. J. (2022). Toward an Automated-Algebra Framework for High Orders in the Virial Expansion of Quantum Matter. Condensed Matter, 7(1), 13. https://doi.org/10.3390/condmat7010013