Density Collective Modes of Exciton Superfluidity in Bilayer Systems
Abstract
1. Introduction
2. Materials and Methods
3. Results
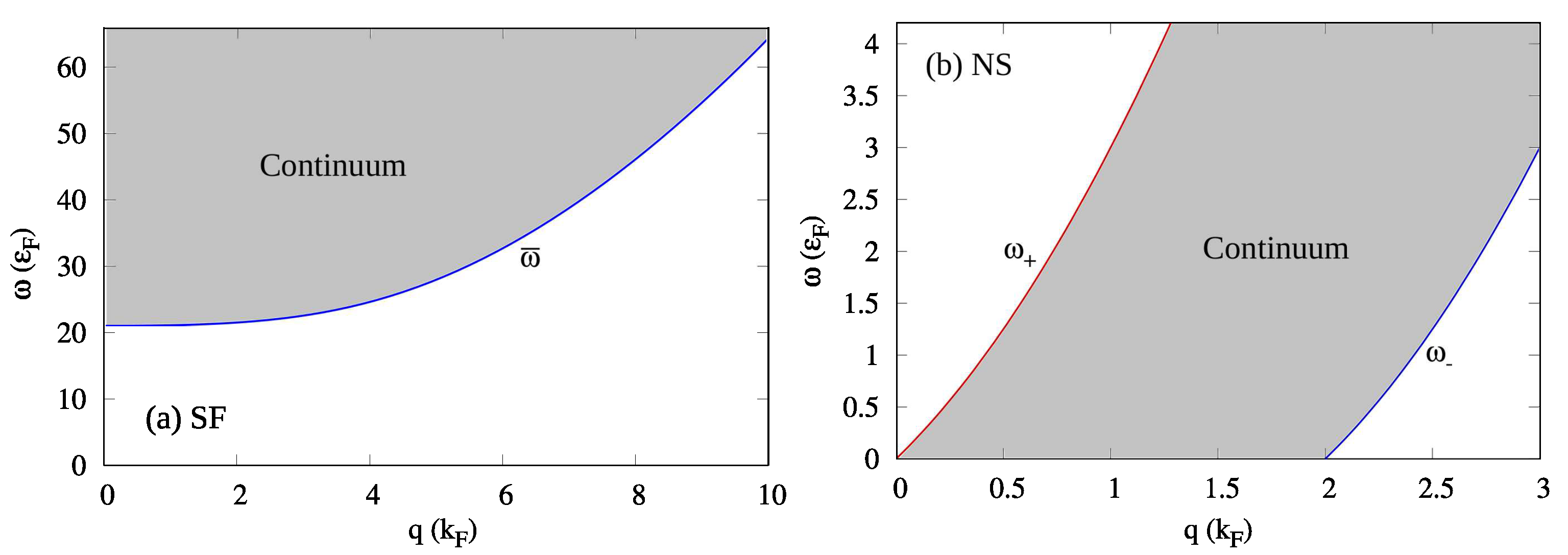
3.1. Density Collective Modes in the BEC Regime
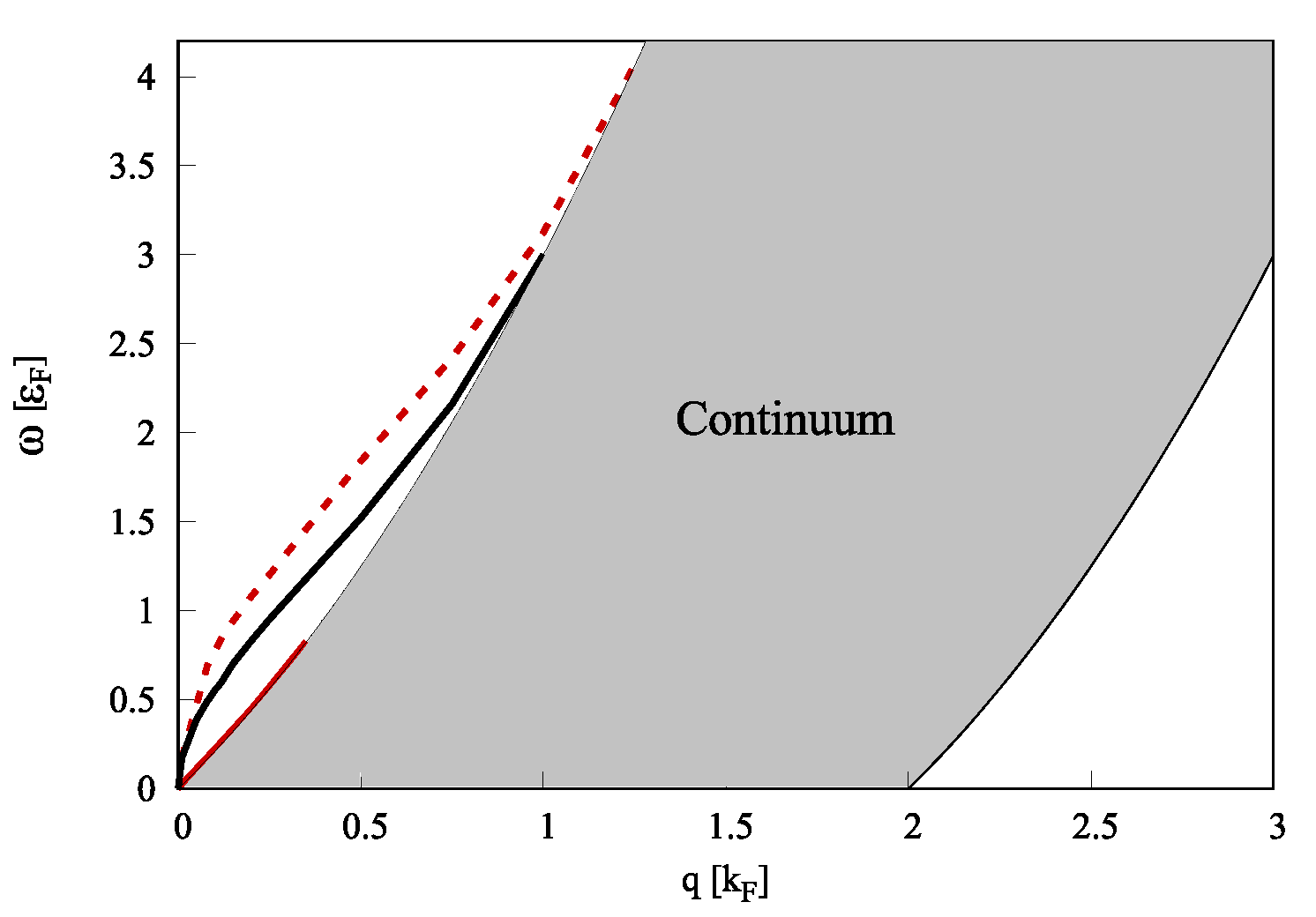
3.2. Density Collective Modes in the Crossover Regime
4. Superfluid Collective Modes in Ultracold Atoms and Exciton Bilayers Compared
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| BCS | Bardeen–Cooper–Schrieffer |
| BEC | Bose–Einstein Condensation |
| RPA | Random Phase Approximation |
| AB | Anderson–Bogoliubov |
| TMD | Transition Metal Dichalcogenide |
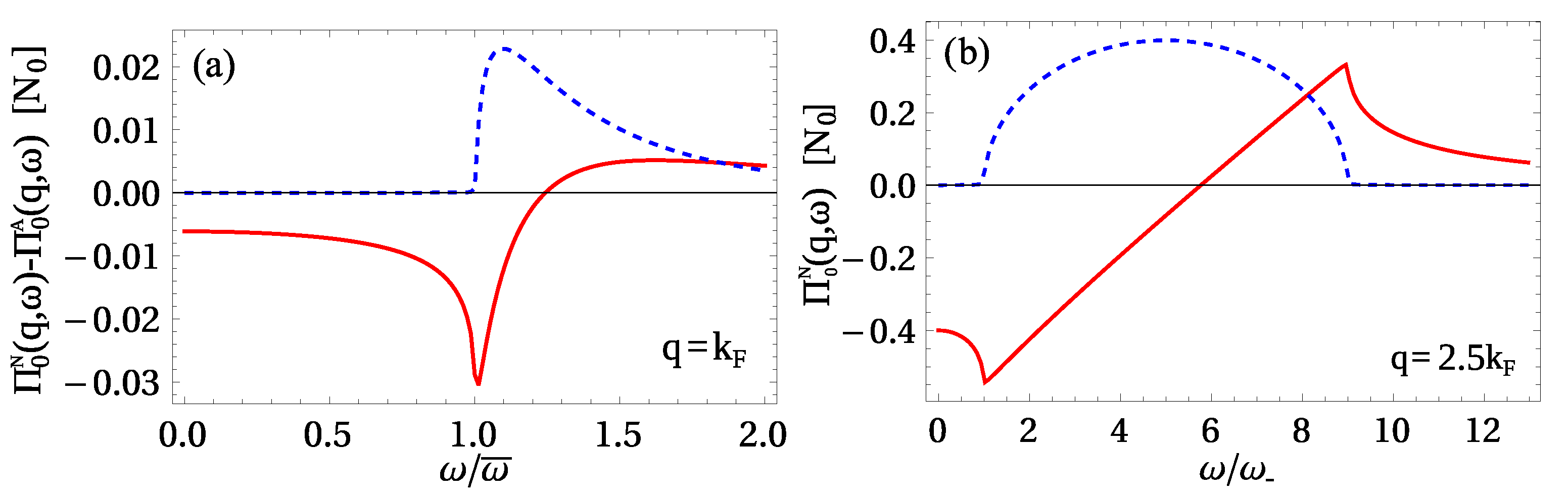
Appendix A. Normal State Collective Modes
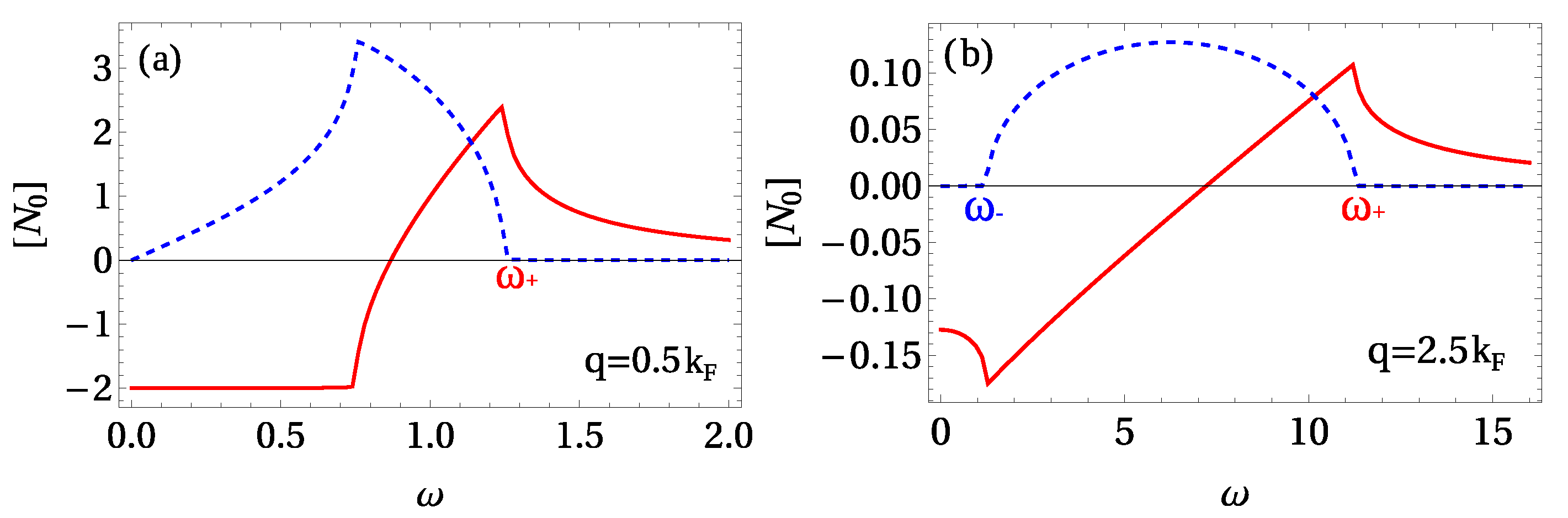
References
- Burg, G.W.; Prasad, N.; Kim, K.; Taniguchi, T.; Watanabe, K.; MacDonald, A.H.; Register, L.F.; Tutuc, E. Strongly Enhanced Tunneling at Total Charge Neutrality in Double-Bilayer Graphene-WSe2 Heterostructures. Phys. Rev. Lett. 2018, 120, 177702. [Google Scholar] [CrossRef]
- Wang, Z.; Rhodes, D.A.; Watanabe, K.; Taniguchi, T.; Hone, J.C.; Shan, J.; Mak, K.F. Evidence of high-temperature exciton condensation in two-dimensional atomic double layers. Nature 2019, 574, 76–80. [Google Scholar] [CrossRef]
- Ma, L.; Nguyen, P.X.; Wang, Z.; Zeng, Y.; Watanabe, K.; Taniguchi, T.; MacDonald, A.H.; Mak, K.F.; Shan, J. Strongly correlated excitonic insulator in atomic double layers. Nature 2021, 598, 585–589. [Google Scholar] [CrossRef]
- Gamucci, A.; Spirito, D.; Carrega, M.; Karmakar, B.; Lombardo, A.; Bruna, M.; Pfeiffer, L.; West, K.; Ferrari, A.; Polini, M.; et al. Anomalous low-temperature Coulomb drag in graphene-GaAs heterostructures. Nat. Commun. 2014, 5, 5824. [Google Scholar] [CrossRef]
- Gu, J.; Ma, L.; Liu, S.; Watanabe, K.; Taniguchi, T.; Hone, J.C.; Shan, J.; Mak, K.F. Dipolar excitonic insulator in a moiré lattice. Nat. Phys. 2022, 18, 395–400. [Google Scholar] [CrossRef]
- Nguyen, P.X.; Ma, L.; Chaturvedi, R.; Watanabe, K.; Taniguchi, T.; Shan, J.; Mak, K.F. Perfect Coulomb drag in a dipolar excitonic insulator. arXiv 2023, arXiv:2309.14940. [Google Scholar]
- Conti, S.; Perali, A.; Hamilton, A.R.; Milošević, M.V.; Peeters, F.M.; Neilson, D. Chester Supersolid of Spatially Indirect Excitons in Double-Layer Semiconductor Heterostructures. Phys. Rev. Lett. 2023, 130, 057001. [Google Scholar] [CrossRef]
- Kogan, V.G.; Tavger, B.A. Possibility of Pair Correlation of Electrons and Holes in a Sandwich Consisting of n- and p-Type Semiconductor Films. In Physics of p-n Junctions and Semiconductor Devices; Springer: Boston, MA, USA, 1971; pp. 39–45. [Google Scholar] [CrossRef]
- Lozovik, Y.E.; Yudson, V.I. A new mechanism for superconductivity: Pairing between spatially separated electrons and holes. Sov. Phys. JETP 1976, 44, 389–397. [Google Scholar]
- Perali, A.; Neilson, D.; Hamilton, A.R. High-temperature superfluidity in double-bilayer graphene. Phys. Rev. Lett. 2013, 110, 146803. [Google Scholar] [CrossRef]
- Neilson, D.; Perali, A.; Hamilton, A.R. Excitonic superfluidity and screening in electron-hole bilayer systems. Phys. Rev. B 2014, 89, 060502. [Google Scholar] [CrossRef]
- Nilsson, F.; Aryasetiawan, F. Effects of dynamical screening on the BCS-BEC crossover in double bilayer graphene: Density functional theory for exciton bilayers. Phys. Rev. Mater. 2021, 5, L050801. [Google Scholar] [CrossRef]
- De Palo, S.; Tramonto, F.; Moroni, S.; Senatore, G. Quadriexcitons and excitonic condensate in a symmetric electron-hole bilayer with valley degeneracy. Phys. Rev. B 2023, 107, L041409. [Google Scholar] [CrossRef]
- De Palo, S.; Trevisanutto, P.E.; Senatore, G.; Vignale, G. Collective excitations and quantum incompressibility in electron-hole bilayers. Phys. Rev. B 2021, 104, 115165. [Google Scholar] [CrossRef]
- Fetter, A.L. Electrodynamics of a layered electron gas. II. Periodic array. Ann. Phys. 1974, 88, 1–25. [Google Scholar] [CrossRef]
- Neilson, D.; Świerkowski, L.; Szymański, J.; Liu, L. Excitations of the strongly correlated electron liquid in coupled layers. Phys. Rev. Lett. 1993, 71, 4035–4038. [Google Scholar] [CrossRef] [PubMed]
- Pascucci, F.; Conti, S.; Perali, A.; Tempere, J.; Neilson, D. Effects of intralayer correlations on electron-hole double-layer superfluidity. Phys. Rev. B 2024, 109, 094512. [Google Scholar] [CrossRef]
- Altland, A.; Simons, B.D. Condensed Matter Field Theory; Cambridge University Press: Cambridge, UK, 2010. [Google Scholar]
- Sodemann, I.; Pesin, D.A.; MacDonald, A.H. Interaction-enhanced coherence between two-dimensional Dirac layers. Phys. Rev. B 2012, 85, 195136. [Google Scholar] [CrossRef]
- Lozovik, Y.E.; Ogarkov, S.L.; Sokolik, A.A. Condensation of electron-hole pairs in a two-layer graphene system: Correlation effects. Phys. Rev. B 2012, 86, 045429. [Google Scholar] [CrossRef]
- Lozovik, Y.E.; Sokolik, A.A. Multi-band pairing of ultrarelativistic electrons and holes in graphene bilayer. Phys. Lett. A 2009, 374, 326. [Google Scholar] [CrossRef]
- Mazloom, A.; Abedinpour, S.H. Interplay of interlayer pairing and many-body screening in a bilayer of dipolar fermions. Phys. Rev. B 2018, 98, 014513. [Google Scholar] [CrossRef]
- Giuliani, G.; Vignale, G. Quantum Theory of the Electron Liquid; Masters Series in Physics and Astronomy; Cambridge University Press: Cambridge, UK, 2005. [Google Scholar]
- Liu, L.; Świerkowski, L.; Neilson, D.; Szymański, J. Static and dynamic properties of coupled electron-electron and electron–hole layers. Phys. Rev. B 1996, 53, 7923–7931. [Google Scholar] [CrossRef]
- Maezono, R.; López Ríos, P.; Ogawa, T.; Needs, R.J. Excitons and biexcitons in symmetric electron-hole bilayers. Phys. Rev. Lett. 2013, 110, 216407. [Google Scholar] [CrossRef]
- Nikolaev, V.V.; Portnoi, M.E. Mott transition of spatially indirect excitons. In Proceedings of the Optical Science and Technology, the SPIE 49th Annual Meeting, Denver, CO, USA, 2 August 2004; SPIE: Denver, CO, USA, 2004; Volume 5509, pp. 187–193. [Google Scholar] [CrossRef]
- Kurkjian, H.; Tempere, J.; Klimin, S.N. Linear response of a superfluid Fermi gas inside its pair-breaking continuum. Sci. Rep. 2020, 10, 11591. [Google Scholar] [CrossRef]
- Hoinka, S.; Dyke, P.; Lingham, M.G.; Kinnunen, J.J.; Bruun, G.M.; Vale, C.J. Goldstone mode and pair-breaking excitations in atomic Fermi superfluids. Nat. Phys. 2017, 13, 943–946. [Google Scholar] [CrossRef]
- Djadaojee, L.; Douillet, A.; Grucker, J. Stimulated Brillouin Gain Spectroscopy of Superfluid Helium-4. J. Low Temp. Phys. 2021, 203, 234–243. [Google Scholar] [CrossRef]
- Anderson, P.W. Random–Phase Approximation in the Theory of Superconductivity. Phys. Rev. 1958, 112, 1900–1916. [Google Scholar] [CrossRef]
- Van Loon, S.; Sá de Melo, C.A.R. Effects of Quantum Fluctuations on the Low-Energy Collective Modes of Two-Dimensional Superfluid Fermi Gases from the BCS to the Bose Limit. Phys. Rev. Lett. 2023, 131, 113001. [Google Scholar] [CrossRef]
- Klimin, S.N.; Tempere, J.; Repplinger, T.; Kurkjian, H. Collective excitations of a charged Fermi superfluid in the BCS-BEC crossover. New J. Phys. 2023, 25, 063011. [Google Scholar] [CrossRef]
- Ohashi, Y.; Griffin, A. Superfluidity and collective modes in a uniform gas of Fermi atoms with a Feshbach resonance. Phys. Rev. A 2003, 67, 063612. [Google Scholar] [CrossRef]
- Donnelly, R.J. The two-fluid theory and second sound in liquid helium. Phys. Today 2009, 62, 34–39. [Google Scholar] [CrossRef]
- Ville, J.L.; Saint-Jalm, R.; Le Cerf, E.; Aidelsburger, M.; Nascimbène, S.; Dalibard, J.; Beugnon, J. Sound Propagation in a Uniform Superfluid Two-Dimensional Bose Gas. Phys. Rev. Lett. 2018, 121, 145301. [Google Scholar] [CrossRef] [PubMed]
- Singh, G.; Jouan, A.; Herranz, G.; Scigaj, M.; Sánchez, F.; Benfatto, L.; Caprara, S.; Grilli, M.; Saiz, G.; Couëdo, F.; et al. Gap suppression at a Lifshitz transition in a multi-condensate superconductor. Nat. Mater. 2019, 18, 948. [Google Scholar] [CrossRef]
- Pekker, D.; Varma, C. Amplitude/Higgs Modes in Condensed Matter Physics. Annu. Rev. Condens. Matter Phys. 2015, 6, 269–297. [Google Scholar] [CrossRef]
- Fogler, M.M.; Butov, L.V.; Novoselov, K.S. High-temperature superfluidity with indirect excitons in van der Waals heterostructures. Nat. Commun. 2014, 5, 4555. [Google Scholar] [CrossRef]
- Conti, S.; Neilson, D.; Peeters, F.M.; Perali, A. Transition metal dichalcogenides as strategy for high temperature electron-hole superfluidity. Condens. Matter 2020, 5, 22. [Google Scholar] [CrossRef]
- Ng, R.C.; El Sachat, A.; Cespedes, F.; Poblet, M.; Madiot, G.; Jaramillo-Fernandez, J.; Florez, O.; Xiao, P.; Sledzinska, M.; Sotomayor-Torres, C.M.; et al. Excitation and detection of acoustic phonons in nanoscale systems. Nanoscale 2022, 14, 13428–13451. [Google Scholar] [CrossRef]
- Monien, H.; Zawadowski, A. Theory of Raman scattering with final-state interaction in high-Tc BCS superconductors: Collective modes. Phys. Rev. B 1990, 41, 8798–8810. [Google Scholar] [CrossRef] [PubMed]
- Lindhard, J. On the properties of a gas of charged particles. Kgl. Dan. Videnskab. Selsk. Mat. Fys. Medd. 1954, 28, 8. [Google Scholar]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Pascucci, F.; Conti, S.; Neilson, D.; Perali, A.; Tempere, J. Density Collective Modes of Exciton Superfluidity in Bilayer Systems. Condens. Matter 2025, 10, 7. https://doi.org/10.3390/condmat10010007
Pascucci F, Conti S, Neilson D, Perali A, Tempere J. Density Collective Modes of Exciton Superfluidity in Bilayer Systems. Condensed Matter. 2025; 10(1):7. https://doi.org/10.3390/condmat10010007
Chicago/Turabian StylePascucci, Filippo, Sara Conti, David Neilson, Andrea Perali, and Jacques Tempere. 2025. "Density Collective Modes of Exciton Superfluidity in Bilayer Systems" Condensed Matter 10, no. 1: 7. https://doi.org/10.3390/condmat10010007
APA StylePascucci, F., Conti, S., Neilson, D., Perali, A., & Tempere, J. (2025). Density Collective Modes of Exciton Superfluidity in Bilayer Systems. Condensed Matter, 10(1), 7. https://doi.org/10.3390/condmat10010007






