Edelstein Effect in Isotropic and Anisotropic Rashba Models
Abstract
1. Introduction
2. Results
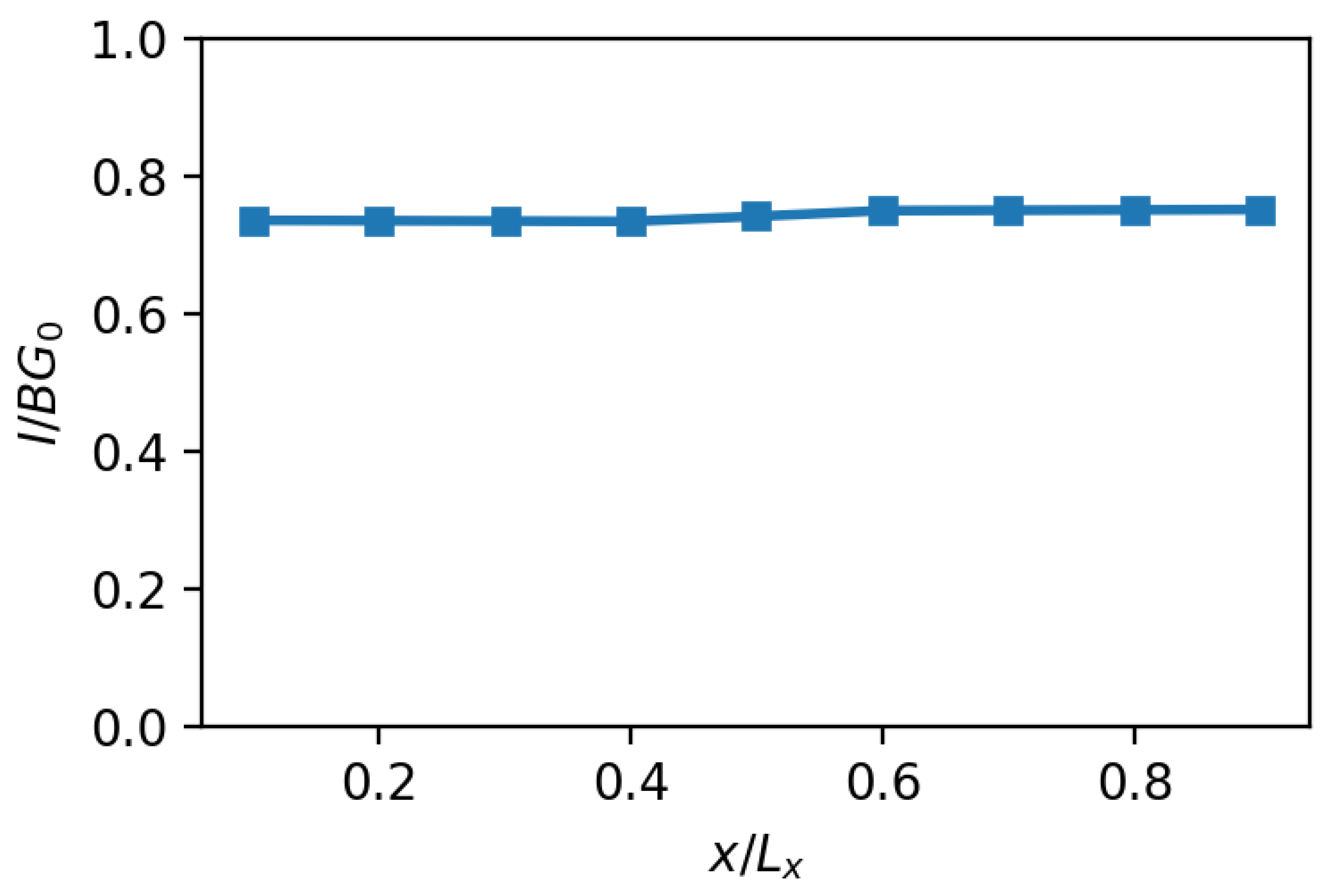
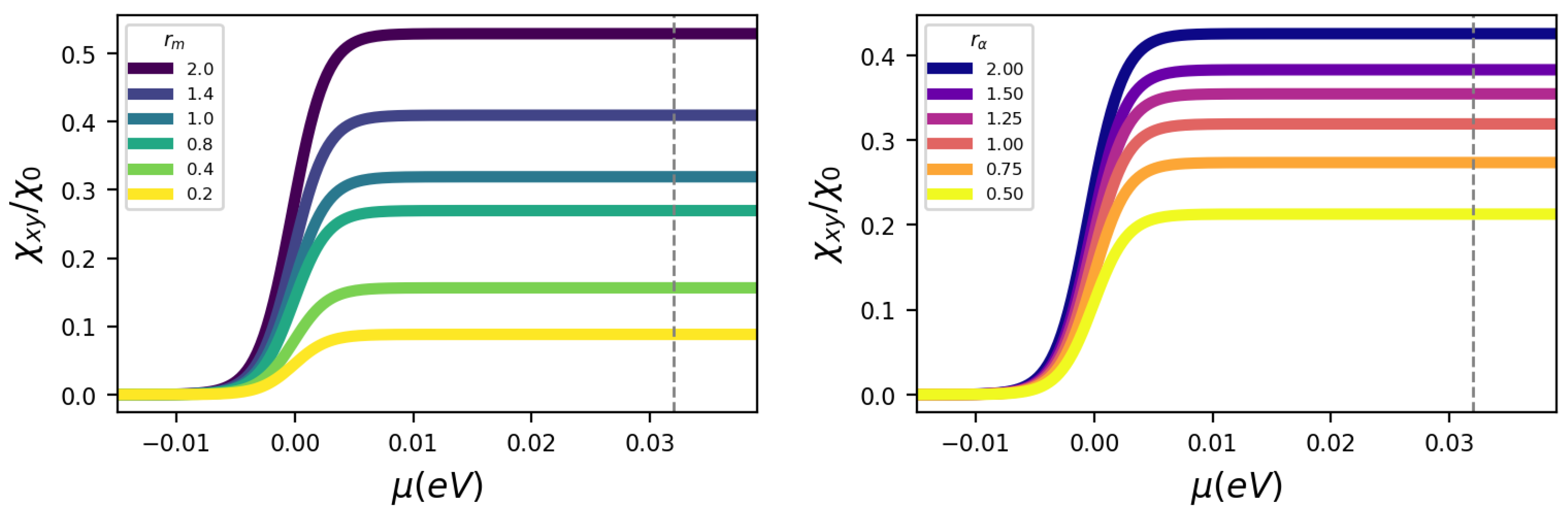
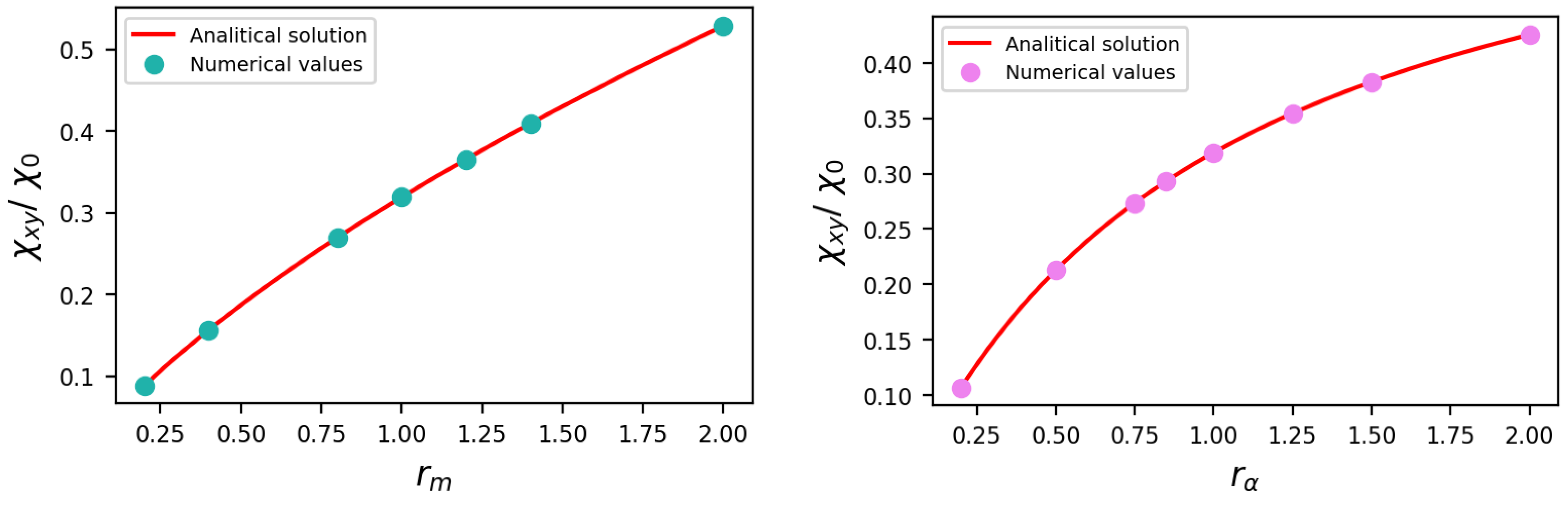
2.1. DEE: Analytical and Numerical Calculation of Spin Density
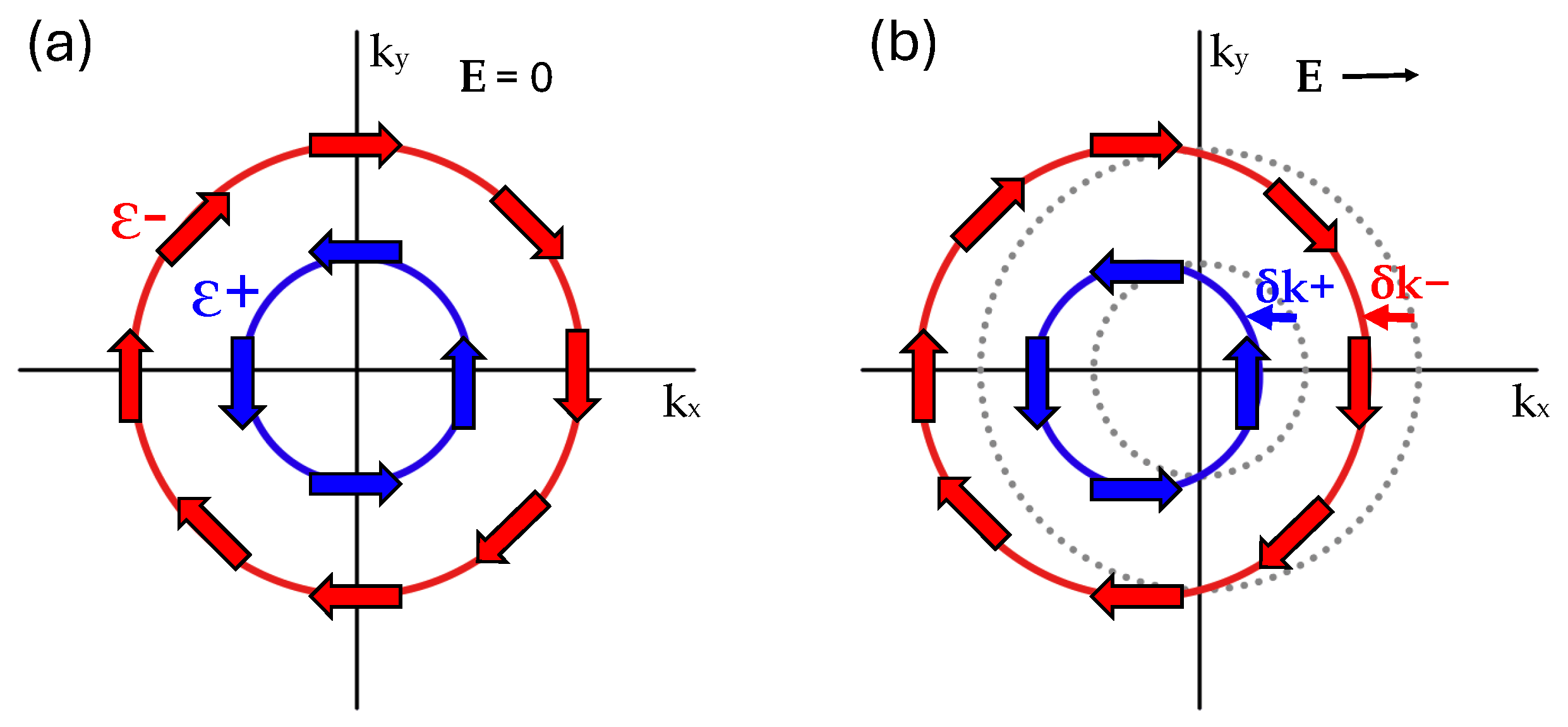
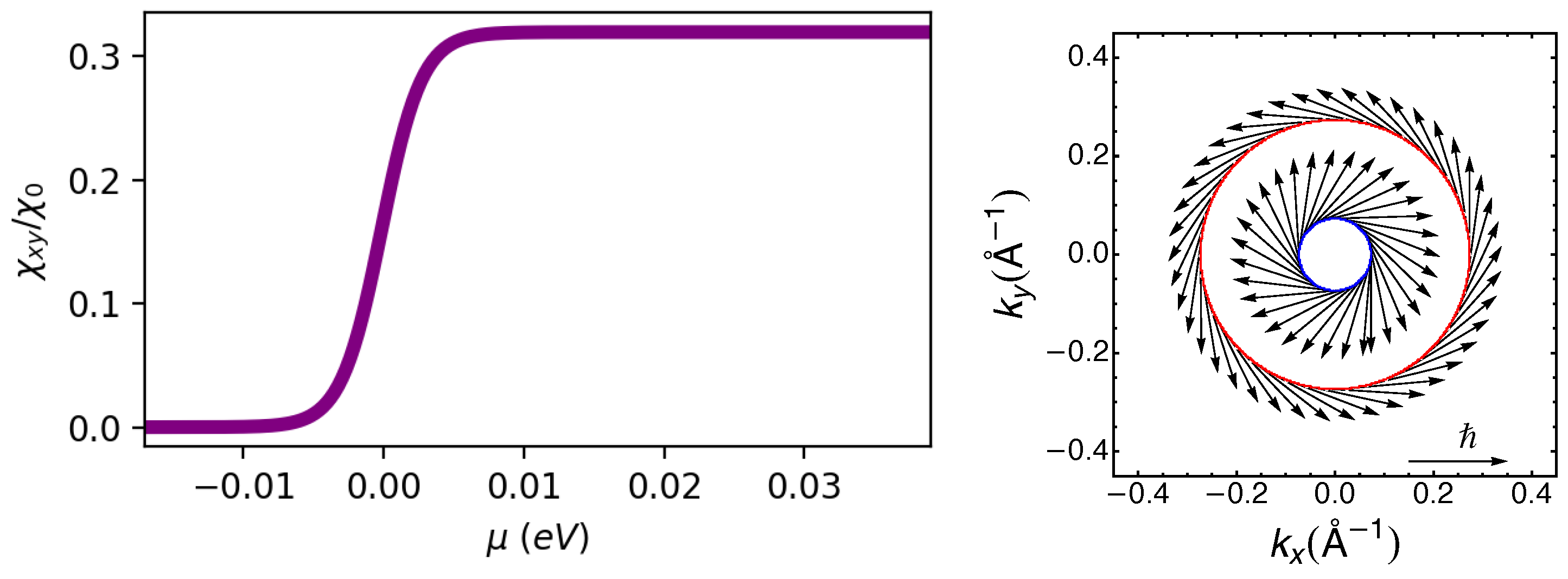
2.1.1. DEE in Isotropic Rashba Model
- In the LDR, the expression for the spin density becomes
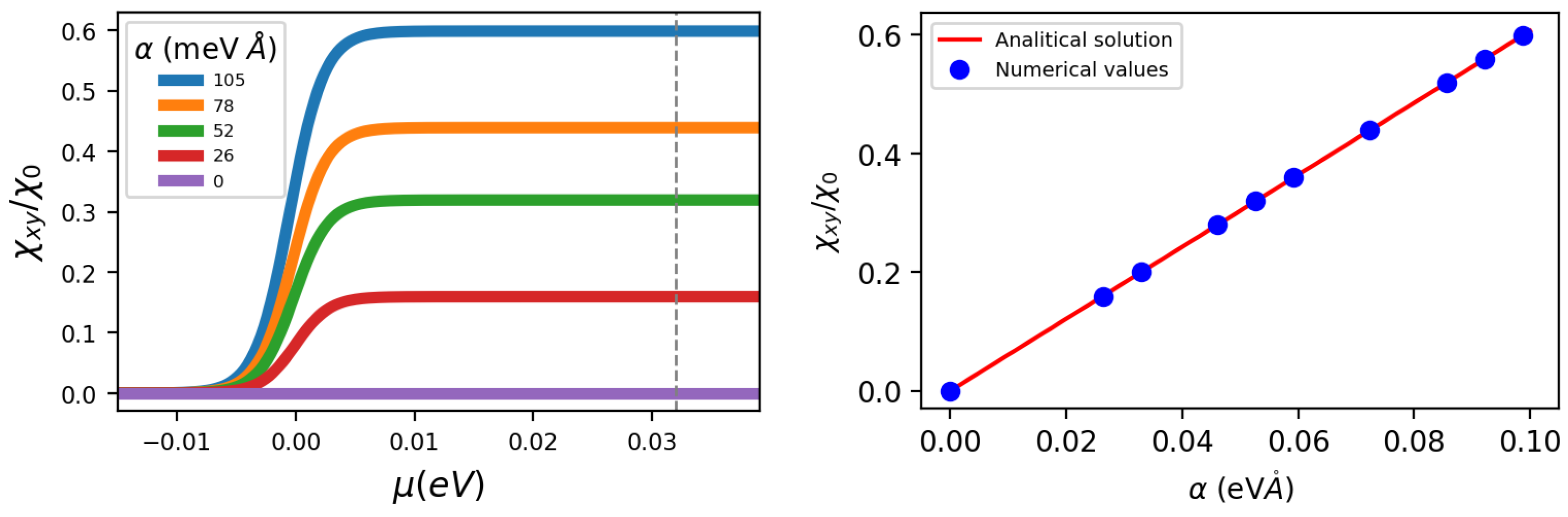
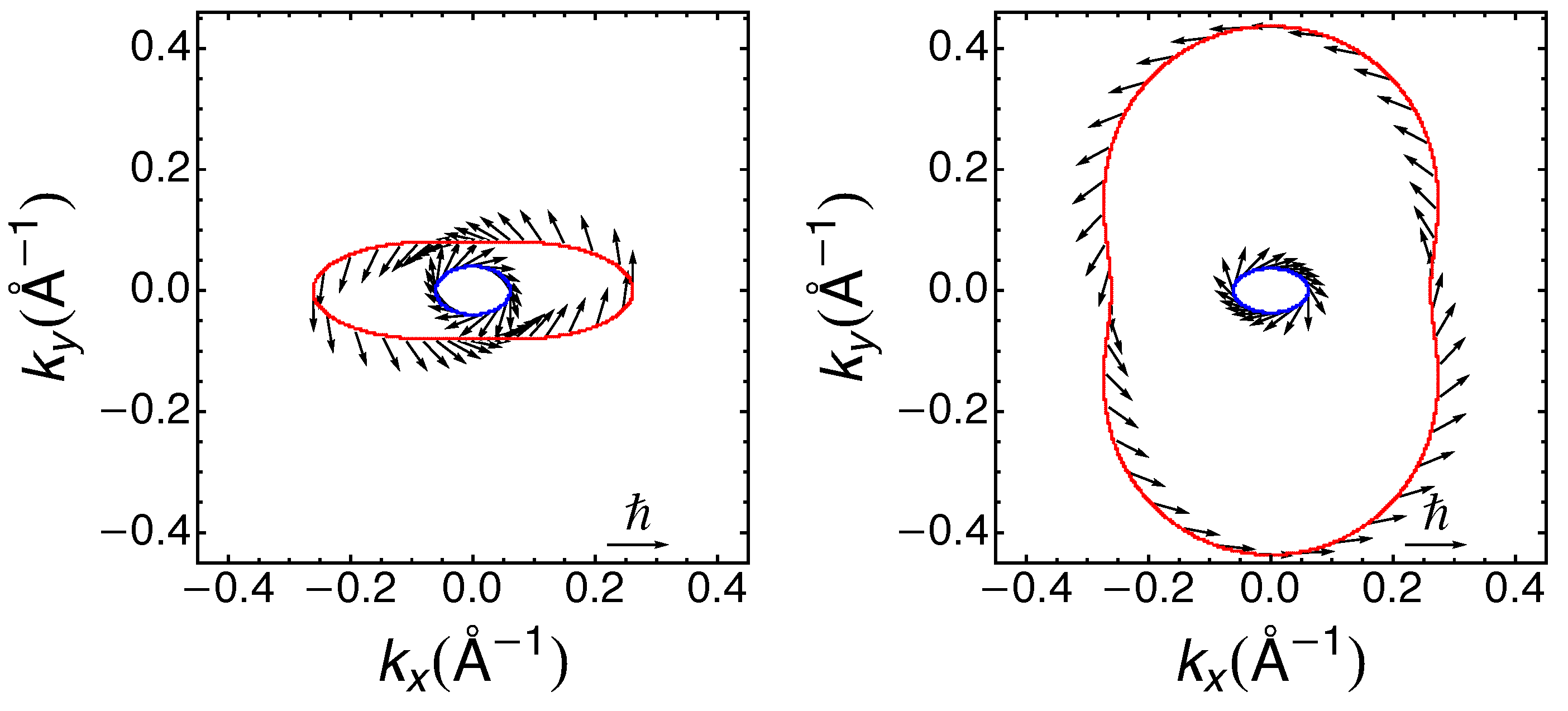
2.1.2. DEE in Anisotropic Case: The Symmetry
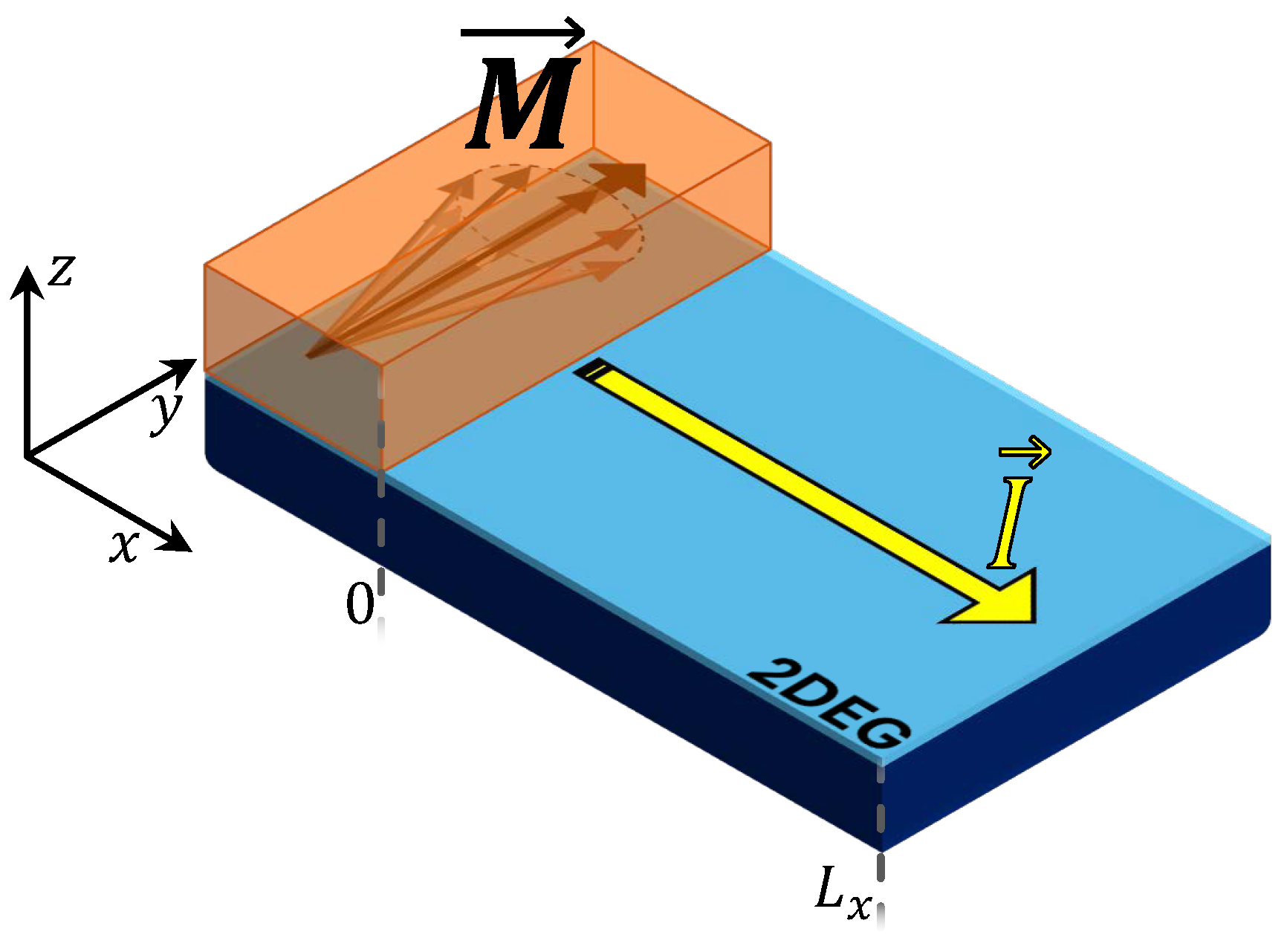
2.2. IEE: Inverse Edelstein Effect
3. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| DEE | Direct Edelstein Effect |
| IEE | Inverse Edelstein Effect |
| HDR | High-Density Regime |
| LDR | Low-Density Regime |
Appendix A
Appendix A.1
Appendix A.2
Appendix B
- The integral in the complex plane reads , with
Appendix C
Appendix D
References
- Wolf, S.; Awschalom, D.; Buhrman, R.; Daughton, J.; von Molnár, v.S.; Roukes, M.; Chtchelkanova, A.Y.; Treger, D. Spintronics: A spin-based electronics vision for the future. Science 2001, 294, 1488–1495. [Google Scholar] [CrossRef]
- Schmidt, G. Concepts for spin injection into semiconductors—A review. J. Phys. D Appl. Phys. 2005, 38, R107. [Google Scholar] [CrossRef]
- Soumyanarayanan, A.; Reyren, N.; Fert, A.; Panagopoulos, C. Emergent phenomena induced by spin–orbit coupling at surfaces and interfaces. Nature 2016, 539, 509–517. [Google Scholar] [CrossRef]
- Dieny, B.; Prejbeanu, I.L.; Garello, K.; Gambardella, P.; Freitas, P.; Lehndorff, R.; Raberg, W.; Ebels, U.; Demokritov, S.O.; Akerman, J.; et al. Opportunities and challenges for spintronics in the microelectronics industry. Nat. Electron. 2020, 3, 446–459. [Google Scholar] [CrossRef]
- Go, D.; Jo, D.; Lee, H.W.; Kläui, M.; Mokrousov, Y. Orbitronics: Orbital currents in solids. Europhys. Lett. 2021, 135, 37001. [Google Scholar] [CrossRef]
- Manchon, A.; Koo, H.C.; Nitta, J.; Frolov, S.M.; Duine, R.A. New perspectives for Rashba spin–orbit coupling. Nat. Mater. 2015, 14, 871–882. [Google Scholar] [CrossRef]
- Trama, M.; Cataudella, V.; Perroni, C.A.; Romeo, F.; Citro, R. Effect of Confinement and Coulomb Interactions on the Electronic Structure of the (111) LaAlO3/SrTiO3 Interface. Nanomaterials 2023, 13, 819. [Google Scholar] [CrossRef] [PubMed]
- Zhai, J.; Trama, M.; Liu, H.; Zhu, Z.; Zhu, Y.; Perroni, C.A.; Citro, R.; He, P.; Shen, J. Large Nonlinear Transverse Conductivity and Berry Curvature in KTaO3 Based Two-Dimensional Electron Gas. Nano Lett. 2023, 23, 11892–11898. [Google Scholar] [CrossRef]
- Trama, M.; Cataudella, V.; Perroni, C.; Romeo, F.; Citro, R. Gate tunable anomalous Hall effect: Berry curvature probe at oxides interfaces. Phys. Rev. B 2022, 106, 075430. [Google Scholar] [CrossRef]
- Chen, Y.; d’Antuono, M.; Trama, M.; Preziosi, D.; Jouault, B.; Teppe, F.; Consejo, C.; Perroni, C.A.; Citro, R.; Stornaiuolo, D.; et al. Dirac-Like Fermions Anomalous Magneto-Transport in a Spin-Polarized Oxide 2D Electron System. Adv. Mater. 2025, 37, 2410354. [Google Scholar] [CrossRef]
- Guarcello, C.; Maiellaro, A.; Settino, J.; Gaiardoni, I.; Trama, M.; Romeo, F.; Citro, R. Probing Topological Superconductivity of oxide nanojunctions using fractional Shapiro steps. Chaos Solitons Fractals 2024, 189, 115596. [Google Scholar] [CrossRef]
- Maiellaro, A.; Settino, J.; Guarcello, C.; Romeo, F.; Citro, R. Hallmarks of orbital-flavored Majorana states in Josephson junctions based on oxide nanochannels. Phys. Rev. B 2023, 107, L201405. [Google Scholar] [CrossRef]
- Bychkov, Y.A.; Rashba, É.I. Properties of a 2D electron gas with lifted spectral degeneracy. JETP Lett. 1984, 39, 78–81. [Google Scholar]
- Rashba, E.I. Spin-orbit coupling in condensed matter physics. Sov. Phys. Solid State 1960, 2, 1109. [Google Scholar]
- Maiellaro, A.; Trama, M.; Settino, J.; Guarcello, C.; Romeo, F.; Citro, R. Engineered Josephson diode effect in kinked Rashba nanochannels. SciPost Phys. 2024, 17, 101. [Google Scholar] [CrossRef]
- Amin, V.; Stiles, M. Spin transport at interfaces with spin-orbit coupling: Phenomenology. Phys. Rev. B 2016, 94, 104420. [Google Scholar] [CrossRef] [PubMed]
- Gariglio, S.; Caviglia, A.; Triscone, J.M.; Gabay, M. A spin–orbit playground: Surfaces and interfaces of transition metal oxides. Rep. Prog. Phys. 2018, 82, 012501. [Google Scholar] [CrossRef]
- Vaz, D.C.; Noël, P.; Johansson, A.; Göbel, B.; Bruno, F.Y.; Singh, G.; McKeown-Walker, S.; Trier, F.; Vicente-Arche, L.M.; Sander, A.; et al. Mapping spin–charge conversion to the band structure in a topological oxide two-dimensional electron gas. Nat. Mater. 2019, 18, 1187–1193. [Google Scholar] [CrossRef]
- Inoue, J.i.; Bauer, G.E.; Molenkamp, L.W. Diffuse transport and spin accumulation in a Rashba two-dimensional electron gas. Phys. Rev. B 2003, 67, 033104. [Google Scholar] [CrossRef]
- Trier, F.; Noël, P.; Kim, J.V.; Attané, J.P.; Vila, L.; Bibes, M. Oxide spin-orbitronics: Spin–charge interconversion and topological spin textures. Nat. Rev. Mater. 2022, 7, 258–274. [Google Scholar] [CrossRef]
- Vicente-Arche, L.; Bréhin, J.; Varotto, S.; Cosset-Cheneau, M.; Mallik, S.; Salazar, R.; Noël, P.; Vaz, D.C.; Trier, F.; Bhattacharya, S.; et al. Spin–charge interconversion in KTaO3 2D electron gases. Adv. Mater. 2021, 33, 2102102. [Google Scholar] [CrossRef] [PubMed]
- Trama, M.; Cataudella, V.; Perroni, C.A.; Romeo, F.; Citro, R. Tunable spin and orbital Edelstein effect at (111) LaAlO3/SrTiO3 interface. Nanomaterials 2022, 12, 2494. [Google Scholar] [CrossRef]
- Varotto, S.; Johansson, A.; Göbel, B.; Vicente-Arche, L.M.; Mallik, S.; Bréhin, J.; Salazar, R.; Bertran, F.; Fèvre, P.L.; Bergeal, N.; et al. Direct visualization of Rashba-split bands and spin/orbital-charge interconversion at KTaO3 interfaces. Nat. Commun. 2022, 13, 6165. [Google Scholar] [CrossRef] [PubMed]
- Sinova, J.; Culcer, D.; Niu, Q.; Sinitsyn, N.; Jungwirth, T.; MacDonald, A.H. Universal intrinsic spin Hall effect. Phys. Rev. Lett. 2004, 92, 126603. [Google Scholar] [CrossRef] [PubMed]
- Bibes, M.; Villegas, J.E.; Barthélémy, A. Ultrathin oxide films and interfaces for electronics and spintronics. Adv. Phys. 2011, 60, 5–84. [Google Scholar] [CrossRef]
- Gambardella, P.; Miron, I.M. Current-induced spin–orbit torques. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2011, 369, 3175–3197. [Google Scholar] [CrossRef]
- Trama, M.; Gaiardoni, I.; Guarcello, C.; Facio, J.I.; Maiellaro, A.; Romeo, F.; Citro, R.; Brink, J.v.d. Non-linear anomalous Edelstein response at altermagnetic interfaces. arXiv 2024, arXiv:2410.18036. [Google Scholar]
- Seibold, G.; Caprara, S.; Grilli, M.; Raimondi, R. Theory of the spin galvanic effect at oxide interfaces. Phys. Rev. Lett. 2017, 119, 256801. [Google Scholar] [CrossRef]
- Edelstein, V.M. Spin polarization of conduction electrons induced by electric current in two-dimensional asymmetric electron systems. Solid State Commun. 1990, 73, 233–235. [Google Scholar] [CrossRef]
- Leiva-Montecinos, S.; Henk, J.; Mertig, I.; Johansson, A. Spin and orbital Edelstein effect in a bilayer system with Rashba interaction. Phys. Rev. Res. 2023, 5, 043294. [Google Scholar] [CrossRef]
- El Hamdi, A.; Chauleau, J.Y.; Boselli, M.; Thibault, C.; Gorini, C.; Smogunov, A.; Barreteau, C.; Gariglio, S.; Triscone, J.M.; Viret, M. Observation of the orbital inverse Rashba–Edelstein effect. Nat. Phys. 2023, 19, 1855–1860. [Google Scholar] [CrossRef]
- Aronov, A.; Lyanda-Geller, Y.B. Nuclear electric resonance and orientation of carrier spins by an electric field. Sov. J. Exp. Theor. Phys. Lett. 1989, 50, 431. [Google Scholar]
- Johansson, A.; Göbel, B.; Henk, J.; Bibes, M.; Mertig, I. Spin and orbital Edelstein effects in a two-dimensional electron gas: Theory and application to SrTiO 3 interfaces. Phys. Rev. Res. 2021, 3, 013275. [Google Scholar] [CrossRef]
- Johansson, A.; Henk, J.; Mertig, I. Theoretical aspects of the Edelstein effect for anisotropic two-dimensional electron gas and topological insulators. Phys. Rev. B 2016, 93, 195440. [Google Scholar] [CrossRef]
- Shen, K.; Vignale, G.; Raimondi, R. Microscopic theory of the inverse Edelstein effect. Phys. Rev. Lett. 2014, 112, 096601. [Google Scholar] [CrossRef]
- Kato, Y.; Myers, R.; Gossard, A.; Awschalom, D. Current-induced spin polarization in strained semiconductors. Phys. Rev. Lett. 2004, 93, 176601. [Google Scholar] [CrossRef]
- Silov, A.Y.; Blajnov, P.; Wolter, J.; Hey, R.; Ploog, K.; Averkiev, N. Current-induced spin polarization at a single heterojunction. Appl. Phys. Lett. 2004, 85, 5929–5931. [Google Scholar] [CrossRef]
- Gorini, C.; Raimondi, R.; Schwab, P. Onsager relations in a two-dimensional electron gas with spin-orbit coupling. Phys. Rev. Lett. 2012, 109, 246604. [Google Scholar] [CrossRef]
- Sánchez, J.R.; Vila, L.; Desfonds, G.; Gambarelli, S.; Attané, J.; De Teresa, J.; Magén, C.; Fert, A. Spin-to-charge conversion using Rashba coupling at the interface between non-magnetic materials. Nat. Commun. 2013, 4, 2944. [Google Scholar] [CrossRef] [PubMed]
- Vignale, G.; Tokatly, I. Theory of the nonlinear Rashba-Edelstein effect: The clean electron gas limit. Phys. Rev. B 2016, 93, 035310. [Google Scholar] [CrossRef]
- Hirsch, J. Spin hall effect. Phys. Rev. Lett. 1999, 83, 1834. [Google Scholar] [CrossRef]
- Valenzuela, S.O.; Tinkham, M. Direct electronic measurement of the spin Hall effect. Nature 2006, 442, 176–179. [Google Scholar] [CrossRef]
- Kimura, T.; Otani, Y.; Sato, T.; Takahashi, S.; Maekawa, S. Room-temperature reversible spin Hall effect. Phys. Rev. Lett. 2007, 98, 156601. [Google Scholar] [CrossRef] [PubMed]
- Werake, L.K.; Ruzicka, B.A.; Zhao, H. Observation of intrinsic inverse spin Hall effect. Phys. Rev. Lett. 2011, 106, 107205. [Google Scholar] [CrossRef]
- Murakami, S.; Nagaosa, N.; Zhang, S.C. Dissipationless quantum spin current at room temperature. Science 2003, 301, 1348–1351. [Google Scholar] [CrossRef]
- Jungwirth, T.; Wunderlich, J.; Olejník, K. Spin Hall effect devices. Nat. Mater. 2012, 11, 382–390. [Google Scholar] [CrossRef] [PubMed]
- Kronmüller, H. Handbook of Magnetism and Advanced Magnetic Materials; John Wiley & Sons: Hoboken, NJ, USA, 2007; Volume 1, 879p. [Google Scholar]
- Wunderlich, J.; Kaestner, B.; Sinova, J.; Jungwirth, T. Experimental Observation of the Spin-Hall Effect in a Two-Dimensional Spin-Orbit Coupled Semiconductor System. Phys. Rev. Lett. 2005, 94, 047204. [Google Scholar] [CrossRef] [PubMed]
- Mahfouzi, F.; Nagaosa, N.; Nikolić, B.K. Spin-to-charge conversion in lateral and vertical topological-insulator/ferromagnet heterostructures with microwave-driven precessing magnetization. Phys. Rev. B 2014, 90, 115432. [Google Scholar] [CrossRef]
- Isasa, M.; Martínez-Velarte, M.C.; Villamor, E.; Magén, C.; Morellón, L.; De Teresa, J.M.; Ibarra, M.R.; Vignale, G.; Chulkov, E.V.; Krasovskii, E.E.; et al. Origin of inverse Rashba-Edelstein effect detected at the Cu/Bi interface using lateral spin valves. Phys. Rev. B 2016, 93, 014420. [Google Scholar] [CrossRef]
- Luo, W.; Deng, W.; Geng, H.; Chen, M.; Shen, R.; Sheng, L.; Xing, D. Perfect inverse spin Hall effect and inverse Edelstein effect due to helical spin-momentum locking in topological surface states. Phys. Rev. B 2016, 93, 115118. [Google Scholar] [CrossRef]
- Geng, H.; Deng, W.Y.; Ren, Y.J.; Sheng, L.; Xing, D.Y. Unified semiclassical approach to electronic transport from diffusive to ballistic regimes. Chin. Phys. B 2016, 25, 097201. [Google Scholar] [CrossRef]
- Geng, H.; Luo, W.; Deng, W.; Sheng, L.; Shen, R.; Xing, D. Theory of inverse edelstein effect of the surface states of a topological insulator. Sci. Rep. 2017, 7, 3755. [Google Scholar] [CrossRef] [PubMed]
- Landauer, R. Spatial variation of currents and fields due to localized scatterers in metallic conduction. IBM J. Res. Dev. 1957, 1, 223–231. [Google Scholar] [CrossRef]
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gaiardoni, I.; Trama, M.; Maiellaro, A.; Guarcello, C.; Romeo, F.; Citro, R. Edelstein Effect in Isotropic and Anisotropic Rashba Models. Condens. Matter 2025, 10, 15. https://doi.org/10.3390/condmat10010015
Gaiardoni I, Trama M, Maiellaro A, Guarcello C, Romeo F, Citro R. Edelstein Effect in Isotropic and Anisotropic Rashba Models. Condensed Matter. 2025; 10(1):15. https://doi.org/10.3390/condmat10010015
Chicago/Turabian StyleGaiardoni, Irene, Mattia Trama, Alfonso Maiellaro, Claudio Guarcello, Francesco Romeo, and Roberta Citro. 2025. "Edelstein Effect in Isotropic and Anisotropic Rashba Models" Condensed Matter 10, no. 1: 15. https://doi.org/10.3390/condmat10010015
APA StyleGaiardoni, I., Trama, M., Maiellaro, A., Guarcello, C., Romeo, F., & Citro, R. (2025). Edelstein Effect in Isotropic and Anisotropic Rashba Models. Condensed Matter, 10(1), 15. https://doi.org/10.3390/condmat10010015






