Numerical Study of the Hydrodynamic Response of Biodegradable Drifting Fish Aggregating Devices in Regular Waves
Abstract
1. Introduction
2. Methodology
2.1. Description of Bio-DFADs
2.2. Wave Conditions
2.3. Numerical Model
2.3.1. Governing Equations and Turbulence Model
- Continuity equation:
- Momentum equation:
2.3.2. Turbulence Model
- k equation:
- ε equation:
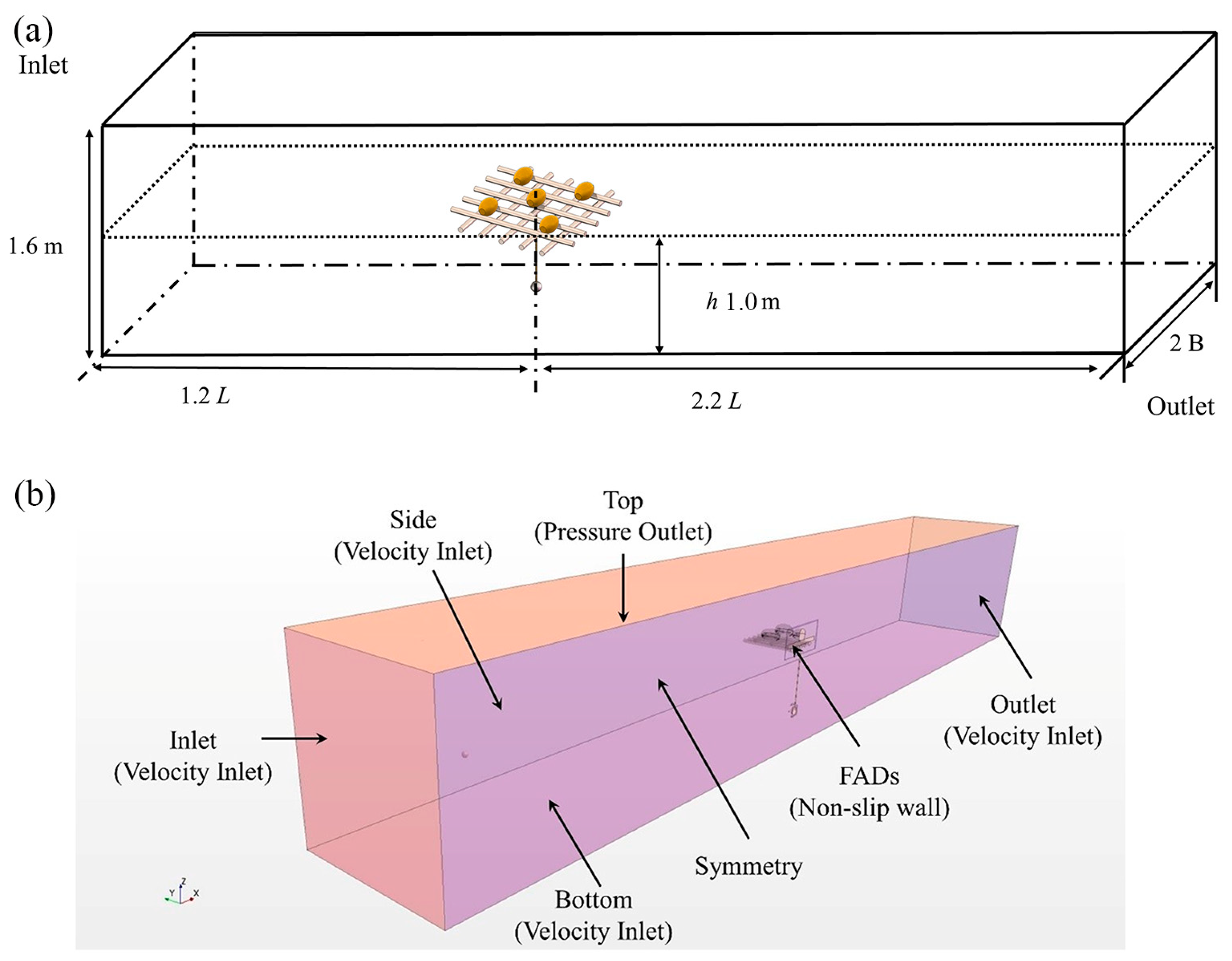
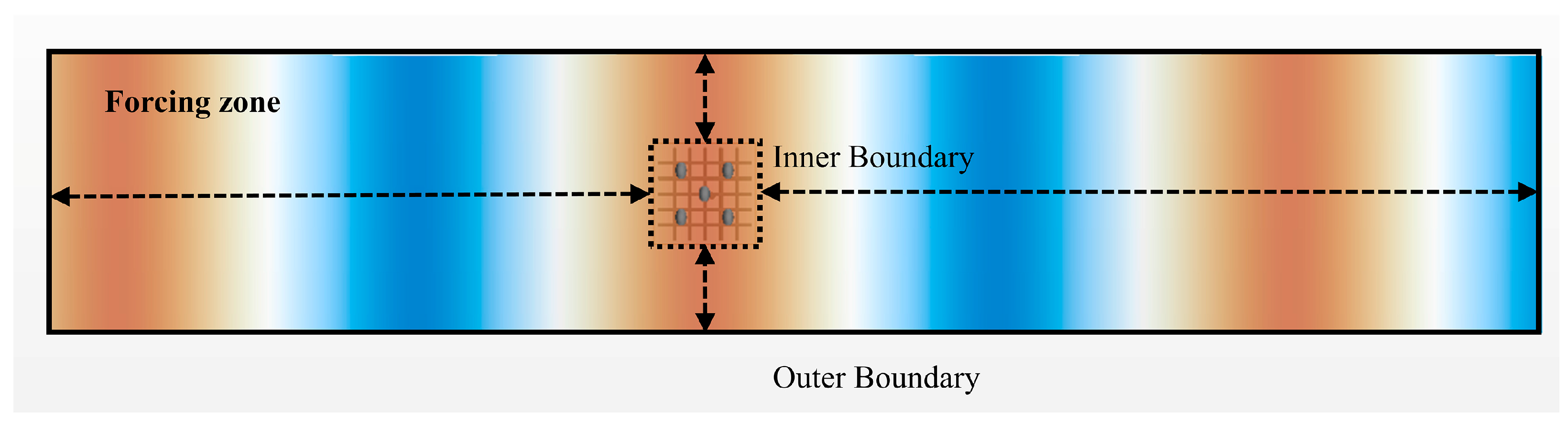
2.3.3. Computational Domain and Boundary Conditions
- Translate equation:
- Rotate equation:
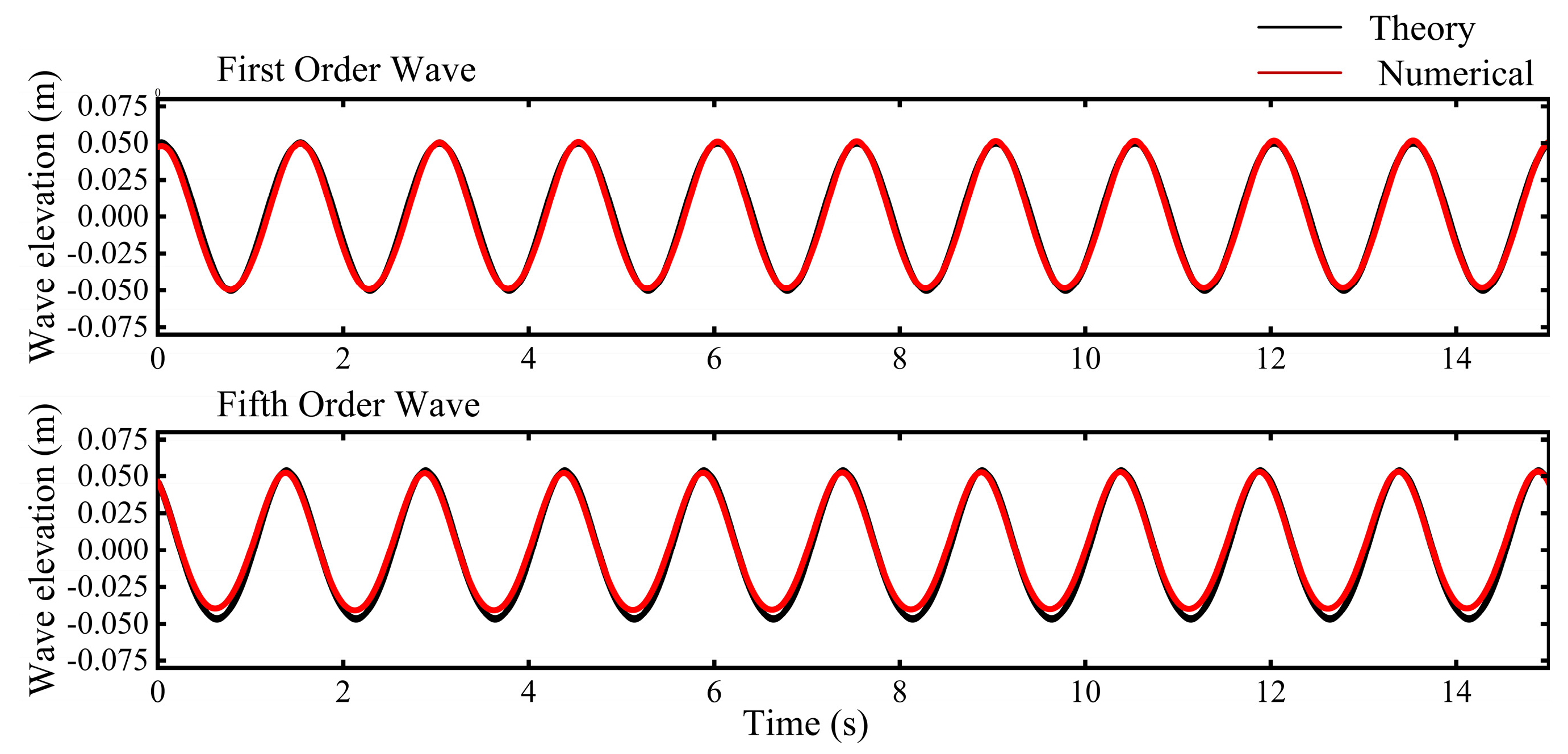
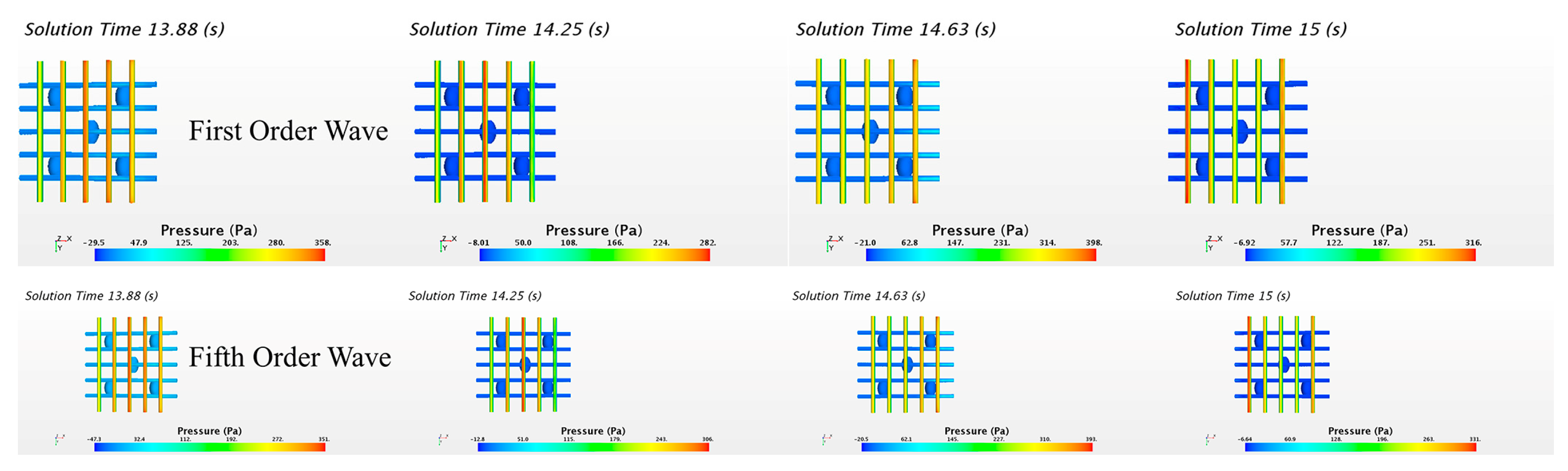
2.4. Verification of the Numerical Model
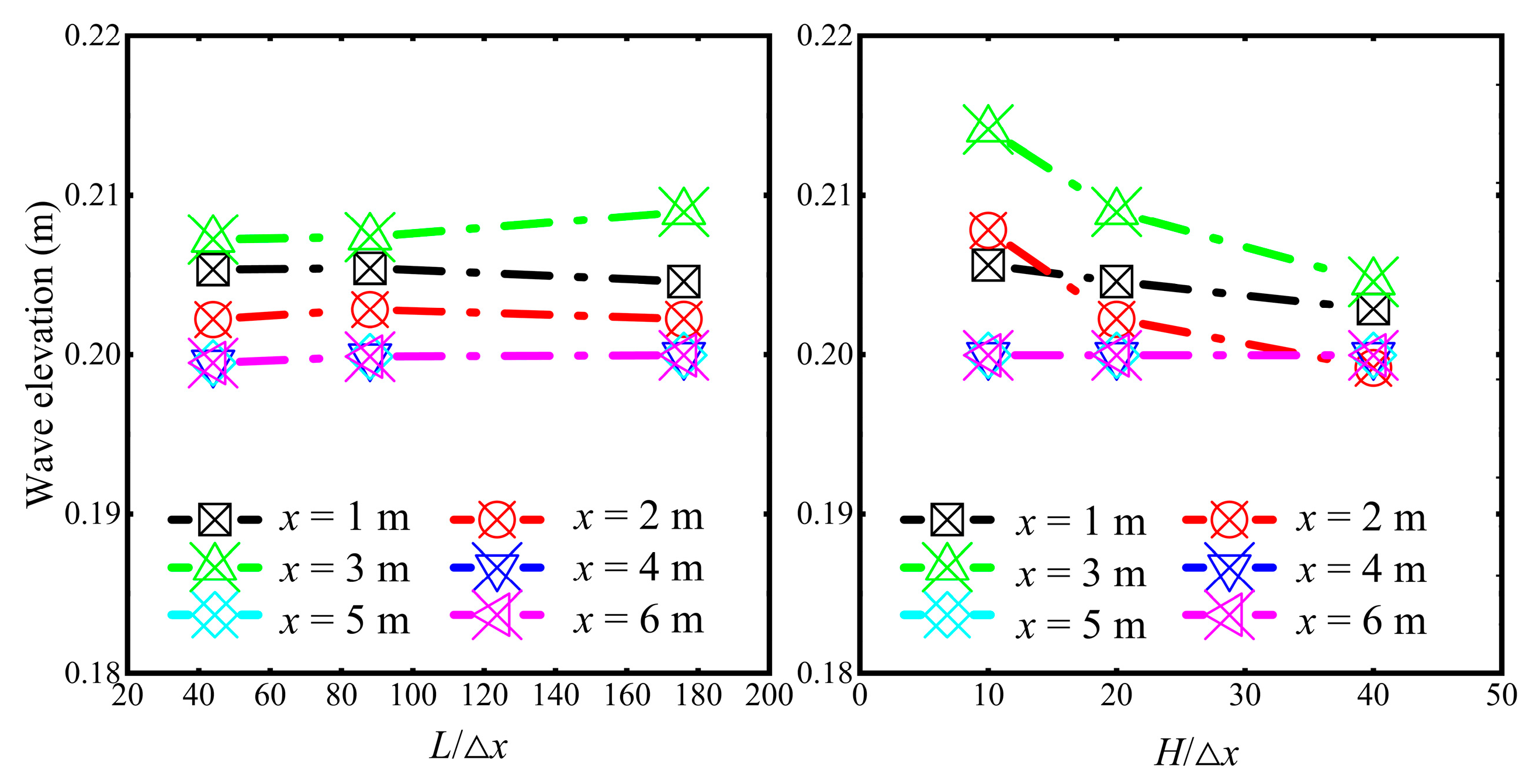
2.4.1. Mesh Sensitivity Analysis
2.4.2. Time-Step Sensitivity Analysis
2.5. Mesh Generation
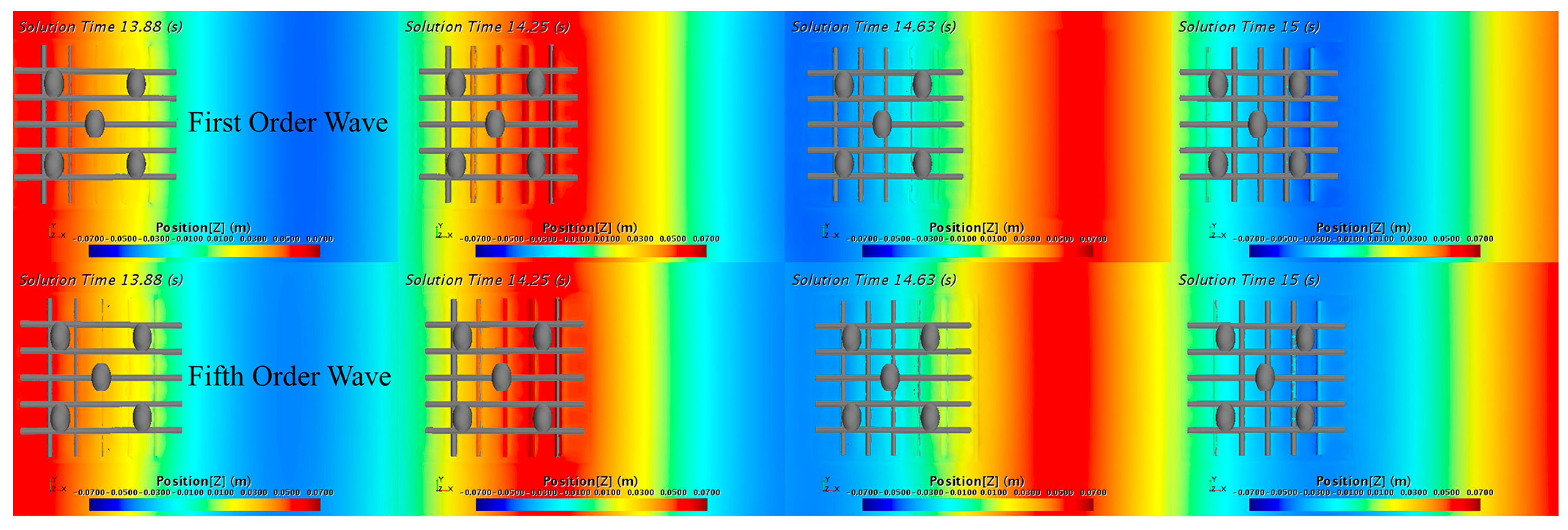
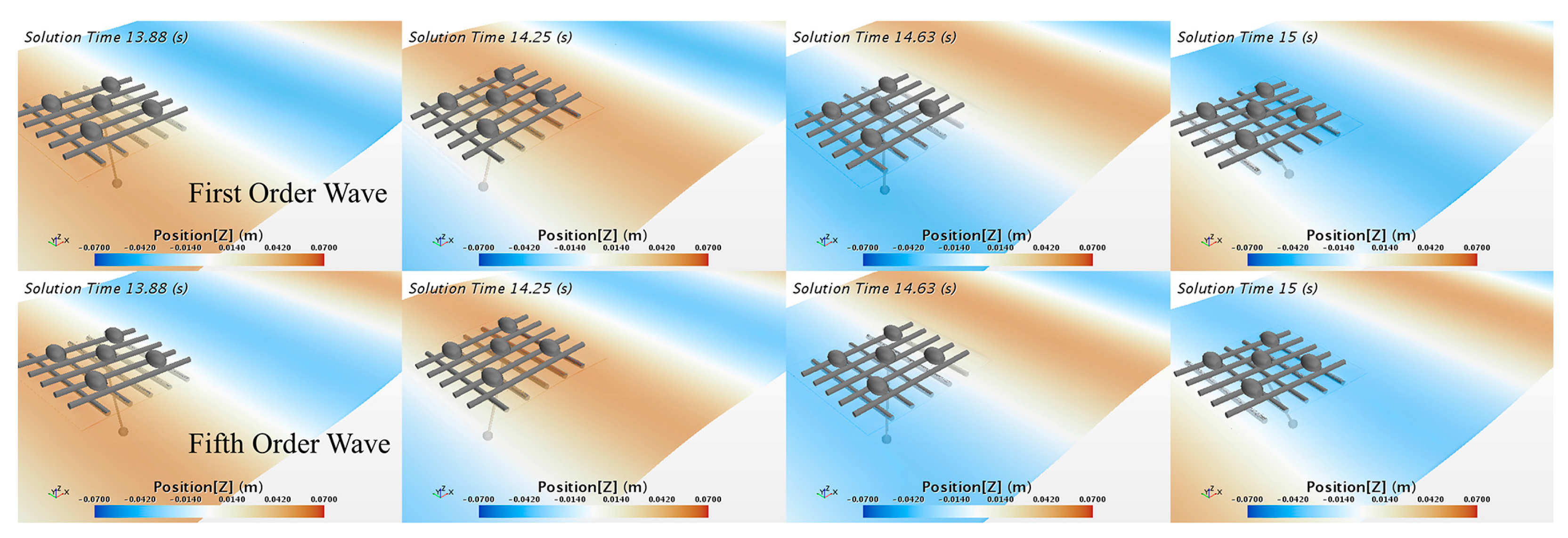
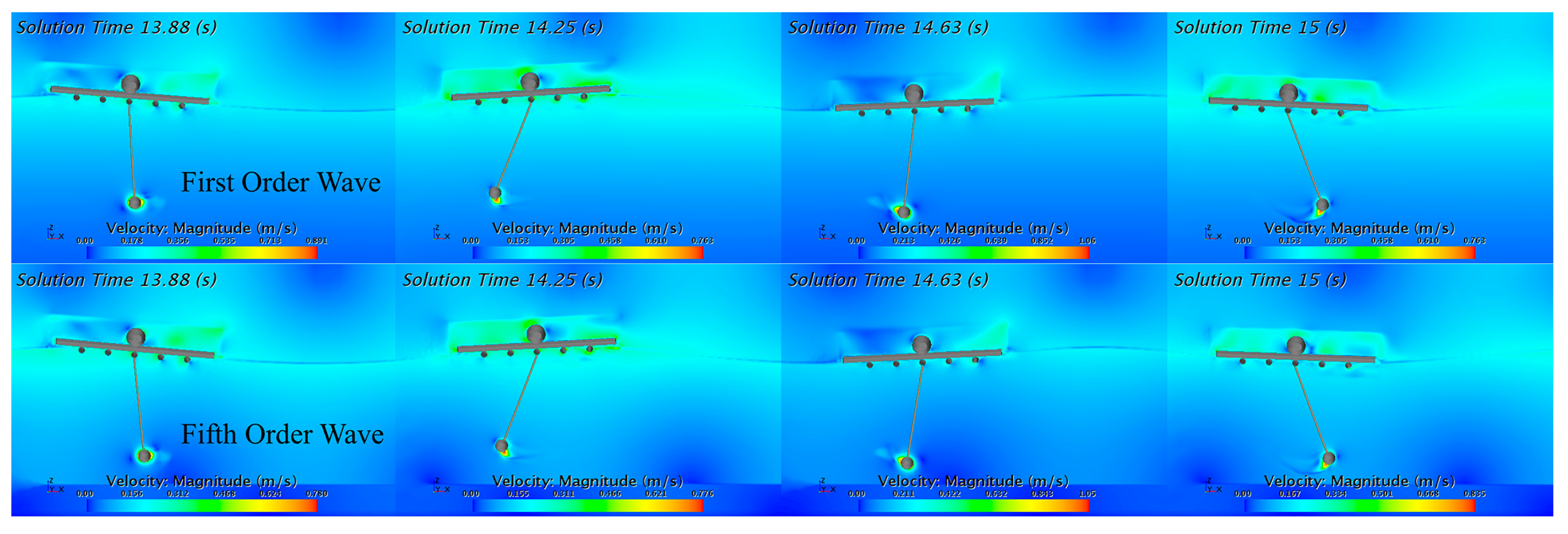
3. Results and Analysis
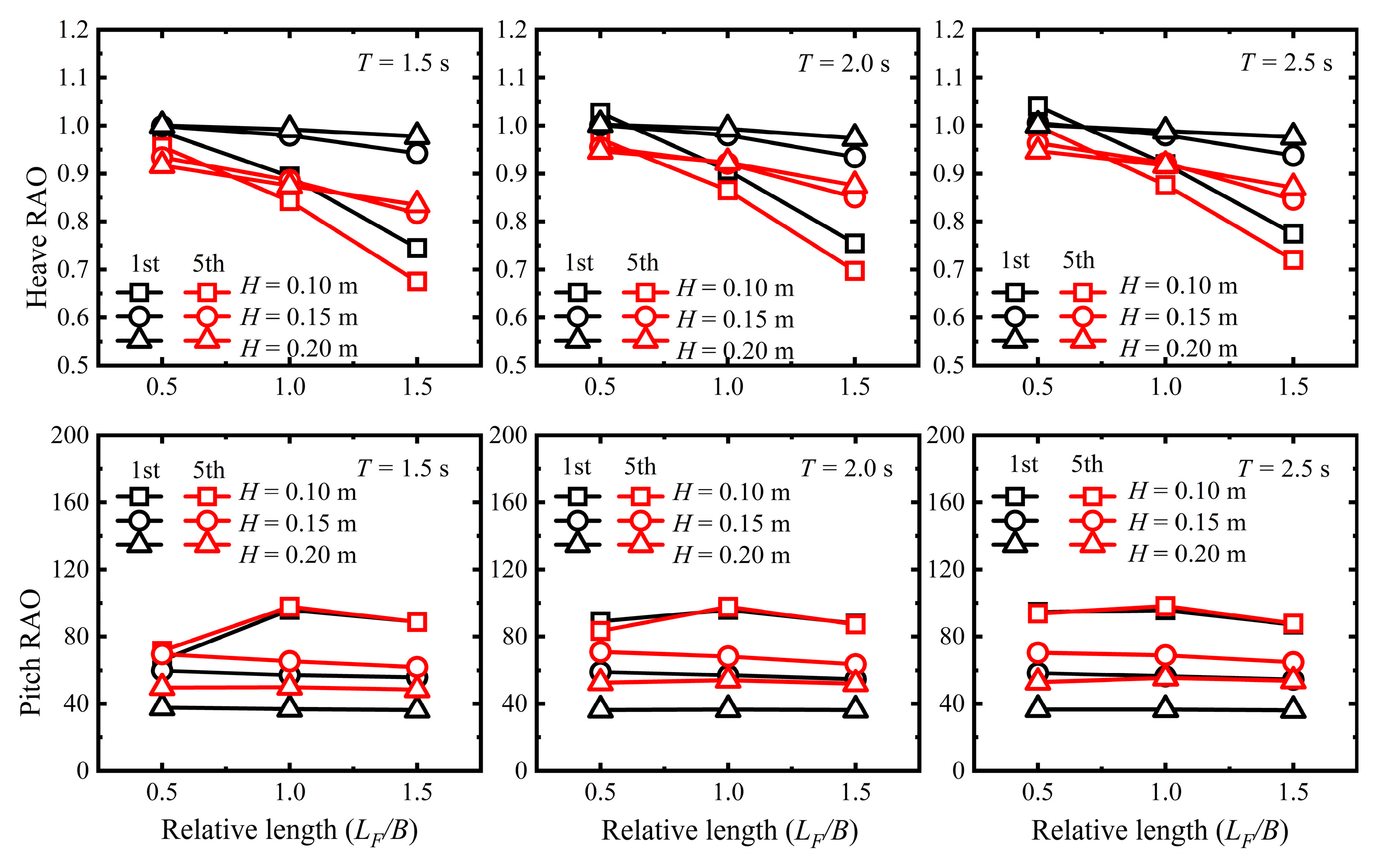
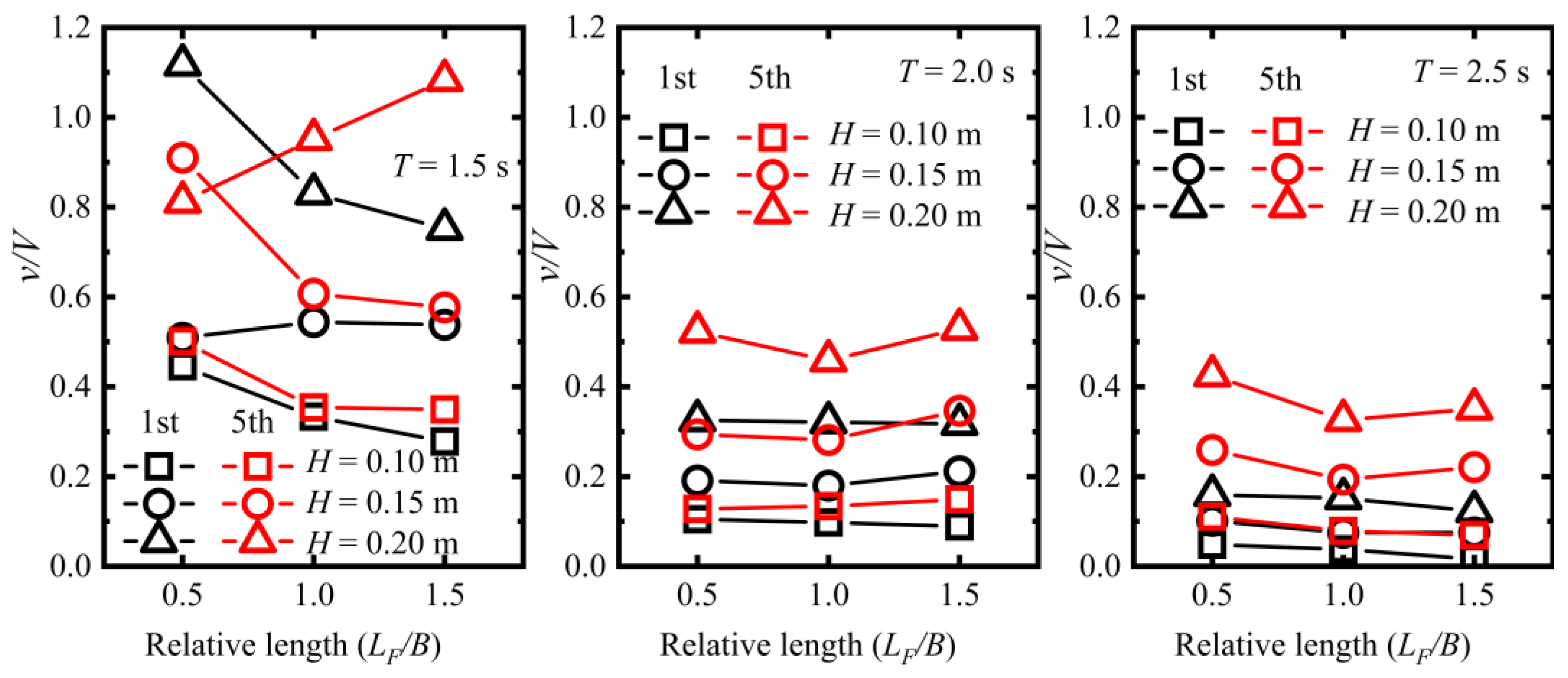
3.1. Influence of Relative Length on the Hydrodynamic Performance of Bio-DFADs
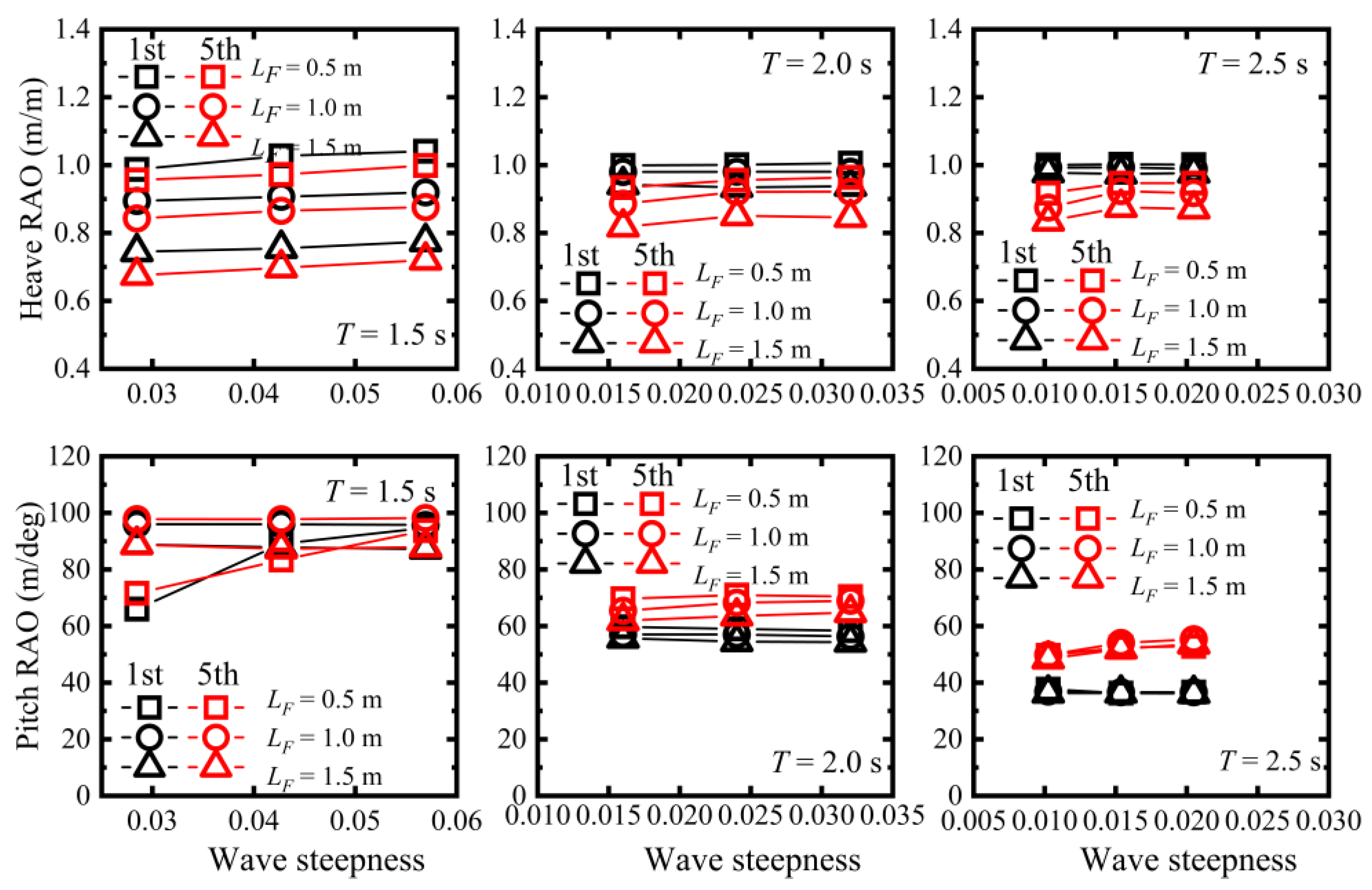
3.2. Influence of Wave Steepness on the Hydrodynamic Performance of Bio-DFADs
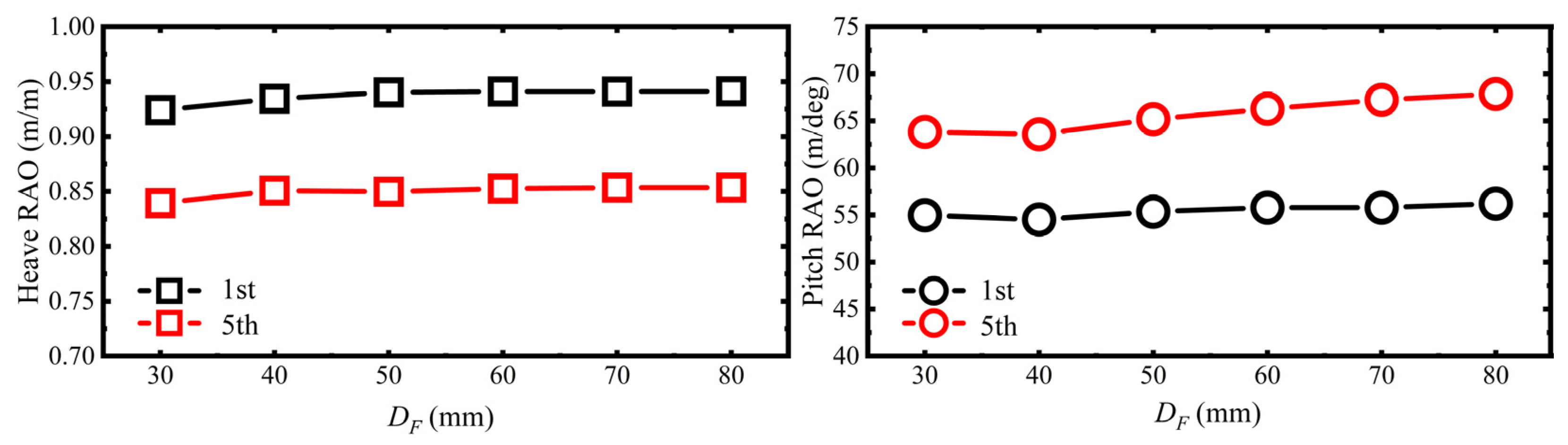
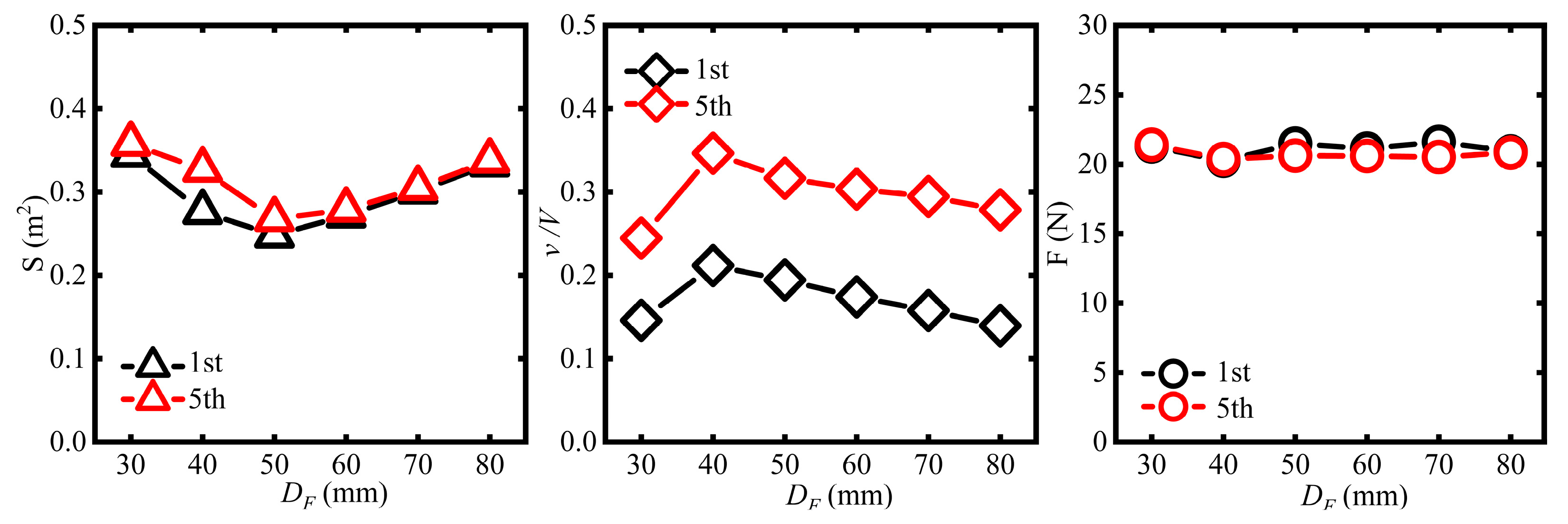
3.3. Influence of Balsa Wood Diameter on the Hydrodynamic Performance of Bio-DFADs
4. Conclusions
- The relative length LF/B and wave height H had a significant influence on the heave RAO, pitch RAO, and relative velocity of Bio-DFADs. The wave period T also has a significant influence on the relative velocity. For a lower heave RAO, pitch RAO, and relative velocity, a larger relative length (LF/B = 1.5) is recommended.
- Wave steepness and length had a significant influence on the heave RAO, pitch RAO, and relative velocity. As the wave period increased, the influence of the wavelength on the heave RAO, pitch RAO, and relative velocity became increasingly insignificant. For a lower heave RAO, pitch RAO, and relative velocity, a longer length (LF = 1.5 m) is recommended.
- The diameter of the balsa wood had a significant influence on the relative velocity and wetted area but had no significant influence on rope tension. Therefore, balsa wood with a smaller diameter (such as DF = 30 mm) should be selected based on the dimensional parameters of Bio-DFADs and regular wave conditions.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Imzilen, T.; Lett, C.; Chassot, E.; Maufroy, A.; Goujon, M.; Kaplan, D.M. Recovery at sea of abandoned, lost or discarded drifting fish aggregating devices. Nat. Sustain. 2022, 5, 593–602. [Google Scholar] [CrossRef]
- Maufroy, A.; Chassot, E.; Joo, R.; Kaplan, D.M. Large-Scale Examination of Spatio-Temporal Patterns of Drifting Fish Aggregating Devices (dFADs) from Tropical Tuna Fisheries of the Indian and Atlantic Oceans. PLoS ONE 2015, 10, e0128023. [Google Scholar] [CrossRef]
- Imzilen, T.; Lett, C.; Chassot, E.; Kaplan, D.M. Spatial management can significantly reduce dFAD beachings in Indian and Atlantic Ocean tropical tuna purse seine fisheries. Biol. Conserv. 2021, 254, 108939. [Google Scholar] [CrossRef]
- ISSF. Status of the World Fisheries for Tuna; ISSF Technical Report; International Seafood Sustainability Foundation: Pittsburgh, PA, USA, 2023. [Google Scholar]
- Bode, M.; Game, E.T.; Wegmann, A.; Pollock, K. Fish aggregating devices could enhance the effectiveness of blue water marine protected areas. Conserv. Lett. 2023, 16, e12984. [Google Scholar] [CrossRef]
- Escalle, L.; Hare, S.R.; Vidal, T.; Brownjohn, M.; Hamer, P.; Pilling, G. Quantifying drifting Fish Aggregating Device use by the world’s largest tuna fishery. ICES J. Mar. Sci. 2021, 78, 2432–2447. [Google Scholar] [CrossRef]
- Murua, J.; Moreno, G.; Hall, M.; Dagorn, L.; Itano, D.; Restrepo, V. Towards Global Non-Entangling Fish Aggregating Device (FAD) Use in Tropical Tuna Purse Seine Fisheries through a Participatory Approach; ISSF Technical Report; International Seafood Sustainability Foundation: Washington, DC, USA, 2017. [Google Scholar]
- Zudaire, I.; Moreno, G.; Murua, J.; Murua, H.; Tolotti, M.; Roman, M.; Hall, M.; Lopez, J.; Grande, M.; Merino, G.; et al. Biodegradable DFADs: Current Status and Prospects. In Proceedings of the 2nd IOTC Ad Hoc Working Group on FADs (WGFAD02) 2021, Online/Virtual, 4–6 October 2021; IOTC-2021-WGFAD02-09, 9p. Available online: https://archimer.ifremer.fr/doc/00727/83862/ (accessed on 9 February 2021).
- Zudaire, I.; Santiago, J.; Grande, M.; Murua, H.; Adam, P.A.; Nogués, P.; Collier, T.; Morgan, M.; Khan, N.; Baguette, F.; et al. FAD Watch: A collaborative initiative to minimize the impact of FADs in coastal ecosystems. In Proceedings of the 14th IOTC Working Party on Ecosystems and Bycatch 2018, Cape Town, South Africa, 10–14 September 2018. [Google Scholar]
- Moreno, G.; Salvador, J.; Zudaire, I.; Murua, J.; Pelegrí, J.L.; Uranga, J.; Murua, H.; Grande, M.; Santiago, J.; Restrepo, V. The jelly-FAD: A paradigm shift in the design of biodegradable fish aggregating devices. Mar. Policy 2022, 174, 105352. [Google Scholar] [CrossRef]
- Dagorn, L.; Holland, K.N.; Restrepo, V.; Moreno, G. Is it good or bad to fish with FADs? What are the real impacts of the use of drifting FADs on pelagic marine ecosystems? Fish Fish. 2013, 14, 391–415. [Google Scholar] [CrossRef]
- Pons, M.; Kaplan, D.; Moreno, G.; Escalle, L.; Abascal, F.; Hall, M.; Restrepo, V.; Hilborn, R. Benefits, concerns, and solutions of fishing for tunas with drifting fish aggregation devices. Fish Fish. 2023, 24, 979–1002. [Google Scholar] [CrossRef]
- Wan, R.; Zhang, T.; Zhou, C.; Zhao, F.; Wang, W. Experimental and numerical investigations of hydrodynamic response of biodegradable drifting Fish Aggregating Devices (FADs) in waves. Ocean Eng. 2022, 244, 110436. [Google Scholar] [CrossRef]
- Rahmawati, S.; Mutsuda, H.; Doi, Y.; Moriyama, Y. Characteristics of a fish aggregating device with ocean energy harvester. J. Mar. Sci. Technol. 2018, 23, 435–452. [Google Scholar] [CrossRef]
- Du, X.; Mutsuda, H.; Tanaka, Y.; Nakashima, T.; Kanehira, T.; Taniguchi, N.; Moriyama, Y. Experimental and numerical studies on working parameter selections of a piezoelectric-painted-based ocean energy harvester attached to fish aggregating devices. Energy Sustain. Dev. 2022, 71, 73–88. [Google Scholar] [CrossRef]
- Hou, T.; Sun, H.; Jiao, B.; Wang, G.; Lin, H.; Liu, H.; Gao, B. Numerical and experimental study of oil boom motion response and oil-stopping effect under wave-current action. Ocean Eng. 2024, 291, 116439. [Google Scholar] [CrossRef]
- Moreno, G.; Murua, J.; Kebe, P.; Scott, J.; Restrepo, V. Design Workshop on the Use of Biodegradable Fish Aggregating Devices in Ghanaian Purse Seine and Pole and Line Tuna Fleets; ISSF Technical Report; International Seafood Sustainability Foundation: Washington, DC, USA, 2018. [Google Scholar]
- Moreno, G.; Salvador, J.; Murua, J.; Phillip, N.B., Jr.; Murua, H.; Escalle, L.; Ashigbui, B.; Zudaire, I.; Pilling, G.; Restrepo, V. A multidisciplinary approach to build new designs of biodegradable Fish Aggregating Devices (FADs). In Scientific Committee Sixteenth Regular Session; WCPFC: Washington, DC, USA, 2020. [Google Scholar]
- Ferziger, J.H.; Perić, M. Computational Methods for Fluid Dynamics; Springer: New York, NY, USA, 2002. [Google Scholar]
- Lin, P.; Liu, P.L.F. A Numerical Study of Breaking Waves in the Surf Zone. J. Fluid Mech. 1998, 359, 239–264. [Google Scholar] [CrossRef]
- Rodi, W. Turbulence Models and Their Application in Hydraulics; Routledge: London, UK, 2000; p. 124. [Google Scholar] [CrossRef]
- Hirt, C.W.; Nichols, B.D. Volume of Fluid (VOF) method for the dynamics of free boundaries. J. Comput. Phys. 1981, 39, 201–225. [Google Scholar] [CrossRef]
- Ravenna, R.; Song, S.; Shi, W.; Sant, T.; Muscat-Fenech, C.D.M.; Tezdogan, T.; Demirel, Y.K. CFD analysis of the effect of heterogeneous hull roughness on ship resistance. Ocean Eng. 2022, 258, 111733. [Google Scholar] [CrossRef]
- Fenton, J.D. A fifth-order Stokes theory for steady waves. J. Waterw. Port Coast. Ocean Eng. 1985, 111, 216–234. [Google Scholar] [CrossRef]
- Kraskowski, M. Simulating hull dynamics in waves using a RANSE code. Ship Technol. Res. 2010, 57, 120–127. [Google Scholar] [CrossRef]
- Ha, T.; Lee, J.W.; Cho, Y.-S. Internal Wave Maker for Navier-Stokes Equations in a Three-Dimensional Numerical Model. J. Coast Res. 2011, 511–515. Available online: http://www.jstor.org/stable/26482225 (accessed on 9 May 2011).
- Kim, J.; O’Sullivan, J.; Read, A. Ringing analysis of a vertical cylinder by Euler overlay method. In: International Conference on Offshore Mechanics and Arctic Engineering. Am. Soc. Mech. Eng. 2012, 44915, 855–866. [Google Scholar] [CrossRef]
- Wang, X.; Xie, J.; Luo, Y.; Wang, X.; Guo, G.; You, X. Experimental Investigation of the Hydrodynamic Characteristics of Longline Aquaculture Facilities under Current and Wave Conditions. Fishes 2023, 8, 204. [Google Scholar] [CrossRef]
- Xu, P.; Zhang, Z.; Li, S.; Song, Q.; Liu, W. Numerical Investigation into the Dynamic Responses of Floating Photovoltaic Platform and Mooring Line Structures under Freak Waves. J. Mar. Sci. Eng. 2024, 12, 96. [Google Scholar] [CrossRef]
- Cutajar, C.; Sant, T.; Farrugia, R.N.; Buhagiar, D. Analysis of the Wave Attenuating and Dynamic Behaviour of a Floating Breakwater Integrating a Hydro-Pneumatic Energy Storage System. J. Mar. Sci. Eng. 2023, 11, 2189. [Google Scholar] [CrossRef]
- Gao, Q.; Song, L.; Yao, J. RANS Prediction of Wave-Induced Ship Motions, and Steady Wave Forces and Moments in Regular Waves. J. Mar. Sci. Eng. 2021, 9, 1459. [Google Scholar] [CrossRef]
- Dai, J.; Wang, C.M.; Utsunomiya, T.; Duan, W. Review of recent research and developments on floating breakwaters. Ocean Eng. 2018, 158, 132–151. [Google Scholar] [CrossRef]
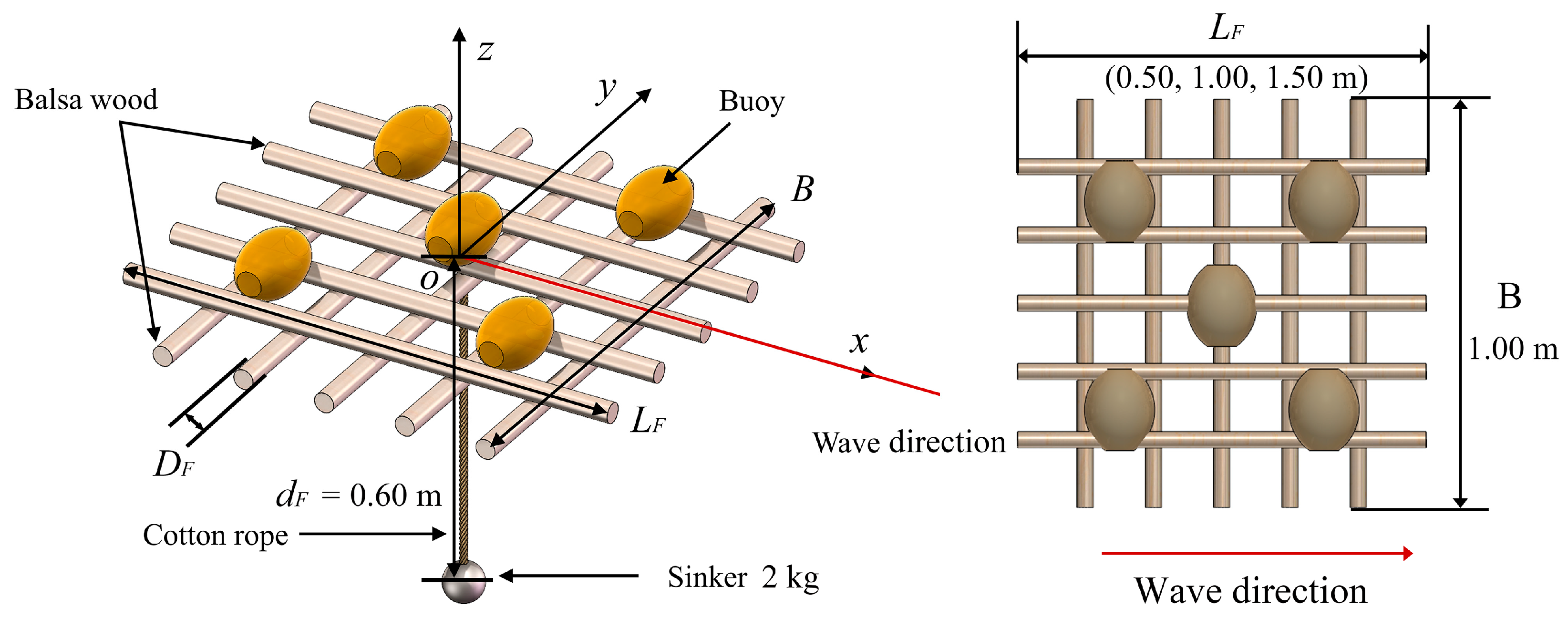




| Main Characteristics | Parameter | Value (m) |
|---|---|---|
| Lengths of Bio-DFADs | LF | 0.50, 1.00, 1.50 |
| Width of Bio-DFADs | B | 1.00 |
| Diameters of balsa wood | DF | 0.03, 0.04, 0.05, 0.06, 0.07, 0.08 |
| Length of rope | dF | 0.60 |
| Model No. | LF (m) | DF (m) | Sinker (kg) |
|---|---|---|---|
| 1 | 0.50 | 0.04 | 2.00 |
| 2 | 1.00 | 0.04 | 2.00 |
| 3 | 1.50 | 0.04 | 2.00 |
| 4 | 1.50 | 0.03 | 2.00 |
| 5 | 1.50 | 0.05 | 2.00 |
| 6 | 1.50 | 0.06 | 2.00 |
| 7 | 1.50 | 0.07 | 2.00 |
| 8 | 1.50 | 0.08 | 2.00 |
| DF (m) | 0.03 | 0.04 | 0.05 | 0.06 | 0.07 | 0.08 | |
|---|---|---|---|---|---|---|---|
| LF (m) | |||||||
| 0.50 | 182.32 N | 216.30 N | 259.98 N | 313.38 N | 376.48 N | 449.30 N | |
| 1.00 | 196.88 N | 242.19 N | 300.44 N | 371.62 N | 455.77 N | 552.85 N | |
| 1.50 | 211.44 N | 268.07 N | 340.88 N | 429.87 N | 535.05 N | 656.40 N | |
| Wave Type | Wave Period T (s) | Wave Height H (m) |
|---|---|---|
| First-order wave | 1.5 | 0.10 |
| 2.0 | 0.15 | |
| 2.5 | 0.20 | |
| 1.5 | 0.10 | |
| Fifth-order wave | 2.0 | 0.15 |
| 2.5 | 0.20 |
| Region | Time Step (s) | Cell Number (N) |
|---|---|---|
| Wave height (1 H) | 0.005 | 10, 20, 40 |
| Wavelength (1 L) | 0.005 | 44, 88, 176 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhang, T.; Liu, Z.; Zhang, J.; Su, X.; Chen, J.; Wan, R. Numerical Study of the Hydrodynamic Response of Biodegradable Drifting Fish Aggregating Devices in Regular Waves. Fishes 2024, 9, 112. https://doi.org/10.3390/fishes9040112
Zhang T, Liu Z, Zhang J, Su X, Chen J, Wan R. Numerical Study of the Hydrodynamic Response of Biodegradable Drifting Fish Aggregating Devices in Regular Waves. Fishes. 2024; 9(4):112. https://doi.org/10.3390/fishes9040112
Chicago/Turabian StyleZhang, Tongzheng, Zhiqiang Liu, Junbo Zhang, Xing Su, Junlin Chen, and Rong Wan. 2024. "Numerical Study of the Hydrodynamic Response of Biodegradable Drifting Fish Aggregating Devices in Regular Waves" Fishes 9, no. 4: 112. https://doi.org/10.3390/fishes9040112
APA StyleZhang, T., Liu, Z., Zhang, J., Su, X., Chen, J., & Wan, R. (2024). Numerical Study of the Hydrodynamic Response of Biodegradable Drifting Fish Aggregating Devices in Regular Waves. Fishes, 9(4), 112. https://doi.org/10.3390/fishes9040112







