Network Topology of Wing Veins in Hawaiian Flies Mitigates Allometric Dilemma
Abstract
1. Introduction
2. Materials and Methods
2.1. Characterization of the Forewing of the Hawaiian Fly
2.2. Calculation of the Hemolymph Flow
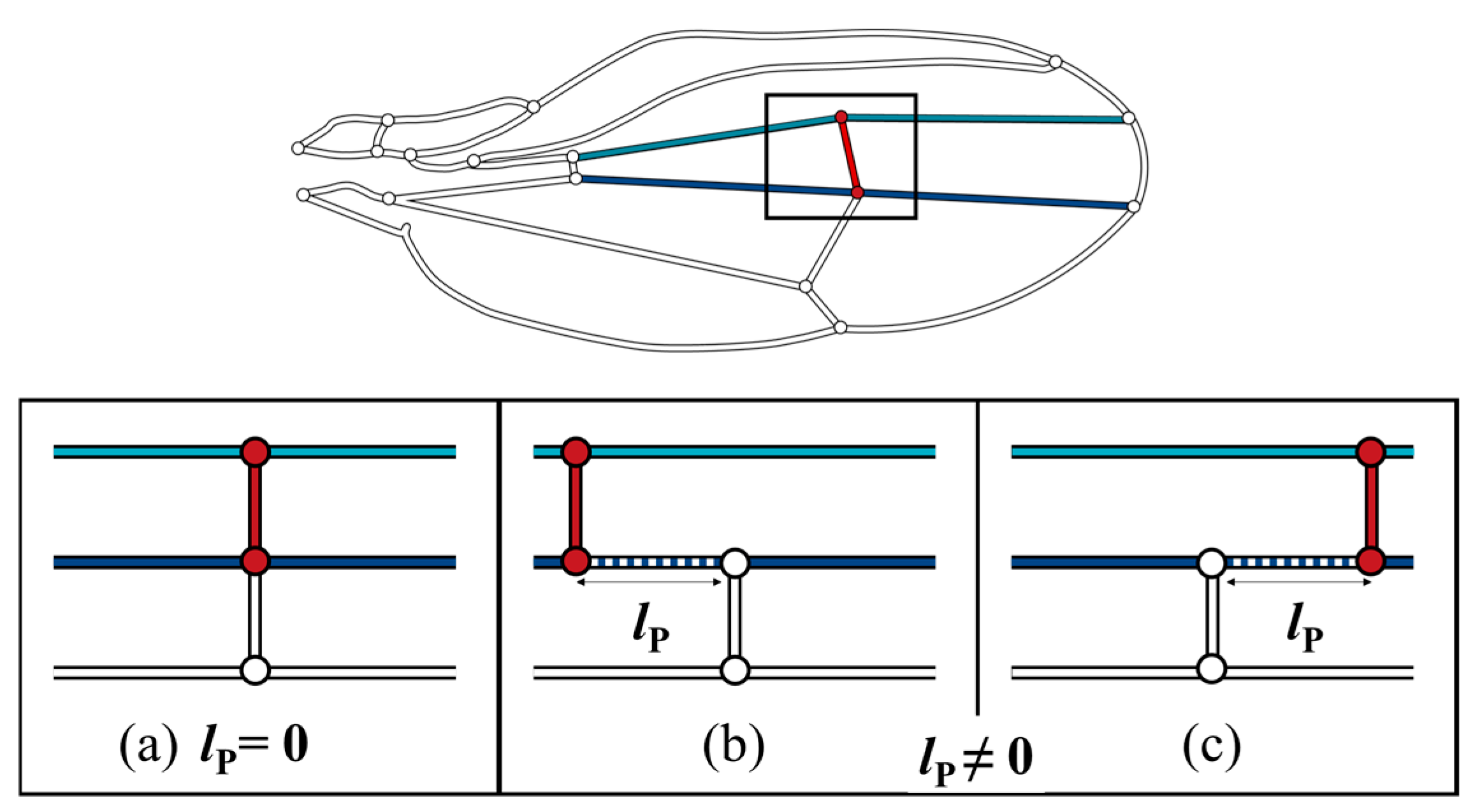
2.3. Network Modeling for the Repositioning of the ECV
3. Results
3.1. Effect of the ECV’s Presence on Hemolymph Flow
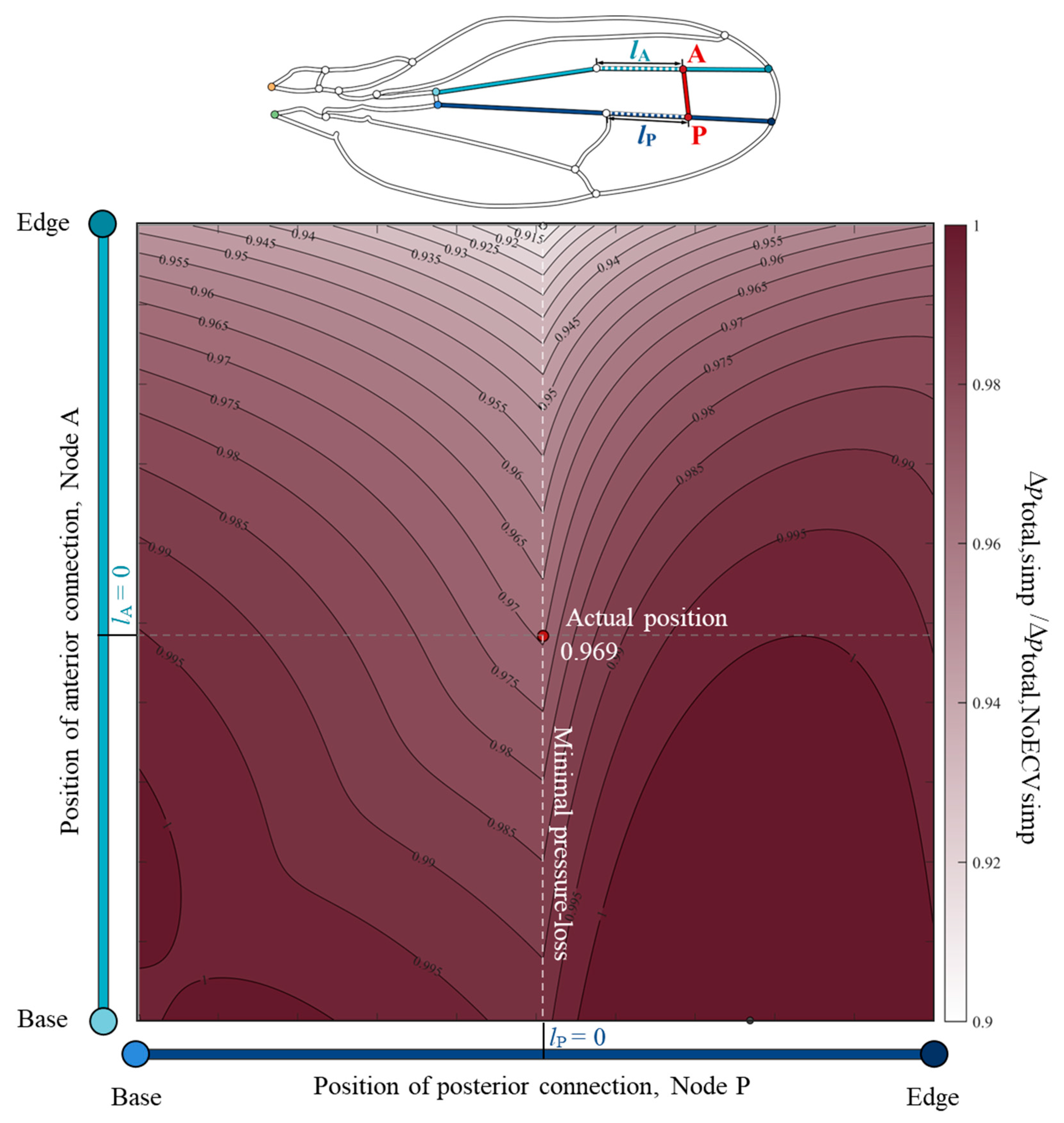
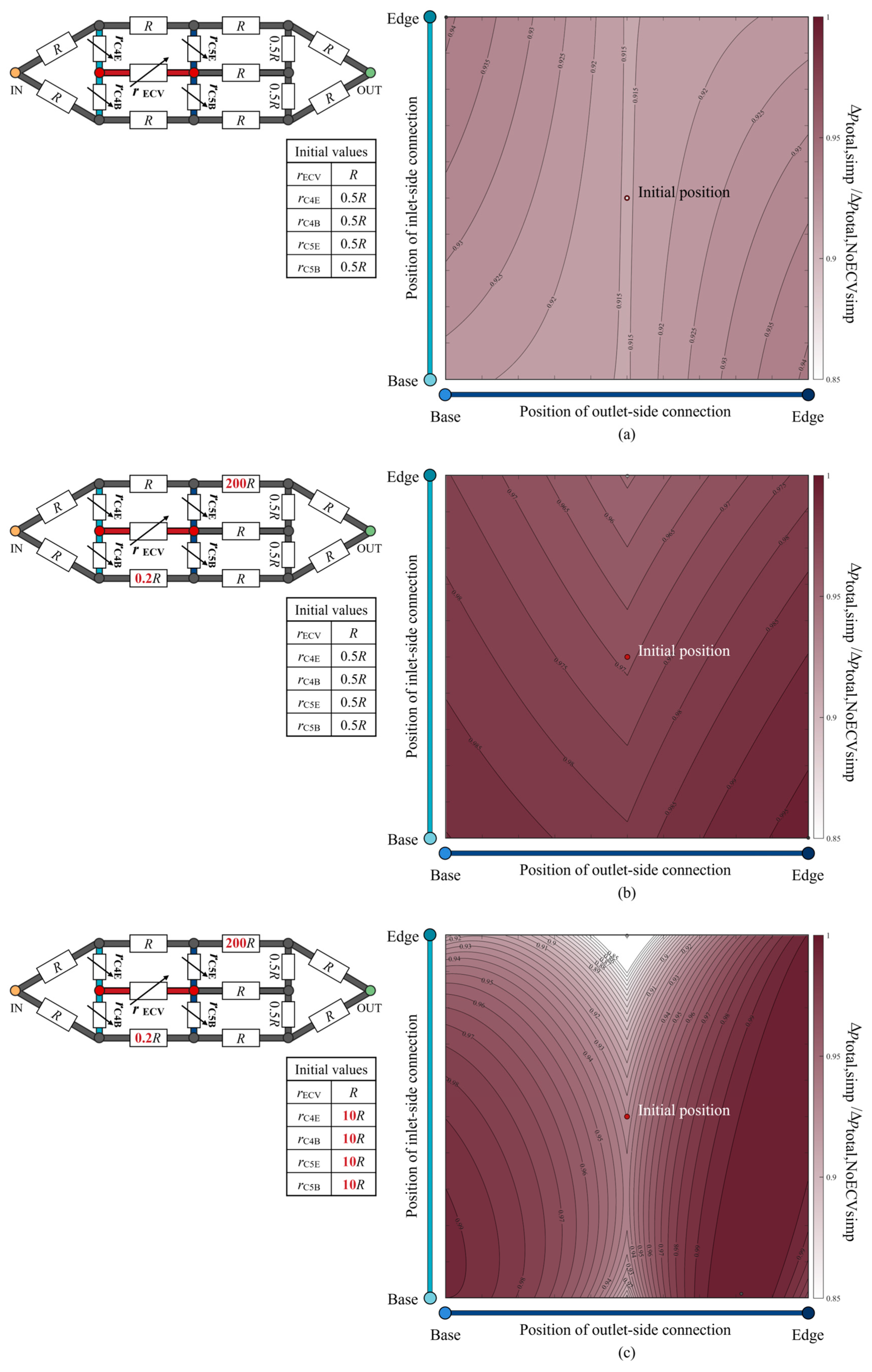
3.2. Positional Effect of the ECV
4. Discussion
4.1. How the Presence of the ECV Reduced the Total Pressure Loss
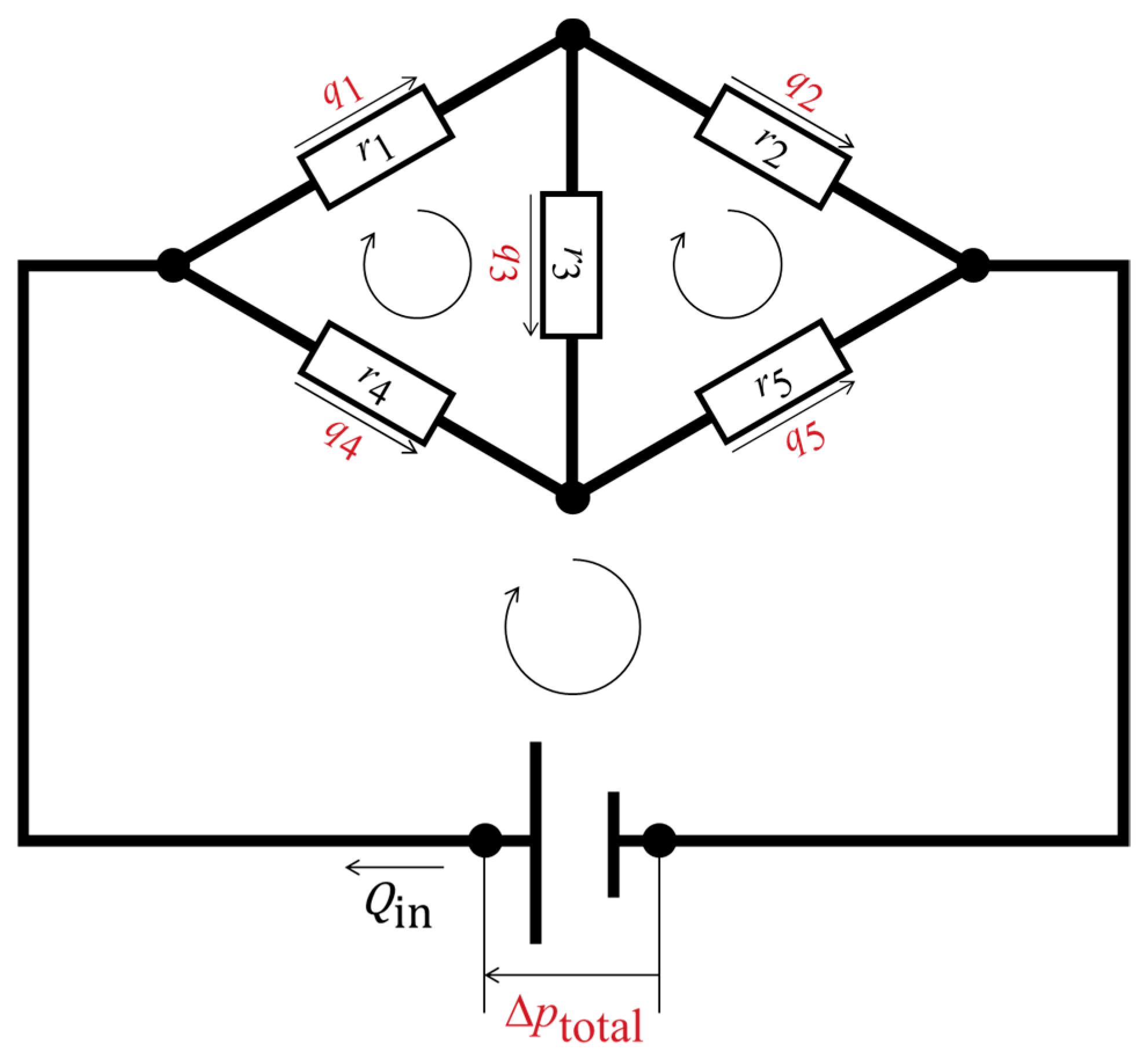
4.2. Demonstration of the Hemodynamic Effects of the Presence of the ECV in Idealized Circuits
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Arnold, J.W. Blood Circulation in Insect Wings. Mem. Entomol. Soc. Can. 1964, 96, 5–60. [Google Scholar] [CrossRef]
- Chintapalli, R.T.V.; Hillyer, J.F. Hemolymph Circulation in Insect Flight Appendages: Physiology of the Wing Heart and Circulatory Flow in the Wings of the Mosquito Anopheles gambiae. J. Exp. Biol. 2016, 219, 3945–3951. [Google Scholar] [CrossRef] [PubMed]
- Salcedo, M.K.; Jun, B.H.; Socha, J.J.; Pierce, N.E.; Vlachos, P.P.; Combes, S.A. Complex Hemolymph Circulation Patterns in Grasshopper Wings. Commun. Biol. 2023, 6, 313. [Google Scholar] [CrossRef]
- Pass, G.; Tögel, M.; Krenn, H.; Paululat, A. The Circulatory Organs of Insect Wings: Prime Examples for the Origin of Evolutionary Novelties. Zool. Anz. J. Comp. Zool. 2015, 256, 82–95. [Google Scholar] [CrossRef]
- Hillyer, J.F.; Pass, G. The Insect Circulatory System: Structure, Function, and Evolution. Annu. Rev. Entomol. 2020, 65, 121–143. [Google Scholar] [CrossRef] [PubMed]
- Salcedo, M.K.; Socha, J.J. Circulation in Insect Wings. Integr. Comp. Biol. 2020, 60, 1208–1220. [Google Scholar] [CrossRef] [PubMed]
- Mengesha, T.E.; Vallance, R.R.; Mittal, R. Stiffness of Desiccating Insect Wings. Bioinspir. Biomim. 2011, 6, 014001. [Google Scholar] [CrossRef] [PubMed]
- Lietz, C.; Schaber, C.F.; Gorb, S.N.; Rajabi, H. The Damping and Structural Properties of Dragonfly and Damselfly Wings during Dynamic Movement. Commun. Biol. 2021, 4, 737. [Google Scholar] [CrossRef] [PubMed]
- Munson, B.R. Fundamentals of Fluid Mechanics; John Wiley & Sons, Inc.: Hoboken, NJ, USA, 2002. [Google Scholar]
- Schachat, S.R.; Boyce, C.K.; Payne, J.L.; Lentink, D. Lepidoptera Demonstrate the Relevance of Murray’s Law to Circulatory Systems with Tidal Flow. BMC Biol. 2021, 19, 204. [Google Scholar] [CrossRef]
- Sugiyama, K.; Kubota, Y.; Mochizuki, O. Circuit Analogy Unveiled the Haemodynamic Effects of the Posterior Cross Vein in the Wing Vein Networks. PLoS ONE 2024, 19, e0301030. [Google Scholar] [CrossRef]
- Edwards, K.A.; Doescher, L.T.; Kaneshiro, K.Y.; Yamamoto, D. A Database of Wing Diversity in the Hawaiian Drosophila. PLoS ONE 2007, 2, e487. [Google Scholar] [CrossRef] [PubMed]
- Carson, H.L.; Clayton, F.E.; Stalker, H.D. Karyotypic Stability and Speciation in Hawaiian Drosophila. Proc. Natl. Acad. Sci. USA 1967, 57, 1280–1285. [Google Scholar] [CrossRef] [PubMed]
- Torquato, L.S.; Mattos, D.; Matta, B.P.; Bitner-Mathé, B.C. Cellular Basis of Morphological Variation and Temperature-Related Plasticity in Drosophila melanogaster Strains with Divergent Wing Shapes. Genetica 2014, 142, 495–505. [Google Scholar] [CrossRef] [PubMed]
- Church, S.H.; Extavour, C.G. Phylotranscriptomics Reveals Discordance in the Phylogeny of Hawaiian Drosophila and Scaptomyza (Diptera: Drosophilidae). Mol. Biol. Evol. 2022, 39, msac012. [Google Scholar] [CrossRef] [PubMed]
- Spieth, H.T. Courthip Patterns and Evolution of Drosophila adiastola and planitibia Species Subgroup. Evolution 1978, 32, 435–451. [Google Scholar] [CrossRef] [PubMed]
- Shingleton, A.W.; Mirth, C.K.; Bates, P.W. Developmental Model of Static Allometry in Holometabolous Insects. Proc. R. Soc. B Biol. Sci. 2008, 275, 1875–1885. [Google Scholar] [CrossRef] [PubMed]
- Craddock, E.M.; Kambysellis, M.P.; Franchi, L.; Francisco, P.; Grey, M.; Hutchinson, A.; Nanhoo, S.; Antar, S. Ultrastructural Variation and Adaptive Evolution of the Ovipositor in the Endemic Hawaiian Drosophilidae. J. Morphol. 2018, 279, 1725–1752. [Google Scholar] [CrossRef] [PubMed]
- Santos, M.; Fowler, K.; Partridge, L. Gene–Environment Interaction for Body Size and Larval Density in Drosophila melanogaster: An Investigation of Effects on Development Time, Thorax Length and Adult Sex Ratio. Heredity 1994, 72, 515–521. [Google Scholar] [CrossRef] [PubMed]
- Tögel, M.; Pass, G.; Paululat, A. In Vivo Imaging of Drosophila Wing Heart Development during Pupal Stages. Int. J. Dev. Biol. 2013, 57, 13–24. [Google Scholar] [CrossRef]
- Tögel, M.; Meyer, H.; Lehmacher, C.; Heinisch, J.J.; Pass, G.; Paululat, A. The bHLH Transcription Factor Hand Is Required for Proper Wing Heart Formation in Drosophila. Dev. Biol. 2013, 381, 446–459. [Google Scholar] [CrossRef]
- Steinbrecht, R.A. Freeze-Substitution for Morphological and Immunocytochemical Studies in Insects. Microsc. Res. Tech. 1993, 24, 488–504. [Google Scholar] [CrossRef] [PubMed]
- Zabihihesari, A.; Parand, S.; Rezai, P. PDMS-Based Microfluidic Capillary Pressure-Driven Viscometry and Application to Drosophila melanogaster’s Hemolymph. Microfluid. Nanofluid. 2023, 27, 8. [Google Scholar] [CrossRef]
- Kenny, M.C.; Giarra, M.N.; Granata, E.; Socha, J.J. How Temperature Influences the Viscosity of Hornworm Hemolymph. J. Exp. Biol. 2018, 221, jeb186338. [Google Scholar] [CrossRef] [PubMed]
- Brasovs, A.; Palaoro, A.V.; Aprelev, P.; Beard, C.E.; Adler, P.H.; Kornev, K.G. Haemolymph Viscosity in Hawkmoths and Its Implications for Hovering Flight. Proc. R. Soc. B Biol. Sci. 2023, 290, 20222185. [Google Scholar] [CrossRef] [PubMed]
- Oh, K.W.; Lee, K.; Ahn, B.; Furlani, E.P. Design of Pressure-Driven Microfluidic Networks Using Electric Circuit Analogy. Lab Chip 2012, 12, 515–545. [Google Scholar] [CrossRef] [PubMed]
- Gompper, G.; Fedosov, D.A. Modeling Microcirculatory Blood Flow: Current State and Future Perspectives. WIREs Syst. Biol. Med. 2016, 8, 157–168. [Google Scholar] [CrossRef]
- Rousset, N.; Lohasz, C.; Boos, J.A.; Misun, P.M.; Cardes, F.; Hierlemann, A. Circuit-Based Design of Microfluidic Drop Networks. Micromachines 2022, 13, 1124. [Google Scholar] [CrossRef]
- Wang, Y.; Yin, Y.; Zheng, G.; Yao, H. Driving Mechanism of Dragonfly’s Wing Flapping Pattern for Liquid Circulation inside Wing. Anim. Biol. 2020, 71, 85–101. [Google Scholar] [CrossRef]
- Muijres, F.T.; Elzinga, M.J.; Melis, J.M.; Dickinson, M.H. Flies Evade Looming Targets by Executing Rapid Visually Directed Banked Turns. Science 2014, 344, 172–177. [Google Scholar] [CrossRef]
- Chen, L.; Wu, J. Coexistence of dual wing–wake interaction mechanisms during the rapid rotation of flapping wings. J. Fluid Mech. 2024, 987, A16. [Google Scholar] [CrossRef]
- Bergou, A.J.; Ristroph, L.; Guckenheimer, J.; Cohen, I.; Wang, Z.J. Fruit Flies Modulate Passive Wing Pitching to Generate In-Flight Turns. Phys. Rev. Lett. 2010, 104, 148101. [Google Scholar] [CrossRef] [PubMed]
- Vincent, J.F.V.; Wegst, U.G.K. Design and Mechanical Properties of Insect Cuticle. Arthropod Struct. Dev. 2004, 33, 187–199. [Google Scholar] [CrossRef] [PubMed]
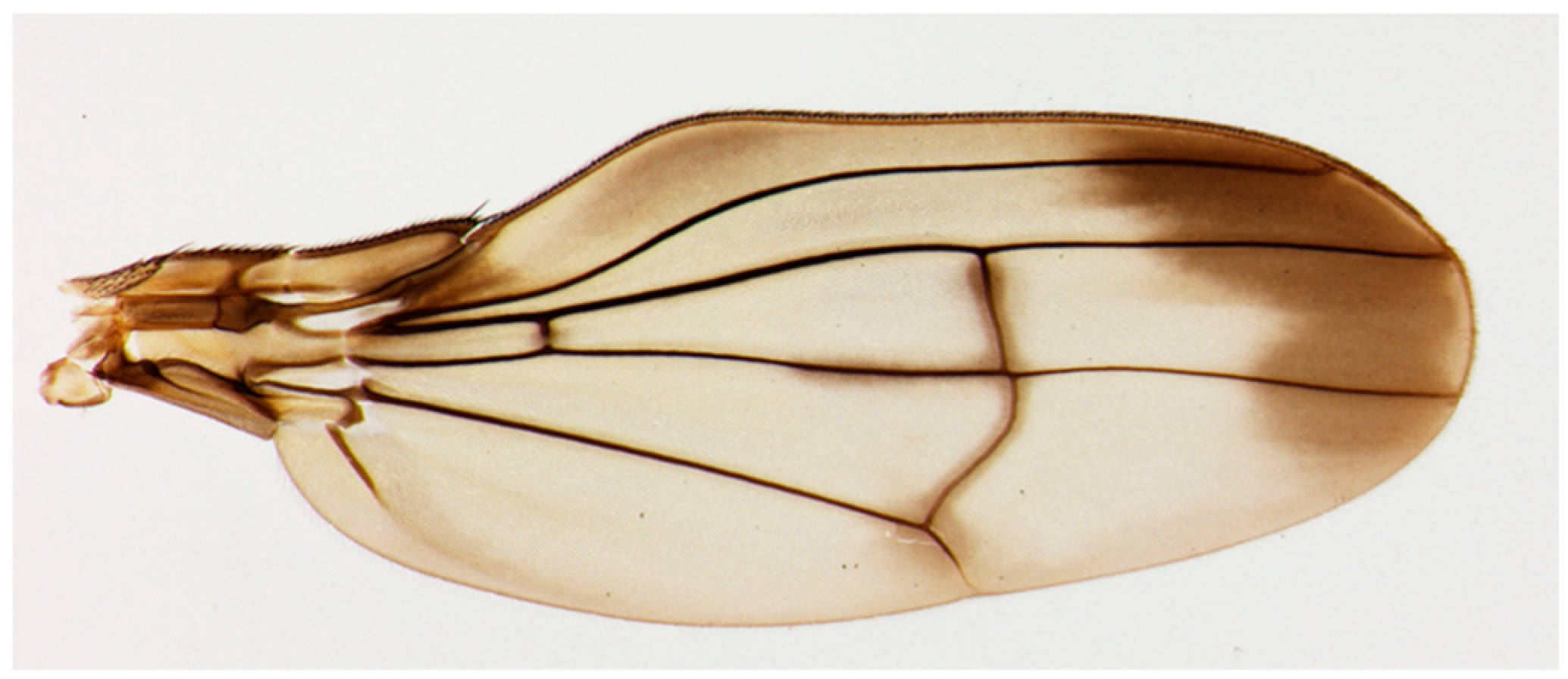
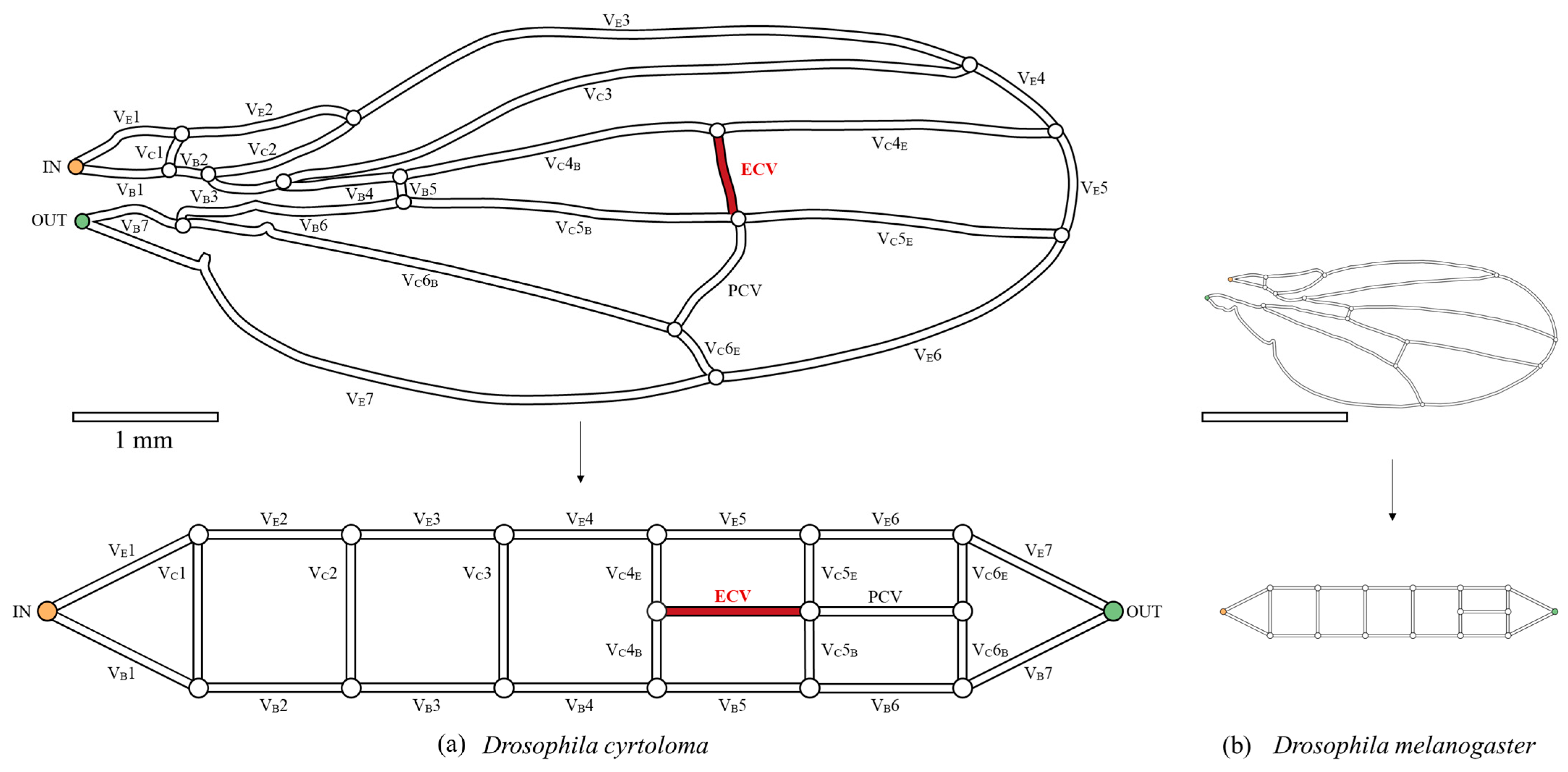



| Vein | Length: ln [mm] | Outer Diameter [mm] | Inner Diameter: dn [mm] | ||
|---|---|---|---|---|---|
| VE1 | 8.9 × 10−1 | ±0.028 | 7.6 × 10−2 | ±0.028 | 1.5 × 10−2 |
| VE2 | 1.2 × 100 | ±0.0092 | 6.0 × 10−2 | ±0.038 | 1.2 × 10−2 |
| VE3 | 4.5 × 100 | ±0.0024 | 5.5 × 10−2 | ±0.012 | 1.1 × 10−2 |
| VE4 | 7.7 × 10−1 | ±0.0050 | 4.3 × 10−2 | ±0.012 | 8.6 × 10−3 |
| VE5 | 7.4 × 10−1 | ±0.0017 | 2.6 × 10−2 | ±0.0027 | 5.2 × 10−3 |
| VE6 | 2.7 × 100 | ±0.0088 | 1.5 × 10−2 | ±0.0015 | 2.9 × 10−3 |
| VE7 | 4.9 × 100 | ±0.038 | 1.9 × 10−2 | ±0.0074 | 3.8 × 10−3 |
| VB1 | 5.8 × 10−1 | ±0.0022 | 1.6 × 10−1 | ±0.013 | 3.2 × 10−2 |
| VB2 | 2.5 × 10−1 | ±0.0019 | 1.4 × 10−1 | ±0.040 | 2.8 × 10−2 |
| VB3 | 5.5 × 10−1 | ±0.015 | 3.5 × 10−2 | ±0.0057 | 7.0 × 10−3 |
| VB4 | 8.3 × 10−1 | ±0.0057 | 4.2 × 10−2 | ±0.0029 | 8.3 × 10−3 |
| VB5 | 1.6 × 10−1 | ±0.0026 | 4.0 × 10−2 | ±0.0046 | 7.9 × 10−3 |
| VB6 | 1.6 × 100 | ±0.031 | 3.5 × 10−2 | ±0.0020 | 7.0 × 10−3 |
| VB7 | 7.2 × 10−1 | ±0.042 | 5.2 × 10−2 | ±0.016 | 1.0 × 10−2 |
| VC1 | 2.6 × 10−1 | ±0.024 | 1.1 × 10−1 | ±0.042 | 2.3 × 10−2 |
| VC2 | 1.2 × 100 | ±0.0017 | 7.9 × 10−2 | ±0.029 | 1.6 × 10−2 |
| VC3 | 5.0 × 100 | ±0.015 | 3.7 × 10−2 | ±0.0073 | 7.4 × 10−3 |
| VC4E | 2.4 × 100 | ±0.016 | 3.0 × 10−2 | ±0.0058 | 6.1 × 10−3 |
| VC4B | 2.3 × 100 | ±0.0045 | 4.8 × 10−2 | ±0.0067 | 9.5 × 10−3 |
| VC5E | 2.3 × 100 | ±0.0047 | 2.3 × 10−2 | ±0.0019 | 4.6 × 10−3 |
| VC5B | 2.3 × 100 | ±0.0047 | 3.0 × 10−2 | ±0.0050 | 6.0 × 10−3 |
| VC6E | 4.4 × 10−1 | ±0.0096 | 2.1 × 10−2 | ±0.0095 | 4.2 × 10−3 |
| VC6B | 3.5 × 100 | ±0.0046 | 3.0 × 10−2 | ±0.0070 | 6.0 × 10−3 |
| ECV | 6.2 × 10−1 | ±0.0038 | 3.7 × 10−2 | ±0.0036 | 7.4 × 10−3 |
| PCV | 9.3 × 10−1 | ±0.0061 | 3.5 × 10−2 | ±0.0029 | 7.0 × 10−3 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Sugiyama, K.; Kubota, Y.; Mochizuki, O. Network Topology of Wing Veins in Hawaiian Flies Mitigates Allometric Dilemma. Biomimetics 2024, 9, 451. https://doi.org/10.3390/biomimetics9080451
Sugiyama K, Kubota Y, Mochizuki O. Network Topology of Wing Veins in Hawaiian Flies Mitigates Allometric Dilemma. Biomimetics. 2024; 9(8):451. https://doi.org/10.3390/biomimetics9080451
Chicago/Turabian StyleSugiyama, Kazuki, Yoshihiro Kubota, and Osamu Mochizuki. 2024. "Network Topology of Wing Veins in Hawaiian Flies Mitigates Allometric Dilemma" Biomimetics 9, no. 8: 451. https://doi.org/10.3390/biomimetics9080451
APA StyleSugiyama, K., Kubota, Y., & Mochizuki, O. (2024). Network Topology of Wing Veins in Hawaiian Flies Mitigates Allometric Dilemma. Biomimetics, 9(8), 451. https://doi.org/10.3390/biomimetics9080451






