Ecuadorian Woods: Building Material Selection Using an Entropy-COPRAS Comparative Analysis Based on the Characterization of Ecuadorian Oak and Guayacan Timber
Abstract
1. Introduction
2. Materials and Methods
2.1. Characterization of Ecuadorian Oak and Guayacan Timber
2.2. Thermal Tests
2.3. Mechanical Test
2.3.1. Parallel Compression
2.3.2. Perpendicular Compression
2.3.3. Bending Test
2.3.4. Tension Test
2.4. Moisture Content (MC) Tests
2.5. Multicriteria Decision Methods
Entropy-Weighted COPRAS Method
- These criteria are normalized and then calculated in entropy Equation (14).
- 2.
- The last step weighs each criterion with Equation (15) [37].
- The weighted matrix is found by multiplying each objective value by the weighted value found in the entropy method, as shown in Equation (17).
- 2.
- The weighted normalized scores are calculated considering the maximization of the objectives with Equation (18). This means that for all the criteria a higher score is better, except for the thermal conductivity where a lower score is better. Equation (19) refers to minimizations.
- 3.
- The calculation of comparative significance Qi determinates the relative importance of each alternative, as shown in Equation (20).
- 4.
3. Results and Discussion
3.1. Thermal Characterization of Guayacan and Ecuadorian Oak
3.2. Mechanical Tests of Ecuadorian Oaks and Guayacan Timber
3.3. Moisture Content of Ecuadorian Oaks and Guayacan Timber
3.4. Comparison by Entropy-COPRAS MCDM
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
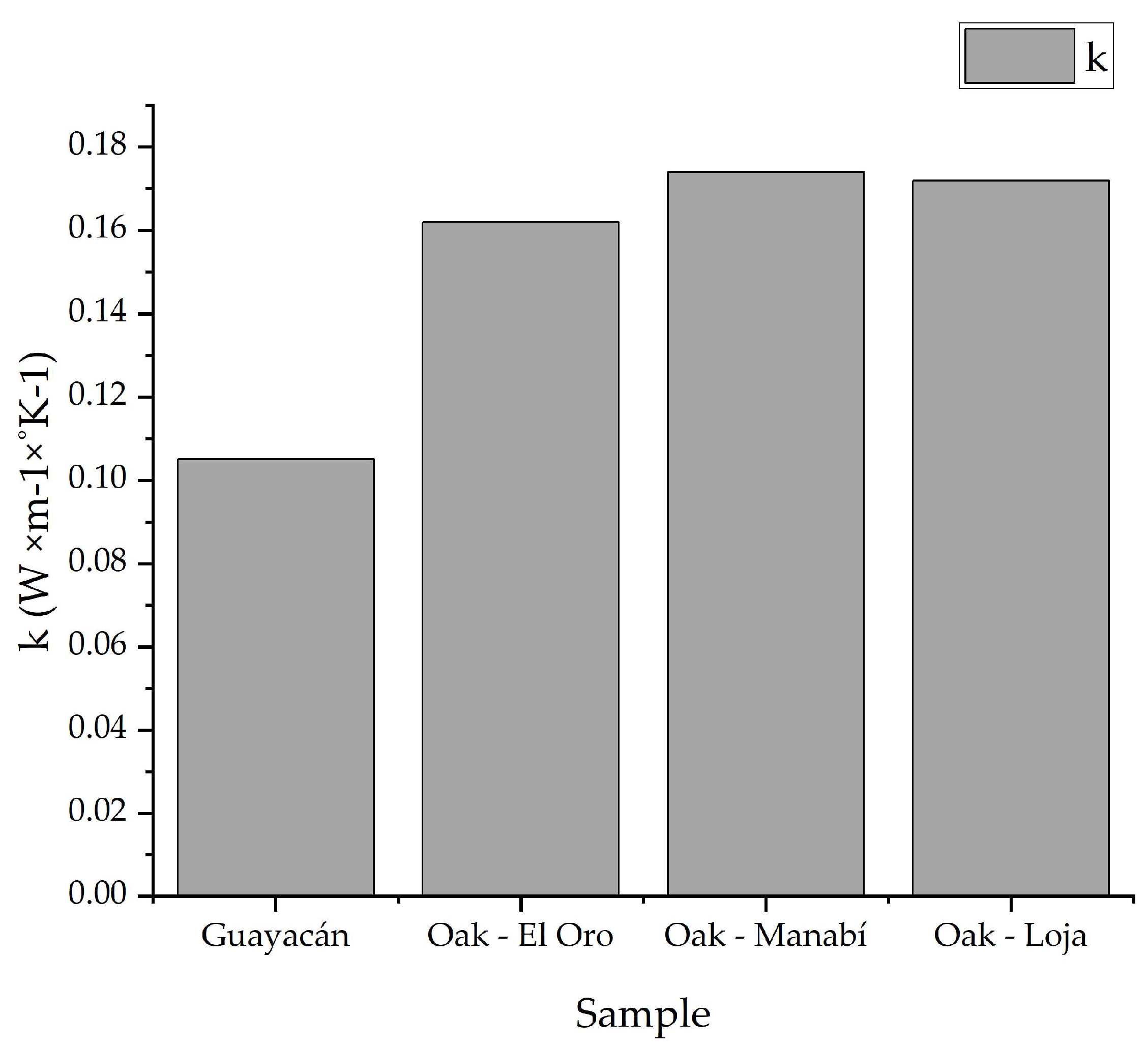
Appendix A.1. Thermal Conductivity Results
| Sample | k (W × m−1 × K−1) |
|---|---|
| G1TC | 0.092 |
| G2TC | 0.115 |
| G3TC | 0.109 |
| Average | 0.105 |
| Std. Deviation | 0.009 |
| Sample | k (W × m−1 × K−1) |
|---|---|
| O1TC-ElOro | 0.176 |
| O2TC-ElOro | 0.142 |
| O3TC-ElOro | 0.169 |
| Average | 0.162 |
| Std. Deviation | 0.014 |
| Sample | k (W × m−1 × K−1) |
|---|---|
| O1TC-Manabi | 0.174 |
| O2TC-Manabi | 0.181 |
| O3TC-Manabi | 0.168 |
| Average | 0.174 |
| Std. Deviation | 0.005 |
| Sample | k (W × m−1 × K−1) |
|---|---|
| O1TC-Loja | 0.168 |
| O2TC-Loja | 0.174 |
| O3TC-Loja | 0.176 |
| Average | 0.172 |
| Std. Deviation | 0.003 |
Appendix A.2. Mechanical Results
| Sample | E (GPa) | Sample | σc (MPa) | Sample | σb (MPa) | Sample | σt (MPa) |
|---|---|---|---|---|---|---|---|
| G1PC | 46.43 | G1PrC | 7.35 | G1B | 56.62 | G1T | 70.79 |
| G2PC | 56.15 | G2PrC | 6.93 | G2B | 65.13 | G2T | 91.38 |
| G3PC | 41.20 | G3PrC | 7.57 | G3B | 54.94 | G3T | 74.03 |
| G4PC | 36.20 | G4PrC | 6.81 | G4B | 57.27 | G4T | 99.34 |
| G5PC | 49.21 | G5PrC | 7.34 | G5B | 56.48 | G5T | 64.81 |
| Average | 45.33 | 7.19 | 57.98 | 79.03 | |||
| Std. Deviation | 7.621 | 0.32 | 4.03 | 14.62 |
| Sample | E (GPa) | Sample | σc (MPa) | Sample | σb (MPa) | Sample | σt (MPa) |
|---|---|---|---|---|---|---|---|
| O1PC-ElOro | 92.84 | O1PrC-ElOro | 20.49 | O1B-ElOro | 86.05 | O1T-ElOro | 191.25 |
| O2PC-ElOro | 99.75 | O2PrC-ElOro | 20.46 | O2B-ElOro | 88.18 | O2T-ElOro | 173.54 |
| O3PC-ElOro | 72.48 | O3PrC-ElOro | 19.34 | O3B-ElOro | 89.80 | O3T-ElOro | 177.50 |
| O4PC-ElOro | 72.4 | O4PrC-ElOro | 19.23 | O4B-ElOro | 86.73 | O4T-ElOro | 201.88 |
| O5PC-ElOro | 86.22 | O5PrC-ElOro | 20.62 | O5B-ElOro | 82.56 | O5T-ElOro | 202.92 |
| Average | 84.73 | 20.02 | 86.66 | 189.41 | |||
| Std. Deviation | 10.91 | 0.61 | 2.42 | 12.12 |
| Sample | E (GPa) | Sample | σc (MPa) | Sample | σb (MPa) | Sample | σt (MPa) |
|---|---|---|---|---|---|---|---|
| O1PC-Manabi | 92.55 | O1PrC-Manabi | 20.44 | O1B-Manabi | 86.31 | O1T-Manabi | 200.63 |
| O2PC-Manabi | 113.69 | O2PrC-Manabi | 20.39 | O2B-Manabi | 88.35 | O2T-Manabi | 192.71 |
| O3PC-Manabi | 118.41 | O3PrC-Manabi | 20.12 | O3B-Manabi | 77.11 | O3T-Manabi | 158.13 |
| O4PC-Manabi | 91.36 | O4PrC-Manabi | 20.20 | O4B-Manabi | 85.63 | O4T-Manabi | 167.08 |
| O5PC-Manabi | 128.86 | O5PrC-Manabi | 20.69 | O5B-Manabi | 82.90 | O5T-Manabi | 169.38 |
| Average | 108.9 | 20.36 | 84.06 | 177.58 | |||
| Std. Deviation | 14.74 | 0.19 | 3.88 | 16.22 |
| Sample | E (GPa) | Sample | σc (MPa) | Sample | σb (MPa) | Sample | σt (MPa) |
|---|---|---|---|---|---|---|---|
| O1PC-Loja | 171.95 | O1PrC-Loja | 20.20 | O1B-Loja | 79.07 | O1T-Loja | 171.04 |
| O2PC-Loja | 193.66 | O2PrC-Loja | 20.42 | O2B-Loja | 81.88 | O2T-Loja | 182.08 |
| O3PC-Loja | 151.36 | O3PrC-Loja | 19.08 | O3B-Loja | 80.43 | O3T-Loja | 148.75 |
| O4PC-Loja | 167.60 | O4PrC-Loja | 19.49 | O4B-Loja | 77.62 | O4T-Loja | 173.96 |
| O5PC-Loja | 191.72 | O5PrC-Loja | 20.52 | O5B-Loja | 84.35 | O5T-Loja | 157.92 |
| Average | 175.25 | 19.94 | 80.67 | 166.75 | |||
| Std. Deviation | 15.81 | 0.56 | 2.32 | 11.89 |
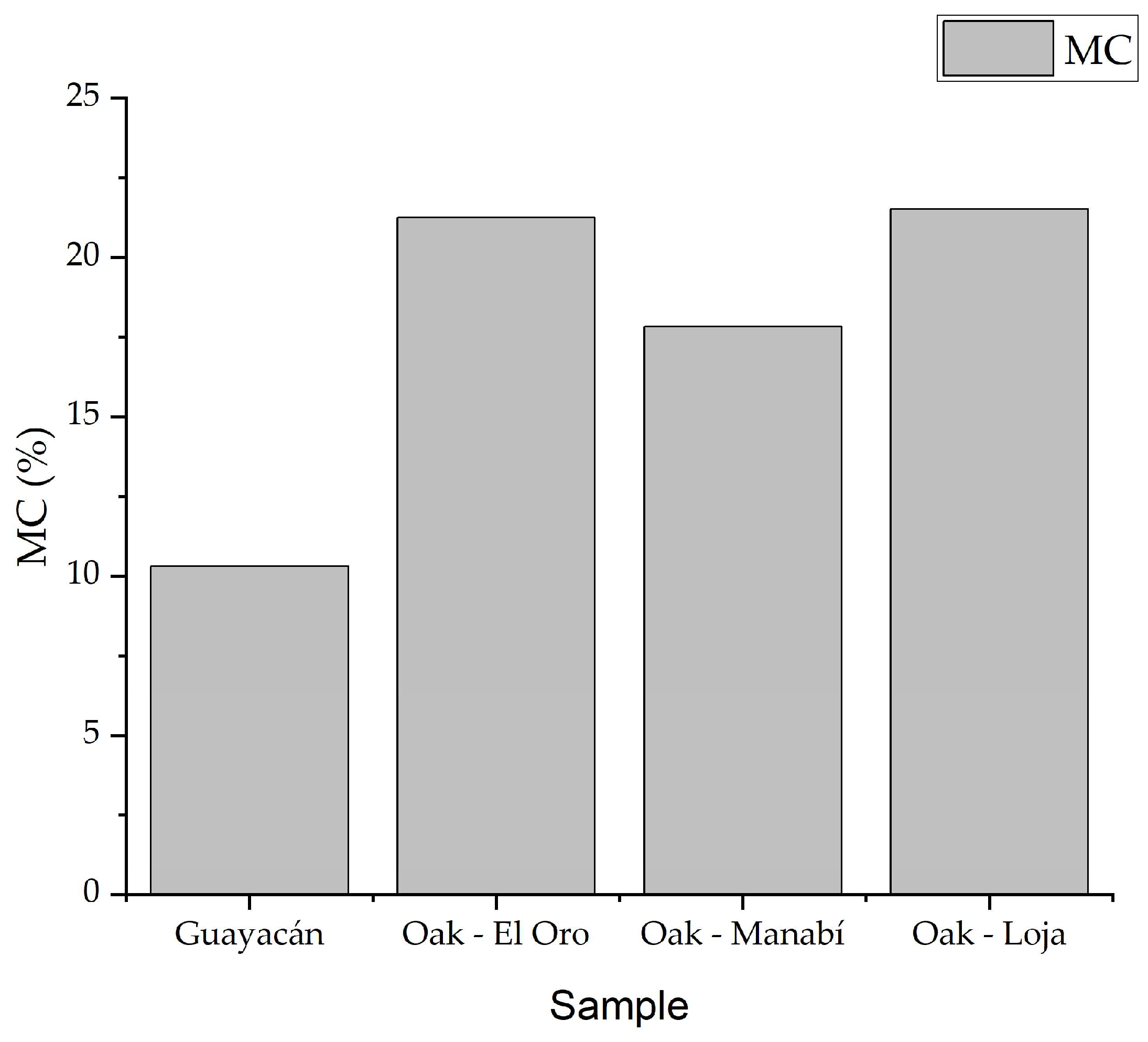
Appendix A.3. Moisture Content Results
| Sample | ww (gr) | wd (gr) | MC (%) |
|---|---|---|---|
| G1MC | 30.9 | 28 | 10.36 |
| G2MC | 32.5 | 29.3 | 10.92 |
| G3MC | 29.3 | 26.4 | 10.98 |
| G4MC | 29.4 | 26.6 | 10.53 |
| G5MC | 28 | 25.7 | 8.95 |
| Average | 10.31 | ||
| Std. Deviation | 0.82 | ||
| Sample | ww (gr) | wd (gr) | MC (%) |
|---|---|---|---|
| O1MC-ElOro | 64.08 | 52.33 | 22.45 |
| O2MC-ElOro | 65.5 | 53.01 | 23.56 |
| O3MC-ElOro | 64.76 | 55 | 17.74 |
| O4MC-ElOro | 60.24 | 48.24 | 24.87 |
| O5MC-ElOro | 66.56 | 53.08 | 25.39 |
| Average | 21.25 | ||
| Std. Deviation | 2.52 | ||
| Sample | ww (gr) | wd (gr) | MC (%) |
|---|---|---|---|
| O1MC-Manabi | 64.33 | 53.98 | 19.17 |
| O2MC- Manabi | 61.14 | 54.50 | 17.68 |
| O3MC- Manabi | 63.70 | 54.59 | 16.68 |
| O4MC- Manabi | 61.15 | 52.15 | 17.25 |
| O5MC- Manabi | 65.65 | 53.91 | 21.77 |
| Average | 17.84 | ||
| Std. Deviation | 1.02 | ||
| Sample | ww (gr) | wd (gr) | MC (%) |
|---|---|---|---|
| O1MC-Loja | 66.25 | 54.44 | 21.69 |
| O2MC- Loja | 65.33 | 53.52 | 22.06 |
| O3MC- Loja | 67.63 | 55.08 | 22.78 |
| O4MC- Loja | 67.06 | 56.09 | 19.55 |
| O5MC- Loja | 67.05 | 55.17 | 21.53 |
| Average | 22.17 | ||
| Std. Deviation | 0.45 | ||
References
- Schubert, M.; Panzarasa, G.; Burgert, I. Sustainability in Wood Products: A New Perspective for Handling Natural Diversity. Chem. Rev. 2023, 123, 1889–1924. [Google Scholar] [CrossRef] [PubMed]
- Hossain, M.U.; Poon, C.S. Comparative LCA of wood waste management strategies generated from building construction activities. J. Clean. Prod. 2018, 177, 387–397. [Google Scholar] [CrossRef]
- Asdrubali, F.; Ferracuti, B.; Lombardi, L.; Guattari, C.; Evangelisti, L.; Grazieschi, G. A review of structural, thermo-physical, acoustical, and environmental properties of wooden materials for building applications. Build. Environ. 2017, 114, 307–332. [Google Scholar] [CrossRef]
- Araya, R.; Guillaumet, A.; Valle, D.; Duque, M.d.P.; Gonzalez, G.; Cabrero, J.M.; De León, E.; Castro, F.; Gutierrez, C.; Negrão, J.; et al. Development of Sustainable Timber Construction in Ibero-America: State of the Art in the Region and Identification of Current International Gaps in the Construction Industry. Sustainability 2022, 14, 1170. [Google Scholar] [CrossRef]
- Nunes, G.; de Melo Moura, J.D.; Güths, S.; Atem, C.; Giglio, T. Thermo-energetic performance of wooden dwellings: Benefits of cross-laminated timber in Brazilian climates. J. Build. Eng. 2020, 32, 101468. [Google Scholar] [CrossRef]
- Wang, Z.; Xue, Q.; Ji, Y.; Yu, Z. Indoor environment quality in a low-energy residential building in winter in Harbin. Build. Environ. 2018, 135, 194–201. [Google Scholar] [CrossRef]
- Keller, M.; Asner, G.P.; Blate, G.; McGlocklin, J.; Merry, F.; Peña-Claros, M.; Zweede, J. Timber production in selectively logged tropical forests in South America. Front. Ecol. Environ. 2007, 5, 213–216. [Google Scholar] [CrossRef]
- Silvero, F.; Rodrigues, F.; Montelpare, S.; Spacone, E.; Varum, H. The path towards buildings energy efficiency in South American countries. Sustain. Cities Soc. 2019, 44, 646–665. [Google Scholar] [CrossRef]
- Carrasco, A.; Cristian, T.; Crespo, E.; Mejía, E. Domestic timber market. For. Use Timber Mark. Ecuadorian Amaz. 2014, 111, 28–44. [Google Scholar]
- Peck, R.B.; Bishop, J.P. Management of secondary tree species in agroforestry systems to improve production sustainability in Amazonian Ecuador. Agrofor. Syst. 1992, 17, 53–63. [Google Scholar] [CrossRef]
- Vázquez, F.J.A.; Guitián, M.A.R. Bioclimatic and phytosociological diagnosis of the species of the Nothofagus genus (Nothofagaceae) in South America. Int. J. Geobot. Res. 2011, 1, 1–20. [Google Scholar] [CrossRef]
- Sánchez-Pinillos, M.; Coll, L.; De Cáceres, M.; Ameztegui, A. Assessing the persistence capacity of communities facing natural disturbances on the basis of species response traits. Ecol. Indic. 2016, 66, 76–85. [Google Scholar] [CrossRef]
- Muñoz, G.R.; Gete, A.R.; Saavedra, F.P. Implications in the design of a method for visual grading and mechanical testing of hardwood structural timber for designation within the European strength classes. For. Syst. 2011, 20, 235. [Google Scholar] [CrossRef]
- Aronson, J.; Toledo, C.S. Caesalpinia paraguariensis (Fabaceae): Forage tree for all seasons. Econ. Bot. 1992, 46, 121–132. [Google Scholar] [CrossRef]
- ISO 5151:2017; Non-Ducted Air Conditioners and Heat Pumps—Testing and Rating for Performance. International Standard Organization: Geneva, Switzerland, 2017; p. 74. Available online: https://www.iso.org/standard/63409.html (accessed on 15 May 2024).
- ANSI/ASHRAE Standard 16-2016; Method of Testing For Rating Room Air Conditioners, Packaged Terminal Air Conditioners, and Packaged Terminal Heat Pumps for Cooling and Heating Capacity. ANSI/ASHRAE: Peachtree Corners, GA, USA, 2016; pp. 1–44.
- AHRI Standard 340/360; Standard for Performance Rating of Commercial and Industrial Unitary Air-conditioning and Heat Pump Equipment. AHRI: Arlington, VA, USA, 2015; pp. 1–62. Available online: https://www.ahrinet.org/system/files/2023-06/AHRI_Standard_340-360_2015.pdf (accessed on 9 June 2024).
- Ghani, S.; Gamaledin, S.M.A.; Rashwan, M.M.; Atieh, M.A. Experimental investigation of double-pipe heat exchangers in air conditioning applications. Energy Build. 2018, 158, 801–811. [Google Scholar] [CrossRef]
- Kodur, V.K.R.; Harmathy, T.Z. Properties of Building Materials. In SFPE Handbook of Fire Protection Engineering; Springer: New York, NY, USA, 2016; pp. 277–324. [Google Scholar] [CrossRef]
- Damfeu, J.C.; Meukam, P.; Jannot, Y.; Wati, E. Modelling and experimental determination of thermal properties of local wet building materials. Energy Build. 2017, 135, 109–118. [Google Scholar] [CrossRef]
- Kouroussis, G.; Fekih, L.B.; Descamps, T. Assessment of timber element mechanical properties using experimental modal analysis. Constr. Build. Mater. 2017, 134, 254–261. [Google Scholar] [CrossRef]
- Darmono; Ma’arif, F.; Widodo, S.; Pamungkas, S. Analysis of Mechanical Properties of Timber Materials. J. Phys. Conf. Ser. 2019, 1387, 012093. [Google Scholar] [CrossRef]
- Tupėnaitė, L.; Žilėnaitė, V.; Kanapeckienė, L.; Sajjadian, S.M.; Gečys, T.; Sakalauskienė, L.; Naimavičienė, J. Multiple criteria assessment of high-rise timber buildings. Eng. Struct. Technol. 2020, 11, 87–94. [Google Scholar] [CrossRef]
- Chen, C.-H. A Novel Multi-Criteria Decision-Making Model for Building Material Supplier Selection Based on Entropy-AHP Weighted TOPSIS. Entropy 2020, 22, 259. [Google Scholar] [CrossRef]
- Reddy, A.S.; Kumar, P.R.; Raj, P.A. Entropy-based fuzzy TOPSIS framework for selection of a sustainable building material. Int. J. Constr. Manag. 2019, 22, 1194–1205. [Google Scholar] [CrossRef]
- Movaffaghi, H.; Yitmen, I. Multi-criteria decision analysis of timber–concrete composite floor systems in multi-storey wooden buildings. Civ. Eng. Environ. Syst. 2021, 38, 161–175. [Google Scholar] [CrossRef]
- Motuzienė, V.; Rogoža, A.; Lapinskienė, V.; Vilutienė, T. Construction solutions for energy efficient single-family house based on its life cycle multi-criteria analysis: A case study. J. Clean. Prod. 2016, 112, 532–541. [Google Scholar] [CrossRef]
- Cavalli, A.; Cibecchini, D.; Togni, M.; Sousa, H.S. A review on the mechanical properties of aged wood and salvaged timber. Constr. Build. Mater. 2016, 114, 681–687. [Google Scholar] [CrossRef]
- ISO 8302:1991; Thermal Insulation Determination of Steady-State Thermal Resistance and Related Properties. Guarded Hot Plate Apparatus. International Organization for Standardization: Geneva, Switzerland, 1991; p. 47.
- ASTM C177-19; Standard Test Method for Steady-State Heat Flux Measurements and Thermal Transmission Properties by Means of the Guarded-Hot-Plate Apparatus. ASTM International: West Conshohocken, PA, USA, 2023; p. 23. [CrossRef]
- ASTM D143-14; Standard Test Methods for Small Clear Specimens of Timber. ASTM International: West Conshohocken, PA, USA, 2021; p. 31. [CrossRef]
- ASTM D4442-20; Standard Test Methods for Direct Moisture Content Measurement of Wood and Wood-Based Materials. ASTM International: West Conshohocken, PA, USA, 2020; p. 5.
- Otten, K.A.; Brischke, C.; Meyer, C. Material moisture content of wood and cement mortars—Electrical resistance-based measurements in the high ohmic range. Constr. Build. Mater. 2017, 153, 640–646. [Google Scholar] [CrossRef]
- Nicolalde, J.F.; Yaselga, J.; Martínez-Gómez, J. Selection of a Sustainable Structural Beam Material for Rural Housing in Latin América by Multicriteria Decision Methods Means. Appl. Sci. 2022, 12, 1393. [Google Scholar] [CrossRef]
- Granta-Design, L. CES-Edupack; Granta Design Limited: Cambridge, UK, 2019. [Google Scholar]
- Shi, J.; Sun, J. Prefabrication Implementation Potential Evaluation in Rural Housing Based on Entropy Weighted TOPSIS Model: A Case Study of Counties in Chongqing, China. Sustainability 2023, 15, 4906. [Google Scholar] [CrossRef]
- Zhu, Y.; Tian, D.; Yan, F. Effectiveness of Entropy Weight Method in Decision-Making. Math. Probl. Eng. 2020, 2020, 3564835. [Google Scholar] [CrossRef]
- Nabavi, S.R.; Wang, Z.; Rangaiah, G.P. Sensitivity Analysis of Multi-Criteria Decision-Making Methods for Engineering Applications. Ind. Eng. Chem. Res. 2023, 62, 6707–6722. [Google Scholar] [CrossRef]
- Mousavi-Nasab, S.H.; Sotoudeh-Anvari, A. A new multi-criteria decision making approach for sustainable material selection problem: A critical study on rank reversal problem. J. Clean. Prod. 2018, 182, 466–484. [Google Scholar] [CrossRef]
- Brancheriau, L.; Bailleres, H.; Guitard, D. Comparison between modulus of elasticity values calculated using 3 and 4 point bending tests on wooden samples. Wood Sci. Technol. 2002, 36, 367–383. [Google Scholar] [CrossRef]
- Chun, Q.; Meng, Z.; Han, Y. Research on Mechanical Properties of Main Joints of Chinese Traditional Timber Buildings with the Type of Post-and-Lintel Construction. Int. J. Archit. Herit. 2017, 11, 247–260. [Google Scholar] [CrossRef]
- Namari, S.; Drosky, L.; Pudlitz, B.; Haller, P.; Sotayo, A.; Bradley, D.; Mehra, S.; O’Ceallaigh, C.; Harte, A.M.; El-Houjeyri, I. Mechanical properties of compressed wood. Constr. Build. Mater. 2021, 301, 124269. [Google Scholar] [CrossRef]
- Haller, P.; Wehsener, J. Festigkeitsuntersuchungen an Fichtenpressholz (FPH). Holz als Roh und Werkst. 2004, 62, 452–454. [Google Scholar] [CrossRef]
- Anh, L.D.H.; Pásztory, Z. An overview of factors influencing thermal conductivity of building insulation materials. J. Build. Eng. 2021, 44, 102604. [Google Scholar] [CrossRef]









| Material | Index | Thermal Conductivity | Flexural Modulus | Compressive Strength | Bending Strength | Tensile Strength |
|---|---|---|---|---|---|---|
| (W × m−1 × K−1) | (GPa) | (MPa) | (MPa) | (MPa) | ||
| Bamboo * | M1 | 0.18 | 17 | 60 | 35.9 | 160 |
| Guayacan | M2 | 0.105 | 36.2 | 6.81 | 54.94 | 64.81 |
| Oak El Oro | M3 | 0.162 | 72.4 | 19.23 | 82.56 | 173.54 |
| Oak Manabi | M4 | 0.176 | 91.36 | 20.12 | 77.11 | 158.13 |
| Oak Loja | M5 | 0.172 | 151.36 | 19.08 | 77.62 | 148.75 |
| Criteria | Entropy | Weight | Rank |
|---|---|---|---|
| Thermal conductivity | 0.99 | 0.212 | 1 |
| Modulus of elasticity | 0.87 | 0.187 | 4 |
| Compressive strength | 0.86 | 0.184 | 5 |
| Bending strength | 0.98 | 0.209 | 2 |
| Tensile strength | 0.97 | 0.208 | 3 |
| Material | Qi | Ui | Rank |
|---|---|---|---|
| Bamboo | 0.203 | 86% | 4 |
| Guayacan | 0.144 | 61% | 5 |
| Oak El Oro | 0.209 | 89% | 2 |
| Oak Manabi | 0.208 | 88% | 3 |
| Oak Loja | 0.236 | 100% | 1 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Nicolalde, J.F.; Martínez-Gómez, J.; Dávila, P.; Medrano-Barboza, J.; Molina-Osejos, J.V. Ecuadorian Woods: Building Material Selection Using an Entropy-COPRAS Comparative Analysis Based on the Characterization of Ecuadorian Oak and Guayacan Timber. Biomimetics 2024, 9, 443. https://doi.org/10.3390/biomimetics9070443
Nicolalde JF, Martínez-Gómez J, Dávila P, Medrano-Barboza J, Molina-Osejos JV. Ecuadorian Woods: Building Material Selection Using an Entropy-COPRAS Comparative Analysis Based on the Characterization of Ecuadorian Oak and Guayacan Timber. Biomimetics. 2024; 9(7):443. https://doi.org/10.3390/biomimetics9070443
Chicago/Turabian StyleNicolalde, Juan Francisco, Javier Martínez-Gómez, Paúl Dávila, Johanna Medrano-Barboza, and Jaime Vinicio Molina-Osejos. 2024. "Ecuadorian Woods: Building Material Selection Using an Entropy-COPRAS Comparative Analysis Based on the Characterization of Ecuadorian Oak and Guayacan Timber" Biomimetics 9, no. 7: 443. https://doi.org/10.3390/biomimetics9070443
APA StyleNicolalde, J. F., Martínez-Gómez, J., Dávila, P., Medrano-Barboza, J., & Molina-Osejos, J. V. (2024). Ecuadorian Woods: Building Material Selection Using an Entropy-COPRAS Comparative Analysis Based on the Characterization of Ecuadorian Oak and Guayacan Timber. Biomimetics, 9(7), 443. https://doi.org/10.3390/biomimetics9070443









