Design of Bionic Foot Inspired by the Anti-Slip Cushioning Mechanism of Yak Feet
Abstract
1. Introduction
2. Materials and Methods
2.1. Ethical Declaration
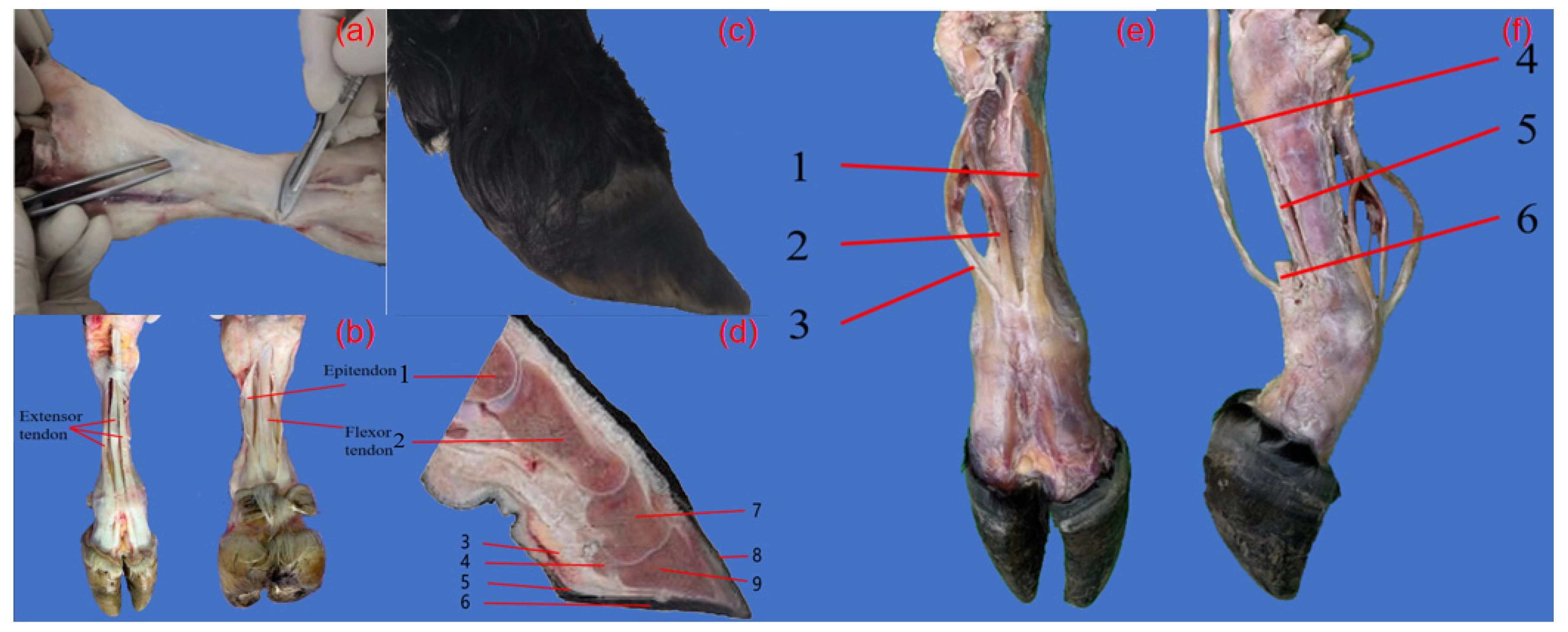
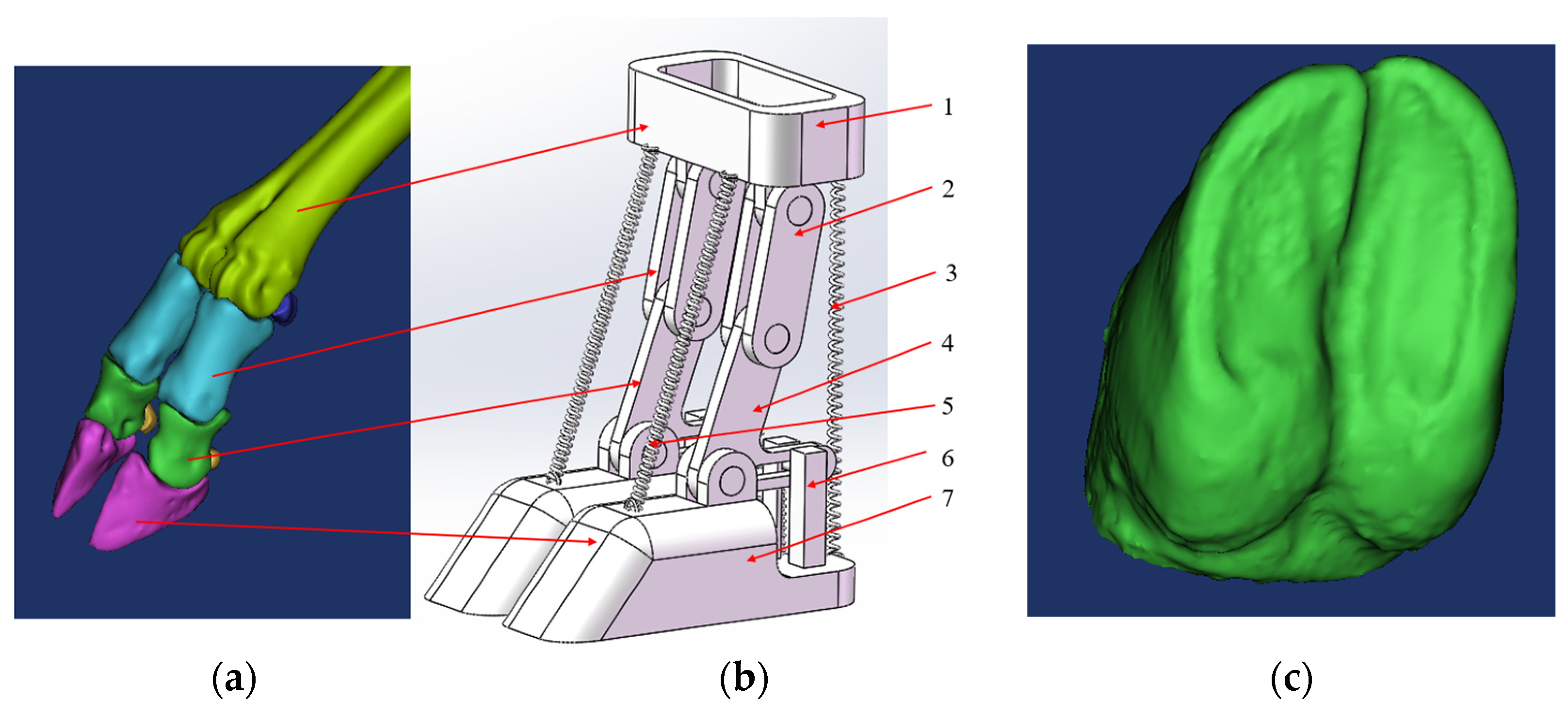
2.2. Anatomical Observations of Yak Foot
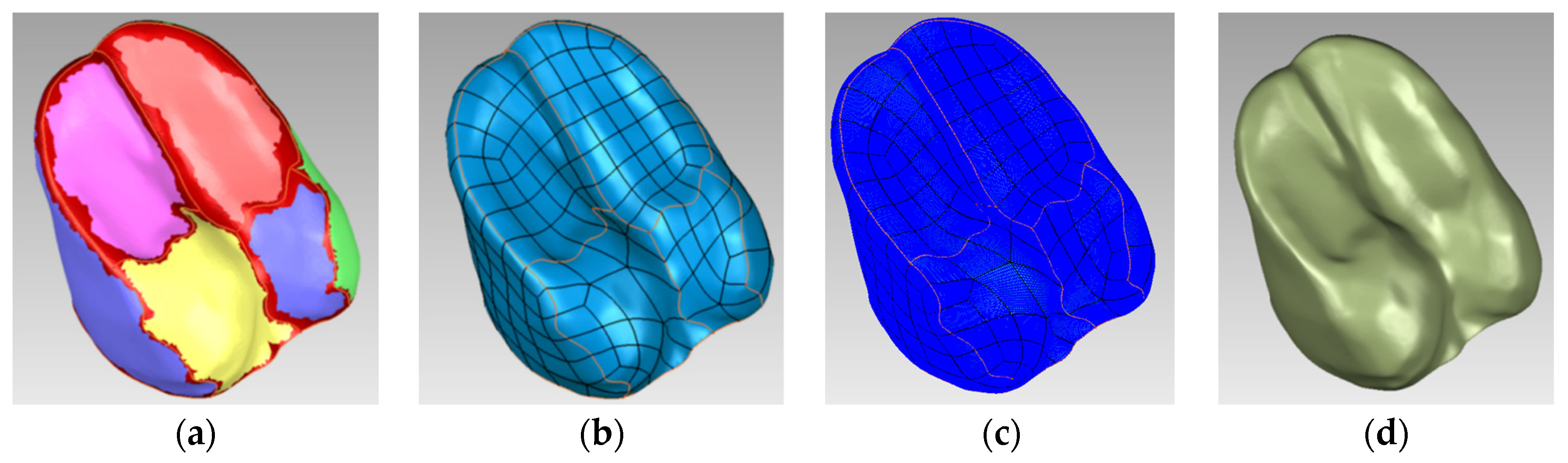
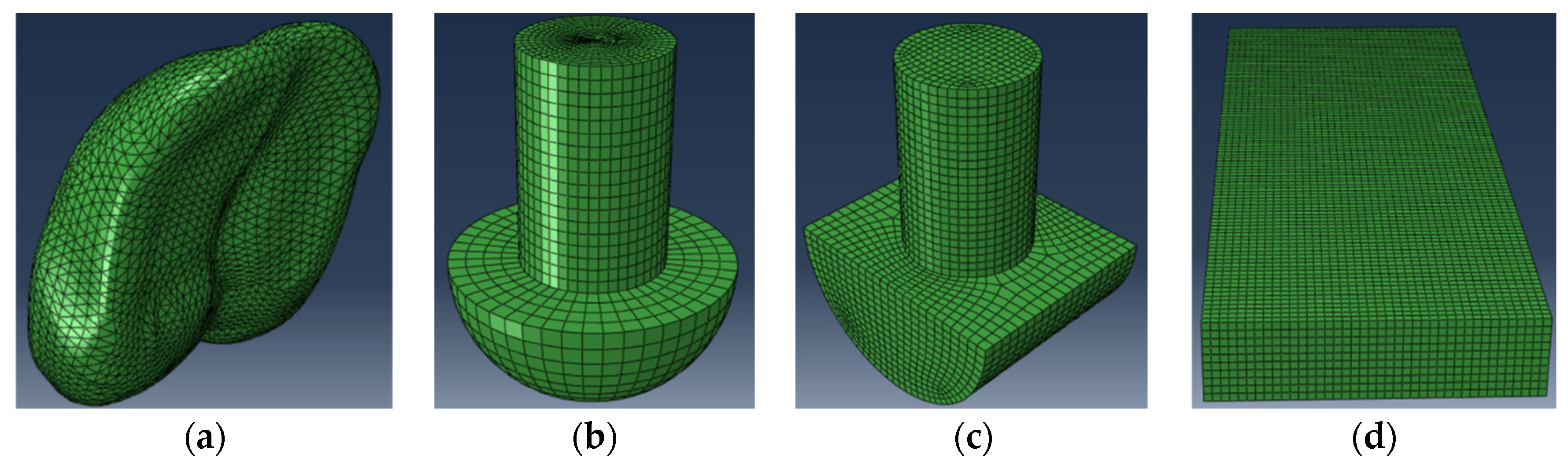
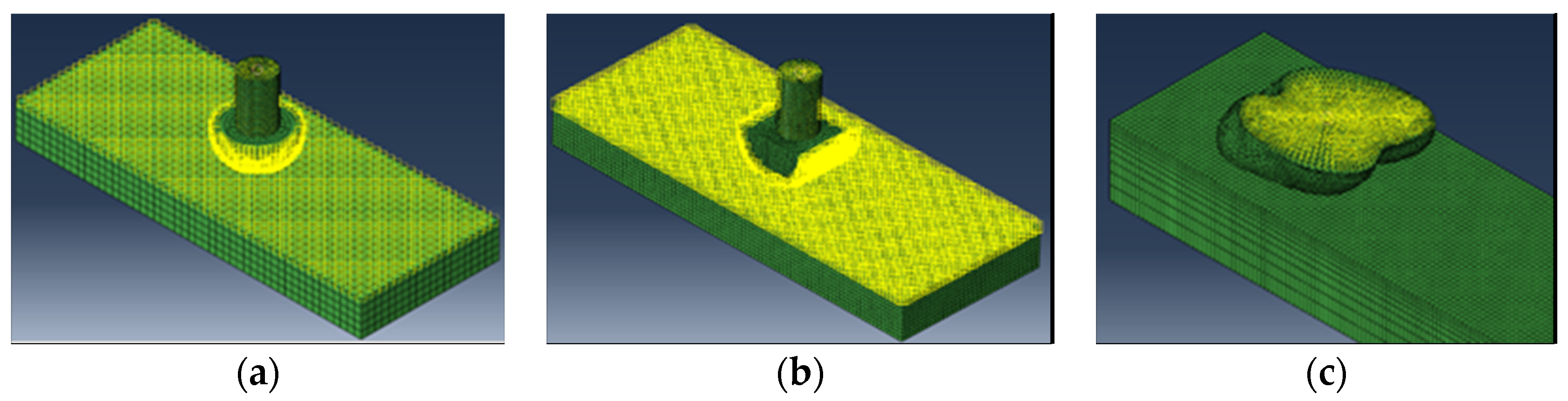
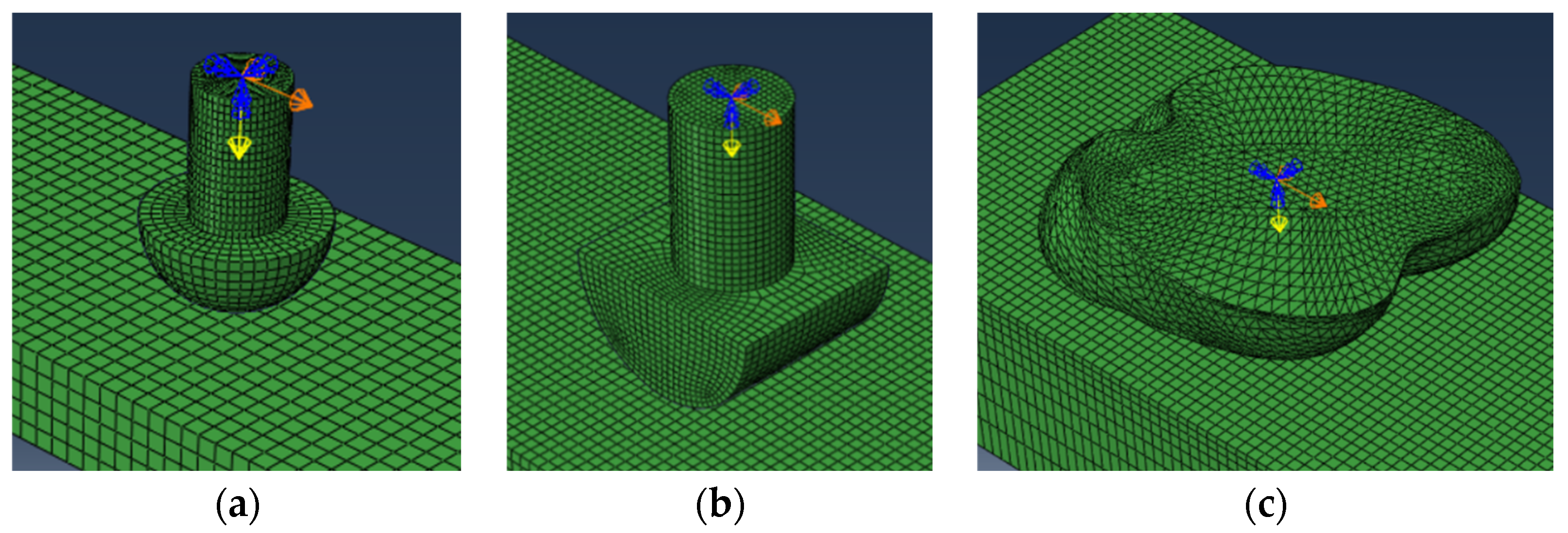
2.3. Bionic Foot End Model Construction and Simulation
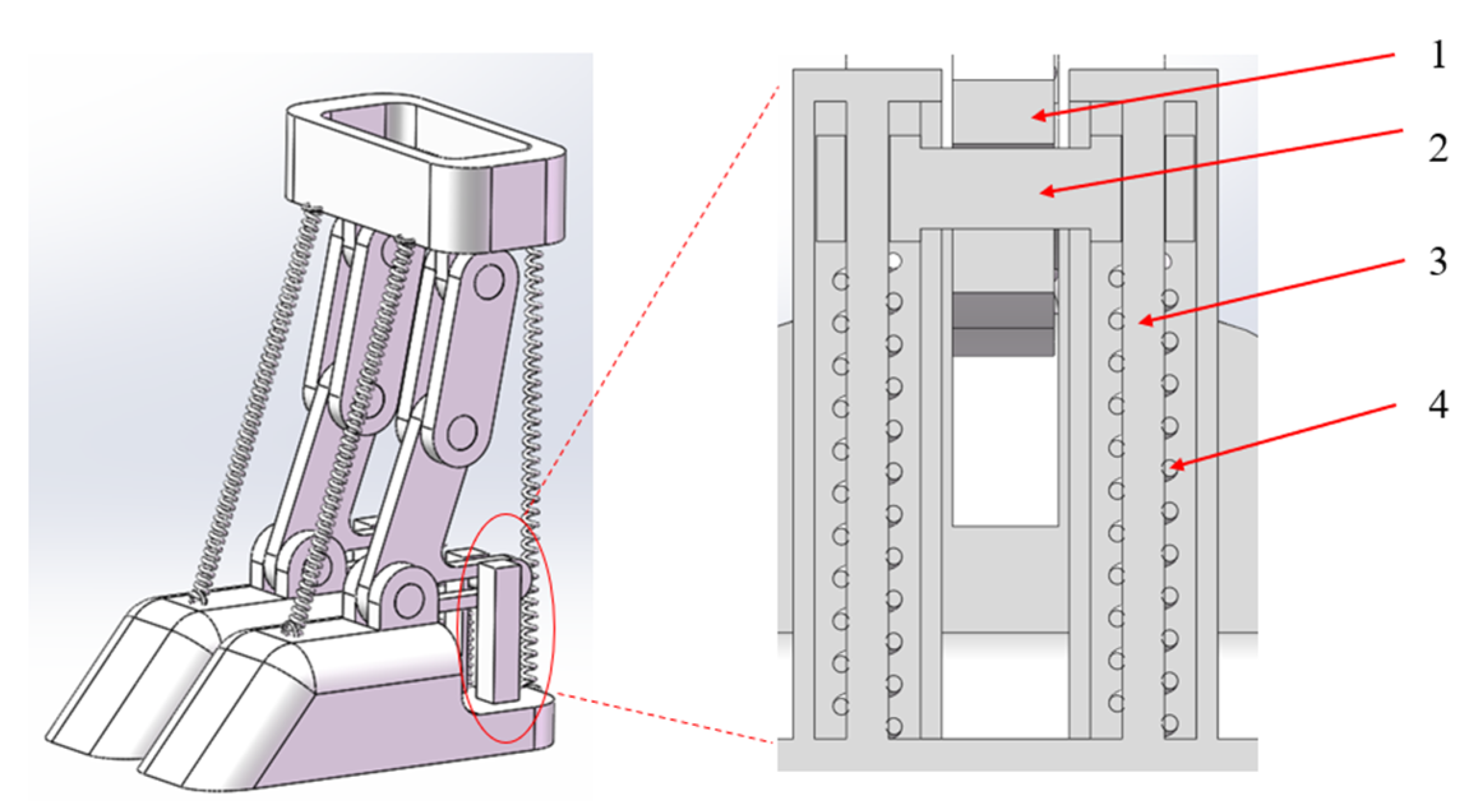
2.4. Bionic Foot Model Construction and Simulation
3. Result and Analysis
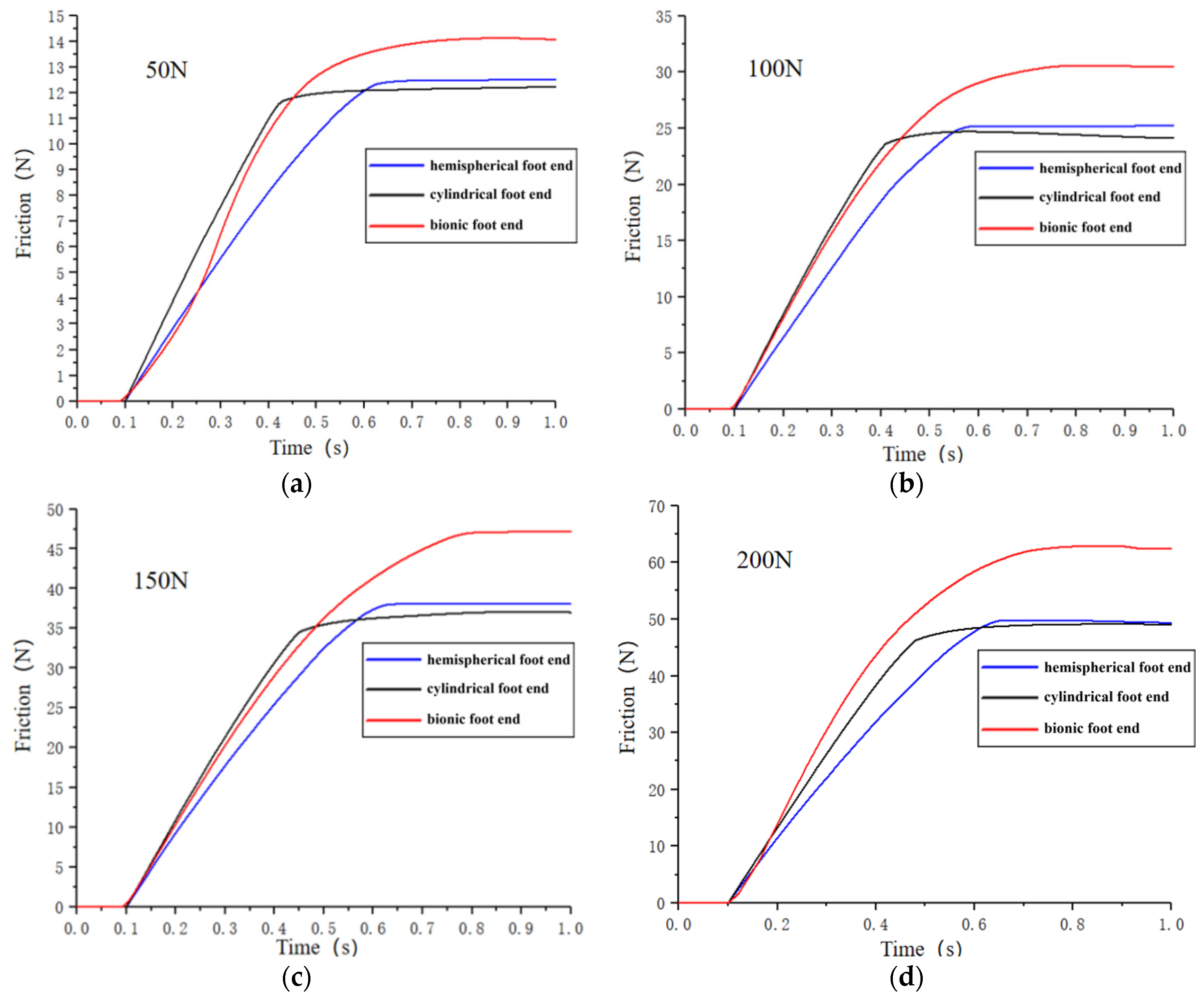
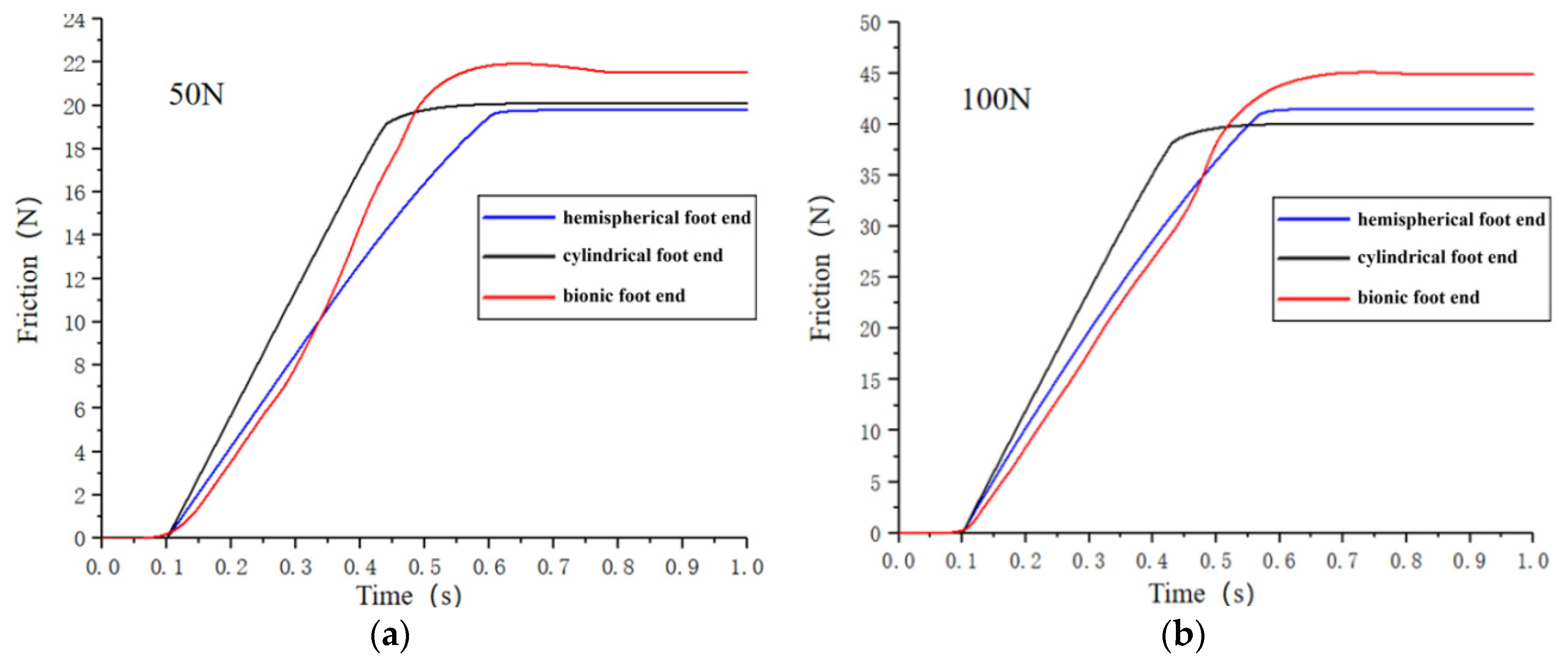
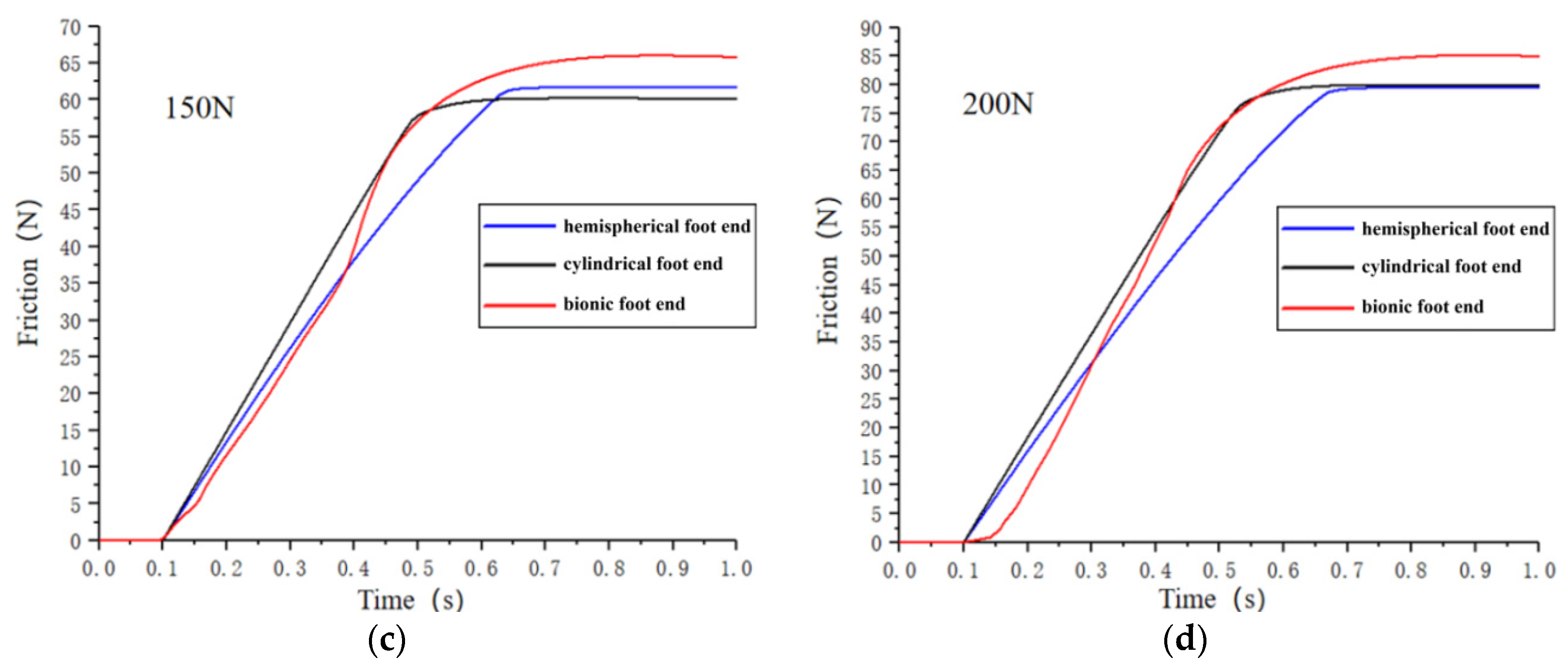
3.1. Anti-Slip Properties of the Foot End
3.2. Bionic Foot Cushioning Properties
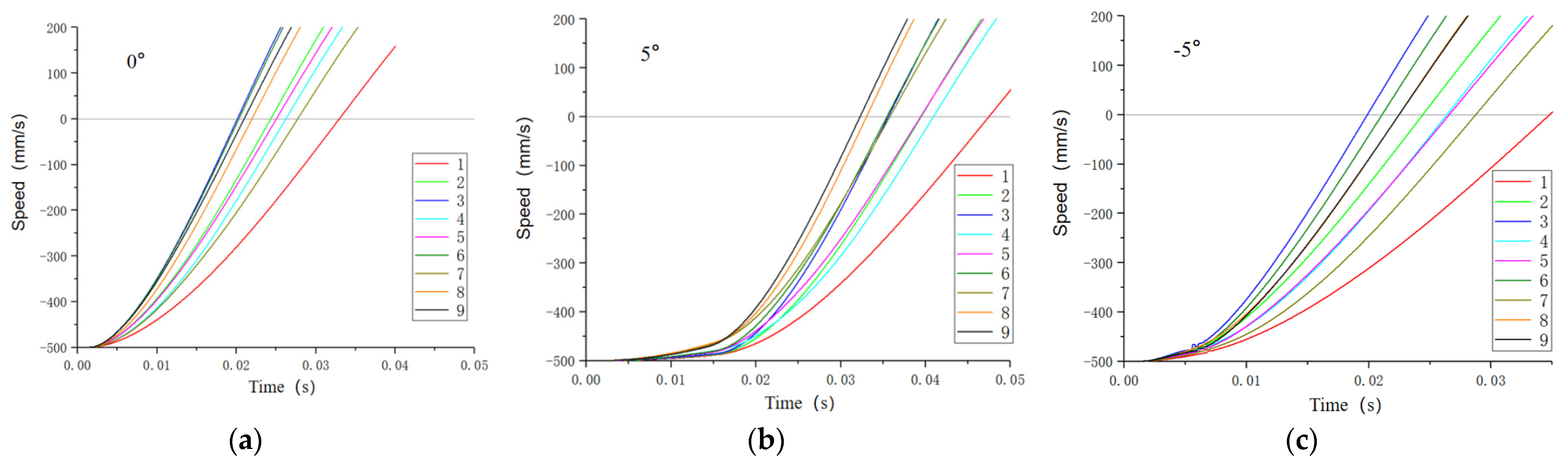
3.3. Regression Analysis
4. Discussion
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Sherrod, V.; Johnson, C.; Killpack, M.D. Design Optimization for Rough Terrain Traversal Using a Compliant, Continuum-Joint, Quadruped Robot. Front. Robot. AI 2022, 9, 860020. [Google Scholar] [CrossRef] [PubMed]
- Zhao, X.; Wu, Y.; You, Y.; Laurenzi, A.; Tsagarakis, N. Variable stiffness locomotion with guaranteed stability for quadruped robots traversing uneven terrains. Front. Robot. AI 2022, 9, 874290. [Google Scholar] [CrossRef] [PubMed]
- Uno, K.; Valsecchi, G.; Hutter, M.; Yoshida, K. Simulation-Based Climbing Capability Analysis for Quadrupedal Robots. In Proceedings of the Climbing and Walking Robots Conference, Takarazuka, Japan, 30 August–1 September 2021; pp. 179–191. [Google Scholar]
- She, H.; Zhang, W.; Huang, H.; Yu, Z. Anti-skid Foot Design for a Humanoid Robot. In Proceedings of the 2014 IEEE International Conference on Robotics and Biomimetics, Bali, Indonesia, 5–10 December 2014; pp. 1112–1117. [Google Scholar]
- Zhang, L.; Liu, X.; Ren, P.; Gao, Z.; Li, A. Design and Research of a Flexible Foot for a Multi-Foot Bionic Robot. Appl. Sci. 2019, 9, 3451. [Google Scholar] [CrossRef]
- Melo, K.; Horvat, T.; Ijspeert, A.J. Minimalist Design of a 3-Axis Passive Compliant Foot for Sprawling Posture Robots. In Proceedings of the 2nd IEEE International Conference on Soft Robotics, Seoul, Republic of Korea, 14–18 April 2019; pp. 788–794. [Google Scholar]
- Chen, T.; Li, Y.; Rong, X. Whole body motion planning and control of a quardruped robot for challenging terrain. J. Field Robot. 2019, 41, 307–316. [Google Scholar]
- Jin, W.C. The Dynamics of Legged Locomotion. J. Huaihai Inst. Technol. 2019, 28, 15–20. [Google Scholar]
- Ma, G. Comparative Experimental Study of Ground Reaction Force on Hemispherical Foot and Bionic Equinus of Quadruped Robot. In Proceedings of the IEEE 4th Information Technology and Mechatronics Engineering Conference, Chongqing, China, 14–16 December 2018; pp. 1883–1886. [Google Scholar]
- Huiseok, M.; Jungsoo, C.; Kyungchul, K. Retractable cleat mechanism of legged robots’ foot on various terrain. In Proceedings of the 18th International Conference on Control, Automation and Systems, PyeongChang, Republic of Korea, 7–20 October 2018; pp. 918–921. [Google Scholar]
- Hauser, S.; Mutlu, M.; Banzet, P.; Ijspeert, A.J. Compliant universal grippers as adaptive feet in legged robots. Adv. Robot. 2018, 32, 825–836. [Google Scholar] [CrossRef]
- Zang, X.; Liu, Y.; Li, W.; Lin, Z.; Zhao, J. Design and Experimental Development of a Pneumatic Stiffness Adjustable Foot System for Biped Robots Adaptable to Bumps on the Ground. Appl. Sci. 2017, 7, 1005. [Google Scholar] [CrossRef]
- He, G.; Hu, P.; Ji, A.; Zhu, D.; Liao, W. Stress and Sinking Property Analysis of Legged Robot Foot with Toe Structure in Soft Soil. In Proceedings of the International Conference on Computer Systems, Electronics and Control, Dalian, China, 25–27 December 2017; pp. 131–135. [Google Scholar]
- Zheng, Y.; Xu, K.; Tian, Y.; Deng, H.; Ding, X. Bionic Design and Analysis of a Novel Quadruped Robot with a Multistage Buffer System. Chin. J. Mech. Eng. 2022, 35, 32. [Google Scholar] [CrossRef]
- Torres-Pardo, A.; Pinto-Fernández, D.; Garabini, M.; Angelini, F.; Rodriguez-Cianca, D.; Massardi, S.; Tornero-López, J.; Moreno, J.C.; Torricelli, D. Legged locomotion over irregular terrains: State of the art of human and robot performance. Bioinspir. Biomim. 2022, 17, 061002. [Google Scholar] [CrossRef]
- Qian, Z. Dynamic Finite Element Modeling and Biomechanical Function Coupling Analysis of the Human Foot Comple during Locomotion. Ph.D. Thesis, Jilin University, Changchun, China, 2010. [Google Scholar]
- Alexander, R.M.; Bennett, M.B.; Ker, R.F. Mechanical properties and function of the paw pads of some mammals. J. Zool. 1986, 209, 405–419. [Google Scholar] [CrossRef]
- Biewener, A.A. Biomechanics of mammalian terrestrial locomotion. Science 1990, 250, 1097–1103. [Google Scholar] [CrossRef] [PubMed]
- David, A.; Chardonnet, J.R.; Kheddar, A.; Kaneko, K.; Yokoi, K. Study of an external passive shock-absorbing mechanism for walking robots. In Proceedings of the Humanoids 2008–8th IEEE-RAS International Conference on Humanoid Robots, Daejeon, Republic of Korea, 1–3 December 2008; p. 450. [Google Scholar]
- Spröwitz, A.; Tuleu, A.; Vespignani, M.; Ajallooeian, M.; Badri, E.; Ijspeert, A.J. Towards dynamic trot gait locomotion: Design, control, and experiments with Cheetah-cub, a compliant quadruped robot. Int. J. Robot. Res. 2013, 32, 932–950. [Google Scholar] [CrossRef]
- Zhang, C.; Chen, H.; Zhang, Q. Design and simulation analysis of a camel-like foot. Dual-Use Technol. Prod. 2012, 6, 55–58. [Google Scholar]
- Wan, H. Research on Foot Bionics of Cross-Sand Mechanical Legs Based on the Bioassembly Characteristics of Ostrich Feet. Master’s Thesis, Jilin University, Changchun, China, 2019. [Google Scholar]
- Kim, S.; Spenko, M.; Trujillo, S.; Heyneman, B.; Santos, D.; Cutkosky, M. Smooth vertical surface climbing with directional adhesion. IEEE Trans. Robot. 2008, 24, 65–74. [Google Scholar]
- Lu, S.; Guo, C.; Dai, Z. Bionic structural design of a footed robot foot. Mech. Manuf. Autom. 2012, 41, 160–161. [Google Scholar]
- Spurrier, S.; Allen, T.; Grant, R.A. Investigating Foot Morphology in Rock Climbing Mammals: Inspiration for Biomimetic Climbing shoes. Biomimetics 2023, 8, 8. [Google Scholar] [CrossRef]
- Chang, T.; Liu, X.; Gu, X.; Guo, Z. Design of bionic quadruped robot and stress analysis for foot end with kinematics. Comput. Eng. 2017, 43, 292–297. [Google Scholar]
- Zhang, Q. Study on Anti-Slip Cushioning Characteristics of Goat Foot and Bionic Foot Design. Master’s Thesis, Jilin University, Changchun, China, 2019. [Google Scholar]
- Zhao, D.; Chen, K. A study on the design and gait planning of a wheel-footed mobile robot for unstructured terrain. J. Mech. Eng. 2013, 2, 72. [Google Scholar]
- Song, X.; Pan, J.; Lin, F.; Zhang, X.; Chen, C.; Huang, D. Cockroach-inspired Traversing Narrow Obstacles for a Sprawled Hexapod Robot. J. Bionic Eng. 2022, 19, 1288–1301. [Google Scholar] [CrossRef]
- Chen, J.; Liu, C.; Zhao, H.; Zhu, Y.; Zhao, J. Learning to Identify Footholds from Geometric Characteristics for a Six-legged Robot over Rugged Terrain. J. Bionic Eng. 2020, 17, 512–522. [Google Scholar] [CrossRef]
- Zhong, K.H.T. Zhongke Wisto’s driverless mine transportation robot “ZaisHan CarMo” officially put into operation. Robot. Appl. 2023, 4, 4. [Google Scholar]
- Zhang, X.; Lin, X.; Dai, F. Design of Intelligent Saving Robot Based on Six-legged Robot. In Proceedings of the 2018 International Conference on Artificial Life and Robotics (ICAROB 2018), Beppu, Japan, 1–4 February 2018; pp. 649–654. [Google Scholar]
- Focchi, M.; Bensaadallah, M.; Frego, M.; Peer, A.; Fontanelli, D.; DelPrete, A.; Palopoli, L. CLIO: A Novel Robotic Solution for Exploration and Rescue Missions in Hostile Mountain Environments. In Proceedings of the 2023 IEEE International Conference on Robotics and Automation (ICRA 2023), London, UK, 29 May–2 June 2023; pp. 7742–7748. [Google Scholar]
- Zhou, Y.; Zhou, S.; Wang, M.; Chen, A. Multitarget Search Algorithm Using Swarm Robots in an Unknown 3D Mountain Environment. Appl. Sci. 2023, 13, 1969. [Google Scholar] [CrossRef]
- Zhang, J.; Li, M.; Cao, J.; Dou, Y.; Xiong, X. Research on Bionic Jumping and Soft Landing of Single Leg System in Quadruped Robot. J. Bionic Eng. 2023, 20, 2088–2107. [Google Scholar] [CrossRef]
- Zhang, T.; Gao, X.; Xu, L. Progress in the study of environmental adaptation of highland domesticated animals. Livest. J. Pastor. Vet. Med. 2020, 51, 1475–1487. [Google Scholar]
- Yi, H.; Xu, Z.; Xin, X.; Zhou, L.; Luo, X. Bio-inspired Leg Design for a Heavy-Duty Hexapod Robot. J. Bionic Eng. 2022, 19, 975–990. [Google Scholar] [CrossRef]
- Smith, B. Life on the Rocks: A Portrait of the American Mountain Goat; University Press of Colorado: Denver, CO, USA, 2014. [Google Scholar]
- Kui, H.; Liu, X.; Liu, J.; Liang, W.; Zhang, S.; Qian, Z.; Ren, L. The passive contact stability of blue sheep hoof based on structure, mechanical properties, and surface morphology. Front. Bioeng. Biotechnol. 2020, 8, 363. [Google Scholar] [CrossRef]
- Tian, W.; Liu, H.; Zhang, Q.; Su, B.; Xu, W.; Cong, Q. Cushion mechanism of goat hoof bulb tissues. Appl. Bionics Biomech. 2019, 2019, 3021576. [Google Scholar] [CrossRef] [PubMed]
- Tian, W.; Wang, J.; Li, M.; Cong, Q. Design and optimization of vibration reduction structure imitating pore structure in goat Capsula ungulae. J. Vib. Eng. 2018, 31, 352–357. [Google Scholar]
- Zhang, R.; Li, G.; Qiao, Y.; Jiang, L.; Li, J. Analysis of the effect of reindeer-like plantar morphology tread unit on the anti-skid performance of tires. Trans. Chin. Soc. Agric. Eng. 2019, 35, 47–54. [Google Scholar]
- Zhang, R.; Yang, M.; Liu, H.; Zeng, G.; Pan, R.; Li, J. Numerical simulation of sand fixation and flow limitation characteristics of the two-toed plantar surface of the ostrich. J. Jilin Univ. (Eng. Technol. Ed.) 2015, 2, 508–515. [Google Scholar]
- Weissengruber, G.E.; Egger, G.F.; Hutchinson, J.R.; Groenewald, H.B.; Elsässer, L.; Famini, D.; Forstenpointner, G. The structure of the cushions in the feet of African elephants (Loxodonta africana). J. Anat. 2006, 209, 789–792. [Google Scholar] [CrossRef]

| Materials | C10 (MPa) | C01 (MPa) | Poisson’s | Densities (1 × 10−9 t/mm3) | Shore Hardness (HA) |
|---|---|---|---|---|---|
| Hard rubber | 0.5792 | 0.1448 | 0.5 | 1.112 | 60 |
| Ground Type | Densities (t/mm3) | Young’s Modulus E (MPa) | Poisson’s | Friction Angle (°) | Stress Ratio | Expansion Angle (°) |
|---|---|---|---|---|---|---|
| Soil | 1.79 × 10−9 | 1.14 | 0.3 | 12.21 | 1 | 0 |
| Rock | 2.72 | 75,000 | 0.3 | 51.8 | 29.5 | 0 |
| Ground Type | Soil | Rock |
|---|---|---|
| coefficient of friction | 0.25 | 0.4 |
| Densities (g/cm3) | Modulus of Elasticity (GPa) | Poisson’s | Tensile Strength (MPa) | Yield Strength (MPa) |
|---|---|---|---|---|
| 2.7 | 70 | 0.3 | 290 | 240 |
| Factor | z1 (N/mm) | z2 (N/mm) | z3 (N/mm) |
|---|---|---|---|
| Level | |||
| 1 | 50 | 50 | 50 |
| 2 | 100 | 100 | 100 |
| 3 | 150 | 150 | 150 |
| Test Number | Factor | 0° Impact Time (s) | 5° Impact Time (s) | −5° Impact Time (s) | ||
|---|---|---|---|---|---|---|
| z1 | z2 | z3 | ||||
| 1 | 1 (50) | 1 (50) | 1 (50) | 0.0329 | 0.0474 | 0.0348 |
| 2 | 1 | 2 (100) | 2 (100) | 0.0243 | 0.0394 | 0.0244 |
| 3 | 1 | 3 (150) | 3 (150) | 0.0201 | 0.0356 | 0.0199 |
| 4 | 2 (100) | 1 | 3 | 0.0262 | 0.0409 | 0.0263 |
| 5 | 2 | 2 | 1 | 0.025 | 0.0394 | 0.0265 |
| 6 | 2 | 3 | 2 | 0.0203 | 0.0354 | 0.0211 |
| 7 | 3 (150) | 1 | 2 | 0.0277 | 0.0358 | 0.0287 |
| 8 | 3 | 2 | 3 | 0.0219 | 0.033 | 0.0225 |
| 9 | 3 | 3 | 1 | 0.021 | 0.0323 | 0.0224 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Tian, W.; Zhou, K.; Chen, Z.; Shen, Z.; Wang, Z.; Jiang, L.; Cong, Q. Design of Bionic Foot Inspired by the Anti-Slip Cushioning Mechanism of Yak Feet. Biomimetics 2024, 9, 260. https://doi.org/10.3390/biomimetics9050260
Tian W, Zhou K, Chen Z, Shen Z, Wang Z, Jiang L, Cong Q. Design of Bionic Foot Inspired by the Anti-Slip Cushioning Mechanism of Yak Feet. Biomimetics. 2024; 9(5):260. https://doi.org/10.3390/biomimetics9050260
Chicago/Turabian StyleTian, Weijun, Kuiyue Zhou, Zhu Chen, Ziteng Shen, Zhirui Wang, Lei Jiang, and Qian Cong. 2024. "Design of Bionic Foot Inspired by the Anti-Slip Cushioning Mechanism of Yak Feet" Biomimetics 9, no. 5: 260. https://doi.org/10.3390/biomimetics9050260
APA StyleTian, W., Zhou, K., Chen, Z., Shen, Z., Wang, Z., Jiang, L., & Cong, Q. (2024). Design of Bionic Foot Inspired by the Anti-Slip Cushioning Mechanism of Yak Feet. Biomimetics, 9(5), 260. https://doi.org/10.3390/biomimetics9050260






