Simulation and Structural Analysis of a Flexible Coupling Bionic Desorption Mechanism Based on the Engineering Discrete Element Method
Abstract
1. Introduction
2. Materials and Methods
2.1. Parameter Acquisition of Bionic Prototype
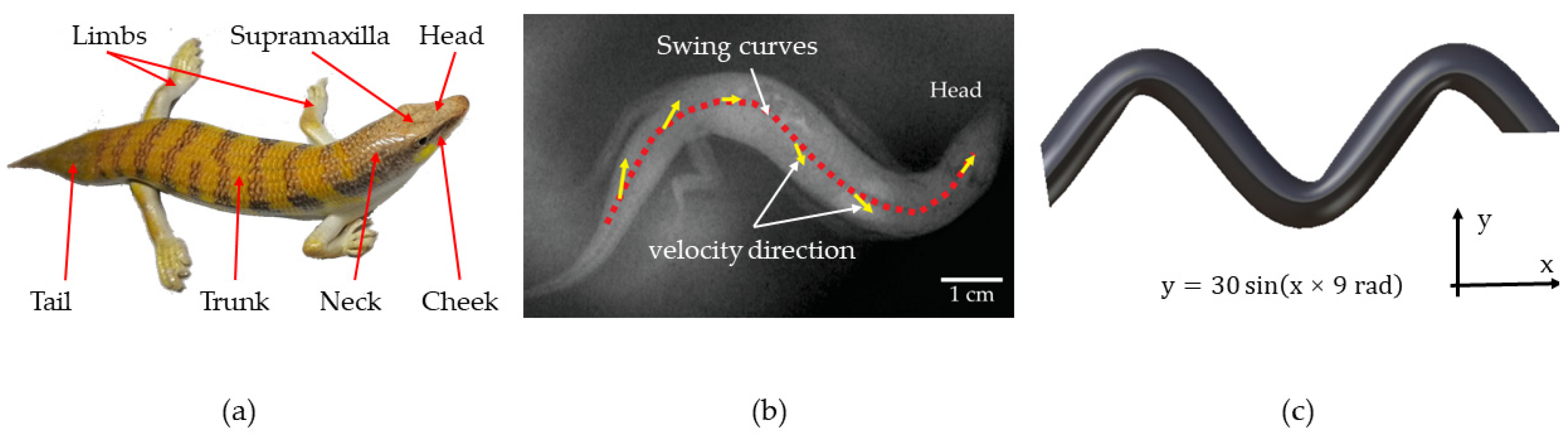
2.1.1. Body Motion Curve Acquisition and Model Establishment
2.1.2. Head Contour Acquisition and Model Establishment
2.1.3. Microstructure Parameter Acquisition and Model Establishment
2.2. Simulation of EDEM
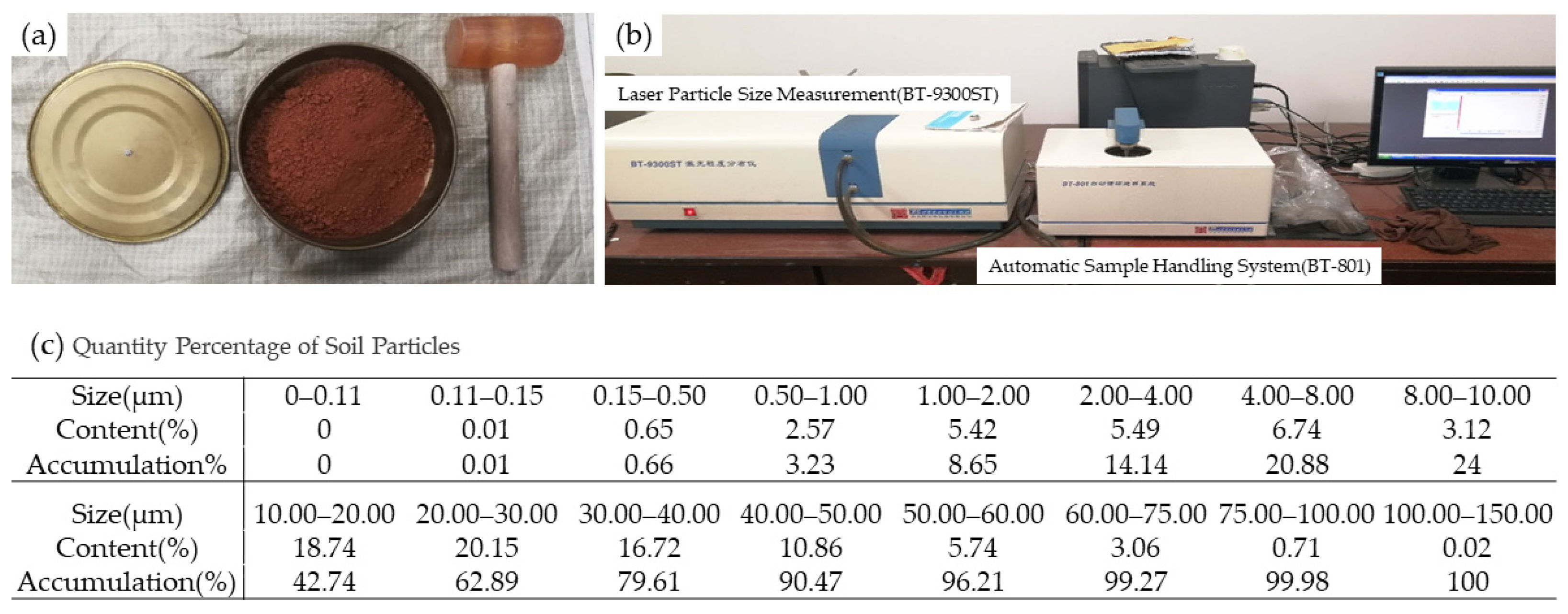
2.2.1. Measurement of Soil Parameters
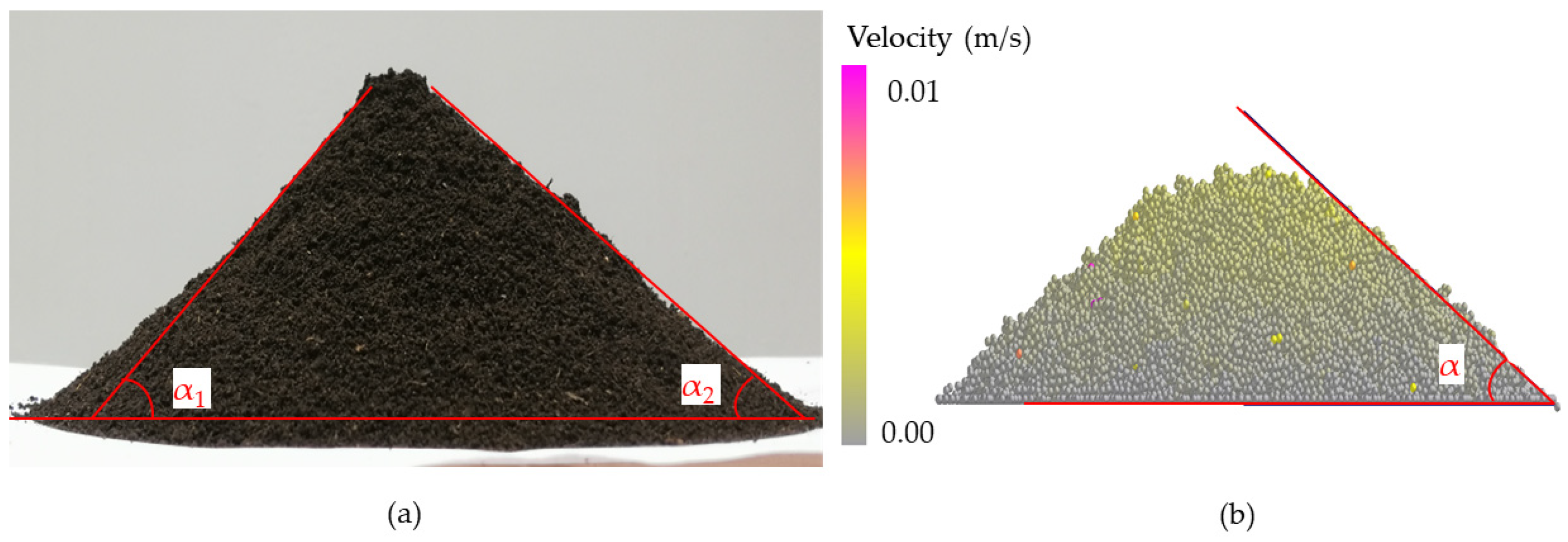
2.2.2. Calibration of Soil Simulation Parameters
2.2.3. Simulation and Analysis of Sandfish Body Swing Mode
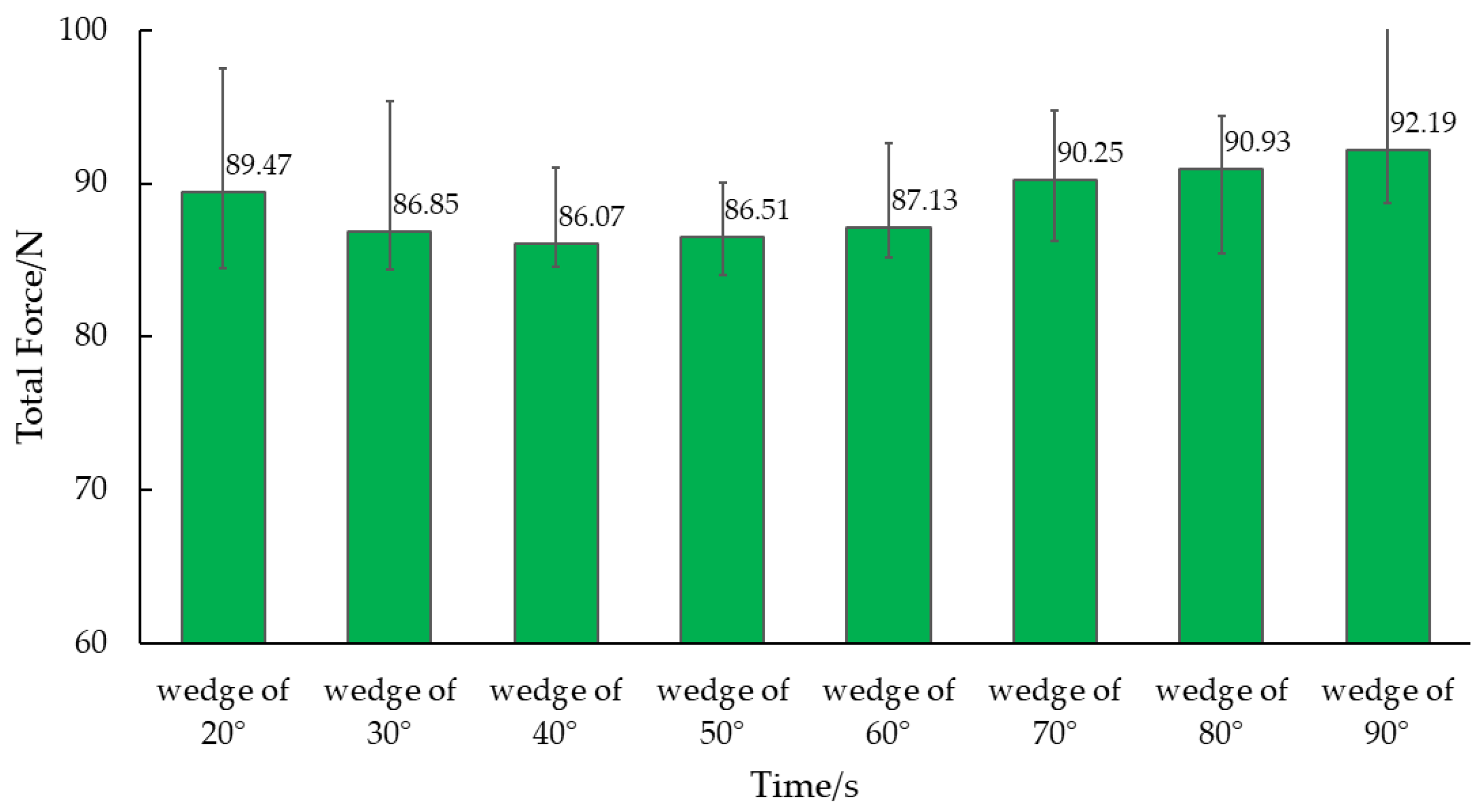
2.2.4. Simulation and Analysis of Wedge Structure and Serrated Structure
3. Results
3.1. Bionic Prototype Structure Organization
3.1.1. Body Motion Curve Acquisition and Model Establishment of Sandfish
3.1.2. Head Contour Acquisition and Model Establishment of Sandfish
3.1.3. Microstructure Model Establishment of Scale Surface
3.2. Simulation of EDEM
3.2.1. Soil Parameter Calibration
3.2.2. Soil Parameter Calibration of EDEM
3.2.3. EDEM Simulation of Sandfish Body Swing
3.2.4. Simulation of the Wedge Structure and Serrated Structure
4. Discussion
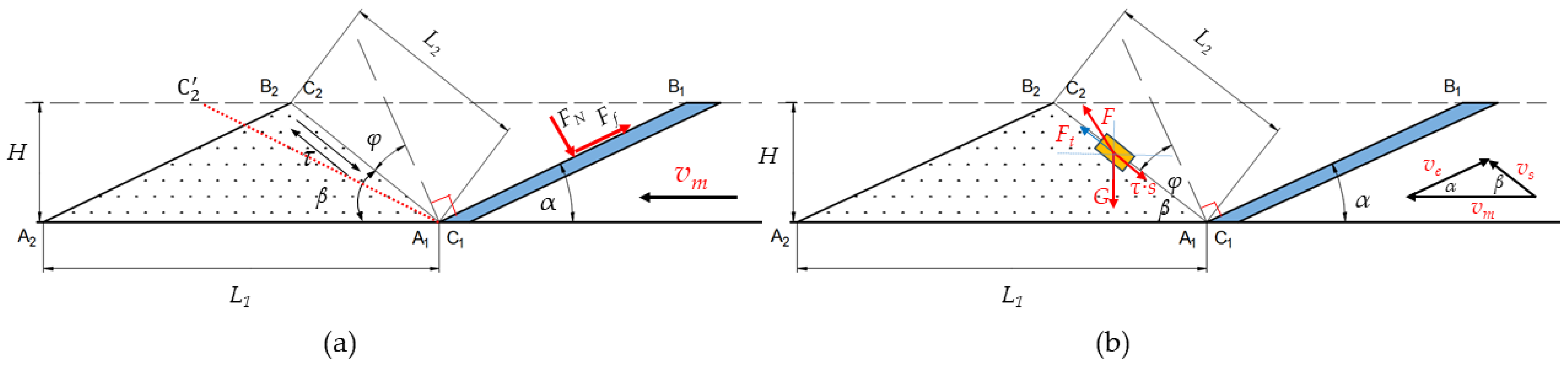
4.1. Bionic Body Swing Desorption Mechanism of Sandfish
4.2. The Interaction Mechanism between Bionic Sandfish Wedge and Soil
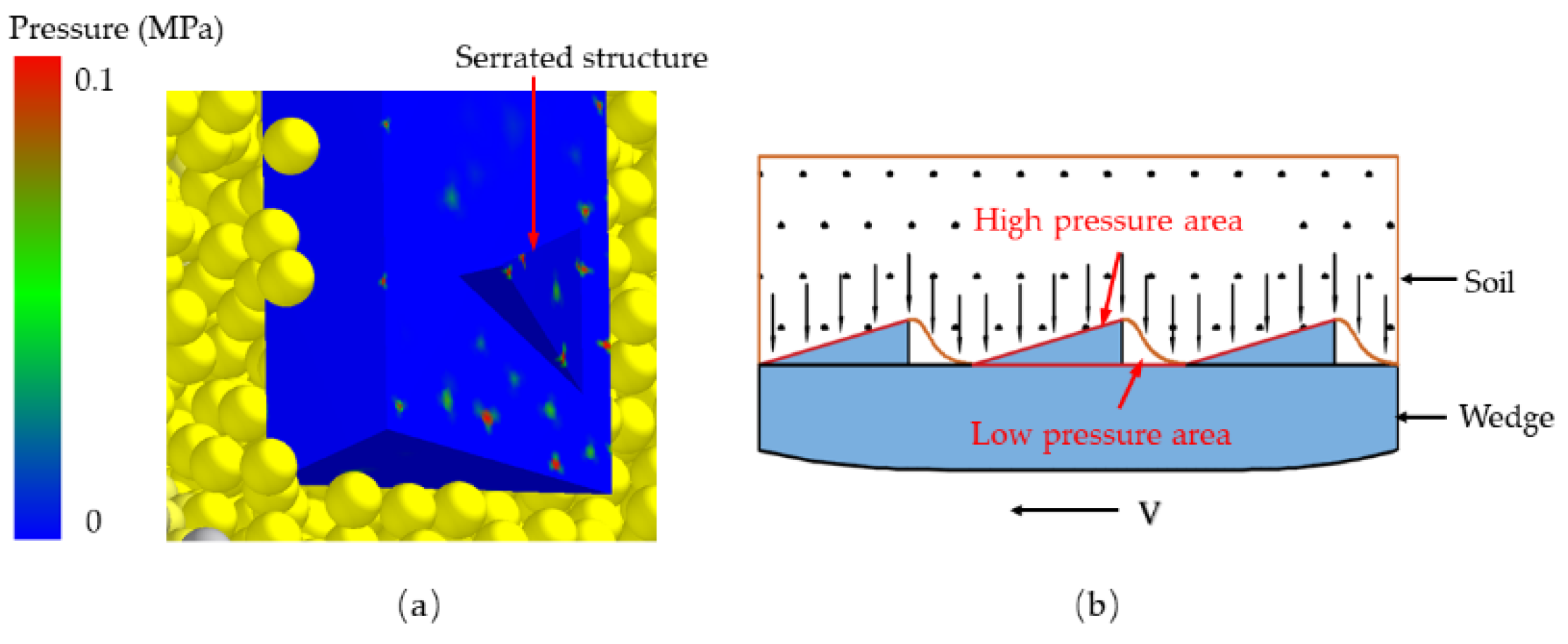
4.3. The Anti-Adhesion Mechanism of Bionic Serrated Structure
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Merkisz, J.; Lijewski, P.; Fuc, P.; Siedlecki, M.; Weymann, S. The Use of the PEMS Equipment for the Assessment of Farm Fieldwork Energy Consumption. Appl. Eng. Agric. 2015, 31, 875–879. [Google Scholar] [CrossRef]
- Scarpare, F.V.; de Jong van Lier, Q.; de Camargo, L.; Pires, R.C.M.; Ruiz-Corrêa, S.T.; Bezerra, A.H.F.; Gava, G.J.C.; Dias, C.T.S. Tillage Effects on Soil Physical Condition and Root Growth Associated with Sugarcane Water Availability. Soil. Tillage Res. 2019, 187, 110–118. [Google Scholar] [CrossRef]
- Zhou, D.; Hou, P.; Xin, Y.; Wu, B.; Tong, J.; Yu, H.; Qi, J.; Zhang, J.; Zhang, Q. Resistance and Consumption Reduction Mechanism of Bionic Vibration and Verification of Field Subsoiling Experiment. Appl. Sci. 2021, 11, 10480. [Google Scholar] [CrossRef]
- Wang, Y.; N Osman, A.; Zhang, D.; Yang, L.; Cui, T.; Zhong, X. Optimized Design and Field Experiment of a Staggered Vibrating Subsoiler for Conservation Tillage. Int. J. Agric. Biol. Eng. 2019, 12, 59–65. [Google Scholar] [CrossRef]
- Mehrang Marani, S.; Shahgholi, G.; Moinfar, A. Effect of Nano Coating Materials on Reduction of Soil Adhesion and External Friction. Soil. Tillage Res. 2019, 193, 42–49. [Google Scholar] [CrossRef]
- Wang, Y.; Zhang, D.; Yang, L.; Cui, T.; Jing, H.; Zhong, X. Modeling the Interaction of Soil and a Vibrating Subsoiler Using the Discrete Element Method. Comput. Electron. Agric. 2020, 174, 105518. [Google Scholar] [CrossRef]
- Zhang, X.R.; Wang, C.; Chen, Z.H.; Zeng, Z.W. Design and Experiment of a Bionic Vibratory Subsoiler for Banana Fields in Southern China. Int. J. Agric. Biol. Eng. 2016, 9, 75–83. [Google Scholar] [CrossRef]
- Massah, J.; Rahmani Fard, M.; Aghel, H. An Optimized Bionic Electro-Osmotic Soil-Engaging Implement for Soil Adhesion Reduction. J. Terramech 2021, 95, 1–6. [Google Scholar] [CrossRef]
- Li, J.; Tong, J.; Ren, L.; Chen, B. Applications of Bionics Non-Smooth Surface to Reduce Draft Resistance against Soil. In Proceedings of the 14th International Conference of the Society for Terrain-Vehicle Systems, Vicksburg, MS, USA, 1 January 2002; pp. 414–422. [Google Scholar]
- Ren, L.; Wang, S.; Tian, X.; Han, Z.; Yan, L.; Qiu, Z. Non-Smooth Morphologies of Typical Plant Leaf Surfaces and Their Anti-Adhesion Effects. J. Bionic Eng. 2007, 4, 33–40. [Google Scholar] [CrossRef]
- Li, J.; Tong, J.; Hu, B.; Ma, Y. Biomimetic Functional Surface of Reducing Soil Adhesion on 65Mn Steel. Adv. Mech. Eng. 2019, 11, 1687814019889801. [Google Scholar] [CrossRef]
- Zhang, Y.; Zhou, C.; Ren, L. Biology Coupling Characteristics of Mole Crickets’ Soil-Engaging Components. J. Bionic Eng. 2008, 5, 164–171. [Google Scholar] [CrossRef]
- Grützmacher, P.G.; Profito, F.J.; Rosenkranz, A. Multi-Scale Surface Texturing in Tribology-Current Knowledge and Future Perspectives. Lubricants 2019, 7, 95. [Google Scholar] [CrossRef]
- Hsu, S.M.; Jing, Y.; Zhao, F. Self-Adaptive Surface Texture Design for Friction Reduction across the Lubrication Regimes. Surf. Topogr. 2016, 4, 014004. [Google Scholar] [CrossRef]
- Schille, J.; Schneider, L.; Mauersberger, S.; Szokup, S.; Höhn, S.; Pötschke, J.; Reiß, F.; Leidich, E.; Löschner, U. High-Rate Laser Surface Texturing for Advanced Tribological Functionality. Lubricants 2020, 8, 33. [Google Scholar] [CrossRef]
- Costa, H.L.; Schille, J.; Rosenkranz, A. Tailored Surface Textures to Increase Friction—A Review. Friction 2022, 10, 1285–1304. [Google Scholar] [CrossRef]
- Ren, L. Progress in the Bionic Study on Anti-Adhesion and Resistance Reduction of Terrain Machines. Sci. China 2009, 52, 273–284. [Google Scholar] [CrossRef]
- Li, M.; Chen, D.; Zhang, S.; Tong, J. Biomimeitc Design of a Stubble-Cutting Disc Using Finite Element Analysis. J. Bionic Eng. 2013, 10, 118–127. [Google Scholar] [CrossRef]
- Xu, S.; Wang, C.; Mao, R.; Liang, X.; Wang, H.; Lin, Z.; Li, J.; Li, S.; Jiang, J.; Zhang, T.; et al. Surface Structure Change Properties: Auto-Soft Bionic Fibrous Membrane in Reducing Postoperative Adhesion. Bioact. Mater. 2022, 12, 16–29. [Google Scholar] [CrossRef]
- Luo, X.; Dong, X.; Zhao, H.; Hu, T.S.; Lan, X.; Ding, L.; Li, J.; Ni, H.; Contreras, J.A.; Zeng, H.; et al. Near-Infrared Responsive Gecko-Inspired Flexible Arm Gripper. Mater. Today Phys. 2022, 29, 100919. [Google Scholar] [CrossRef]
- Ding, Y.; Li, C.; Goldman, D.I. Swimming in the Desert. Phys. Today 2013, 66, 68–69. [Google Scholar] [CrossRef]
- Maladen, R.D.; Ding, Y.; Umbanhowar, P.B.; Kamor, A.; Goldman, D.I. Mechanical Models of Sandfish Locomotion Reveal Principles of High Performance Subsurface Sand-Swimming. J. R. Soc. Interface 2011, 8, 1332–1345. [Google Scholar] [CrossRef] [PubMed]
- Ding, Y.; Sharpe, S.S.; Masse, A.; Goldman, D.I. Mechanics of Undulatory Swimming in a Frictional Fluid. PLoS Comput. Biol. 2012, 8, e1002810. [Google Scholar] [CrossRef] [PubMed]
- Vihar, B.; Hanisch, F.G.; Baumgartner, W. Neutral Glycans from Sandfish Skin Can Reduce Friction of Polymers. J. R. Soc. Interface 2016, 13, 20160103. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Baumgartner, W.; Saxe, F.; Weth, A.; Hajas, D.; Sigumonrong, D.; Emmerlich, J.; Singheiser, M.; Bohme, W.; Schneider, J.M. The Sandfish’s Skin: Morphology, Chemistry and Reconstruction. J. Bionic Eng. 2007, 4, 1–9. [Google Scholar] [CrossRef]
- Wu, W.; Lutz, C.; Mersch, S.; Thelen, R.; Greiner, C.; Gomard, G.; Hölscher, H. Characterization of the Microscopic Tribological Properties of Sandfish (Scincus scincus) Scales by Atomic Force Microscopy. Beilstein J. Nanotechnol. 2018, 9, 2618–2627. [Google Scholar] [CrossRef] [PubMed]
- Staudt, K.; Böhme, W.; Baumgartner, W. Comparative Investigations of the Sandfish’s β-Keratin (Reptilia: Scincidae: Scincus Scincus). Part 2: Glycan-Based Friction Reduction. J. Biomim. Biomater. Tissue Eng. 2012, 16, 1–9. [Google Scholar] [CrossRef]
- Staudt, K.; Saxe, F.P.M.; Schmied, H.; Soeur, R.; Böhme, W.; Baumgartner, W. Comparative Investigations of the Sandfish’s β-Keratin (Reptilia: Scincidae: Scincus scincus). Part 1: Surface and Molecular Examinations. J. Biomim. Biomater. Tissue Eng. 2012, 15, 1–16. [Google Scholar] [CrossRef]
- Goldman, D.I. Colloquium: Biophysical Principles of Undulatory Self-Propulsion in Granular Media. Rev. Mod. Phys. 2014, 86, 943–958. [Google Scholar] [CrossRef]
- Maladen, R.D.; Ding, Y.; Umbanhowar, P.B.; Goldman, D.I. Undulatory Swimming in Sand: Experimental and Simulation Studies of a Robotic Sandfish. Int. J. Rob. Res. 2011, 30, 793–805. [Google Scholar] [CrossRef]
- O’Kelly, B.C. Accurate Determination of Moisture Content of Organic Soils Using the Oven Drying Method. Dry. Technol. 2004, 22, 1767–1776. [Google Scholar] [CrossRef]
- Qiao, Y.; Zhu, H.; Zhong, H.; Li, Y. Stratified Data Reconstruction and Spatial Pattern Analyses of Soil Bulk Density in the Northern Grasslands of China. ISPRS Int. J. Geoinf. 2020, 9, 682. [Google Scholar] [CrossRef]
- Murano, H.; Takata, Y.; Isoi, T. Origin of the Soil Texture Classification System Used in Japan. Soil. Sci. Plant Nutr. 2015, 61, 688–697. [Google Scholar] [CrossRef]
- Yang, Q.; Shi, L.; Shi, A.; He, M.; Zhao, X.; Zhang, L.; Addy, M. Determination of Key Soil Characteristic Parameters Using Angle of Repose and Direct Shear Stress Test. Int. J. Agric. Biol. Eng. 2023, 16, 143–150. [Google Scholar] [CrossRef]
- Huang, G.; Jiao, G.H.; Wen, D.Z.; Zhou, C.Q.; Wu, K.M. Mechanical Properties of Heat Treated 65Mn Steel Produced by Compact Strip Production. Adv. Mat. Res. 2010, 168–170, 832–836. [Google Scholar] [CrossRef]
- Chen, J.; Krengel, D.; Nishiura, D.; Furuichi, M.; Matuttis, H.-G. A Force–Displacement Relation Based on the JKR Theory for DEM Simulations of Adhesive Particles. Powder Technol. 2023, 427, 118742. [Google Scholar] [CrossRef]
- Shaolong, S.; Zhihui, T.; Xuan, Z.; Jinbao, L.; Xiangjin, M.; Yuchao, L. Calibration of the Discrete Element Parameters for the Soil Model of Cotton Field after Plowing in Xinjiang of China. Trans. Chin. Soc. Agric. Eng. 2021, 37, 63–70. [Google Scholar]
- Huang, S.; Lu, C.; Li, H.; He, J.; Wang, Q.; Yuan, P.; Xu, J.; Jiang, S.; He, D. Calibration of Acoustic-Soil Discrete Element Model and Analysis of Influencing Factors on Accuracy. Remote Sens. 2023, 15, 943. [Google Scholar] [CrossRef]
- Zhao, J.Z.; Pan, G.; Gao, S. Research on the Mechanism of Drag Reduction by Wall Vibration through Lattice Boltzmann Method. Mod. Phys. Lett. B 2020, 34, 2050295. [Google Scholar] [CrossRef]
- Koolen, A.J.; Kuipers, H. Agricultural Soil Mechanics; Springer: Berlin/Heidelberg, Germany, 1983; Volume 13, ISBN 978-3-642-69012-9. [Google Scholar]
- Li, J.; Li, Y.; Zhang, Z. Characteristics of Sliding Resistance Reduction between Soil and Flexible Body Surface of Yellow Mouse’s Living Body. Trans. Chin. Soc. Agric. Mach. 2004, 35, 161–164. [Google Scholar]
- Sun, J.; Li, J.; Cheng, H.; Dai, Z.; Rer, L. Restudies on Body Surface of Dung Beetle and Application of Us Bionics Flexible Technique. J. Bionics Eng. 2004, 1, 53–60. [Google Scholar] [CrossRef]







| Physical Quantity | Group 1 | Group 2 | Group 3 |
|---|---|---|---|
| (g) | 499.52 | 499.48 | 500.32 |
| (g) | 410.56 | 415.12 | 412.52 |
| (%) | 21.67 | 20.32 | 21.28 |
| Physical Quantity | Group 1 | Group 2 | Group 3 |
|---|---|---|---|
| (kg) | 0.153 | 0.172 | 0.164 |
| (m3) | 9.8 × 10−5 | 9.8 × 10−5 | 9.8 × 10−5 |
| (kg·m−3) | 1561 | 1755 | 1673 |
| Soil Particle | Sand (0.02–2 mm) | Silt (0.002–0.02 mm) | Clay (<0.002 mm) |
|---|---|---|---|
| Mass percentage (%) | 57.26 | 34.09 | 8.65 |
| EDEM Parameters | Factor | Level | |||
|---|---|---|---|---|---|
| −1 | 0 | 1 | |||
| Bulk material | Poisson ratio | A | 0.3 | 0.4 | 0.5 |
| Solids density (kg·m−3) | B | 1600 | 2100 | 2600 | |
| Shear modulus (MPa) | C | 10 | 20 | 30 | |
| Particle–particle interaction | Coefficient of restitution | D | 0.01 | 0.2 | 0.4 |
| Coefficient of static friction | E | 0.2 | 0.7 | 1.2 | |
| Coefficient of rolling friction | F | 0.1 | 0.2 | 0.7 | |
| Particle–steel interaction | Coefficient of restitution | G | 0.01 | 0.2 | 0.4 |
| Coefficient of static friction | H | 0.2 | 0.7 | 1.2 | |
| Coefficient of rolling friction | J | 0.1 | 0.3 | 0.7 | |
| Physical interaction model | Hertz–Mindlin with JKR (J·m−2) | K | 0 | 2 | 4 |
| Run | Factor | Response (°) | ||||||||||
|---|---|---|---|---|---|---|---|---|---|---|---|---|
| A | B | C | D | E | F | G | H | J | K | L | ||
| 1 | 0.5 | 2600 | 30 | 0.01 | 0.2 | 0.1 | 0.4 | 0.2 | 0.7 | 4 | −1 | 32.65 |
| 2 | 0.5 | 2600 | 10 | 0.4 | 1.2 | 0.7 | 0.01 | 0.2 | 0.1 | 4 | −1 | 31.8 |
| 3 | 0.5 | 1600 | 10 | 0.01 | 1.2 | 0.1 | 0.4 | 1.2 | 0.1 | 4 | 1 | 32.36 |
| 4 | 0.3 | 1600 | 10 | 0.4 | 0.2 | 0.7 | 0.4 | 0.2 | 0.7 | 4 | 1 | 56.36 |
| 5 | 0.3 | 2600 | 30 | 0.01 | 1.2 | 0.7 | 0.4 | 0.2 | 0.1 | 0 | 1 | 46.63 |
| 6 | 0.3 | 2600 | 10 | 0.4 | 1.2 | 0.1 | 0.4 | 1.2 | 0.7 | 0 | −1 | 25.9 |
| 7 | 0.5 | 1600 | 30 | 0.4 | 1.2 | 0.1 | 0.01 | 0.2 | 0.7 | 0 | 1 | 23.37 |
| 8 | 0.3 | 1600 | 10 | 0.01 | 0.2 | 0.1 | 0.01 | 0.2 | 0.1 | 0 | −1 | 22.36 |
| 9 | 0.5 | 1600 | 30 | 0.4 | 0.2 | 0.7 | 0.4 | 1.2 | 0.1 | 0 | −1 | 45.16 |
| 10 | 0.5 | 2600 | 10 | 0.01 | 0.2 | 0.7 | 0.01 | 1.2 | 0.7 | 0 | 1 | 54.52 |
| 11 | 0.3 | 2600 | 30 | 0.4 | 0.2 | 0.1 | 0.01 | 1.2 | 0.1 | 4 | 1 | 27.47 |
| 12 | 0.3 | 1600 | 30 | 0.01 | 1.2 | 0.7 | 0.01 | 1.2 | 0.7 | 4 | −1 | 50.61 |
| Factor | Standardized Effect | Sum of Squares | Contribution | Significance |
|---|---|---|---|---|
| A-A | −1.58 | 7.49 | 1.25 | 8 |
| B-B | −1.88 | 10.57 | 1.76 | 7 |
| C-C | 0.43 | 0.5547 | 0.0924 | 11 |
| D-D | −4.84 | 70.37 | 11.72 | 2 |
| E-E | −4.64 | 64.68 | 10.77 | 4 |
| F-F | 10.16 | 309.88 | 51.61 | 1 |
| G-G | 4.82 | 69.79 | 11.62 | 3 |
| H-H | 3.81 | 43.47 | 7.24 | 5 |
| J-J | 1.27 | 4.86 | 0.8101 | 9 |
| K-K | 2.22 | 14.79 | 2.46 | 6 |
| AB | −5.37 | 4 | 0.6665 | 10 |
| Run | Significant Factors | Response (°) | Relative Error (%) | |||
|---|---|---|---|---|---|---|
| D | E | F | G | |||
| 1 | 0.4 | 1.2 | 0.1 | 0.01 | 21.16 | 60.08% |
| 2 | 0.3 | 0.95 | 0.25 | 0.1 | 31.11 | 40.87% |
| 3 | 0.2 | 0.7 | 0.4 | 0.2 | 42.67 | 19.49% |
| 4 | 0.1 | 0.45 | 0.55 | 0.3 | 50.76 | 4.23% |
| 5 | 0.01 | 0.2 | 0.7 | 0.4 | 62.55 | 18.02% |
| Run | Factor | Response (°) | |||
|---|---|---|---|---|---|
| D | E | F | G | ||
| 1 | 0.105 | 0.7 | 0.55 | 0.4 | 45.52 |
| 2 | 0.105 | 0.45 | 0.55 | 0.3 | 48.54 |
| 3 | 0.01 | 0.2 | 0.55 | 0.3 | 62.37 |
| 4 | 0.2 | 0.45 | 0.55 | 0.4 | 53.39 |
| 5 | 0.105 | 0.2 | 0.55 | 0.4 | 55.94 |
| 6 | 0.105 | 0.2 | 0.4 | 0.3 | 47.9 |
| 7 | 0.2 | 0.45 | 0.7 | 0.3 | 61.38 |
| 8 | 0.2 | 0.45 | 0.55 | 0.2 | 42.46 |
| 9 | 0.2 | 0.7 | 0.55 | 0.3 | 45.4 |
| 10 | 0.2 | 0.2 | 0.55 | 0.3 | 59.94 |
| 11 | 0.2 | 0.45 | 0.4 | 0.3 | 31.18 |
| 12 | 0.105 | 0.45 | 0.55 | 0.3 | 52.23 |
| 13 | 0.01 | 0.45 | 0.55 | 0.4 | 55.95 |
| 14 | 0.105 | 0.45 | 0.4 | 0.4 | 44.27 |
| 15 | 0.105 | 0.45 | 0.7 | 0.2 | 60.94 |
| 16 | 0.105 | 0.45 | 0.55 | 0.3 | 52.15 |
| 17 | 0.105 | 0.7 | 0.7 | 0.3 | 52.68 |
| 18 | 0.105 | 0.2 | 0.55 | 0.2 | 58.34 |
| 19 | 0.105 | 0.7 | 0.55 | 0.2 | 48.62 |
| 20 | 0.105 | 0.45 | 0.55 | 0.3 | 51.82 |
| 21 | 0.105 | 0.45 | 0.4 | 0.2 | 41.12 |
| 22 | 0.01 | 0.45 | 0.4 | 0.3 | 46.97 |
| 23 | 0.01 | 0.45 | 0.7 | 0.3 | 66.67 |
| 24 | 0.105 | 0.45 | 0.7 | 0.4 | 55.18 |
| 25 | 0.01 | 0.45 | 0.55 | 0.2 | 76.03 |
| 26 | 0.01 | 0.7 | 0.55 | 0.3 | 68.02 |
| 27 | 0.105 | 0.2 | 0.7 | 0.3 | 71.68 |
| 28 | 0.105 | 0.7 | 0.4 | 0.3 | 39.11 |
| 29 | 0.105 | 0.45 | 0.55 | 0.3 | 53.52 |
| EDEM Simulation Parameters | Factor | Value |
|---|---|---|
| Sandy loam soil | Poisson ratio | 0.4 |
| Solids density (kg·m−3) | 2100 | |
| Shear modulus (MPa) | 20 | |
| 65 Mn steel | Poisson ratio | 0.3 |
| Solids density (kg·m−3) | 7850 | |
| Shear modulus (MPa) | 7.9 × 104 | |
| Particle–particle interaction | Coefficient of restitution | 0.187 |
| Coefficient of static friction | 0.54 | |
| Coefficient of rolling friction | 0.462 | |
| Particle–steel interaction | Coefficient of restitution | 0.355 |
| Coefficient of static friction | 0.7 | |
| Coefficient of rolling friction | 0.5 | |
| Physical interaction model | Hertz–Mindlin with JKR (J·m−2) | 4 |
| Factor | Level | |
|---|---|---|
| −1 | 1 | |
| Distribution method | parallel | staggered |
| Distribution density | sparse | dense |
| Serrated tail height | low | high |
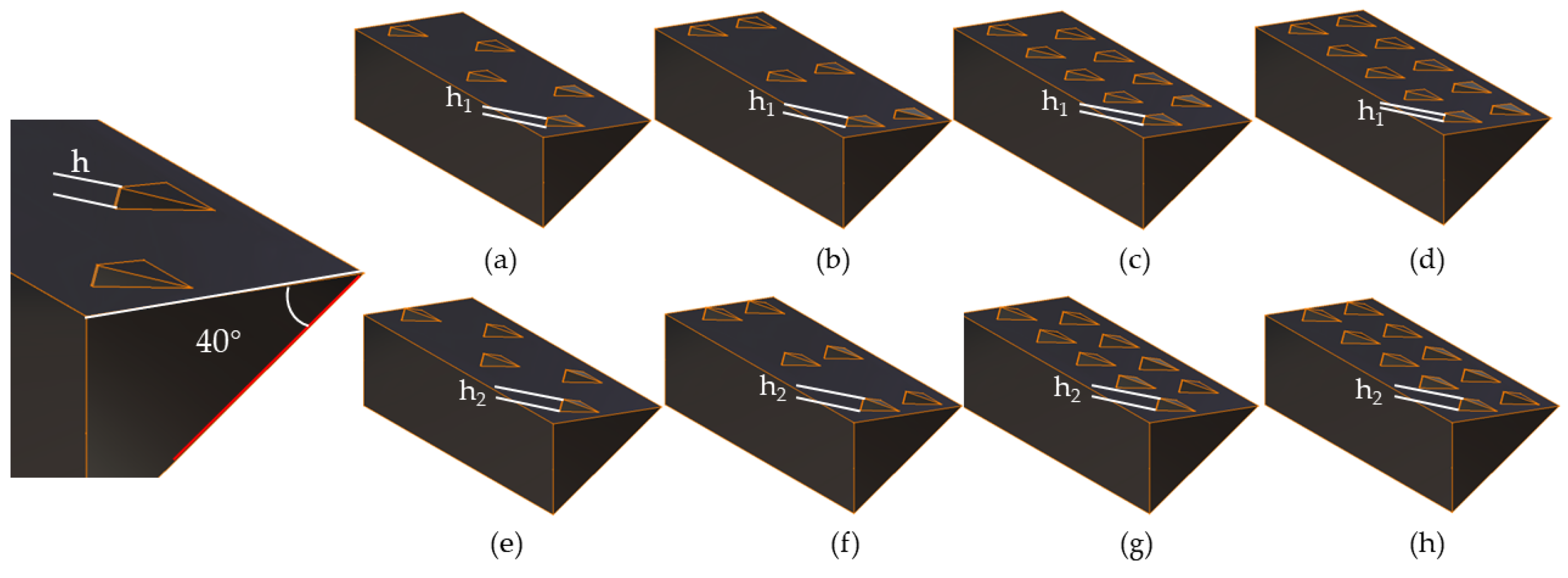
| Wedges | Wedge of 40° | a | b | c | d | e | f | g | h |
|---|---|---|---|---|---|---|---|---|---|
| Motion Resistance | 87.09 | 80.89 | 81.30 | 80.93 | 81.16 | 78.01 | 78.87 | 79.40 | 79.50 |
| Drag Reduction Percentage | 7.12% | 6.65% | 7.07% | 6.81% | 10.73% | 9.44% | 8.83% | 8.72% |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, J.; Qi, H.; Ma, Y.; Gao, P.; Wu, B. Simulation and Structural Analysis of a Flexible Coupling Bionic Desorption Mechanism Based on the Engineering Discrete Element Method. Biomimetics 2024, 9, 224. https://doi.org/10.3390/biomimetics9040224
Li J, Qi H, Ma Y, Gao P, Wu B. Simulation and Structural Analysis of a Flexible Coupling Bionic Desorption Mechanism Based on the Engineering Discrete Element Method. Biomimetics. 2024; 9(4):224. https://doi.org/10.3390/biomimetics9040224
Chicago/Turabian StyleLi, Jinguang, Hongyan Qi, Yunhai Ma, Peng Gao, and Baoguang Wu. 2024. "Simulation and Structural Analysis of a Flexible Coupling Bionic Desorption Mechanism Based on the Engineering Discrete Element Method" Biomimetics 9, no. 4: 224. https://doi.org/10.3390/biomimetics9040224
APA StyleLi, J., Qi, H., Ma, Y., Gao, P., & Wu, B. (2024). Simulation and Structural Analysis of a Flexible Coupling Bionic Desorption Mechanism Based on the Engineering Discrete Element Method. Biomimetics, 9(4), 224. https://doi.org/10.3390/biomimetics9040224







