Study of the Influence of Boundary Conditions on Corneal Deformation Based on the Finite Element Method of a Corneal Biomechanics Model
Abstract
1. Introduction
2. Materials and Methods
2.1. Patients
2.2. Material Definition
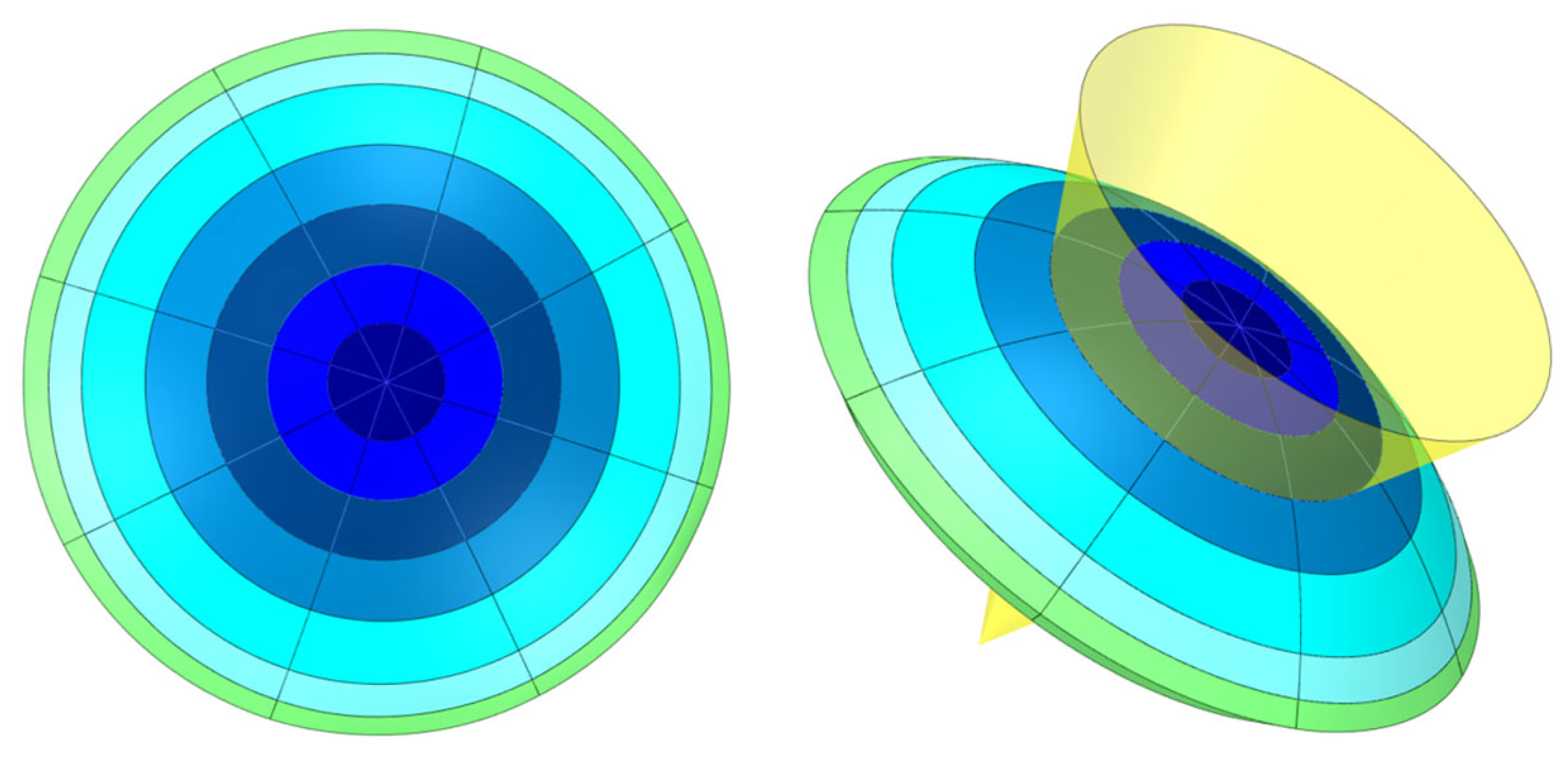
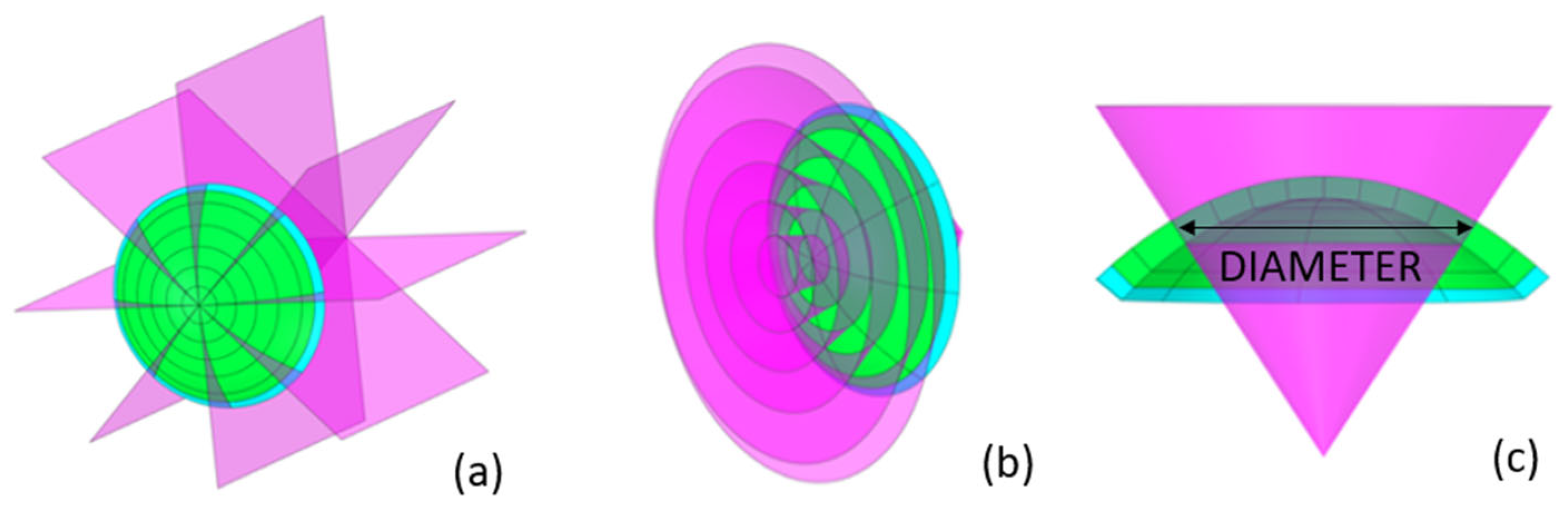
2.3. Finite Element Model Definition
2.4. Calculation of the Stress and Strain Field in the Measurement Phase
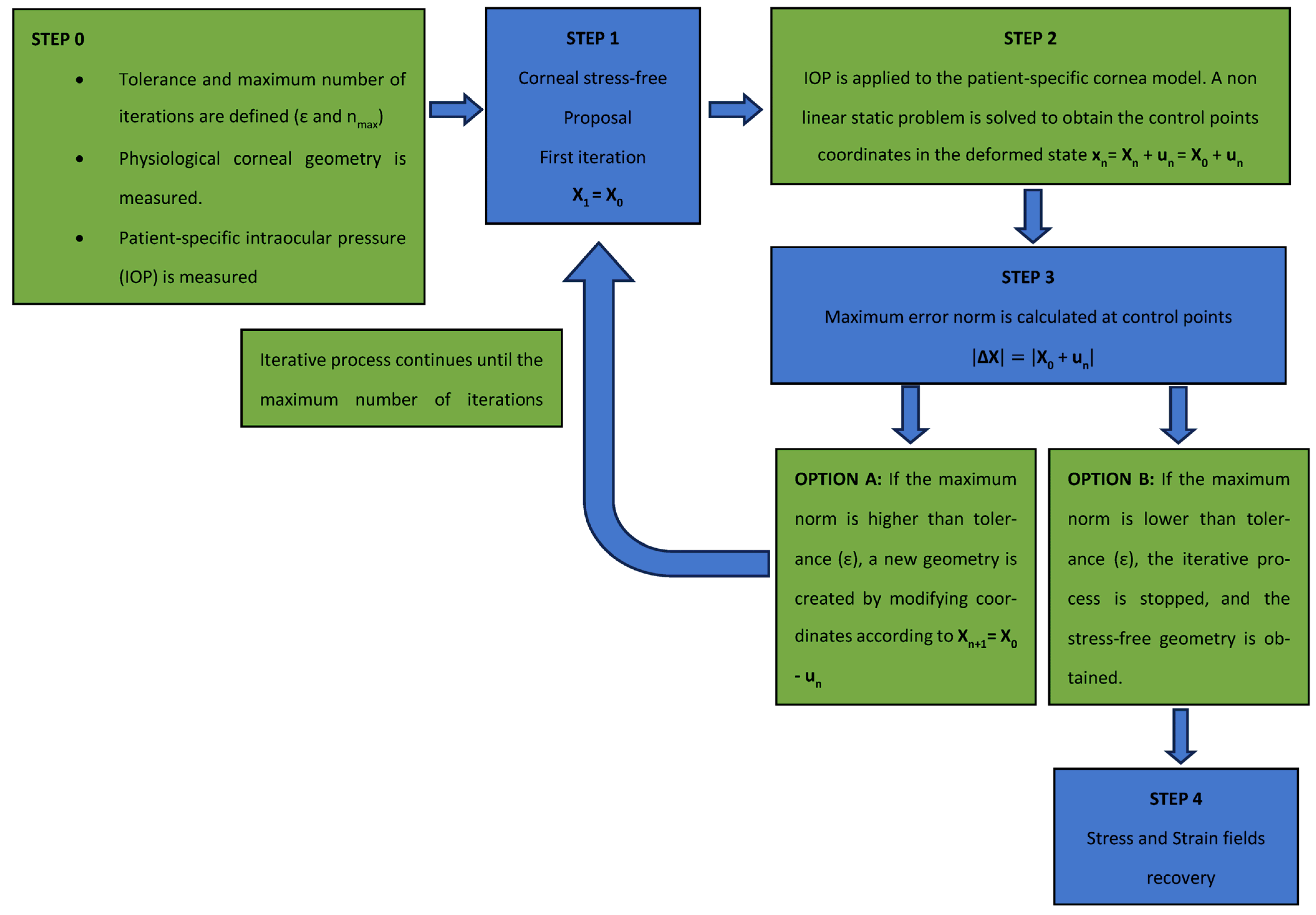
2.4.1. Displacements Method
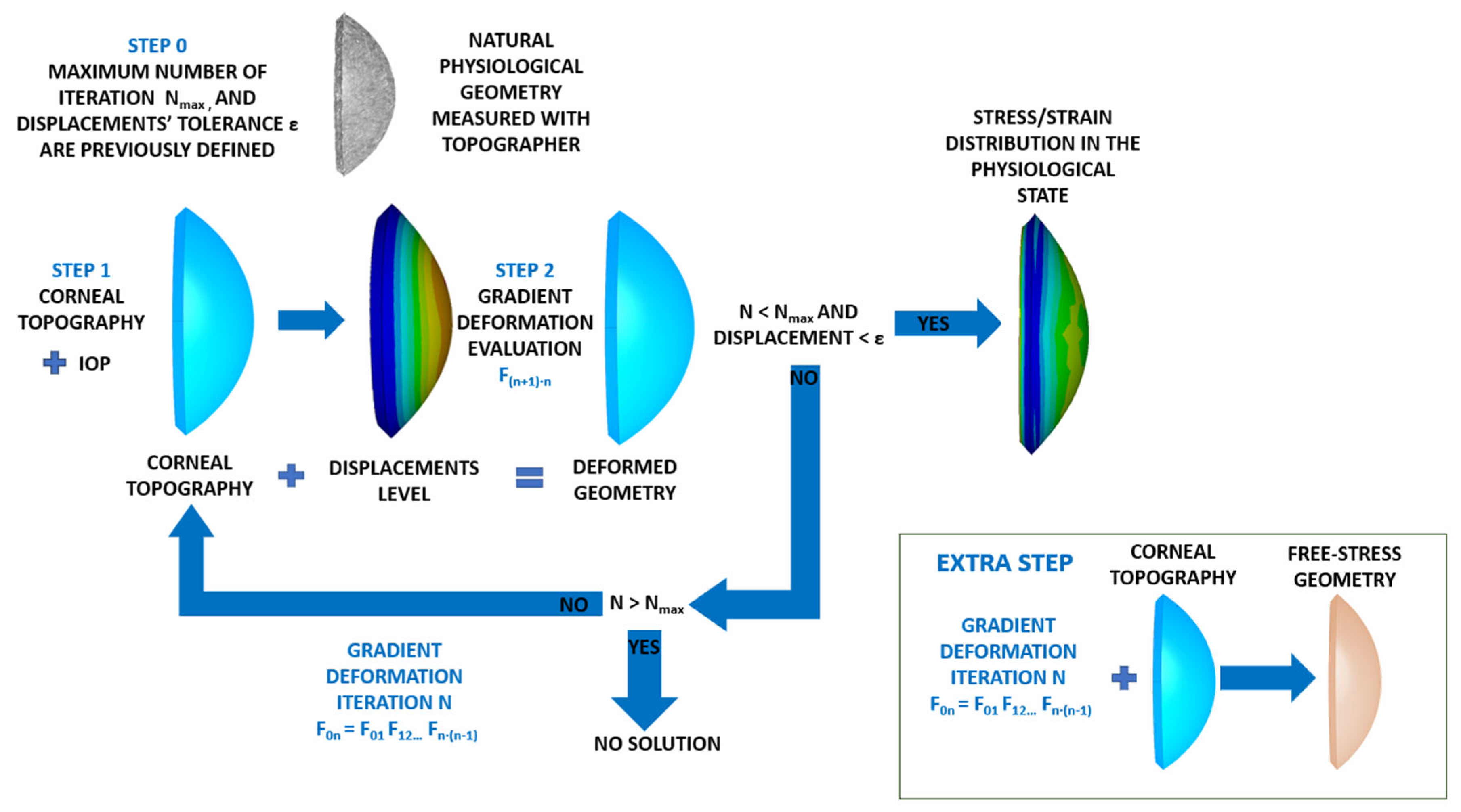
2.4.2. Prestress Method
2.5. Calculation of Displacements and the Stress Field in the Measurement Phase
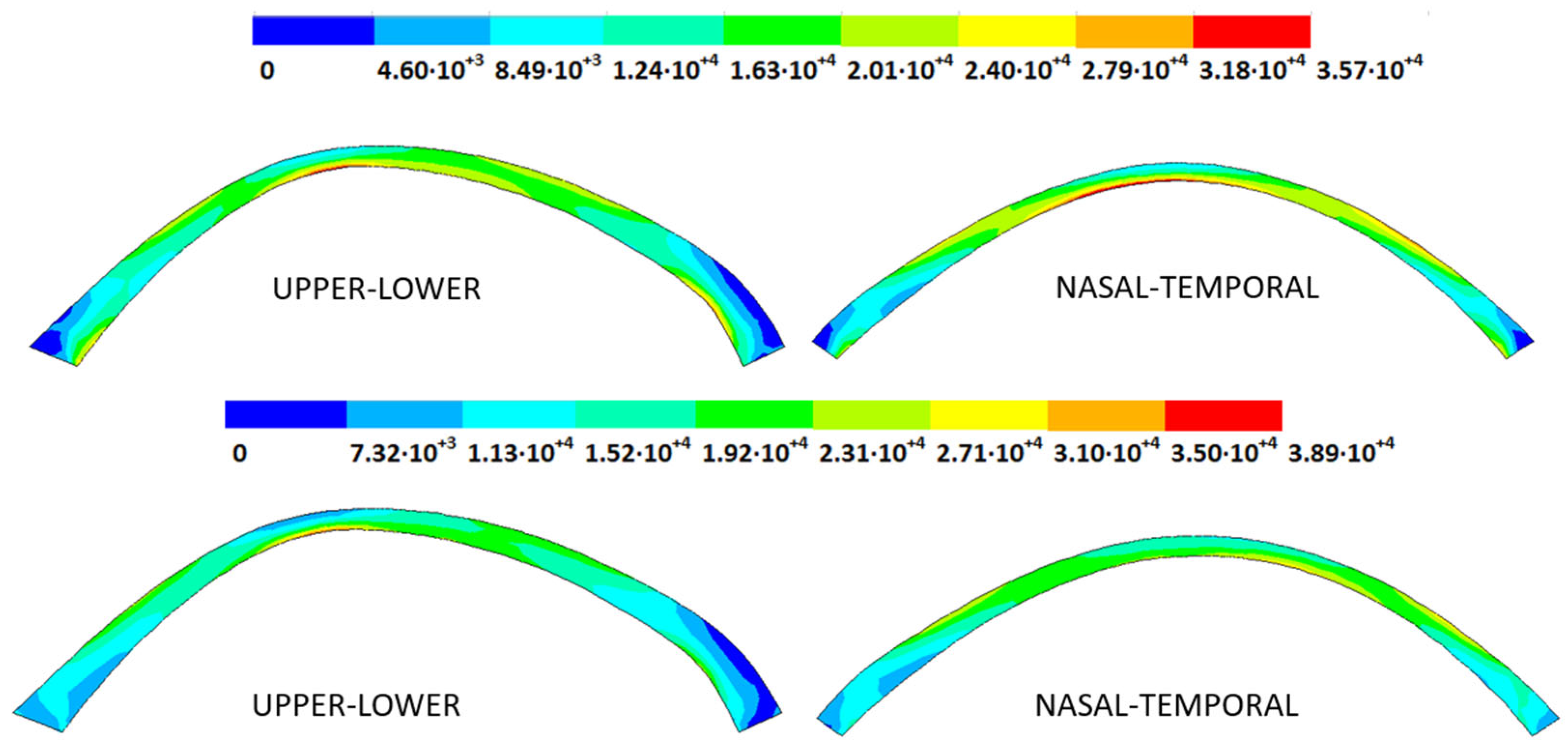
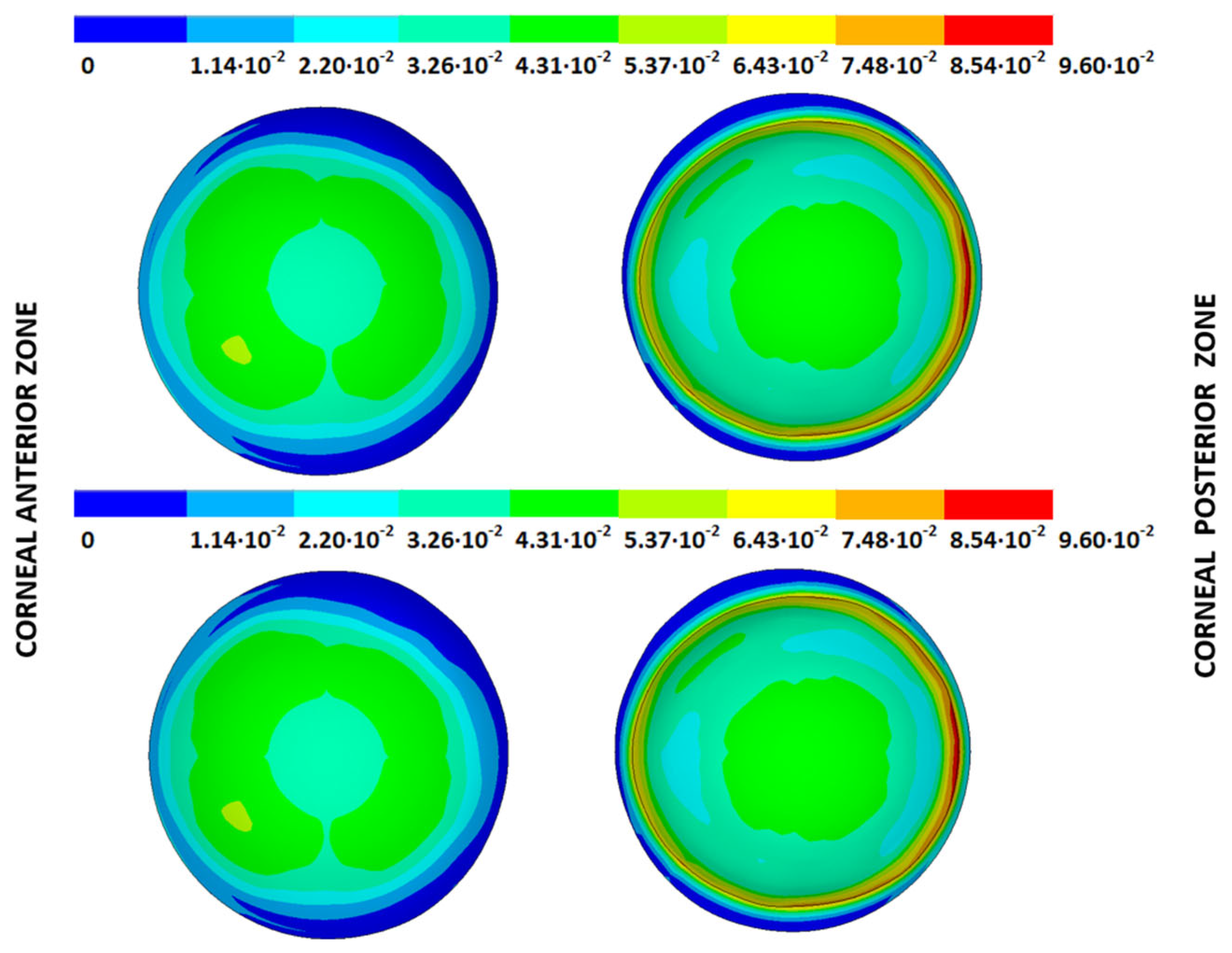
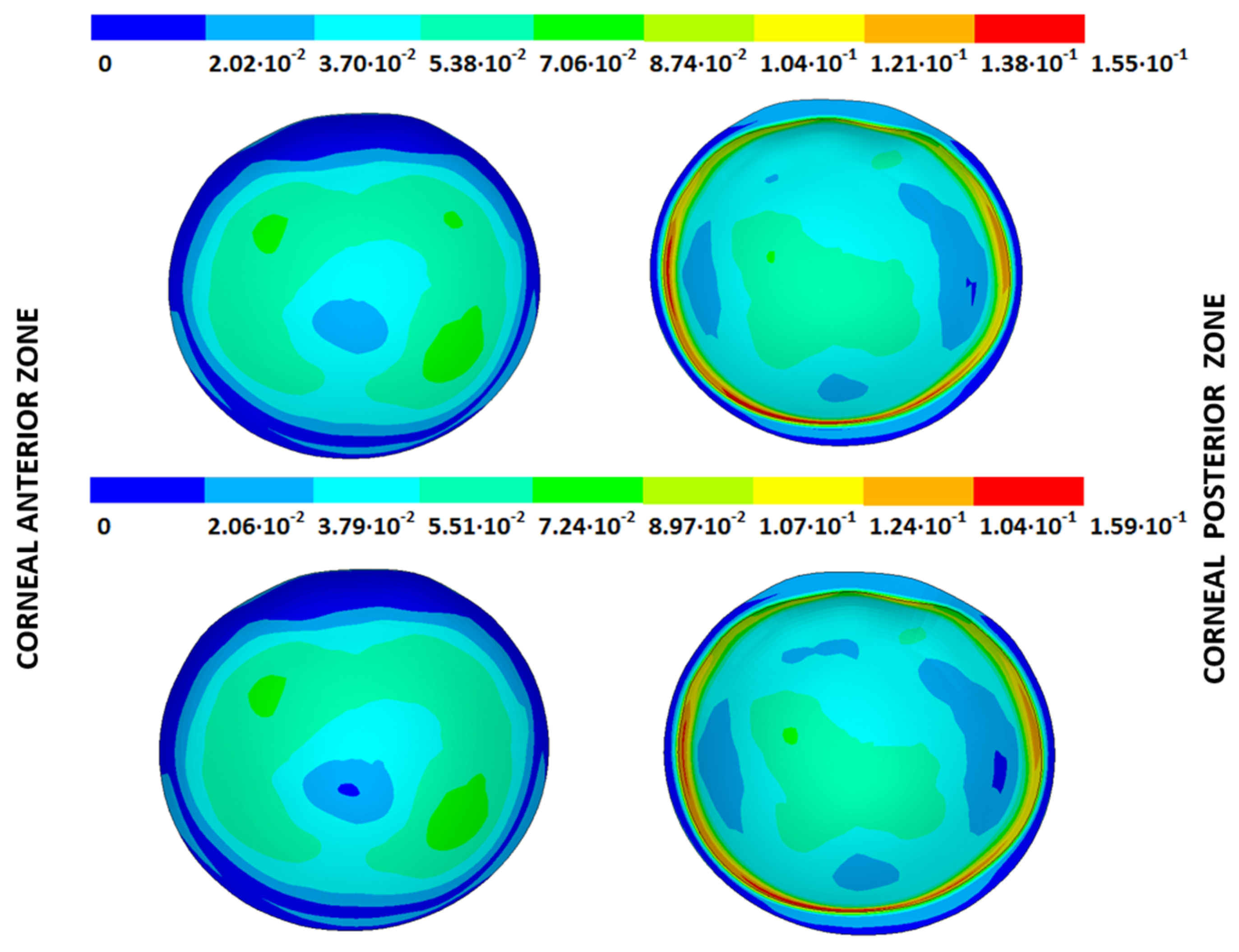
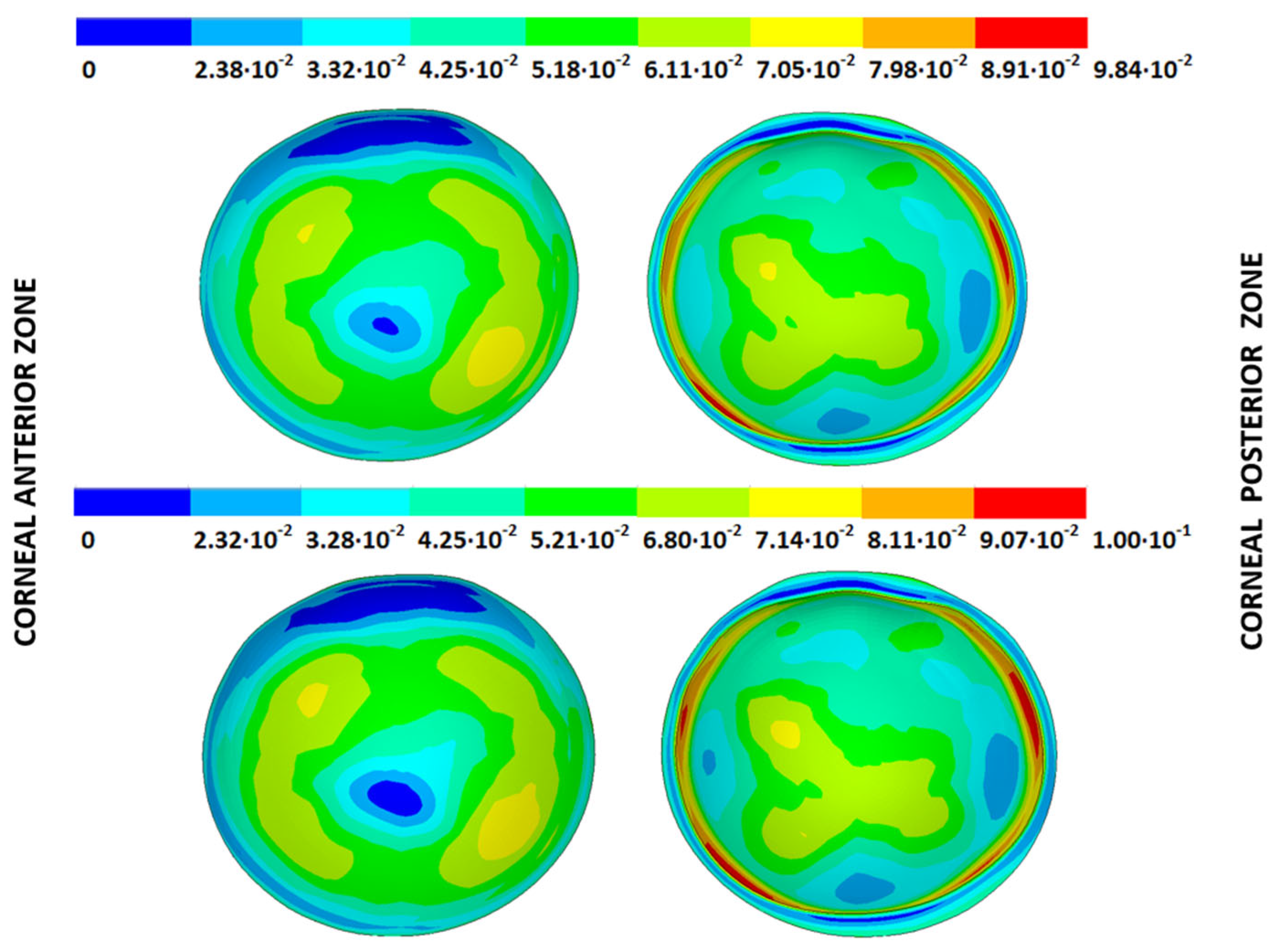
3. Results and Discussion
3.1. Stress-Free Geometry (SFG)
3.2. Estimated Physiological Geometry (EPG)
3.3. Stress Fields in the Estimated Physiological Geometry
3.4. Strain Fields in the Physiological State
3.5. Computational Time Analysis
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
List of Acronyms
| EGP | Estimated physiological geometry |
| SFG | Stress-free geometry |
| IOP | Intraocular pressure |
| Material constant of the Mooney–Rivlin model | |
| k0 | Bulk factor |
| k1 | Hyperelastic material parameter to consider the influence of collagen fibril stiffness. |
| k2 | Hyperelastic material parameter to consider the influence of collagen fibrils in large extensions. |
| Jacobian of the deformation gradient tensor | |
| nmax | Maximum number of iterations |
| Isochoric Cauchy–Green deformation tensor | |
| F | Deformation gradient tensor |
| Fnm | Deformation gradient tensor from step n to step m |
| Initial preferential orientation of collagen fibril family 1. | |
| Deformed preferential orientation of collagen fibril family 1. | |
| Initial preferential orientation of collagen fibril family 2. | |
| Deformed preferential orientation of collagen fibril family 2. | |
| x | Deformed vector |
| xn | Deformed vector in the iteration n |
| X | Reference vector |
| Xn | Reference vector in the iteration n |
| Displacement vector in the iteration n | |
| Strain energy density | |
| Volumetric strain energy density | |
| Isotropic isochoric strain energy density | |
| Anisotropic isochoric strain energy density | |
| (i = 1,9) | Invariants of the modified right Cauchy stress tensor |
| ε | Tolerance |
References
- Piñero, D.P.; Alcón, N. Corneal biomechanics: A review. Clin. Exp. Optom. 2015, 98, 107–116. [Google Scholar] [CrossRef] [PubMed]
- Pandolfi, A. The influence of the collagen architecture on the mechanical response of the human cornea. In Computational Methods in Applied Sciences; Springer Nature: Cham, Switzerland, 2018; Volume 46, pp. 337–355. [Google Scholar]
- Meek, K.M.; Boote, C. The use of X-ray scattering techniques to quantify the orientation and distribution of collagen in the corneal stroma. Prog. Retin. Eye Res. 2009, 28, 369–392. [Google Scholar] [CrossRef] [PubMed]
- Jin, S.X.; Dackowski, E.; Chuck, R.S. Risk factors for postlaser refractive surgery corneal ectasia. Curr. Opin. Ophthalmol. 2020, 31, 288–292. [Google Scholar] [CrossRef] [PubMed]
- Mas Tur, V.; MacGregor, C.; Jayaswal, R.; O’Brart, D.; Maycock, N. A review of keratoconus: Diagnosis, pathophysiology, and genetics. Surv. Ophthalmol. 2017, 62, 770–783. [Google Scholar] [CrossRef] [PubMed]
- Roberts, C.J.; Dupps, W.J., Jr. Biomechanics of corneal ectasia and biomechanical treatments. J. Cataract. Refract. Surg. 2014, 40, 991–998. [Google Scholar] [CrossRef]
- D’Oria, F.; Bagaglia, S.A.; Alio del Barrio, J.L.; Alessio, G.; Alio, J.L.; Mazzotta, C. Refractive surgical correction and treatment of keratoconus. Surv. Ophthalmol. 2024, 69, 122–139. [Google Scholar] [CrossRef]
- Pandolfi, A. Cornea modelling. Eye Vis. 2020, 7, 2. [Google Scholar] [CrossRef]
- Li, F.; Wang, K.; Liu, Z. In Vivo Biomechanical Measurements of the Cornea. Bioengineering 2023, 10, 120. [Google Scholar]
- Canales, C.; García-Herrera, C.; Rivera, E.; Macías, D.; Celentano, D. Anisotropic Hyperelastic Material Characterization: Stability Criterion and Inverse Calibration with Evolutionary Strategies. Mathematics 2023, 11, 922. [Google Scholar] [CrossRef]
- Simonini, I.; Angelillo, M.; Pandolfi, A. Theoretical and numerical analysis of the corneal air puff test. J. Mech. Phys. Solids 2016, 93, 118–134. [Google Scholar] [CrossRef]
- Chagnon, G.; Rebouah, M.; Favier, D. Hyperelastic Energy Densities for Soft Biological Tissues: A Review. J. Elast. 2015, 120, 129–160. [Google Scholar] [CrossRef]
- Zhang, J.; Zhong, Y.; Gu, C. Deform. Models for Surgical Simulation: A Survey. IEEE Rev. Biomed. Eng. 2018, 11, 143–164. [Google Scholar] [CrossRef]
- Alastrué, V.; Calvo, B.; Peña, E.; Doblaré, M. Biomechanical modeling of refractive corneal surgery. J. Biomech. Eng. 2006, 128, 150–160. [Google Scholar] [CrossRef]
- Daxer, A.; Fratzl, P. Collagen fibril orientation in the human corneal stroma and its implication in keratoconus. Investig. Ophthalmol. Vis. Sci. 1997, 38, 121–129. [Google Scholar]
- Whitford, C.; Studer, H.; Boote, C.; Meek, K.M.; Elsheikh, A. Biomechanical model of the human cornea: Considering shear stiffness and regional variation of collagen anisotropy and density. J. Mech. Behav. Biomed. Mater. 2015, 42, 76–87. [Google Scholar] [CrossRef] [PubMed]
- Issarti, I.; Koppen, C.; Rozema, J.J. Influence of the eye globe design on biomechanical analysis. Comput. Biol. Med. 2021, 135, 104612. [Google Scholar] [CrossRef] [PubMed]
- Nejad, T.M.; Foster, C.; Gongal, D. Finite element modelling of cornea mechanics: A review. Arq. Bras. Oftalmol. 2014, 77, 60–65. [Google Scholar] [CrossRef] [PubMed]
- Nguyen, B.A.; Roberts, C.J.; Reilly, M.A. Biomechanical Impact of the Sclera on Corneal Deformation Response to an Air-Puff: A Finite-Element Study. Front. Bioeng. Biotechnol. 2019, 6, 210. [Google Scholar] [CrossRef] [PubMed]
- Fang, L.; Wang, Y.; Yang, R.; Deng, S.; Deng, J.; Wan, L. Effects of the LASIK flap thickness on corneal biomechanical behavior: A finite element analysis. BMC Ophthalmol. 2020, 20, 67. [Google Scholar] [CrossRef] [PubMed]
- Martínez-Abad, A.; Piñero, D.P. New perspectives on the detection and progression of keratoconus. J. Cataract. Refract. Surg. 2017, 43, 1213–1227. [Google Scholar] [CrossRef] [PubMed]
- Lanchares, E.; Calvo, B.; Cristóbal, J.A.; Doblaré, M. Finite element simulation of arcuates for astigmatism correction. J. Biomech. 2008, 41, 797–805. [Google Scholar] [CrossRef]
- Pandolfi, A.; Holzapfel, G.A. Three-dimensional modeling and computational analysis of the human cornea considering distributed collagen fibril orientations. J. Biomech. Eng. 2008, 130, 061006. [Google Scholar] [CrossRef]
- Pandolfi, A.; Manganiello, F. A model for the human cornea. Constitutive behavior and numerical analysis. Biomech. Model. Mechanobiol. 2006, 5, 237–246. [Google Scholar] [CrossRef]
- Whitford, C.; Movchan, N.V.; Studer, H.; Elsheikh, A. A viscoelastic anisotropic hyperelastic constitutive model of the human cornea. Biomech. Model. Mechanobiol. 2018, 17, 19–29. [Google Scholar] [CrossRef] [PubMed]
- Falgayrettes, N.; Patoor, E.; Cleymand, F.; Zevering, Y.; Perone, J.-M. Biomechanics of keratoconus: Two numerical studies. PLoS ONE 2023, 18, e0278455. [Google Scholar] [CrossRef] [PubMed]
- Muench, S.; Roellig, M.; Balzani, D. A new Method for the in vivo identification of material properties of the human eye: Feasibility analysis based on synthetic data. Biomech. Model. Mechanobiol. 2021, 21, 401–418. [Google Scholar] [CrossRef] [PubMed]
- Rahmati, S.M.; Razaghi, R.; Karimi, A. Biomechanics of the keratoconic cornea: Theory, segmentation, pressure distribution, and coupled FE-optimization algorithm. J. Mech. Behav. Biomed. Mater. 2021, 113, 104155. [Google Scholar] [CrossRef] [PubMed]
- Simonini, I.; Pandolfi, A. Customized finite element modelling of the human cornea. PLoS ONE 2015, 10, e0130426. [Google Scholar] [CrossRef] [PubMed]
- Cavas-Martínez, F.; Bataille, L.; Fernández-Pacheco, D.G.; Cañavate, F.J.F.; Alio, J.L. Keratoconus Detection Based on a New Corneal Volumetric Analysis. Sci. Rep. 2017, 7, 15837. [Google Scholar] [CrossRef] [PubMed]
- Toprak, I.; Cavas, F.; Velázquez, J.S.; del Barrio, J.L.A.; Alio, J.L. A three-dimensional morpho-volumetric similarity study of Down syndrome keratopathy vs. keratoconus. Eye Vis. 2023, 10, 4. [Google Scholar] [CrossRef]
- Cavas-Martínez, F.; Bataille, L.; Fernández-Pacheco, D.G.; Cañavate, F.J.F.; Alió, J.L. A new approach to keratoconus detection based on corneal morphogeometric analysis. PLoS ONE 2017, 12, e0184569. [Google Scholar] [CrossRef]
- Moore, J.; Shu, X.; Lopes, B.T.; Wu, R.; Abass, A. Limbus misrepresentation in parametric eye models. PLoS ONE 2020, 15, e0236096. [Google Scholar] [CrossRef] [PubMed]
- Corbett, M.; Maycock, N.; Rosen, E.; O’Brart, D. Normal Topography. In Corneal Topography: Principles and Applications; Corbett, M., Maycock, N., Rosen, E., O’Brart, D., Eds.; Springer International Publishing: Cham, Switzerland, 2019; pp. 85–102. [Google Scholar] [CrossRef]
- Elsheikh, A.; Whitford, C.; Hamarashid, R.; Kassem, W.; Joda, A.; Büchler, P. Stress free configuration of the human eye. Med. Eng. Phys. 2013, 35, 211–216. [Google Scholar] [CrossRef] [PubMed]
- Pandolfi, A.; Montanino, A. On the recovery of the stress-free configuration of the human cornea. Model. Artif. Intell. Ophthalmol. 2020, 2, 11–33. [Google Scholar] [CrossRef]
- Gee, M.W.; Förster, C.; Wall, W.A. A computational strategy for prestressing patient-specific biomechanical problems under finite deformation. Int. J. Numer. Methods Biomed. Eng. 2010, 26, 52–72. [Google Scholar] [CrossRef]
- Grytz, R.; Downs, J.C. A forward incremental prestressing method with application to inverse parameter estimations and eye-specific simulations of posterior scleral shells. Comput. Methods Biomech. Biomed. Eng. 2013, 16, 768–780. [Google Scholar] [CrossRef]
- Carvalho, L.A.; Prado, M.; Cunha, R.H.; Costa Neto, A.; Paranhos, A., Jr.; Schor, P.; Chamon, W. Keratoconus prediction using a finite element model of the cornea with local biomechanical properties. Arq. Bras. Oftalmol. 2009, 72, 139–145. [Google Scholar] [CrossRef]
- Sinha Roy, A.; Dupps, W.J., Jr. Patient-specific computational modeling of keratoconus progression and differential responses to collagen cross-linking. Investig. Ophthalmol. Vis. Sci. 2011, 52, 9174–9187. [Google Scholar] [CrossRef] [PubMed]
- Voorhees, A.; Ho, L.; Jan, N.-J.; Tran, H.; van der Merwe, Y.; Chan, K.; Sigal, I. Whole-globe biomechanics using high-field MRI. Exp. Eye Res. 2017, 160, 85–95. [Google Scholar] [CrossRef] [PubMed]
- Chang, S.H.; Zhou, D.; Eliasy, A.; Elsheikh, Y.C.L.A. Experimental evaluation of stiffening effect induced by UVA/Riboflavin corneal crosslinking using intact porcine eye globes. PLoS ONE 2020, 15, e0240724. [Google Scholar] [CrossRef]
- Montanino, A.; Gizzi, A.; Vasta, M.; Angelillo, M.; Pandolfi, A. Modeling the biomechanics of the human cornea accounting for local variations of the collagen fibril architecture. ZAMM—J. Appl. Math. Mech./Z. Für Angew. Math. Und Mech. 2018, 98, 2122–2134. [Google Scholar] [CrossRef]
- Miller, K.; Joldes, G.; Lance, D.; Wittek, A. Total Lagrangian Explicit Dynamics Finite Element Algorithm for computing soft tissue deformation. Commun. Numer. Methods Eng. 2006, 23, 121–134. [Google Scholar] [CrossRef]
- Zhang, J. A direct Jacobian total Lagrangian explicit dynamics finite element algorithm for real-time simulation of hyperelastic materials. Int. J. Numer. Methods Eng. 2021, 122, 5744–5772. [Google Scholar] [CrossRef]








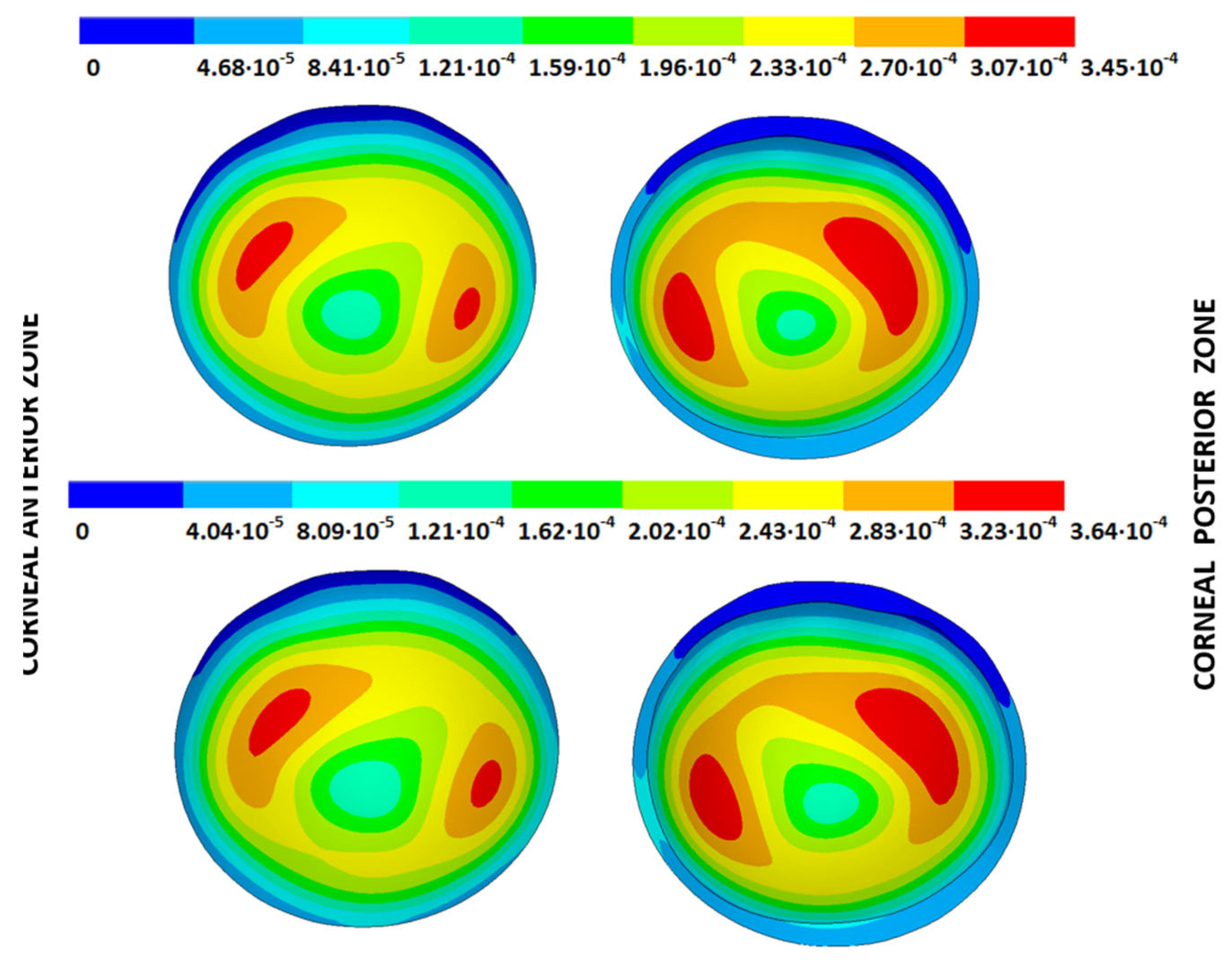

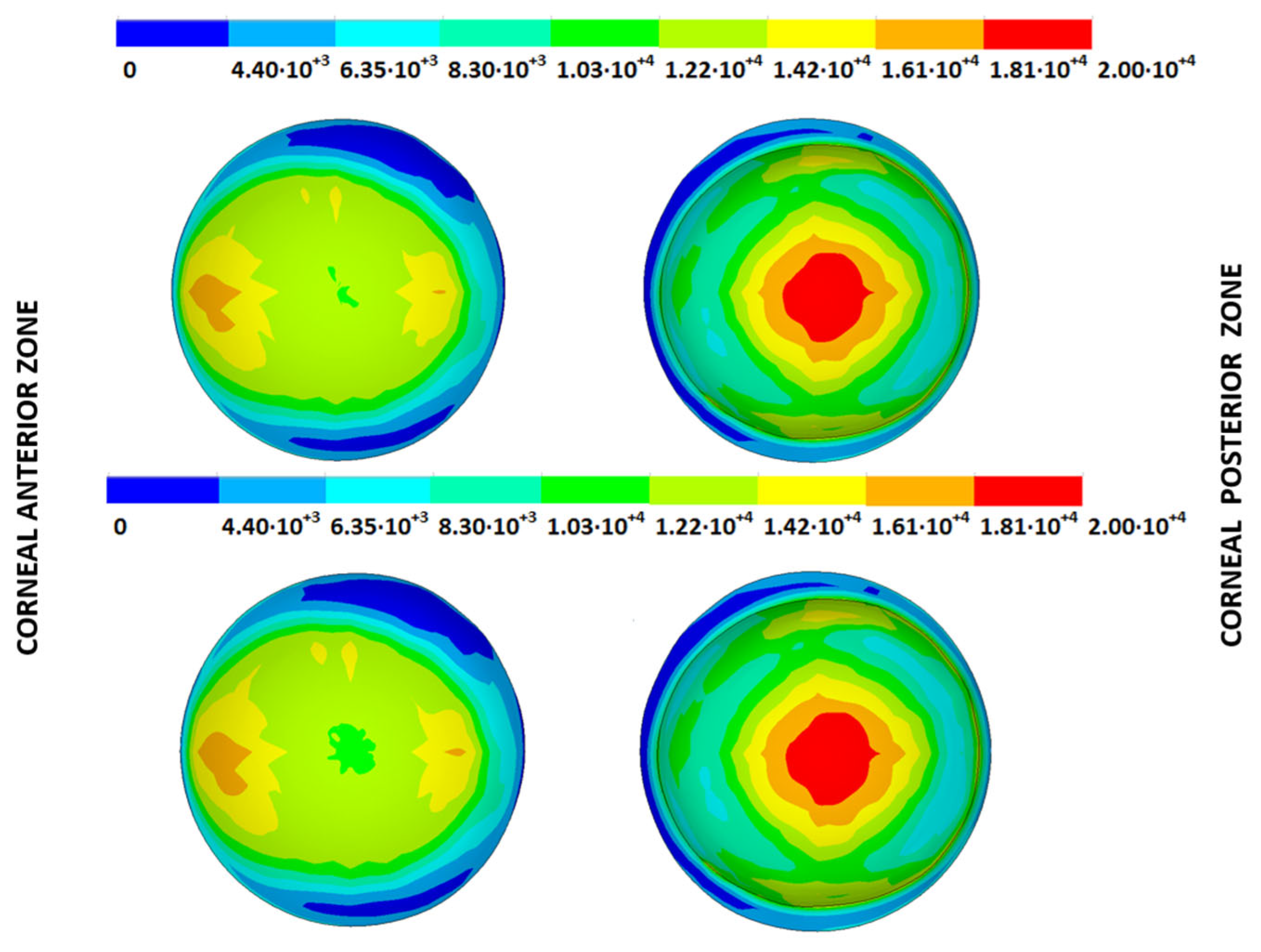
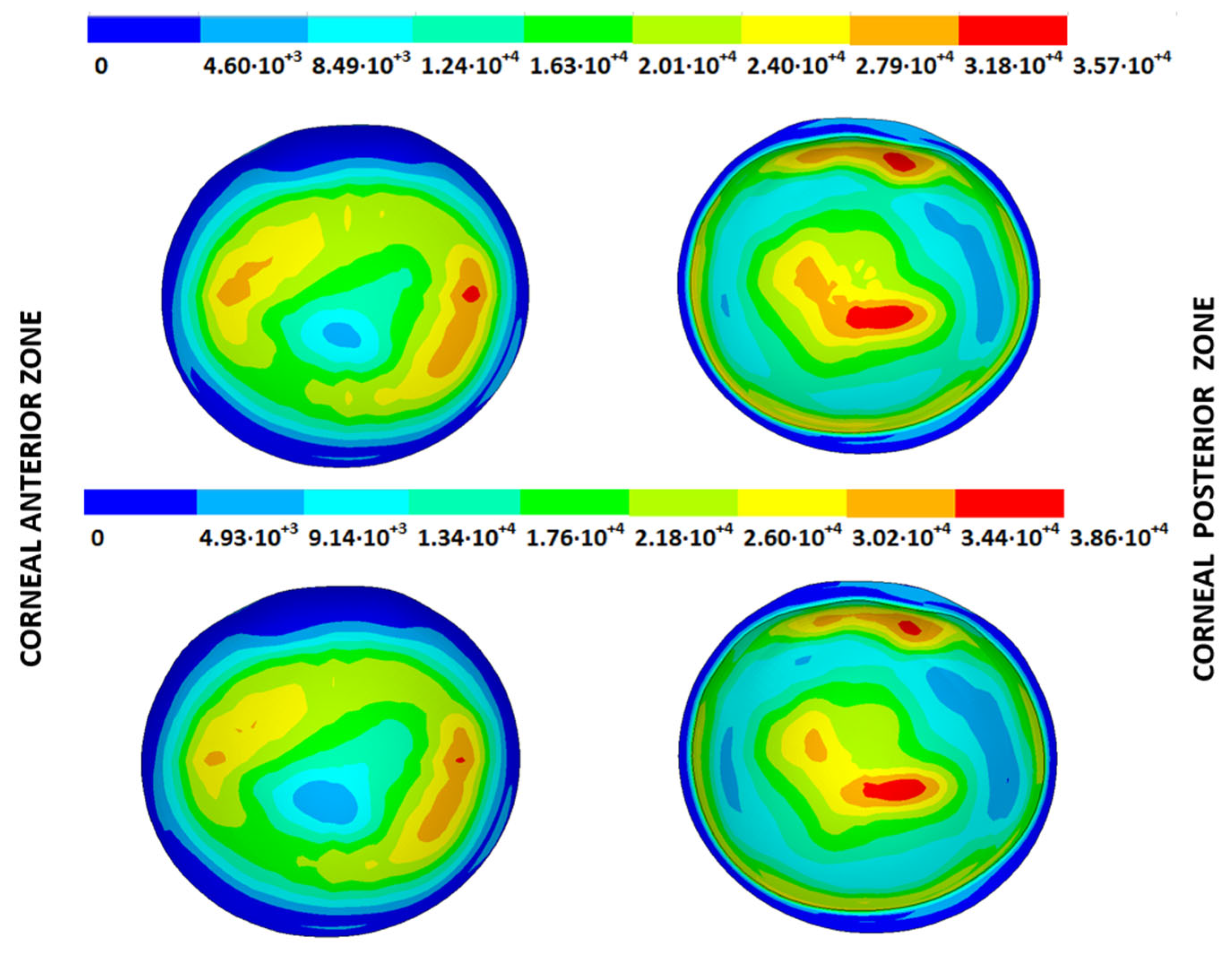

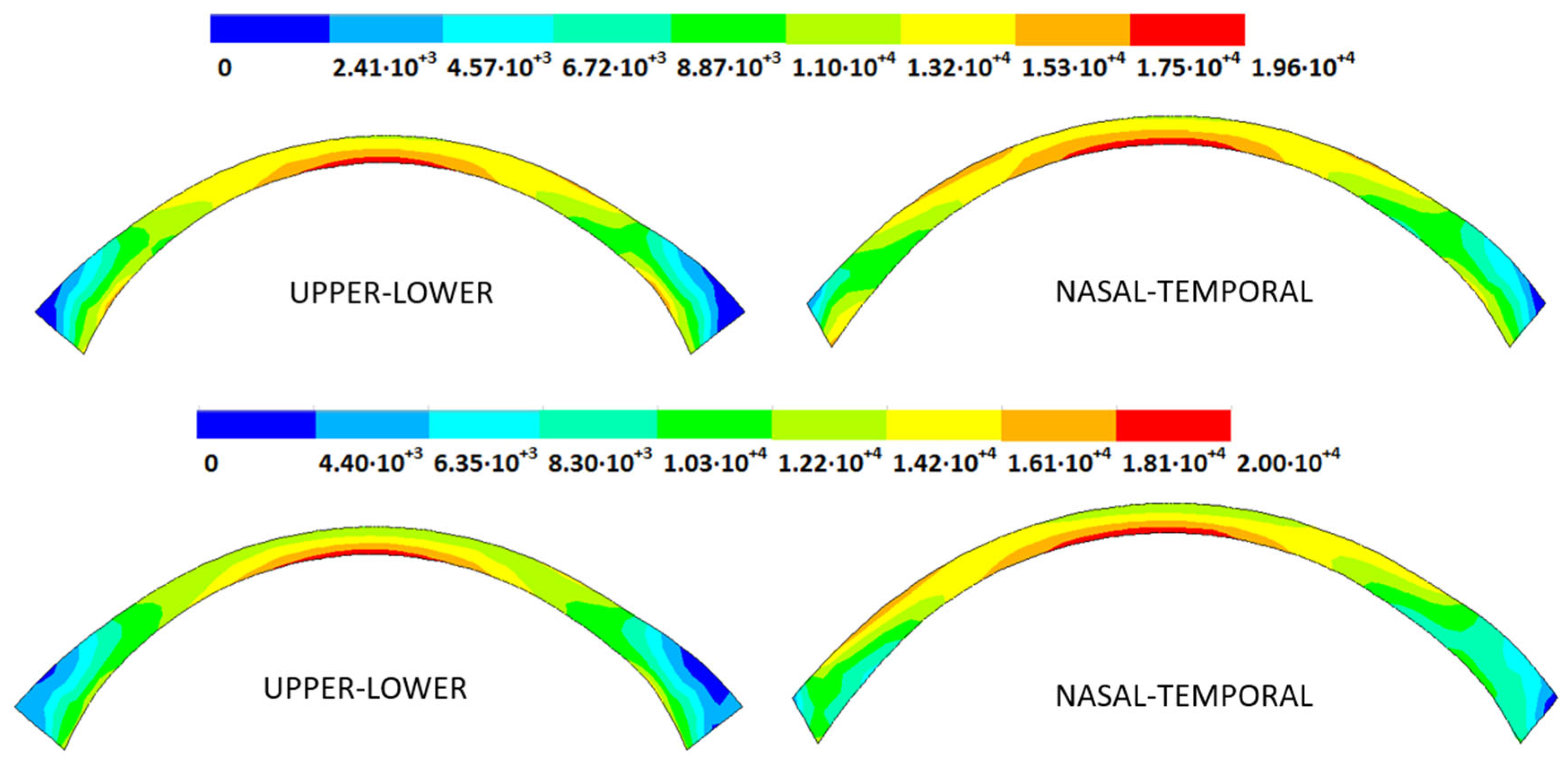

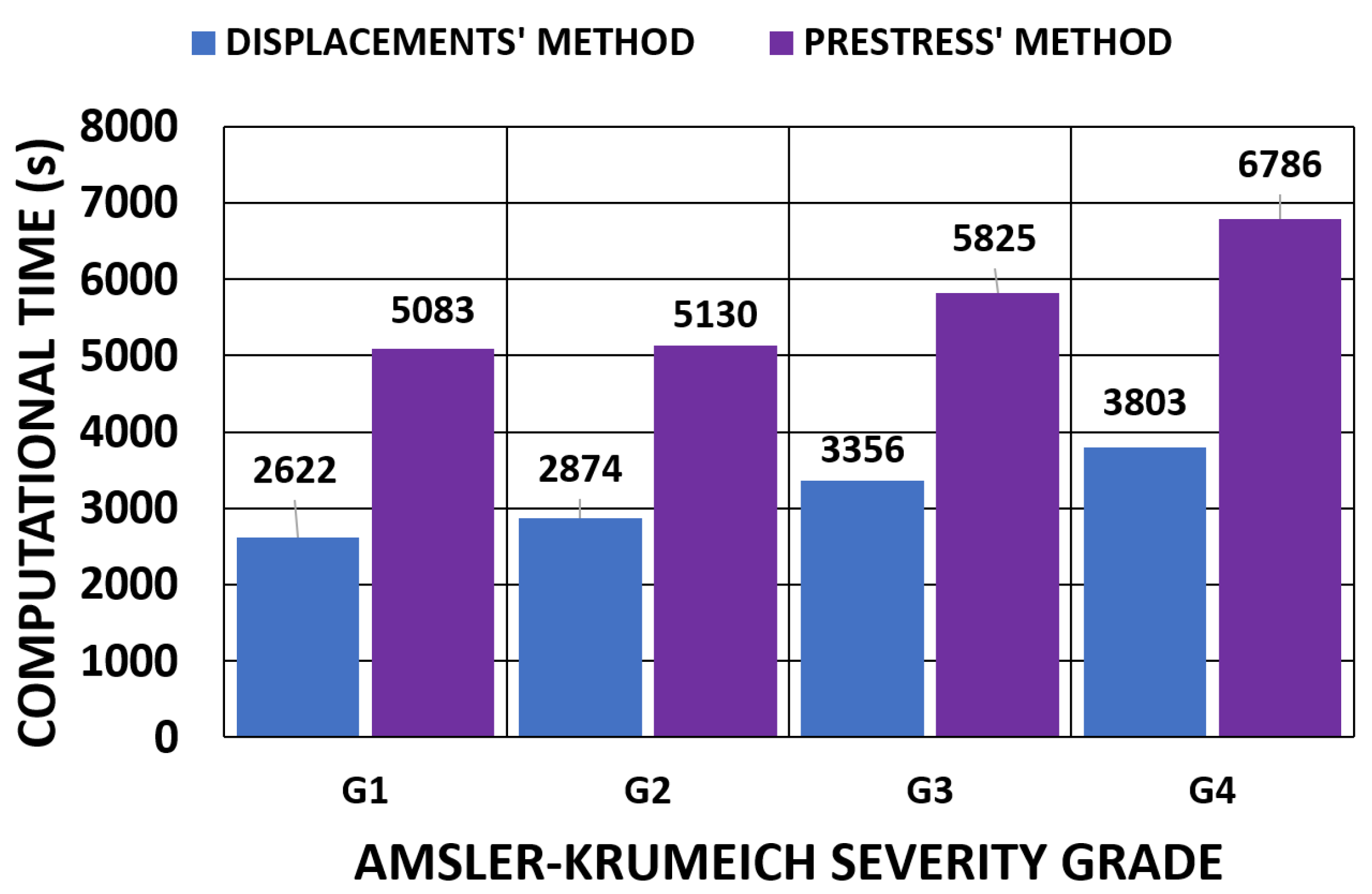

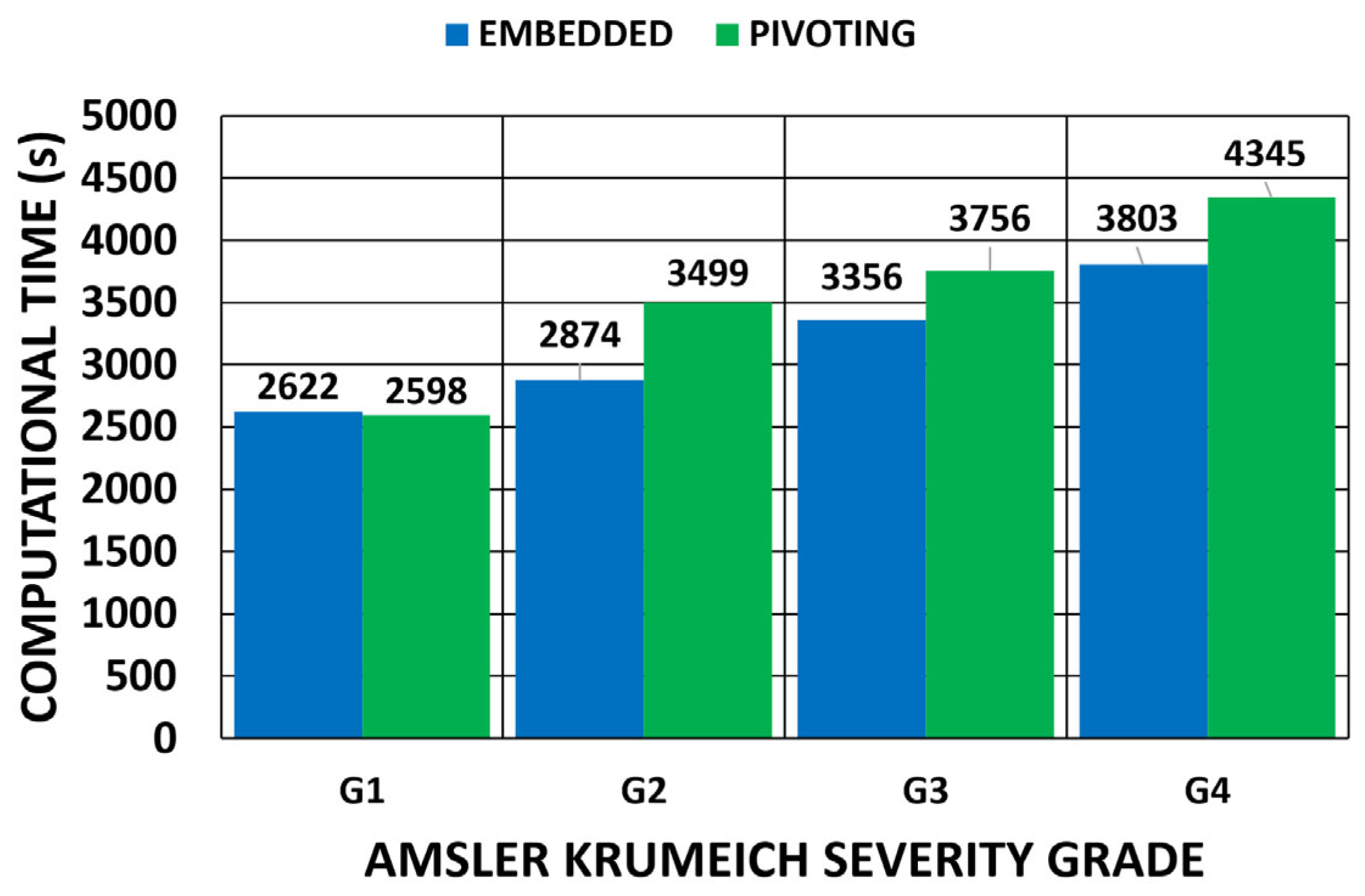
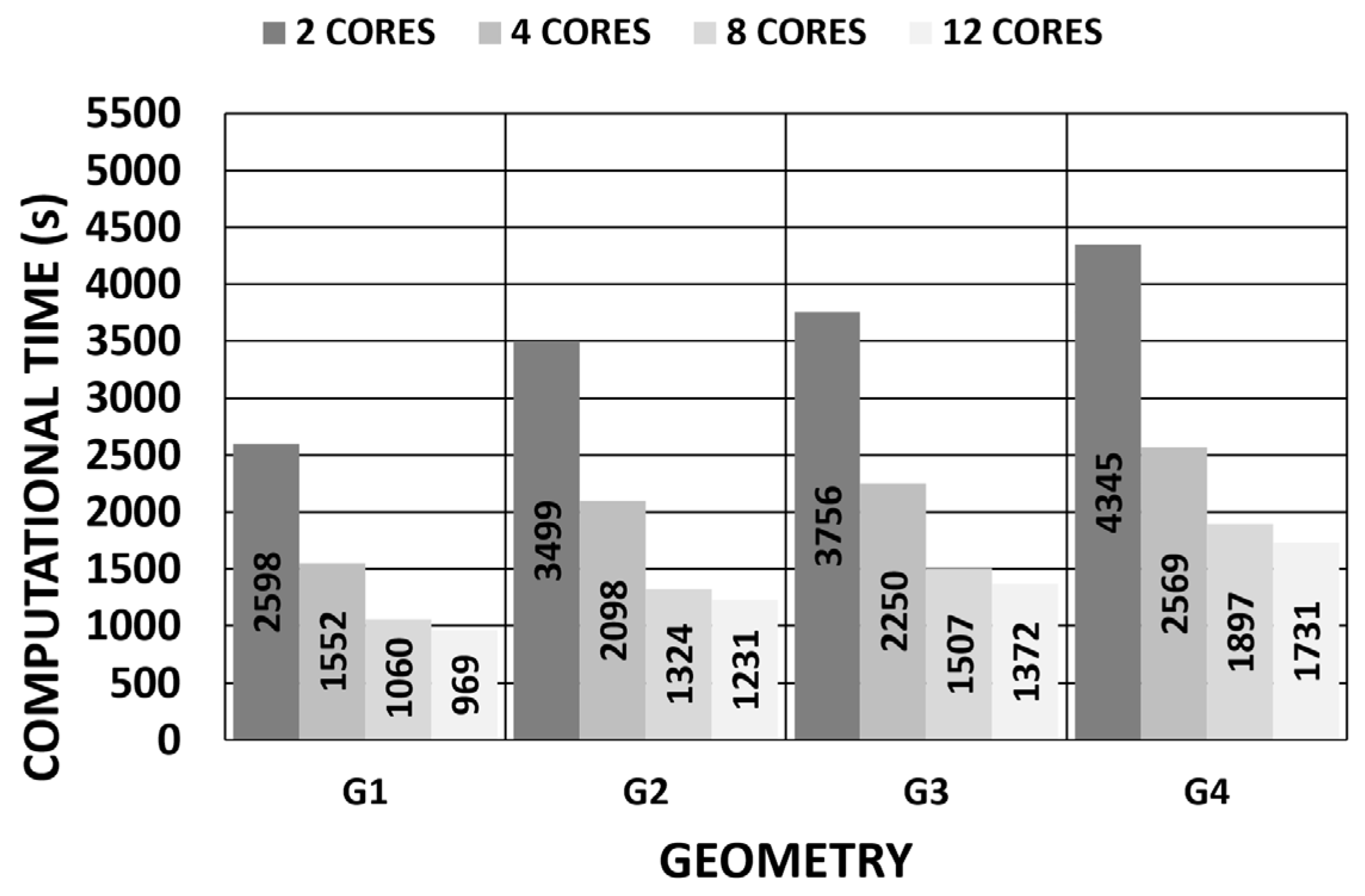
| A-K | IOP (mm) | Mean K (D) | Age (y) | Gender | Eye | AXL (mm) | Manifest Sphere (D) | Manifest Cylinder (D) | Cylinder Axis (°) | Spherical Equivalent (D) | Decimal CDVA |
|---|---|---|---|---|---|---|---|---|---|---|---|
| G1 | 15 | 47.29 | 49 | M | OS | 21.81 | 0.5 | −0.5 | 100 | 0.62 | 1.05 |
| G2 | 13 | 51.59 | 55 | F | OD | 24.1 | 2 | −5.5 | 85 | −0.40 | 0.55 |
| G3 | 14 | 53.77 | 26 | F | OS | 25.39 | −0.5 | −2.5 | 120 | −2 | 0.65 |
| G4 | 17 | 69.1 | 33 | M | OD | 23.61 | 0 | −2.5 | 60 | −1 | 0.15 |
| Material Constants | a1 (Pa) | a2 (Pa) | k1 (Pa) | k2 (−) |
|---|---|---|---|---|
| Central, N-T and S-I zones | 40,000 | −10,000 | 50,000 | 200 |
| Transition zones | 40,000 | −10,000 | 37,500 | 200 |
| Central oblique zones | 40,000 | −10,000 | 25,000 | 200 |
| Limbus | 40,000 | −10,000 | 50,000 | 200 |
| Stress-Free Geometry (m) | Displacements Method | Prestress Method | |||
|---|---|---|---|---|---|
| Geometry | IOP (mmHg) | Embedded | Pivoting | Embedded | Pivoting |
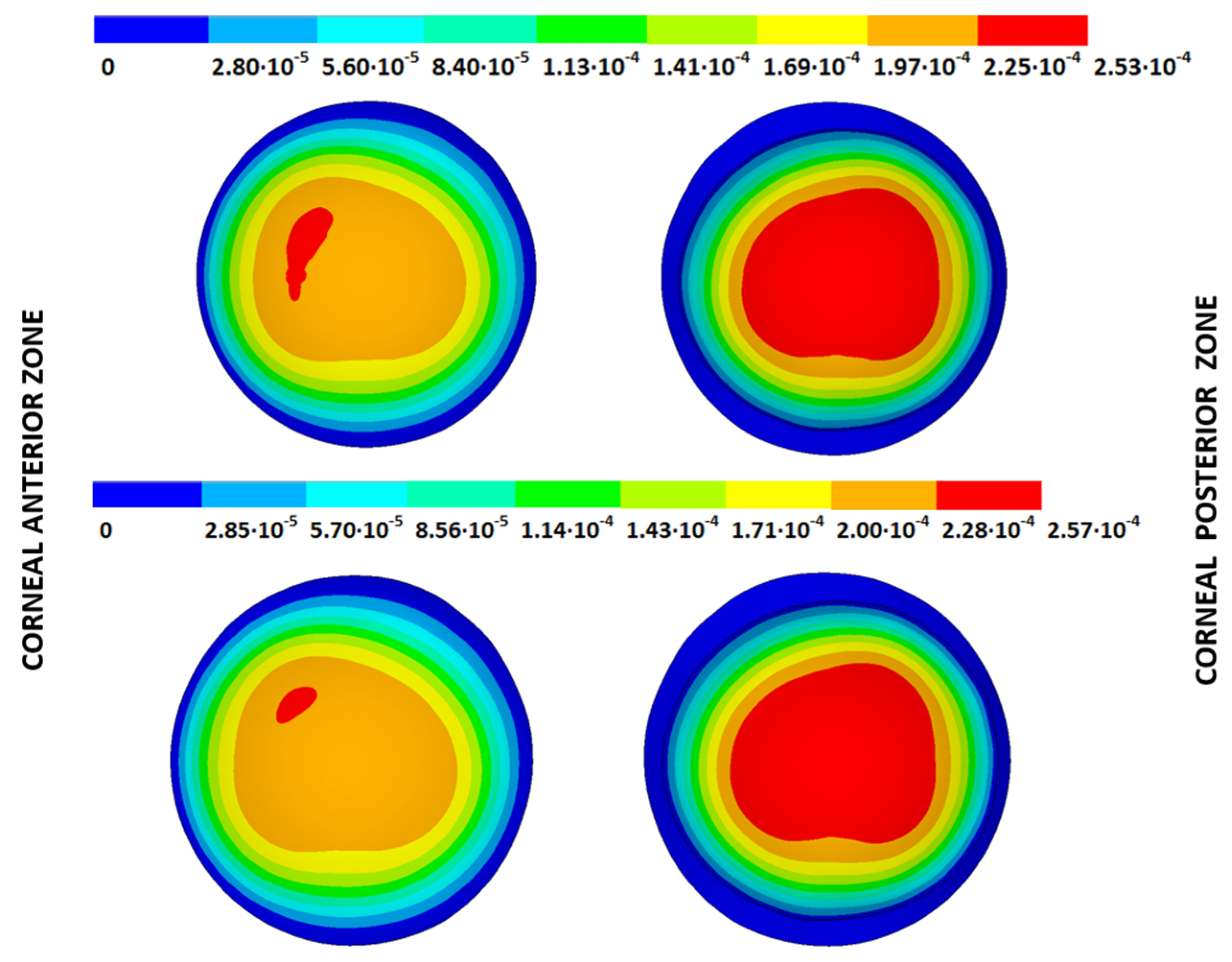
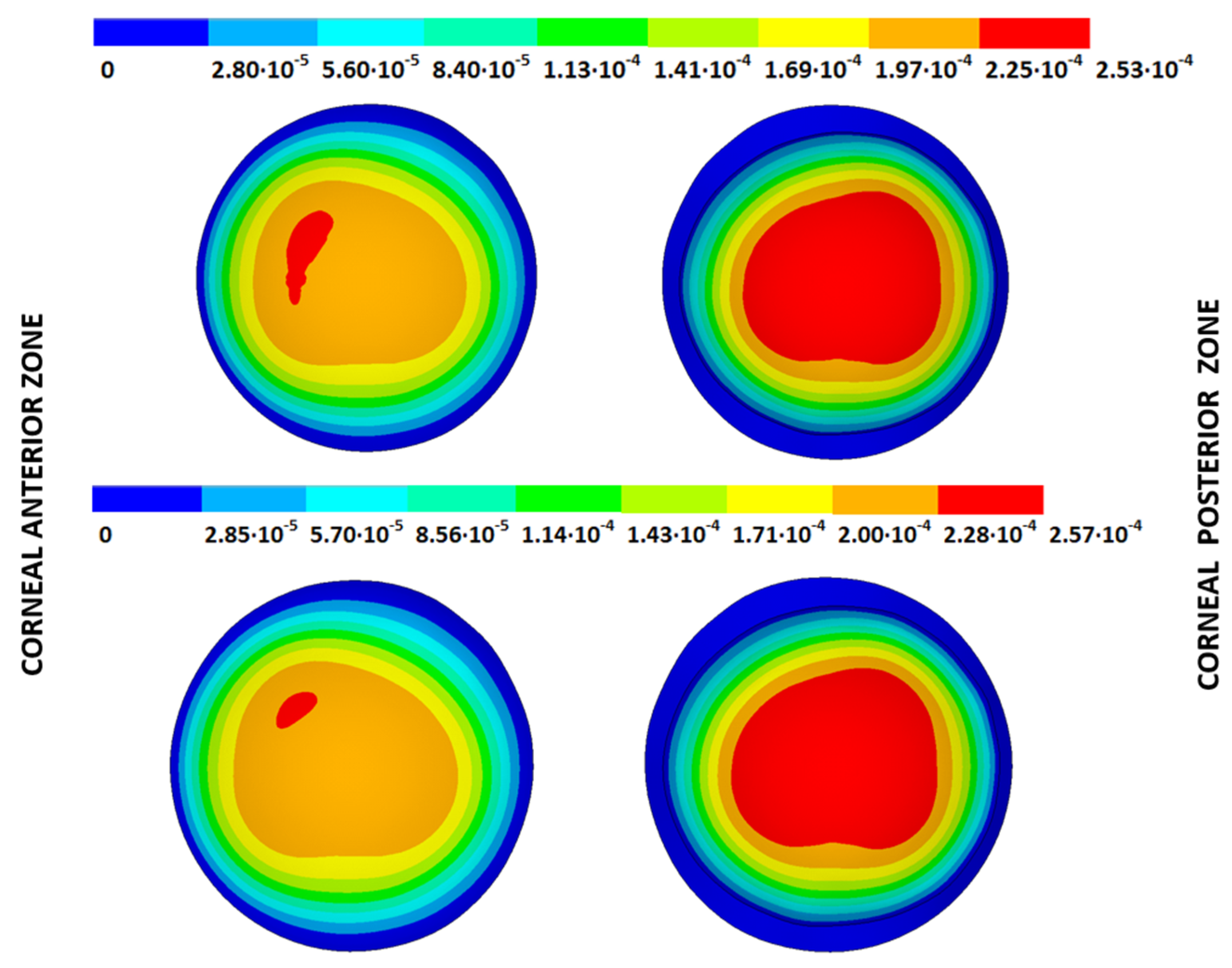
| G1 | 15 | 2.53·10−4 | 2.37·10−4 | 2.57·10−4 | 2.41·10−4 |
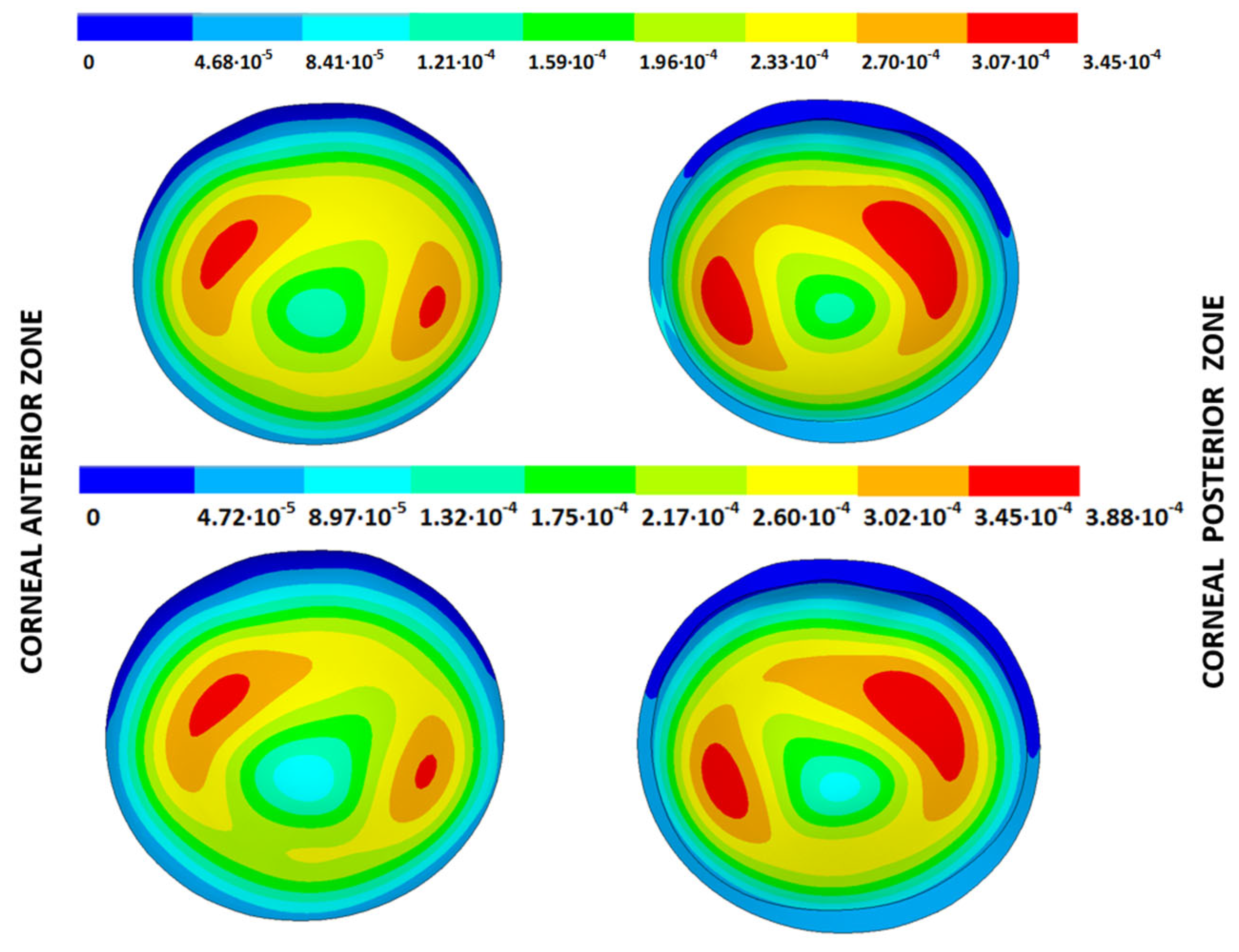
| G2 | 13 | 2.87·10−4 | 4.23·10−4 | 2.96·10−4 | 4.35·10−4 |
| G3 | 14 | 3.99·10−4 | 3.45·10−4 | 4.22·10−4 | 3.88·10−4 |
| G4 | 17 | 4.78·10−4 | 6.71·10−4 | 4.98·10−4 | 7.42·10−4 |
| G1 | Embedded | Pivoting |
|---|---|---|
| Maximum (μm) | 8.36 | 7.47 |
| Mean (μm) | 2.92 | 3.07 |
| Standard deviation (μm) | 1.83 | 1.41 |
| G3 | Embedded | Pivoting |
| Maximum (μm) | 43.54 | 43.54 |
| Mean (μm) | 13.18 | 15.59 |
| Standard deviation (μm) | 11.35 | 10.53 |
| Estimated Physiological Geometry (m) | Displacements Method | Prestress Method | |||
|---|---|---|---|---|---|
| Geometry | IOP (mmHg) | Embedded | Pivoting | Embedded | Pivoting |
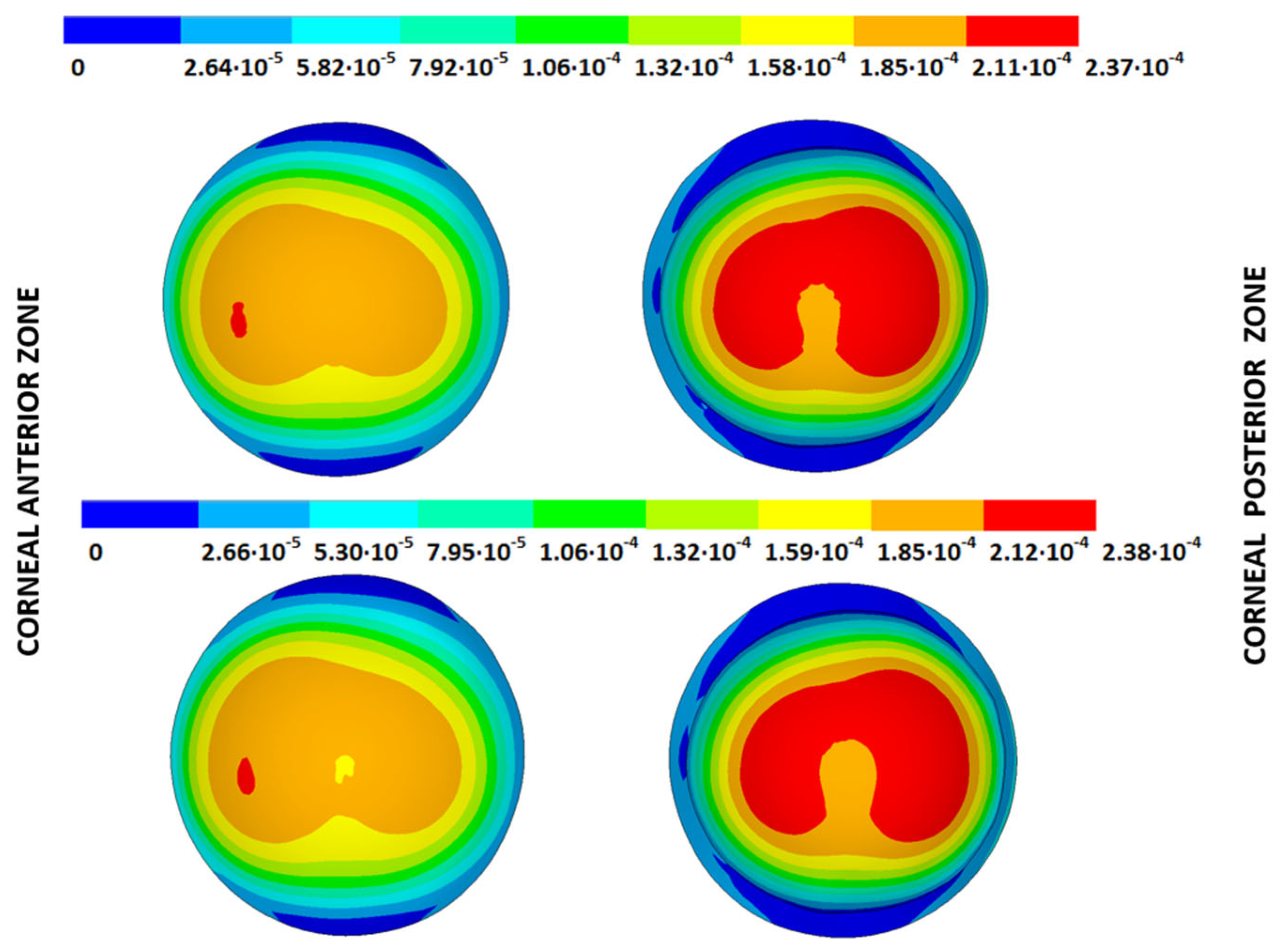
| G1 | 15 | 2.53·10−4 | 2.37·10−4 | 2.54·10−4 | 2.38·10−4 |
| G2 | 13 | 2.87·10−4 | 4.23·10−4 | 2.90·10−4 | 4.28·10−4 |
| G3 | 14 | 3.99·10−4 | 3.45·10−4 | 4.17·10−4 | 3.64·10−4 |
| G4 | 17 | 4.78·10−4 | 6.71·10−4 | 4.89·10−4 | 7.08·10−4 |
| G1 | Embedded | Pivoting |
|---|---|---|
| Maximum (μm) | 0.12 | 0.14 |
| Mean (μm) | 0.04 | 0.05 |
| Standard deviation (μm) | 0.03 | 0.03 |
| G3 | Embedded | Pivoting |
| Maximum (μm) | 0.11 | 0.11 |
| Mean (μm) | 0.04 | 0.05 |
| Standard deviation (μm) | 0.03 | 0.03 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Gómez, C.; Piñero, D.P.; Paredes, M.; Alió, J.L.; Cavas, F. Study of the Influence of Boundary Conditions on Corneal Deformation Based on the Finite Element Method of a Corneal Biomechanics Model. Biomimetics 2024, 9, 73. https://doi.org/10.3390/biomimetics9020073
Gómez C, Piñero DP, Paredes M, Alió JL, Cavas F. Study of the Influence of Boundary Conditions on Corneal Deformation Based on the Finite Element Method of a Corneal Biomechanics Model. Biomimetics. 2024; 9(2):73. https://doi.org/10.3390/biomimetics9020073
Chicago/Turabian StyleGómez, Carmelo, David P. Piñero, Manuel Paredes, Jorge L. Alió, and Francisco Cavas. 2024. "Study of the Influence of Boundary Conditions on Corneal Deformation Based on the Finite Element Method of a Corneal Biomechanics Model" Biomimetics 9, no. 2: 73. https://doi.org/10.3390/biomimetics9020073
APA StyleGómez, C., Piñero, D. P., Paredes, M., Alió, J. L., & Cavas, F. (2024). Study of the Influence of Boundary Conditions on Corneal Deformation Based on the Finite Element Method of a Corneal Biomechanics Model. Biomimetics, 9(2), 73. https://doi.org/10.3390/biomimetics9020073










