Numerical Investigation of Dimensionless Parameters in Carangiform Fish Swimming Hydrodynamics
Abstract
1. Introduction
2. Methodology
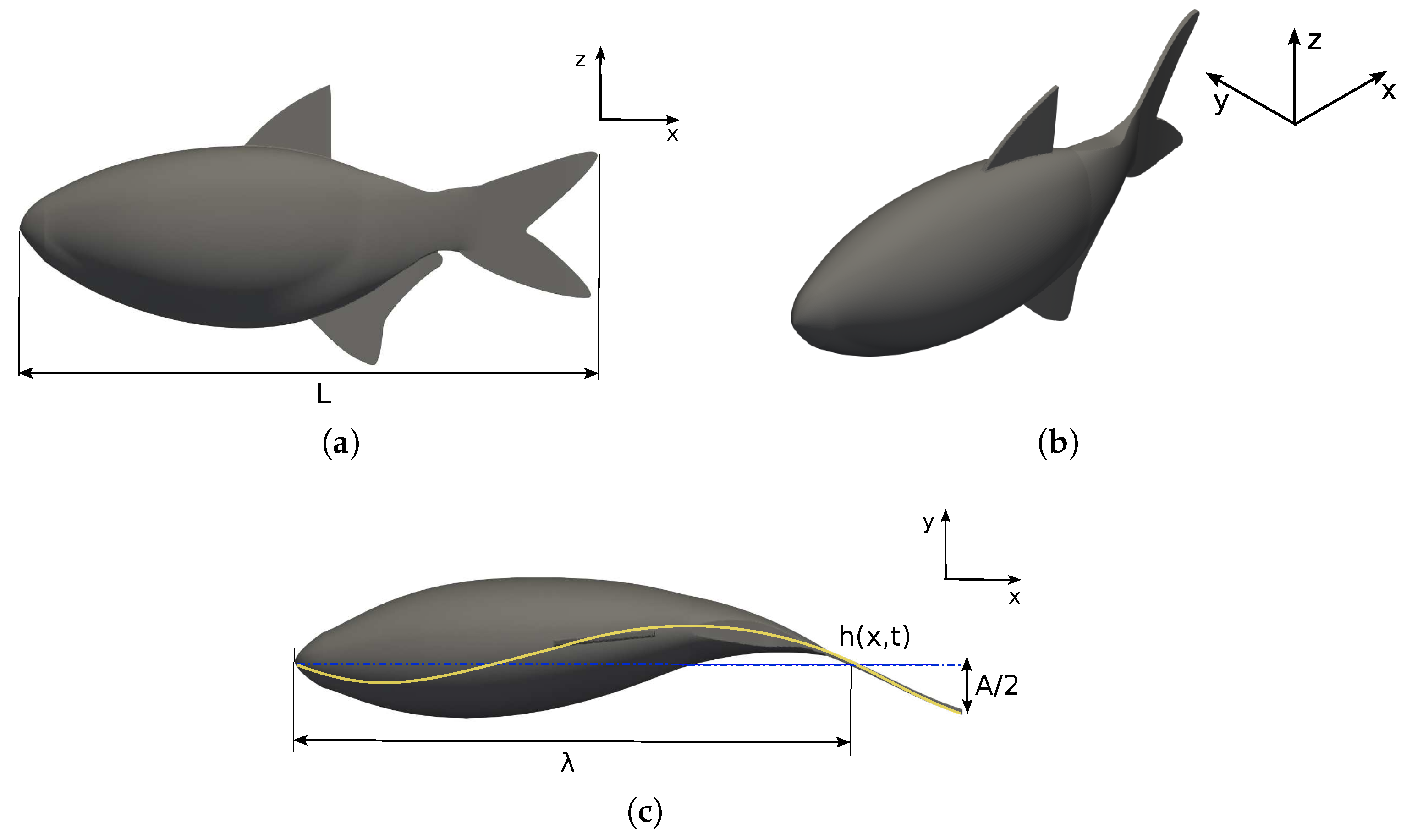
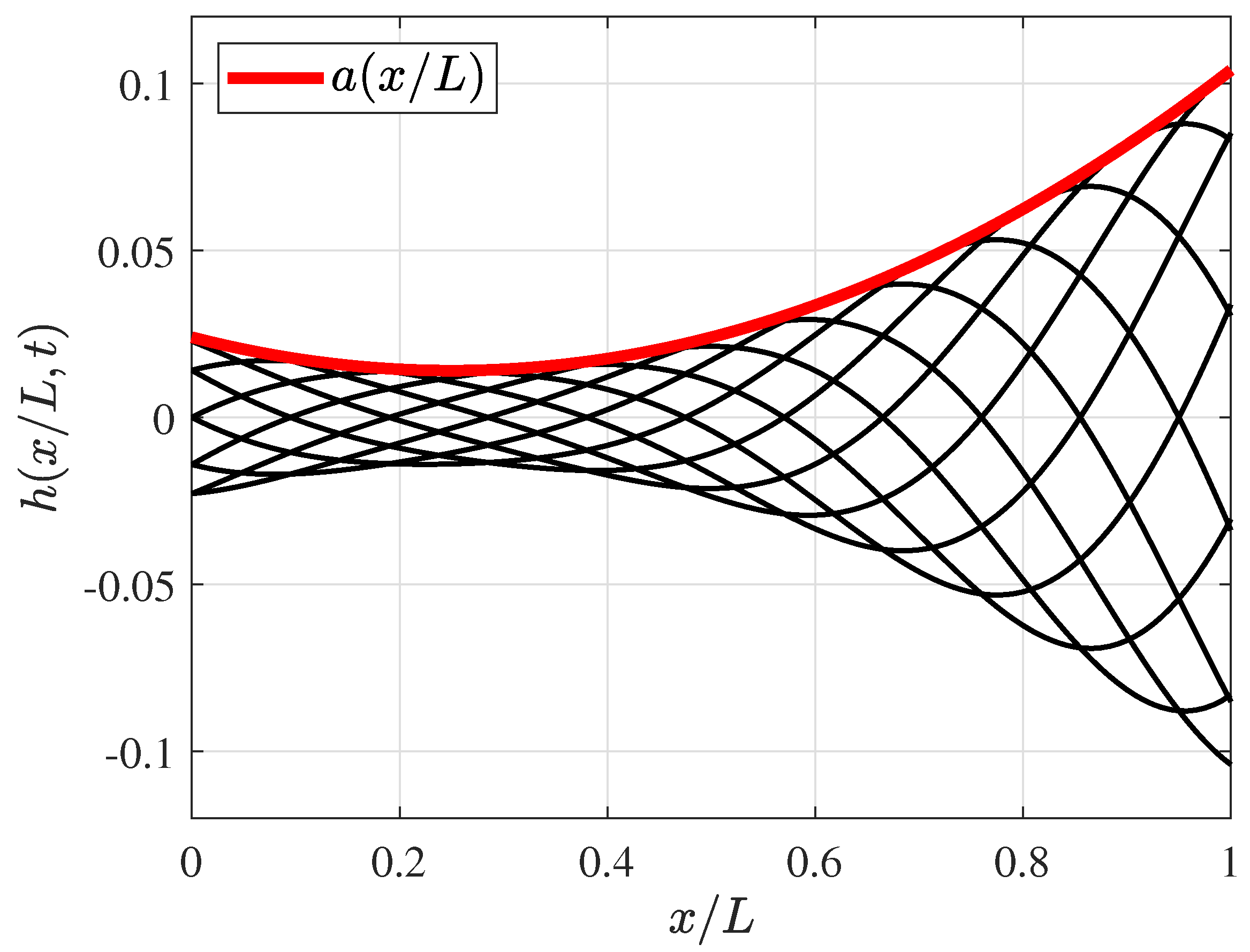
2.1. Fish Swimming Characteristics
2.2. Hydrodynamic Efficiency Metrics, Power Consumption, and Hydrodynamic Forces
2.3. Numerical Setup
3. Results and Discussion
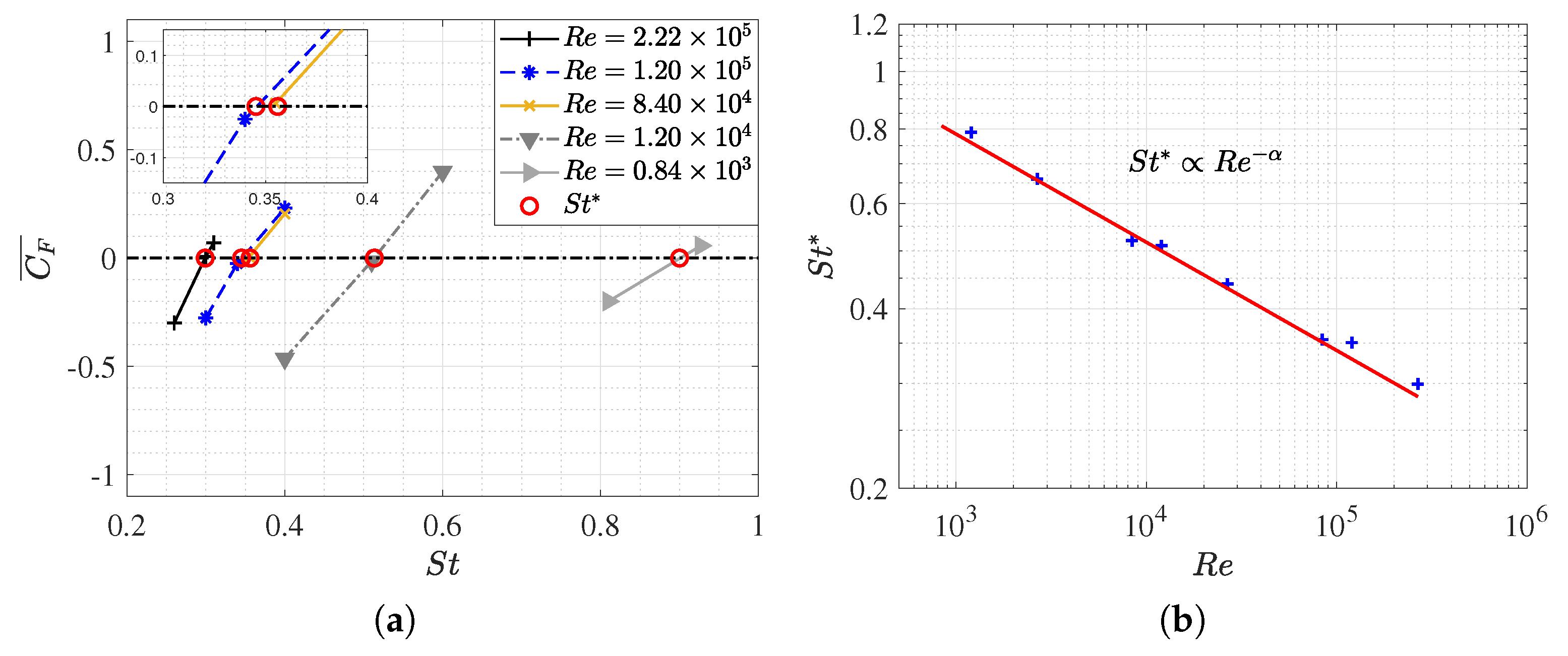
3.1. Equilibrium of the Strouhal Number and Reynolds Number
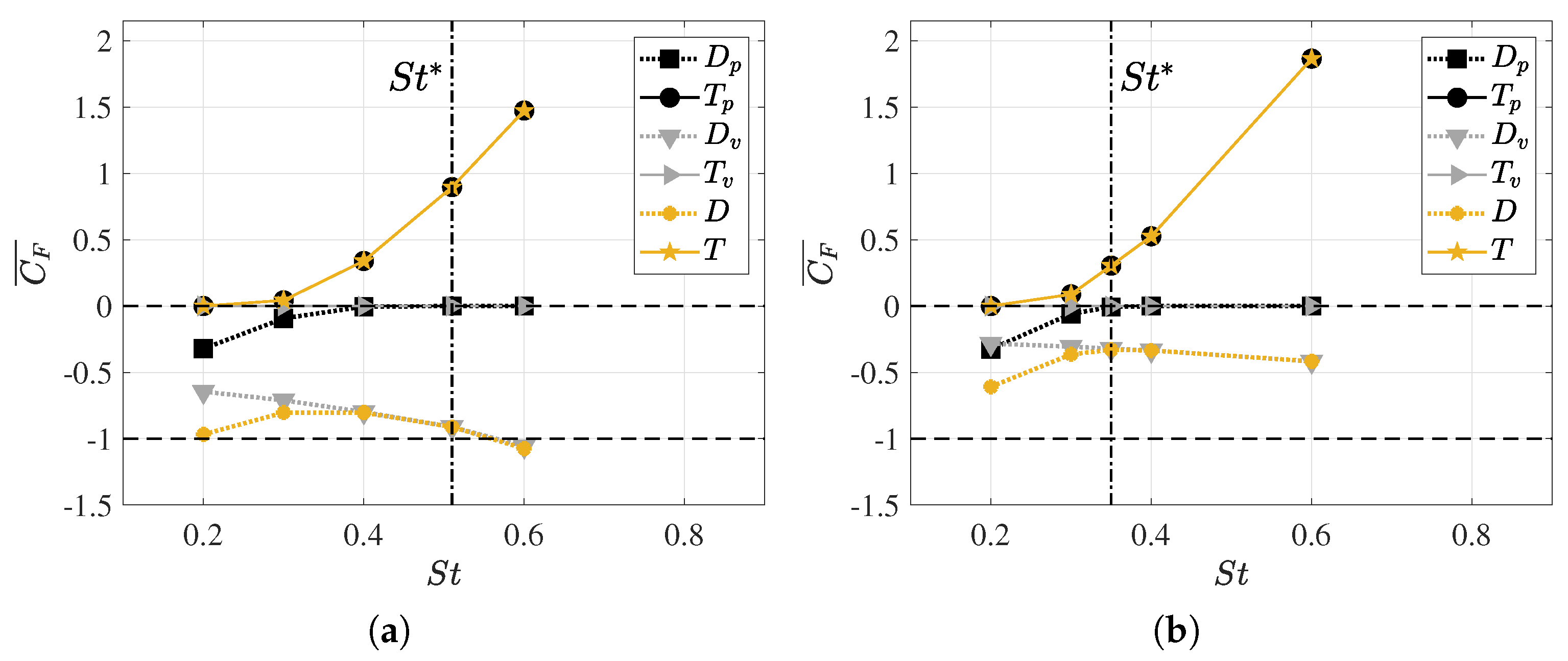
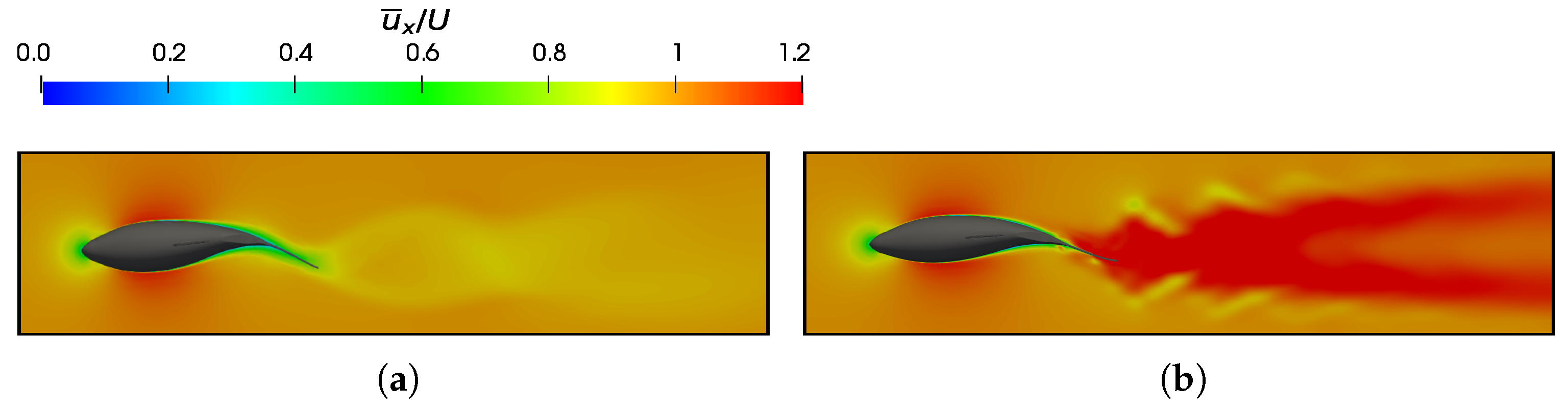
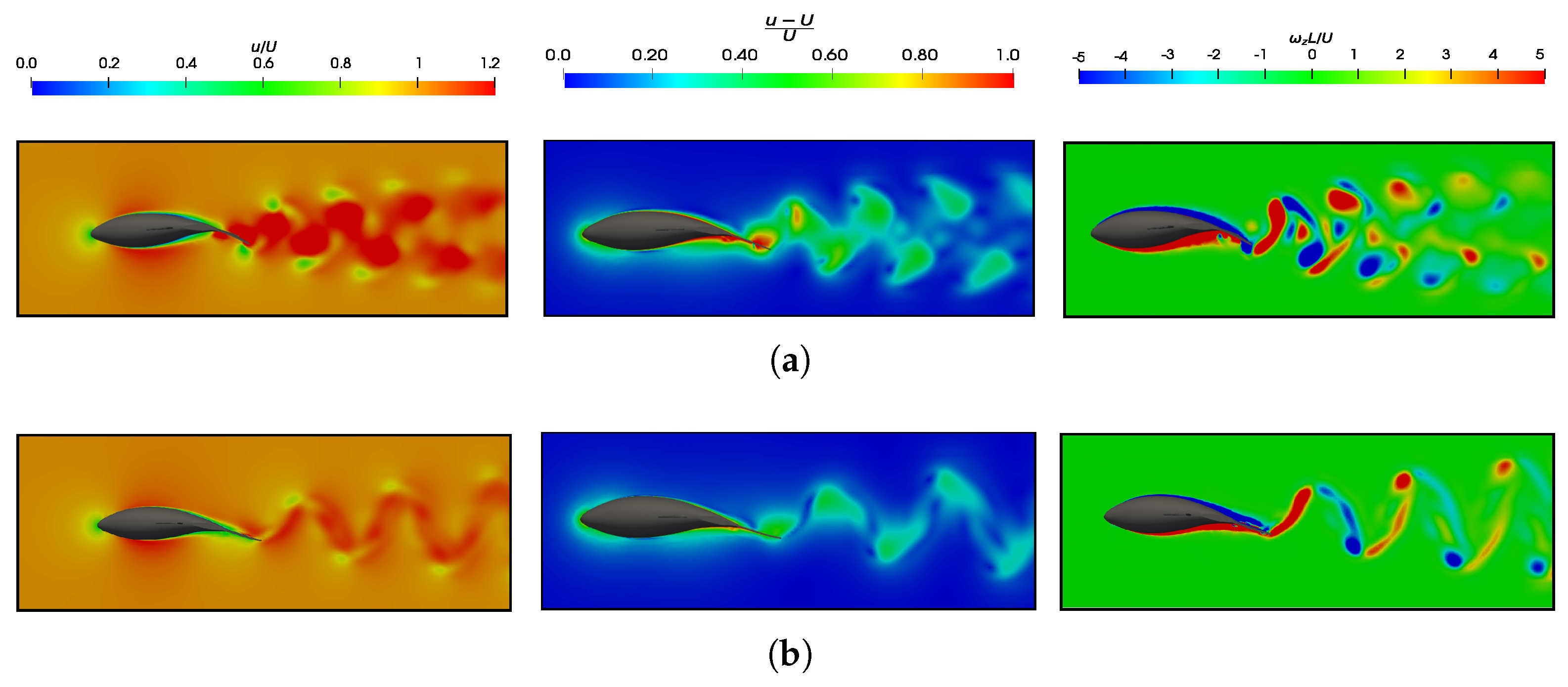
3.2. Hydrodynamic Force Coefficients: Swimming Drag and Thrust
3.3. Reynolds Number Effect on Efficiency Metrics
3.4. Slip Number and Wavelength Effect on Efficiency Metrics
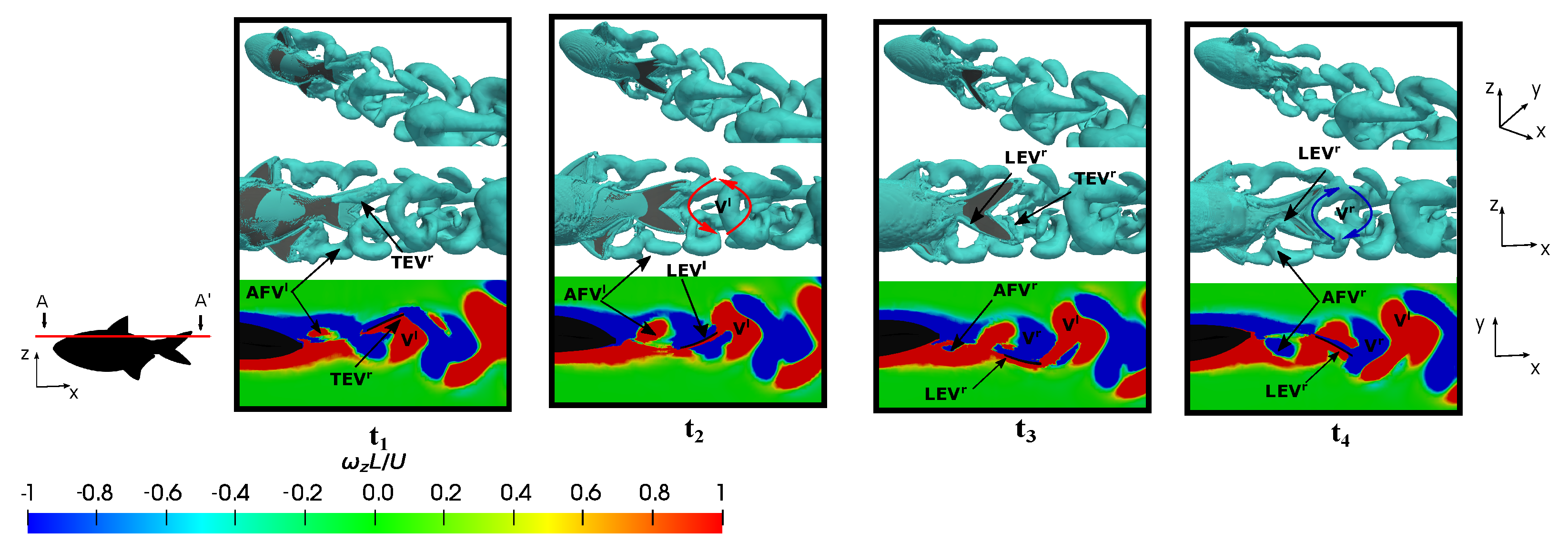
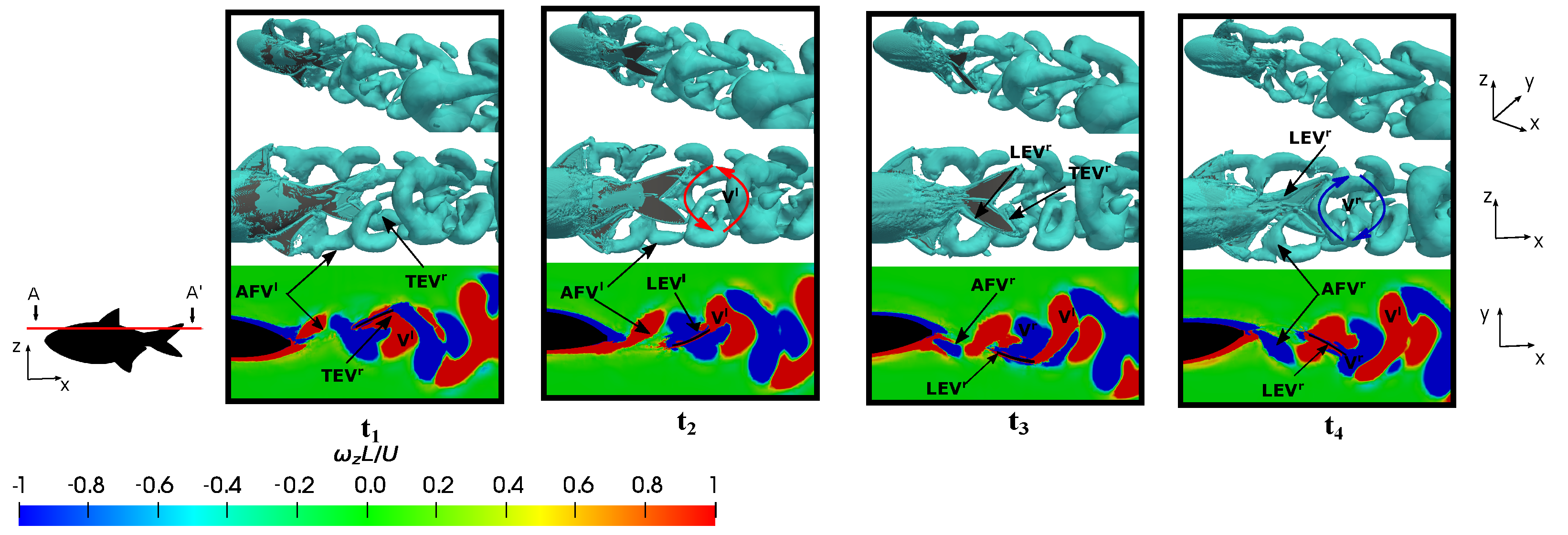
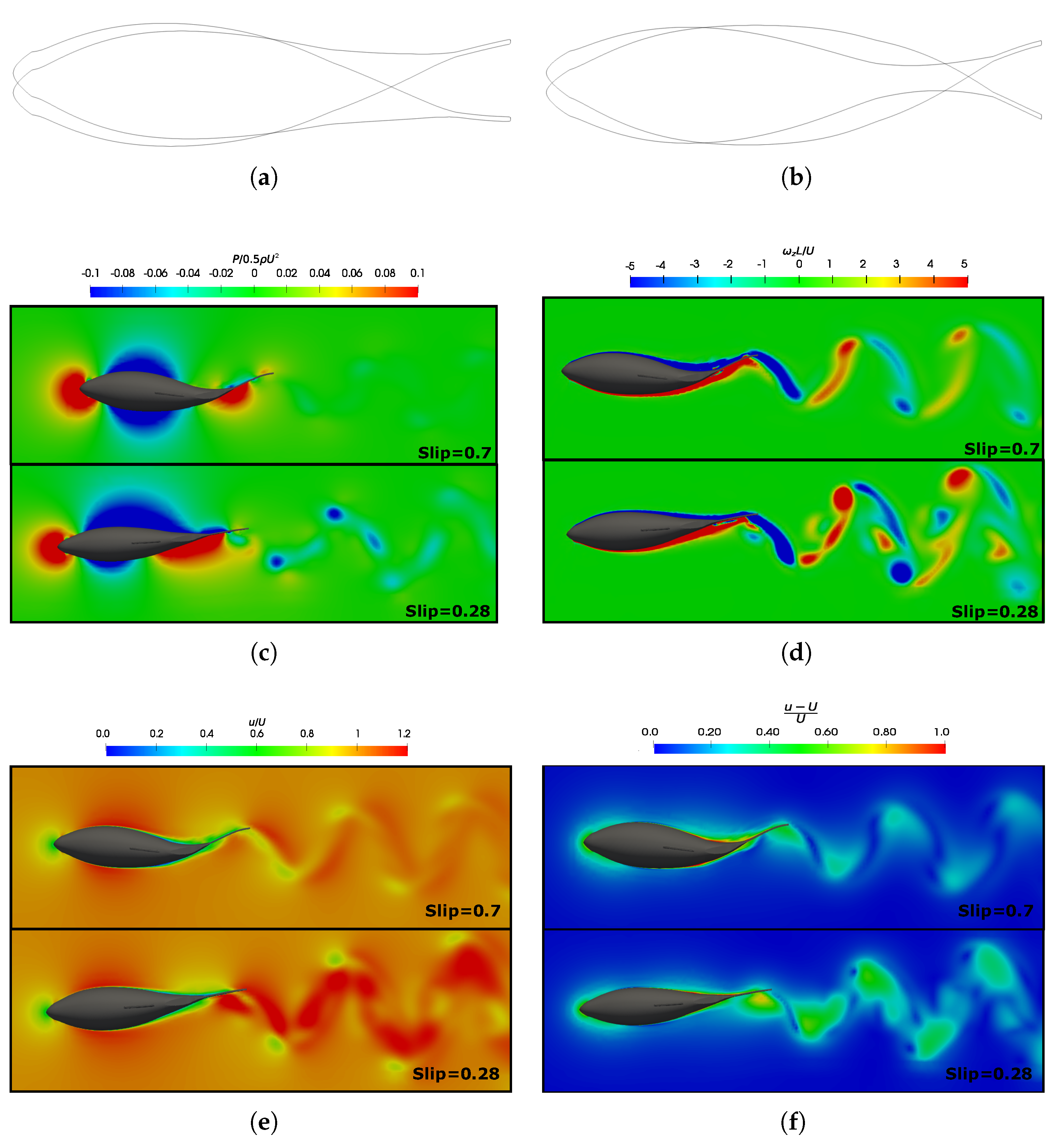
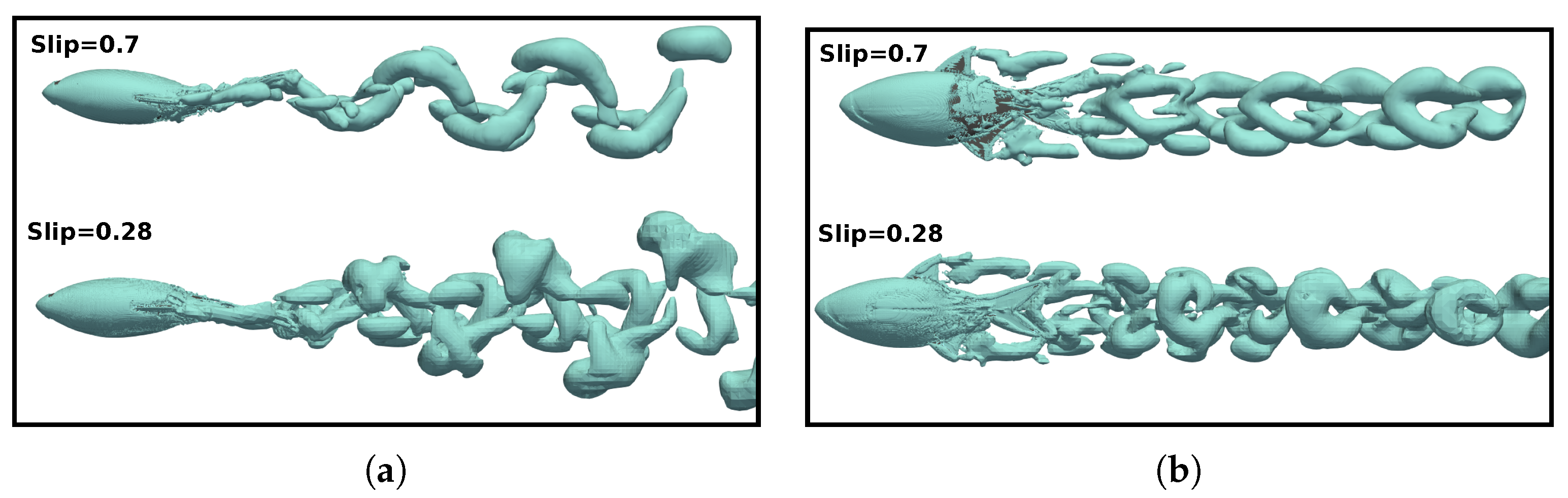
3.5. Vortex Dynamics
3.5.1. Leading-Edge Vortex Generation
3.5.2. Wake Vortex-Induced Scenarios
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Appendix A
| U (m/s) | /L | f () | |||
|---|---|---|---|---|---|
| 2.22 | 0.95 | 24.1 | 0.26 | 0.81 | |
| 27.7 * | 0.30 * | 0.70 * | |||
| 28.7 | 0.31 | 0.68 | |||
| 1.00 | 0.95 | 8.3 | 0.20 | 1.052 | |
| 12.5 | 0.30 | 0.70 | |||
| 14.6 * | 0.35 * | 0.60 * | |||
| 16.7 | 0.40 | 0.53 | |||
| 25.0 | 0.60 | 0.35 | |||
| 0.70 | 0.95 | 10.4 * | 0.36 * | 0.60 * | |
| 11.7 | 0.40 | 0.53 | |||
| 1.00 | 0.95 | 8.33 | 0.20 | 1.052 | |
| 12.5 | 0.30 | 0.70 | |||
| 16.7 | 0.40 | 0.53 | |||
| 21.3 * | 0.51 * | 0.41 * | |||
| 25.0 | 0.60 | 0.35 | |||
| 0.70 | 0.95 | 23.6 | 0.81 | 0.26 | |
| 26.3 * | 0.90 * | 0.23 * | |||
| 27.1 | 0.93 | 0.24 |
| U (m/s) | /L | f () | |||
|---|---|---|---|---|---|
| 2.22 | 0.95 | 27.7 | 0.30 | 0.70 | |
| 1.00 | 0.67 | ||||
| 1.10 | 0.60 | ||||
| 1.25 | 0.53 | ||||
| 1.60 | 0.42 | ||||
| 2.40 | 0.28 | ||||
| 1.00 | 0.85 | 14.6 | 0.34 | 0.70 | |
| 0.95 | 0.60 | ||||
| 1.10 | 0.55 | ||||
| 1.25 | 0.48 | ||||
| 1.43 | 0.42 | ||||
| 2.15 | 0.26 | ||||
| 0.70 | 0.95 | 22.2 | 0.36 | 0.60 | |
| 1.20 | 0.48 | ||||
| 1.60 | 0.36 | ||||
| 2.00 | 0.28 | ||||
| 2.22 | 0.65 | 40.7 | 0.44 | 0.70 | |
| 0.75 | 0.60 | ||||
| 1.10 | 0.42 | ||||
| 1.25 | 0.36 | ||||
| 1.60 | 0.28 | ||||
| 1.00 | 0.56 | 21.3 | 0.51 | 0.70 | |
| 0.63 | 0.63 | ||||
| 0.95 | 0.42 | ||||
| 1.40 | 0.28 | ||||
| 0.70 | 0.95 | 15.2 | 0.52 | 0.41 | |
| 2.22 | 0.95 | 61.1 | 0.66 | 0.32 | |
| 1.25 | 0.24 | ||||
| 1.00 | 0.95 | 33.4 | 0.80 | 0.26 | |
| 1.25 | 0.20 |
References
- Vepa, R. Biomimetic Robotics; Cabridge University Press: Cambridge, UK, 2009. [Google Scholar] [CrossRef]
- Triantafyllou, M.S.; Weymouth, G.D.; Miao, J. Biomimetic Survival Hydrodynamics and Flow Sensing. Annu. Rev. Fluid Mech. 2016, 48, 1–24. [Google Scholar] [CrossRef]
- Katzschmann, R.K.; DelPreto, J.; MacCurdy, R.; Rus, D. Exploration of underwater life with an acoustically controlled soft robotic fish. Sci. Robot. 2018, 3, eaar3449. [Google Scholar] [CrossRef]
- Fish, F.E. Advantages of aquatic animals as models for bio-inspired drones over present AUV technology. Bioinspir. Biomim. 2020, 15, 025001. [Google Scholar] [CrossRef]
- Wright, M.; Xiao, Q.; Dai, S.; Post, M.; Yue, H.; Sarkar, B. Design and development of modular magnetic bio-inspired autonomous underwater robot—MMBAUV. Ocean Eng. 2023, 273, 113968. [Google Scholar] [CrossRef]
- Horgan, J.; Toal, D. Review of Machine Vision Applications in Unmanned Underwater Vehicles. In Proceedings of the 2006 9th International Conference on Control, Automation, Robotics and Vision, Singapore, 5–8 December 2006; pp. 1–6. [Google Scholar] [CrossRef]
- Chutia, S.; Kakoty, N.M.; Deka, D. A Review of Underwater Robotics, Navigation, Sensing Techniques and Applications. In Proceedings of the AIR ’17: Proceedings of the Advances in Robotics, New Delhi, India, 28 June–2 July 2017. [Google Scholar] [CrossRef]
- Maertens, A.P.; Triantafyllou, M.S.; Yue, D.K. Efficiency of fish propulsion. BIoinspir. Biomim. 2015, 10, 046013. [Google Scholar] [CrossRef]
- Sfakiotakis, M.; Lane, D.M.; Davies, J.B.C. Review of fish swimming modes for aquatic locomotion. IEEE J. Ocean. Eng. 1999, 24, 237–252. [Google Scholar] [CrossRef]
- Gazzola, M.; Argentina, M.; Mahadevan, L. Scaling macroscopic aquatic locomotion. Nat. Phys. 2014, 10, 758–761. [Google Scholar] [CrossRef]
- Feng, Y.; Xu, J.; Su, Y. Effect of trailing-edge shape on the swimming performance of a fish-like swimmer under self-propulsion. Ocean Eng. 2023, 287, 115849. [Google Scholar] [CrossRef]
- Lighthill, M. Note on the swimming of slender fish. J. Fluid Mech. 1960, 9, 305–371. [Google Scholar] [CrossRef]
- Macias, M.M.; Souza, I.F.; Junior, A.C.P.B.; Oliveira, T.F. Three-dimensional viscous wake flow in fish swimming—A CFD study. Mech. Res. Commun. 2020, 107, 103547. [Google Scholar] [CrossRef]
- Eloy, C. Optimal Strouhal number for swimming animals. J. Fluids Struct. 2011, 30, 205–218. [Google Scholar] [CrossRef]
- Borazjani, I.; Sotiropoulos, F. Numerical investigation of the hydrodynamics of carangiform swimming in the transitional and inertial flow regimes. J. Exp. Biol. 2008, 211, 1541–1558. [Google Scholar] [CrossRef] [PubMed]
- Yu, C.L.; Ting, S.C.; Hsu, Y.H.; Yeh, M.K.; Yang, J.T. Mechanical capability and timing of a fish to maneuver from a steady straight-line swimming state. Mech. Res. Commun. 2012, 39, 59–64. [Google Scholar] [CrossRef]
- Ju, I.; Yun, D. Hydraulic variable stiffness mechanism for swimming locomotion optimization of soft robotic fish. Ocean Eng. 2023, 286, 115551. [Google Scholar] [CrossRef]
- Wei, C.; Hu, Q.; Liu, Y.; Yin, S.; Chen, Z.; Ji, X. Performance evaluation and optimization for two-dimensional fish-like propulsion. Ocean Eng. 2021, 233, 109191. [Google Scholar] [CrossRef]
- Scaradozzi, D.; Palmieri, G.; Costa, D.; Pinelli, A. BCF swimming locomotion for autonomous underwater robots: A review and a novel solution to improve control and efficiency. Ocean Eng. 2017, 130, 437–453. [Google Scholar] [CrossRef]
- Rosen, M.W. Water Flow about a Swimming Fish; US Naval Ordnance Test Station: China Lake, CA, USA, 1959. [Google Scholar]
- Webb, P.W. Entrainment by river chub Nocomis micropogon and smallmouth bass Micropterus dolomieu on cylinders. J. Exp. Biol. 1998, 201, 2403–2412. [Google Scholar] [CrossRef]
- Cui, Z.; Gu, X.; Kangkang, L.; Jiang, H.; Li, K.; Jiang, H. CFD Studies of the Effects of Waveform on Swimming Performance of Carangiform Fish. Appl. Sci. 2017, 7, 149. [Google Scholar] [CrossRef]
- Zhang, S.; Qian, Y.; Liao, P.; Qin, F.; Yang, J. Design and Control of an Agile Robotic Fish with Integrative Biomimetic Mechanisms. IEEE/ASME Trans. Mechatron. 2016, 21, 1846–1857. [Google Scholar] [CrossRef]
- Ma, S.; Zhao, Q.; Ding, M.; Zhang, M.; Zhao, L.; Huang, C.; Zhang, J.; Liang, X.; Yuan, J.; Wang, X.; et al. A Review of Robotic Fish Based on Smart Materials. Biomimetics 2023, 8, 227. [Google Scholar] [CrossRef]
- Zhu, Q.; Wolfgang, M.J.; Yue, D.K.P.; Triantafyllou, M.S. Three-dimensional flow structures and vorticity control in fish-like swimming. J. Fluid Mech. 2002, 468, 1–28. [Google Scholar] [CrossRef]
- Thekkethil, N.; Sharma, A.; Agrawal, A. Unified hydrodynamics study for various types of fishes-like undulating rigid hydrofoil in a free stream flow. Phys. Fluids 2018, 30, 077107. [Google Scholar] [CrossRef]
- Gibouin, F.; Raufaste, C.; Bouret, Y.; Argentina, M. Study of the thrust-drag balance with a swimming robotic fish. Phys. Fluids 2018, 30, 091901. [Google Scholar] [CrossRef]
- Singh, N.; Gupta, A.; Mukherjee, S. A dynamic model for underwater robotic fish with a servo actuated pectoral fin. SN Appl. Sci. 2019, 1, 659. [Google Scholar] [CrossRef]
- Brooks, S.A.; Green, M.A. Experimental Study of Body-Fin Interaction and Vortex Dynamics Generated by a Two Degree-Of-Freedom Fish Model. Biomimetics 2019, 4, 67. [Google Scholar] [CrossRef] [PubMed]
- Kurt, M.; Eslam Panah, A.; Moored, K.W. Flow Interactions Between Low Aspect Ratio Hydrofoils in In-line and Staggered Arrangements. Biomimetics 2020, 5, 13. [Google Scholar] [CrossRef] [PubMed]
- Barrett, D.S.; Triantafyllou, M.S.; Yue, D.K.P.; Grosenbaugh, M.A.; Wolfgang, M.J. Drag reduction in fish-like locomotion. J. Fluid Mech. 1999, 392, 183–212. [Google Scholar] [CrossRef]
- Ogami, Y. A three-dimensional source-vorticity method for simulating incompressible potential flows around a deforming body without the Kutta condition. Comput. Fluids 2017, 154, 184–199. [Google Scholar] [CrossRef]
- Borazjani, I.; Sotiropoulos, F. On the role of form and kinematics on the hydrodynamics of self-propelled body/caudal fin swimming. J. Exp. Biol. 2010, 213, 89–107. [Google Scholar] [CrossRef]
- Liu, G.; Ren, Y.; Dong, H.; Akanyeti, O.; Liao, J.C.; Lauder, G.V. Computational analysis of vortex dynamics and performance enhancement due to body-fin and fin-fin interactions in fish-like locomotion. J. Fluid Mech. 2017, 829, 65–88. [Google Scholar] [CrossRef]
- Li, N.; Zhuang, J.; Zhu, Y.; Su, G.; Su, Y. Fluid dynamics of a self-propelled biomimetic underwater vehicle with pectoral fins. J. Ocean Eng. Sci. 2021, 6, 160–169. [Google Scholar] [CrossRef]
- Zhang, D.; Huang, Q.G.; Pan, G.; Yang, L.; Huang, W.X. Vortex dynamics and hydrodynamic performance enhancement mechanism in batoid fish oscillatory swimming. J. Fluid Mech. 2022, 930, A28. [Google Scholar] [CrossRef]
- Zhang, F.; Pang, J.; Wu, Z.; Liu, J.; Zhong, Y. Effects of Different Motion Parameters on the Interaction of Fish School Subsystems. Biomimetics 2023, 8, 510. [Google Scholar] [CrossRef] [PubMed]
- Li, Z.; Xia, D.; Zhou, X.; Cao, J.; Chen, W.; Wang, X. The hydrodynamics of self-rolling locomotion driven by the flexible pectoral fins of 3-D bionic dolphin. J. Ocean Eng. Sci. 2022, 7, 29–40. [Google Scholar] [CrossRef]
- Chang, X.; Zhang, L.; He, X. Numerical study of the thunniform mode of fish swimming with different Reynolds number and caudal fin shape. Comput. Fluids 2012, 68, 54–70. [Google Scholar] [CrossRef]
- Li, N.; Liu, H.; Su, Y. Numerical study on the hydrodynamics of thunniform bio-inspired swimming under self-propulsion. PLoS ONE 2017, 12, e0174740. [Google Scholar] [CrossRef]
- Adkins, D.; Yan, Y.Y. CFD Simulation of Fish-like Body Moving in Viscous Liquid. J. Bionic Eng. 2006, 3, 147–153. [Google Scholar] [CrossRef]
- Bottom, R.G.; Borazjani, I.; Blevins, E.L.; Lauder, G.V. Hydrodynamics of swimming in stingrays: Numerical simulations and the role of the leading-edge vortex. J. Fluid Mech. 2016, 788, 407–443. [Google Scholar] [CrossRef]
- Ogunka, U.E.; Daghooghi, M.; Akbarzadeh, A.M.; Borazjani, I. The Ground Effect in Anguilliform Swimming. Biomimetics 2020, 5, 9. [Google Scholar] [CrossRef]
- Drucker, E.G.; Lauder, G.V. A hydrodynamic analysis of fish swimming speed: Wake structure and locomotor force in slow and fast labriform swimmers. J. Exp. Biol. 2000, 203, 2379–2393. [Google Scholar] [CrossRef]
- Wolfgang, M.; Anderson, J.; Grosenbaugh, M.; Yue, D.; Triantafyllou, M. Near-body flow dynamics in swimming fish. J. Exp. Biol. 1999, 202 Pt 17, 2303–2327. [Google Scholar] [CrossRef] [PubMed]
- Cui, Z.; Yang, Z.; Jiang, H. Sharp interface immersed boundary method for simulating three-dimensional swimming fish. Eng. Appl. Comput. Fluid Mech. 2020, 14, 534–544. [Google Scholar] [CrossRef]
- Videler, J.J.; Hess, F. Fast Continuous Swimming of Two Pelagic Predators, Saithe (Pollachius Virens) and Mackerel (Scomber Scombrus): A Kinematic Analysis. J. Exp. Biol. 1984, 109, 209–228. [Google Scholar] [CrossRef]
- Gray, J. Studies in Animal Locomotion: VI. The Propulsive Powers of the Dolphin. J. Exp. Biol. 1936, 13, 192–199. [Google Scholar] [CrossRef]
- Lighthill, J. Large-Amplitude Elongated-Body Theory of Fish Locomotion. R. Soc. 1971, 179, 125–138. [Google Scholar] [CrossRef]
- Ehrenstein, U.; Marquillie, M.; Eloy, C. Skin friction on a flapping plate in uniform flow. Philos. Trans. R. Soc. A Math. Phys. Eng. Sci. 2014, 372, 20130345. [Google Scholar] [CrossRef]
- Anderson, E.J.; Mcgillis, W.R.; Grosenbaugh, M.A. The boundary layer of swimming fish. J. Exp. Biol. 2001, 204, 81–102. [Google Scholar] [CrossRef]
- Maertens, A.P.; Gao, A.; Triantafyllou, M.S. Optimal undulatory swimming for a single fish-like body and for a pair of interacting swimmers. J. Fluid Mech. 2017, 813, 301–345. [Google Scholar] [CrossRef]
- Li, Y.; Song, J.; Zhong, Y.; Yin, B. The roles of fish median fins on the hydrodynamics and muscle actuation in carangiform swimming. J. Fluids Struct. 2023, 123, 104000. [Google Scholar] [CrossRef]
- Senturk, U.; Smits, A.J. Reynolds Number Scaling of the Propulsive Performance of a Pitching Airfoil. AIAA J. 2019, 57, 2663–2669. [Google Scholar] [CrossRef]
- Smits, A.J. Undulatory and oscillatory swimming. J. Fluid Mech. 2019, 874, P1. [Google Scholar] [CrossRef]
- Buchholz, J.H.J.; Smits, A.J. The wake structure and thrust performance of a rigid low-aspect-ratio pitching panel. J. Fluid Mech. 2008, 603, 331–365. [Google Scholar] [CrossRef] [PubMed]
- Kohannim, S.; Iwasaki, T. Analytical insights into optimality and resonance in fish swimming. J. R. Soc. Interface 2014, 11, 20131073. [Google Scholar] [CrossRef] [PubMed]
- Wu, B.; Shu, C.; Lee, H.; Wan, M. Numerical study on the hydrodynamic performance of an unconstrained carangiform swimmer. Phys. Fluids 2022, 34, 121902. [Google Scholar] [CrossRef]
- Yu, C.L.; Hsu, Y.H.; Yang, J.T. The dependence of propulsive performance on the slip number in an undulatory swimming fish. Ocean Eng. 2013, 70, 51–60. [Google Scholar] [CrossRef]
- Wu, Y.T.; Porte-Agel, F.; Porté-Agel, F. Large-Eddy Simulation of Wind-Turbine Wakes: Evaluation of Turbine Parametrisations. Bound.-Layer Meteorol. 2011, 138, 345–366. [Google Scholar] [CrossRef]
- Borazjani, I.; Daghooghi, M. The fish tail motion forms an attached leading edge vortex. Proc. R. Soc. B Biol. Sci. 2013, 280, 20122071. [Google Scholar] [CrossRef]
- Mignano, A.; Kadapa, S.; Tangorra, J.; Lauder, G. Passing the Wake: Using Multiple Fins to Shape Forces for Swimming. Biomimetics 2019, 4, 23. [Google Scholar] [CrossRef] [PubMed]
- Xiong, Z.; Liu, X. Numerical investigation on evolutionary characteristics of the leading-edge vortex induced by flapping caudal fin. Phys. Fluids 2019, 31, 125117. [Google Scholar] [CrossRef]
- Han, P.; Lauder, G.V.; Dong, H. Hydrodynamics of median-fin interactions in fish-like locomotion: Effects of fin shape and movement. Phys. Fluids 2020, 32, 011902. [Google Scholar] [CrossRef]
- Tack, N.B.; Gemmell, B.J. A tale of two fish tails: Does a forked tail really perform better than a truncate tail when cruising? J. Exp. Biol. 2022, 225, jeb244967. [Google Scholar] [CrossRef]
- Botelho, H.A.; de Assis Lago, A.; da Costa, A.L.; Costa, A.C.; Reis Neto, R.V.; Silva, Z.; Ribeiro, F.M.; Café, M.B.; de Freitas, R.T.F. Application of morphometric measures in estimation of body weight and discrimination of Astyanax lacustris and Astyanax fasciatus. Aquac. Res. 2019, 50, 2429–2436. [Google Scholar] [CrossRef]
- Videler, J.J.; Wardle, C.S. Fish swimming stride by stride: Speed limits and endurance. Rev. Fish Biol. Fish. 1991, 1, 23–40. [Google Scholar] [CrossRef]
- Menter, F.R.; Langtry, R.; Völker, S.; Huang, P.G. Transition Modelling for General Purpose CFD Codes. Flow Turbul. Combust. 2006, 77, 277–303. [Google Scholar] [CrossRef]
- Schlichting, H.; Kestin, J. Boundary Layer Theory; Springer: Berlin/Heidelberg, Germany, 1961; Volume 121. [Google Scholar]
- Cheng, M.; Zhu, Y. The state of the art of wind energy conversion systems and technologies: A review. Energy Convers. Manag. 2014, 88, 332–347. [Google Scholar] [CrossRef]
- Tytell, E.D. Median fin function in bluegill sunfish Lepomis macrochirus: Streamwise vortex structure during steady swimming. J. Exp. Biol. 2006, 209, 1516–1534. [Google Scholar] [CrossRef] [PubMed]
- Namshad, T.; Shrivastava, M.; Agrawal, A.; Sharma, A. Effect of wavelength of fish-like undulation of a hydrofoil in a free-stream flow. Sādhanā 2017, 42, 585–595. [Google Scholar] [CrossRef]



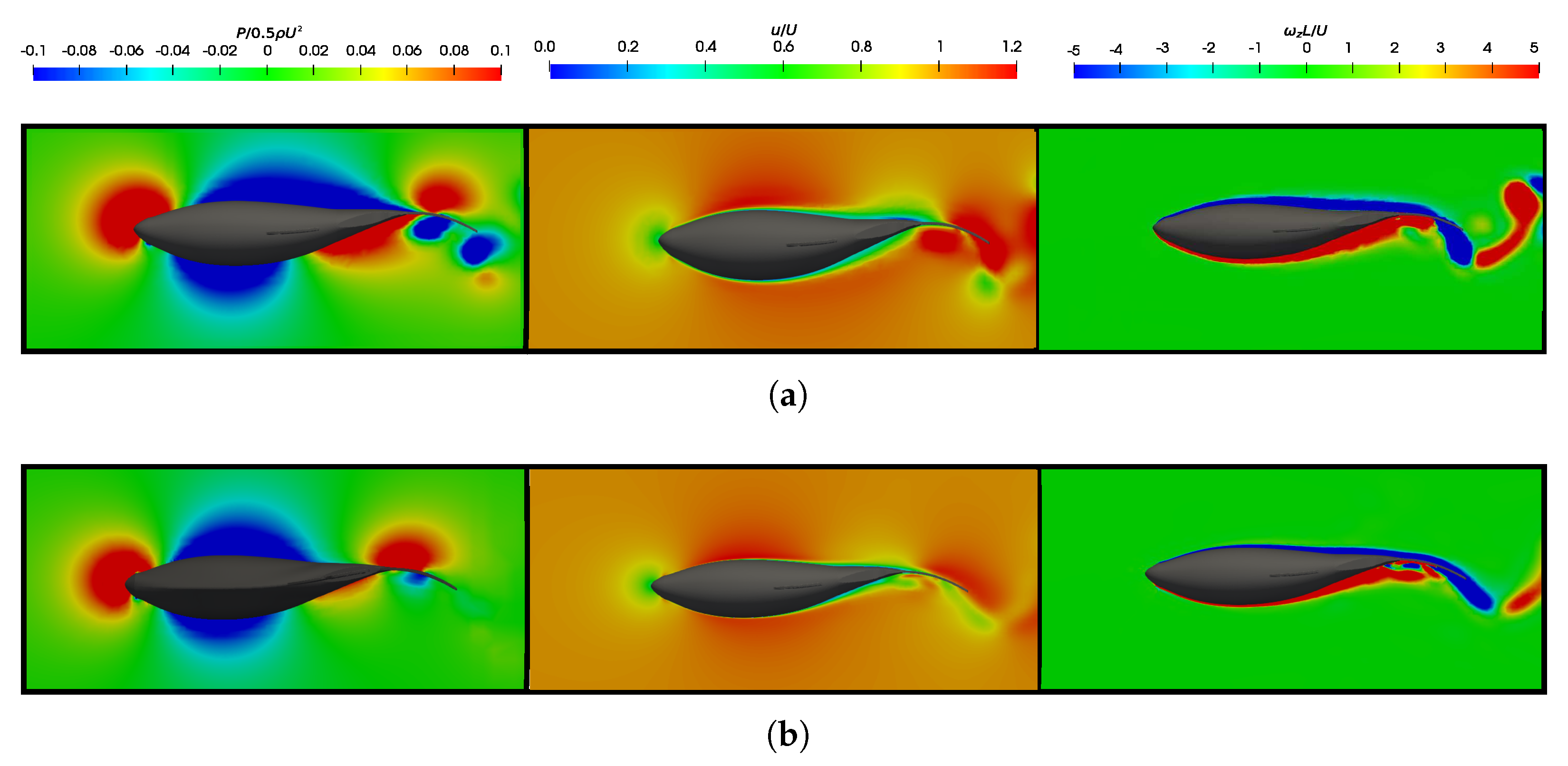
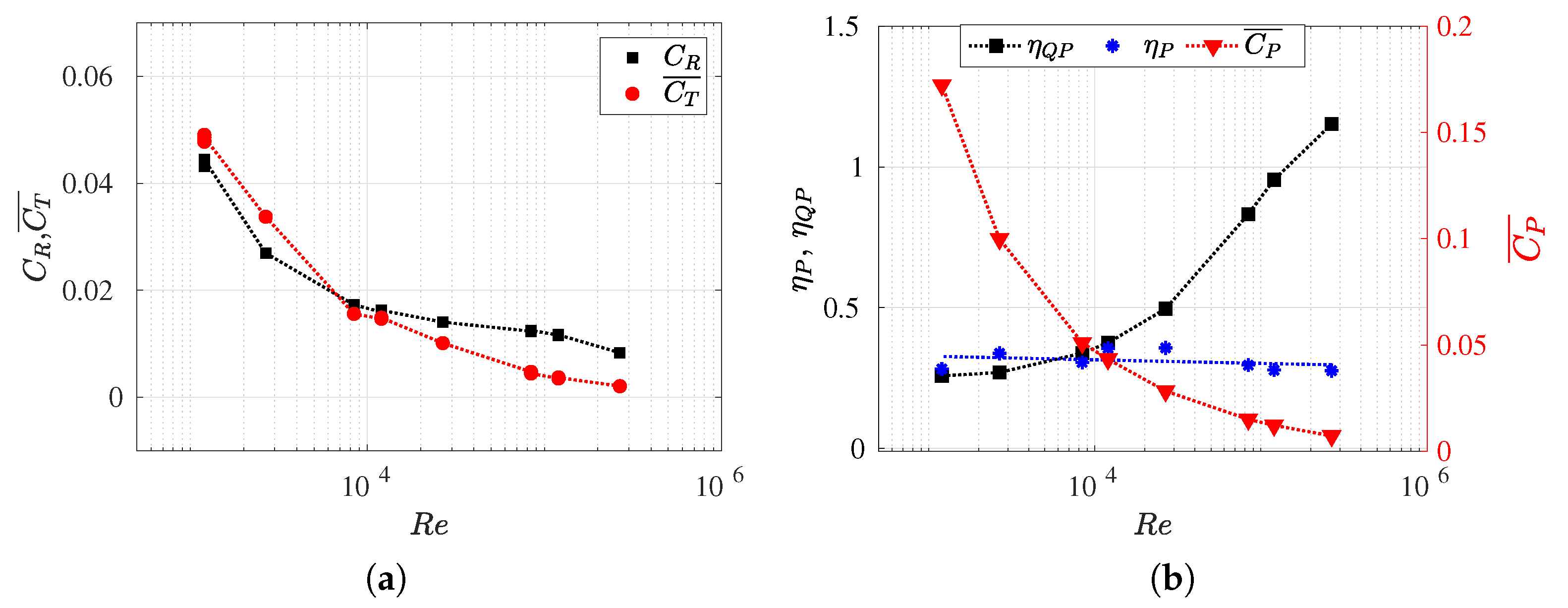


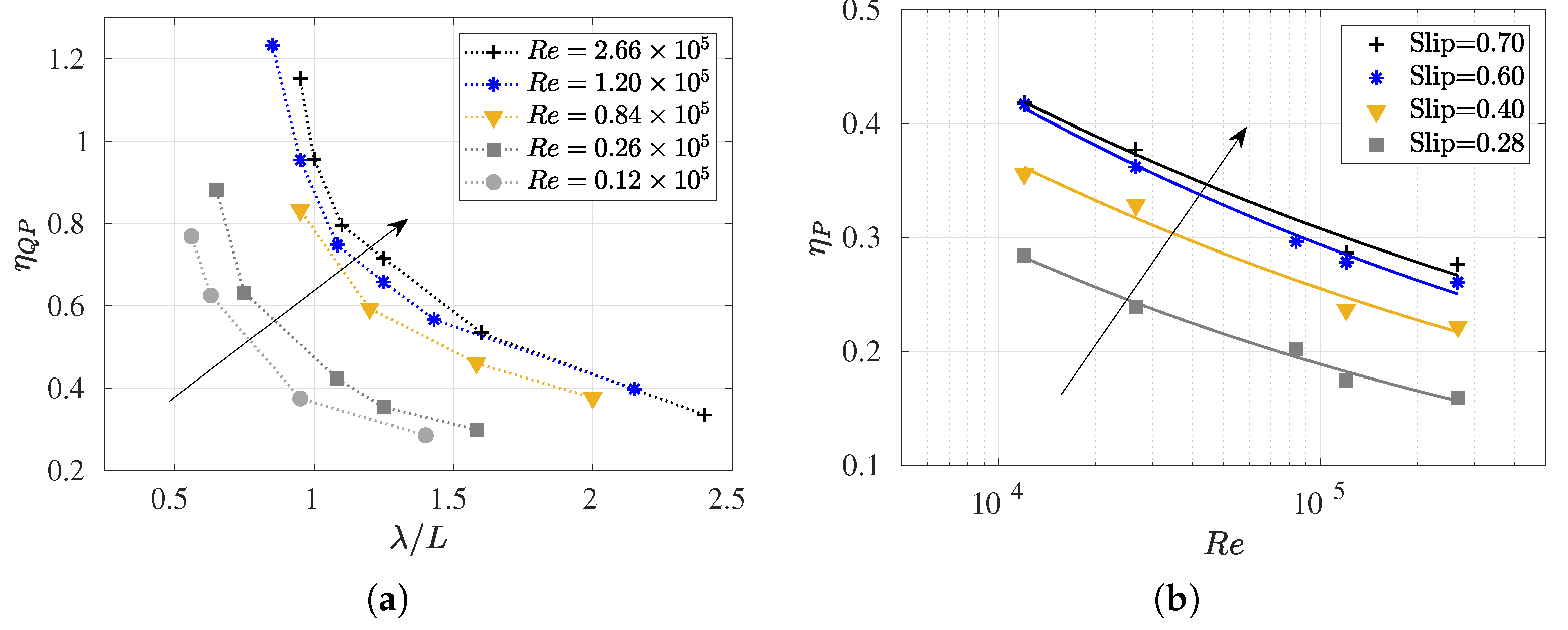


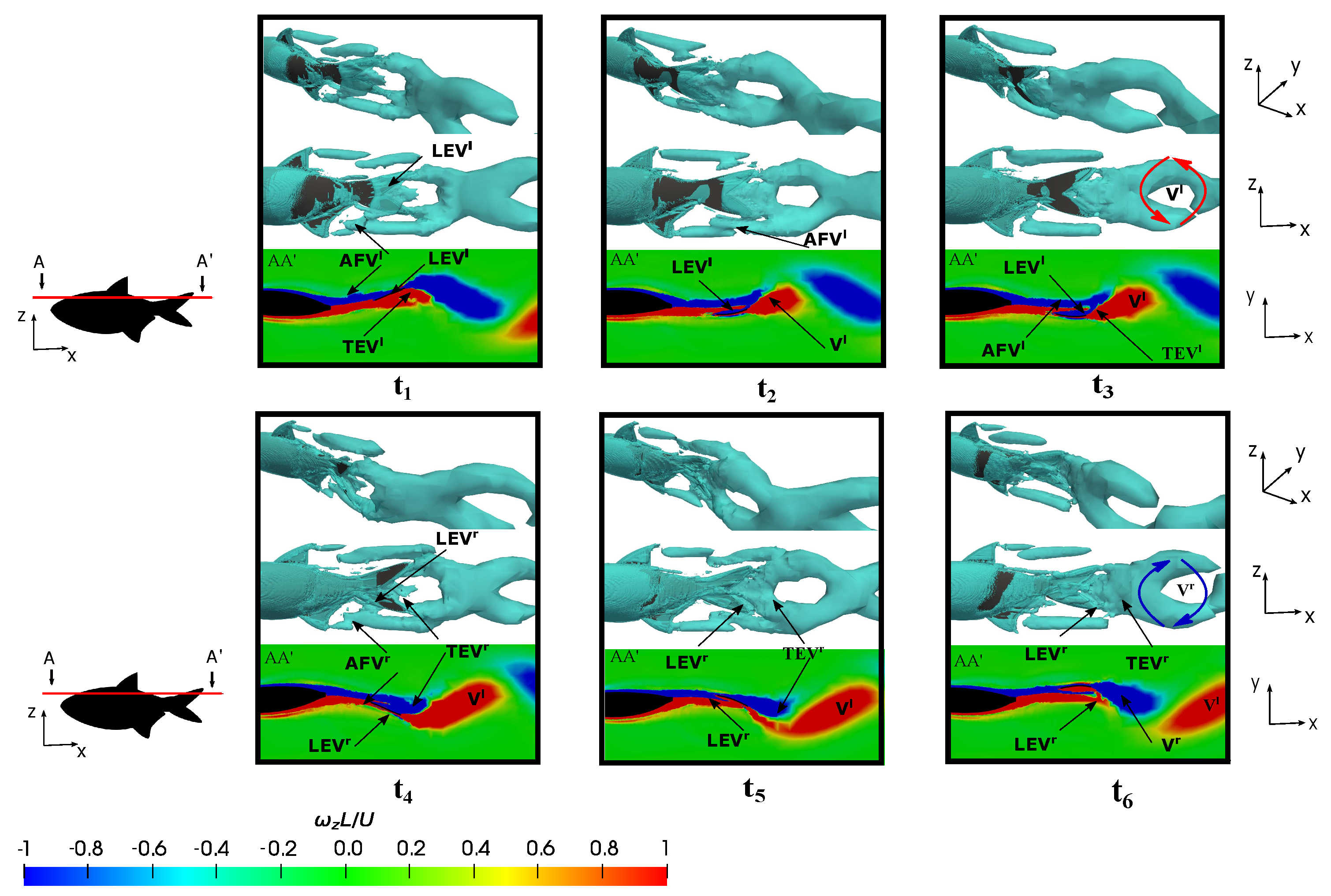

| Grid | Nodes | Error on (%) | |||
|---|---|---|---|---|---|
| Coarse | 0.0093L | 2.48 | 0.0328 | 3.8% | |
| Medium | 0.0051L | 0.36 | 0.0341 | 0.6% | |
| Fine | 0.0027L | 0.16 | 0.0339 | - |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Macías, M.M.; García-Ortiz, J.H.; Oliveira, T.F.; Brasil Junior, A.C.P. Numerical Investigation of Dimensionless Parameters in Carangiform Fish Swimming Hydrodynamics. Biomimetics 2024, 9, 45. https://doi.org/10.3390/biomimetics9010045
Macías MM, García-Ortiz JH, Oliveira TF, Brasil Junior ACP. Numerical Investigation of Dimensionless Parameters in Carangiform Fish Swimming Hydrodynamics. Biomimetics. 2024; 9(1):45. https://doi.org/10.3390/biomimetics9010045
Chicago/Turabian StyleMacías, Marianela Machuca, José Hermenegildo García-Ortiz, Taygoara Felamingo Oliveira, and Antonio Cesar Pinho Brasil Junior. 2024. "Numerical Investigation of Dimensionless Parameters in Carangiform Fish Swimming Hydrodynamics" Biomimetics 9, no. 1: 45. https://doi.org/10.3390/biomimetics9010045
APA StyleMacías, M. M., García-Ortiz, J. H., Oliveira, T. F., & Brasil Junior, A. C. P. (2024). Numerical Investigation of Dimensionless Parameters in Carangiform Fish Swimming Hydrodynamics. Biomimetics, 9(1), 45. https://doi.org/10.3390/biomimetics9010045








