Biomimetic Design of a Tendon-Driven Myoelectric Soft Hand Exoskeleton for Upper-Limb Rehabilitation
Abstract
1. Introduction
2. Materials and Methods
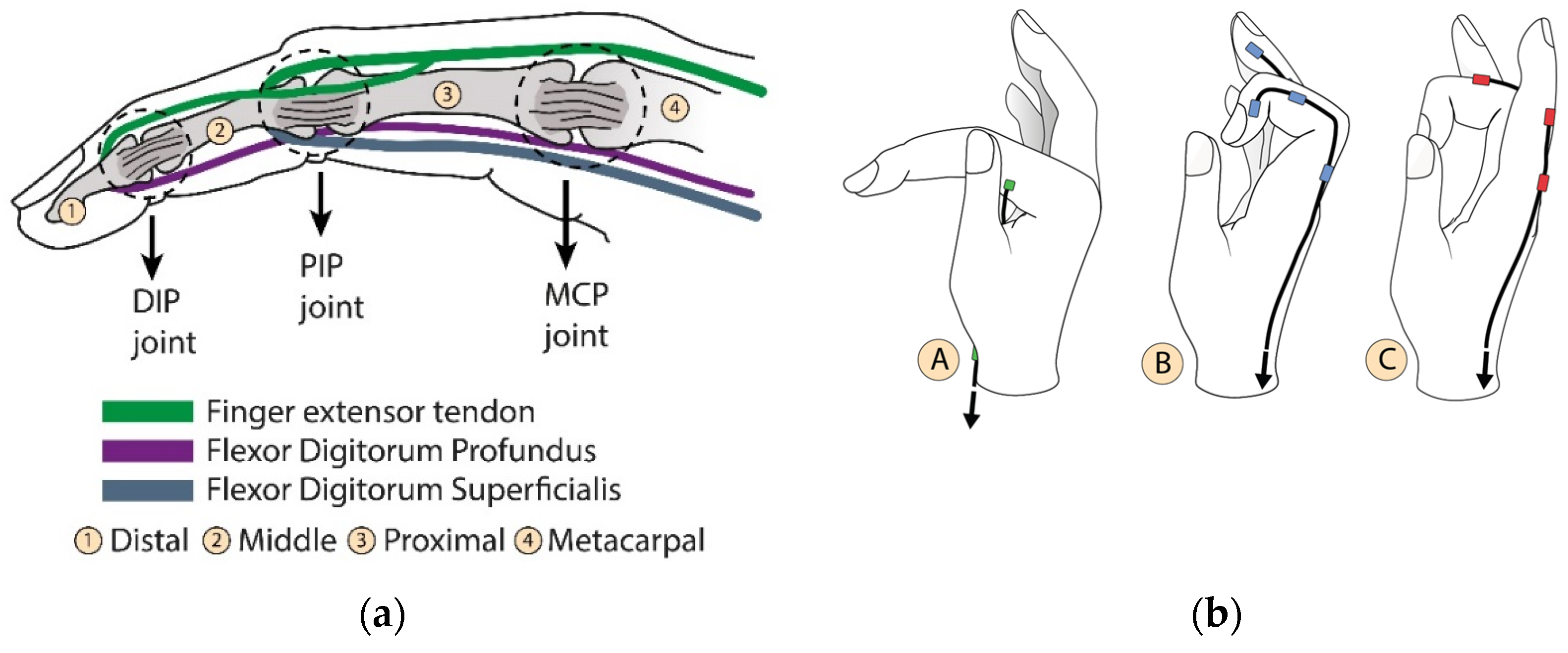
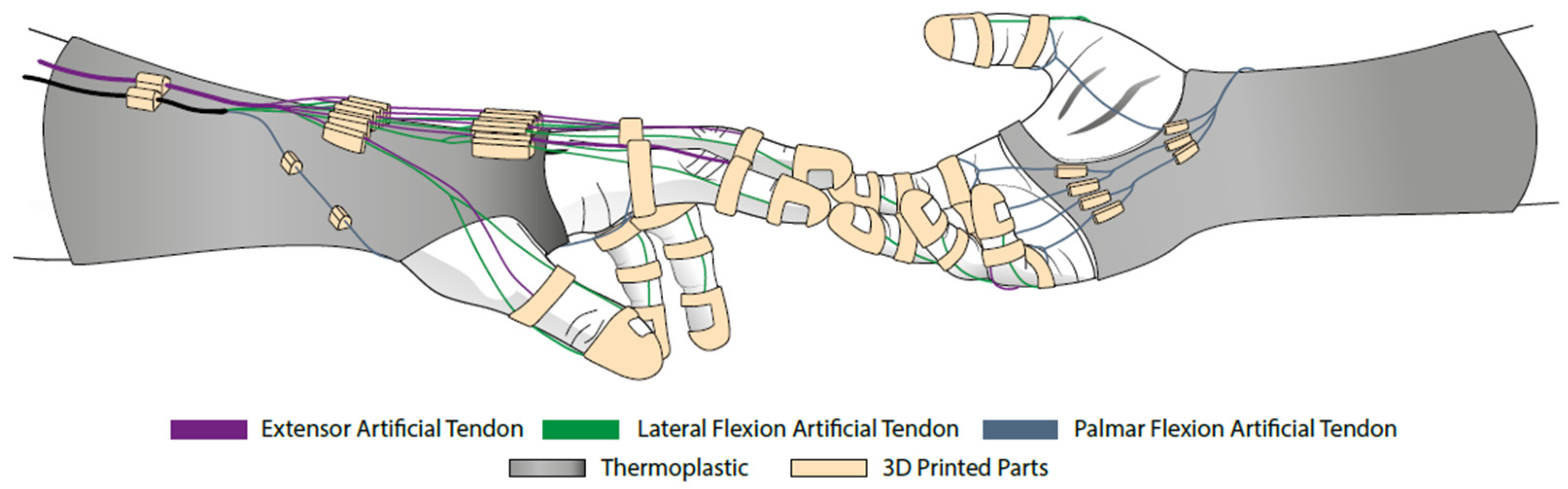
2.1. Bioinspired Design
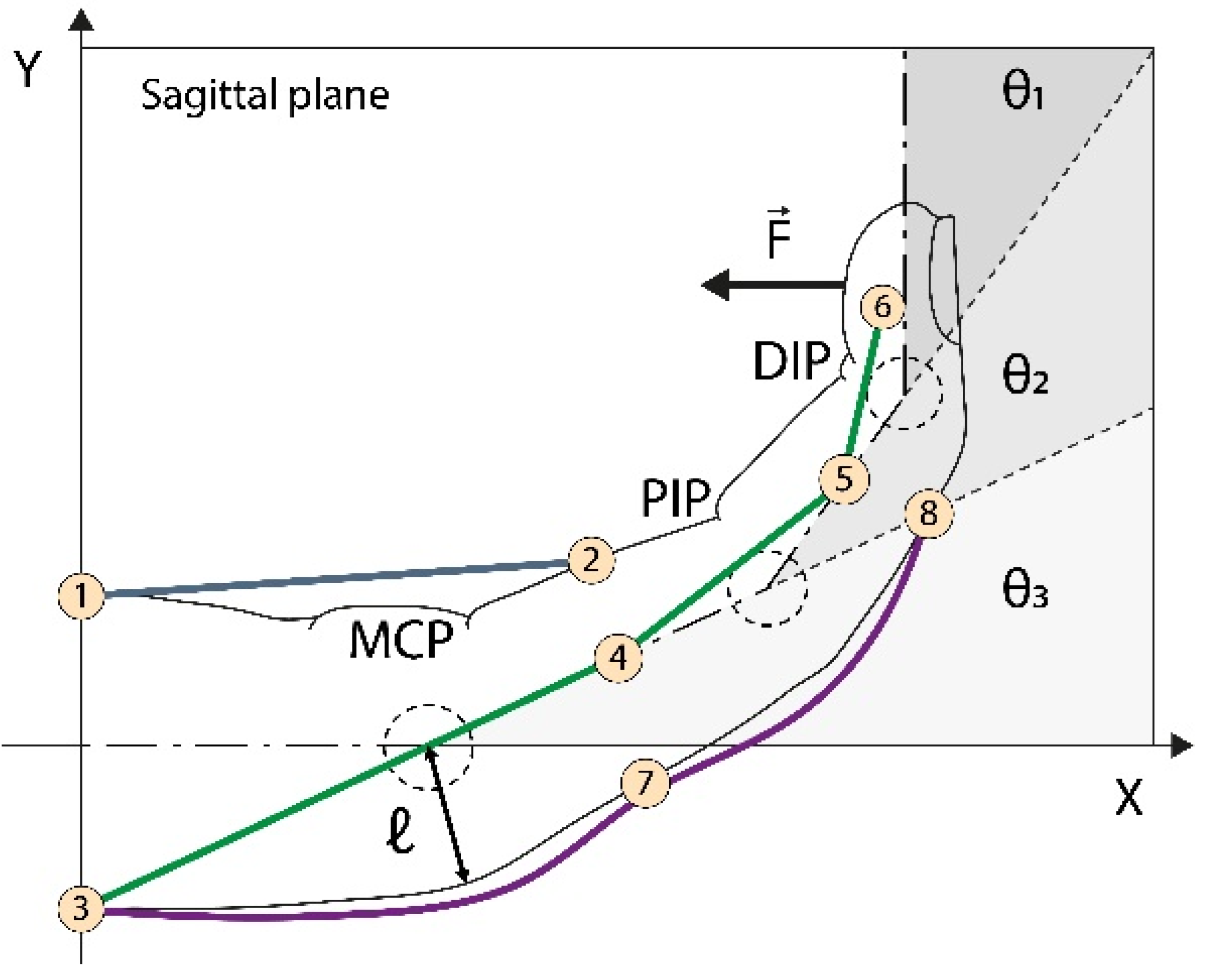
2.2. Hand Exoskeleton Kinematics
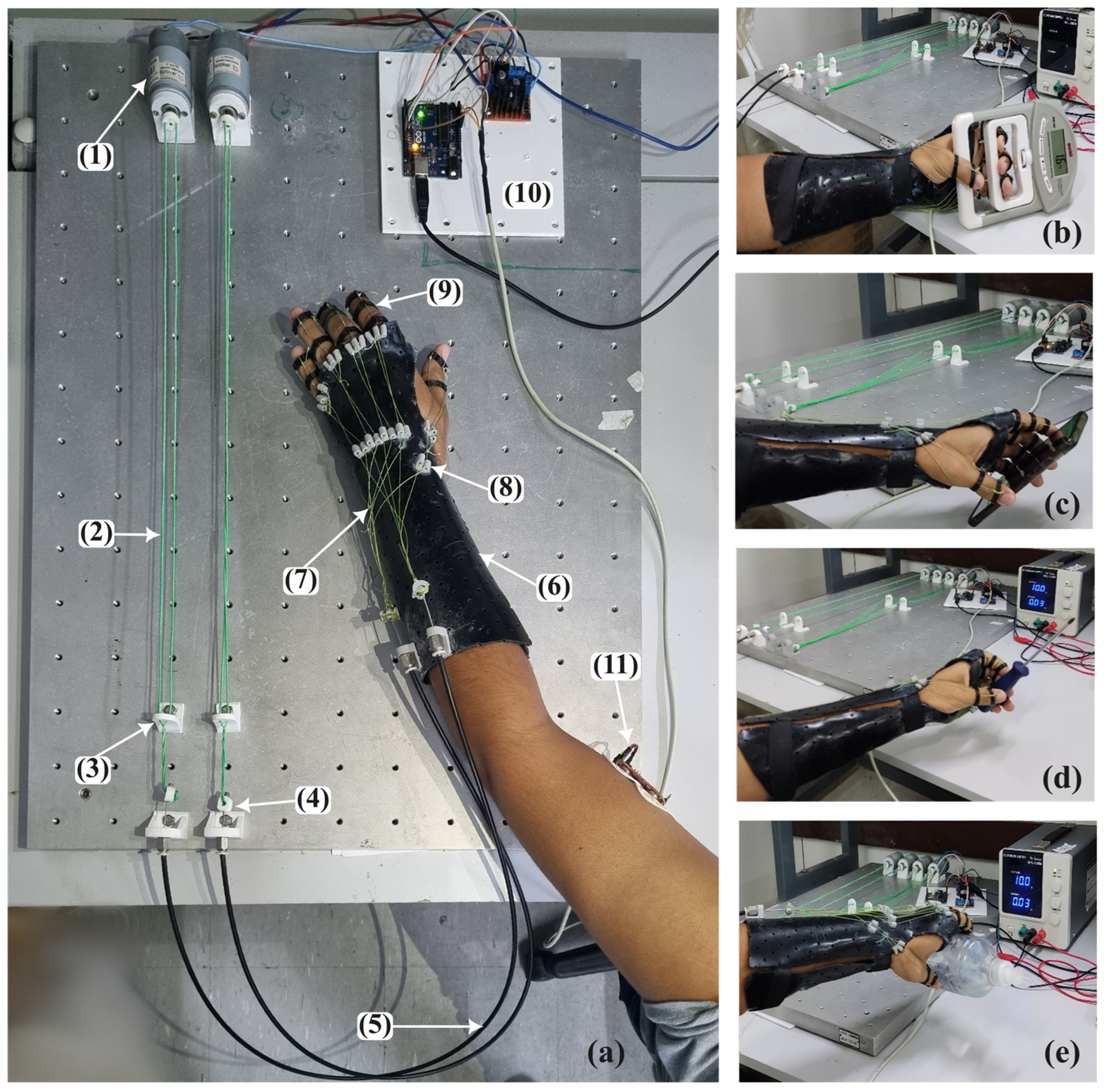
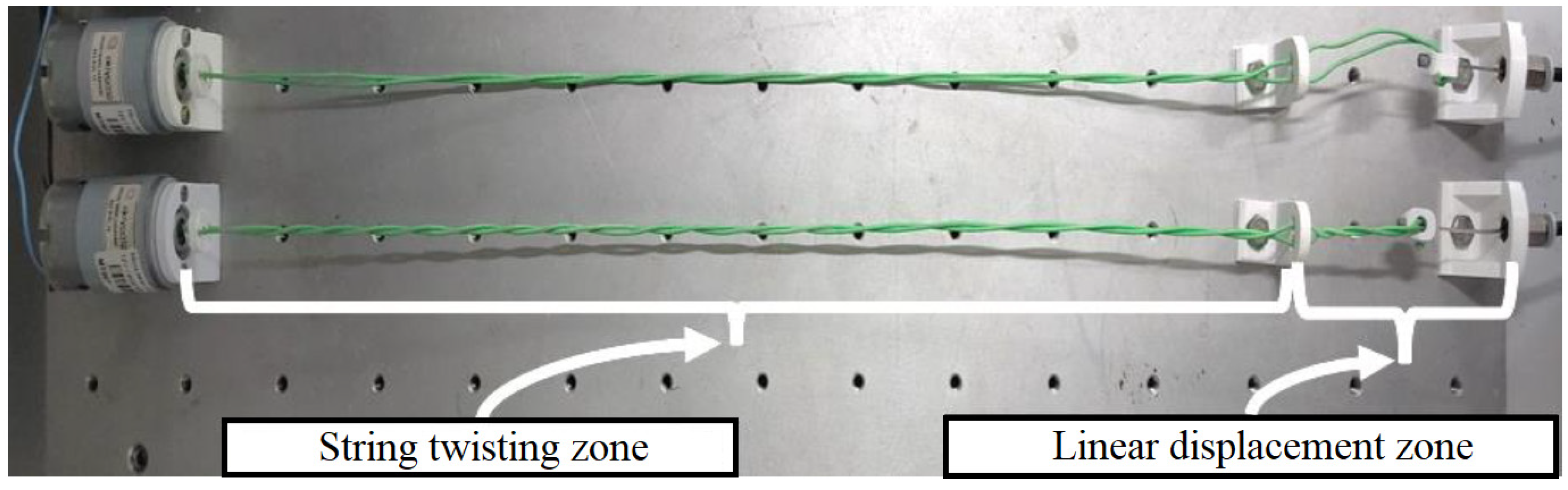
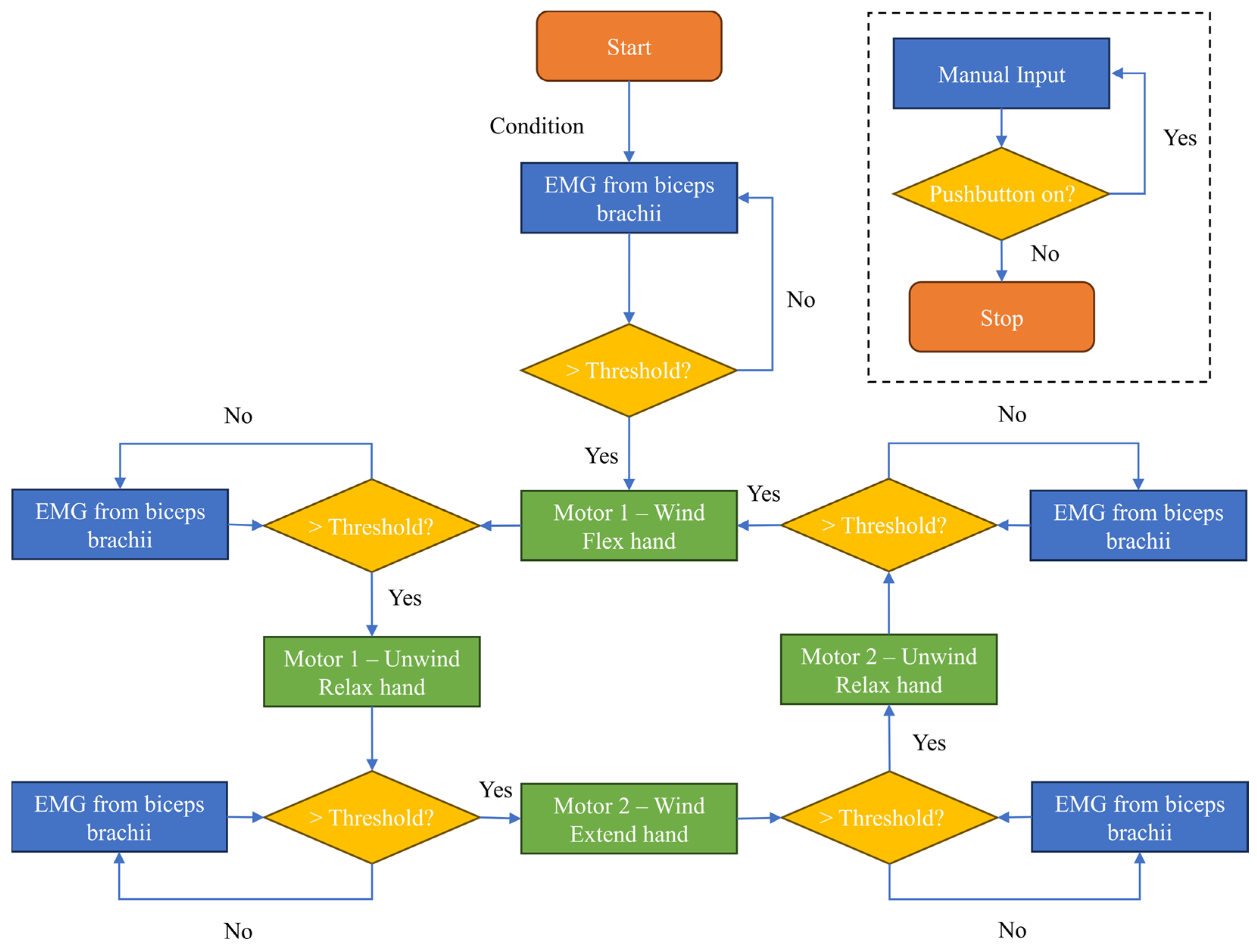
2.3. Physical Prototype and Experimental Setup
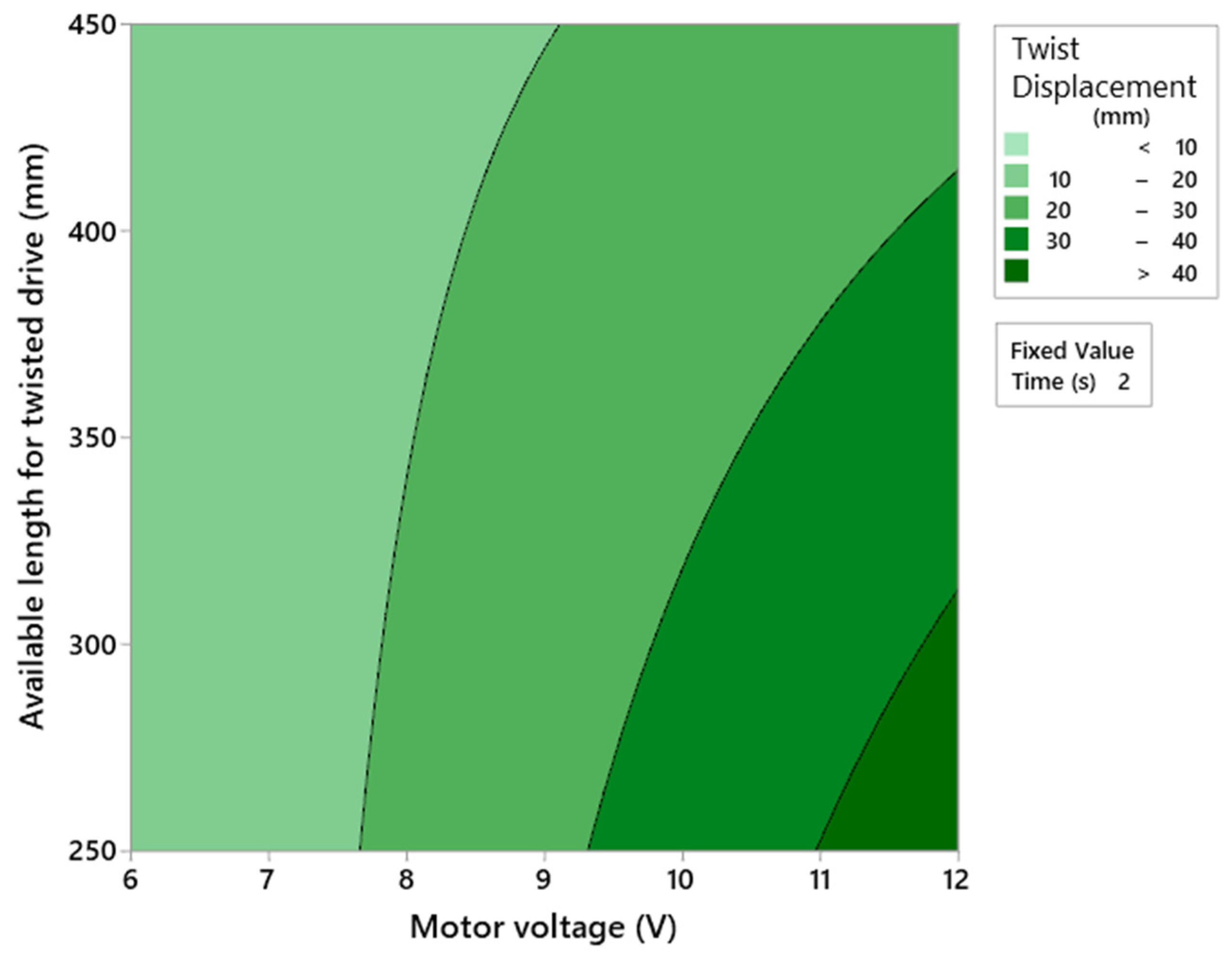
2.4. Experimental Protocol and Data Analysis
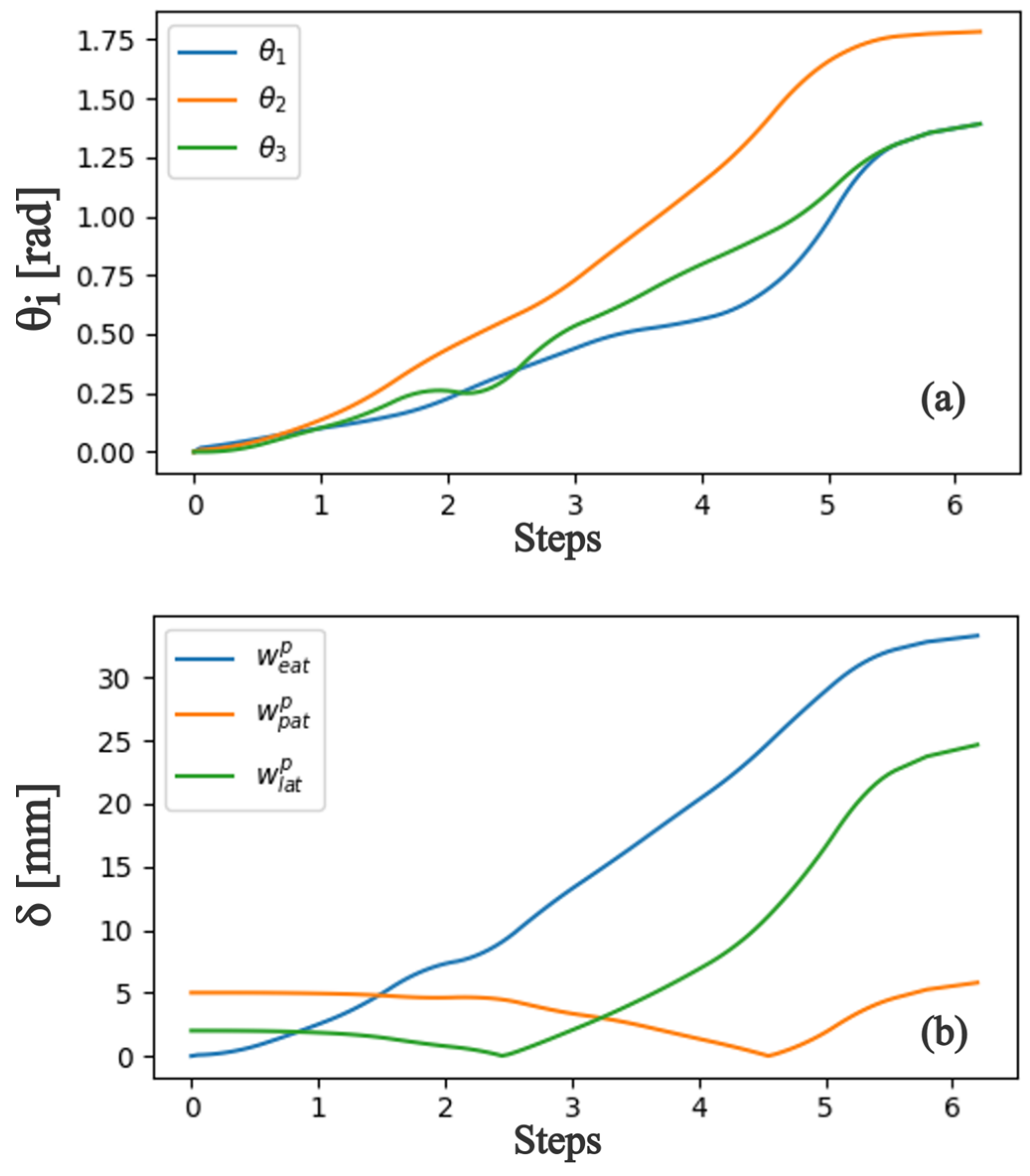
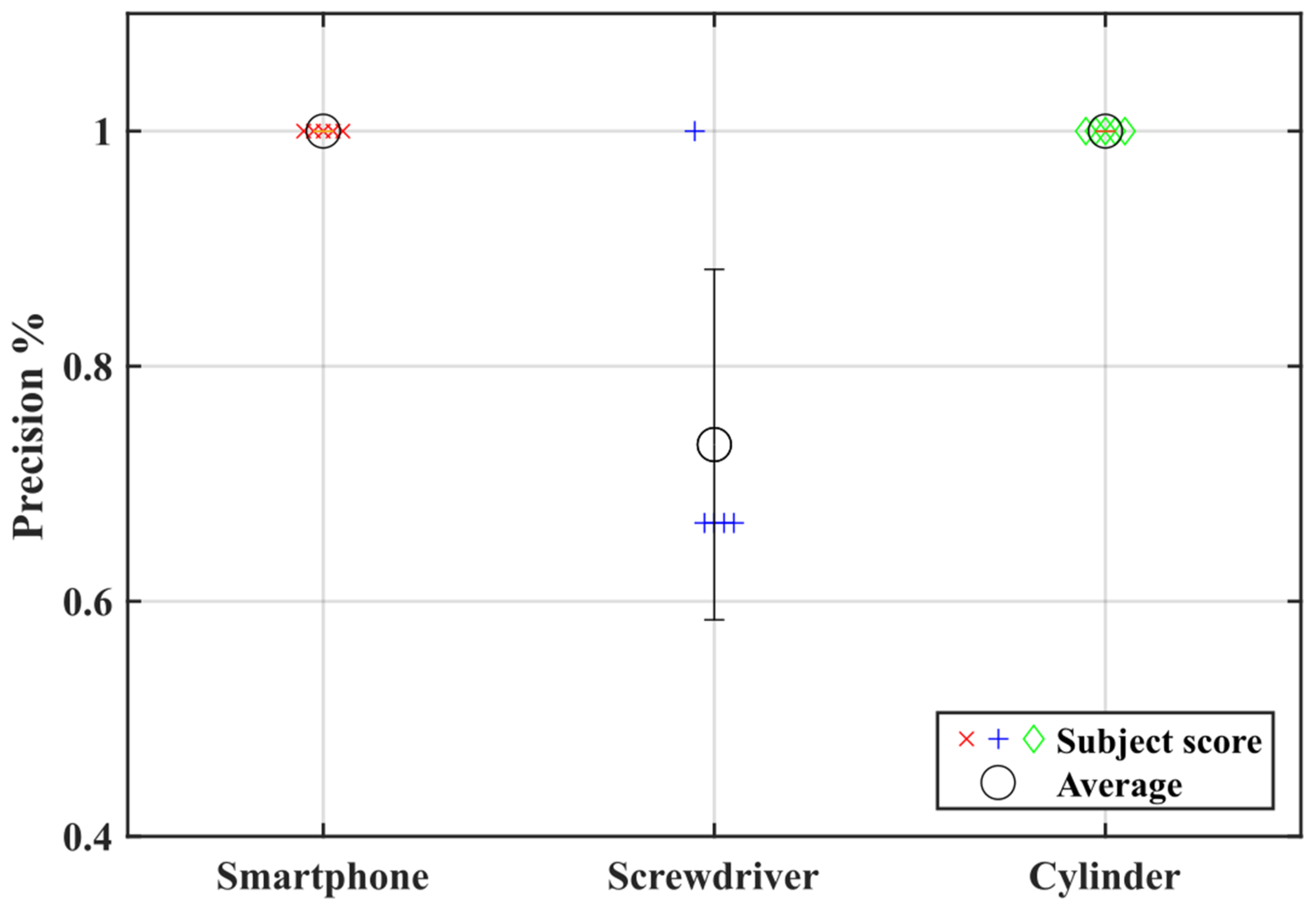
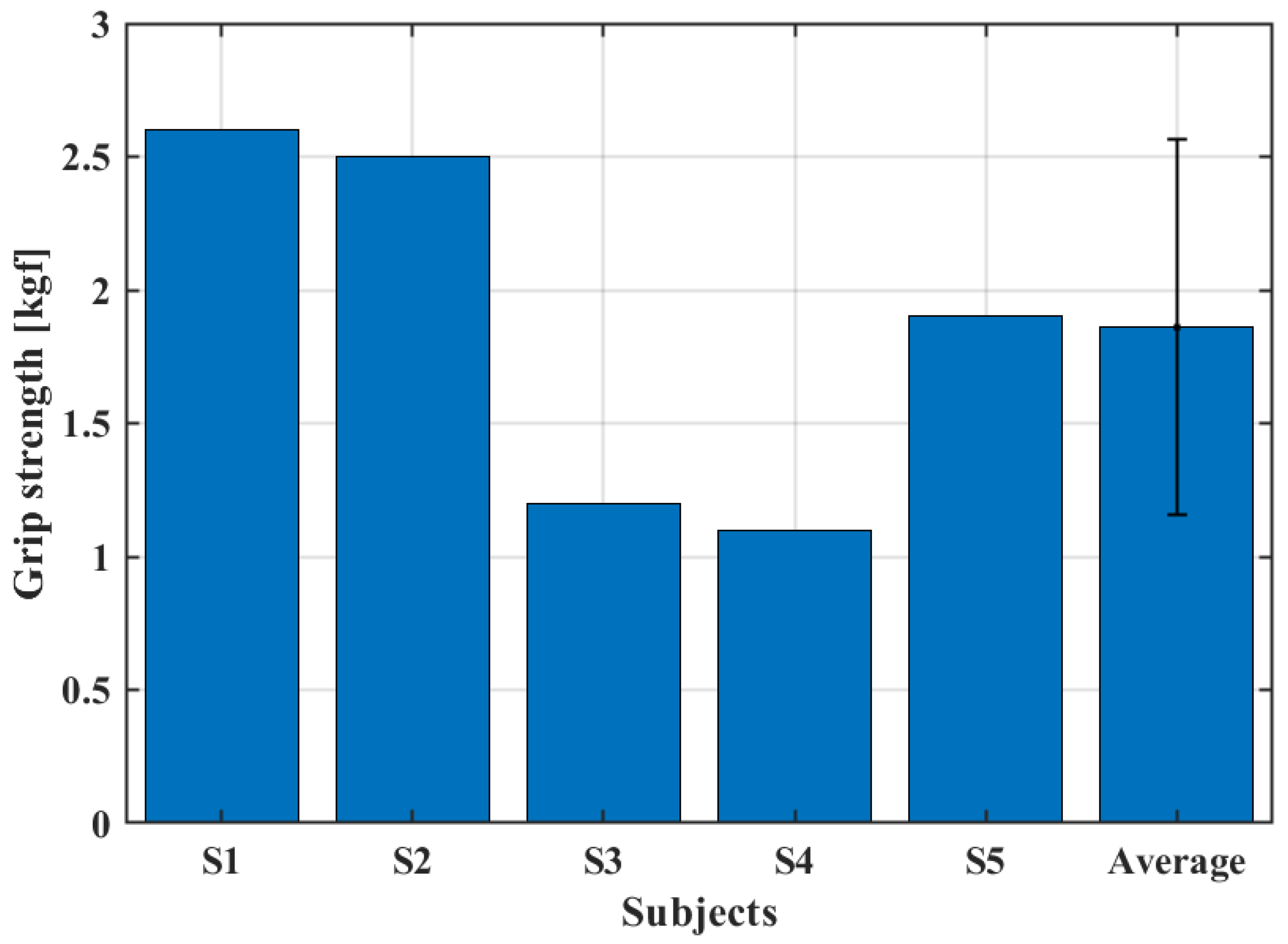
3. Results and Discussion
4. Conclusions
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
References
- Padilla-Magaña, J.F.; Peña-Pitarch, E.; Sánchez-Suarez, I.; Ticó-Falguera, N. Quantitative Assessment of Hand Function in Healthy Subjects and Post-Stroke Patients with the Action Research Arm Test. Sensors 2022, 22, 3604. [Google Scholar] [CrossRef] [PubMed]
- Raghavan, P. Upper Limb Motor Impairment after Stroke. Phys. Med. Rehabil. Clin. 2015, 26, 599–610. [Google Scholar] [CrossRef]
- Langhorne, P.; Coupar, F.; Pollock, A. Motor Recovery after Stroke: A Systematic Review. Lancet Neurol. 2009, 8, 741–754. [Google Scholar] [CrossRef] [PubMed]
- Vos, T.; Abajobir, A.A.; Abate, K.H.; Abbafati, C.; Abbas, K.M.; Abd-Allah, F.; Abdulkader, R.S.; Abdulle, A.M.; Abebo, T.A.; Abera, S.F.; et al. Global, Regional, and National Incidence, Prevalence, and Years Lived with Disability for 328 Diseases and Injuries for 195 Countries, 1990–2016: A Systematic Analysis for the Global Burden of Disease Study 2016. Lancet 2017, 390, 1211–1259. [Google Scholar] [CrossRef]
- Feigin, V.L.; Stark, B.A.; Johnson, C.O.; Roth, G.A.; Bisignano, C.; Abady, G.G.; Abbasifard, M.; Abbasi-Kangevari, M.; Abd-Allah, F.; Abedi, V.; et al. Global, Regional, and National Burden of Stroke and Its Risk Factors, 1990–2019: A Systematic Analysis for the Global Burden of Disease Study 2019. Lancet Neurol. 2021, 20, 795–820. [Google Scholar] [CrossRef]
- Hatem, S.M.; Saussez, G.; della Faille, M.; Prist, V.; Zhang, X.; Dispa, D.; Bleyenheuft, Y. Rehabilitation of Motor Function after Stroke: A Multiple Systematic Review Focused on Techniques to Stimulate Upper Extremity Recovery. Front. Hum. Neurosci. 2016, 10, 442. [Google Scholar]
- Lang, C.E.; MacDonald, J.R.; Reisman, D.S.; Boyd, L.; Kimberley, T.J.; Schindler-Ivens, S.M.; Hornby, T.G.; Ross, S.A.; Scheets, P.L. Observation of Amounts of Movement Practice Provided During Stroke Rehabilitation. Arch. Phys. Med. Rehabil. 2009, 90, 1692–1698. [Google Scholar] [CrossRef]
- Waddell, K.J.; Birkenmeier, R.L.; Moore, J.L.; Hornby, T.G.; Lang, C.E. Feasibility of High-Repetition, Task-Specific Training for Individuals with Upper-Extremity Paresis. Am. J. Occup. Ther. 2014, 68, 444–453. [Google Scholar] [CrossRef]
- Kleim, J.A.; Jones, T.A. Principles of Experience-Dependent Neural Plasticity: Implications for Rehabilitation After Brain Damage. J. Speech Lang. Hear. Res. 2008, 51, S225–S239. [Google Scholar] [CrossRef]
- Bertani, R.; Melegari, C.; De Cola, M.C.; Bramanti, A.; Bramanti, P.; Calabrò, R.S. Effects of Robot-Assisted Upper Limb Rehabilitation in Stroke Patients: A Systematic Review with Meta-Analysis. Neurol. Sci. 2017, 38, 1561–1569. [Google Scholar] [CrossRef]
- Heo, P.; Gu, G.M.; Lee, S.; Rhee, K.; Kim, J. Current Hand Exoskeleton Technologies for Rehabilitation and Assistive Engineering. Int. J. Precis. Eng. Manuf. 2012, 13, 807–824. [Google Scholar] [CrossRef]
- Leonardis, D.; Barsotti, M.; Loconsole, C.; Solazzi, M.; Troncossi, M.; Mazzotti, C.; Castelli, V.P.; Procopio, C.; Lamola, G.; Chisari, C.; et al. An EMG-Controlled Robotic Hand Exoskeleton for Bilateral Rehabilitation. IEEE Trans. Haptics 2015, 8, 140–151. [Google Scholar] [CrossRef]
- Gasser, B.W.; Bennett, D.A.; Durrough, C.M.; Goldfarb, M. Design and Preliminary Assessment of Vanderbilt Hand Exoskeleton. In Proceedings of the 2017 International Conference on Rehabilitation Robotics (ICORR), London, UK, 17–20 July 2017; pp. 1537–1542. [Google Scholar]
- Andrade, R.M.; Bonato, P. The Role Played by Mass, Friction, and Inertia on the Driving Torques of Lower-Limb Gait Training Exoskeletons. IEEE Trans. Med. Robot. Bionics 2021, 3, 125–136. [Google Scholar] [CrossRef]
- de Andrade, R.M.; Fabriz Ulhoa, P.H.; Fragoso Dias, E.A.; Filho, A.B.; Vimieiro, C.B.S. Design and testing a highly backdrivable and kinematic compatible magneto-rheological knee exoskeleton. J. Intell. Mater. Syst. Struct. 2023, 34, 653–663. [Google Scholar] [CrossRef]
- Noronha, B.; Accoto, D. Exoskeletal Devices for Hand Assistance and Rehabilitation: A Comprehensive Analysis of State-of-the-Art Technologies. IEEE Trans. Med. Robot. Bionics 2021, 3, 525–538. [Google Scholar] [CrossRef]
- Novelli, G.L.; Vargas, G.G.; Andrade, R.M. Dielectric elastomer actuators as artificial muscles for wearable robots. J. Intell. Mater. Syst. Struct. 2023, 34, 1007–1025. [Google Scholar] [CrossRef]
- Pinskier, J.; Shirinzadeh, B. Topology optimization of leaf flexures for stiffness ratio maximization in compliant mechanisms. In Proceedings of the 2018 IEEE/ASME International Conference on Advanced Intelligent Mechatronics (AIM), Auckland, New Zealand, 9–12 July 2018; pp. 407–412. [Google Scholar] [CrossRef]
- Sun, Y.; Lueth, T.C. Enhancing Torsional Stiffness of Continuum Robots Using 3-D Topology Optimized Flexure Joints. IEEE/ASME Trans. Mechatron. 2023. [Google Scholar] [CrossRef]
- In, H.; Kang, B.B.; Sin, M.; Cho, K.-J. Exo-Glove: A Wearable Robot for the Hand with a Soft Tendon Routing System. IEEE Robot. Autom. Mag. 2015, 22, 97–105. [Google Scholar] [CrossRef]
- Nycz, C.J.; Bützer, T.; Lambercy, O.; Arata, J.; Fischer, G.S.; Gassert, R. Design and Characterization of a Lightweight and Fully Portable Remote Actuation System for Use with a Hand Exoskeleton. IEEE Robot. Autom. Lett. 2016, 1, 976–983. [Google Scholar] [CrossRef]
- Xiloyannis, M.; Cappello, L.; Khanh, D.B.; Yen, S.-C.; Masia, L. Modelling and Design of a Synergy-Based Actuator for a Tendon-Driven Soft Robotic Glove. In Proceedings of the 2016 6th IEEE International Conference on Biomedical Robotics and Biomechatronics (BioRob), Singapore, 26–29 June 2016; pp. 1213–1219. [Google Scholar]
- Palli, G.; Natale, C.; May, C.; Melchiorri, C.; Wurtz, T. Modeling and Control of the Twisted String Actuation System. IEEE/ASME Trans. Mechatron. 2013, 18, 664–673. [Google Scholar] [CrossRef]
- Würtz, T.; May, C.; Holz, B.; Natale, C.; Palli, G.; Melchiorri, C. The Twisted String Actuation System: Modeling and Control. In Proceedings of the 2010 IEEE/ASME International Conference on Advanced Intelligent Mechatronics, Montreal, QC, Canada, 6–9 July 2010; pp. 1215–1220. [Google Scholar]
- Hosseini, M.; Sengül, A.; Pane, Y.; De Schutter, J.; Bruyninck, H. ExoTen-Glove: A Force-Feedback Haptic Glove Based on Twisted String Actuation System. In Proceedings of the 2018 27th IEEE International Symposium on Robot and Human Interactive Communication (RO-MAN), Nanjing, China, 27–31 August 2018; pp. 320–327. [Google Scholar] [CrossRef]
- Standring, S. Gray’s Anatomy E-Book: The Anatomical Basis of Clinical Practice; Elsevier Health Sciences: Philadelphia, PA, USA, 2015. [Google Scholar]
- Cornejo, J.; Cornejo-Aguilar, J.A.; Vargas, M.; Helguero, C.G.; De Andrade, R.M.; Torres-Montoya, S.; Asensio-Salazar, J.; Rivero Calle, A.; Martínez Santos, J.; Damon, A. Anatomical Engineering and 3D Printing for Surgery and Medical Devices: International Review and Future Exponential Innovations. BioMed Res. Int. 2022, 2022, 6797745. [Google Scholar] [CrossRef] [PubMed]
- Lourenço, B.; Neto, V.; Andrade, R.d. A Concept Design of an Adaptive Tendon Driven Mechanism for Active Soft Hand Orthosis. Proceedings 2020, 64, 21. [Google Scholar]
- Lorrain, T.; Jiang, N.; Farina, D. Influence of the training set on the accuracy of surface EMG classification in dynamic contractions for the control of multifunction prostheses. J. NeuroEngineering Rehabil. 2011, 8, 25. [Google Scholar] [CrossRef] [PubMed]
- Suthar, B.; Usman, M.; Seong, H.; Gaponov, I.; Ryu, J. Preliminary Study of Twisted String Actuation Through a Conduit Toward Soft and Wearable Actuation. In Proceedings of the IEEE International Conference on Robotics and Automation (ICRA), Brisbane, QLD, Australia, 21–25 May 2018; pp. 2260–2265. [Google Scholar]
- Jeong, S.H.; Kim, K.; Kim, S. Designing Anthropomorphic Robot Hand with Active Dual-Mode Twisted String Actuation Mechanism and Tiny Tension Sensors. IEEE Robot. Autom. Lett. 2017, 2, 1571–1578. [Google Scholar] [CrossRef]
- Rúbio, G.d.P.; Martins Ferreira, F.M.R.; Brandão, F.H.d.L.; Machado, V.F.; Tonelli, L.G.; Martins, J.S.R.; Kozan, R.F.; Vimieiro, C.B.S. Evaluation of Commercial Ropes Applied as Artificial Tendons in Robotic Rehabilitation Orthoses. Appl. Sci. 2020, 10, 920. [Google Scholar] [CrossRef]
- De Luca, C.J. Surface Electromyography: Detection and Recording; Delsys: Natick, MA, USA, 2002. [Google Scholar]
- Fiorezi, G.G.; dos Santos de Moraes, J.; Ulhoa, P.H.F.; de Andrade, R.M. Biomimetic design of a planar torsional spring to an active knee prosthesis actuator using FEM analysis. Proceedings 2020, 64, 30. [Google Scholar]
- Massy-Westropp, N.M.; Gill, T.K.; Taylor, A.W.; Bohannon, R.W.; Hill, C.L. Hand Grip Strength: Age and gender stratified normative data in a population-based study. BMC Res. Notes 2011, 4, 127. [Google Scholar] [CrossRef]


Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Silva, R.C.; Lourenço, B.G.; Ulhoa, P.H.F.; Dias, E.A.F.; da Cunha, F.L.; Tonetto, C.P.; Villani, L.G.; Vimieiro, C.B.S.; Lepski, G.A.; Monjardim, M.; et al. Biomimetic Design of a Tendon-Driven Myoelectric Soft Hand Exoskeleton for Upper-Limb Rehabilitation. Biomimetics 2023, 8, 317. https://doi.org/10.3390/biomimetics8030317
Silva RC, Lourenço BG, Ulhoa PHF, Dias EAF, da Cunha FL, Tonetto CP, Villani LG, Vimieiro CBS, Lepski GA, Monjardim M, et al. Biomimetic Design of a Tendon-Driven Myoelectric Soft Hand Exoskeleton for Upper-Limb Rehabilitation. Biomimetics. 2023; 8(3):317. https://doi.org/10.3390/biomimetics8030317
Chicago/Turabian StyleSilva, Rodrigo C., Bruno. G. Lourenço, Pedro H. F. Ulhoa, Eduardo A. F. Dias, Fransergio L. da Cunha, Cristiane P. Tonetto, Luis G. Villani, Claysson B. S. Vimieiro, Guilherme A. Lepski, Marina Monjardim, and et al. 2023. "Biomimetic Design of a Tendon-Driven Myoelectric Soft Hand Exoskeleton for Upper-Limb Rehabilitation" Biomimetics 8, no. 3: 317. https://doi.org/10.3390/biomimetics8030317
APA StyleSilva, R. C., Lourenço, B. G., Ulhoa, P. H. F., Dias, E. A. F., da Cunha, F. L., Tonetto, C. P., Villani, L. G., Vimieiro, C. B. S., Lepski, G. A., Monjardim, M., & Andrade, R. M. (2023). Biomimetic Design of a Tendon-Driven Myoelectric Soft Hand Exoskeleton for Upper-Limb Rehabilitation. Biomimetics, 8(3), 317. https://doi.org/10.3390/biomimetics8030317






