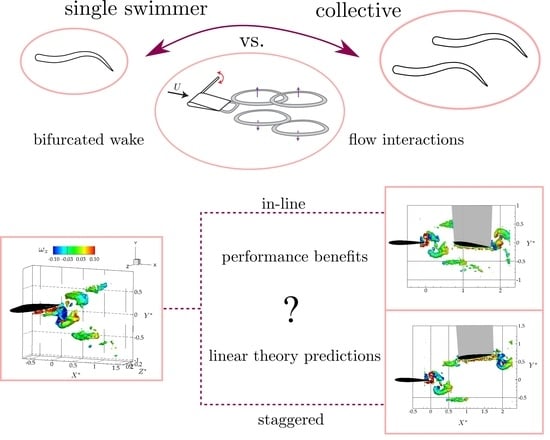
Flow Interactions Between Low Aspect Ratio Hydrofoils in In-line and Staggered Arrangements
Abstract
1. Introduction
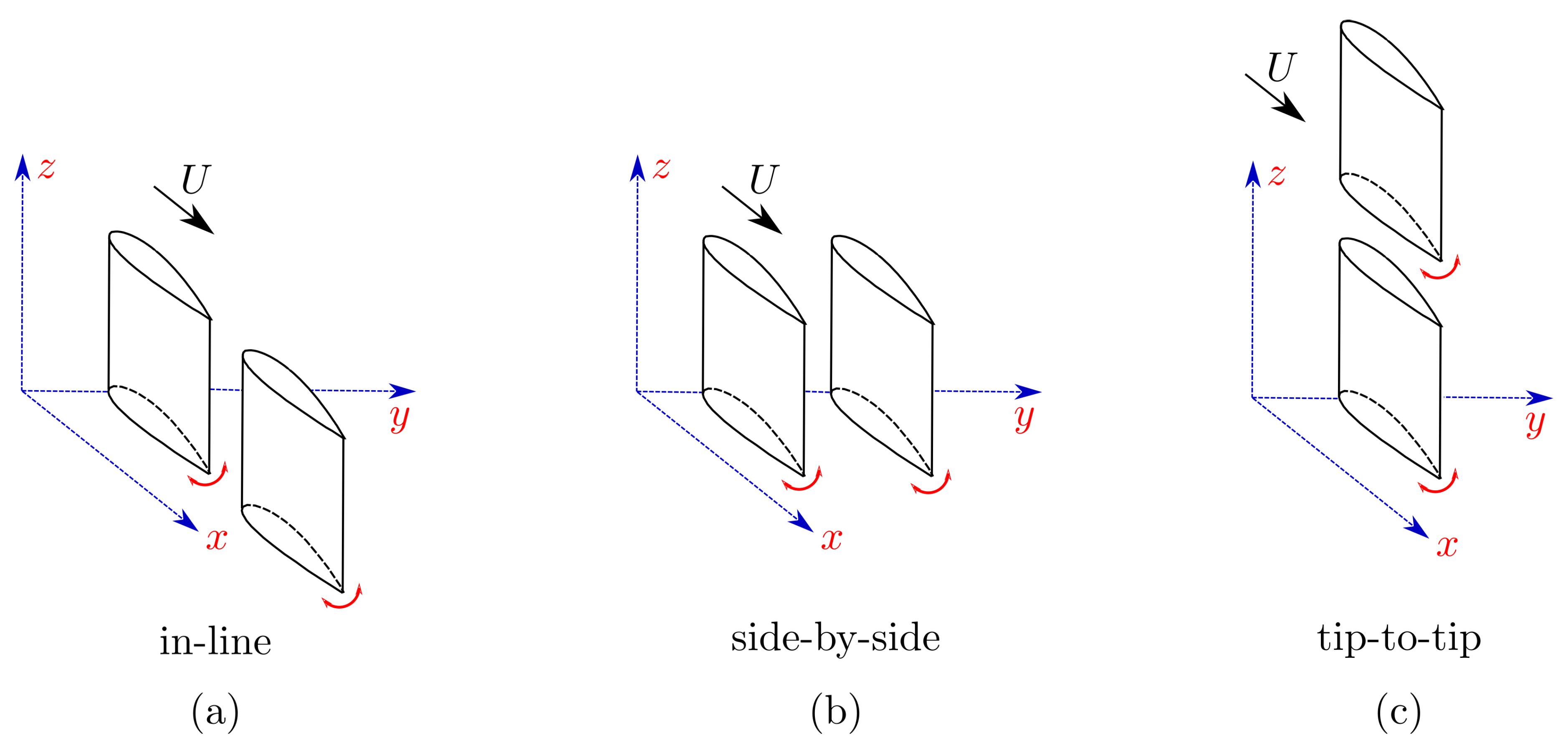
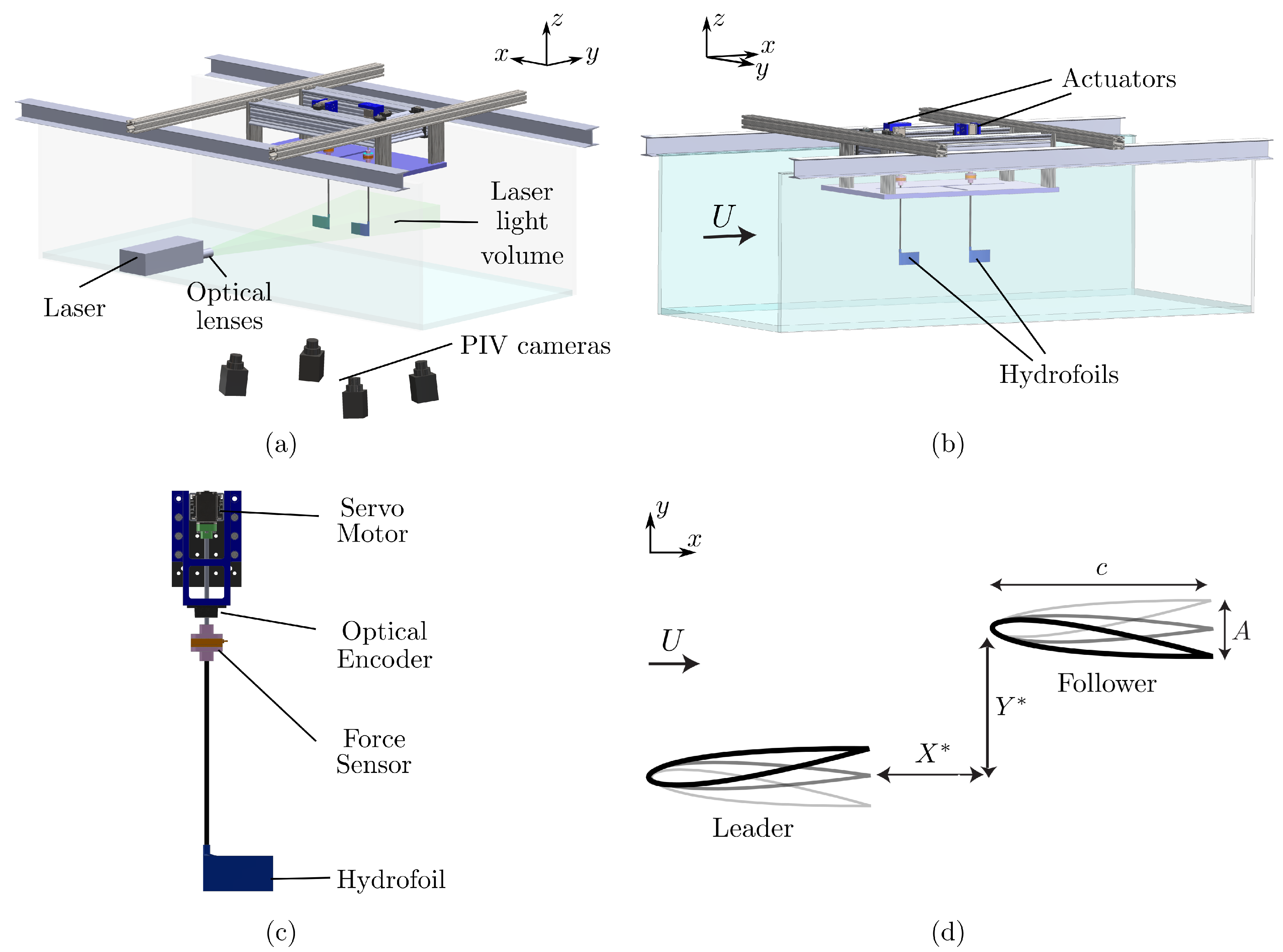
2. Experimental Setup and Methods
3. Results
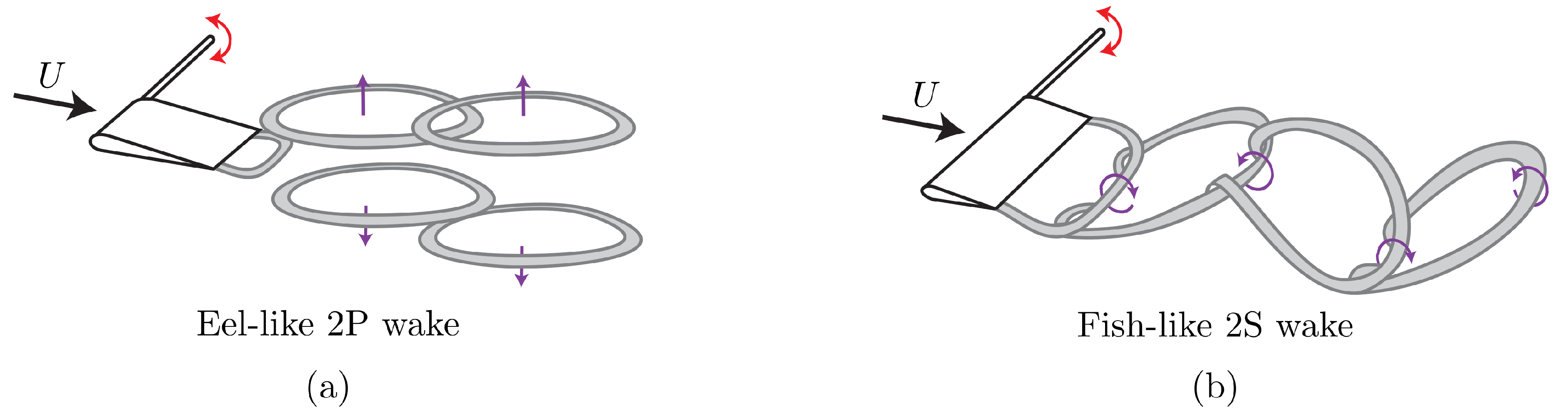
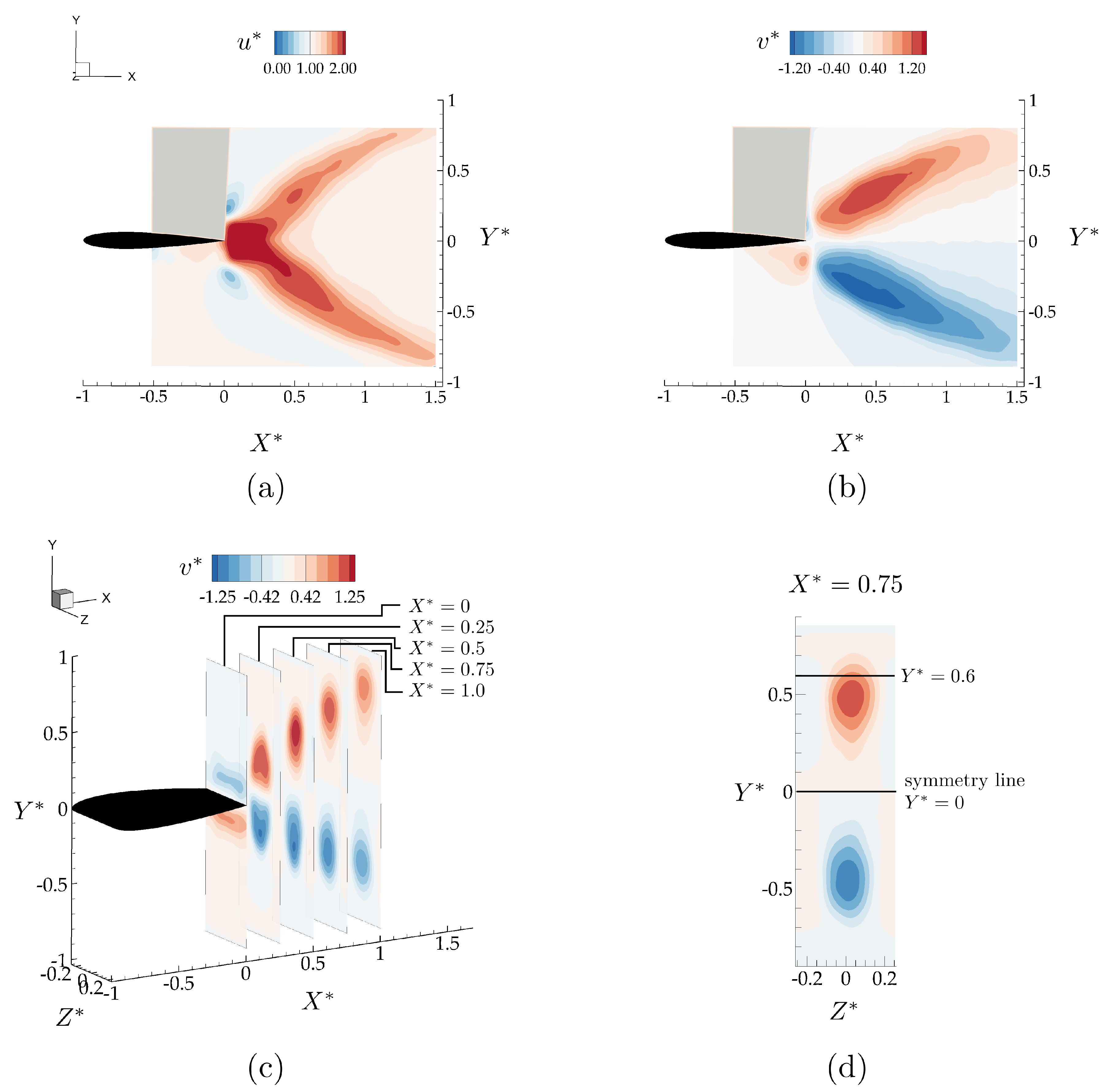
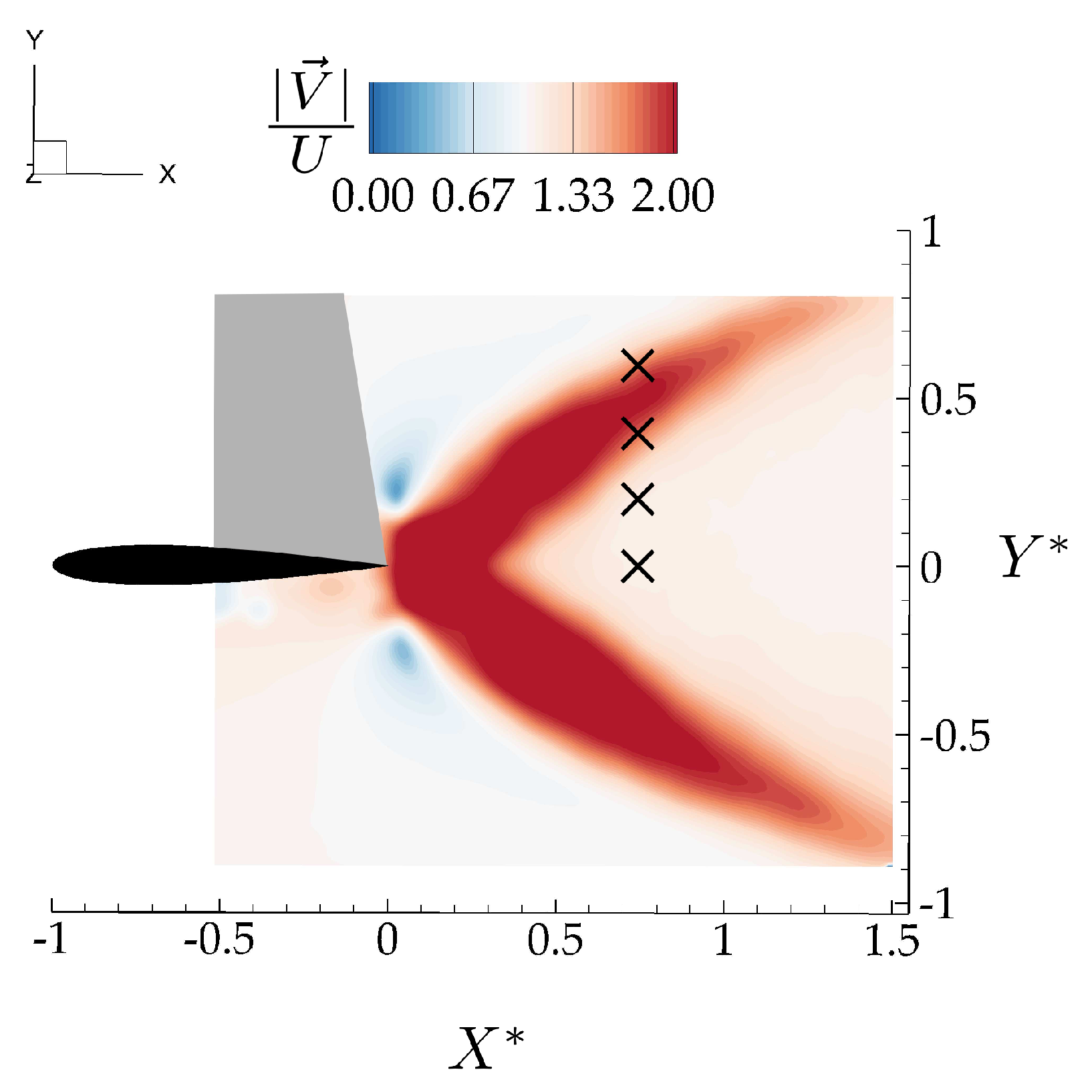
3.1. Isolated Hydrofoil Performance and Wake Measurements
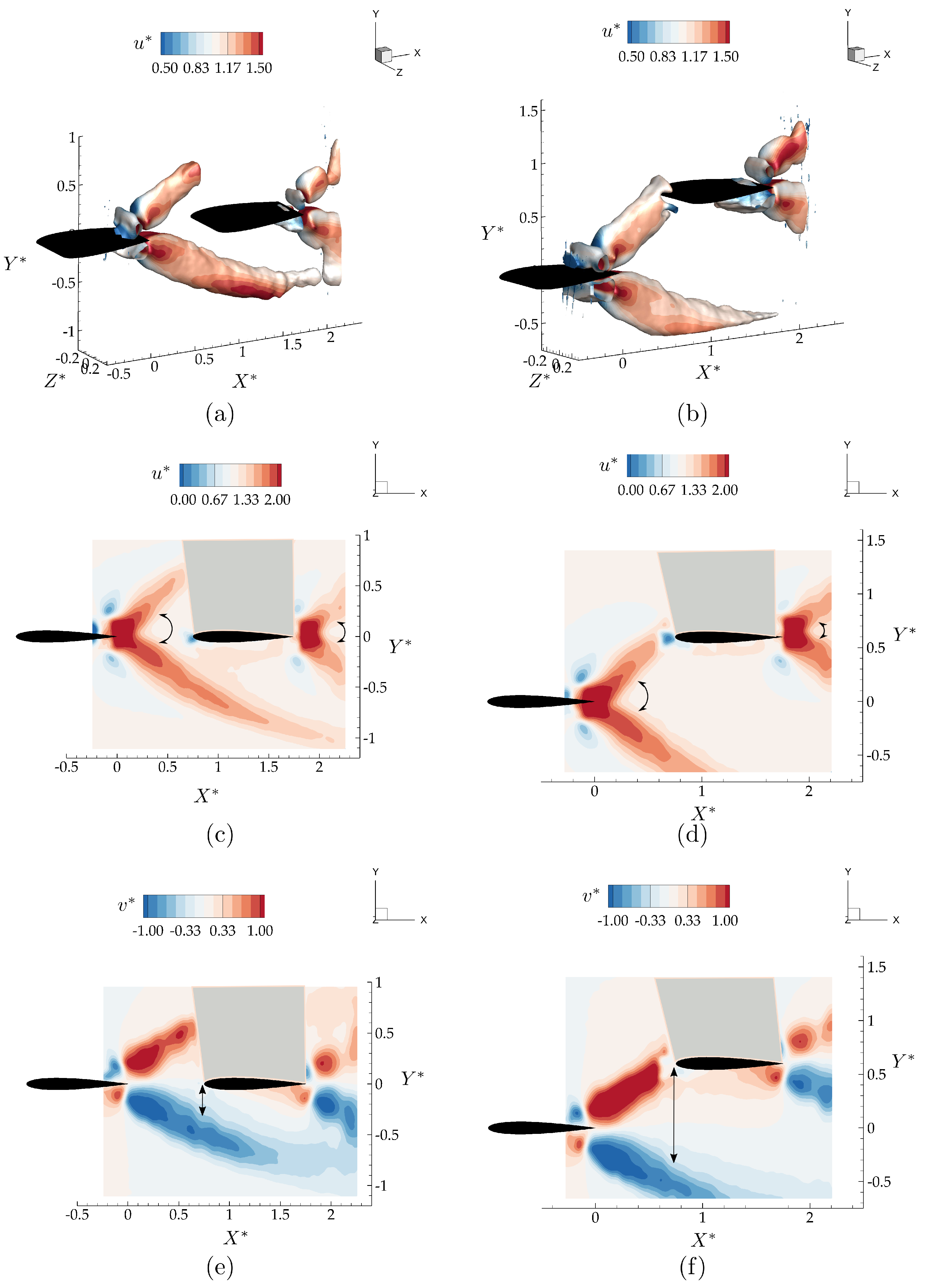
3.2. Wake Measurements of Two Interacting Hydrofoils
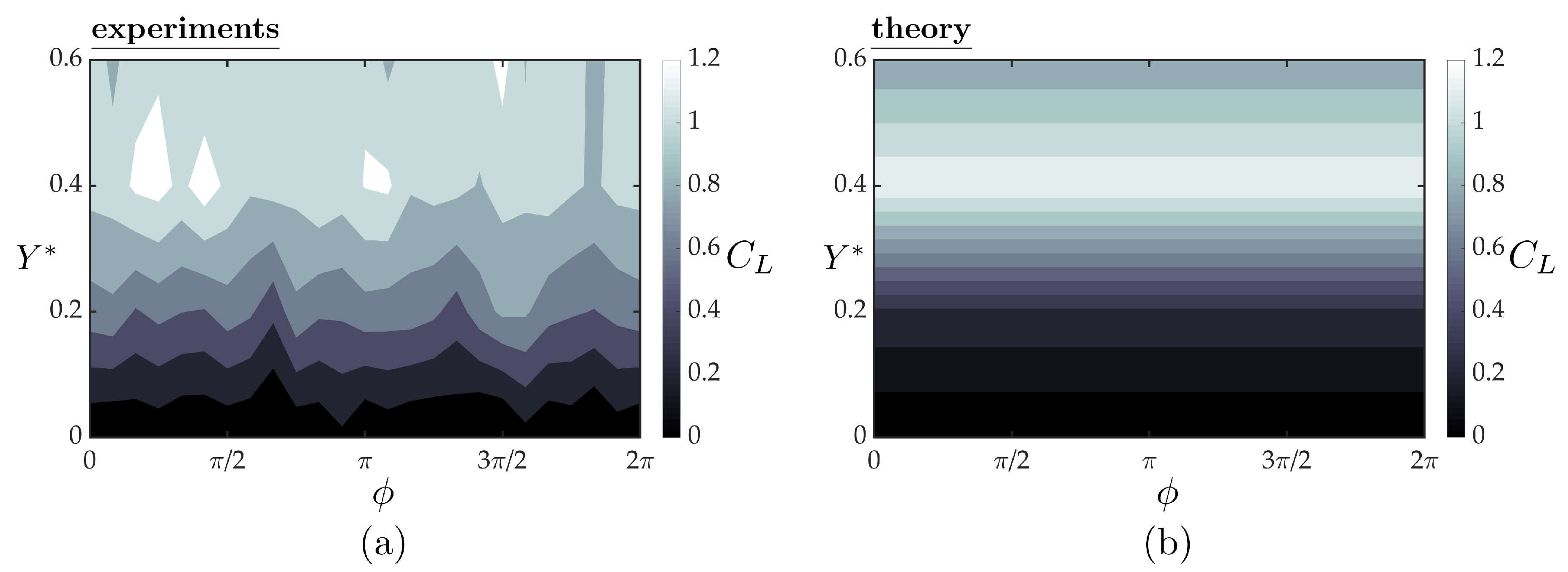
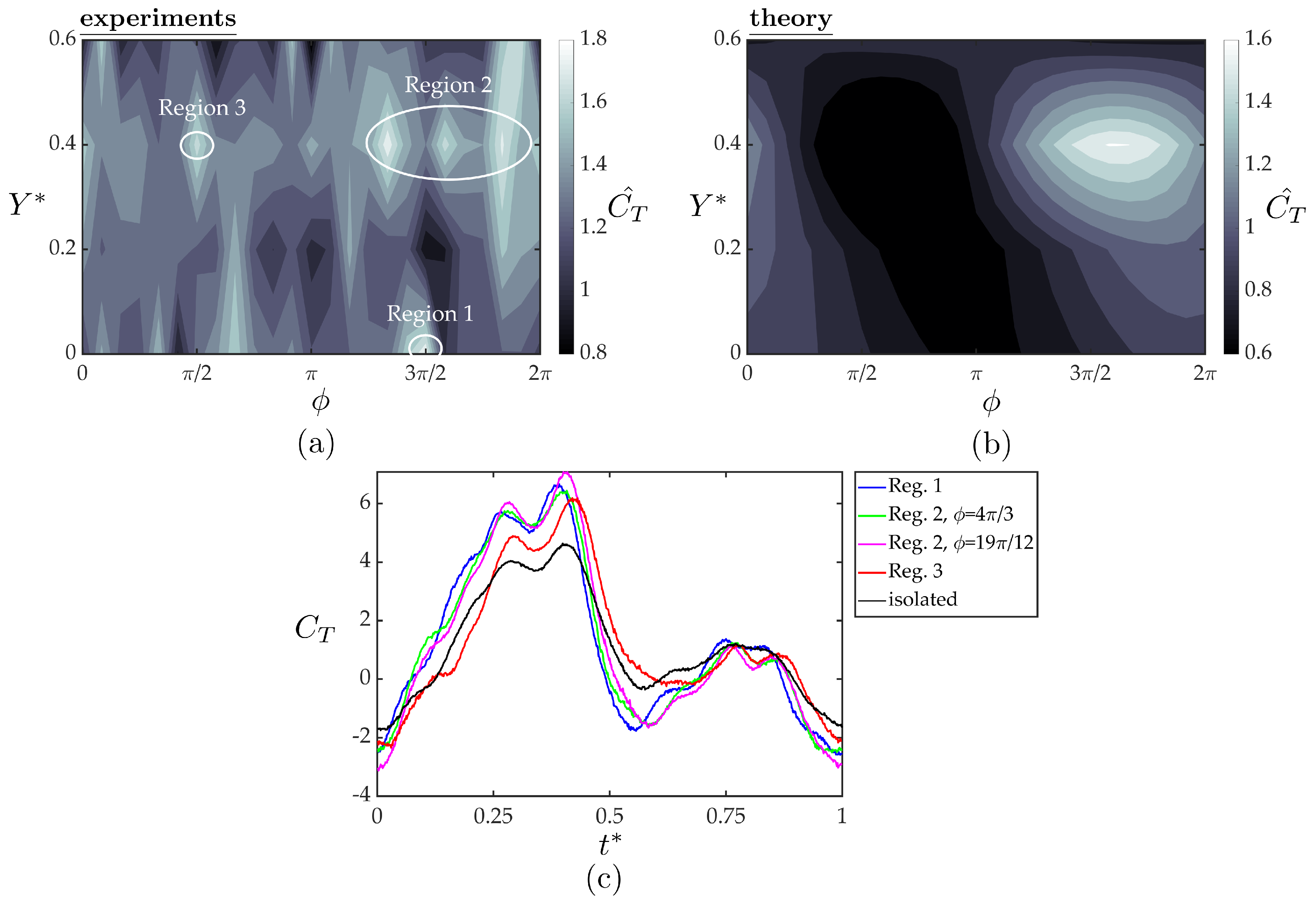
3.3. Linear Unsteady Hydrofoil Theory
3.4. Follower Performance Comparison with Theory
4. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Wynne-Edwards, V.C. Animal Dispersion: In Relation to Social Behaviour; Oliver and Boyd: London, UK, 1962. [Google Scholar]
- Tinbergen, J. Social Behaviour in Animals: With Special Reference to vertebrates; Springer Science & Business Media: Berlin, Germany, 2012. [Google Scholar]
- Weihs, D. Hydromechanics of fish schooling. Nature 1973, 241, 290–291. [Google Scholar] [CrossRef]
- Weimerskirch, H.; Martin, J.; Clerquin, Y.; Alexandre, P.; Jiraskova, S. Energy saving in flight formation. Nature 2001, 413, 697. [Google Scholar] [CrossRef]
- Drucker, E.G.; Lauder, G.V. Locomotor function of the dorsal fin in teleost fishes: Experimental analysis of wake forces in sunfish. J. Exp. Biol. 2001, 204, 2943–2958. [Google Scholar]
- Standen, E.; Lauder, G.V. Hydrodynamic function of dorsal and anal fins in brook trout (Salvelinus fontinalis). J. Exp. Biol. 2007, 210, 325–339. [Google Scholar] [CrossRef]
- Partridge, B.L.; Pitcher, T.; Cullen, J.M.; Wilson, J. The three-dimensional structure of fish schools. Behav. Ecol. Sociobiol. 1980, 6, 277–288. [Google Scholar] [CrossRef]
- Portugal, S.J.; Hubel, T.Y.; Fritz, J.; Heese, S.; Trobe, D.; Voelkl, B.; Hailes, S.; Wilson, A.M.; Usherwood, J.R. Upwash exploitation and downwash avoidance by flap phasing in ibis formation flight. Nature 2014, 505, 399–402. [Google Scholar] [CrossRef]
- Ashraf, I.; Bradshaw, H.; Ha, T.T.; Halloy, J.; Godoy-Diana, R.; Thiria, B. Simple phalanx pattern leads to energy saving in cohesive fish schooling. Proc. Natl. Acad. Sci. USA 2017, 114, 9599–9604. [Google Scholar] [CrossRef] [PubMed]
- Akhtar, I.; Mittal, R.; Lauder, G.V.; Drucker, E. Hydrodynamics of a biologically inspired tandem flapping foil configuration. Theor. Comput. Fluid Dyn. 2007, 21, 155–170. [Google Scholar] [CrossRef]
- Rival, D.; Hass, G.; Tropea, C. Recovery of energy from leading-and trailing-edge vortices in tandem-airfoil configurations. J. Aircr. 2011, 48, 203–211. [Google Scholar] [CrossRef]
- Boschitsch, B.M.; Dewey, P.A.; Smits, A.J. Propulsive performance of unsteady tandem hydrofoils in an in-line configuration. Phys. Fluids 2014, 26, 051901. [Google Scholar] [CrossRef]
- Broering, T.M.; Lian, Y.; Henshaw, W. Numerical investigation of energy extraction in a tandem flapping wing configuration. AIAA J. 2012, 50, 2295–2307. [Google Scholar] [CrossRef]
- Broering, T.M.; Lian, Y.S. The effect of phase angle and wing spacing on tandem flapping wings. Acta Mech. Sin. 2012, 28, 1557–1571. [Google Scholar] [CrossRef]
- Liu, G.; Ren, Y.; Dong, H.; Akanyeti, O.; Liao, J.C.; Lauder, G.V. Computational analysis of vortex dynamics and performance enhancement due to body–fin and fin–fin interactions in fish-like locomotion. J. Fluid Mech. 2017, 829, 65–88. [Google Scholar] [CrossRef]
- Gong, W.Q.; Jia, B.B.; Xi, G. Experimental study on mean thrust of two plunging wings in Tandem. AIAA J. 2015. [Google Scholar] [CrossRef]
- Gong, W.Q.; Jia, B.B.; Xi, G. Experimental study on instantaneous thrust and lift of two plunging wings in tandem. Exp. Fluids 2016, 57, 8. [Google Scholar] [CrossRef]
- Muscutt, L.; Weymouth, G.; Ganapathisubramani, B. Performance augmentation mechanism of in-line tandem flapping foils. J. Fluid Mech. 2017, 827, 484–505. [Google Scholar] [CrossRef]
- Kurt, M.; Moored, K.W. Flow interactions of two-and three-dimensional networked bio-inspired control elements in an in-line arrangement. Bioinspiration Biomimetics 2018, 13, 045002. [Google Scholar] [CrossRef]
- Dewey, P.A.; Quinn, D.B.; Boschitsch, B.M.; Smits, A.J. Propulsive performance of unsteady tandem hydrofoils in a side-by-side configuration. Phys. Fluids 2014, 26, 041903. [Google Scholar] [CrossRef]
- Dong, G.J.; Lu, X.Y. Characteristics of flow over traveling wavy foils in a side-by-side arrangement. Phys. Fluids 2007, 19, 057107. [Google Scholar] [CrossRef]
- Kurt, M.; Moored, K.W. Unsteady Performance of Finite-Span Pitching Propulsors in Side-by-Side Arrangements. In Proceedings of the 2018 Fluid Dynamics Conference, Atlanta, GA, USA, 25–29 June 2018; p. 3732. [Google Scholar]
- Godoy-Diana, R.; Vacher, J.; Raspa, V.; Thiria, B. On the Fluid Dynamical Effects of Synchronization in Side-by-Side Swimmers. Biomimetics 2019, 4, 77. [Google Scholar] [CrossRef]
- Shoele, K.; Zhu, Q. Performance of synchronized fins in biomimetic propulsion. Bioinspiration Biomimetics 2015, 10, 026008. [Google Scholar] [CrossRef] [PubMed]
- Daghooghi, M.; Borazjani, I. The hydrodynamic advantages of synchronized swimming in a rectangular pattern. Bioinspiration Biomimetics 2015, 10, 056018. [Google Scholar] [CrossRef] [PubMed]
- Maertens, A.P.; Gao, A.; Triantafyllou, M.S. Optimal undulatory swimming for a single fish-like body and for a pair of interacting swimmers. J. Fluid Mech. 2017, 813, 301–345. [Google Scholar] [CrossRef]
- Novati, G.; Verma, S.; Alexeev, D.; Rossinelli, D.; van Rees, W.M.; Koumoutsakos, P. Synchronisation through learning for two self-propelled swimmers. Bioinspiration Biomimetics 2017, 12, 036001. [Google Scholar] [CrossRef] [PubMed]
- Filella, A.; Nadal, F.; Sire, C.; Kanso, E.; Eloy, C. Model of collective fish behavior with hydrodynamic interactions. Phys. Rev. Lett. 2018, 120, 198101. [Google Scholar] [CrossRef] [PubMed]
- Lighthill, M. Large-amplitude elongated-body theory of fish locomotion. Proc. R. Soc. Lond. B Biol. Sci. 1971, 179, 125–138. [Google Scholar]
- Gopalkrishnan, R.; Triantafyllou, M.; Triantafyllou, G.; Barrett, D. Active vorticity control in a shear flow using a flapping foil. J. Fluid Mech. 1994, 274, 1–21. [Google Scholar] [CrossRef]
- Warkentin, J.; DeLaurier, J. Experimental aerodynamic study of tandem flapping membrane wings. J. Aircr. 2007, 44, 1653–1661. [Google Scholar] [CrossRef]
- Verma, S.; Novati, G.; Koumoutsakos, P. Efficient collective swimming by harnessing vortices through deep reinforcement learning. Proc. Natl. Acad. Sci. USA 2018, 115, 5849–5854. [Google Scholar] [CrossRef]
- Liao, J.C.; Beal, D.N.; Lauder, G.V.; Triantafyllou, M.S. The Kármán gait: Novel body kinematics of rainbow trout swimming in a vortex street. J. Exp. Biol. 2003, 206, 1059–1073. [Google Scholar] [CrossRef]
- Lauder, G.V.; Tytell, E.D. Hydrodynamics of undulatory propulsion. Fish Physiol. 2005, 23, 425–468. [Google Scholar]
- Tytell, E.D.; Lauder, G.V. The hydrodynamics of eel swimming: I. Wake structure. J. Exp. Biol. 2004, 207, 1825–1841. [Google Scholar] [CrossRef] [PubMed]
- Buchholz, J.H.; Smits, A.J. The wake structure and thrust performance of a rigid low-aspect-ratio pitching panel. J. Fluid Mech. 2008, 603, 331–365. [Google Scholar] [CrossRef] [PubMed]
- Dong, H.; Mittal, R.; Najjar, F. Wake topology and hydrodynamic performance of low-aspect-ratio flapping foils. J. Fluid Mech. 2006, 566, 309–343. [Google Scholar] [CrossRef]
- Von Ellenrieder, K.D.; Parker, K.; Soria, J. Flow structures behind a heaving and pitching finite-span wing. J. Fluid Mech. 2003, 490, 129–138. [Google Scholar] [CrossRef]
- Senturk, U.; Smits, A.J. Numerical simulations of the flow around a square pitching panel. J. Fluids Struct. 2018, 76, 454–468. [Google Scholar] [CrossRef]
- Jeong, J.; Hussain, F. On the identification of a vortex. J. Fluid Mech. 1995, 285, 69–94. [Google Scholar] [CrossRef]
- Wagner, H. Über die Entstehung des dynamischen Auftriebes von Tragflügeln. J. Appl. Math. Mech. Für Angew. Math. Und Mech. 1925, 5, 17–35. [Google Scholar] [CrossRef]
- von Kármán, T.; Sears, W.R. Airfoil theory for non-uniform motion. J. Aeronaut. Sci. 1938, 5, 379–390. [Google Scholar] [CrossRef]
- Theodorsen, T. General Theory of Aerodynamic Instability and the Mechanism of Flutter; Technical report; Ames Research Center, NASA: Mountain View, CA, USA, 1935.
- Garrick, I.E. Propulsion of a Flapping and Oscillating Airfoil; Technical Report; Langley Memorial Aeronautical Laboratory: Langley Field, VA, USA, 1937. [Google Scholar]
- Bisplinghoff, R.L.; Ashley, H.; Halfman, R.L. Aeroelasticity; Courier Corporation, Dover Publications: Minoela, NY, USA, 2013. [Google Scholar]
- Brennen, C.E. A Review of Added Mass and Fluid Inertial Forces; Technical Report; Naval Civil Engineering Laboratory: Sierra Madre, CA, USA, 1982. [Google Scholar]
- Ayancik, F.; Zhong, Q.; Quinn, D.B.; Brandes, A.; Bart-Smith, H.; Moored, K.W. Scaling laws for the propulsive performance of three-dimensional pitching propulsors. J. Fluid Mech. 2019, 871, 1117–1138. [Google Scholar] [CrossRef]
- Helmbold, H.B. Der unverwundene ellipsenflugel als tragende flanche. Jahrbuch 1942, I111–I113. [Google Scholar]
- Anderson, J.D. Aircraft Performance and Design; WCB/McGraw-Hill: Boston, MA, USA, 1999. [Google Scholar]
- Taira, K.; Colonius, T. Three-dimensional flows around low-aspect-ratio flat-plate wings at low Reynolds numbers. J. Fluid Mech. 2009, 623, 187–207. [Google Scholar] [CrossRef]



| 0–0.6 | increments | |
| 0–2 | increments | |
| k | ||
| 7.5° | () | |
| f | 1.5 |
| Coefficients | |
|---|---|
© 2020 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kurt, M.; Eslam Panah, A.; Moored, K.W. Flow Interactions Between Low Aspect Ratio Hydrofoils in In-line and Staggered Arrangements. Biomimetics 2020, 5, 13. https://doi.org/10.3390/biomimetics5020013
Kurt M, Eslam Panah A, Moored KW. Flow Interactions Between Low Aspect Ratio Hydrofoils in In-line and Staggered Arrangements. Biomimetics. 2020; 5(2):13. https://doi.org/10.3390/biomimetics5020013
Chicago/Turabian StyleKurt, Melike, Azar Eslam Panah, and Keith W. Moored. 2020. "Flow Interactions Between Low Aspect Ratio Hydrofoils in In-line and Staggered Arrangements" Biomimetics 5, no. 2: 13. https://doi.org/10.3390/biomimetics5020013
APA StyleKurt, M., Eslam Panah, A., & Moored, K. W. (2020). Flow Interactions Between Low Aspect Ratio Hydrofoils in In-line and Staggered Arrangements. Biomimetics, 5(2), 13. https://doi.org/10.3390/biomimetics5020013