Corrugation at the Trailing Edge Enhances the Aerodynamic Performance of a Three-Dimensional Wing During Gliding Flight
Abstract
1. Introduction
2. Materials and Methods
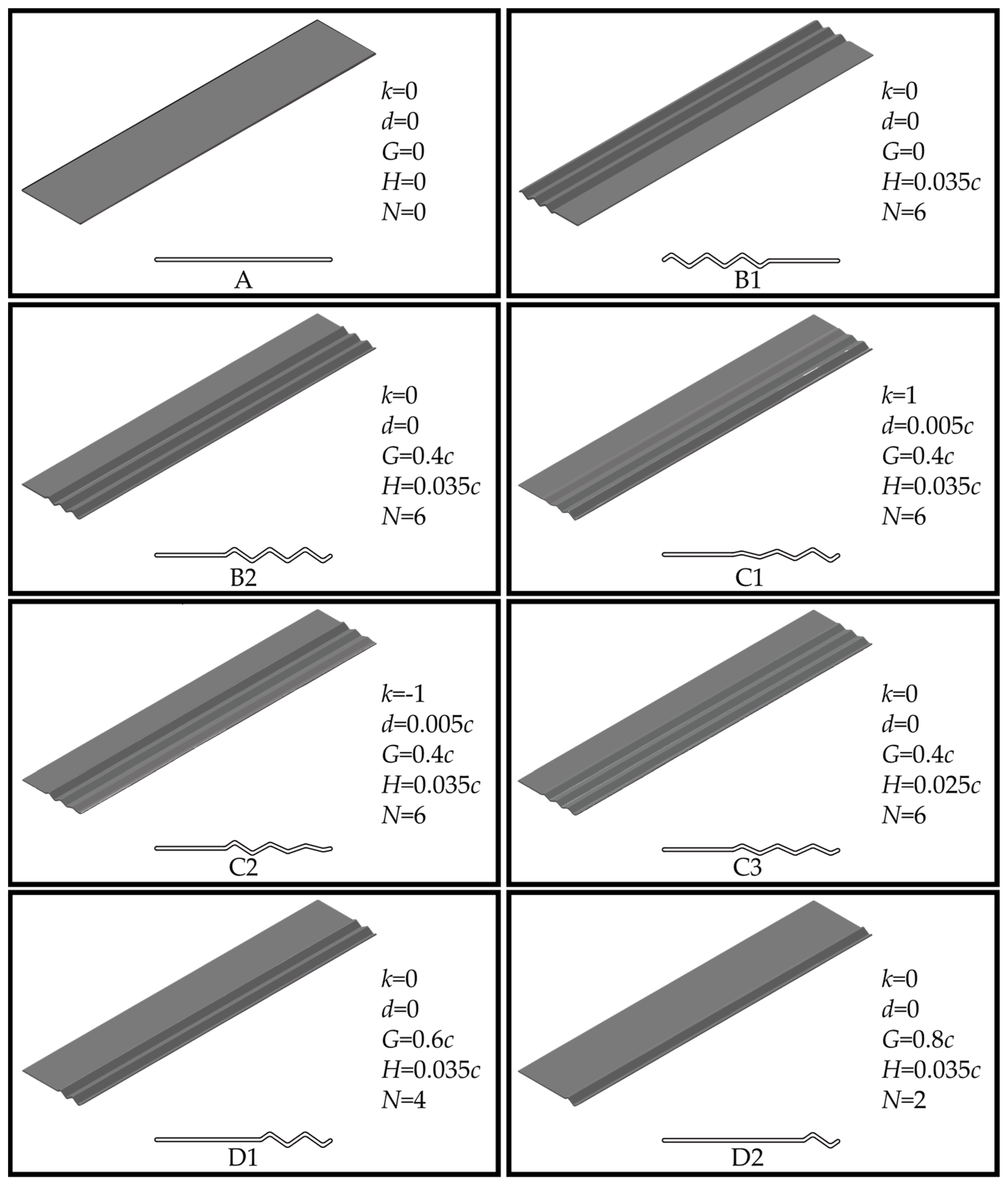
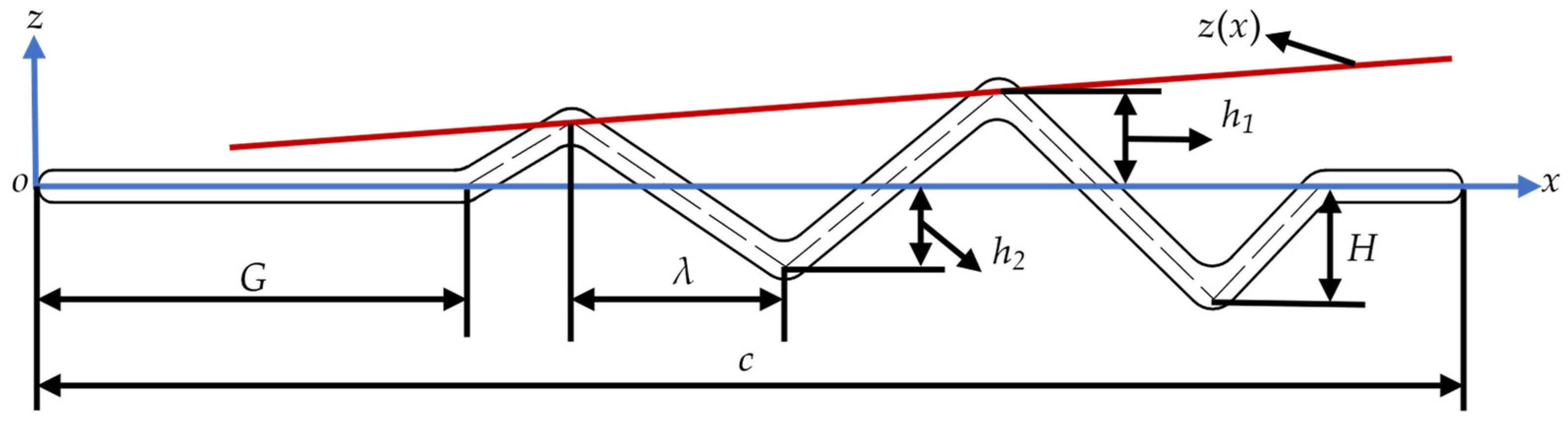
2.1. Wing Model Design
2.2. The Flow Equations and Solution Method
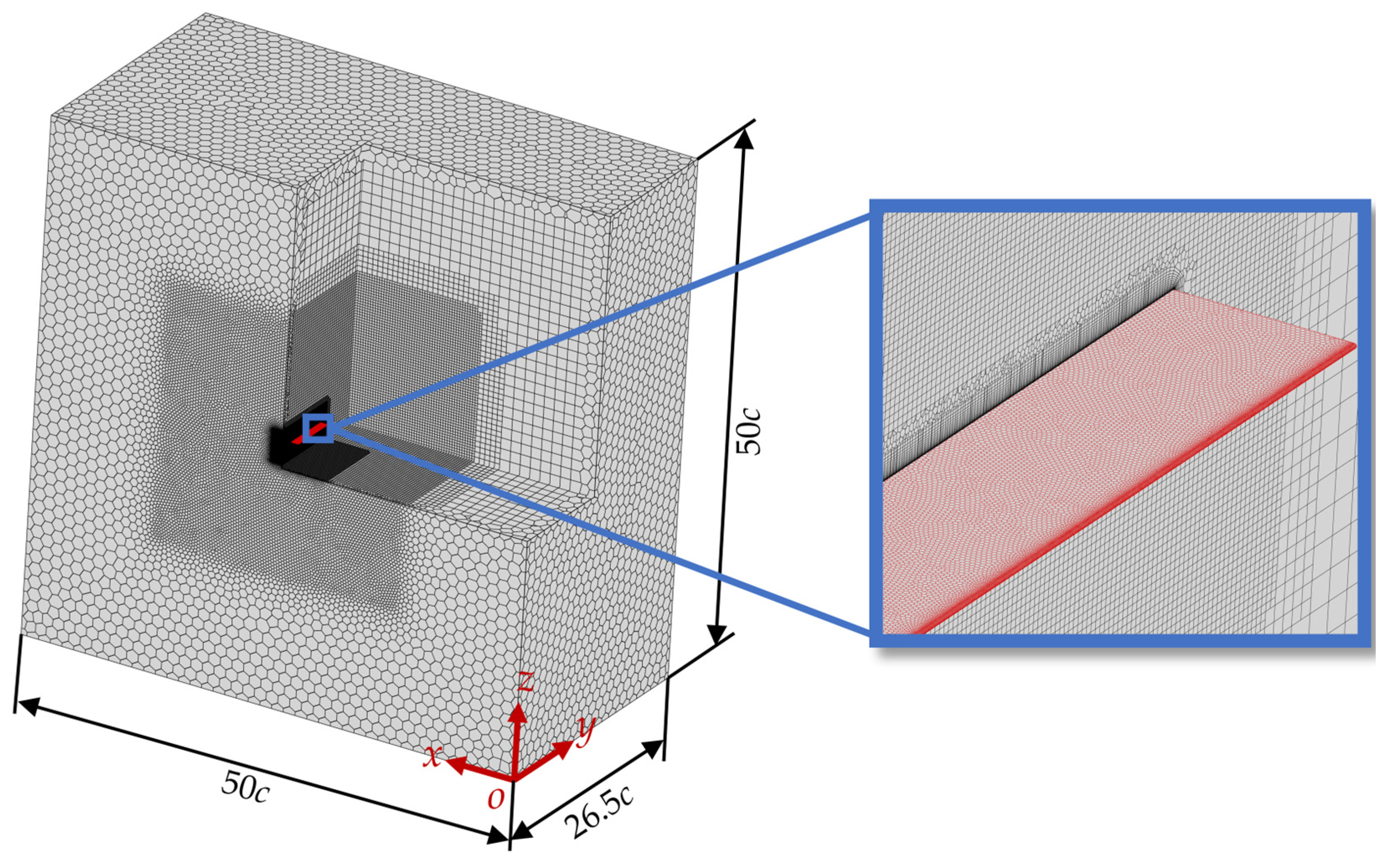
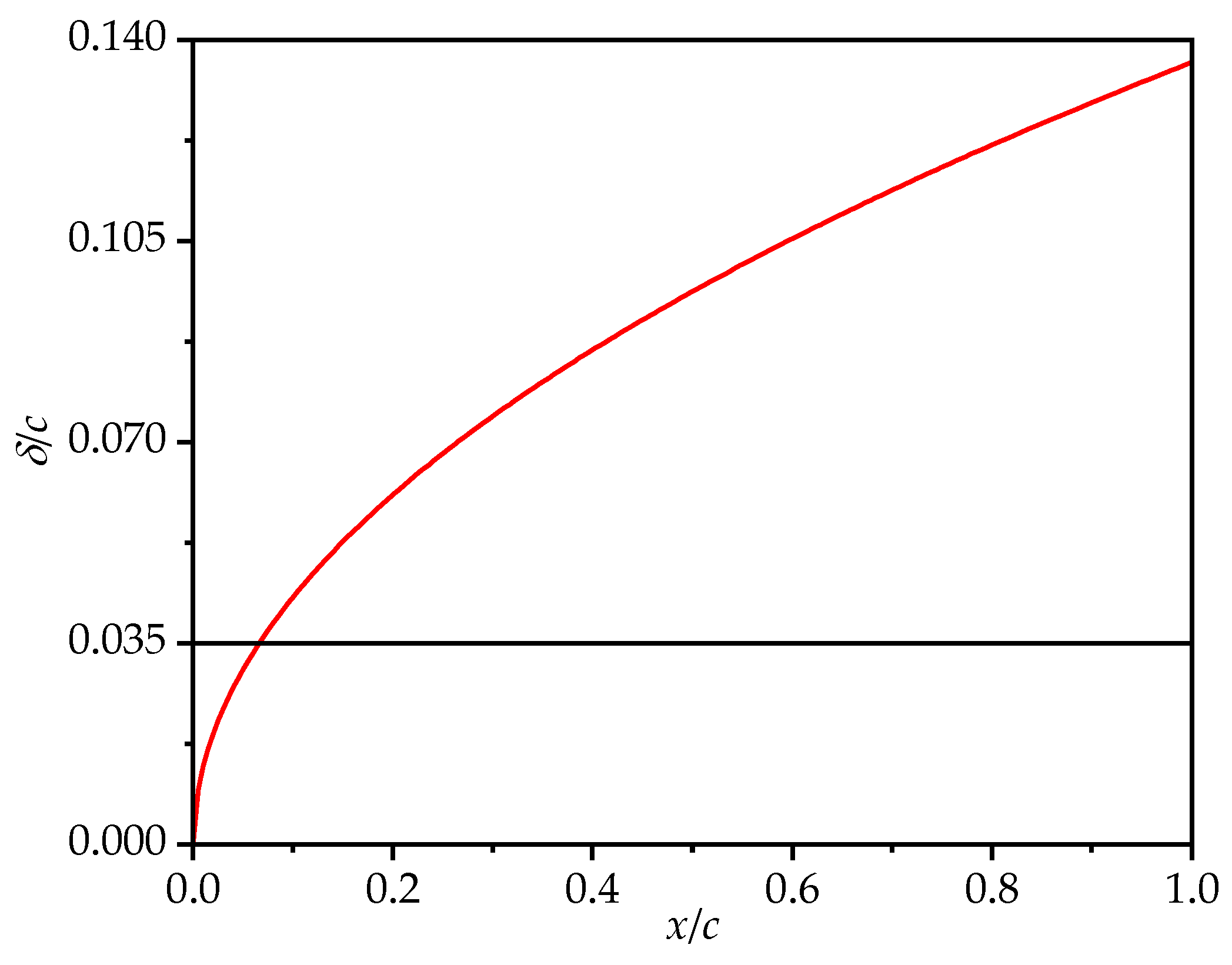
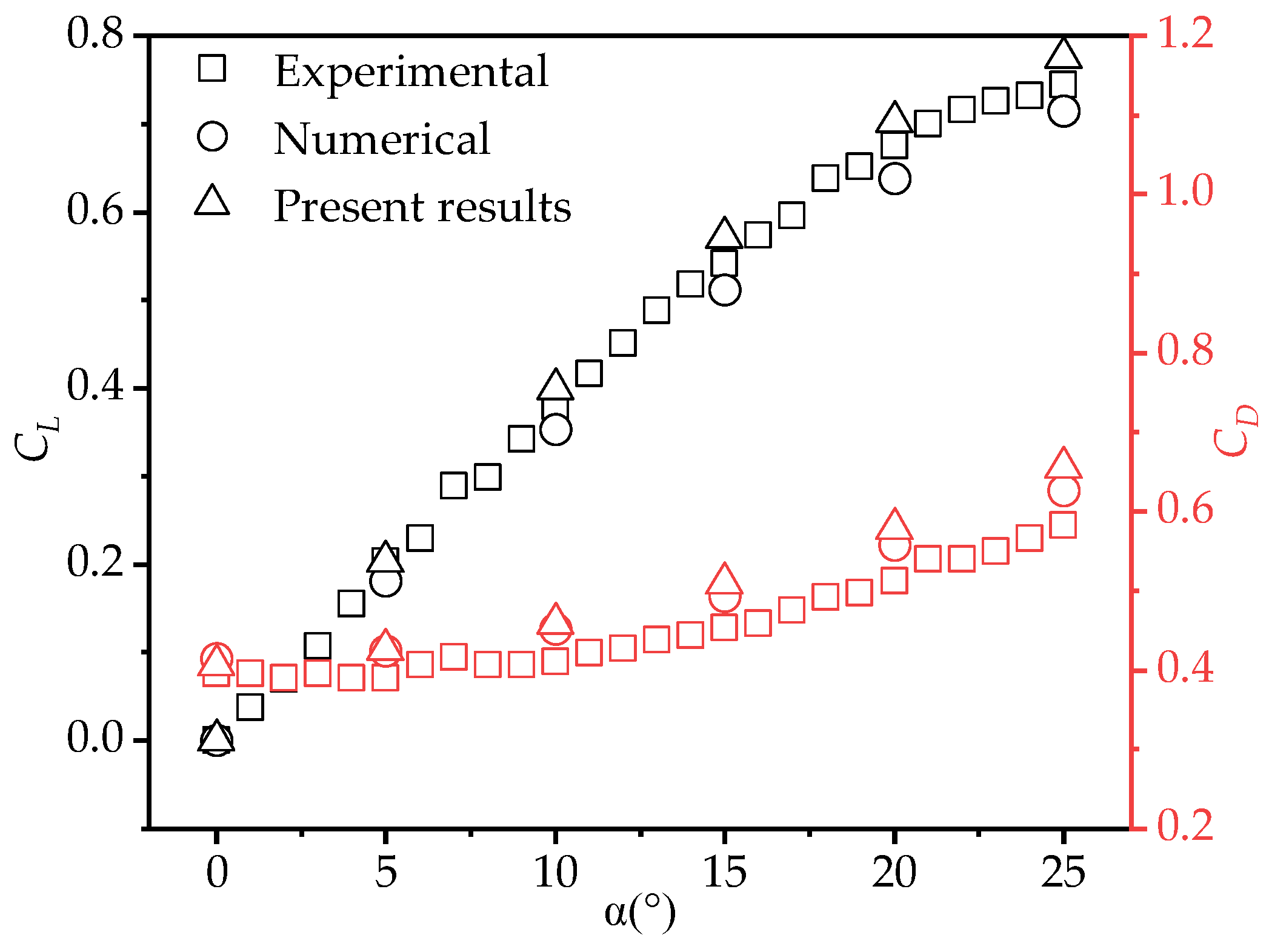
2.3. Verification and Validation
3. Results and Discussion
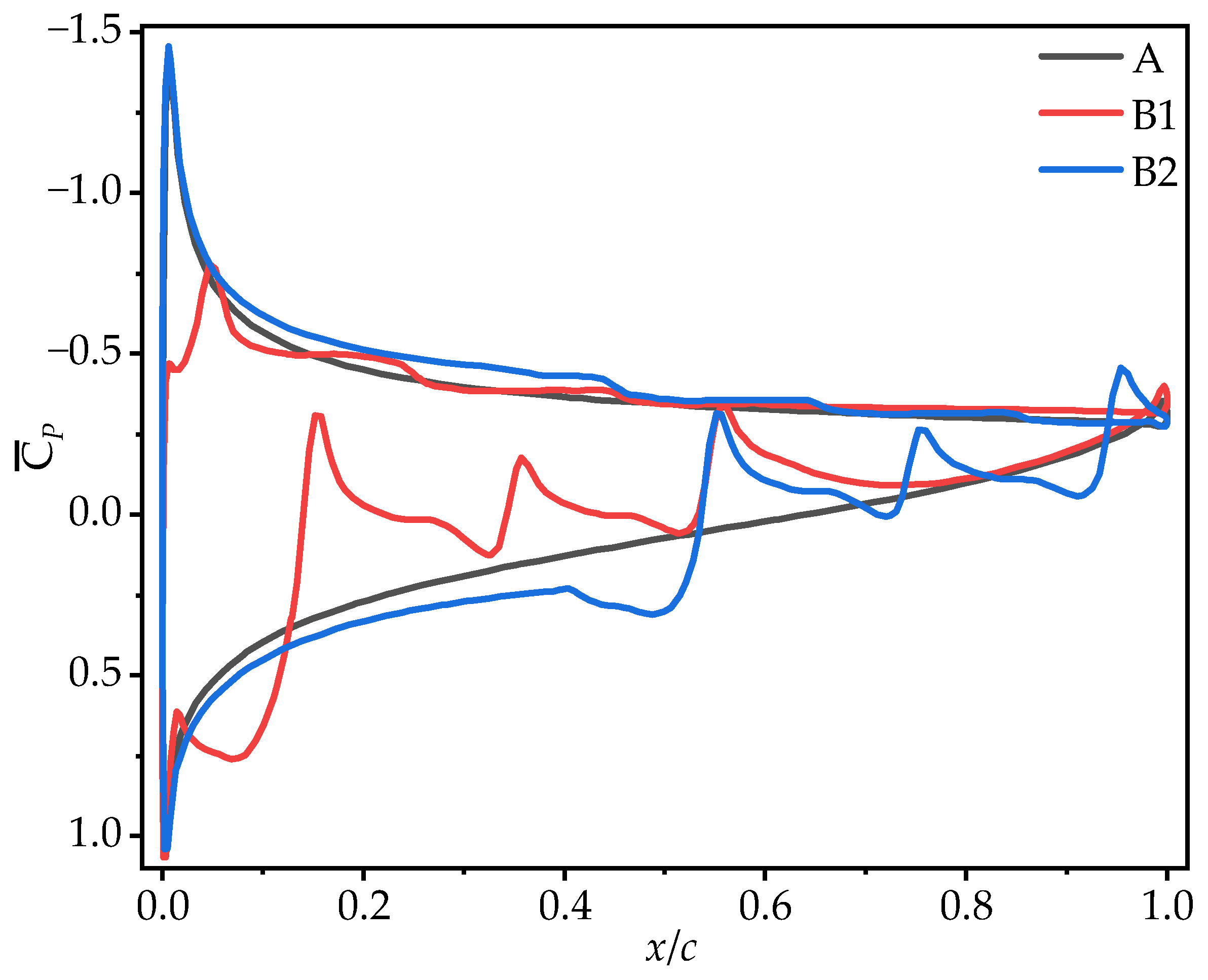
3.1. Effect of Chordwise Corrugation Position
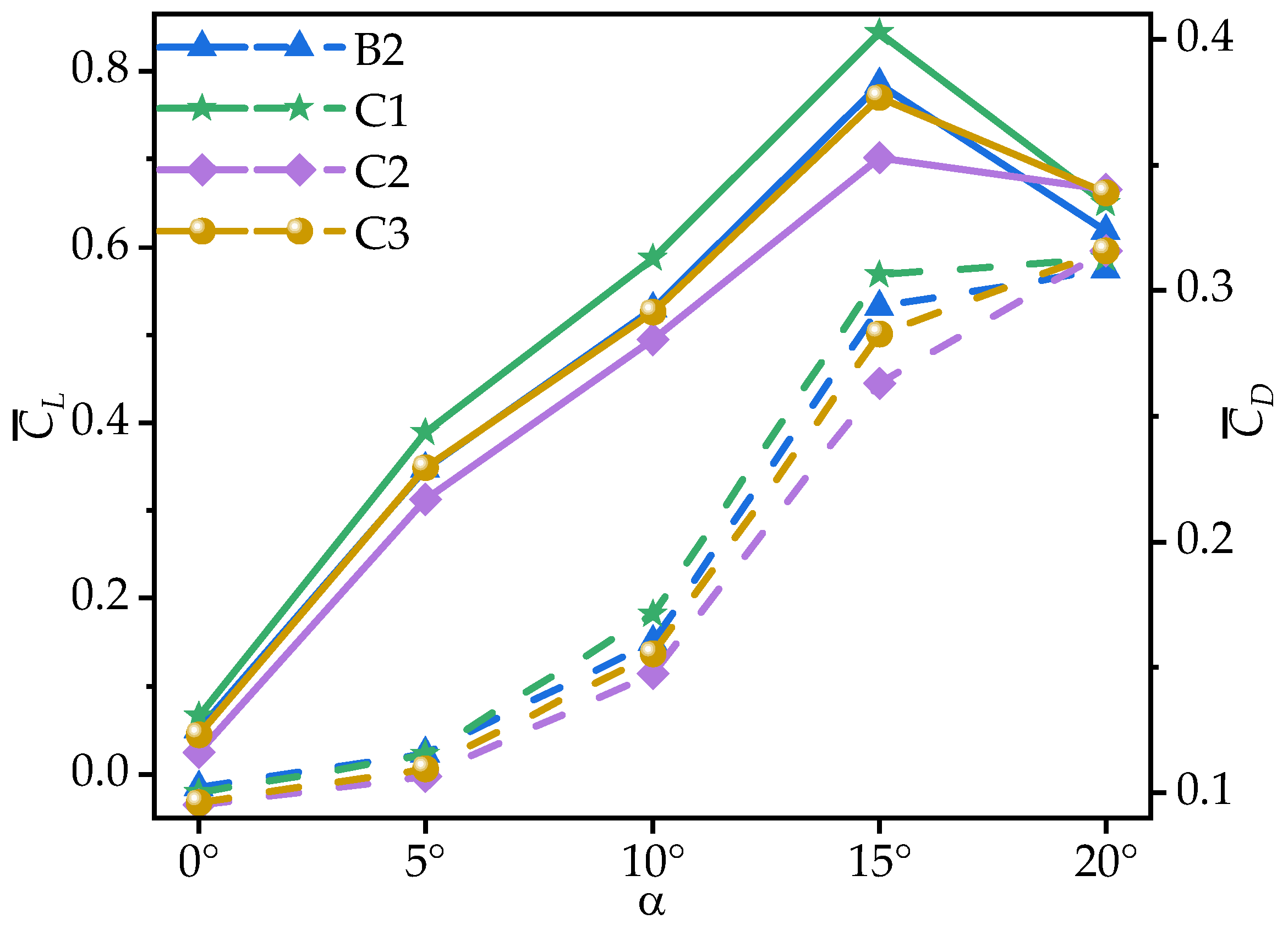
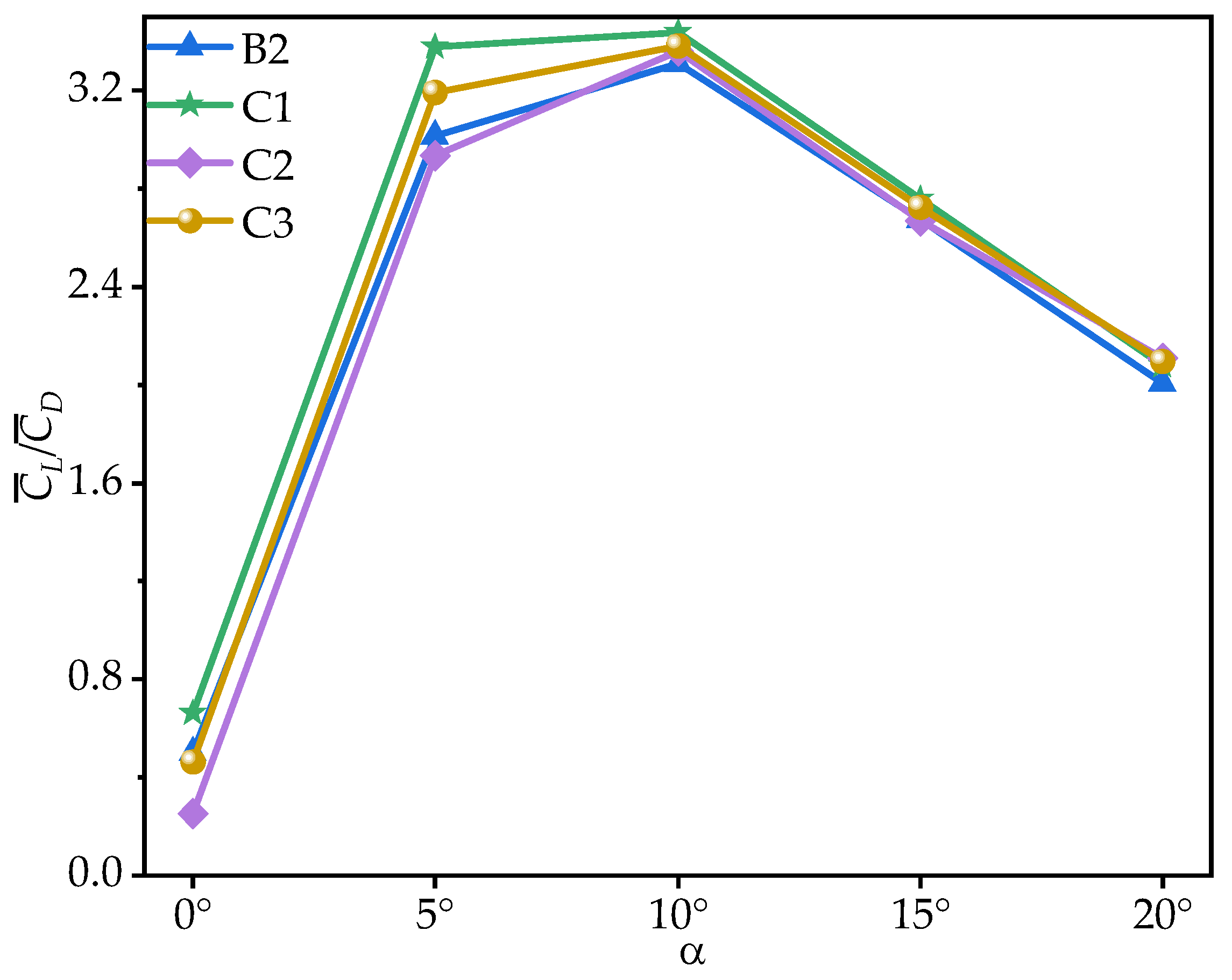
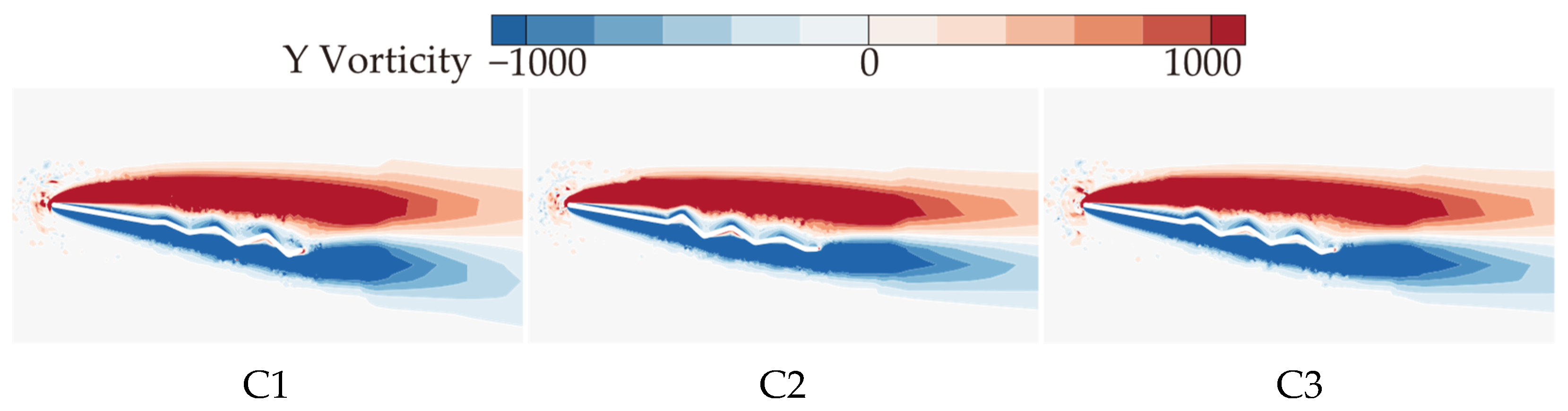
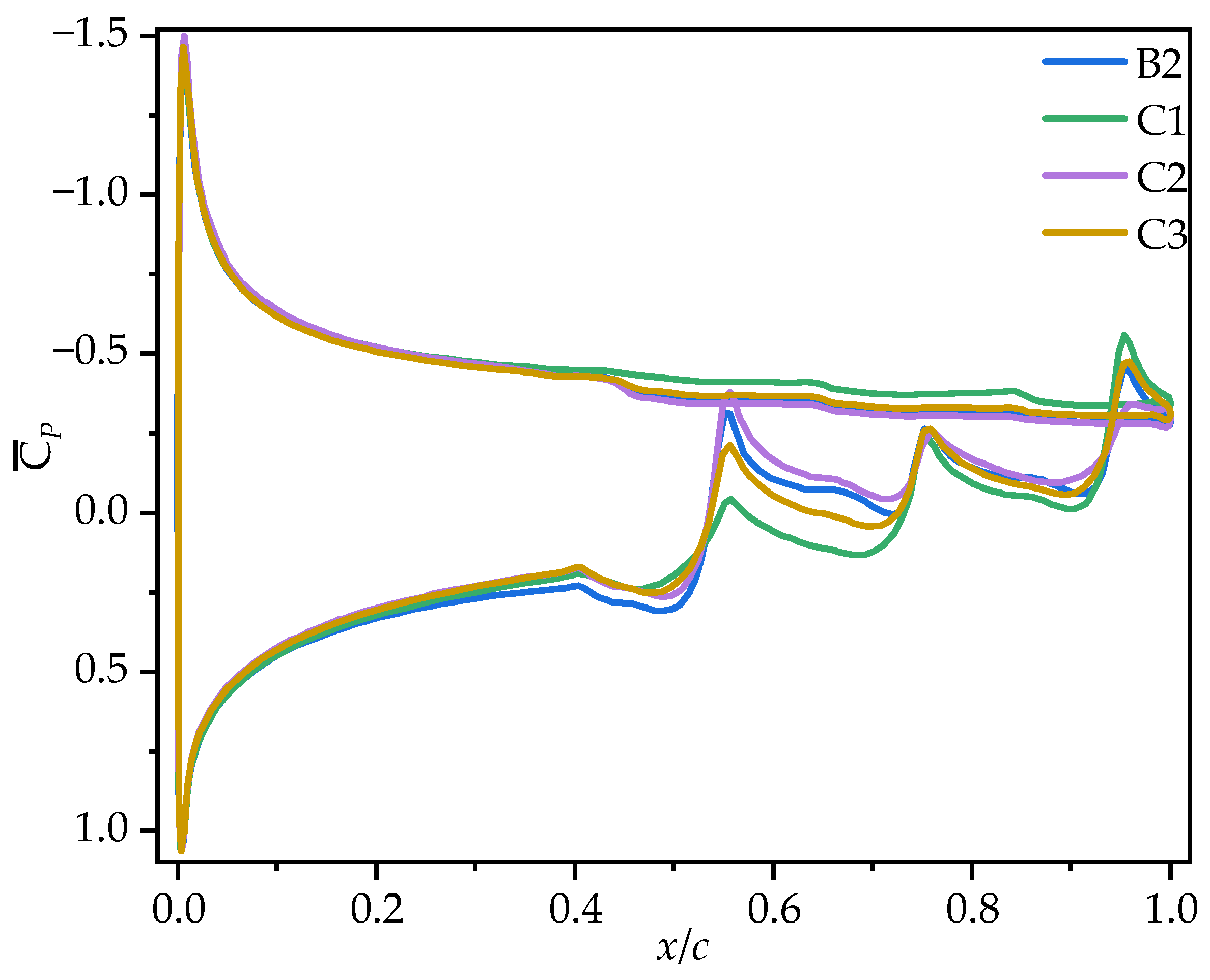
3.2. Effect of Linear Variation in Corrugation Amplitude
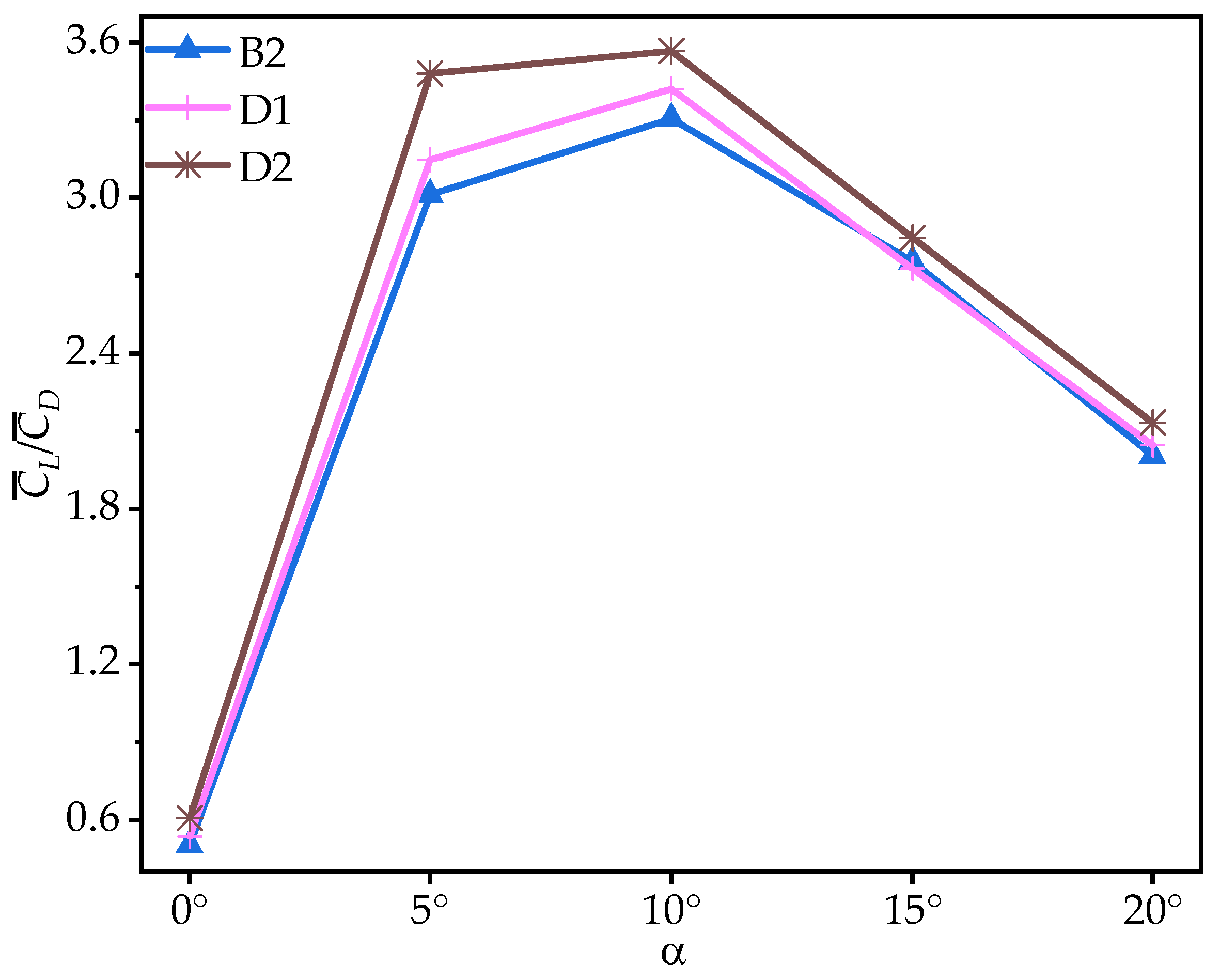
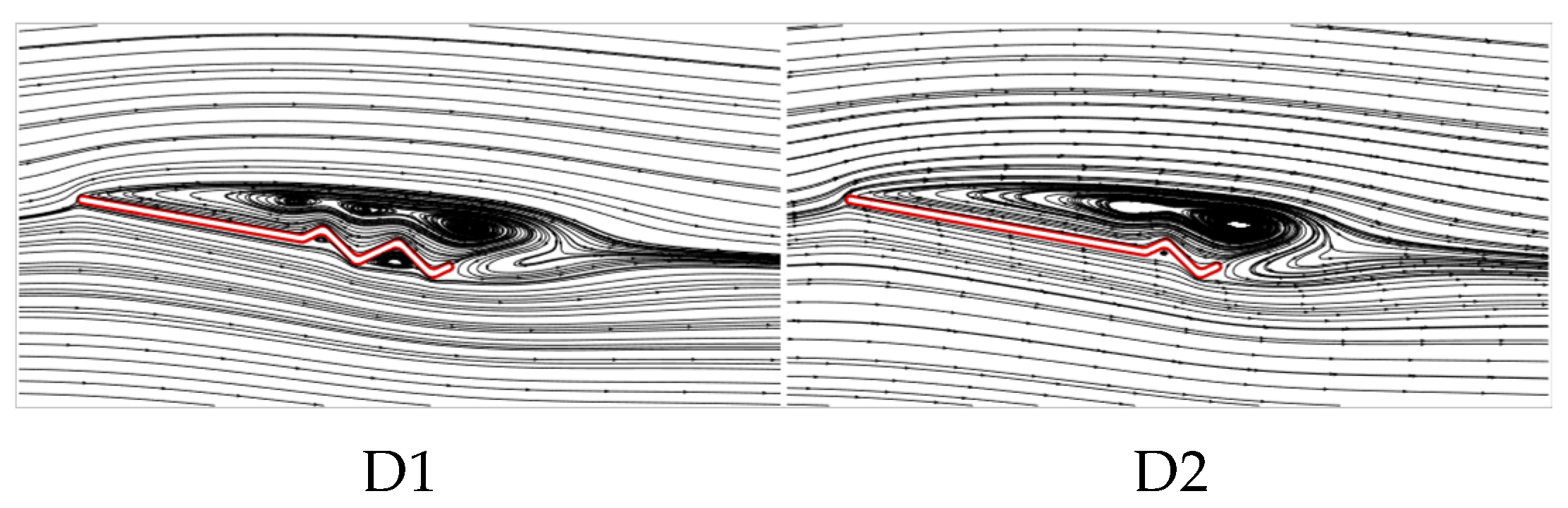
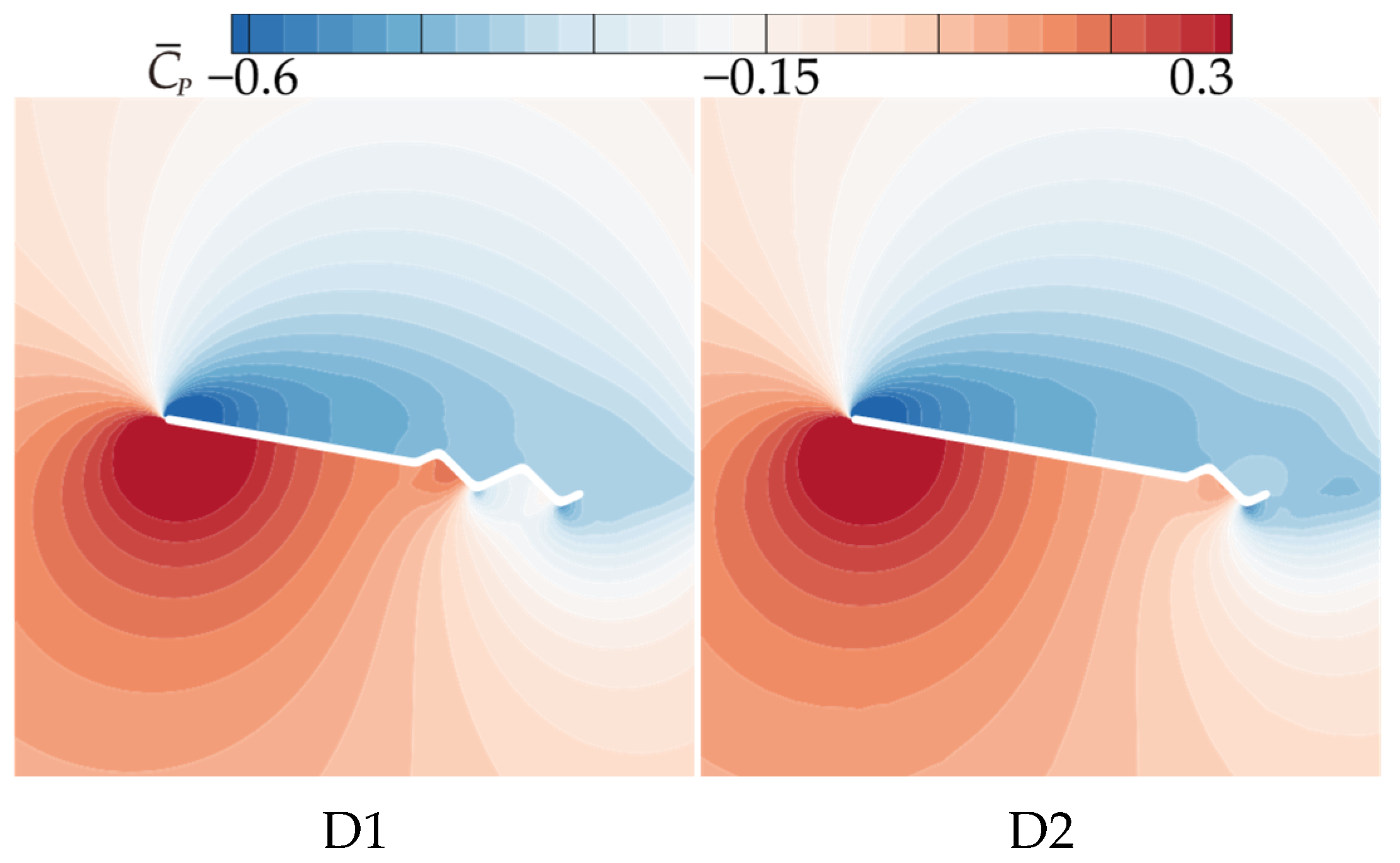
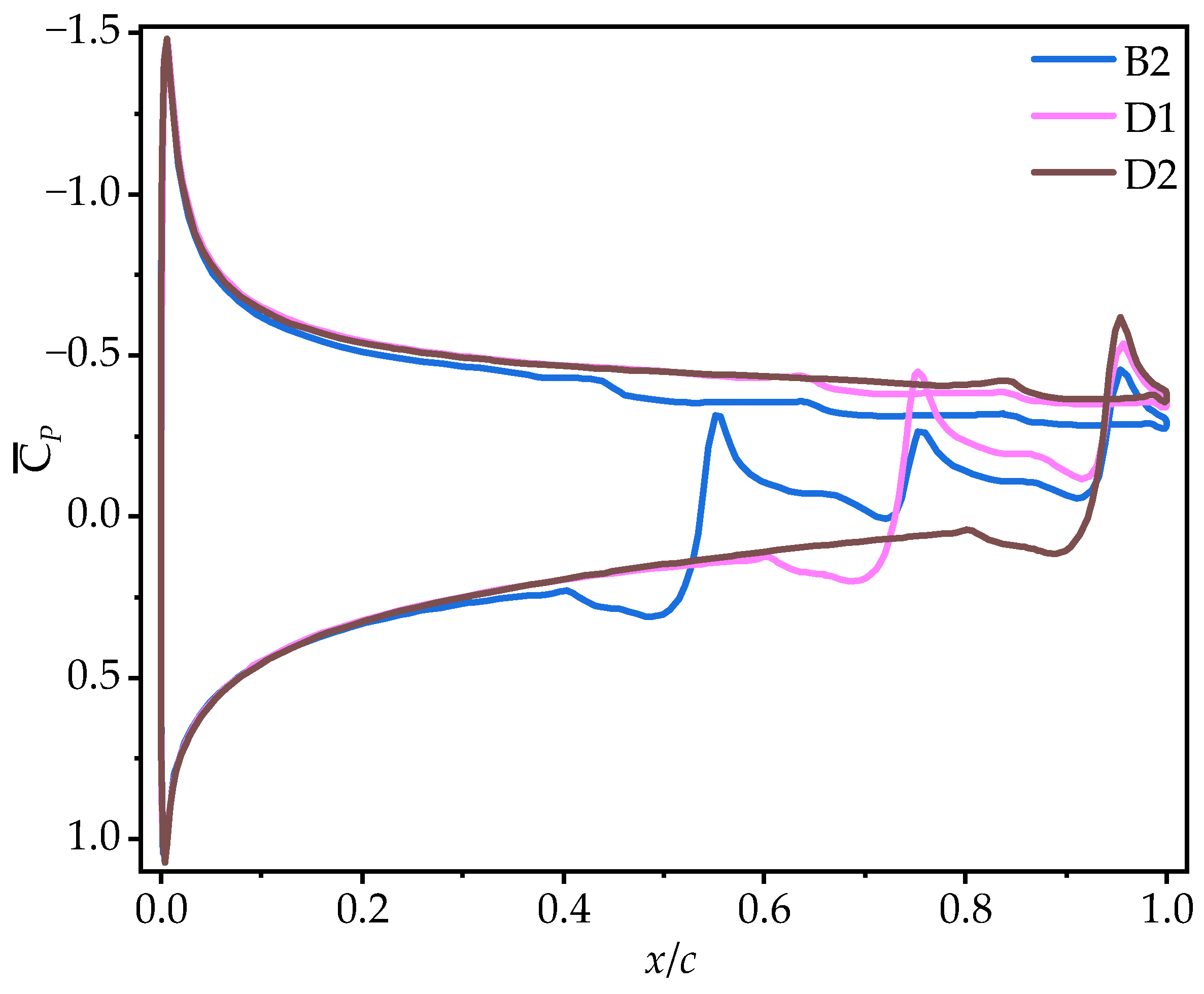
3.3. Effect of the Number of Trailing-Edge Corrugations
4. Conclusions
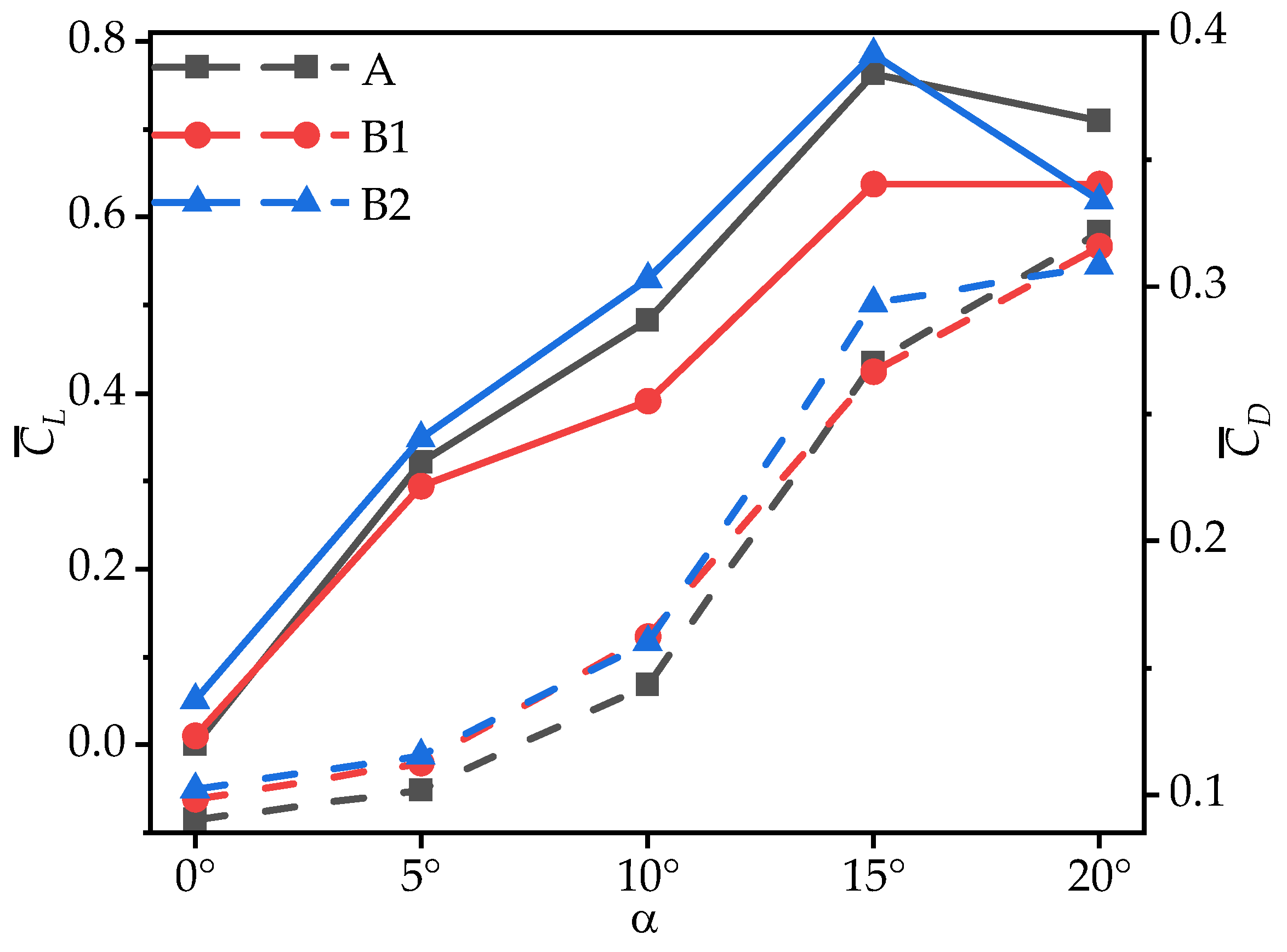
- Trailing-edge corrugations (B2) significantly enhanced aerodynamic performance compared to leading-edge configurations (B1). The closer proximity of corrugations toward the trailing edge promoted stronger LEV adhesion to the upper surface, expanded the low-pressure region, and increased the lift coefficient by up to 9.70% relative to a flat plate (A) at = 10°.
- A linear increase in corrugation amplitude toward the trailing edge (C1) delayed LEV shedding and reduced vortex generation in lower-surface grooves. This configuration achieved a 28.99% increase in lift coefficient and a 31.96% improvement in lift-to-drag ratio compared to uniform-amplitude corrugations (B2).
- Reducing the number of trailing-edge corrugations (e.g., D2 with one corrugation) consolidated localized vortices into larger, coherent LEVs, further increasing upper-surface suction. On the other hand, the number of negative pressure peaks at the corrugations decreases on the lower surface, thus enhancing the pressure difference between the upper and lower surfaces. D2 exhibited a 20.09% increase in lift coefficient and a 20.91% improvement in lift-to-drag ratio compared to B2.
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Song, F.; Yan, Y.; Sun, J. Review of Insect-Inspired Wing Micro Air Vehicle. Arthropod Struct. Dev. 2023, 72, 101225. [Google Scholar] [CrossRef] [PubMed]
- Murphy, J.; Hu, H. An Experimental Investigation on a Bio-Inspired Corrugated Airfoil. In Proceedings of the 47th AIAA Aerospace Sciences Meeting Including the New Horizons Forum and Aerospace Exposition, Orlando, FL, USA, 5 January 2009; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2009. [Google Scholar]
- Salami, E.; Ward, T.A.; Montazer, E.; Ghazali, N.N.N. A Review of Aerodynamic Studies on Dragonfly Flight. Proc. Inst. Mech. Eng. Part C J. Mech. Eng. Sci. 2019, 233, 6519–6537. [Google Scholar] [CrossRef]
- Bomphrey, R.J.; Nakata, T.; Henningsson, P.; Lin, H.-T. Flight of the Dragonflies and Damselflies. Phil. Trans. R. Soc. B 2016, 371, 20150389. [Google Scholar] [CrossRef] [PubMed]
- Lian, Y.; Broering, T.; Hord, K.; Prater, R. The Characterization of Tandem and Corrugated Wings. Prog. Aerosp. Sci. 2014, 65, 41–69. [Google Scholar] [CrossRef]
- Okamoto, M.; Yasuda, K.; Azuma, A. Aerodynamic Characteristics of the Wings and Body of a Dragonfly. J. Exp. Biol. 1996, 199, 281–294. [Google Scholar] [CrossRef]
- Kesel, A.B. Aerodynamic Characteristics of Dragonfly Wing Sections Compared with Technical Aerofoils. J. Exp. Biol. 2000, 203, 3125–3135. [Google Scholar] [CrossRef]
- Rees, C.J.C. Aerodynamic Properties of an Insect Wing Section and a Smooth Aerofoil Compared. Nature 1975, 258, 141–142. [Google Scholar] [CrossRef]
- Newman, B.G.; Savage, S.B.; Schouella, D. Model Test on a Wing Section of an Aeschna Dragonfly. In Scale Effects in Animal Locomotion: Based on the Proceedings of an International Symposium Held at Cambridge University, September, 1975; Academic Press: London, UK, 1977; pp. 445–477. [Google Scholar]
- Buckholz, R.H. The Functional Role of Wing Corrugations in Living Systems. J. Fluids Eng. 1986, 108, 93–97. [Google Scholar] [CrossRef]
- Wakeling, J.M.; Ellington, C.P. Dragonfly Flight: I. Gliding Flight and Steady-State Aerodynamic Forces. J. Exp. Biol. 1997, 200, 543–556. [Google Scholar] [CrossRef]
- Levy, D.-E.; Seifert, A. Simplified Dragonfly Airfoil Aerodynamics at Reynolds Numbers below 8000. Phys. Fluids 2009, 21, 071901. [Google Scholar] [CrossRef]
- Narita, Y.; Chiba, K. Aerodynamics on a Faithful Hindwing Model of a Migratory Dragonfly Based on 3d Scan Data. J. Fluids Struct. 2024, 125, 104080. [Google Scholar] [CrossRef]
- Moscato, G.; Romano, G. Biomimetic Wings for Micro Air Vehicles. Biomimetics 2024, 9, 553. [Google Scholar] [CrossRef] [PubMed]
- Chitsaz, N.; Marian, R.; Chahl, J. Experimental Method for 3D Reconstruction of Odonata Wings (Methodology and Dataset). PLoS ONE 2020, 15, e0232193. [Google Scholar] [CrossRef] [PubMed]
- Ren, L.; Li, X. Functional Characteristics of Dragonfly Wings and Its Bionic Investigation Progress. Sci. China Technol. Sci. 2013, 56, 884–897. [Google Scholar] [CrossRef]
- Chandra, S. Low Reynolds Number Flow over Low Aspect Ratio Corrugated Wing. Int. J. Aeronaut. Space Sci. 2020, 21, 627–637. [Google Scholar] [CrossRef]
- XU Na; ZHOU Shuaizhi; MOU Xiaolei Influence of corrugation position on aerodynamic performance of dragonfly gliding airfoils. J. Aerosp. Power 2021, 36, 1434–1442. [CrossRef]
- Rohit, B.; Reddy, S.S.R. Computations of Flow Past the Corrugated Airfoil of Drosophila Melanogaster at Ultra Low Reynolds Number. JAFM 2021, 14, 417–427. [Google Scholar] [CrossRef]
- Meng, X.G.; Sun, M. Aerodynamic Effects of Wing Corrugation at Gliding Flight at Low Reynolds Numbers. Phys. Fluids 2013, 25, 071905. [Google Scholar] [CrossRef]
- Mukherjee, P.; Pawar, A.A.; Sanat Ranjan, K.; Saha, S. Corrugation Assisted Enhancement of Aerodynamic Characteristics of Delta Wing for Micro Aerial Vehicle. J. Aircr. 2021, 58, 300–309. [Google Scholar] [CrossRef]
- Guilarte Herrero, A.; Noguchi, A.; Kusama, K.; Shigeta, T.; Nagata, T.; Nonomura, T.; Asai, K. Effects of Compressibility and Reynolds Number on the Aerodynamics of a Simplified Corrugated Airfoil. Exp. Fluids 2021, 62, 63. [Google Scholar] [CrossRef]
- Chitsaz, N.; Siddiqui, K.; Marian, R.; Chahl, J.S. Numerical and Experimental Analysis of 3d Micro-Corrugated Wing in Gliding Flight. J. Fluids Eng. 2022, 144, 011205. [Google Scholar] [CrossRef]
- Zhang, Z.; Yin, Y.; Zhong, Z.; Zhao, H. Aerodynamic Performance of Dragonfly Wing with Well-Designed Corrugated Section in Gliding Flight. Comput. Model. Eng. Sci. 2015, 109, 285–302. [Google Scholar]
- Shabbir, Z.; Shah, H.R.; Mansoor, A.; Ahmed, A.; Tayyab, S.M. Effects of Varying Peak Shape on Aerodynamic Performance of a Corrugated Airfoil. In Proceedings of the AIAA Scitech 2020 Forum, Orlando, FL, USA, 6 January 2020; American Institute of Aeronautics and Astronautics: Reston, VA, USA, 2020. [Google Scholar]
- Murphy, J.T.; Hu, H. An Experimental Study of a Bio-Inspired Corrugated Airfoil for Micro Air Vehicle Applications. Exp. Fluids 2010, 49, 531–546. [Google Scholar] [CrossRef]
- Wang, X.; Zhang, Z.; Ren, H.; Chen, Y.; Wu, B. Role of Soft Matter in the Sandwich Vein of Dragonfly Wing in Its Configuration and Aerodynamic Behaviors. J. Bionic Eng. 2017, 14, 557–566. [Google Scholar] [CrossRef]
- Chitsaz, N.; Marian, R.; Chitsaz, A.; Chahl, J.S. Parametric and Statistical Study of the Wing Geometry of 75 Species of Odonata. Appl. Sci. 2020, 10, 5389. [Google Scholar] [CrossRef]
- Rees, C.J.C. Form and Function in Corrugated Insect Wings. Nature 1975, 256, 200–203. [Google Scholar] [CrossRef]
- Du, G.; Sun, M. Aerodynamic Effects of Corrugation and Deformation in Flapping Wings of Hovering Hoverflies. J. Theor. Biol. 2012, 300, 19–28. [Google Scholar] [CrossRef]
- Meng, X.G.; Xu, L.; Sun, M. Aerodynamic Effects of Corrugation in Flapping Insect Wings in Hovering Flight. J. Exp. Biol. 2011, 214, 432–444. [Google Scholar] [CrossRef] [PubMed]
- Meng, X.; Sun, M. Aerodynamic Effects of Corrugation in Flapping Insect Wings in Forward Flight. J. Bionic Eng. 2011, 8, 140–150. [Google Scholar] [CrossRef]
- Au, L.T.K.; Phan, H.V.; Park, S.H.; Park, H.C. Effect of Corrugation on the Aerodynamic Performance of Three-Dimensional Flapping Wings. Aerosp. Sci. Technol. 2020, 105, 106041. [Google Scholar] [CrossRef]
- Dao, T.T.; Loan Au, T.K.; Park, S.H.; Park, H.C. Effect of Wing Corrugation on the Aerodynamic Efficiency of Two-Dimensional Flapping Wings. Appl. Sci. 2020, 10, 7375. [Google Scholar] [CrossRef]
- Ansari, M.I.; Siddique, M.H.; Samad, A.; Anwer, S.F. On the Optimal Morphology and Performance of a Modeled Dragonfly Airfoil in Gliding Mode. Phys. Fluids 2019, 31, 051904. [Google Scholar] [CrossRef]
- Kim, W.-K.; Ko, J.H.; Park, H.C.; Byun, D. Effects of Corrugation of the Dragonfly Wing on Gliding Performance. J. Theor. Biol. 2009, 260, 523–530. [Google Scholar] [CrossRef] [PubMed]
- Chen, Y.H.; Skote, M. Gliding Performance of 3-D Corrugated Dragonfly Wing with Spanwise Variation. J. Fluids Struct. 2016, 62, 1–13. [Google Scholar] [CrossRef]
- Luo, Y.; He, G.; Liu, H.; Wang, Q.; Song, H. Aerodynamic Performance of Dragonfly Forewing–Hindwing Interaction in Gliding Flight. IOP Conf. Ser. Mater. Sci. Eng. 2019, 538, 012048. [Google Scholar] [CrossRef]
- Liu, C.; Du, R.; Li, F.; Sun, J. Bioinspiration of the Vein Structure of Dragonfly Wings on Its Flight Characteristics. Microsc. Res. Tech. 2022, 85, 829–839. [Google Scholar] [CrossRef]
- Shen, H.; Cao, K.; Liu, C.; Mao, Z.; Li, Q.; Han, Q.; Sun, Y.; Yang, Z.; Xu, Y.; Wu, S.; et al. Kinematics and Flow Field Analysis of Allomyrina Dichotoma Flight. Biomimetics 2024, 9, 777. [Google Scholar] [CrossRef]
- Hord, K.; Liang, Y. Numerical Investigation of the Aerodynamic and Structural Characteristics of a Corrugated Airfoil. J. Aircr. 2012, 49, 749–757. [Google Scholar] [CrossRef]
- Taira, K.; Colonius, T. Three-Dimensional Flows around Low-Aspect-Ratio Flat-Plate Wings at Low Reynolds Numbers. J. Fluid Mech. 2009, 623, 187–207. [Google Scholar] [CrossRef]








| Researcher | Dimensionality | Corrugation | Re | |
|---|---|---|---|---|
| Chordwise corrugation position | Xu [18] | 2D | At leading, middle, and trailing edge | 1500 |
| Meng [20] | 3D | Close to the leading edge | 1000 | |
| Rohit [19] | 2D | At leading and trailing edge | 1000 | |
| This study | 3D | At leading and trailing edge | 1350 | |
| Corrugation amplitude | Zhang [24] | 2D | Global variation in all corrugation amplitudes | 500~12,000 |
| Shabbir [25] | 2D | Amplitude variation in the first two leading-edge corrugations | 58,000, 125,000 | |
| Wang [27] | 3D | Global variation in corrugation amplitude | 50,000~100,000 | |
| This study | 3D | Chordwise linear variation in corrugation amplitude | 1350 | |
| Number of corrugations | Rohit [19] | 2D | Variation in the number of leading-edge corrugations | 1000 |
| This study | 3D | Variation in the number of trailing-edge corrugations | 1350 |
| Time Step (s) | () | () | ||
|---|---|---|---|---|
| 2 × 10−4 | 0.756 | 1.05% | 0.268 | 0.74% |
| 1 × 10−4 | 0.764 | 0.13% | 0.270 | 0% |
| 5 × 10−5 | 0.763 | 0.270 |
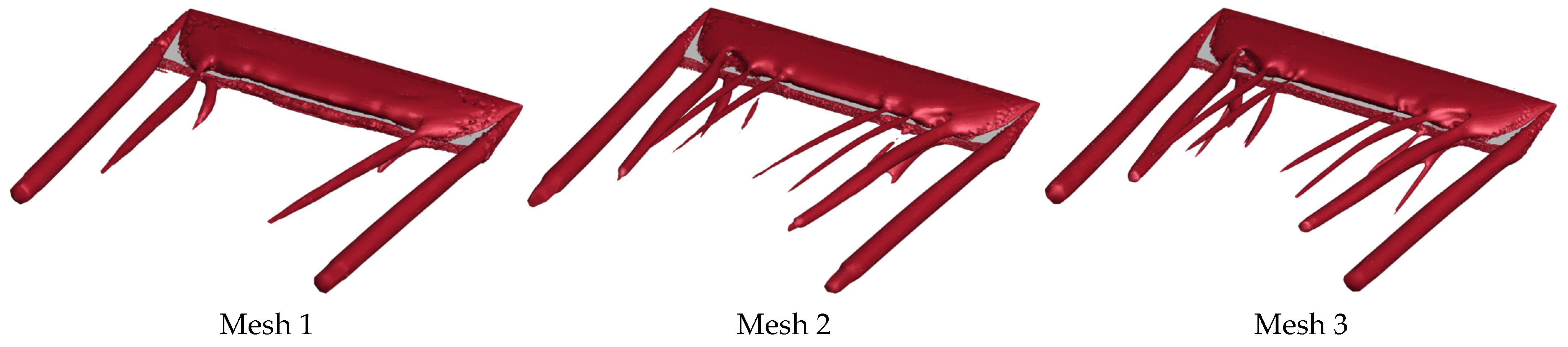
| Mesh Numbers (Million) | () | () | |||
|---|---|---|---|---|---|
| Mesh 1 | 2.1 | 0.733 | 4.06% | 0.261 | 3.33% |
| Mesh 2 | 5.0 | 0.764 | 2.55% | 0.270 | 2.17% |
| Mesh 3 | 10.0 | 0.784 | 0.276 |
| Wing | 0° | 5° | 10° | 15° | 20° | |
|---|---|---|---|---|---|---|
| B1 | ↑ | 8.26% ↓ | 19.11% ↓ | 16.48% ↓ | 10.09% ↓ | |
| B2 | ↑ | 8.49% ↑ | 9.70% ↑ | 2.79% ↑ | 12.84% ↓ | |
| B1 | 8.87% ↑ | 10.60% ↑ | 13.00% ↑ | 1.26% ↓ | 1.75% ↓ | |
| B2 | 13.30% ↑ | 13.61% ↑ | 11.60% ↑ | 8.74% ↑ | 4.09% ↓ | |
| B1 | ↑ | 17.06% ↓ | 28.41% ↓ | 15.41% ↓ | 8.49% ↓ | |
| B2 | ↑ | 4.51% ↓ | 1.70% ↓ | 5.47% ↓ | 9.13% ↓ |
| Wing | 0° | 5° | 10° | 15° | 20° | |
|---|---|---|---|---|---|---|
| C1 | 28.99% ↑ | 11.76% ↑ | 10.85% ↑ | 7.53% ↑ | 5.06% ↑ | |
| C2 | 52.72% ↓ | 10.24% ↓ | 6.50% ↓ | 10.55% ↓ | 7.63% ↑ | |
| C3 | 13.42% ↓ | 0.33% ↑ | 0.69% ↓ | 1.81% ↓ | 7.01% ↑ | |
| C1 | 2.25% ↓ | 0.26% ↓ | 6.77% ↑ | 4.22% ↑ | 1.45% ↑ | |
| C2 | 6.85% ↓ | 7.76% ↓ | 7.93% ↓ | 10.39% ↓ | 2.46% ↑ | |
| C3 | 5.87% ↓ | 5.21% ↓ | 2.87% ↓ | 3.68% ↓ | 2.48% ↑ | |
| C1 | 31.96% ↑ | 12.05% ↑ | 3.83% ↑ | 3.17% ↑ | 3.56% ↑ | |
| C2 | 49.77% ↓ | 2.69% ↓ | 1.55% ↑ | 0.18% ↓ | 5.05% ↑ | |
| C3 | 8.03% ↓ | 5.84% ↑ | 2.25% ↑ | 1.94% ↑ | 4.41% ↑ |
| Wing | 0° | 5° | 10° | 15° | 20° | |
|---|---|---|---|---|---|---|
| D1 | 5.84% ↑ | 3.76% ↑ | 10.00% ↑ | 3.32% ↑ | 1.34% ↑ | |
| D2 | 19.84% ↑ | 17.39% ↑ | 20.09% ↑ | 15.11% ↑ | 9.51% ↑ | |
| D1 | 0.49% ↓ | 0.62% ↓ | 6.33% ↑ | 1.16% ↑ | 0.56% ↓ | |
| D2 | 0.88% ↓ | 1.60% ↑ | 11.28% ↑ | 8.10% ↑ | 3.07% ↑ | |
| D1 | 6.26% ↑ | 4.41% ↑ | 3.45% ↑ | 2.14% ↑ | 1.91% ↑ | |
| D2 | 20.91% ↑ | 15.54% ↑ | 7.92% ↑ | 6.49% ↑ | 6.25% ↑ |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Li, K.; Xu, N.; Zhong, L.; Mou, X. Corrugation at the Trailing Edge Enhances the Aerodynamic Performance of a Three-Dimensional Wing During Gliding Flight. Biomimetics 2025, 10, 329. https://doi.org/10.3390/biomimetics10050329
Li K, Xu N, Zhong L, Mou X. Corrugation at the Trailing Edge Enhances the Aerodynamic Performance of a Three-Dimensional Wing During Gliding Flight. Biomimetics. 2025; 10(5):329. https://doi.org/10.3390/biomimetics10050329
Chicago/Turabian StyleLi, Kaipeng, Na Xu, Licheng Zhong, and Xiaolei Mou. 2025. "Corrugation at the Trailing Edge Enhances the Aerodynamic Performance of a Three-Dimensional Wing During Gliding Flight" Biomimetics 10, no. 5: 329. https://doi.org/10.3390/biomimetics10050329
APA StyleLi, K., Xu, N., Zhong, L., & Mou, X. (2025). Corrugation at the Trailing Edge Enhances the Aerodynamic Performance of a Three-Dimensional Wing During Gliding Flight. Biomimetics, 10(5), 329. https://doi.org/10.3390/biomimetics10050329





