Effects of Wing Kinematics on Aerodynamics Performance for a Pigeon-Inspired Flapping Wing
Abstract
1. Introduction
2. Methodology
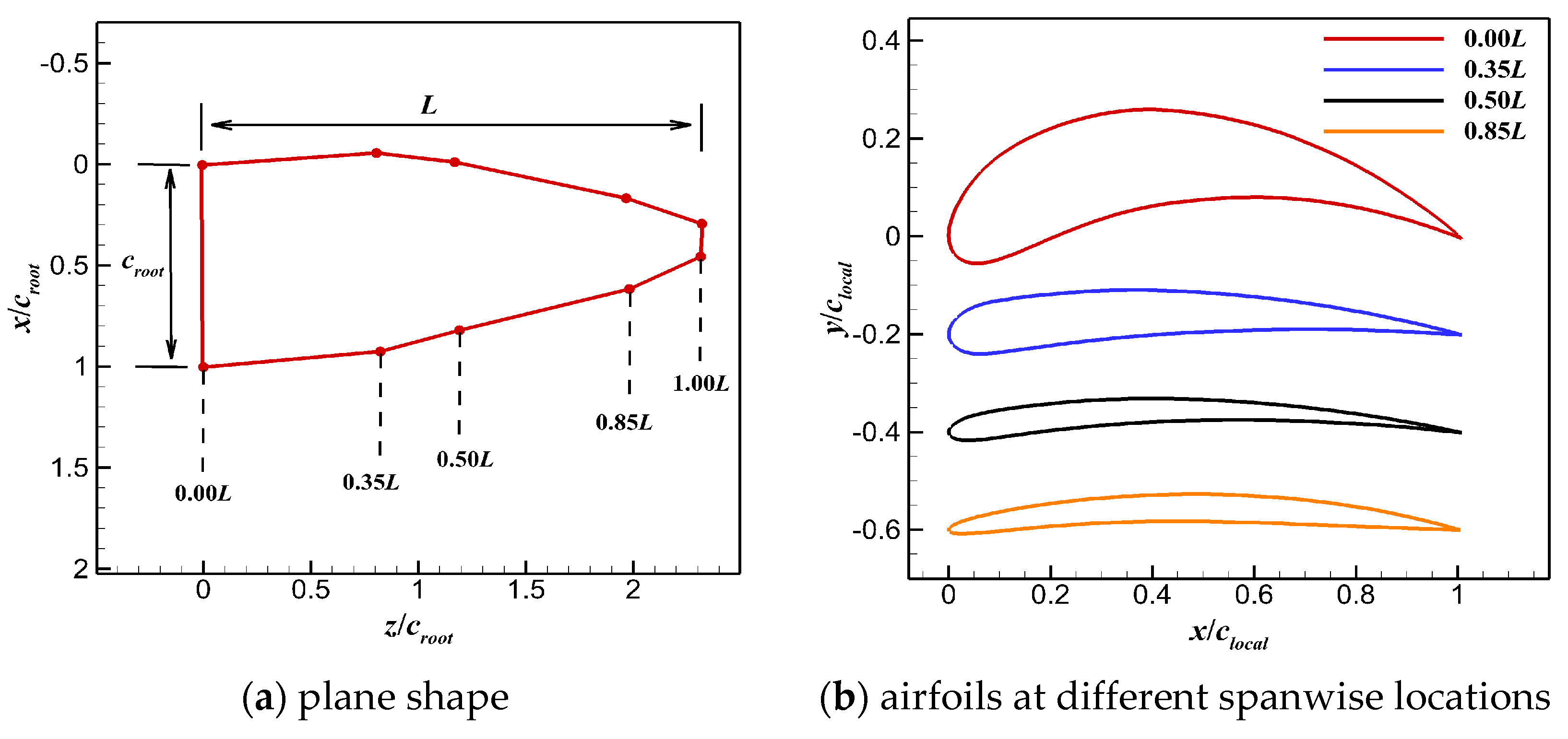
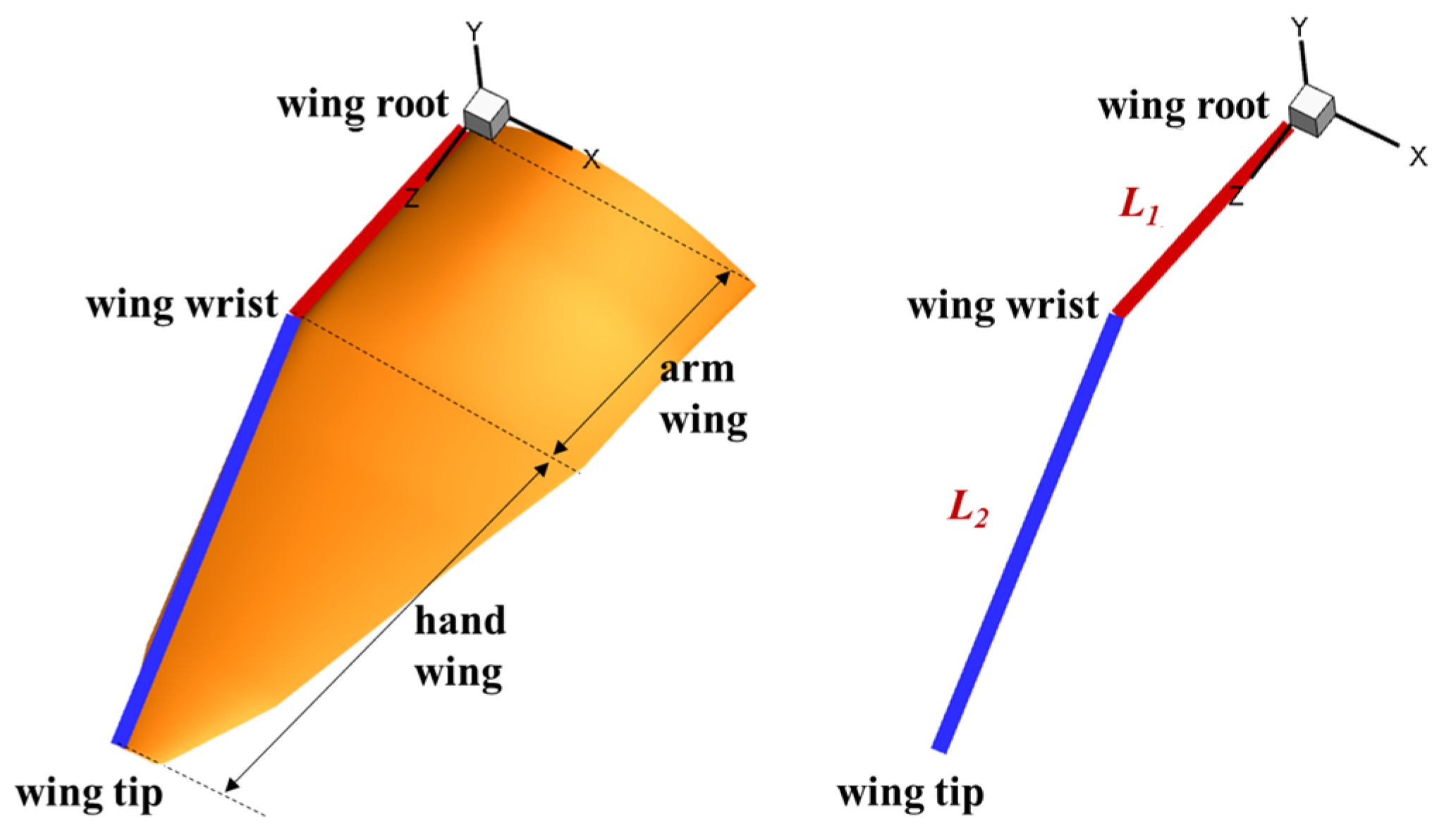
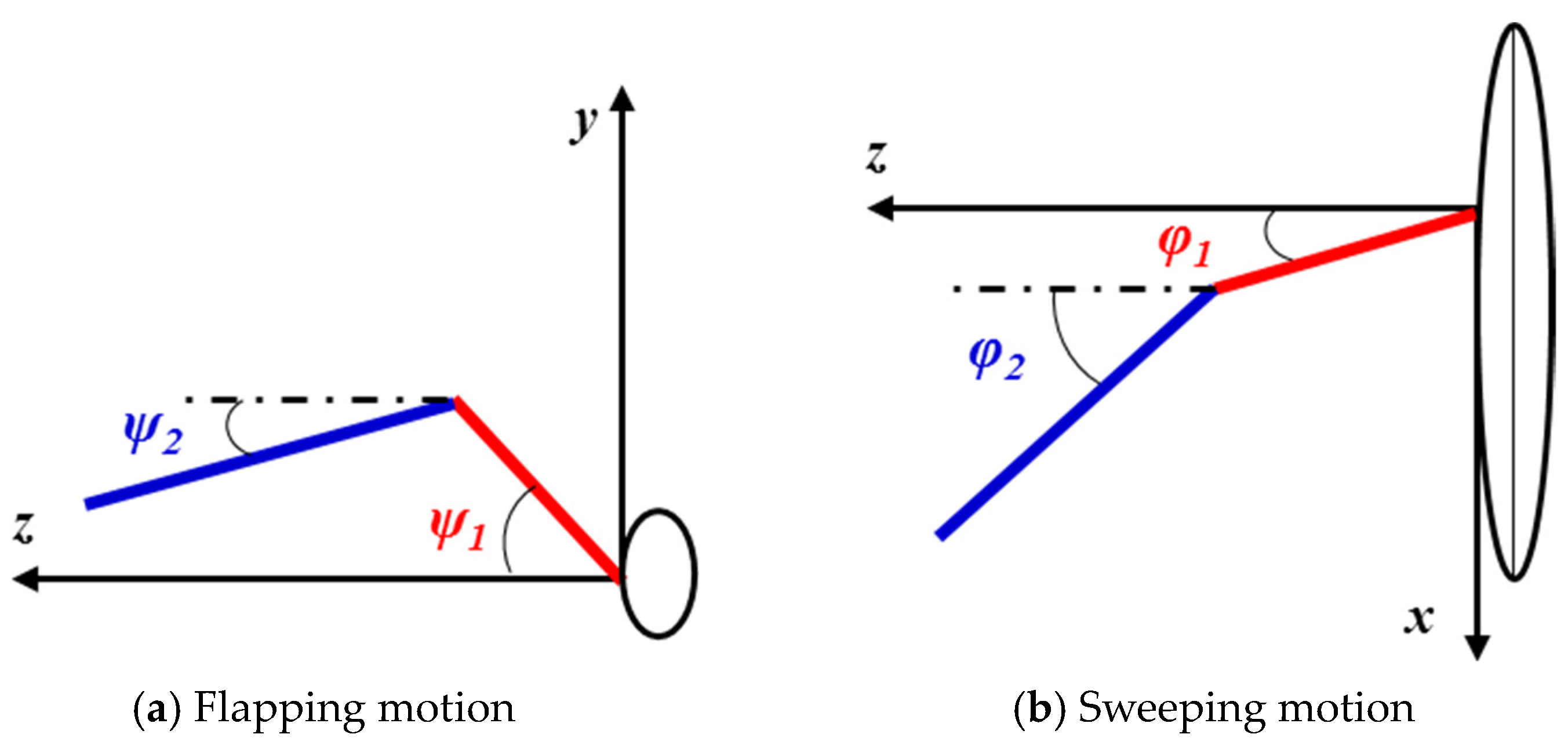
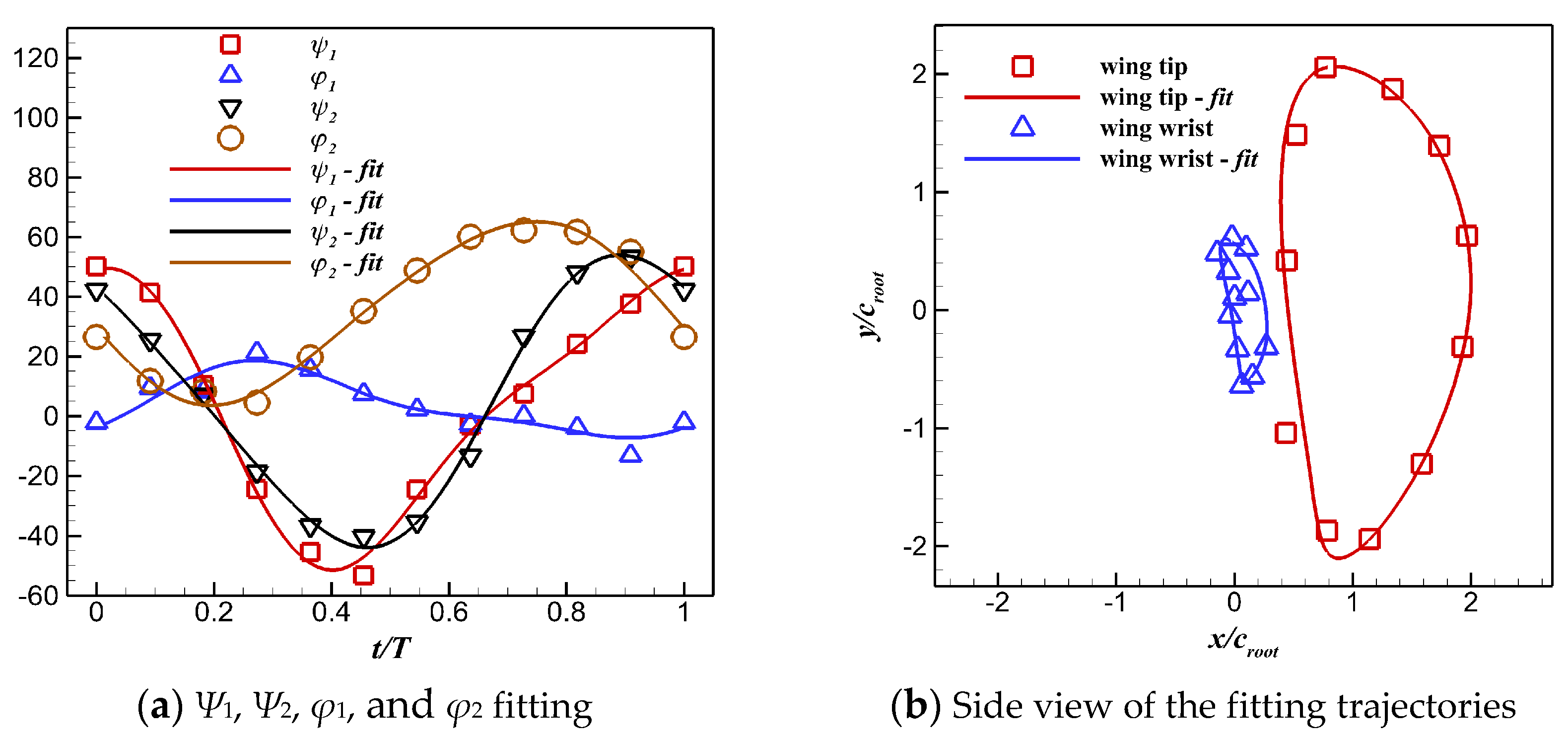
2.1. Geometry and Kinematic Models
2.2. Aerodynamic Performance
2.3. Numerical Simulation Methods
2.3.1. Flow Solver
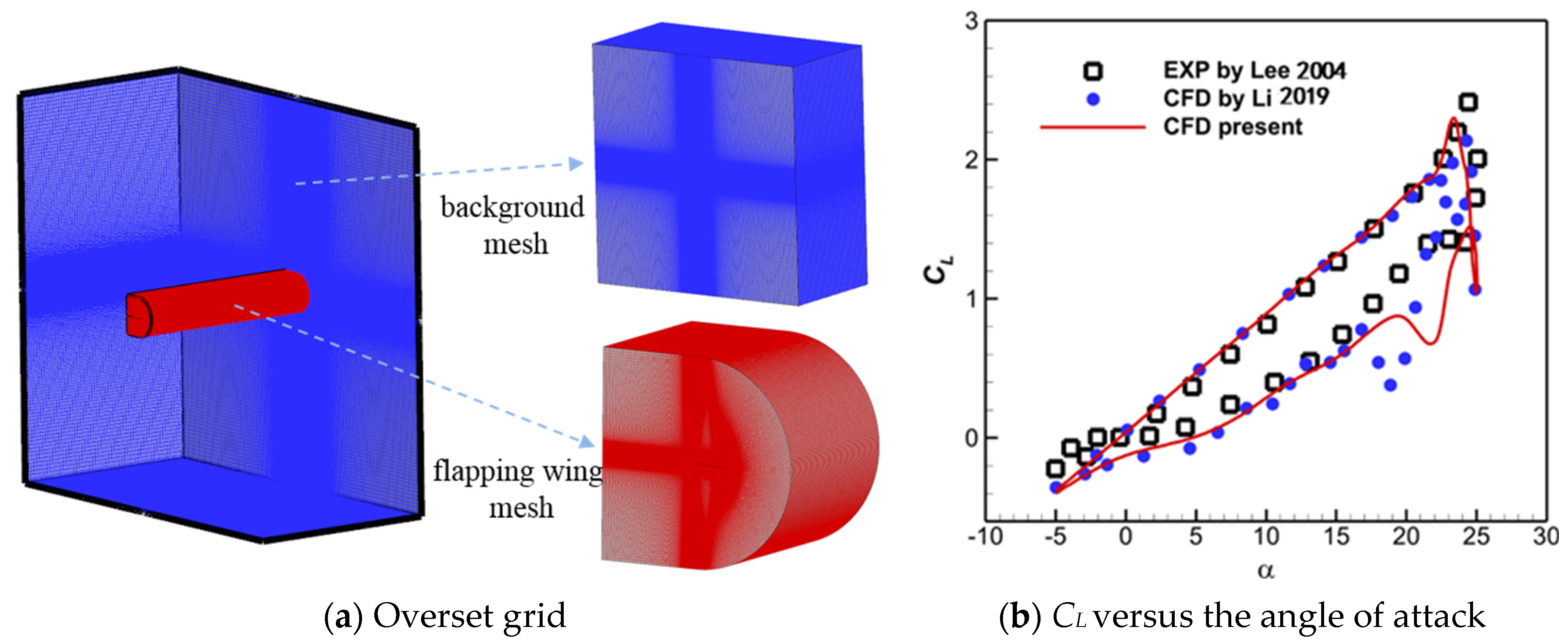
2.3.2. Convergence Study of Grid and Time Resolution
3. Results and Discussion
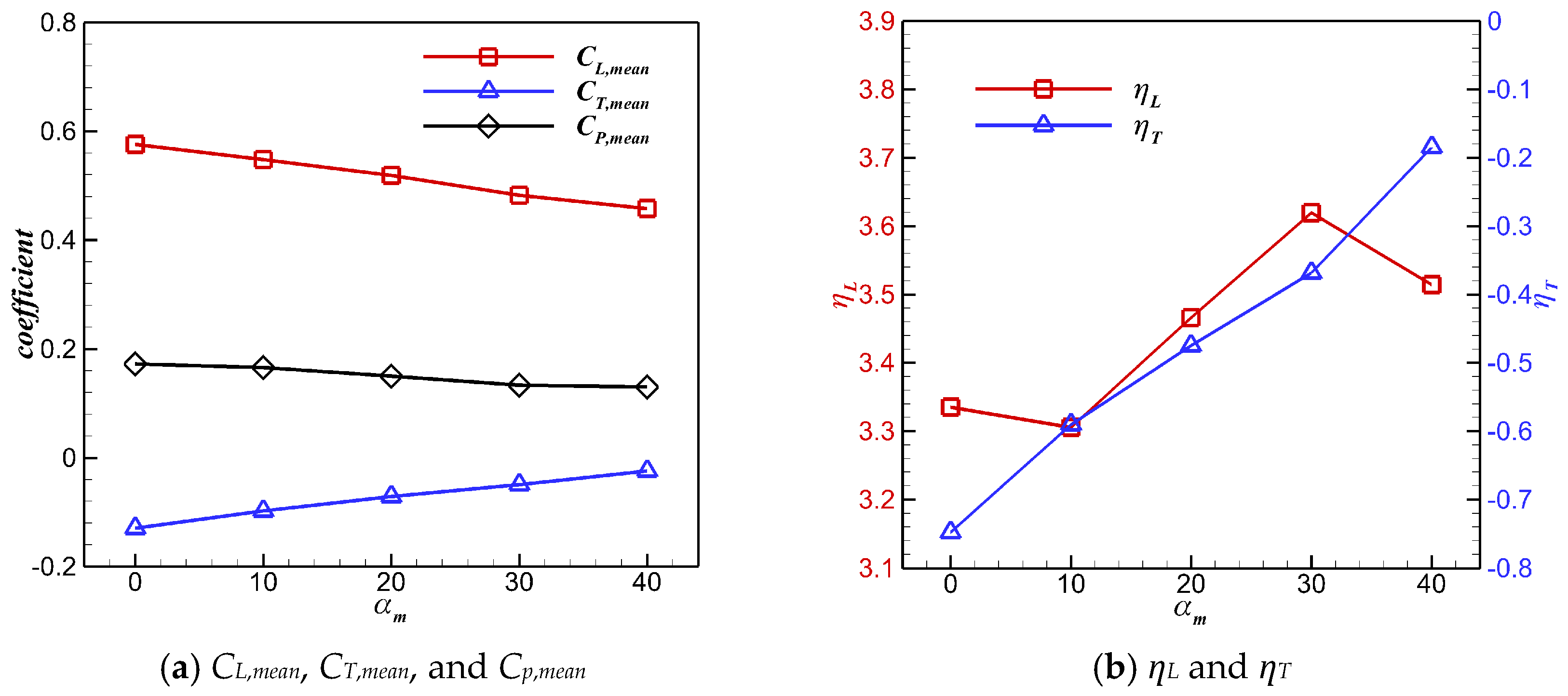
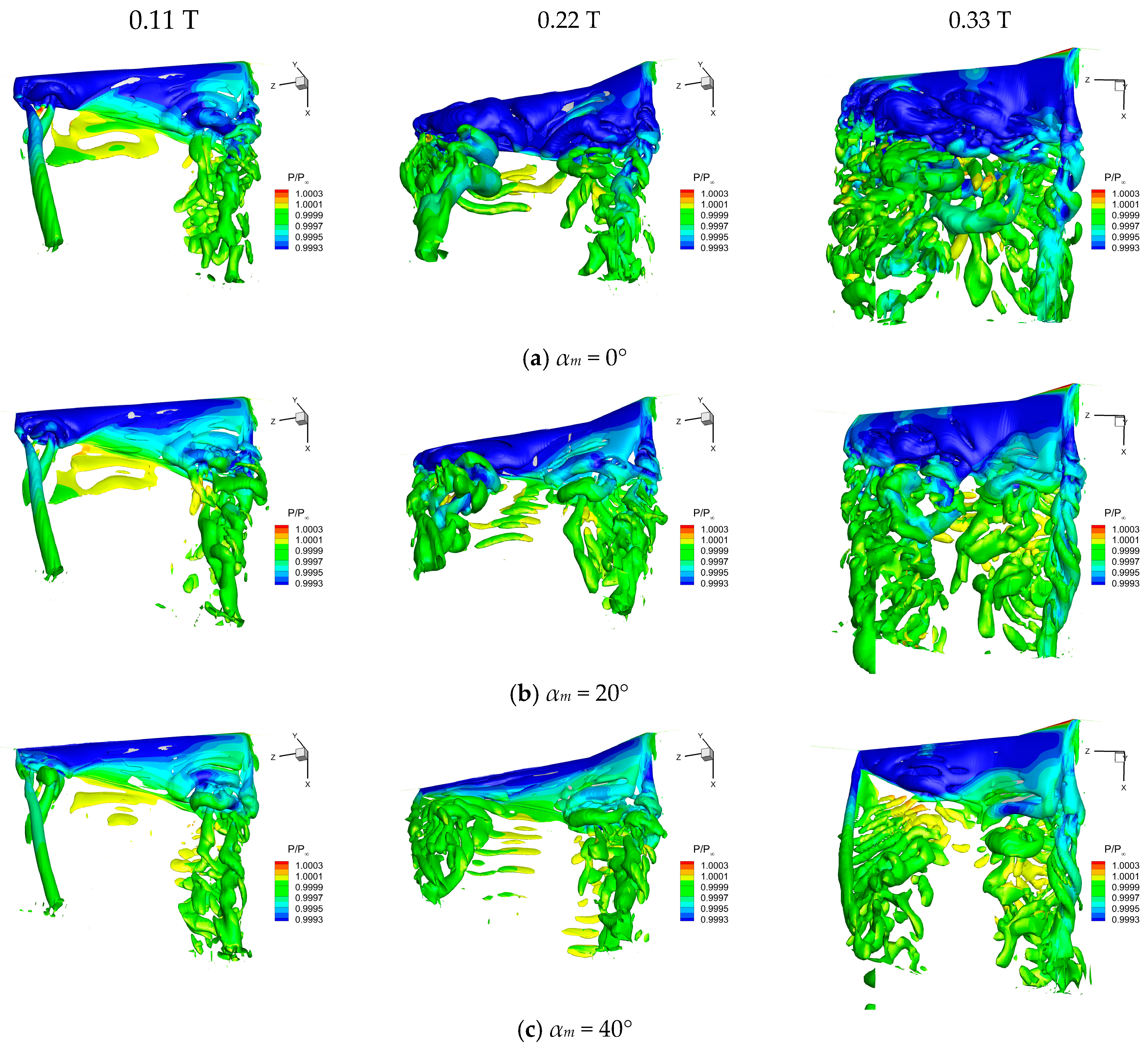
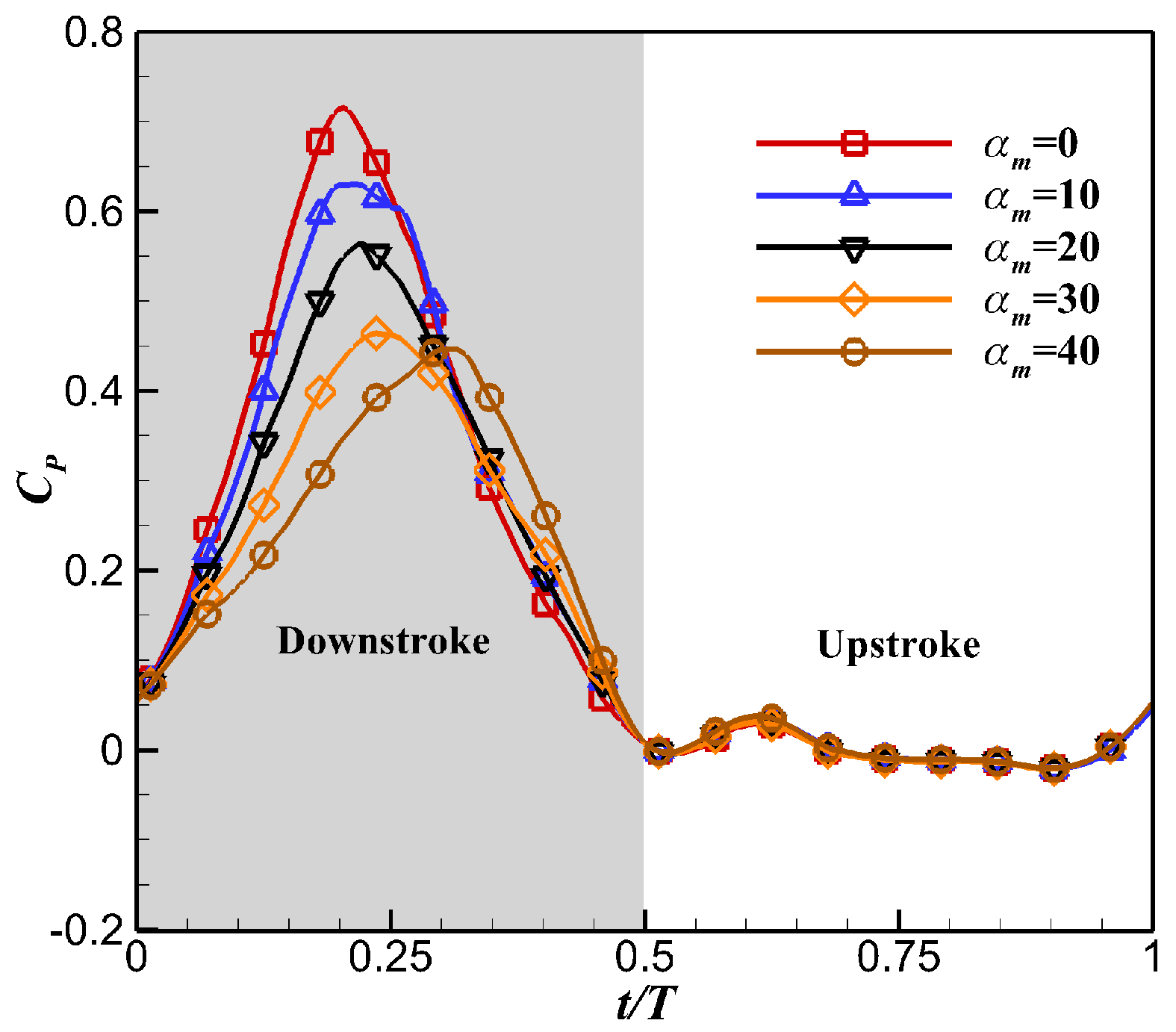
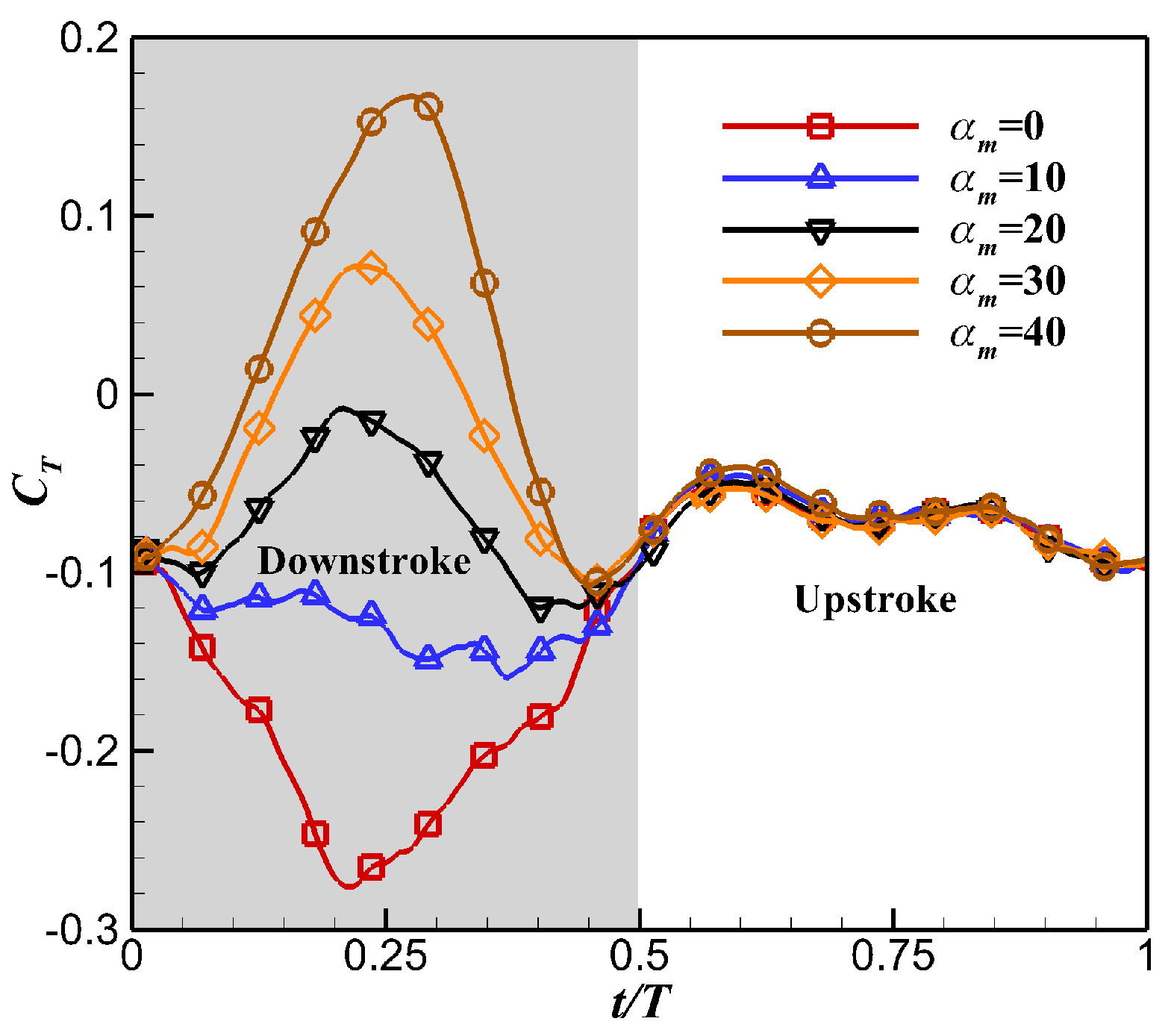
3.1. Effect of the Twisting Motion on Aerodynamics
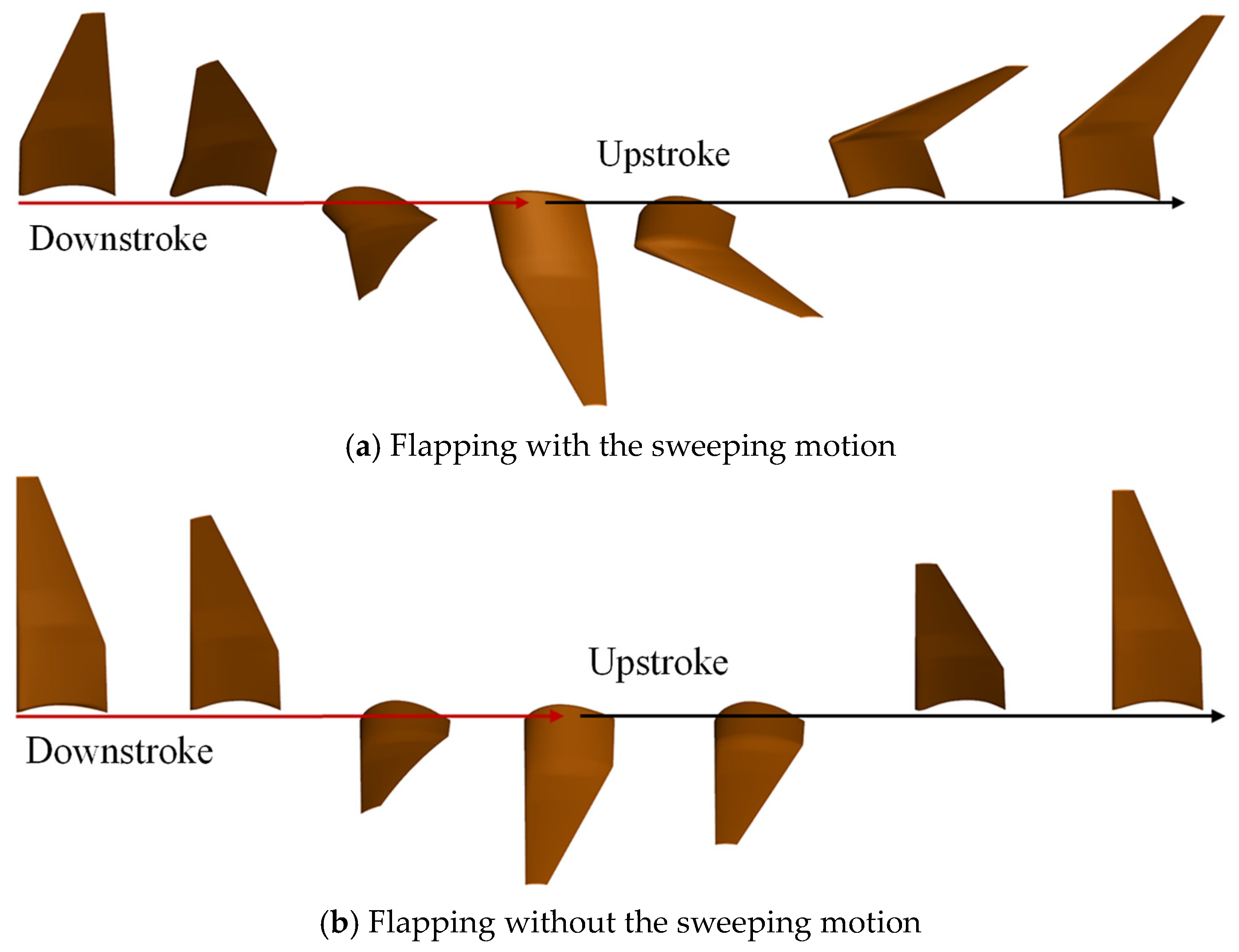
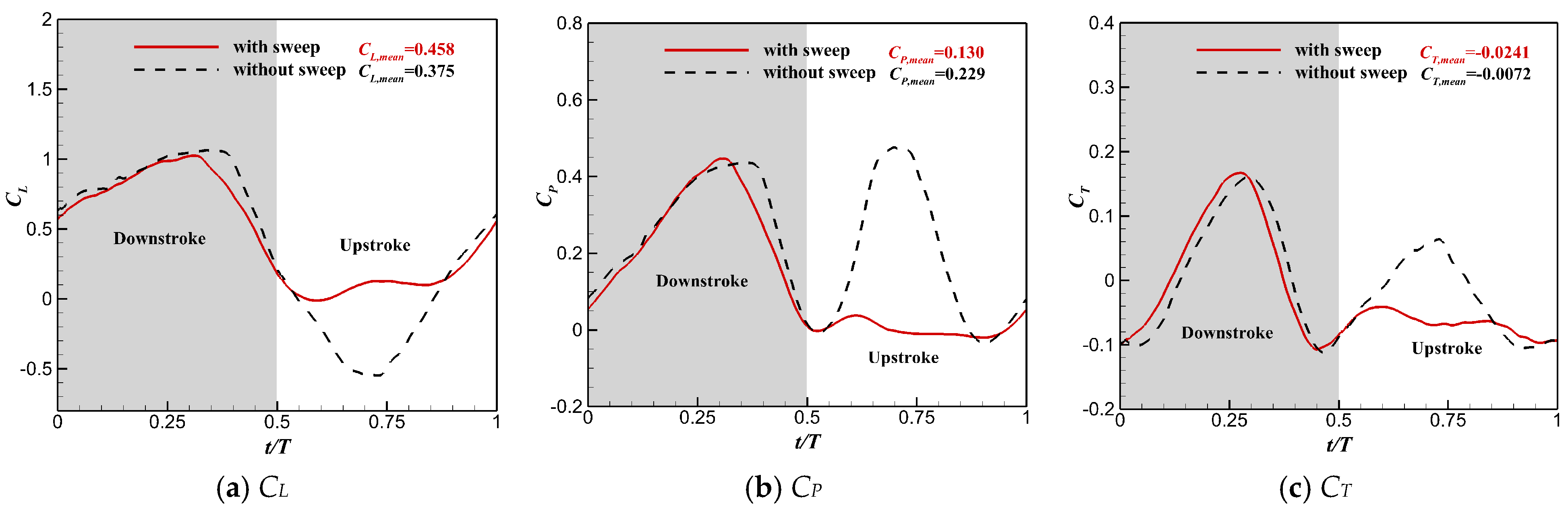
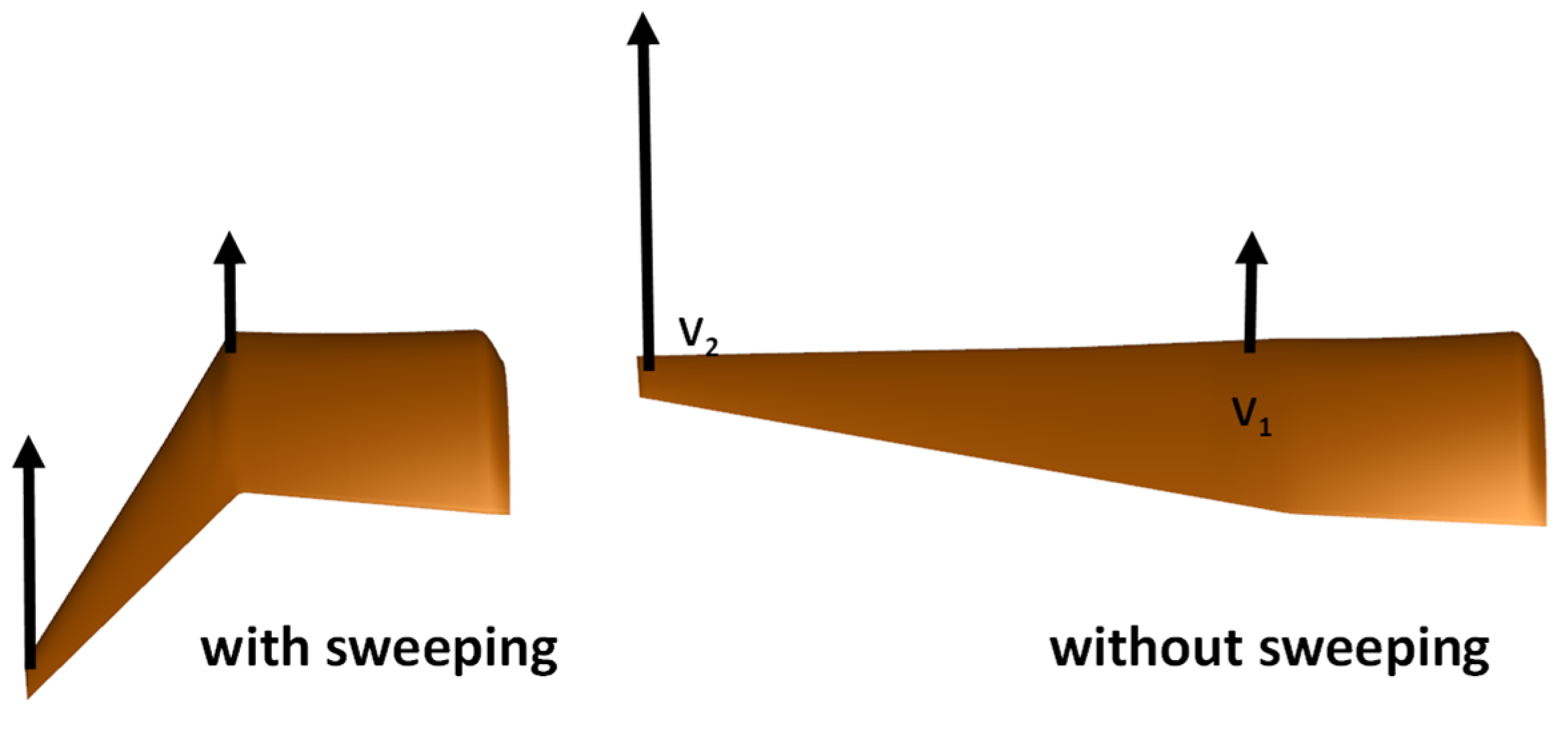
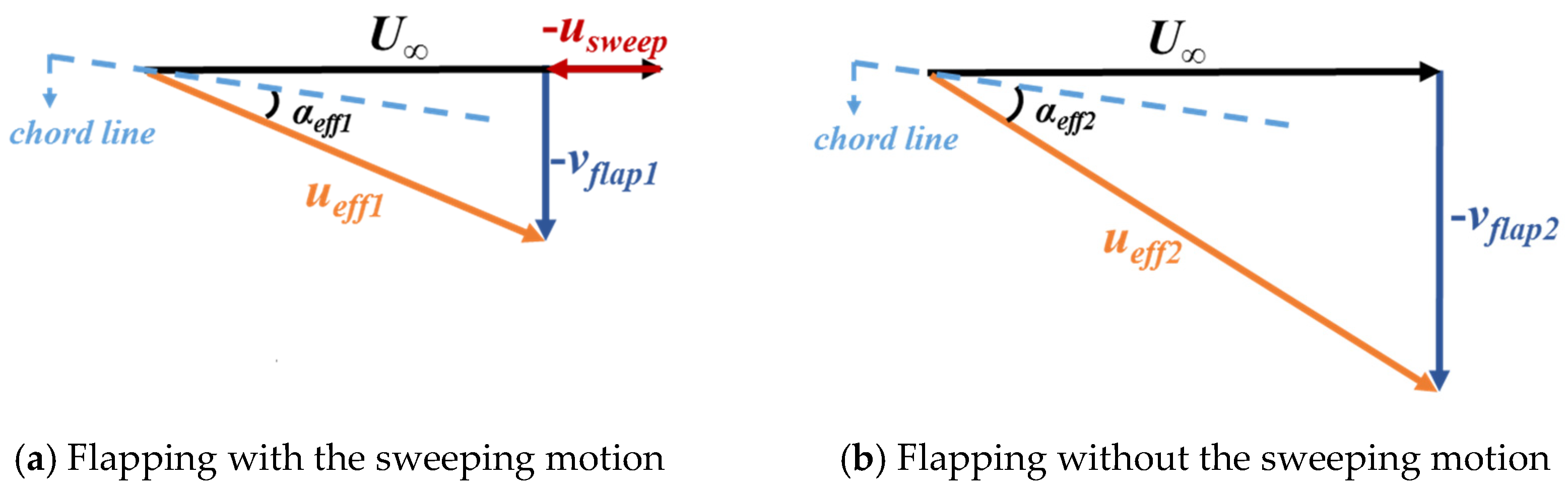
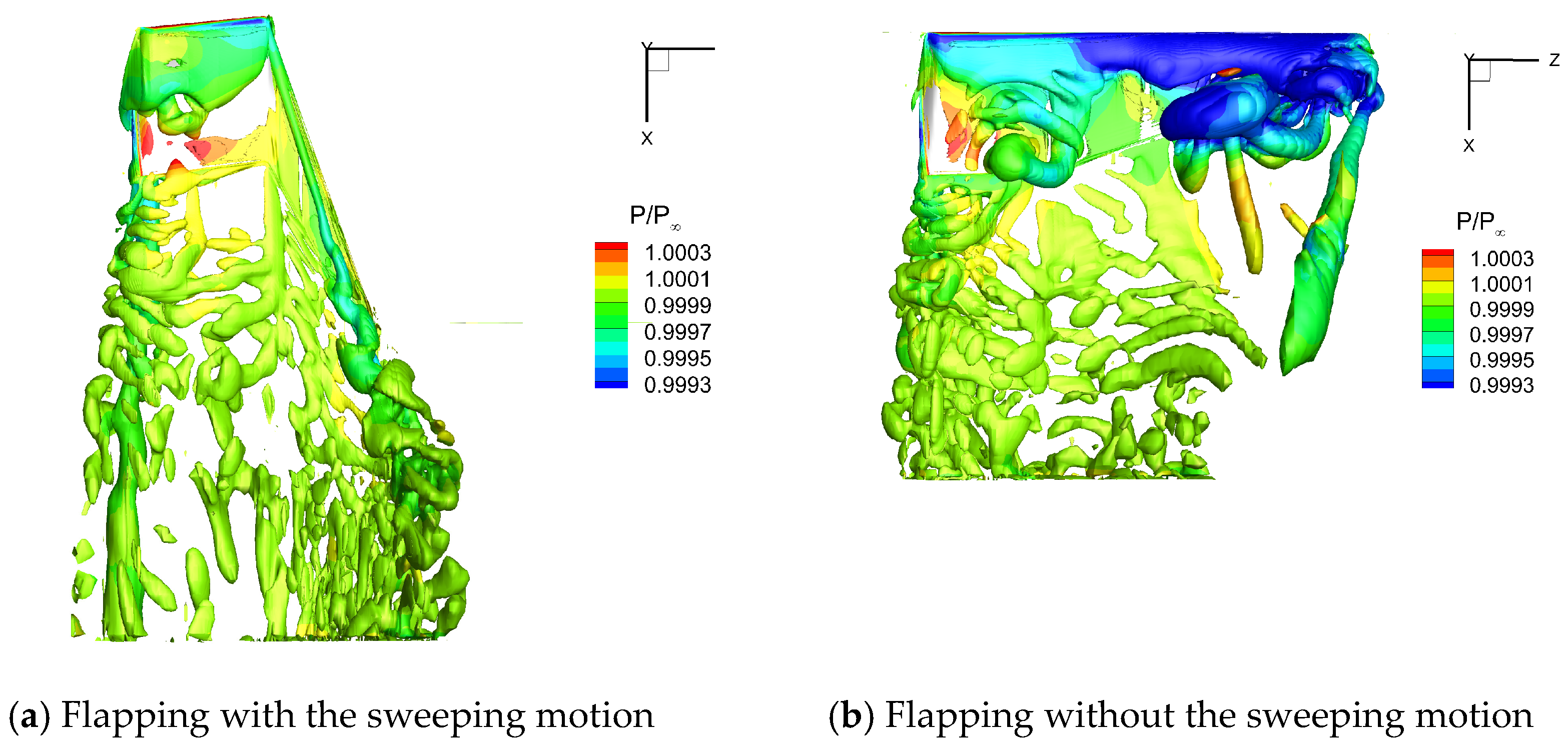
3.2. Effect of the Sweeping Motion on Aerodynamics
4. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Liu, T.; Kuykendoll, K.; Rhew, R.; Jones, S. Avian wings. In Proceedings of the 24th AIAA Aerodynamic Measurement Technology and Ground Testing Conference, Portland, OR, USA, 28 June–1 July 2004; pp. 2004–2186. [Google Scholar]
- Liu, T.; Kuykendoll, K.; Rhew, R.; Jones, S. Avian Wing Geometry and Kinematics. AIAA J. 2006, 44, 954–963. [Google Scholar] [CrossRef]
- Klän, S.; Bachmann, T.; Klaas, M.; Wagner, H.; Schröder, W. Experimental analysis of the flow field over a novel owl based airfoil. Exp. Fluids 2009, 46, 975–989. [Google Scholar] [CrossRef]
- Bachmann, T. Anatomical, Morphometrical and Biomechanical Studies of Barn Owls’ and Pigeons’ Wings. Ph.D. Thesis, RWTH Aachen University, Aachen, Germany, 2010. [Google Scholar]
- Carruthers, A.C.; Walker, S.M.; Thomas, A.L.R.; Taylor, G.K. Aerodynamics of aerofoil sections measured on a free-flying bird. P. I Mech. Eng. G-J. Aer. 2010, 224, 855–864. [Google Scholar] [CrossRef]
- Chen, K.; Liu, Q.P.; Sun, W.L.; Liao, G.H.; Ren, L.Q. Wing Kinematics of Long Eared Owl and Sparrow Hawk in Flapping Flight. Appl. Mech. Mater. 2014, 461, 297–304. [Google Scholar] [CrossRef]
- Crandell, K.E.; Tobalske, B.W. Kinematics and aerodynamics of avian upstrokes during slow flight. J. Exp. Biol. 2015, 218, 2518. [Google Scholar] [CrossRef]
- Wolf, T.; Konrath, R. Avian wing geometry and kinematics of a free-flying barn owl in flapping flight. Exp. Fluids 2015, 56, 28. [Google Scholar] [CrossRef]
- Stowers, A.K.; Matloff, L.Y.; Lentink, D. How pigeons couple three-dimensional elbow and wrist motion to morph their wings. J. R. Soc. Interface 2017, 14, 20170224. [Google Scholar] [CrossRef]
- Deetjen, M.E.; Biewener, A.A.; Lentink, D. High-speed surface reconstruction of a flying bird using structured light. J. Exp. Biol. 2017, 220, 1956. [Google Scholar] [CrossRef]
- Berg, A.M.; Biewener, A.A. Wing and body kinematics of takeoff and landing flight in the pigeon (Columba livia). J. Exp. Biol. 2010, 213, 1651–1658. [Google Scholar] [CrossRef]
- Carruthers, A.C.; Thomas, A.L.R.; Taylor, G.K. Automatic aeroelastic devices in the wings of a steppe eagle Aquila nipalensis. J. Exp. Biol. 2007, 210, 4136–4149. [Google Scholar] [CrossRef]
- Ros, I.G.; Bassman, L.C.; Badger, M.A.; Pierson, A.N.; Biewener, A.A. Pigeons steer like helicopters and generate down and upstroke lift during low speed turns. Proc. Natl. Acad. Sci. USA 2011, 108, 19990–19995. [Google Scholar] [CrossRef] [PubMed]
- Chin, D.D.; Lentink, D. Flapping wing aerodynamics: From insects to vertebrates. J. Exp. Biol. 2016, 219, 920–932. [Google Scholar] [CrossRef] [PubMed]
- Knoller, R. Die Gesetze des Luftwiderstandes (The Laws of Wind Resistance); Flug-und Motortechnik: Wien, Austria, 1909; Volume 3, pp. 1–7. [Google Scholar]
- Betz, A. Ein beitrag zur erklaerung segelfluges. Z Flugtech Mot. 1912, 3, 269–272. [Google Scholar]
- Ashraf, M.A.; Young, J.; Lai, J.C.S. Oscillation frequency and amplitude effects on plunging airfoil propulsion and flow periodicity. AIAA J. 2012, 50, 2308–2324. [Google Scholar] [CrossRef]
- Yu, M.; Wang, Z.J.; Hu, H. High fidelity numerical simulation of airfoil thickness and kinematics effects on flapping airfoil propulsion. J. Fluid. Struct. 2013, 42, 166–186. [Google Scholar] [CrossRef]
- Koochesfahani, M.M. Vortical patterns in the wake of an oscillating airfoil. AIAA J. 1989, 27, 1200–1205. [Google Scholar] [CrossRef]
- Chang, X.; Zhang, L.; Ma, R.; Wang, N. Numerical investigation on aerodynamic performance of a bionic flapping wing. Appl. Math. Mech. 2019, 40, 1625–1646. [Google Scholar] [CrossRef]
- Bansmer, S.E.; Radespiel, R. Flapping flight: High thrust and propulsive efficiency due to forward gliding oscillations. AIAA J. 2012, 50, 2937–2942. [Google Scholar] [CrossRef]
- Wang, S.; Zhang, X.; He, G.; Liu, T. Lift enhancement by dynamically changing wingspan in forward flapping flight. Phys. Fluids 2014, 26, 061903. [Google Scholar] [CrossRef]
- Bahlman, J.W.; Swartz, S.M.; Breuer, K.S. How wing kinematics affect power requirements and aerodynamic force production in a robotic bat wing. Bioinspir Biomim. 2014, 9, 025008. [Google Scholar] [CrossRef]
- Wang, S.; Zhang, X.; He, G.; Liu, T. Lift enhancement by bats’ dynamically changing wingspan. J. R. Soc. Interface 2015, 12, 20150821. [Google Scholar] [CrossRef] [PubMed]
- Tobalske, B.W.; Dial, K.P. Flight kinematics of black-billed magpies and pigeons over a wide range of speeds. J. Exp. Biol. 1996, 199, 263–280. [Google Scholar] [CrossRef] [PubMed]
- Lee, T.; Gerontakos, P. Investigation of flow over an oscillating airfoil. J. Fluid. Mech. 2004, 512, 313–341. [Google Scholar] [CrossRef]
- Li, X.; Feng, L.H.; Li, Z.Y. Flow mechanism for the effect of pivot point on the aerodynamic characteristics of a pitching airfoil and its manipulation. Phys. Fluids 2019, 31, 087108. [Google Scholar] [CrossRef]

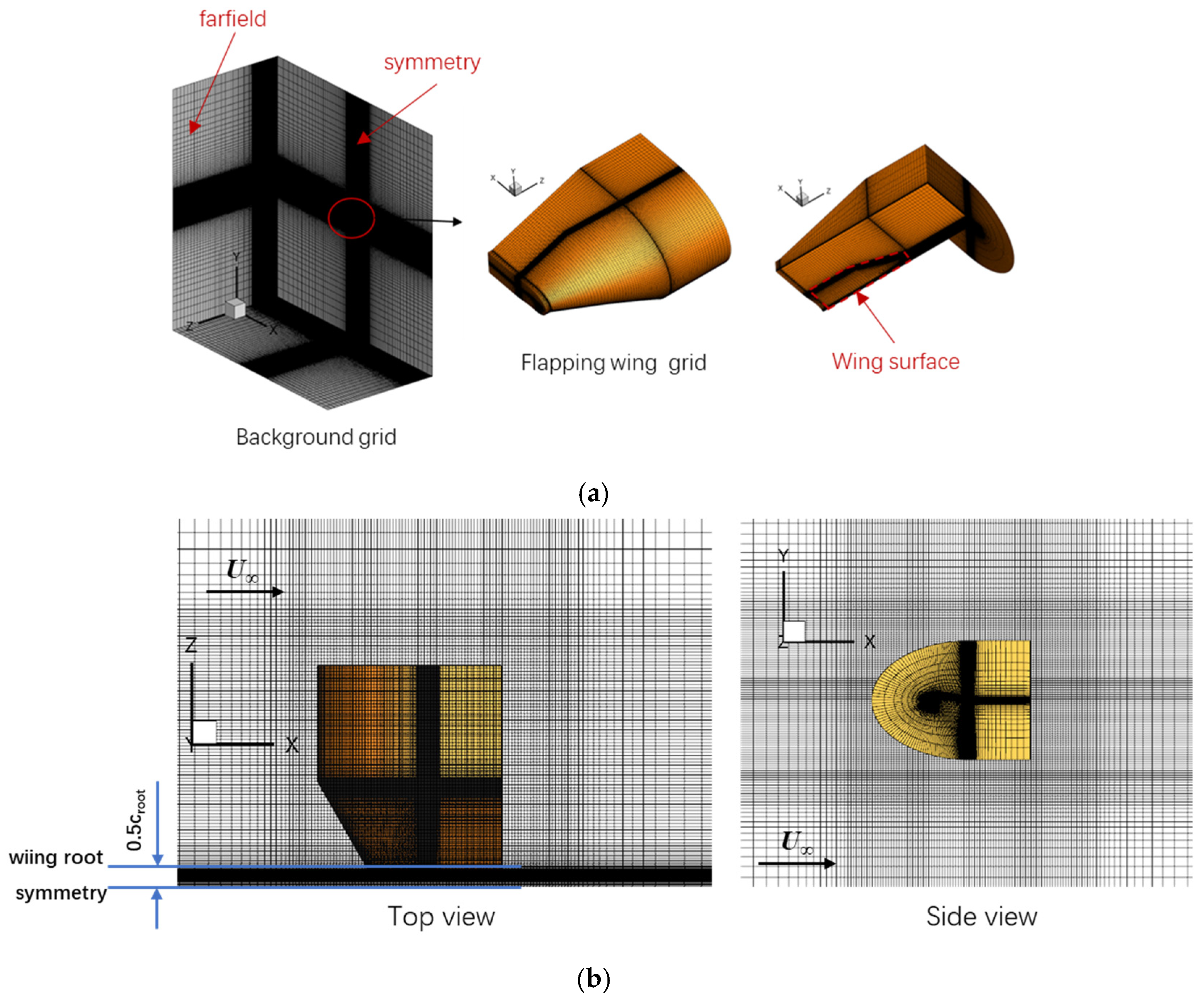
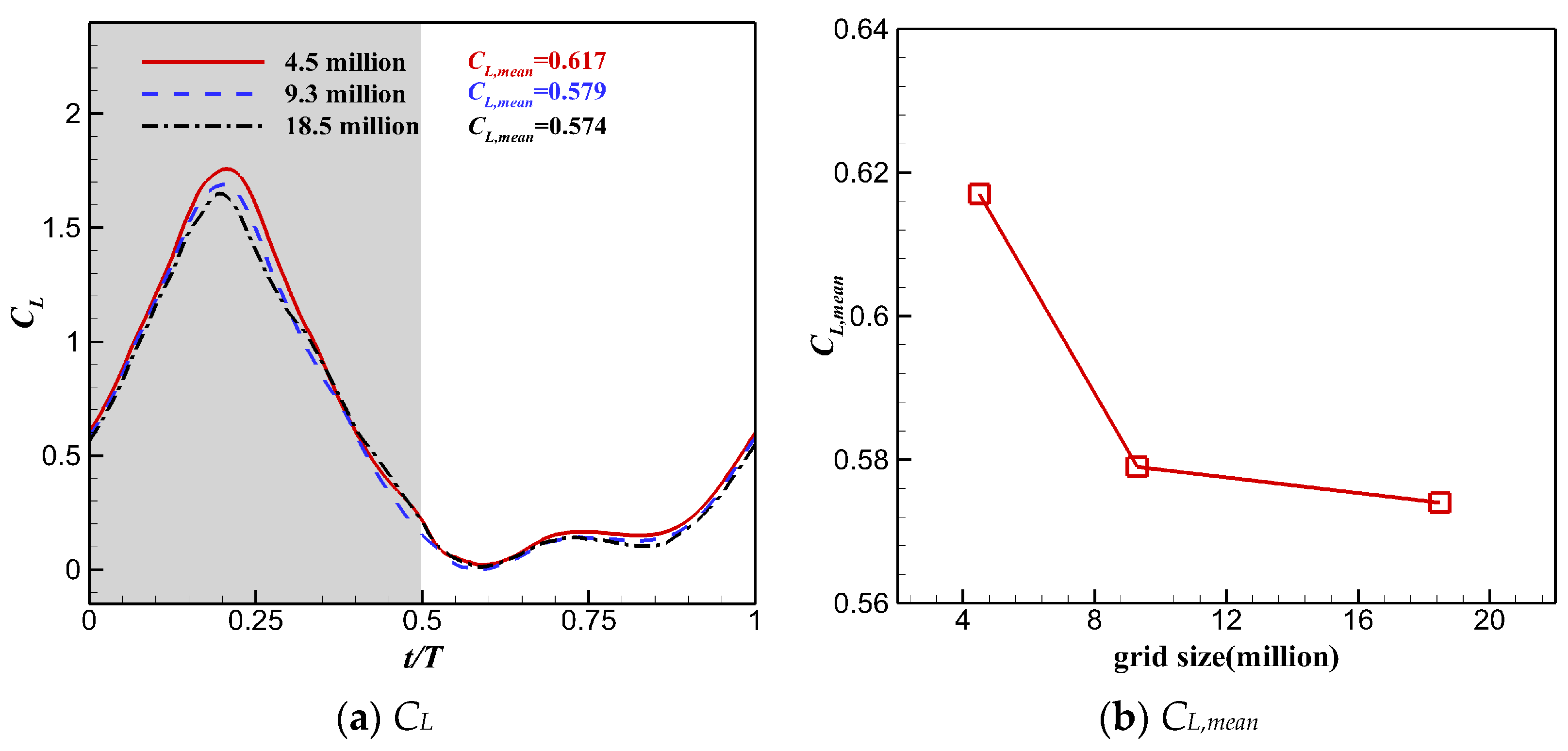
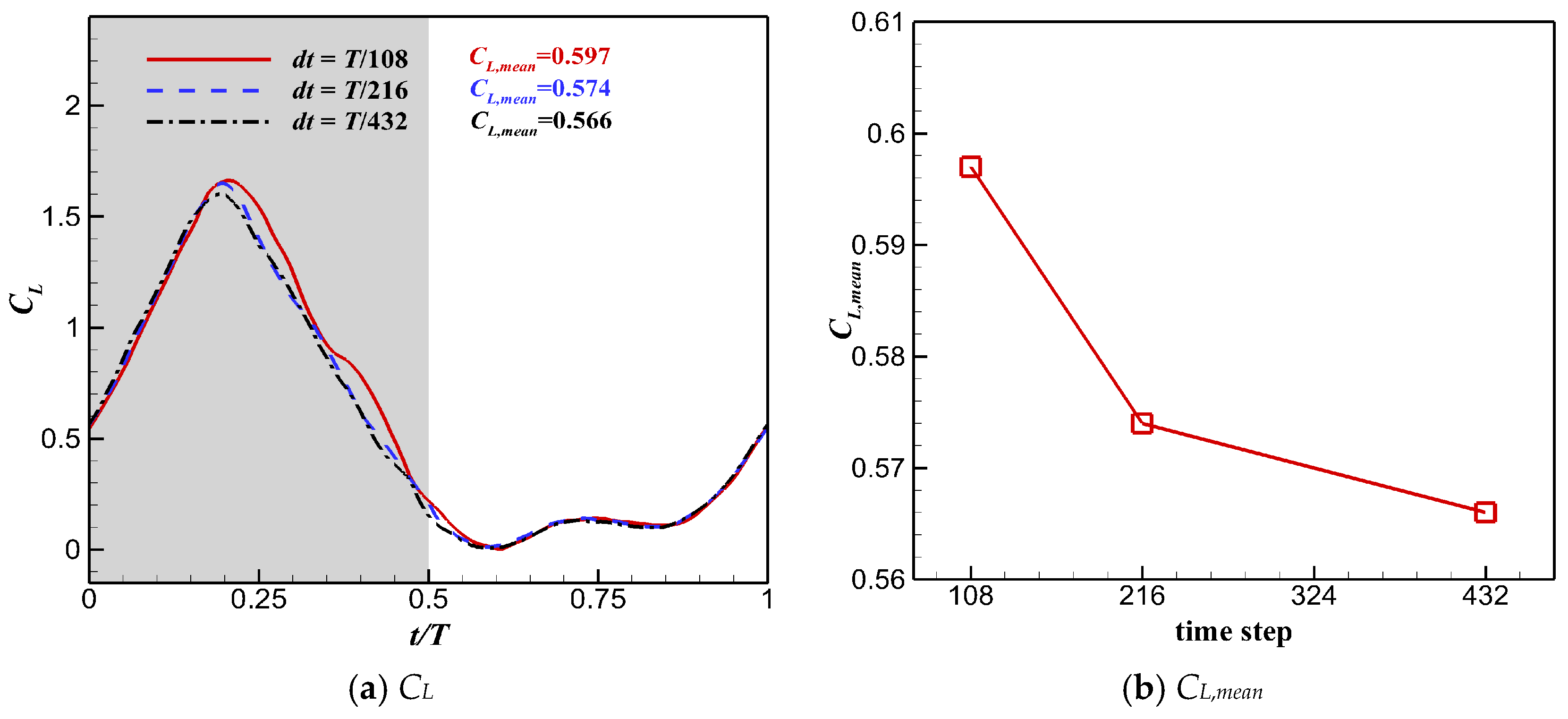




| Parameters | Far Field Length (c) | Grid Cell Number | Total Grid Volume |
|---|---|---|---|
| Coarse grid | 30 | 123 × 133 × 133 | 2.2 million |
| Medium grid | 30 | 145 × 155 × 205 | 4.6 million |
| Fine grid | 30 | 189 × 201 × 221 | 8.4 million |
| Parameters | Grid Cell Number | Total Grid Volume | First Grid Layer Spacing (c) | Spacing Increase Ratio |
|---|---|---|---|---|
| Coarse grid | 265 × 57 × 133 | 2.0 million | 3.5 × 10−4 | 1.1 |
| Medium grid | 321 × 81 × 151 | 3.9 million | 2.6 × 10−4 | 1.09 |
| Fine grid | 401 × 107 × 193 | 8.3 million | 1.8 × 10−4 | 1.08 |
| Parameter | CL,mean | CT,mean | CP,mean |
|---|---|---|---|
| Far field radius (c) | 0.574 | −0.1277 | 0.173 |
| Parameters | CL,mean | CT,mean | CP,mean | ƞL | ƞT |
|---|---|---|---|---|---|
| Without twisting | 0.576 | −0.1291 | 0.173 | 3.33 | −0.746 |
| With twisting | 0.458 | −0.0241 | 0.130 | 3.52 | −0.185 |
| Parameters | CL,mean | CT,mean | CP,mean | ƞL | ƞT |
|---|---|---|---|---|---|
| Without the sweeping motion | 0.375 | −0.0072 | 0.229 | 1.64 | −0.031 |
| With the sweeping motion | 0.458 | −0.0241 | 0.130 | 3.52 | −0.185 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wu, T.; Wang, K.; Jia, Q.; Ding, J. Effects of Wing Kinematics on Aerodynamics Performance for a Pigeon-Inspired Flapping Wing. Biomimetics 2025, 10, 328. https://doi.org/10.3390/biomimetics10050328
Wu T, Wang K, Jia Q, Ding J. Effects of Wing Kinematics on Aerodynamics Performance for a Pigeon-Inspired Flapping Wing. Biomimetics. 2025; 10(5):328. https://doi.org/10.3390/biomimetics10050328
Chicago/Turabian StyleWu, Tao, Kai Wang, Qiang Jia, and Jie Ding. 2025. "Effects of Wing Kinematics on Aerodynamics Performance for a Pigeon-Inspired Flapping Wing" Biomimetics 10, no. 5: 328. https://doi.org/10.3390/biomimetics10050328
APA StyleWu, T., Wang, K., Jia, Q., & Ding, J. (2025). Effects of Wing Kinematics on Aerodynamics Performance for a Pigeon-Inspired Flapping Wing. Biomimetics, 10(5), 328. https://doi.org/10.3390/biomimetics10050328