1. Introduction
Multi-legged robots offer superior flexibility and mobility in complex uneven-terrain environments inaccessible to wheeled or tracked vehicles [
1]. Compared to quadruped robots, hexapod robots have a higher stability and load capacity, although at the cost of certain aspects of their dynamic performance [
2]. Hydraulic hexapod robots (HHRs) exhibit superior force output capabilities, power-to-weight ratios, and control robustness through hydraulic actuation. These advantages make HHRs particularly suitable for executing heavy-load tasks and performing critical missions in disaster response scenarios. However, hydraulic actuation also introduces increased complexity and control challenges, lower energy efficiency, and higher energy consumption. Consequently, energy-saving strategies for hydraulic robots have become a critical area of research.
Many advanced hydraulic legged robots have been successfully researched and developed, such as Boston Dynamics’ Big Dog [
3]; IIT’s HyQ [
4], HyQ2Max [
5], and miniHyQ [
6]; Shandong University’s SCalf [
7,
8]; Chiba University’s COMET [
9,
10]; NTUA’s HexaTerra [
11]; and SJTU’s Baby Elephant [
12], JINPOONG [
13], LSHDSL-robot [
14], and MBBOT [
15]. While hydraulic legged robots exhibit superior force output and power-to-weight ratios, their energy conversion efficiency remains markedly inferior to their biological counterparts of comparable masses under most working conditions [
16].
At present, three primary methods are employed to reduce the energy consumption of hydraulic legged robots: (1) the optimization of gait and foot trajectories; (2) the optimization of their mechanical structure; and (3) the optimization of their hydraulic actuators and hydraulic system architecture.
Optimization of gait and foot trajectories: Yang et al. [
17] developed a model incorporating both mechanical power and heat rate for the SCalf-III. By optimizing the foot trajectory with the objective of energy efficiency, they achieved a 7.55% reduction in the robot’s energy consumption. Hua et al. [
18] proposed an energy-efficient gait based on the segmented spline interpolation curve, enabling the SCalf-II to maintain low energy consumption on unknown terrain. Tani et al. [
19] developed a novel approach to the trajectory design of hydraulic legged robots by incorporating the properties of limited-power hydraulic pumps. This method effectively improves energy utilization while ensuring better power matching. Optimization of the mechanical structure: Zhai et al. [
20] proposed an archive-based micro genetic algorithm (AMGA) to optimize mechanical structure and gait parameters, resulting in a 40% reduction in energy consumption compared to the original structure. Liu et al. [
21] presented a switchable parallel elastic actuator design for the monopedal switchable parallel elastic actuator robot (SPEAR), which adjusts the stiffness of the parallel elastic actuators to enhance energy efficiency without compromising mobility. Yin et al. [
22] designed an origami-based decoupling clutch for energy-efficient legged robots, which switches the connection between the parallel spring and the robotic leg without requiring additional control effort. This decoupling clutch reduces the required knee flexion torque and energy consumption by 37.6% and 31%, respectively. Mazumdar et al. [
23,
24] developed a novel parallel spring mechanism to enhance energy efficiency in bipedal robots. Optimization of the hydraulic actuators and hydraulic system architecture: Fan et al. [
25,
26] proposed an unpowered hydraulic auxiliary system to enhance the loading capability and energy efficiency of legged robots. This system provides continuous support force to assist the knee joint actuator in bearing the load without consuming additional energy, resulting in a 16.9% improvement in energy efficiency during walking for a bipedal hydraulic-assisted electric leg prototype (HyELeg). Both Xue et al. [
27] and Hua et al. [
28] have developed multi-pressure hydraulic systems for hydraulic quadruped robots to reduce energy consumption. Xue et al. [
29] proposed a novel variable-effective-area cylinder that reduces overall machine energy consumption by reducing the throttling loss and allowing energy recovery. Hua et al. [
30] developed a hydraulically compliant servo actuator (HPCA) to enhance energy efficiency by utilizing the characteristics of the pressure-transition dynamics of an asymmetric hydraulic cylinder with a symmetrical valve configuration.
To improve the low energy efficiency of hydraulic legged robots, this article designs a hydraulic hexapod robot equipped with a two-level pressure system (TPS) with separate-meter-in and separate-meter-out (SMISMO) configurations. Previous studies have investigated the control performance of robotic joints by employing the TPS [
31]. Compared to the traditional one-level pressure system (OPS) commonly employed in hydraulic robots, the TPS comprises high-pressure and low-pressure oil sources, which supply the actuator with the required pressure, which is based on load requirements. Both simulation and experimental results demonstrate that the TPS exhibits superior energy efficiency and an adequate control performance compared to the OPS, confirming that the TPS can replace the OPS to achieve stable and energy-efficient robotic motion.
In summary, the main contributions of this article are as follows: (1) the proposal of the TPS, inspired by mammalian energy supply mechanisms, to improve energy efficiency in hydraulic robots and (2) the verification of the TPS’s energy-saving and control performance, which demonstrates that the TPS can replace the OPS to enable stable and energy-efficient robotic motion.
The remainder of this article is structured as follows:
Section 2 analyzes the energy transfer process and energy loss of the robot.
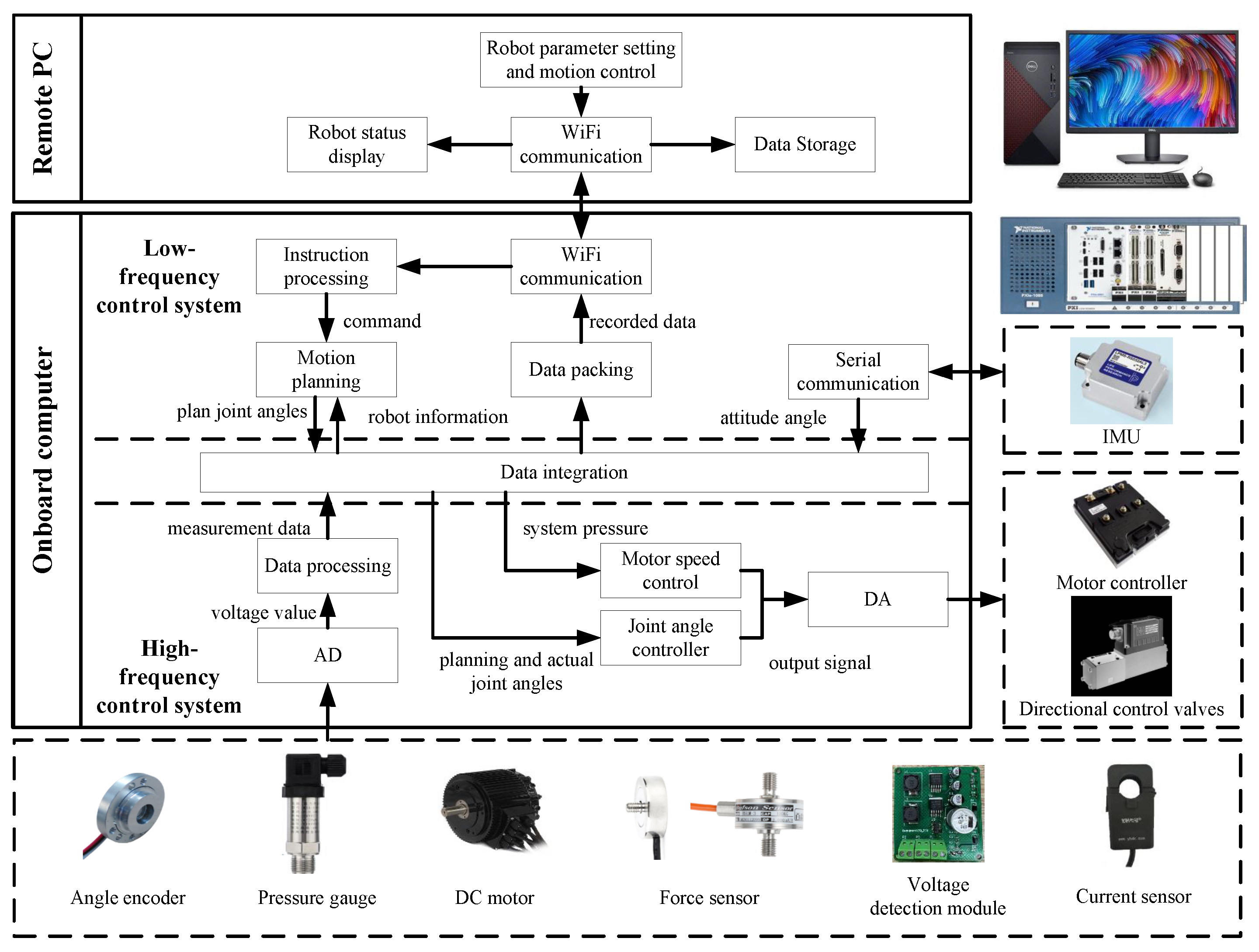
Section 3 introduces an overview of the HHR, including its mechanical structure, hydraulic system architecture, and control system configuration.
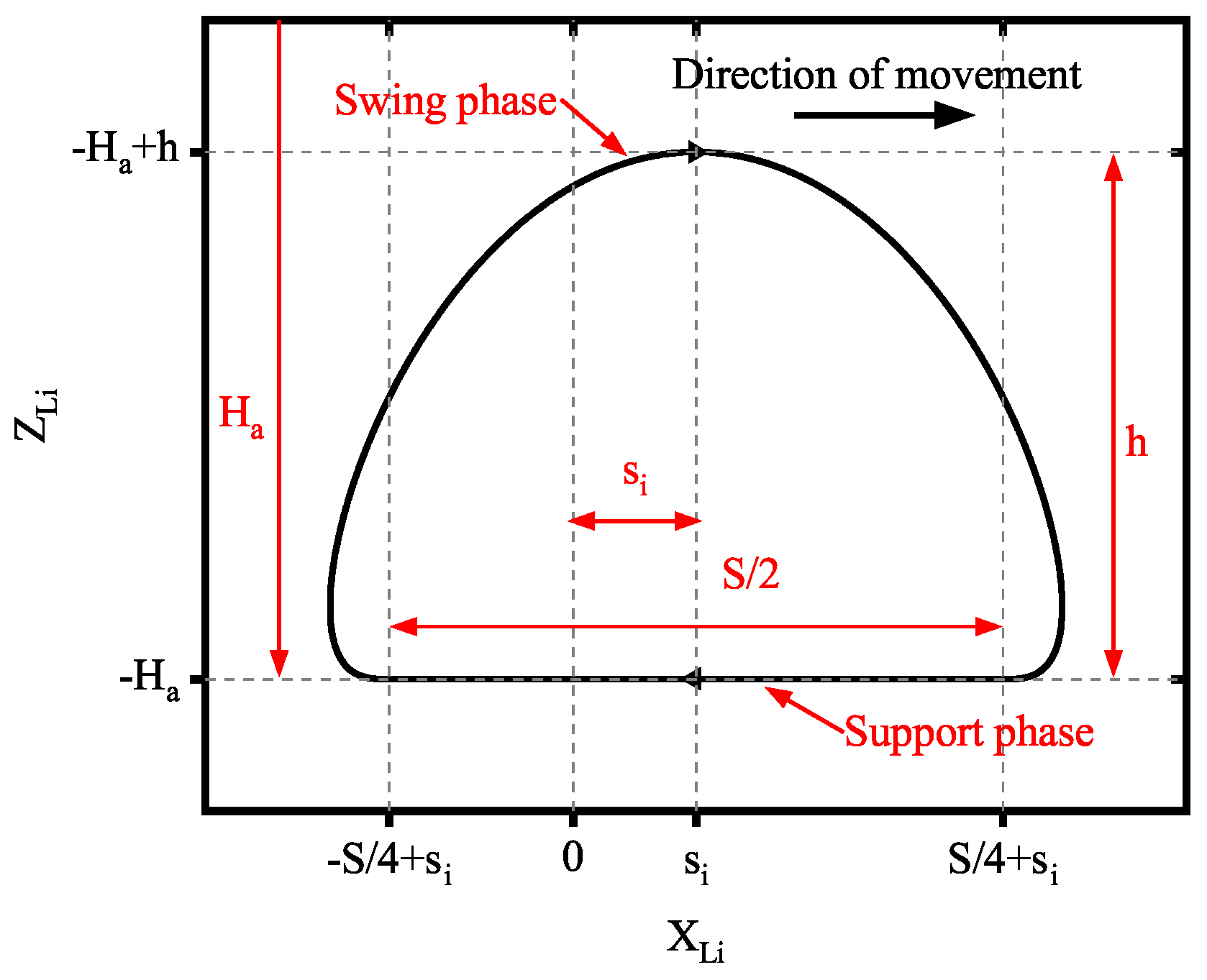
Section 4 establishes the kinematics model and hydraulic model of the HHR.
Section 5 presents the energy-saving and control performance of both hydraulic systems through simulation and experimental results.
Section 6 concludes this article.
2. Energy Consumption Analysis
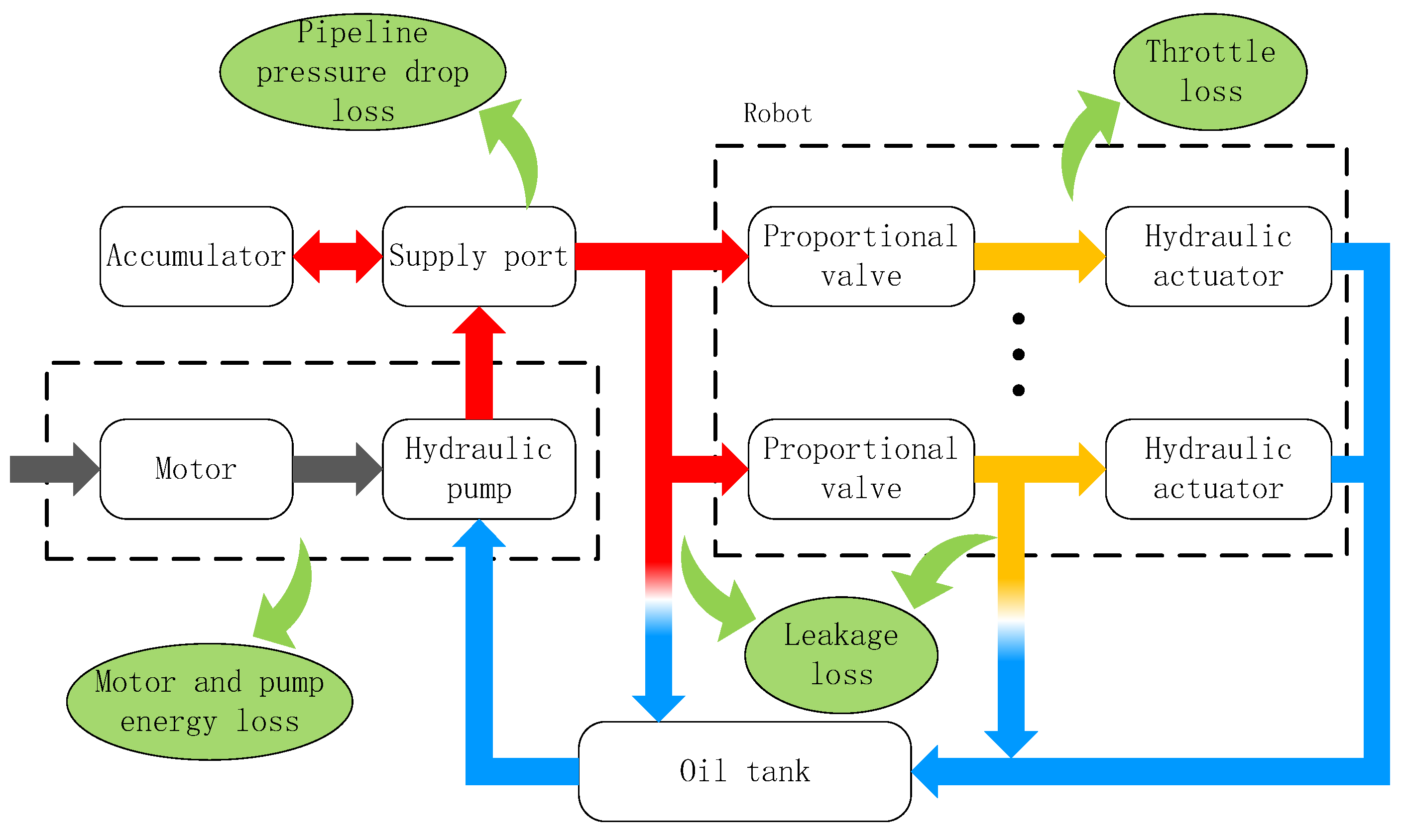
In hydraulic legged robots, the hydraulic pump initially transforms mechanical energy into hydraulic fluid energy, which is subsequently converted back to mechanical energy through actuator systems. As illustrated in
Figure 1, these energy conversion processes result in various forms of power dissipation. Prior to implementing energy efficiency optimization, a critical analysis is required to identify the primary loss mechanisms and assess their potential for energy saving. For analytical clarity, these losses are quantified in terms of power rather than cumulative energy. The total energy loss can be calculated by integrating the power loss over the duration of operation.
2.1. Motor and Pump Energy Loss
The sample manual provides the efficiency of the motor and pump, as well as the leakage coefficients of the hydraulic components. Therefore, the motor and pump energy loss can be calculated as follows:
where
is the comprehensive efficiency of the motor and the pump and
and
are the system’s pressure and flow.
2.2. Pipeline Pressure Drop Loss
In the valve control system, the oil flowing through the pipeline induces a pressure drop, resulting in energy loss. Assuming the pipeline specifications are the same, the pipeline pressure drop loss can be expressed as follows:
where
is the pressure drop;
is the fluid resistance coefficient;
and
d are the length and diameter of the pipeline, respectively;
and
v are the flow rate and velocity of the oil through the pipeline; and
is the oil’s density.
2.3. Leakage Loss
Proportional valves exhibit inevitable internal leakage caused by mechanical clearance. For the ith valve, the leakage flow
demonstrates proportionality to both the valve leakage coefficient
and the pressure differential
across the valve. The total leakage loss is calculated as follows:
2.4. Throttle Loss
In valve control systems, the spool displacement of proportional valves is regulated to supply the actuator with the required flow rate. However, a small displacement of the valve spool typically results in a pressure drop, leading to throttle loss. The throttle loss can be quantified based on the relationship between the required mechanical power for robotic motion and the hydraulic power transmitted through the valve. The throttle loss can be expressed as follows:
where
representw the flow rate supplied to the ith actuator via the valve and
and
represent the corresponding actuator’s load force and piston velocity, respectively.
2.5. Total Power Loss
The main types of power loss seen during the energy transfer process have been introduced. Based on Formulas (
1)–(
4), the total power loss can be expressed as follows:
The partial parameters in the formula correspond to fundamental hardware properties. The simplified expression, after incorporating these parameters, can be expressed as
where
represents the motor and pump loss coefficient;
represents the pipeline pressure drop loss coefficient;
represents the leakage loss coefficient; and
represents the power required for hydraulic actuator movement.
The formula indicates that enhancing the performance of the robot’s drive equipment, control valves, and actuators can reduce unnecessary losses. However, most research cannot balance energy efficiency and cost performance. Furthermore, achieving high integration and performance in the design of hydraulic components remains a significant challenge. Therefore, most research focuses on the impact of mechanical structures, hydraulic system design, and motion planning on the energy consumption of robots, focusing on the reduction of the flow rate supplied to the actuator and the power required for the robot to move.
This article focuses on system pressure and reduces throttling losses by optimizing the hydraulic system structure to match the system pressure to the actuator’s required pressure. It also proposes a TPS with SMISMO configurations, which can enhance robot energy efficiency by reducing throttling loss.
Compared to traditional hydraulic systems, this system can provide high-pressure or low-pressure oil sources to the hydraulic actuators based on the force conditions of the robot leg, which improves the robot’s pressure matching under different conditions. The specific robot model and hydraulic system model used will be introduced in the following sections.
5. Results and Discussion
5.1. Simulation
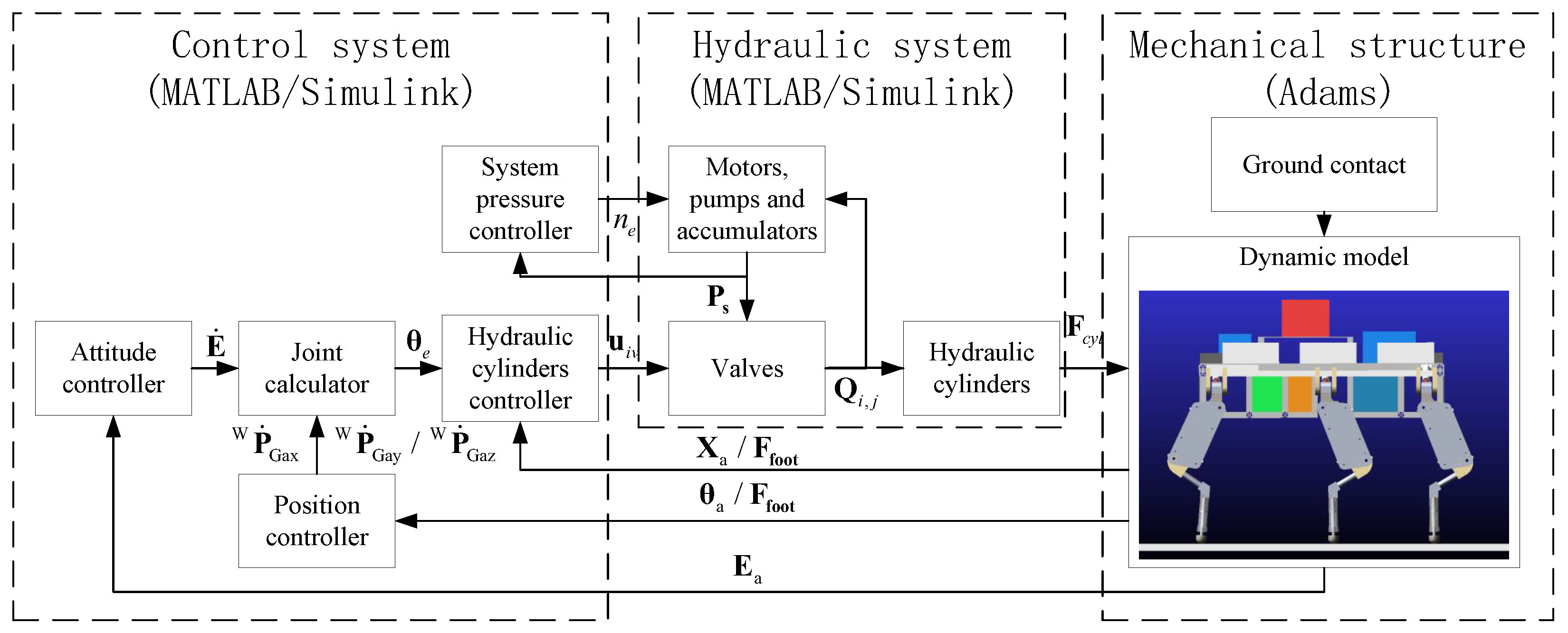
The HHR’s co-simulation architecture includes the control system, hydraulic system, and mechanical model. The control system and hydraulic system were developed in MATLAB/Simulink, while the mechanical model was built in Adams. The schematic of this co-simulation architecture is provided in
Figure 8.
The interface for the interaction between Adams and MATLAB/Simulink is encapsulated in an adams_sub module. This module transmits the output forces of the hydraulic system as inputs to Adams while returning the parameters of the robot simulation dynamics as output to MATLAB/Simulink. The hydraulic system parameters are shown in
Table 3. In the mechanical model, the proper configuration of the ground contact model is crucial, as it directly influences the foot contact force and impacts the motion of the HHR. The relevant parameters are listed in
Table 4.
In the simulation, the high pressure and low pressure of the TPS are 16 MPa and 8 MPa, respectively, while the system pressure of the OPS is 16 MPa. The robot moves using a preplanned tripod gait at a speed of 0.05 m/s and with a cycle time of 10 s. The total simulation time is 60 s, with the first 20 s allocated for robot posture adjustment, followed by 40 s of constant-speed linear motion.
Figure 9 shows the power consumption of the robot when it is equipped with the OPS or the TPS during the simulation. And
Figure 10 presents the calculated, based on Formula (
25), and simulated power of the robot, when equipped with the two different hydraulic systems, within a single gait cycle. Based on the simulation results, the average power of the robot during constant-speed linear motion can be calculated. The average power of the robot with the TPS is 1.079 kW, representing a 39.13% reduction compared to the 1.77 kW average power needed with the OPS. Additionally, the peak power of the robot is reduced by 41.3% with the TPS compared to the OPS.
Compared to the OPS, the TPS offers high-pressure and low-pressure oil sources, enhancing energy efficiency by reducing throttling loss. However, using two-level pressure of the oil sources inevitably results in pressure fluctuations during valve switching, which can impact the robot’s control accuracy. From an energy-saving perspective, a slight reduction in control precision is acceptable to enhance the robot’s energy efficiency. The simulation results demonstrate that the TPS shows a superior energy-saving performance compared to the OPS, necessitating a comparison of the control performance of the two hydraulic systems.
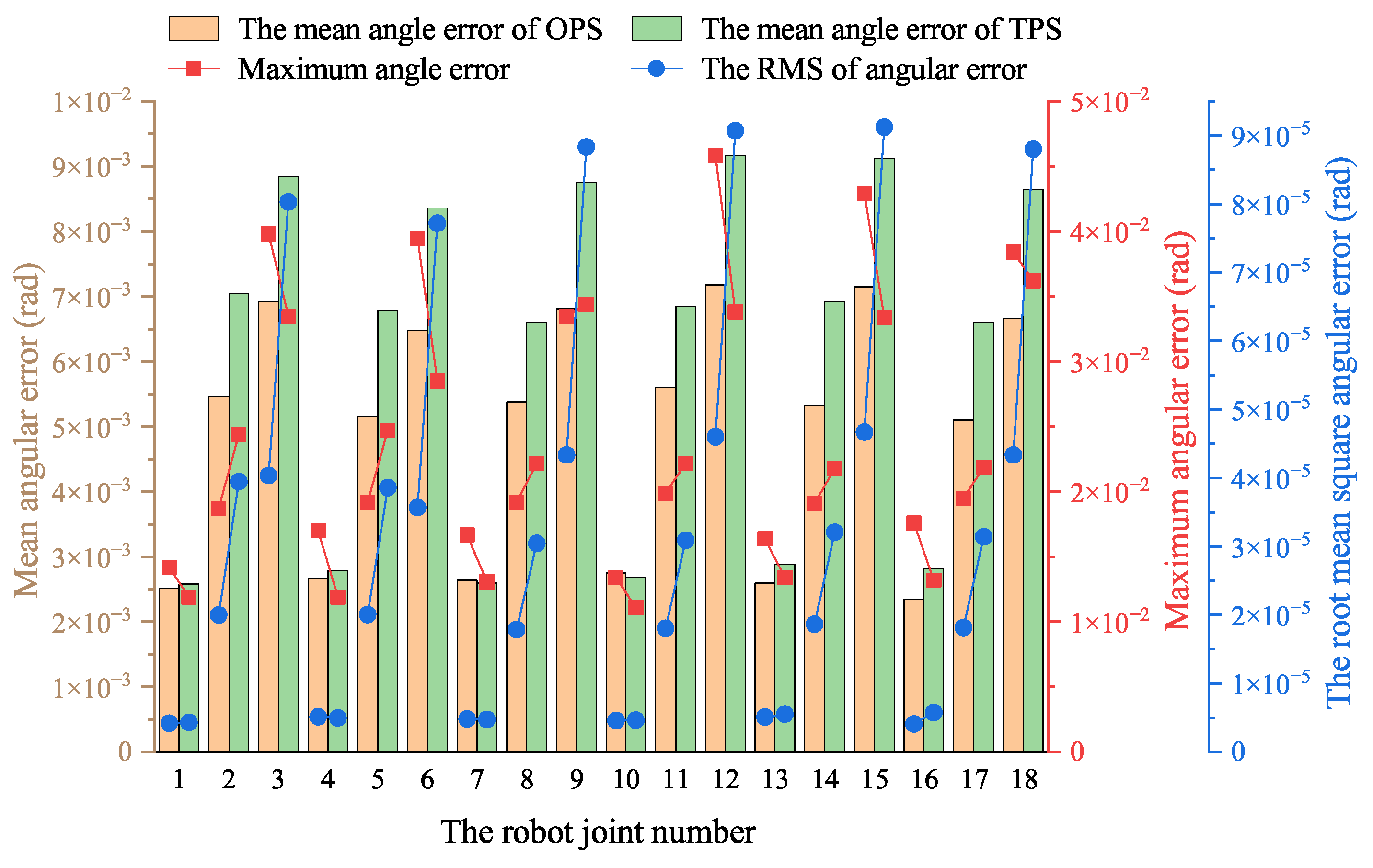
Figure 11 illustrates the mean angular error, maximum angular error, and root mean square (RMS) of the joint angle error of the robot when equipped with the OPS or TPS in simulation.
As shown in
Figure 11, the mean joint angle errors in the TPS are generally higher than those in the OPS, while the maximum error varies. Considering potential contingencies in the simulation, these results indicate that the control performance of the two hydraulic systems is comparable and within an acceptable range. Additionally, the RMS of the angular error is greater in the TPS than in the OPS, suggesting that pressure surges during the switching between the two different-pressure oil sources affect the actuator’s control accuracy, leading to minor fluctuations in the joint angles.
By analyzing the power consumption and control performance of the two hydraulic systems, it is demonstrated that, compared to the traditional OPS, the TPS reduces throttling loss when the robot moves while maintaining acceptable control accuracy not compromising its motion performance. The TPS enhances the robot’s energy efficiency and reduces the power consumed during movement.
5.2. Experiment
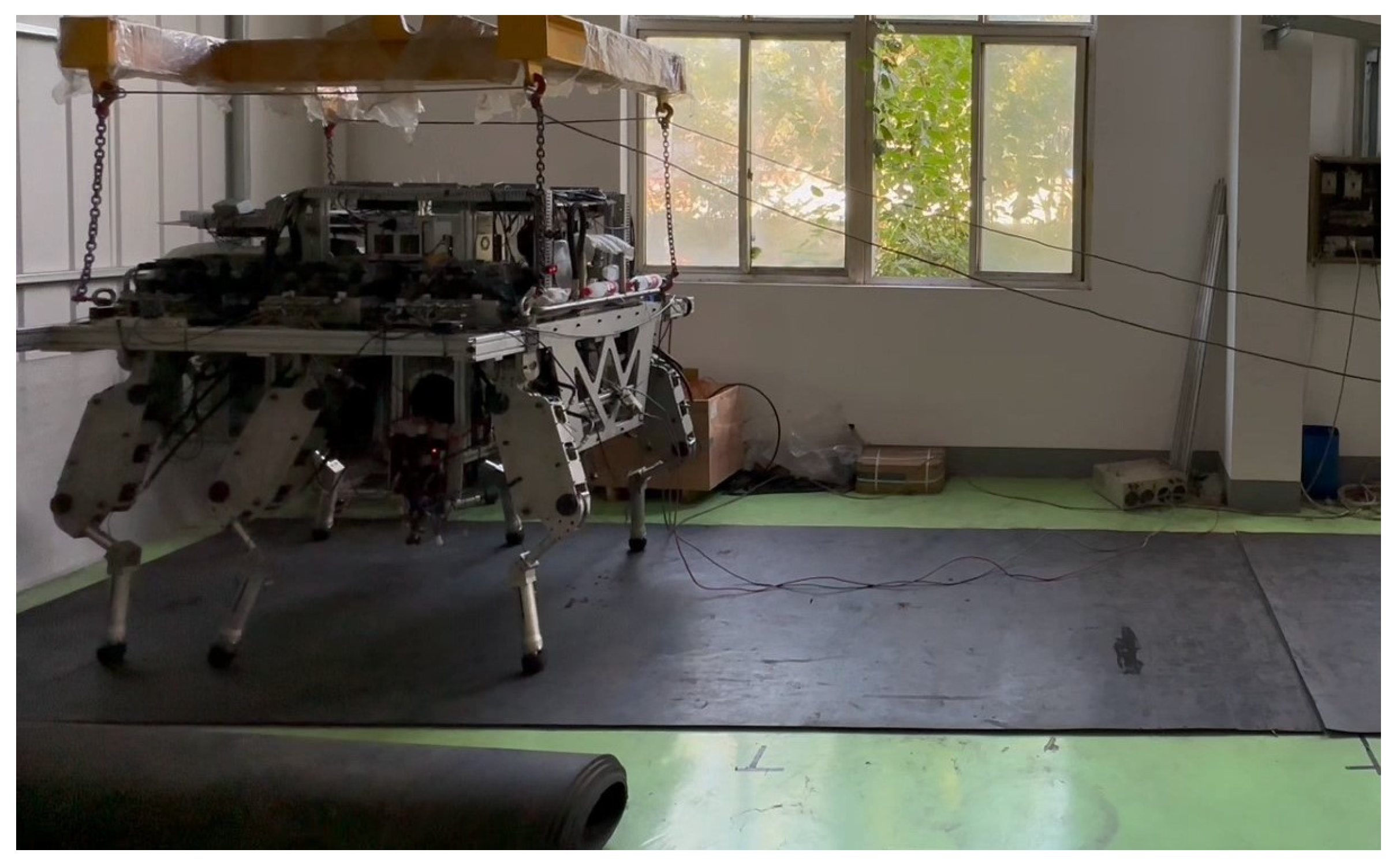
To further validate the energy-saving performance of the proposed TPS, a physical experiment is conducted on an HHR equipped with the TPS.
Figure 12 shows the experimental setup and environment of the HHR; the robot traversed from the left side to the right side of the test area. The structure of the HHR used in this experiment is outlined in
Section 3. To reduce experimental costs, industrial-grade motors and proportional directional valves were employed, which may create certain performance limitations. Nevertheless, this also demonstrates that the TPS has relatively low hardware requirements, as it can achieve a satisfactory energy-saving performance even with hardware of limited capabilities. In the experiment, the robot’s OPS employed a traditional one-level pressure SMISMO hydraulic system, similar to the proposed TPS described in this article. The two hydraulic systems can be switched by changing the number of corresponding proportional valves. Additionally, the gait parameters used in the experiment are the same as those in the simulation.
Consistent with the simulation, the system pressure for the OPS used in the experiment was set to 16 MPa, while the TPS’s system pressures were set to 16 MPa and 8 MPa. To minimize the influence of structural and control factors on the robot’s motion, a preplanned tripod gait was employed to maintain constant-speed movement without adjustment phases.
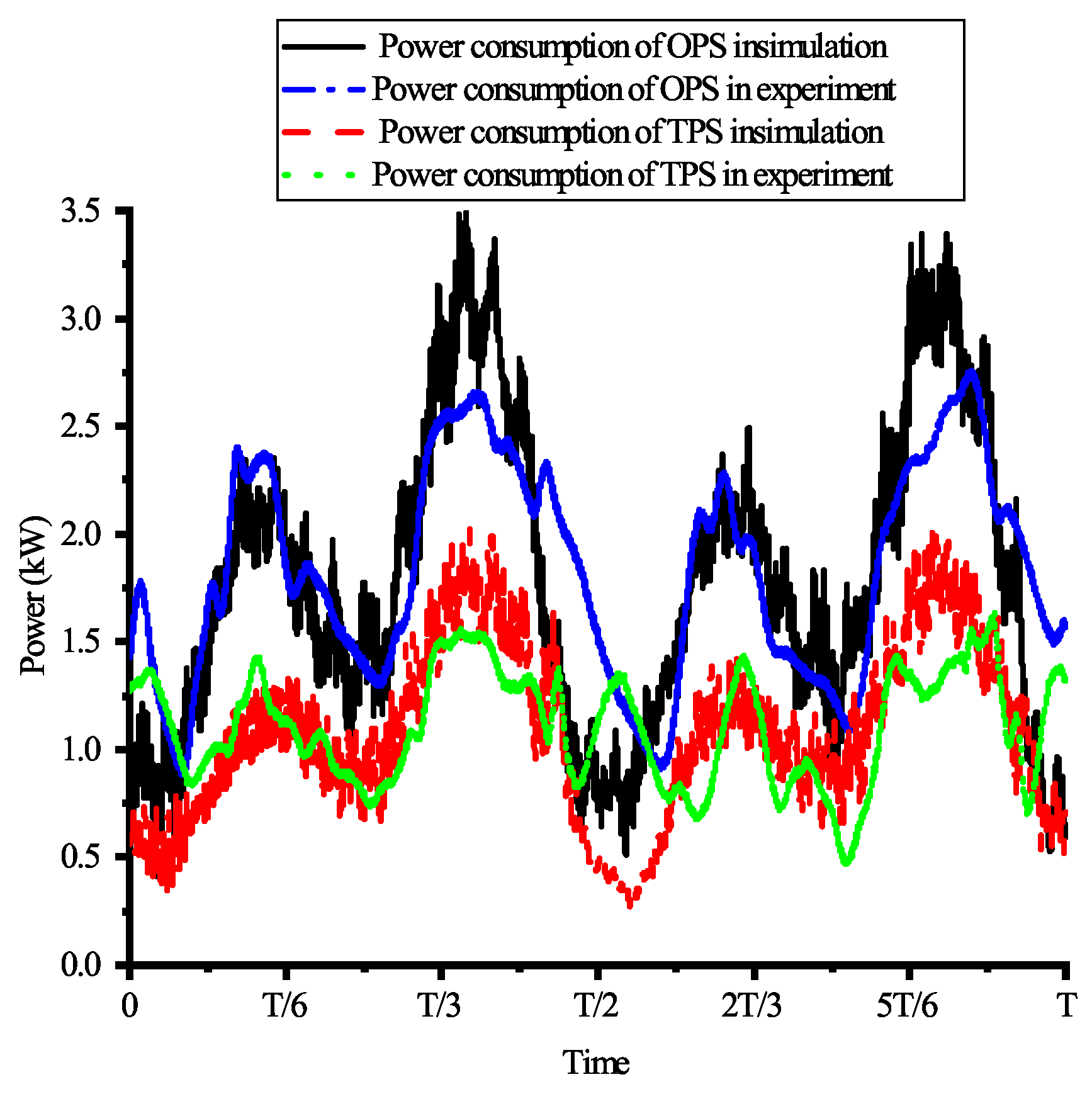
As illustrated in
Figure 13, the robot’s motion power is calculated based on the motor’s input voltage and current, which include all power consumption losses described in Formula (
5). Experimental data indicate that the average movement power consumption of the robot is 11.7 kW and 8.3 kW with the OPS and TPS, respectively. Compared to the OPS, the power consumption of the TPS is consistently lower throughout the motion, resulting in a 28.8% reduction in average power consumption.
The results indicate that the robot’s power consumption in the experiment is significantly higher than in the simulation, although the trend in power variation is the same. This discrepancy is primarily due to the simplifications made in the simulation, where leakage loss and pipeline pressure drops are neglected. Additionally, the industrial-grade proportional valves used in the experimental hydraulic system exhibit non-negligible leakage. Furthermore, damping orifices were used in the hydraulic system to help control the robot root joints, further exacerbating leakage loss. These factors collectively resulted in the experimental power consumption being substantially higher than in the simulation.
This article primarily focuses on the impact of the throttling loss on the robot’s energy consumption; therefore, the following assumptions are made: (1) throttling losses are negligible when the robot is stationary; (2) the combined efficiency of the motor and pump remains constant; and (3) the leakage loss and pipeline pressure drop loss are essentially unchanged. Based on these assumptions, the robot’s power consumption, including its throttling loss, can be estimated using the equation below.
where
is the estimated power used for the robot’s movement;
is the robot’s power, calculated based on the motor’s input voltage and current; and
is the power used by the robot while standing.
Using the method described above, the power consumption of the robot is estimated for both hydraulic systems. The TPS demonstrated a power consumption of 0.98 kW, representing a 42.7% reduction compared to the 1.71 kW of power consumed by the OPS.
Figure 14 compares the estimated power consumption of both hydraulic systems in the experiment with their power consumption as simulated over one cycle. As illustrated in the figure, while there are discrepancies between the experimentally estimated power and the simulated power of the hydraulic systems, the overall trends remain consistent.
The experimental results indicate that the TPS significantly enhanced robotic energy efficiency by reducing throttling losses compared to the OPS. Given the TPS’s superior energy conservation characteristics, it is essential to evaluate both systems’ control performance to ensure they have comparable control capabilities.
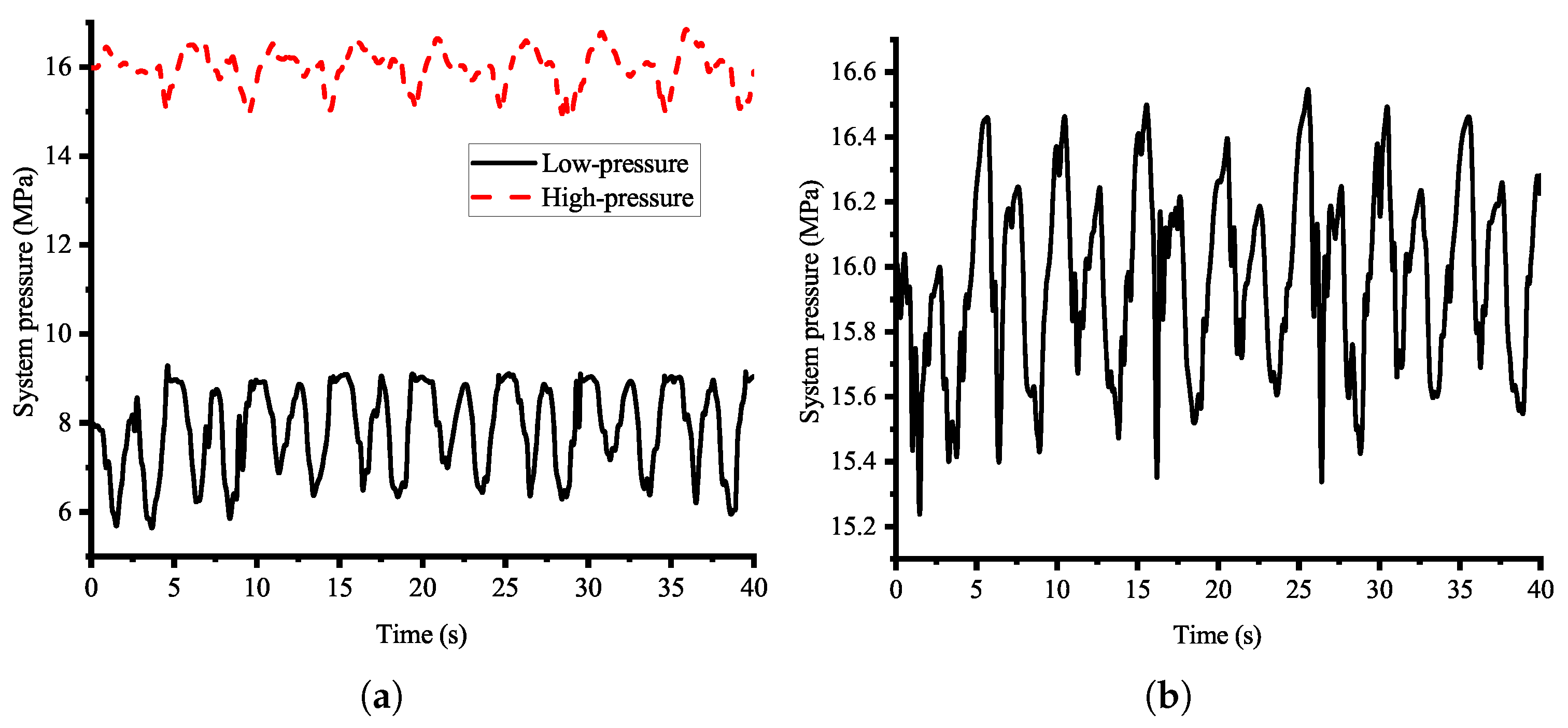
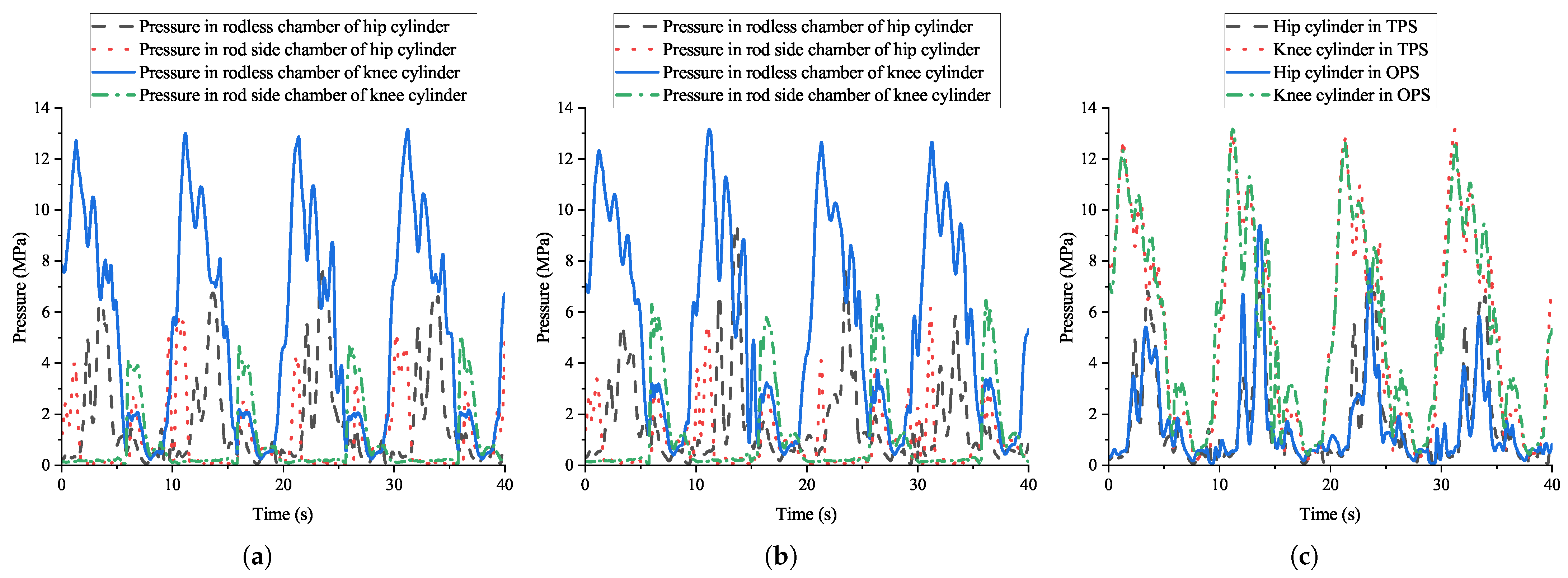
Figure 15 shows the system pressure variations of the TPS and OPS during the robot’s motion. The TPS’s average system pressures are 15.99 MPa and 7.89 MPa, respectively, while that of the OPS is 15.97 MPa. Both hydraulic systems exhibited similar high-pressure fluctuations. The effects of the two hydraulic systems on pressure variations in both chambers of the hydraulic cylinders during motion were analyzed. For clarity and reliability, the left middle leg with the highest load was selected as the representative case.
Figure 16 shows the experimentally measured pressure in both chambers of the hip and knee joints’ cylinders. The results reveal that both chambers exhibit periodic pressure fluctuations with similar trends, and the period of these matches the 10 s gait cycle. This phase-synchronous fluctuation demonstrates comparable control characteristics between the two systems. These findings confirm that the pressure compatibility is enhanced when the cylinders are supplied by the low pressure of the TPS.
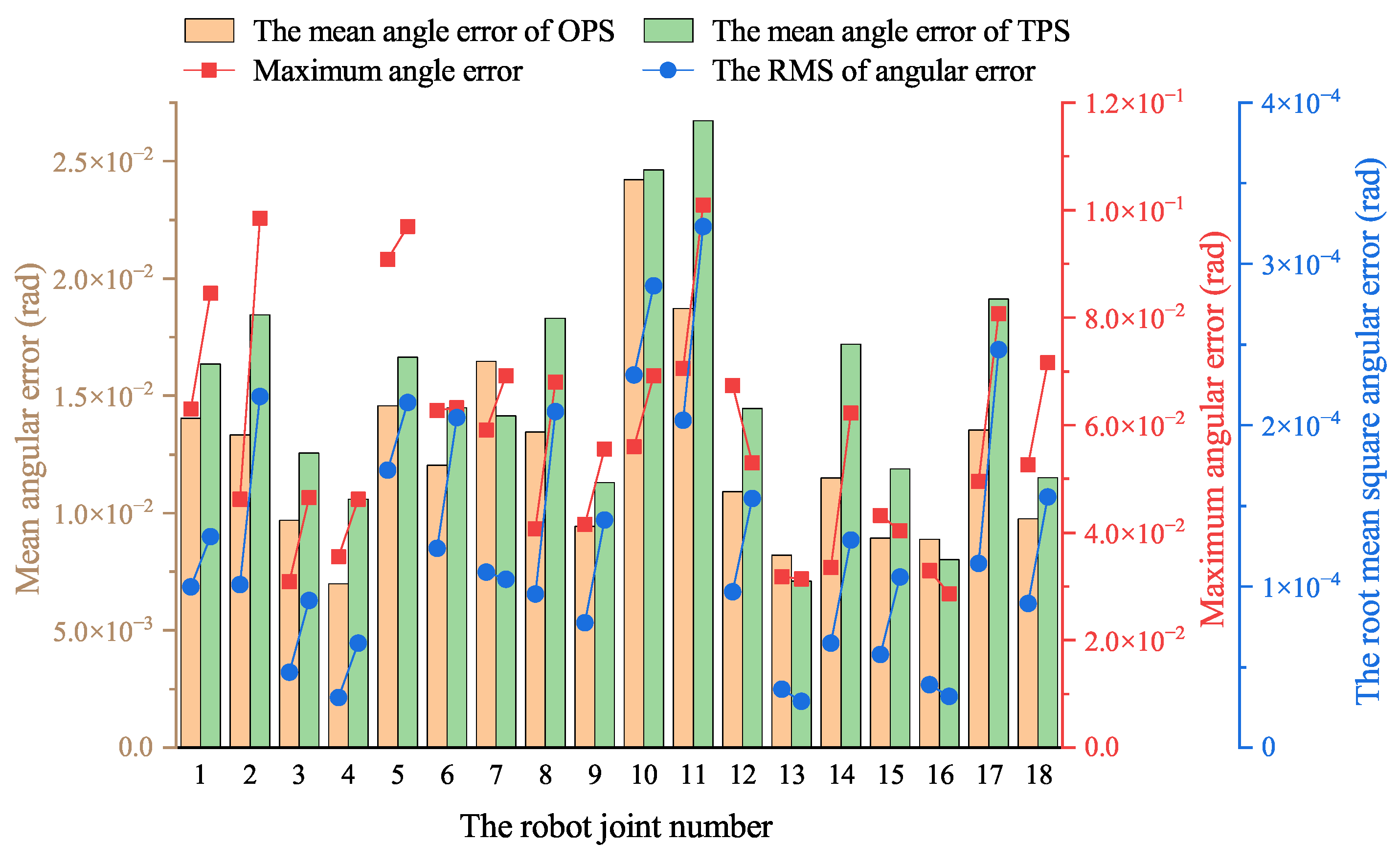
Figure 17 illustrates the mean angular error, maximum angular error, and RMS of the robot’s joint angular error for both the OPS and TPS as measured in the experiment, which leads to similar conclusions as those made from the simulation. As shown in the figure, the mean joint angular errors and RMS of the TPS are generally higher than those of the OPS, while the maximum error varies. Considering this experiment’s complexity and potential accidentally dissimilar conditions, these results indicate that the control performance of the two hydraulic systems is comparable and within an acceptable range.
In conclusion, the TPS demonstrates superior energy efficiency while maintaining a comparable control performance to that of the OPS. Therefore, the TPS can replace the OPS to achieve stable, energy-efficient robotic motion.
6. Conclusions
This article proposes a hydraulic system with two pressure levels to reduce the large throttling loss in traditional single-pressure multi-actuator hydraulic systems and comprehensively analyzes the energy-saving principles and efficiency of this hydraulic system. An overview of the mechanical structure, hydraulic system structure, and control system structure of a robot equipped with the energy-efficient two-pressure-level hydraulic system is given, as well as a kinematics model and hydraulic system model.
Compared to the traditional OPS, the proposed TPS can provide a high-pressure or low-pressure oil supply based on the load of the hydraulic actuators required to meet the robot’s motion, which reduces throttling loss by enhancing the matching between the system pressure and the actuator’s required pressure. The experimental and simulation results further confirm the energy-saving advantages of the TPS. In the simulation, the average power consumption of the robot with the TPS was 1.079 kW, representing a 39.13% reduction compared to the average 1.77 kW of power required by the OPS. Similarly, in the experiment, the TPS demonstrated a power consumption of 8.3 kW, which is a 28.8% reduction compared to the 11.7 kW of power consumed by the OPS. The significant difference in power consumption between the experimental and simulation results can primarily be attributed to the industrial-grade valves used in the experiment, which have considerable leakage loss. This loss was not accounted for in the simulation. From the results, it can be seen that the TPS exhibits pressure fluctuations during pressure switching, which may affect its control accuracy. However, the reduction in accuracy is minimal and within acceptable limits. Therefore, given its superior energy-saving performance, the TPS is well suited for use in heavy-load robots. Future work will focus on optimizing its mechanical structure, integrating hydraulic components, and reducing weight to minimize energy loss and enhance control accuracy during robot movement.