A Computational Study on the Hydrodynamics of Bio-Inspired Quadrupedal Paddling
Abstract
1. Introduction
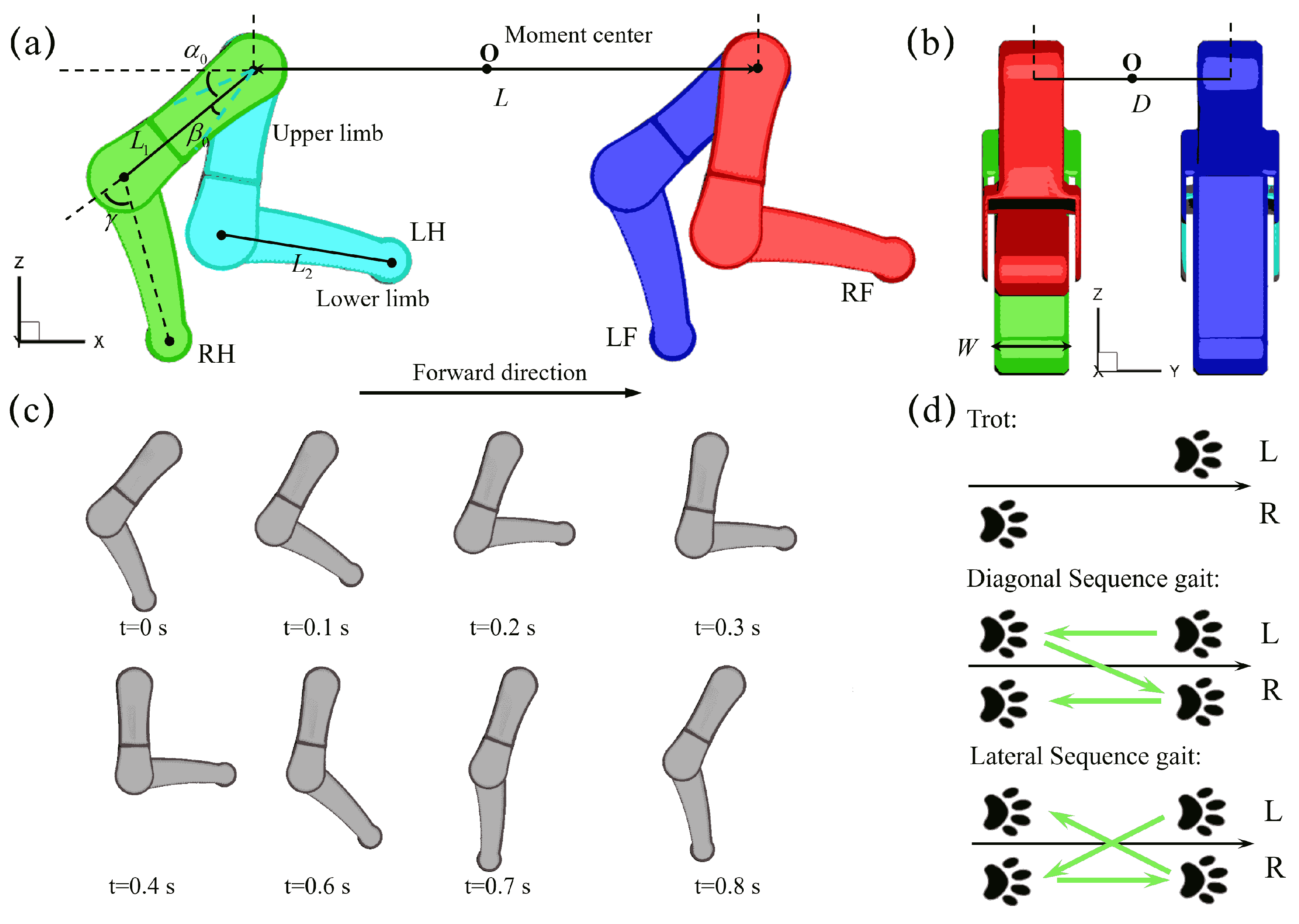
2. Model Description and the Numerical Approach
3. Results
3.1. Hydrodynamics of a Single Paddling Leg
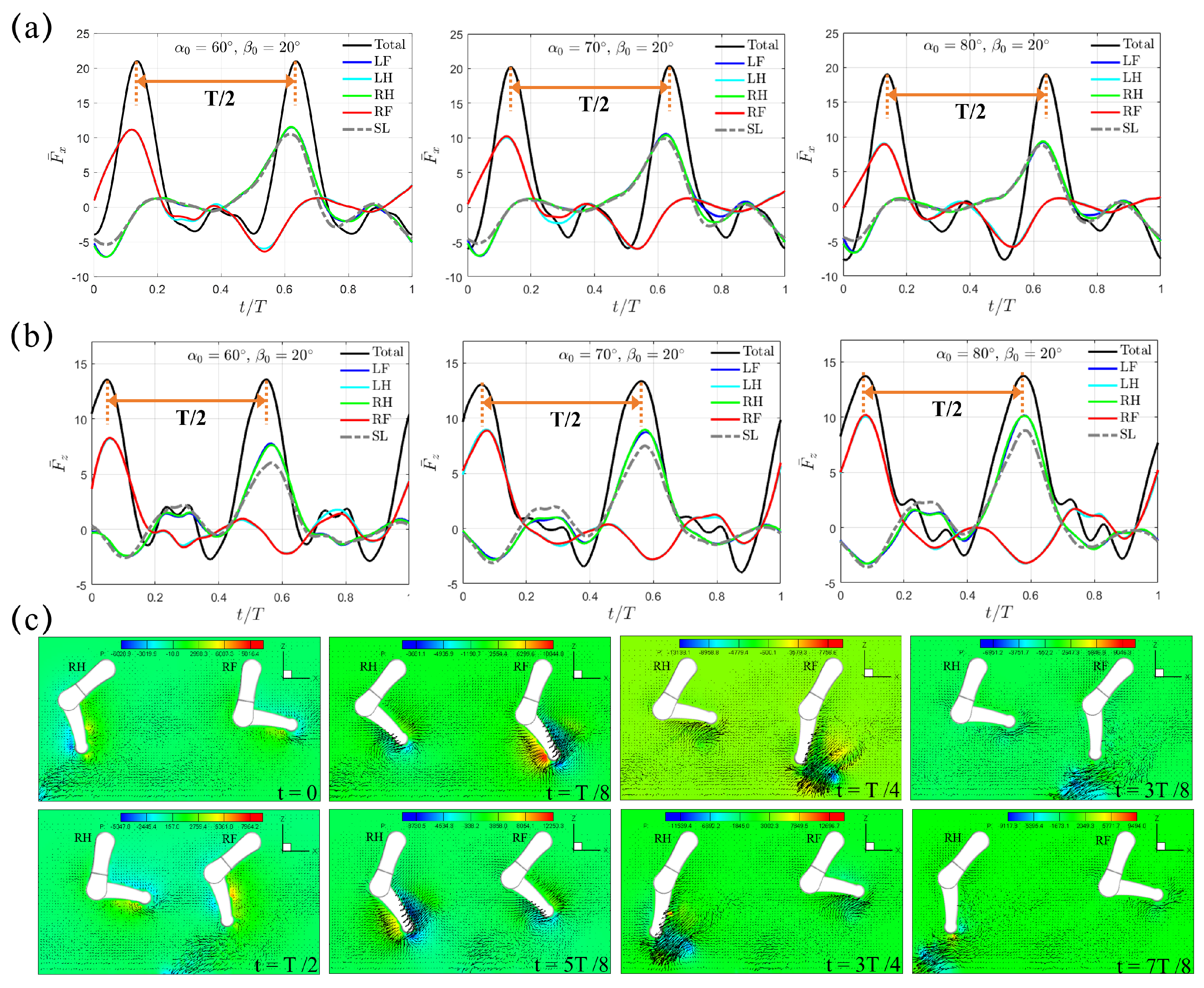
3.2. Quadrupedal Paddling Using the Trotting Gait
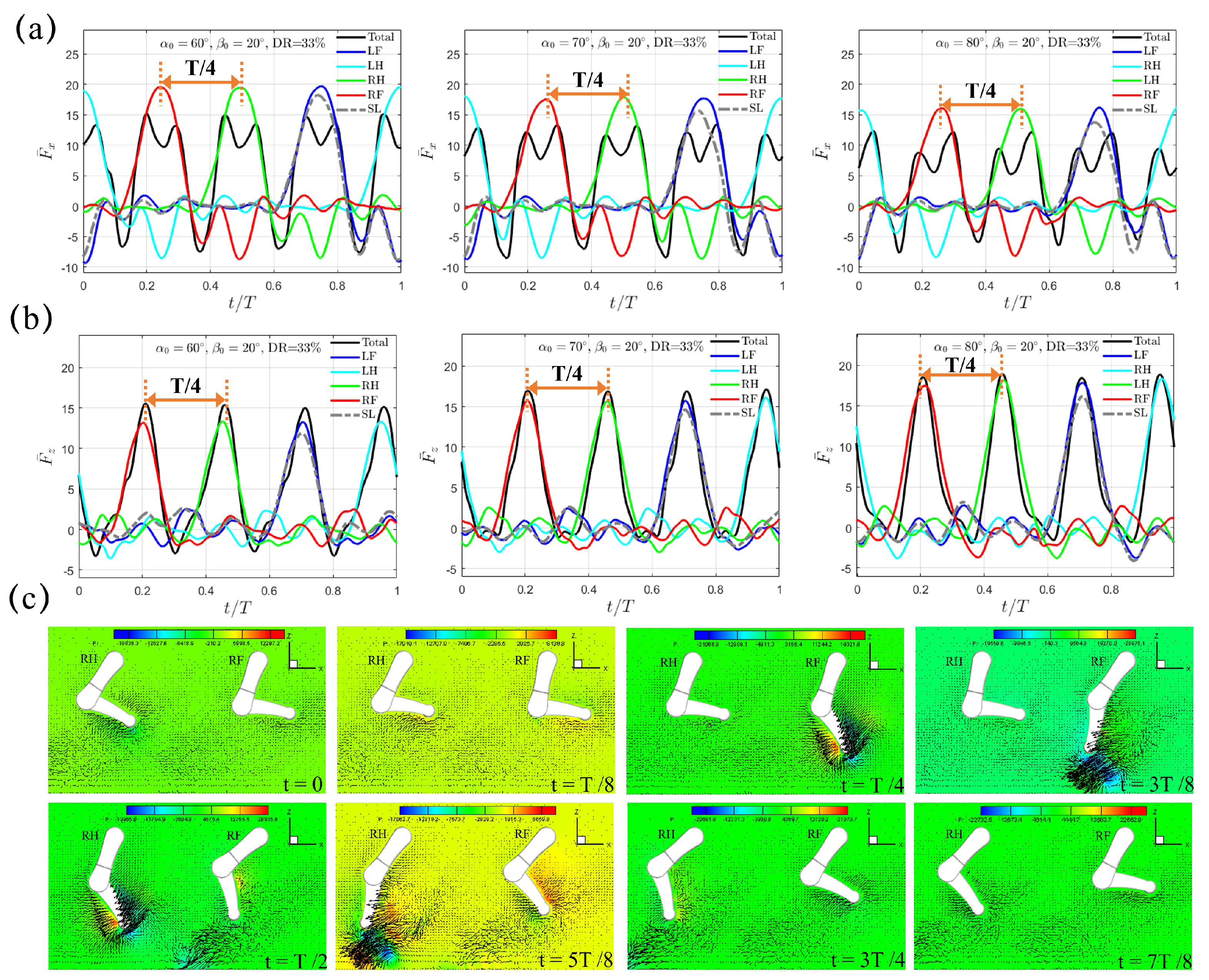
3.3. Quadrupedal Paddling Using the Diagonal Sequence Gait
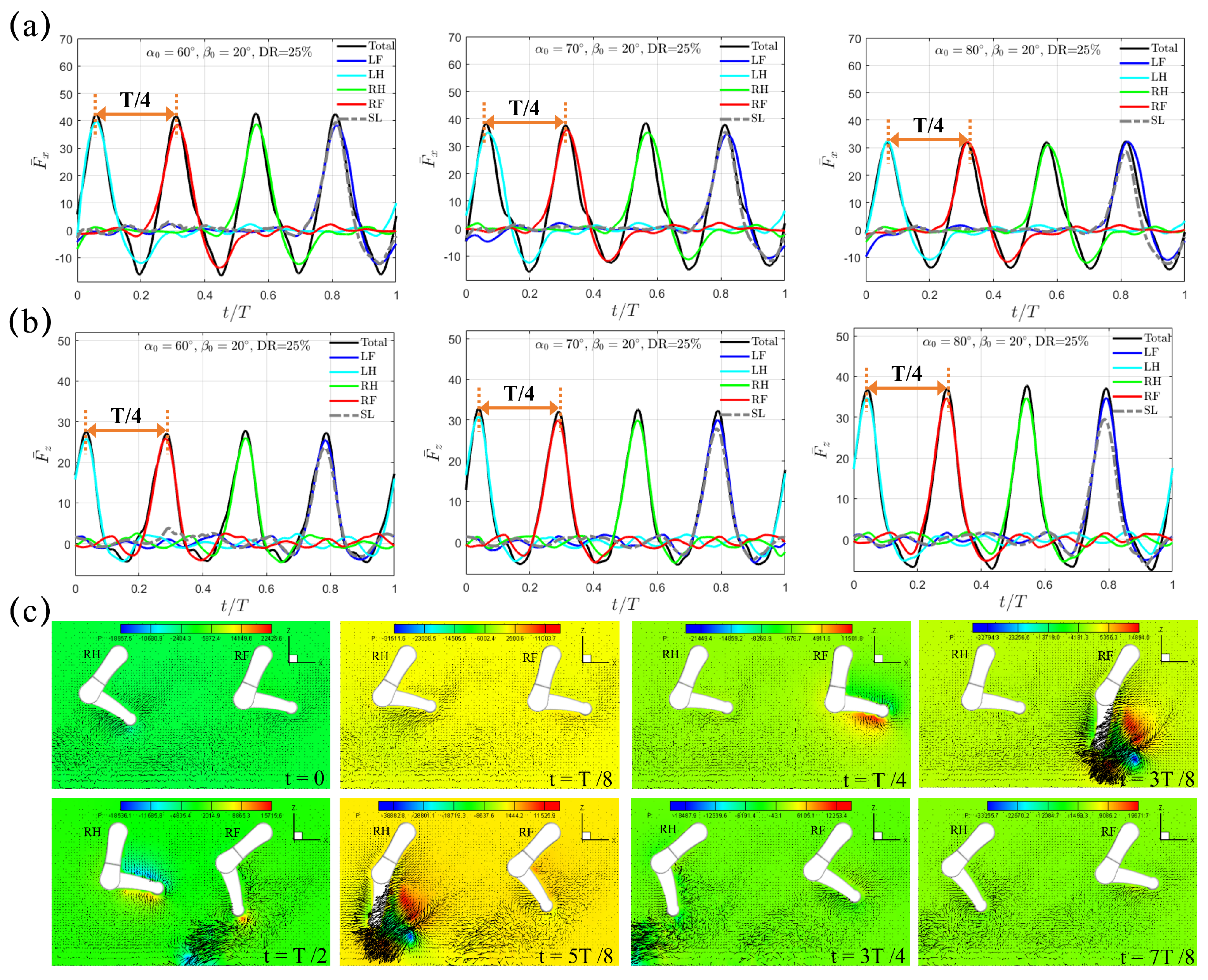
3.4. Quadrupedal Paddling Using the Lateral Sequence Gait
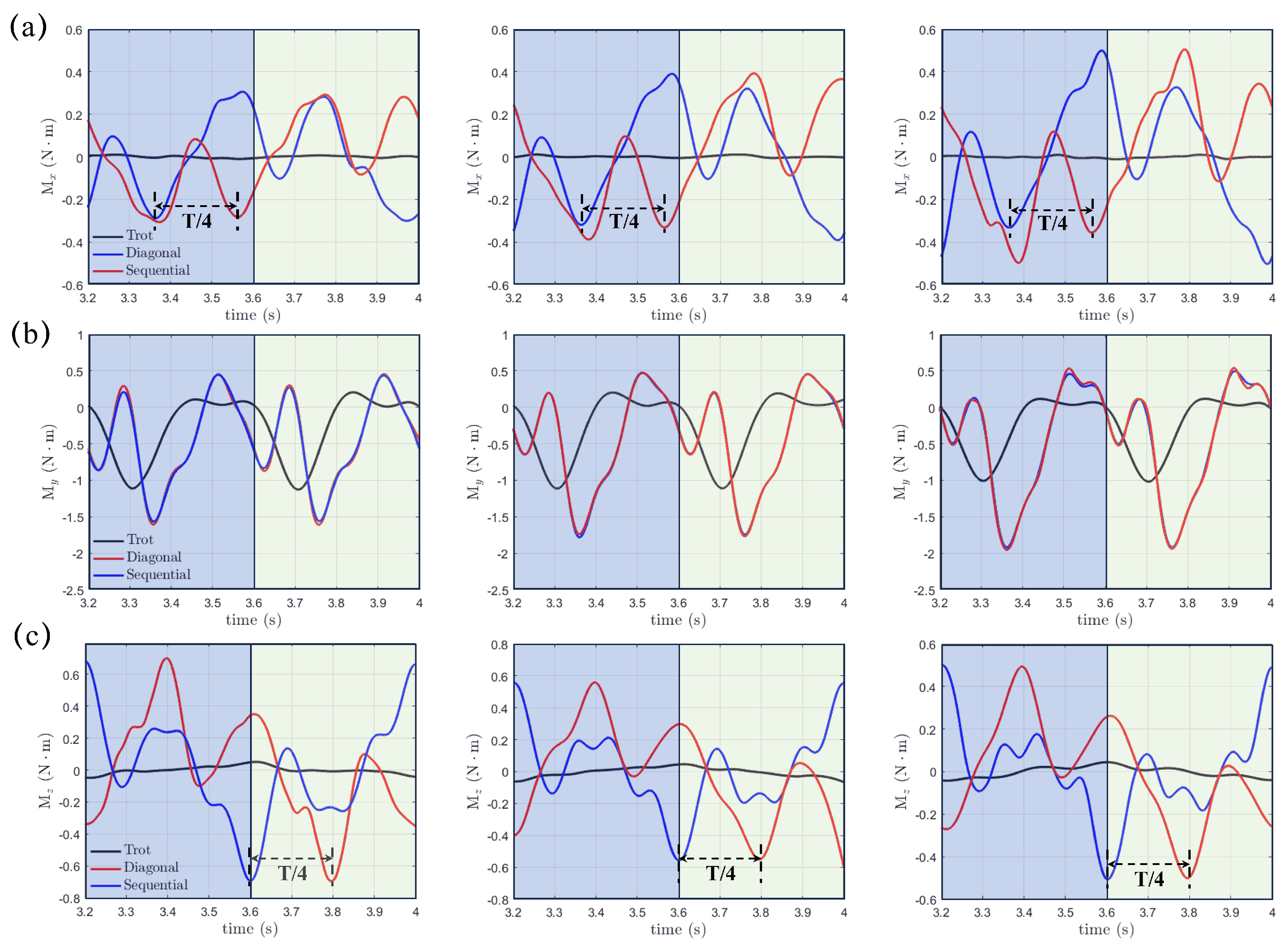
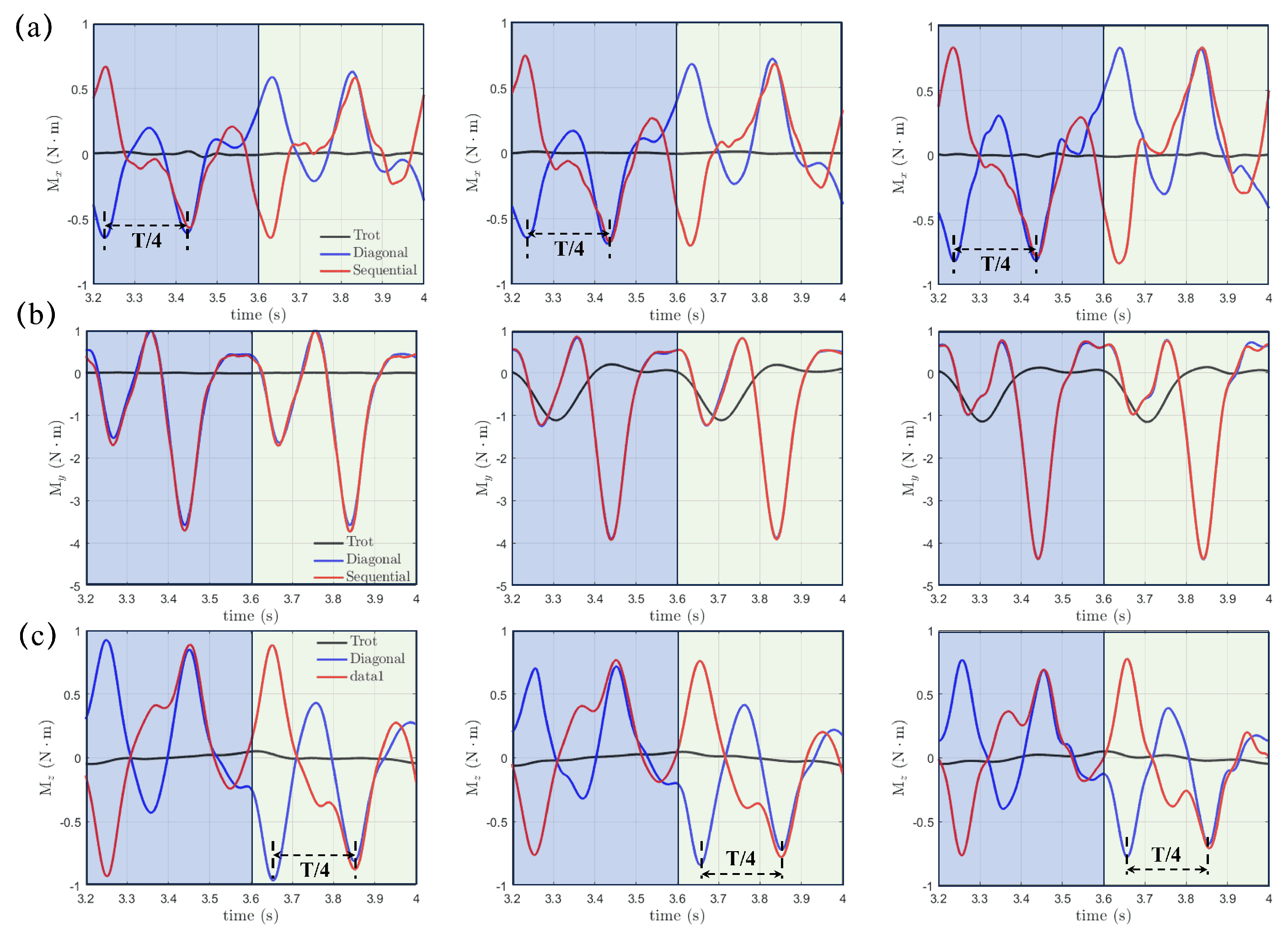
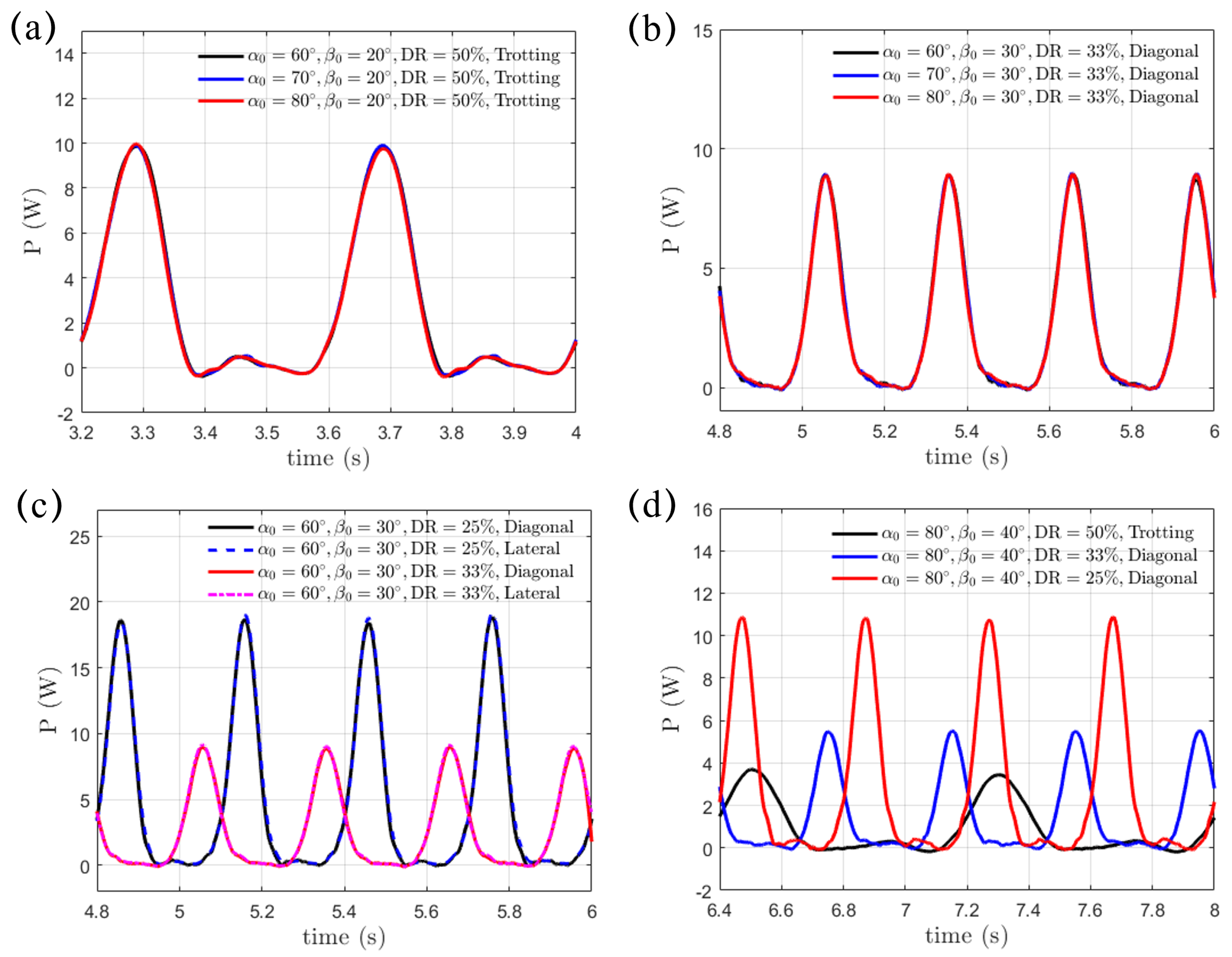
3.5. Moments and Energetics of Quadrupedal Paddling
4. Discussion
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Data Availability Statement
Conflicts of Interest
Appendix A

References
- Bai, X.J.; Shang, J.Z.; Luo, Z.R.; Jiang, T.; Yin, Q. Development of amphibious biomimetic robots. J. Zhejiang Univ. Sci. A 2022, 23, 157–187. [Google Scholar] [CrossRef]
- Yu, J.; Ding, R.; Yang, Q.; Tan, M.; Wang, W.; Zhang, J. On a bio-inspired amphibious robot capable of multimodal motion. IEEE/ASME Trans. Mechatron. 2011, 17, 847–856. [Google Scholar] [CrossRef]
- Zhang, S.; Zhou, Y.; Xu, M.; Liang, X.; Liu, J.; Yang, J. AmphiHex-I: Locomotory performance in amphibious environments with specially designed transformable flipper legs. IEEE/ASME Trans. Mechatron. 2015, 21, 1720–1731. [Google Scholar] [CrossRef]
- Greiner, H.; Shectman, A.; Won, C.; Elsley, R.; Beith, P. Autonomous legged underwater vehicles for near land warfare. In Proceedings of the Symposium on Autonomous Underwater Vehicle Technology, Monterey, CA, USA, 2–6 June 1996; IEEE: Piscataway, NJ, USA, 1996; pp. 41–48. [Google Scholar]
- Saranli, U.; Buehler, M.; Koditschek, D.E. Design, modeling and preliminary control of a compliant hexapod robot. In Proceedings of the 2000 ICRA IEEE International Conference on Robotics and Automation, San Francisco, CA, USA, 24–28 April 2000; IEEE: Piscataway, NJ, USA, 2000; Volume 3, pp. 2589–2596. [Google Scholar]
- Prahacs, C.; Saudners, A.; Smith, M.K.; McMordie, D.; Buehler, M. Towards legged amphibious mobile robotics. In Proceedings of the Canadian Engineering Education Association (CEEA), Montreal, QC, Canada, 29–30 July 2004. [Google Scholar]
- Zhong, B.; Zhou, Y.; Li, X.; Xu, M.; Zhang, S. Locomotion performance of the amphibious robot on various terrains and underwater with flexible flipper legs. J. Bionic Eng. 2016, 13, 525–536. [Google Scholar] [CrossRef]
- Hirose, S.; Mori, M. Biologically inspired snake-like robots. In Proceedings of the 2004 IEEE International Conference on Robotics and Biomimetics, Shenyang, China, 22–26 August 2004; IEEE: Piscataway, NJ, USA, 2004; pp. 1–7. [Google Scholar]
- Hirose, S.; Yamada, H. snake-like robots machine design of biologically inspired robots. IEEE Robot. Autom. Mag. 2009, 16, 88–98. [Google Scholar] [CrossRef]
- Matsuo, T.; Yokoyama, T.; Ueno, D.; Ishii, K. Biomimetic motion control system based on a CPG for an amphibious multi-link mobile robot. J. Bionic Eng. 2008, 5, 91–97. [Google Scholar] [CrossRef]
- Ijspeert, A.J.; Crespi, A.; Ryczko, D.; Cabelguen, J.M. From swimming to walking with a salamander robot driven by a spinal cord model. Science 2007, 315, 1416–1420. [Google Scholar] [CrossRef] [PubMed]
- Xia, M.; Wang, H.; Yin, Q.; Shang, J.; Luo, Z.; Zhu, Q. Design and mechanics of a composite wave-driven soft robotic fin for biomimetic amphibious robot. J. Bionic Eng. 2023, 20, 934–952. [Google Scholar] [CrossRef]
- Fish, F.E. Transitions from drag-based to lift-based propulsion in mammalian swimming. Am. Zool. 1996, 36, 628–641. [Google Scholar] [CrossRef]
- Li, Y.; Fish, F.; Chen, Y.; Ren, T.; Zhou, J. Bio-inspired robotic dog paddling: Kinematic and hydro-dynamic analysis. Bioinspir. Biomim. 2019, 14, 066008. [Google Scholar] [CrossRef] [PubMed]
- Lee, J.; Hwangbo, J.; Wellhausen, L.; Koltun, V.; Hutter, M. Learning quadrupedal locomotion over challenging terrain. Sci. Robot. 2020, 5, eabc5986. [Google Scholar] [CrossRef]
- Tang, Y.; Tan, J.; Harada, T. Learning agile locomotion via adversarial training. In Proceedings of the 2020 IEEE/RSJ International Conference On Intelligent Robots And Systems (IROS), Las Vegas, NV, USA, 24 October 2020–24 January 2021; IEEE: Piscataway, NJ, USA, 2020; pp. 6098–6105. [Google Scholar]
- Fu, Z.; Kumar, A.; Malik, J.; Pathak, D. Minimizing energy consumption leads to the emergence of gaits in legged robots. arXiv 2021, arXiv:2111.01674. [Google Scholar]
- Choi, S.; Ji, G.; Park, J.; Kim, H.; Mun, J.; Lee, J.H.; Hwangbo, J. Learning quadrupedal locomotion on deformable terrain. Sci. Robot. 2023, 8, eade2256. [Google Scholar] [CrossRef]
- Hao, T.; Xu, D.; Yan, S. Quadrupedal Locomotion in an Energy-efficient Way Based on Reinforcement Learning. Int. J. Control Autom. Syst. 2024, 22, 1613–1623. [Google Scholar] [CrossRef]
- Lee, S.; Nahrendra, I.; Lee, D.; Yu, B.; Oh, M.; Myung, H. DreamFLEX: Learning Fault-Aware Quadrupedal Locomotion Controller for Anomaly Situation in Rough Terrains. arXiv 2025, arXiv:2502.05817. [Google Scholar]
- Dudek, G.; Giguere, P.; Prahacs, C.; Saunderson, S.; Sattar, J.; Torres-Mendez, L.A.; Jenkin, M.; German, A.; Hogue, A.; Ripsman, A.; et al. Aqua: An amphibious autonomous robot. Computer 2007, 40, 46–53. [Google Scholar] [CrossRef]
- Zhang, S.; Liang, X.; Xu, L.; Xu, M. Initial development of a novel amphibious robot with transformable fin-leg composite propulsion mechanisms. J. Bionic Eng. 2013, 10, 434–445. [Google Scholar] [CrossRef]
- Wang, C.; Xie, G.; Yin, X.; Li, L.; Wang, L. CPG-based locomotion control of a quadruped amphibious robot. In Proceedings of the 2012 IEEE/ASME International Conference on Advanced Intelligent Mechatronics (AIM), Kaohsiung, Taiwan, 11–14 July 2012; IEEE: Piscataway, NJ, USA, 2012; pp. 1–6. [Google Scholar]
- Alben, S. Flapping propulsion using a fin ray. J. Fluid Mech. 2012, 705, 149–164. [Google Scholar] [CrossRef][Green Version]
- Maertens, A.P.; Gao, A.; Triantafyllou, M.S. Optimal undulatory swimming for a single fish-like body and for a pair of interacting swimmers. J. Fluid Mech. 2017, 813, 301–345. [Google Scholar] [CrossRef]
- Dagenais, P.; Aegerter, C.M. How shape and flapping rate affect the distribution of fluid forces on flexible hydrofoils. J. Fluid Mech. 2020, 901, A1. [Google Scholar] [CrossRef]
- Fernández-Gutiérrez, D.; Van Rees, W.M. Effect of leading-edge curvature actuation on flapping fin performance. J. Fluid Mech. 2021, 921, A22. [Google Scholar] [CrossRef]
- Chao, L.M.; Alam, M.M.; Cheng, L. Hydrodynamic performance of slender swimmer: Effect of travelling wavelength. J. Fluid Mech. 2022, 947, A8. [Google Scholar] [CrossRef]
- Zhang, D.; Huang, Q.G.; Pan, G.; Yang, L.M.; Huang, W.X. Vortex dynamics and hydrodynamic performance enhancement mechanism in batoid fish oscillatory swimming. J. Fluid Mech. 2022, 930, A28. [Google Scholar] [CrossRef]
- Georgiades, C.; Nahon, M.; Buehler, M. Simulation of an underwater hexapod robot. Ocean Eng. 2009, 36, 39–47. [Google Scholar] [CrossRef]
- Chen, G.; Tu, J.; Ti, X.; Wang, Z.; Hu, H. Hydrodynamic model of the beaver-like bendable webbed foot and paddling characteristics under different flow velocities. Ocean Eng. 2021, 234, 109179. [Google Scholar] [CrossRef]
- Zhang, C.; Guy, R.D.; Mulloney, B.; Zhang, Q.; Lewis, T.J. Neural mechanism of optimal limb coordination in crustacean swimming. Proc. Natl. Acad. Sci. USA 2014, 111, 13840–13845. [Google Scholar] [CrossRef]
- Baines, R.; Patiballa, S.K.; Booth, J.; Ramirez, L.; Sipple, T.; Garcia, A.; Fish, F.; Kramer-Bottiglio, R. Multi-environment robotic transitions through adaptive morphogenesis. Nature 2022, 610, 283–289. [Google Scholar] [CrossRef] [PubMed]
- Fish, F.E.; DiNenno, N.K.; Trail, J. The “dog paddle”: Stereotypic swimming gait pattern in different dog breeds. Anat. Rec. 2021, 304, 90–100. [Google Scholar] [CrossRef] [PubMed]
- Khalid, M.S.U.; Akhtar, I.; Imtiaz, H.; Dong, H.; Wu, B. On the hydrodynamics and nonlinear interaction between fish in tandem configuration. Ocean Eng. 2018, 157, 108–120. [Google Scholar] [CrossRef]
- Sun, X.; Ji, F.; Zhong, S.; Huang, D. Numerical study of an undulatory airfoil with different leading edge shape in power-extraction regime and propulsive regime. Renew. Energy 2020, 146, 986–996. [Google Scholar] [CrossRef]
- Tian, F.B. Hydrodynamic effects of mucus on swimming performance of an undulatory foil by using the DSD/SST method. Comput. Mech. 2020, 65, 751–761. [Google Scholar] [CrossRef]
- Khalid, M.S.U.; Wang, J.; Dong, H.; Liu, M. Flow transitions and mapping for undulating swimmers. Phys. Rev. Fluids 2020, 5, 063104. [Google Scholar] [CrossRef]
- Mittal, R.; Dong, H.; Bozkurttas, M.; Najjar, F.; Vargas, A.; Von Loebbecke, A. A versatile sharp interface immersed boundary method for incompressible flows with complex boundaries. J. Comput. Phys. 2008, 227, 4825–4852. [Google Scholar] [CrossRef] [PubMed]
- Luo, H.; Dai, H.; de Sousa, P.J.F.; Yin, B. On the numerical oscillation of the direct-forcing immersed-boundary method for moving boundaries. Comput. Fluids 2012, 56, 61–76. [Google Scholar] [CrossRef]
- Chen, Y.; Luo, H. A computational study of the three-dimensional fluid–structure interaction of aortic valve. J. Fluids Struct. 2018, 80, 332–349. [Google Scholar] [CrossRef]
- Chen, Y.; Luo, H. Pressure distribution over the leaflets and effect of bending stiffness on fluid–structure interaction of the aortic valve. J. Fluid Mech. 2020, 883, A52. [Google Scholar] [CrossRef]
- Tian, F.B.; Dai, H.; Luo, H.; Doyle, J.F.; Rousseau, B. Fluid–structure interaction involving large deformations: 3D simulations and applications to biological systems. J. Comput. Phys. 2014, 258, 451–469. [Google Scholar] [CrossRef] [PubMed]




| Authors | Year | Leg Number | Simulation Approach | Application Type |
|---|---|---|---|---|
| Zhang, Calvin, et al. [32] | 2014 | 4 | Immersed Boundary Method | NA |
| Chen, Gang, et al. [31] | 2021 | 2 | Sliding/dynamic mesh | Fluent |
| Baines, Robert, et al. [33] | 2022 | 4 | SIMPLEC solver | Fluent |
| (m/s) | T (s) | ||||||
|---|---|---|---|---|---|---|---|
| 0.8 | 10.53 | ||||||
| 0.8 | 9.90 | ||||||
| 0.8 | 8.79 | ||||||
| 1.2 | 8.26 | ||||||
| 1.2 | 7.71 | ||||||
| 1.2 | 7.15 | ||||||
| 1.6 | 6.62 | ||||||
| 1.6 | 5.71 | ||||||
| 1.6 | 5.37 | ||||||
| 0.8 | 18.24 | ||||||
| 0.8 | 15.68 | ||||||
| 0.8 | 13.75 | ||||||
| 1.2 | 17.50 | ||||||
| 1.2 | 15.57 | ||||||
| 1.2 | 13.95 | ||||||
| 1.6 | 14.06 | ||||||
| 1.6 | 12.38 | ||||||
| 1.6 | 11.11 | ||||||
| 0.8 | 39.32 | ||||||
| 0.8 | 34.98 | ||||||
| 0.8 | 28.54 | ||||||
| 1.2 | 31.81 | ||||||
| 1.2 | 28.68 | ||||||
| 1.2 | 25.86 | ||||||
| 1.6 | 22.80 | ||||||
| 1.6 | 21.08 | ||||||
| 1.6 | 18.95 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Wang, Y.; Cai, Y.; Xie, B.; Zhu, C.; Li, Y.; Chen, Y. A Computational Study on the Hydrodynamics of Bio-Inspired Quadrupedal Paddling. Biomimetics 2025, 10, 148. https://doi.org/10.3390/biomimetics10030148
Wang Y, Cai Y, Xie B, Zhu C, Li Y, Chen Y. A Computational Study on the Hydrodynamics of Bio-Inspired Quadrupedal Paddling. Biomimetics. 2025; 10(3):148. https://doi.org/10.3390/biomimetics10030148
Chicago/Turabian StyleWang, Yihan, Yumeng Cai, Bin Xie, Chi Zhu, Yunquan Li, and Ye Chen. 2025. "A Computational Study on the Hydrodynamics of Bio-Inspired Quadrupedal Paddling" Biomimetics 10, no. 3: 148. https://doi.org/10.3390/biomimetics10030148
APA StyleWang, Y., Cai, Y., Xie, B., Zhu, C., Li, Y., & Chen, Y. (2025). A Computational Study on the Hydrodynamics of Bio-Inspired Quadrupedal Paddling. Biomimetics, 10(3), 148. https://doi.org/10.3390/biomimetics10030148






