Terahertz Constant Velocity Flying Spot for 3D Tomographic Imaging
Abstract
1. Introduction
2. Materials and Methods
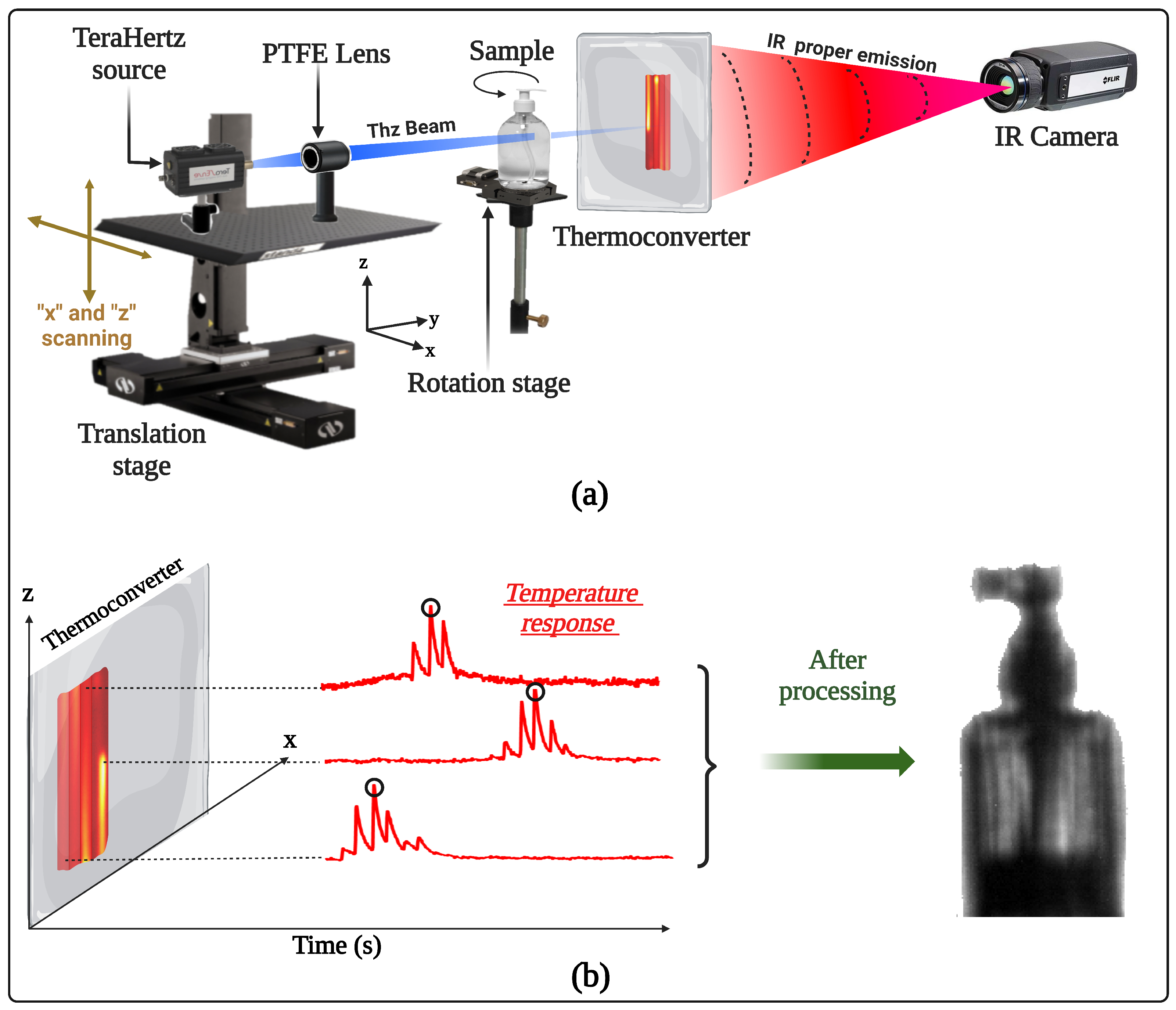
2.1. Experimental Setup
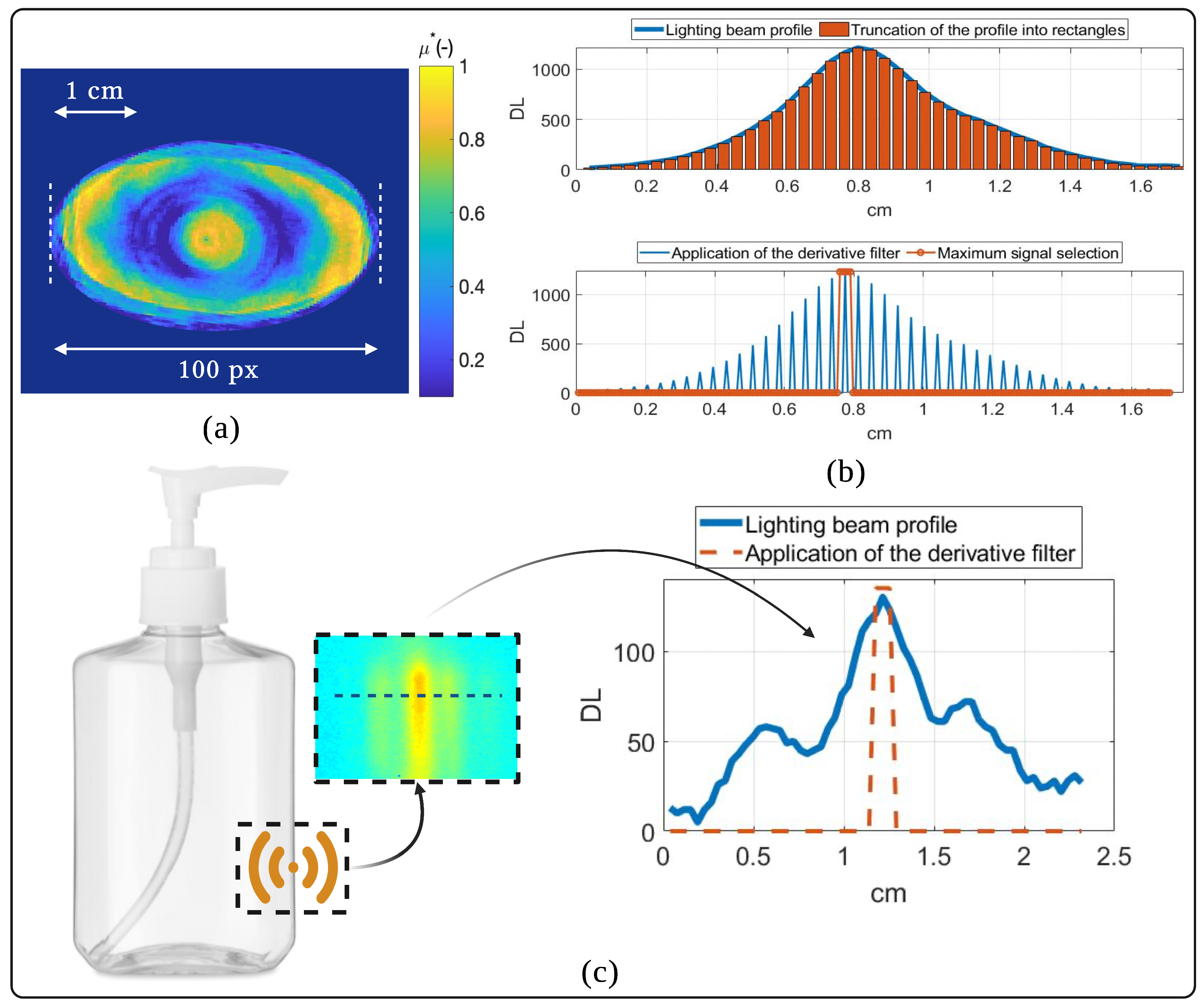
2.2. Measurement Procedure
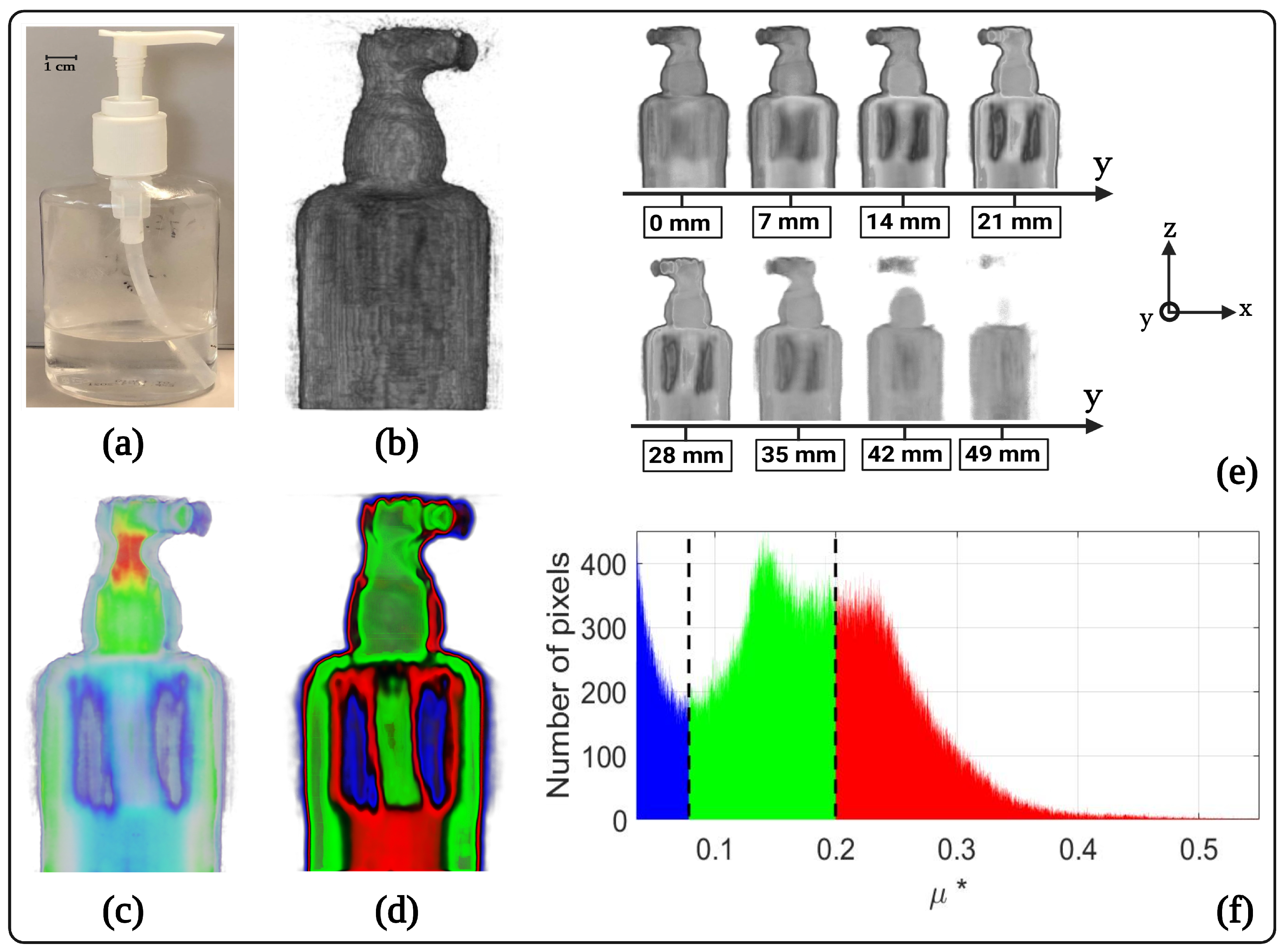
3. Results and Discussion
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Abbreviations
| 2D, 3D | Two-dimensional, three-dimensional |
| C | Concentration |
| GHz | GigaHertz |
| IR | InfraRed |
| THz | TeraHertz |
| T | Temperature |
| Angle | |
| Wavelength |
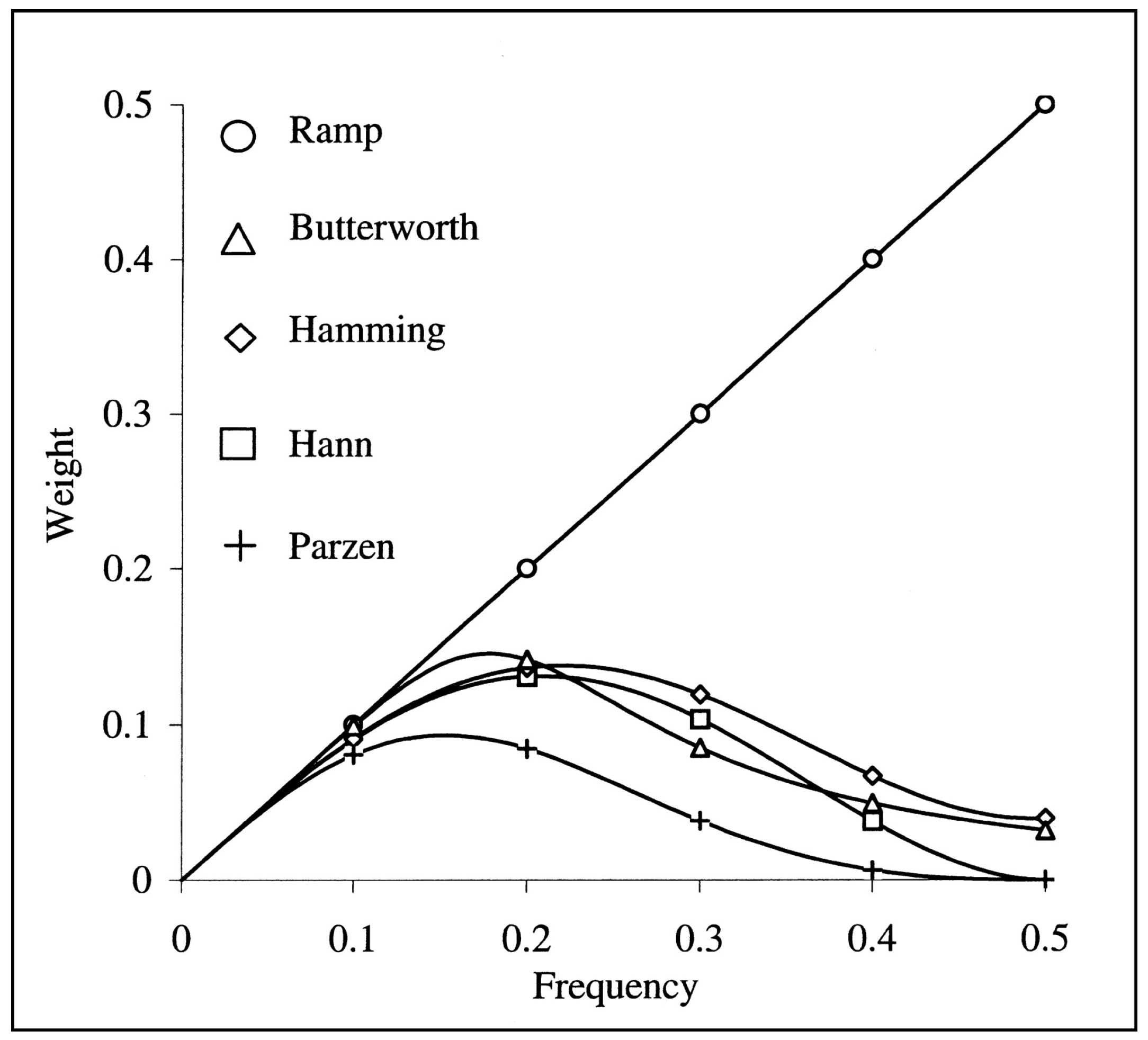
Appendix A. Ramp Filter
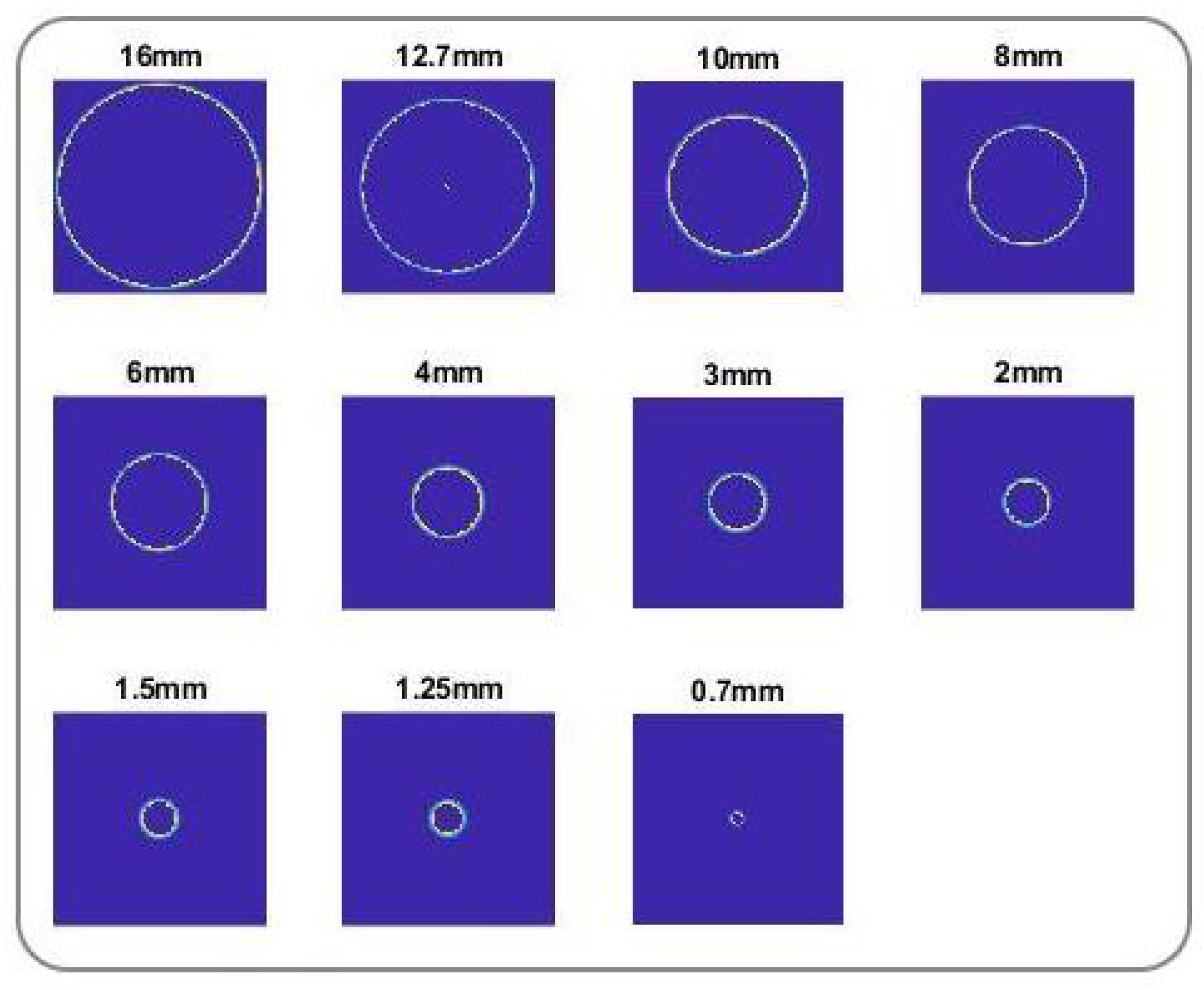
Appendix B. Spatial Resolution and Resolution Limit


References
- Withers, P.J.; Bouman, C.; Carmignato, S.; Cnudde, V.; Grimaldi, D.; Hagen, C.K.; Maire, E.; Manley, M.; Du Plessis, A.; Stock, S.R. X-ray computed tomography. Nat. Rev. Methods Prim. 2021, 1, R29. [Google Scholar] [CrossRef]
- Piovesan, A.; Vancauwenberghe, V.; Van De Looverbosch, T.; Verboven, P.; Nicolaï, B. X-ray computed tomography for 3D plant imaging. Trends Plant Sci. 2021, 26, 1171–1185. [Google Scholar] [CrossRef] [PubMed]
- McIntosh, R.; Nicastro, D.; Mastronarde, D. New views of cells in 3D: An introduction to electron tomography. Trends Cell Biol. 2005, 15, 43–51. [Google Scholar] [CrossRef] [PubMed]
- Ohmae, E.; Ouchi, Y.; Oda, M.; Suzuki, T.; Nobesawa, S.; Kanno, T.; Yoshikawa, E.; Futatsubashi, M.; Ueda, Y.; Okada, H.; et al. Cerebral hemodynamics evaluation by near-infrared time-resolved spectroscopy: Correlation with simultaneous positron emission tomography measurements. Neuroimage 2006, 29, 697–705. [Google Scholar] [CrossRef] [PubMed]
- Aouali, A.; Chevalier, S.; Sommier, A.; Abisset-Chavanne, E.; Batsale, J.C.; Pradere, C. 3D infrared thermospectroscopic imaging. Sci. Rep. 2020, 10, 22310. [Google Scholar] [CrossRef]
- Guillet, J.P.; Recur, B.; Frederique, L.; Bousquet, B.; Canioni, L.; Manek-Hönninger, I.; Desbarats, P.; Mounaix, P. Review of terahertz tomography techniques. J. Infrared Millim. Terahertz Waves 2014, 35, 382–411. [Google Scholar] [CrossRef]
- Taday, P.F. Applications of terahertz spectroscopy to pharmaceutical sciences. Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Eng. Sci. 2004, 362, 351–364. [Google Scholar] [CrossRef]
- Cook, D.J.; Decker, B.K.; Maislin, G.; Allen, M.G. Through container THz sensing: Applications for explosives screening. In Proceedings of the Terahertz and Gigahertz Electronics and Photonics III; International Society for Optics and Photonics: Washington, DC, USA, 2004; Volume 5354, pp. 55–62. [Google Scholar]
- Mueller, E.R. Terahertz radiation: Applications and sources. Ind. Phys. 2003, 9, 27–30. [Google Scholar]
- Federici, J.F.; Schulkin, B.; Huang, F.; Gary, D.; Barat, R.; Oliveira, F.; Zimdars, D. THz imaging and sensing for security applications—Explosives, weapons and drugs. Semicond. Sci. Technol. 2005, 20, S266. [Google Scholar] [CrossRef]
- Chan, W.L.; Deibel, J.; Mittleman, D.M. Imaging with terahertz radiation. Rep. Prog. Phys. 2007, 70, 1325. [Google Scholar] [CrossRef]
- Kawase, K.; Ogawa, Y.; Watanabe, Y.; Inoue, H. Non-destructive terahertz imaging of illicit drugs using spectral fingerprints. Opt. Express 2003, 11, 2549–2554. [Google Scholar] [CrossRef] [PubMed]
- White, J.; Zimdars, D. Time domain terahertz non destructive evaluation of water intrusion in composites and corrosion under insulation. In Proceedings of the 2007 Conference on Lasers and Electro-Optics (CLEO), Baltimore, MD, USA, 6–11 May 2007; pp. 1–2. [Google Scholar]
- Ospald, F.; Zouaghi, W.; Beigang, R.; Matheis, C.; Jonuscheit, J.; Recur, B.; Guillet, J.P.; Mounaix, P.; Vleugels, W.; Bosom, P.V.; et al. Aeronautics composite material inspection with a terahertz time-domain spectroscopy system. Opt. Eng. 2013, 53, 031208. [Google Scholar] [CrossRef]
- Beckmann, J.; Richter, H.; Zscherpel, U.; Ewert, U.; Weinzierl, J.; Schmidt, L.P.; Rutz, F.; Koch, M.; Hübers, H.W.; Richter, H. Imaging Capability of Terahertz and Millimeter-Wave Instrumentations for NDT of Polymer Materials. In Proceedings of the 9th European Conference on NDT, Berlin, Germany, 25–29 September 2006. [Google Scholar]
- Markelz, A.; Roitberg, A.; Heilweil, E.J. Pulsed terahertz spectroscopy of DNA, bovine serum albumin and collagen between 0.1 and 2.0 THz. Chem. Phys. Lett. 2000, 320, 42–48. [Google Scholar] [CrossRef]
- Pickwell, E.; Wallace, V. Biomedical applications of terahertz technology. J. Phys. Appl. Phys. 2006, 39, R301. [Google Scholar] [CrossRef]
- Zhang, X.C. Three-dimensional terahertz wave imaging. Philos. Trans. R. Soc. Lond. Ser. A Math. Phys. Eng. Sci. 2004, 362, 283–299. [Google Scholar] [CrossRef]
- Wang, D.; Ning, R.; Li, G.; Zhao, J.; Wang, Y.; Rong, L. 3D image reconstruction of terahertz computed tomography at sparse angles by total variation minimization. Appl. Opt. 2022, 61, B1–B7. [Google Scholar] [CrossRef]
- Balacey, H.; Recur, B.; Perraud, J.B.; Sleiman, J.B.; Guillet, J.P.; Mounaix, P. Advanced processing sequence for 3-D THz imaging. IEEE Trans. Terahertz Sci. Technol. 2016, 6, 191–198. [Google Scholar] [CrossRef]
- Yim, J.H.; Kim, S.y.; Kim, Y.; Cho, S.; Kim, J.; Ahn, Y.H. Rapid 3D-imaging of semiconductor chips using THz time-of-flight technique. Appl. Sci. 2021, 11, 4770. [Google Scholar] [CrossRef]
- Lewis, R. A review of terahertz sources. J. Phys. D Appl. Phys. 2014, 47, 374001. [Google Scholar] [CrossRef]
- Lewis, R. A review of terahertz detectors. J. Phys. D Appl. Phys. 2019, 52, 433001. [Google Scholar] [CrossRef]
- Rogalski, A.; Sizov, F. Terahertz detectors and focal plane arrays. Opto-Electron. Rev. 2011, 19, 346–404. [Google Scholar] [CrossRef]
- Nemoto, N.; Higuchi, T.; Kanda, N.; Konishi, K.; Kuwata-Gonokami, M. Highly precise and accurate terahertz polarization measurements based on electro-optic sampling with polarization modulation of probe pulses. Opt. Express 2014, 22, 17915–17929. [Google Scholar] [CrossRef]
- Aouali, A.; Chevalier, S.; Sommier, A.; Ayadi, M.; Batsale, J.C.; Balageas, D.; Pradere, C. Ultra-broadband contactless imaging power meter. Appl. Opt. 2021, 60, 7995–8005. [Google Scholar] [CrossRef]
- Romano, M.; Chulkov, A.; Sommier, A.; Balageas, D.; Vavilov, V.; Batsale, J.; Pradere, C. Broadband Sub-terahertz Camera Based on Photothermal Conversion and IR Thermography. J. Infrared Millim. Terahertz Waves 2016, 37, 448–461. [Google Scholar] [CrossRef]
- Centrone, A. Infrared imaging and spectroscopy beyond the diffraction limit. Annu. Rev. Anal. Chem. 2015, 8, 101–126. [Google Scholar] [CrossRef] [PubMed]
- Wilmink, G.J.; Ibey, B.L.; Rivest, B.D.; Grundt, J.E.; Roach, W.P.; Tongue, T.D.; Schulkin, B.J.; Laman, N.; Peralta, X.G.; Roth, C.C.; et al. Development of a compact terahertz time-domain spectrometer for the measurement of the optical properties of biological tissues. J. Biomed. Opt. 2011, 16, 047006. [Google Scholar] [CrossRef] [PubMed]
- Settles, G.S. Schlieren and Shadowgraph Techniques: Visualizing Phenomena in Transparent Media; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2001. [Google Scholar]
- Dumouchel, C. On the experimental investigation on primary atomization of liquid streams. Exp. Fluids 2008, 45, 371–422. [Google Scholar] [CrossRef]
- Bruyant, P.P. Analytic and iterative reconstruction algorithms in SPECT. J. Nucl. Med. 2002, 43, 1343–1358. [Google Scholar] [PubMed]

Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aouali, A.; Chevalier, S.; Sommier, A.; Pradere, C. Terahertz Constant Velocity Flying Spot for 3D Tomographic Imaging. J. Imaging 2023, 9, 112. https://doi.org/10.3390/jimaging9060112
Aouali A, Chevalier S, Sommier A, Pradere C. Terahertz Constant Velocity Flying Spot for 3D Tomographic Imaging. Journal of Imaging. 2023; 9(6):112. https://doi.org/10.3390/jimaging9060112
Chicago/Turabian StyleAouali, Abderezak, Stéphane Chevalier, Alain Sommier, and Christophe Pradere. 2023. "Terahertz Constant Velocity Flying Spot for 3D Tomographic Imaging" Journal of Imaging 9, no. 6: 112. https://doi.org/10.3390/jimaging9060112
APA StyleAouali, A., Chevalier, S., Sommier, A., & Pradere, C. (2023). Terahertz Constant Velocity Flying Spot for 3D Tomographic Imaging. Journal of Imaging, 9(6), 112. https://doi.org/10.3390/jimaging9060112





