A Finite-Difference Approach for Plasma Microwave Imaging Profilometry
Abstract
1. Introduction
2. Mathematical Formulation
3. Microwave Imaging Profilometry
3.1. Linearized Approach for Plasma Slabs
3.2. Sparsity-Promoting Recovery Approaches
4. Numerical Assessment Towards Benchmark Examples
- A single transmitting and receiving antenna measuring the reflection coefficient in a free-space homogeneous background (reflection-only measurement);
- Single transmitting and receiving antenna measuring the reflection coefficient in presence of a PEC surface;
- A transmitting and receiving antenna measuring the reflection coefficient and a receiving antenna measuring the transmission coefficient in a homogeneous free-space background (reflection and transmission measurement).
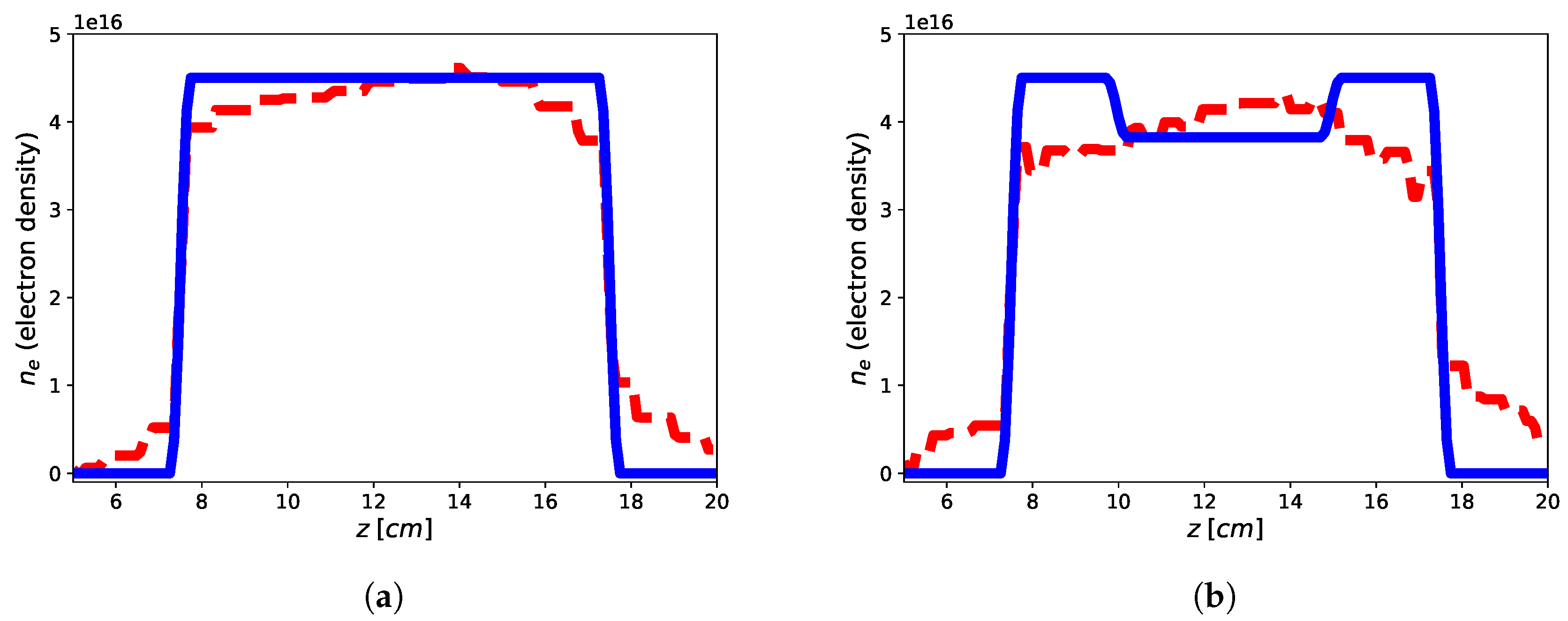
4.1. Reflection-Only Measurement with Single Antenna
4.2. Reflection-Transmission Measurement with Two Antennas
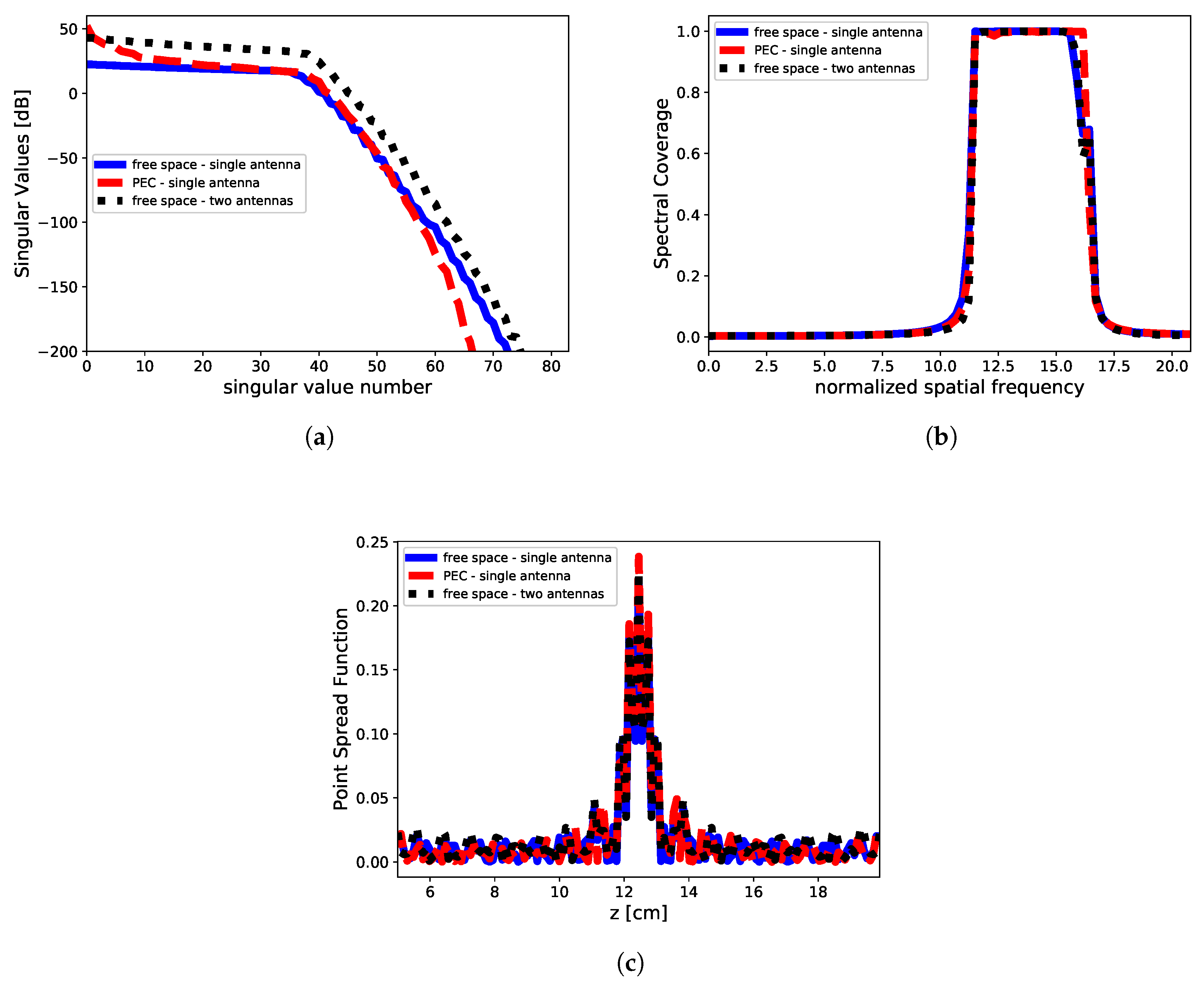
5. Discussion of the Results through Singular Value Decomposition Analysis
- behavior of the singular values —the logarithmic plot of the singular values as ordered in non-increasing fashion. Indeed, as the scattering operator is a compact one [18], its singular values exhibits an exponential decay after a given threshold index I (analytically expressed by (17)), which indicates the maximum number of the degrees of freedom, and hence of the parameters which can be conveyed back by the recovery procedure;
- spectral coverage (SC) defined as:wherein is the Fourier Transform of the left singular vectors and the truncation index used as regularization parameter in TSVD approach [10]. It is a measure of the class of profiles which can be actually retrieved in the object domain by the inversion process [10].
- point spread function (PSF) defined as [27]:wherein stands for conjugation, and the abscissa with respect the PSF is considered. For the case at hand, we set cm, which is the center of the imaging domain. The point spread function is a direct measure of the ultimate attainable spatial resolution [27].
6. Conclusions
Author Contributions
Funding
Acknowledgments
Conflicts of Interest
References
- Park, S.; Jang, J.; Choe, W. Sparse data recovery of tomographic diagnostics for ultra-large-area plasmas. Plasma Sour. Sci. Technol. 2019, 28, 035012. [Google Scholar] [CrossRef]
- Geller, R. Electron Cyclotron Resonance Ion Sources and ECR Plasmas; Routledge: London, UK, 2018. [Google Scholar]
- Torrisi, G.; Sorbello, G.; Leonardi, O.; Mascali, D.; Celona, L.; Gammino, S. A new launching scheme for ECR plasma based on two-waveguides-array. Microw. Opt. Technol. Lett. 2016, 58, 2629–2634. [Google Scholar] [CrossRef]
- Mazzucato, E.; Munsat, T.; Park, H.; Deng, B.; Domier, C.; Luhmann, N., Jr.; Donné, A.; van de Pol, M. Fluctuation measurements in tokamaks with microwave imaging reflectometry. Phys. Plasmas 2002, 9, 1955–1961. [Google Scholar] [CrossRef]
- Mascali, D.; Torrisi, G.; Leonardi, O.; Sorbello, G.; Castro, G.; Celona, L.; Miracoli, R.; Agnello, R.; Gammino, S. The first measurement of plasma density in an ECRIS-like device by means of a frequency-sweep microwave interferometer. Rev. Sci. Instrum. 2016, 87, 095109. [Google Scholar] [CrossRef] [PubMed]
- Pastorino, M. Microwave Imaging; Wiley Online Library: Hoboken, NJ, USA, 2010. [Google Scholar]
- Di Donato, L.; Morabito, A.F.; Torrisi, G.; Isernia, T.; Sorbello, G. Electromagnetic Inverse Profiling for Plasma Diagnostics via Sparse Recovery Approaches. IEEE Trans. Plasma Sci. 2019, 47, 1781–1787. [Google Scholar] [CrossRef]
- Gilmore, C.; LoVetri, J. Enhancement of microwave tomography through the use of electrically conducting enclosures. Inverse Probl. 2008, 24, 035008. [Google Scholar] [CrossRef][Green Version]
- Solimene, R.; Maisto, M.A.; Pierri, R. Inverse scattering in the presence of a reflecting plane. J. Opt. 2015, 18, 025603. [Google Scholar] [CrossRef]
- Pierri, R.; Persico, R.; Bernini, R. Information content of the Born field scattered by an embedded slab: multifrequency, multiview, and multifrequency—Multiview cases. JOSA A 1999, 16, 2392–2399. [Google Scholar] [CrossRef]
- Sullivan, D.M. Electromagnetic Simulation Using the FDTD Method; John Wiley & Sons: Hoboken, NJ, USA, 2013. [Google Scholar]
- Sacks, Z.S.; Kingsland, D.M.; Lee, R.; Lee, J.F. A perfectly matched anisotropic absorber for use as an absorbing boundary condition. IEEE Trans. Antennas Propag. 1995, 43, 1460–1463. [Google Scholar] [CrossRef]
- Tonti, E. The Mathematical Structure of Classical and Relativistic Physics; Springer: Berlin, Germany, 2013. [Google Scholar]
- Laudani, R.; Calvagna, A.; Sorbello, G.; Janner, D.; Tramontana, E. Analysis of the Discretization Error at Material Interfaces in Staggered Grids. IEEE Trans. Antennas Propag. 2010, 58, 1653–1661. [Google Scholar] [CrossRef]
- Clemens, M.; Weiland, T. Discrete electromagnetics: Maxwell’s equations tailored to numerical simulations. Int. Compumag Soc. Newsl. 2001, 8, 13–20. [Google Scholar]
- Abubakar, A.; Hu, W.; Van Den Berg, P.; Habashy, T. A finite-difference contrast source inversion method. Inverse Probl. 2008, 24, 065004. [Google Scholar] [CrossRef]
- Colton, D.; Kress, R. Inverse Acoustic and Electromagnetic Scattering Theory; Springer-Verlag: Berlin, Germany, 1992. [Google Scholar]
- Bertero, M.; Boccacci, P. Introduction to Inverse Problems in Imaging; Institute of Physics: Bristol, UK, 1998. [Google Scholar]
- Slaney, M.; Kak, A.C.; Larsen, L.E. Limitations of imaging with first-order diffraction tomography. IEEE Trans. Microw. Theory Tech. 1984, 32, 860–874. [Google Scholar] [CrossRef]
- Stix, T. Waves in Plasmas; American Institute of Physics: College Park, MD, USA, 1992. [Google Scholar]
- Di Donato, L.; Palmeri, R.; Sorbello, G.; Isernia, T.; Crocco, L. Assessing the capabilities of a new linear inversion method for quantitative microwave imaging. Int. J. Antennas Propag. 2015, 2015. [Google Scholar] [CrossRef]
- Di Donato, L.; Palmeri, R.; Sorbello, G.; Isernia, T.; Crocco, L. A new linear distorted-wave inversion method for microwave imaging via virtual experiments. IEEE Trans. Microw. Theory Tech. 2016, 64, 2478–2488. [Google Scholar] [CrossRef]
- Donoho, D.L. Compressed sensing. IEEE Trans. Inf. Theory 2006, 52, 1289–1306. [Google Scholar] [CrossRef]
- Baraniuk, R.G. Compressive sensing [lecture notes]. IEEE Signal Process. Mag. 2007, 24, 118–121. [Google Scholar] [CrossRef]
- Rumpf, R.C. Simple implementation of arbitrarily shaped total-field/scattered-field regions in finite-difference frequency-domain. Prog. Electromagn. Res. 2012, 36, 221–248. [Google Scholar] [CrossRef]
- CVXPY 1.0. 2019. Available online: https://www.cvxpy.org/ (accessed on 9 July 2019).
- Pierri, R.; Brancaccio, A.; Leone, G.; Soldovieri, F. Electromagnetic prospection via homogeneous and inhomogeneous plane waves: the case of an embedded slab. AEU-Int. J. Electron. Commun. 2002, 56, 11–18. [Google Scholar] [CrossRef]



© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Di Donato, L.; Mascali, D.; Morabito, A.F.; Sorbello, G. A Finite-Difference Approach for Plasma Microwave Imaging Profilometry. J. Imaging 2019, 5, 70. https://doi.org/10.3390/jimaging5080070
Di Donato L, Mascali D, Morabito AF, Sorbello G. A Finite-Difference Approach for Plasma Microwave Imaging Profilometry. Journal of Imaging. 2019; 5(8):70. https://doi.org/10.3390/jimaging5080070
Chicago/Turabian StyleDi Donato, Loreto, David Mascali, Andrea F. Morabito, and Gino Sorbello. 2019. "A Finite-Difference Approach for Plasma Microwave Imaging Profilometry" Journal of Imaging 5, no. 8: 70. https://doi.org/10.3390/jimaging5080070
APA StyleDi Donato, L., Mascali, D., Morabito, A. F., & Sorbello, G. (2019). A Finite-Difference Approach for Plasma Microwave Imaging Profilometry. Journal of Imaging, 5(8), 70. https://doi.org/10.3390/jimaging5080070






