Tip Crack Imaging on Transparent Materials by Digital Holographic Microscopy
Abstract
:1. Introduction
2. Key Principle of DHM
3. Preparation the Transparent Sample
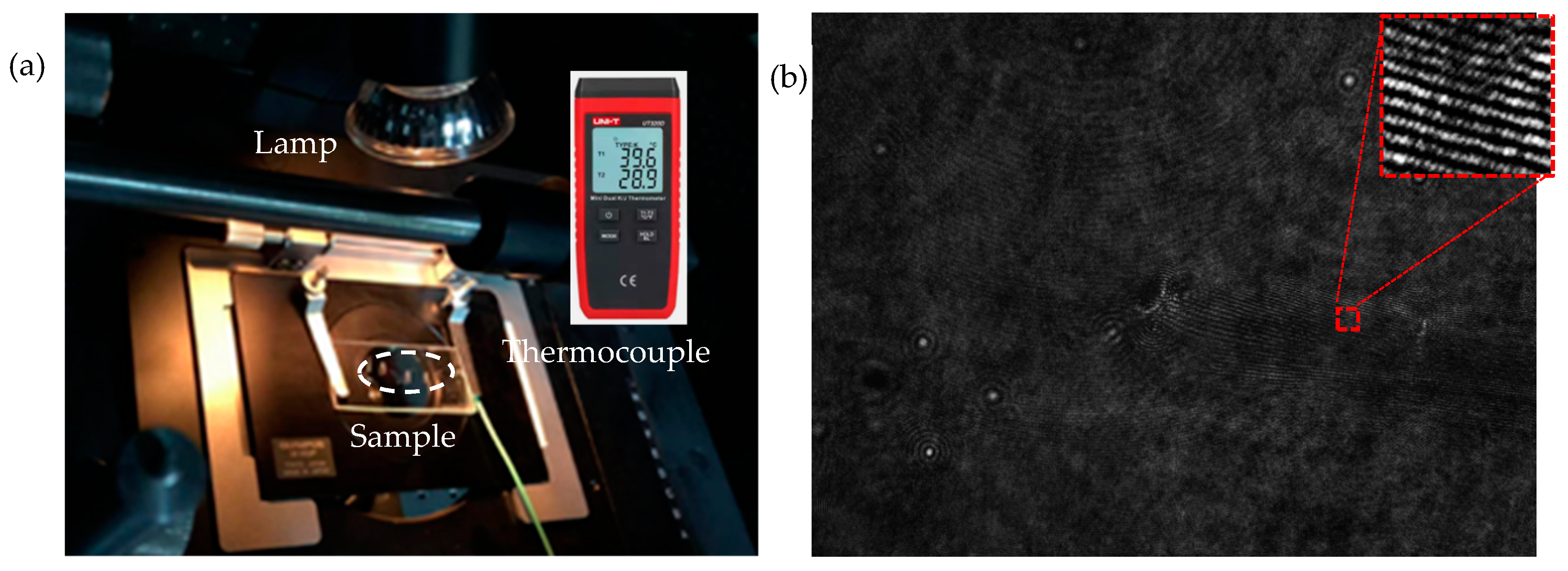
4. Experimental Setup
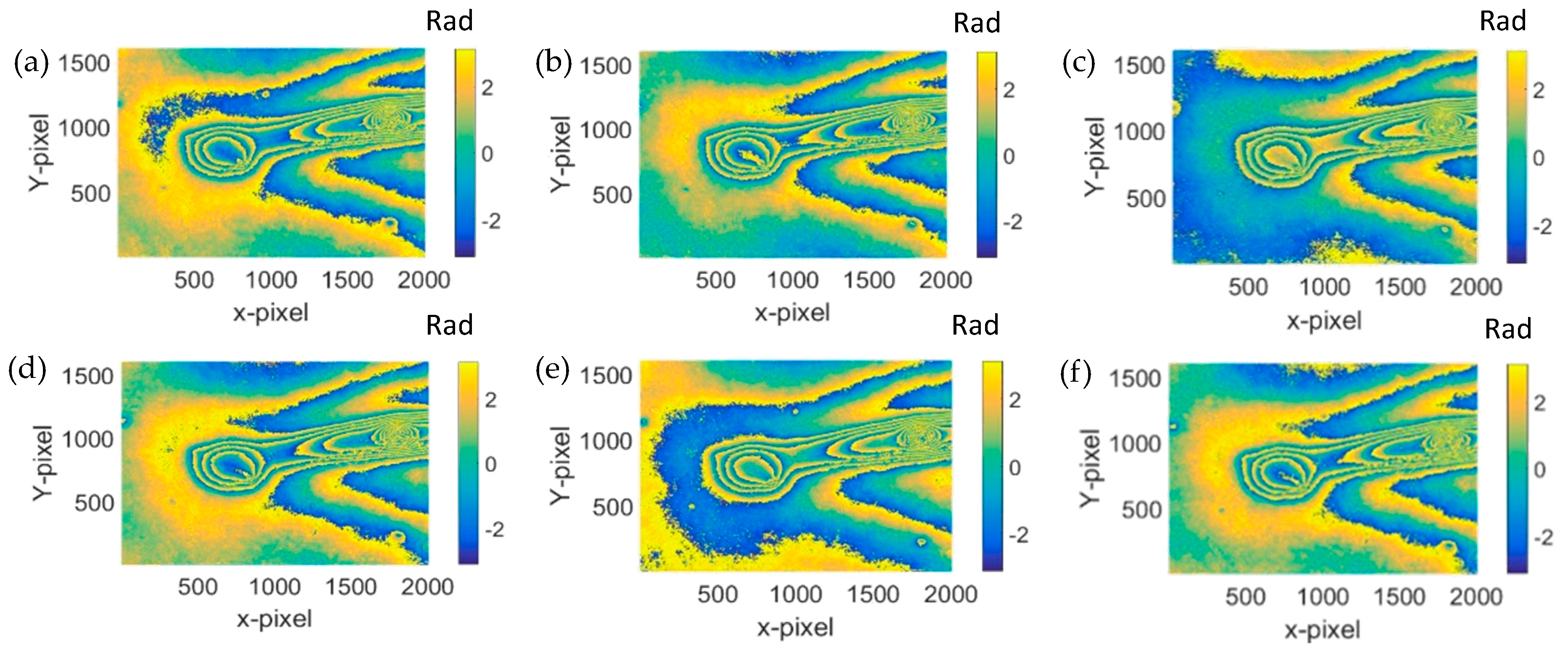
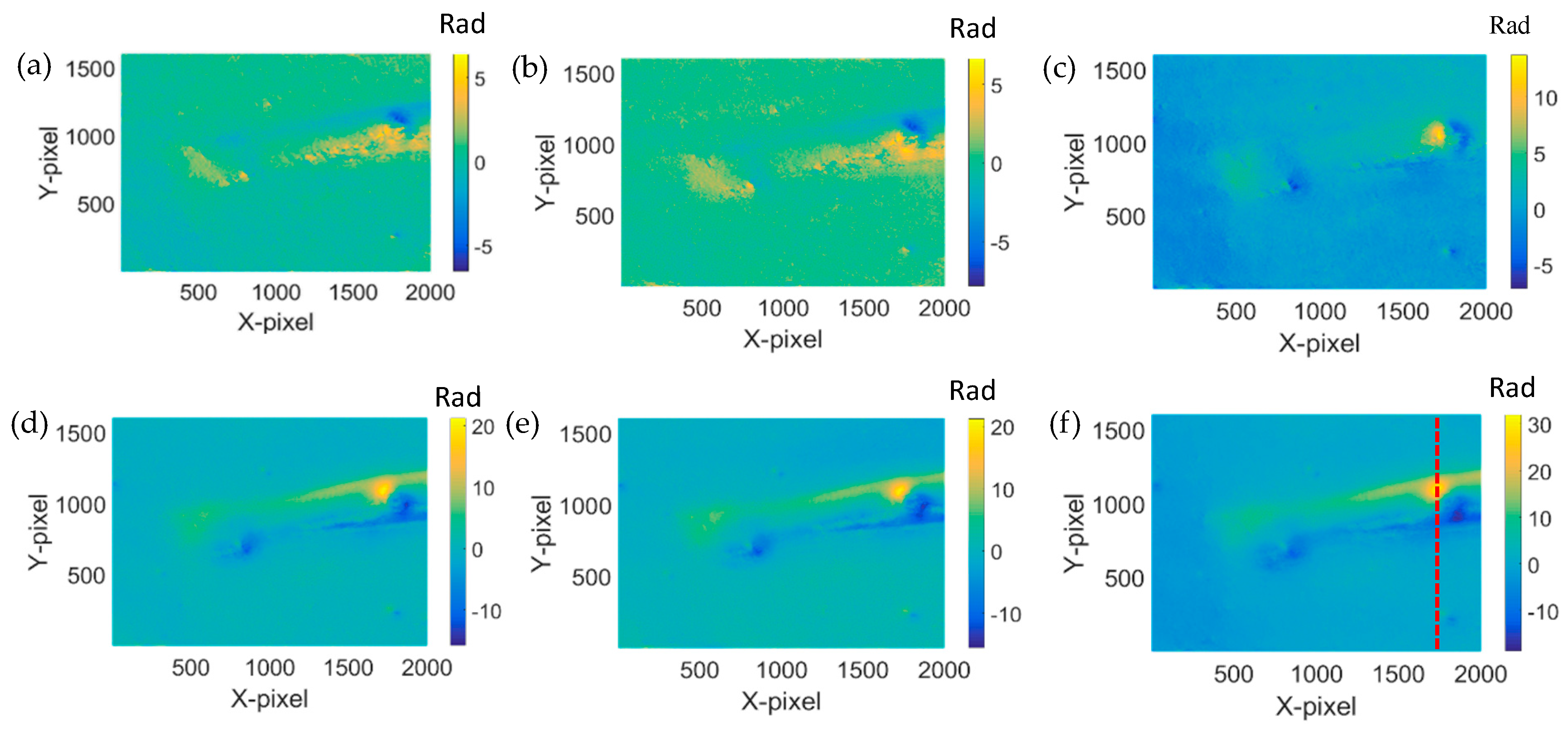
5. Experimental Results and Discussion
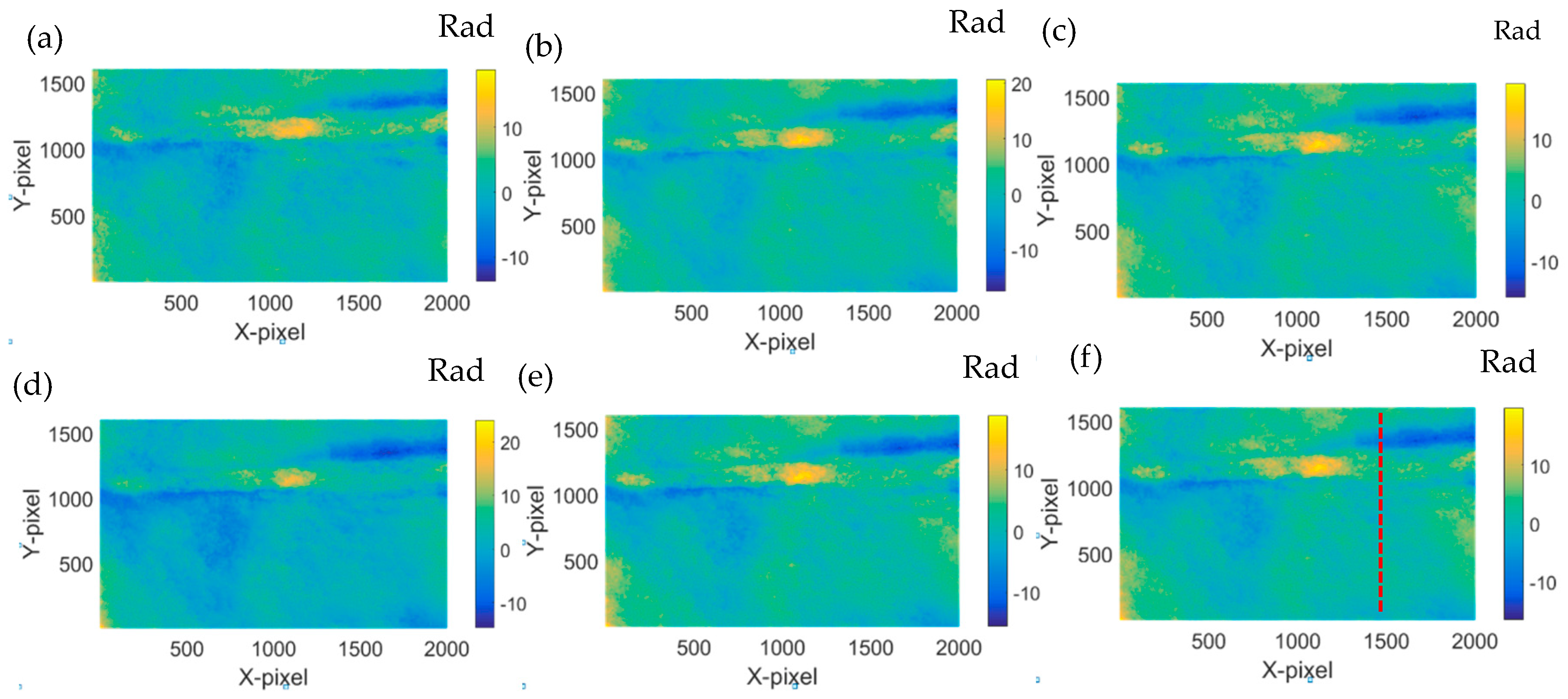
6. Comparison Experiment Setup and Results
7. Conclusions
Author Contributions
Funding
Conflicts of Interest
References
- Malowany, K.; Tymińska-Widmer, L.; Malesa, M. Application of 3D digital image correlation to track displacements and strains of canvas paintings exposed to relative humidity changes. Appl. Opt. 2014, 53, 1739–1749. [Google Scholar] [CrossRef] [PubMed]
- Paoletti, D.; Spagnolo, G.S.; Facchini, M.; Zanetta, P. Artwork diagnostics with fiber-optic digital speckle pattern interferometry. Appl. Opt. 1993, 32, 6236–6241. [Google Scholar] [CrossRef] [PubMed]
- Gao, P.F.; Lei, Z.N.; Wang, X.X.; Zhan, M. Deformation in fatigue crack tip plastic zone and its role in crack propagation of titanium alloy with tri-modal microstructure. Mater. Sci. Eng. A 2019, 739, 198–202. [Google Scholar] [CrossRef]
- Nowella, D.; Dragnevskib, K.I.; O’Connorb, S.J. Investigation of fatigue crack models by micro-scale measurement of crack tip deformation. Int. J. Fatigue 2018, 115, 20–26. [Google Scholar] [CrossRef]
- Cuche, E.; Marquet, P.; Depeursinge, C. Simultaneous amplitude-contrast and quantitative phase-contrast microscopy by numerical reconstruction of Fresnel off-axis holograms. Appl. Opt. 1999, 34, 6994–7001. [Google Scholar] [CrossRef] [PubMed]
- John, C.M.; Ting, C.K.; Anna, S.; Alexander, K. Design of a dual wavelength digital holographic imaging system for the examination of layered structures. In SPIE Optical Systems Design; SPIE: Frankfurt, Germany, 2018. [Google Scholar]
- Paturzo, M.; Pagliarulo, V.; Bianco, V.; Memmolo, P.; Miccio, L.; Merola, F.; Ferraro, P. Digital Holography, a metrological tool for quantitative analysis: Trends and future applications. Opt. Lasers Eng. 2018, 104, 32–47. [Google Scholar] [CrossRef]
- Zhou, W.J.; Peng, K.Q.; Yu, Y.J. Surface roughness measurement and analysis of mechanical parts based on digital holography. Adv. Manuf. 2016, 4, 217–224. [Google Scholar] [CrossRef]
- Fernández, M.V.; Gonçalves, E.; Rivera, J.L.V.; Ricardo, J.; Fernández Abreu, M.E.; Vaz, M.A.P. Development of Digital Holographic Microscopy by reflection for analysis of surface. Results Phys. 2018, 11, 182–187. [Google Scholar]
- Di, J.L.; Li, Y.; Wang, K.Q.; Zhao, J.L. Quantitative and Dynamic Phase Imaging of Biological Cells by the Use of the Digital Holographic Microscopy Based on a Beam Displacer Unit. IEEE Photonics J. 2018, 10, 6900510. [Google Scholar] [CrossRef]
- Wang, J.; Dong, L.; Chen, H.; Huang, S.J. Birefringence measurement of biological tissue based on polarization sensitive digital holographic microscopy. Appl. Phys. B 2018, 124, 240. [Google Scholar] [CrossRef]
- Trivedi, V.; Joglekar, M.; Mahajan, S.; Patel, N.; Chhaniwal, V.; Javidi, B.; Anand, A. Digital holographic imaging of refractive index distributions for defect detection. Opt. Laser Technol. 2019, 111, 439–446. [Google Scholar] [CrossRef]
- Asgaria, P.; Pourvaisb, Y.; Abdollahib, P.; Moradid, A.R.; Khamedib, R.; Darudia, A. Digital holographic microscopy as a new technique for quantitative measurement of microstructural corrosion in austenitic stainless steel. Appl. Mater. Des. 2017, 125, 109–115. [Google Scholar] [CrossRef]
- Pouli, P.; Melessanaki, K.; Giakoumaki, A.; Argyropoulos, V.; Anglos, D. Measuring the thickness of protective coatings on historic metal objects using nanosecond and femtosecond LIBS depth profiling. Spectrochim. Acta Part B At. Spectrosc. 2005, 60, 1163–1171. [Google Scholar] [CrossRef]
- Tornari, V.; Tsiranidou, E.; Bernikola, E. Crack-Growth on Canvas Paintings during Transport Simulation Monitored with Digital Holographic Speckle Interferometry. Adv. Res. 2014, 2, 967–986. [Google Scholar] [CrossRef]
- Tornari, V.; Bernikola, E.; Nevin, A.; Kouloumpi, E.; Doulgeridis, M.; Fotakis, C. Fully-Non-Contact Masking-Based Holography Inspection on Dimensionally Responsive. Artwork Mater. 2008, 8, 8401–8422. [Google Scholar]
- Bernikola, E.; Nevin, A.; Tornari, V. Rapid initial dimensional changes in wooden panel paintings due to simulated climate-induced alterations monitored by digital coherent out-of-plane interferometry. Appl. Phys. A 2009, 95, 387–399. [Google Scholar] [CrossRef]
- Tornari, V.; Bernikola, E.; Tsigarida, N.; Andrianakis, M.; Hatzigiannakis, K.; Leissner, J. Preventive deformation measurements on cultural heritage materials based on non-contact surface response of model samples. Stud. Conserv. 2015, 60, 143–158. [Google Scholar] [CrossRef]
- Tornari, V.; Tsiranidou, E.; Bernikola, E. Interference fringe-patterns association to defect-types in artwork conservation: An experiment and research validation review. Appl. Phys. A 2012, 106, 397–410. [Google Scholar] [CrossRef]
- Suzuki, S.; Sakaue, K.; Morita, Y.; Mori, T. Method for successive photographing of rapid crack bifurcation by means of high-speed holographic microscopy. In Proceedings of the 24th International Congress on High-Speed Photography and Photonics, Sendai, Japan, 17 April 2001. [Google Scholar]
- Schnars, U.; Juptner, P.O. Digital recording and numerical reconstruction of holograms. Meas. Sci. Technol. 2002, 13, R85–R101. [Google Scholar] [CrossRef]
- Poon, T.C.; Liu, J.P. Introduction to Modern Digital Holography: With MATLAB; Cambridge University: Cambridge, UK, 2014; pp. 9–24. [Google Scholar]
- Dennis, C.; Louis, A. Minimum L(p)-norm two-dimensional phase unwrapping. J. Opt. Soc. Am. 1996, 13, 1999–2013. [Google Scholar]








| No. of Hologram | 0 | 1 | 2 | 3 | 4 | 5 | 6 |
| Heating time (h) | 0 | 12 | 16 | 20 | 24 | 36 | 40 |
| Temperature (°C) | 22.3 | 36.5 | 36.8 | 36.8 | 38 | 37.1 | 37.8 |
| No. of Hologram | 0 | 1 | 2 | 3 | 4 | 5 | 6 |
| Heating time (h) | 0 | 12 | 16 | 20 | 24 | 36 | 40 |
| Temperature (°C) | 20.0 | 34.7 | 36.4 | 37.3 | 37.6 | 37.0 | 37.8 |
| No. of Hologram | 0 | 1 | 2 | 3 | 4 | 5 | 6 |
| Heating time (h) | 0 | 12 | 16 | 20 | 24 | 36 | 40 |
| Temperature (°C) | 19.3 | 19.2 | 19.5 | 20.0 | 19.3 | 18.8 | 20.1 |
© 2019 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (http://creativecommons.org/licenses/by/4.0/).
Share and Cite
Zhou, W.-J.; Li, B.-Y.; Shen, H.-X.; He, D.-K.; Zhang, H.-B.; Yu, Y.-J.; Tornari, V. Tip Crack Imaging on Transparent Materials by Digital Holographic Microscopy. J. Imaging 2019, 5, 80. https://doi.org/10.3390/jimaging5100080
Zhou W-J, Li B-Y, Shen H-X, He D-K, Zhang H-B, Yu Y-J, Tornari V. Tip Crack Imaging on Transparent Materials by Digital Holographic Microscopy. Journal of Imaging. 2019; 5(10):80. https://doi.org/10.3390/jimaging5100080
Chicago/Turabian StyleZhou, Wen-Jing, Bo-Yu Li, Hong-Xia Shen, Deng-Ke He, Hong-Bo Zhang, Ying-Jie Yu, and Vivi Tornari. 2019. "Tip Crack Imaging on Transparent Materials by Digital Holographic Microscopy" Journal of Imaging 5, no. 10: 80. https://doi.org/10.3390/jimaging5100080
APA StyleZhou, W.-J., Li, B.-Y., Shen, H.-X., He, D.-K., Zhang, H.-B., Yu, Y.-J., & Tornari, V. (2019). Tip Crack Imaging on Transparent Materials by Digital Holographic Microscopy. Journal of Imaging, 5(10), 80. https://doi.org/10.3390/jimaging5100080






