Reduction of Uneven Brightness and Ghosts of Aerial Images Using a Prism in a Micromirror Array Plate
Abstract
1. Introduction
2. Conventional Method
2.1. Principle of the MMAP
2.2. Method of Ghost Reduction
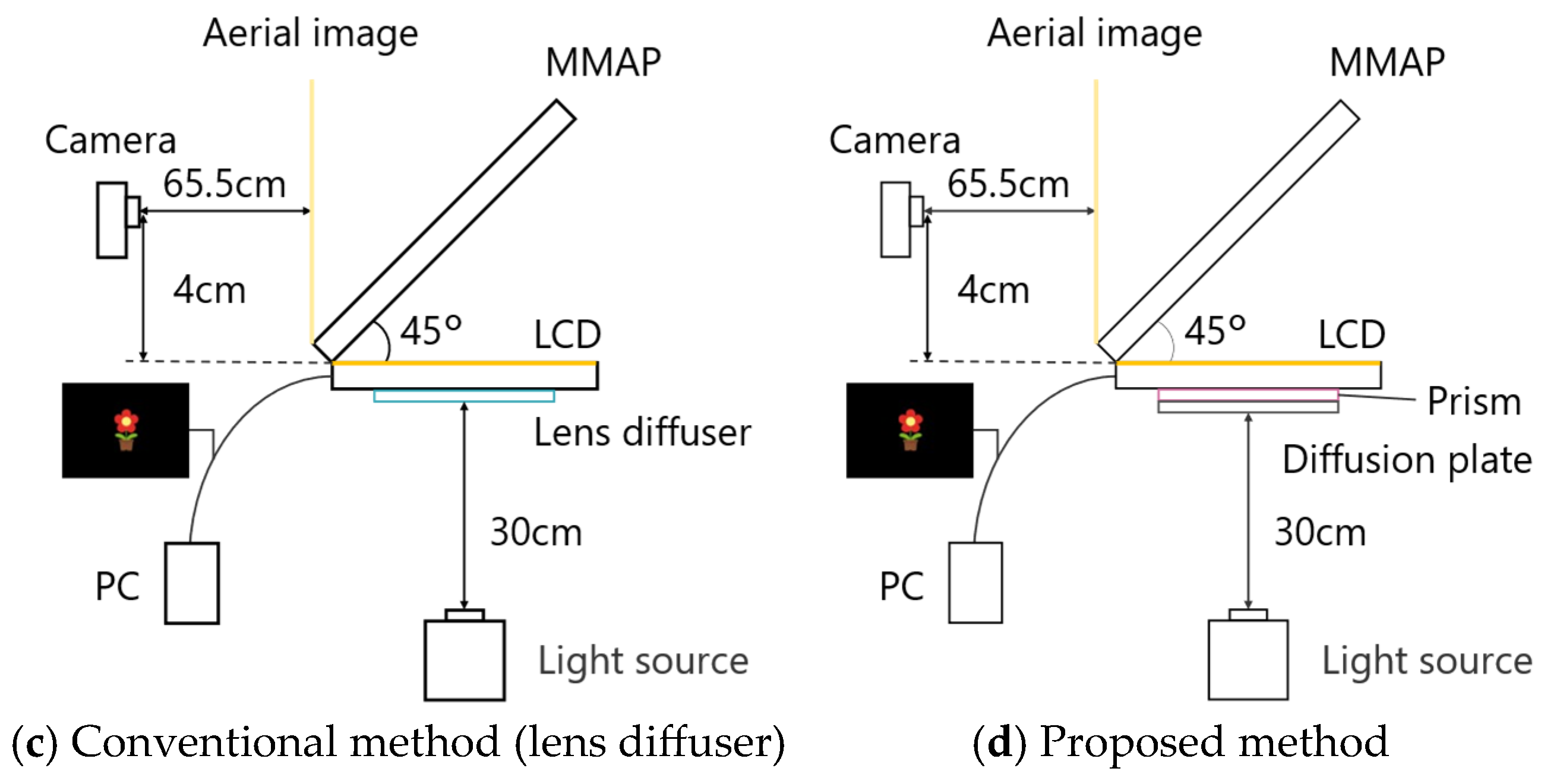
3. Proposed Method
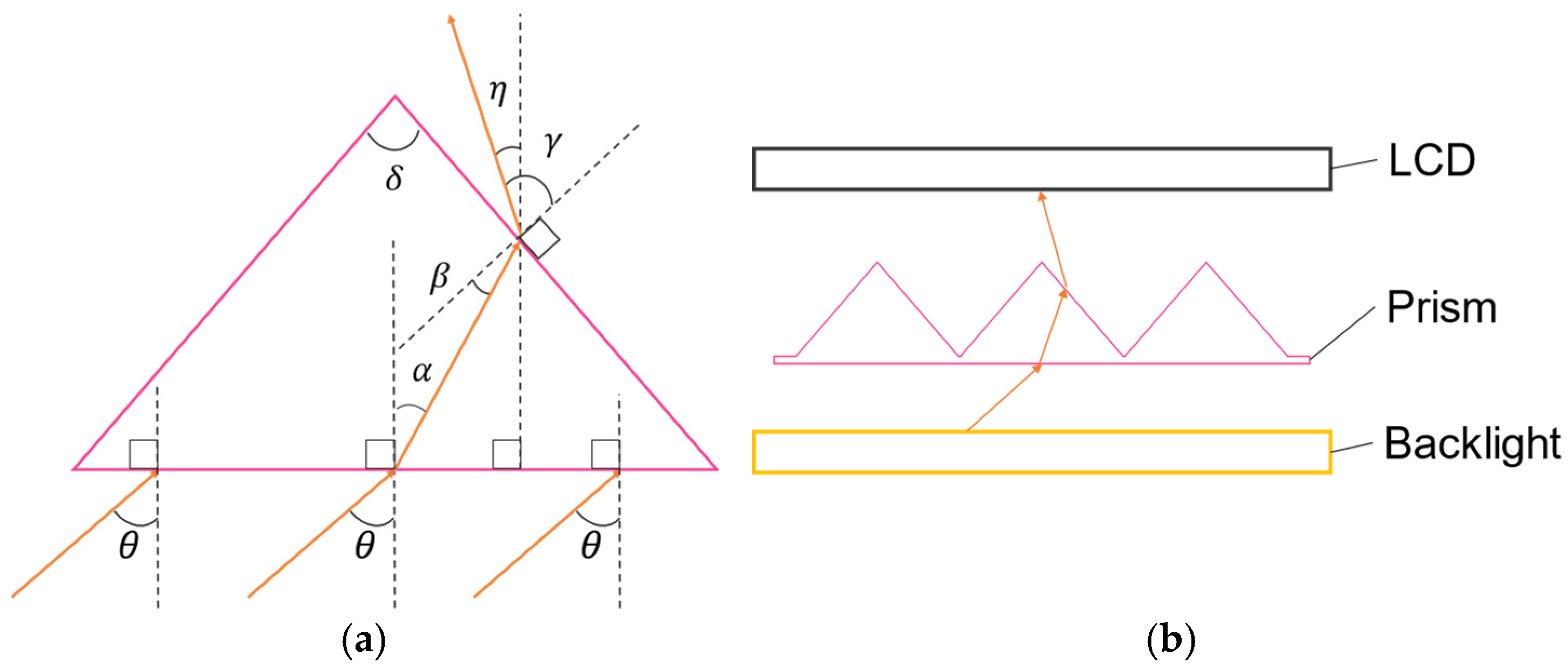
3.1. Principle of the Proposed Method
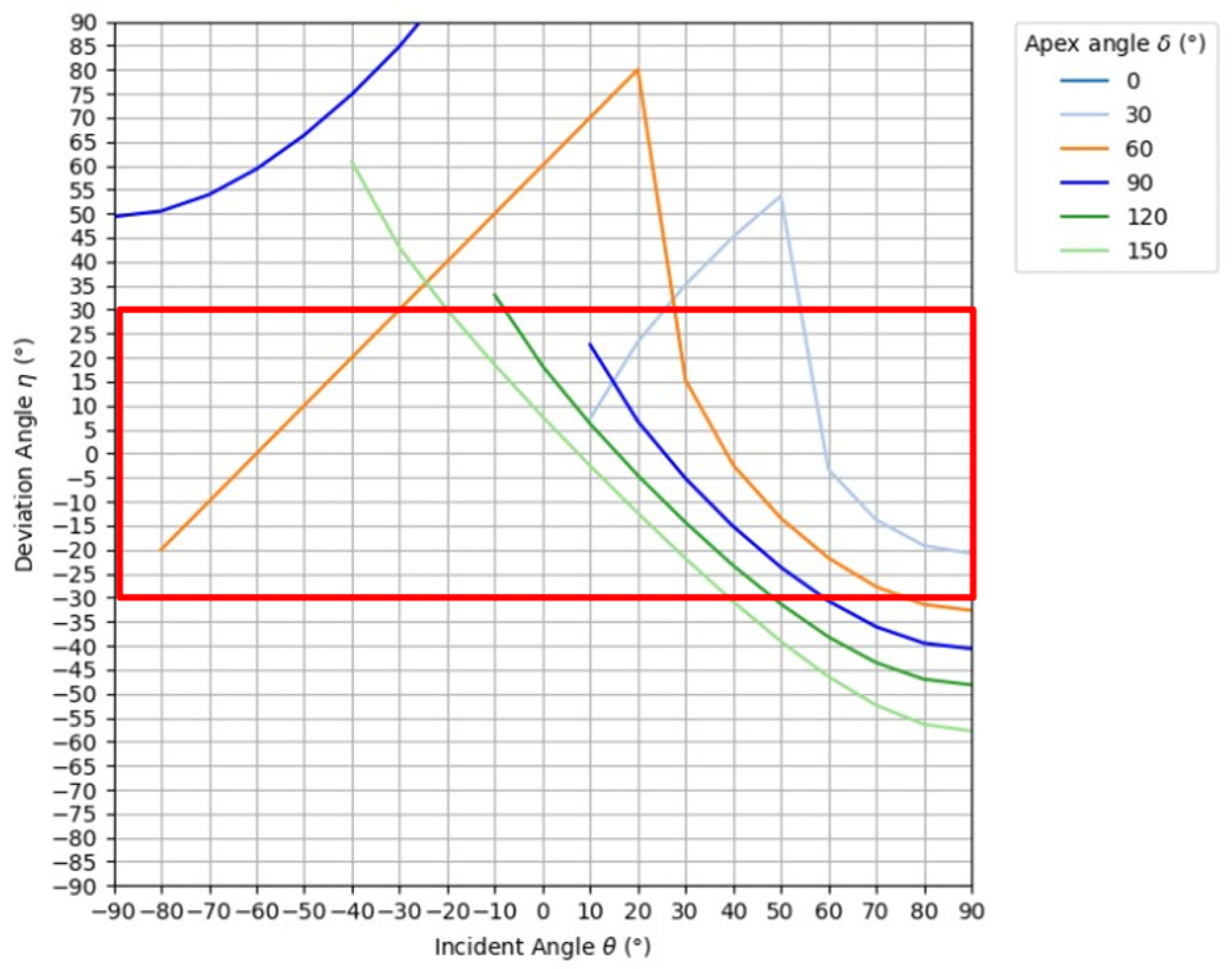
3.2. Simulation of the Prism
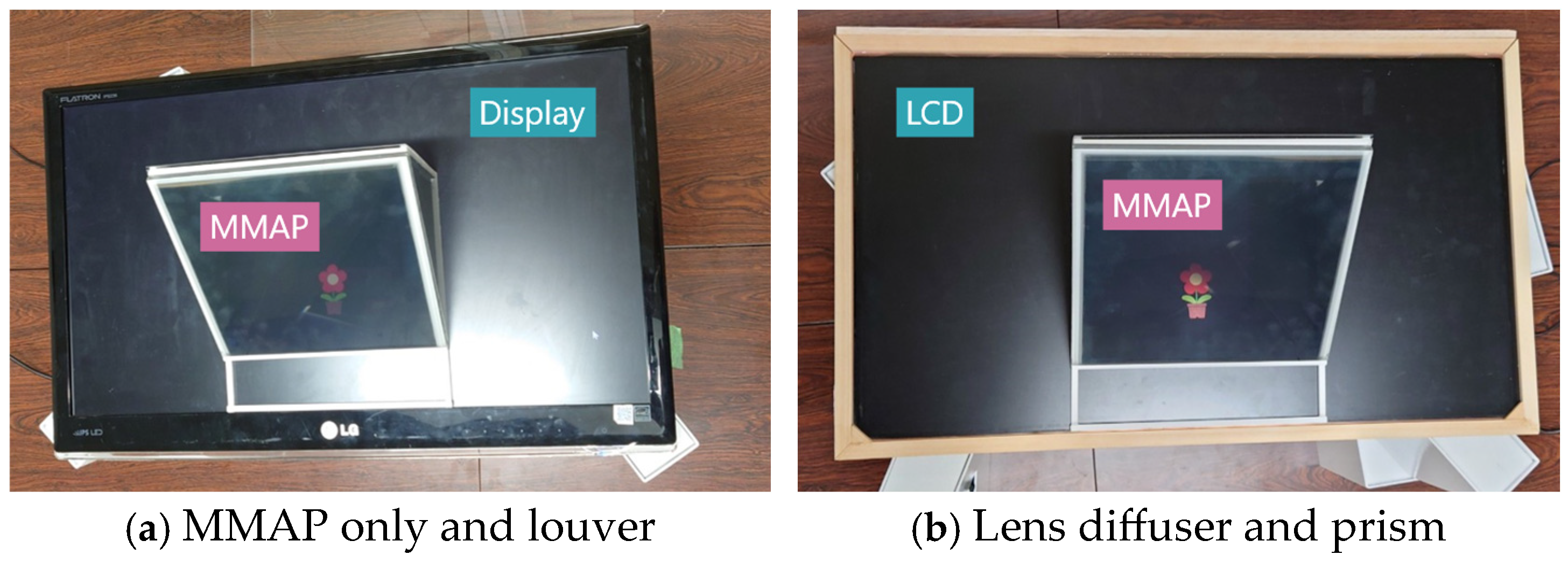
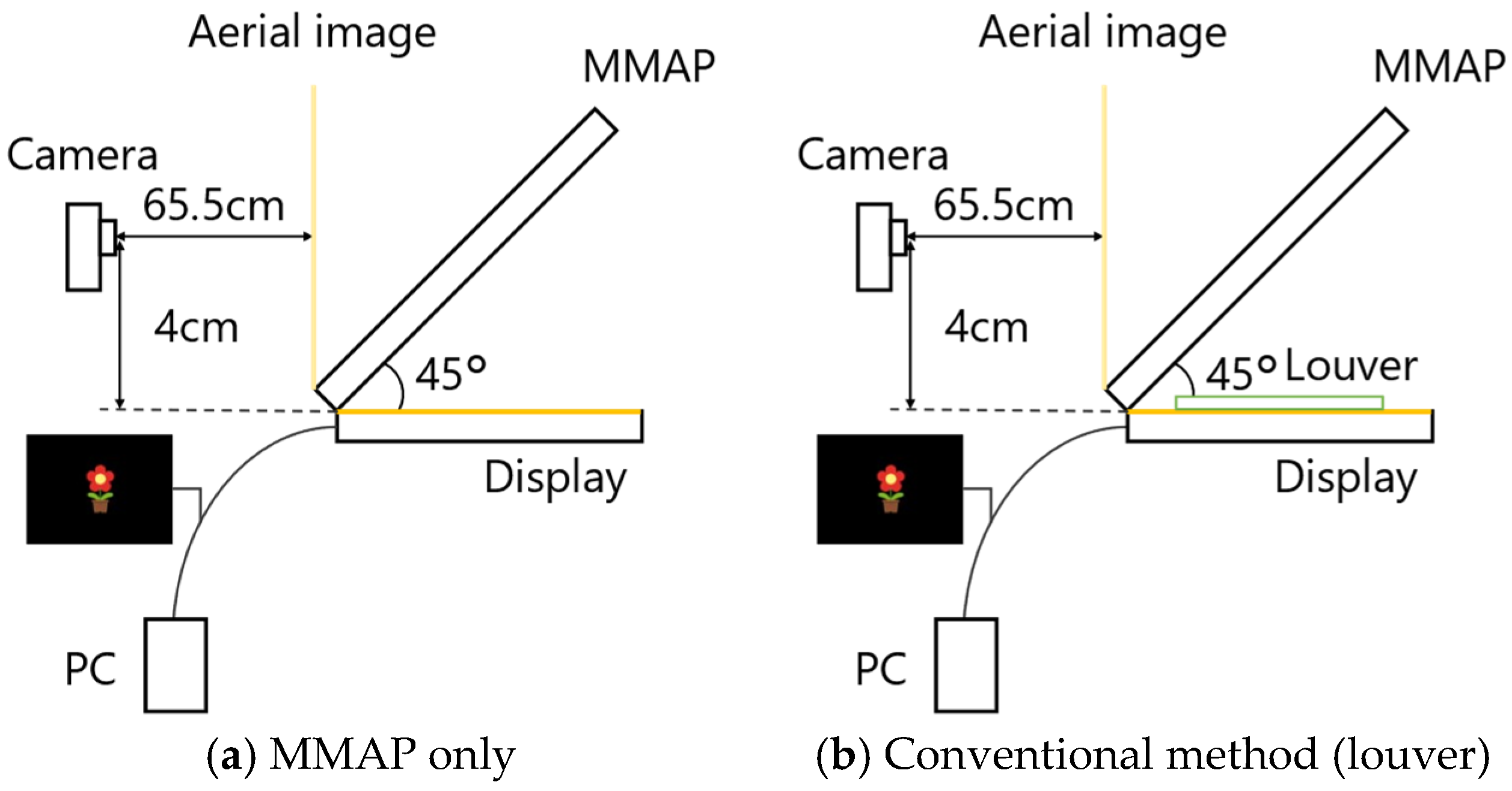
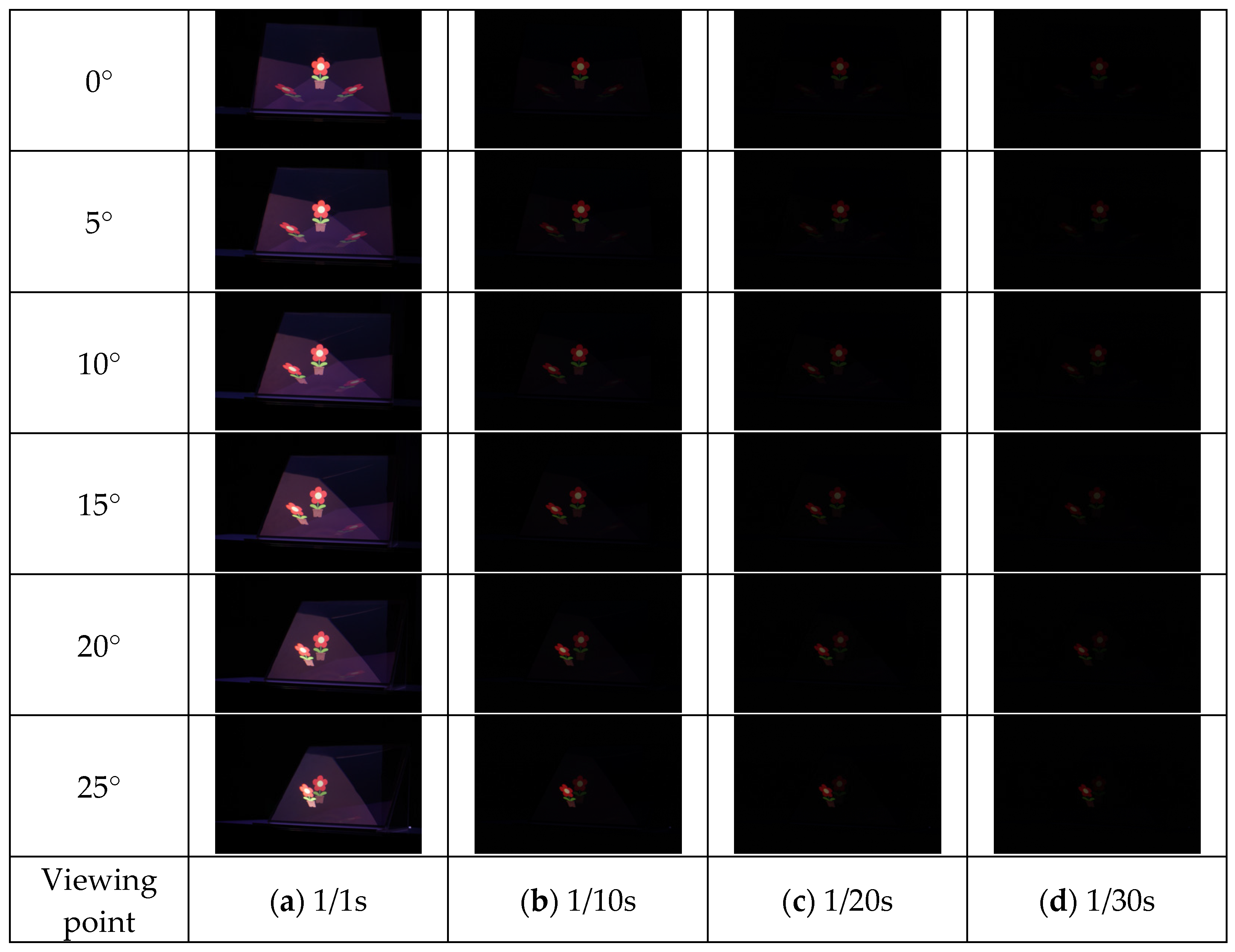
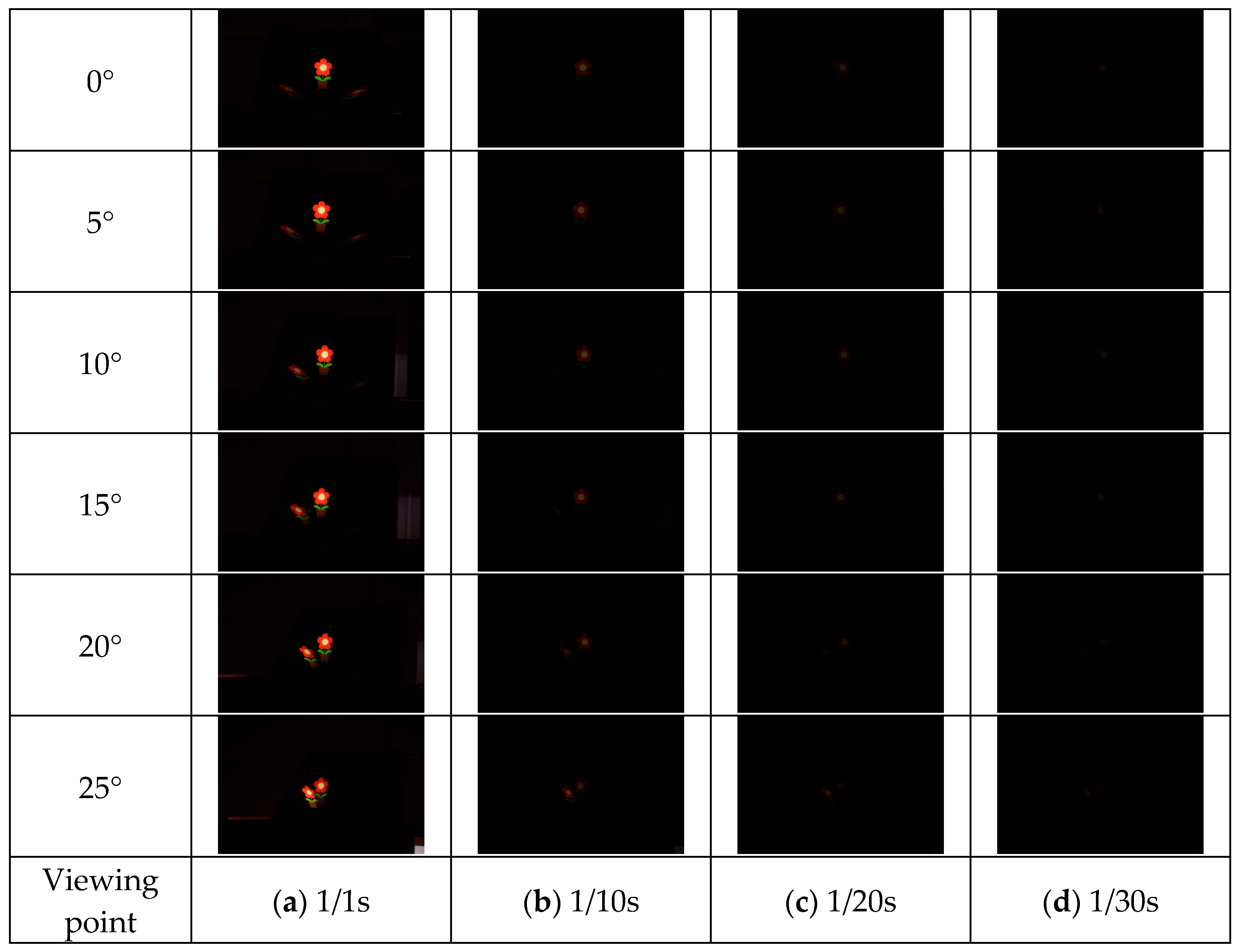
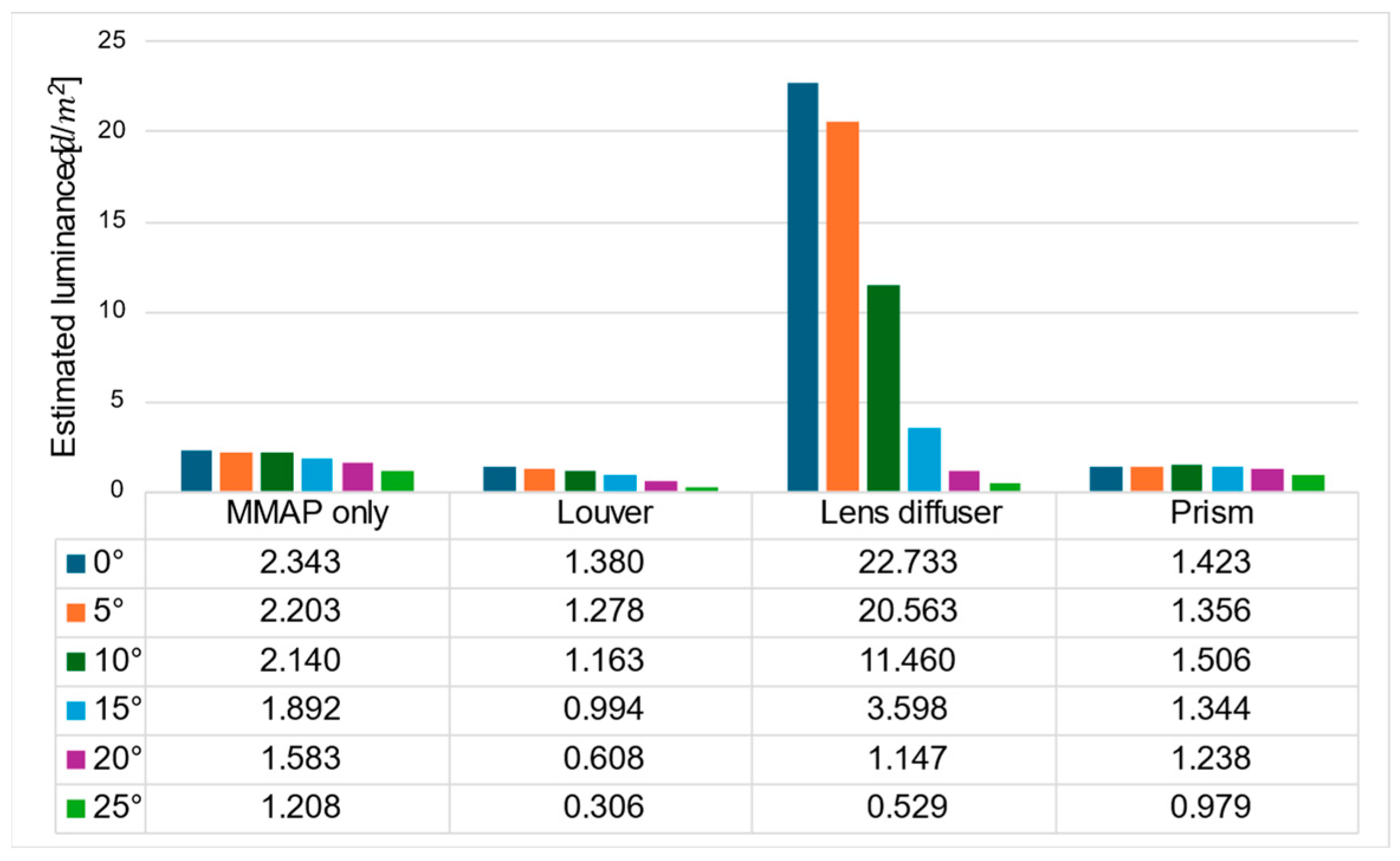
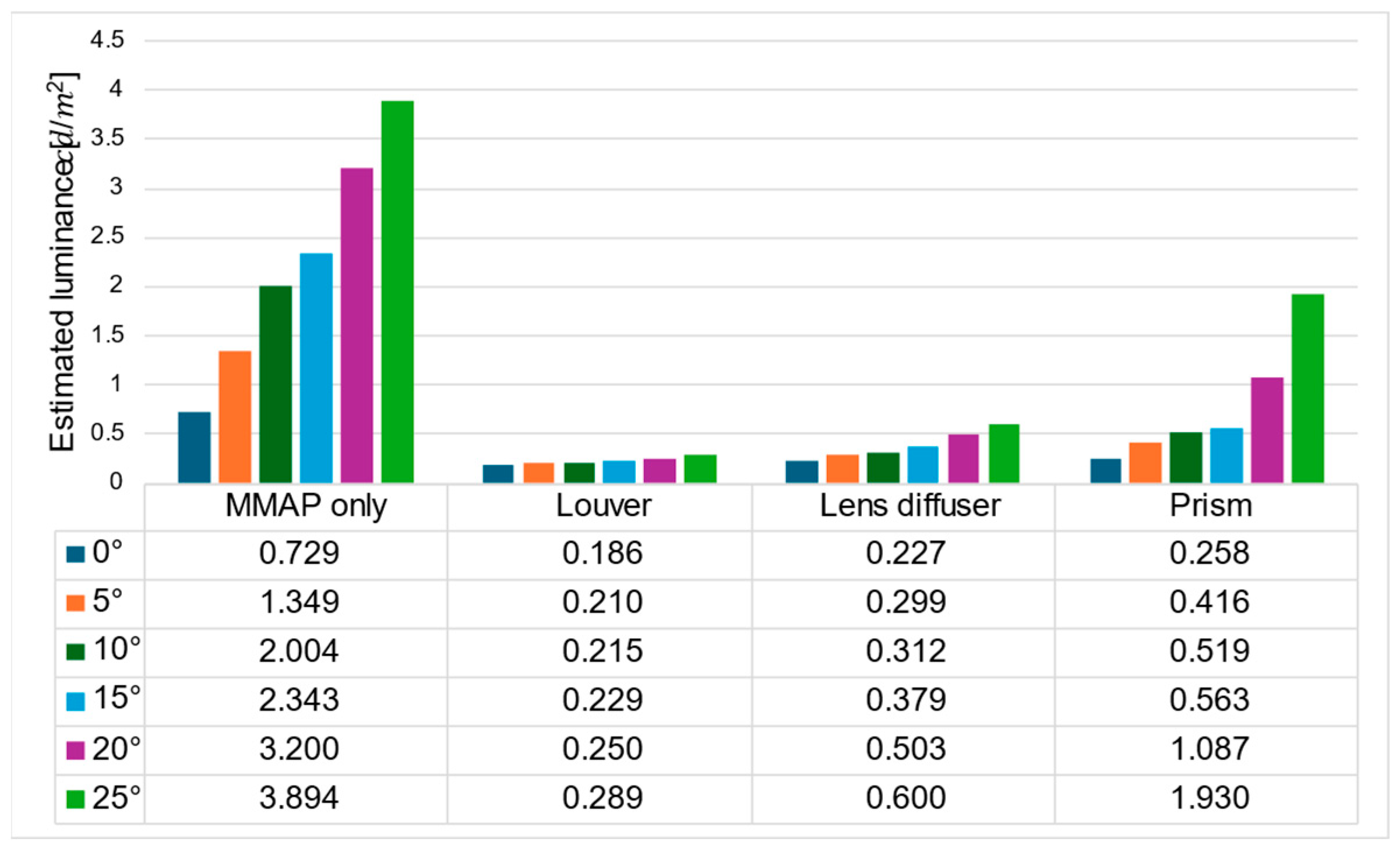
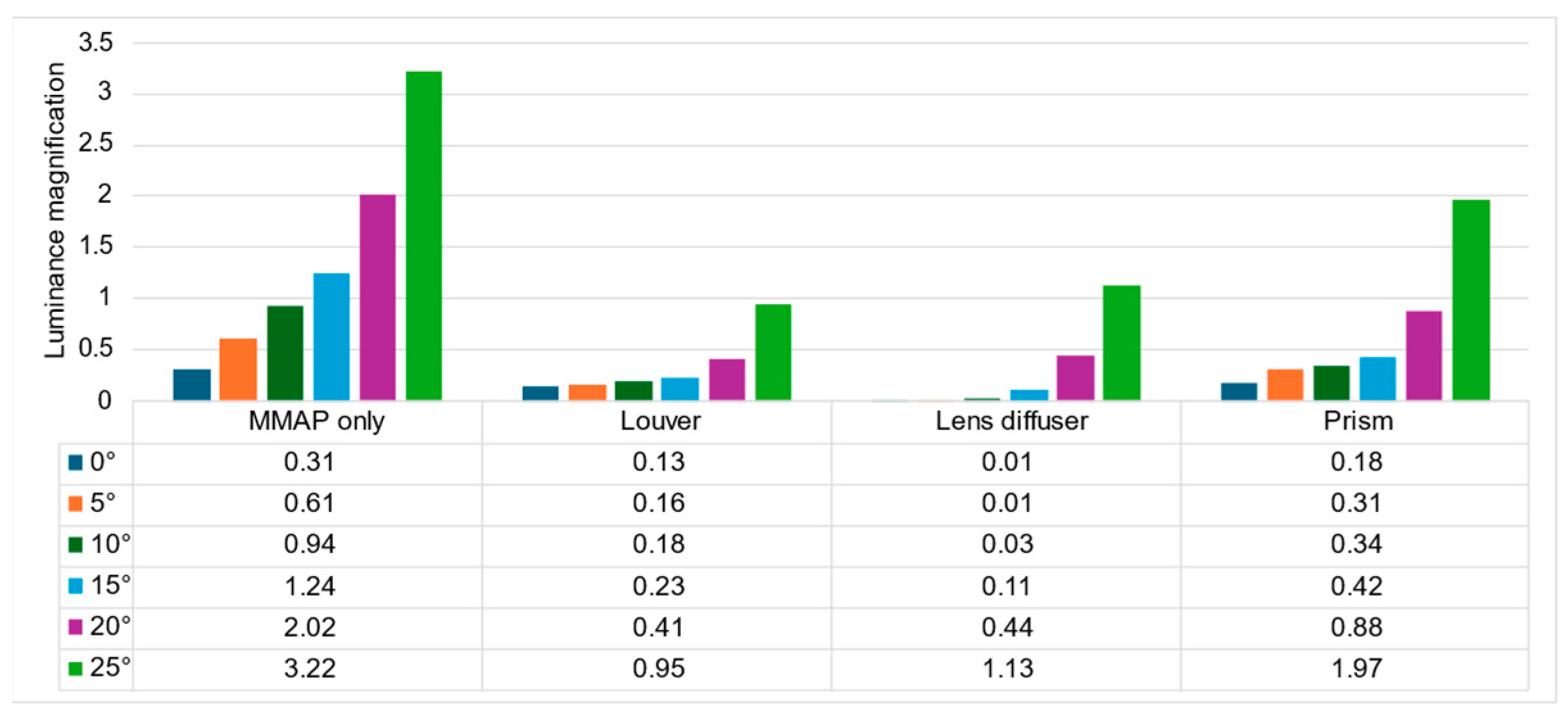
4. Experiments and Results
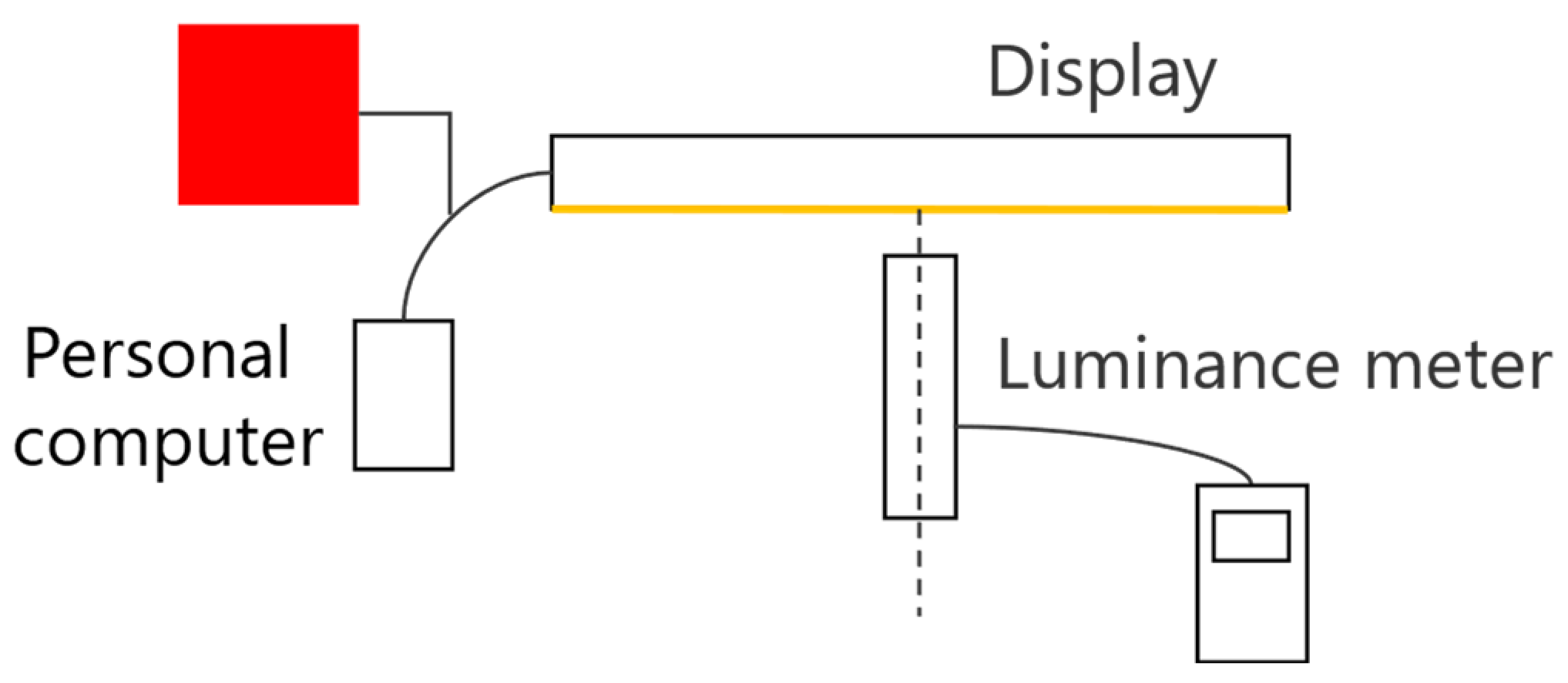
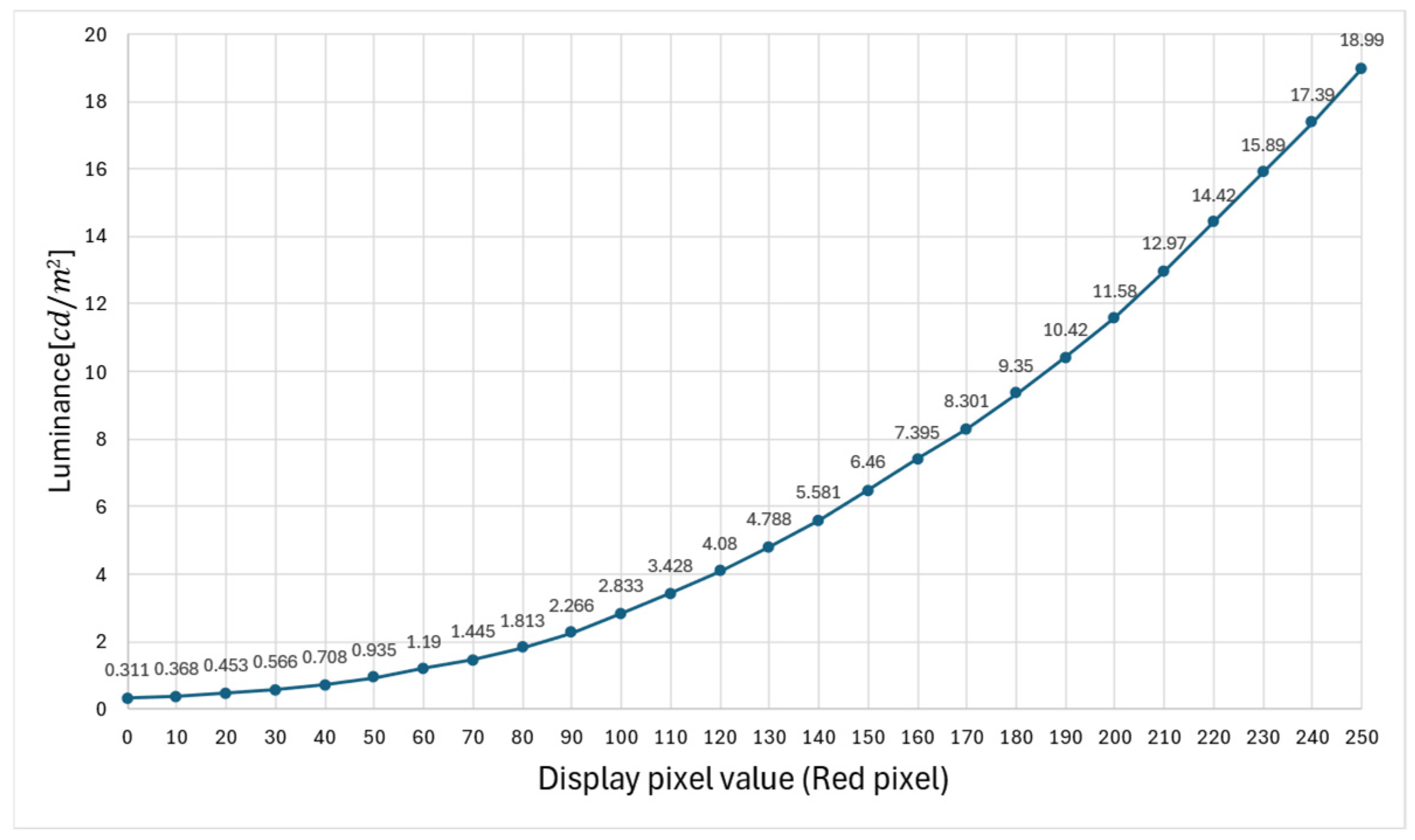
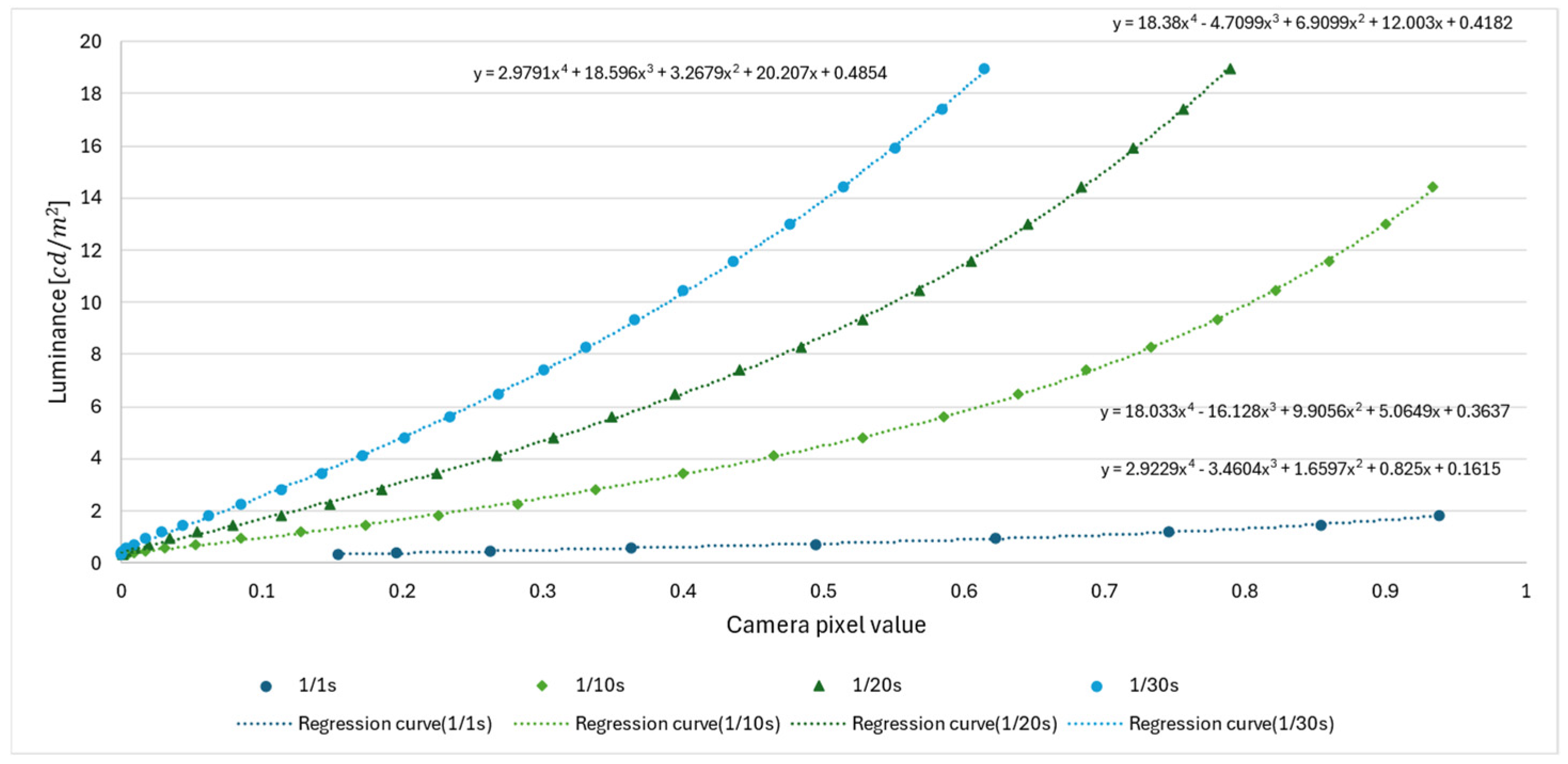
4.1. Determining the Relationship Between the Luminance of Light Sources and the Camera Pixel Values
4.1.1. Calculation of Luminance in Camera-Captured Images
4.1.2. Experiments on Capturing Aerial Images
5. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Yamamoto, H. Fundamentals and Social Implementations of Aerial Display. J. Inst. Image Inf. Telev. Eng. 2021, 75, 181–187. [Google Scholar]
- Kuwayama, T. Aerial display technology to realize a non-contact society. ITE Tech. Rep. 2021, 45, 25–32. [Google Scholar]
- Zhang, N.; Huang, T.; Zhang, X.; Hu, C.; Liao, H. Omnidirectional 3D autostereoscopic aerial display with continuous parallax. J. Opt. Soc. Am. A 2022, 39, 782–792. [Google Scholar] [CrossRef] [PubMed]
- ASKA3D. Available online: https://aska3d.com/ja/ (accessed on 17 December 2024).
- Yamamoto, H.; Tomiyama, Y.; Suyama, S. Floating aerial LED signage based on aerial imaging by retro-reflection (AIRR). Opt. Express 2014, 22, 26919–26924. [Google Scholar] [CrossRef] [PubMed]
- Otao, K.; Itoh, Y.; Takazawa, K.; Osone, H.; Ochiai, Y. Transmissive mirror device based near-eye displays with wide field of view. In Proceedings of the ACM SIGGRAPH 2018 Emerging Technologies (SIGGRAPH ’18), Vancouver, BC, Canada, 12–16 August 2018; Association for Computing Machinery: New York, NY, USA, 2018; pp. 1–2. [Google Scholar] [CrossRef]
- Zhang, H.L.; Deng, H.; Ren, H.; Yang, X.; Xing, Y.; Li, D.H.; Wang, Q.H. Method to eliminate pseudoscopic issue in an integral imaging 3D display by using a transmissive mirror device and light filter. Opt. Lett. 2020, 45, 351–354. [Google Scholar] [CrossRef]
- Miyazaki, D.; Onoda, S.; Maeda, Y.; Mukai, T. Blurring correction for aerial image formed by dihedral corner reflector array. In Proceedings of the 2015 11th Conference on Lasers and Electro-Optics Pacific Rim (CLEO-PR), Busan, Republic of Korea, 24–28 August 2015; pp. 1–2. [Google Scholar] [CrossRef]
- Yamaguchi, A.; Maekawa, S.; Yamane, T.; Okada, I.; Utsumi, Y. Fabrication of a Dihedral Corner Reflector Array for a Floating Image Manufactured by X-ray Lithography Using Synchrotron Radiation. Trans. Jpn. Inst. Electron. Packag. 2015, 8, 23–28. [Google Scholar] [CrossRef]
- Yoshimizu, Y.; Iwase, E. Radially Arranged Dihedral Corner Reflector Array for Wide Viewing Angle of Seamless Floating Image. In Proceedings of the 2019 IEEE 32nd International Conference on Micro Electro Mechanical Systems (MEMS), Seoul, Republic of Korea, 27–31 January 2019; pp. 274–277. [Google Scholar] [CrossRef]
- Hiratani, K.; Iwai, D.; Kageyama, Y.; Punpongsanon, P.; Hiraki, T.; Sato, K. Shadowless Projection Mapping using Retrotransmissive Optics. IEEE Trans. Vis. Comput. Graph. 2023, 29, 2280–2290. [Google Scholar] [CrossRef] [PubMed]
- Sato, Y.; Osada, Y.; Kobayashi, R.; Bao, Y. Aerial floating 3D display device with lenticular-type naked-eye 3D display and a crossed-mirror array. Appl. Opt. 2021, 60, 8267–8276. [Google Scholar] [CrossRef] [PubMed]
- Yuto, O.; Yue, B. Design of Ghost-free Aerial Display by Using Polarizing Plate and Dihedral Corner Reflector Array. In Proceedings of the 2021 5th International Conference on Imaging, Signal Processing and Communications (ICISPC), Kumamoto, Japan, 23–25 July 2021; pp. 67–70. [Google Scholar] [CrossRef]
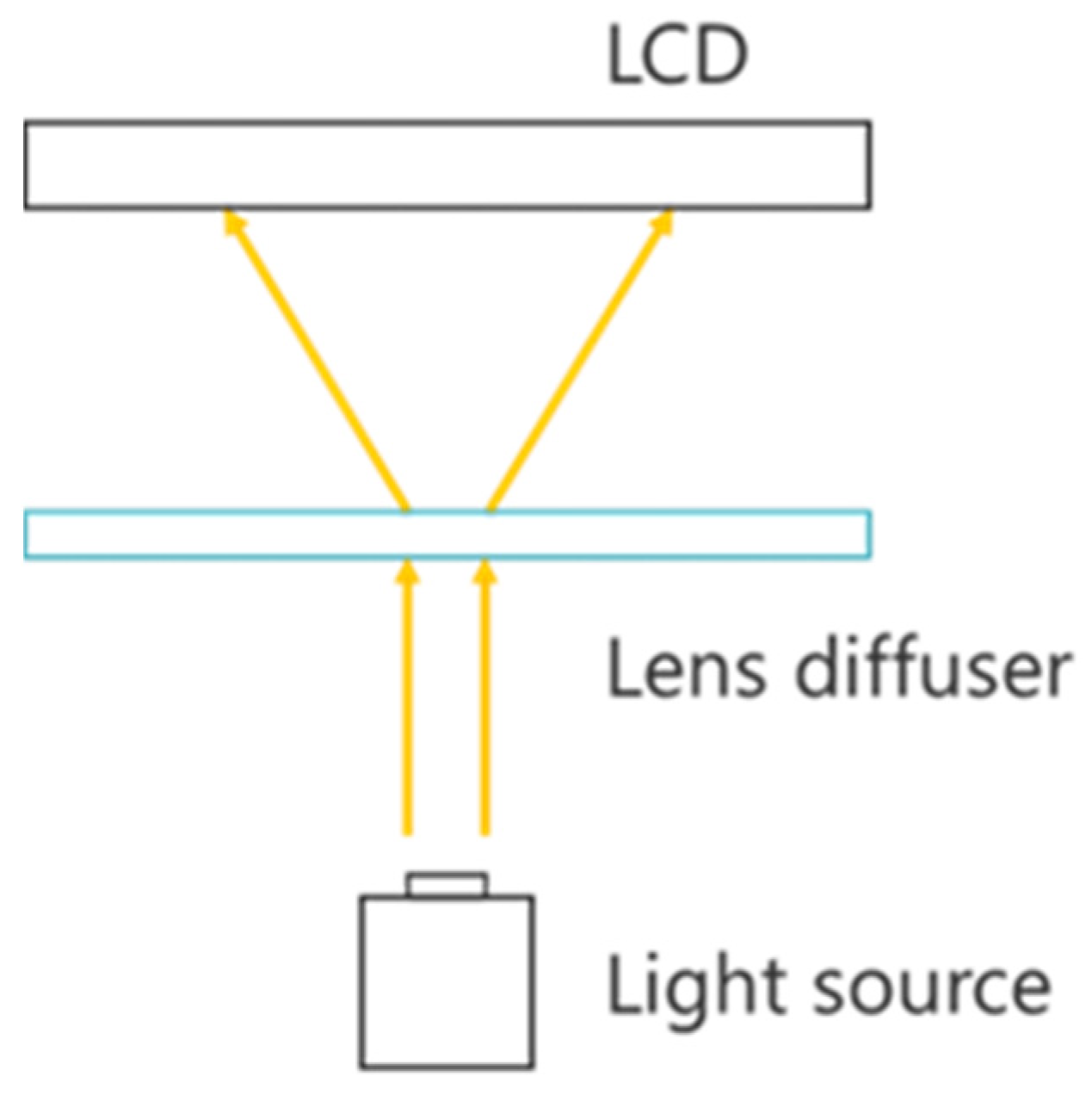
- Kurihara, A.; Bao, Y. Ghost reduction and brightness enhancement of aerial images using lens diffusers in MMAP. Opt. Contin. 2024, 3, 1706–1721. [Google Scholar] [CrossRef]
- Yamamoto, H. Recent Developments and Prospective Applications of Aerial Display; CMC Publishing Co., Ltd.: Tokyo, Japan, 2018; pp. 35–45. [Google Scholar]
- Industrial Lens Diffuser: LSD. Available online: https://www.osc-japan.com/lsd-corp/ (accessed on 17 December 2024).
- Yoshinori, M.; Akio, S.; Akira, T.; Masahiro, T.; Kazumasa, K.; Hiroaki, Y.; Masayuki, K. New backliting technologies for liquid crystal display (IV). ITEJ Tech. Rep. 1993, 17, 33–38. [Google Scholar]
- Kawai, H. Plastic molding materials for precision optics. Jpn. J. Opt. 1995, 24, 69–75. [Google Scholar]
- Junichi, S. Introduction to Optics; Corona Publishing Co., Ltd.: Tokyo, Japan, 1997; pp. 132–137. [Google Scholar]









| First Layer | |||
|---|---|---|---|
| Odd Times | Even Times | ||
| Second layer | Odd times | Aerial image | Ghost |
| Even times | Ghost | Transmitted light | |
| Equipment | Parameters | Specification |
|---|---|---|
| MMAP | model number | ASKA3D-200NT |
| size | 200 × 200 mm | |
| pitch | 0.3 mm | |
| viewing angle | 40° | |
| material | Optical Resins | |
| Display, LCD | model number | LG Electronics IPS236V |
| resolution | 1920 × 1080 pixels (23 inch) Used 125 × 125 mm inside | |
| LED | model number | OHM LED E26 L70313 |
| Prism array | model number | LPV-200S-90-0.05 |
| size | 200 × 200 × 2 mm | |
| apex angle | 90° | |
| prism pitch | 0.05 mm | |
| material | PMMA | |
| index of refraction | 1.492 | |
| Diffusion plate | Disassembly source | IBM T221TFT LCD |
| Lens diffuser | model number | LSD10PC10-5 |
| diffusion angle [deg] | 10 | |
| size | 5.0 × 5.0 inch | |
| material | polycarbonate | |
| Louver | model number | Hikari kogyo Looknon-N8 |
| viewing angle | 60° | |
| visible light transmittance | 71.2% | |
| material | PET | |
| Camera | model number | SONY α6000 |
| lens | E 30mm F3.5 Macro |
| Aerial Image | Ghost | |||||||
|---|---|---|---|---|---|---|---|---|
| 1/1 s | 1/10 s | 1/20 s | 1/30 s | 1/1 s | 1/10 s | 1/20 s | 1/30 s | |
| 0.994 | 0.285 | 0.149 | 0.089 | 0.534 | 0.059 | 0.024 | 0.011 | |
| 0.975 | 0.268 | 0.138 | 0.083 | 0.829 | 0.160 | 0.073 | 0.040 | |
| 0.974 | 0.266 | 0.141 | 0.081 | 0.958 | 0.249 | 0.128 | 0.074 | |
| 0.936 | 0.233 | 0.120 | 0.068 | 0.994 | 0.290 | 0.157 | 0.094 | |
| 0.882 | 0.191 | 0.093 | 0.053 | 0.999 | 0.409 | 0.231 | 0.150 | |
| 0.772 | 0.141 | 0.061 | 0.034 | 1.000 | 0.498 | 0.288 | 0.189 | |
| Aerial Image | Ghost | |||||||
|---|---|---|---|---|---|---|---|---|
| 1/1 s | 1/10 s | 1/20 s | 1/30 s | 1/1 s | 1/10 s | 1/20 s | 1/30 s | |
| 0.845 | 0.162 | 0.073 | 0.042 | 0.028 | 0.001 | 0.000 | 0.000 | |
| 0.809 | 0.148 | 0.067 | 0.037 | 0.053 | 0.001 | 0.000 | 0.000 | |
| 0.767 | 0.130 | 0.058 | 0.032 | 0.059 | 0.001 | 0.000 | 0.000 | |
| 0.694 | 0.105 | 0.044 | 0.024 | 0.073 | 0.002 | 0.001 | 0.000 | |
| 0.419 | 0.040 | 0.016 | 0.008 | 0.093 | 0.003 | 0.001 | 0.000 | |
| 0.144 | 0.006 | 0.002 | 0.001 | 0.129 | 0.005 | 0.001 | 0.001 | |
| Aerial Image | Ghost | |||||||
|---|---|---|---|---|---|---|---|---|
| 1/1 s | 1/10 s | 1/20 s | 1/30 s | 1/1 s | 1/10 s | 1/20 s | 1/30 s | |
| 0.998 | 1.000 | 0.999 | 0.923 | 0.071 | 0.002 | 0.001 | 0.000 | |
| 0.998 | 1.000 | 0.970 | 0.842 | 0.138 | 0.005 | 0.002 | 0.001 | |
| 1.000 | 0.921 | 0.690 | 0.535 | 0.149 | 0.007 | 0.002 | 0.001 | |
| 1.000 | 0.457 | 0.264 | 0.174 | 0.208 | 0.013 | 0.004 | 0.002 | |
| 0.759 | 0.130 | 0.057 | 0.030 | 0.315 | 0.025 | 0.009 | 0.004 | |
| 0.342 | 0.029 | 0.011 | 0.006 | 0.416 | 0.038 | 0.015 | 0.007 | |
| Aerial image | Ghost | |||||||
|---|---|---|---|---|---|---|---|---|
| 1/1 s | 1/10 s | 1/20 s | 1/30 s | 1/1 s | 1/10 s | 1/20 s | 1/30 s | |
| 0.859 | 0.168 | 0.078 | 0.044 | 0.100 | 0.004 | 0.001 | 0.000 | |
| 0.843 | 0.159 | 0.072 | 0.040 | 0.239 | 0.017 | 0.005 | 0.003 | |
| 0.881 | 0.180 | 0.082 | 0.049 | 0.334 | 0.027 | 0.010 | 0.004 | |
| 0.834 | 0.158 | 0.071 | 0.040 | 0.381 | 0.033 | 0.012 | 0.006 | |
| 0.801 | 0.142 | 0.063 | 0.035 | 0.726 | 0.118 | 0.052 | 0.029 | |
| 0.859 | 0.168 | 0.078 | 0.044 | 0.100 | 0.004 | 0.001 | 0.000 | |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2025 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Shoji, K.; Osada, Y.; Kurihara, A.; Bao, Y. Reduction of Uneven Brightness and Ghosts of Aerial Images Using a Prism in a Micromirror Array Plate. J. Imaging 2025, 11, 75. https://doi.org/10.3390/jimaging11030075
Shoji K, Osada Y, Kurihara A, Bao Y. Reduction of Uneven Brightness and Ghosts of Aerial Images Using a Prism in a Micromirror Array Plate. Journal of Imaging. 2025; 11(3):75. https://doi.org/10.3390/jimaging11030075
Chicago/Turabian StyleShoji, Kaito, Yuto Osada, Atsutoshi Kurihara, and Yue Bao. 2025. "Reduction of Uneven Brightness and Ghosts of Aerial Images Using a Prism in a Micromirror Array Plate" Journal of Imaging 11, no. 3: 75. https://doi.org/10.3390/jimaging11030075
APA StyleShoji, K., Osada, Y., Kurihara, A., & Bao, Y. (2025). Reduction of Uneven Brightness and Ghosts of Aerial Images Using a Prism in a Micromirror Array Plate. Journal of Imaging, 11(3), 75. https://doi.org/10.3390/jimaging11030075






