Analysis of Essential Features and Optimal Operational Parameters of an RF-ICP Torch for Waste Treatment Applications
Abstract
1. Introduction
2. Results
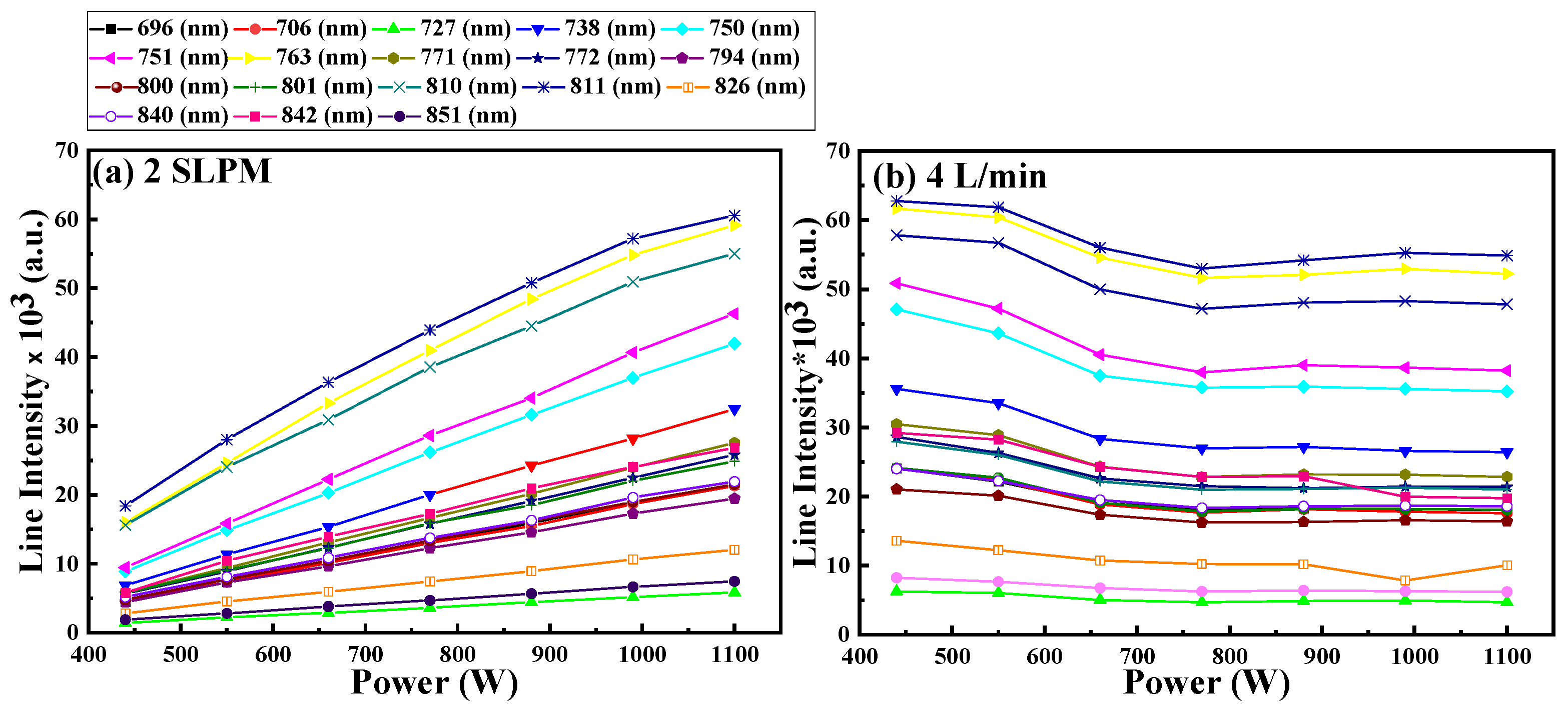
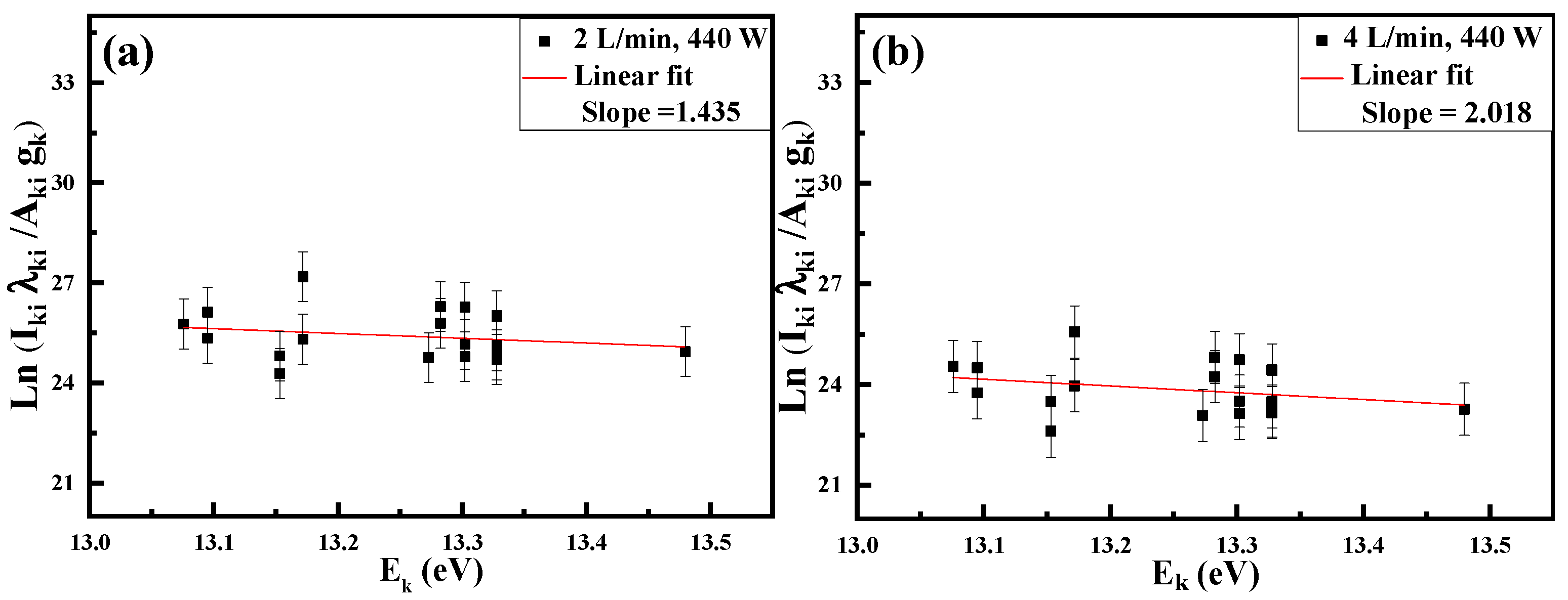
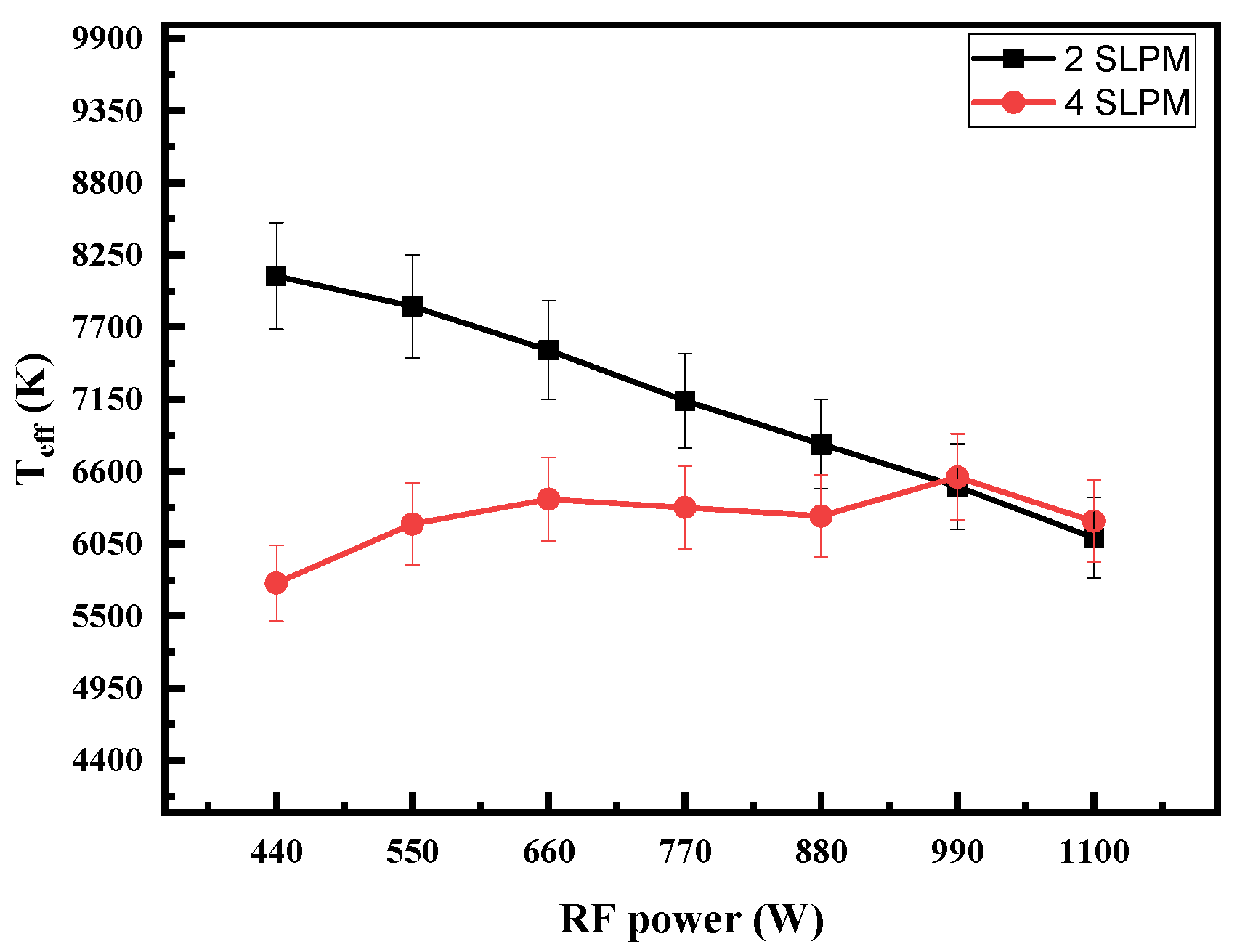
2.1. Excitation Temperature
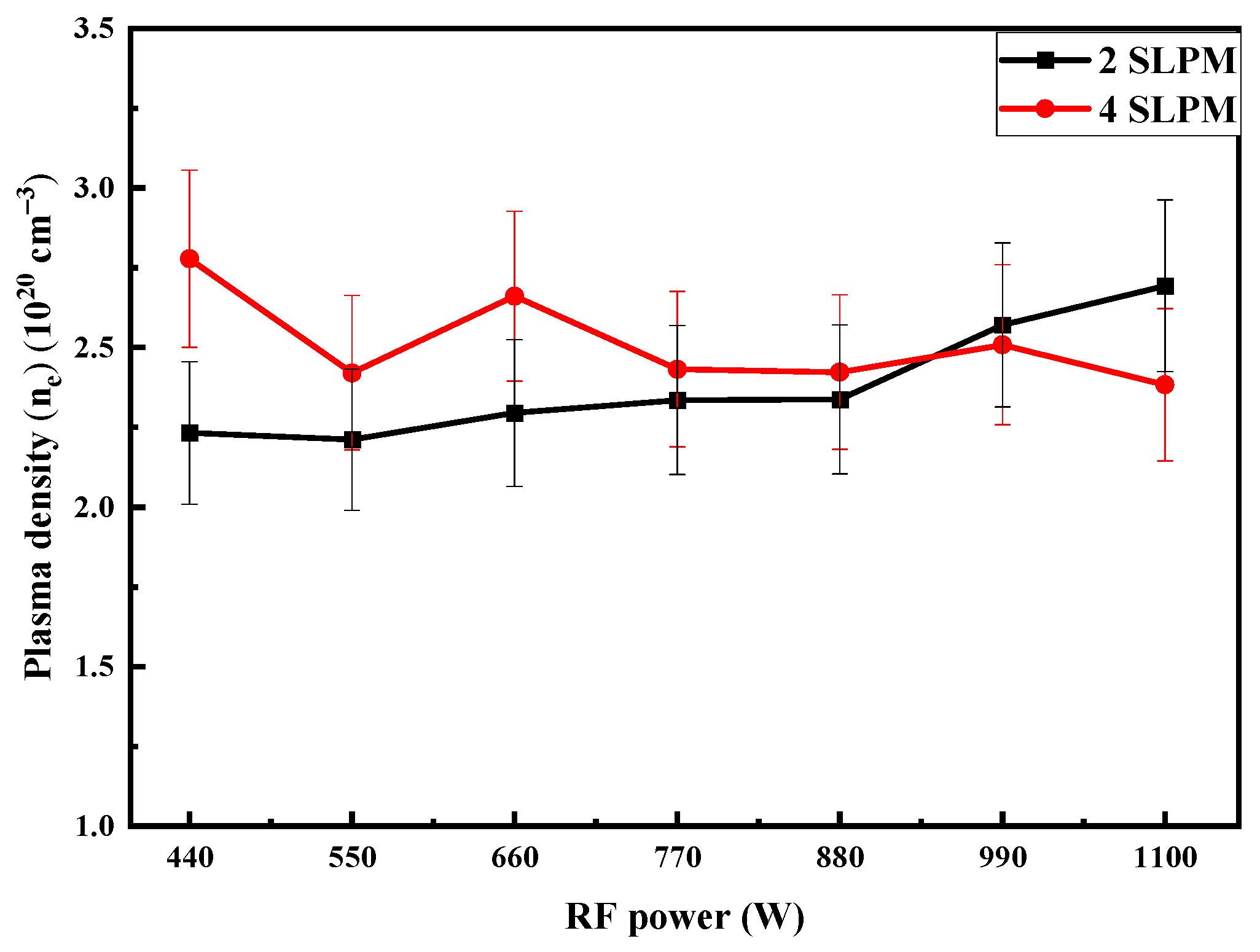
2.2. Electron Density
3. Material and Methods
4. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
References
- Lombardi, L.; Carnevale, E.; Corti, A. A Review of Technologies and Performances of Thermal Treatment Systems for Energy Recovery from Waste. Waste Manag. 2015, 37, 26–44. [Google Scholar] [CrossRef] [PubMed]
- Brunner, P.H. WTE: Thermal Waste Treatment for Sustainable Waste Management. In Recovery of Materials and Energy from Urban Wastes; Springer New York: New York, NY, USA, 2019; pp. 523–536. [Google Scholar]
- Leckner, B. Process Aspects in Combustion and Gasification Waste-to-Energy (WtE) Units. Waste Manag. 2015, 37, 13–25. [Google Scholar] [CrossRef] [PubMed]
- Panepinto, D.; Tedesco, V.; Brizio, E.; Genon, G. Environmental Performances and Energy Efficiency for MSW Gasification Treatment. Waste Biomass Valorization 2015, 6, 123–135. [Google Scholar] [CrossRef]
- Manisalidis, I.; Stavropoulou, E.; Stavropoulos, A.; Bezirtzoglou, E. Environmental and Health Impacts of Air Pollution: A Review. Front. Public Health 2020, 8, 505570. [Google Scholar] [CrossRef] [PubMed]
- Dong, J.; Tang, Y.; Nzihou, A.; Chi, Y.; Weiss-Hortala, E.; Ni, M. Life Cycle Assessment of Pyrolysis, Gasification and Incineration Waste-to-Energy Technologies: Theoretical Analysis and Case Study of Commercial Plants. Sci. Total Environ. 2018, 626, 744–753. [Google Scholar] [CrossRef] [PubMed]
- Safavi, S.M.; Richter, C.; Unnthorsson, R. Dioxin and Furan Emissions from Gasification. In Gasification; Silva, V., Tuna, C.E., Eds.; IntechOpen: Rijeka, Croatia, 2021; p. 5. ISBN 978-1-83968-796-9. [Google Scholar]
- Lopes, E.J.; Okamura, L.A.; Yamamoto, C.I. Formation of dioxins and furans during municipal solid waste gasification. Braz. J. Chem. Eng. 2015, 32, 87–97. [Google Scholar] [CrossRef]
- Magoua Mbeugang, C.F.; Li, B.; Lin, D.; Xie, X.; Wang, S.; Wang, S.; Zhang, S.; Huang, Y.; Liu, D.; Wang, Q. Hydrogen Rich Syngas Production from Sorption Enhanced Gasification of Cellulose in the Presence of Calcium Oxide. Energy 2021, 228, 120659. [Google Scholar] [CrossRef]
- Cortazar, M.; Santamaria, L.; Lopez, G.; Alvarez, J.; Zhang, L.; Wang, R.; Bi, X.; Olazar, M. A Comprehensive Review of Primary Strategies for Tar Removal in Biomass Gasification. Energy Convers. Manag. 2023, 276, 116496. [Google Scholar] [CrossRef]
- Yadav, A.; Karmakar, S.; Kar, S.; Kumar, M. Numerical Modelling of a Direct Current Non-Transferred Thermal Plasma Torch for Optimal Performance. Contrib. Plasma Phys. 2023, 63, e202200088. [Google Scholar] [CrossRef]
- Li, J.; Liu, K.; Yan, S.; Li, Y.; Han, D. Application of Thermal Plasma Technology for the Treatment of Solid Wastes in China: An Overview. Waste Manag. 2016, 58, 260–269. [Google Scholar] [CrossRef]
- Nema, S.K.; Ganeshprasad, K.S. Plasma Pyrolysis of Medical Waste. Curr. Sci. 2002, 83, 271–278. [Google Scholar]
- Bosmans, A.; Vanderreydt, I.; Geysen, D.; Helsen, L. The Crucial Role of Waste-to-Energy Technologies in Enhanced Landfill Mining: A Technology Review. J. Clean. Prod. 2013, 55, 10–23. [Google Scholar] [CrossRef]
- Rueda, Y.G.; Helsen, L. The Role of Plasma in Syngas Tar Cracking. Biomass Convers. Biorefin. 2020, 10, 857–871. [Google Scholar] [CrossRef]
- Mostaghimi, J.; Boulos, M.I. Thermal Plasma Sources: How Well Are They Adopted to Process Needs? Plasma Chem. Plasma Process. 2015, 35, 421–436. [Google Scholar] [CrossRef]
- Munir, M.T.; Mardon, I.; Al-Zuhair, S.; Shawabkeh, A.; Saqib, N.U. Plasma Gasification of Municipal Solid Waste for Waste-to-Value Processing. Renew. Sustain. Energy Rev. 2019, 116, 109461. [Google Scholar] [CrossRef]
- Pancholi, K.C.; Sen, N.; Singh, K.K.; Vincent, T.; Kaushik, C.P. Transient Heat Transfer during Startup of a Thermal Plasma Chamber: Numerical Insights. Prog. Nucl. Energy 2022, 152, 104371. [Google Scholar] [CrossRef]
- Tang, L.; Huang, H. Some observations from studies of rf plasma pyrolysis of waste tires. Chem. Eng. Commun. 2010, 197, 1541–1552. [Google Scholar] [CrossRef]
- Khongkrapan, P.; Thanompongchart, P.; Tippayawong, N.; Kiatsiriroat, T. Microwave Plasma Assisted Pyrolysis of Refuse Derived Fuels. Cent. Eur. J. Eng. 2014, 4, 72–79. [Google Scholar] [CrossRef]
- Zhang, Q.; Dor, L.; Fenigshtein, D.; Yang, W.; Blasiak, W. Gasification of Municipal Solid Waste in the Plasma Gasification Melting Process. Appl. Energy 2012, 90, 106–112. [Google Scholar] [CrossRef]
- Sanito, R.C.; You, S.-J.; Wang, Y.-F. Application of Plasma Technology for Treating E-Waste: A Review. J. Environ. Manag. 2021, 288, 112380. [Google Scholar] [CrossRef]
- Gao, P.; Jia, C.-C.; Cao, W.-B.; Wang, C.-C.; Xu, G.-L.; Liang, D.; Cui, Z.-W. Dielectric Properties of AlN/Mo Composite Ceramics Prepared by Spark Plasma Sintering Method at Different Processing Conditions. Rare Met. 2022, 41, 1369–1374. [Google Scholar] [CrossRef]
- Muro-Fraguas, I.; Sainz-García, A.; López, M.; Rojo-Bezares, B.; Múgica-Vidal, R.; Sainz-García, E.; Toledano, P.; Sáenz, Y.; González-Marcos, A.; Alba-Elías, F. Antibiofilm Coatings through Atmospheric Pressure Plasma for 3D Printed Surgical Instruments. Surf. Coat. Technol. 2020, 399, 126163. [Google Scholar] [CrossRef] [PubMed]
- Boulos, M.I.; Fauchais, P.L.; Pfender, E. The Plasma State. In Handbook of Thermal Plasmas; Springer: Cham, Switzerland, 2016; pp. 1–53. [Google Scholar] [CrossRef]
- Conrads, H.; Schmidt, M. Plasma Generation and Plasma Sources. Plasma Sources Sci. Technol. 2000, 9, 441–454. [Google Scholar] [CrossRef]
- Reed, T.B. Induction-Coupled Plasma Torch. J. Appl. Phys. 1961, 32, 821–824. [Google Scholar] [CrossRef]
- Fazekas, P.; Czégény, Z.; Mink, J.; Bódis, E.; Klébert, S.; Németh, C.; Keszler, A.M.; Károly, Z.; Szépvölgyi, J. Decomposition of Poly(Vinyl Chloride) in Inductively Coupled Radiofrequency Thermal Plasma. Chem. Eng. J. 2016, 302, 163–171. [Google Scholar] [CrossRef]
- Major, K.; Veilleux, J.; Brisard, G. Lithium Iron Phosphate Powders and Coatings Obtained by Means of Inductively Coupled Thermal Plasma. J. Therm. Spray Technol. 2016, 25, 357–364. [Google Scholar] [CrossRef]
- Darthout, É.; Gitzhofer, F. Thermal Cycling and High-Temperature Corrosion Tests of Rare Earth Silicate Environmental Barrier Coatings. J. Therm. Spray Technol. 2017, 26, 1823–1837. [Google Scholar] [CrossRef]
- Darthout, É.; Gitzhofer, F. Structure Stabilization by Zirconia Pinning Effect of Y2Si2O7 Environmental Barrier Coatings Synthesized by Solution Precursor Plasma Spraying Process. Surf. Coat. Technol. 2017, 309, 1081–1088. [Google Scholar] [CrossRef]
- Deng, Z.-Q.; Mao, J.; Liu, M.; Deng, C.-M.; Ma, J.-T. Regional Characteristic of 7YSZ Coatings Prepared by Plasma Spray-Physical Vapor Deposition Technique. Rare Met. 2021, 40, 3308–3315. [Google Scholar] [CrossRef]
- Berghaus, J.O.; Meunier, J.L.; Gitzhofer, F. Monitoring and Control of RF Thermal Plasma Diamond Deposition via Substrate. Meas. Sci. Technol. 2003, 15, 161. [Google Scholar] [CrossRef]
- Aldeeb, M.A.; Morgan, N.; Abouelsayed, A.; Amin, K.M.; Hassaballa, S. Correlation of Acetylene Plasma Discharge Environment and the Optical and Electronic Properties of the Hydrogenated Amorphous Carbon Films. Diam. Relat. Mater. 2019, 96, 74–84. [Google Scholar] [CrossRef]
- Todorovic-Marković, B.; Marković, Z.; Mohai, I.; Károly, Z.; Gál, L.; Föglein, K.; Szabó, P.T.; Szépvölgyi, J. Efficient Synthesis of Fullerenes in RF Thermal Plasma Reactor. Chem. Phys. Lett. 2003, 378, 434–439. [Google Scholar] [CrossRef]
- Keszler, A.M.; Kováts, É.; Bódis, E.; Károly, Z.; Szépvölgyi, J. Effect of Metallic and Non-Metallic Additives on the Synthesis of Fullerenes in Thermal Plasma. Condens. Matter 2022, 7, 44. [Google Scholar] [CrossRef]
- Tong, J.B.; Lu, X.; Liu, C.C.; Pi, Z.Q.; Zhang, R.J.; Qu, X.H. Numerical Simulation and Prediction of Radio Frequency Inductively Coupled Plasma Spheroidization. Appl. Therm. Eng. 2016, 100, 1198–1206. [Google Scholar] [CrossRef]
- Yu, C.; Zhou, X.; Wang, D.; Van Linh, N.; Liu, W. Study on the RF Inductively Coupled Plasma Spheroidization of Refractory W and W-Ta Alloy Powders. Plasma Sci. Technol. 2017, 20, 014019. [Google Scholar] [CrossRef]
- Park, J.Y.; Park, K.B.; Kang, J.W.; Kim, H.G.; Hwang, N.M.; Park, H.K. Spheroidization Behavior of Water-Atomized 316 Stainless Steel Powder by Inductively-Coupled Thermal Plasma. Mater. Today Commun. 2020, 25, 101488. [Google Scholar] [CrossRef]
- Kim, K.H.; Choi, H.; Han, C. Tungsten Micropowder/Copper Nanoparticle Core/Shell-Structured Composite Powder Synthesized by Inductively Coupled Thermal Plasma Process. Metall. Mater. Trans. A 2017, 48, 439–445. [Google Scholar] [CrossRef]
- Dhamale, G.D.; Tak, A.K.; Mathe, V.L.; Ghorui, S. Nucleation and Growth of Y2O3 Nanoparticles in a RF-ICTP Reactor: A Discrete Sectional Study Based on CFD Simulation Supported with Experiments. J. Phys. D Appl. Phys. 2018, 51, 255202. [Google Scholar] [CrossRef]
- Oh, J.-W.; Na, H.; Cho, Y.S.; Choi, H. In Situ Synthesis of Bimetallic Tungsten-Copper Nanoparticles via Reactive Radio-Frequency (RF) Thermal Plasma. Nanoscale Res. Lett. 2018, 13, 220. [Google Scholar] [CrossRef]
- Zhang, X.; Hayashida, R.; Tanaka, M.; Watanabe, T. Synthesis of Carbon-Coated Silicon Nanoparticles by Induction Thermal Plasma for Lithium Ion Battery. Powder Technol. 2020, 371, 26–36. [Google Scholar] [CrossRef]
- Kim, K.S.; Couillard, M.; Shin, H.; Plunkett, M.; Ruth, D.; Kingston, C.T.; Simard, B. Role of Hydrogen in High-Yield Growth of Boron Nitride Nanotubes at Atmospheric Pressure by Induction Thermal Plasma. ACS Nano 2018, 12, 884–893. [Google Scholar] [CrossRef]
- Kim, K.S.; Kingston, C.T.; Ruth, D.; Barnes, M.; Simard, B. Synthesis of High Quality Single-Walled Carbon Nanotubes with Purity Enhancement and Diameter Control by Liquid Precursor Ar–H2 Plasma Spraying. Chem. Eng. J. 2014, 250, 331–341. [Google Scholar] [CrossRef]
- Li, J.; Hu, R.; Qu, H.; Su, Y.; Wang, N.; Su, H.; Gu, X. Radio-Frequency Thermal Plasma-Induced Novel Chainmail-like Core-Shell MoO2 as Highly Stable Catalyst for Converting Syngas to Higher Alcohols. Appl. Catal. B 2019, 249, 63–71. [Google Scholar] [CrossRef]
- Takigawa, A.; Kim, T.-H.; Igami, Y.; Umemoto, T.; Tsuchiyama, A.; Koike, C.; Matsuno, J.; Watanabe, T. Formation of Transition Alumina Dust around Asymptotic Giant Branch Stars: Condensation Experiments Using Induction Thermal Plasma Systems. Astrophys. J. Lett. 2019, 878, L7. [Google Scholar] [CrossRef]
- Tanaka, Y. Recent Development of New Inductively Coupled Thermal Plasmas for Materials Processing. Adv. Phys. X 2021, 6, 1867637. [Google Scholar] [CrossRef]
- Causa, F.; Gittini, G.; Minelli, D.; Mellera, V.; Uccello, A.; Nardone, A.; Ripamonti, F. Plasma Parameters Profiles from Langmuir Probe Measurements in Low-Density, Low-Temperature Plasmas in an Axial Magnetic Field. Plasma Sources Sci. Technol. 2022, 31, 075007. [Google Scholar] [CrossRef]
- Passoth, E.; Kudrna, P.; Csambal, C.; Behnke, J.F.; Tichý, M.; Helbig, V. An Experimental Study of Plasma Density Determination by a Cylindrical Langmuir Probe at Different Pressures and Magnetic Fields in a Cylindrical Magnetron Discharge in Heavy Rare Gases. J. Phys. D Appl. Phys. 1997, 30, 1763. [Google Scholar] [CrossRef]
- Mascali, D.; Gambino, N.; Miracoli, R.; Gammino, S.; Torrisi, L.; Maimone, F.; Tumino, L. Plasma Parameters Measurements by Means of Langmuir Probe. Radiat. Eff. Defects Solids 2008, 163, 471–478. [Google Scholar] [CrossRef]
- Lindner, J.; Ross, U.; Roddatis, V.; Jooss, C. Langmuir Analysis of Electron Beam Induced Plasma in Environmental TEM. Ultramicroscopy 2023, 243, 113629. [Google Scholar] [CrossRef] [PubMed]
- Giannakaris, N.; Gürtler, G.; Stehrer, T.; Mair, M.; Pedarnig, J.D. Optical Emission Spectroscopy of an Industrial Thermal Atmospheric Pressure Plasma Jet: Parametric Study of Electron Temperature. Spectrochim. Acta Part B Spectrosc. 2023, 207, 106736. [Google Scholar] [CrossRef]
- Ohno, N.; Razzak, M.A.; Ukai, H.; Takamura, S.; Uesugi, Y. Validity of Electron Temperature Measurement by Using Boltzmann Plot Method in Radio Frequency Inductive Discharge in the Atmospheric Pressure Range. Plasma Fusion Res. 2006, 1, 28. [Google Scholar] [CrossRef]
- Zhang, H.; Yuan, F.; Chen, Q. Optical Emission Spectroscopy Diagnostics of Atmospheric Pressure Radio Frequency Ar-H2Inductively Coupled Thermal Plasma. IEEE Trans. Plasma Sci. 2020, 48, 3621–3628. [Google Scholar] [CrossRef]
- Tu, X.; Chéron, B.G.; Yan, J.H.; Cen, K.F. Electrical and Spectroscopic Diagnostic of an Atmospheric Double Arc Argon Plasma Jet. Plasma Sources Sci. Technol. 2007, 16, 803. [Google Scholar] [CrossRef]
- Nikiforov, A.Y.; Leys, C.; Gonzalez, M.A.; Walsh, J.L. Electron Density Measurement in Atmospheric Pressure Plasma Jets: Stark Broadening of Hydrogenated and Non-Hydrogenated Lines. Plasma Sources Sci. Technol. 2015, 24, 034001. [Google Scholar] [CrossRef]
- Chen, C.; Fu, W.; Zhang, C.; Lu, D.; Han, M.; Yan, Y. Langmuir Probe Diagnostics with Optical Emission Spectrometry (OES) for Coaxial Line Microwave Plasma. Appl. Sci. 2020, 10, 8117. [Google Scholar] [CrossRef]
- Wu, J.; Zhang, P.; Yu, D.; Zhang, S.; Xin, Q.; Wan, Y. Monitoring and Diagnosis of the Inductively Coupled Atmospheric Pressure Plasma Jet for Deterministic Optical Processing. Optik 2020, 214, 164815. [Google Scholar] [CrossRef]
- Griem, H.R. Stark Broadening. Adv. At. Mol. Phys. 1976, 11, 331–359. [Google Scholar] [CrossRef]
- Sadeghzadeh Lari, E.; Ranjbar Askari, H.; Meftah, M.T.; Shariat, M. Calculation of Electron Density and Temperature of Plasmas by Using New Stark Broadening Formula of Helium Lines. Phys. Plasmas 2019, 26, 023519. [Google Scholar] [CrossRef]
- Christova, M.; Castanos-Martinez, E.; Calzada, M.D.; Kabouzi, Y.; Luque, J.M.; Moisan, M. Electron Density and Gas Temperature from Line Broadening in an Argon Surface-Wave-Sustained Discharge at Atmospheric Pressure. Appl. Spectrosc. 2004, 58, 1032–1037. [Google Scholar] [CrossRef]
- Bhatt, K.P.; Patel, S.; Upadhyay, D.S.; Patel, R.N. A Critical Review on Solid Waste Treatment Using Plasma Pyrolysis Technology. Chem. Eng. Process.-Process Intensif. 2022, 177, 108989. [Google Scholar] [CrossRef]
- Galiwango, E.; Gabbar, H.A. Synergistic Interactions, Kinetic and Thermodynamic Analysis of Co-Pyrolysis of Municipal Paper and Polypropylene Waste. Waste Manag. 2022, 146, 86–93. [Google Scholar] [CrossRef]
- Ganguli, A.; Tarey, R. Understanding Plasma Sources. Curr. Sci. 2002, 83, 279–290. [Google Scholar]
- Aldeeb, M.A.; Morgan, N.; Abouelsayed, A.; Amin, K.M.; Hassaballa, S. Electrical and Optical Characterization of Acetylene RF CCP for Synthesis of Different Forms of Hydrogenated Amorphous Carbon Films. Plasma Chem. Plasma Process. 2020, 40, 387–406. [Google Scholar] [CrossRef]
- Abbass, Q.; Ahmed, N.; Ahmed, R.; Baig, M.A. A Comparative Study of Calibration Free Methods for the Elemental Analysis by Laser Induced Breakdown Spectroscopy. Plasma Chem. Plasma Process. 2016, 36, 1287–1299. [Google Scholar] [CrossRef]
- Younus, M.; Rehman, N.U.; Shafiq, M.; Naeem, M.; Zaka-Ul-Islam, M.; Zakaullah, M. Evolution of Plasma Parameters in an Ar–N 2 /He Inductive Plasma Source with Magnetic Pole Enhancement. Plasma Sci. Technol. 2017, 19, 025402. [Google Scholar] [CrossRef]
- Godyak, V.A. Electrical and Plasma Parameters of ICP with High Coupling Efficiency. Plasma Sources Sci. Technol. 2011, 20, 025004. [Google Scholar] [CrossRef]
- Godyak, V.A.; Piejak, R.B.; Alexandrovich, B.M. Electron Energy Distribution Function Measurements and Plasma Parameters in Inductively Coupled Argon Plasma. Plasma Sources Sci. Technol. 2002, 11, 525–543. [Google Scholar] [CrossRef]
- Jun-Feng, Z.; Xin-Chao, B.; Qiang, C.; Fu-Ping, L.; Zhong-Wei, L. Diagnosis of Methane Plasma Generated in an Atmospheric Pressure DBD Micro-Jet by Optical Emission Spectroscopy. Chin. Phys. Lett. 2009, 26, 035203. [Google Scholar] [CrossRef]
- Kolobov, V. The Anomalous Skin Effect in Bounded Systems. In Electron Kinetics and Applications of Glow Discharges; Kluwer Academic Publishers: Boston, MA, USA, 1995; pp. 293–311. [Google Scholar]
- Boffard, J.B.; Jung, R.O.; Lin, C.C.; Wendt, A.E. Measurement of Metastable and Resonance Level Densities in Rare-Gas Plasmas by Optical Emission Spectroscopy. Plasma Sources Sci. Technol. 2009, 18, 035017. [Google Scholar] [CrossRef]
- Nijdam, S.; Teunissen, J.; Ebert, U. The Physics of Streamer Discharge Phenomena. Plasma Sources Sci. Technol. 2020, 29, 103001. [Google Scholar] [CrossRef]
- Tamošiūnas, A.; Valatkevičius, P.; Valinčius, V.; Grigaitienė, V.; Kavaliauskas, Ž. Diagnostic Methods Used for Atmospheric Pressure Thermal Arc Plasma. Phys. Scr. 2014, T161, 014059. [Google Scholar] [CrossRef]
- Ali, A.M.; Abu Hassan, M.A.; Ibrahim, R.R.K.; Jalil, A.A.; Mat Nayan, N.H.; Abdulkarim, B.I.; Sabeen, A.H. Analysis of Solid Residue and Flue Gas from Thermal Plasma Treatment of Petroleum Sludge. J. Environ. Chem. Eng. 2019, 7, 103207. [Google Scholar] [CrossRef]
- Pourali, M. Application of Plasma Gasification Technology in Waste. to Energy—Challenges and Opportunities. IEEE Trans. Sustain. Energy 2010, 1, 125–130. [Google Scholar] [CrossRef]
- Balas, M.; Lisy, M.; Kracik, P.; Pospisil, J. Municipal solid waste gasification within waste-to-energy processing. MM Sci. J. 2017, 2017, 1783–1788. [Google Scholar] [CrossRef]
- Huang, H.; Tang, L.; Wu, C.Z. Characterization of Gaseous and Solid Product from Thermal Plasma Pyrolysis of Waste Rubber. Environ. Sci. Technol. 2003, 37, 4463–4467. [Google Scholar] [CrossRef] [PubMed]



| λ (nm) | Ek (eV) | Aik (106 S−1) | gk |
|---|---|---|---|
| 696 | 13.328 | 6.40 | 3 |
| 706 | 13.302 | 3.80 | 5 |
| 727 | 13.328 | 1.83 | 3 |
| 738 | 13.302 | 8.50 | 5 |
| 750 | 13.479 | 45 | 1 |
| 751 | 13.273 | 40 | 1 |
| 763 | 13.172 | 24 | 5 |
| 771 | 13.153 | 5.20 | 3 |
| 772 | 13.328 | 11.7 | 3 |
| 794 | 13.283 | 18.6 | 3 |
| 800 | 13.172 | 49 | 5 |
| 801 | 13.095 | 9.30 | 5 |
| 810 | 13.153 | 25 | 3 |
| 811 | 13.076 | 33 | 7 |
| 826 | 13.328 | 15.3 | 3 |
| 840 | 13.302 | 22.3 | 5 |
| 842 | 13.095 | 21.5 | 5 |
| 851 | 13.283 | 13.9 | 3 |
| 2 SLPM | 4 SLPM | ||||
|---|---|---|---|---|---|
| Power (W) | (nm) | (nm) | Power (W) | (nm) | (nm) |
| 440 | 1.431 | 1.126 | 440 | 1.440 | 1.138 |
| 550 | 1.406 | 1.096 | 550 | 1.358 | 1.037 |
| 660 | 1.415 | 1.108 | 660 | 1.461 | 1.162 |
| 770 | 1.402 | 1.091 | 770 | 1.372 | 1.055 |
| 880 | 1.377 | 1.061 | 880 | 1.363 | 1.044 |
| 990 | 1.436 | 1.133 | 990 | 1.419 | 1.113 |
| 1100 | 1.445 | 1.143 | 1100 | 1.346 | 1.023 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2024 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Aldeeb, M.A.; Abu Darda, S.; Damideh, V.; Hassen, I.; Gabbar, H.A. Analysis of Essential Features and Optimal Operational Parameters of an RF-ICP Torch for Waste Treatment Applications. Recycling 2024, 9, 20. https://doi.org/10.3390/recycling9010020
Aldeeb MA, Abu Darda S, Damideh V, Hassen I, Gabbar HA. Analysis of Essential Features and Optimal Operational Parameters of an RF-ICP Torch for Waste Treatment Applications. Recycling. 2024; 9(1):20. https://doi.org/10.3390/recycling9010020
Chicago/Turabian StyleAldeeb, Mustafa A., Sharif Abu Darda, Vahid Damideh, Isaac Hassen, and Hossam A. Gabbar. 2024. "Analysis of Essential Features and Optimal Operational Parameters of an RF-ICP Torch for Waste Treatment Applications" Recycling 9, no. 1: 20. https://doi.org/10.3390/recycling9010020
APA StyleAldeeb, M. A., Abu Darda, S., Damideh, V., Hassen, I., & Gabbar, H. A. (2024). Analysis of Essential Features and Optimal Operational Parameters of an RF-ICP Torch for Waste Treatment Applications. Recycling, 9(1), 20. https://doi.org/10.3390/recycling9010020







