Towards Determining an Engineering Stress-Strain Curve and Damage of the Cylindrical Lithium-Ion Battery Using the Cylindrical Indentation Test
Abstract
1. Introduction
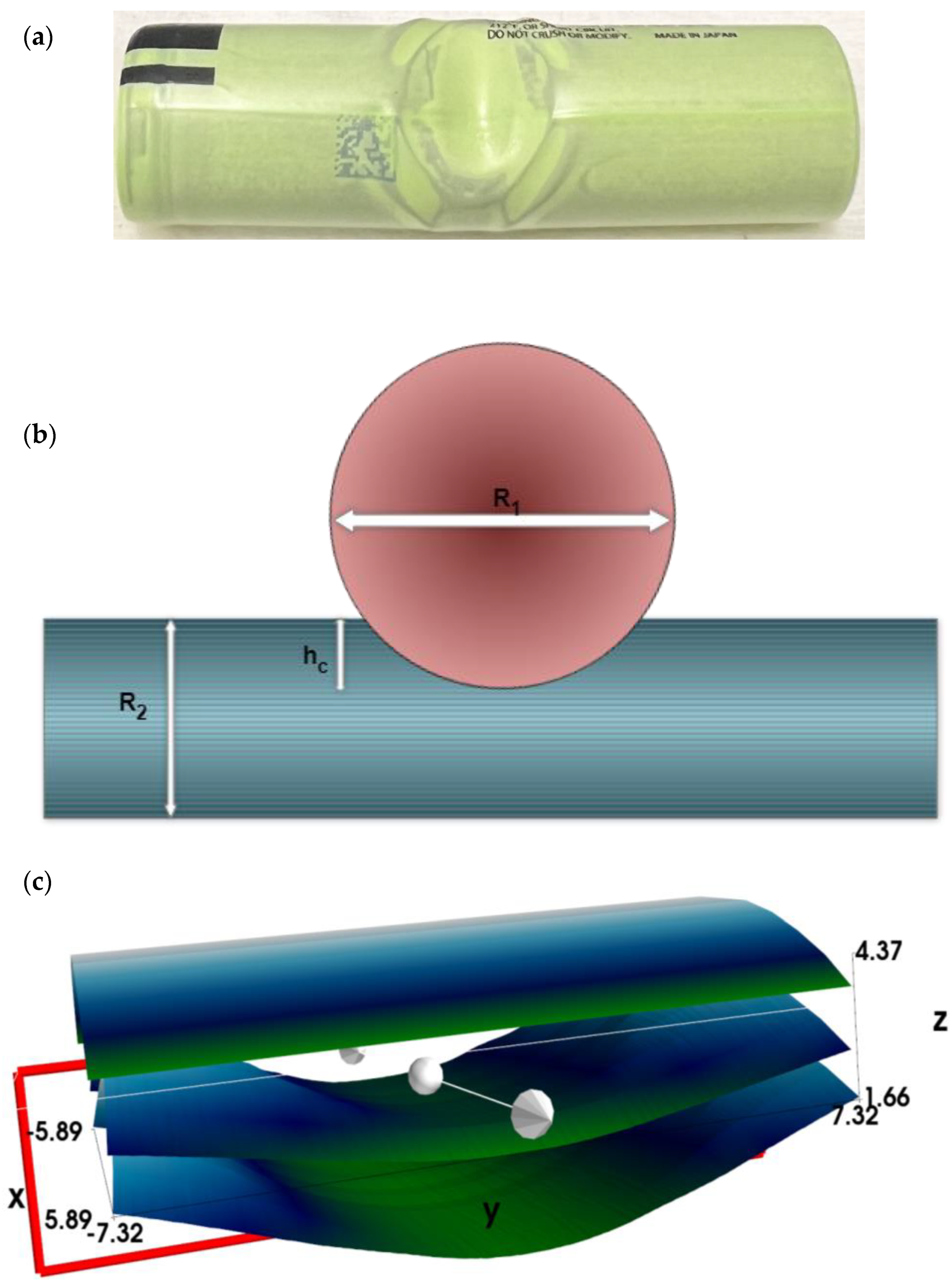
2. Materials and Methods
3. Results and Discussion
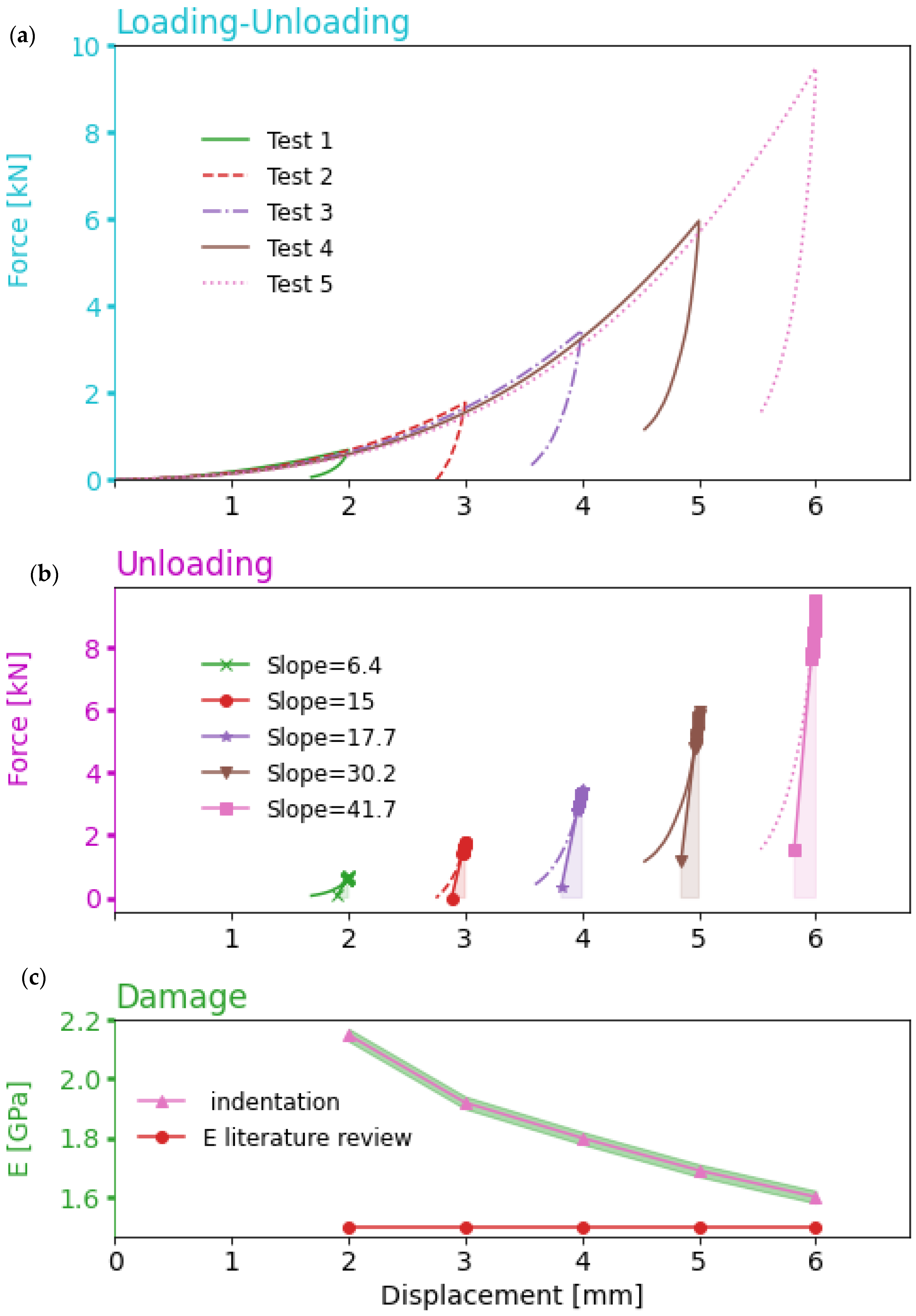
3.1. Measurement of Young’s Modulus Reduction
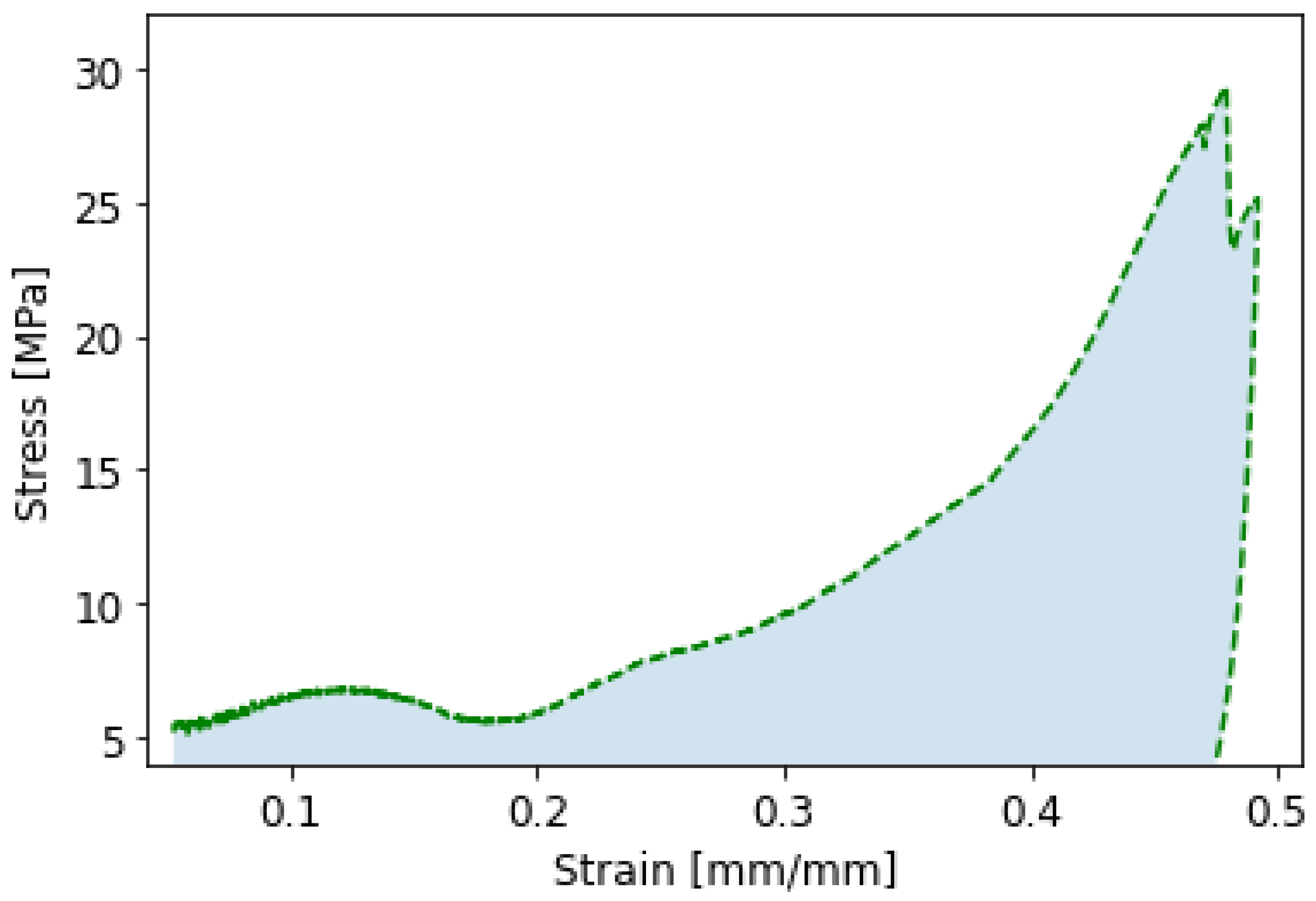
3.2. Analytical Approach to Convert Force-Depth into Stress-Strain Relation
3.3. Fundamental Assumptions
3.4. Free-Energy Function and Damage Evolution
- ➢
- 4 defining anisotropic hyperelastic part
- ➢
- 19 defining damage evolution plus the modulation function .
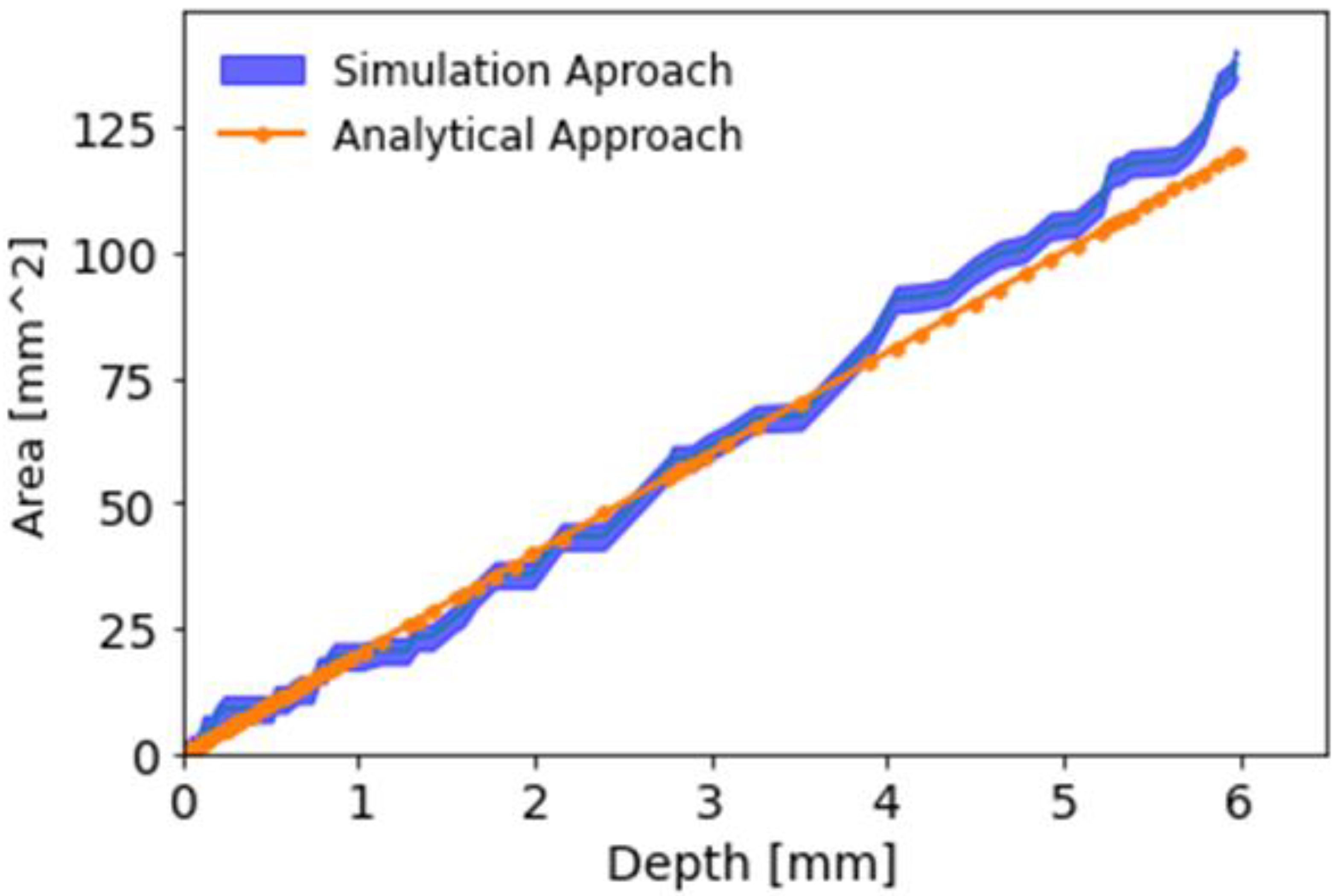
3.5. Model Validation
4. Conclusions
Author Contributions
Funding
Informed Consent Statement
Data Availability Statement
Conflicts of Interest
References
- Masias, A.; Felten, N.; Sakamoto, J. Characterizing the mechanical behavior of lithium in compression. J. Mater. Res. 2021, 36, 729–739. [Google Scholar] [CrossRef]
- Kermani, G.; Keshavarzi, M.M.; Sahraei, E. Deformation of lithium-ion batteries under axial loading: Analytical model and Representative Volume Element. Energy Rep. 2021, 7, 2849–2861. [Google Scholar] [CrossRef]
- Spielbauer, M.; Berg, P.; Soellner, J.; Peters, J.; Schaeufl, F.; Rosenmüller, C.; Bohlen, O.; Jossen, A. Experimental investigation of the failure mechanism of 18650 lithium-ion batteries due to shock and drop. J. Energy Storage 2021, 43, 103213. [Google Scholar] [CrossRef]
- Zhu, F.; Zhou, R.; Sypeck, D.J. Numerical modeling and safety design for lithium-ion vehicle battery modules subject to crush loading. Energies 2020, 14, 118. [Google Scholar] [CrossRef]
- Kisters, T.; Sahraei, E.; Wierzbicki, T. Dynamic impact tests on lithium-ion cells. Int. J. Impact Eng. 2017, 108, 205–216. [Google Scholar] [CrossRef]
- Luo, H.; Xia, Y.; Zhou, Q. Mechanical damage in a lithium-ion pouch cell under indentation loads. J. Power Source 2017, 357, 61–70. [Google Scholar] [CrossRef]
- Keshavarzi, M.M.; Gilaki, M.; Sahraei, E. Characterization of in-situ material properties of pouch lithium-ion batteries in tension from three-point bending tests. Int. J. Mech. Sci. 2022, 219, 107090. [Google Scholar] [CrossRef]
- Zhu, X.; Wang, H.; Wang, X.; Gao, Y.; Allu, S.; Cakmak, E.; Wang, Z. Internal short circuit and failure mechanisms of lithium-ion pouch cells under mechanical indentation abuse conditions: An experimental study. J. Power Source 2020, 455, 227939. [Google Scholar] [CrossRef]
- Lian, J.; Koch, M.; Li, W.; Wierzbicki, T.; Zhu, J. Mechanical deformation of Lithium-ion pouch cells under in-plane loads—Part II: Computational modeling. J. Electrochem. Soc. 2020, 167, 090556. [Google Scholar] [CrossRef]
- Gao, Z.; Zhang, X.; Xiao, Y.; Gao, H.; Wang, H.; Piao, C. Influence of low-temperature charge on the mechanical integrity behavior of 18650 lithium-ion battery cells subject to lateral compression. Energies 2019, 12, 797. [Google Scholar] [CrossRef][Green Version]
- Ren, F.; Cox, T.; Wang, H. Thermal runaway risk evaluation of Li-ion cells using a pinch–torsion test. J. Power Source 2014, 249, 156–162. [Google Scholar] [CrossRef]
- Ellersdorfer, C.; Höschele, P.; Heider, E.; Kovachev, G.; Gstrein, G. Safety Assessment of High Dynamic Pre-Loaded Lithium Ion Pouch Cells. Batteries 2023, 9, 71. [Google Scholar] [CrossRef]
- Gilaki, M.; Song, Y.; Sahraei, E. Homogenized characterization of cylindrical Li-ion battery cells using elliptical approximation. Int. J. Energy Res. 2022, 46, 5908–5923. [Google Scholar] [CrossRef]
- Kermani, G.; Dixon, B.; Sahraei, E. Elliptical lithium-ion batteries: Transverse and axial loadings under wet/dry conditions. Energy Sci. Eng. 2019, 7, 890–898. [Google Scholar] [CrossRef][Green Version]
- Aphale, S.; Murugkar, M.; Lulla, S.; Date, P. Deformation and Failure Behavior of Cylindrical Lithium-Ion Batteries Subjected to Mechanical Loading; SAE Technical Paper: Warrendale, PA, USA, 2020; pp. 0148–7191. [Google Scholar]
- Avdeev, I.; Gilaki, M. Structural analysis and experimental characterization of cylindrical lithium-ion battery cells subject to lateral impact. J. Power Source 2014, 271, 382–391. [Google Scholar] [CrossRef]
- Raffler, M.; Sevarin, A.; Ellersdorfer, C.; Heindl, S.F.; Breitfuss, C.; Sinz, W. Finite element model approach of a cylindrical lithium ion battery cell with a focus on minimization of the computational effort and short circuit prediction. J. Power Source 2017, 360, 605–617. [Google Scholar] [CrossRef]
- Sheikh, M.; Elmarakbi, A.; Elkady, M. Thermal runaway detection of cylindrical 18650 lithium-ion battery under quasi-static loading conditions. J. Power Source 2017, 370, 61–70. [Google Scholar] [CrossRef][Green Version]
- Chung, S.H.; Tancogne-Dejean, T.; Zhu, J.; Luo, H.; Wierzbicki, T. Failure in lithium-ion batteries under transverse indentation loading. J. Power Source 2018, 389, 148–159. [Google Scholar] [CrossRef]
- Muresanu, A.D.; Dudescu, M.C. Numerical and Experimental Evaluation of a Battery Cell under Impact Load. Batteries 2022, 8, 48. [Google Scholar] [CrossRef]
- Zhu, F.; Zhou, R.; Sypeck, D.; Deng, J.; Bae, C. Failure behavior of prismatic Li-ion battery cells under abuse loading condition-A combined experimental and computational study. J. Energy Storage 2022, 48, 103969. [Google Scholar] [CrossRef]
- Li, W.; Xing, B.; Watkins, T.R.; Xia, Y.; Wang, H.; Zhu, J. Damage of prismatic lithium-ion cells subject to bending: Test, model, and detection. EcoMat 2022, 4, e12257. [Google Scholar] [CrossRef]
- Wang, H.; Simunovic, S.; Maleki, H.; Howard, J.N.; Hallmark, J.A. Internal configuration of prismatic lithium-ion cells at the onset of mechanically induced short circuit. J. Power Source 2016, 306, 424–430. [Google Scholar] [CrossRef][Green Version]
- Lamb, J.; Orendorff, C.J. Evaluation of mechanical abuse techniques in lithium ion batteries. J. Power Source 2014, 247, 189–196. [Google Scholar] [CrossRef]
- Mao, B.; Chen, H.; Cui, Z.; Wu, T.; Wang, Q. Failure mechanism of the lithium ion battery during nail penetration. Int. J. Heat Mass Transf. 2018, 122, 1103–1115. [Google Scholar] [CrossRef]
- Feng, X.; Sun, J.; Ouyang, M.; Wang, F.; He, X.; Lu, L.; Peng, H. Characterization of penetration induced thermal runaway propagation process within a large format lithium ion battery module. J. Power Source 2015, 275, 261–273. [Google Scholar] [CrossRef]
- Ma, T.; Chen, L.; Liu, S.; Zhang, Z.; Xiao, S.; Fan, B.; Liu, L.; Lin, C.; Ren, S.; Wang, F. Mechanics-morphologic coupling studies of commercialized lithium-ion batteries under nail penetration test. J. Power Source 2019, 437, 226928. [Google Scholar] [CrossRef]
- Aiello, L.; Gstrein, G.; Erker, S.; Kaltenegger, B.; Ellersdorfer, C.; Sinz, W. Optimized Nail for Penetration Test on Lithium-Ion Cells and Its Utilization for the Validation of a Multilayer Electro-Thermal Model. Batteries 2022, 8, 32. [Google Scholar] [CrossRef]
- Doose, S.; Haselrieder, W.; Kwade, A. Effects of the Nail Geometry and Humidity on the Nail Penetration of High-Energy Density Lithium Ion Batteries. Batteries 2021, 7, 6. [Google Scholar] [CrossRef]
- Sahraei, E.; Meier, J.; Wierzbicki, T. Characterizing and modeling mechanical properties and onset of short circuit for three types of lithium-ion pouch cells. J. Power Source 2014, 247, 503–516. [Google Scholar] [CrossRef]
- Sahraei, E.; Campbell, J.; Wierzbicki, T. Modeling and short circuit detection of 18650 Li-ion cells under mechanical abuse conditions. J. Power Source 2012, 220, 360–372. [Google Scholar] [CrossRef]
- Sahraei, E.; Kahn, M.; Meier, J.; Wierzbicki, T. Modelling of cracks developed in lithium-ion cells under mechanical loading. RSC Adv. 2015, 5, 80369–80380. [Google Scholar] [CrossRef]
- Wang, W.; Yang, S.; Lin, C. Clay-like mechanical properties for the jellyroll of cylindrical Lithium-ion cells. Appl. Energy 2017, 196, 249–258. [Google Scholar] [CrossRef]
- Cai, W.; Wang, H.; Maleki, H.; Howard, J.; Lara-Curzio, E. Experimental simulation of internal short circuit in Li-ion and Li-ion-polymer cells. J. Power Source 2011, 196, 7779–7783. [Google Scholar] [CrossRef]
- Sahraei, E.; Hill, R.; Wierzbicki, T. Calibration and finite element simulation of pouch lithium-ion batteries for mechanical integrity. J. Power Source 2012, 201, 307–321. [Google Scholar] [CrossRef]
- Greve, L.; Fehrenbach, C. Mechanical testing and macro-mechanical finite element simulation of the deformation, fracture, and short circuit initiation of cylindrical Lithium ion battery cells. J. Power Source 2012, 214, 377–385. [Google Scholar] [CrossRef]
- Wang, Q.; Mao, B.; Stoliarov, S.I.; Sun, J. A review of lithium ion battery failure mechanisms and fire prevention strategies. Prog. Energy Combust. Sci. 2019, 73, 95–131. [Google Scholar] [CrossRef]
- Xu, J.; Liu, B.; Hu, D. State of Charge Dependent Mechanical Integrity Behavior of 18650 Lithium-ion Batteries. Sci. Rep. 2016, 6, 21829. [Google Scholar] [CrossRef] [PubMed][Green Version]
- Zhu, J.; Zhang, X.; Sahraei, E.; Wierzbicki, T. Deformation and failure mechanisms of 18650 battery cells under axial compression. J. Power Source 2016, 336, 332–340. [Google Scholar] [CrossRef]
- Song, Y.; Gilaki, M.; Keshavarzi, M.M.; Sahraei, E. A universal anisotropic model for a lithium-ion cylindrical cell validated under axial, lateral, and bending loads. Energy Sci. Eng. 2022, 10, 1431–1448. [Google Scholar] [CrossRef]
- Wierzbicki, T.; Sahraei, E. Homogenized mechanical properties for the jellyroll of cylindrical Lithium-ion cells. J. Power Source 2013, 241, 467–476. [Google Scholar] [CrossRef]
- Wang, L.; Yin, S.; Xu, J. A detailed computational model for cylindrical lithium-ion batteries under mechanical loading: From cell deformation to short-circuit onset. J. Power Source 2019, 413, 284–292. [Google Scholar] [CrossRef]
- Zhu, J.; Li, W.; Wierzbicki, T.; Xia, Y.; Harding, J. Deformation and failure of lithium-ion batteries treated as a discrete layered structure. Int. J. Plast. 2019, 121, 293–311. [Google Scholar] [CrossRef]
- Li, W.; Zhu, J. A Large Deformation and Fracture Model of Lithium-Ion Battery Cells Treated as a Homogenized Medium. J. Electrochem. Soc. 2020, 167, 120504. [Google Scholar] [CrossRef]
- Voyiadjis, G.Z.; Akbari, E.; Kattan, P.I. Damage model for lithium-ion batteries with experiments and simulations. J. Energy Storage 2023, 57, 106285. [Google Scholar] [CrossRef]
- Ali, M.Y.; Lai, W.-J.; Pan, J. Computational models for simulation of a lithium-ion battery module specimen under punch indentation. J. Power Source 2015, 273, 448–459. [Google Scholar] [CrossRef]
- Lai, W.-J.; Ali, M.Y.; Pan, J. Mechanical behavior of representative volume elements of lithium-ion battery modules under various loading conditions. J. Power Source 2014, 248, 789–808. [Google Scholar] [CrossRef]
- Murakami, S. Continuum Damage Mechanics: A Continuum Mechanics Approach to the Analysis of Damage and Fracture; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2012; Volume 185. [Google Scholar]
- Chow, C.; Wang, J. An anisotropic theory of continuum damage mechanics for ductile fracture. Eng. Fract. Mech. 1987, 27, 547–558. [Google Scholar] [CrossRef]
- Perzyna, P. The constitutive equations for rate sensitive plastic materials. Q. Appl. Math. 1963, 20, 321–332. [Google Scholar] [CrossRef][Green Version]
- Almeida, R. A Caputo fractional derivative of a function with respect to another function. Commun. Nonlinear Sci. Numer. Simul. 2017, 44, 460–481. [Google Scholar] [CrossRef][Green Version]
- Hertz, H. Miscellaneous Papers; Macmillan: Stuttgart, Germany, 1896. [Google Scholar]
- Wang, H.; Duan, X.; Liu, B. The Anisotropic Homogenized Model for Pouch Type Lithium-Ion Battery Under Various Abuse Loadings. J. Electrochem. Energy Convers. Storage 2021, 18, 021015. [Google Scholar] [CrossRef]
- Popov, V.L. Contact Mechanics and Friction; Springer: Berlin/Heidelberg, Germany, 2010. [Google Scholar]
- Yang, S.; Wang, W.; Lin, C.; Shen, W.; Li, Y. Improved constitutive model of the jellyroll for cylindrical lithium ion batteries considering microscopic damage. Energy 2019, 185, 202–212. [Google Scholar] [CrossRef]
- Xu, B.; Chen, X. Determining engineering stress–strain curve directly from the load–depth curve of spherical indentation test. J. Mater. Res. 2010, 25, 2297–2307. [Google Scholar] [CrossRef]
- Kalidindi, S.R.; Pathak, S. Determination of the effective zero-point and the extraction of spherical nanoindentation stress–strain curves. Acta Mater. 2008, 56, 3523–3532. [Google Scholar] [CrossRef]
- Voyiadjis, G.Z.; Sumelka, W. Brain modelling in the framework of anisotropic hyperelasticity with time fractional damage evolution governed by the Caputo-Almeida fractional derivative. J. Mech. Behav. Biomed. Mater. 2019, 89, 209–216. [Google Scholar] [CrossRef] [PubMed]
- Sumelka, W.; Łuczak, B.; Gajewski, T.; Voyiadjis, G.Z. Modelling of AAA in the framework of time-fractional damage hyperelasticity. Int. J. Solids Struct. 2020, 206, 30–42. [Google Scholar] [CrossRef]
- FindFit. Available online: https://reference.wolfram.com/language/ref/FindFit.html (accessed on 20 March 2023).



Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Voyiadjis, G.Z.; Akbari, E.; Łuczak, B.; Sumelka, W. Towards Determining an Engineering Stress-Strain Curve and Damage of the Cylindrical Lithium-Ion Battery Using the Cylindrical Indentation Test. Batteries 2023, 9, 233. https://doi.org/10.3390/batteries9040233
Voyiadjis GZ, Akbari E, Łuczak B, Sumelka W. Towards Determining an Engineering Stress-Strain Curve and Damage of the Cylindrical Lithium-Ion Battery Using the Cylindrical Indentation Test. Batteries. 2023; 9(4):233. https://doi.org/10.3390/batteries9040233
Chicago/Turabian StyleVoyiadjis, George Z., Edris Akbari, Bartosz Łuczak, and Wojciech Sumelka. 2023. "Towards Determining an Engineering Stress-Strain Curve and Damage of the Cylindrical Lithium-Ion Battery Using the Cylindrical Indentation Test" Batteries 9, no. 4: 233. https://doi.org/10.3390/batteries9040233
APA StyleVoyiadjis, G. Z., Akbari, E., Łuczak, B., & Sumelka, W. (2023). Towards Determining an Engineering Stress-Strain Curve and Damage of the Cylindrical Lithium-Ion Battery Using the Cylindrical Indentation Test. Batteries, 9(4), 233. https://doi.org/10.3390/batteries9040233








