Arcing in Li-Ion Batteries
Abstract
:1. Introduction
1.1. Battery Systems in Electric Vehicles
1.2. Thermal Runaway of Li-Ion Cells
1.3. Electric Arcing Inside a Battery Pack
1.4. Electric Breakdown
1.5. Goals
2. Materials and Methods
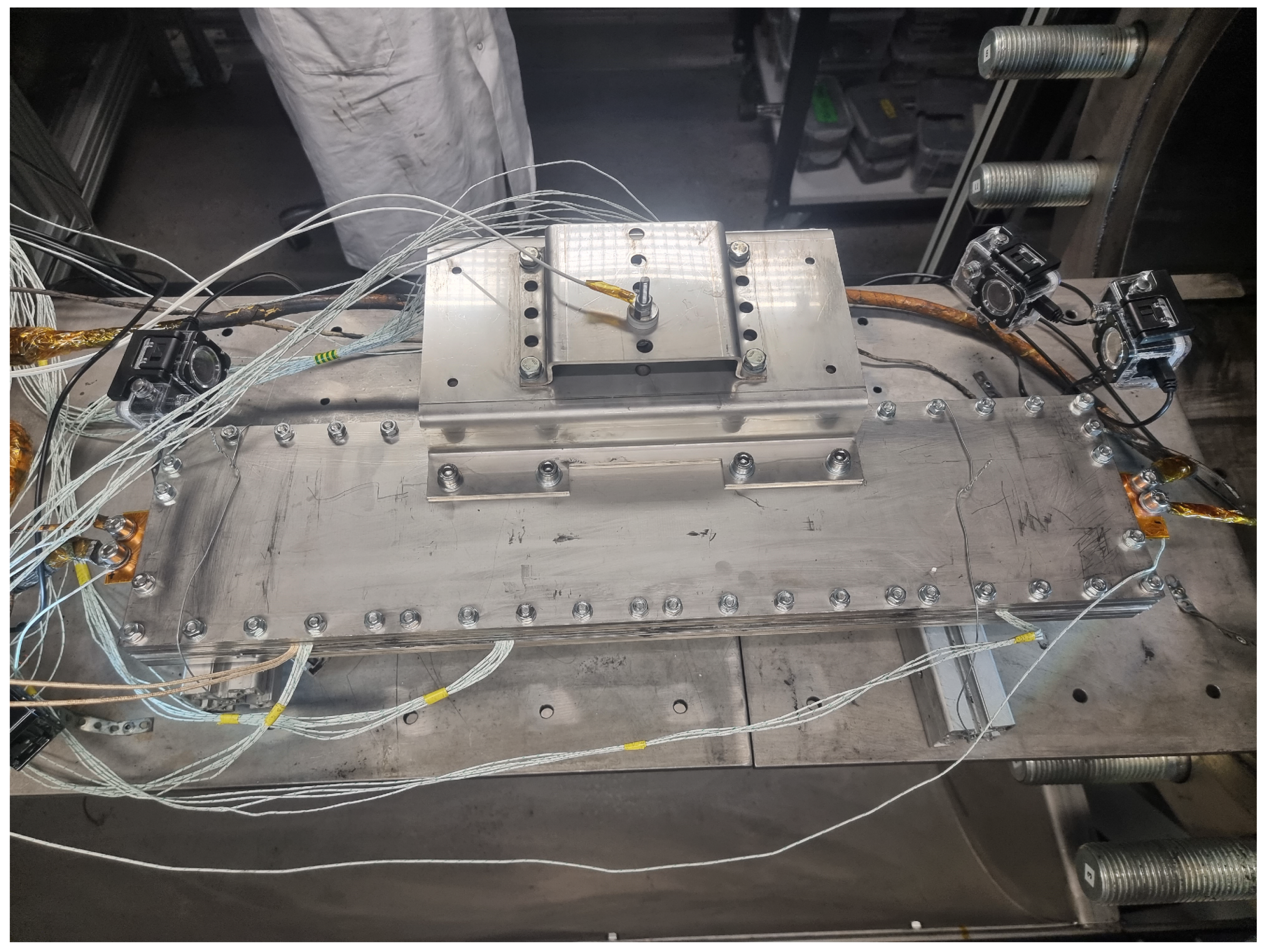
2.1. Used Li-Ion Cell
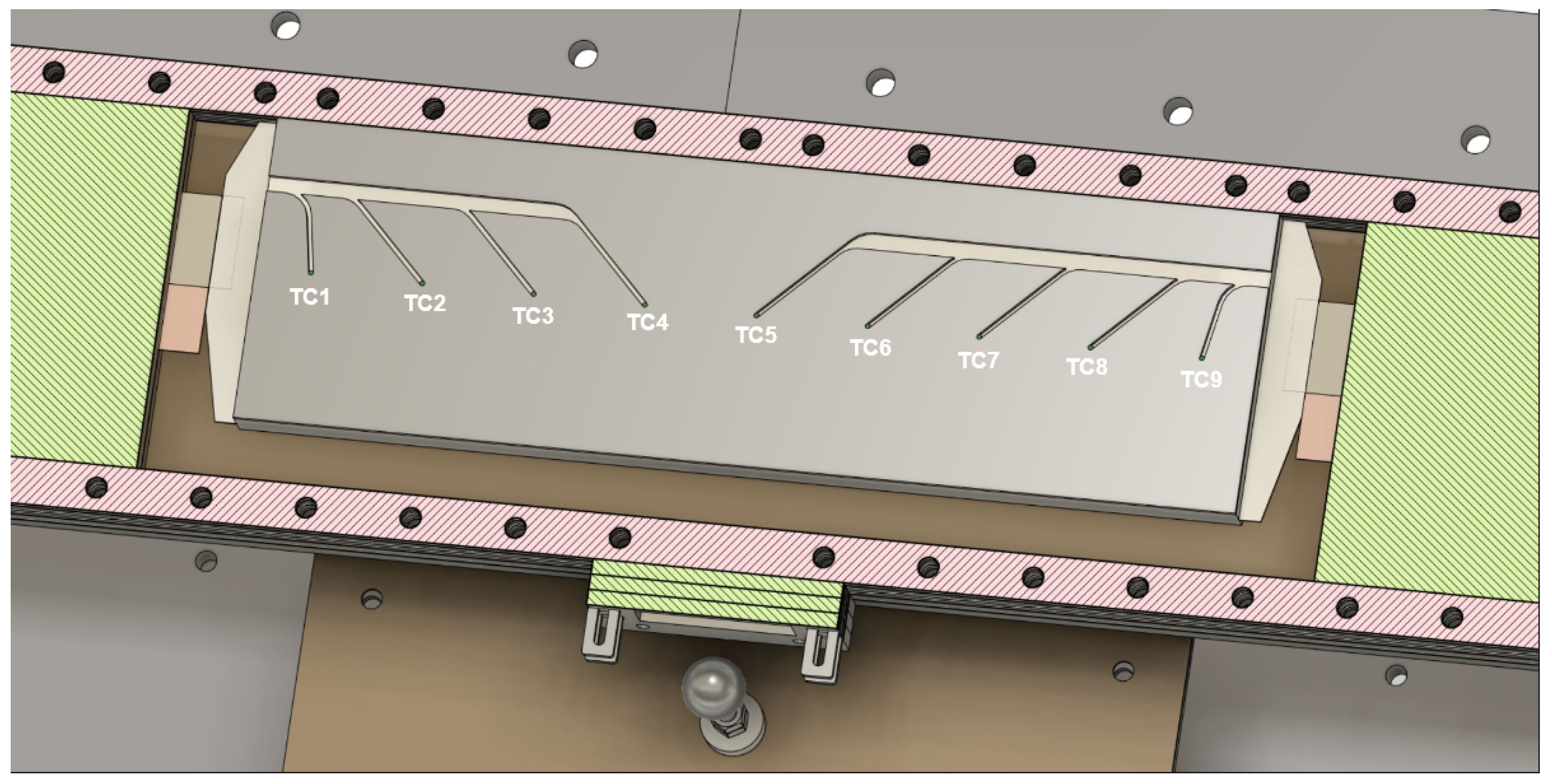
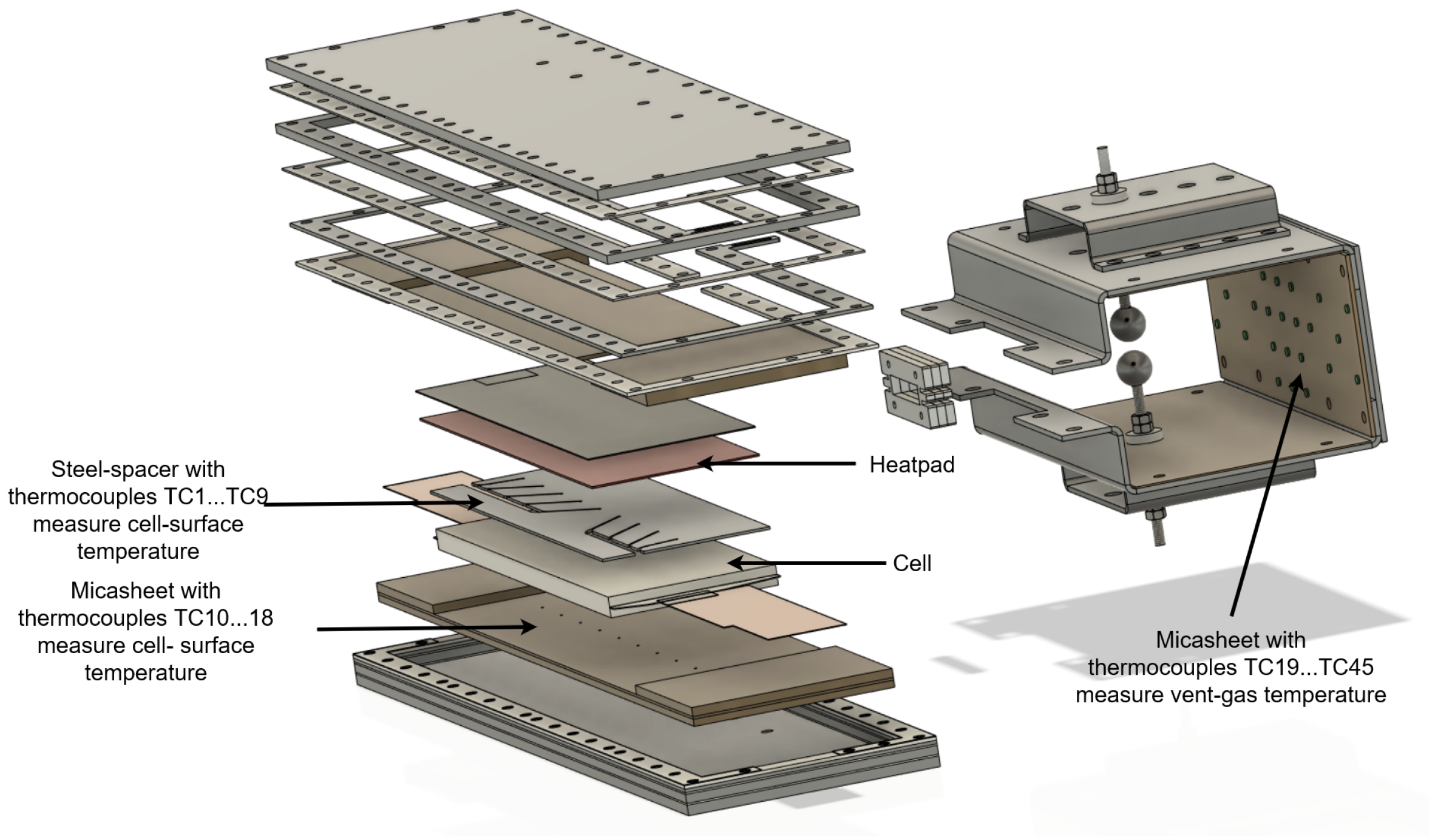
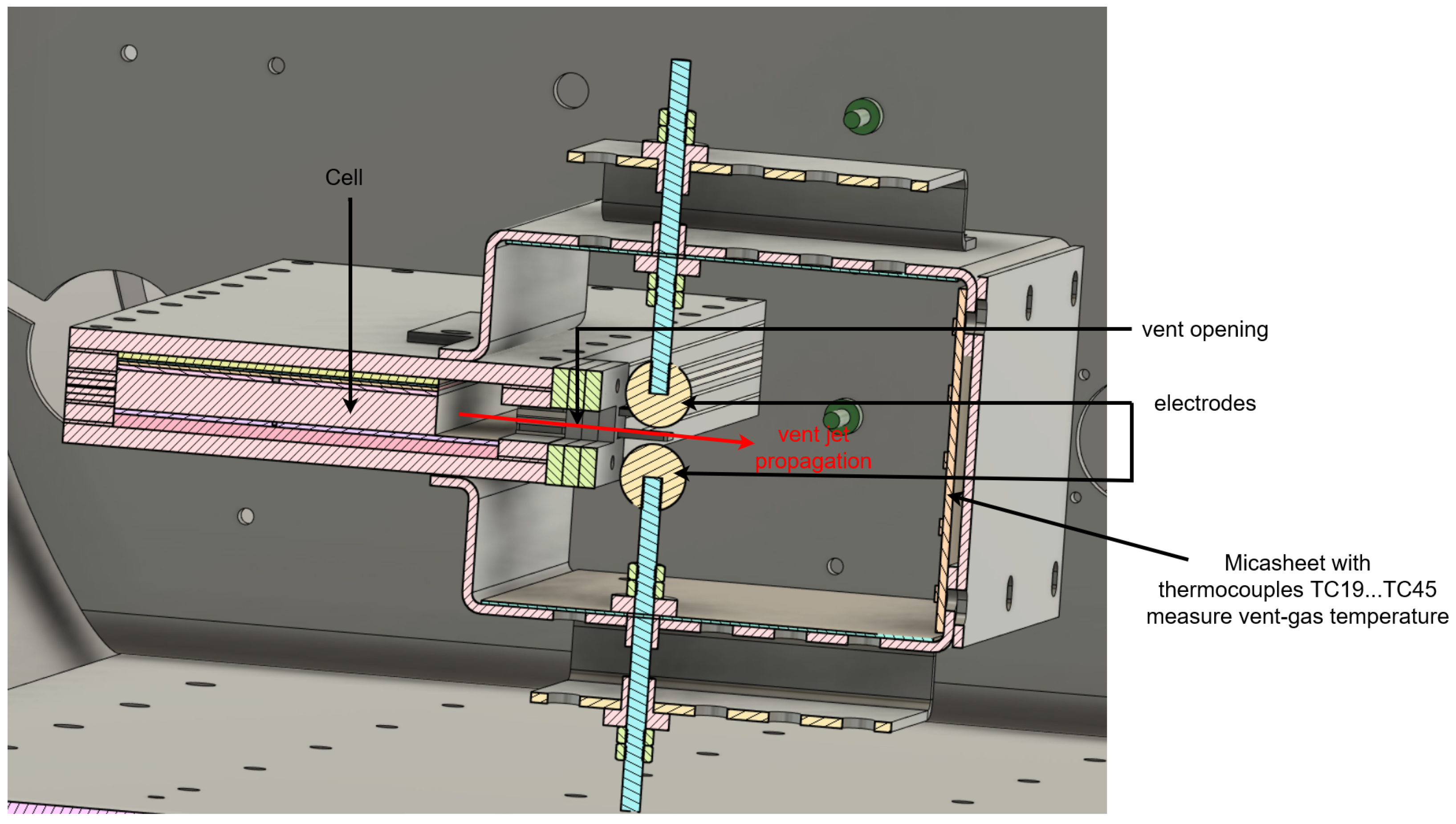
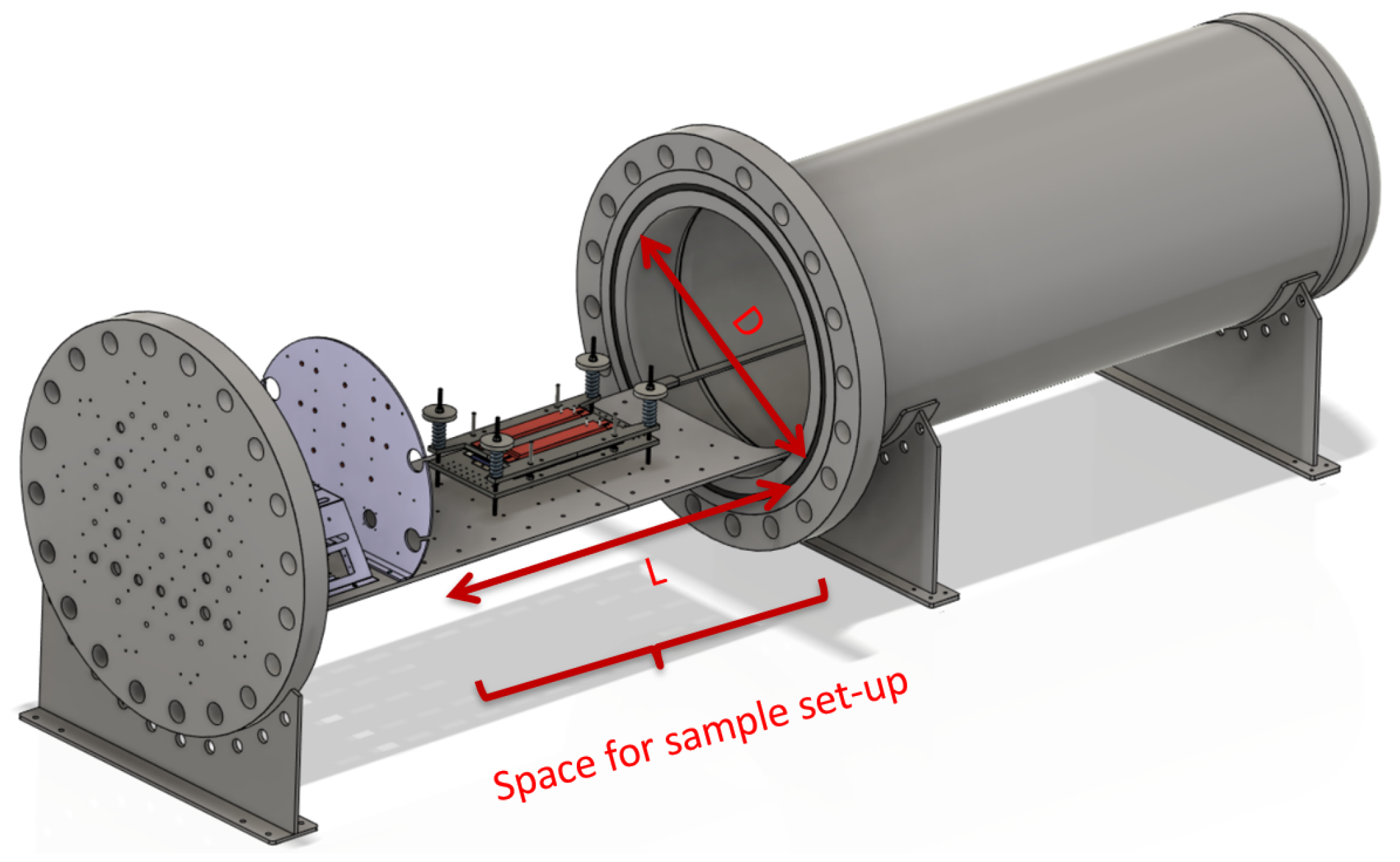
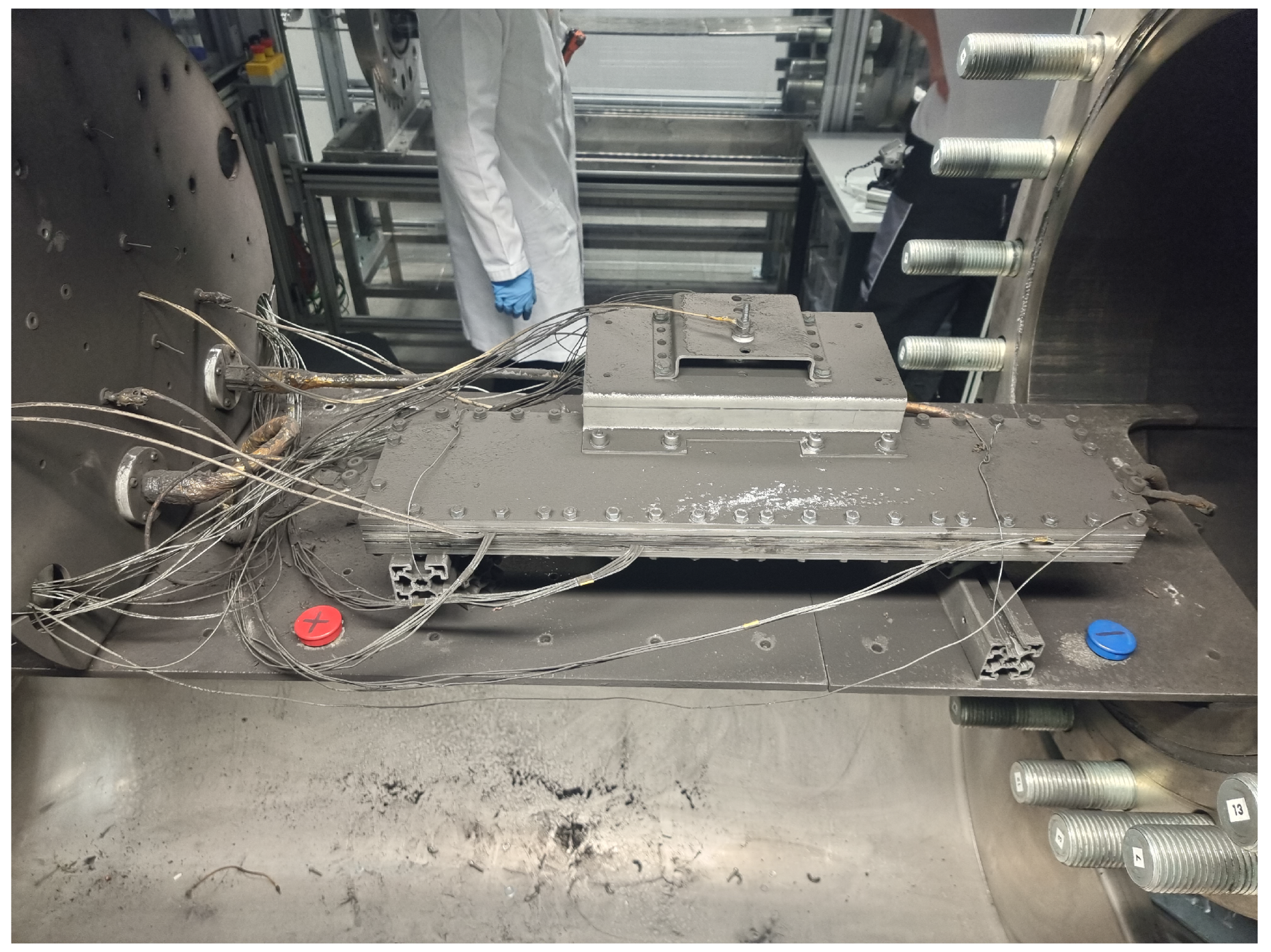
2.2. Test Setup
2.3. Reactor
2.4. Gas Analysis
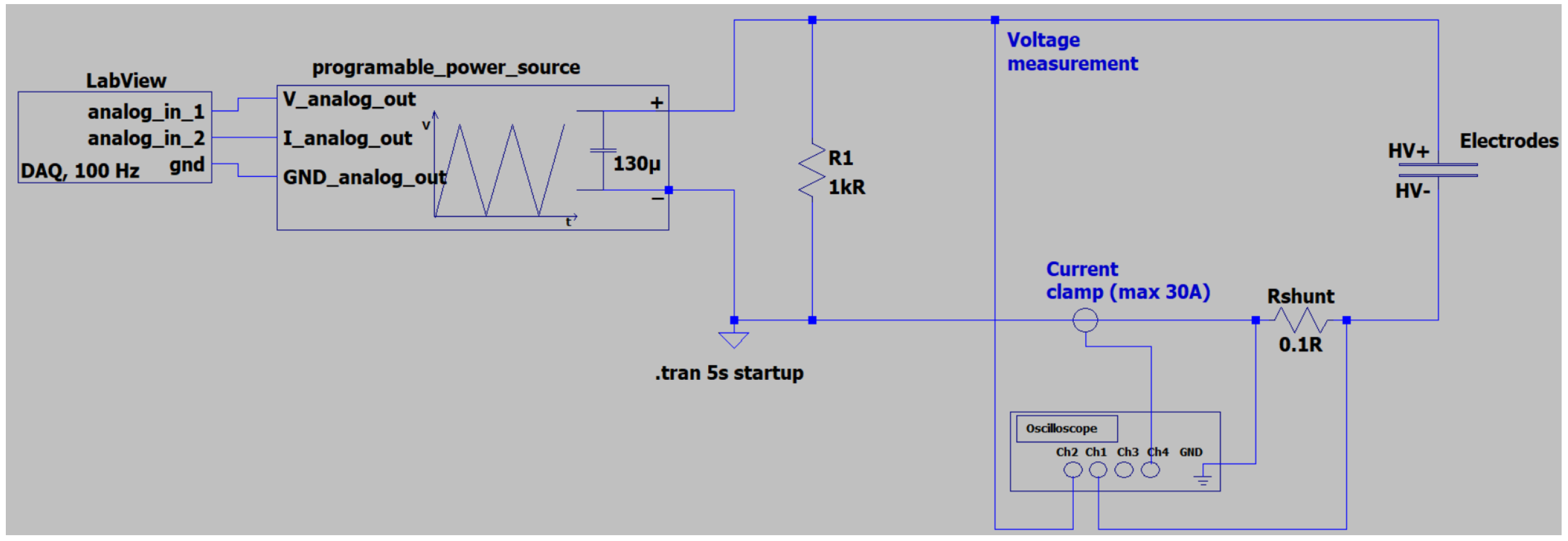
2.5. Electric Circuit
- Oscilloscope Picoscope6402 (Pico Technology, Saint Neots, UK)
- Isolated programmable power supply: EA-PSI 95100-10 DT (EA Elektro-Automatik, Viersen, Germany) max 500 V, max 10 A;
- capacitor (RS components, Gmünd, Austria) inside the power supply;
- power resistors (RS components, Gmünd, Austria) 1 ;
- high quality shunt (Isabellenhuette Heusler, Dillenburg, Germany) with ;
- Current clamp: Pico technology TA189 (Pico Technology, Saint Neots, UK) DC to 100 kHz Max 30 A. Overload 500 A, for 60 .
2.6. Experiment Method
3. Results
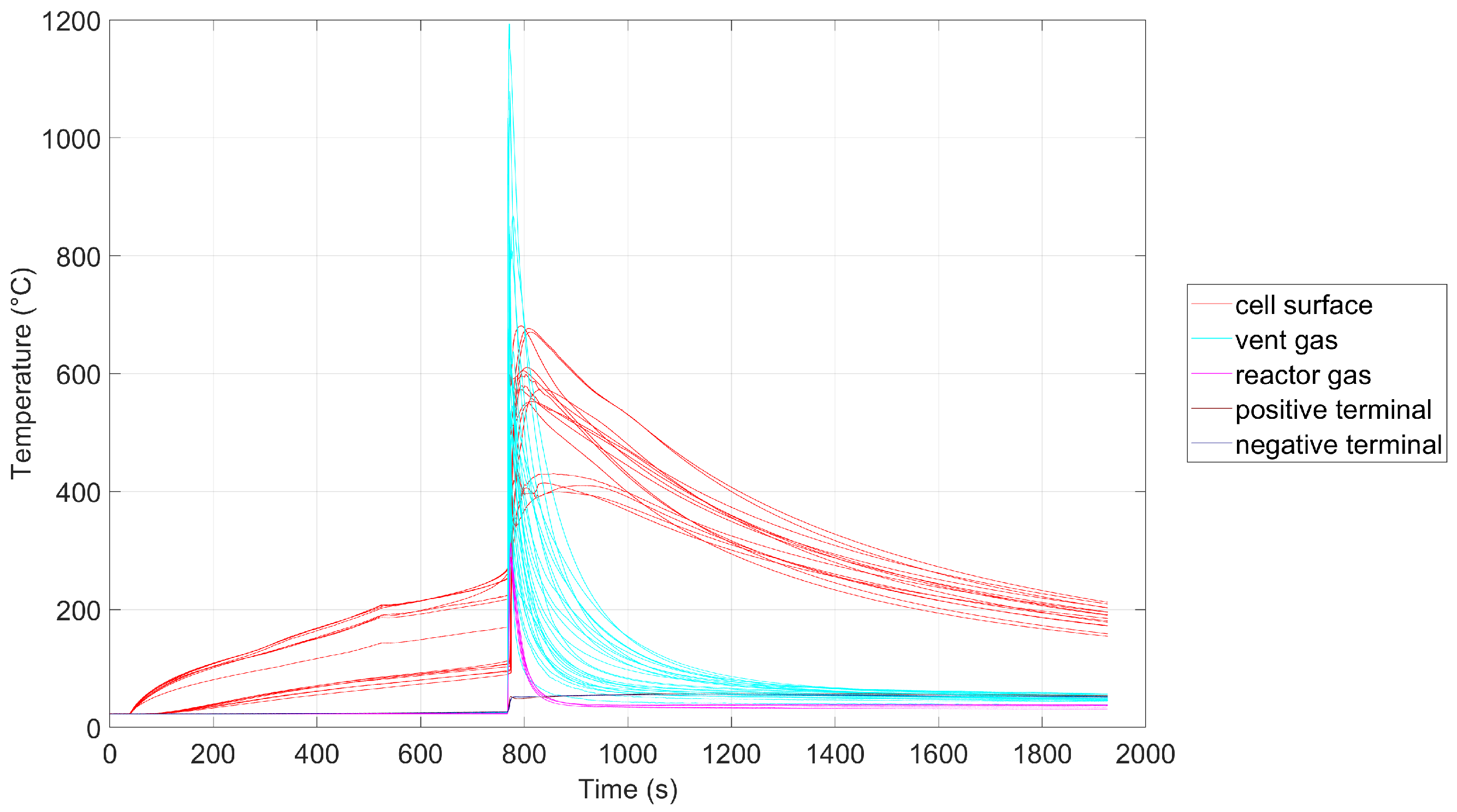
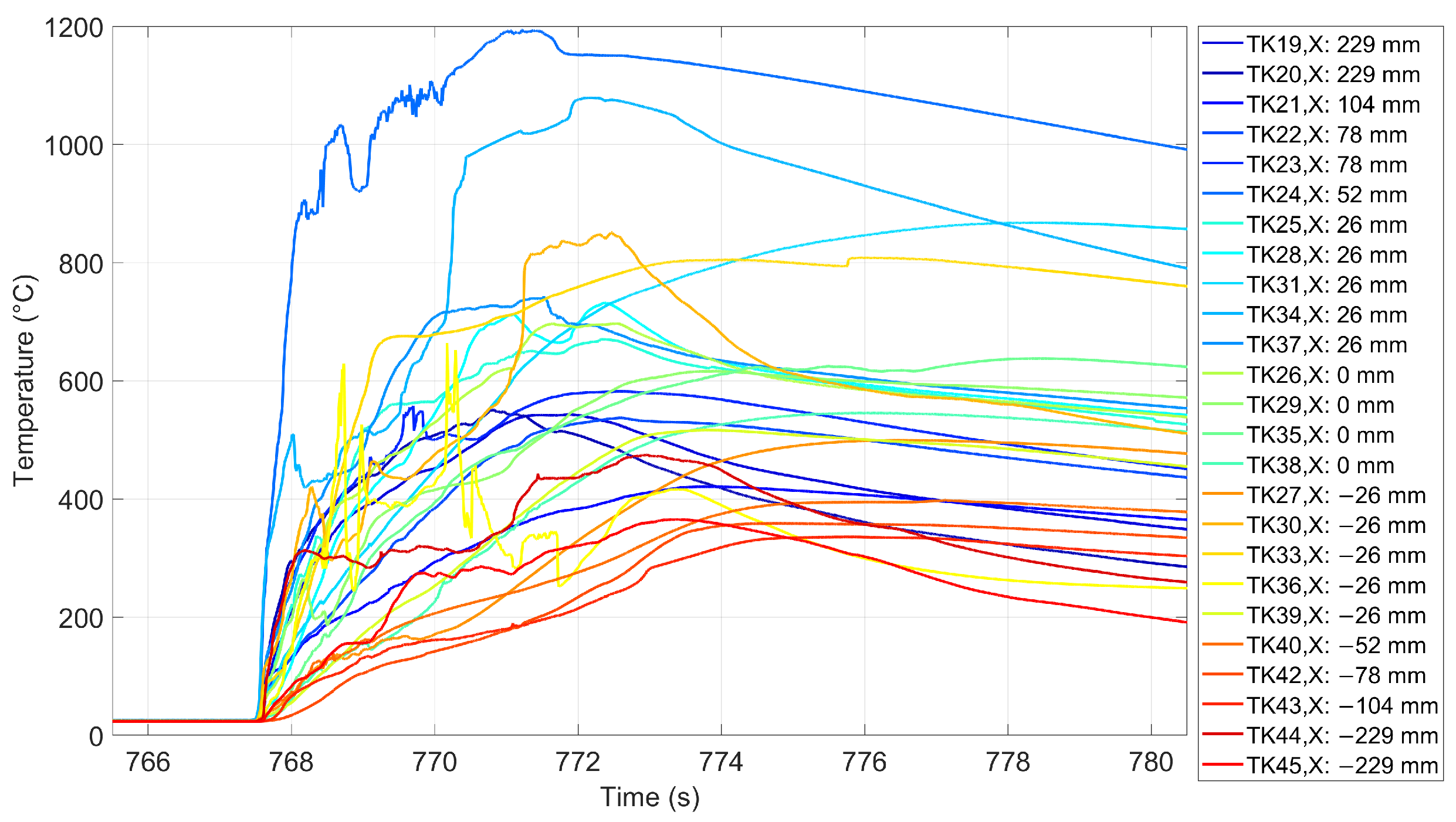
3.1. Cell Response
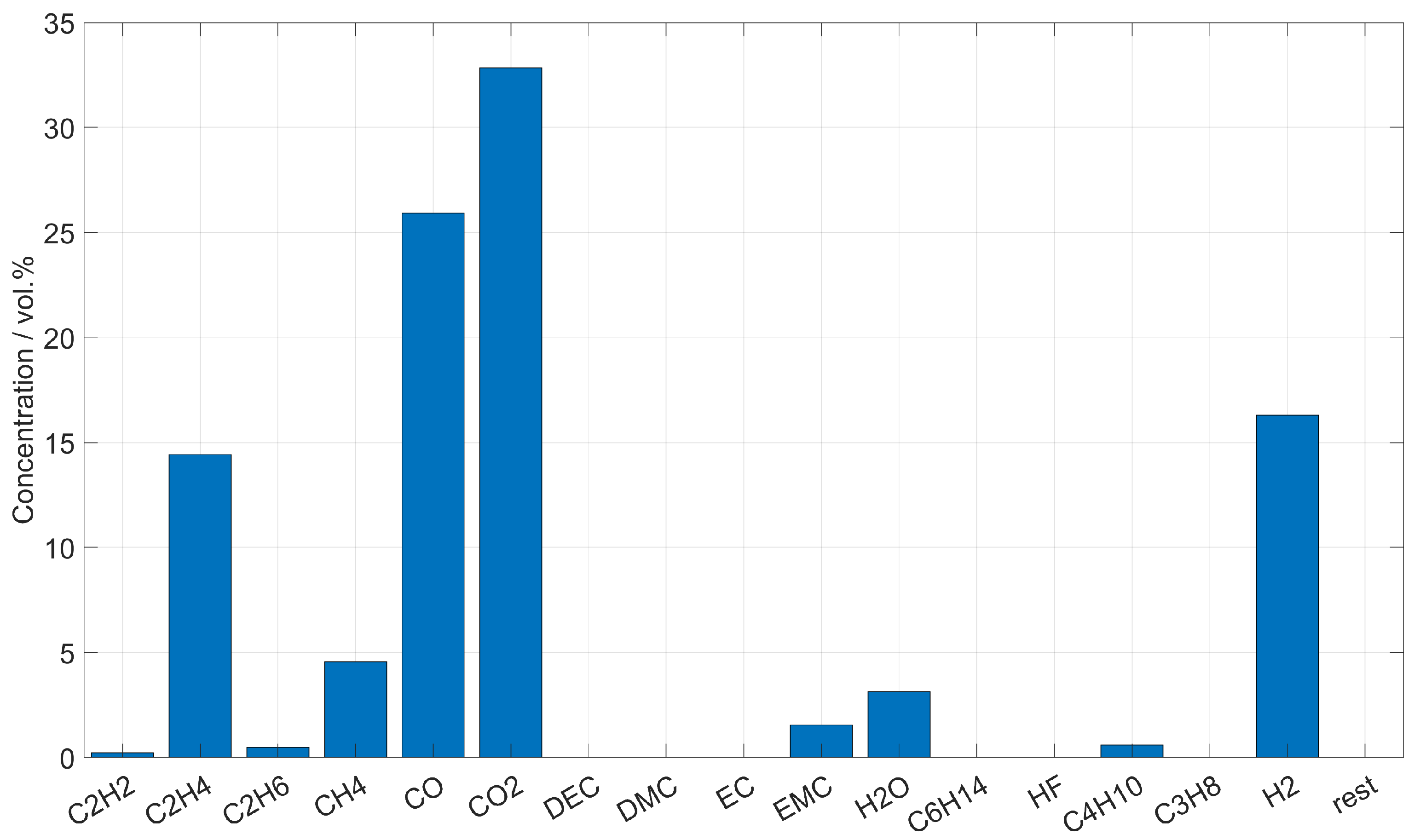
3.2. Gas Analysis
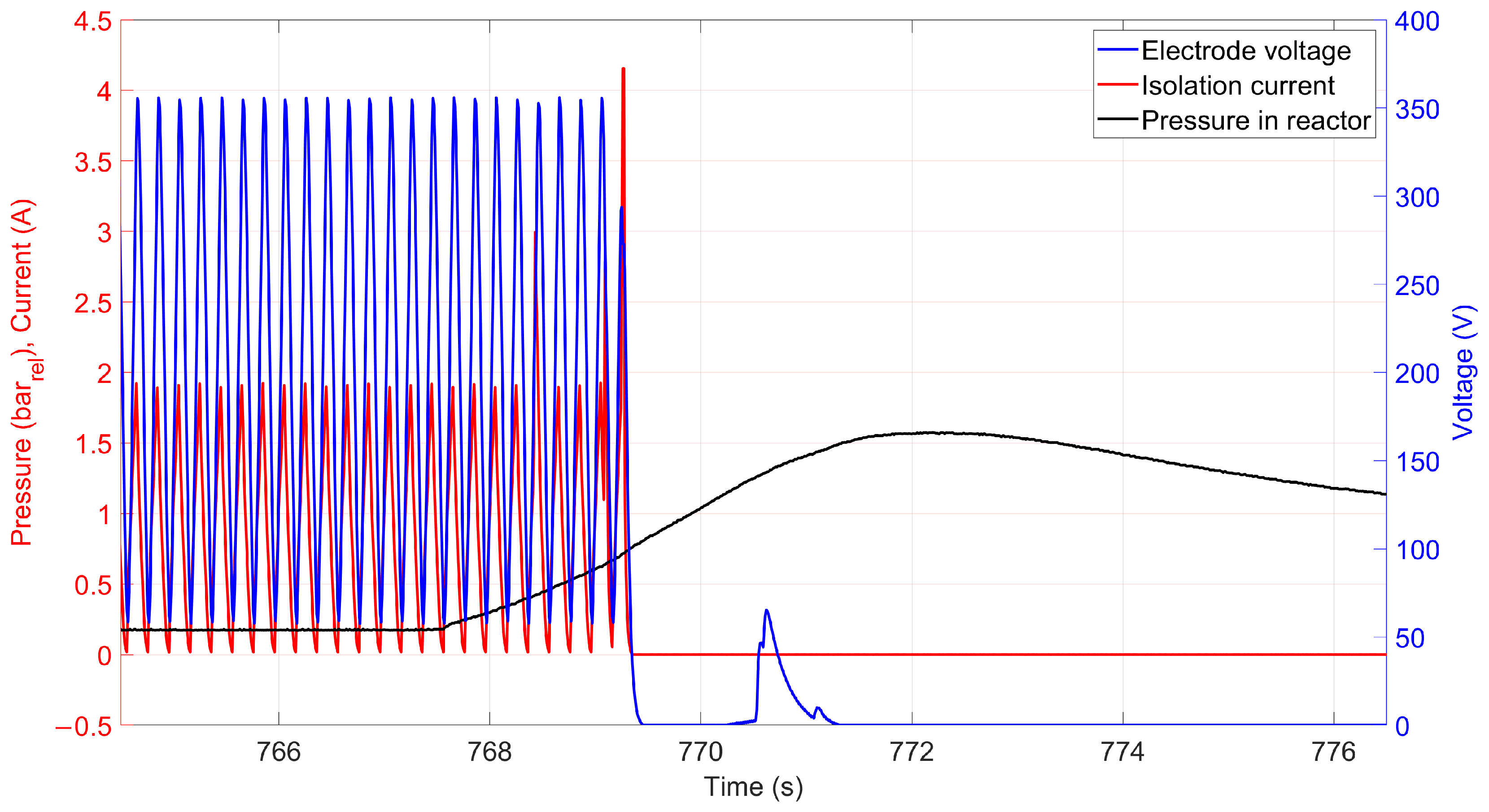
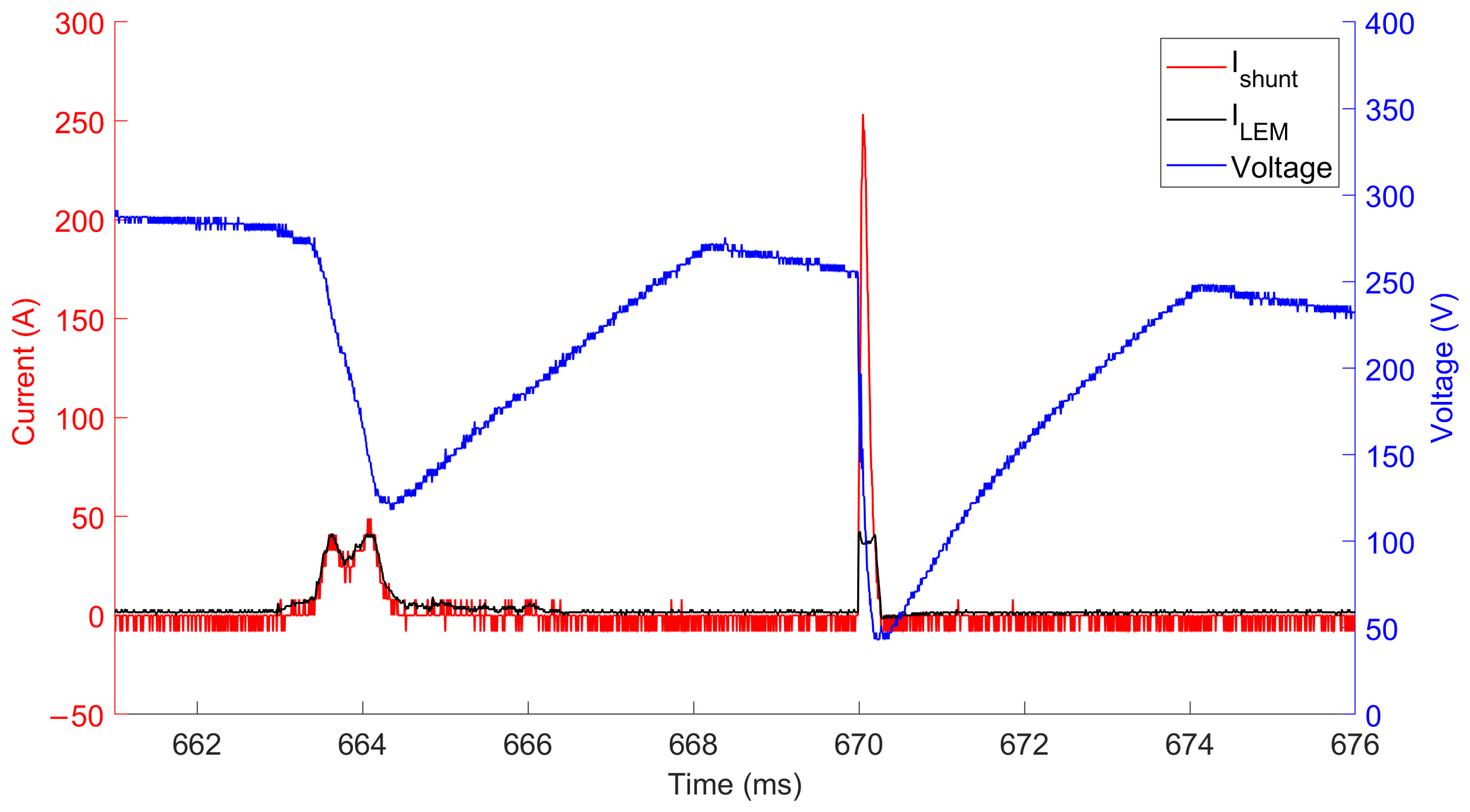
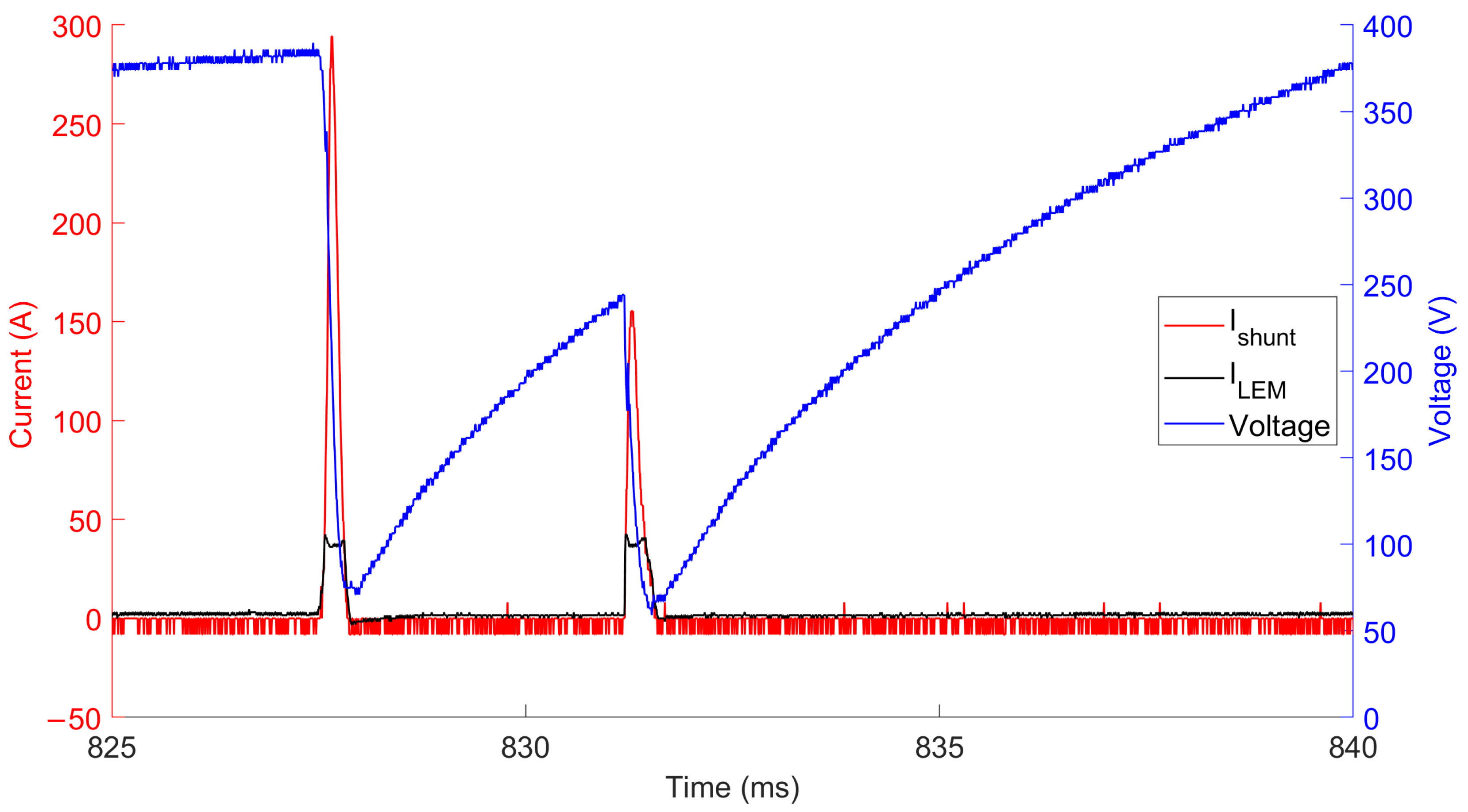
3.3. Electric Characteristics of the Vent-Gas-Jet
3.4. Cell and Ejected Material after Thermal Runaway
- 14 pieces ;
- 46 pieces 5 to 10 ;
- 48 pieces .
4. Discussion
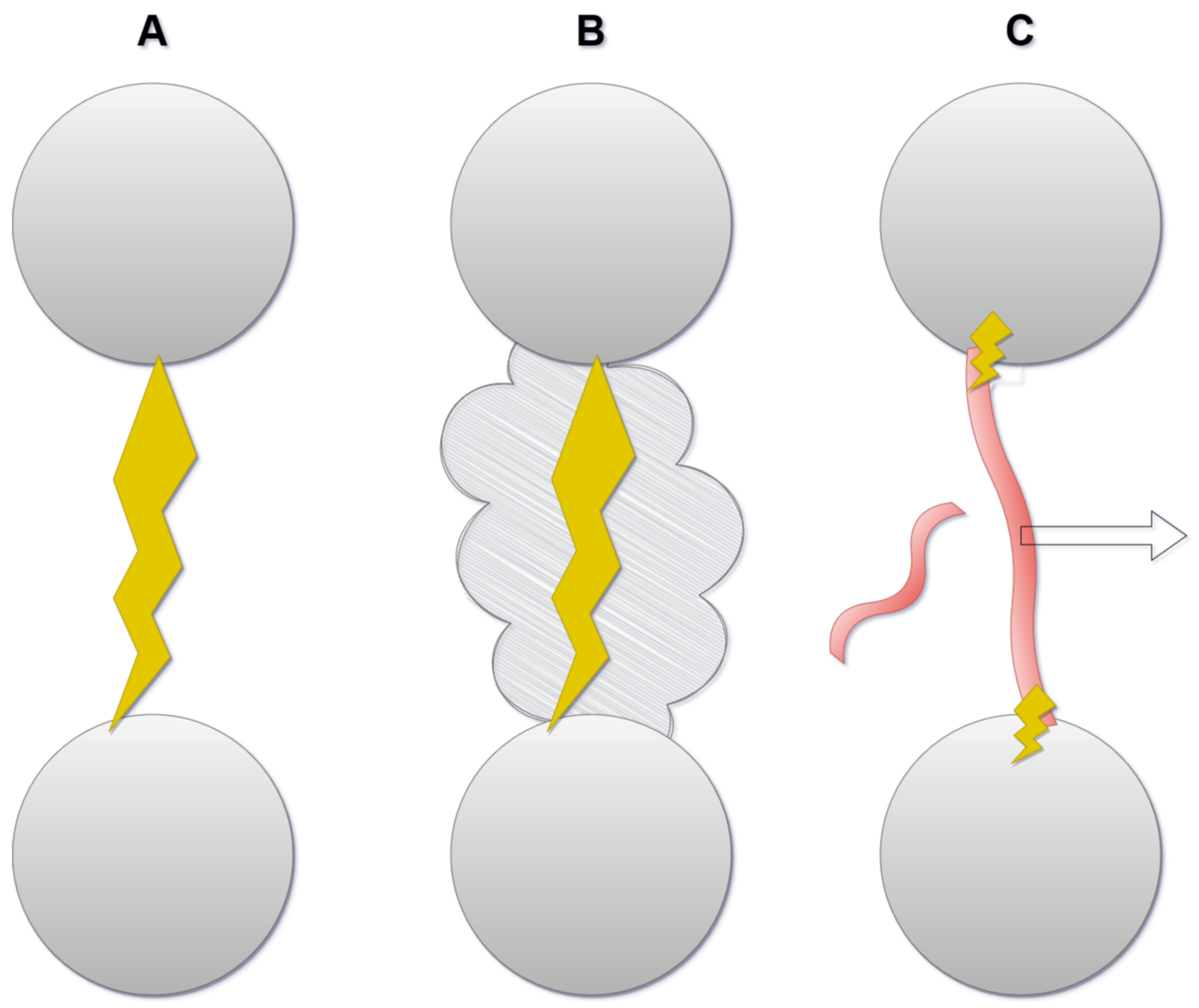
4.1. Arcing through Gas
4.2. Dust as Arcing Starter
4.3. Copper Pieces as Arcing Starter
5. Conclusions
Author Contributions
Funding
Data Availability Statement
Conflicts of Interest
Abbreviations
| EV | Electric vehicle |
| BMS | Battery management system |
| TR | Thermal runaway |
| TC | Thermocouple |
| PTFE | Polytetrafluoroethylene |
| DAQ | Data acquisition system |
Appendix A. Dust Resistivity Measurement
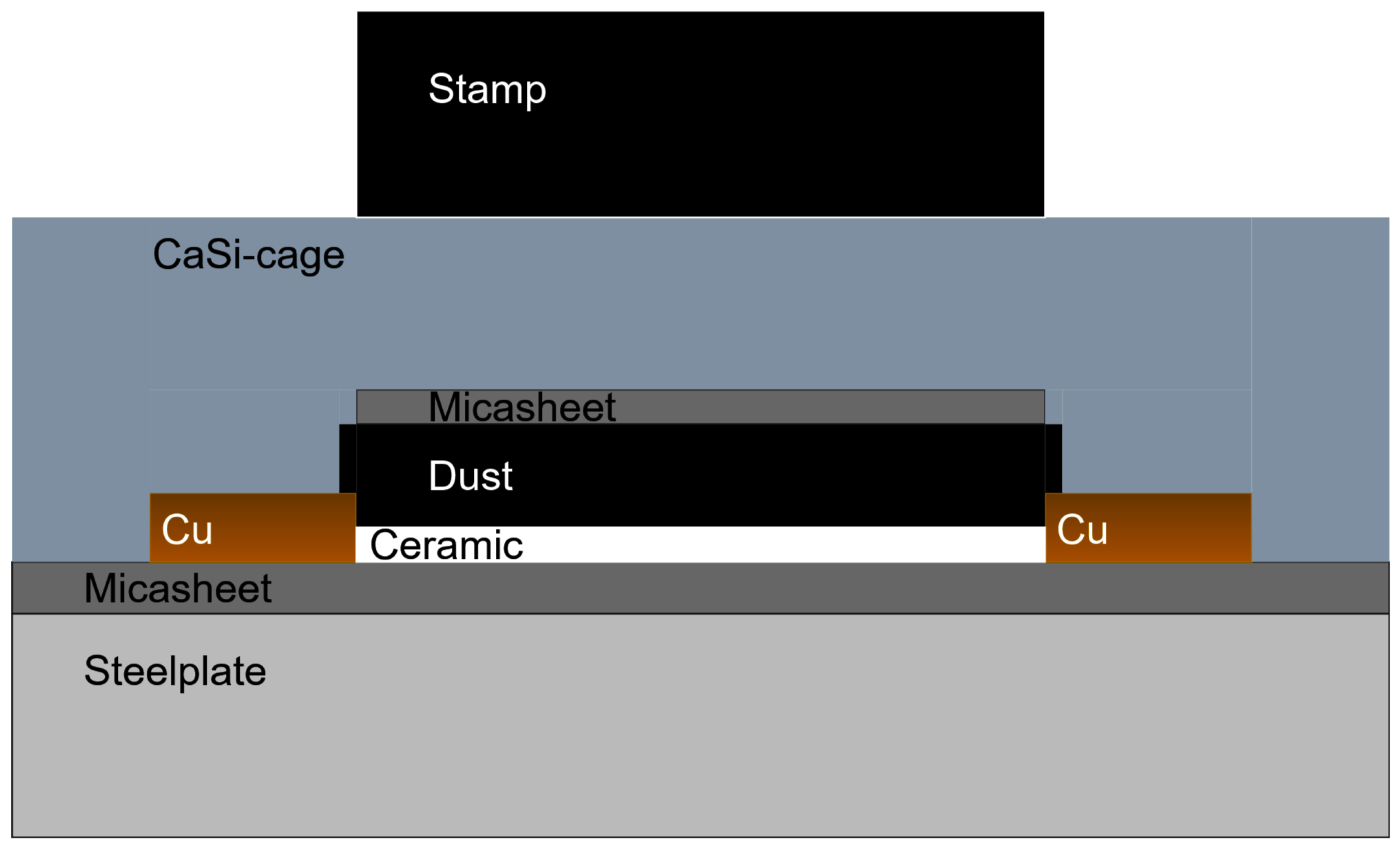

References
- IEA. Global Sales and Sales Market Share of Electric Cars, 2010–2021. Available online: https://www.iea.org/data-and-statistics/charts/global-sales-and-sales-market-share-of-electric-cars-2010-2021 (accessed on 24 October 2023).
- Muralidharan, N.; Self, E.C.; Dixit, M.; Du, Z.; Essehli, R.; Amin, R.; Nanda, J.; Belharouak, I. Next-Generation Cobalt-Free Cathodes—A Prospective Solution to the Battery Industry’s Cobalt Problem. Adv. Energy Mater. 2022, 12, 2103050. [Google Scholar] [CrossRef]
- Patel, D.; Robinson, J.B.; Ball, S.; Brett, D.J.L.; Shearing, P.R. Thermal Runaway of a Li-Ion Battery Studied by Combined ARC and Multi-Length Scale X-ray CT. J. Electrochem. Soc. 2020, 167, 090511. [Google Scholar] [CrossRef]
- Liao, Z.; Zhang, S.; Li, K.; Zhao, M.; Qiu, Z.; Han, D.; Zhang, G.; Habetler, T.G. Hazard analysis of thermally abused lithium-ion batteries at different state of charges. J. Energy Storage 2020, 27, 101065. [Google Scholar] [CrossRef]
- Yang, Y.; Fang, D.; Maleki, A.; Kohzadi, S.; Liu, Y.; Chen, Y.; Liu, R.; Gao, G.; Zhi, J. Characterization of Thermal-Runaway Particles from Lithium Nickel Manganese Cobalt Oxide Batteries and Their Biotoxicity Analysis. Acs Appl. Energy Mater. 2021, 4, 10713–10720. [Google Scholar] [CrossRef]
- Zou, K.; He, K.; Lu, S. Venting composition and rate of large-format LiNi0.8Co0.1Mn0.1O2 pouch power battery during thermal runaway. Int. J. Heat Mass Transf. 2022, 195, 123133. [Google Scholar] [CrossRef]
- Lai, X.; Yao, J.; Jin, C.; Feng, X.; Wang, H.; Xu, C.; Zheng, Y. A Review of Lithium-Ion Battery Failure Hazards: Test Standards, Accident Analysis, and Safety Suggestions. Batteries 2022, 8, 248. [Google Scholar] [CrossRef]
- Lebedeva, N.; Leite Patrao, R.; Lazareanu, M.; Pfrang, A.; Da Costa Barata, R. JRC’s thermal runaway propagation test campaign at pack and vehicle level. In Proceedings of the 24th GTR EVS Meeting, Online Event, 8–10 June 2022. [Google Scholar]
- Doan, D.R. Arc Flash Calculations for Exposures to DC Systems. IEEE Trans. Ind. Appl. 2010, 46, 2299–2302. [Google Scholar] [CrossRef]
- Xiong, R.; Ma, S.; Li, H.; Sun, F.; Li, J. Toward a Safer Battery Management System: A Critical Review on Diagnosis and Prognosis of Battery Short Circuit. iScience 2020, 23, 101010. [Google Scholar] [CrossRef] [PubMed]
- Abaza, A.; Ferrari, S.; Wong, H.K.; Lyness, C.; Moore, A.; Weaving, J.; Blanco-Martin, M.; Dashwood, R.; Bhagat, R. Experimental study of internal and external short circuits of commercial automotive pouch lithium-ion cells. J. Energy Storage 2018, 16, 211–217. [Google Scholar] [CrossRef]
- Rheinfeld, A.; Noel, A.; Wilhelm, J.; Kriston, A.; Pfrang, A.; Jossen, A. Quasi-Isothermal External Short Circuit Tests Applied to Lithium-Ion Cells: Part I. Measurements. J. Electrochem. Soc. 2018, 165, A3427. [Google Scholar] [CrossRef]
- Yang, R.; Xiong, R.; Shen, W. Experimental Study on External Short Circuit and Overcharge of Lithium-ion Battery Packs for Electric Vehicles. In Proceedings of the 2020 4th International Conference on Green Energy and Applications (ICGEA), Singapore, 7–9 March 2020; pp. 1–6. [Google Scholar] [CrossRef]
- Fu, Y.; Zhang, P.; Verboncoeur, J.; Wang, X. Electrical breakdown from macro to micro/nano scales: A tutorial and a review of the state of the art. Plasma Res. Express 2020, 2, 013001. [Google Scholar] [CrossRef]
- Ledinki, T.; Golubkov, A.; Schweighofer, O.; Erker, S. Arcing in Li-Ion Batteries: Supplementary Information; TU Graz: Graz, Austria, 2023. [Google Scholar] [CrossRef]
- Golubkov, A.W.; Scheikl, S.; Planteu, R.; Voitic, G.; Wiltsche, H.; Stangl, C.; Fauler, G.; Thaler, A.; Hacker, V. Thermal runaway of commercial 18650 Li-ion batteries with LFP and NCA cathodes – impact of state of charge and overcharge. RSC Adv. 2015, 5, 57171–57186. [Google Scholar] [CrossRef]
- Zhao, H.; Li, X.; Jia, S.; Murphy, A.B. Prediction of the critical reduced electric field strength for carbon dioxide and its mixtures with 50% O2 and 50% H2 from Boltzmann analysis for gas temperatures up to 3500 K at atmospheric pressure. J. Phys. D Appl. Phys. 2014, 47, 325203. [Google Scholar] [CrossRef]
- Lehr, J.; Ron, P. Electrical Breakdown in Gases. In Foundations of Pulsed Power Technology; John Wiley & Sons, Ltd: Hoboken, NJ, USA, 2017; pp. 369–438. [Google Scholar] [CrossRef]
- Nybeck, C.N.; Wetz, D.A.; Dodson, D.A.; Heinzel, J.M. Investigation of the Dielectric Breakdown Strength of Vented Li-Ion Electrolyte. IEEE Trans. Plasma Sci. 2018, 46, 3438–3443. [Google Scholar] [CrossRef]














| t/s | /°C | Event |
|---|---|---|
| 0 | 23 | Start of Experiment |
| 36.7 | 23 | Cell heater ON, power |
| 503.3 | 131 | First cell case temperature (TK7) reaches °C |
| 766.6 | 171 | 1 before start of 90% of the main gas release (immediately before start of TR). |
| 767.6 | 171 | Start of 90% of the main gas release (pressure starts to increase rapidly). |
| 767.6 | 171 | First vent gas temperature (TK34) reaches °C |
| 768.4 | 182 | Arcing event 1 |
| 769.1 | 218 | Arcing event 2 and 3 |
| 769.2 | 220 | Arcing event 4 and 5 |
| 769.4 | 220 | 400 V power source switches off due to overload |
| 770.9 | 218 | First reactor gas temperature (TK56) reaches °C |
| 771.3 | 229 | Voltage of the cell drops below 90% of . |
| 772.1 | 247 | Max. over-pressure (peak-baseline) inside the reactor bar. |
| 773.8 | 312 | Voltage of the cell drops below 50% of . |
| 775.1 | 378 | Last cell case temperature (TK10) reaches °C |
| 794.2 | 539 | Maximal local cell case temperature (TK5) °C. |
| 794.6 | 540 | Cell heater OFF, power |
| 800 | 547 | Maximal average cell case temperature = 547 °C. |
| Sample | / | R/ |
|---|---|---|
| V0 | 39.67 | 6.29 |
| V1 | 54.46 | 8.64 |
| V2 | 14,272.15 | 2263.56 |
| V2 | 111.66 | 17.71 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Ledinski, T.; Golubkov, A.W.; Schweighofer, O.; Erker, S. Arcing in Li-Ion Batteries. Batteries 2023, 9, 540. https://doi.org/10.3390/batteries9110540
Ledinski T, Golubkov AW, Schweighofer O, Erker S. Arcing in Li-Ion Batteries. Batteries. 2023; 9(11):540. https://doi.org/10.3390/batteries9110540
Chicago/Turabian StyleLedinski, Theo, Andrey W. Golubkov, Oskar Schweighofer, and Simon Erker. 2023. "Arcing in Li-Ion Batteries" Batteries 9, no. 11: 540. https://doi.org/10.3390/batteries9110540
APA StyleLedinski, T., Golubkov, A. W., Schweighofer, O., & Erker, S. (2023). Arcing in Li-Ion Batteries. Batteries, 9(11), 540. https://doi.org/10.3390/batteries9110540






