Effect of Phase Change Materials on Lithium-Ion Plate Batteries
Abstract
1. Introduction
2. Problem Definition
3. Governing Equations
4. Numerical Method
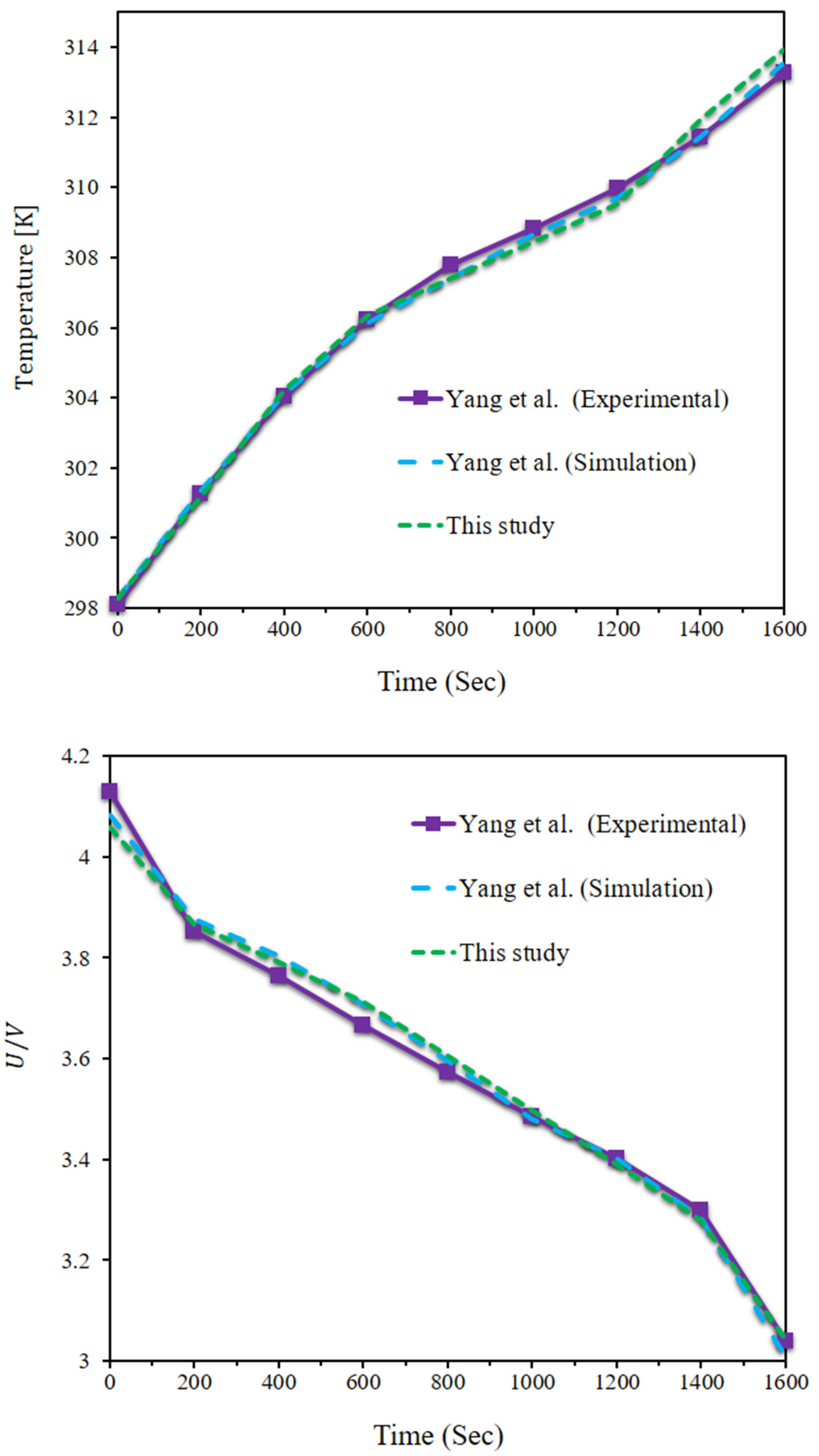
5. Validation and Grid Study
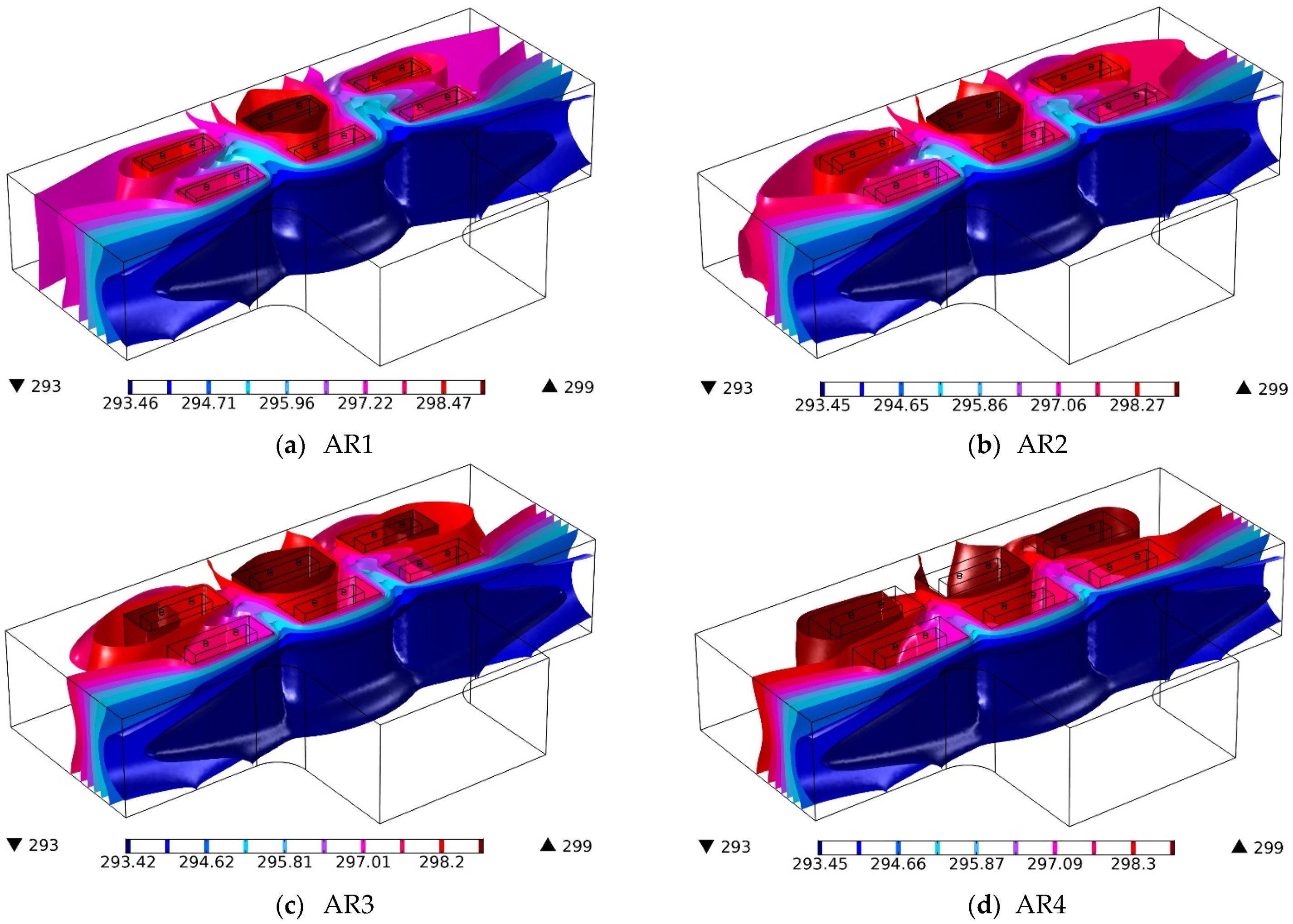
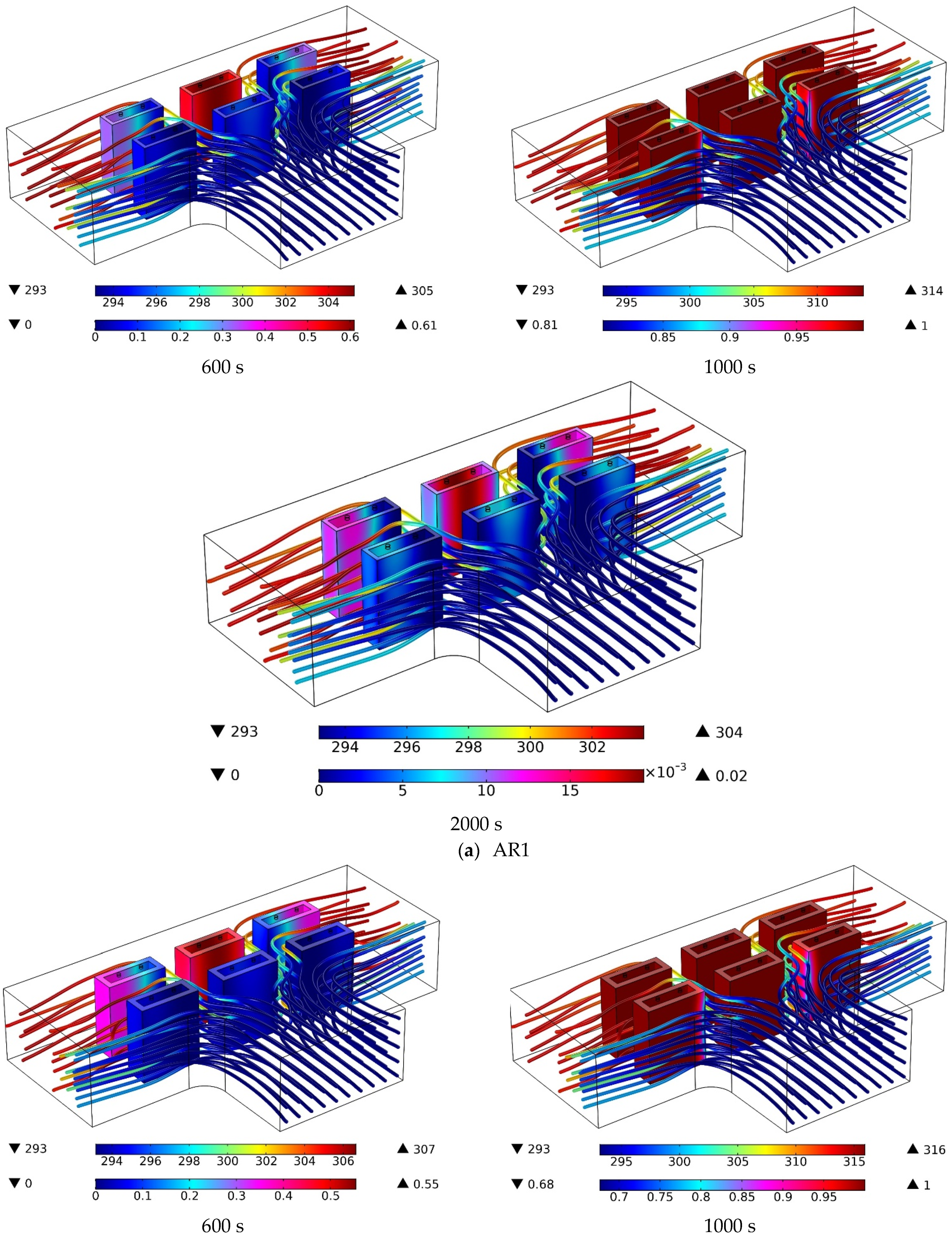
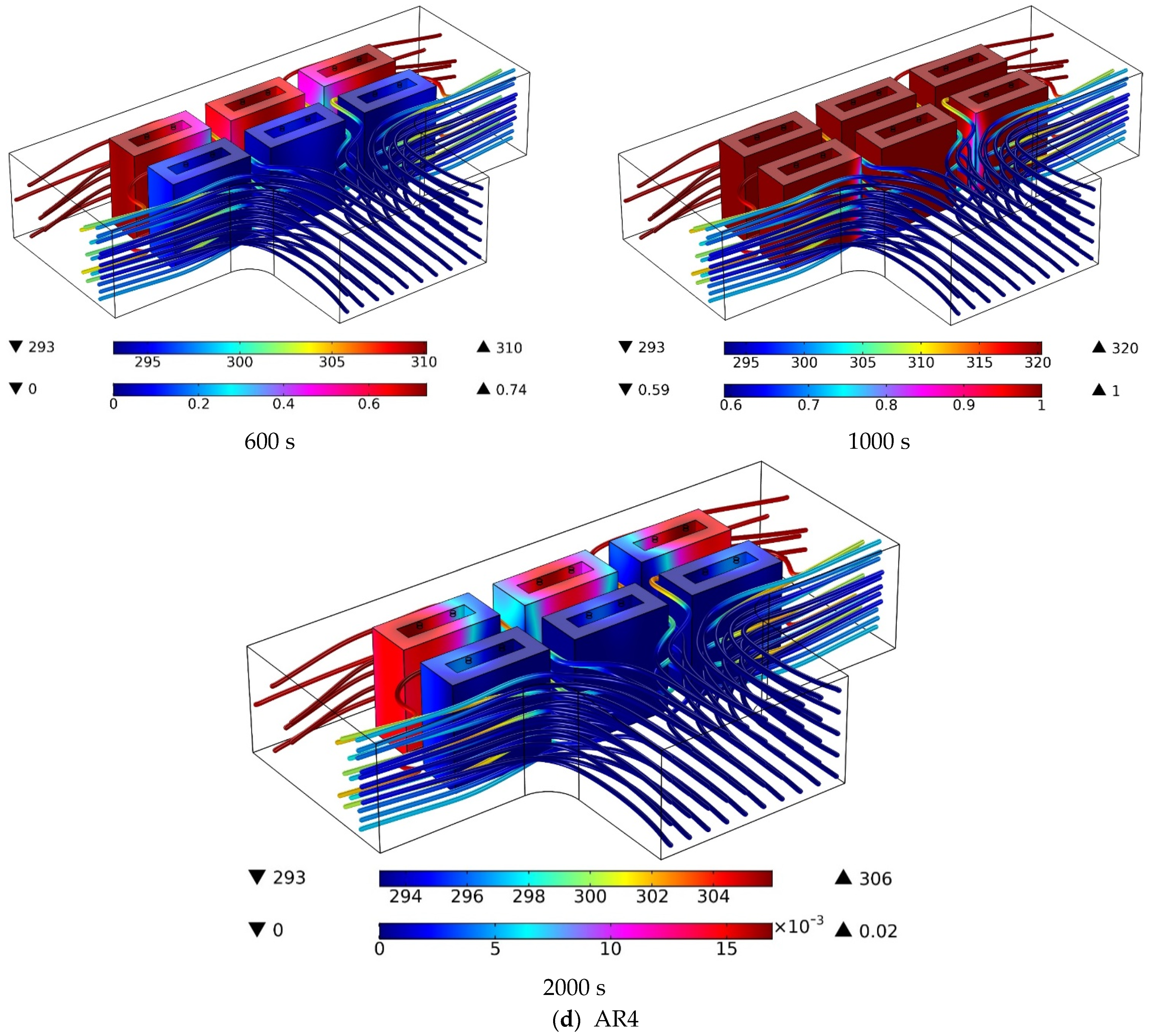
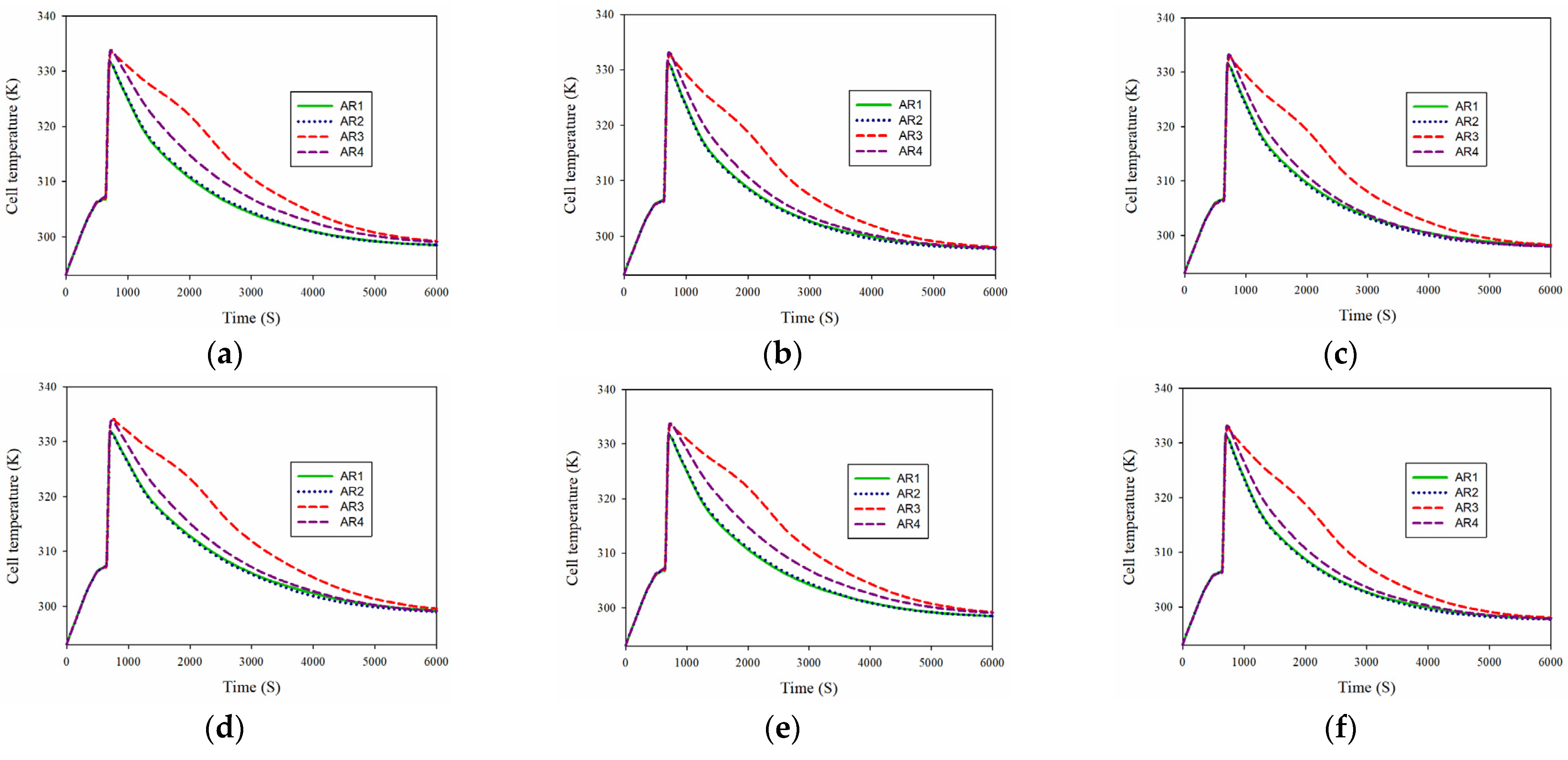
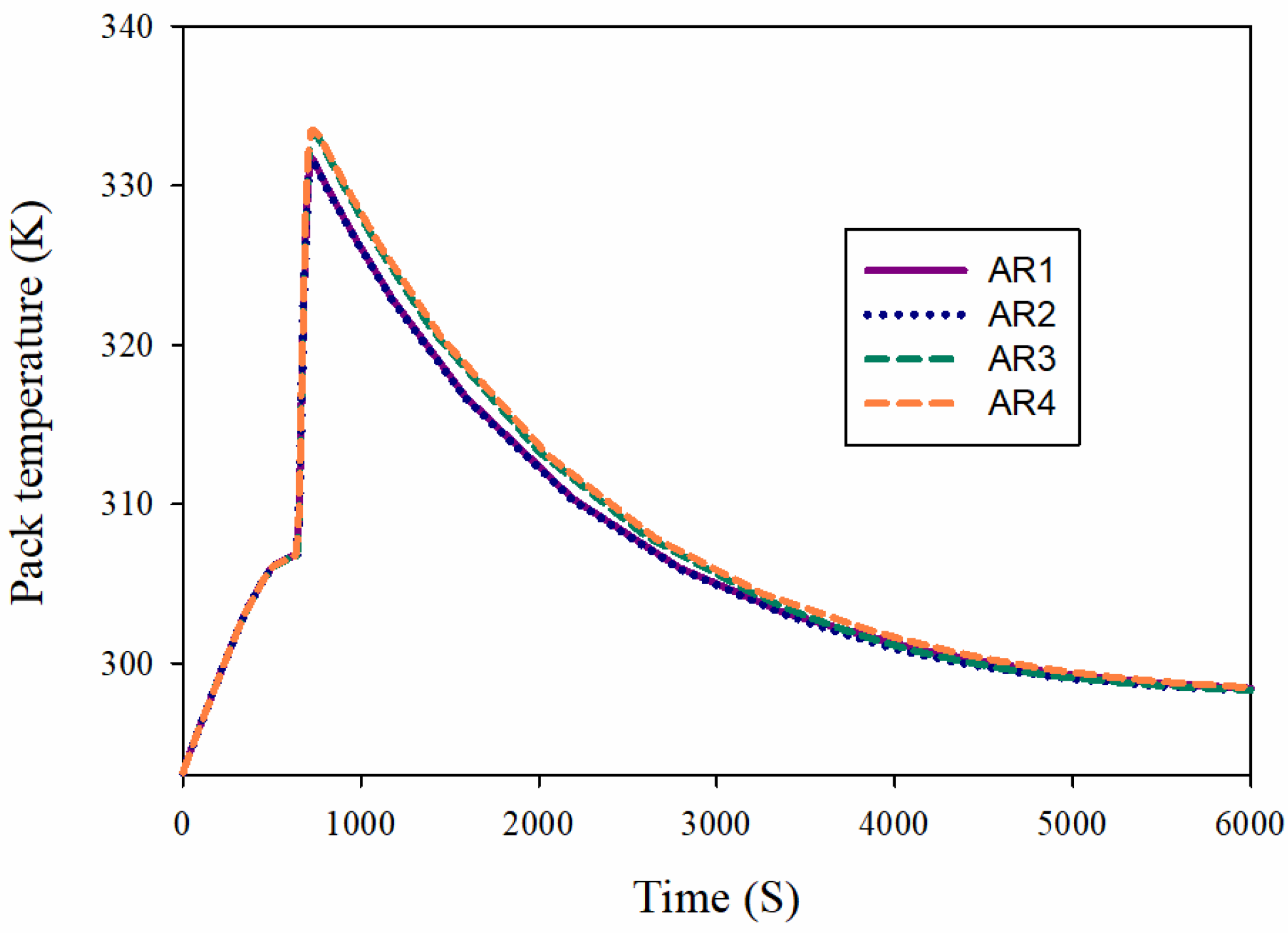
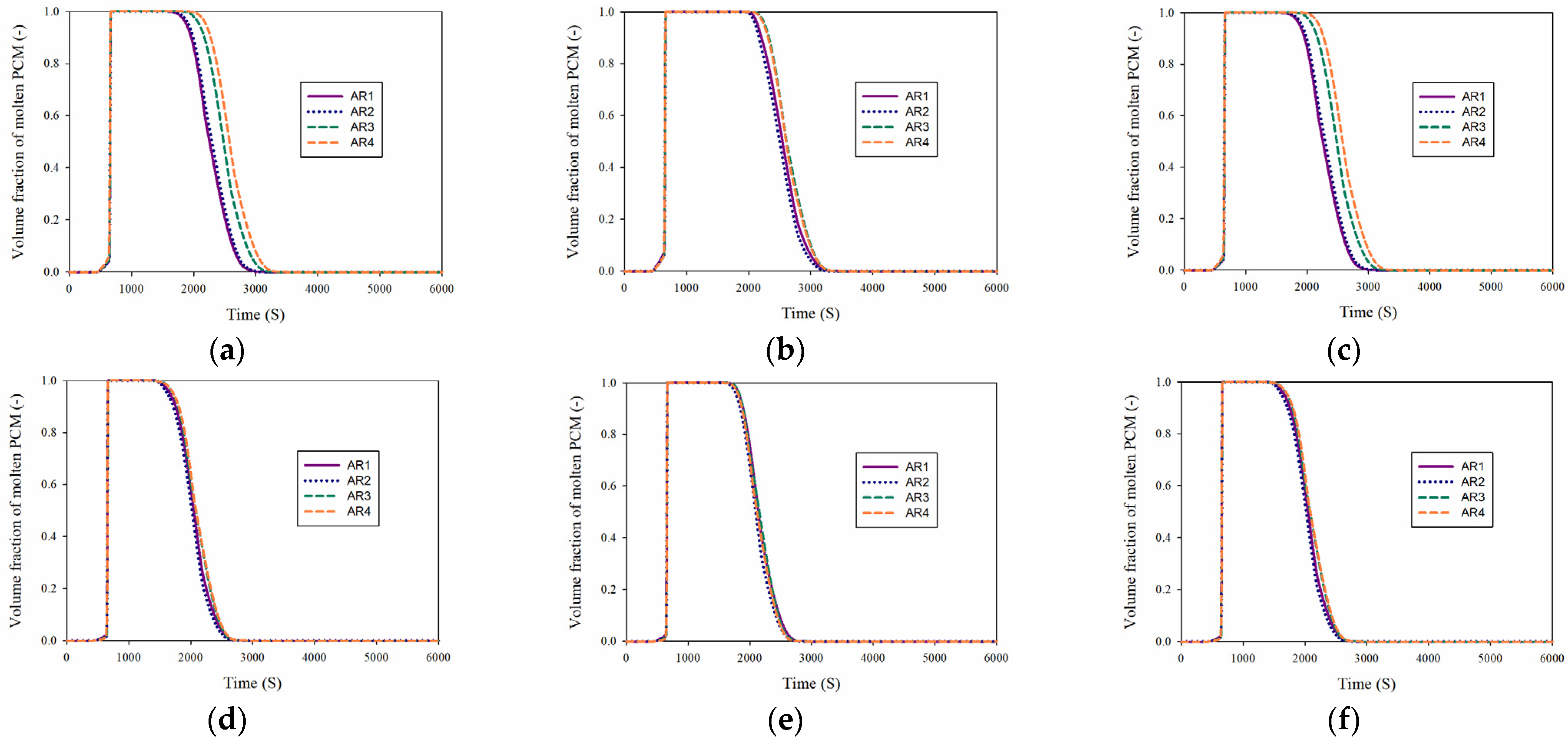
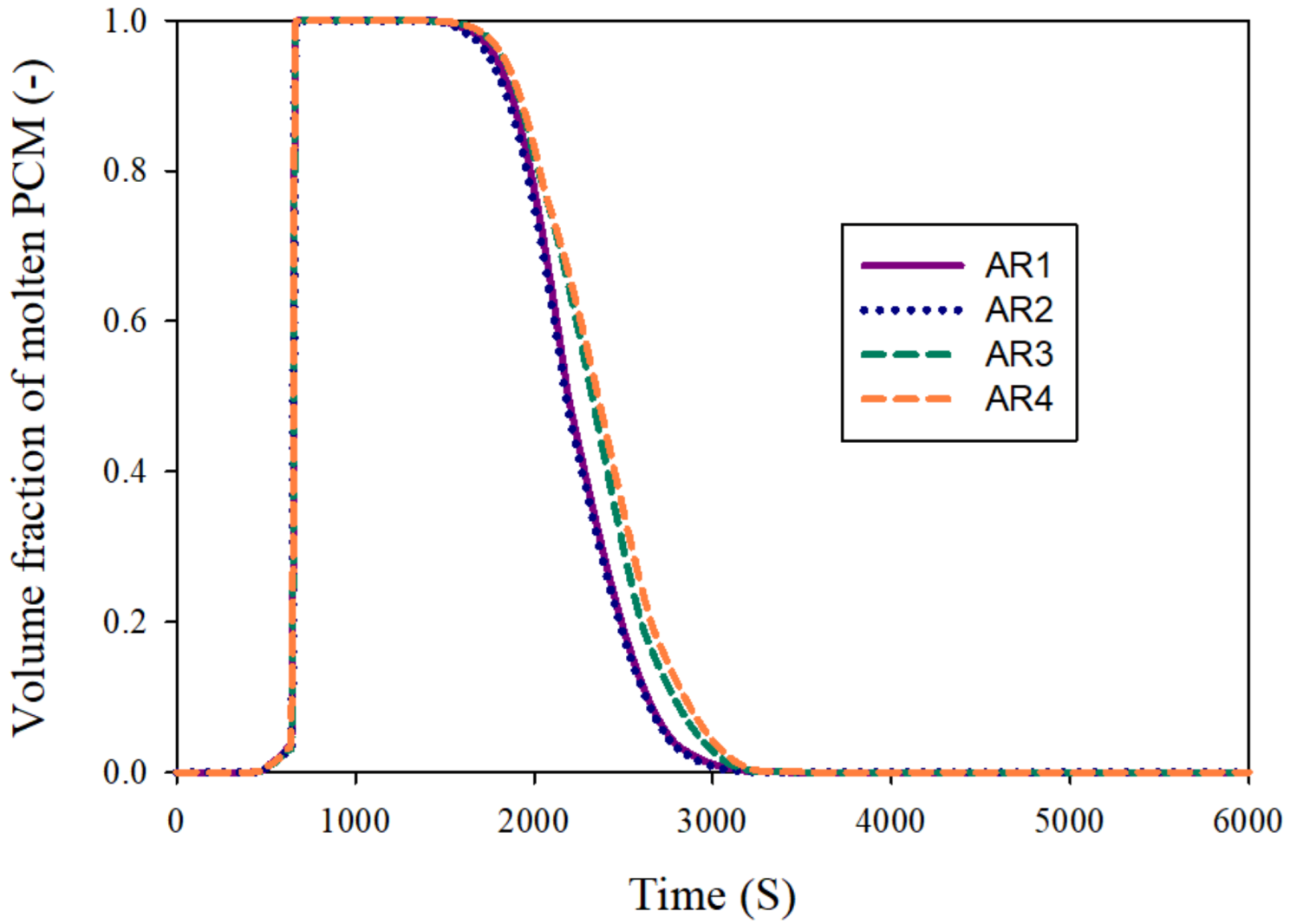
6. Results and Discussion
7. Conclusions
Author Contributions
Funding
Data Availability Statement
Acknowledgments
Conflicts of Interest
Nomenclature
| Specific heat | PCM | Phase change material | |
| Concentration of lithium ions in the solid (mol/dm3) | PVOF | PCM volume fraction | |
| c | Salt concentration(mol/dm3) | TMGT | Thermal management of battery |
| Ds | Lithium diffusion coefficient in the solid electrode (cm2/s) | TOB | Temperature of the battery |
| E | Specific energy (Wh/kg) | Greek symbols | |
| F | Faraday constant (96,485 C/mol) | λ | PCM of VOF |
| g | Gravitational acceleration [m/s2] | Thermal diffusivity | |
| h | Enthalpy | ε | Volume fraction |
| Current (A) | Dynamic viscosity | ||
| k | Thermal conductivity | Density | |
| Pressure | ϕ | Electrical potential (V) | |
| Electrical resistivity () | η | Electrode potential (V) | |
| T | Temperature [K] | σ | Solid matrix electronic conductivity (S cm−1) |
| t | Time (s) | Subscripts | |
| Chemical reaction heat | eff | Effective | |
| u,v | Velocity components in x and y directions | f | Fluid |
| x,y | Cartesian coordinates, | l | Liquid |
| V | Operating voltage of the battery (V) | s | Solid |
| U | Open circuit voltage (V) | + | Positive electrode |
| Abbreviations | 1 | Solid phase of the electrode | |
| AR | Aspect ratio | 2 | Solution phase of the electrode |
| BTCL | Battery cells | i | Layer in lithium ion battery |
| BTPC | Battery pack | J | Joule heat |
| FEM | finite element method | ||
| LIN | Lithium-ion | ||
References
- Gibbons, L.; Javed, S. A review of HVAC solution-sets and energy performace of nearly zero-energy multi-story apartment buildings in Nordic climates by statistical analysis of environmental performance certificates and literature review. Energy 2022, 238, 121709. [Google Scholar] [CrossRef]
- Tian, G.; Fan, Y.; Gao, M.; Wang, H.; Zheng, H.; Liu, J.; Liu, C. Indoor thermal environment of thin membrane structure Buildings: A review. Energy Build. 2021, 234, 110704. [Google Scholar] [CrossRef]
- Jurasz, J.; Canales, F.A.; Kies, A.; Guezgouz, M.; Beluco, A. A review on the complementarity of renewable energy sources: Concept, metrics, application and future research directions. Sol. Energy 2020, 195, 703–724. [Google Scholar] [CrossRef]
- Lowitzsch, J.; Hoicka, C.E.; van Tulder, F.J. Renewable energy communities under the 2019 European Clean Energy Package–Governance model for the energy clusters of the future? Renew. Sustain. Energy Rev. 2020, 122, 109489. [Google Scholar] [CrossRef]
- Bamisile, O.; Obiora, S.; Huang, Q.; Yimen, N.; Idriss, I.A.; Cai, D.; Dagbasi, M. Impact of economic development on CO2 emission in Africa; the role of BEVs and hydrogen production in renewable energy integration. Int. J. Hydrogen Energy 2021, 46, 2755–2773. [Google Scholar] [CrossRef]
- Shaqsi, A.Z.A.; Sopian, K.; Al-Hinai, A. Review of energy storage services, applications, limitations, and benefits. Energy Rep. 2020, 6, 288–306. [Google Scholar] [CrossRef]
- Agrawal, T.; Ajitkumar, R.; Prakash, R.; Nandan, G. Sodium Silicide As A Hydrogen Source For Portable Energy Devices: A Review. Mater. Today: Proc. 2018, 5, 3563–3570. [Google Scholar] [CrossRef]
- Østergaard, P.A.; Duic, N.; Noorollahi, Y.; Mikulcic, H.; Kalogirou, S. Sustainable development using renewable energy technology. Renew. Energy 2020, 146, 2430–2437. [Google Scholar] [CrossRef]
- Kehrein, P.; Van Loosdrecht, M.; Osseweijer, P.; Garfí, M.; Dewulf, J.; Posada, J. A critical review of resource recovery from municipal wastewater treatment plants–market supply potentials, technologies and bottlenecks. Environ. Sci. Water Res. Technol. 2020, 6, 877–910. [Google Scholar] [CrossRef]
- Lyu, P.; Liu, X.; Qu, J.; Zhao, J.; Huo, Y.; Qu, Z.; Rao, Z. Recent advances of thermal safety of lithium ion battery for energy storage. Energy Storage Mater. 2020, 31, 195–220. [Google Scholar] [CrossRef]
- Killer, M.; Farrokhseresht, M.; Paterakis, N.G. Implementation of large-scale Li-ion battery energy storage systems within the EMEA region. Appl. Energy 2020, 260, 114166. [Google Scholar] [CrossRef]
- Zhang, D.; Tan, C.; Ou, T.; Zhang, S.; Li, L.; Ji, X. Constructing advanced electrode materials for low-temperature lithium-ion batteries: A review. Energy Rep. 2022, 8, 4525–4534. [Google Scholar] [CrossRef]
- Shi, Q.; Zhou, J.; Ullah, S.; Yang, X.; Tokarska, K.; Trzebicka, B.; Ta, H.Q.; Rümmeli, M.H. A review of recent developments in Si/C composite materials for Li-ion batteries. Energy Storage Mater. 2021, 34, 735–754. [Google Scholar] [CrossRef]
- Bose, B.; Garg, A.; Panigrahi, B.; Kim, J. Study on Li-ion battery fast charging strategies: Review, challenges and proposed charging framework. J. Energy Storage 2022, 55, 105507. [Google Scholar] [CrossRef]
- Palacín, M.R. Understanding ageing in Li-ion batteries: A chemical issue. Chem. Soc. Rev. 2018, 47, 4924–4933. [Google Scholar] [CrossRef] [PubMed]
- Raijmakers, L.; Danilov, D.; Eichel, R.-A.; Notten, P. A review on various temperature-indication methods for Li-ion batteries. Appl. Energy 2019, 240, 918–945. [Google Scholar] [CrossRef]
- Sharma, D.K.; Prabhakar, A. A review on air cooled and air centric hybrid thermal management techniques for Li-ion battery packs in electric vehicles. J. Energy Storage 2021, 41, 102885. [Google Scholar] [CrossRef]
- Qian, S.; Yu, J.; Yan, G. A review of regenerative heat exchange methods for various cooling technologies. Renew. Sustain. Energy Rev. 2017, 69, 535–550. [Google Scholar] [CrossRef]
- Okonkwo, E.C.; Wole-Osho, I.; Almanassra, I.W.; Abdullatif, Y.M.; Al-Ansari, T. An updated review of nanofluids in various heat transfer devices. J. Therm. Anal. Calorim. 2021, 145, 2817–2872. [Google Scholar] [CrossRef]
- Yao, J. A Review of Industrial Heat Exchange Optimization. IOP Conf. Ser. Earth Environ. Sci. 2018, 108, 042036. [Google Scholar] [CrossRef]
- Khan, M.; Swierczynski, M.; Kær, S. Towards an Ultimate Battery Thermal Management System: A Review. Batteries 2017, 3, 9. [Google Scholar] [CrossRef]
- Al-Zareer, M.; Dincer, I.; Rosen, M.A. A review of novel thermal management systems for batteries. Int. J. Energy Res. 2018, 42, 3182–3205. [Google Scholar] [CrossRef]
- Xiao, X.; Zhang, P.; Li, M. Preparation and thermal characterization of paraffin/metal foam composite phase change material. Appl. Energy 2013, 112, 1357–1366. [Google Scholar] [CrossRef]
- Wang, Z.; Zhang, Z.; Jia, L.; Yang, L.-X. Paraffin and paraffin/aluminum foam composite phase change material heat storage experimental study based on thermal management of Li-ion battery. Appl. Therm. Eng. 2015, 78, 428–436. [Google Scholar] [CrossRef]
- Li, W.; Qu, Z.; He, Y.; Tao, Y. Experimental study of a passive thermal management system for high-powered lithium ion batteries using porous metal foam saturated with phase change materials. J. Power Sources 2014, 255, 9–15. [Google Scholar] [CrossRef]
- Jilte, R.; Afzal, A.; Panchal, S. A novel battery thermal management system using nano-enhanced phase change materials. Energy 2021, 219, 119564. [Google Scholar] [CrossRef]
- Choudhari, V.; Dhoble, A.; Panchal, S. Numerical analysis of different fin structures in phase change material module for battery thermal management system and its optimization. Int. J. Heat Mass Transf. 2020, 163, 120434. [Google Scholar] [CrossRef]
- Deng, D. Li-ion batteries: Basics, progress, and challenges. Energy Sci. Eng. 2015, 3, 385–418. [Google Scholar] [CrossRef]
- Zubi, G.; Dufo-López, R.; Carvalho, M.; Pasaoglu, G. The lithium-ion battery: State of the art and future perspectives. Renew. Sustain. Energy Rev. 2018, 89, 292–308. [Google Scholar] [CrossRef]
- Doyle, M.; Newman, J.; Gozdz, A.S.; Schmutz, C.N.; Tarascon, J.-M. Comparison of Modeling Predictions with Experimental Data from Plastic Lithium Ion Cells. J. Electrochem. Soc. 1996, 143, 1890. [Google Scholar] [CrossRef]
- Al-Zareer, M.; Dincer, I.; Rosen, M.A. Electrochemical modeling and performance evaluation of a new ammonia-based battery thermal management system for electric and hybrid electric vehicles. Electrochimica Acta 2017, 247, 171–182. [Google Scholar] [CrossRef]
- Amiribavandpour, P.; Shen, W.; Mu, D.; Kapoor, A. An improved theoretical electrochemical-thermal modelling of lithium-ion battery packs in electric vehicles. J. Power Sources 2015, 284, 328–338. [Google Scholar] [CrossRef]
- Karimi, G.; Li, X. Thermal management of lithium-ion batteries for electric vehicles. Int. J. Energy Res. 2013, 37, 13–24. [Google Scholar] [CrossRef]
- Panchal, S.; Khasow, R.; Dincer, I.; Agelin-Chaab, M.; Fraser, R.; Fowler, M. Thermal design and simulation of mini-channel cold plate for water cooled large sized prismatic lithium-ion battery. Appl. Therm. Eng. 2017, 122, 80–90. [Google Scholar] [CrossRef]
- Khetib, Y.; Alotaibi, A.A.; Alshahri, A.H.; Cheraghan, G.; Sharifpur, M.; Meyer, J.P. Study on the Effect of Hole Size of Trombe Wall in the Presence of Phase Change Material for Different Times of a Day in Winter and Summer. Processes 2021, 9, 1886. [Google Scholar] [CrossRef]
- Kant, K.; Shukla, A.; Sharma, A.; Henry Biwole, P. Heat transfer study of phase change materials with graphene nano particle for thermal energy storage. Sol. Energy 2017, 146, 453–463. [Google Scholar] [CrossRef]
- Yang, W.; Zhou, F.; Liu, Y.; Xu, S.; Chen, X. Thermal performance of honeycomb-like battery thermal management system with bionic liquid mini-channel and phase change materials for cylindrical lithium-ion battery. Appl. Therm. Eng. 2021, 188, 116649. [Google Scholar] [CrossRef]
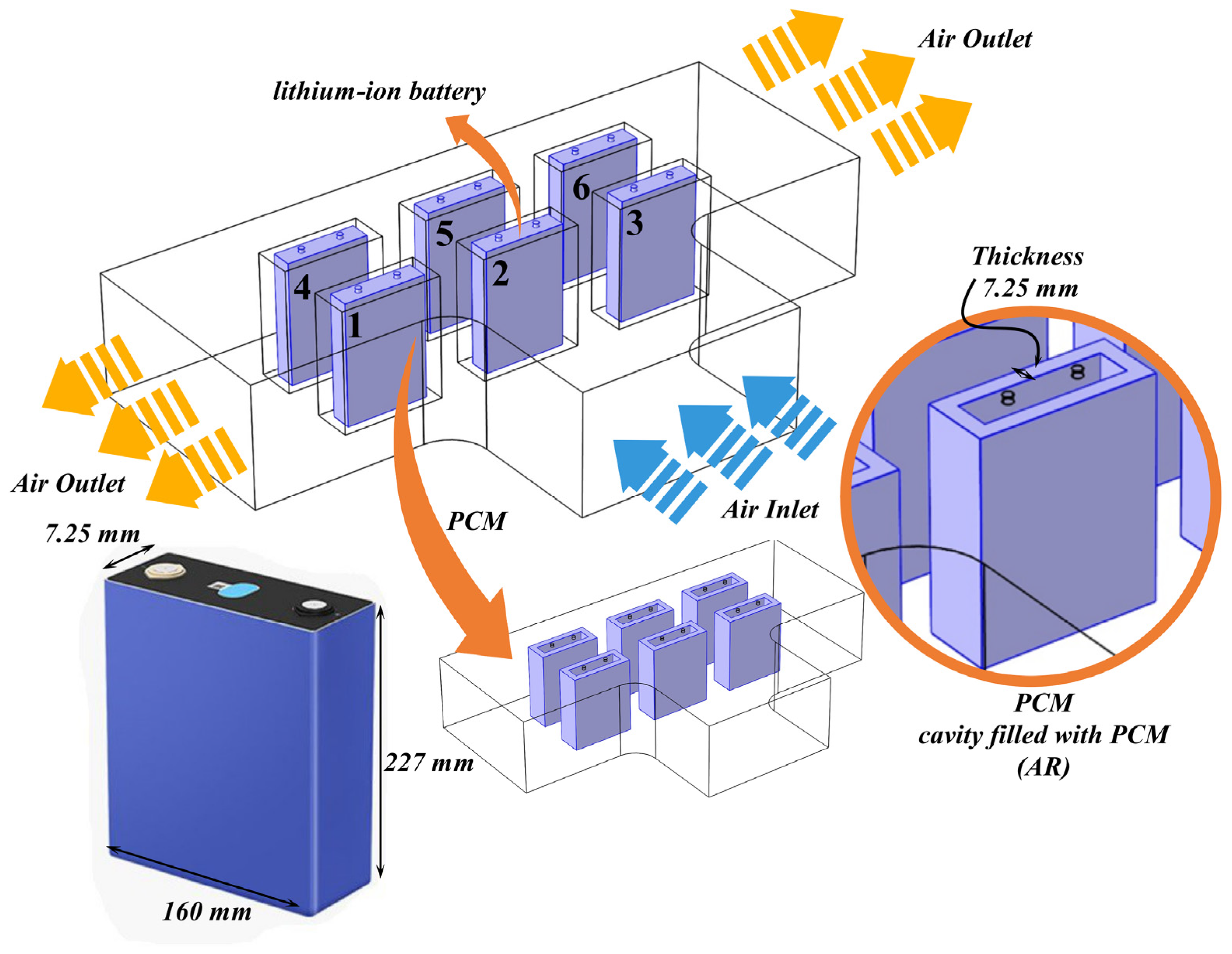




| Cathode Material: LiFePO4 |
| Anode Material: Graphite |
| Electrolyte: Carbonate based |
| Nominal Capacity: 20.0 Ah |
| Nominal Voltage: 3.3 V |
| Properties | CaCl2.6H2O | Air |
|---|---|---|
| Specific heat (J/kg K) | 1400 (Solid) | 1.012 |
| 2100 (Liquid) | ||
| Latent heat of fusion (kJ/kg) | 192 | - |
| Density (kg/m3) | 1802 (Solid) | 1.225 |
| 1562 (Liquid) | ||
| Thermal conductivity (W/m.k) | 1.008 (Solid) | 0.025 |
| 0.561 (Liquid) | ||
| Melting point (°C) | 29 | - |
| Grid | 367091 | 408092 | 461283 | 502967 | 554032 | 593482 |
| T | 298.44 | 298.21 | 297.98 | 297.89 | 297.84 | 297.84 |
| AR | 1 | 2 | 3 | 4 |
| Pressure drop (mPa) | 1.28 | 1.32 | 1.35 | 1.46 |
Disclaimer/Publisher’s Note: The statements, opinions and data contained in all publications are solely those of the individual author(s) and contributor(s) and not of MDPI and/or the editor(s). MDPI and/or the editor(s) disclaim responsibility for any injury to people or property resulting from any ideas, methods, instructions or products referred to in the content. |
© 2023 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Mustafa, J.; Alqaed, S.; Husain, S.; Jamil, B.; Sharifpur, M.; Cheraghian, G. Effect of Phase Change Materials on Lithium-Ion Plate Batteries. Batteries 2023, 9, 60. https://doi.org/10.3390/batteries9010060
Mustafa J, Alqaed S, Husain S, Jamil B, Sharifpur M, Cheraghian G. Effect of Phase Change Materials on Lithium-Ion Plate Batteries. Batteries. 2023; 9(1):60. https://doi.org/10.3390/batteries9010060
Chicago/Turabian StyleMustafa, Jawed, Saeed Alqaed, Shahid Husain, Basharat Jamil, Mohsen Sharifpur, and Goshtasp Cheraghian. 2023. "Effect of Phase Change Materials on Lithium-Ion Plate Batteries" Batteries 9, no. 1: 60. https://doi.org/10.3390/batteries9010060
APA StyleMustafa, J., Alqaed, S., Husain, S., Jamil, B., Sharifpur, M., & Cheraghian, G. (2023). Effect of Phase Change Materials on Lithium-Ion Plate Batteries. Batteries, 9(1), 60. https://doi.org/10.3390/batteries9010060










