Intrinsic Defects, Diffusion and Dopants in AVSi2O6 (A = Li and Na) Electrode Materials
Abstract
1. Introduction
2. Computational Methods
3. Results and Discussion
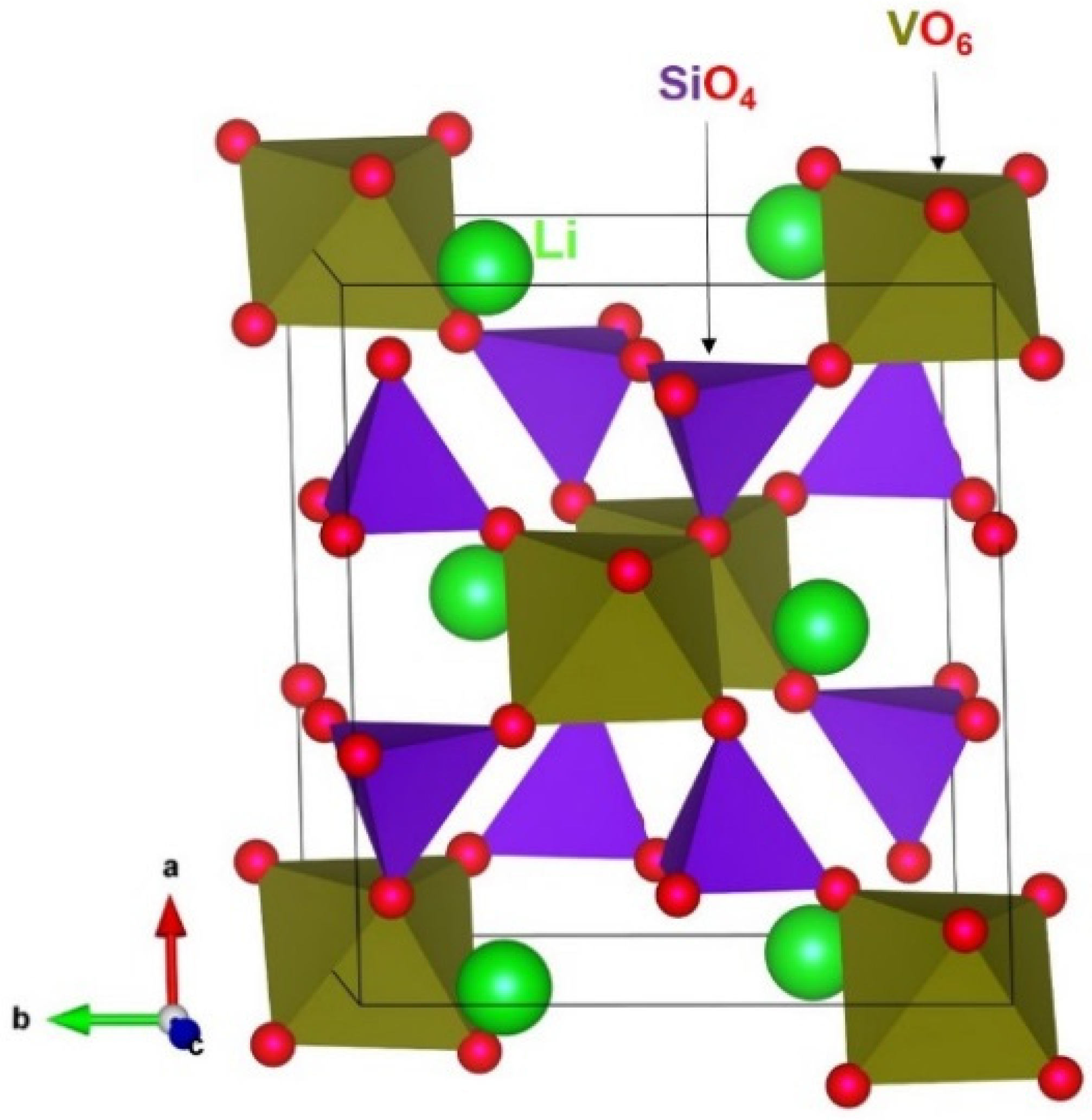
3.1. Crystal Structures of LiVSi2O6 and NaVSi2O6
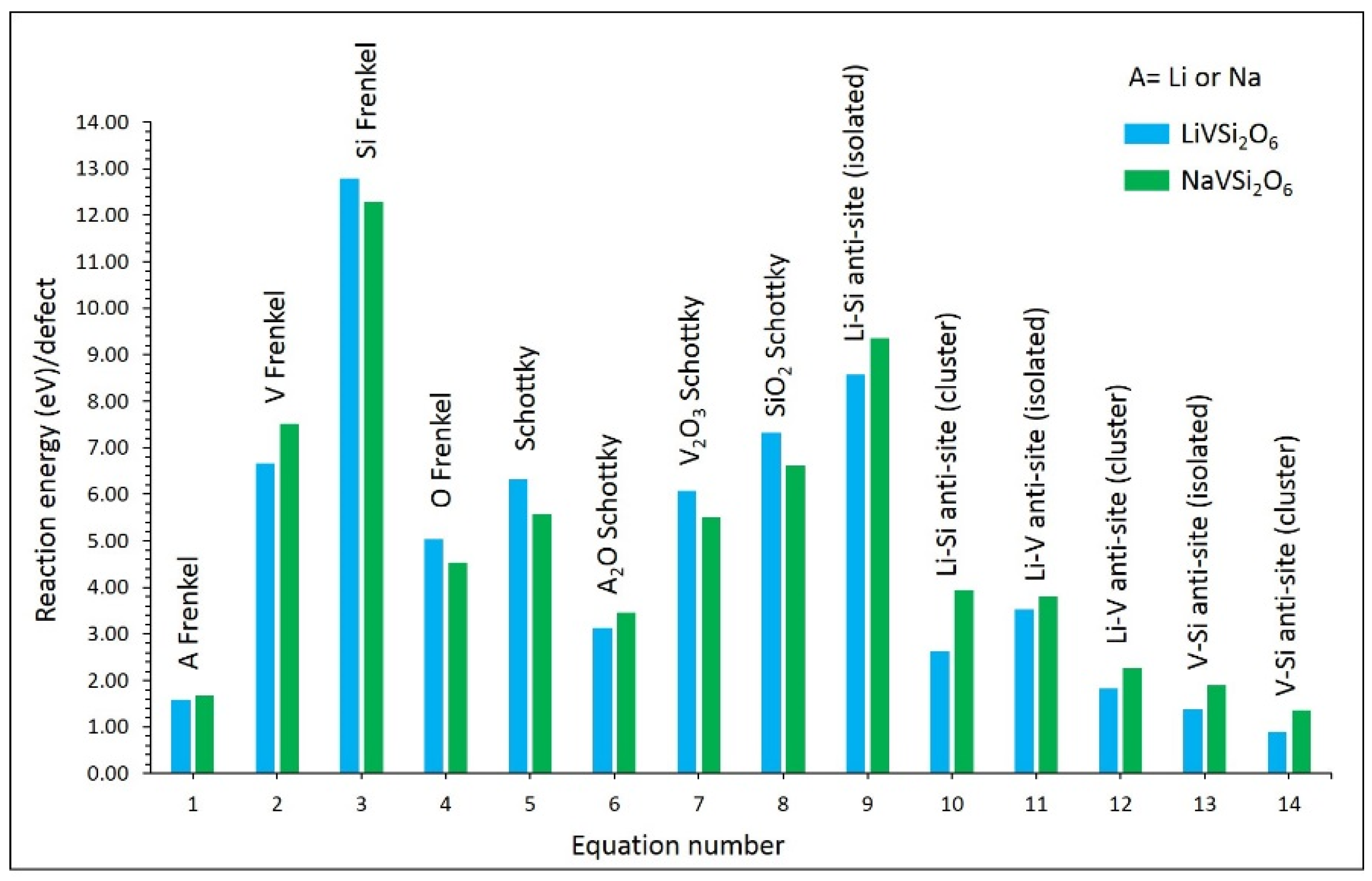
3.2. Defect Properties
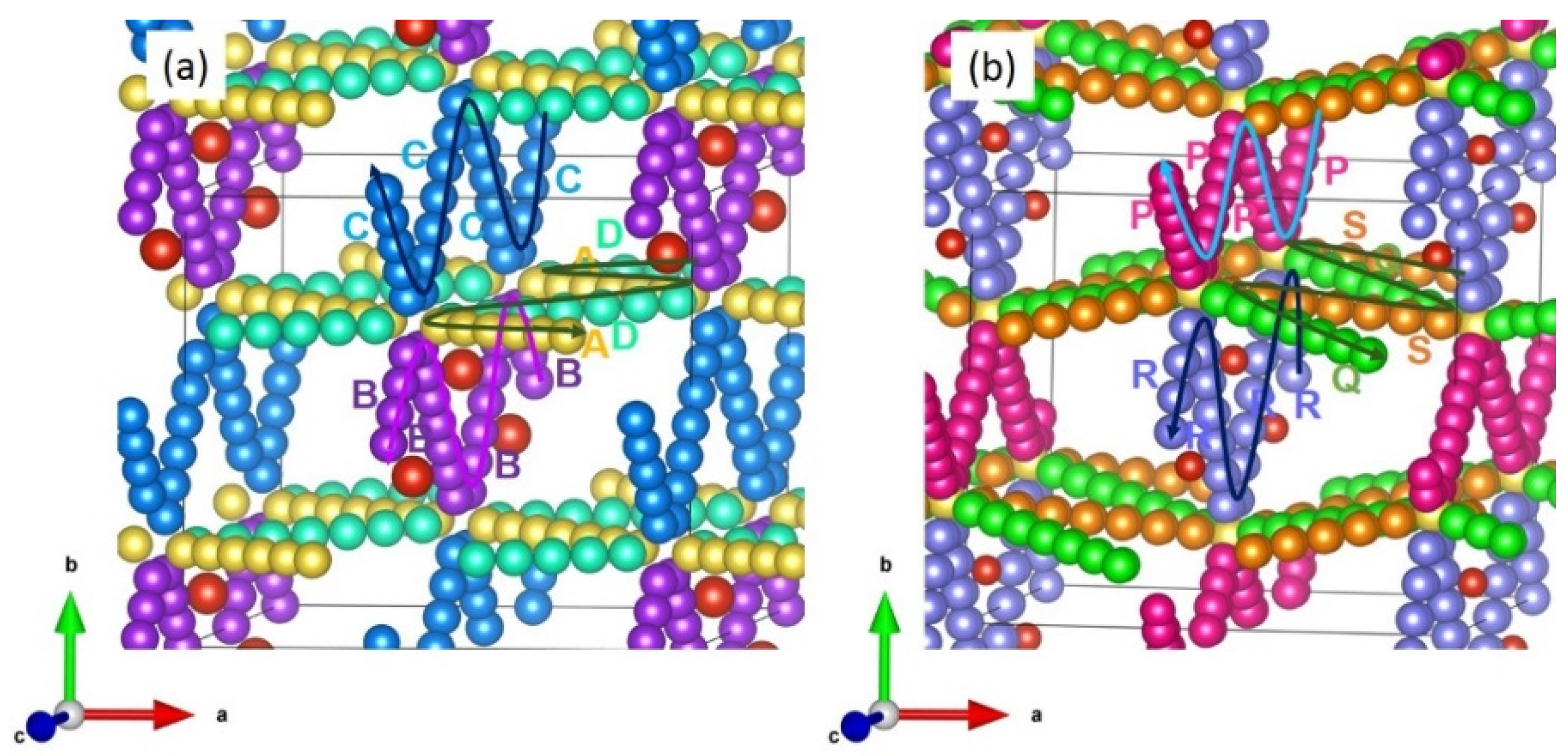
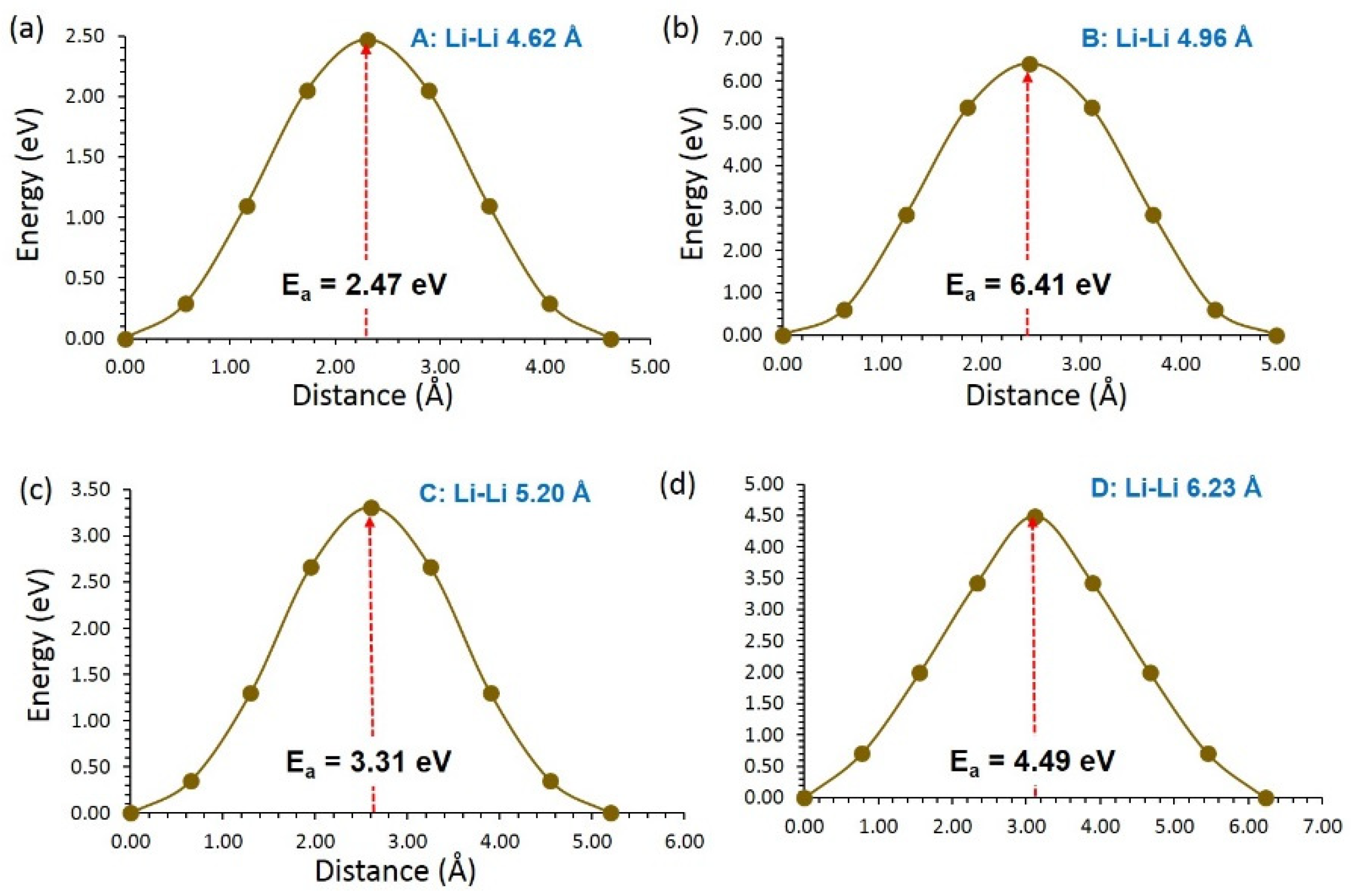
3.3. Diffusion of Li and Na Ions
3.4. Solution of Dopants
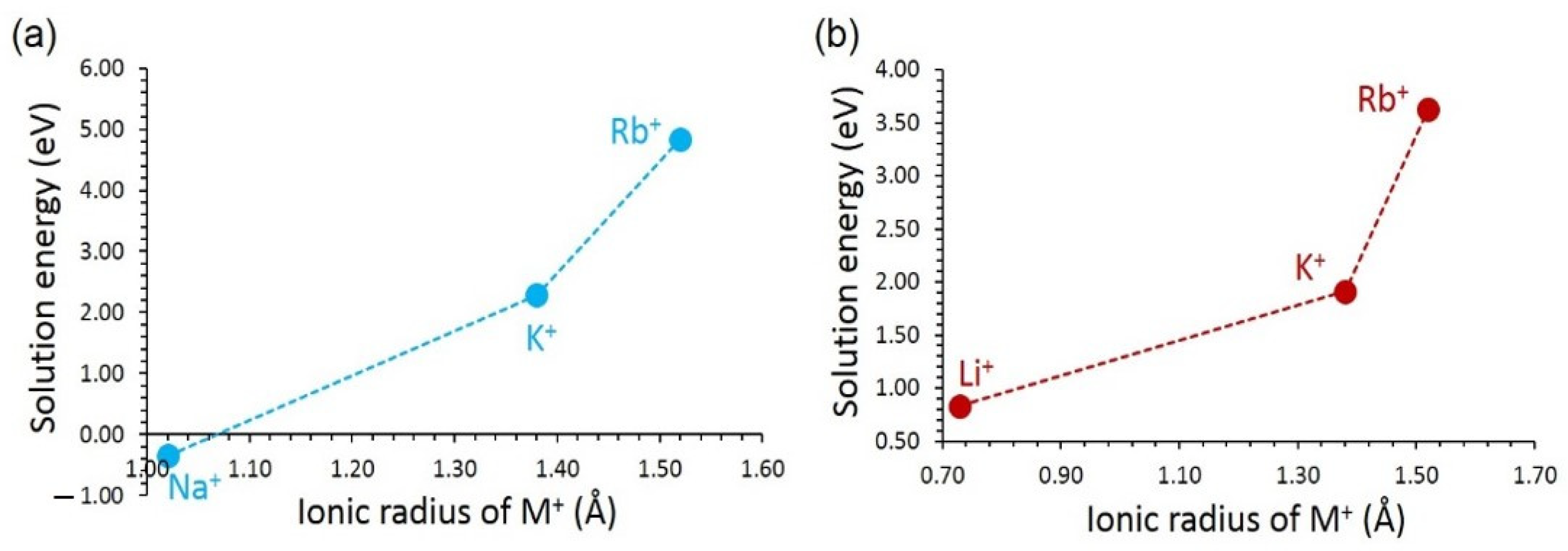
3.4.1. Monovalent Dopants
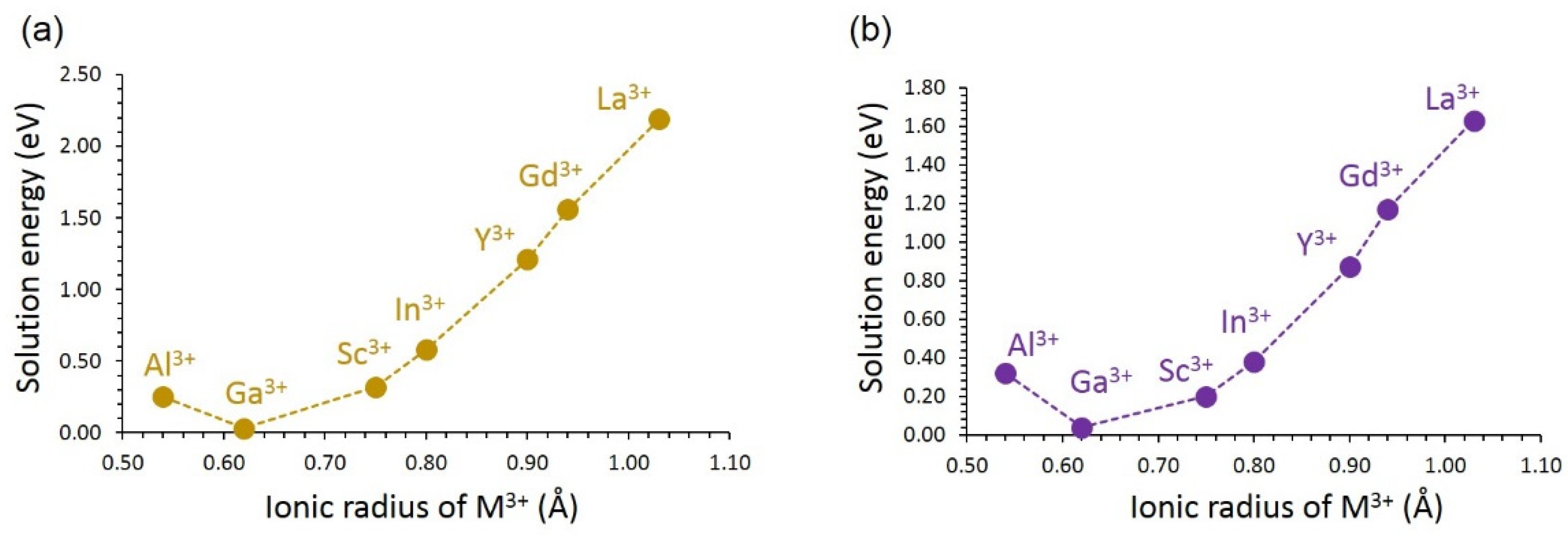
3.4.2. Trivalent Dopants
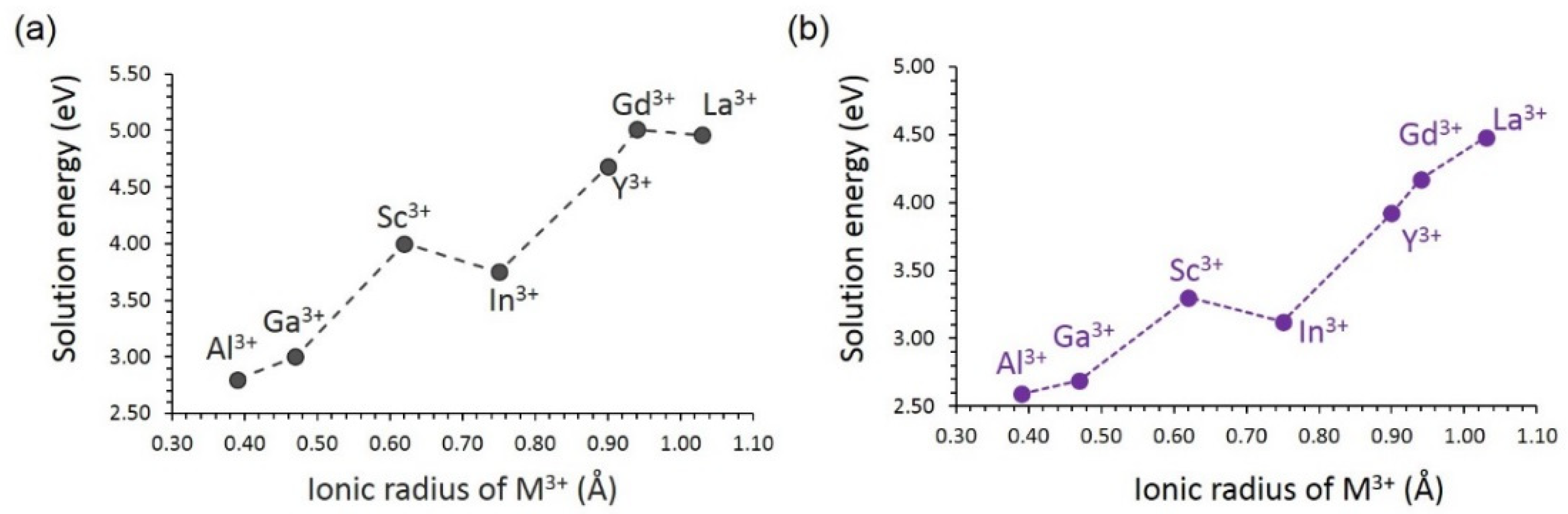
3.4.3. Tetravalent Dopants
4. Conclusions
Supplementary Materials
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- Liang, Y.; Zhao, C.-Z.; Yuan, H.; Chen, Y.; Zhang, W.; Huang, J.-Q.; Yu, D.; Liu, Y.; Titirici, M.M.; Chueh, Y.L.; et al. A review of rechargeable batteries for portable electronic devices. InfoMat 2019, 1, 6–32. [Google Scholar] [CrossRef]
- Goodenough, J.B.; Park, K.-S. The Li-Ion Rechargeable Battery: A Perspective. J. Am. Chem. Soc. 2013, 135, 1167–1176. [Google Scholar] [CrossRef] [PubMed]
- Kim, T.; Song, W.; Son, D.-Y.; Ono, L.K.; Qi, Y. Lithium-ion batteries: Outlook on present, future, and hybridized technologies. J. Mater. Chem. A 2019, 7, 2942–2964. [Google Scholar] [CrossRef]
- Chen, W.; Liang, J.; Yang, Z.; Li, G. A Review of Lithium-Ion Battery for Electric Vehicle Applications and Beyond. Energy Procedia 2019, 158, 4363–4368. [Google Scholar] [CrossRef]
- Karabelli, D.; Singh, S.; Kiemel, S.; Koller, J.; Konarov, A.; Stubhan, F.; Miehe, R.; Weeber, M.; Bakenov, Z.; Birke, K.P. Sodium-Based Batteries: In Search of the Best Compromise Between Sustainability and Maximization of Electric Performance. Front. Energy Res. 2020, 8, 349. [Google Scholar] [CrossRef]
- Islam, M.S.; Fisher, C.A.J. Lithium and sodium battery cathode materials: Computational insights into voltage, diffusion and nanostructural properties. Chem. Soc. Rev. 2014, 43, 185–204. [Google Scholar] [CrossRef]
- Bao, L.; Gao, W.; Su, Y.; Wang, Z.; Li, N.; Chen, S.; Wu, F. Progression of the silicate cathode materials used in lithium ion batteries. Chin. Sci. Bull. 2013, 58, 575–584. [Google Scholar] [CrossRef]
- Ling, J.; Karuppiah, C.; Krishnan, S.G.; Reddy, M.V.; Misnon, I.I.; Ab Rahim, M.H.; Yang, C.C.; Jose, R. Phosphate Polyanion Materials as High-Voltage Lithium-Ion Battery Cathode: A Review. Energy Fuels 2021, 35, 10428–10450. [Google Scholar] [CrossRef]
- Niu, Y.; Zhang, Y.; Xu, M. A review on pyrophosphate framework cathode materials for sodium-ion batteries. J. Mater. Chem. A 2019, 7, 15006–15025. [Google Scholar] [CrossRef]
- Liu, C.; Wang, Y.; Sun, J.; Chen, A. A Review on Applications of Layered Phosphorus in Energy Storage. Trans. Tianjin Univ. 2020, 26, 104–126. [Google Scholar] [CrossRef]
- Yang, S.-H.; Xue, H.; Guo, S.-P. Borates as promising electrode materials for rechargeable batteries. Coord. Chem. Rev. 2021, 427, 213551. [Google Scholar] [CrossRef]
- Roy, B.; Cherepanov, P.; Nguyen, C.; Forsyth, C.; Pal, U.; Mendes, T.C.; Howlett, P.; Forsyth, M.; MacFarlane, D.; Kar, M. Lithium Borate Ester Salts for Electrolyte Application in Next-Generation High Voltage Lithium Batteries. Adv. Energy Mater. 2021, 11, 2101422. [Google Scholar] [CrossRef]
- Zhang, Q.; Ji, S.; Yan, C.; Wang, X. Insights into the porosity and electrochemical performance of nano Li2FeSiO4 and Li2FeSiO4/C composite cathode materials. Mater. Technol. 2021, 1–10. [Google Scholar] [CrossRef]
- Li, L.; Han, E.; Pei, X.; Fu, C.; Zhang, M. The research on the electrochemical performance of Li2FeSiO4/mgx and Li2FeSiO4/cux. Inorg. Nano-Met. Chem. 2021, 51, 1536–1545. [Google Scholar] [CrossRef]
- Fisher, C.A.J.; Hart Prieto, V.M.; Islam, M.S. Lithium Battery Materials LiMPO4 (M = Mn, Fe, Co, and Ni): Insights into Defect Association, Transport Mechanisms, and Doping Behavior. Chem. Mater. 2008, 20, 5907–5915. [Google Scholar] [CrossRef]
- Clark, J.M.; Barpanda, P.; Yamada, A.; Islam, M.S. Sodium-ion battery cathodes Na2FeP2O7 and Na2MnP2O7: Diffusion behaviour for high rate performance. J. Mater. Chem. A 2014, 2, 11807–11812. [Google Scholar] [CrossRef]
- Kalantarian, M.M.; Hafizi-Barjini, M.; Momeni, M. Ab Initio Study of AMBO3 (A = Li, Na and M = Mn, Fe, Co, Ni) as Cathode Materials for Li-Ion and Na-Ion Batteries. ACS Omega 2020, 5, 8952–8961. [Google Scholar] [CrossRef]
- Nytén, A.; Kamali, S.; Häggström, L.; Gustafsson, T.; Thomas, J.O. The lithium extraction/insertion mechanism in Li2FeSiO4. J. Mater. Chem. 2006, 16, 2266–2272. [Google Scholar] [CrossRef]
- Yang, F.; Xia, Z.; Huang, S.; Zhang, X.; Song, Y.; Xiao, G.; Shao, G.; Liu, Y.; Deng, H.; Jiang, D.; et al. High field phase transition of cathode material Li2MnSiO4 for lithium-ion battery. Mater. Res. Express 2020, 7, 026104. [Google Scholar] [CrossRef]
- Eshetu, G.G.; Zhang, H.; Judez, X.; Adenusi, H.; Armand, M.; Passerini, S.; Figgemeier, E. Production of high-energy Li-ion batteries comprising silicon-containing anodes and insertion-type cathodes. Nat. Commun. 2021, 12, 5459. [Google Scholar] [CrossRef]
- Nishimura, S.-I.; Hayase, S.; Kanno, R.; Yashima, M.; Nakayama, N.; Yamada, A. Structure of Li2FeSiO4. J. Am. Chem. Soc. 2008, 130, 13212–13213. [Google Scholar] [CrossRef] [PubMed]
- Politaev, V.V.; Petrenko, A.A.; Nalbandyan, V.B.; Medvedev, B.S.; Shvetsova, E.S. Crystal structure, phase relations and electrochemical properties of monoclinic Li2MnSiO4. J. Solid State Chem. 2007, 180, 1045–1050. [Google Scholar] [CrossRef]
- Zhang, P.; Xu, Y.; Zheng, F.; Wu, S.Q.; Yang, Y.; Zhu, Z.-Z. Ion diffusion mechanism in Pn NaxLi2-xMnSiO4. CrystEngComm 2015, 17, 2123–2128. [Google Scholar] [CrossRef]
- Li, L.; Han, E.; Qiao, S.; Liu, H.; Shi, Y.; Yuan, W. Synthesis characterization and improved electrochemical performance of Li2FeSiO4/C as cathode for lithium-ion battery by metal doping. Prog. Nat. Sci. Mater. Int. 2019, 29, 111–118. [Google Scholar] [CrossRef]
- Kumar, A.; Jayakumar, O.D.; Bashiri, P.; Nazri, G.A.; Naik, V.M.; Naik, R. Mg doped Li2FeSiO4/C nanocomposites synthesized by the solvothermal method for lithium ion batteries. Dalton Trans. 2017, 46, 12908–12915. [Google Scholar] [CrossRef] [PubMed]
- Li, L.; Jia, T.; Liu, C.; Han, E.; Yang, Y.; Qin, Y.; Zhang, M. The modification of Li2FeSiO4 materials by dual doping with Ag and PO43− or BO33−. Ionics 2021, 27, 1887–1898. [Google Scholar] [CrossRef]
- Kim, C.; Yoo, G.W.; Son, J.T. Barium Doped Li2FeSiO4 Cathode Material for Li-Ion Secondary Batteries. J. Nanosci. Nanotechnol. 2015, 15, 8808–8812. [Google Scholar] [CrossRef]
- Li, S.-D.; Gao, K. Insights into manganese and nickel co-doped Li2FeSiO4 cathodes for lithium-ion battery. Ionics 2021, 27, 2345–2352. [Google Scholar] [CrossRef]
- Kuganathan, N.; Chroneos, A. Defects, Dopants and Sodium Mobility in Na2MnSiO4. Sci. Rep. 2018, 8, 14669. [Google Scholar] [CrossRef]
- Kuganathan, N.; Islam, M.S. Li2MnSiO4 Lithium Battery Material: Atomic-Scale Study of Defects, Lithium Mobility, and Trivalent Dopants. Chem. Mater. 2009, 21, 5196–5202. [Google Scholar] [CrossRef]
- Zhu, L.; Zeng, Y.-R.; Wen, J.; Li, L.; Cheng, T.-M. Structural and electrochemical properties of Na2FeSiO4 polymorphs for sodium-ion batteries. Electrochim. Acta 2018, 292, 190–198. [Google Scholar] [CrossRef]
- Law, M.; Ramar, V.; Balaya, P. Na2MnSiO4 as an attractive high capacity cathode material for sodium-ion battery. J. Power Sources 2017, 359, 277–284. [Google Scholar] [CrossRef]
- Hou, P.; Feng, J.; Wang, Y.; Wang, L.; Li, S.; Yang, L.; Luo, S.H. Study on the properties of Li2MnSiO4 as cathode material for lithium-ion batteries by sol-gel method. Ionics 2020, 26, 1611–1616. [Google Scholar] [CrossRef]
- Ni, J.; Kawabe, Y.; Morishita, M.; Watada, M.; Takeichi, N.; Sakai, T. Pyroxene LiVSi2O6 as an electrode material for Li-ion batteries. J. Power Sources 2010, 195, 8322–8326. [Google Scholar] [CrossRef]
- Hameed, A.S.; Reddy, M.V.; AlQaradawi, S.Y.; Adams, S. Synthesis, structural and lithium storage studies of graphene-LiVSi2O6 composites. Ionics 2019, 25, 1559–1566. [Google Scholar] [CrossRef]
- Wei, Y.; Zhang, Y.; Huang, Y.; Wang, X.; Cheng, W.; Sun, Y.; Jia, D.; Tang, X. Simple synthesis and electrochemical performance of NaVSi2O6 as a new sodium-ion cathode material. Int. J. Energy Res. 2021, 45, 10746–10751. [Google Scholar] [CrossRef]
- Wong, L.L.; Chen, H.; Adams, S. Design of fast ion conducting cathode materials for grid-scale sodium-ion batteries. Phys. Chem. Chem. Phys. 2017, 19, 7506–7523. [Google Scholar] [CrossRef]
- Yeandel, S.R.; Chapman, B.J.; Slater, P.R.; Goddard, P. Structure and Lithium-Ion Dynamics in Fluoride-Doped Cubic Li7La3Zr2O12 (LLZO) Garnet for Li Solid-State Battery Applications. J. Phys. Chem. C 2018, 122, 27811–27819. [Google Scholar] [CrossRef]
- Byrne, E.H.; Raiteri, P.; Gale, J.D. Computational Insight into Calcium–Sulfate Ion Pair Formation. J. Phys. Chem. C 2017, 121, 25956–25966. [Google Scholar] [CrossRef]
- Taylor, F.H.; Buckeridge, J.; Catlow, C.R.A. Defects and Oxide Ion Migration in the Solid Oxide Fuel Cell Cathode Material LaFeO3. Chem. Mater. 2016, 28, 8210–8220. [Google Scholar] [CrossRef]
- Treacher, J.C.; Wood, S.M.; Islam, M.S.; Kendrick, E. Na2CoSiO4 as a cathode material for sodium-ion batteries: Structure, electrochemistry and diffusion pathways. Phys. Chem. Chem. Phys. 2016, 18, 32744–32752. [Google Scholar] [CrossRef] [PubMed]
- Tripathi, R.; Wood, S.M.; Islam, M.S.; Nazar, L.F. Na-ion mobility in layered Na2FePO4F and olivine Na[Fe,Mn]PO4. Energy Environ. Sci. 2013, 6, 2257–2264. [Google Scholar] [CrossRef]
- Panchmatia, P.M.; Orera, A.; Kendrick, E.; Hanna, J.V.; Smith, M.E.; Slater, P.R.; Islam, M.S. Protonic defects and water incorporation in Si and Ge-based apatite ionic conductors. J. Mater. Chem. 2010, 20, 2766–2772. [Google Scholar] [CrossRef][Green Version]
- Gale, J.D.; Rohl, A.L. The General Utility Lattice Program (GULP). Mol. Simul. 2003, 29, 291–341. [Google Scholar] [CrossRef]
- Gale, J.D. GULP: A computer program for the symmetry-adapted simulation of solids. J. Chem. Soc. Faraday Trans. 1997, 93, 629–637. [Google Scholar] [CrossRef]
- Mott, N.F.; Littleton, M.J. Conduction in polar crystals. I. Electrolytic conduction in solid salts. Trans. Faraday Soc. 1938, 34, 485–499. [Google Scholar] [CrossRef]
- Woodley, S.M.; Catlow, C.R.A.; Piszora, P.; Stempin, K.; Wolska, E. Computer Modeling Study of the Lithium Ion Distribution in Quaternary Li–Mn–Fe–O Spinels. J. Solid State Chem. 2000, 153, 310–316. [Google Scholar] [CrossRef]
- Kuganathan, N.; Chroneos, A. Defects and dopant properties of Li3V2(PO4)3. Sci. Rep. 2019, 9, 333. [Google Scholar] [CrossRef]
- Minervini, L.; Grimes, R.W.; Sickafus, K.E. Disorder in Pyrochlore Oxides. J. Am. Ceram. Soc. 2000, 83, 1873–1878. [Google Scholar] [CrossRef]
- Redhammer, G.J.; Roth, G. Structural variation and crystal chemistry of LiMe3+Si2O6 clinopyroxenes Me3+ = Al, Ga, Cr, V.; Fe, Sc and In. Z. Für Krist.—Cryst. Mater. 2004, 219, 278–294. [Google Scholar] [CrossRef]
- Ohashi, H.; Osawa, T.; Sato, A. NaVSi2O6. Acta Crystallogr. Sect. C 1994, 50, 1652–1655. [Google Scholar] [CrossRef]
- Momma, K.; Izumi, F. VESTA: A three-dimensional visualization system for electronic and structural analysis. J. Appl. Crystallogr. 2008, 41, 653–658. [Google Scholar] [CrossRef]
- Kröger, F.A.; Vink, H.J. Relations between the Concentrations of Imperfections in Crystalline Solids. In Solid State Physics; Seitz, F., Turnbull, D., Eds.; Academic Press: Cambridge, MA, USA, 1956; Volume 3, pp. 307–435. [Google Scholar]
- Kempaiah Devaraju, M.; Duc Truong, Q.; Hyodo, H.; Sasaki, Y.; Honma, I. Synthesis, characterization and observation of antisite defects in LiNiPO4 nanomaterials. Sci. Rep. 2015, 5, 11041. [Google Scholar] [CrossRef] [PubMed]
- Liu, H.; Choe, M.J.; Enrique, R.A.; Orvañanos, B.; Zhou, L.; Liu, T.; Thornton, K.; Grey, C.P. Effects of Antisite Defects on Li Diffusion in LiFePO4 Revealed by Li Isotope Exchange. J. Phys. Chem. C 2017, 121, 12025–12036. [Google Scholar] [CrossRef]
- Gardiner, G.R.; Islam, M.S. Anti-Site Defects and Ion Migration in the LiFe0.5Mn0.5PO4 Mixed-Metal Cathode Material. Chem. Mater. 2010, 22, 1242–1248. [Google Scholar] [CrossRef]
- Islam, M.S.; Driscoll, D.J.; Fisher, C.A.J.; Slater, P.R. Atomic-Scale Investigation of Defects, Dopants, and Lithium Transport in the LiFePO4 Olivine-Type Battery Material. Chem. Mater. 2005, 17, 5085–5092. [Google Scholar] [CrossRef]
- Heath, J.; Chen, H.; Islam, M.S. MgFeSiO4 as a potential cathode material for magnesium batteries: Ion diffusion rates and voltage trends. J. Mater. Chem. A 2017, 5, 13161–13167. [Google Scholar] [CrossRef]
- Stokes, S.J.; Islam, M.S. Defect chemistry and proton-dopant association in BaZrO3 and BaPrO3. J. Mater. Chem. 2010, 20, 6258–6264. [Google Scholar] [CrossRef]
- Shannon, R. Revised effective ionic radii and systematic studies of interatomic distances in halides and chalcogenides. Acta Crystallogr. Sect. A 1976, 32, 751–767. [Google Scholar] [CrossRef]



| Interaction | A/eV | ρ/Å | C/eV·Å6 | Y/e | K/eV·Å−2 |
|---|---|---|---|---|---|
| LiVSi2O6 | |||||
| Li+–O2− | 479.837 | 0.3000 | 0.00 | 1.00 | 99,999 |
| V3+–O2− | 1410.82 | 0.3117 | 0.00 | 2.04 | 196.3 |
| Si4+–O2− | 1283.91 | 0.32052 | 10.66 | 4.00 | 99,999 |
| O2−–O2− | 9547.96 | 0.2192 | 32.0 | −2.04 | 6.3 |
| NaVSi2O6 | |||||
| Na+–O2− | 1497.830598 | 0.287483 | 0.00 | 1.00 | 99,999 |
| V3+–O2− | 1410.82 | 0.3117 | 0.00 | 2.04 | 196.3 |
| Si4+–O2− | 1283.91 | 0.32052 | 10.66 | 4.00 | 99,999 |
| O2−–O2− | 22,764.0 | 0.1490 | 27.89 | −2.80 | 74.92 |
| Three body | |||||
| Bonds | K (eV. rad−2) | θ0 (o) | |||
| O2−–Si4+–O2− | 2.09724 | 109.5 | |||
| Parameter | Calculated | Experiment | ∆|(%) |
|---|---|---|---|
| LiVSi2O6 [50] | |||
| a (Å) | 9.543 | 9.657 | 1.19 |
| b (Å) | 8.608 | 8.623 | 0.17 |
| c (Å) | 5.399 | 5.287 | 2.11 |
| α = γ (°) | 90.0 | 90.0 | 0.00 |
| β (°) | 109.97 | 110.15 | 0.16 |
| V (Å3) | 416.80 | 413.31 | 0.84 |
| NaVSi2O6 [51] | |||
| a (Å) | 9.634 | 9.634 | 0.00 |
| b (Å) | 8.594 | 8.741 | 1.68 |
| c (Å) | 5.249 | 5.296 | 0.88 |
| α = γ (°) | 90.0 | 90.0 | 0.00 |
| β (°) | 104.89 | 106.91 | 1.89 |
| V (Å3) | 420.04 | 426.72 | 1.57 |
| LiVSi2O6 | ||
| Migration Hop | Separation (Å) | Activation Energy (eV) |
| A | 4.62 | 2.47 |
| B | 4.96 | 6.41 |
| C | 5.02 | 3.31 |
| D | 6.23 | 4.49 |
| NaVSi2O6 | ||
| P | 4.23 | 3.95 |
| Q | 4.89 | 2.88 |
| R | 5.88 | 11.78 |
| S | 6.12 | 7.97 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the author. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Kuganathan, N. Intrinsic Defects, Diffusion and Dopants in AVSi2O6 (A = Li and Na) Electrode Materials. Batteries 2022, 8, 20. https://doi.org/10.3390/batteries8030020
Kuganathan N. Intrinsic Defects, Diffusion and Dopants in AVSi2O6 (A = Li and Na) Electrode Materials. Batteries. 2022; 8(3):20. https://doi.org/10.3390/batteries8030020
Chicago/Turabian StyleKuganathan, Navaratnarajah. 2022. "Intrinsic Defects, Diffusion and Dopants in AVSi2O6 (A = Li and Na) Electrode Materials" Batteries 8, no. 3: 20. https://doi.org/10.3390/batteries8030020
APA StyleKuganathan, N. (2022). Intrinsic Defects, Diffusion and Dopants in AVSi2O6 (A = Li and Na) Electrode Materials. Batteries, 8(3), 20. https://doi.org/10.3390/batteries8030020







