Assessing the Feasibility of a Cold Start Procedure for Solid State Batteries in Automotive Applications
Abstract
:1. Introduction
2. Literature Review
2.1. Solid-State Batteries
2.2. Battery Heating Techniques
3. Methods
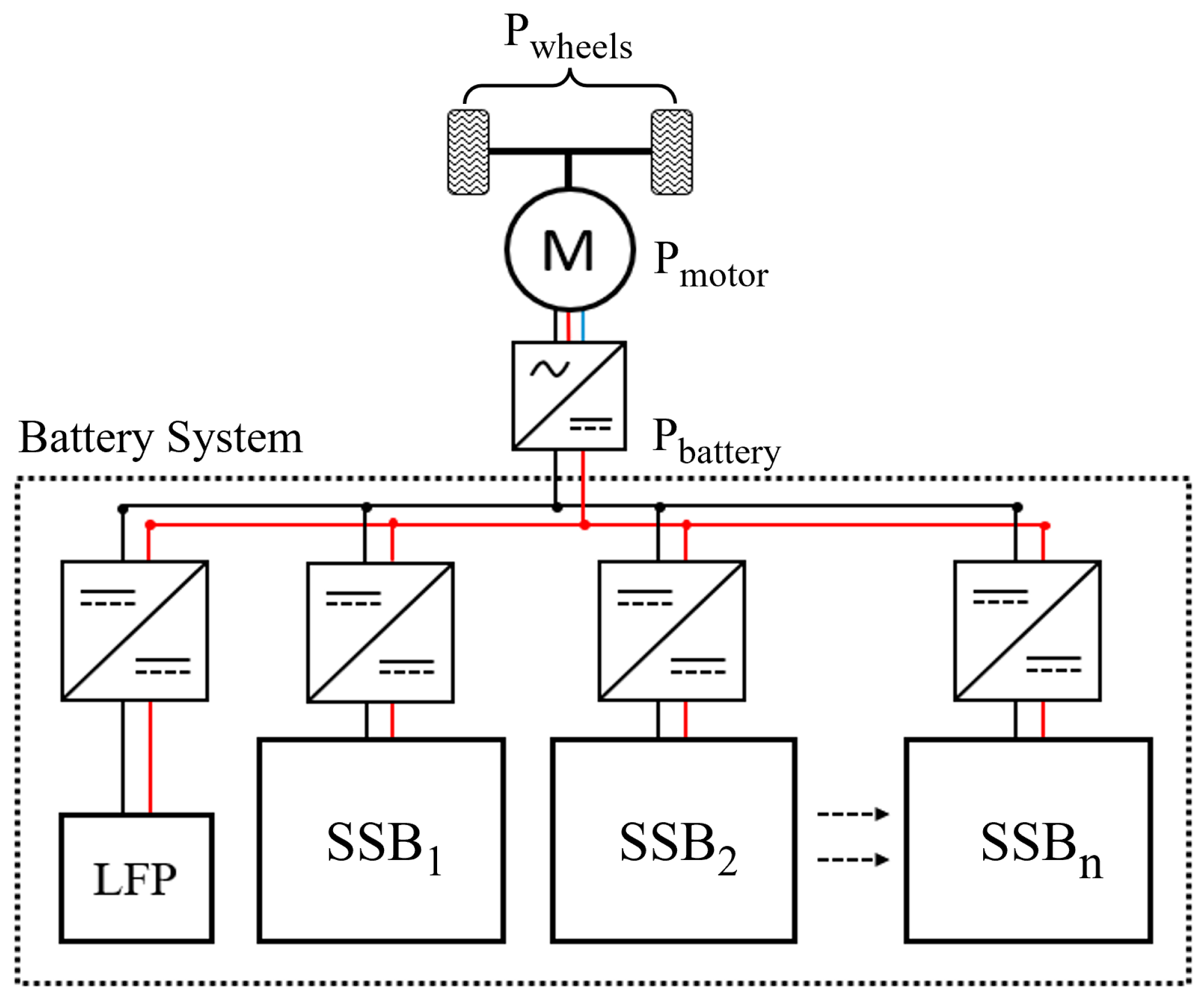
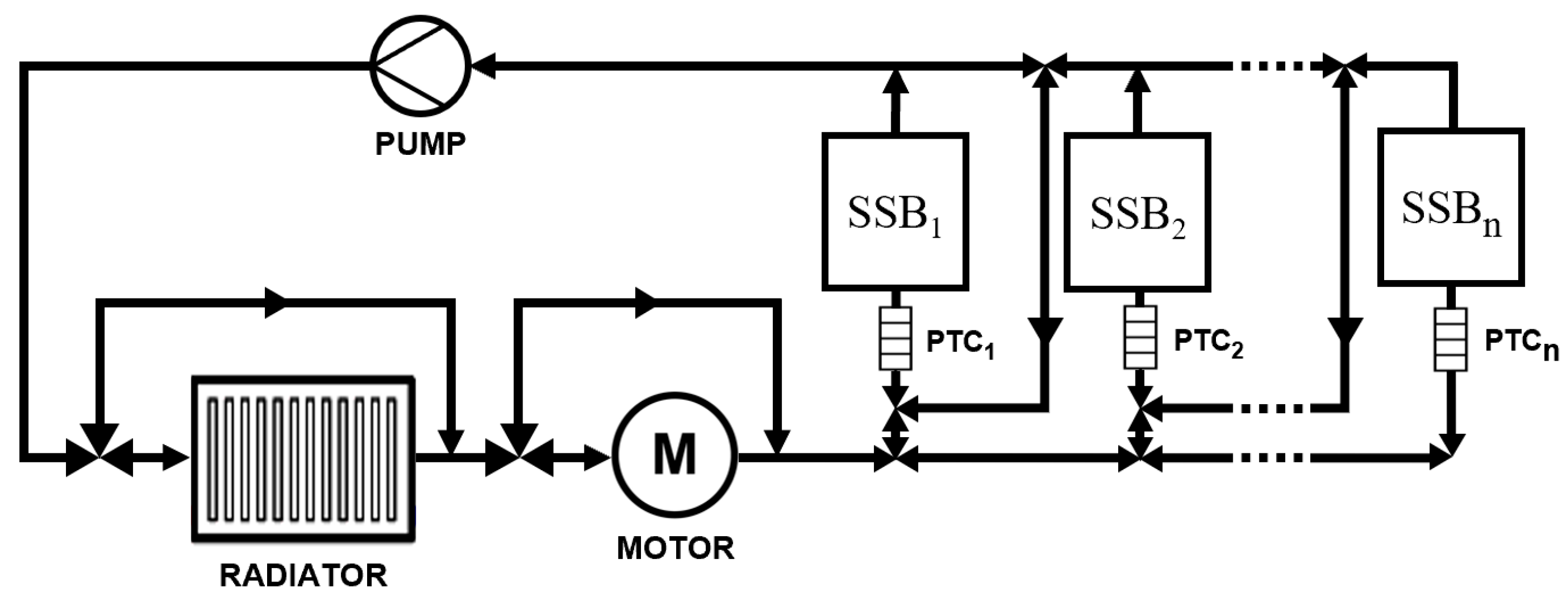
3.1. Powertrain Architecture
3.2. Model Architecture
3.3. Vehicle Model
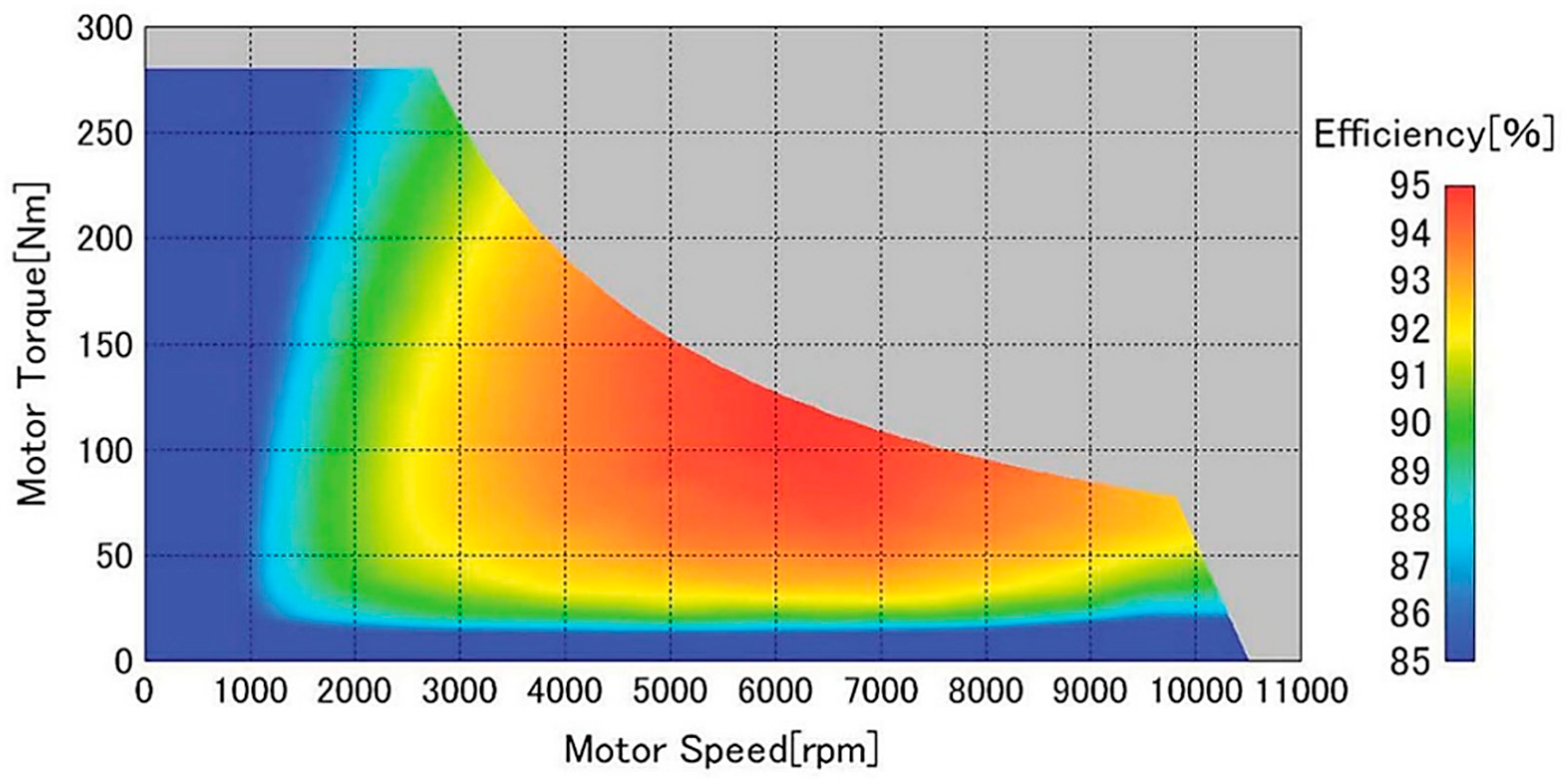
3.4. Motor Model
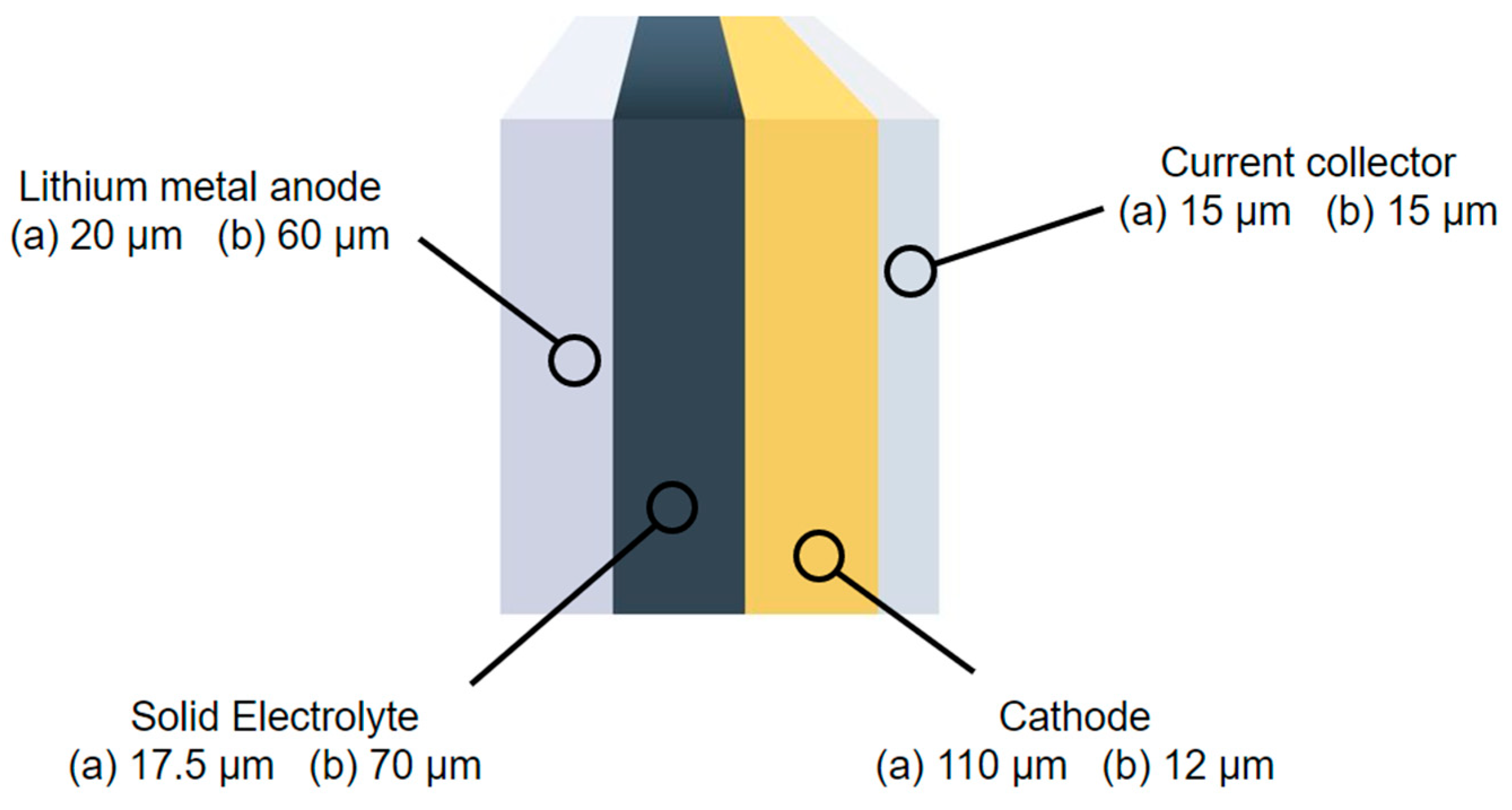
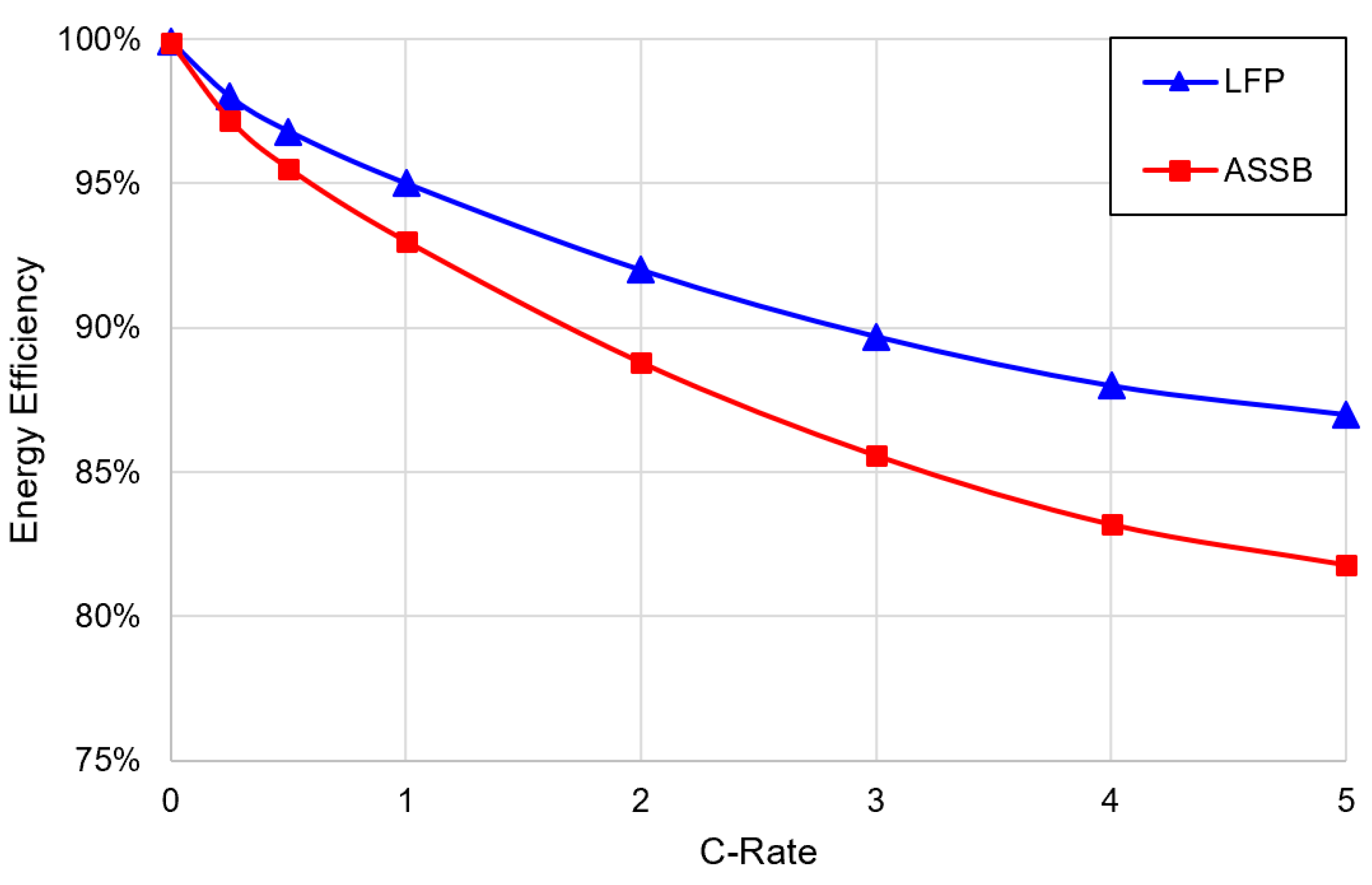
3.5. Battery Model
4. Results
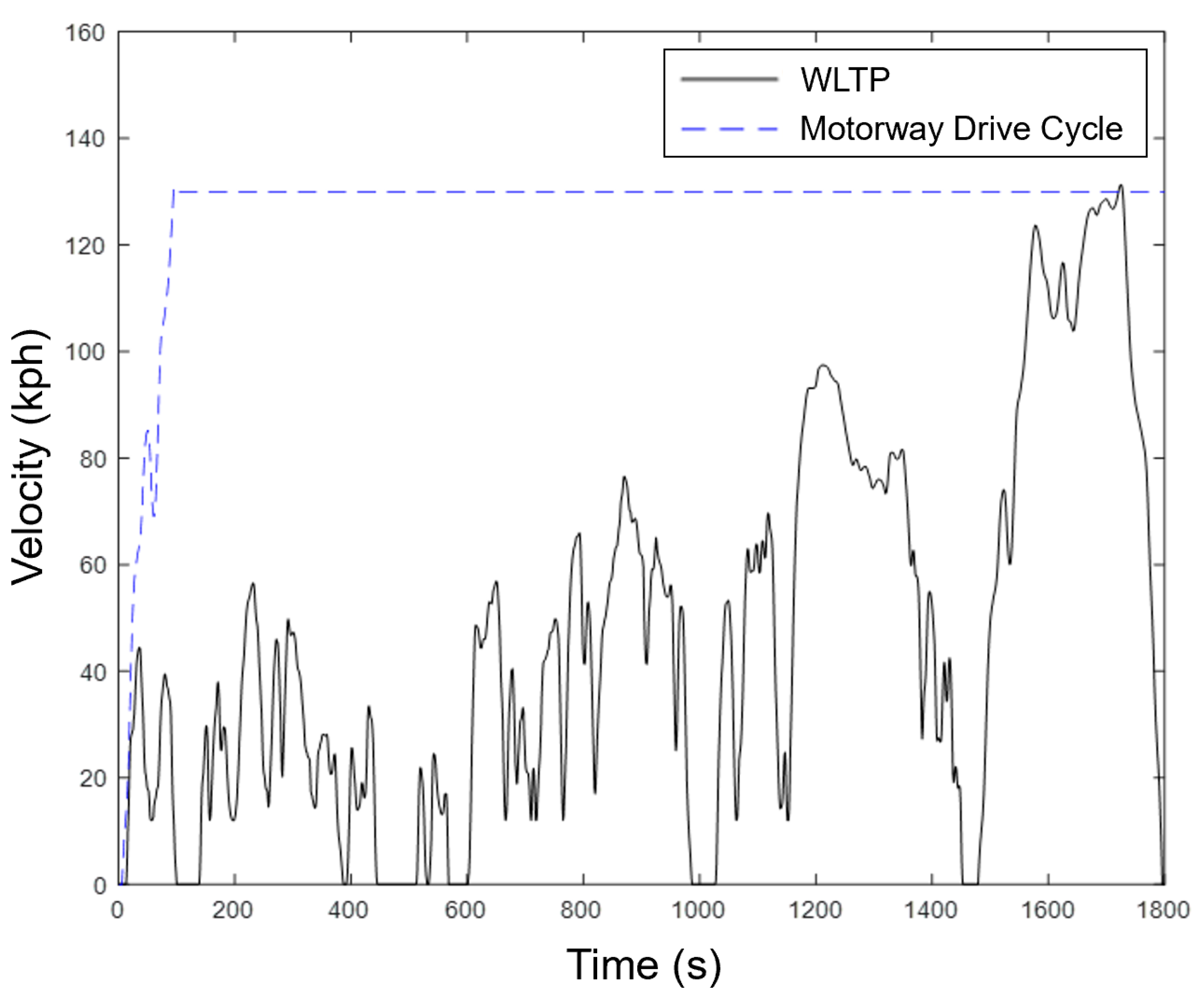
4.1. Drive Cycles
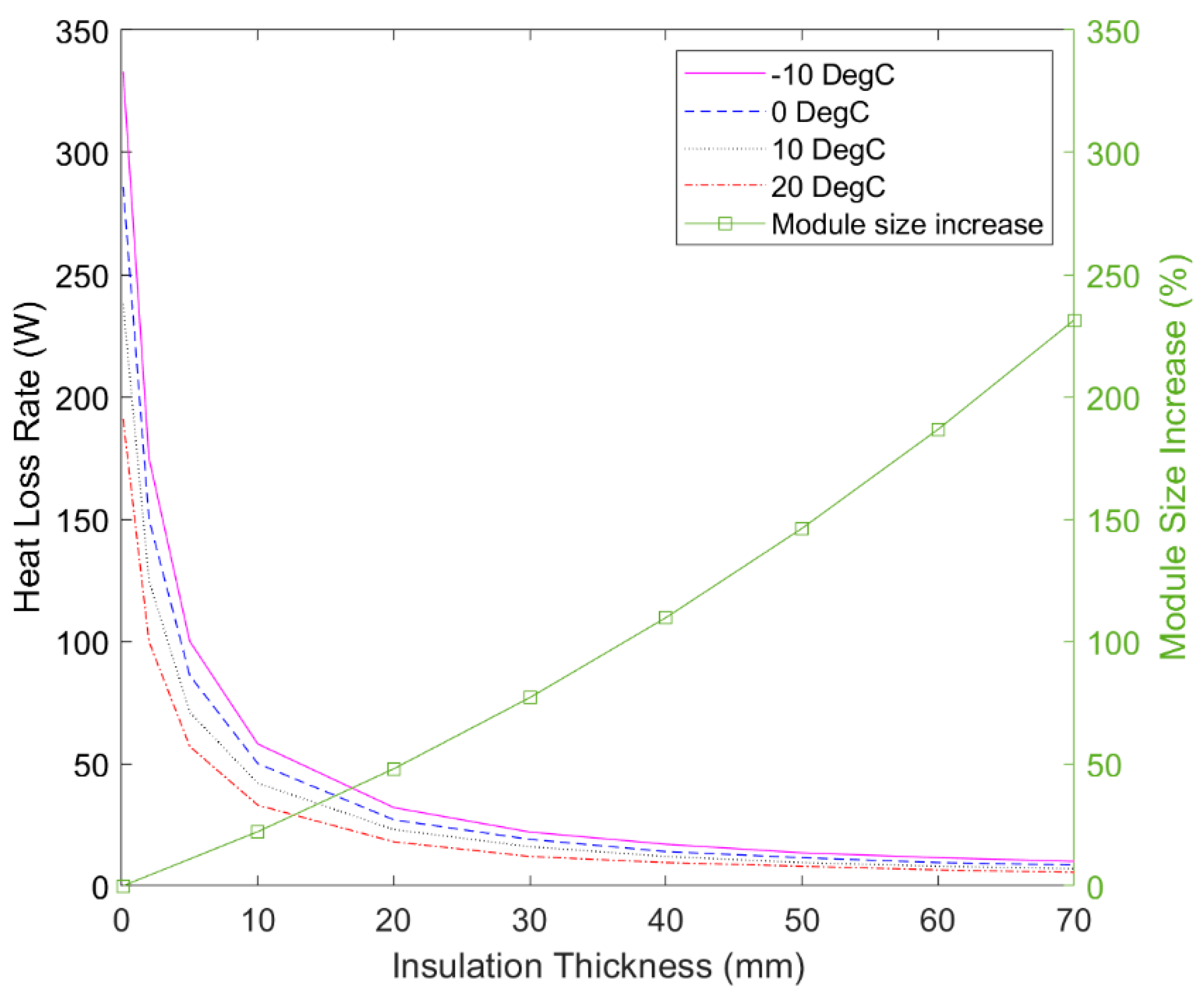
4.2. Sub-Pack Insulation
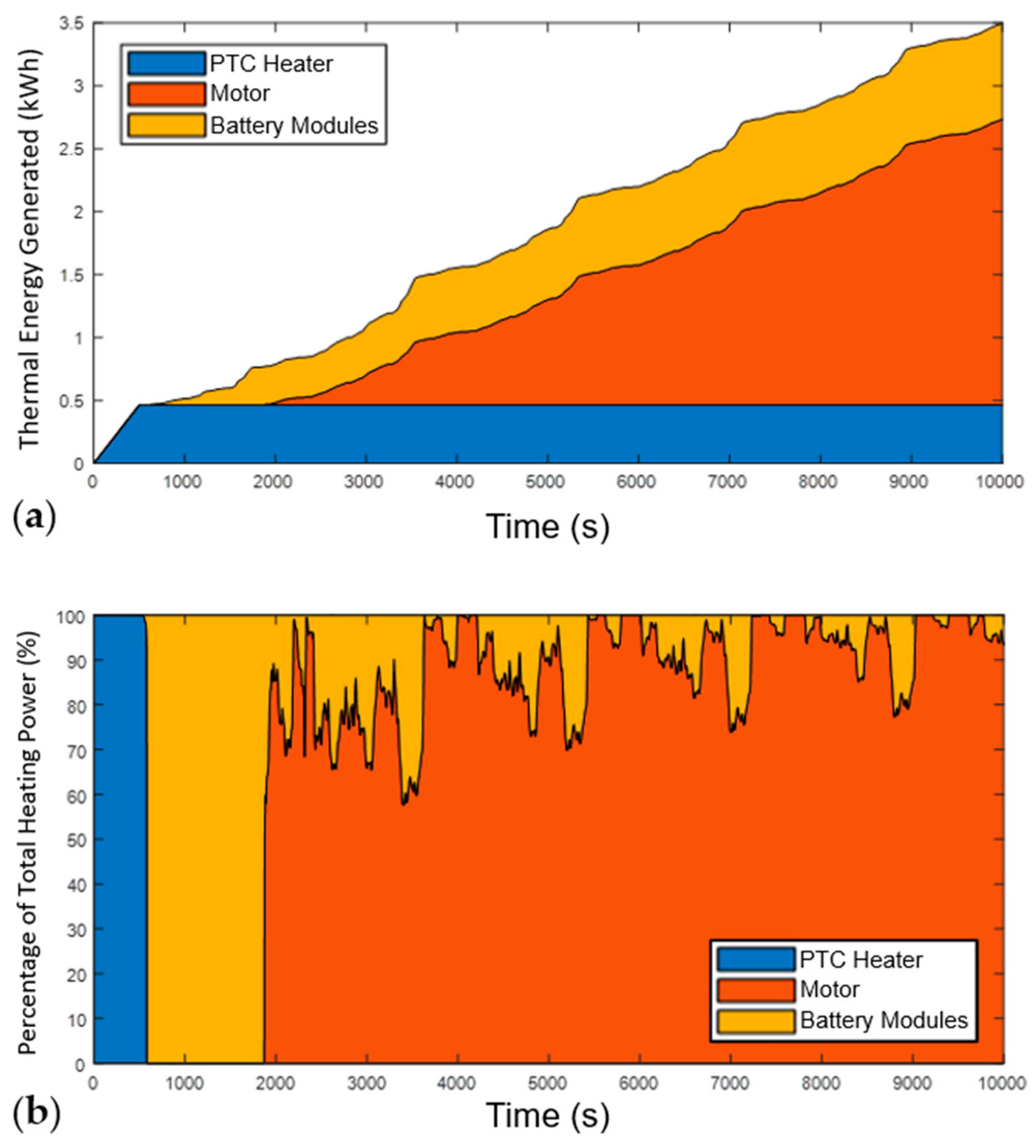
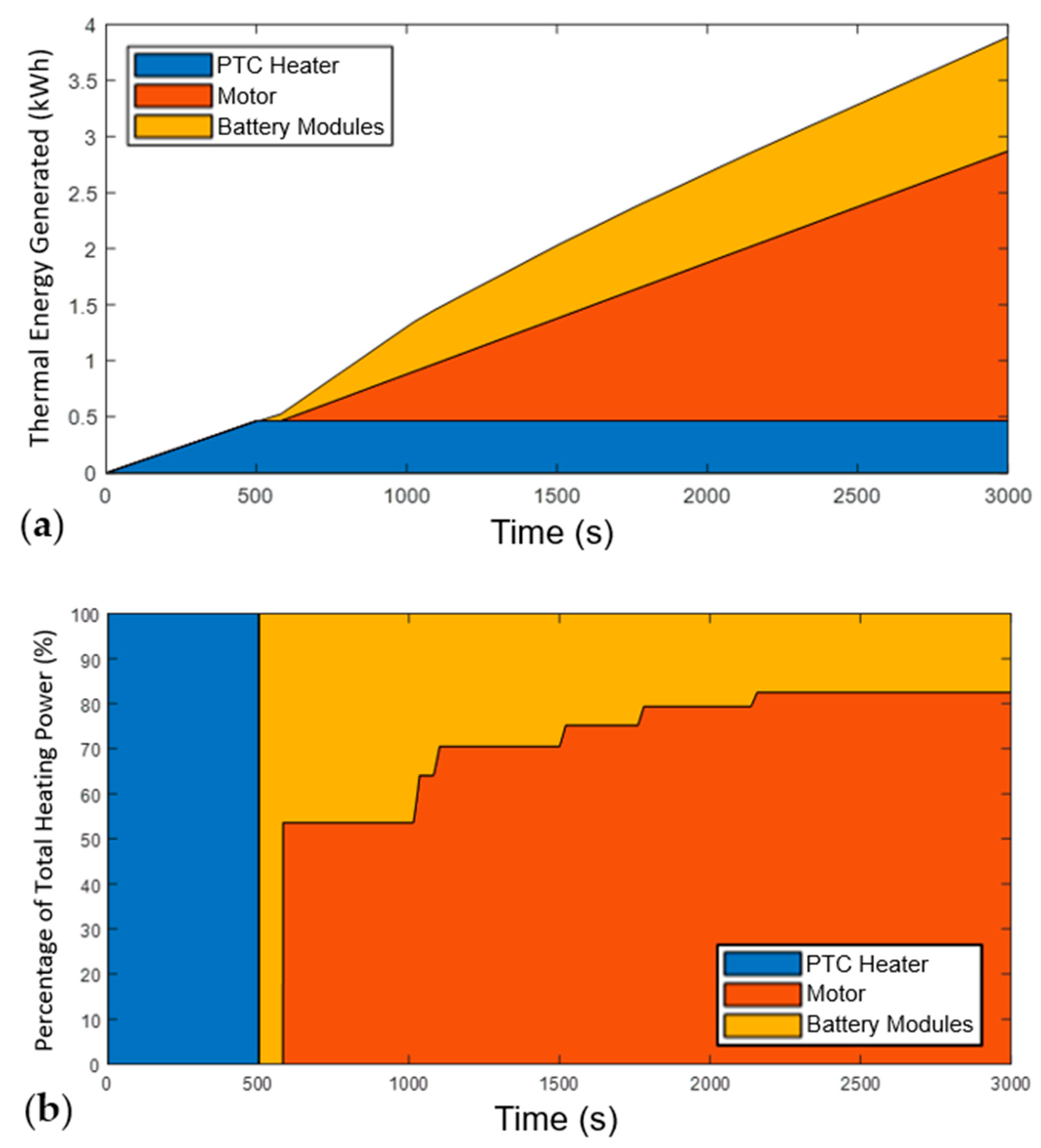
4.3. WLTP Cold Start Testing
4.4. Motorway Cold Start Testing
5. Discussion
5.1. System Considerations and Limitations
5.2. Applications of Findings
5.3. Future Work
6. Conclusions
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
References
- The Faraday Institution. Solid-State Batteries: The Technology of the 2030s but the Research Challenge of the 2020s; The Faraday Institution: Cambridge, UK, 2021. [Google Scholar]
- Chen, S.; Dai, F.; Cai, M. Opportunities and Challenges of High-Energy Lithium Metal Batteries for Electric Vehicle Applications. ACS Energy Lett. 2020, 5, 3140–3151. [Google Scholar] [CrossRef]
- United Nations. Paris Agreement; UN: Paris, France, 2015. [Google Scholar]
- HM Government Department for Transport. ‘The Road to Zero’ Next Steps towards Cleaner Road Transport and Delivering Our Industrial Strategy; Department for Transport: London, UK, 2018.
- California Governor’s Office of Business and Economic Development. California Zero-Emission Vehicle Market Development Strategy; GO-Biz: Sacramento, CA, USA, 2021.
- Zhang, T.; He, W.; Zhang, W.; Wang, T.; Li, P.; Sun, Z.; Yu, X. Designing composite solid-state electrolytes for high performance lithium ion or lithium metal batteries. Chem. Sci. 2020, 11, 8686–8707. [Google Scholar] [CrossRef]
- Janek, J.; Zeier, W. A solid future for battery development. Nat. Energy 2016, 1, 16141. [Google Scholar] [CrossRef]
- BlueSolutions. Lmp® (Lithium Metal Polymer) Technology, Unique All-Solid-State Battery; Bolloré Group: Puteaux, France, 2019. [Google Scholar]
- Citroen. 2016 Citroën E-Méhari—Owner’s Manual; Citroën: Paris, France, 2016. [Google Scholar]
- Pasta, M.; Armstrong, D.; Brown, Z.L.; Bu, J.; Castell, M.R.; Chen, P.; Cocks, A.; Corr, S.A.; Cussen, E.J.; Darnbrough, E.; et al. 2020 roadmap on solid-state batteries. J. Phys. Energy 2020, 2, 032008. [Google Scholar] [CrossRef]
- Zhang, D.; Xu, X.; Qin, Y.; Ji, S.; Huo, Y.; Wang, Z.; Liu, Z.; Shen, J.; Liu, J. Recent Progress in Organic–Inorganic Composite Solid Electrolytes for All-Solid-State 68 Lithium Batteries. Chem. A Eur. J. 2020, 26, 1720–1736. [Google Scholar] [CrossRef]
- Zhang, S.; Li, Z.; Guo, Y.; Cai, L.; Manikandan, P.; Zhao, K.; Li, Y.; Pol, V.G. Room-temperature, high-voltage solid-state lithium battery with composite solid polymer electrolyte with in-situ thermal safety study. Chem. Eng. J. 2020, 400, 125996. [Google Scholar] [CrossRef]
- Oh, J.; He, L.; Chua, B.; Zeng, K.; Lu, L. Inorganic sodium solid-state electrolyte and interface with sodium metal for room-temperature metal solid-state batteries. Energy Storage Mater. 2021, 34, 28–44. [Google Scholar] [CrossRef]
- Zhou, Q.; Ma, J.; Dong, S.; Li, X.; Cui, G. Intermolecular Chemistry in Solid Polymer Electrolytes for High-Energy-Density Lithium Batteries. Adv. Mater. 2019, 31, 1902029. [Google Scholar] [CrossRef]
- Zhu, Y.; Pande, V.; Li, L.; Wen, B.; Pan, M.S.; Wang, D.; Ma, Z.-F.; Viswanathan, V.; Chiang, Y.-M. Design principles for self-forming interfaces enabling stable lithium-metal anodes. Proc. Natl. Acad. Sci. USA 2020, 117, 27195–27203. [Google Scholar] [CrossRef]
- Khurana, R.; Schaefer, J.; Archer, L.; Coates, G. Suppression of Lithium Dendrite Growth Using Cross-Linked Polyethylene/Poly(ethylene oxide) Electrolytes: A New Approach for Practical Lithium-Metal Polymer Batteries. J. Am. Chem. Soc. 2014, 136, 7395–7402. [Google Scholar] [CrossRef]
- Li, S.; Wang, H.; Cuthbert, J.; Liu, T.; Whitacre, J.; Matyjaszewski, K. A Semiliquid Lithium Metal Anode. Joule 2019, 3, 1637–1646. [Google Scholar] [CrossRef]
- Xia, S.; Wu, X.; Zhang, Z.; Cui, Y.; Lui, W. Practical Challenges and Future Perspectives of All-Solid-State Lithium-Metal Batteries. Chem 2019, 5, 753–785. [Google Scholar] [CrossRef]
- Liu, H.; Cheng, X.; Huang, J.; Yuan, H.; Lu, Y.; Yan, C.; Zhu, G.; Xu, R.; He, C. Controlling Dendrite Growth in Solid-State Electrolytes. ACS Energy Lett. 2020, 5, 833–843. [Google Scholar] [CrossRef]
- Pesaran, A.; Santhanagopalan, S.; Kim, G. Addressing the Impact of Temperature Extremes on Large Format Li-Ion Batteries for Vehicle Applications. In Proceedings of the 30th International Battery Seminar, Ft. Lauderdale, FL, USA, 11–14 March 2013. [Google Scholar]
- Guo, L.; Wang, Z.; Wang, J.; Luo, Q.; Liu, J. Effects of the environmental temperature and heat dissipation condition on the thermal runaway of lithium ion batteries during the charge-discharge process. J. Loss Prev. Process. Ind. 2017, 49, 953–960. [Google Scholar] [CrossRef]
- Agwu, D.; Opara, F.; Chukwuchekwa, N.; Dike, D.; Uzoechi, L. Review of Comparative Battery Energy Storage Systems (Bess) For Energy Storage Applications in Tropical Environments. In Proceedings of the IEEE NIGERCON2017—3rd International Conference on Electro-Technology for National Development, Owerri, Nigeria, 7–10 November 2017. [Google Scholar]
- Wang, Q.; Jiang, B.; Li, B.; Yan, Y. A critical review of thermal management models and solutions of lithium-ion batteries for the development of pure electric vehicles. Renew. Sustain. Energy Rev. 2016, 64, 106–128. [Google Scholar] [CrossRef]
- An, Z.; Jia, L.; Ding, Y.; Dang, C.; Li, X. A review on lithium-ion power battery thermal management technologies and thermal safety. J. Therm. Sci. 2017, 26, 391–412. [Google Scholar] [CrossRef]
- Ji, Y.; Wang, C. Heating strategies for Li-ion batteries operated from subzero temperatures. Electrochim. Acta 2013, 107, 664–674. [Google Scholar] [CrossRef]
- Hu, X.; Zheng, Y.; Howey, D.; Perez, H.; Foley, A.; Pecht, M. Battery warm-up methodologies at subzero temperatures for automotive applications: Recent advances and perspectives. Prog. Energy Combust. Sci. 2020, 77, 100806. [Google Scholar] [CrossRef]
- Yates, M.; Akrami, M.; Javadi, A. Analysing the performance of liquid cooling designs in cylindrical lithium-ion batteries. J. Energy Storage 2021, 33, 100913. [Google Scholar] [CrossRef]
- Fan, R.; Zhang, C.; Wang, Y.; Ji, C.; Meng, Z.; Xu, L.; Ou, Y.; Chin, C.S. Numerical study on the effects of battery heating in cold climate. J. Energy Storage 2019, 26, 100969. [Google Scholar] [CrossRef]
- Jiang, G.; Zhuang, L.; Hu, Q.; Liu, Z.; Huang, J. An investigation of heat transfer and capacity fade in a prismatic Li-ion battery based on an electrochemical-thermal coupling model. Appl. Therm. Eng. 2020, 171, 115080. [Google Scholar] [CrossRef]
- Wang, Y.; Rao, Z.; Liu, S.; Li, X.; Li, H.; Xiong, R. Evaluating the performance of liquid immersing preheating system for Lithium-ion battery pack. Appl. Therm. Eng. 2021, 190, 116811. [Google Scholar] [CrossRef]
- Tzermias, G.; Akehurst, S.; Burke, R.; Brace, C.; George, S.; Bernards, J.; Smith, C. Methodology for the Optimisation of Battery Hybrid Energy Storage Systems for Mass and Volume Using a Power-To-Energy Ratio Analysis. Batteries 2021, 7, 37. [Google Scholar] [CrossRef]
- Omar, N.; Bossche, P.; Coosemans, T.; Mierlo, J. Peukert Revisited—Critical Appraisal and Need for Modification for Lithium-Ion Batteries. Energies 2013, 6, 5625–5641. [Google Scholar] [CrossRef]
- PowerStream Technology. Introduction of INR18650-25R; PowerStream Technology: Orem, UT, USA, 2013; Available online: https://www.powerstream.com/p/INR18650-25R-datasheet.pdf (accessed on 1 October 2021).
- Fiori, C.; Ahn, K.; Rakha, H. Power-based electric vehicle energy consumption model: Model development and validation. Appl. Energy 2016, 168, 257–268. [Google Scholar] [CrossRef]
- Spanoudakis, P.; Tsourveloudis, N.; Doitsidis, L.; Karapidakis, E. Experimental Research of Transmissions on Electric Vehicles’ Energy Consumption. Energies 2019, 12, 388. [Google Scholar] [CrossRef] [Green Version]
- Sato, Y.; Ishikawa, S.; Okubo, T.; Abe, M.; Tamai, K. Development of High Response Motor and Inverter System for the Nissan LEAF Electric Vehicle. SAE Tech. Pap. Ser. 2011, 7. [Google Scholar] [CrossRef]
- Rassolkin, A.; Orlova, S.; Kallaste, A.; Gevorkov, L. Re-Use and Recycling of Different Electrical Machines. Latv. J. Phys. Tech. Sci. 2018, 55, 13–23. [Google Scholar] [CrossRef] [Green Version]
- Schnell, J.; Gunther, T.; Knoche, T.; Vieder, C.; Kohler, L.; Just, A.; Keller, M.; Passerini, S.; Reinhart, G. All-solid-state lithium-ion and lithium metal batteries—paving the way to large-scale production. J. Power Sources 2018, 382, 160–175. [Google Scholar] [CrossRef]
- Blue Solutions. Lithium Metal Polymer Battery Having a High Energy Density; US 2019/0088997; Blue Solutions: Ergué Gabéric, France, 2019. [Google Scholar]
- Silveria, A.; Santana, M.; Tanabe, E.; Bertuol, D. Recovery of valuable materials from spent lithium ion batteries using electrostatic separation. Int. J. Miner. Process. 2017, 169, 91–98. [Google Scholar] [CrossRef]
- Gratz, E.; Sa, Q.; Apelian, D.; Wang, Y. A closed loop process for recycling spent lithium ion batteries. J. Power Sources 2014, 262, 255–262. [Google Scholar] [CrossRef]
- Herrmann, M. Packaging—Materials review. AIP Conf. Proc. 2014, 1597, 121–133. [Google Scholar]
- Maleki, H.; Hallaj, S.; Selman, R.; Dinwiddie, R.; Wang, H. Thermal Properties of Lithium-Ion Battery and Components. Electrochem. Soc. 1999, 146, 947. [Google Scholar] [CrossRef]
- Saw, L.; Tay, A.; Zhang, L. Thermal management of lithium-ion battery pack with liquid cooling. In Proceedings of the 2015 31st Thermal Measurement, Modeling & Management Symposium (SEMI-THERM), San Jose, CA, USA, 15–19 March 2015. [Google Scholar]
- Villano, P.; Carewska, M.; Passerini, S. Specific heat capacity of lithium polymer battery components. Thermochim. Acta 2003, 402, 219–224. [Google Scholar] [CrossRef]
- Randau, S.; Weber, D.A.; Kötz, O.; Koerver, R.; Braun, P.; Weber, A.; Ivers-Tiffée, E.; Adermann, T.; Kulisch, J.; Zeier, W.G.; et al. Benchmarking the performance of all-solid-state lithium batteries. Nat. Energy 2020, 5, 259–270. [Google Scholar] [CrossRef]
- Stan, A.; Swierczynski, M.; Stroe, D.; Teodorescu, R.; Andreasen, S.J.; Moth, K. A comparative study of lithium ion to lead acid batteries for use in UPS applications. In Proceedings of the 2014 IEEE 36th International Telecommunications Energy Conference (INTELEC), Vancouver, BC, Canada, 28 September–2 October 2014. [Google Scholar]
- Löbberding, H.; Wessel, S.; Offermanns, C.; Kehrer, M.; Rother, J.; Heimes, H.; Kampker, A. From Cell to Battery System in BEVs: Analysis of System Packing Efficiency and Cell Types. World Electr. Veh. J. 2020, 11, 77. [Google Scholar] [CrossRef]
- Kuipers, M.; Friedrich, E.; Meier, S.; Dirk, U. An in-depth View into the Tesla Model S Module Part Two: Module Characterization and Comparison to Other State of the Art EV Battery Systems. In Proceedings of the 9th Battery Power Conference, Aachen, Germany, 29–30 March 2017. [Google Scholar]
- Kosky, P.; Balmer, R.; Keat, W.; Wise, G. Exploring Engineering, 5th ed.; Elsevier: Amsterdam, The Netherlands, 2020. [Google Scholar]
- IRENA. Renewable Power Generation Costs in 2019; International Renewable Energy Agency: Abu Zabi, United Arab Emirates, 2020. [Google Scholar]
- Zhang, D.; Xu, X.; Huang, X.; Shi, Z.; Wang, Z.; Liu, Z.; Hu, R.; Liu, J.; Zhu, M. A flexible composite solid electrolyte with a highly stable interphase for dendrite-free and durable all-solid-state lithium metal batteries. J. Mater. Chem. A 2020, 8, 18043–18054. [Google Scholar] [CrossRef]
- Sun, Y.; Jin, F.; Li, J.; Liu, B.; Chen, X.; Dong, H.; Mao, Y.; Gu, W.; Xu, J.; Shen, Y.; et al. Composite Solid Electrolyte for Solid-State Lithium Batteries Workable at Room Temperature. ACS Appl. Energy Mater. 2020, 3, 12127–12133. [Google Scholar] [CrossRef]


| Parameter | Value |
|---|---|
| Mass, | 1580 kg |
| Frontal Area, | 2.33 m2 |
| Drag Coefficient, | 0.28 |
| Rolling Resistance Coefficient, | 0.02 |
| Parameter | Value |
|---|---|
| Tire Diameter | 0.64 m |
| Drivetrain gear ratio | 8.19 |
| Motor mass | 56 kg |
| Motor specific heat | 856 J/kg∙K |
| Component | Material | Density (kg/m3) | Layer Percentage (%) | Specific Heat (J/kg∙K) | |
|---|---|---|---|---|---|
| (a) | (b) | ||||
| Solid electrolyte | P(EO)20LiBETI | 900 | 10.8 | 44.6 | 1730 [45] |
| Anode | Lithium | 534 | 12.3 | 38.2 | 3550 |
| Cathode | V2O5–C–PEG–PEO | 3000 | 67.7 | 7.6 | 935 [45] |
| Collector (+/−) | Aluminum | 2700 | 9.2 | 9.6 | 900 |
| Case | Aluminum | 900 | |||
| Average Specific Heat Capacity (J/kg∙K) | 1010 | 1550 | |||
| Parameter | Value |
|---|---|
| Sub-pack capacity | 10 kWh |
| Number of sub-packs | 6 |
| Sub-pack mass | 40.6 kg |
| Sub-pack volume | 23 L |
| Sub-pack surface area | 0.49 m2 |
| ASSB specific heat capacity | 1010 J/kg∙K |
| Maximum heating power | 132.7 W/L |
| Natural convective heat transfer | 10 W/m2K |
| Insulation conductivity | 0.02 W/m∙K |
| Insultation thickness | 0.02 m |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2022 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Hughes, R.; Vagg, C. Assessing the Feasibility of a Cold Start Procedure for Solid State Batteries in Automotive Applications. Batteries 2022, 8, 13. https://doi.org/10.3390/batteries8020013
Hughes R, Vagg C. Assessing the Feasibility of a Cold Start Procedure for Solid State Batteries in Automotive Applications. Batteries. 2022; 8(2):13. https://doi.org/10.3390/batteries8020013
Chicago/Turabian StyleHughes, Ryan, and Christopher Vagg. 2022. "Assessing the Feasibility of a Cold Start Procedure for Solid State Batteries in Automotive Applications" Batteries 8, no. 2: 13. https://doi.org/10.3390/batteries8020013
APA StyleHughes, R., & Vagg, C. (2022). Assessing the Feasibility of a Cold Start Procedure for Solid State Batteries in Automotive Applications. Batteries, 8(2), 13. https://doi.org/10.3390/batteries8020013






