Absolute Local Quantification of Li as Function of State-of-Charge in All-Solid-State Li Batteries via 2D MeV Ion-Beam Analysis
Abstract
:1. Introduction
2. Results
2.1. Ceramic Based Cells (Ex-Situ)
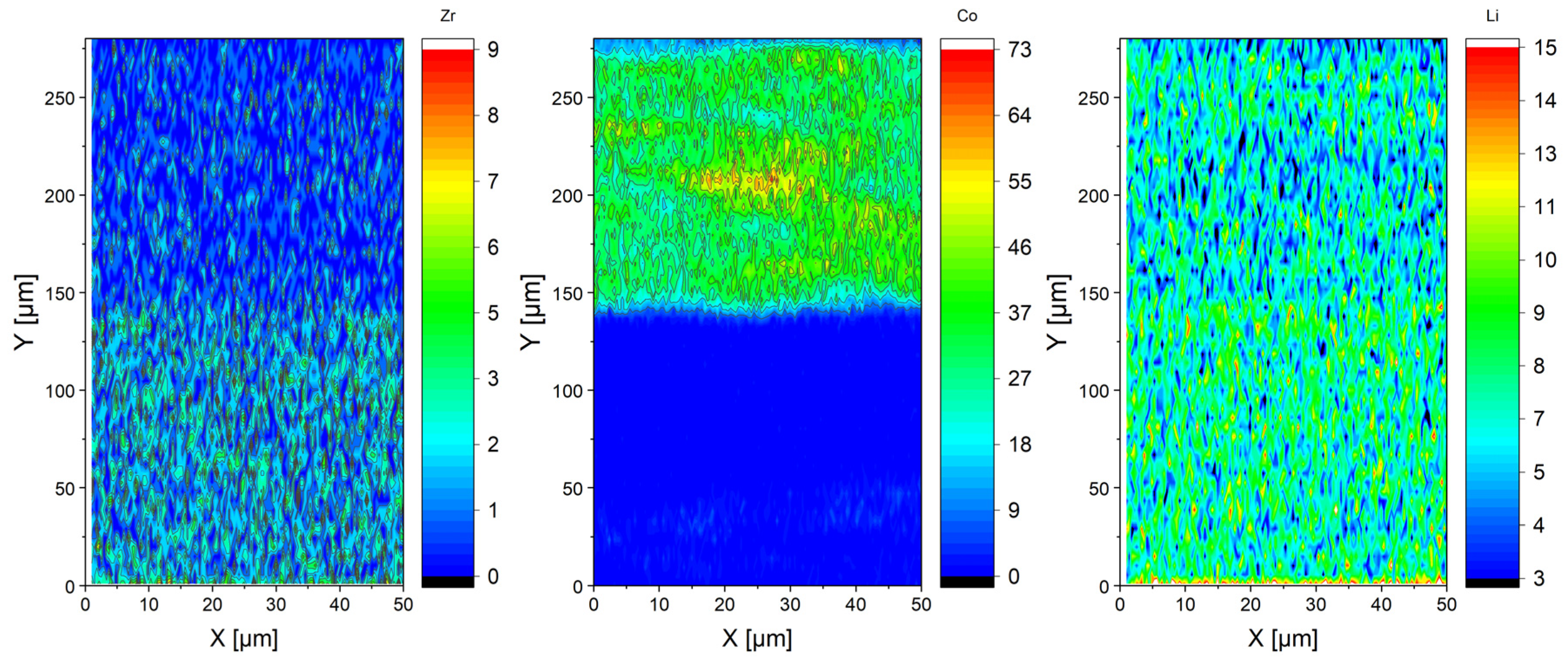
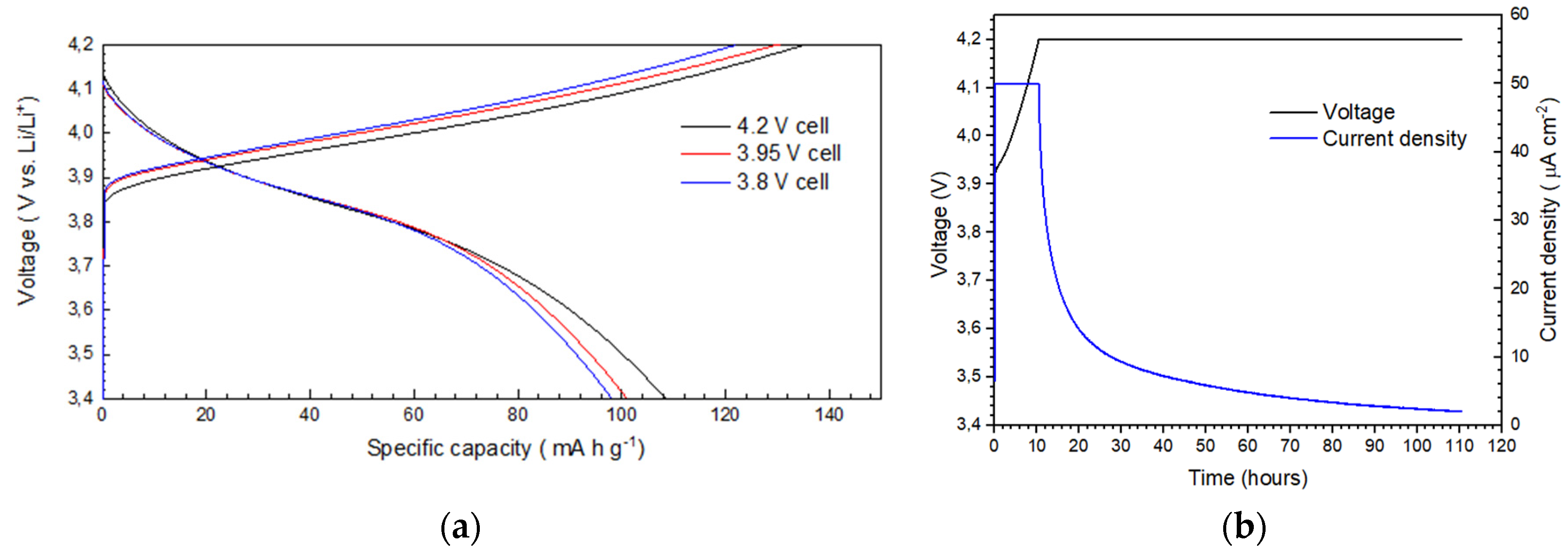
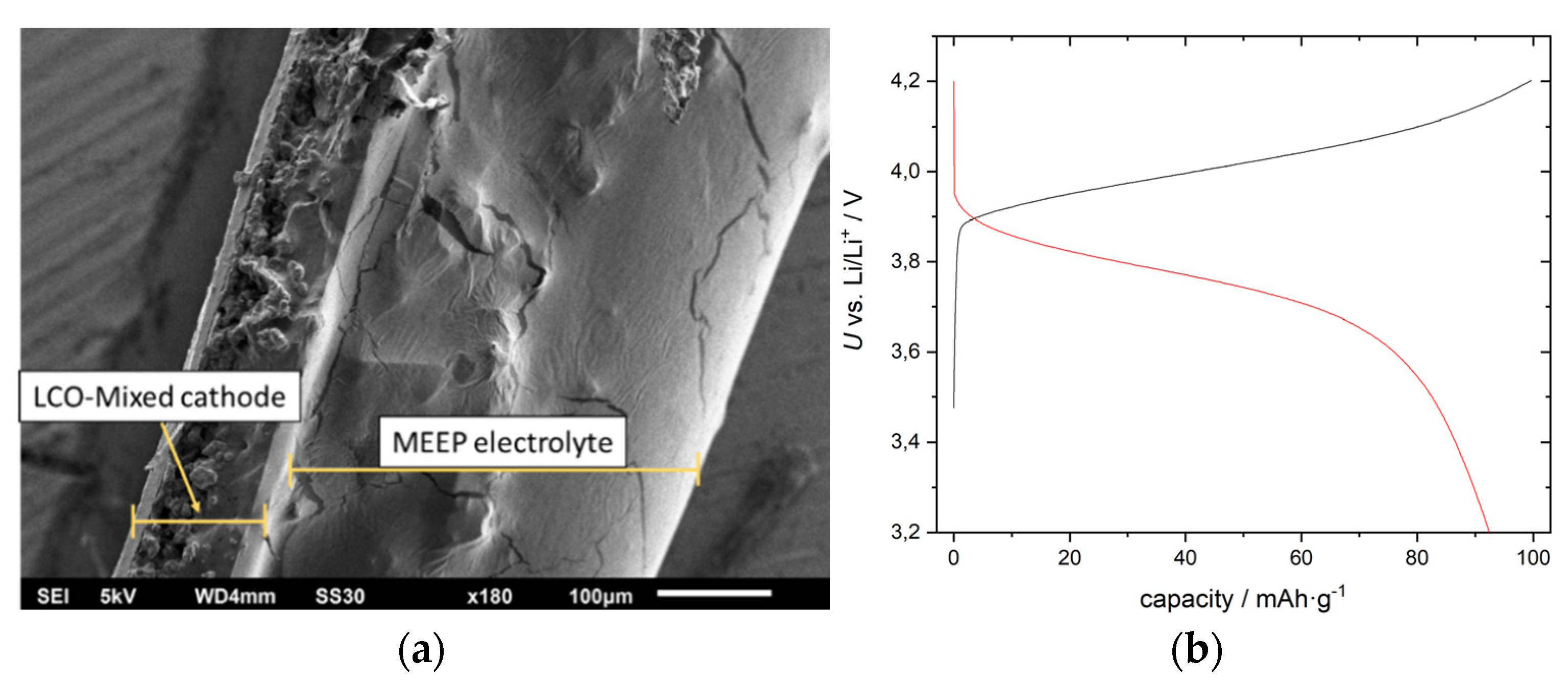
2.2. Polymer Based Cells (Ex-Situ)
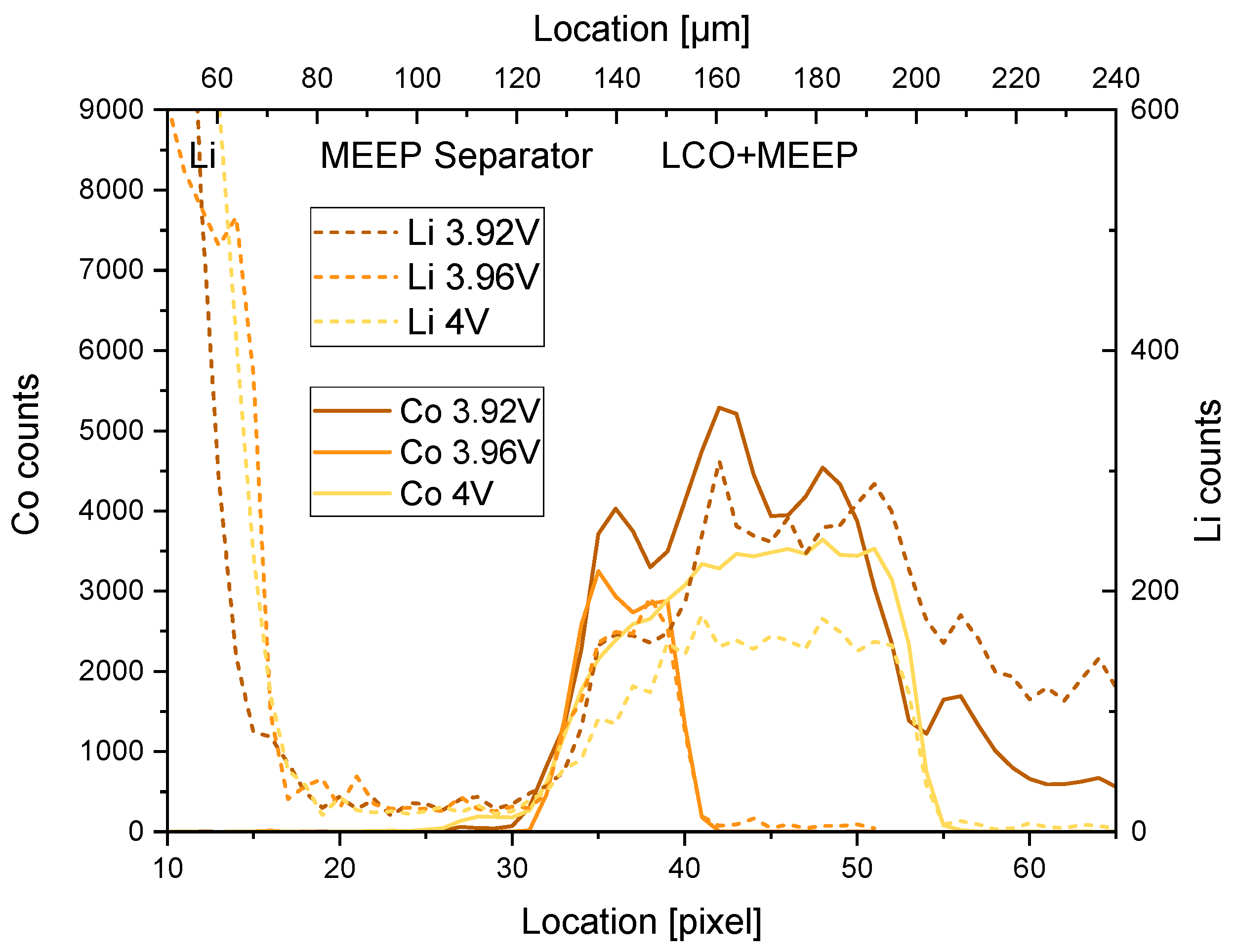
2.3. Operando Polymer Based Cell
3. Discussion
4. Materials and Methods
4.1. Ceramic-Based Cell Preparation
4.2. Polymer-Based Cell Preparation
4.3. Ion-Beam Analysis
4.4. Ion-Beam Analysis Spectrum Evaluation Methodology
4.5. Cell Preparation
Preparation of Polymer All-Solid-State Batteries
Supplementary Materials
Author Contributions
Funding
Institutional Review Board Statement
Informed Consent Statement
Data Availability Statement
Acknowledgments
Conflicts of Interest
Appendix A
| LCO | g/mol | g/cm3 | mol LCO/cm3 | Li atoms/cm3 |
| x = 1 | 97.9 | 5.05 | 0.0516 | 0.0516 |
| x = 0.5 | 94.4 | 4.87 | 0.0516 | 0.0258 |
| LLZO | g/mol | g/cm3 | mol LLZO/cm3 | Li atoms/cm3 |
| 0.4 Ta | 873.6285 | 5.4 | 0.0062 | 0.0399 |
| x in LixCoO2 | Li-atoms/cm3 | at. Li/cm3 in Mixed Cathode | Calculated Relative Concentration Change | SimNRA PIGE Signal |
|---|---|---|---|---|
| 1 | 0.0516 | 0.0459 | 1 | 1.0000 |
| 0.9 | 0.0464 | 0.0433 | 0.9420 | 0.9476 |
| 0.8 | 0.0413 | 0.0406 | 0.8839 | 0.8875 |
| 0.7 | 0.0361 | 0.0379 | 0.8259 | 0.8375 |
| 0.6 | 0.0309 | 0.0353 | 0.7678 | 0.7797 |
| 0.5 | 0.0258 | 0.0326 | 0.7098 | 0.7177 |
References
- Nazri, G.-A.; Pistoia, G. Lithium Batteries: Science and Technology; Springer Science & Business Media: Berlin/Heidelberg, Germany, 2008. [Google Scholar]
- Kim, I.-S.; Kumta, P.N. High capacity Si/C nanocomposite anodes for Li-ion batteries. J. Power Sources 2004, 136, 145–149. [Google Scholar] [CrossRef]
- Marinaro, M.; Yoon, D.-h.; Gabrielli, G.; Stegmaier, P.; Figgemeier, E.; Spurk, P.C.; Nelis, D.; Schmidt, G.; Chauveau, J.; Axmann, P.; et al. High performance 1.2 Ah Si-alloy/Graphite| LiNi0. 5Mn0. 3Co0. 2O2 prototype Li-ion battery. J. Power Sources 2017, 357, 188–197. [Google Scholar] [CrossRef]
- Xia, Y.; Zheng, J.; Wang, C.; Gu, M. Designing principle for Ni-rich cathode materials with high energy density for practical applications. Nano Energy 2018, 49, 434–452. [Google Scholar] [CrossRef]
- Rozier, P.; Tarascon, J.M. Review—Li-Rich Layered Oxide Cathodes for Next-Generation Li-Ion Batteries: Chances and Challenges. J. Electrochem. Soc. 2015, 162, A2490. [Google Scholar] [CrossRef]
- Fuller, T.F.; Doyle, M.; Newman, J. Simulation and Optimization of the Dual Lithium Ion Insertion Cell. J. Electrochem. Soc. 1994, 141, 1. [Google Scholar] [CrossRef] [Green Version]
- Dai, Y.; Srinivasan, V. On graded electrode porosity as a design tool for improving the energy density of batteries. J. Electrochem. Soc. 2015, 163, A406. [Google Scholar] [CrossRef]
- Janek, J.; Zeier, W.G. A solid future for battery development. Nat. Energy 2016, 1, 16141. [Google Scholar] [CrossRef]
- Doyle, M.; Fuller, T.F.; Newman, J. Modeling of galvanostatic charge and discharge of the lithium/polymer/insertion cell. J. Electrochem. Soc. 1993, 140, 1526. [Google Scholar] [CrossRef]
- de Vidst, P.; White, R.E. Governing equations for transport in porous electrodes. J. Electrochem. Soc. 1997, 144, 1343. [Google Scholar] [CrossRef]
- Ramadass, P.; Haran, B.; Gomadam, P.M.; White, R.; Popov, B.N. Development of first principles capacity fade model for Li-ion cells. J. Electrochem. Soc. 2004, 151, A196. [Google Scholar] [CrossRef]
- Garcia, R.E.; Chiang, Y.M.; Carter, W.C.; Limthongkul, P.; Bishop, C.M. Microstructural modeling and design of rechargeable lithium-ion batteries. J. Electrochem. Soc. 2005, 152, A255. [Google Scholar] [CrossRef]
- Latz, A.; Zausch, J. Thermodynamic consistent transport theory of Li-ion batteries. J. Power Sources 2011, 196, 3296–3302. [Google Scholar] [CrossRef] [Green Version]
- Latz, A.; Zausch, J. Multiscale modeling of lithium ion batteries: Thermal aspects. Beilstein J. Nanotechnol. 2015, 6, 987–1007. [Google Scholar] [CrossRef] [Green Version]
- Less, G.B.; Seo, J.H.; Han, S.; Sastry, A.M.; Zausch, J.; Latz, A.; Schmidt, S.; Wieser, C.; Kehrwald, D.; Fell, S. Micro-scale modeling of Li-ion batteries: Parameterization and validation. J. Electrochem. Soc. 2012, 159, A697. [Google Scholar] [CrossRef]
- Latz, A.; Zausch, J. Available online: http://www.itwm.fraunhofer.de/best (accessed on 31 January 2021).
- Danner, T.; Singh, M.; Hein, S.; Kaiser, J.; Hahn, H.; Latz, A. Thick electrodes for Li-ion batteries: A model based analysis. J. Power Sources 2016, 334, 191–201. [Google Scholar] [CrossRef] [Green Version]
- Latz, A.; Danner, T.; Horstmann, B.; Jahnke, T. Microstructure-and Theory-Based Modeling and Simulation of Batteries and Fuel Cells. Chem. Ing. Tech. 2019, 91, 758–768. [Google Scholar] [CrossRef]
- Kremer, L.S.; Danner, T.; Hein, S.; Hoffmann, A.; Prifling, B.; Schmidt, V.; Latz, A.; Wohlfahrt-Mehrens, M. Influence of the Electrolyte Salt Concentration on the Rate Capability of Ultra-Thick NCM 622 Electrodes. Batter. Supercaps 2020, 3. [Google Scholar] [CrossRef]
- Kremer, L.S.; Hoffmann, A.; Danner, T.; Hein, S.; Prifling, B.; Westhoff, D.; Dreer, C.; Latz, A.; Schmidt, V.; Wohlfahrt-Mehrens, M. Manufacturing Process for Improved Ultra-Thick Cathodes in High-Energy Lithium-Ion Batteries. Energy Technol. 2020, 8, 1900167. [Google Scholar] [CrossRef]
- Liu, D.; Shadike, Z.; Lin, R.; Qian, K.; Li, H.; Li, K.; Wang, S.; Yu, Q.; Liu, M.; Ganapathy, S.; et al. Review of recent development of in situ/operando characterization techniques for lithium battery research. Adv. Mater. 2019, 31, 1806620. [Google Scholar] [CrossRef] [PubMed]
- Grey, C.P.; Tarascon, J.M. Sustainability and in situ monitoring in battery development. Nat. Mater. 2017, 16, 45–56. [Google Scholar] [CrossRef]
- Sathiya, M.; Leriche, J.B.; Salager, E.; Gourier, D.; Tarascon, J.M.; Vezin, H. Electron paramagnetic resonance imaging for real-time monitoring of Li-ion batteries. Nat. Commun. 2015, 6, 6276. [Google Scholar] [CrossRef] [PubMed] [Green Version]
- Chandrashekar, S.; Trease, N.M.; Chang, H.J.; Du, L.-S.; Grey, C.P.; Jerschow, A. 7 Li MRI of Li batteries reveals location of microstructural lithium. Nat. Mater. 2012, 11, 311–315. [Google Scholar] [CrossRef]
- Gong, Y.; Zhang, J.; Jiang, L.; Shi, J.-A.; Zhang, Q.; Yang, Z.; Zou, D.; Wang, J.; Yu, X.; Xiao, R.; et al. In Situ Atomic-Scale Observation of Electrochemical Delithiation Induced Structure Evolution of LiCoO2 Cathode in a Working All-Solid-State Battery. J. Am. Chem. Soc. 2017, 139, 4274–4277. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.; Santhanagopalan, D.; Zhang, W.; Wang, F.; Xin, H.L.; He, K.; Li, J.; Dudney, N.; Meng, Y.S. In situ STEM-EELS observation of nanoscale interfacial phenomena in all-solid-state batteries. Nano Lett. 2016, 16, 3760–3767. [Google Scholar] [CrossRef] [PubMed]
- Hong, L.; Li, L.; Chen-Wiegart, Y.-K.; Wang, J.; Xiang, K.; Gan, L.; Li, W.; Meng, F.; Wang, F.; Wang, J.; et al. Two-dimensional lithium diffusion behavior and probable hybrid phase transformation kinetics in olivine lithium iron phosphate. Nat. Commun. 2017, 8, 1–13. [Google Scholar] [CrossRef] [Green Version]
- Kuppan, S.; Xu, Y.; Liu, Y.; Chen, G. Phase transformation mechanism in lithium manganese nickel oxide revealed by single-crystal hard X-ray microscopy. Nat. Commun. 2017, 8, 14309. [Google Scholar] [CrossRef] [Green Version]
- Oudenhoven, J.F.M.; Labohm, F.; Mulder, M.; Niessen, R.A.H.; Mulder, F.M.; Notten, P.H.L. In situ neutron depth profiling: A powerful method to probe lithium transport in micro-batteries. Adv. Mater. 2011, 23, 4103–4106. [Google Scholar] [CrossRef]
- Harks, P.-P.R.M.L.; Verhallen, T.W.; George, C.; van den Biesen, J.K.; Liu, Q.; Wagemaker, M.; Mulder, F.M. Spatiotemporal quantification of lithium both in electrode and in electrolyte with atomic precision via operando neutron absorption. J. Am. Chem. Soc. 2019, 141, 14280–14287. [Google Scholar] [CrossRef] [Green Version]
- Habedank, J.B.; Günter, F.J.; Billot, N.; Gilles, R.; Neuwirth, T.; Reinhart, G.; Zaeh, M.F. Rapid electrolyte wetting of lithium-ion batteries containing laser structured electrodes: In situ visualization by neutron radiography. Int. J. Adv. Manuf. Technol. 2019, 102, 2769–2778. [Google Scholar] [CrossRef]
- Surblé, S.; Paireau, C.; Martin, J.F.; Tarnopolskiy, V.; Gauthier, M.; Khodja, H.; Daniel, L.; Patoux, S. Operando analysis of lithium profiles in Li-ion batteries using nuclear microanalysis. J. Power Sources 2018, 393, 37–42. [Google Scholar] [CrossRef] [Green Version]
- Habrioux, A.; Surblé, S.; Berger, P.; Khodja, H.; D’Affroux, A.; Mailley, S.; Gutel, T.; Patoux, S. Nuclear microanalysis of lithium dispersion in LiFePO4 based cathode materials for Li-ion batteries. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. Atoms 2012, 290, 13–18. [Google Scholar] [CrossRef]
- Gonzalez-Arrabal, R.; Panizo-Laiz, M.; Fujita, K.; Mima, K.; Yamazaki, A.; Kamiya, T.; Orikasa, Y.; Uchimoto, Y.; Sawada, H.; Okuda, C.; et al. Meso-scale characterization of lithium distribution in lithium-ion batteries using ion beam analysis techniques. J. Power Sources 2015, 299, 587–595. [Google Scholar] [CrossRef] [Green Version]
- Mima, K.; Gonzalez-Arrabal, R.; Azuma, H.; Yamazaki, A.; Okuda, C.; Ukyo, Y.; Sawada, H.; Fujita, K.; Kato, Y.; Perlado, J.M.; et al. Li distribution characterization in Li-ion batteries positive electrodes containing LixNi0.8Co0.15Al0.05O2 secondary particles (0.75 ⩽ x ⩽ 1.0). Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. Atoms 2012, 290, 79–84. [Google Scholar] [CrossRef] [Green Version]
- Yamazaki, A.; Orikasa, Y.; Chen, K.; Uchimoto, Y.; Kamiya, T.; Koka, M.; Satoh, T.; Mima, K.; Kato, Y.; Fujita, K. In-situ measurement of the lithium distribution in Li-ion batteries using micro-IBA techniques. Nucl. Instrum. Methods Phys. Res. Sect. B Beam Interact. Mater. Atoms 2016, 371, 298–302. [Google Scholar] [CrossRef] [Green Version]
- Kato, Y.; Ogumi, Z.; Perlado, M. Diagnostics of Lithium-Ion Batteries: Overview, Simulation, and diagnostics; Pan Stanford Publishing: Singapore, 2019. [Google Scholar]
- Manthiram, A.; Yu, X.; Wang, S. Lithium battery chemistries enabled by solid-state electrolytes. Nat. Rev. Mater. 2017, 2, 16103. [Google Scholar] [CrossRef]
- Kamaya, N.; Homma, K.; Yamakawa, Y.; Hirayama, M.; Kanno, R.; Yonemura, M.; Kamiyama, T.; Kato, Y.; Hama, S.; Kawamoto, K.; et al. A lithium superionic conductor. Nat. Mater. 2011, 10, 682–686. [Google Scholar] [CrossRef]
- Kato, Y.; Hori, S.; Saito, T.; Suzuki, K.; Hirayama, M.; Mitsui, A.; Yonemura, M.; Iba, H.; Kanno, R. High-power all-solid-state batteries using sulfide superionic conductors. Nat. Energy 2016, 1, 16030. [Google Scholar] [CrossRef]
- Lee, Y.-G.; Fujiki, S.; Jung, C.; Suzuki, N.; Yashiro, N.; Omoda, R.; Ko, D.-S.; Shiratsuchi, T.; Sugimoto, T.; Ryu, S.; et al. High-energy long-cycling all-solid-state lithium metal batteries enabled by silver–carbon composite anodes. Nat. Energy 2020, 5, 299–308. [Google Scholar] [CrossRef]
- Yamada, Y.; Suzuki, K.; Yoshino, K.; Taminato, S.; Satoh, T.; Finsterbusch, M.; Kamiya, T.; Yamazaki, A.; Kato, Y.; Fujita, K.; et al. Ex-situ Analysis of Lithium Distribution in a Sulfide-based All-solid-state Lithium Battery by Particle-induced X-ray and Gamma-ray Emission Measurements. Electrochemistry 2020, 88, 45–49. [Google Scholar] [CrossRef] [Green Version]
- Finsterbusch, M.; Danner, T.; Tsai, C.-L.; Uhlenbruck, S.; Latz, A.; Guillon, O. High Capacity Garnet-Based All-Solid-State Lithium Batteries: Fabrication and 3D-Microstructure Resolved Modeling. ACS Appl. Mater. Interfaces 2018, 10, 22329–22339. [Google Scholar] [CrossRef] [PubMed]
- Tsai, C.-L.; Ma, Q.; Dellen, C.; Lobe, S.; Vondahlen, F.; Windmüller, A.; Grüner, D.; Zheng, H.; Uhlenbruck, S.; Finsterbusch, M.; et al. A garnet structure-based all-solid-state Li battery without interface modification: Resolving incompatibility issues on positive electrodes. Sustain. Energy Fuels 2019, 3, 280–291. [Google Scholar] [CrossRef]
- Jankowsky, S.; Hiller, M.M.; Wiemhöfer, H.D. Preparation and electrochemical performance of polyphosphazene based salt-in-polymer electrolyte membranes for lithium ion batteries. J. Power Sources 2014, 253, 256–262. [Google Scholar] [CrossRef]
- Jankowsky, S.; Hiller, M.M.; Stolina, R.; Wiemhöfer, H.D. Performance of polyphosphazene based gel polymer electrolytes in combination with lithium metal anodes. J. Power Sources 2015, 273, 574–579. [Google Scholar] [CrossRef]
- Yabuuchi, N.; Kawamoto, Y.; Hara, R.; Ishigaki, T.; Hoshikawa, A.; Yonemura, M.; Kamiyama, T.; Komaba, S. A Comparative Study of LiCoO2 Polymorphs: Structural and Electrochemical Characterization of O2-, O3-, and O4-type Phases. Inorg. Chem. 2013, 52, 9131–9142. [Google Scholar] [CrossRef] [PubMed]
- Okumura, T.; Yamaguchi, Y.; Shikano, M.; Kobayashi, H. Correlation of lithium ion distribution and X-ray absorption near-edge structure in O3-and O2-lithium cobalt oxides from first-principle calculation. J. Mater. Chem. 2012, 22, 17340–17348. [Google Scholar] [CrossRef]
- Nitta, N.; Wu, F.; Lee, J.T.; Yushin, G. Li-ion battery materials: Present and future. Mater. Today 2015, 18, 252–264. [Google Scholar] [CrossRef]
- Koka, M.; Ishii, Y.; Yamada, N.; Okubo, T.; Kada, W.; Kitamura, A.; Iwata, Y.; Kamiya, T.; Sato, T. Light Ion Microbeam Analysis/Processing System and Its Improvement; No. JAEA-TECHNOLOGY--2016-006; Japan Atomic Energy Agency: Naka-gun, Japan, 2016. [Google Scholar]
- Mayer, M. SIMNRA User’s Guide; Report IPP 9/113; Max-Planck-Institut für Plasmaphysik: Garching, Germany, 1997. [Google Scholar]
- Wang, B.; Rivard, E.; Manners, I. A New High-Yield Synthesis of Cl3PNSiMe3, a Monomeric Precursor for the Controlled Preparation of High Molecular Weight Polyphosphazenes. Inorg. Chem. 2002, 41, 1690–1691. [Google Scholar] [CrossRef] [PubMed]
- Wang, Z.; Manners, I. Synthesis and characterization of water-soluble cationic and anionic polythionylphosphazene polyelectrolytes. Macromolecules 2005, 38, 5047–5054. [Google Scholar] [CrossRef]
- Allcock, H.R.; Reeves, S.D.; de Denus, C.R.; Crane, C.A. Influence of Reaction Parameters on the Living Cationic Polymerization of Phosphoranimines to Polyphosphazenes. Macromolecules 2001, 34, 748–754. [Google Scholar] [CrossRef]
- Paulsdorf, J.; Kaskhedikar, N.; Burjanadze, M.; Obeidi, S.; Stolwijk, N.A.; Wilmer, D.; Wiemhöfer, H.D. Synthesis and ionic conductivity of polymer electrolytes based on a polyphosphazene with short side groups. Chem. Mater. 2006, 18, 1281–1288. [Google Scholar]



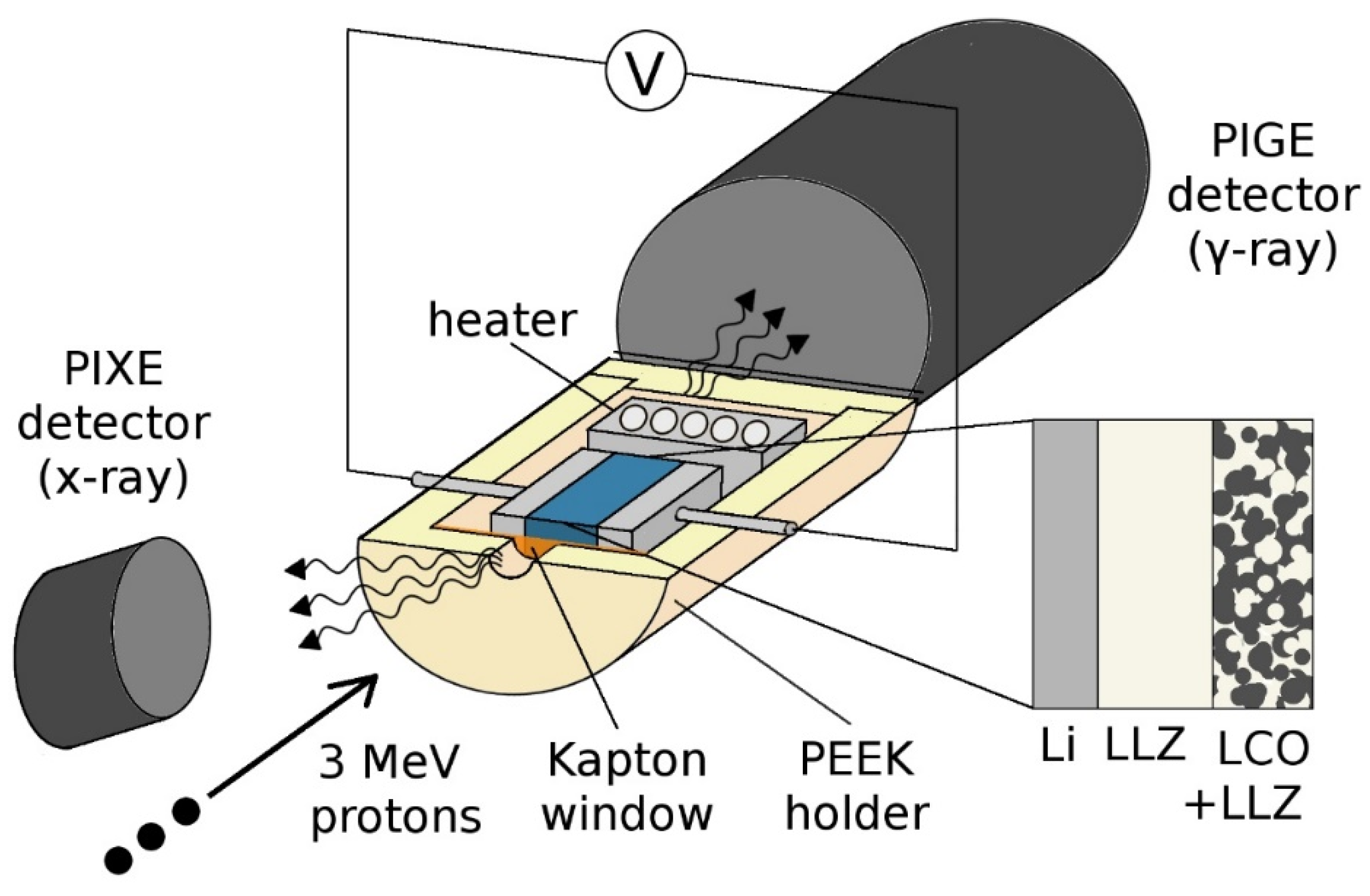

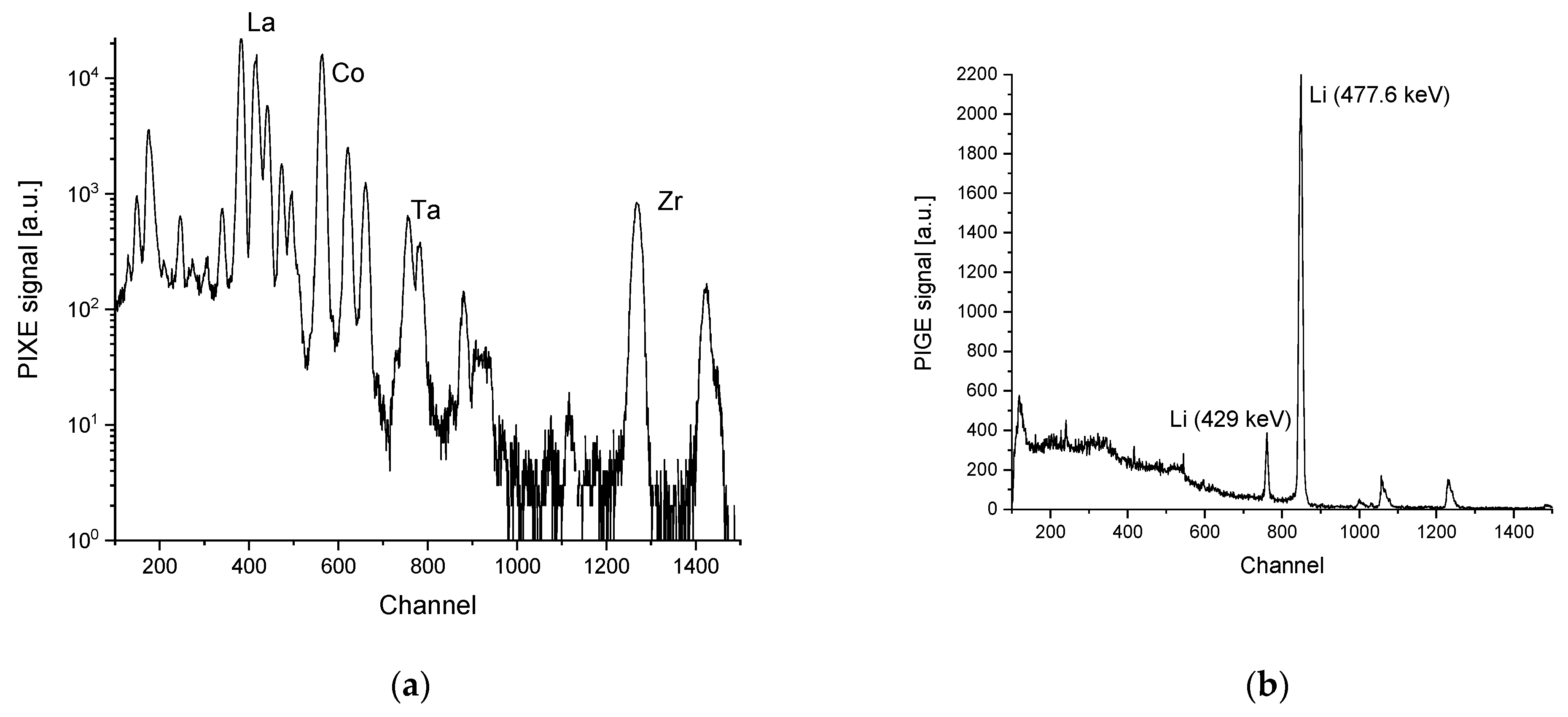

| Charging [V] | Mean PIGE Li Signal [a.u.] | Relative PIGE Li-Signal | X in LixCoO2 via IBA | Derived IBA SoC [%] | SoC Relative (2nd Cycle) [%] |
|---|---|---|---|---|---|
| As-prep | 904 | 1 | 1 | 0 | - |
| 3.8 | 847 | 0.937 | 0.865 | 28 | 0 |
| 3.95 | 818 | 0.905 | 0.783 | 43.4 | 24 |
| 4.2 | 710 | 0.785 | 0.54 | 92 | 100 |
Publisher’s Note: MDPI stays neutral with regard to jurisdictional claims in published maps and institutional affiliations. |
© 2021 by the authors. Licensee MDPI, Basel, Switzerland. This article is an open access article distributed under the terms and conditions of the Creative Commons Attribution (CC BY) license (https://creativecommons.org/licenses/by/4.0/).
Share and Cite
Möller, S.; Satoh, T.; Ishii, Y.; Teßmer, B.; Guerdelli, R.; Kamiya, T.; Fujita, K.; Suzuki, K.; Kato, Y.; Wiemhöfer, H.-D.; et al. Absolute Local Quantification of Li as Function of State-of-Charge in All-Solid-State Li Batteries via 2D MeV Ion-Beam Analysis. Batteries 2021, 7, 41. https://doi.org/10.3390/batteries7020041
Möller S, Satoh T, Ishii Y, Teßmer B, Guerdelli R, Kamiya T, Fujita K, Suzuki K, Kato Y, Wiemhöfer H-D, et al. Absolute Local Quantification of Li as Function of State-of-Charge in All-Solid-State Li Batteries via 2D MeV Ion-Beam Analysis. Batteries. 2021; 7(2):41. https://doi.org/10.3390/batteries7020041
Chicago/Turabian StyleMöller, Sören, Takahiro Satoh, Yasuyuki Ishii, Britta Teßmer, Rayan Guerdelli, Tomihiro Kamiya, Kazuhisa Fujita, Kota Suzuki, Yoshiaki Kato, Hans-Dieter Wiemhöfer, and et al. 2021. "Absolute Local Quantification of Li as Function of State-of-Charge in All-Solid-State Li Batteries via 2D MeV Ion-Beam Analysis" Batteries 7, no. 2: 41. https://doi.org/10.3390/batteries7020041
APA StyleMöller, S., Satoh, T., Ishii, Y., Teßmer, B., Guerdelli, R., Kamiya, T., Fujita, K., Suzuki, K., Kato, Y., Wiemhöfer, H.-D., Mima, K., & Finsterbusch, M. (2021). Absolute Local Quantification of Li as Function of State-of-Charge in All-Solid-State Li Batteries via 2D MeV Ion-Beam Analysis. Batteries, 7(2), 41. https://doi.org/10.3390/batteries7020041






